Analysis and Optimization of a Moving Magnet Permanent Magnet Synchronous Planar Motor with Split Halbach Arrays
Abstract
1. Introduction
2. Topology and Electromagnetic Principle of PMSPM
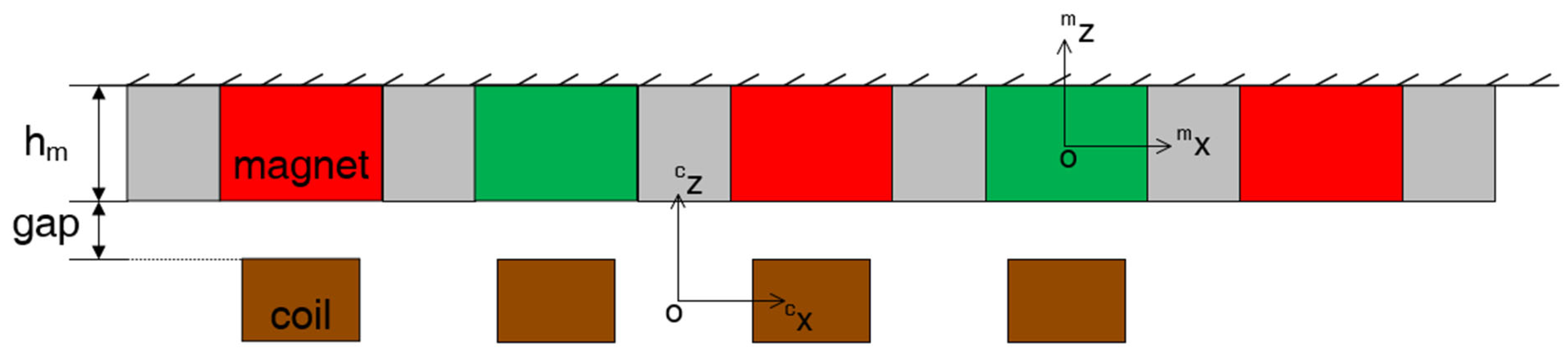
2.1. Analysis of Halbach PM Arrays
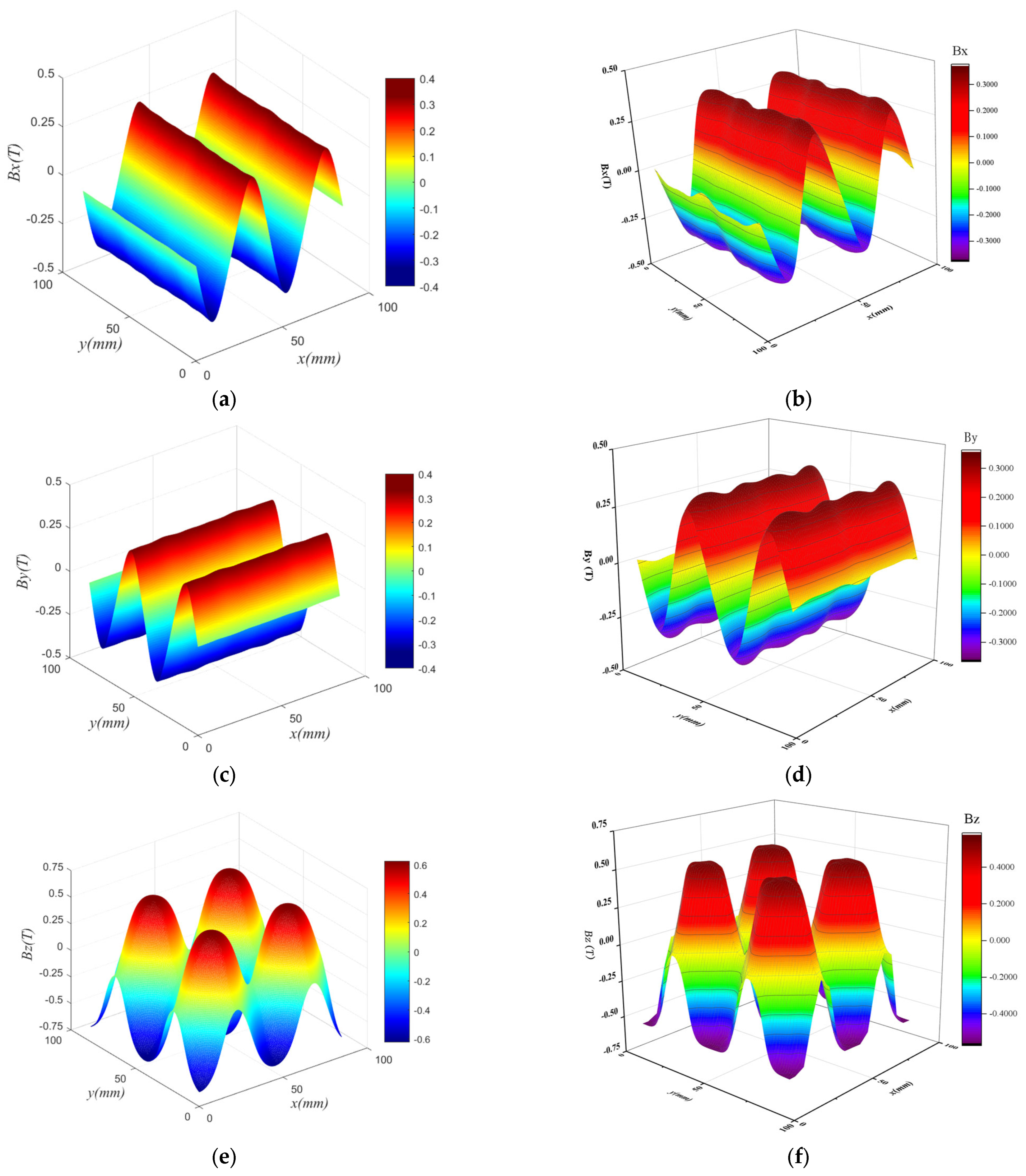
2.2. Thrust Analysis of PMSPM
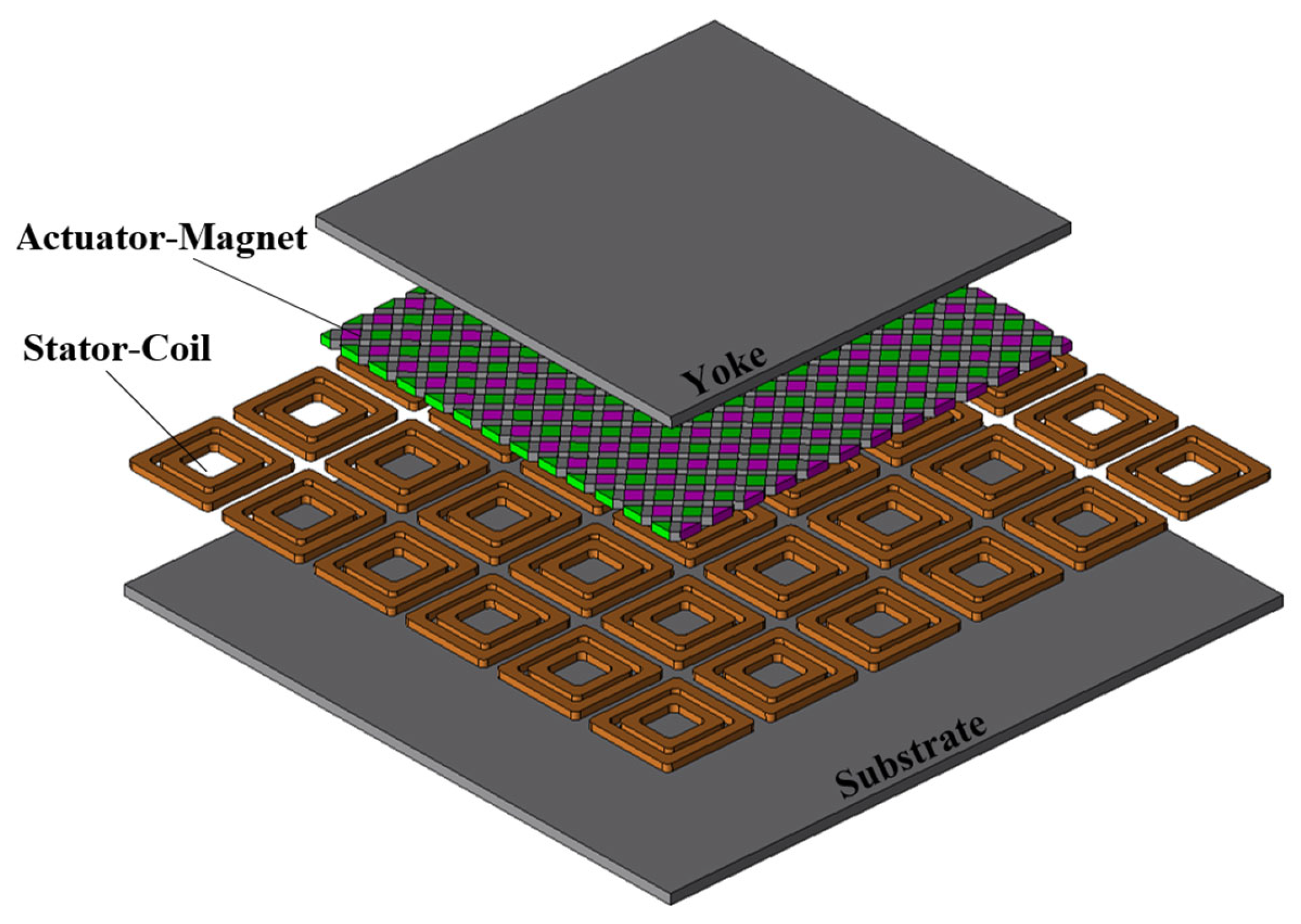
3. Original Topology of the PMSPM
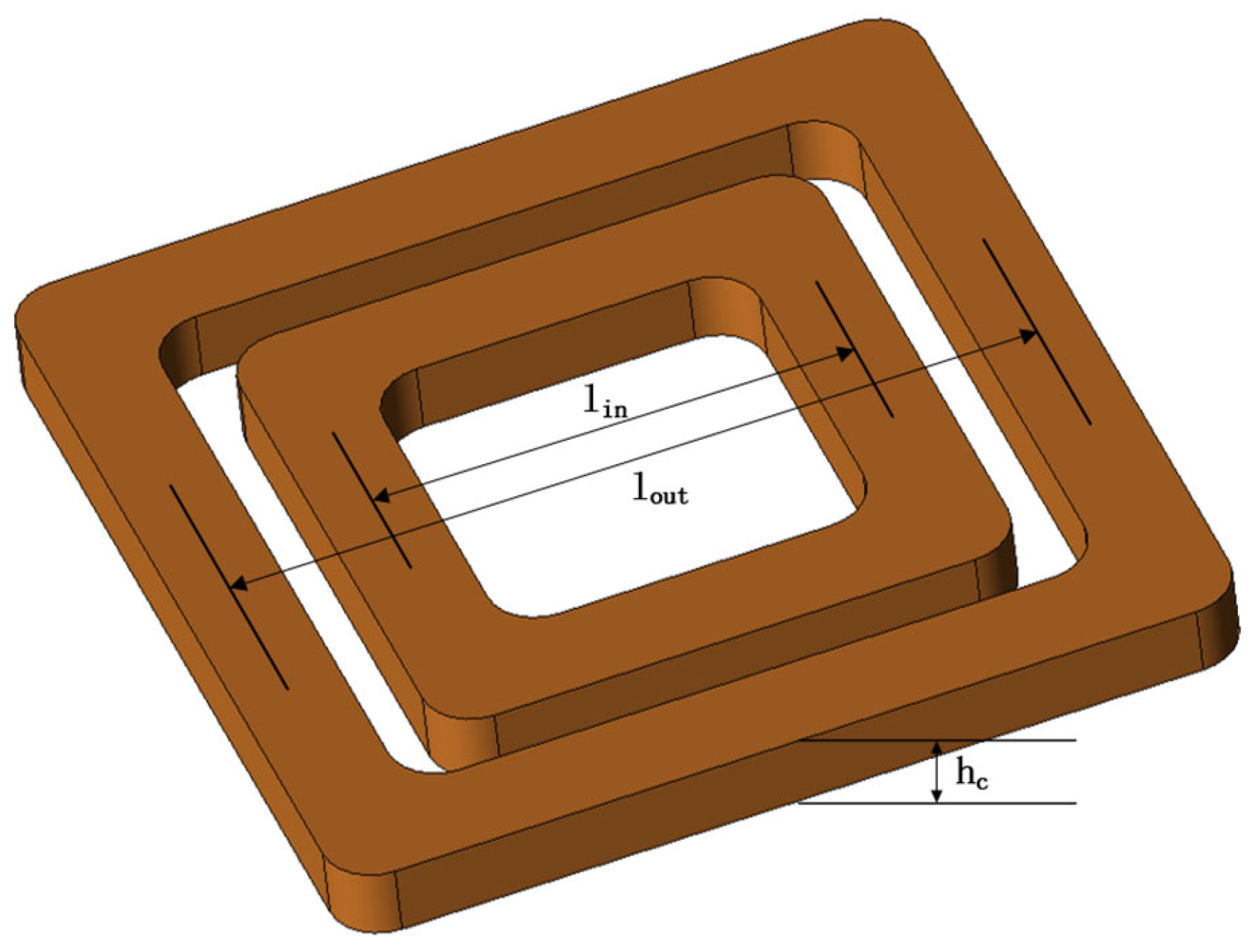
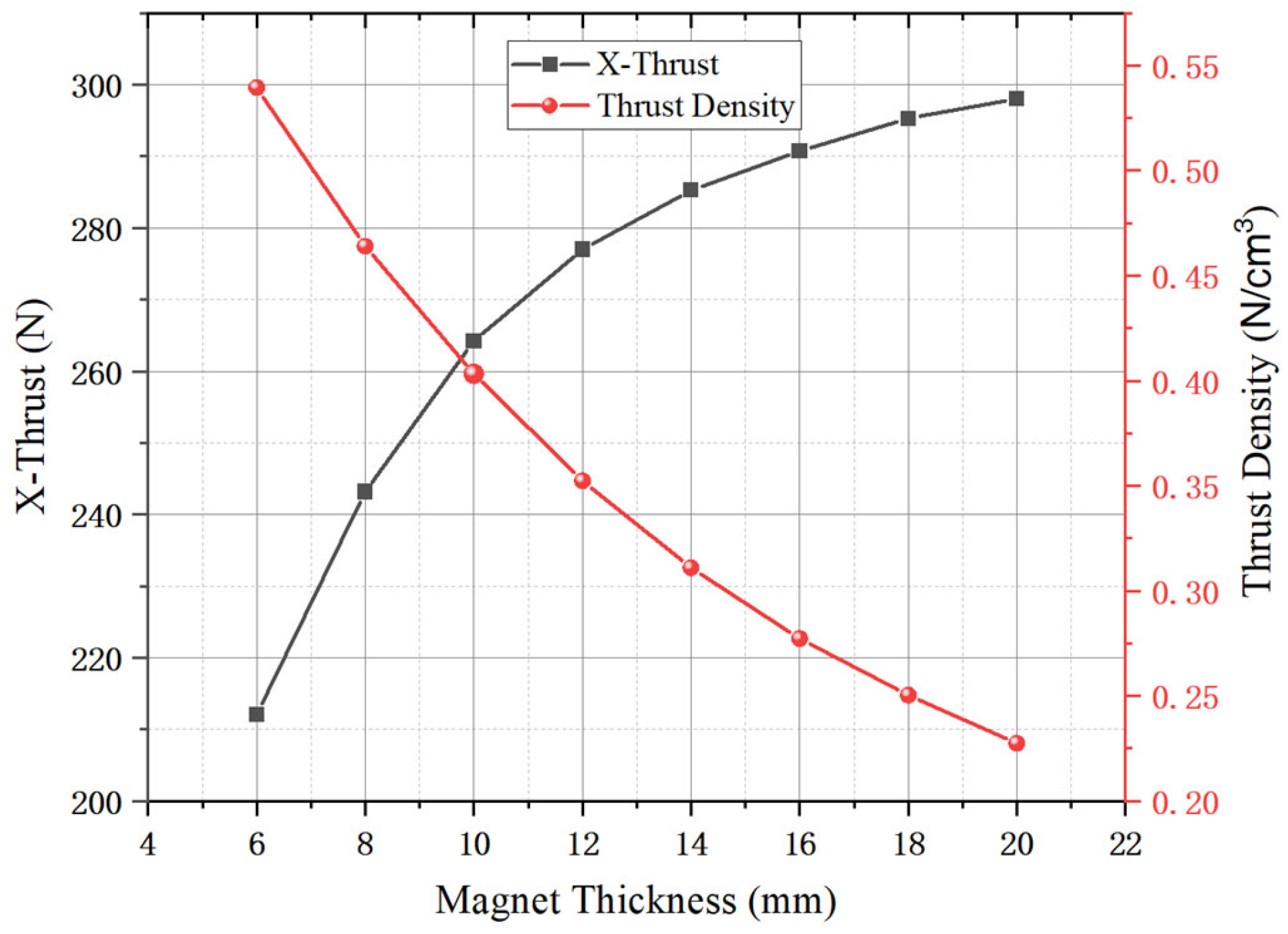
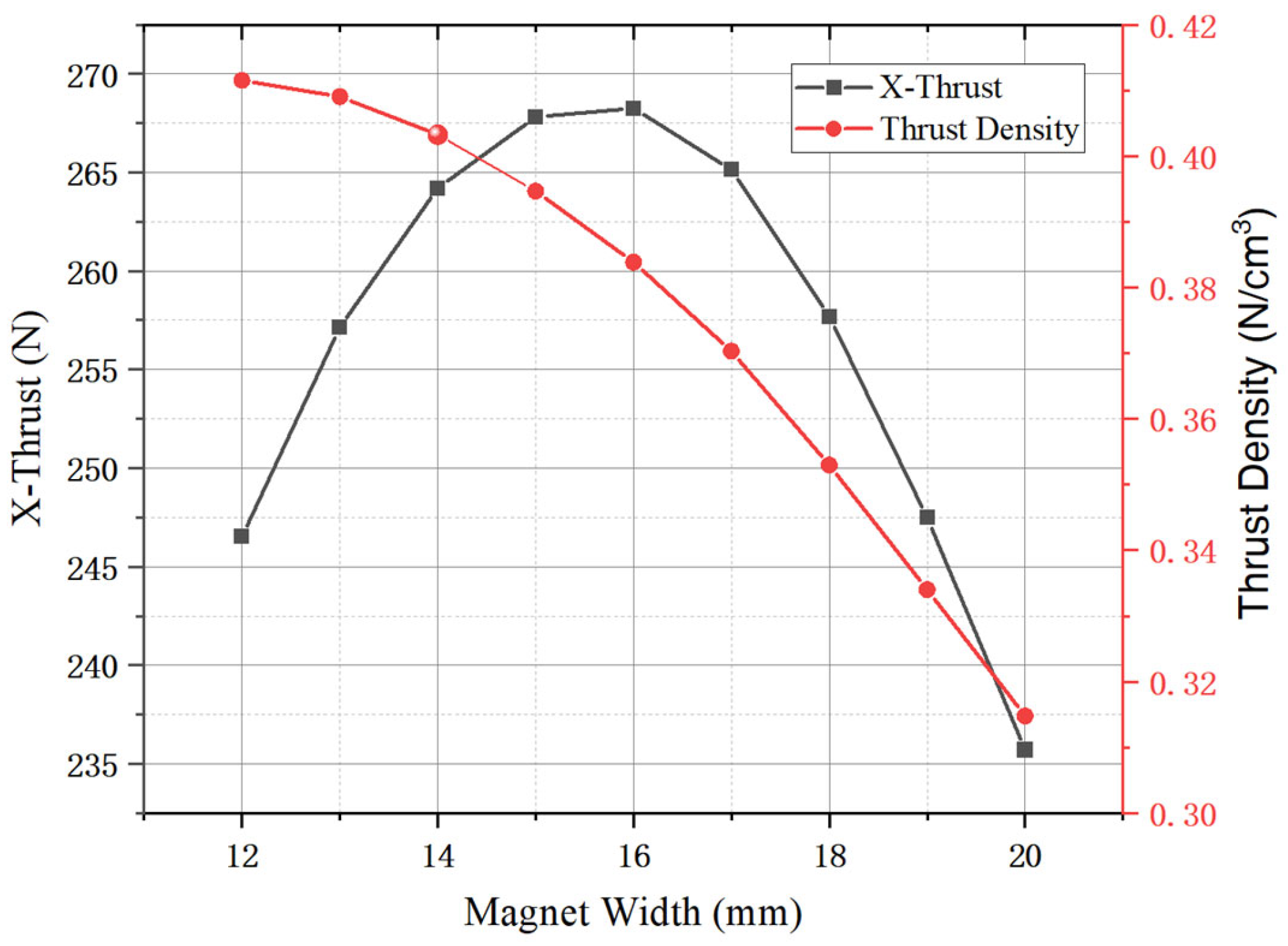
3.1. Topology and Parameters
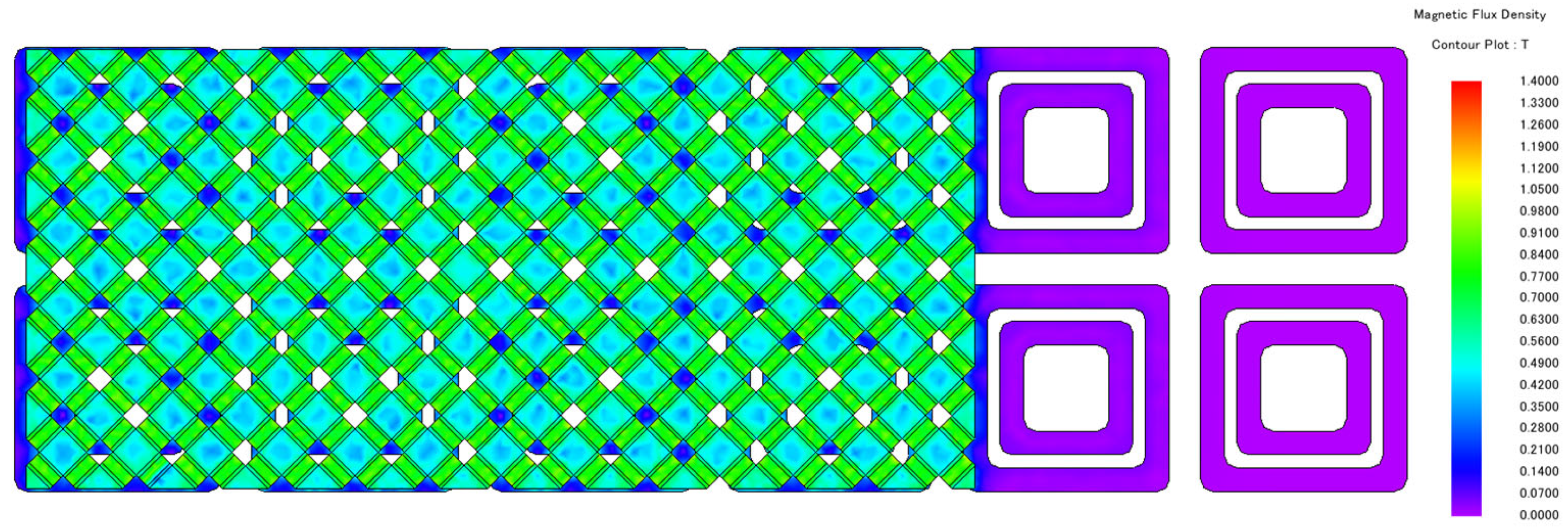
3.2. Parametric Modeling and Optimization
3.3. Performance and Conclusions
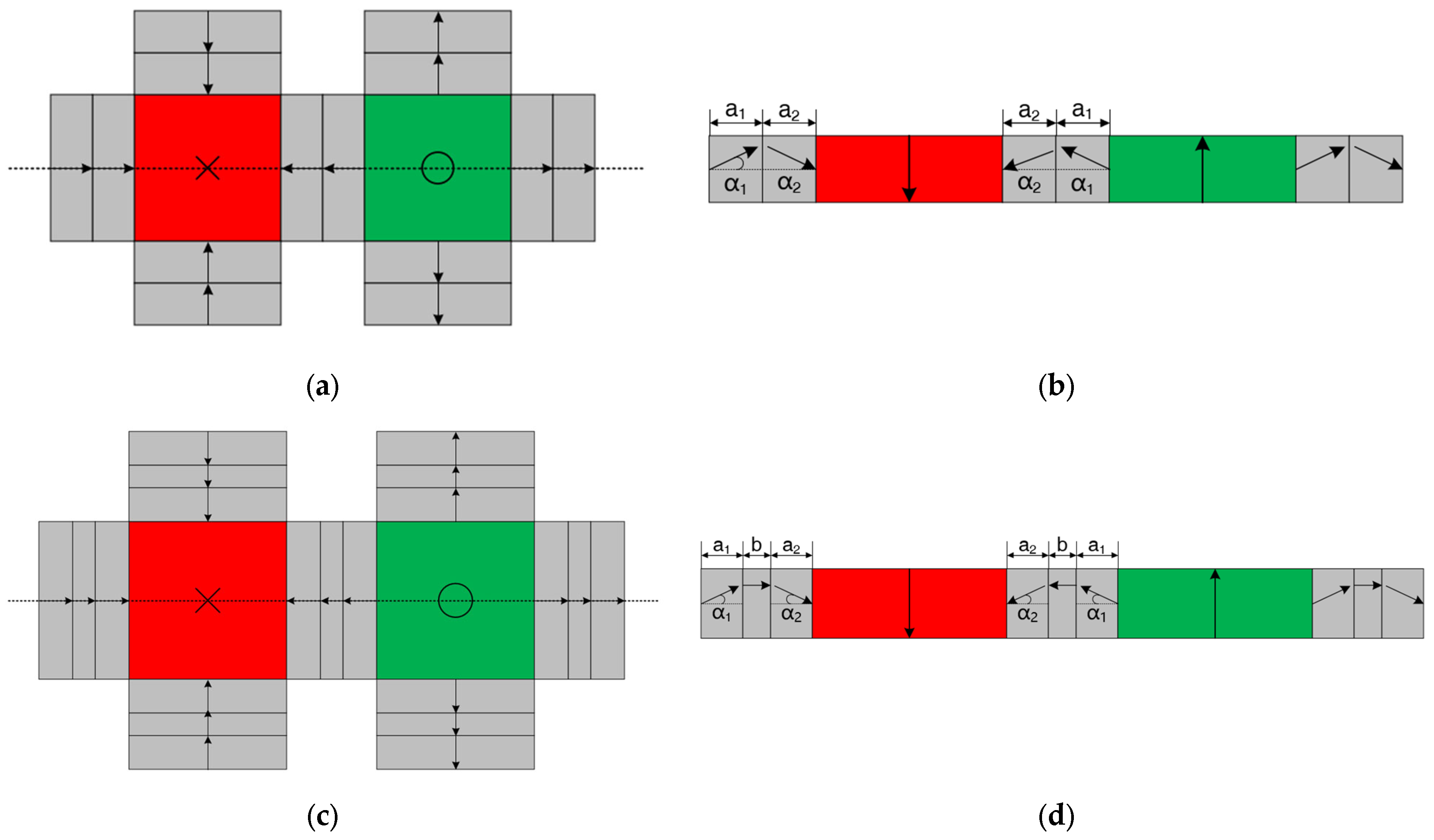
4. PMSPM with Auxiliary Split Permanent Magnet
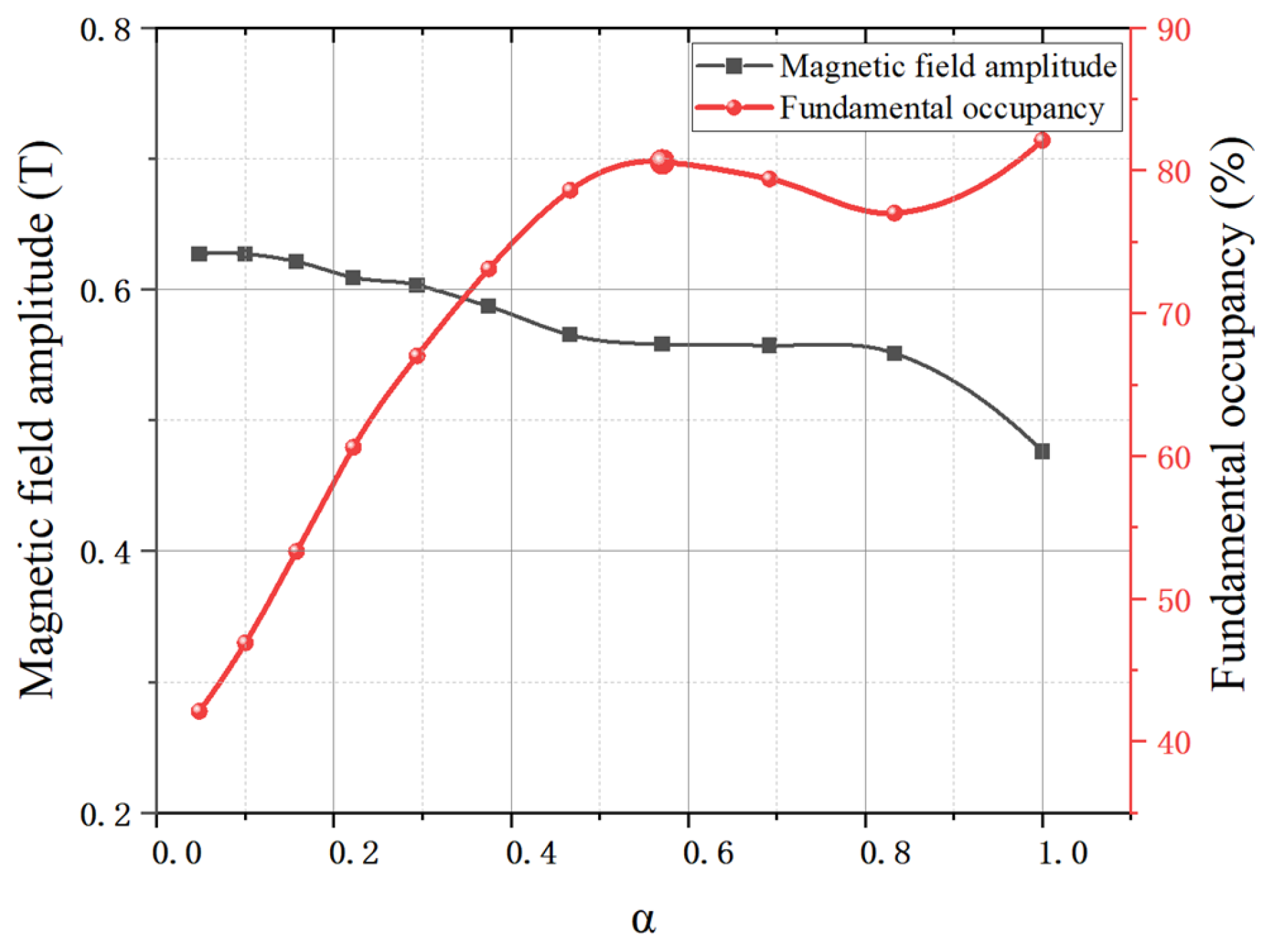
4.1. Novel Auxiliary Permanent Magnet Split Structure
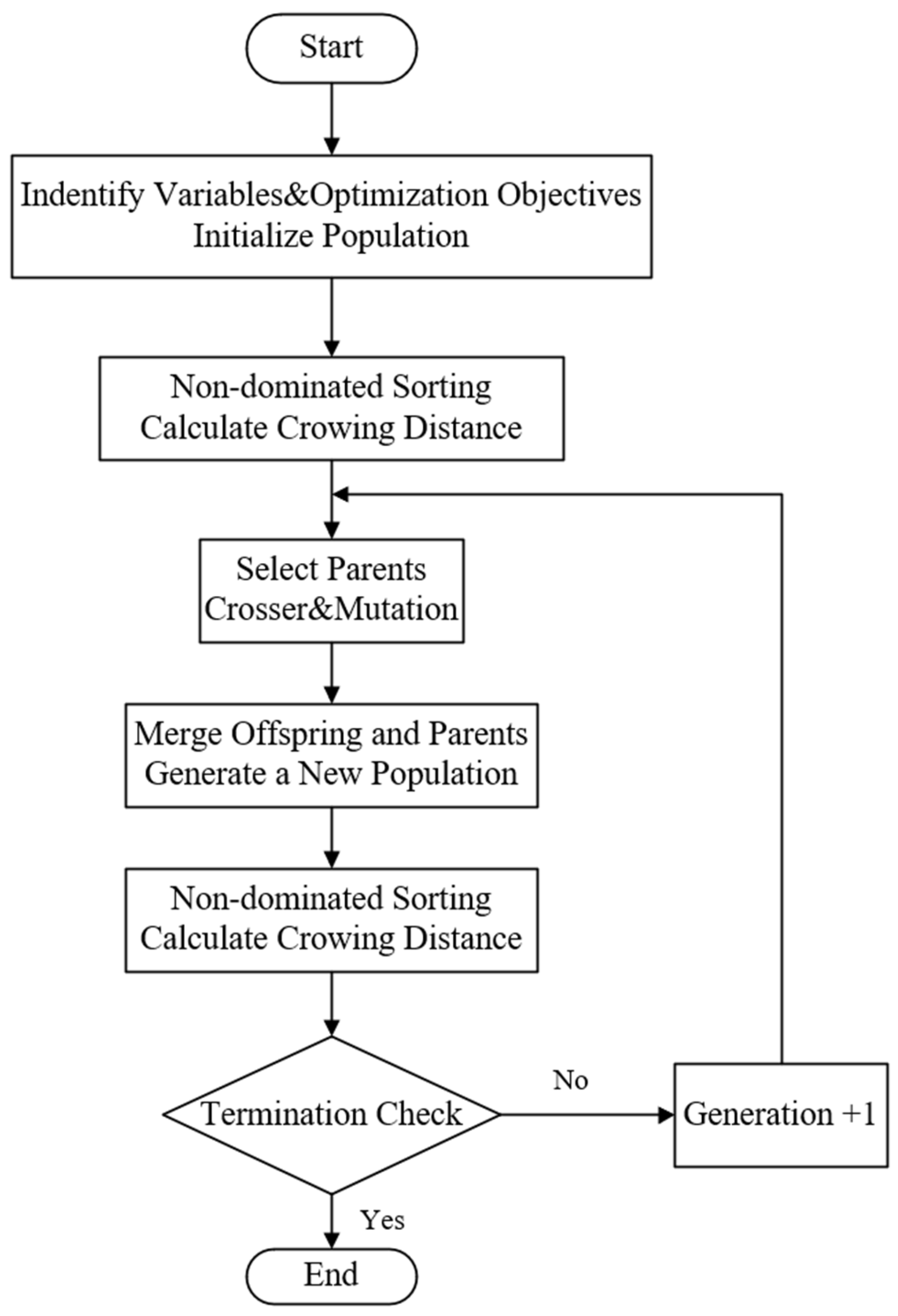
4.2. Multi-Objective Optimization Based on NSGA-II
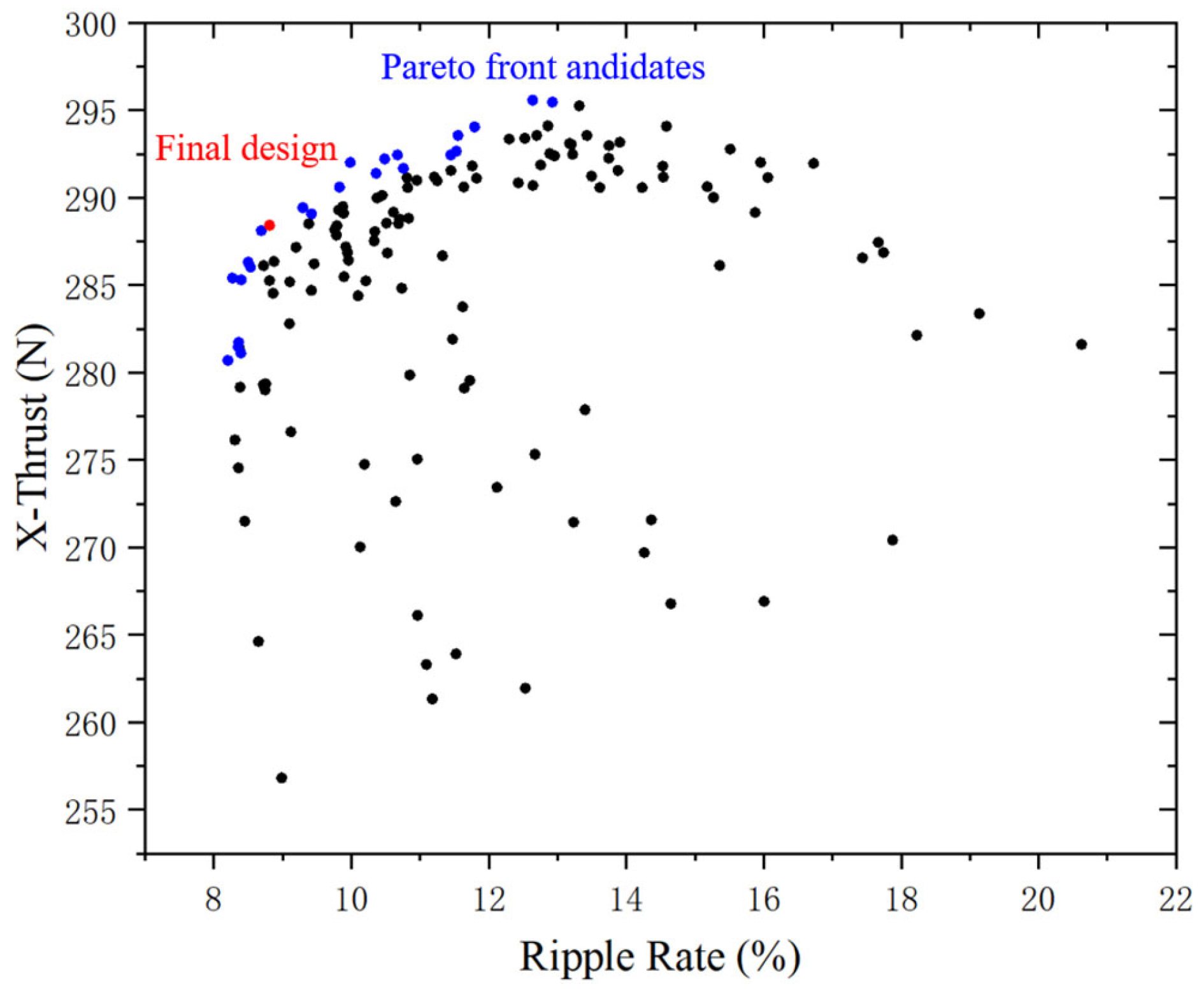
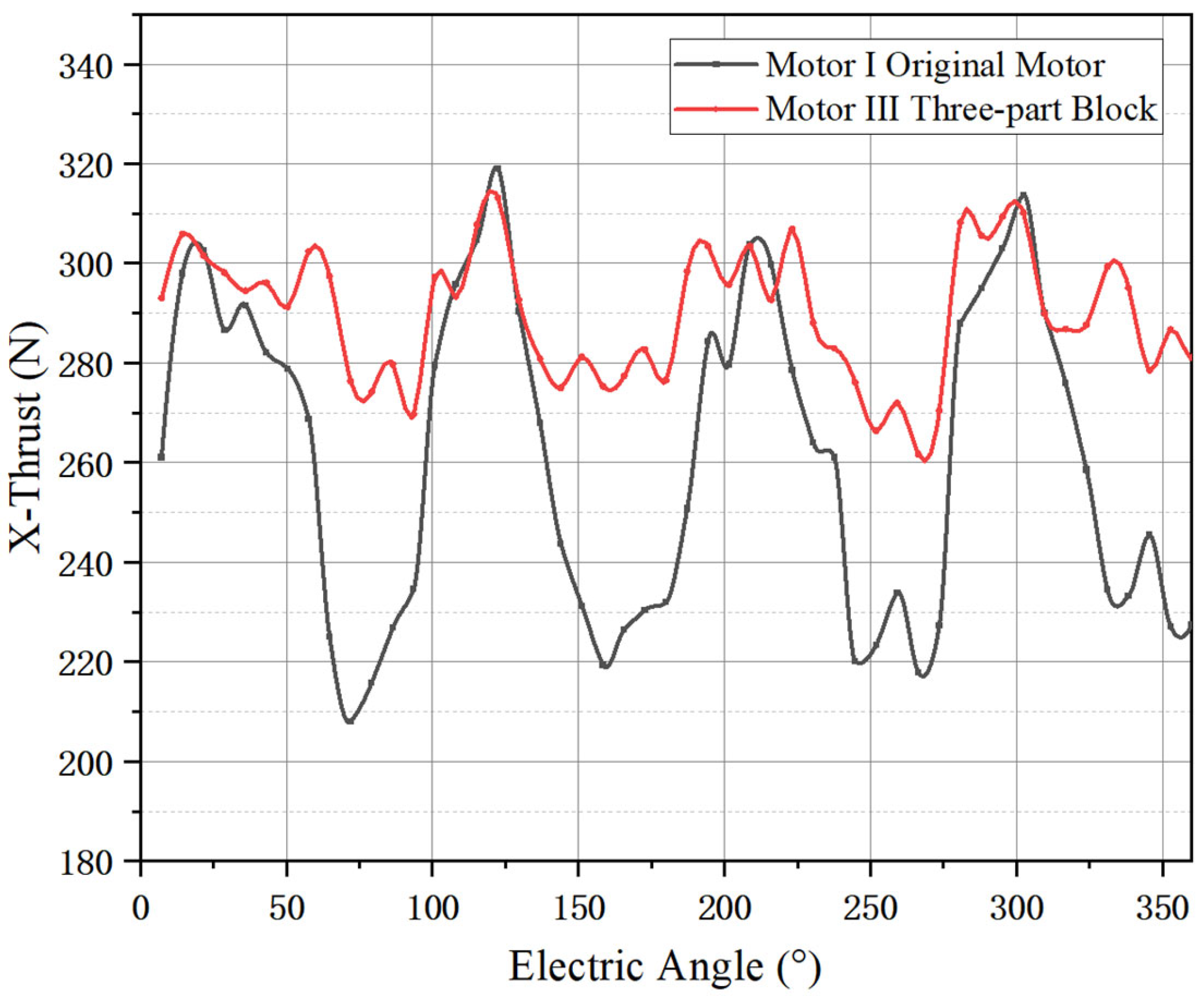
4.3. Final Optimization Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Rui, H.; Shihong, W.; Qiuying, Z.; Chunling, C.; Fengli, J.; Bo, H. Characteristics analysis of the synchronous permanent magnet planar motor with new permanent magnet array. In Proceedings of the 2012 IEEE International Conference on Mechatronics and Automation, Chengdu, China, 5–8 August 2012; pp. 904–908. [Google Scholar]
- Zhang, L.; Kou, B.; Li, L.; Zhao, B. Modeling and Design of an Integrated Winding Synchronous Permanent Magnet Planar Motor. IEEE Trans. Plasma Sci. 2013, 41, 1214–1219. [Google Scholar] [CrossRef]
- Min, W.; Zhang, M.; Zhu, Y.; Liu, F.; Duan, G.; Hu, J.; Yin, W. Analysis and Design of Novel Overlapping Ironless Windings for Planar Motors. IEEE Trans. Magn. 2011, 47, 4635–4642. [Google Scholar] [CrossRef]
- Zhu, H.; Teo, T.J.; Pang, C.K. Analysis of force harmonics and eddy current damping for 2 DOF moving magnet linear motor. In Proceedings of the IECON 2015—41st Annual Conference of the IEEE Industrial Electronics Society, Yokohama, Japan, 9–12 November 2015; pp. 001055–001060. [Google Scholar]
- Xu, F.; Peng, R.; Zheng, T.; Xu, X. Development and Validation of Numerical Magnetic Force and Torque Model for Magnetically Levitated Actuator. IEEE Trans. Magn. 2019, 55, 4900109. [Google Scholar] [CrossRef]
- Jansen, J.W.; van Lierop, C.M.M.; Lomonova, E.A.; Vandenput, A.J.A. Modeling of Magnetically Levitated Planar Actuators With Moving Magnets. IEEE Trans. Magn. 2007, 43, 15–25. [Google Scholar] [CrossRef]
- Jansen, J.W.; van Lierop, C.M.M.; Lomonova, E.A.; Vandenput, A.J.A. Magnetically Levitated Planar Actuator with Moving Magnets. IEEE Trans. Ind. Appl. 2008, 44, 1108–1115. [Google Scholar] [CrossRef]
- Hofmann, T.; Huepfel, M.; de Haas, O.; Jocher, A. Scalable construction, and test of optimal linear Halbach arrays for mobile applications. J. Magn. Magn. Mater. 2024, 596, 171967. [Google Scholar] [CrossRef]
- Zhang, Y.; Ding, K.; Du, S. Design Study of a High Loading Superconducting Magnetically Levitated Planar Motor. IEEE Trans. Appl. Supercond. 2021, 31, 3601504. [Google Scholar] [CrossRef]
- Zhu, H.; Pang, C.K.; Teo, T.J.; Marek, L.T. Modeling and design of a size and mass reduced magnetically levitated planar positioner. In Proceedings of the IECON 2014—40th Annual Conference of the IEEE Industrial Electronics Society, Dallas, TX, USA, 29 October–1 November 2014; pp. 2366–2371. [Google Scholar]
- Raich, H.; Blümler, P. Design and construction of a dipolar Halbach array with a homogeneous field from identical bar magnets: Magnetic Resonance Imaging applications. Magn. Reson. Mater. Phys. Biol. Med. 2004, 17, 318–329. [Google Scholar]
- Park, S.; Kim, D.; Lee, J. Topology optimization of Halbach arrays for suppressing local magnetic field fluctuations using finite element analysis and genetic algorithms. J. Magn. Magn. Mater. 2021, 537, 168215. [Google Scholar]
- Jizhong, C.; Yiming, Z.; Chunyan, X. A More Homogeneous, Less Massive Halbach Magnet array for Portable NMR. In Proceedings of the 2007 8th International Conference on Electronic Measurement and Instruments, Xi’an, China, 16–18 August 2007; pp. 1-330–1-336. [Google Scholar]
- Yu, Z.; Yan, L.; Zhou, Y.; Liu, J.; He, X.; Aboelhassan, A. Performance Analysis of PMSM Based on Improved Trapezoidal Halbach Magnet Arrays. In Proceedings of the 2022 IEEE 17th Conference on Industrial Electronics and Applications (ICIEA), Chengdu, China, 16–19 December 2022; pp. 1267–1271. [Google Scholar]
- Niu, L.; Zhang, S.; Tang, Y.; Hu, W. Optimization of flux density distribution for planar motor Halbach permanent magnet array. In Proceedings of the 2020 7th International Forum on Electrical Engineering and Automation (IFEEA), Hefei, China, 25–27 September 2020; pp. 534–538. [Google Scholar]
- Jiao, Z.; Wang, T.; Yan, L. Design of a Tubular Linear Oscillating Motor with a Novel Compound Halbach Magnet Array. IEEE/ASME Trans. Mechatron. 2017, 22, 498–508. [Google Scholar] [CrossRef]
- Bashyam, A.; Li, M.; Cima, M.J. Design and experimental validation of Unilateral Linear Halbach magnet arrays for single-sided magnetic resonance. J. Magn. Reson. 2018, 292, 36–43. [Google Scholar] [CrossRef] [PubMed]
- Habib, A.; Che, H.S.; Rahim, N.A.; Tousizadeh, M.; Sulaiman, E. A fully coreless Multi-Stator Multi-Rotor (MSMR) AFPM generator with combination of conventional and Halbach magnet arrays. Alex. Eng. J. 2020, 59, 589–600. [Google Scholar] [CrossRef]
- Cao, J.; Zhu, Y.; Wang, J.; Yin, W.; Duan, G. Analysis and comparison of two-dimensional permanent-magnet arrays for planar motor. IEEE Trans. Magn. 2004, 40, 3490–3494. [Google Scholar] [CrossRef]
- Ni, Y.; Jiang, X.; Xiao, B.; Wang, Q. Analytical Modeling and Optimization of Dual-Layer Segmented Halbach Permanent-Magnet Machines. IEEE Trans. Magn. 2020, 56, 8100811. [Google Scholar] [CrossRef]




| Symbol | Quantity | Value |
|---|---|---|
| hm | magnetic thickness | 10 mm |
| τm | main magnetic width | 14 mm |
| τ | magnetic polar distance | 22 mm |
| τn | magnetic polar distance rotates 45° | mm |
| hc | coil height | 7.1 mm |
| wc | coil thickness | 10.15 mm |
| lin | inner circle distance | 3 τn |
| lout | outer circle distance | 5 τn |
| gap | air gap height | 1 mm |
| Case | b | a | X-Thrust | Ripple Rate |
|---|---|---|---|---|
| 1 | 3.47 | 13.4 | 230.84 | 10.9% |
| 2 | 3.73 | 10.8 | 229.41 | 15.3% |
| 3 | 3.01 | 18.2 | 239.04 | 11.5% |
| 4 | 2.22 | 14.9 | 257.39 | 18.7% |
| 5 | 2.06 | 5.8 | 270.42 | 17.8% |
| 6 | 2.69 | 5.2 | 263.31 | 11.1% |
| 7 | 3.30 | 15.9 | 231.67 | 12.8% |
| 8 | 2.72 | 1.6 | 288.83 | 10.8% |
| Symbol | Quantity | Value |
|---|---|---|
| a1, a2 | auxiliary PM split block first, third width | 2.7 mm |
| b | middle width of auxiliary PM split block | 2.6 mm |
| α1, α2 | auxiliary PM block magnetization angle | 48° |
| Motor | Thrust | Thrust Density | Ripple Rate |
|---|---|---|---|
| I | 264 | 13.4 | 19.4% |
| II | 277 | 10.8 | 15.3% |
| III | 288 | 18.2 | 8.8% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, R.; Zhang, L.; Shi, C.; Zhao, C.; Yang, K. Analysis and Optimization of a Moving Magnet Permanent Magnet Synchronous Planar Motor with Split Halbach Arrays. Energies 2025, 18, 1388. https://doi.org/10.3390/en18061388
Wang R, Zhang L, Shi C, Zhao C, Yang K. Analysis and Optimization of a Moving Magnet Permanent Magnet Synchronous Planar Motor with Split Halbach Arrays. Energies. 2025; 18(6):1388. https://doi.org/10.3390/en18061388
Chicago/Turabian StyleWang, Ronglu, Lu Zhang, Chenyang Shi, Chunqiu Zhao, and Kai Yang. 2025. "Analysis and Optimization of a Moving Magnet Permanent Magnet Synchronous Planar Motor with Split Halbach Arrays" Energies 18, no. 6: 1388. https://doi.org/10.3390/en18061388
APA StyleWang, R., Zhang, L., Shi, C., Zhao, C., & Yang, K. (2025). Analysis and Optimization of a Moving Magnet Permanent Magnet Synchronous Planar Motor with Split Halbach Arrays. Energies, 18(6), 1388. https://doi.org/10.3390/en18061388







