Optimization of Heat Transfer Performances Within Porous Solar Receivers—A Comprehensive Review
Abstract
1. Introduction
2. Optimization of Porous Solar Receivers
2.1. Open Porous Solar Receivers
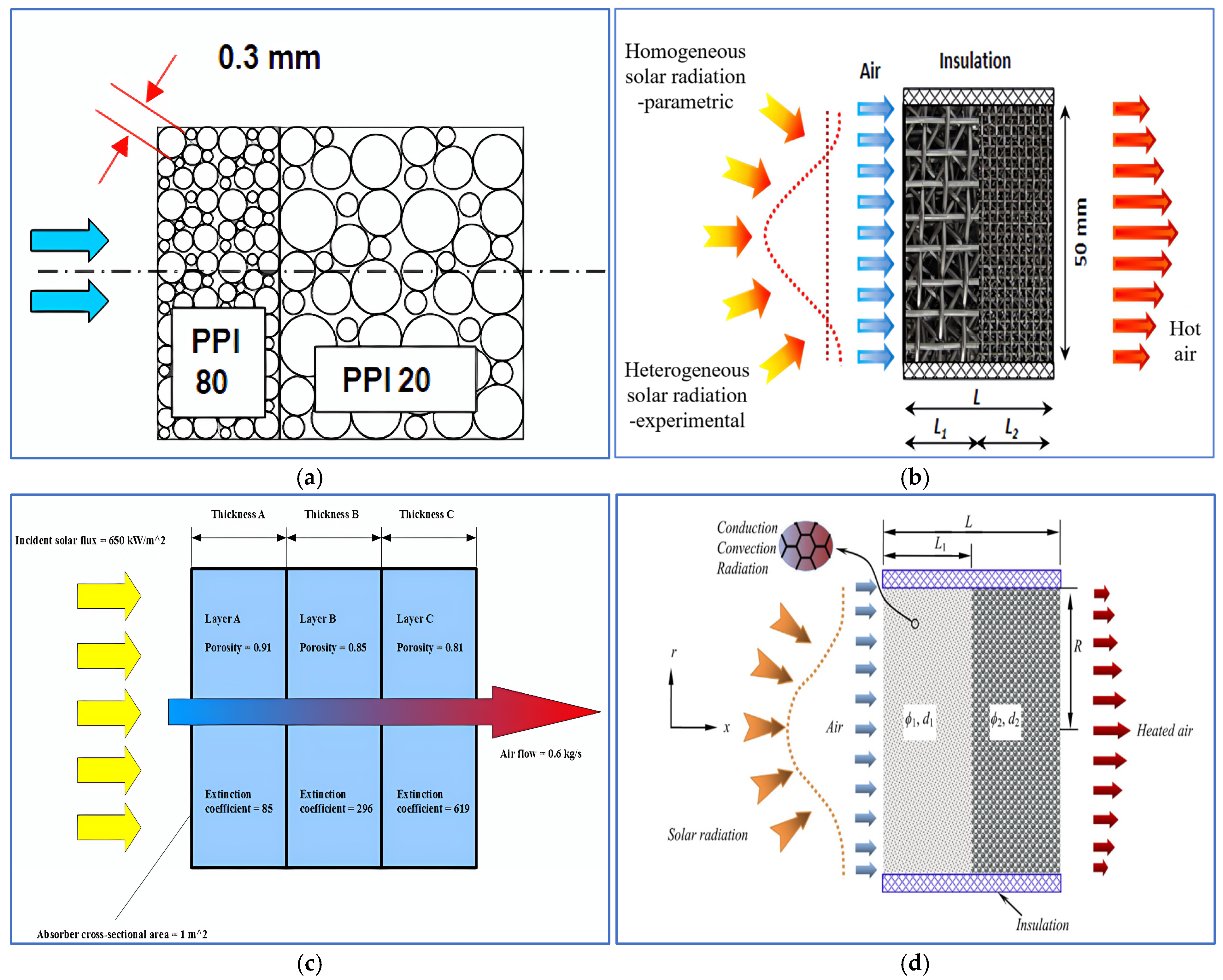
2.1.1. Optimization of Pore Parameters
| Reference | Year | Method | Material | Direction | Configuration | Pore Parameters |
|---|---|---|---|---|---|---|
| Fend et al. [17] | 2004 | Experiment | Sic | Axial | Double-layer | Porosity |
| Avila-Marin et al. [20] | 2014 | Experiment | Wire meshes | Double-layer | Porosity | |
| Avila-Marin et al. [18] | 2019 | Simulation | Wire meshes | Multi-layer | Porosity | |
| Avila-Marin et al. [19] | 2022 | Experiment Simulation | Wire meshes | Double-layer | Porosity | |
| Zaversky et al. [21] | 2017 | Experiment Simulation | Ceramic foam | Multi-layer | Porosity, PPI | |
| Chen et al. [22] | 2015 | Simulation | Ceramic foam | Double-layer | Cell size, porosity | |
| Chen et al. [23] | 2017 | Simulation | Ceramic foam | Radial | Composite | Cell size, porosity |
| Chen et al. [24] | 2019 | Simulation | Metal foams | Double-layer | Porosity, PPI | |
| M. Pelanconi et al. [25] | 2019 | Experiment Simulation | Al2O3 | Composite | Cell size | |
| Du et al. [26] | 2020 | Experiment Simulation | Inconel 718 | Multi-layer | Porosity, PPI | |
| Li et al. [27] | 2021 | Simulation | Porous material | Bidirectional | Multi-layer | Pore diameter, porosity |
| Sonika Sharma et al. [28] | 2022 | Simulation | Porous material | Multi-layer | Pore diameter, porosity | |
| Sonika Sharma et al. [29] | 2024 | Simulation | Porous material | Multi-layer | Pore diameter, porosity |
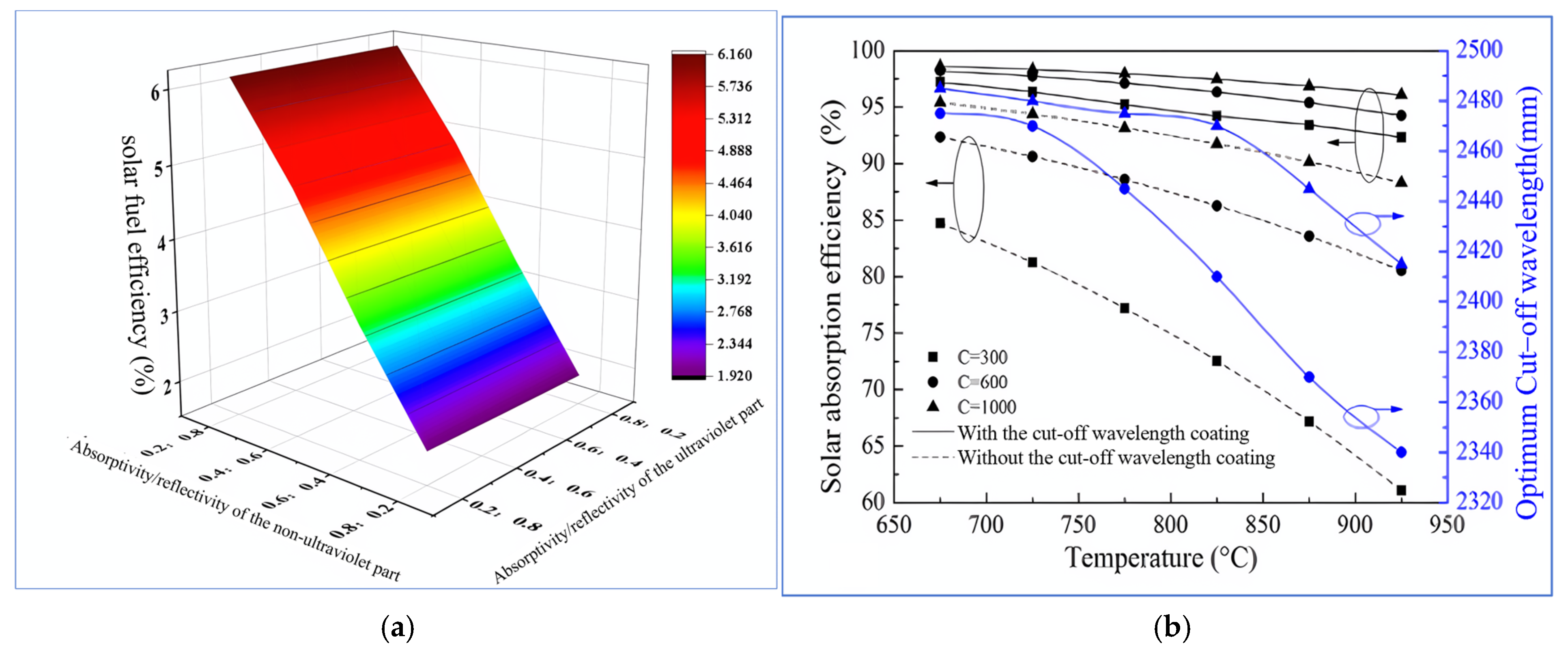
2.1.2. Optimization of Optical Properties
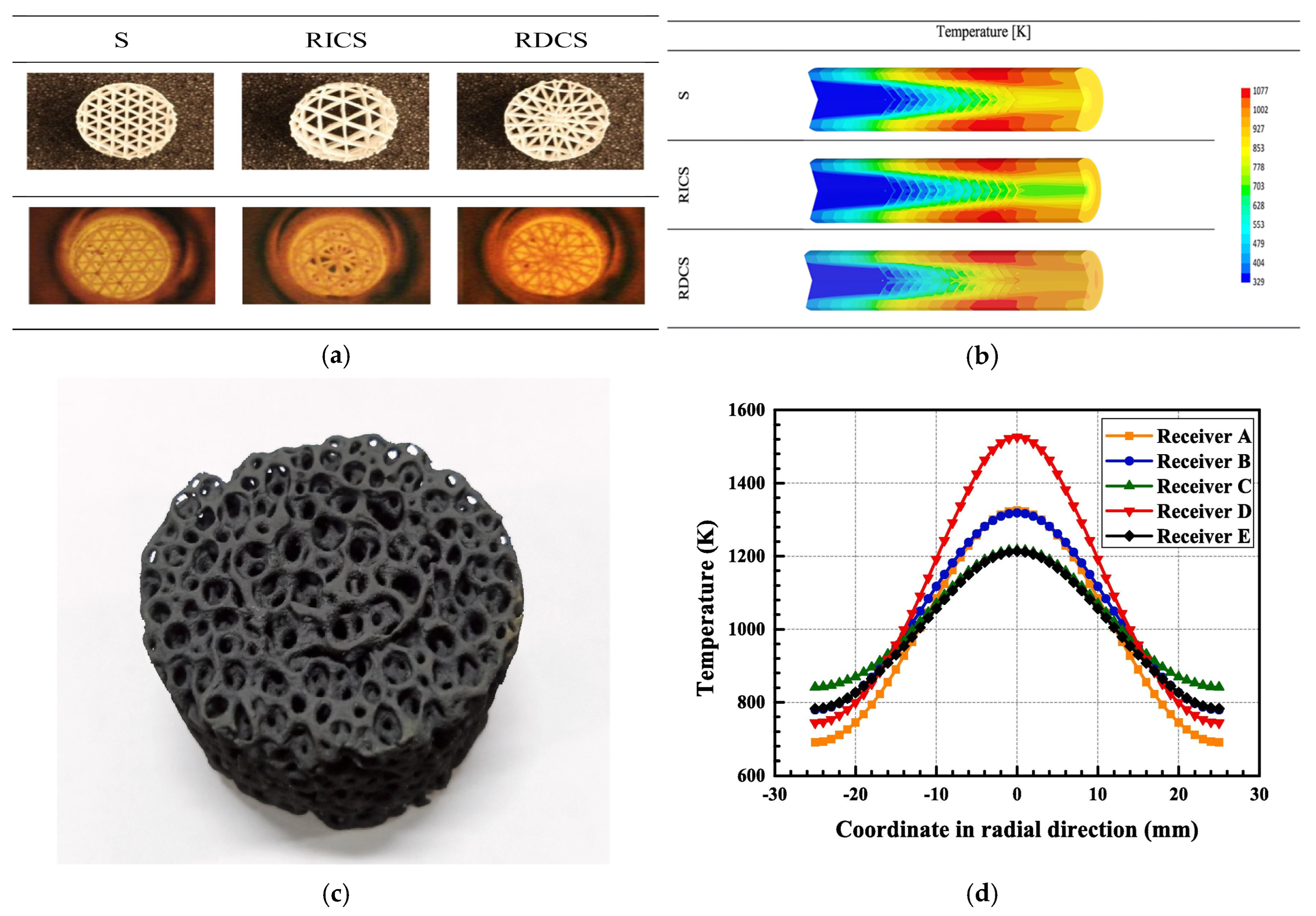
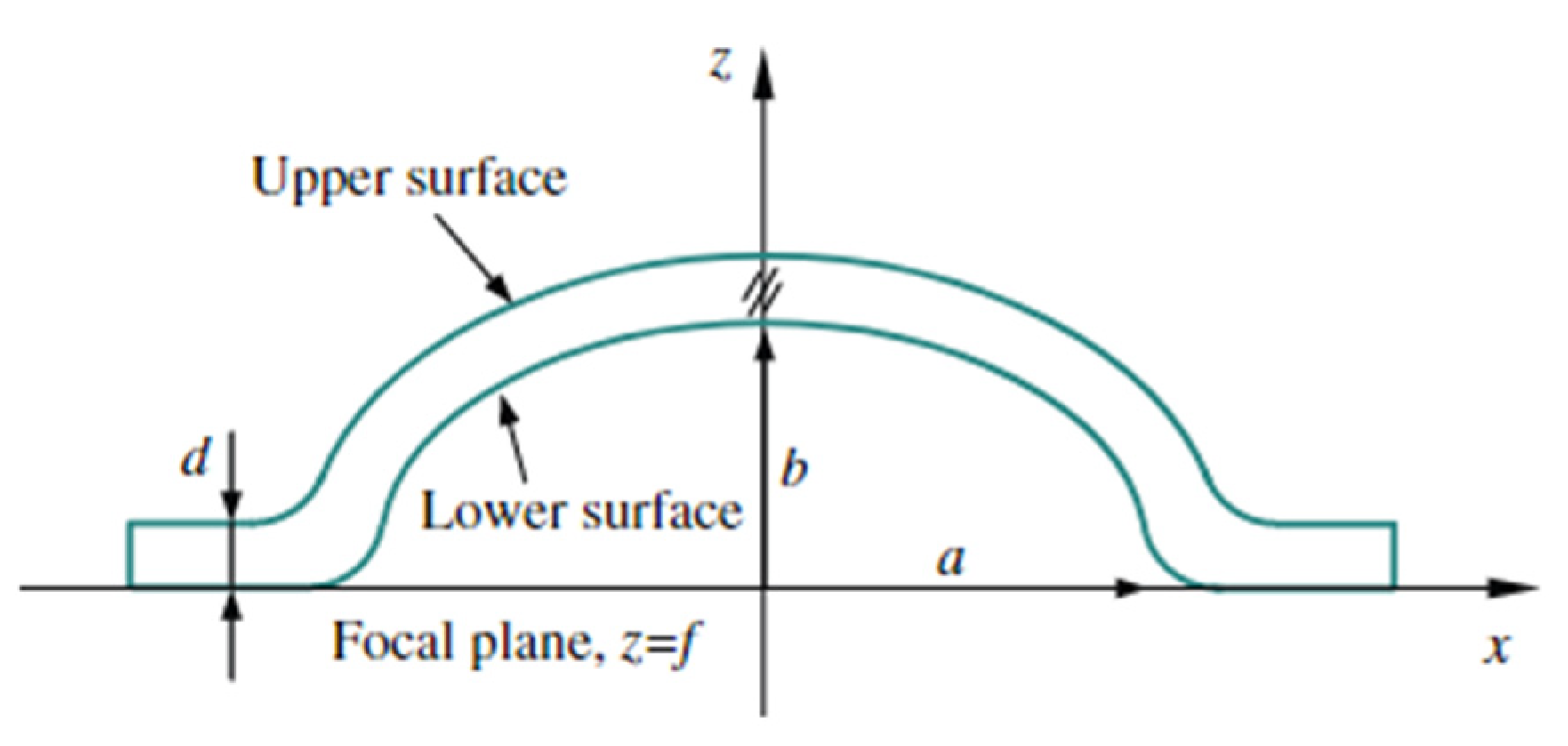
2.1.3. Optimization of the Configuration Shape
2.2. Optimization of Pressured Solar Porous Receivers
2.2.1. Optimization of Pore Parameters
2.2.2. Optimization of Optical Properties
2.2.3. Optimization of the Configuration Shape
| Reference | Year | Material | Optical Window Shape |
|---|---|---|---|
| Karni et al. [40] | 1998 | quartz glass | Frustum of a circular cone window |
| Maag and Steinfeld et al. [55] | 2011 | quartz glass; sapphire | Flat window |
| Dai et al. [54] | 2014 | quartz glass; sapphire | Ellipsoidal curved optical window |
| Li et al. [57] | 2019 | quartz glass | Dual window |
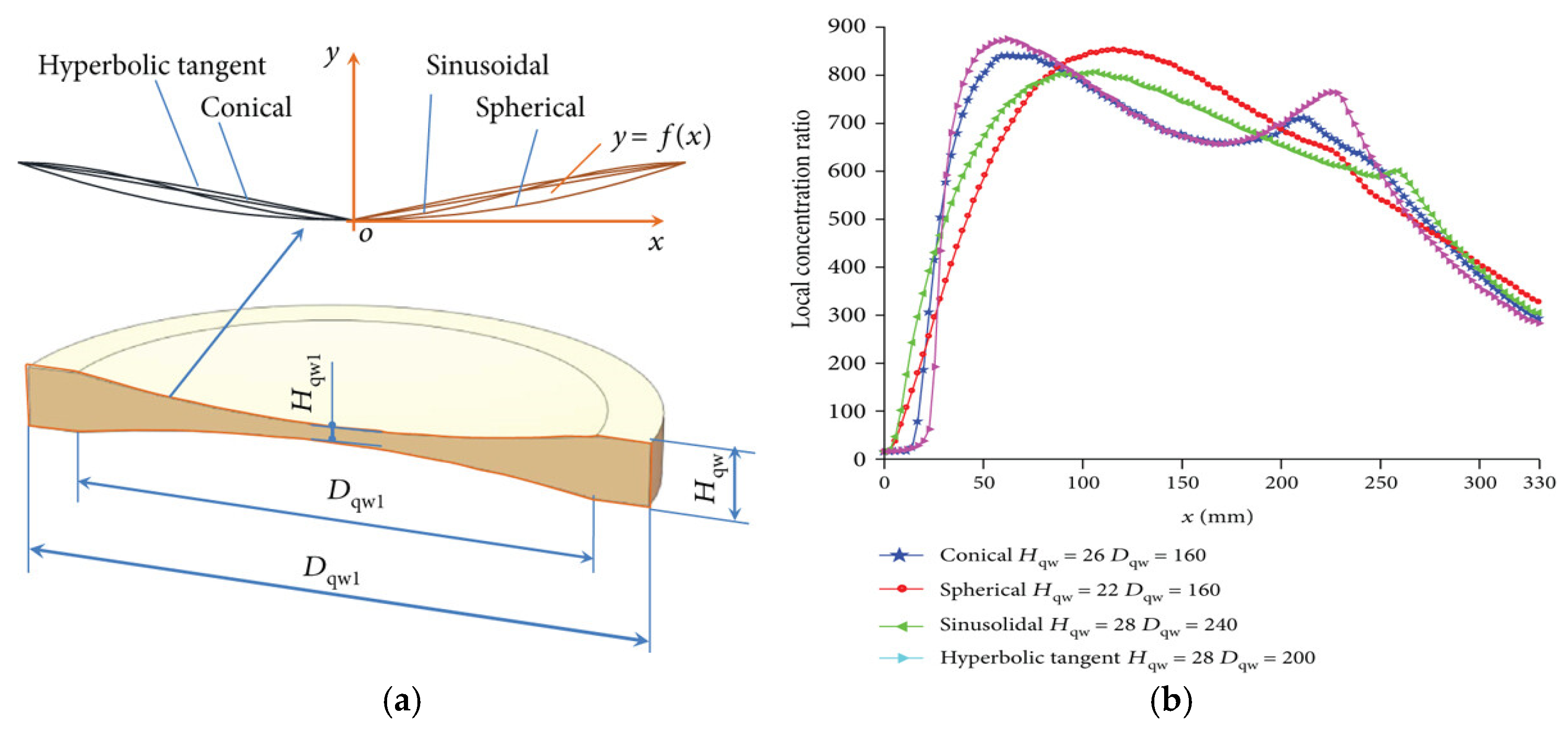
| Nie et al. [56] | 2020 | quartz glass | Four types of concave quartz windows (conical, spherical, sinusoidal, and hyperbolic tangent shapes) |
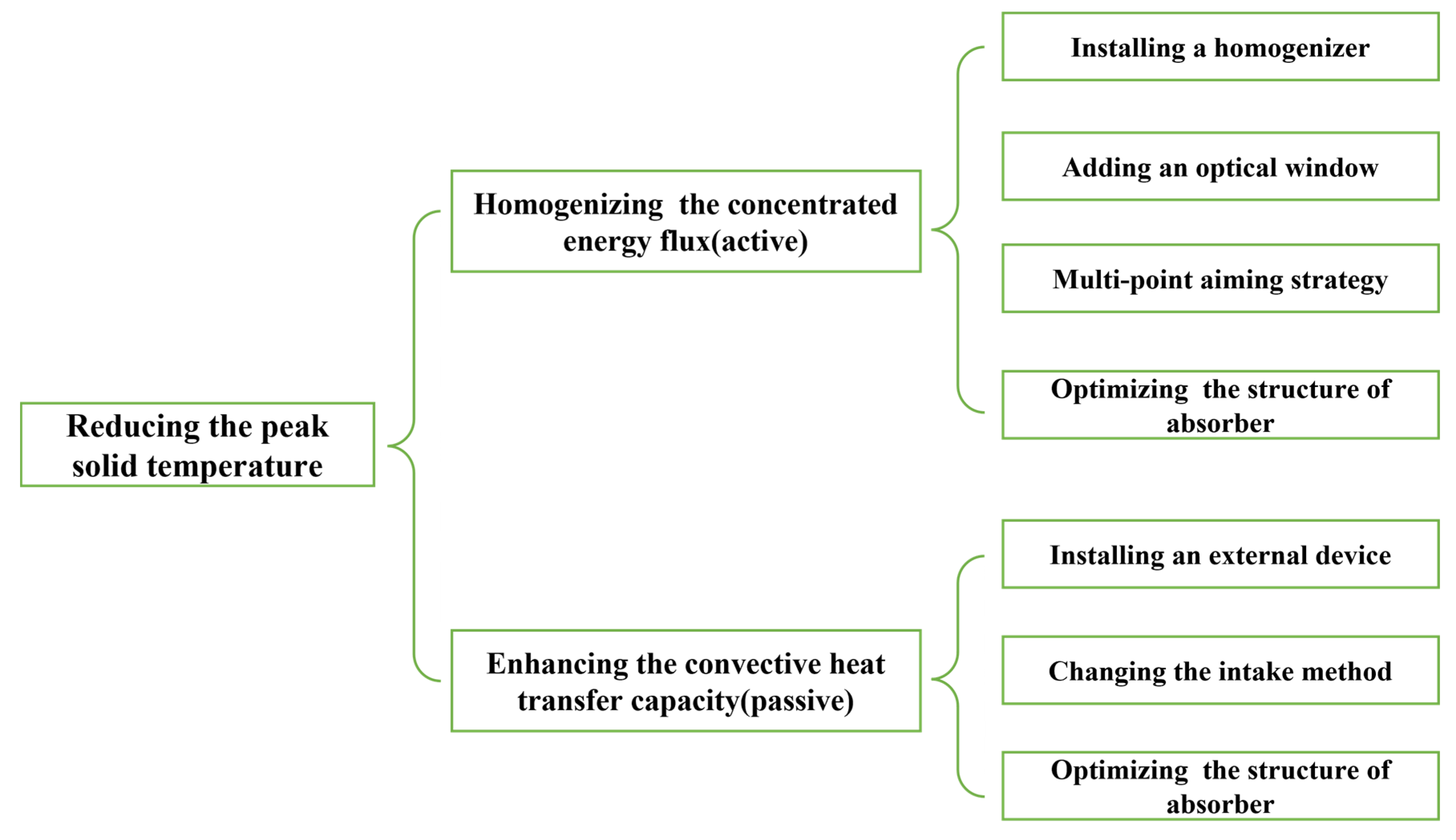
3. Reducing the Peak Solid Temperature
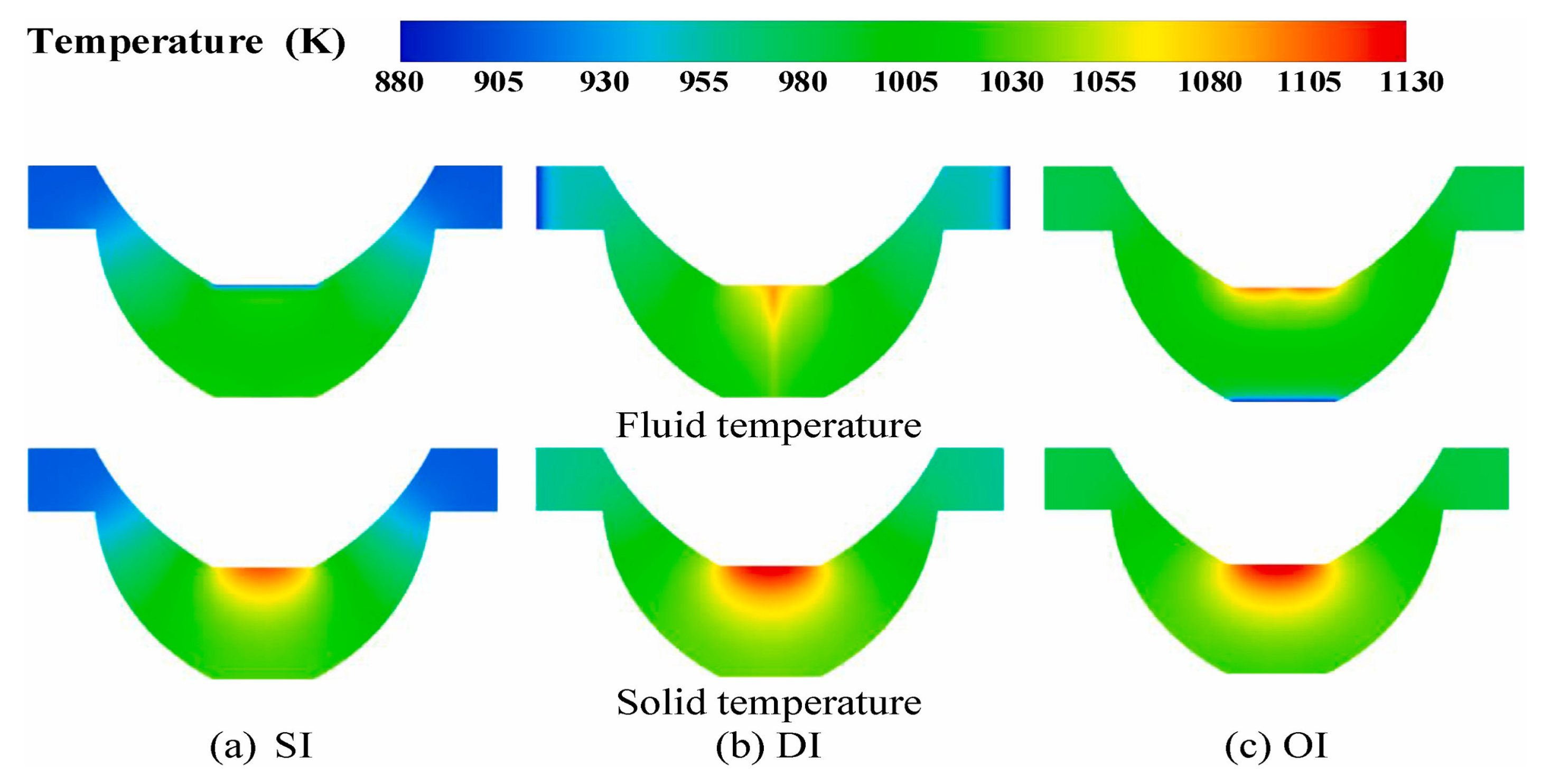
3.1. Homogenizing the Concentrated Energy Flux
3.2. Enhancing the Mass Flow of the HTF
4. Heat Transfer Model
4.1. Convection Heat Transfer
- (1)
- Volume-Averaging Method
- (2)
- Pore-scale model
4.2. Radiation Heat Transfer
4.3. Optimization Method
4.4. Experimental Measurement
5. Outlook and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Acronyms
| CPC | compound parabolic concentrator |
| CSF | concentrated solar flux |
| DOM | discrete ordinates method |
| DSDC | discrete solar dish concentrator |
| FEM | finite element method |
| FVM | finite volume method |
| HTF | heat transfer fluid |
| LTE | local thermal equilibrium |
| LTNE | local thermal non-equilibrium |
| MCM | Monte Carlo method |
| OPSR | open porous solar receivers |
| PCA | porous catalytic absorber |
| PEC | performance evaluation criterion |
| PSR | porous solar receivers |
| PSM | pore scale method |
| PPSR | pressured porous solar receivers |
| Ro | Rosseland |
| RTE | radiation transfer equation |
| SSR | surface solar receivers |
| VAM | volume-averaging method |
| VSR | volumetric solar receivers |
| Greek Symbols | |
| a | absorption coefficient |
| cp | specific heat |
| dp | pore parameter (m) |
| G | projected radiation |
| hv | volumetric convective heat transfer coefficient (W/(m2·K)) |
| total radiation intensity in each direction | |
| k | conductivity |
| n | refractive index |
| p | pressure (Pa) |
| RDi-j | radiative transfer factor |
| Sr | energy source term (W/m3) |
| unit vector in the direction of scattering | |
| unit vector in the direction of the incident radiation | |
| T | temperature (K) |
| U | superficial velocity (m/s) |
| u | velocity (m/s) |
| β | attenuation coefficient |
| ϕ | porosity |
| isotropic phase function | |
| λ | thermal conductivity (W/(m·K)) |
| μf | dynamic viscosity (Pa·s) |
| fluid density (kg/m3) | |
| reflectivity of the skeleton of the porous foam | |
| σ | Stefan–Boltzmann constant |
| σs | scattering coefficient |
| Subscripts | |
| eff | effective value |
| f | fluid |
| s | solid |
References
- He, Y.-L.; Du, S.; Shen, S. Advances in porous volumetric solar receivers and enhancement of volumetric absorption. Energy Rev. 2023, 2, 100035. [Google Scholar] [CrossRef]
- Avila-Marin, A.L.; Fernandez-Reche, J.; Martinez-Tarifa, A. Modelling strategies for porous structures as solar receivers in central receiver systems: A review. Renew. Sustain. Energy Rev. 2019, 111, 15–33. [Google Scholar] [CrossRef]
- Capuano, R.; Fend, T.; Schwarzbözl, P.; Smirnova, O.; Stadler, H.; Hoffschmidt, B.; Pitz-Paal, R. Numerical models of advanced ceramic absorbers for volumetric solar receivers. Renew. Sustain. Energy Rev. 2016, 58, 656–665. [Google Scholar] [CrossRef]
- Ávila-Marín, A.L. Volumetric receivers in Solar Thermal Power Plants with Central Receiver System technology: A review. Sol. Energy 2011, 85, 891–910. [Google Scholar] [CrossRef]
- Ho, C.K.; Iverson, B.D. Review of high-temperature central receiver designs for concentrating solar power. Renew. Sustain. Energy Rev. 2014, 29, 835–846. [Google Scholar] [CrossRef]
- Hachicha, A.A.; Yousef, B.A.; Said, Z.; Rodríguez, I. A review study on the modeling of high-temperature solar thermal collector systems. Renew. Sustain. Energy Rev. 2019, 112, 280–298. [Google Scholar] [CrossRef]
- Fend, T.; Hoffschmidt, B.; Pitz-Paal, R.; Reutter, O.; Rietbrock, P. Porous materials as open volumetric solar receivers: Experimental determination of thermophysical and heat transfer properties. Energy 2004, 29, 823–833. [Google Scholar] [CrossRef]
- Dai, G.; Huangfu, J.; Wang, X.; Du, S.; Zhao, T. A Review of Radiative Heat Transfer in Fixed-Bed Particle Solar Receivers. Sustainability 2023, 15, 9918. [Google Scholar] [CrossRef]
- Sedighi, M.; Padilla, R.V.; Lake, M.; Rose, A.; Lim, Y.Y.; Novak, J.P.; Taylor, R.A. Design of high-temperature atmospheric and pressurised gas-phase solar receivers: A comprehensive review on numerical modeling and performance parameters. Sol. Energy 2020, 201, 701–723. [Google Scholar] [CrossRef]
- Kribus, A.; Gray, Y.; Grijnevich, M.; Mittelman, G.; Mey-Cloutier, S.; Caliot, C. The promise and challenge of solar volumetric absorbers. Sol. Energy 2014, 110, 463–481. [Google Scholar] [CrossRef]
- Zhang, W.; Li, Q.; Qiu, Y. Design and optimization of a solar-driven methane dry reforming reactor by developing an optical-thermal-chemical model. Chem. Eng. J. 2024, 483, 149094. [Google Scholar] [CrossRef]
- Ma, T.; Fu, M.; Cong, J.; Zhang, X.; Zhang, Q.; Sayfieva, K.F.; Chang, Z.; Li, X. Analysis of heat and mass transfer in a porous solar thermochemical reactor. Energy 2024, 294, 130842. [Google Scholar] [CrossRef]
- Lougou, B.G.; Wu, L.; Ma, D.; Geng, B.; Jiang, B.; Han, D.; Zhang, H.; Łapka, P.; Shuai, Y. Efficient conversion of solar energy through a macroporous ceramic receiver coupling heat transfer and thermochemical reactions. Energy 2023, 271, 126989. [Google Scholar] [CrossRef]
- Li, M.J.; Tao, W.Q. Review of methodologies and polices for evaluation of energy efficiency in high energy-consuming industry. Appl. Energy 2017, 187, 203–215. [Google Scholar] [CrossRef]
- Wang, F.; Shuai, Y.; Tan, H.; Yu, C. Thermal performance analysis of porous media receiver with concentrated solar irradiation. Int. J. Heat Mass Transf. 2013, 62, 247–254. [Google Scholar] [CrossRef]
- Huang, H.; Lin, M. Optimization of solar receivers for high-temperature solar conversion processes: Direct vs. Indirect illumination designs. Appl. Energy 2021, 304, 117675. [Google Scholar] [CrossRef]
- Fend, T.; Pitz-Paal, R.; Reutter, O.; Hoffschmidt, B. Two novel high-porosity materials as volumetric receivers for concentrated solar radiation. Sol. Energy Mater. Sol. Cells 2004, 84, 291–304. [Google Scholar] [CrossRef]
- Avila-Marin, A.L.; Fernandez-Reche, J.; Caliot, C.; Martinez-Tarifa, A.; de Lara, M.A. CFD numerical model for open volumetric receivers with graded porosity dense wire meshes and experimental validation. AIP Conf. Proc. 2019, 2126, 030005. [Google Scholar]
- Avila-Marin, A.L.; Alvarez-Lara, M.; Fernandez-Reche, J. Experimental Results of Gradual Porosity Wire Mesh Absorber for Volumetric Receivers. Energy Procedia 2014, 49, 275–283. [Google Scholar] [CrossRef]
- Avila-Marin, A.L. CFD parametric analysis of wire meshes open volumetric receivers with axial-varied porosity and comparison with small-scale solar receiver tests. Renew. Energy 2022, 193, 1094–1105. [Google Scholar] [CrossRef]
- Zaversky, F.; Aldaz, L.; Sánchez, M.; Ávila-Marín, A.L.; Roldán, M.I.; Fernández-Reche, J.; Füssel, A.; Beckert, W.; Adler, J. Numerical and experimental evaluation and optimization of ceramic foam as solar absorber—Single-layer vs. multi-layer configurations. Appl. Energy 2018, 210, 351–375. [Google Scholar] [CrossRef]
- Chen, X.; Xia, X.L.; Meng, X.L.; Dong, X.H. Thermal performance analysis on a volumetric solar receiver with double-layer ceramic foam. Energy Convers. Manag. 2015, 97, 282–289. [Google Scholar] [CrossRef]
- Chen, X.; Xia, X.L.; Yan, X.W.; Sun, C. Heat transfer analysis of a volumetric solar receiver with composite porous structure. Energy Convers. Manag. 2017, 136, 262–269. [Google Scholar] [CrossRef]
- Chen, X.; Xia, X.; Sun, C.; Wang, F.; Liu, R. Performance evaluation of a double-pipe heat exchanger with uniform and graded metal foams. Heat Mass Transf. 2020, 56, 291–302. [Google Scholar] [CrossRef]
- Pelanconi, M.; Barbato, M.; Zavattoni, S.; Vignoles, G.L.; Ortona, A. Thermal design, optimization and additive manufacturing of ceramic regular structures to maximize the radiative heat transfer. Mater. Des. 2019, 163, 107539. [Google Scholar] [CrossRef]
- Du, S.; Xia, T.; He, Y.L.; Li, Z.Y.; Li, D.; Xie, X.Q. Experiment and optimization study on the radial graded porous volumetric solar receiver matching non-uniform solar flux distribution. Appl. Energy 2020, 275, 115343. [Google Scholar] [CrossRef]
- Li, J.B.; Wang, P.; Liu, D.Y. Optimization on the gradually varied pore structure distribution for the irradiated absorber. Energy 2022, 240, 122787. [Google Scholar] [CrossRef]
- Sharma, S.; Talukdar, P. Thermo-mechanical analysis of a porous volumetric solar receiver subjected to concentrated solar radiation. Sol. Energy 2022, 247, 41–54. [Google Scholar] [CrossRef]
- Sharma, S.; Talukdar, P. Thermo-mechanical performance enhancement of volumetric solar receivers using graded porous absorbers. Energy 2024, 304, 132070. [Google Scholar] [CrossRef]
- Menigault, T.; Flamant, G.; Rivoire, B. Advanced high-temperature two-slab selective volumetric receiver. Sol. Energy Mater. 1991, 24, 192–203. [Google Scholar] [CrossRef]
- Zhu, Q.; Xuan, Y. Improving the performance of volumetric solar receivers with a spectrally selective gradual structure and swirling characteristics. Energy 2019, 172, 467–476. [Google Scholar] [CrossRef]
- Ali, M.; Rady, M.; Attia, M.A.; Ewais, E.M. Consistent coupled optical and thermal analysis of volumetric solar receivers with honeycomb absorbers. Renew. Energy 2020, 145, 1849–1861. [Google Scholar] [CrossRef]
- Dai, G.; Chen, X.; Zhuang, Y. Thermal radiation performances of solar volumetric absorbers made up of multiple tubes and porous. Acta Energiae Solaris Sin. 2022, 43, 186–190. [Google Scholar]
- Chen, X.; Lyu, J.; Sun, C.; Xia, X.; Wang, F. Pore-scale evaluation on a volumetric solar receiver with different optical property control strategies. Energy 2023, 278, 128006. [Google Scholar] [CrossRef]
- Du, S.; Li, M.J.; He, Y.L.; Shen, S. Conceptual design of porous volumetric solar receiver using molten salt as heat transfer fluid. Appl. Energy 2021, 301, 117400. [Google Scholar] [CrossRef]
- Du, S.; He, Y.L. Investigation and optimization on spectrally selective absorption for enhanced thermal performance in porous volumetric solar receivers. Sol. Energy Mater. Sol. Cells 2024, 277, 113135. [Google Scholar] [CrossRef]
- Luque, S.; Menéndez, G.; Roccabruna, M.; González-Aguilar, J.; Crema, L.; Romero, M. Exploiting volumetric effects in novel additively manufactured open solar receivers. Sol. Energy 2018, 174, 342–351. [Google Scholar] [CrossRef]
- Capuano, R.; Fend, T.; Stadler, H.; Hoffschmidt, B.; Pitz-Paal, R. Optimized volumetric solar receiver: Thermal performance prediction and experimental validation. Renew. Energy 2017, 114, 556–566. [Google Scholar] [CrossRef]
- Avila-Marin, A.L.; Fernandez-Reche, J.; Carballo, J.A.; Carra, M.E.; Gianella, S.; Ferrari, L.; Sanchez-Señoran, D. CFD analysis of the performance impact of geometrical shape on volumetric absorbers in a standard cup. Renew. Energy 2022, 201, 256–272. [Google Scholar] [CrossRef]
- Karni, J.; Kribus, A.; Ostraich, B.; Kochavi, E. A High-Pressure Window for Volumetric Solar Receivers. J. Sol. Energy Eng. 1998, 120, 101–107. [Google Scholar] [CrossRef]
- Wang, P.; Li, J.B.; Bai, F.W.; Liu, D.Y.; Xu, C.; Zhao, L.; Wang, Z.F. Experimental and theoretical evaluation on the thermal performance of a windowed volumetric solar receiver. Energy 2017, 119, 652–661. [Google Scholar] [CrossRef]
- Villafán-Vidales, H.; Abanades, S.; Caliot, C.; Romero-Paredes, H. Heat transfer simulation in a thermochemical solar reactor based on a volumetric porous receiver. Appl. Therm. Eng. 2011, 31, 3377–3386. [Google Scholar] [CrossRef]
- Wang, F.; Shi, X.; Zhang, C.; Cheng, Z.; Chen, X. Effects of non-uniform porosity on thermochemical performance of solar driven methane reforming. Energy 2020, 191, 116575. [Google Scholar] [CrossRef]
- Chen, X.; Wang, F.; Yan, X.; Han, Y.; Cheng, Z.; Jie, Z. Thermochemical performance of solar driven CO2 reforming of methane in volumetric reactor with gradual foam structure. Energy 2018, 151, 545–555. [Google Scholar] [CrossRef]
- Zhang, H.; Shuai, Y.; Lougou, B.G.; Jiang, B.; Yang, D.; Pan, Q.; Wang, F.; Huang, X. Effects of foam structure on thermochemical characteristics of porous-filled solar reactor. Energy 2022, 239, 122219. [Google Scholar] [CrossRef]
- Godini, A.; Kheradmand, S. Optimization of volumetric solar receiver geometry and porous media specifications. Renew. Energy 2021, 172, 574–581. [Google Scholar] [CrossRef]
- Yao, J.L.; Zhang, Z.Y.; Xu, B.Y.; Li, T.; Xie, T. Spectral properties regulation for the performance improvement of solar-driven methane dry reforming reactor based on the photothermal synergistic catalysis. Fuel 2025, 380, 133228. [Google Scholar] [CrossRef]
- Vicente, G.S.; Germán, N.; Farchado, M.; Morales, A. Antireflective coatings on quartz glass for high temperature solar receivers. AIP Conf. Proc. 2018, 2033, 220006. [Google Scholar]
- Röger, M.; Rickers, C.; Uhlig, R.; Neumann, F.; Polenzky, C. Infrared-Reflective Coating on Fused Silica for a Solar High-Temperature Receiver. J. Sol. Energy Eng. 2009, 131, 021004. [Google Scholar] [CrossRef]
- Jin, J.; Wei, X.; Liu, M.; Yu, Y.; Li, W.; Kong, H.; Hao, Y. A solar methane reforming reactor design with enhanced efficiency. Appl. Energy 2018, 226, 797–807. [Google Scholar] [CrossRef]
- Shuai, Y.; Xia, X.L.; Tan, H.P. Radiation performance of dish solar concentrator/cavity receiver systems. Sol. Energy 2008, 82, 13–21. [Google Scholar] [CrossRef]
- Meng, X.L.; Xia, X.L.; Sellami, N.; Mallick, T. Coupled heat transfer performance of a high temperature cup shaped porous absorber. Energy Convers. Manag. 2016, 110, 327–337. [Google Scholar] [CrossRef]
- Li, T.; Xie, L.; Zhao, B.; Shen, W.; Liu, Y. Analysis on the Effects of Different Receiver Structures and Porous Parameters on the Volumetric Effects and Heat Transfer Performance of Porous Volumetric Solar Receiver. Int. J. Energy Res. 2023, 2023, 3289428. [Google Scholar] [CrossRef]
- Dai, G.L.; Xia, X.L.; Hou, G.F. Transmission performances of solar windows exposed to concentrated sunlight. Sol. Energy 2014, 103, 125–133. [Google Scholar] [CrossRef]
- Maag, G.; Falter, C.; Steinfeld, A. Temperature of a Quartz/Sapphire Window in a Solar Cavity-Receiver. J. Sol. Energy Eng. 2011, 133, 014501. [Google Scholar] [CrossRef]
- Nie, D.Z.; Peng, Y.D.; Yan, J.; Mi, C.J.; Liu, Y.X.; Tian, Y. Improvement in the Flux Uniformity of the Solar Dish Concentrator System through a Concave Quartz Window. Int. J. Photoenergy 2020, 2020, 2421391. [Google Scholar] [CrossRef]
- Li, X.L.; Xia, X.L.; Li, Z.H.; Chen, X. Effects of double windows on optical and thermal performance of solar receivers under concentrated irradiation. Sol. Energy 2019, 184, 331–344. [Google Scholar] [CrossRef]
- Barreto, G.; Canhoto, P.; Collares-Pereira, M. Three-dimensional modelling and analysis of solar radiation absorption in porous volumetric receivers. Appl. Energy 2018, 215, 602–614. [Google Scholar] [CrossRef]
- Khanna, S.; Kedare, S.B.; Singh, S. Deflection and stresses in absorber tube of solar parabolic trough due to circumferential and axial flux variations on absorber tube supported at multiple points. Sol. Energy 2014, 99, 134–151. [Google Scholar] [CrossRef]
- Gray, V.; Truong-Ba, H.; Picotti, G.; Cholette, M.E. Using statistical analysis to understand creep modelling error and the ramifications for Solar Tower receivers. Sol. Energy Mater. Sol. Cells 2023, 257, 112408. [Google Scholar] [CrossRef]
- Walczak, M.; Pineda, F.; Fernández, Á.G.; Mata-Torres, C.; Escobar, R.A. Materials corrosion for thermal energy storage systems in concentrated solar power plants. Renew. Sustain. Energy Rev. 2018, 86, 22–44. [Google Scholar] [CrossRef]
- Perrero, M.; Papurello, D. Solar Disc Concentrator: Material Selection for the Receiver. Energies 2023, 16, 6870. [Google Scholar] [CrossRef]
- Salem, J.A.; Quinn, G.D. Failure Analysis of Sapphire Refractive Secondary Concentrators; NASA: Washington, DC, USA, 2009.
- He, Y.L.; Wang, K.; Qiu, Y.; Du, B.C.; Liang, Q.; Du, S. Review of the solar flux distribution in concentrated solar power: Non-uniform features, challenges, and solutions. Appl. Therm. Eng. 2019, 149, 448–474. [Google Scholar] [CrossRef]
- Yan, J.; Peng, Y.D.; Cheng, Z.R. Optimization of a discrete dish concentrator for uniform flux distribution on the cavity receiver of solar concentrator system. Renew. Energy 2018, 129, 431–445. [Google Scholar] [CrossRef]
- Mey-Cloutier, S.; Caliot, C.; Kribus, A.; Gray, Y.; Flamant, G. Experimental study of ceramic foams used as high temperature volumetric solar absorber. Sol. Energy 2016, 136, 226–235. [Google Scholar] [CrossRef]
- Faizan, M.; Almerbati, A.; Yilbas, B. A novel approach for volumetric solar receiver performance assessments. Appl. Therm. Eng. 2022, 211, 118487. [Google Scholar] [CrossRef]
- Shuai, Y.; Wang, F.-Q.; Xia, X.-L.; Tan, H.-P.; Liang, Y.-C. Radiative properties of a solar cavity receiver/reactor with quartz window. Int. J. Hydrogen Energy 2011, 36, 12148–12158. [Google Scholar]
- Wang, F.; Tan, J.; Ma, L.; Shuai, Y.; Tan, H.; Leng, Y. Thermal performance analysis of porous medium solar receiver with quartz window to minimize heat flux gradient. Sol. Energy 2014, 108, 348–359. [Google Scholar]
- Besarati, S.M.; Goswami, D.Y.; Stefanakos, E.K. Optimal heliostat aiming strategy for uniform distribution of heat flux on the receiver of a solar power tower plant. Energy Convers. Manag. 2014, 84, 234–243. [Google Scholar] [CrossRef]
- Du, B.C.; Qiu, Y.; He, Y.L.; Xue, X.D. Study on heat transfer and stress characteristics of the pressurized volumetric receiver in solar power tower system. Appl. Therm. Eng. 2018, 133, 341–350. [Google Scholar] [CrossRef]
- Wang, K.; He, Y.L.; Xue, X.D.; Du, B.C. Multi-objective optimization of the aiming strategy for the solar power tower with a cavity receiver by using the non-dominated sorting genetic algorithm. Appl. Energy 2017, 205, 399–416. [Google Scholar] [CrossRef]
- Yu, Q.; Wang, Z.; Xu, E. Analysis and improvement of solar flux distribution inside a cavity receiver based on multi-focal points of heliostat field. Appl. Energy 2014, 136, 417–430. [Google Scholar] [CrossRef]
- Wang, P.; Li, J.; Zhou, L.; Liu, D. Acceptance-Rejection Sampling Based Monte Carlo Ray Tracing in Anisotropic Porous Media. Energy 2020, 199, 117455. [Google Scholar] [CrossRef]
- Du, S.; Ren, Q.; He, Y.-L. Optical and radiative properties analysis and optimization study of the gradually-varied volumetric solar receiver. Appl. Energy 2017, 207, 27–35. [Google Scholar] [CrossRef]
- Roldán, M.; Smirnova, O.; Fend, T.; Casas, J.; Zarza, E. Thermal analysis and design of a volumetric solar absorber depending on the porosity. Renew. Energy 2014, 62, 116–128. [Google Scholar] [CrossRef]
- Nimvari, M.E.; Jouybari, N.F.; Esmaili, Q. A new approach to mitigate intense temperature gradients in ceramic foam solar receivers. Renew. Energy 2018, 122, 206–215. [Google Scholar] [CrossRef]
- Ahlbrink, N.; Andersson, J.; Diehl, M.; Pitz-Paal, R. Optimization of the Mass Flow Rate Distribution of an Open Volumetric Air Receiver. J. Sol. Energy Eng. 2013, 135, 041003. [Google Scholar] [CrossRef]
- Du, S.; Li, Z.; He, Y.; Li, D.; Xie, X.; Gao, Y. Experimental and numerical analysis of the hydraulic and thermal performances of the gradually-varied porous volumetric solar receiver. Sci. China Technol. Sci. 2020, 63, 1224–1234. [Google Scholar] [CrossRef]
- Li, X.L.; Xia, X.L.; Sun, C.; Chen, Z.H. Performance analysis on a volumetric solar receiver with an annular inner window. Renew. Energy 2021, 170, 487–499. [Google Scholar] [CrossRef]
- Li, X.L.; Sun, C.; Xia, X.L.; Li, Z.H.; Li, Y. Modeling of coupled heat transfer in a windowed volumetric solar receiver. Sol. Energy 2020, 201, 195–208. [Google Scholar] [CrossRef]
- Röger, M.; Pfänder, M.; Buck, R. Multiple Air-Jet Window Cooling for High-Temperature Pressurized Volumetric Receivers: Testing, Evaluation, and Modeling. J. Sol. Energy Eng. 2006, 128, 265–274. [Google Scholar] [CrossRef]
- Liu, Y.; Xie, L.T.; Shen, W.R.; Xu, C.; Zhao, B.Y. Relative flow direction modes and gradual porous parameters for radiation transport and interactions with thermochemical reaction in porous volumetric solar reactor. Renew. Energy 2023, 203, 612–621. [Google Scholar] [CrossRef]
- Lovegrove, K.; Luzzi, A. Endothermic reactors for an ammonia based thermochemical solar energy storage and transport system. Sol. Energy 1996, 56, 361–371. [Google Scholar] [CrossRef]
- Yuan, J.; Sundén, B. On continuum models for heat transfer in micro/nano-scale porous structures relevant for fuel cells. Int. J. Heat Mass Transf. 2013, 58, 441–456. [Google Scholar] [CrossRef]
- Krishnan, S.; Murthy, J.Y.; Garimella, S.V. Direct Simulation of Transport in Open-Cell Metal Foam. J. Heat Transf. 2006, 128, 793–799. [Google Scholar] [CrossRef]
- Jiang, P.X.; Ren, Z.P. Numerical investigation of forced convection heat transfer in porous media using a thermal non-equilibrium model. Int. J. Heat Fluid Flow 2001, 22, 102–110. [Google Scholar] [CrossRef]
- Vafai, K. Handbook of Porous Media; CRC Press: Boca Raton, FL, USA, 2015. [Google Scholar]
- Yang, K.; Vafai, K. Transient Aspects of Heat Flux Bifurcation in Porous Media: An Exact Solution. J. Heat Transf. 2011, 133, 052602. [Google Scholar] [CrossRef]
- Coutinho, J.A.; Lemos, M.S. Laminar flow with combustion in inert porous media. Int. Commun. Heat Mass Transf. 2012, 39, 896–903. [Google Scholar] [CrossRef]
- Nimvari, M.; Maerefat, M.; Jouybari, N.; El-Hossaini, M. Numerical simulation of turbulent reacting flow in porous media using two macroscopic turbulence models. Comput. Fluids 2013, 88, 232–240. [Google Scholar] [CrossRef]
- Nie, Z.; Lin, Y.; Tong, Q. Modeling structures of open cell foams. Comput. Mater. Sci. 2017, 131, 160–169. [Google Scholar] [CrossRef]
- Petrasch, J.; Meier, F.; Friess, H.; Steinfeld, A. Tomography based determination of permeability, Dupuit–Forchheimer coefficient, and interfacial heat transfer coefficient in reticulate porous ceramics. Int. J. Heat Fluid Flow 2008, 29, 315–326. [Google Scholar] [CrossRef]
- Cuevas, J.; García-Vidal, F.J. Radiative Heat Transfer. ACS Photonics 2018, 5, 3896–3915. [Google Scholar] [CrossRef]
- Cunsolo, S.; Coquard, R.; Baillis, D.; Chiu, W.K.; Bianco, N. Radiative properties of irregular open cell solid foams. Int. J. Therm. Sci. 2017, 117, 77–89. [Google Scholar] [CrossRef]
- Wang, F.; Tan, J.; Yong, S.; Tan, H.; Chu, S. Thermal performance analyses of porous media solar receiver with different irradiative transfer models. Int. J. Heat Mass Transf. 2014, 78, 7–16. [Google Scholar] [CrossRef]
- Du, S.; Li, M.-J.; Ren, Q.; Liang, Q.; He, Y.-L. Pore-scale numerical simulation of fully coupled heat transfer process in porous volumetric solar receiver. Energy 2017, 140, 1267–1275. [Google Scholar] [CrossRef]
- Zhu, Q.; Xuan, Y. Performance analysis of a volumetric receiver composed of packed shaped particles with spectrally dependent emissivity. Int. J. Heat Mass Transf. 2018, 122, 421–431. [Google Scholar] [CrossRef]
- Liu, D.; Li, Q.; Xuan, Y. Reticulated porous volumetric solar receiver designs guided by normal absorptance and hemispherical volumetric emittance investigations. Int. J. Heat Mass Transf. 2017, 114, 1067–1071. [Google Scholar] [CrossRef]
- Cheng, Z.D.; He, Y.L.; Cui, F.Q. Numerical investigations on coupled heat transfer and synthetical performance of a pressurized volumetric receiver with MCRT–FVM method. Appl. Therm. Eng. 2013, 50, 1044–1054. [Google Scholar] [CrossRef]
- Mey, S.; Caliot, C.; Flamant, G.; Kribus, A.; Gray, Y. Optimization of High Temperature SiC Volumetric Solar Absorber. Energy Procedia 2014, 49, 478–487. [Google Scholar] [CrossRef]
- Dietrich, B. Heat transfer coefficients for solid ceramic sponges—Experimental results and correlation. Int. J. Heat Mass Transf. 2013, 61, 627–637. [Google Scholar] [CrossRef]
- Wu, Z.; Caliot, C.; Flamant, G.; Wang, Z. Numerical simulation of convective heat transfer between air flow and ceramic foams to optimise volumetric solar air receiver performances. Int. J. Heat Mass Transf. 2011, 54, 1527–1537. [Google Scholar] [CrossRef]
- Xia, X.L.; Chen, X.; Sun, C.; Li, Z.H.; Liu, B. Experiment on the convective heat transfer from airflow to skeleton in open-cell porous foams. Int. J. Heat Mass Transf. 2017, 106, 83–90. [Google Scholar] [CrossRef]
- George, G.R.; Bockelmann, M.; Schmalhorst, L.; Beton, D.; Gerstle, A.; Lindermeir, A.; Wehinger, G.D. Radial heat transport in a fixed-bed reactor made of metallic foam pellets: Experiment and particle-resolved computational fluid dynamics. Int. J. Heat Mass Transf. 2022, 197, 123376. [Google Scholar] [CrossRef]
- Albanakis, C.; Missirlis, D.; Michailidis, N.; Yakinthos, K.; Goulas, A.; Omar, H.; Tsipas, D.; Granier, B. Experimental analysis of the pressure drop and heat transfer through metal foams used as volumetric receivers under concentrated solar radiation. Exp. Therm. Fluid Sci. 2009, 33, 246–252. [Google Scholar] [CrossRef]
- Wang, P.; Li, J.; Zhou, L.; Vafai, K. Experimental characterization on pore parameter and the irradiation absorption efficiency of a series SiC foam specimens. Energy Convers. Manag. 2020, 212, 112795. [Google Scholar] [CrossRef]
- Lougou, B.G.; Shuai, Y.; Pan, R.; Chaffa, G.; Tan, H. Heat transfer and fluid flow analysis of porous medium solar thermochemical reactor with quartz glass cover. Int. J. Heat Mass Transf. 2018, 127, 61–74. [Google Scholar] [CrossRef]


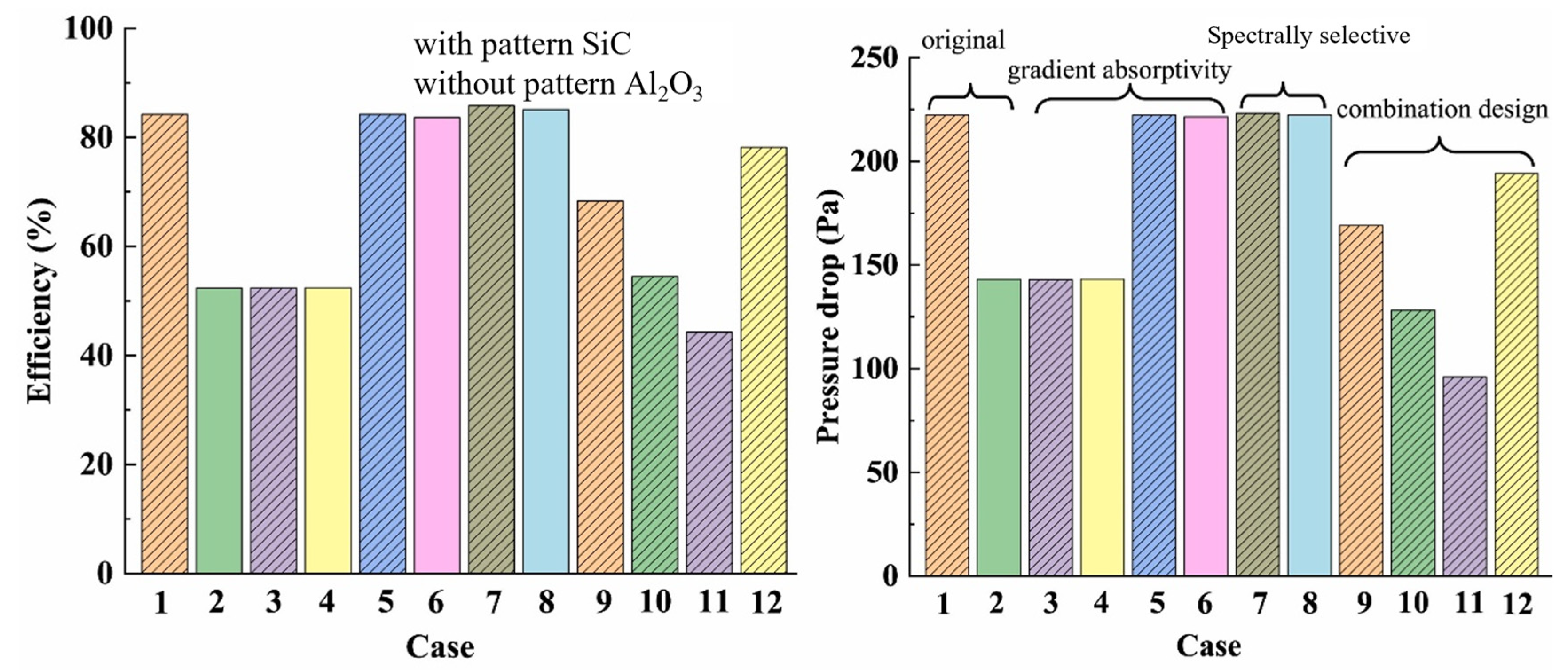
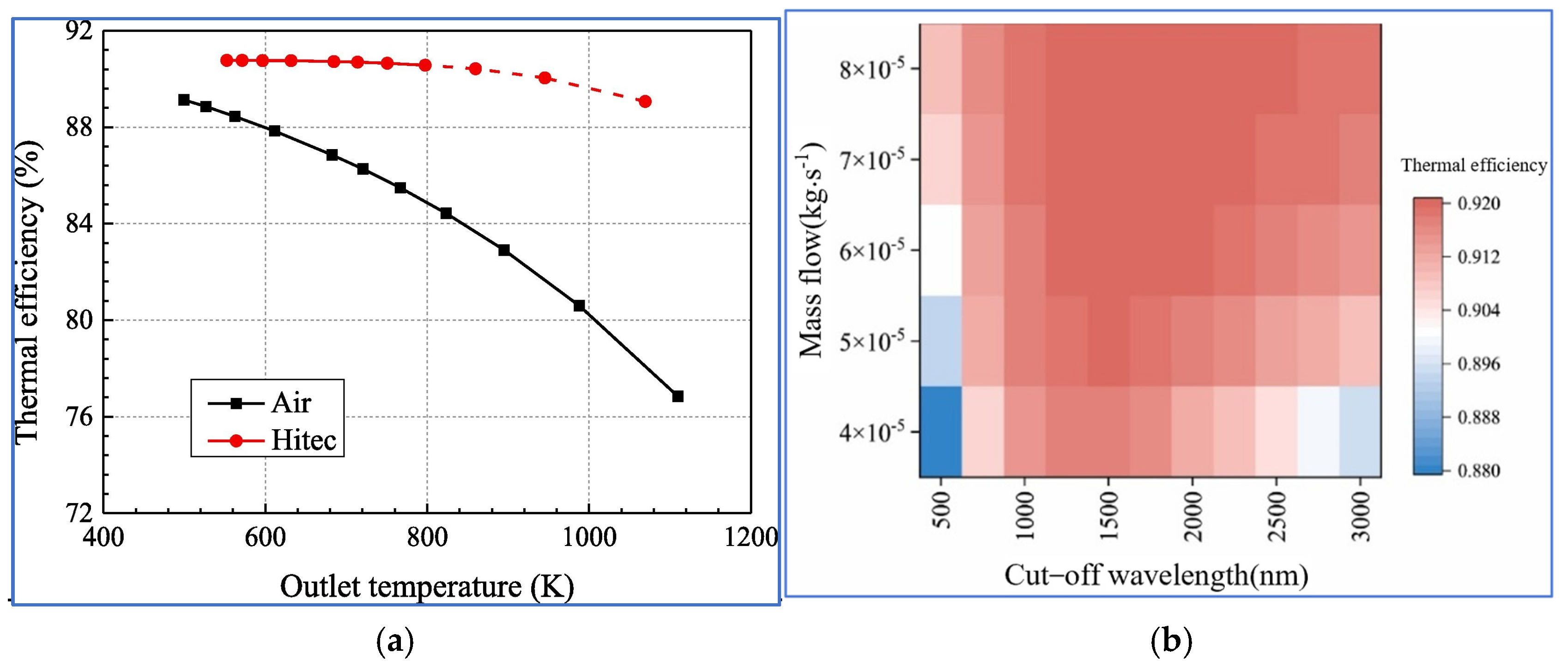

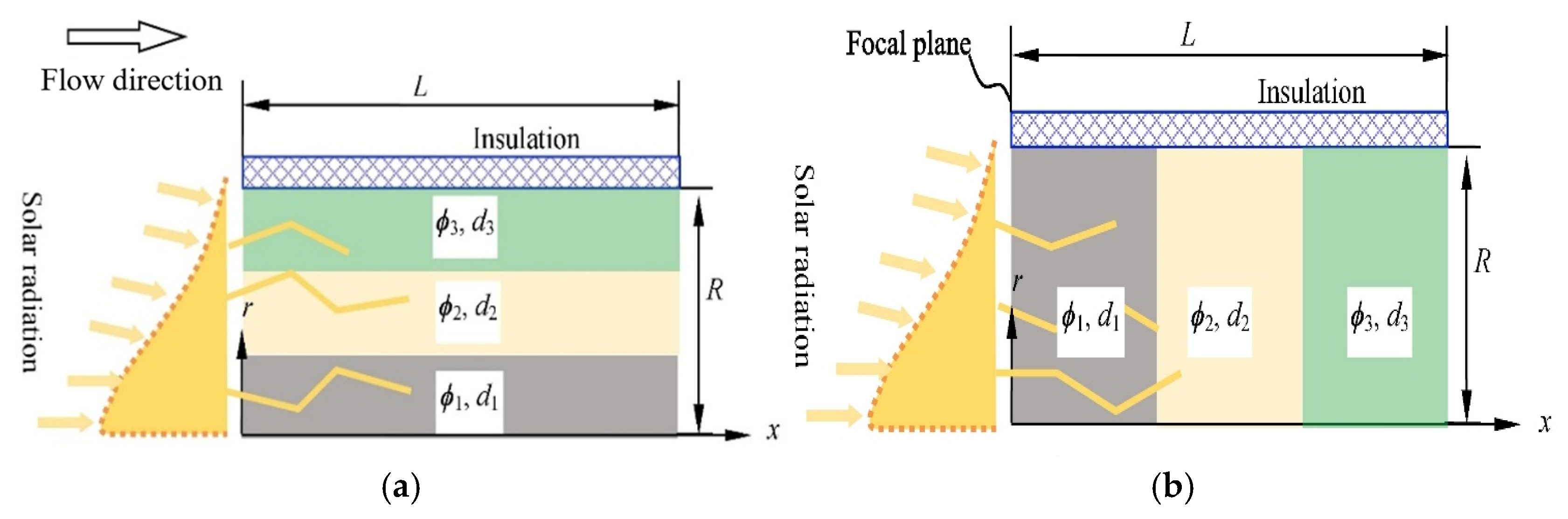
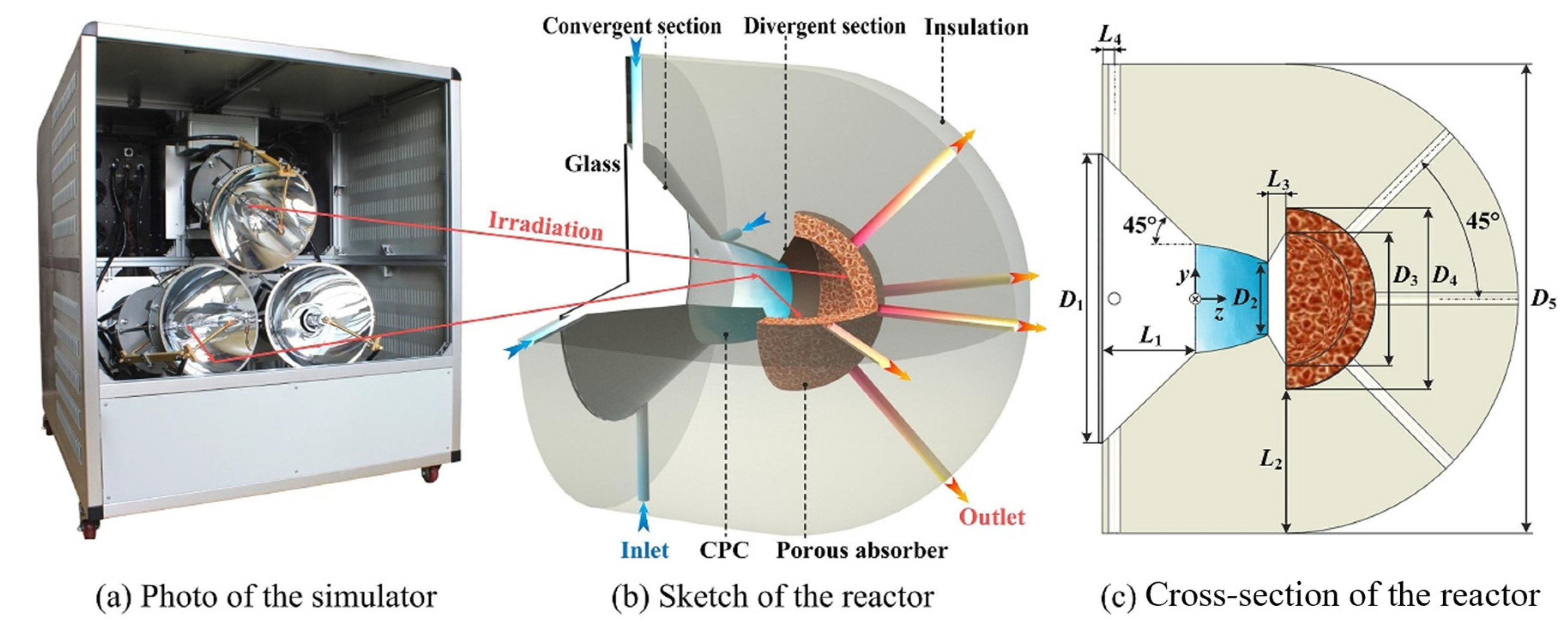





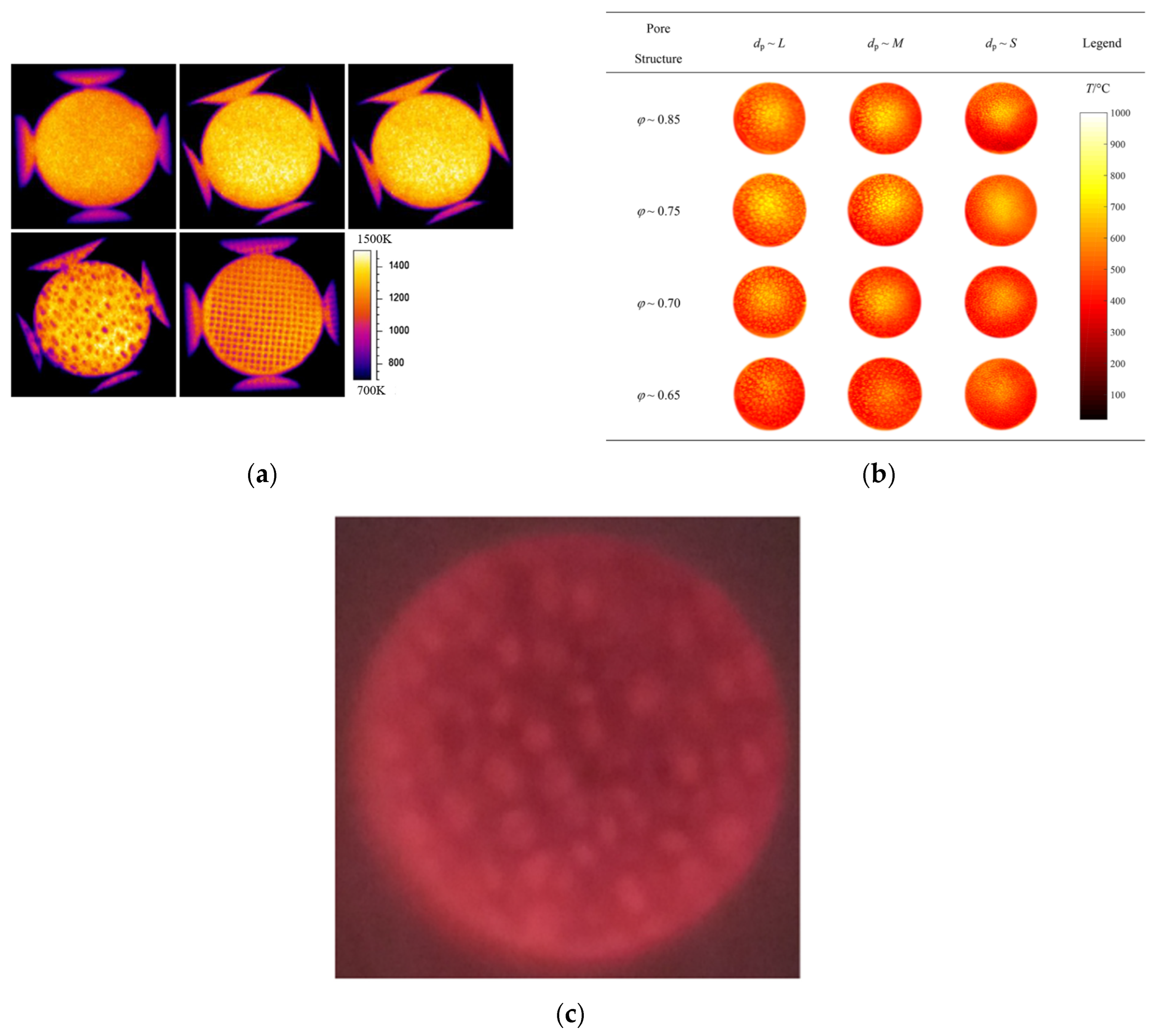
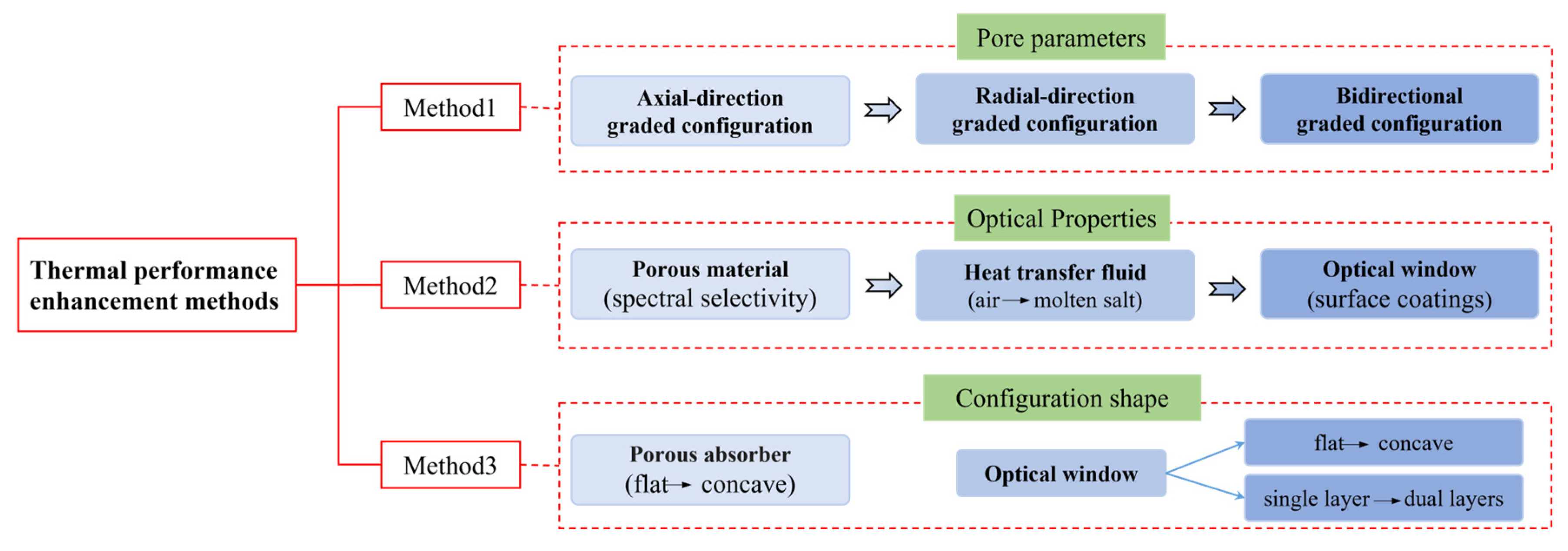
| Reference | Year | Optimization Method | Optical Properties | Efficiency |
|---|---|---|---|---|
| Meni-gault et al. [30] | 1991 | A two-slab selective volumetric receiver (a silica honeycomb and SiC particles) | infrared radiation loss | 78% |
| Zhu and Xuan [31] | 2018 | A multi-layer porous volumetric solar receiver (MoSi2-Si3N4 coating or ideal coating) | cut-off wavelength | 87% |
| Ali et al. [32] | 2020 | Double-layer honeycomb solar receiver (the first layer’s absorption rate is 0.2 and the second layer’s absorption rate is 0.95) | absorptivity and emissivity | 87.56% (optical efficiency) |
| Dai et al. [33] | 2022 | Double-layer absorber (Glass-porous) | infrared radiation loss | 83% |
| Chen et al. [34] | 2023 | Different solar absorbers (silicon carbide and alumina ceramic foam) | absorptivity and emissivity | 85% (best) |
| Du et al. [35] | 2021 | A porous volumetric solar receiver (molten salt replaces air) | infrared radiation loss | 90.4% |
| He et al. [36] | 2024 | A porous volumetric solar receiver Hitec molten | cut-off wavelength | 92% (best) |
| Reference | Year | Method | Material | Direction | Configuration | Pore Parameters |
|---|---|---|---|---|---|---|
| Villafán-Vidales et al. [42] | 2011 | Experiment Simulation | Sic | Uniform | Single-layer | Porosity |
| Chen et al. [44] | 2018 | Simulation | Ru/γ-Al2O3 | Axial, Radial | Multi-layer | Pore diameter, Porosity |
| Wang et al. [43] | 2020 | Simulation | Ru/γ-Al2O3 | Axial | Double-layer | Pore diameter, Porosity |
| Ali et al. [46] | 2021 | Experiment Simulation | Sic | Uniform | Single-layer | Porosity |
| Zhang et al. [45] | 2022 | Simulation | Wire meshes | Axial | Double-layer | Porosity, Mean cell size |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, G.; Liu, Y.; Chen, X.; Zhao, T. Optimization of Heat Transfer Performances Within Porous Solar Receivers—A Comprehensive Review. Energies 2025, 18, 1201. https://doi.org/10.3390/en18051201
Dai G, Liu Y, Chen X, Zhao T. Optimization of Heat Transfer Performances Within Porous Solar Receivers—A Comprehensive Review. Energies. 2025; 18(5):1201. https://doi.org/10.3390/en18051201
Chicago/Turabian StyleDai, Guilong, Yishuo Liu, Xue Chen, and Tian Zhao. 2025. "Optimization of Heat Transfer Performances Within Porous Solar Receivers—A Comprehensive Review" Energies 18, no. 5: 1201. https://doi.org/10.3390/en18051201
APA StyleDai, G., Liu, Y., Chen, X., & Zhao, T. (2025). Optimization of Heat Transfer Performances Within Porous Solar Receivers—A Comprehensive Review. Energies, 18(5), 1201. https://doi.org/10.3390/en18051201