CO2 Sequestration in a Carbonate Saline Aquifer: An Investigation into the Roles of Natural Fractures and Well Placement
Abstract
1. Introduction
2. Methodology
2.1. Model Development
2.2. Sensitivity Analysis Framework
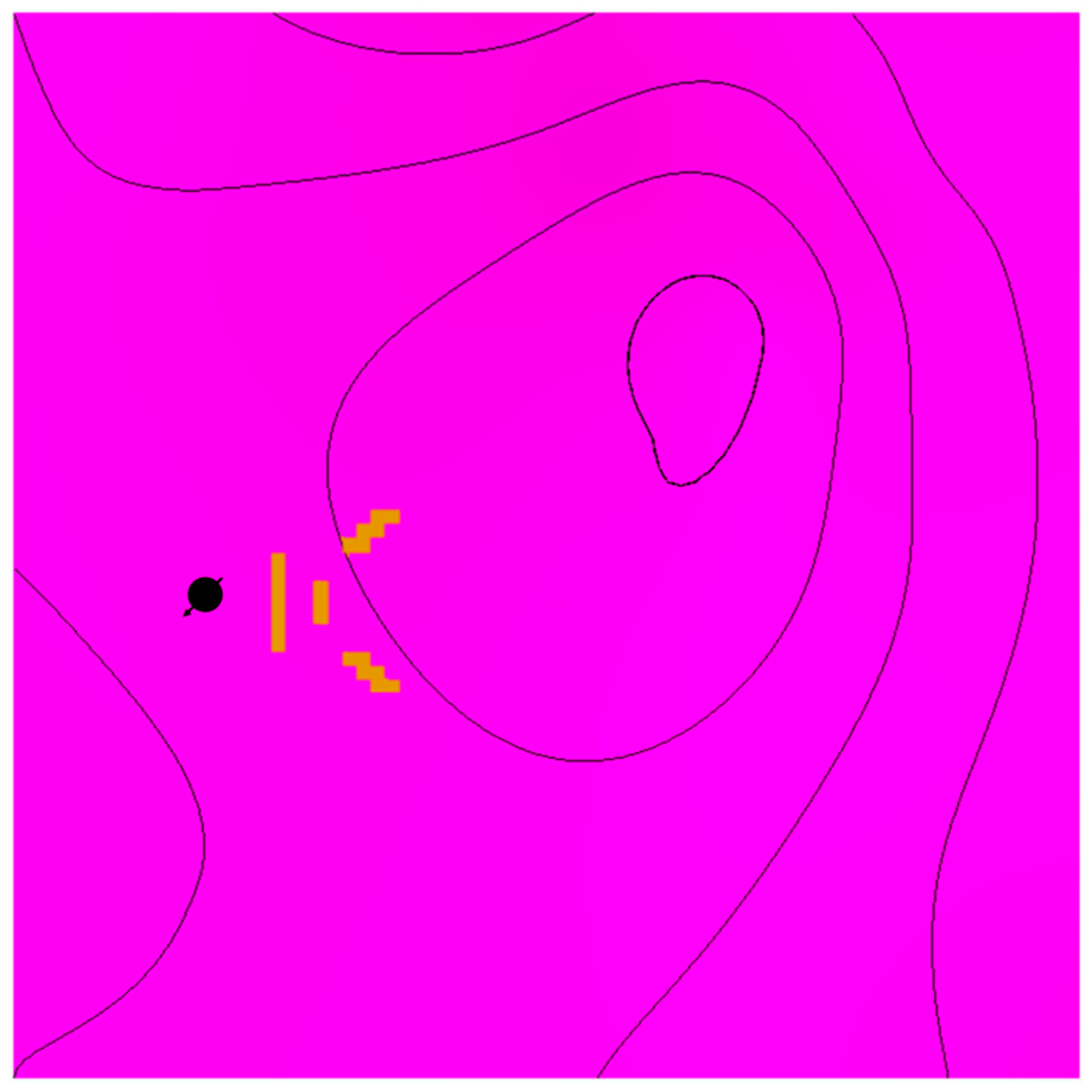
2.2.1. Well Placement and Injection Zone
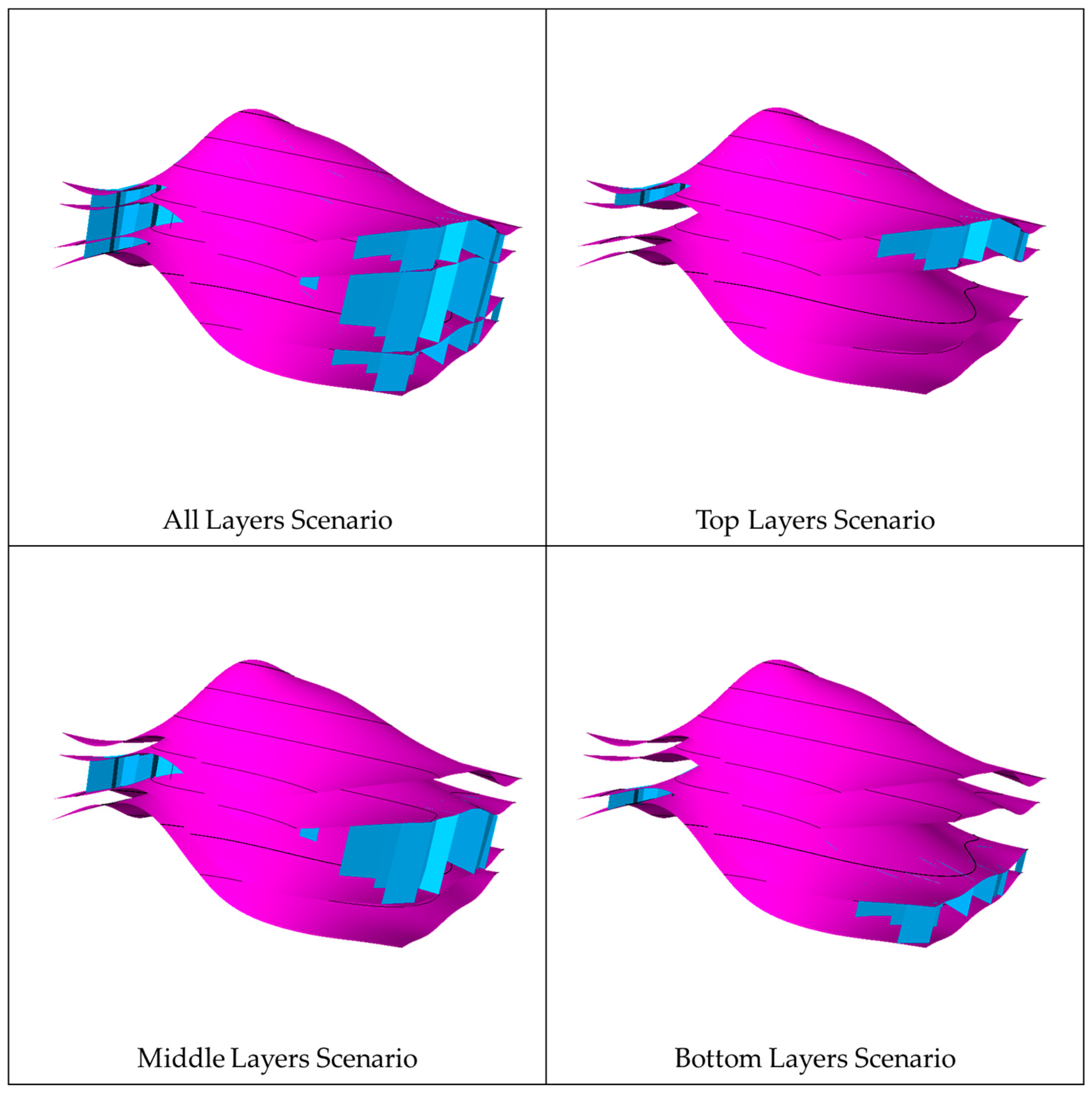
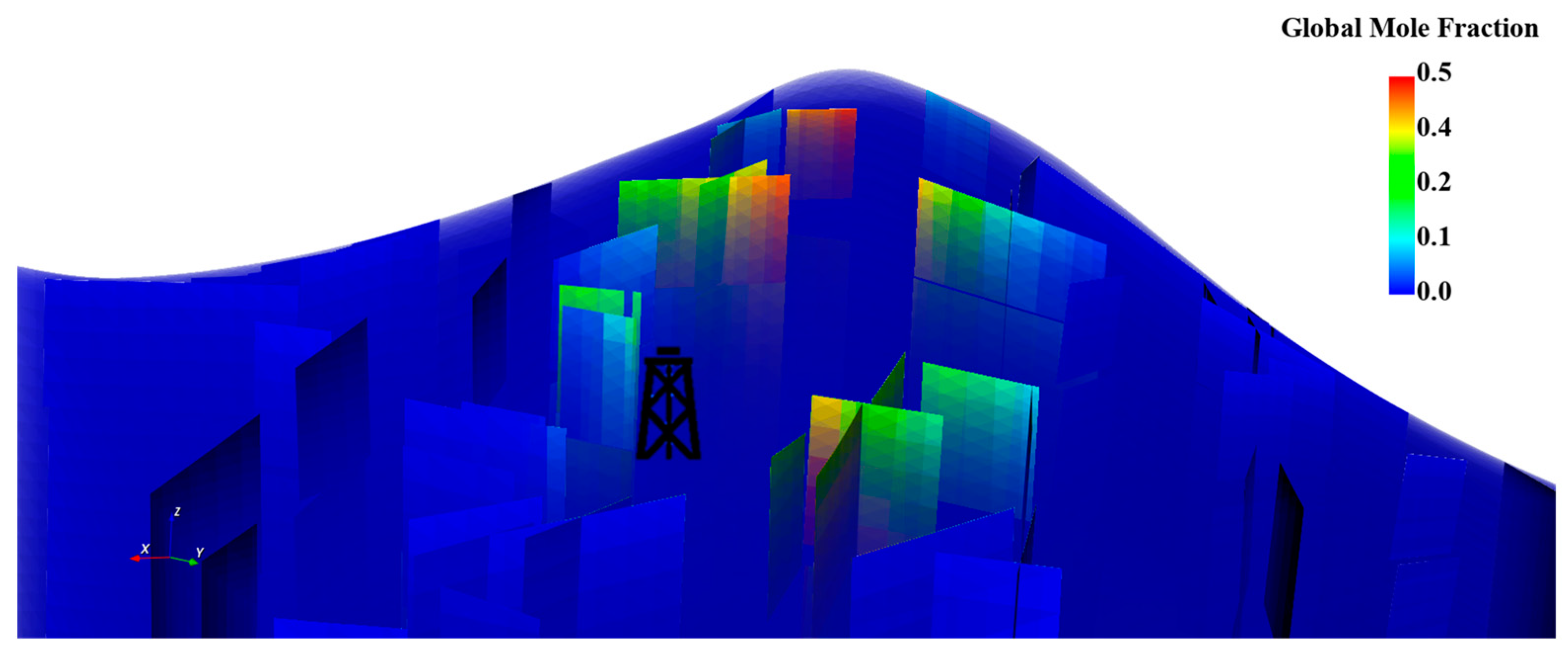
2.2.2. Natural Fractures Network
3. Results and Discussion
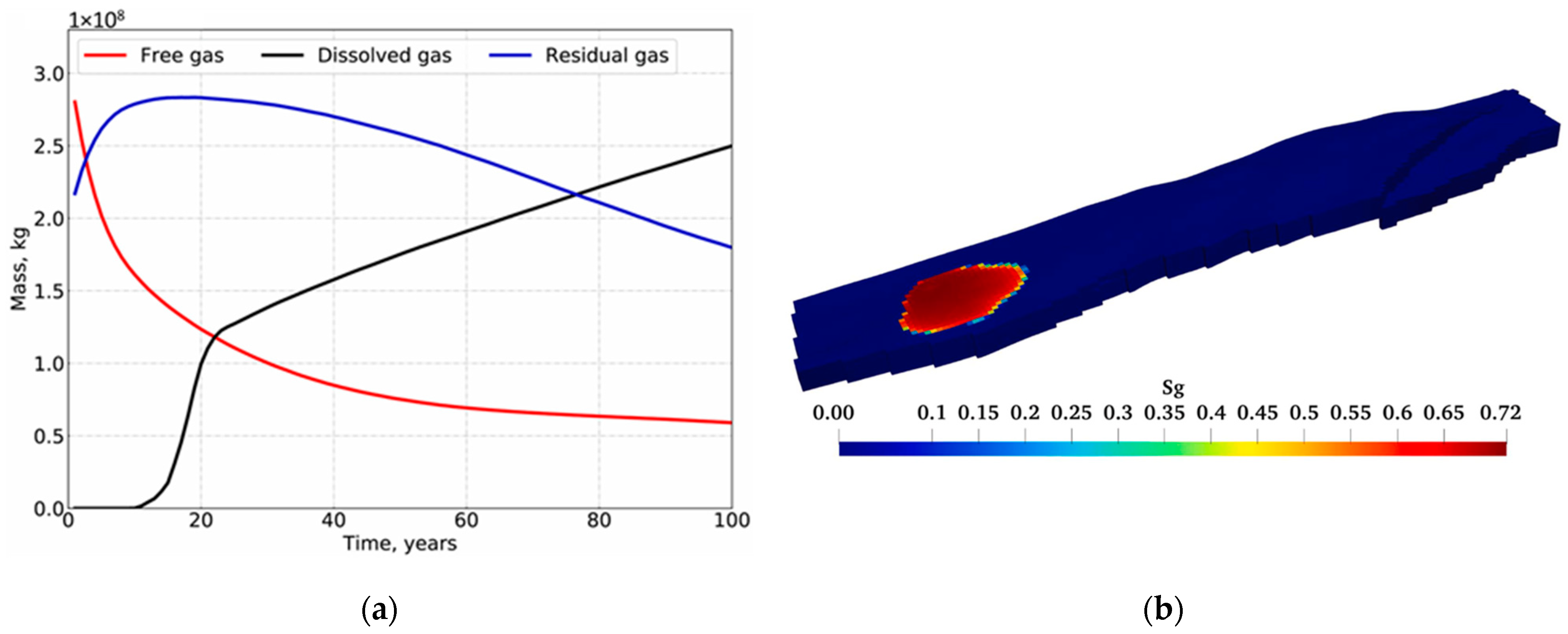
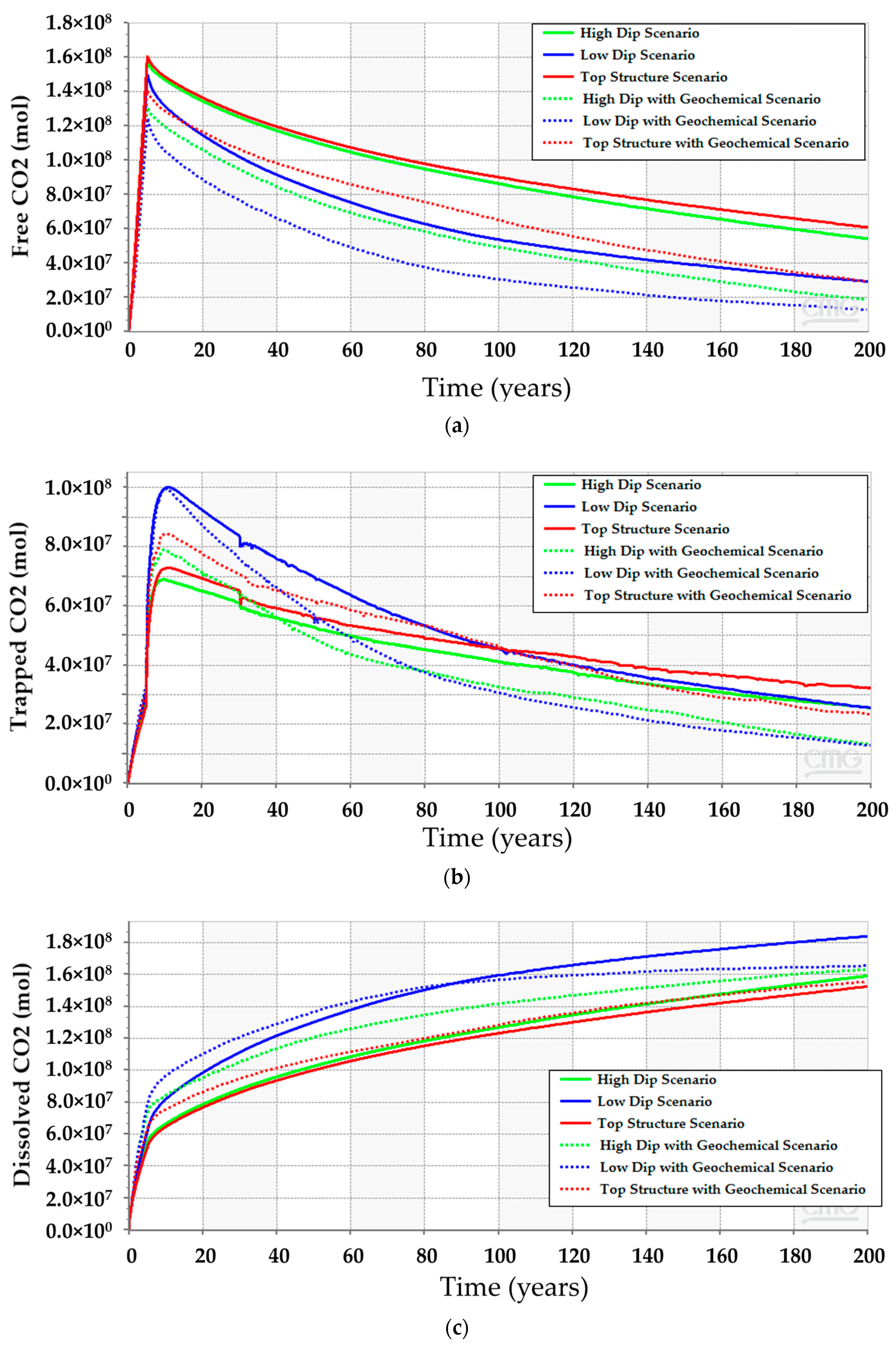
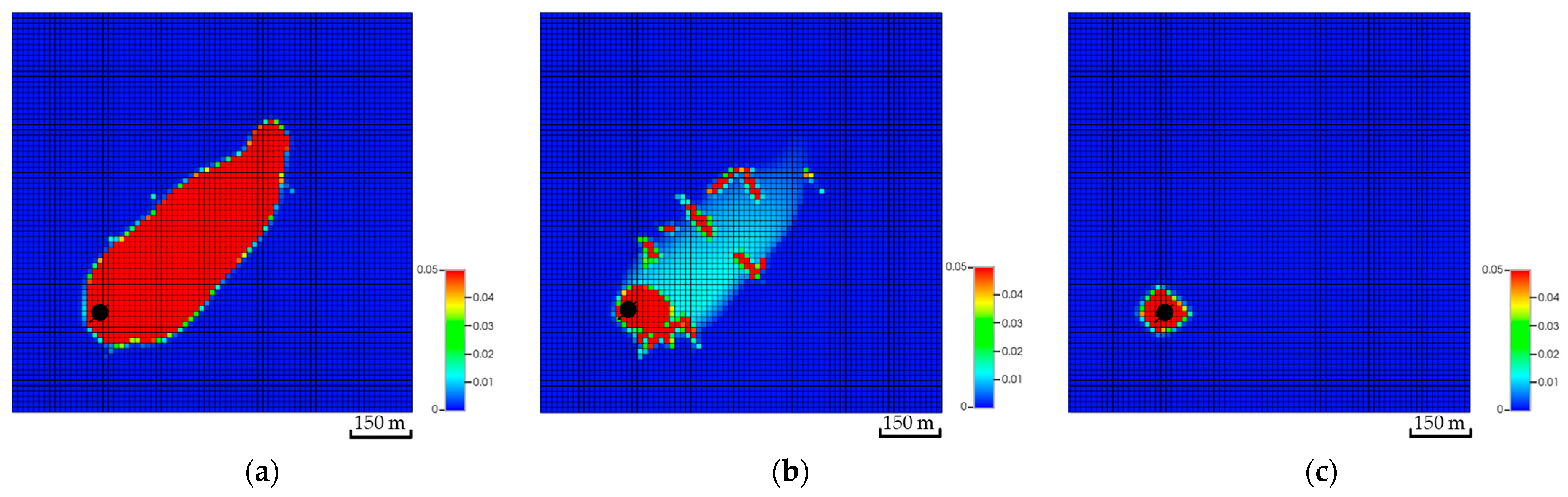
3.1. Well Placement Optimization
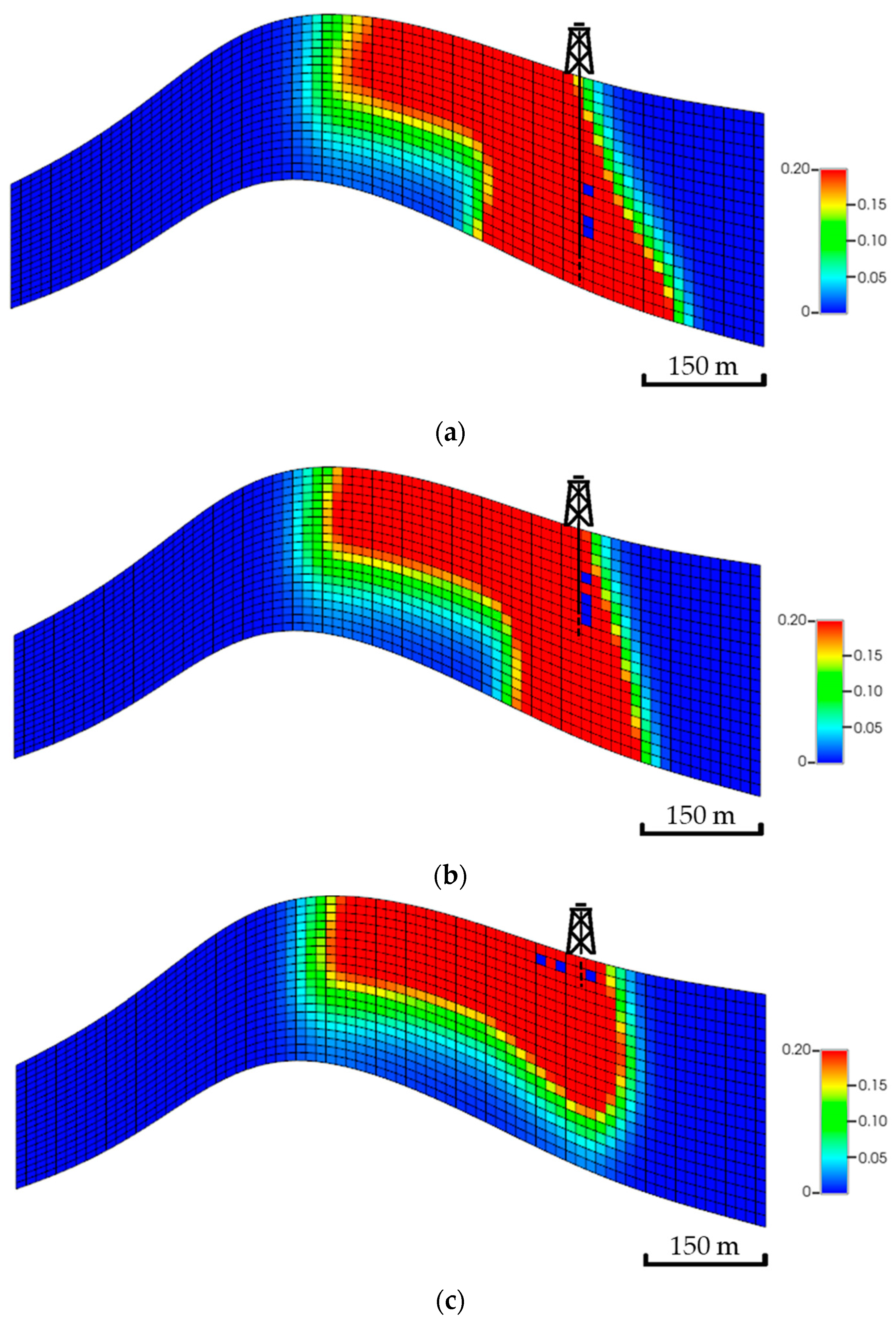
3.1.1. Injection Location
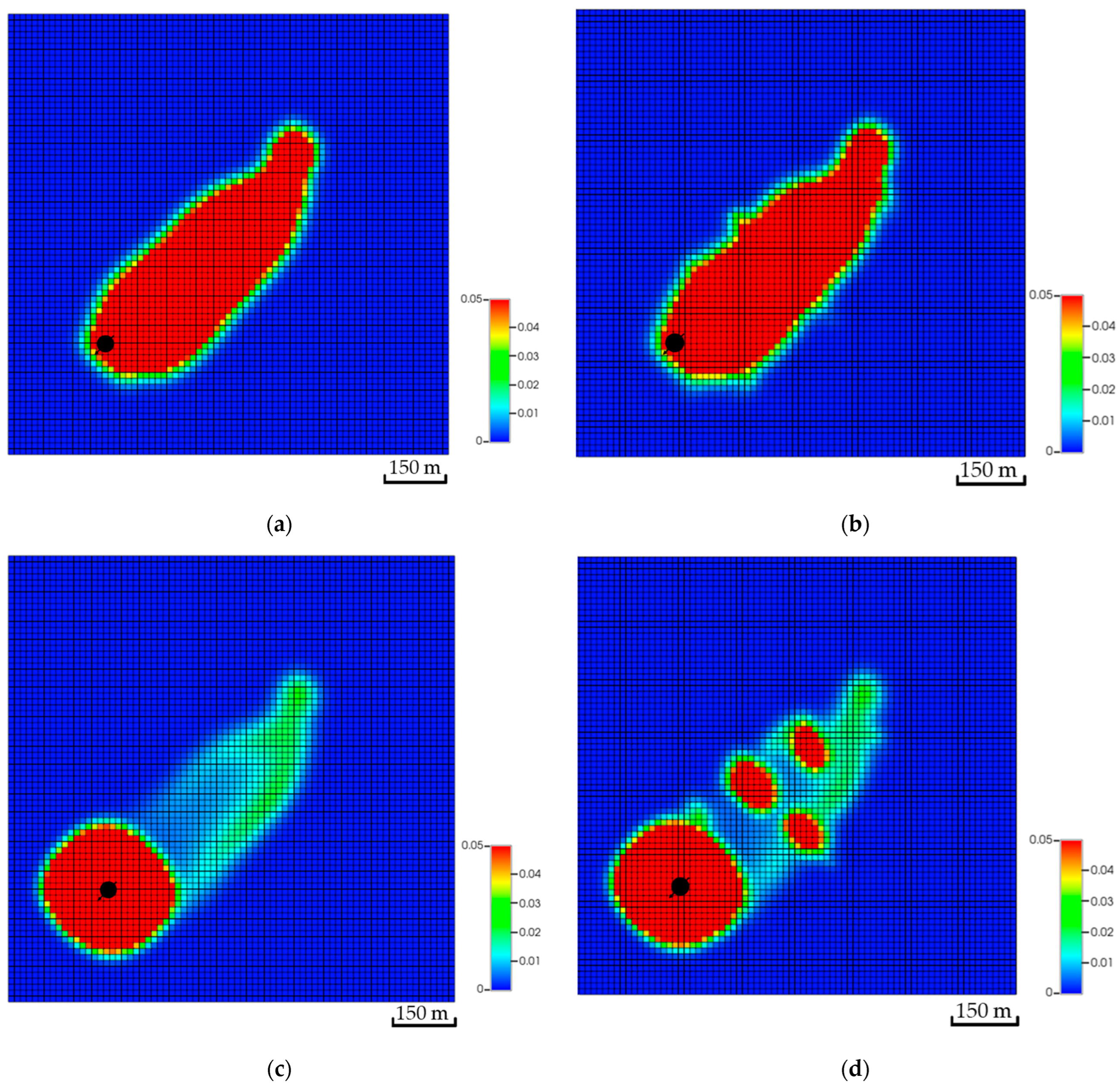
3.1.2. Injection Zone
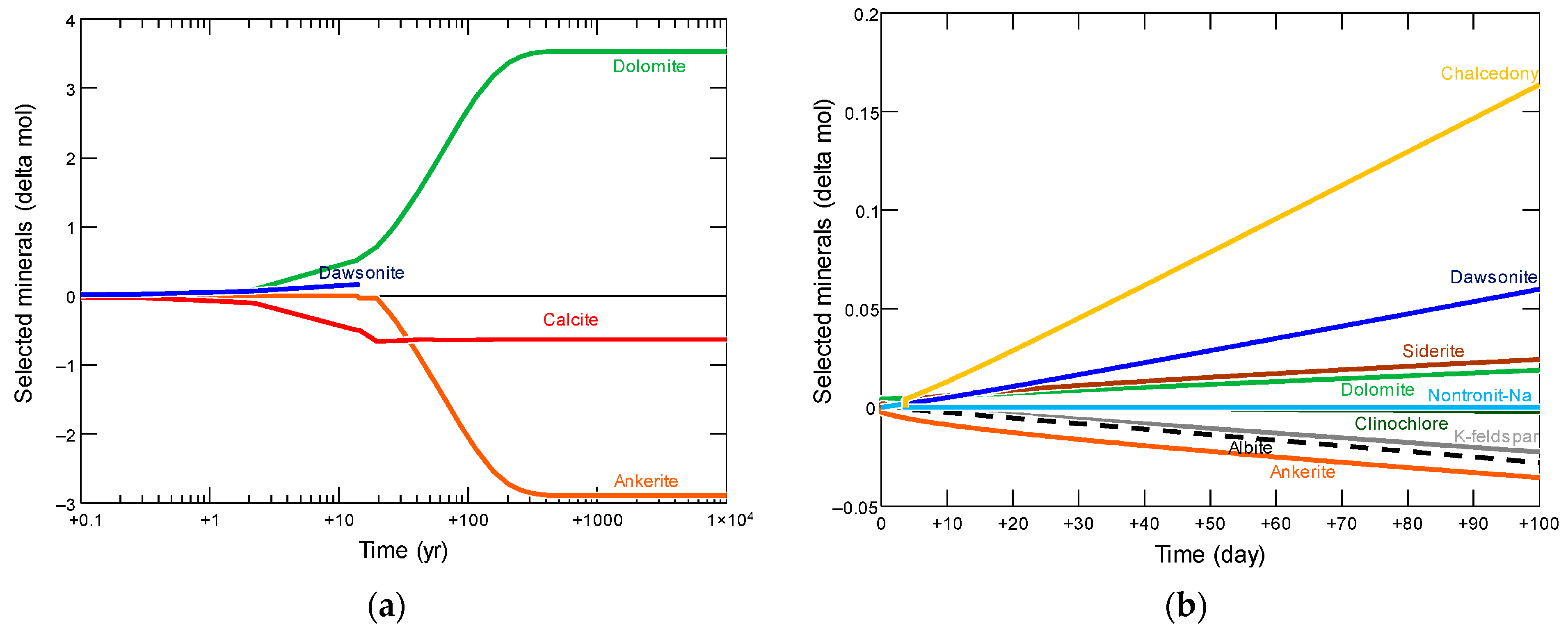
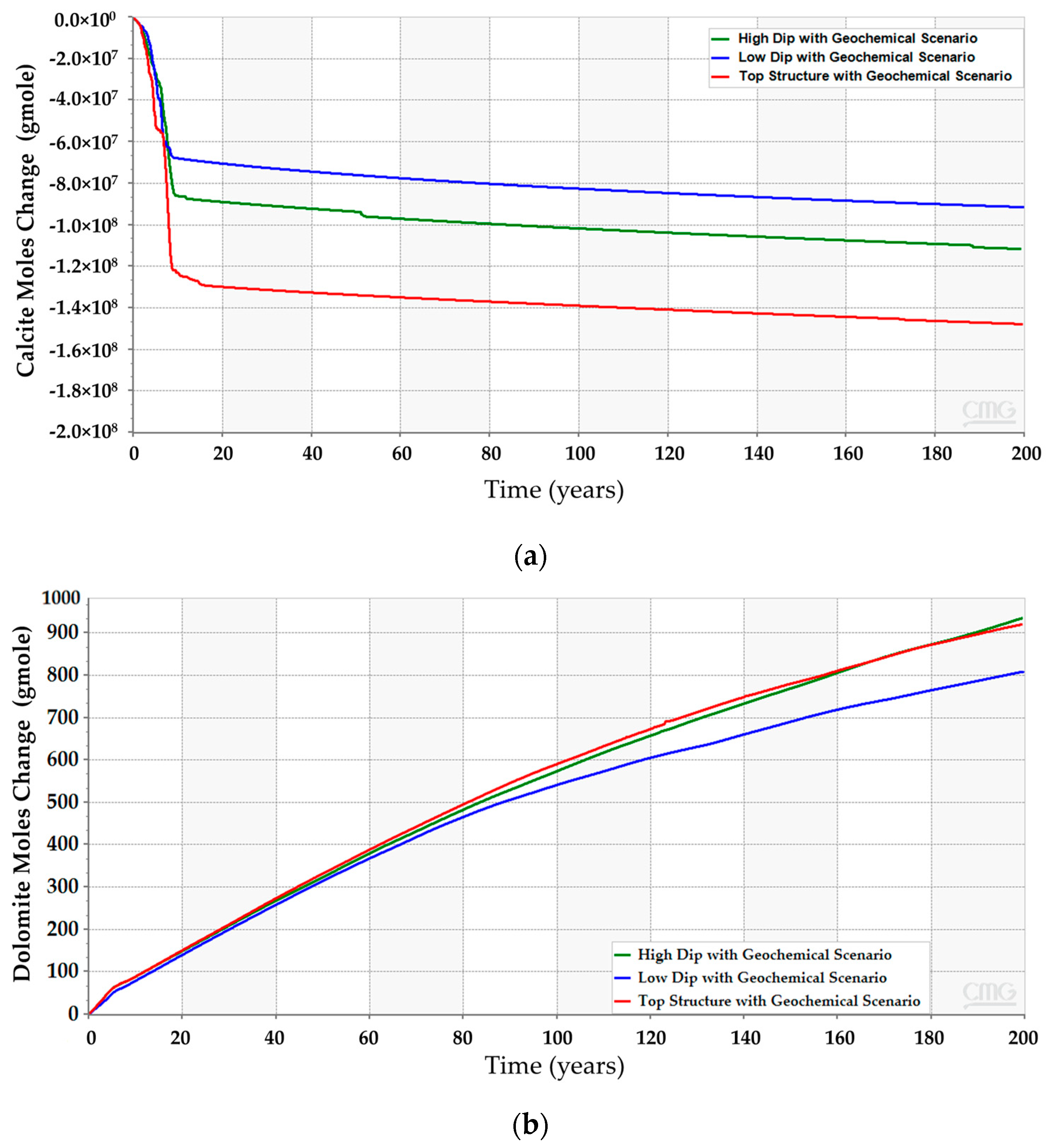
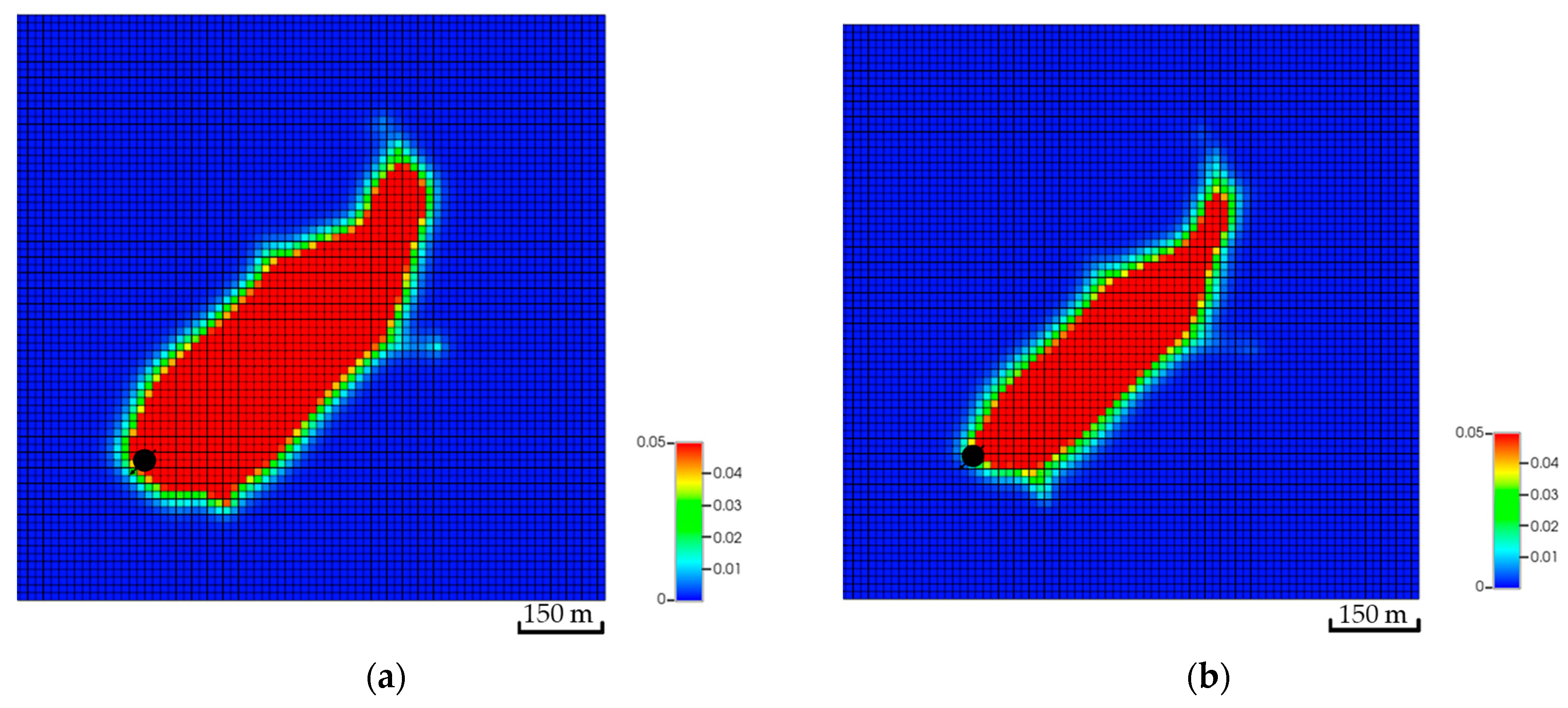
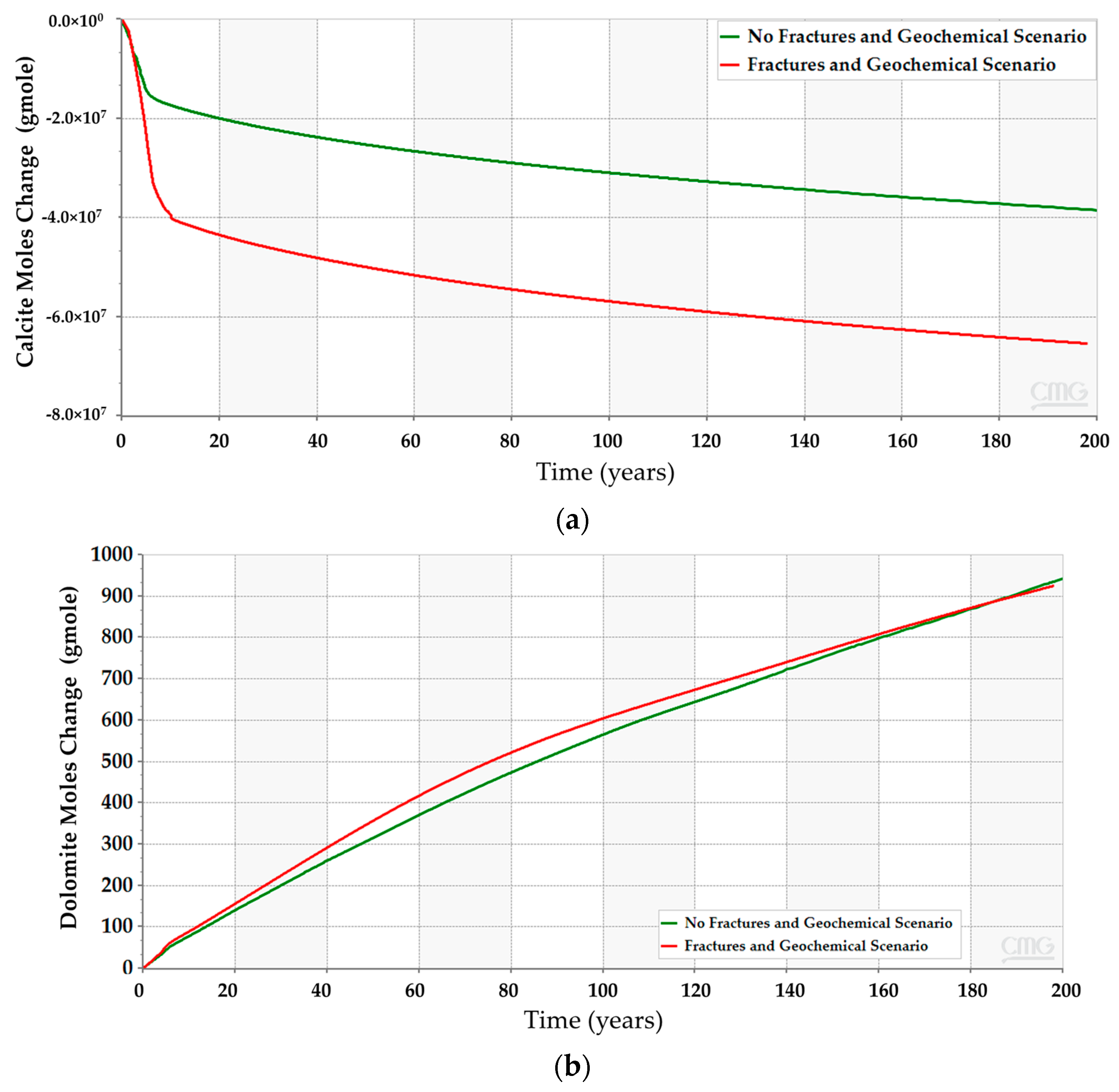
3.2. Natural Fractures Network Influence
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dhakal, S.; Minx, J.C.; Toth, F.L. Emissions Trends and Drivers. In IPCC, 2022: Climate Change 2022—Mitigation of Climate Change; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2023; pp. 215–294. [Google Scholar]
- U.S. Environmental Protection Agency (EPA). Inventory of U.S. Greenhouse Gas Emissions and Sinks: 1990–2022—EPA 430-R-24-004; EPA: Washington, DC, USA, 2024.
- Kuh, K.F. The Law of Climate Change Mitigation: An Overview. Encycl. Anthr. 2017, 1–5, 505–510. [Google Scholar] [CrossRef]
- Butnar, I.; Cronin, J.; Pye, S. Review of Carbon Capture Utilisation and Carbon Capture and Storage in Future EU Decarbonisation Scenarios; UCL Energy Institute: London, UK, 2020. [Google Scholar]
- Paltsev, S.; Morris, J.; Kheshgi, H.; Herzog, H. Hard-to-Abate Sectors: The Role of Industrial Carbon Capture and Storage (CCS) in Emission Mitigation. Appl. Energy 2021, 300, 117322. [Google Scholar] [CrossRef]
- Peridas, G.; Mordick Schmidt, B. The Role of Carbon Capture and Storage in the Race to Carbon Neutrality. Electr. J. 2021, 34, 106996. [Google Scholar] [CrossRef]
- Prepared by Working Group III of the Intergovernmental Panel on Climate Change; Metz, B.; Davidson, O.; Coninck, H.D.; Loos, M.; Meyer, L. IPCC Special Report on Carbon Dioxide Capture and Storage; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Furre, A.K.; Eiken, O.; Alnes, H.; Vevatne, J.N.; Kiær, A.F. 20 Years of Monitoring CO2-Injection at Sleipner. Energy Procedia 2017, 114, 3916–3926. [Google Scholar] [CrossRef]
- Snæbjörnsdóttir, S.Ó.; Sigfússon, B.; Marieni, C.; Goldberg, D.; Gislason, S.R.; Oelkers, E.H. Carbon Dioxide Storage through Mineral Carbonation. Nat. Rev. Earth Environ. 2020, 1, 90–102. [Google Scholar] [CrossRef]
- Ehrenberg, S.N.; Nadeau, P.H. Sandstone vs. Carbonate Petroleum Reservoirs: A Global Perspective on Porosity-Depth and Porosity-Permeability Relationships. Am. Assoc. Pet Geol. Bull. 2005, 89, 435–445. [Google Scholar] [CrossRef]
- Hosa, A.; Esentia, M.; Stewart, J.; Haszeldine, S. Injection of CO2 into Saline Formations: Benchmarking Worldwide Projects. Chem. Eng. Res. Des. 2011, 89, 1855–1864. [Google Scholar] [CrossRef]
- Eiken, O.; Ringrose, P.; Hermanrud, C.; Nazarian, B.; Torp, T.A.; Høier, L. Lessons Learned from 14 Years of CCS Operations: Sleipner, In Salah and Snøhvit. Energy Procedia 2011, 4, 5541–5548. [Google Scholar] [CrossRef]
- Bourne, S.; Crouch, S.; Smith, M. A Risk-Based Framework for Measurement, Monitoring and Verification of the Quest CCS Project, Alberta, Canada. Int. J. Greenh. Gas Control 2014, 26, 109–126. [Google Scholar] [CrossRef]
- Loring, J.S.; Miller, Q.R.S.; Thompson, C.J.; Schaef, H.T. Experimental Studies of Reactivity and Transformations of Rocks and Minerals in Water-Bearing Supercritical CO2. In Science of Carbon Storage in Deep Saline Formations; Elsevier: Amsterdam, The Netherlands, 2019; pp. 63–88. [Google Scholar]
- Rackley, S.A. Mineral Carbonation. In Carbon Capture and Storage; Elsevier: Amsterdam, The Netherlands, 2017; pp. 253–282. [Google Scholar]
- Kim, M.; Kim, K.; Han, W.S.; Oh, J.; Park, E. Density-Driven Convection in a Fractured Porous Media: Implications for Geological CO2 Storage. Water Resour. Res. 2019, 55, 5852–5870. [Google Scholar] [CrossRef]
- Iding, M.; Ringrose, P. Evaluating the Impact of Fractures on the Performance of the In Salah CO2 Storage Site. Int. J. Greenh. Gas Control 2010, 4, 242–248. [Google Scholar] [CrossRef]
- Sohal, M.A.; Le Gallo, Y.; Audigane, P.; de Dios, J.C.; Rigby, S.P. Effect of Geological Heterogeneities on Reservoir Storage Capacity and Migration of CO2 Plume in a Deep Saline Fractured Carbonate Aquifer. Int. J. Greenh. Gas Control 2021, 108, 103306. [Google Scholar] [CrossRef]
- Parkhurst, D.L.; Thorstenson, D.C.; Plummer, N. PHREEQE: A Computer Program for Geochemical Calculations; US Geological Survey, Water Resources Division: Reston, VA, USA, 1980.
- TOUGHREACT. Available online: https://tough.lbl.gov/software/toughreact_v4-13-omp/ (accessed on 11 December 2024).
- Lasaga, A.C. Chemical Kinetics of Water-rock Interactions. J. Geophys. Res. Solid Earth 1984, 89, 4009–4025. [Google Scholar] [CrossRef]
- Li, Y.; Nghiem, L.X. Phase Equilibria of Oil, Gas and Water/Brine Mixtures from a Cubic Equation of State and Henry’s Law. Can. J. Chem. Eng. 1986, 64, 486–496. [Google Scholar] [CrossRef]
- Wang, P.; Stenby, E.H. Phase Equilibrium Calculation in Compositional Reservoir Simulation. Comput. Chem. Eng. 1992, 16, S449–S456. [Google Scholar] [CrossRef]
- Licbinska, M.; Labus, K.; Klempa, M.; Matysek, D.; Vasek, M. Laboratory Experiments and Geochemical Modeling of Gas–Water–Rock Interactions for a CO2 Storage Pilot Project in a Carbonate Reservoir in the Czech Republic. Minerals 2024, 14, 602. [Google Scholar] [CrossRef]
- Lyu, X.; Voskov, D. Advanced Modeling of Enhanced CO2 Dissolution Trapping in Saline Aquifers. Int. J. Greenh. Gas Control 2023, 127, 103907. [Google Scholar] [CrossRef]
- Ranganathan, P.; Van Hemert, P.; Rudolph, E.S.J.; Zitha, P.Z.J. Numerical Modeling of CO2 Mineralisation during Storage in Deep Saline Aquifers. Energy Procedia 2011, 4, 4538–4545. [Google Scholar] [CrossRef]
- Anchliya, A.; Ehlig-Economides, C.; Jafarpour, B. Aquifer Management To Accelerate CO2 Dissolution and Trapping. SPE J. 2012, 17, 805–816. [Google Scholar] [CrossRef]
- Computer Modelling Group Ltd. CMG-GEM Compositional & Unconventional Simulator (Version 2023.30). Available online: https://www.cmgl.ca/solutions/software/gem/ (accessed on 7 November 2024).
- Machado, M.V.B.; Khanal, A.; Delshad, M. Unveiling the Essential Parameters Driving Mineral Reactions during CO2 Storage in Carbonate Aquifers through Proxy Models. Appl. Sci. 2024, 14, 1465. [Google Scholar] [CrossRef]
- Bennion, D.B.; Bachu, S. Drainage and Imbibition Relative Permeability Relationships for Supercritical CO2/Brine and H2S/Brine Systems in Intergranular Sandstone, Carbonate, Shale, and Anhydrite Rocks. SPE Reserv. Eval. Eng. 2008, 11, 487–496. [Google Scholar] [CrossRef]
- Carlson, F.M. Simulation of Relative Permeability Hysteresis to the Nonwetting Phase. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 4–7 October 1981. [Google Scholar]
- Land, C.S. Calculation of Imbibition Relative Permeability for Two- and Three-Phase Flow From Rock Properties. Soc. Pet. Eng. J. 1968, 8, 149–156. [Google Scholar] [CrossRef]
- Burnside, N.M.; Naylor, M. Review and Implications of Relative Permeability of CO2/Brine Systems and Residual Trapping of CO2. Int. J. Greenh. Gas Control 2014, 23, 1–11. [Google Scholar] [CrossRef]
- Li, L.; Lee, S.H. Efficient Field-Scale Simulation of Black Oil in a Naturally Fractured Reservoir Through Discrete Fracture Networks and Homogenized Media. SPE Reserv. Eval. Eng. 2008, 11, 750–758. [Google Scholar] [CrossRef]
- Moinfar, A.; Varavei, A.; Sepehrnoori, K.; Johns, R.T. Development of an Efficient Embedded Discrete Fracture Model for 3D Compositional Reservoir Simulation in Fractured Reservoirs. SPE J. 2014, 19, 289–303. [Google Scholar] [CrossRef]
- Sepehrnoori, K.; Xu, Y.; Yu, W. Embedded Discrete Fracture Modeling and Application in Reservoir Simulation; Elsevier: Amsterdam, The Netherlands, 2020. [Google Scholar]
- Poli, R.E.B.; Barbosa Machado, M.V.; Sepehrnoori, K. Advancements and Perspectives in Embedded Discrete Fracture Models (EDFM). Energies 2024, 17, 3550. [Google Scholar] [CrossRef]
- Barbosa Machado, M.V.; Delshad, M.; Sepehrnoori, K. A Computationally Efficient Approach to Model Reactive Transport during CO2 Storage in Naturally Fractured Saline Aquifers. Geoenergy Sci. Eng. 2024, 236, 212768. [Google Scholar] [CrossRef]








| Property/Parameter | Value |
|---|---|
| Porosity (%) | 15 |
| Horizontal permeability (mD) | 100 |
| Vertical permeability (mD) | 10 |
| Saline water density (kg/m3) | 1020 |
| Reservoir datum depth (m) | 1320 |
| Initial datum reservoir pressure (MPa) | 13 |
| Scenario * | Free CO2 (mol) | Trapped CO2 (mol) | Dissolved CO2 (mol) |
|---|---|---|---|
| A | 6.07 × 107 | 3.22 × 107 | 1.53 × 108 |
| B | 3.86 × 107 | 2.99 × 107 | 1.75 × 108 |
| C | 2.61 × 107 | 2.46 × 107 | 1.87 × 108 |
| Realization | Layers | Free CO2 (mol) | Trapped CO2 (mol) | Dissolved CO2 (mol) |
|---|---|---|---|---|
| 1 | All | 2.49 × 107 | 2.49 × 107 | 1.88 × 108 |
| Top | 2.54 × 107 | 2.54 × 107 | 1.88 × 108 | |
| Middle | 2.58 × 107 | 2.58 × 107 | 1.87 × 108 | |
| Bottom | 2.52 × 107 | 2.52 × 107 | 1.88 × 108 | |
| 2 | All | 2.52 × 107 | 2.51 × 107 | 1.88 × 108 |
| Top | 2.50 × 107 | 2.50 × 107 | 1.88 × 108 | |
| Middle | 2.50 × 107 | 2.50 × 107 | 1.88 × 108 | |
| Bottom | 2.52 × 107 | 2.52 × 107 | 1.88 × 108 | |
| 3 | All | 2.48 × 107 | 2.46 × 107 | 1.88 × 108 |
| Top | 2.49 × 107 | 2.49 × 107 | 1.88 × 108 | |
| Middle | 2.50 × 107 | 2.50 × 107 | 1.88 × 108 | |
| Bottom | 2.54 × 107 | 2.53 × 107 | 1.88 × 108 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al Mulhim, A.K.; Delshad, M.; Sepehrnoori, K. CO2 Sequestration in a Carbonate Saline Aquifer: An Investigation into the Roles of Natural Fractures and Well Placement. Energies 2025, 18, 242. https://doi.org/10.3390/en18020242
Al Mulhim AK, Delshad M, Sepehrnoori K. CO2 Sequestration in a Carbonate Saline Aquifer: An Investigation into the Roles of Natural Fractures and Well Placement. Energies. 2025; 18(2):242. https://doi.org/10.3390/en18020242
Chicago/Turabian StyleAl Mulhim, Abdulrahim K., Mojdeh Delshad, and Kamy Sepehrnoori. 2025. "CO2 Sequestration in a Carbonate Saline Aquifer: An Investigation into the Roles of Natural Fractures and Well Placement" Energies 18, no. 2: 242. https://doi.org/10.3390/en18020242
APA StyleAl Mulhim, A. K., Delshad, M., & Sepehrnoori, K. (2025). CO2 Sequestration in a Carbonate Saline Aquifer: An Investigation into the Roles of Natural Fractures and Well Placement. Energies, 18(2), 242. https://doi.org/10.3390/en18020242







