Small-Disturbance Stability Analysis of Doubly Fed Variable-Speed Pumped Storage Units
Abstract
1. Introduction
2. Mathematical Model of DFIG-VSPS
2.1. DFIG Model
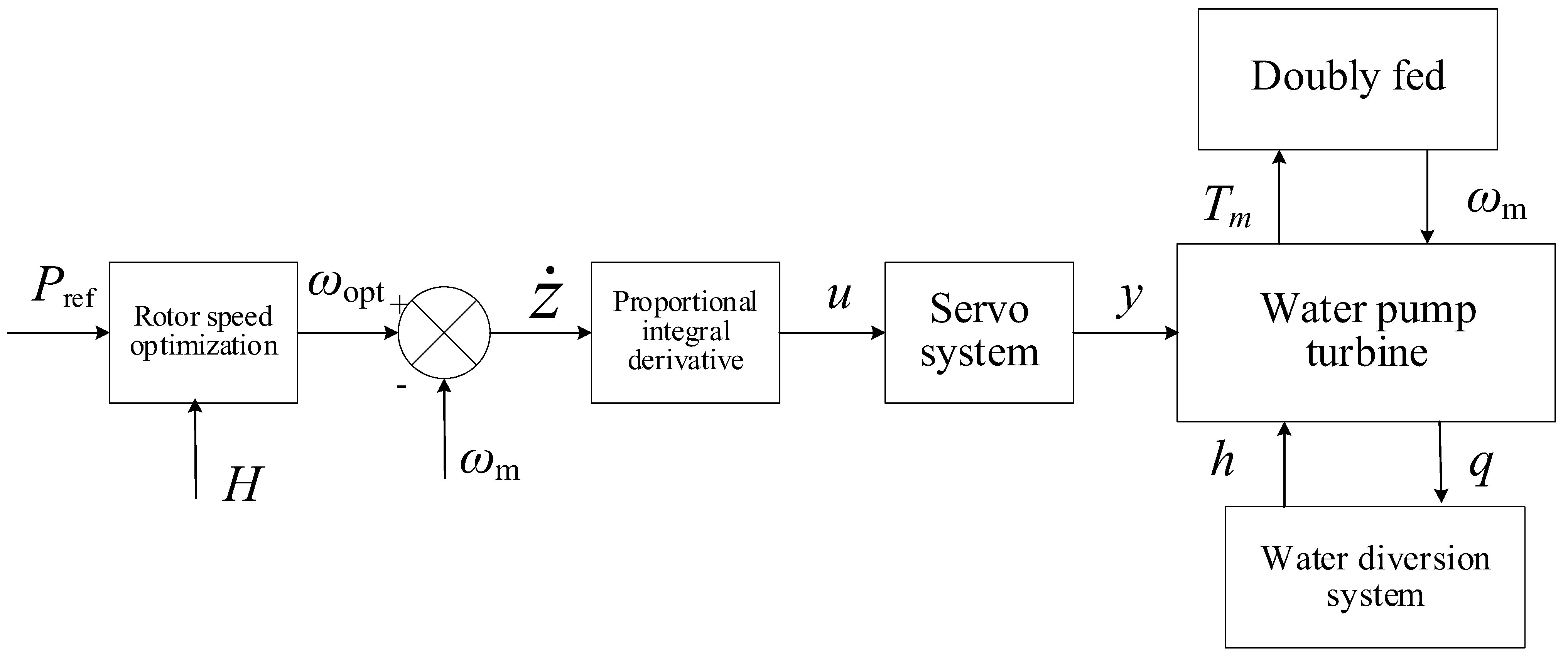
2.2. Pump Turbine and Speed Control System Model
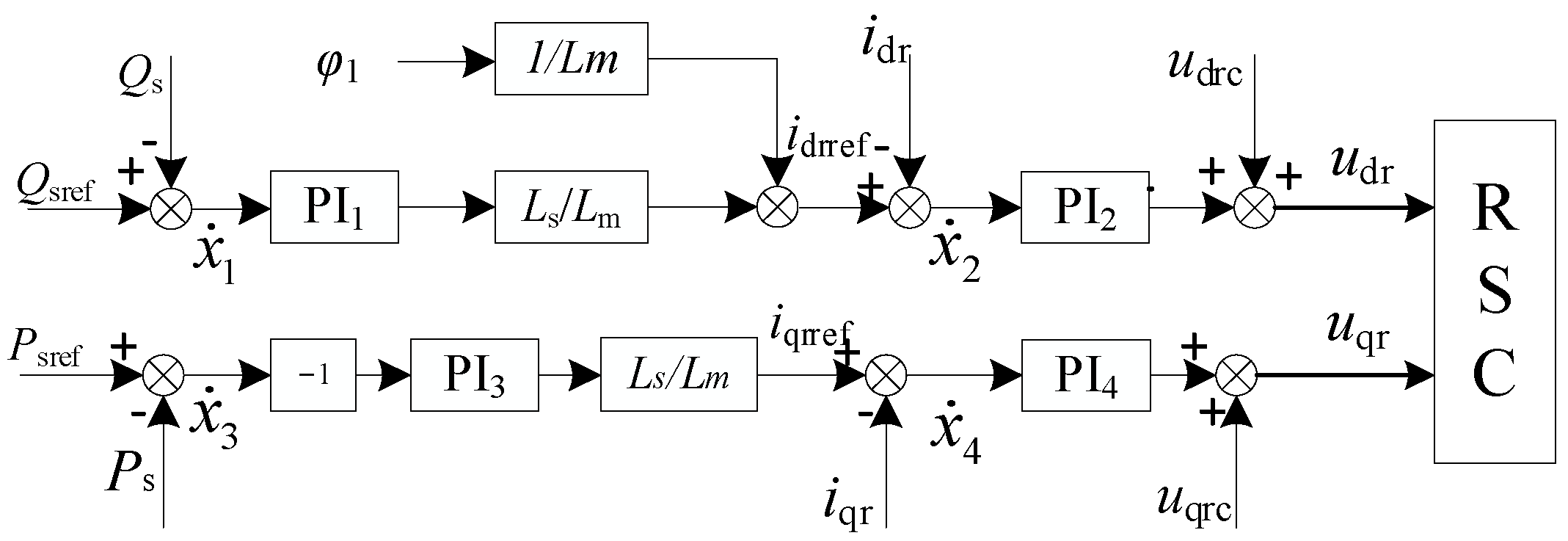
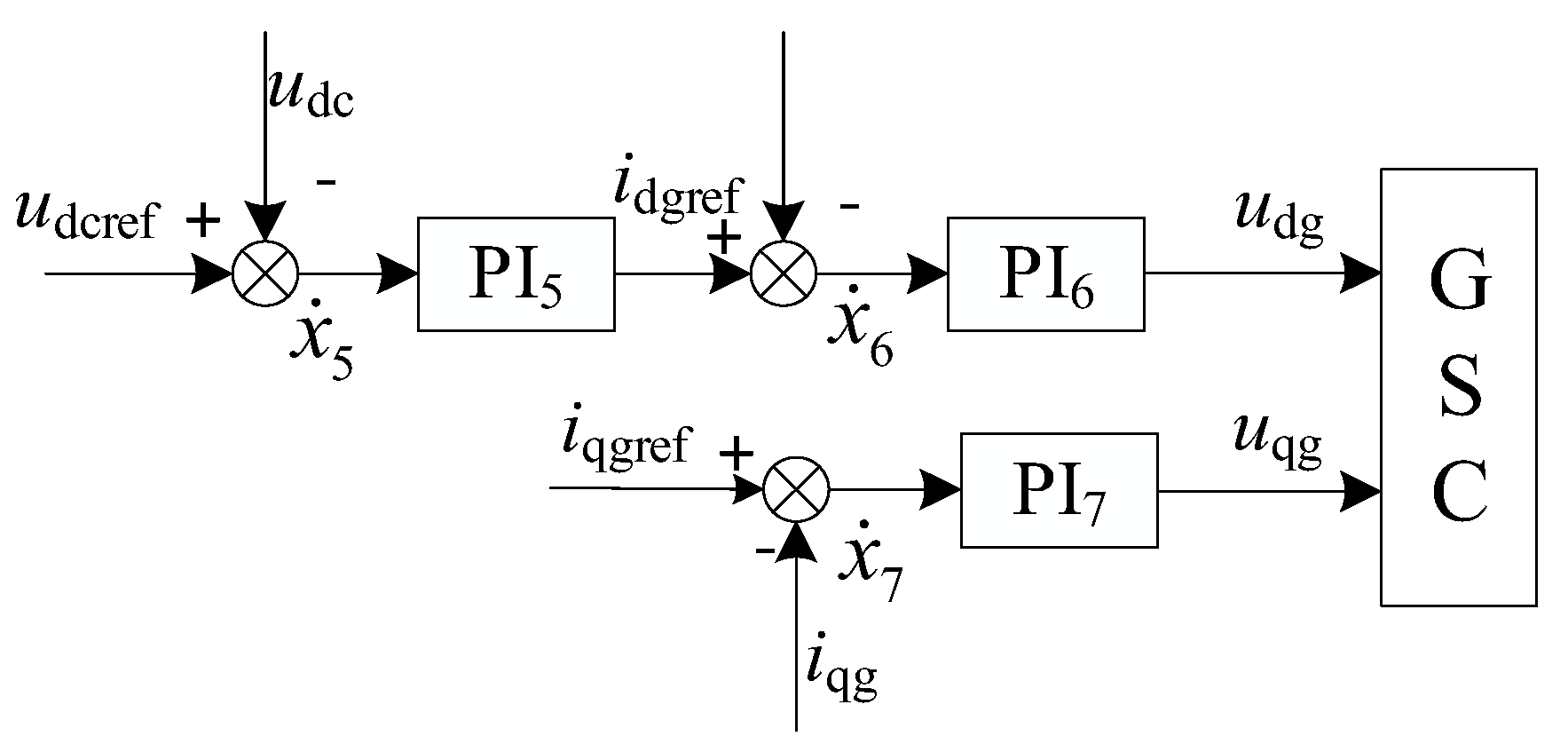
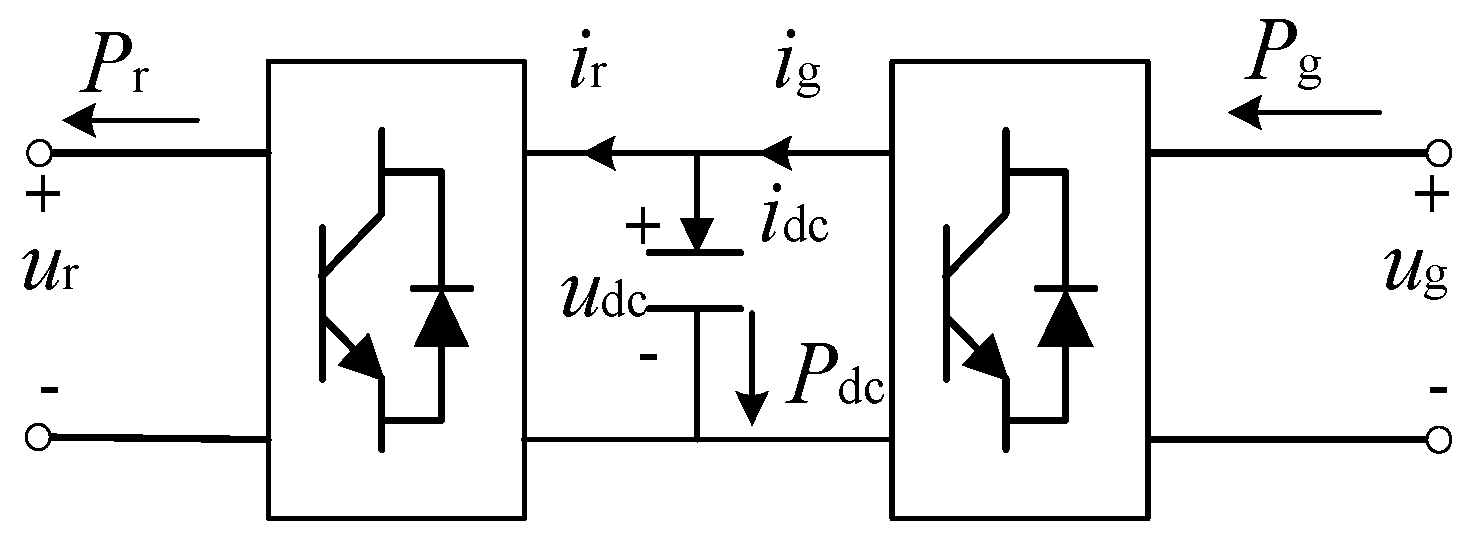
2.3. Converter and Control System Model
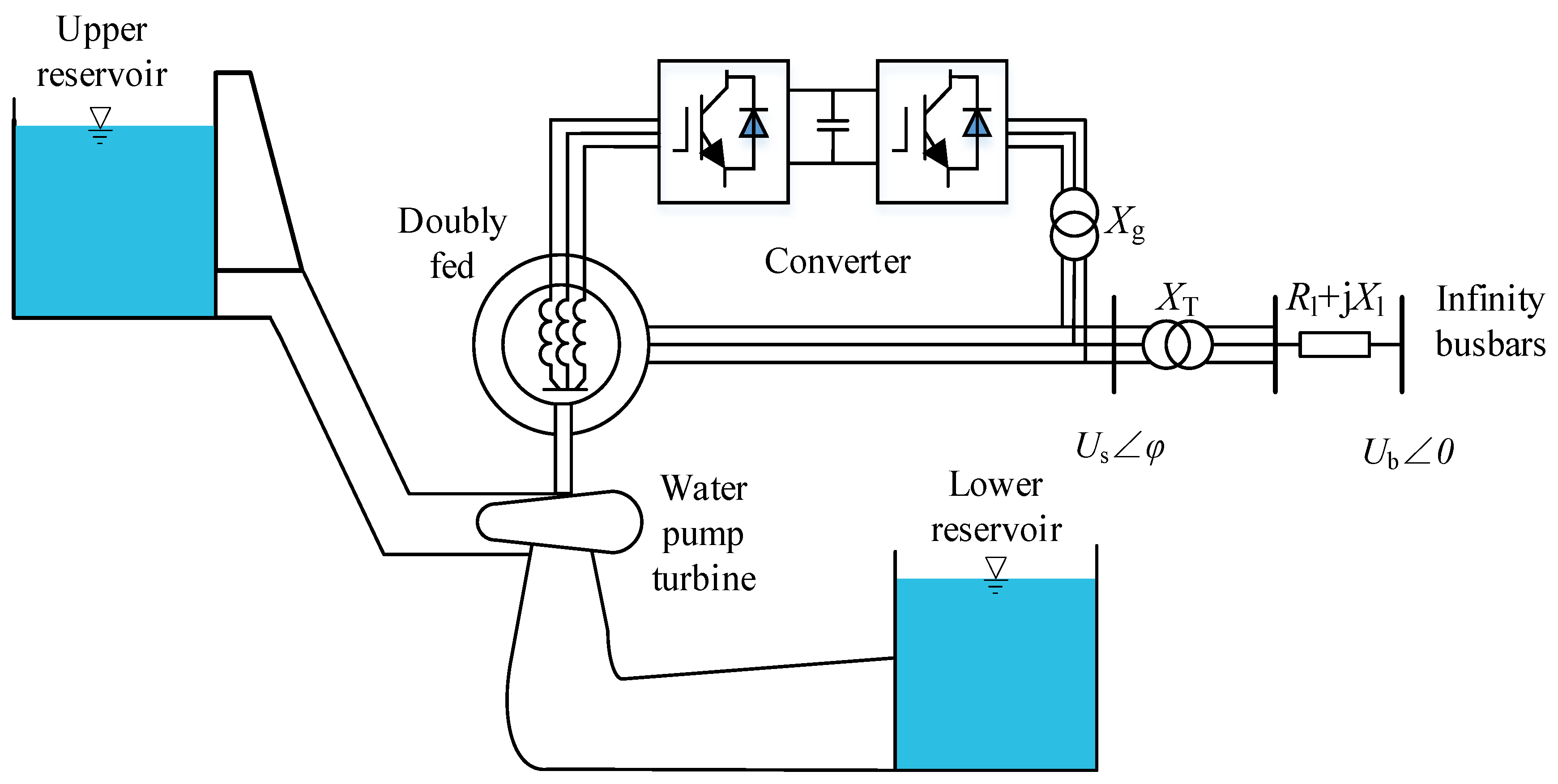
3. Small-Signal Model of the Single-Machine Infinite Bus System
3.1. Single-Machine Infinite Bus System Model
3.2. Small-Signal Model for Simple Systems
4. Simple System Analysis
4.1. State Analysis of OM
4.2. Interactions Between State Variables
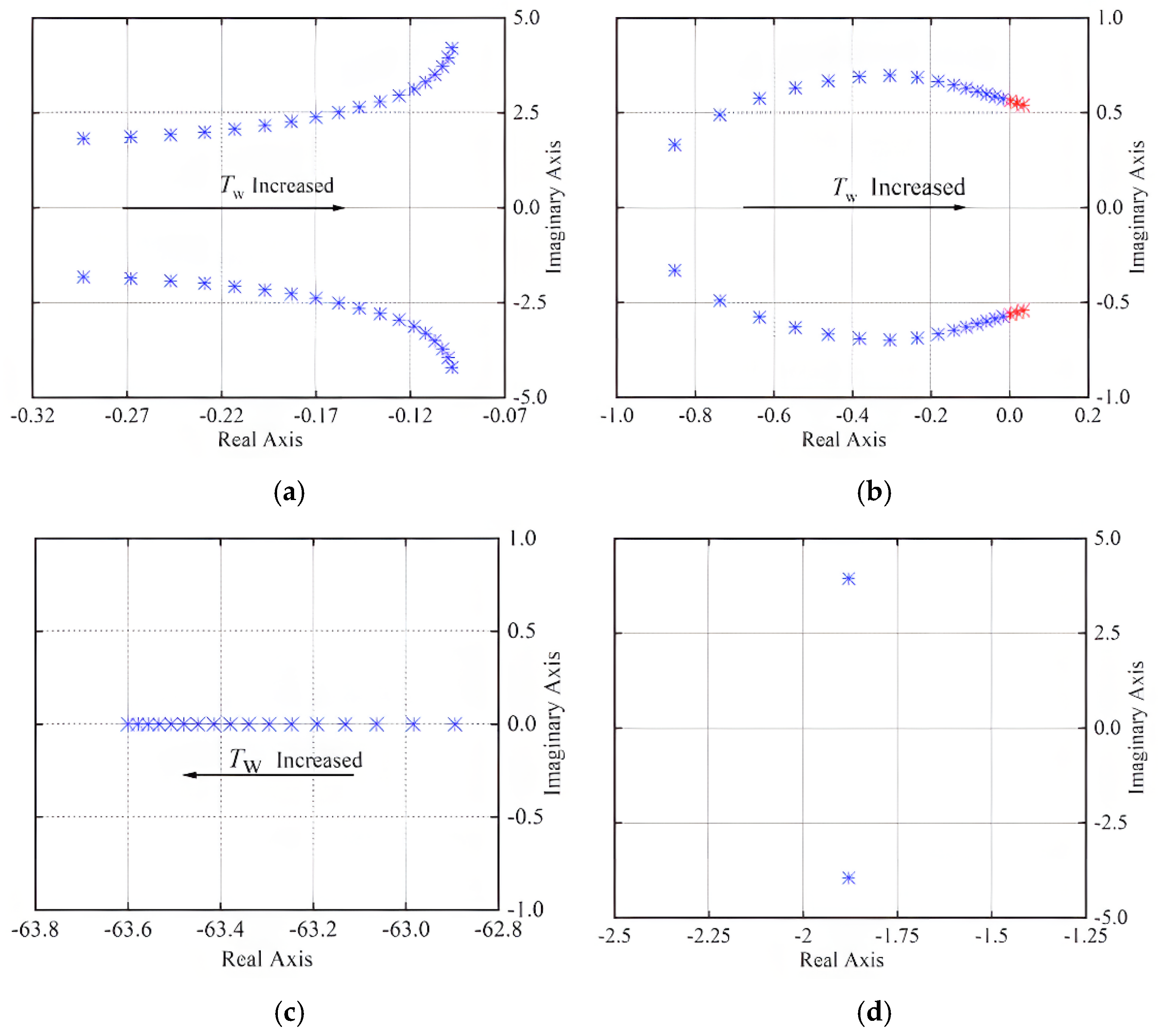
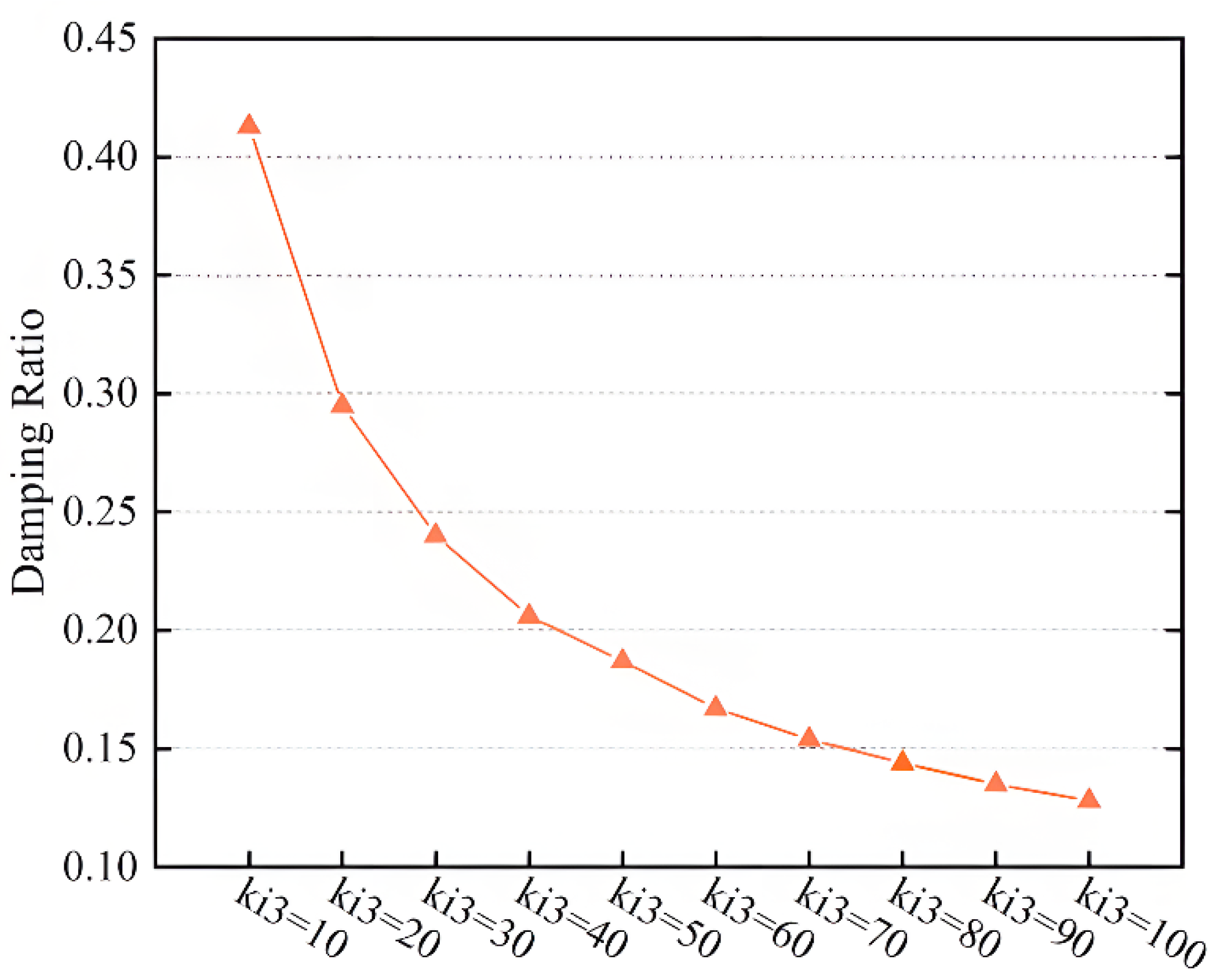
4.3. Influence of System Parameters on Feature Roots
4.3.1. Changing the Inertia Time Constant Tw of Water Flow
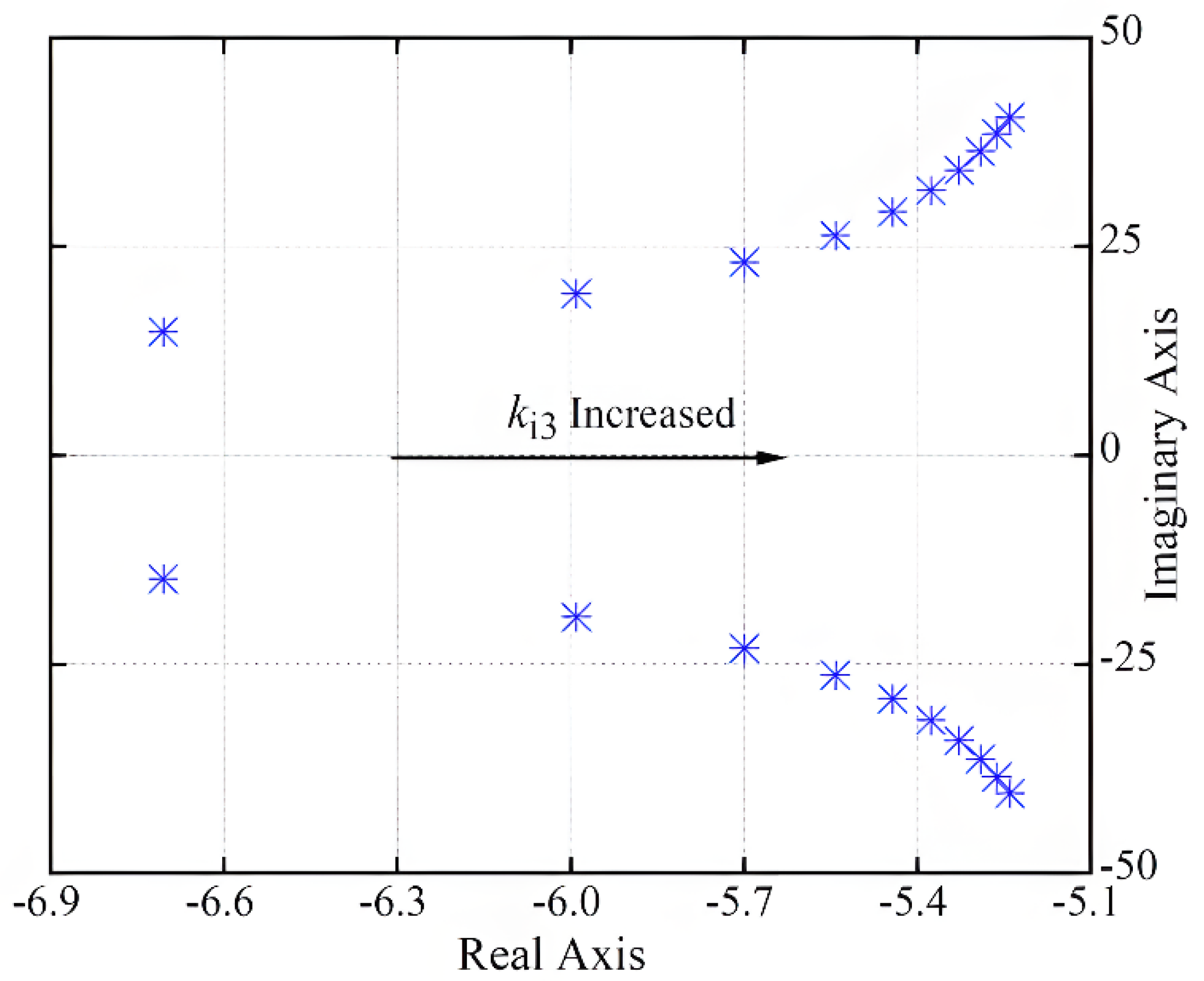
4.3.2. Changing the Control Parameters of the Converter
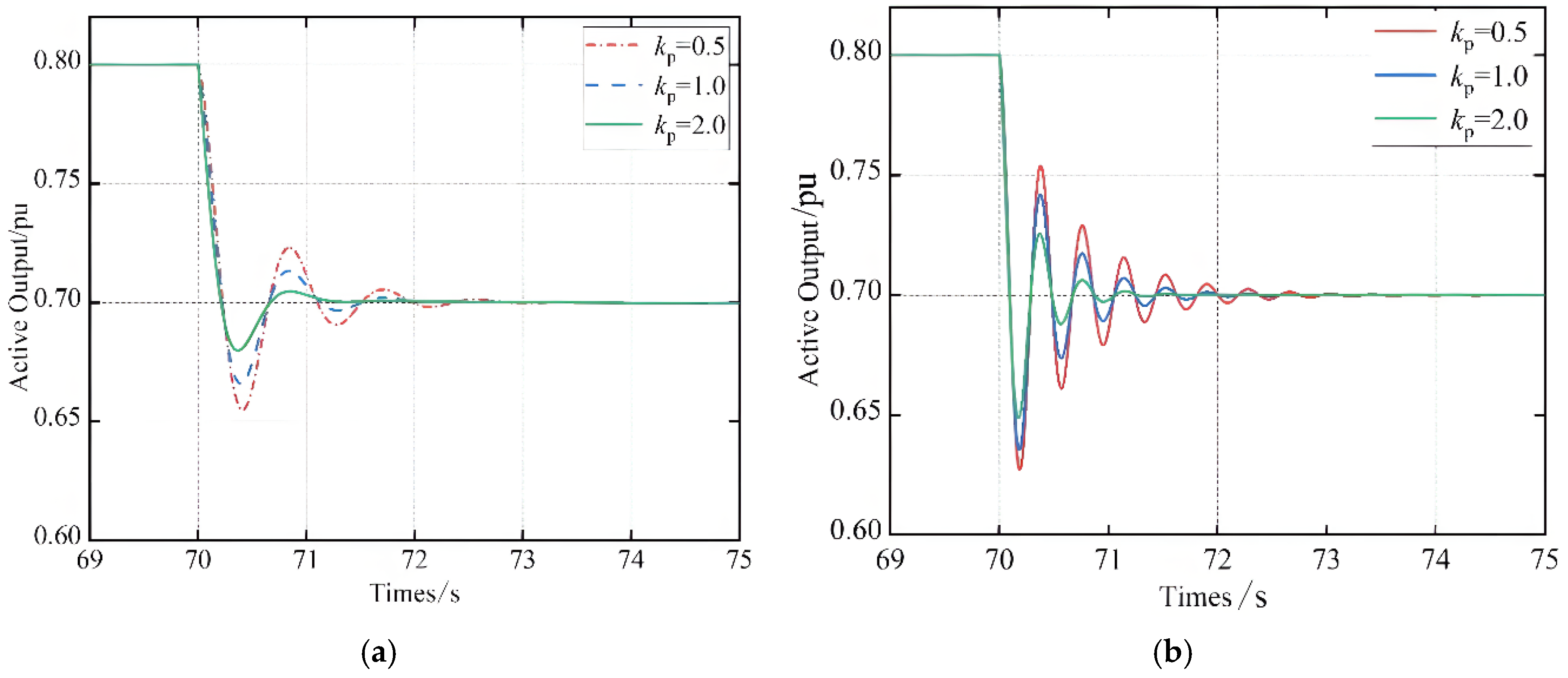
5. Simulation Verification
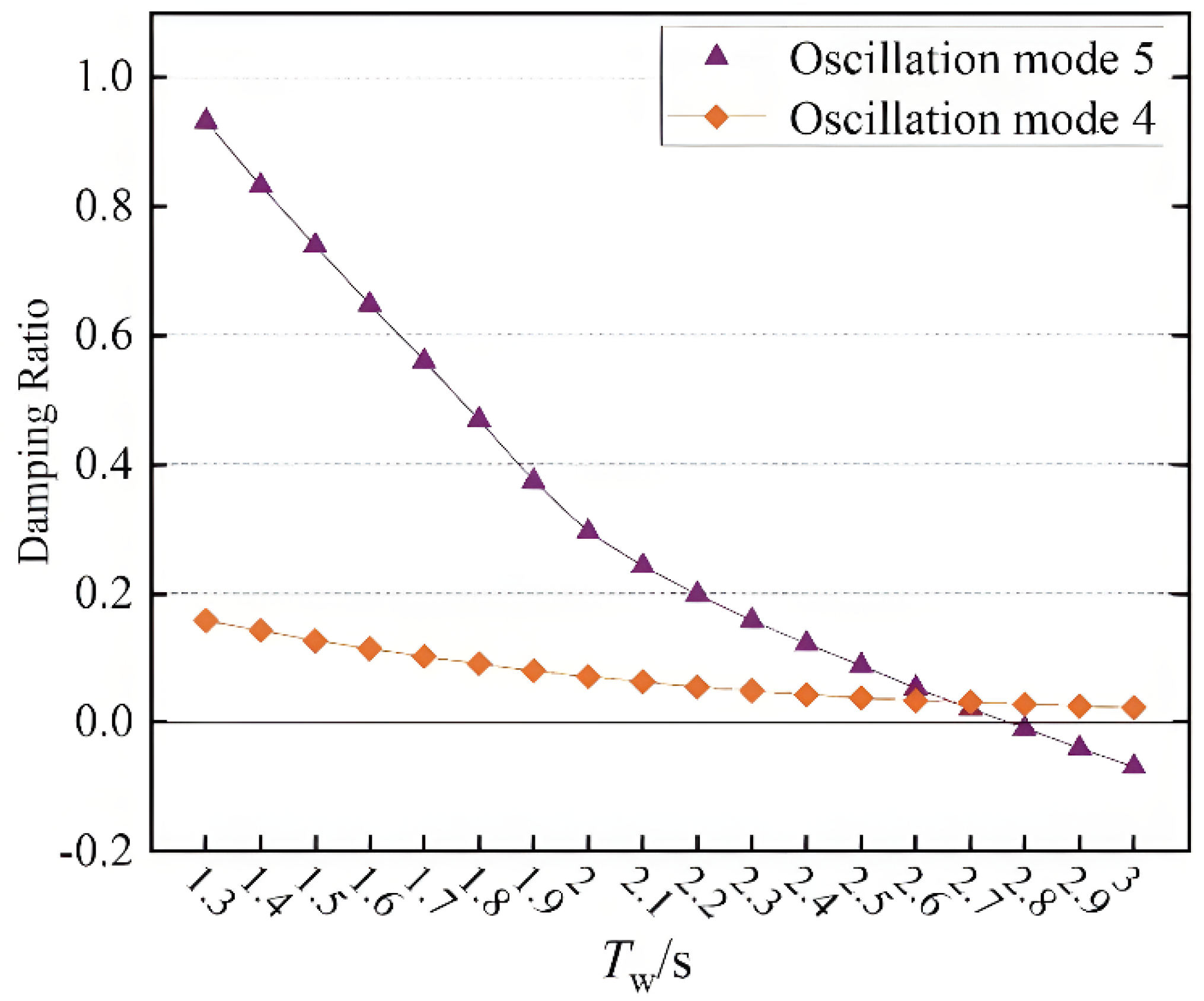
5.1. Impact of Water Flow Inertia Time Constant Tw
5.2. Influence of the Control Parameters of the RSC
6. Small Signal Stability Analysis of Interconnected Systems with DFIG-VSPS
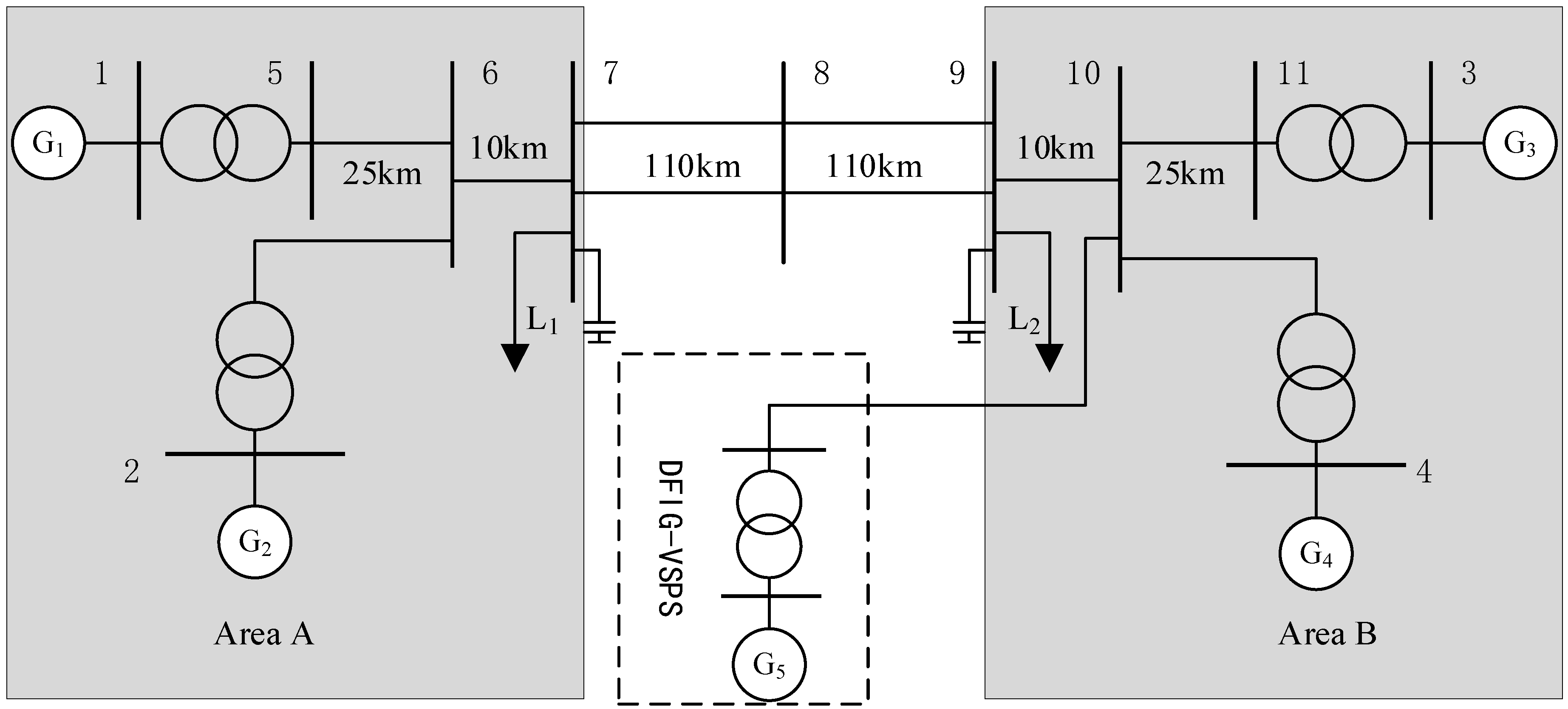
6.1. Four-Machine Two-Area System Model
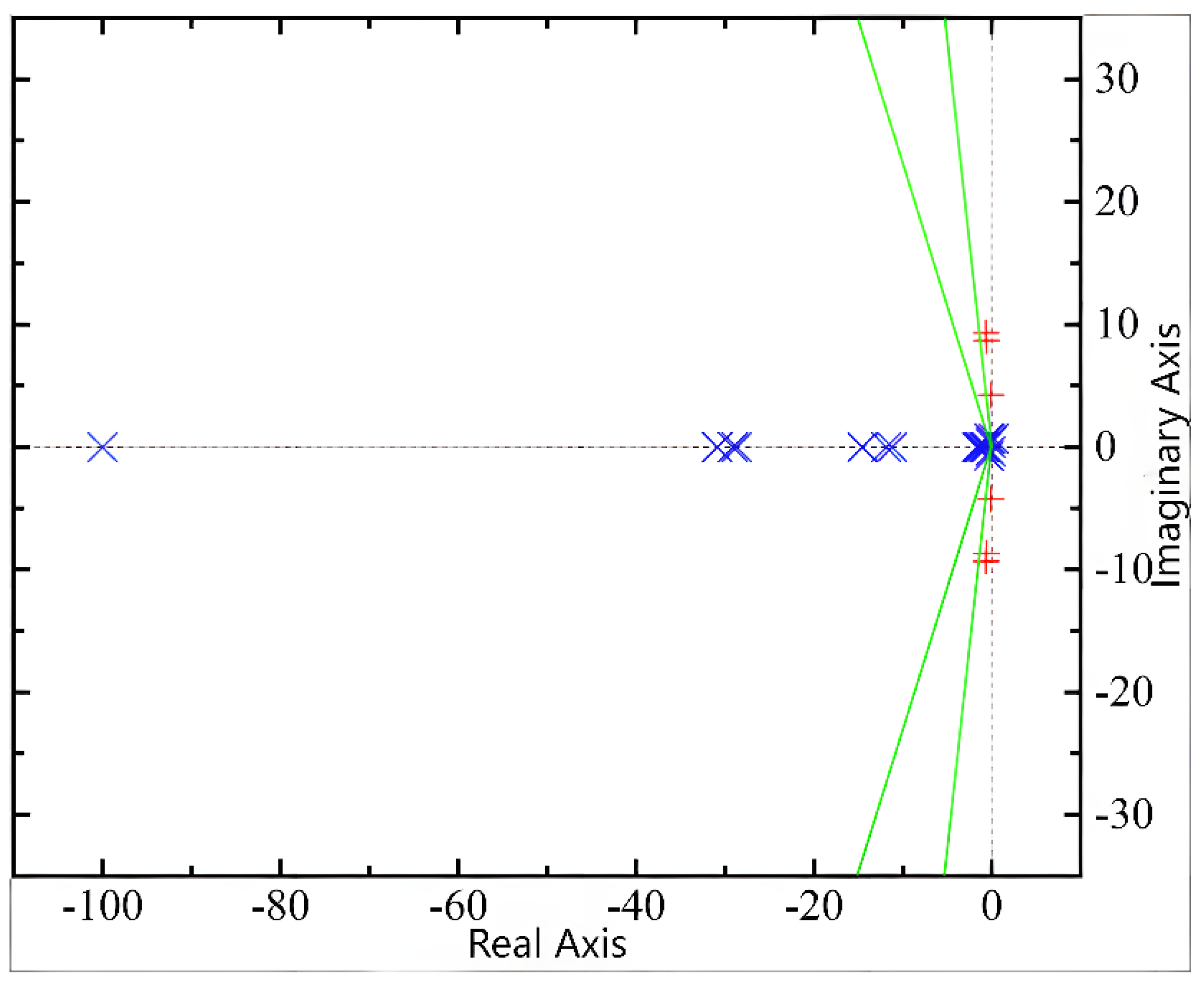
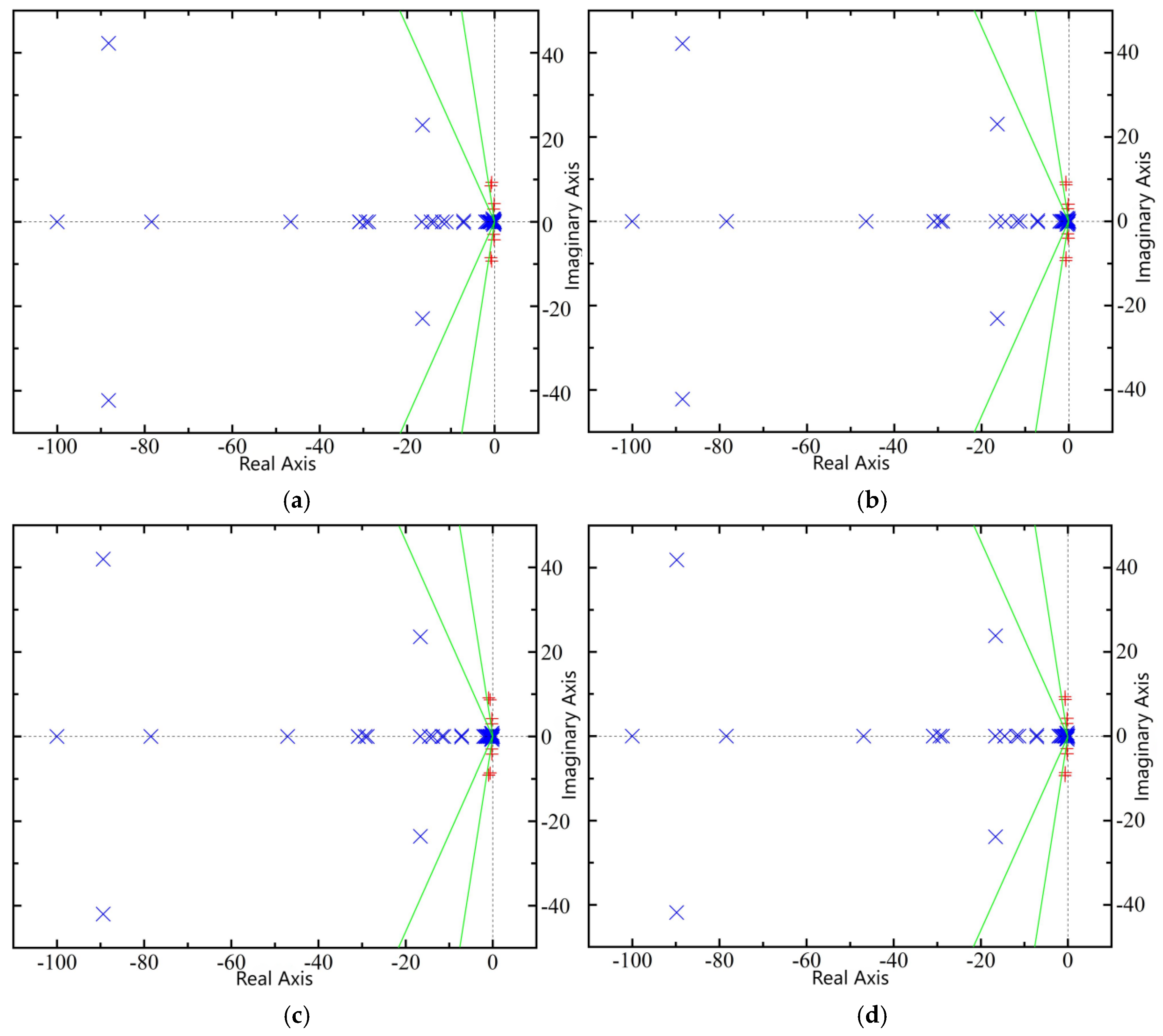
6.2. Eigenvalue Analysis of the System Before and After DFIG-VSPS Integration
6.2.1. Eigenvalue Analysis of the Four-Machine Two-Area System
6.2.2. Eigenvalue Analysis of the System After DFIG-VSPS Integration
- (1)
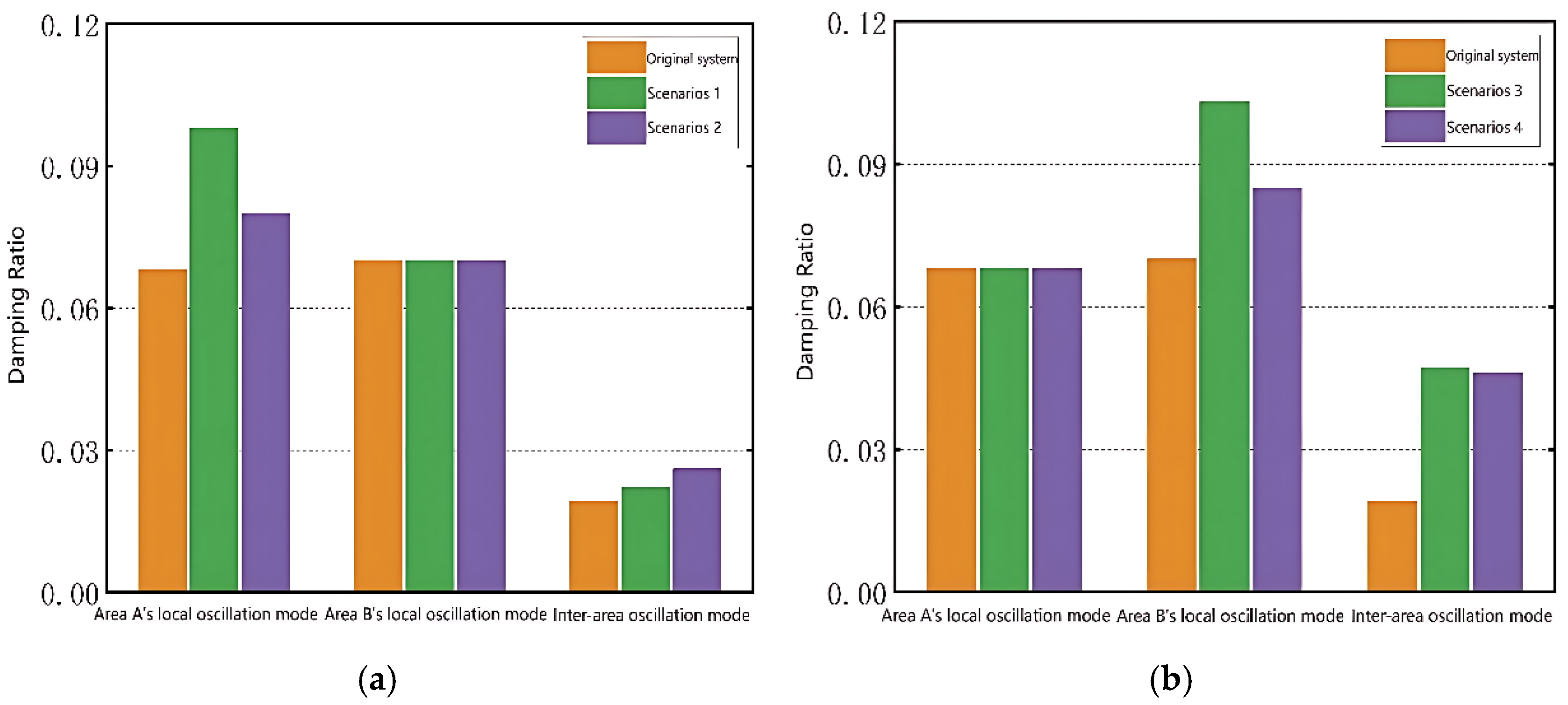
- The integration of DFIG-VSPS introduces a weakly damped oscillation mode to the system, whose characteristics remain unaffected by the unit’s connection location or integration method.
- (2)
- In all four scenarios, DFIG-VSPS integration improves both the local oscillation mode in the connected area and the inter-area oscillation mode to varying degrees, while showing no impact on local oscillation modes outside the connected area.
- (3)
- The improvement effect on regional oscillation modes is more pronounced when DFIG-VSPS is connected to the power-receiving area. The integration approach that maintains power balance by reducing synchronous generator output in the connected area proves more effective in enhancing the local oscillation mode of that area. Therefore, connecting DFIG-VSPS to the power-receiving area (Scenario 3) through synchronous generator output adjustment represents the optimal approach for improving system damping characteristics and strengthening the small signal stability of the interconnected system.
6.2.3. Simulation Verification
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| VSPS | Variable-speed pumped storage |
| DFIG | Doubly fed induction generator |
| DFIG | VSPS doubly fed induction generator-based variable-speed pumped storage |
| PID | Proportional integral derivative |
| DC | Direct current |
| AC | Alternating current |
| OM | Oscillation mode |
| AM | Attenuation mode |
| RSC | Rotor-side converter |
| GSC | Grid-side converter |
| Nomenclature | |
| Stator-side d-axis flux linkage | |
| Stator-side q-axis flux linkage | |
| Rotor-side d-axis magnetic linkage | |
| Rotor-side q-axis magnetic linkage | |
| Stator-side d-axis voltage | |
| Stator-side q-axis voltage | |
| Rotor-side d-axis voltage | |
| Rotor-side q-axis voltage | |
| Stator resistance | |
| Stator-side d-axis current | |
| Stator-side q-axis current | |
| Transient reactance | |
| d-axis transient electromotive force | |
| q-axis transient electromotive force | |
| Stator inductance | |
| Rotor inductance | |
| Mutual inductance | |
| Synchronous speed | |
| Rotor speed | |
| Reference active power | |
| Optimal speed calculation value | |
| Actual speed feedback value | |
| Inertia time constant of water flow | |
| Rotational inertia time constant | |
| Servomotor time constant | |
| Transmission coefficient of pump turbine torque to speed | |
| Transmission coefficient of pump turbine torque to servomotor stroke | |
| Transmission coefficient of pump turbine torque to water head | |
| Transfer coefficient of pump turbine flow rate to speed | |
| Transfer coefficient of pump turbine flow rate to servomotor stroke | |
| Transfer coefficient of pump turbine flow to head | |
| DC bus voltage reference value | |
| DC bus voltage | |
| DC bus current | |
| Grid-side d-axis current | |
| Reference value of grid side q-axis current | |
| Grid-side q-axis current | |
| Output power on the gird side | |
| Output power on the rotor side | |
| DC bus output power | |
| Reactance of step-up transformer | |
| Reactance of transmission lines | |
| Oscillation/attenuation mode (represented by characteristic roots) | |
| Change in feedback value of rotor speed | |
| Change in servomotor stroke | |
| Change in water head | |
| Change in speed | |
| Change in d-axis transient electromotive force | |
| Change in q-axis transient electromotive force | |
| Change in rotor d-axis current | |
| Change in rotor q-axis current | |
| Change in DC bus voltage | |
| Change in intermediate variable i |
References
- Papaefthymiou, S.V.; Lakiotis, V.G.; Margaris, I.D.; Papathanassiou, S.A. Dynamic analysis of island systems with wind-pumped-storage hybrid power stations. Renew. Energy 2015, 74, 544–554. [Google Scholar] [CrossRef]
- Li, J.; Guo, W.; Liu, Y. Nonlinear state feedback-synergetic control for low frequency oscillation suppression in grid-connected pumped storage-wind power interconnection system. J. Energy Storage 2023, 73, 109281. [Google Scholar] [CrossRef]
- Tan, X.; Li, C.; Liu, D.; Wang, H.; Xu, R.; Lu, X.; Zhu, Z. Multi-time scale model reduction strategy of variable-speed pumped storage unit grid-connected system for small-signal oscillation stability analysis. Renew. Energy 2023, 211, 985–1009. [Google Scholar] [CrossRef]
- Mohanpurkar, M.; Ouroua, A.; Hovsapian, R.; Luo, Y.; Singh, M.; Muljadi, E.; Gevorgian, V.; Donalek, P. Real-time co-simulation of adjustable-speed pumped storage hydro for transient stability analysis. Electr. Power Syst. Res. 2018, 154, 276–286. [Google Scholar] [CrossRef]
- Joseph, A.; Desingu, K.; Semwal, R.R.; Chilliah, T.R.; Khare, D. Dynamic performance of pumping mode of 250 MW variable speed hydro-generating unit subjected to power and control circuit faults. IEEE Trans. Energy Convers. 2018, 33, 430–441. [Google Scholar] [CrossRef]
- Desingu, K.; Selvaraj, R.; Chelliah, T.R.; Khare, D. Effective utilization of parallel-connected megawatt three-level back-to-back power converters in variable speed pumped storage units. IEEE Trans. Ind. Appl. 2019, 55, 6414–6426. [Google Scholar] [CrossRef]
- Lung, J.-K.; Lu, Y.; Hung, W.-L.; Kao, W.-S. Modeling and dynamic simulations of doubly fed adjustable-speed pumped storage units. IEEE Trans. Energy Convers. 2007, 22, 250–258. [Google Scholar] [CrossRef]
- Gao, C.; Yu, X.; Nan, H.; Men, C.; Zhao, P.; Fu, J. A fast high-precision model of the doubly-fed pumped storage unit. J. Electr. Eng. Technol. 2021, 16, 797–808. [Google Scholar] [CrossRef]
- Zhao, K.; Xu, Y.; Guo, P.; Qian, Z.; Zhang, Y.; Liu, W. Multi-scale oscillation characteristics and stability analysis of pumped-storage unit under primary frequency regulation condition with low water head grid-connected. Renew. Energy 2022, 189, 1102–1119. [Google Scholar] [CrossRef]
- Li, S.; Xie, H.; Yan, Y.; Wu, T.; Huang, T.; Liu, Y.; Li, C.; Liang, H.; Cao, T. Excitation control of variable speed pumped storage unit for electromechanical transient modeling. Energy Rep. 2022, 8 (Suppl. 8), 818–825. [Google Scholar] [CrossRef]
- Zhang, X.; Zhu, Z.; Fu, Y.; Li, L. Optimized virtual inertia of wind turbine for rotor angle stability in interconnected power systems. Electr. Power Syst. Res. 2020, 180, 106157. [Google Scholar] [CrossRef]
- Gebru, F.M.; Khan, B.; Alhelou, H.H. Analyzing low voltage ride through capability of doubly fed induction generator based wind turbine. Comput. Electr. Eng. 2020, 86, 106727. [Google Scholar] [CrossRef]
- Xu, H.; Li, Z.; Wu, H.; Zhao, R.; Hu, J. Reduced-order modeling of DFIG-based wind turbine connected into weak AC grid based on electromechanical time scale. Energy Rep. 2020, 6 (Suppl. 9), 886–895. [Google Scholar] [CrossRef]
- Han, M.; Kawkabani, B.; Simond, J.-J. Eigenvalues analysis applied to the stability study of a variable speed pump turbine unit. In Proceedings of the 2012 XXth International Conference on Electrical Machines, Marseille, France, 2–5 September 2012; pp. 907–913. [Google Scholar] [CrossRef]
- Wang, F.; Tan, T.; Liu, K.; Zhu, S.; Yang, J.; Li, Y.; Hu, C.; Qin, L. Small signal stability analysis of the joint operation system of variable speed pumped storage units and direct drive wind turbines. Electr. Power Autom. Equip. 2021, 41, 65–72. [Google Scholar] [CrossRef]
- Gao, C.; Yu, X.; Nan, H.; Men, C.; Zhao, P.; Cai, Q.; Fu, J. Stability and dynamic analysis of doubly-fed variable speed pump turbine governing system based on Hopf bifurcation theory. Renew. Energy 2021, 175, 568–579. [Google Scholar] [CrossRef]
- Guo, W.; Xu, X. Sliding mode control of regulating system of pumped storage power station considering nonlinear pump-turbine characteristics. J. Energy Storage 2022, 52, 105071. [Google Scholar] [CrossRef]
- Shi, H.; Wang, Y.; Sun, X.; Chen, G.; Ding, L.; Pan, P.; Zeng, Q. Stability characteristics analysis and ultra-low frequency oscillation suppression strategy for FSC-VSPSU. Int. J. Electr. Power Energy Syst. 2024, 155, 109623. [Google Scholar] [CrossRef]
- Si, Y.; Wang, Z.; Liu, L. Sensitivity analysis of power system small signal stability to geomagnetic disturbances. Int. J. Electr. Power Energy Syst. 2023, 148, 108970. [Google Scholar] [CrossRef]
- de Toledo, P.F.; Bergdahl, B.; Asplund, G. Multiple infeed short circuit ratio—Aspects related to multiple HVDC into one AC network. In Proceedings of the 2005 IEEE/PES Transmission & Distribution Conference & Exposition: Asia and Pacific, Dalian, China, 18 August 2005; pp. 1–6. [Google Scholar] [CrossRef]








| Mode | Order | Eigenvalue | Damping Ratio |
|---|---|---|---|
| OM1 | λ1,2 | –5.443 ± 29.157 | 0.184 |
| OM2 | λ3,4 | –1.879 ± 3.943 | 0.430 |
| OM3 | λ5,6 | –0.128 ± 0.896 | 1.141 |
| OM4 | λ7,8 | –0.195 ± 2.166 | 0.090 |
| OM5 | λ9,10 | –0.377 ± 0.710 | 0.468 |
| AM1 | λ11 | –63.192 | 1 |
| AM2 | λ12 | –7.689 | 1 |
| AM3 | λ13 | –5.818 | 1 |
| AM4 | λ14 | –1.401 | 1 |
| AM5 | λ15 | –0.580 | 1 |
| AM6 | λ16 | –0.560 | 1 |
| Variable | λ1 λ2 | λ3 λ4 | λ5 λ6 | λ7 λ8 | λ9 λ10 | λ11 | λ12 | λ13 | λ14 | λ15 | λ16 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| ∆ωm | 0.01 | 0 | 0 | 0.18 | 0.34 | 0.01 | 0.01 | 0 | 0 | 0.15 | 0 |
| ∆y | 0.01 | 0 | 0 | 0.15 | 0.26 | 0.67 | 0.02 | 0 | 0 | 0.01 | 0 |
| ∆h | 0.01 | 0 | 0 | 0.11 | 0.24 | 0.32 | 0.02 | 0 | 0 | 0 | 0 |
| ∆z | 0 | 0 | 0 | 0.01 | 0.02 | 0 | 0 | 0 | 0 | 0.83 | 0 |
| 0.05 | 0 | 0.02 | 0.22 | 0.05 | 0 | 0.01 | 0.01 | 0.01 | 0 | 0 | |
| 0 | 0 | 0.02 | 0.22 | 0.04 | 0 | 0 | 0.03 | 0.07 | 0 | 0.03 | |
| ∆idr | 0 | 0 | 0 | 0.03 | 0.01 | 0 | 0 | 0.74 | 0.18 | 0 | 0.03 |
| ∆iqr | 0.45 | 0 | 0.01 | 0.04 | 0.01 | 0 | 0.07 | 0.01 | 0 | 0 | 0 |
| ∆x1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0.08 | 0.17 | 0 | 0.8 |
| ∆x2 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0.14 | 0.57 | 0 | 0.12 |
| ∆x3 | 0.36 | 0 | 0 | 0 | 0 | 0 | 0.1 | 0 | 0 | 0 | 0 |
| ∆x4 | 0.12 | 0 | 0 | 0 | 0 | 0 | 0.77 | 0 | 0 | 0 | 0 |
| ∆udc | 0 | 0.03 | 0.45 | 0.02 | 0.01 | 0 | 0 | 0 | 0 | 0 | 0 |
| ∆x5 | 0 | 0.05 | 0.45 | 0.02 | 0.01 | 0 | 0 | 0 | 0 | 0 | 0 |
| ∆x6 | 0 | 0.46 | 0.02 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| ∆x7 | 0 | 0.46 | 0.02 | 0.01 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| Parameter | G1, G2 | G3, G4 |
|---|---|---|
| /pu | 1.8 | 1.8 |
| /pu | 1.7 | 1.7 |
| /pu | 0.3 | 0.3 |
| /pu | 0.55 | 0.55 |
| /pu | 0.25 | 0.25 |
| /pu | 0.25 | 0.25 |
| /s | 8 | 8 |
| /s | 0.4 | 0.4 |
| /s | 0.03 | 0.03 |
| /s | 0.05 | 0.05 |
| /pu | 6.5 | 6.175 |
| Oscillation Mode | Eigenvalue | Damping Ratio | Oscillation Frequency/HZ | Relevant Generating Units |
|---|---|---|---|---|
| 1 | −0.595 ± j8.671 | 0.068 | 1.380 | G1, G2 |
| 2 | −0.658 ± j9.321 | 0.070 | 1.483 | G3, G4 |
| 3 | −0.081 ± j4.237 | 0.019 | 0.674 | G1, G2, G3, G4 |
| Oscillation Mode | Eigenvalue | Damping Ratio | Oscillation Frequency/HZ | Relevant Generating Units | |
|---|---|---|---|---|---|
| Four-Machine Two-Area System | 1 | −0.595 ± j8.671 | 0.068 | 1.380 | G1, G2 |
| 2 | −0.658 ± j9.321 | 0.070 | 1.483 | G3, G4 | |
| 3 | −0.081 ± j4.237 | 0.019 | 0.674 | G1, G2, G3, G4 | |
| Scenario 1 | 1 | −0.857 ± j8.659 | 0.098 | 1.378 | G1, G2 |
| 2 | −0.659 ± j9.321 | 0.070 | 1.483 | G3, G4 | |
| 3 | −0.091 ± j4.218 | 0.022 | 0.671 | G1, G2, G3, G4 | |
| 4 | −0.247 ± j2.981 | 0.083 | 0.474 | DFIG-VSPS | |
| Scenario 2 | 1 | −0.682 ± j8.512 | 0.080 | 1.355 | G1, G2 |
| 2 | −0.659 ± j9.318 | 0.070 | 1.483 | G3, G4 | |
| 3 | −0.107 ± j4.043 | 0.026 | 0.643 | G1, G2, G3, G4 | |
| 4 | −0.249 ± j2.981 | 0.083 | 0.474 | DFIG-VSPS | |
| Scenario 3 | 1 | −0.595 ± j8.669 | 0.068 | 1.380 | G1, G2 |
| 2 | −0.948 ± j9.119 | 0.103 | 1.451 | G3, G4 | |
| 3 | −0.198 ± j4.212 | 0.047 | 0.670 | G1, G2, G3, G4 | |
| 4 | −0.248 ± j2.982 | 0.083 | 0.475 | DFIG-VSPS | |
| Scenario 4 | 1 | −0.595 ± j8.668 | 0.068 | 1.380 | G1, G2 |
| 2 | −0.792 ± j9.325 | 0.085 | 1.484 | G3, G4 | |
| 3 | −0.161 ± j4.199 | 0.038 | 0.668 | G1, G2, G3, G4 | |
| 4 | −0.250 ± j2.981 | 0.084 | 0.474 | DFIG-VSPS |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, X.; Cui, Y.; Qi, H.; Gao, C.; He, Z.; Nan, H. Small-Disturbance Stability Analysis of Doubly Fed Variable-Speed Pumped Storage Units. Energies 2025, 18, 2796. https://doi.org/10.3390/en18112796
Yu X, Cui Y, Qi H, Gao C, He Z, Nan H. Small-Disturbance Stability Analysis of Doubly Fed Variable-Speed Pumped Storage Units. Energies. 2025; 18(11):2796. https://doi.org/10.3390/en18112796
Chicago/Turabian StyleYu, Xiangyang, Yujie Cui, Hao Qi, Chunyang Gao, Ziming He, and Haipeng Nan. 2025. "Small-Disturbance Stability Analysis of Doubly Fed Variable-Speed Pumped Storage Units" Energies 18, no. 11: 2796. https://doi.org/10.3390/en18112796
APA StyleYu, X., Cui, Y., Qi, H., Gao, C., He, Z., & Nan, H. (2025). Small-Disturbance Stability Analysis of Doubly Fed Variable-Speed Pumped Storage Units. Energies, 18(11), 2796. https://doi.org/10.3390/en18112796





