Numerical Simulation and Optimized Field-Driven Design of Triple Periodic Minimal Surface Structure Liquid-Cooling Radiator

Abstract

This work investigates the design of liquid-cooled heat sinks for IGBT modules via optimizing the Triple Periodic Minimal Surface (TPMS) structure. The performance of heat sinks with different porosity TPMS structures was compared through the finite element simulation software Fluent. The results indicate that smaller porosity is conducive to improving the heat dissipation efficiency, but the difference in pressure between the entrance and exit increases. The field-driven design method is further adopted to adjust the porosity according to the temperature field distribution, and the TPMS channel structures were optimized by nTopology software. The results show that the optimized Schwarz P, Gyroid, and Diamond structures have a comparable effect on reducing the maximum surface temperature as that of TPMS structures with uniform porosity; however, the differential pressure at the inlet and outlet decreased remarkably by 94.8%, 90.8%, and 88.9%, respectively, compared to the structure with a uniform porosity of 0.32. The Nusselt numbers of the optimized Gyroid and Diamond structures increased by 19.2% and 12.3%, respectively, compared to their structures with a uniform porosity of 0.84. This study illustrates the advantages of the field-driven design in enhancing the heat dissipation and reducing pressure loss, which provides an effective design solution for the heat dissipation of IGBT modules.

1. Introduction

IGBT modules are advancing towards miniaturization, high power, and high integration, significantly increasing the demand for enhanced heat dissipation capabilities [1]. Traditional air-cooling methods are inadequate for high-power electronic devices [2]. Porous structures, unlike traditional heat dissipation fins [3,4], offer more uniform surface heat dissipation, mitigating local overheating. The Triple Periodic Minimum Surface (TPMS) has emerged as a superior solution for constructing porous structures due to its smooth surface, highly interconnected porous structure, and mathematically controllable geometry [5]. Research has consistently demonstrated that TPMS structures exhibit excellent flow and heat transfer performance. Nada Baobaid et al. [6] explored three TPMS structures as heat sinks under natural convection, using CFDs (computational fluid dynamics) to analyze thermal performance and fluid flow under different environmental conditions. Their findings suggest that TPMS geometries outperform conventional fin-structured heat sinks. Huang et al. [7] developed a multi-scale geometric topology optimization method for thermal performance, incorporating TPMS dots into a porous structure to create a lightweight gradient dot porous structure with superior thermal properties. Li [8] applied TPMS heat sinks on circuit boards, noting minimal flow separation and a 16% higher heat transfer coefficient than traditional heat exchangers.

TPMS structures also exhibit a preponderance in convective heat transfer. Cheng et al. [9] studied the flow, heat transfer, and mechanical properties of four TPMS structures, concluding that TPMS-based heat exchangers surpass conventional designs. Kaur Inderjot et al. [10] compared Gyroid-sheet and Primitive-sheet TPMS surfaces, finding them superior to random metal foam structures. Attarzade et al. [11] designed a Schwarz-D structure heat exchanger, which outperformed conventional designs, especially with thinner walls. While these studies focused on optimized TPMS structures with uniform porosity, they also noted increased differential pressure. The authors of [12,13] indicate that porosity can affect flow resistance and heat transfer, so that controlled porosity can enhance performance. Cheng et al. [14] demonstrated that graded porous structures, created via additive manufacturing, offer better cooling by improving coolant distribution and reducing hot spot temperatures.

Field-driven design, utilizing equations, distances, or simulation results to guide design changes, has been applied across various fields. For example, Tel et al. [15] used nTopology software to design custom osteosynthesis plates, simplifying complex workflows. Baladé [16] used nTopology to design a drive based on a scalar stress field, reducing component weight by 15.8%. Liu [17] explored a stress field-driven sphere filling algorithm, maintaining geometric properties and limiting node distances. Lalegani [18] designed a suspension arm mesh structure with variable thickness, reducing weight without sacrificing strength. Mata [19] applied field-driven design to an unmanned boat wing, creating variable shell thickness based on stress analysis. García-Ávila [20] used nTopology to generate models, create meshes, and perform static analysis, resulting in porous structures with complex, improvable properties.

Field-driven design also optimizes heat exchangers, maximizing heat transfer and minimizing pressure drop [21]. Weems [22] designed a thickness-modified heat sink based on temperature distribution from COMSOL simulations, demonstrating the method’s potential for optimizing conventional designs. Wang et al. [23] proposed a field-driven additive manufacturing framework for designing bifunctional gradient TPMS lattice structures, highlighting their advantages in compressive mechanical response and heat transfer properties.

Despite these advancements, field-driven design for temperature field-based heat sinks with TPMS structures remains underexplored. This study focuses on a liquid-cooled heat sink for a single IGBT module, establishing three TPMS structures (Schwarz P, Gyroid, and Diamond) in the flow channel with seven different porosities. Using Fluent (2022R1) for finite element simulation, the heat dissipation performance of these TPMS structures is compared. nTopology software (nTop 4.2.3 version) is then used to design a TPMS channel structure with variable porosity based on the temperature field, optimizing thermal performance. Finally, the finite element simulation of the optimized channel is compared with the pre-optimized structure to validate the effectiveness of field-driven design in heat dissipation structures.

2. Materials and Methods

2.1. TPMS Structure Heat Sink Model

In this work, the Infineon FF450R12ME4 single IGBT module (Infineon Technologies, Neubiberg, Germany), is set as the heat source, the IGBT module, and the liquid-cooled heat sink model structure schematic shown in Figure 1a. Modelled in SOLIDWORKS 2020, the liquid-cooled radiator consists of a wall panel and fluid flow channels. The overall size is designed as 160 mm × 114 mm × 28 mm, the wall panel thickness is designed as 3 mm, and the fluid inlet and outlet of the liquid-cooled radiator in the length direction are designed as a cylinder with a length of 28 mm and a radius of 5 mm. The structural dimensions of the liquid-cooled radiator are shown in Figure 1b.

There are various methods for generating the coordinates of the TPMS structure. Approximating the periodic surface of the TPMS is generally defined as follows [24]:

$$\mathrm{\varnothing }\left(r\right)={\sum }_{k=1}^K A_k\cos \left[{\displaystyle \frac{2\pi \left(h_k\cdot r\right)}{{\lambda }_k}}+p_k\right]=C$$

(1)

In the above Equation (1), $r$ denotes the position vector in Euclidean space, $A_k$ denotes the amplitude factor, $h_k$ is the kth lattice vector in the reciprocal space, ${\lambda }_k$ is the period wavelength, $p_k$ denotes the phase offset, and C is a constant.

Based on the above implicit functional equations, this work establishes three kinds of TPMS structure channels in the flow channel based on the parametric modelling software nTopology: Schwarz P, Gyroid, and Diamond. The size of each unit cell is set as 22 mm × 22 mm × 22 mm, and seven porosities are generated for each structure: 0.32, 0.38, 0.50, 0.62, 0.68, 0.74, and 0.84. The cross-section of the internal flow path of the radiator is rectangular and the whole flow path is generally a “Z” shape. The cross-sections and fluid shapes of the three TPMS-structured radiators with a porosity of 0.5 are shown in Figure 1c–e.

2.2. Numerical Model

Throughout the simulation process, the heat transfer model operates under the following assumptions:

(1) The fluid flow and heat transfer are three-dimensional steady-state phenomena, with the flow being incompressible and turbulent, and gravitational effects are disregarded.

(2) During the heat transfer process, heat generation within the IGBT module is uniform, neglecting convective heat transfer processes with air as well as thermal radiation.

(3) The coupled calculation method is used to deal with the interaction between solid domains and the coupled heat transfer process between the fluid domain and the solid domain. All channel walls are subjected to no-slip velocity boundary conditions.

The finite element solution is the basis of the CFD calculation. The flow problem of the fluid is carried out around the N-S (Navier–Stokes) equation, and its governing equation is as follows:

$$\rho {\displaystyle \frac{\mathrm{d}u}{\mathrm{d}t}}=\mu {\nabla }^{2}u-\mathrm{grad}p+f$$

(2)

In the above Formula (2), $\rho$ is the liquid density, $u$ is the velocity of the fluid, $\mu$ is the hydrodynamic viscosity, $\mathrm{grad}p$ is the pressure, and $f$ is the volume force.

The continuity equation is used as the mass conservation equation, momentum conservation equation, and energy conservation equation [25].

The mass conservation equation (continuity equation) is as follows:

$$\nabla ·\left(\rho \overrightarrow{v}\right)=0$$

(3)

where $\rho$ is the fluid density and $\overrightarrow{v}$ is the fluid velocity.

The momentum conservation equation is as follows:

$$\nabla ·\left(\rho \overrightarrow{v}\overrightarrow{v}\right)=-\nabla P+\nabla ·\left(\mu \nabla \overrightarrow{v}\right)+\rho \overrightarrow{g}$$

(4)

where $P$ is the pressure, $\mu$ is the dynamic viscosity, and $\overrightarrow{g}$ is the gravitational acceleration.

The energy conservation equation is as follows:

$$\nabla ·\left(\rho E\overrightarrow{v}\right)=-\nabla ·\left(\lambda \nabla T\right)+\nabla ·\left(\mu \overrightarrow{v}·\nabla \overrightarrow{v}\right)+\rho \overrightarrow{v}·\overrightarrow{g}$$

(5)

where $E$ is the total energy per unit volume, $T$ is the fluid temperature, and $\lambda$ is the thermal conductivity.

The convection term adopts the Second-Order Upwind Scheme. The diffusion term uses the Central Difference Scheme.

The computational domain is the flow region of the fluid inside the water-cooled radiator, which is modelled by the k-ε standard turbulence model [26]:

$$\rho {\displaystyle \frac{\partial K}{\partial t}}+\rho {\displaystyle \frac{\left(K{u}_i\right)}{\partial X_i}}={\displaystyle \frac{\partial \left[\left(\mu +\frac{{\mu }_i}{{\sigma }_k}\right)\frac{\partial K}{\partial X_j}\right]}{\partial X_j}}+{\displaystyle \frac{C_{1\epsilon }}{K}}{G}_K-C_{2\epsilon }\rho {\displaystyle \frac{{\epsilon }^2}{K}}$$

(6)

In Equation (6), $K$ is the turbulent kinetic energy and ε is the turbulent dissipation rate, where $C_{1\mathsf{\epsilon }}=1.44$, $C_{2\mathsf{\epsilon }}=1.44$.

The solver uses the SIMPLE algorithm and the hybrid initialization method. When the convergence in X, Y, and Z directions in the residual graph is less than 10⁻³ and the energy convergence is less than 10⁻⁶, the calculation convergence is determined, and the iteration process is terminated, and the other parameters remain the default settings.

2.3. Boundary Conditions

Our previous thesis has simulated the optimal inlet flow rate of the radiator is 1.75 m/s, so the inlet flow rate in this work is selected to be 1.75 m/s. Usually, the magnitude of the Reynolds number Re is calculated to judge the flow state of the fluid inside the liquid-cooled radiator, and the Reynolds number inside the liquid-cooled radiator can be described by Equation (7) [27]:

$$Re={\displaystyle \frac{v_b\ast D\ast \rho }{\mu }}$$

(7)

In the above Equation (7), $v_b$ is the velocity of the fluid, D is the diameter of the flow channel, $\rho$ is the density of the fluid, and $\mu$ is the kinetic viscosity of the fluid. $v_b$, D, $\rho$, and $\mu$ can be chosen as 1.75 m/s, 10 mm, 998.2 kg/m³, and 0.001 kg/(M·s), respectively. The Reynolds number is calculated by Equation (7) to be equal to 17,468.5. For the flow of the liquid-cooled radiator with its circular tube, it is generally considered that the Reynolds number < 2000 is laminar and the Reynolds number > 2000 is turbulent, so the model chosen in this work is a turbulent model. Simulation is carried out using Ansys Fluent and the simulation parameters are set as shown in

Table 1. Simulation parameter settings.

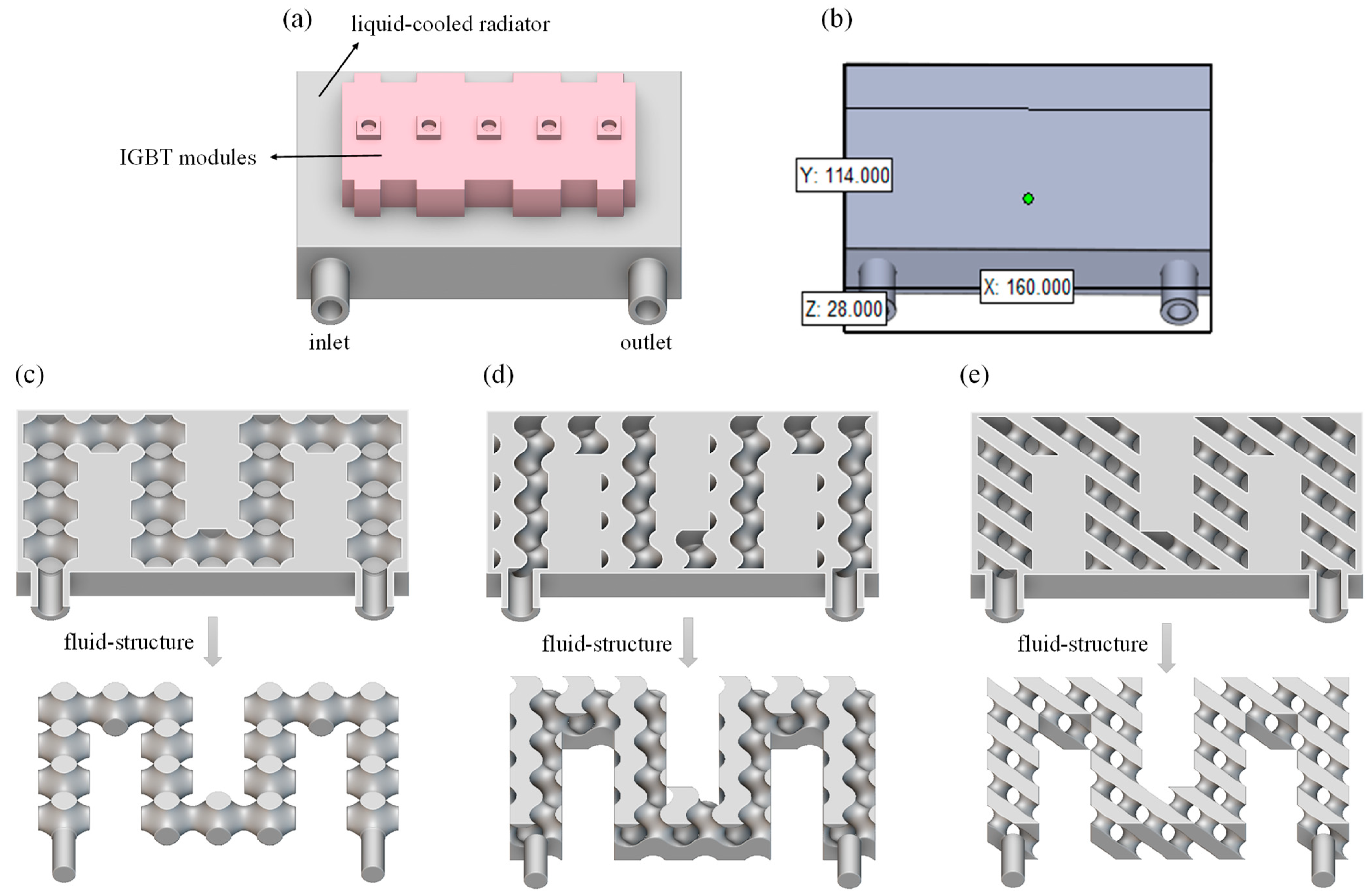
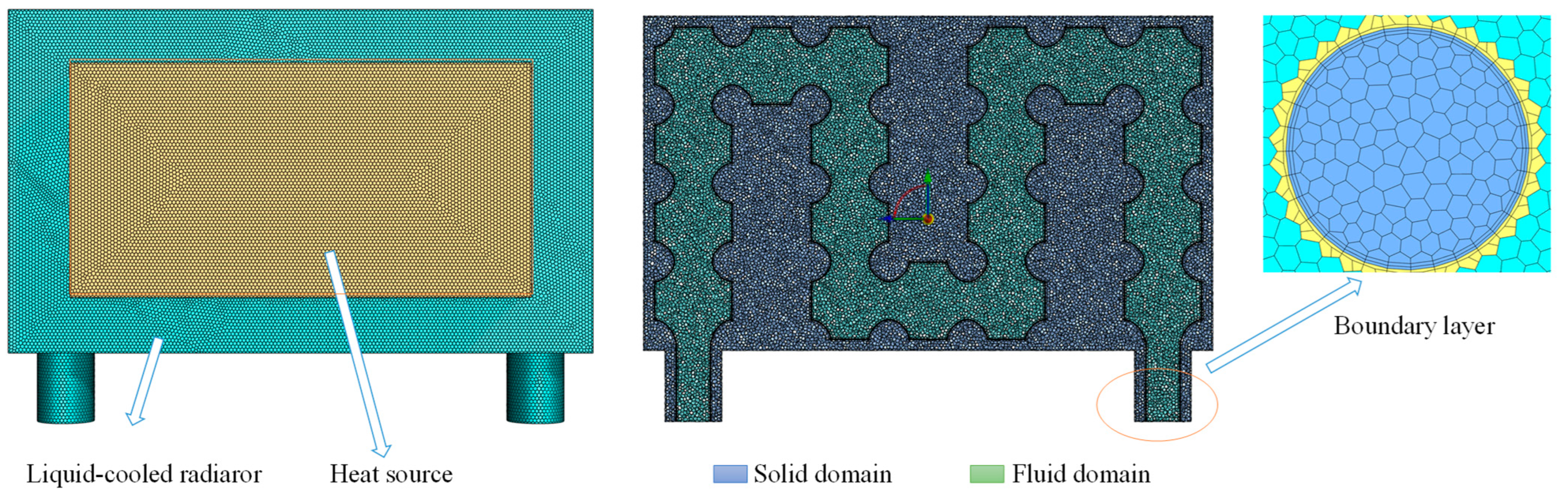
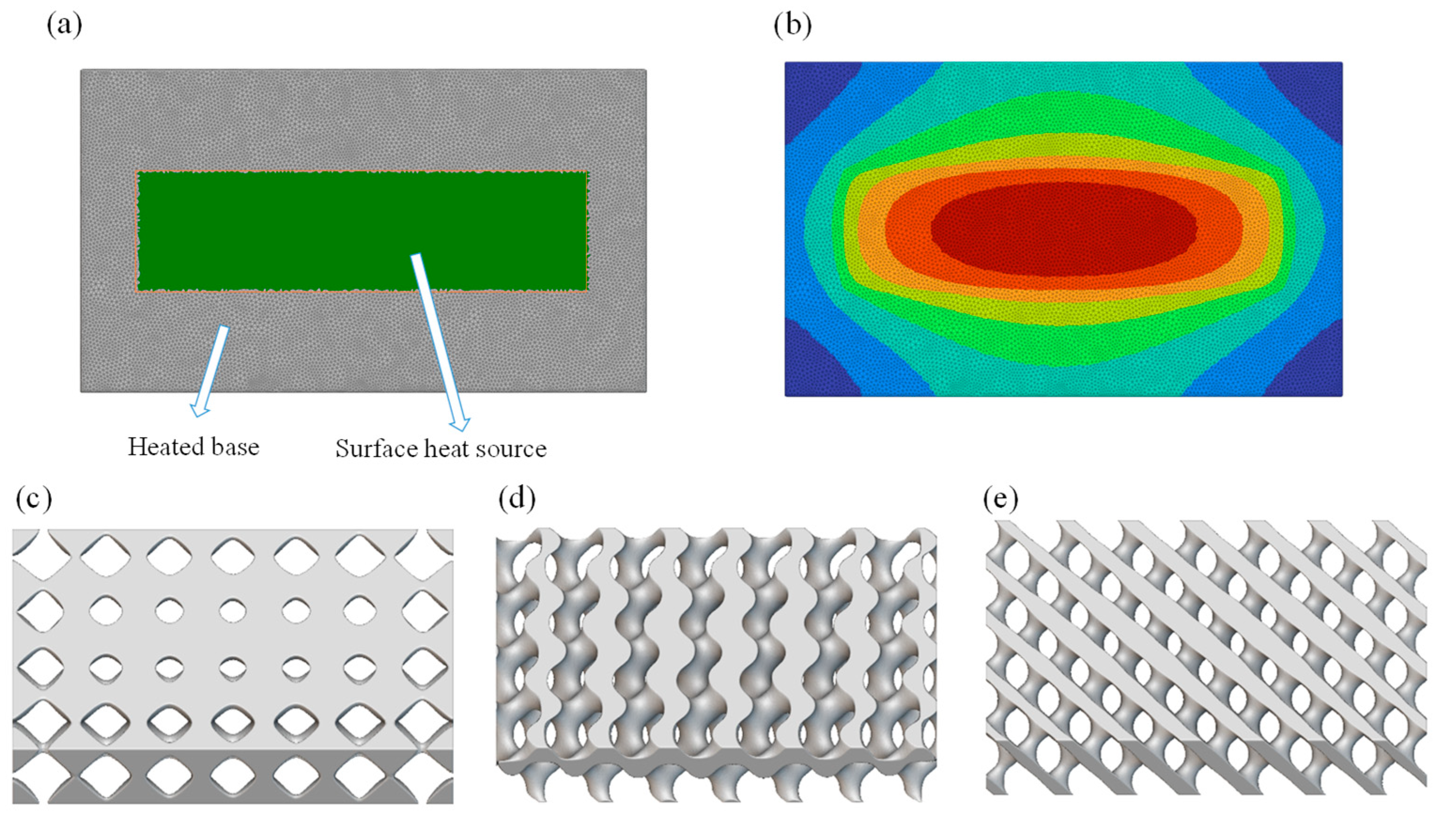
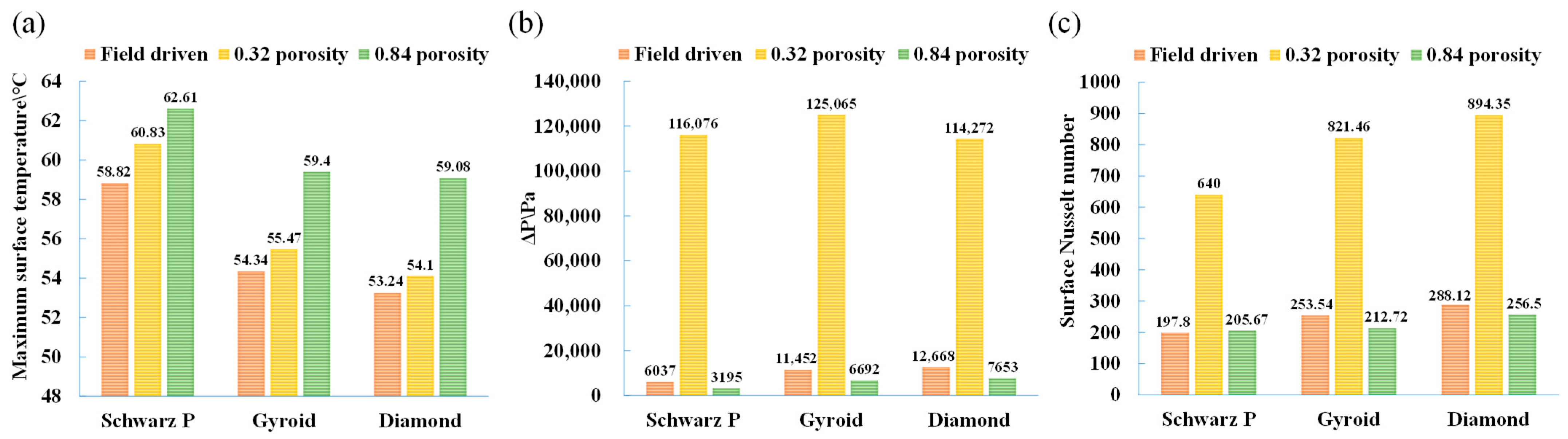
Parameter Type	Setting
Heat source power	2500 W
Radiator Material	Aluminum alloy
Coolant	Water
Inlet temperature	25 °C
Entrance speed	1.75 m/s
Turbulence model	k-epsilon
Contact surface setting	Coupled
Wall conditions	No slip
Solution method	SIMPLE
Initialization methods	Hybrid initialization

.

2.4. Model Convergence Test

When the increase in the number of meshes does not have a significant impact on the fluid simulation results, it indicates that the mesh is reliable, and obtaining appropriate mesh results can reduce computational complexity. For example, the mesh of a liquid-cooled radiator with a Schwarz P structure of 0.5 porosity is obtained by monitoring the pressure difference between the radiator inlet and outlet and the overall maximum temperature under the same boundary conditions. Five models with different meshes were simulated and studied, in which a hexahedral mesh was used in the solid domain and a hexahedral mesh with a boundary layer was used in the fluid domain. The mesh division is shown in Figure 2. As shown in

Table 2. Simulation results of mesh independence.

Mesh	Elements	${\mathit{P}}_{\mathit{i}\mathit{n}}$ [Pa]	$|\left({\mathit{P}}_{\mathit{i}\mathit{n}}^{\mathit{m}}-{\mathit{P}}_{\mathit{i}\mathit{n}}^{\mathit{m}+1}\right)/{\mathit{P}}_{\mathit{i}\mathit{n}}^{\mathit{m}}|$	${\mathit{T}}_{\mathit{m}\mathit{a}\mathit{x}}$ [°C]	$|\left({\mathit{T}}_{\mathit{m}\mathit{a}\mathit{x}}^{\mathit{m}}-{\mathit{T}}_{\mathit{m}\mathit{a}\mathit{x}}^{\mathit{m}+1}\right)/{\mathit{T}}_{\mathit{m}\mathit{a}\mathit{x}}^{\mathit{m}}|$
Mesh1	267,291	4211.22	----	76.11	----
Mesh2	557,938	4226.52	0.36%	76.03	0.09%
Mesh3	623,678	4236.92	0.25%	76.02	0.01%
Mesh4	736,501	4250.53	0.32%	75.98	0.05%
Mesh5	1,023,642	4246.67	0.09%	75.85	0.17%

, the results indicate that when the mesh model is Mesh1, Mesh2, and Mesh3, the obtained inlet and outlet pressure differences are small due to the small number of mesh cells. However, when the mesh is Mesh4, the inlet pressure variation and maximum temperature variation of the radiator are below 1%. This indicates that the simulation results converge when the number of meshes reaches 736,501. In this work, the same convergency analysis is also carried out for the other three models to ensure the reliability of the simulation data.

3. Results

3.1. Analysis of the Thermal Performance of Channels with Different Porosities

The simulation results of liquid-cooled heat sinks with three TPMS channel structures in the range of 0.32–0.84 porosity are shown in Figure 3.

Figure 3a illustrates the trend of the maximum temperature at the surface of the heat sink as a function of the porosity. The results show that the maximum temperatures of Schwarz P, Gyroid, and Diamond structures increase as the porosity increases. This may be attributed to the fact that the structures with large porosity have relatively small heat transfer areas and large pores, which are not favorable for cooling and heat dissipation. In addition, the maximum temperature of 0.32 porosity in the Diamond structure was 8.4% lower than that of the maximum porosity (0.84), and the difference for the Gyroid was 6.6% ostructure and for the Schwarz P structure was 2.8%. It can be seen that the cooling effect of the Diamond structure is the best among the three structures. However, after that, the maximum surface temperature tends to converge with further increasing porosity.

To assess the performance of convective heat transfer, the Nusselt number (Nu) was used. It is a dimensionless measure of convective heat transfer capacity defined as the ratio of the local convective heat transfer coefficient to the thermal conductivity of the fluid, i.e.,

$$Nu={\displaystyle \frac{hL}{k}}$$

(8)

where L is the characteristic length, k is the thermal conductivity of the fluid, and h is the local convective heat transfer coefficient. A higher Nusselt number indicates a higher convective heat transfer efficiency and better performance of the heat sink.

Figure 3b shows the Nusselt number of the heat sink changes with increasing porosity. It can be seen that the Nusselt number of the three structures shows a decreasing trend as the porosity increases. As the porosity increases, the total surface area of the solid surface inside the heat sink decreases, which reduces the contact area for heat transfer from the solid surface to the fluid, thus decreasing the convective heat transfer coefficient. In addition, the Nusselt number exhibited significant improvements for the minimum porosity (0.32) compared to the maximum porosity (0.84), with increases of 211.2% for the Schwarz P structure, 286.2% for the Gyroid structure, and 248.7% for the Diamond structure.

As discussed above, the structure with smaller porosity is advantageous for enhancing heat dissipation in the heat sink. Moreover, the small porosity also plays an important role in distributing the temperature evenly and reducing the hot spots in the heat sink, thus improving the efficiency of the heat sink.

Figure 3c shows the trend of the inlet pressure of the radiator with porosity. The inlet and outlet pressure differences can be defined as

$$\mathsf{\Delta }P=P_{\mathrm{o}\mathrm{u}\mathrm{t}}-P_{\mathrm{i}\mathrm{n}}$$

(9)

For Schwarz P, Gyroid, and Diamond structures, the inlet and outlet pressure drop decreases significantly with increasing porosity, implying that the larger the porosity, the lower the pressure loss. In addition, the minimum porosity of the Schwarz P structure (0.32) increased the differential pressure at the entrance and exit by a factor of 35.3, the Gyroid structure by a factor of 17.7, and the Diamond structure by a factor of 13.9 over the maximum porosity (0.84). Therefore, in heat sink design, it is necessary to balance the entrance and exit pressure drop with the heat dissipation efficiency. The structural morphology plays a decisive role in balancing the heat transfer and pressure drop, which is consistent with the research of Cheng et al. [9]. In their study, the P-type TPMS structure exhibited the lowest specific pressure drop and the highest comprehensive heat transfer coefficient (j/f) under the same porosity (0.442) and flow conditions due to its simplest channel network and lowest node connection complexity (six pillars per node, and a pillar angle of 90°). This is consistent with the rule in this article that “small porosity structures (simple structural morphology) have a higher pressure drop but stronger heat dissipation”, further indicating that the geometric characteristics of TPMS structures (such as the pillar connection method, channel complexity, porosity) are key factors affecting the pressure drop heat transfer balance. Reducing the porosity increases the Nusselt number and decreases the maximum temperature, but at the same time, the inlet and outlet pressure differences are larger.

3.2. Field-Driven Design for Optimizing Flow Path

According to the above numerical analysis of the three TPMS structures with different porosities, it can be seen that the porosity has an intuitive impact on the heat dissipation performance of the heat sink. Therefore, this work proposes a field-driven design to optimize the flow path, using nTopology software to carry out thermal simulation analysis under the same loading conditions, transforming the analysis results into scalar fields, and then carrying out the field-driven generation of the TPMS flow path structure with variable porosity to obtain optimal fluid distribution and improve the performance of the heat sink.

Thermal simulations were conducted using nTopology software. A rectangular base with the same dimensions as the wall panel of the heat sink is first created, and a heat source surface is created on the upper surface. Material properties are then assigned to the model, the heat source is defined as a boundary condition with a specified surface heat flux, and convective boundary loads are set. Next, the model is meshed in order to discretize it into small elements for analysis. Finally, thermal simulations are run to analyze the temperature distribution and heat flow within the model under specific conditions. Figure 4a shows the rectangular base and surface heat sources set up in nTopology, and Figure 4b shows the temperature distribution of the thermal simulation. In this case, the red color represents the region with a higher temperature, and the blue color represents the region with a lower temperature.

Based on the distribution of the temperature field, hot spots and areas of higher cooling demand in the heat sink can be identified. nTopology uses this information to guide the adjustment of the porosity. The porosity of the channel is adjusted according to the changes in the temperature field, decreasing the porosity in places with higher temperatures and increasing the porosity in places with lower temperatures. In this work, the design optimizes the channel structure to generate three kinds of TPMS structure channels with varying porosity from 0.32 to 0.84 according to the temperature field distribution, and the size of the unit cell is still 22 mm × 22 mm × 22 mm. Figure 4c–e shows the three kinds of field-driven designs of the structure with variable porosities.

After design optimization, numerical simulation is required to verify the performance of the optimized design. Using the same boundary conditions and settings, finite element simulations of the improved channel are performed to evaluate its heat dissipation effect under the same operating conditions.

3.3. Thermal Performance Analysis of the Optimized Channels

The overall temperature distributions of the liquid-cooled heat sink with the optimized three TPMS channel structures are shown in Figure 5a–c. From the figure, it can be seen that the temperature around the liquid-cooled heat sink is low, and the highest temperature is concentrated at the junction with the IGBT module. The maximum surface temperature is 58.82 °C for the Schwarz P channel structure, 54.34 °C for the Gyroid channel structure, and 53.24 °C for the Diamond channel structure. The maximum surface temperatures for the minimum porosity (0.32) of the Schwarz P structure, Gyroid structure, and Diamond structure are 60.83, 55.47, and 54.1, respectively. It can be seen that field-driven design has a significant effect on reducing the minimum surface temperature. A comparison of the three images shows that the heat dissipation of the Gyroid and Diamond channel structures is better than that of the Schwarz P structure, which is consistent with the conclusion of uniform porosity above. Figure 6 shows the results of the simulation of the three structural field-driven designs of heat sinks, Schwarz P, Gyroid, and Diamond, compared with the simulation of the heat sinks with 0.32 porosity and 0.84 porosity. Among them, Figure 6a shows the comparison of the maximum surface temperature. The field-driven design reduced the maximum surface temperature in all three structures. The Schwarz P structure, Gyroid structure, and Diamond structure are 3.3%, 2.0%, and 1.6% lower than the maximum temperatures for their respective minimum porosities (0.32). This indicates that the field-driven design is able to optimize the flow paths according to the spatial distribution of heat loads, resulting in more effective temperature reduction and better cooling. As stated in the literature [13], proper porosity is the most effective way to control the heat transfer performance of the TPMS, and this study further confirms that the heat dissipation performance can be significantly improved by optimizing the porosity distribution.

The overall pressure distribution of the internal water path of the optimized three TPMS structures is shown in Figure 5d–f. The pressure is maximum at the inlet of the liquid-cooled radiator and gradually decreases along the outlet direction, forming a pressure gradient and generating fluid-flow dynamics. The maximum pressure of the optimized Schwarz P water circuit structure is 6551.62 Pa, the maximum pressure of the optimized Gyroid water circuit structure is 1.9 times that of the optimized Schwarz P water circuit structure, and the maximum pressure of the optimized Diamond water circuit structure is 2.1 times that of the optimized Schwarz P water circuit structure. The results imply that the maximum differential pressure between the entrance and exit is in the Diamond structure, and the minimum differential pressure at the entrance and exit is in the Schwarz P structure. Figure 6b compares the inlet and outlet pressure differences of the radiator flow paths for different TPMS structures with field-driven design and uniform porosity design. Based on the graphical information combined with the uniform porosity analysis above, it can be seen that the smaller the porosity, the larger the inlet and outlet pressure difference. The differential pressure for the field-driven designs of the three structures—Schwarz P, Gyroid, and Diamond—is reduced compared to their respective 0.32 porosities by 94.8%, 90.8%, and 88.9%. As described in the literature [19], material distribution based on stress distribution can improve structural efficiency and reduce material usage. Similarly, the field-driven design in this work reduces unwanted pressure losses by optimizing the porosity distribution.

Figure 6c shows the surface Nusselt number to compare convective heat transfer efficiency under different TPMS structures. The surface Nusselt number of three structures at 0.32 porosity is larger than that of 0.84 porosity, indicating that the smaller the porosity, the better the convective heat transfer efficiency of the heat sink. The differences in the internal design of the three TPMS structures lead to differences in the convective heat transfer performance of the heat sinks with field-driven designs. The Nusselt number of the field-driven designs of the Gyroid and Diamond structures increased by 19.2% and 12.3% over the 0.84 porosity, and the field-driven design of the Schwarz P structure had the smallest Nusselt number, which was reduced by 3.8% compared to the 0.84 porosity. It indicates that the field-driven design has an optimizing effect on the convective heat transfer efficiency of the Gyroid and Diamond structures.

Based on the comparative results in Figure 6a,c, it can be seen that the field-driven design is also able to significantly reduce the maximum surface temperature and improve the convective heat transfer efficiency in the liquid-cooled system. Both different cooling environments demonstrate the advantages of the field-driven design in enhancing the cooling performance. The optimized plate-fin heat sink in [22] reduces the thermal resistance by 15% to 25% compared to the original structure, which is consistent with the trend of increasing the Nusselt number by 19.2% and 12.3% after optimizing the Gyro and Diamond structures in this paper. Both reflect the improvement effect of field-driven design on heat transfer efficiency. In addition, the authors of [22] emphasize the advantages of field-driven design in reducing material consumption and weight. Their method of adjusting fin thickness through linear interpolation and the strategy of allocating porosity based on the temperature field in this paper both demonstrate the “on-demand optimization” structural design—the former reduces ineffective materials through thickness gradient, while the latter reduces inlet and outlet pressure difference through porosity gradient.

4. Conclusions

In this study, we first investigated the heat dissipation performance of uniformly porous heat sinks with three different TPMS structures. The results showed that as the porosity increased, the maximum temperature on the surface of the heat sink increased and the Nusselt number decreased. Field-driven design adjusts the porosity based on the temperature field and optimizes the internal flow channel structure of the radiator.

Compared with the 0.32 uniform porosity, the optimized structures of Schwarz P, Gyroid, and Diamond reduced the maximum temperature by 3.3%, 2.0%, and 1.6%, respectively, and the inlet/outlet differential pressure by 94.8%, 90.8%, and 88.9%, respectively. Compared with the 0.84 porosity, the Nusselt number of the field-driven Schwarz P structure decreased by 3.8%, while those of Gyroid and Diamond increased by 19.2% and 12.3%, respectively. This research optimizes the heat sink’s internal flow channel via field-driven design, improving heat dissipation efficiency and reducing pressure loss, thus offering an effective solution for IGBT module heat dissipation.

Notably, this study acknowledges several limitations from the perspectives of design and manufacturing.

Design Limitations: The current optimization relies exclusively on steady-state thermal-flow simulations, overlooking dynamic operational conditions of IGBT modules, such as transient thermal loads, flow fluctuations, and temperature oscillations. These dynamic factors could significantly affect the actual heat dissipation performance and pressure stability, requiring further investigation under time-varying scenarios.

Manufacturing and Practical Constraints: The design focuses exclusively on optimizing thermal flow performance without considering manufacturing constraints (e.g., additive manufacturing precision, material limitations, geometric complexity) or conducting a cost-effectiveness analysis. Industrial applicability requires a balance among heat transfer efficiency, pressure loss, manufacturability, and economic viability, which remains unaddressed in this work.

Lack of Experimental Validation: The research lacks physical prototypes and experimental testing of the optimized TPMS structures. While numerical simulations demonstrate promising results, real-world validation through fabrication and testing is critical to verify simulation accuracy, material performance, and structural integrity under practical cooling conditions.

Future work will prioritize addressing these limitations, including dynamic performance analysis via transient CFD simulations, multi-objective optimization that incorporates manufacturing and cost factors, and experimental validation to bridge the gap between simulation and practical application.