Multiphysics-Driven Structural Optimization of Flat-Tube Solid Oxide Electrolysis Cells to Enhance Hydrogen Production Efficiency and Thermal Stress Resistance
Abstract
1. Introduction
2. Model Description
2.1. Geometry
2.2. Governing Equations
2.2.1. Electrochemical Reaction Models
2.2.2. Gas Flow Model
2.2.3. Mass Transfer Model
2.2.4. Heat Transfer Model
2.2.5. Electrode Mesoscopic Structure and Mechanical Model
2.3. Boundary
| Distribution | Current Transport | Mass Transfer | Momentum Transport | Heat Transfer | Thermal Stress |
|---|---|---|---|---|---|
| Fuel inlet | / | Mole fraction | Mass flow rate | Temperature | Free |
| Fuel outlet | / | Convection | Pressure | Heat convection | Free |
| Air inlet | / | Mole fraction | Mass flow rate | Temperature | Free |
| Air outlet | / | Convection | Pressure | Heat convection | Free |
| Fuel interconnector face | 0 V | / | / | Heat isolation | Roller |
| Air interconnector face | Operating voltage | / | / | Heat isolation | Roller |
| Parameters | Porosity | Permeability (m2) | Thermal Conductivity ) | Thermal Capacity ) |
|---|---|---|---|---|
| Fuel active layer | 0.23 | 1 × 10−12 | 6 | 450 |
| Fuel support layer | 0.46 | 1 × 10−12 | 6 | 450 |
| Electrolyte | / | / | 2.7 | 550 |
| Air active layer | 0.3 | 1 × 10−12 | 11 | 430 |
| Interconnector | / | / | 20 | 550 |
2.4. Model Setup
- (1)
- The thickness of the fuel support layer is increased from 5.0 mm to 5.5 mm at an interval of 0.1 mm.
- (2)
- The air rib area portion is increased from 17% to 50%.
- (3)
- The ratio of Ni is increased from 20% to 60%.
3. Results and Discussion
3.1. Model Validation
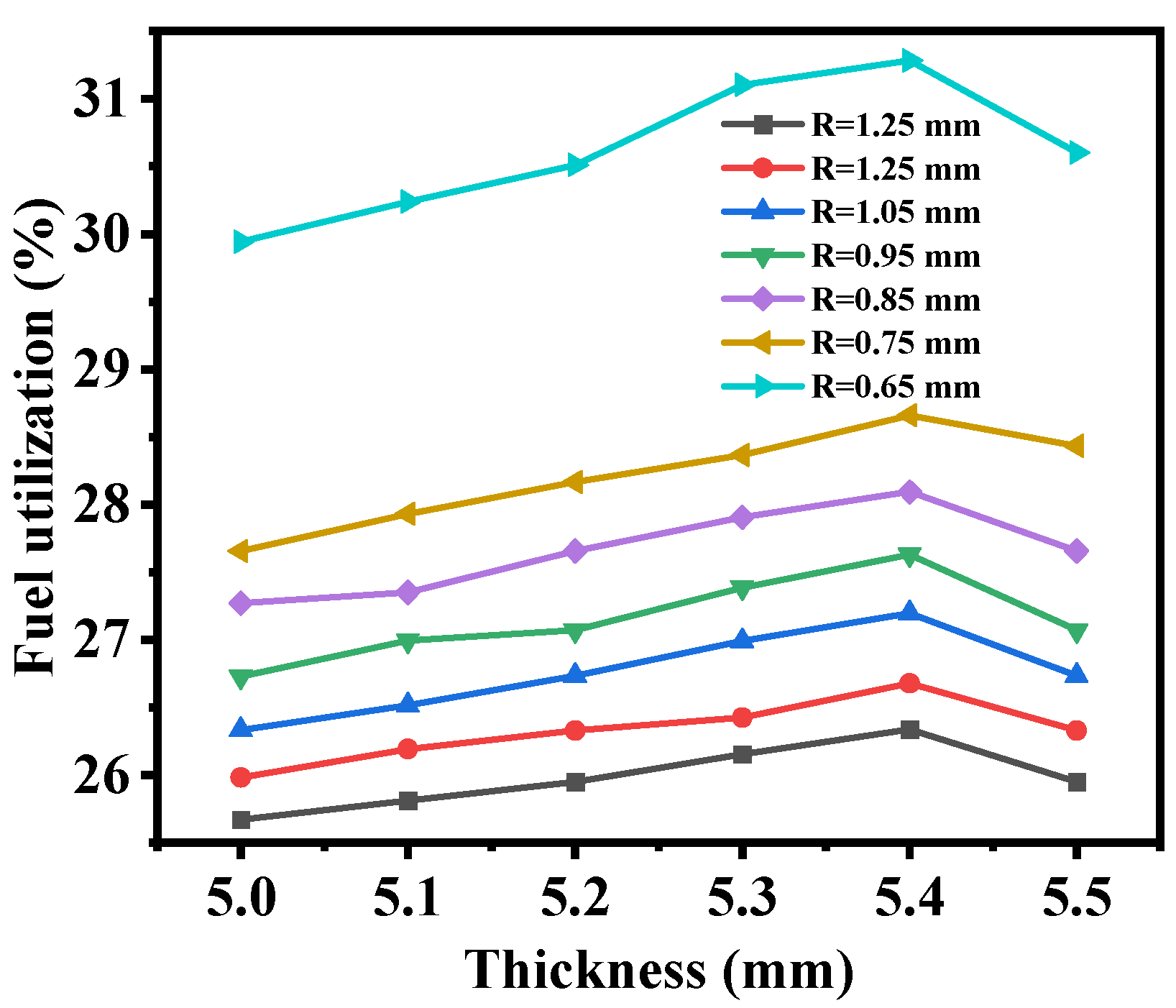
3.2. Macrostructural Thickness of Fuel Support Layer
3.3. Macrostructure Air Electrode Rib Area
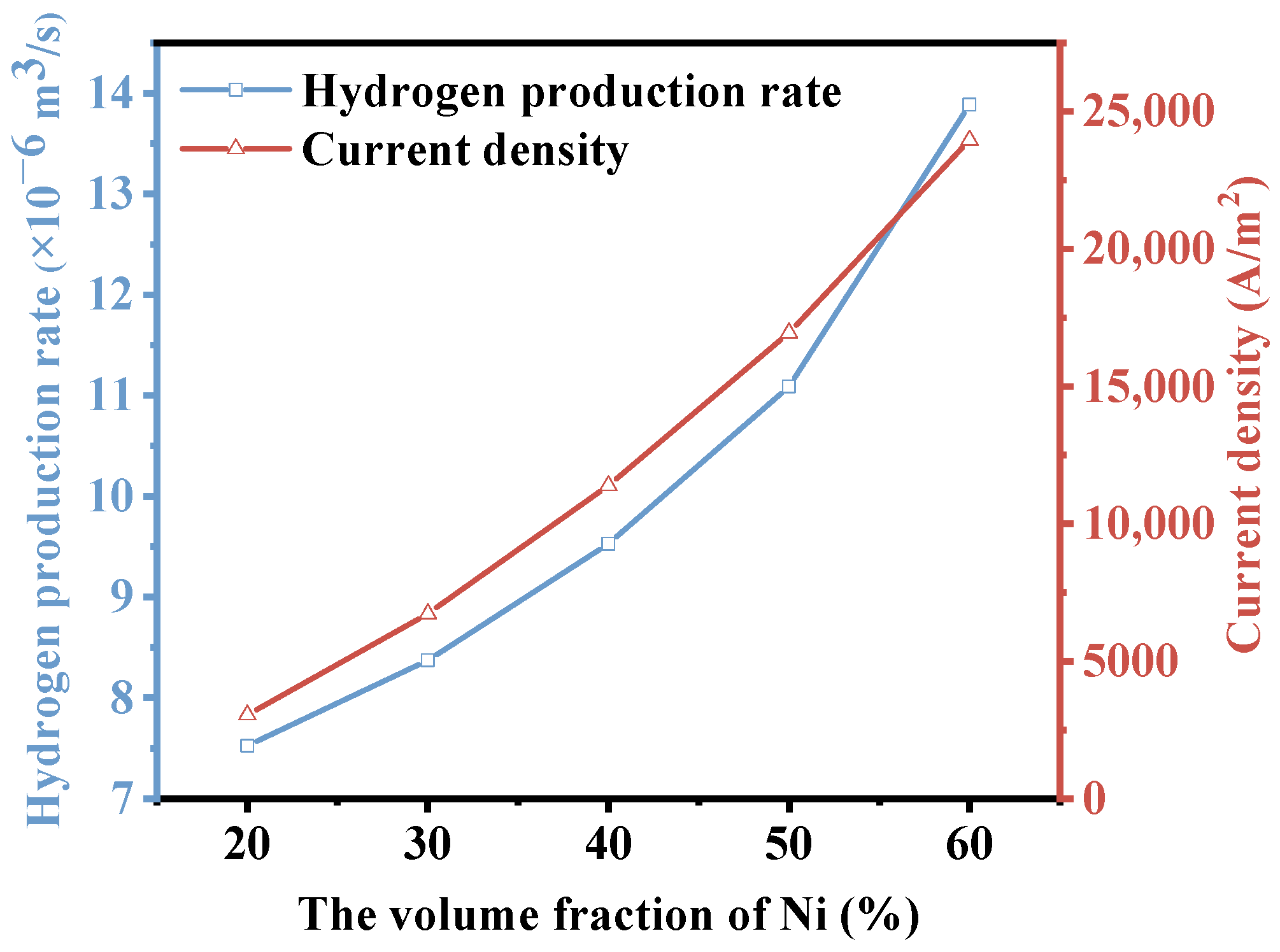
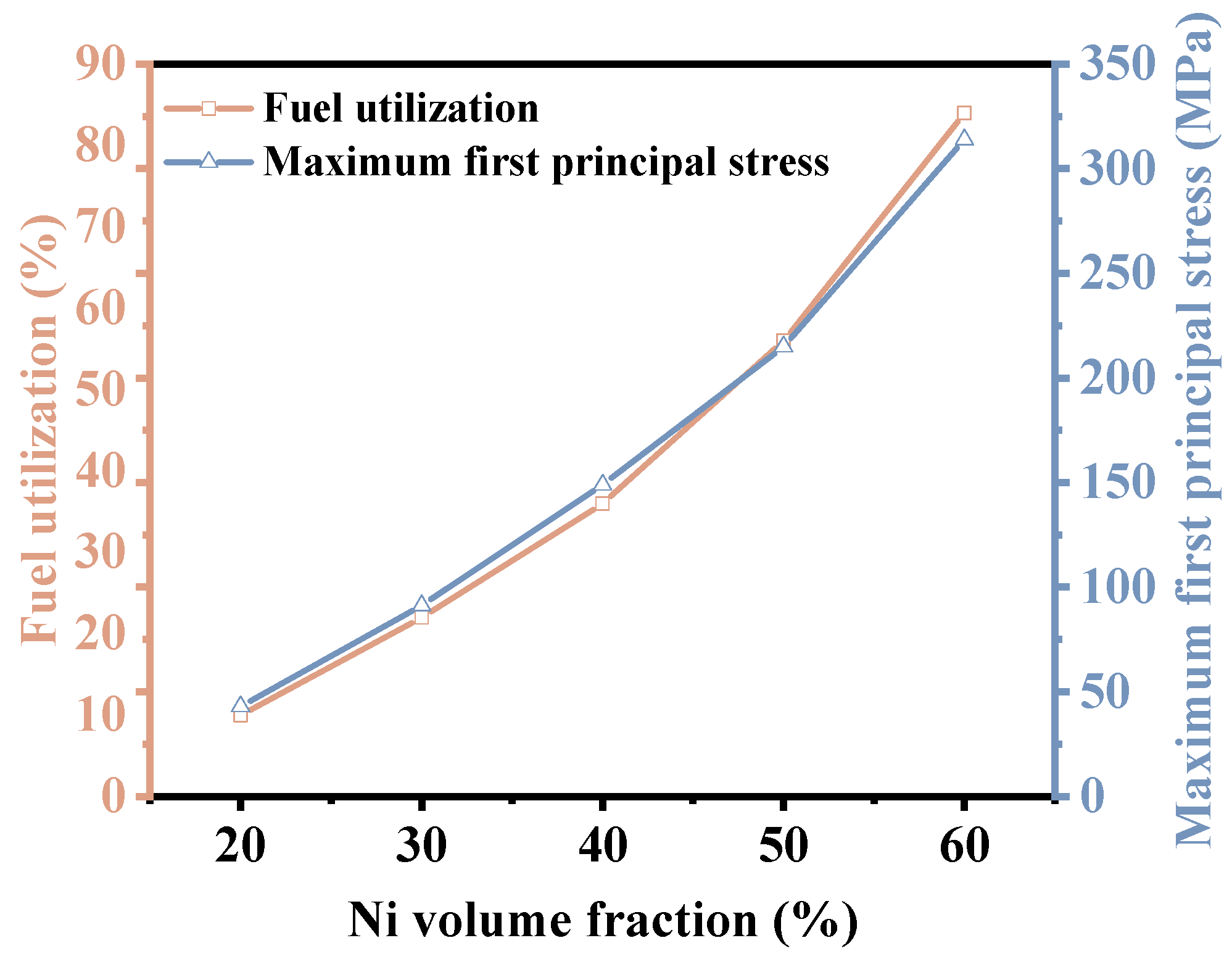
3.4. Microstructure-Ni-YSZ Ratio
4. Conclusions
- (1)
- As the thickness of the fuel support layer increases, the hydrogen production rate and diffusion flux first increase and then decrease, reaching the optimum when the thickness of the fuel electrode support layer is 5.4 mm. Additionally, as the thickness of the fuel support layer increases, the maximum first principal stress gradually decreases.
- (2)
- Although the oxygen generation and current density increase with the increase in the rib area portion, the distribution of oxygen concentration is more uniform, and the thermal stress is lower when the rib area portion is 42%. Therefore, the performance of the SOEC is optimal when the rib area is 42%.
- (3)
- When the Ni volume fraction increases from 20% to 60%, both the hydrogen production rate and thermal stress increase, and the hydrogen production rate increases by 86%. The flow uniformity of H2O gradually decreases. Notably, when the Ni volume fraction is below 50%, the decline in flow uniformity is more significant. After a comprehensive analysis, it can be concluded that the SOEC exhibits a better performance when the Ni volume fraction is 50%.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guo, Y.; Zhang, H. A hybrid system using a looped multi-stage thermoacoustically-driven cryocooler to harvest the waste heat from a direct carbon solid oxide fuel cell. Int. J. Heat Mass Transf. 2021, 169, 120972. [Google Scholar] [CrossRef]
- Peng, H.; Di, Z.; Gong, P.; Yang, F.; Cheng, F. Techno-economic assessment of a chemical looping splitting system for H2 and CO Co-generation. Green Energy Environ. 2023, 8, 338–350. [Google Scholar] [CrossRef]
- Williams, M.; Song, X.; Gemmen, R. Research Needs for the Solid Oxide Electrolyzer (SOEC) with a Proton-Conducting SOEC (H-SOEC) Electrochemical Hydrogen Compressor (EHC) Energy Conversion Network (ECN). ECS Trans. 2021, 103, 451. [Google Scholar] [CrossRef]
- Ma, S.; Xue, D.; Li, Q.; Zheng, J.; Feng, C.; Li, G. Stress Analysis of Solid Oxide Fuel Cell Electrodes Using Functional Gradient Materials. J. Electrochem. Soc. 2023, 170, 034502. [Google Scholar] [CrossRef]
- Xu, X.; Zhou, Q.; Yu, D. The future of hydrogen energy: Bio-hydrogen production technology. Int. J. Hydrogen Energy 2022, 47, 33677–33698. [Google Scholar] [CrossRef]
- Kang, S.; Pan, Z.; Guo, J.; Zhou, Y.; Wang, J.; Fan, L.; Zheng, C.; Cha, S.W.; Zhong, Z. Scientometric analysis of research trends on solid oxide electrolysis cells for green hydrogen and syngas production. Front. Energy 2024, 18, 583–611. [Google Scholar] [CrossRef]
- Laguna-Bercero, M.A. Recent advances in high temperature electrolysis using solid oxide fuel cells: A review. J. Power Sources 2012, 203, 4–16. [Google Scholar] [CrossRef]
- Lahrichi, A.; El Issmaeli, Y.; Kalanur, S.S.; Pollet, B.G. Advancements, strategies, and prospects of solid oxide electrolysis cells (SOECs): Towards enhanced performance and large-scale sustainable hydrogen production. J. Energy Chem. 2024, 94, 688–715. [Google Scholar] [CrossRef]
- Lee, S.-J.; Jung, C.-Y.; Yi, S.-C. Computational analysis on the electrode geometric parameters for the reversible solid oxide cells. Electrochim. Acta 2017, 242, 86–99. [Google Scholar] [CrossRef]
- Li, W.; Shi, Y.; Luo, Y.; Cai, N. Theoretical modeling of air electrode operating in SOFC mode and SOEC mode: The effects of microstructure and thickness. Int. J. Hydrogen Energy 2014, 39, 13738–13750. [Google Scholar] [CrossRef]
- Mahmood, A.; Bano, S.; Yu, J.H.; Lee, K.-H. Performance evaluation of SOEC for CO2/H2O co-electrolysis: Considering the effect of cathode thickness. J. CO2 Util. 2019, 33, 114–120. [Google Scholar] [CrossRef]
- Zhang, X.; Li, A.; Fei, Y.; Sun, M.; Zhu, L.; Huang, Z. Design of biomimetic leaf-like flow fields using three-dimensional numerical simulation for co-electrolysis in solid oxide electrolysis cell. Int. J. Hydrogen Energy 2024, 72, 326–337. [Google Scholar] [CrossRef]
- Tu, Y.; Lin, H.; Chen, M.; Zhang, Z.; Cai, W.; Zhu, Z. Design and numerical analysis of a Y-shaped flow channel for enhanced hydrogen production in solid oxide electrolysis cells. Int. J. Electrochem. Sci. 2024, 19, 100806. [Google Scholar] [CrossRef]
- Pan, H.; Wu, A.; Au, S.F.; Yang, Y.; Song, Z.; Liu, Z.; Gong, X.; Guan, W. Effect of the steam/hydrogen ratio on the performance of flat-tube solid oxide electrolysis cells for seawater. Sustain. Energy Fuels 2023, 7, 3333–3341. [Google Scholar] [CrossRef]
- Lin, Z.; Stevenson, J.W.; Khaleel, M.A. The effect of interconnect rib size on the fuel cell concentration polarization in planar SOFCs. J. Power Sources 2003, 117, 92–97. [Google Scholar] [CrossRef]
- Keane, M.; Fan, H.; Han, M.; Singh, P. Role of initial microstructure on nickel-YSZ cathode degradation in solid oxide electrolysis cells. Int. J. Hydrogen Energy 2014, 39, 18718–18726. [Google Scholar] [CrossRef]
- Janardhanan, V.M.; Monder, D.S. Microkinetic modeling of CO2 reduction on Pt in a solid oxide electrolysis cell. Electrochim. Acta 2022, 410, 139742. [Google Scholar] [CrossRef]
- Nerat, M.; Juričić, Đ. Modelling of anode delamination in solid oxide electrolysis cell and analysis of its effects on electrochemical performance. Int. J. Hydrogen Energy 2018, 43, 8179–8189. [Google Scholar] [CrossRef]
- Ren, B.; Wen, G.; Ricardez–Sandoval, L.; Croiset, E. New mechanistic insights into CO2 reduction in solid oxide electrolysis cell through a multi-scale modelling approach. J. Power Sources 2021, 490, 229488. [Google Scholar] [CrossRef]
- Luo, Y.; Shi, Y.; Li, W.; Cai, N. Elementary reaction modeling of reversible CO/CO2 electrochemical conversion on patterned nickel electrodes. J. Power Sources 2018, 379, 298–308. [Google Scholar] [CrossRef]
- Kamkeng, A.D.N.; Wang, M. Long-term performance prediction of solid oxide electrolysis cell (SOEC) for CO2/H2O co-electrolysis considering structural degradation through modelling and simulation. Chem. Eng. J. 2022, 429, 132158. [Google Scholar] [CrossRef]
- Chatzichristodoulou, C.; Chen, M.; Hendriksen, P.V.; Jacobsen, T.; Mogensen, M.B. Understanding degradation of solid oxide electrolysis cells through modeling of electrochemical potential profiles. Electrochim. Acta 2016, 189, 265–282. [Google Scholar] [CrossRef]
- Wang, Y.; Wu, C.; Zu, B.; Han, M.; Du, Q.; Ni, M.; Jiao, K. Ni migration of Ni-YSZ electrode in solid oxide electrolysis cell: An integrated model study. J. Power Sources 2021, 516, 230660. [Google Scholar] [CrossRef]
- Luo, X.; Wu, A.; Sang, J.; Huang, N.; Han, B.; Wang, C.; Gao, Y.; Guan, W.; Singhal, S.C. The properties of the fuel electrode of solid oxide cells under simulated seawater electrolysis. Int. J. Hydrogen Energy 2023, 48, 10359–10367. [Google Scholar] [CrossRef]
- Liu, Z.; Hu, J.; Wu, A.; Lu, Z.; Guan, W. Stability and energy consumption of solid oxide electrolysis cells under wide fluctuating and stable conditions. J. Power Sources 2024, 616, 235113. [Google Scholar] [CrossRef]
- Xu, Y.; Zhang, J.; Tu, Z. Numerical simulation of flow channel geometries optimization for the planar solid oxide electrolysis cell. Int. J. Hydrogen Energy 2024, 52, 288–301. [Google Scholar] [CrossRef]
- Tu, Y.; Chai, J.; Li, S.; Han, F.; Zhang, Z.; Cai, W. The Study of Multiphysics Field Coupling and Thermal Stress in Three Types of Solid Oxide Electrolysis Cells (SOEC). Int. J. Electrochem. Sci. 2024, 19, 100789. [Google Scholar] [CrossRef]
- Kim, Y.; Lim, K.; Salihi, H.; Heo, S.; Ju, H. The Effects of Stack Configurations on the Thermal Management Capabilities of Solid Oxide Electrolysis Cells. Energies 2023, 17, 125. [Google Scholar] [CrossRef]
- Liu, Z.; Han, B.; Lu, Z.; Guan, W.; Li, Y.; Song, C.; Chen, L.; Singhal, S.C. Efficiency and stability of hydrogen production from seawater using solid oxide electrolysis cells. Appl. Energy 2021, 300, 117439. [Google Scholar] [CrossRef]
- Sun, Y.; Hu, X.; Gao, J.; Han, Y.; Sun, A.; Zheng, N.; Shuai, W.; Xiao, G.; Guo, M.; Ni, M.; et al. Solid oxide electrolysis cell under real fluctuating power supply with a focus on thermal stress analysis. Energy 2022, 261, 125096. [Google Scholar] [CrossRef]
- Li, G.; Liao, Y.; Cheng, J.; Huo, H.; Xu, J. Coupled Electrochemical-Thermo-Stress Analysis for Methanol-Fueled Solid Oxide Fuel Cells. J. Electrochem. Soc. 2024, 171, 054502. [Google Scholar] [CrossRef]
- Yang, G.; Li, Y.; Sang, J.; Wu, A.; Yang, J.; Liang, T.; Xu, J.; Guan, W.; Chai, M.; Singhal, S.C. In-situ analysis of anode atmosphere in a flat-tube solid oxide fuel cell operated with dry reforming of methane. J. Power Sources 2022, 533, 231246. [Google Scholar] [CrossRef]
- Jiang, C.; Gu, Y.; Guan, W.; Zheng, J.; Ni, M.; Zhong, Z. 3D thermo-electro-chemo-mechanical coupled modeling of solid oxide fuel cell with double-sided cathodes. Int. J. Hydrogen Energy 2020, 45, 904–915. [Google Scholar] [CrossRef]
- Zeng, S.; Xu, M.; Parbey, J.; Yu, G.; Andersson, M.; Li, Q.; Li, B.; Li, T. Thermal stress analysis of a planar anode-supported solid oxide fuel cell: Effects of anode porosity. Int. J. Hydrogen Energy 2017, 42, 20239–20248. [Google Scholar] [CrossRef]
- Ni, M.; Leung, M.K.H.; Leung, D.Y.C. Parametric study of solid oxide steam electrolyzer for hydrogen production. Int. J. Hydrogen Energy 2007, 32, 2305–2313. [Google Scholar] [CrossRef]
- Zhang, J.; Guo, H.; Lei, L.; Shen, S.; Zheng, K.; Han, M. A critical review of the Butler-Volmer equation for the activation polarization of solid oxide fuel cells. J. Power Sources 2024, 613, 234871. [Google Scholar] [CrossRef]
- Xu, H.; Chen, B.; Liu, J.; Ni, M. Modeling of direct carbon solid oxide fuel cell for CO and electricity cogeneration. Appl. Energy 2016, 178, 353–362. [Google Scholar] [CrossRef]
- Wang, H.; Xiao, L.; Liu, Y.; Zhang, X.; Zhou, R.; Liu, F.; Yuan, J. Performance and Thermal Stress Evaluation of Full-Scale SOEC Stack Using Multi-Physics Modeling Method. Energies 2023, 16, 7720. [Google Scholar] [CrossRef]
- Gong, C.; Xu, Y.; Cai, S.; Chi, B.; Tu, Z. Comparative study on thermodynamic analysis of solid oxide fuel cells supplied with methanol or ammonia. Int. J. Hydrogen Energy 2024, 50, 1293–1301. [Google Scholar] [CrossRef]
- Hussain, M.M.; Li, X.; Dincer, I. A numerical investigation of modeling an SOFC electrode as two finite layers. Int. J. Hydrogen Energy 2009, 34, 3134–3144. [Google Scholar] [CrossRef]
- Yuan, J.; Sundén, B. On mechanisms and models of multi-component gas diffusion in porous structures of fuel cell electrodes. Int. J. Heat Mass Transf. 2014, 69, 358–374. [Google Scholar] [CrossRef]
- Chan, S.H.; Khor, K.A.; Xia, Z.T. A complete polarization model of a solid oxide fuel cell and its sensitivity to the change of cell component thickness. J. Power Sources 2001, 93, 130–140. [Google Scholar] [CrossRef]
- Yakabe, H.; Hishinuma, M.; Uratani, M.; Matsuzaki, Y.; Yasuda, I. Evaluation and modeling of performance of anode-supported solid oxide fuel cell. J. Power Sources 2000, 86, 423–431. [Google Scholar] [CrossRef]
- Nemat-Alla, M. Reduction of thermal stresses by developing two-dimensional functionally graded materials. Int. J. Solids Struct. 2003, 40, 7339–7356. [Google Scholar] [CrossRef]
- Chan, S.; Xia, Z. Anode micro model of solid oxide fuel cell. J. Electrochem. Soc. 2001, 148, A388. [Google Scholar] [CrossRef]
- Molla, T.T.; Kwok, K.; Frandsen, H.L. Modeling the Mechanical Integrity of Generic Solid Oxide Cell Stack Designs Exposed to Long-term Operation. Fuel Cells 2018, 19, 96–109. [Google Scholar] [CrossRef]
- Zhu, P.; Wu, Z.; Yao, J.; Guo, L.; Yan, H.; Nyamsi, S.N.; Kurko, S.; Yang, F.; Zhang, Z. Multi-physics field modeling of biomass gasification syngas fueled solid oxide fuel cell. J. Power Sources 2021, 512, 230470. [Google Scholar] [CrossRef]
- Xu, Q.; Xia, L.; He, Q.; Guo, Z.; Ni, M. Thermo-electrochemical modelling of high temperature methanol-fuelled solid oxide fuel cells. Appl. Energy 2021, 291, 116832. [Google Scholar] [CrossRef]
- Liu, Y.; Xiao, L.; Wang, H.; Ou, D.; Yuan, J. Numerical Study of H2 Production and Thermal Stress for Solid Oxide Electrolysis Cells with Various Ribs/Channels. Energies 2024, 17, 510. [Google Scholar] [CrossRef]
- Liu, C.; Dang, Z.; Xi, G. Numerical study on thermal stress of solid oxide electrolyzer cell with various flow configurations. Appl. Energy 2024, 353, 122041. [Google Scholar] [CrossRef]
- Zhang, Z.; Yue, D.; Yang, G.; Chen, J.; Zheng, Y.; Miao, H.; Wang, W.; Yuan, J.; Huang, N. Three-dimensional CFD modeling of transport phenomena in multi-channel anode-supported planar SOFCs. Int. J. Heat Mass Transf. 2015, 84, 942–954. [Google Scholar] [CrossRef]
- Yang, C.; Jin, C.; Coffin, A.; Chen, F. Characterization of infiltrated (La0.75Sr0.25)0.95MnO3 as oxygen electrode for solid oxide electrolysis cells. Int. J. Hydrogen Energy 2010, 35, 5187–5193. [Google Scholar] [CrossRef]
- Qi, S.; Xu, L.; Song, Y.; Jiang, W.; Hou, G. Numerical Investigation and Analyzation of an Anode-Supported Tubular Solid Oxide Fuel Cell. In Proceedings of the 2022 4th Asia Energy and Electrical Engineering Symposium (AEEES), Chengdu, China, 25–28 March 2022; pp. 579–582. [Google Scholar]
- Xia, L.; Ni, M.; He, Q.; Xu, Q.; Cheng, C. Optimization of gas diffusion layer in high temperature PEMFC with the focuses on thickness and porosity. Appl. Energy 2021, 300, 117357. [Google Scholar] [CrossRef]
- Zhang, H.; Davison, W. Performance Characteristics of Diffusion Gradients in Thin Films for the in Situ Measurement of Trace Metals in Aqueous Solution. Anal. Chem. 1995, 67, 3391–3400. [Google Scholar] [CrossRef]
- Krishna, R. Uphill diffusion in multicomponent mixtures. Chem Soc Rev 2015, 44, 2812–2836. [Google Scholar] [CrossRef]
- Fadzillah, D.M.; Rosli, M.I.; Talib, M.Z.M.; Kamarudin, S.K.; Daud, W.R.W. Review on microstructure modelling of a gas diffusion layer for proton exchange membrane fuel cells. Renew. Sustain. Energy Rev. 2017, 77, 1001–1009. [Google Scholar] [CrossRef]
- Chang, M.-H.; Chen, F.; Teng, H.-S. Effects of two-phase transport in the cathode gas diffusion layer on the performance of a PEMFC. J. Power Sources 2006, 160, 268–276. [Google Scholar] [CrossRef]
- Li, G.; Shi, W.; Zhang, W.; Yan, D.; Li, J.; Jia, L. Effect of rib width on the thermo-electro-mechanical behavior of solid oxide fuel cells. Sustain. Mater. Technol. 2024, 42, e01186. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, H.; Cai, W.; Ye, W.; Tong, Y.; Cheng, H. Effect of varying rib area portions on the performance of PEM fuel cells: Insights into design and optimization. Renew. Energy 2023, 217, 119185. [Google Scholar] [CrossRef]
- Yang, L.; Nik-Ghazali, N.-N.; Ali, M.A.H.; Chong, W.T.; Yang, Z.; Liu, H. A review on thermal management in proton exchange membrane fuel cells: Temperature distribution and control. Renew. Sustain. Energy Rev. 2023, 187, 113737. [Google Scholar] [CrossRef]
- Mortada, M.; Ramadan, H.S.; Faraj, J.; Faraj, A.; El Hage, H.; Khaled, M. Impacts of reactant flow nonuniformity on fuel cell performance and scaling-up: Comprehensive review, critical analysis and potential recommendations. Int. J. Hydrogen Energy 2021, 46, 32161–32191. [Google Scholar] [CrossRef]
- Wang, Y.; Banerjee, A.; Wehrle, L.; Shi, Y.; Brandon, N.; Deutschmann, O. Performance analysis of a reversible solid oxide cell system based on multi-scale hierarchical solid oxide cell modelling. Energy Convers. Manag. 2019, 196, 484–496. [Google Scholar] [CrossRef]
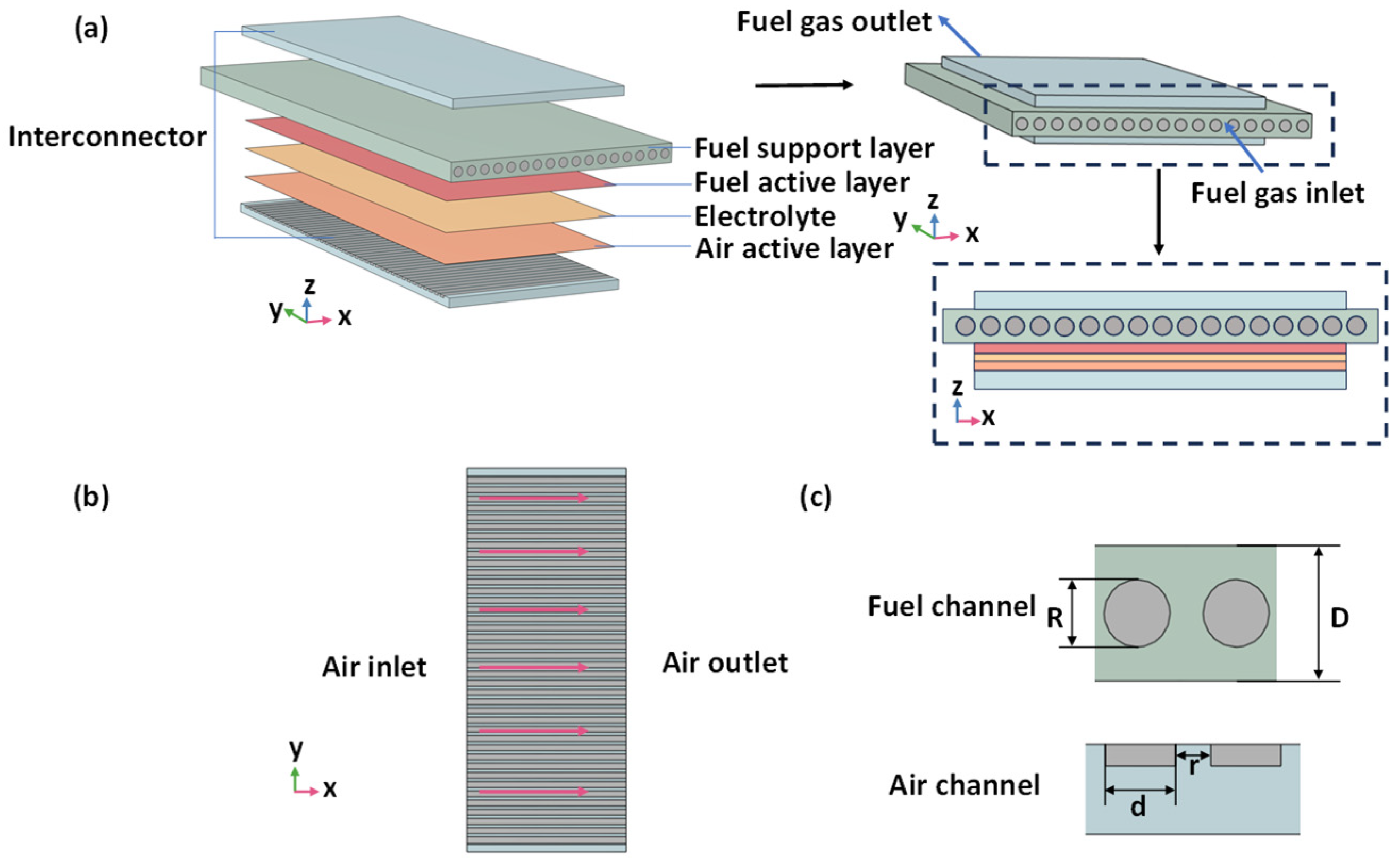

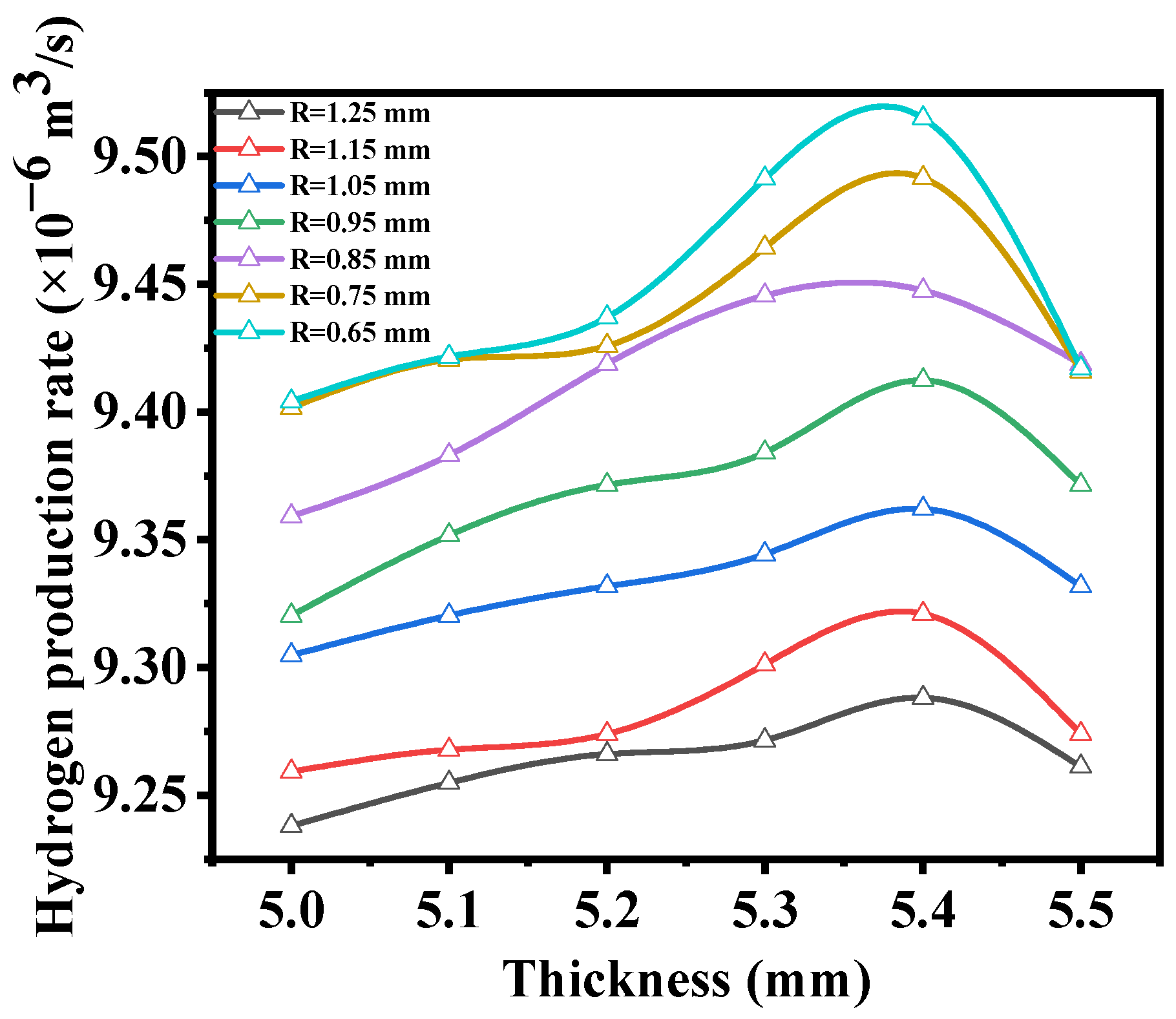
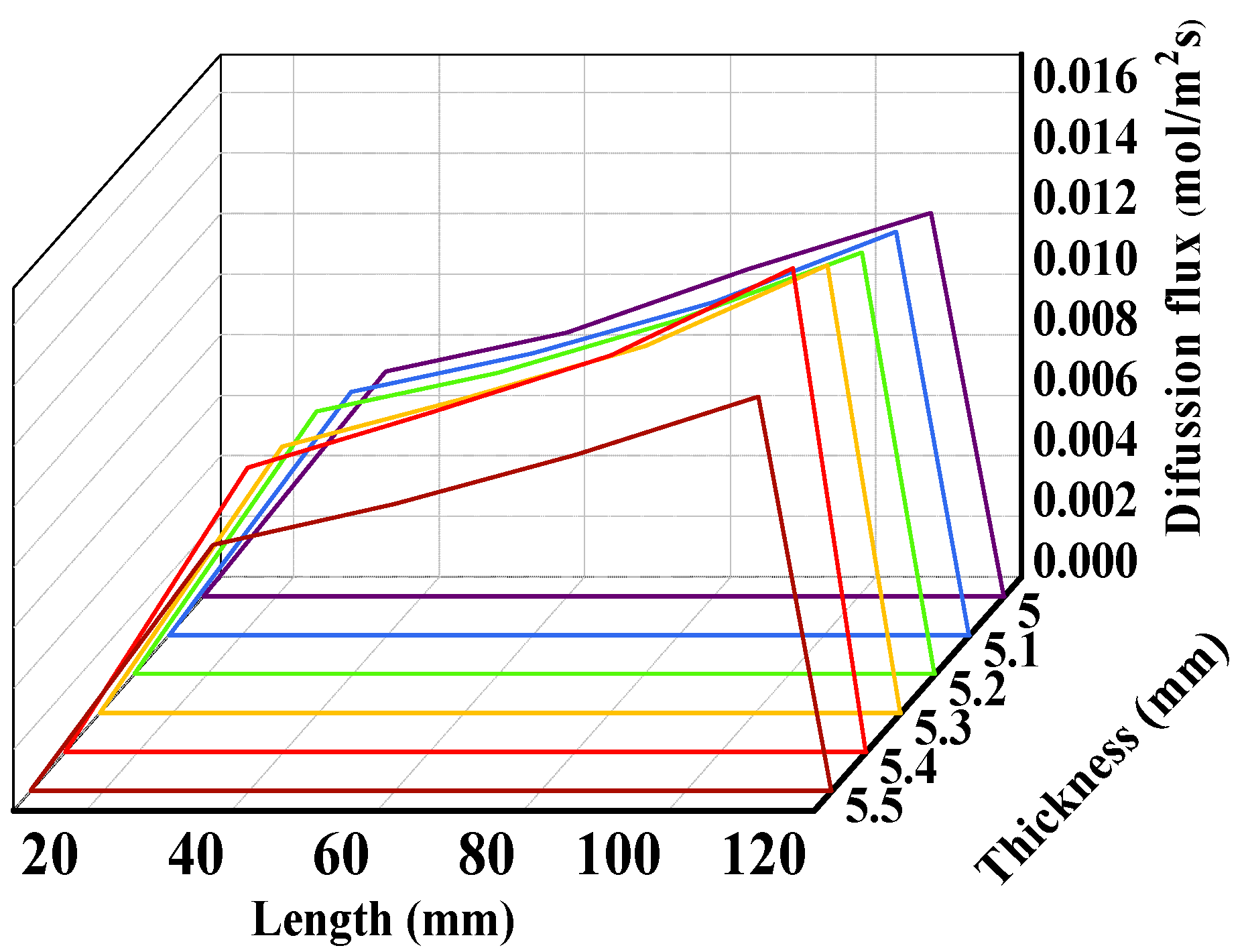
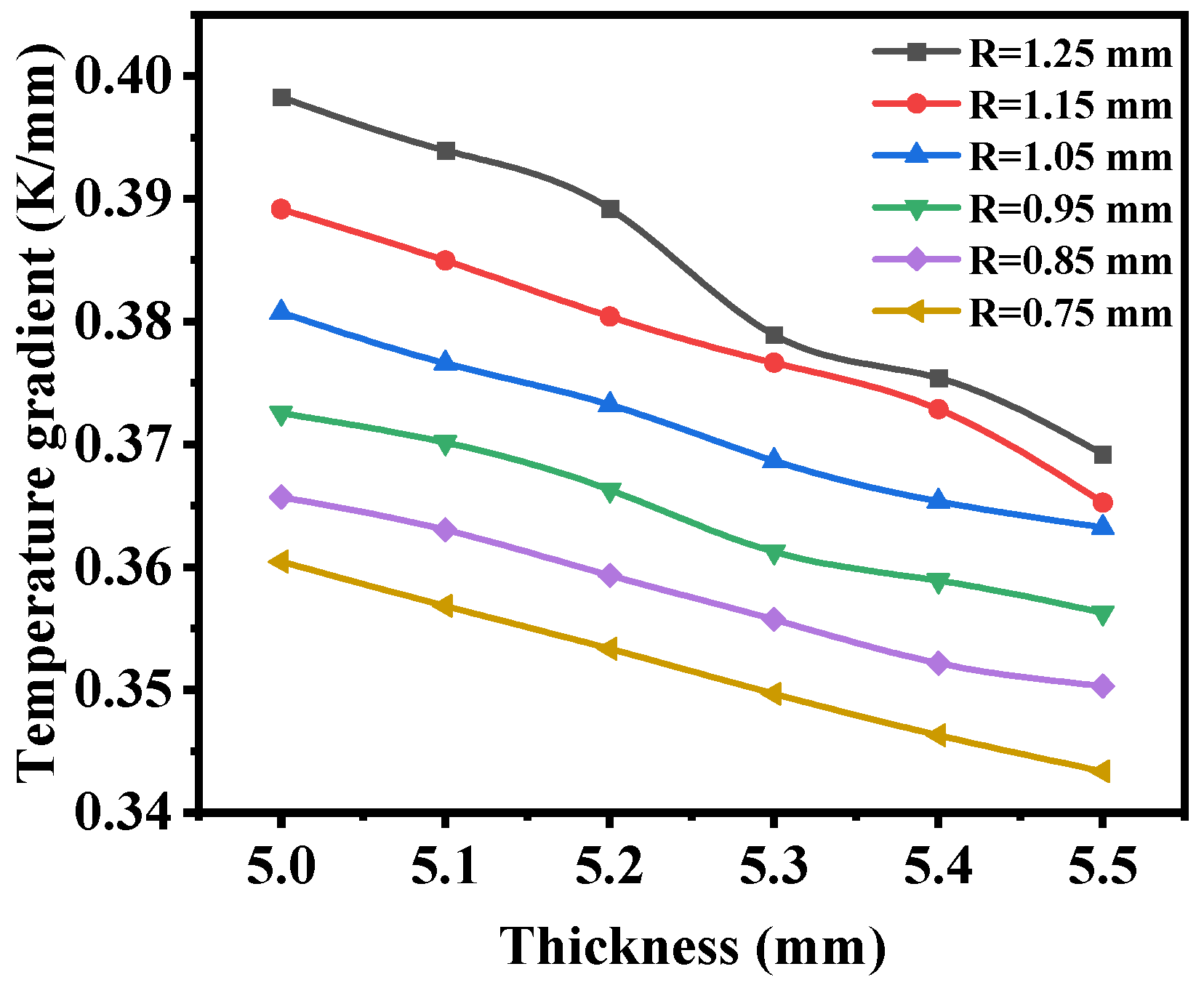






| Parameter | Length × Width × Height (mm) |
|---|---|
| Fuel support layer | 150 × 64 × 5 |
| Fuel active layer | 120 × 50 × 0.02 |
| Electrolyte | 120 × 50 × 0.015 |
| Air active layer | 120 × 50 × 0.02 |
| Interconnector | 125 × 50 × 2.5 |
| Materials | Electric/Ionic Conductivity (S/m) |
|---|---|
| Ni | 9.5 × 107/T × exp (−1150/T) |
| LSCF | 4.2 × 107/T × exp (−1200/T) |
| YSZ | 3.34 × 104 × exp (−10,300/T) |
| μi | A | B | C |
|---|---|---|---|
| H2O | −36.826 | 0.429 | 1.62 |
| H2 | 27.758 | 0.212 | 3.28 |
| N2 | 42.606 | 0.475 | 9.88 |
| O2 | 44.224 | 0.562 | 1.13 |
| Parameters | Value | Unit |
|---|---|---|
| Operating Voltage | 1.0–1.4 | V |
| Operating Temperature | 1073–1123 | K |
| Operating Pressure | 1 | atm |
| Fuel Composition | H2:H2O = 50%:50% | / |
| Air Composition | O2:N2 = 21%:79% | / |
| Fuel Outlet | / | Convection |
| Channel Width (mm) | Rib Width (mm) | Rib Area Portion (%) |
|---|---|---|
| 1.5 | 1.5 | 50 |
| 1.75 | 1.25 | 42 |
| 2.0 | 1 | 33 |
| 2.25 | 0.75 | 25 |
| 2.5 | 0.5 | 17 |
| Volume of Ni (%) | STPB (m2/m3) | CTE (10−6 K−1) | Elastic Modulus (GPa) |
|---|---|---|---|
| 20 | 0.51 × 105 | 9.062 | 130 |
| 30 | 1.43 × 105 | 9.473 | 131 |
| 40 | 3.11 × 105 | 9.884 | 132 |
| 50 | 6.00 × 105 | 10.295 | 133 |
| 60 | 11.2 × 105 | 10.706 | 135 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, S.; Xu, J.; Liao, Y.; Zhao, Y.; Huo, H.; Chu, Z. Multiphysics-Driven Structural Optimization of Flat-Tube Solid Oxide Electrolysis Cells to Enhance Hydrogen Production Efficiency and Thermal Stress Resistance. Energies 2025, 18, 2449. https://doi.org/10.3390/en18102449
Liang S, Xu J, Liao Y, Zhao Y, Huo H, Chu Z. Multiphysics-Driven Structural Optimization of Flat-Tube Solid Oxide Electrolysis Cells to Enhance Hydrogen Production Efficiency and Thermal Stress Resistance. Energies. 2025; 18(10):2449. https://doi.org/10.3390/en18102449
Chicago/Turabian StyleLiang, Shanshan, Jingxiang Xu, Yunfeng Liao, Yu Zhao, Haibo Huo, and Zhenhua Chu. 2025. "Multiphysics-Driven Structural Optimization of Flat-Tube Solid Oxide Electrolysis Cells to Enhance Hydrogen Production Efficiency and Thermal Stress Resistance" Energies 18, no. 10: 2449. https://doi.org/10.3390/en18102449
APA StyleLiang, S., Xu, J., Liao, Y., Zhao, Y., Huo, H., & Chu, Z. (2025). Multiphysics-Driven Structural Optimization of Flat-Tube Solid Oxide Electrolysis Cells to Enhance Hydrogen Production Efficiency and Thermal Stress Resistance. Energies, 18(10), 2449. https://doi.org/10.3390/en18102449








