Multi-Physics Coupling Dynamics Simulation of Thermally Induced Vibration of Magnetically Suspended Rotor in Small and Micro Nuclear Reactors
Abstract
:1. Introduction
2. System Structure and Multi-Physics Coupling Analysis Framework
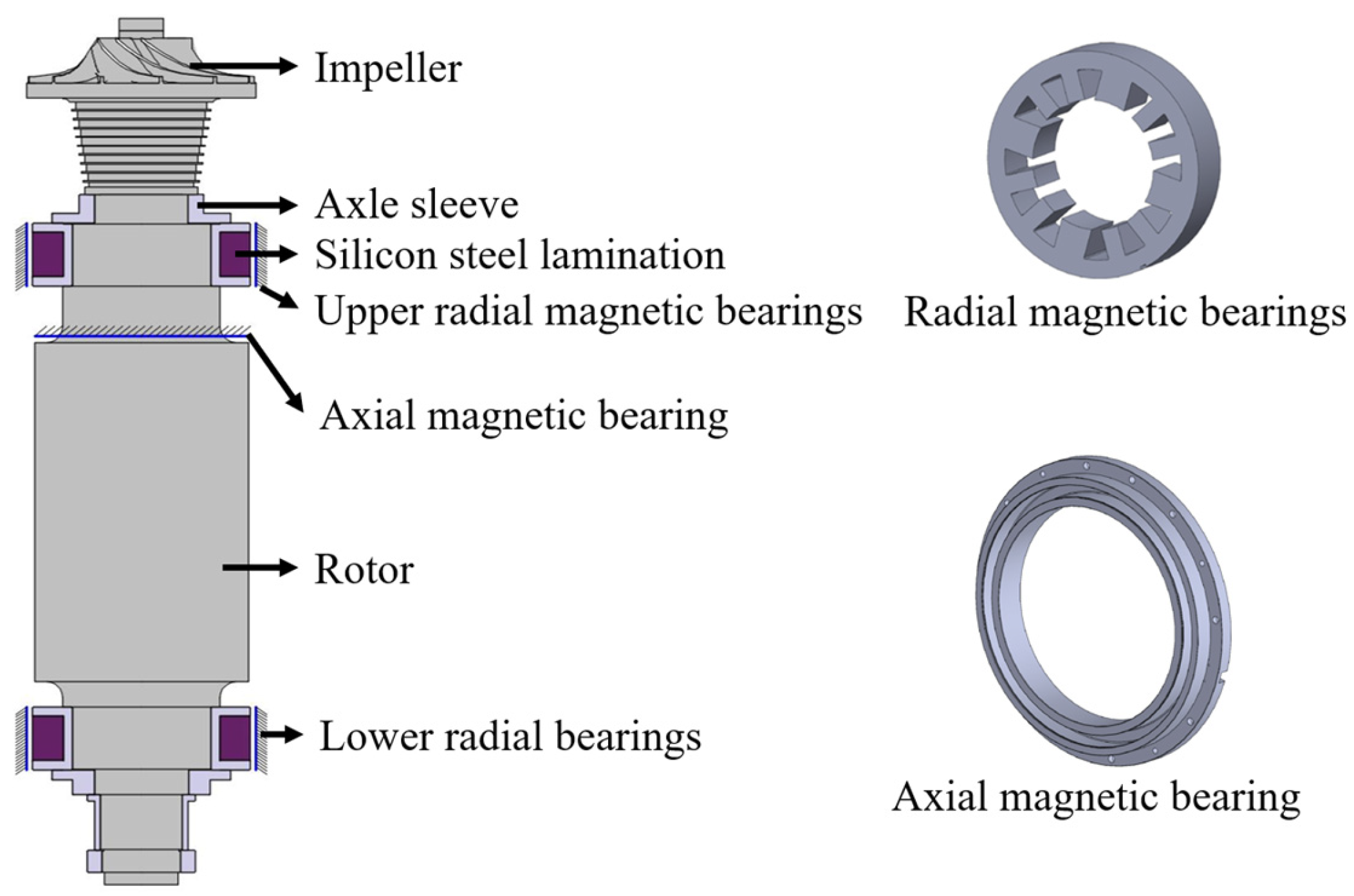
2.1. System Structure
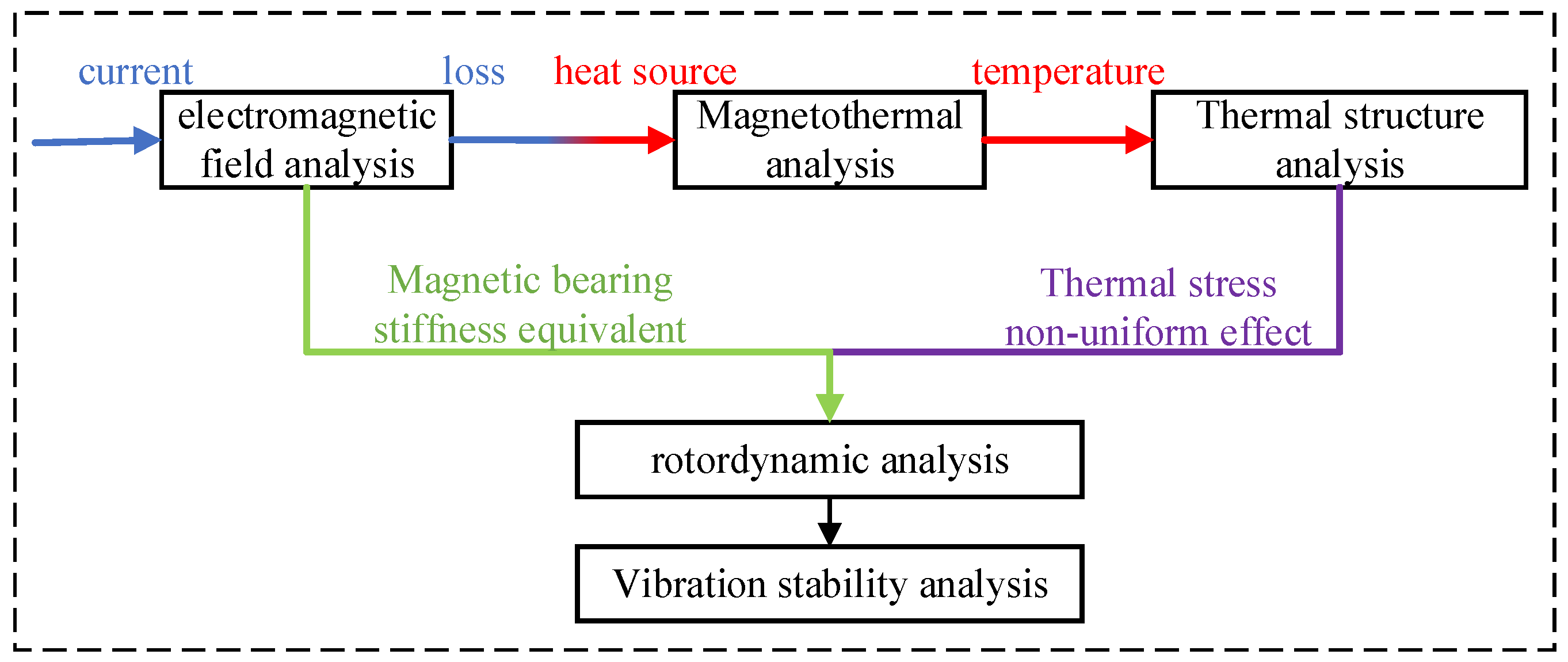
2.2. Multi-Physics Coupled Analysis Architecture
3. Electromagnetic Coupling
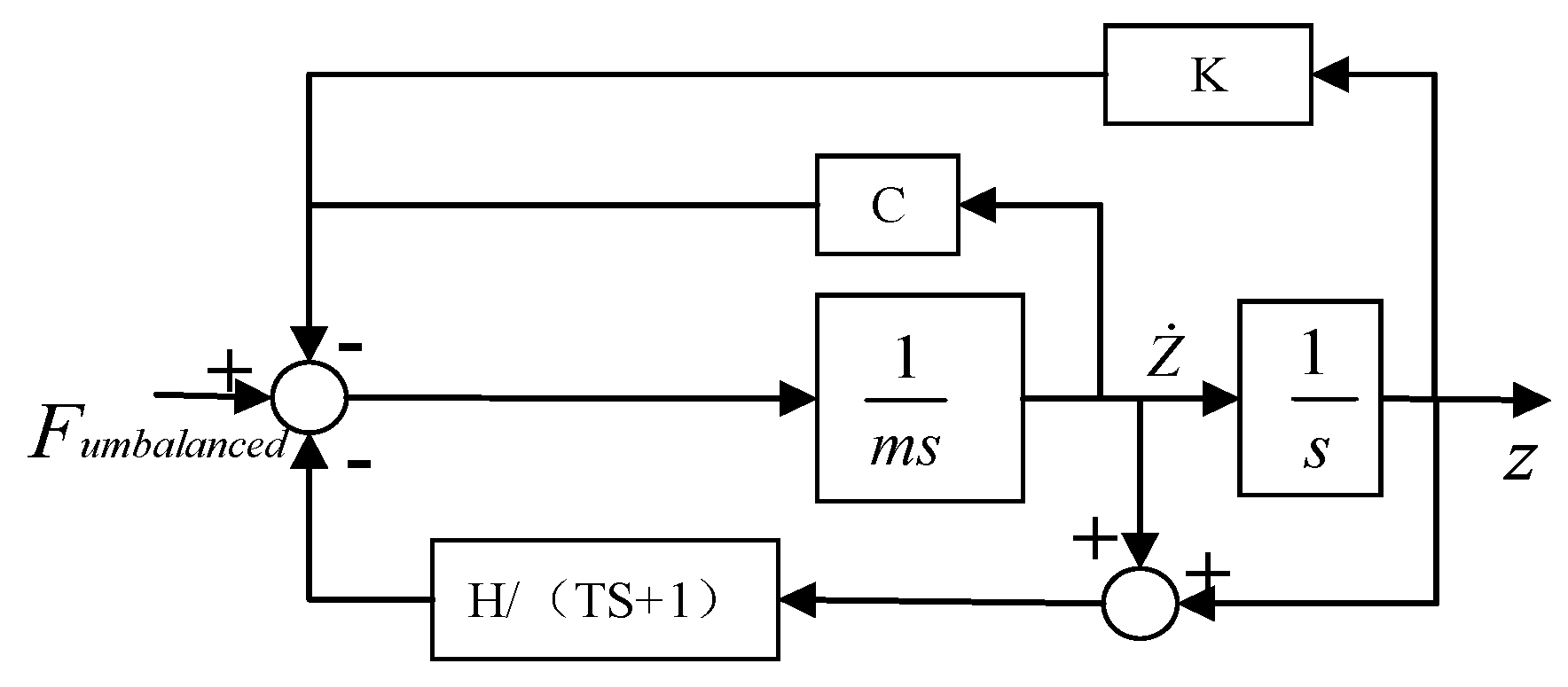
3.1. Equivalent Magnetic Circuit and Stiffness Equivalent
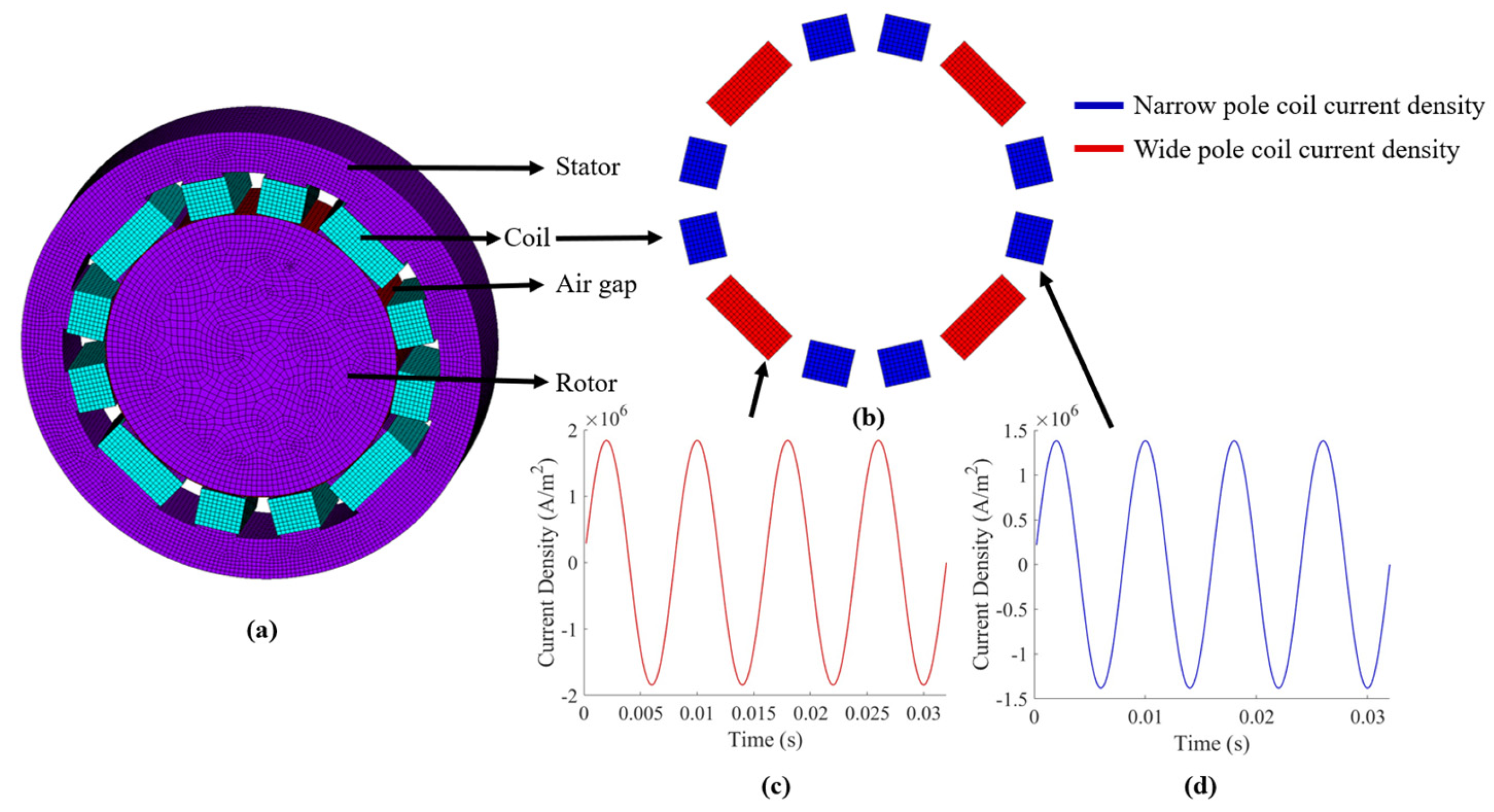
3.2. Electromagnetic Finite Element Calculation of Electromagnetic Bearings
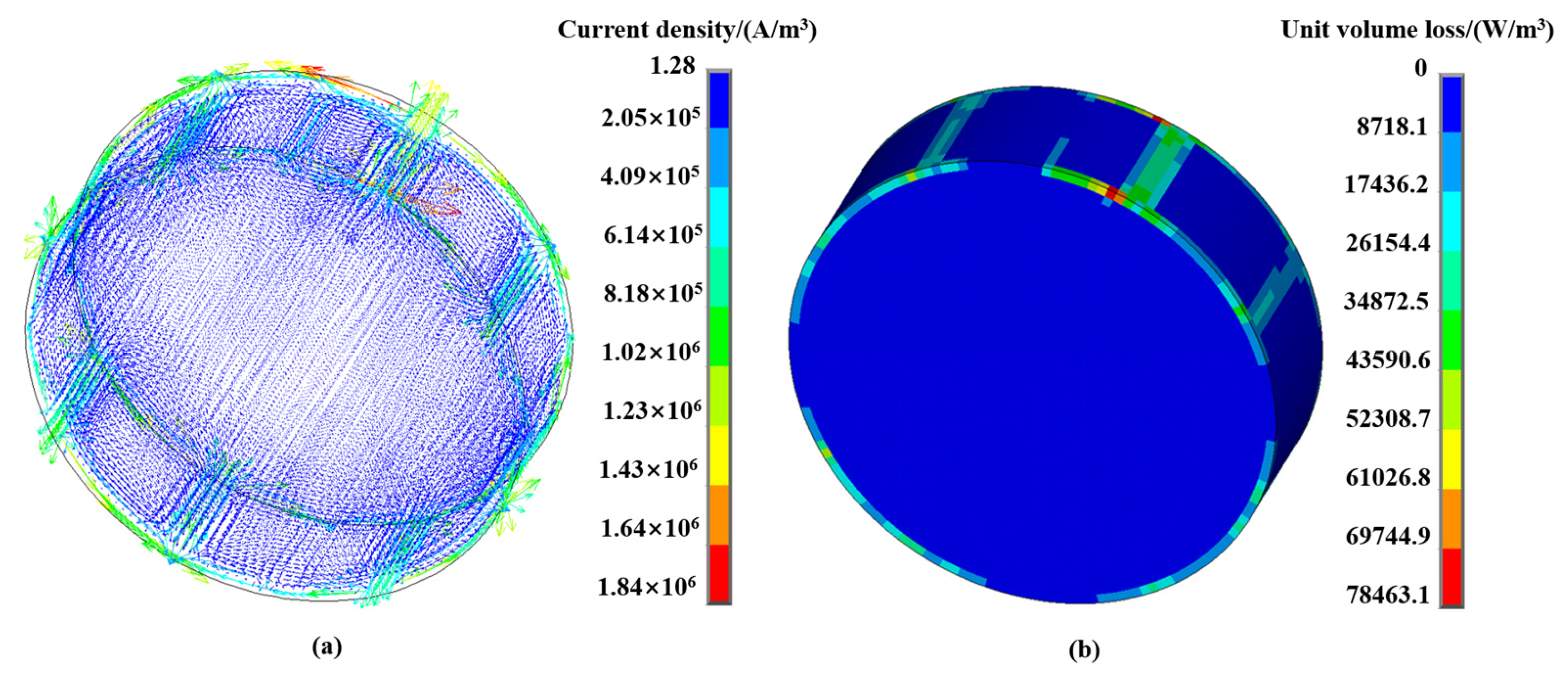
4. Magneto-Thermal Coupling
5. Heat Structural Coupling
6. Rotor Dynamic Analysis
7. System Stability Analysis
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Teräsvirta, A.; Syri, S.; Hiltunen, P. Small Nuclear Reactor-Nordic District Heating Case Study. Energies 2020, 13, 3782. [Google Scholar] [CrossRef]
- Alam, S.B.; Ridwan, T.; Kumar, D.; Almutairi, B.; Goodwin, C.; Parks, G.T. Small modular reactor core design for civil marine propulsion using micro-heterogeneous duplex fuel. Part II: Whole-core analysis. Nucl. Eng. Des. 2019, 346, 176–191. [Google Scholar] [CrossRef]
- Liu, Y.; Zhao, Z.W.; Wang, Y.C.; Zhao, F.; Lu, D.G.; Cao, Q. Dynamic response of a multi-point mooring cylindrical floating nuclear power platform carrying a small-scale reactor. Ocean Eng. 2023, 267, 14. [Google Scholar] [CrossRef]
- Bhowmik, P.K.; Sabharwall, P.; Johnson, J.T.; Retamales, M.E.T.; Wang, C.; Brien, J.E.; Lietwiler, C.; Wu, Q. Scaling methodologies and similarity analysis for thermal hydraulics test facility development for water-cooled small modular reactor. Nucl. Eng. Des. 2024, 424, 15. [Google Scholar] [CrossRef]
- Zheng, Y.; Mo, N.; Sun, Z.; Zhou, Y.; Shi, Z. Study on Unbalanced Magnetic Pulling Analysis and Its Control Method for Primary Helium Circulator of High-Temperature Gas-Cooled Reactor. Energies 2019, 12, 3682. [Google Scholar] [CrossRef]
- Plantegenet, T.; Arghir, M.; Hassini, M.-A.; Jolly, P. The Thermal Unbalance Effect Induced by a Journal Bearing in Rigid and Flexible Rotors: Experimental Analysis. Tribol. Trans. 2019, 63, 52–67. [Google Scholar] [CrossRef]
- Takahashi, N.; Kaneko, S. Thermal instability in a magnetically levitated doubly overhung rotor. J. Sound Vib. 2013, 332, 1188–1203. [Google Scholar] [CrossRef]
- Jin, C.; Su, H.; Dong, Y.; Zhou, J.; Xu, Y.; Yan, X. Iron loss analysis of magnetic bearing system considering magnetic-thermal coupling. Int. J. Appl. Electromagn. Mech. 2022, 70, 1–20. [Google Scholar] [CrossRef]
- Hassini, M.-A. Numerical and experimental analysis of stable, unstable, and limit cycle spiral vibrations. Mech. Syst. Signal Process. 2023, 193, 110258. [Google Scholar] [CrossRef]
- He, Q.; Wang, J.; Li, K.; Wang, X.; Xu, Z.; Zhang, Y. Thermal analysis and thermal management of high power density electric motors for aircraft electrification. Appl. Therm. Eng. 2025, 260, 125006. [Google Scholar] [CrossRef]
- Zhang, X.; Han, B.C.; Liu, X.; Chen, Y.L.; Zhai, L.X. Prediction and experiment of DC- bias iron loss in radial magnetic bearing for a small scale turbomolecular pump. Vacuum 2019, 163, 224–235. [Google Scholar] [CrossRef]
- Zhai, L.X.; Han, B.C.; Liu, X.; Zhao, J.H. Losses estimation, thermal-structure coupled simulation analysis of a magnetic-bearing reaction wheel. Int. J. Appl. Electromagn. Mech. 2019, 60, 33–53. [Google Scholar] [CrossRef]
- Liu, J.; Zhang, P. Thermo-mechanical behavior analysis of motorized spindle based on a coupled model. Adv. Mech. Eng. 2018, 10, 168781401774714. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Y.; Zhao, Y.; Shi, X. Thermal-mechanical coupling calculation method for deformation error of motorized spindle of machine tool. Eng. Fail. Anal. 2021, 128, 105597. [Google Scholar] [CrossRef]
- Sun, M.; Xu, Y.; Zhang, W. Multiphysics Analysis of Flywheel Energy Storage System Based on Cup Winding Permanent Magnet Synchronous Machine. IEEE Trans. Energy Convers. 2023, 38, 2684–2694. [Google Scholar] [CrossRef]
- Wang, K.; Ma, X.; Liu, Q.; Chen, S.H.; Liu, X.Q. Multiphysics Global Design and Experiment of the Electric Machine with a Flexible Roto Supported by Active Magnetic Bearing. IEEE-ASME Trans. Mechatron. 2019, 24, 820–831. [Google Scholar] [CrossRef]
- Guo, S.J.; Bai, C.Q. Experimental and Numerical Research Studies on Multifield Coupling Rotordynamic Behaviors of Motor. Shock. Vib. 2021, 2021, 5550804. [Google Scholar] [CrossRef]
- Scheidler, J.J.; Tallerico, T.F.; Stalcup, E.J.; Duffy, K.P. Thermal, Structural, and Rotordynamics Refinements to the Superconducting Rotor of a 1.4 MW Partially Superconducting Machine for Electrified Aircraft. IEEE Trans. Appl. Supercond. 2025, 35, 5203005. [Google Scholar] [CrossRef]
- ISO 1940-1; Mechanical Vibration—Balance Quality Requirements for Rotors in a Constant (Rigid) State—Part 1: Specification and Verification of Balance Tolerances. The ISO Council: Geneve, Switzerland, 2003.

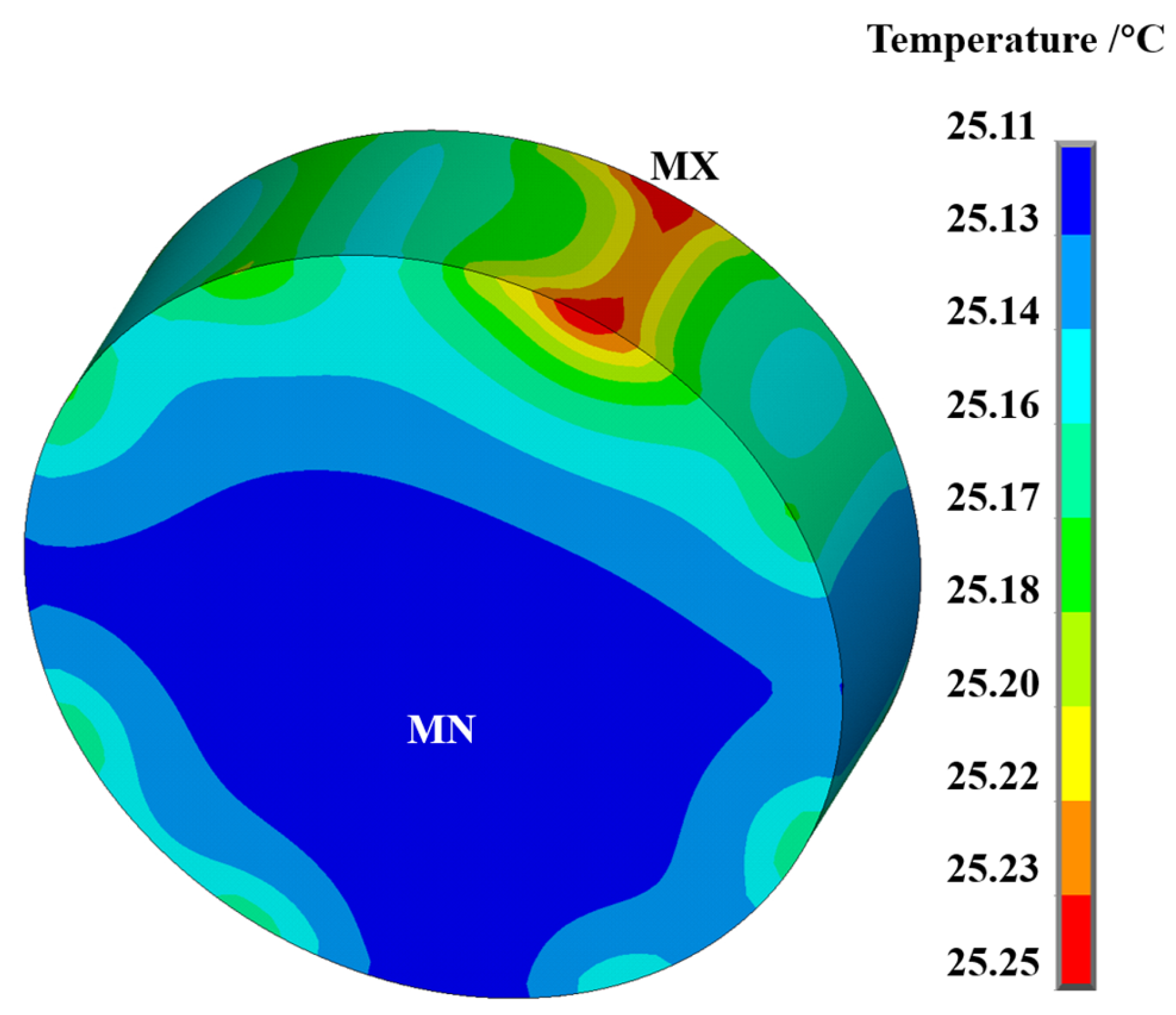
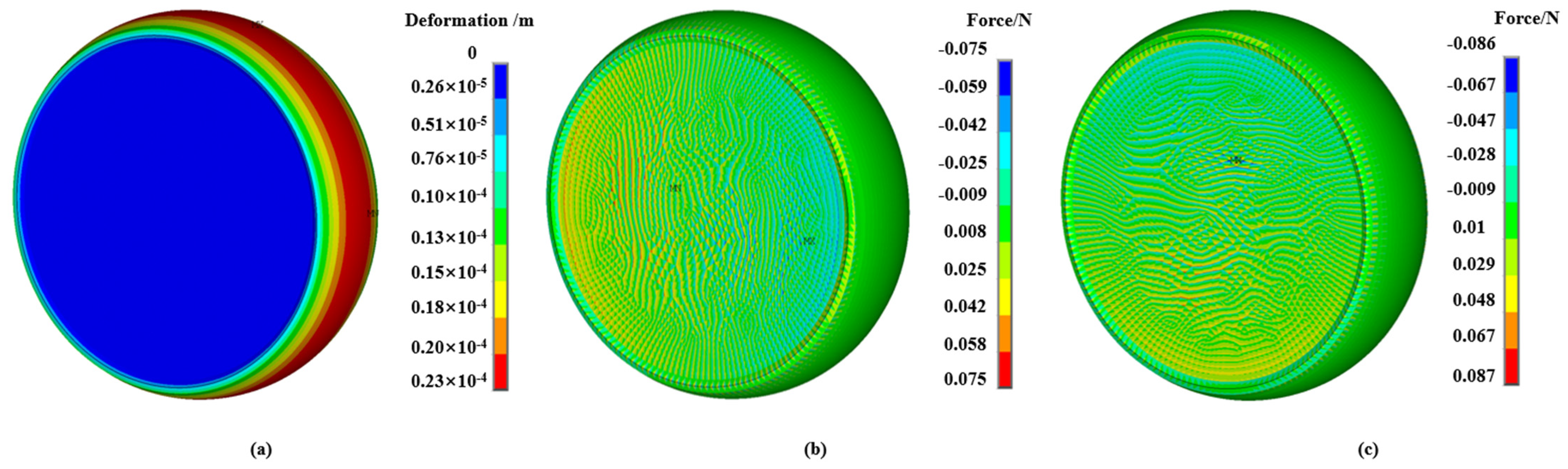

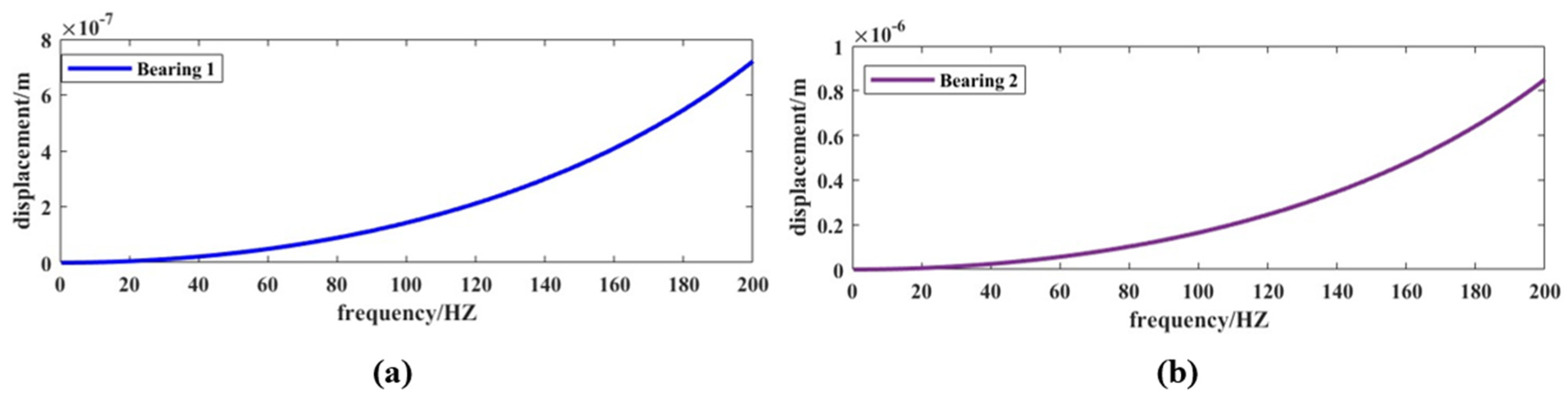
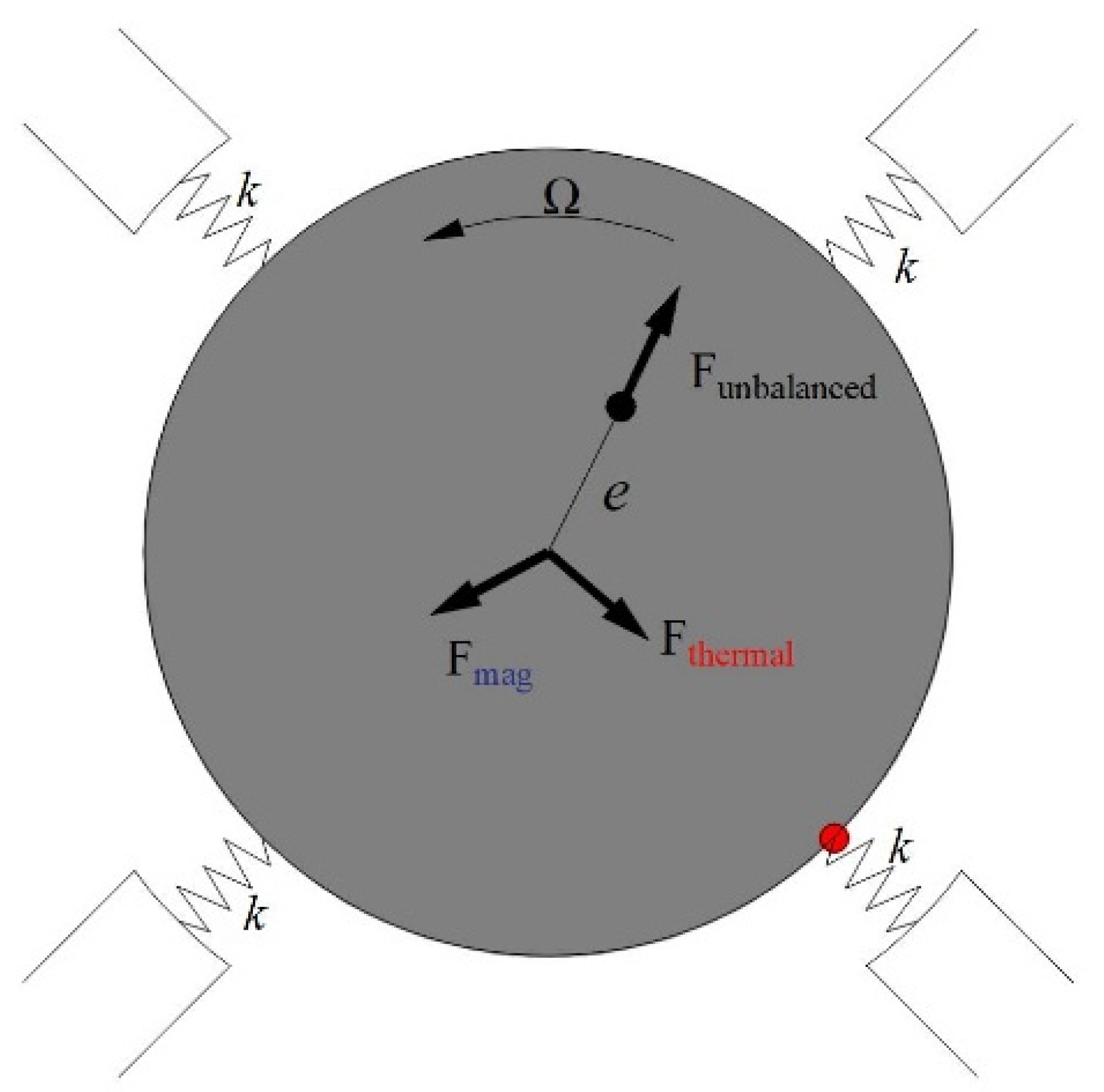

| Parameter Name | Value |
|---|---|
| Turns of wide magnetic pole coil | 32 |
| Turns of narrow magnetic pole coil | 24 |
| Air gap | 1 mm |
| The maximum amplitude of current | 10 A |
| The minimum current amplitude | 5 A |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, Y.; Li, Z.; Xing, D. Multi-Physics Coupling Dynamics Simulation of Thermally Induced Vibration of Magnetically Suspended Rotor in Small and Micro Nuclear Reactors. Energies 2025, 18, 2433. https://doi.org/10.3390/en18102433
Xu Y, Li Z, Xing D. Multi-Physics Coupling Dynamics Simulation of Thermally Induced Vibration of Magnetically Suspended Rotor in Small and Micro Nuclear Reactors. Energies. 2025; 18(10):2433. https://doi.org/10.3390/en18102433
Chicago/Turabian StyleXu, Yihao, Zeguang Li, and Dianchuan Xing. 2025. "Multi-Physics Coupling Dynamics Simulation of Thermally Induced Vibration of Magnetically Suspended Rotor in Small and Micro Nuclear Reactors" Energies 18, no. 10: 2433. https://doi.org/10.3390/en18102433
APA StyleXu, Y., Li, Z., & Xing, D. (2025). Multi-Physics Coupling Dynamics Simulation of Thermally Induced Vibration of Magnetically Suspended Rotor in Small and Micro Nuclear Reactors. Energies, 18(10), 2433. https://doi.org/10.3390/en18102433





