Numerical Investigation on Heat Transfer and Pressure Drop in Silver/Water Nanofluids Flowing Through Tubes with Variable Expansion–Contraction Ratios
Abstract
1. Introduction
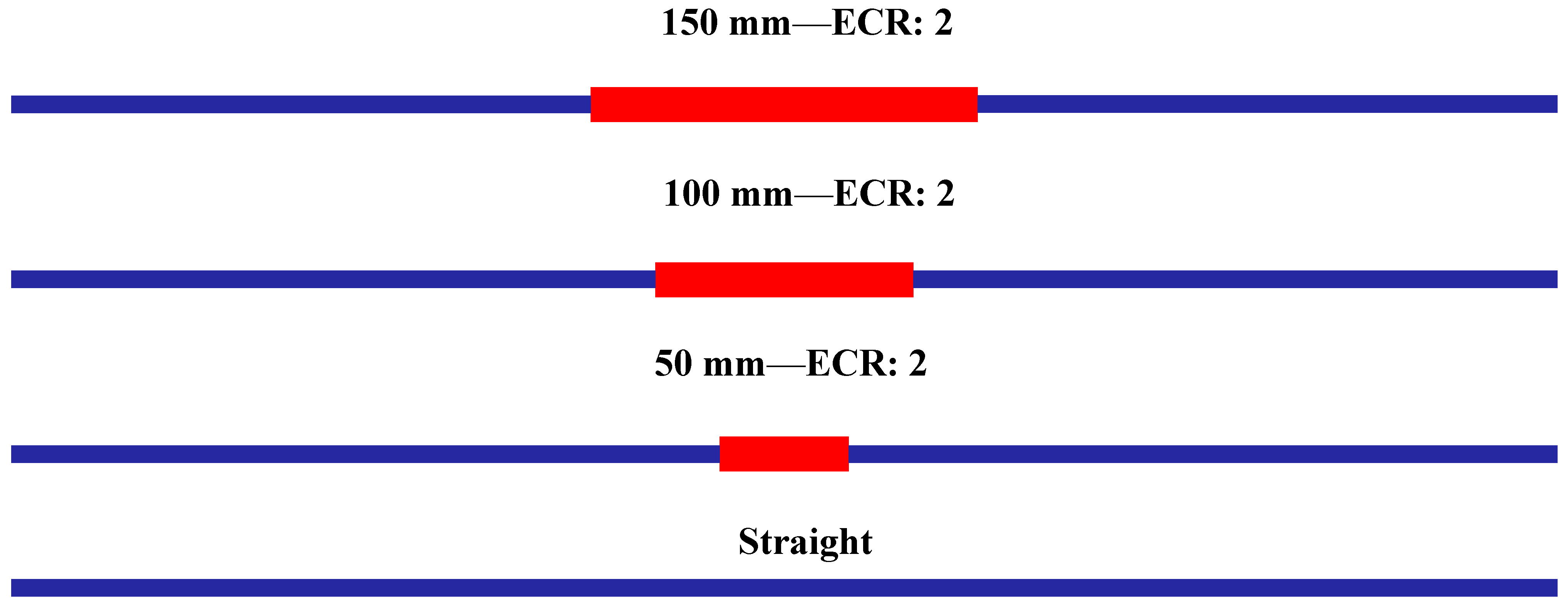
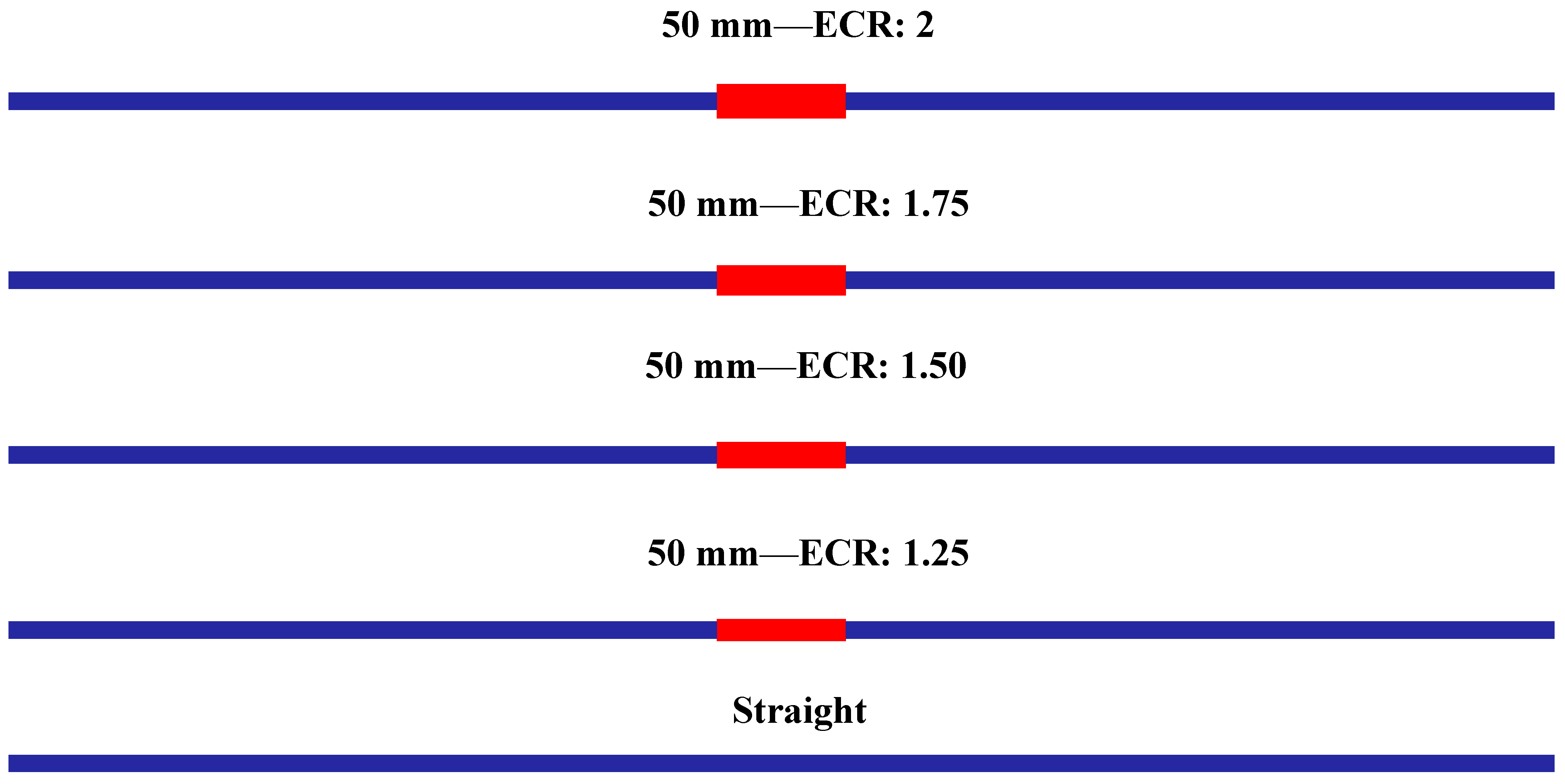
2. The Problem Statement
3. Numerical Procedure
3.1. Governing Equations
3.2. Software Verification and Computational Model Validation
3.2.1. Software Verification
3.2.2. Mesh Independence and Computational Model Validation
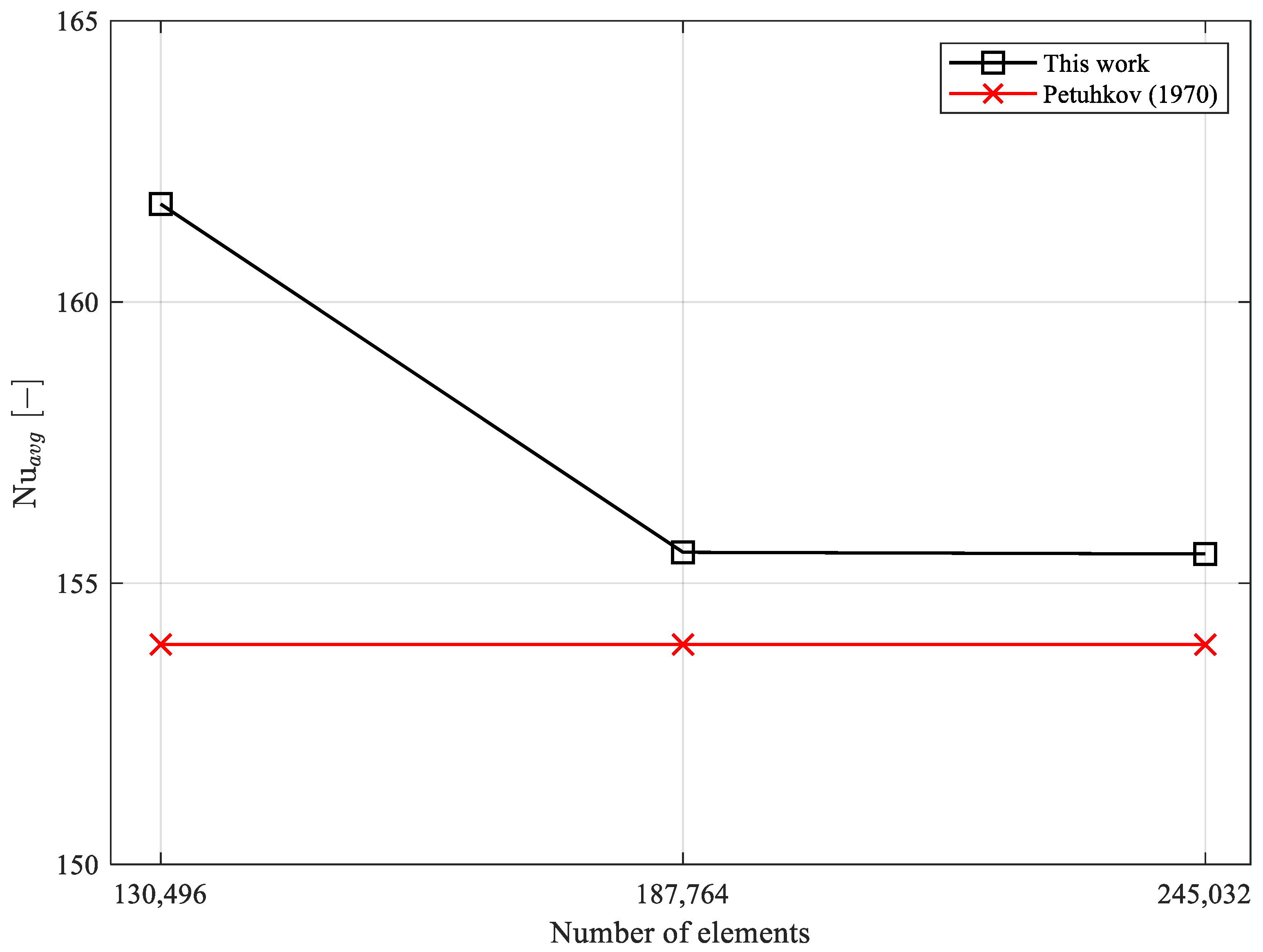
4. Thermophysical Properties
5. Data Reduction
6. Results and Discussions
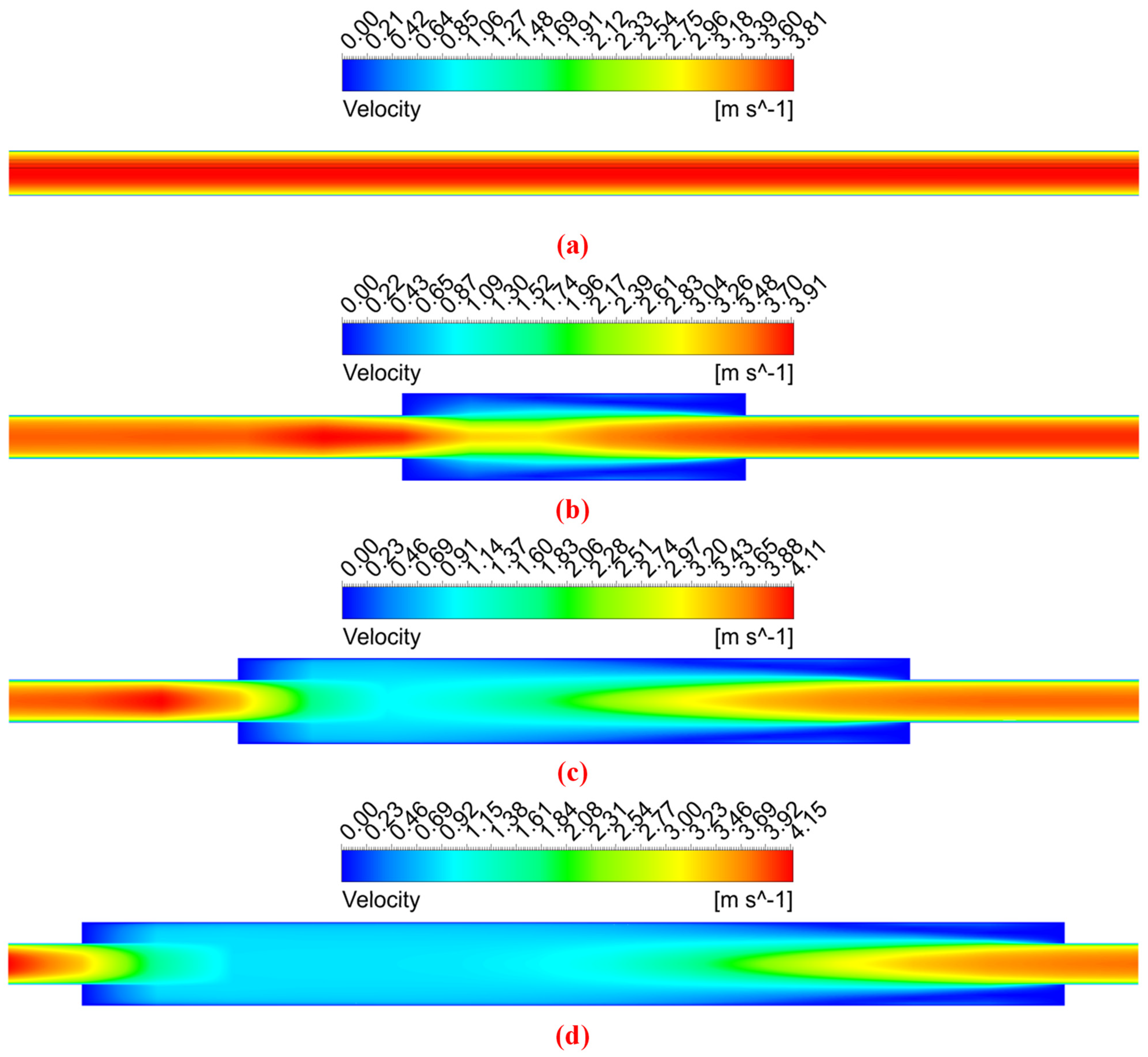
6.1. Local Convective Heat Transfer Coefficient
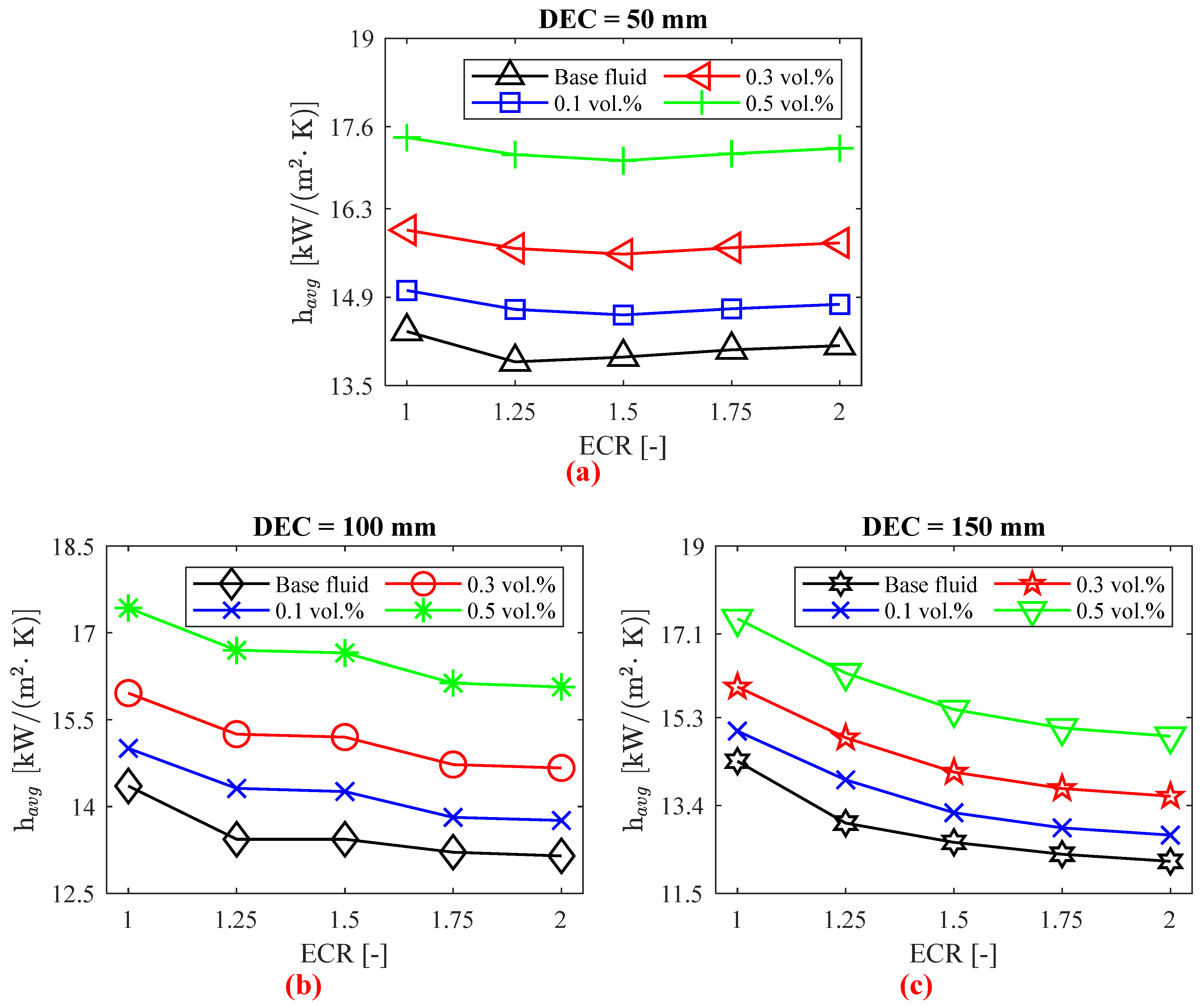
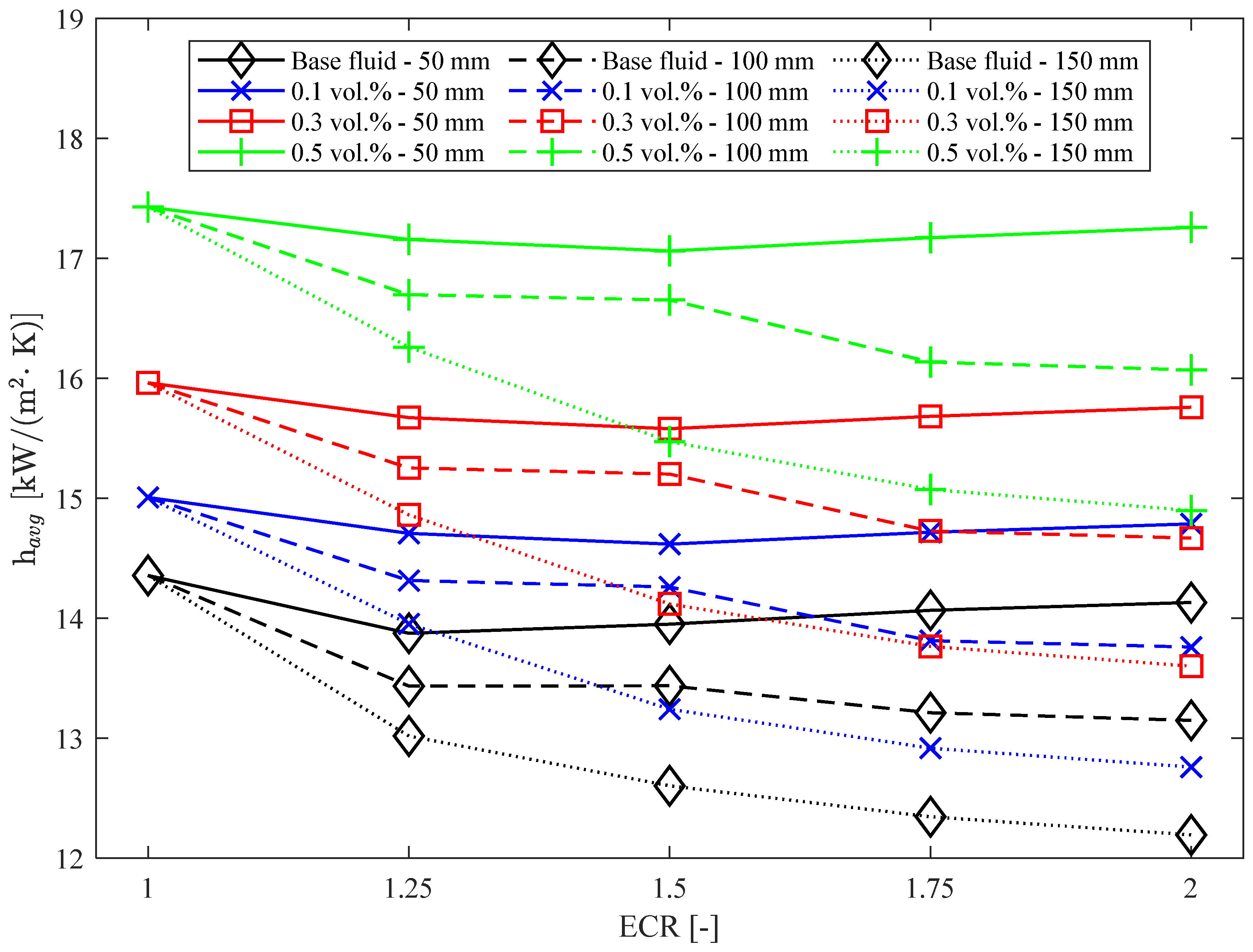
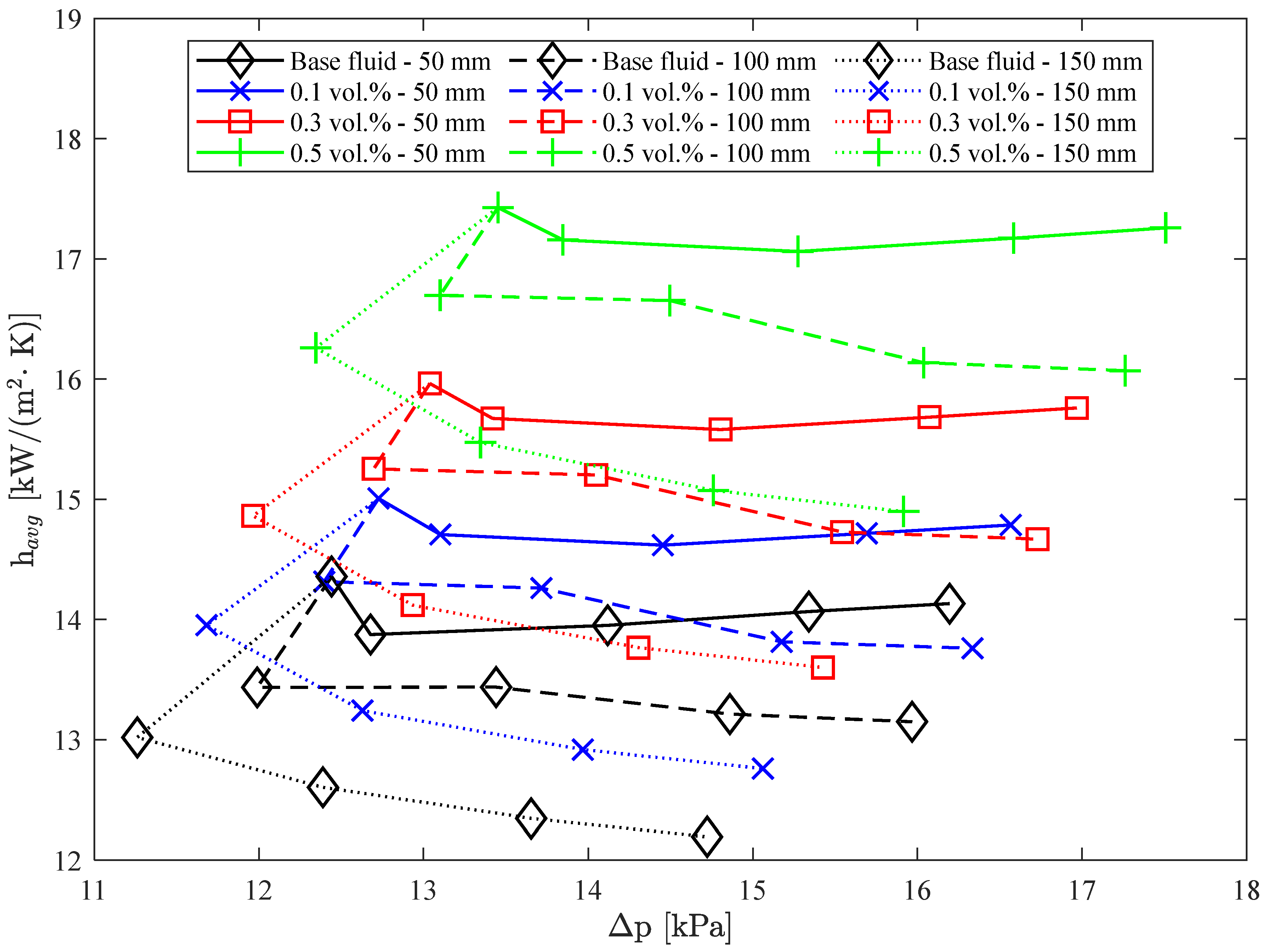
6.2. Average Convective Heat Transfer Coefficient
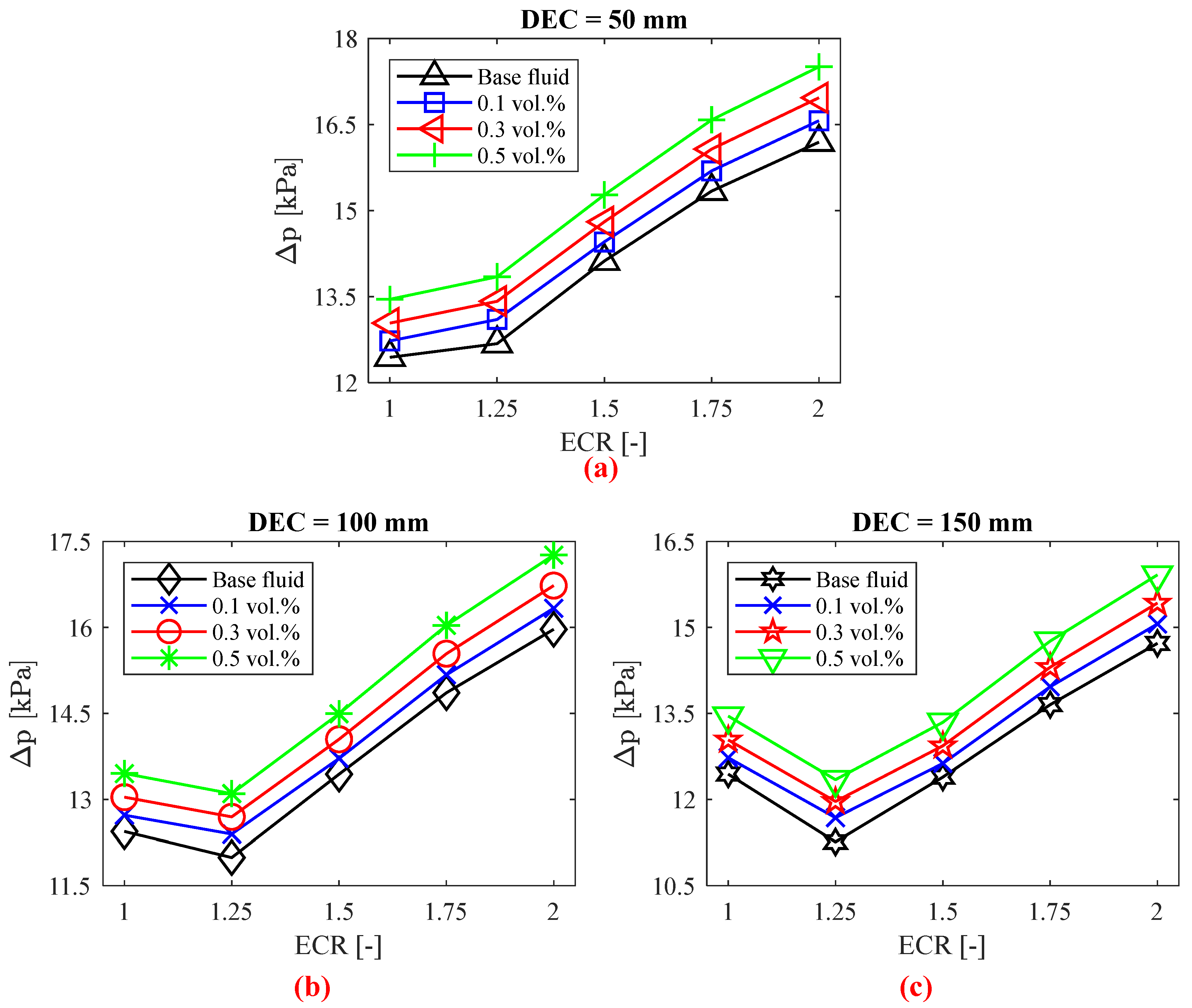
6.3. Pressure Drop
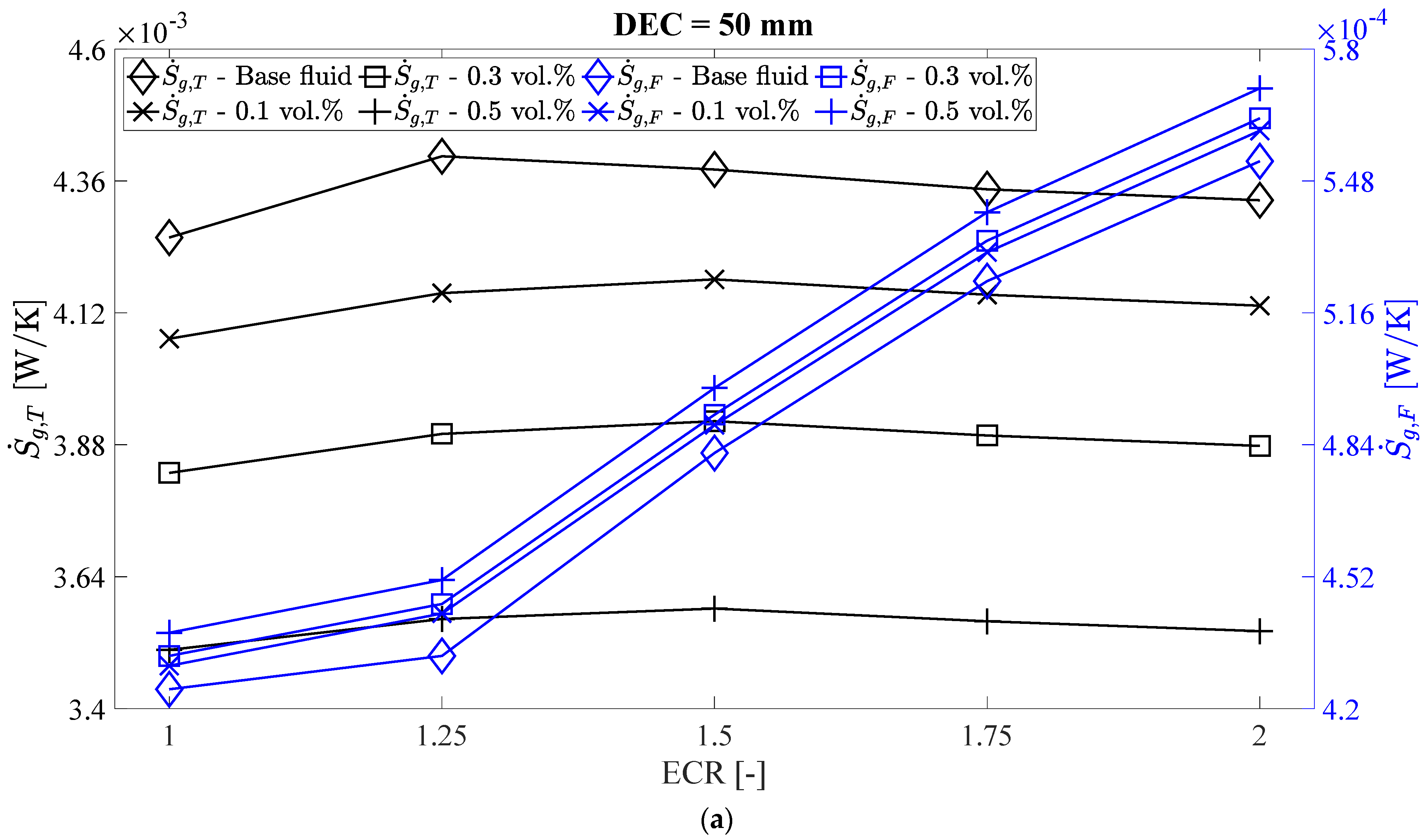
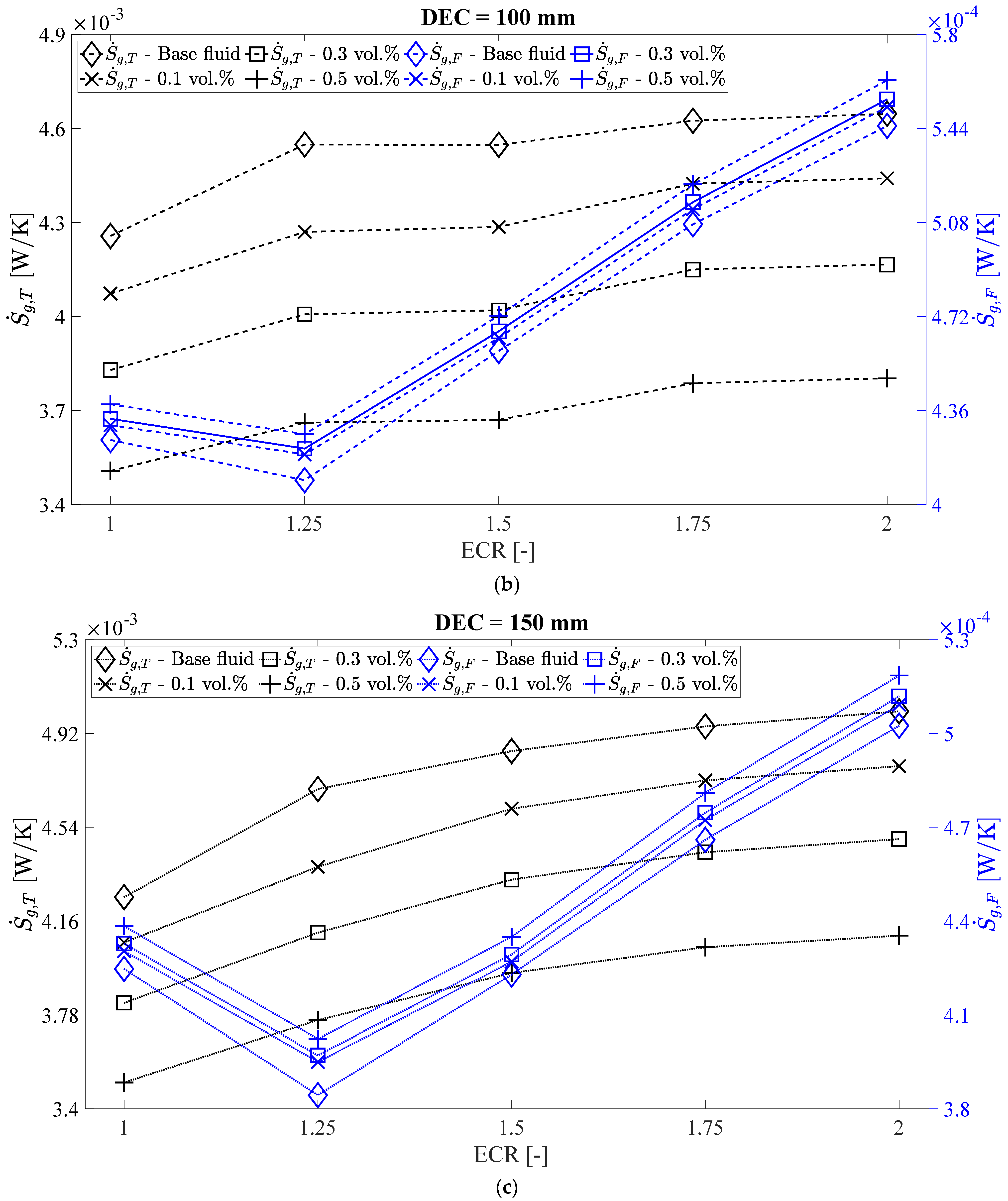
6.4. Entropy Generation
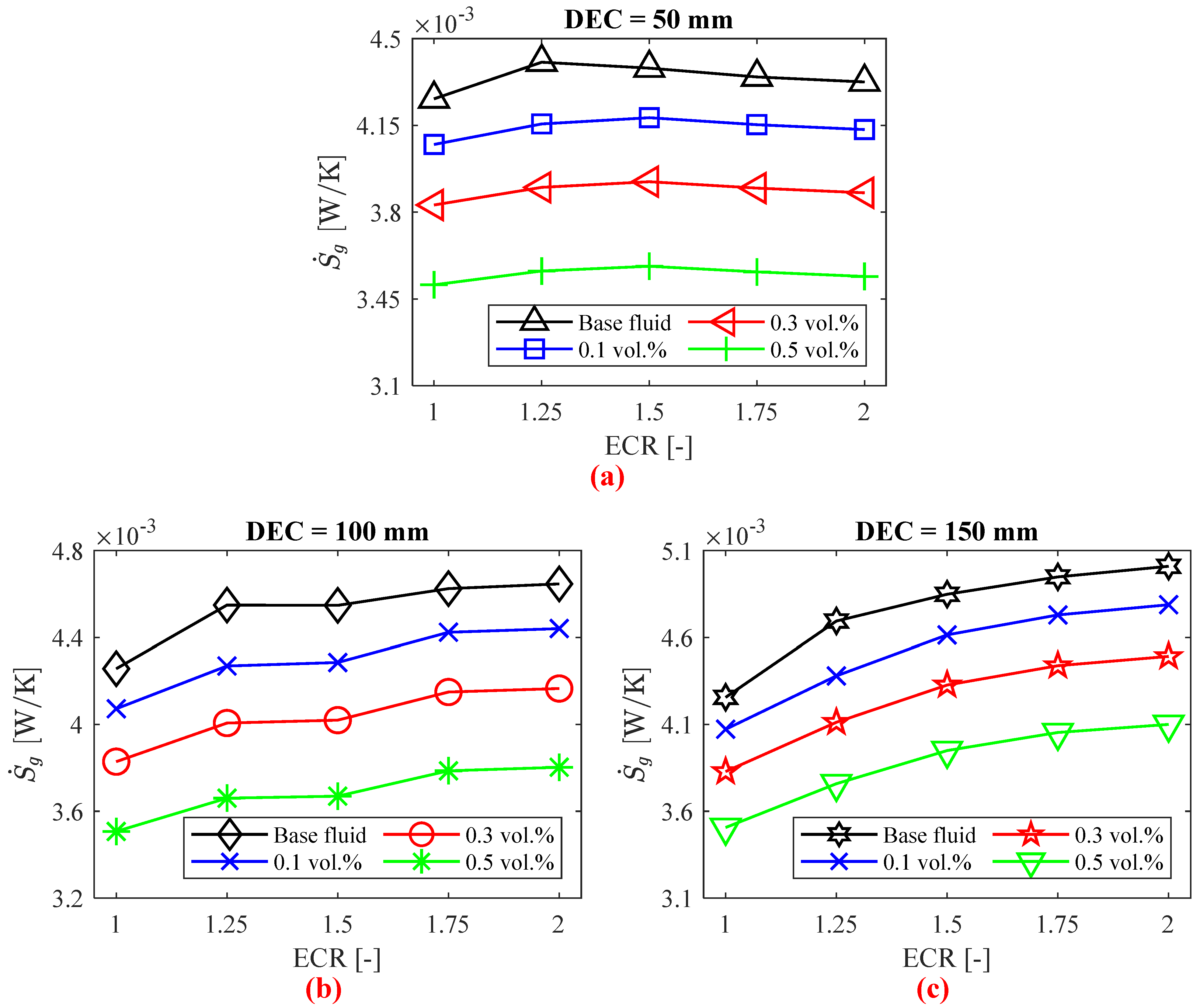
6.5. Total Entropy Generation
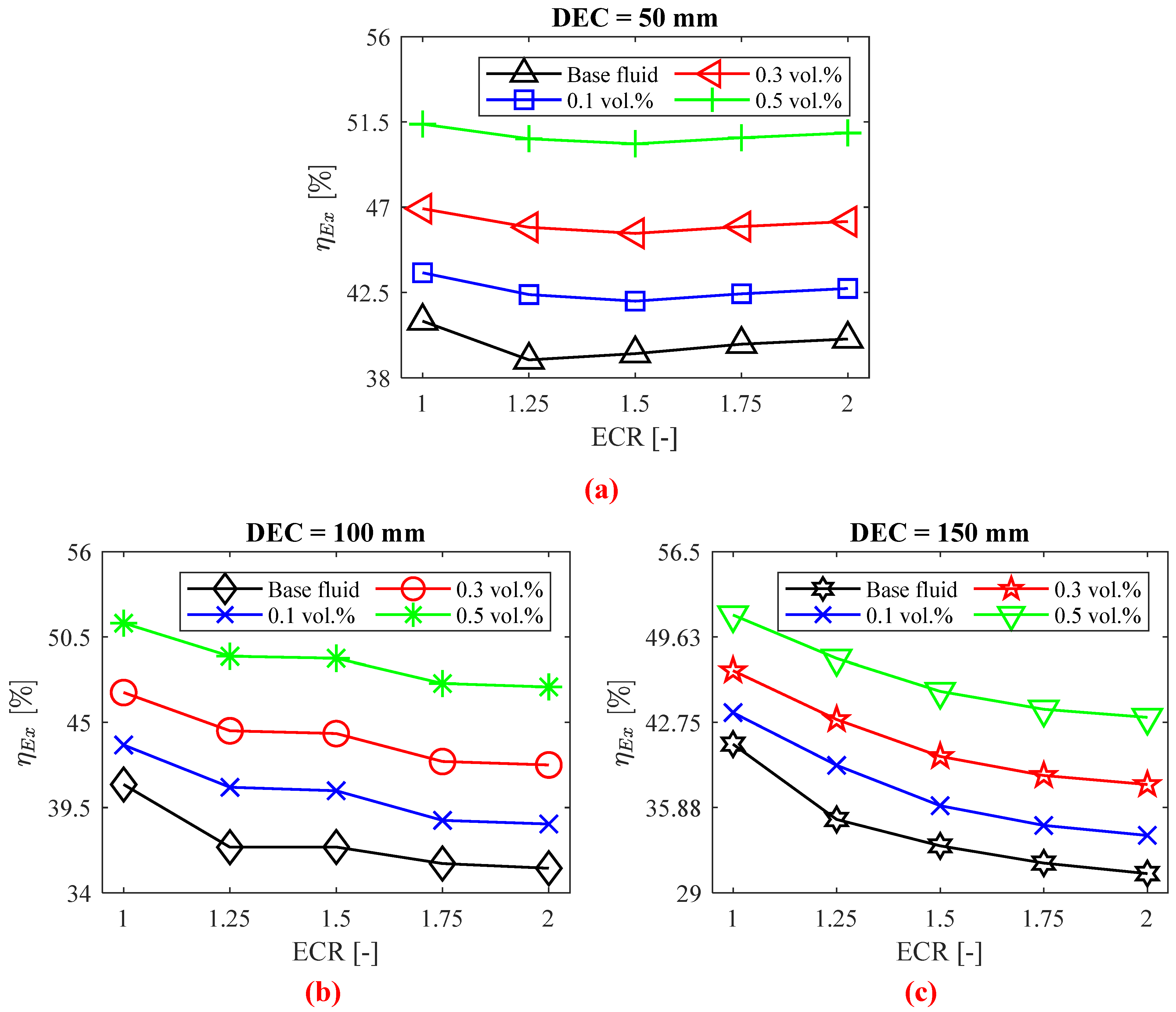
6.6. Exergy Efficiency
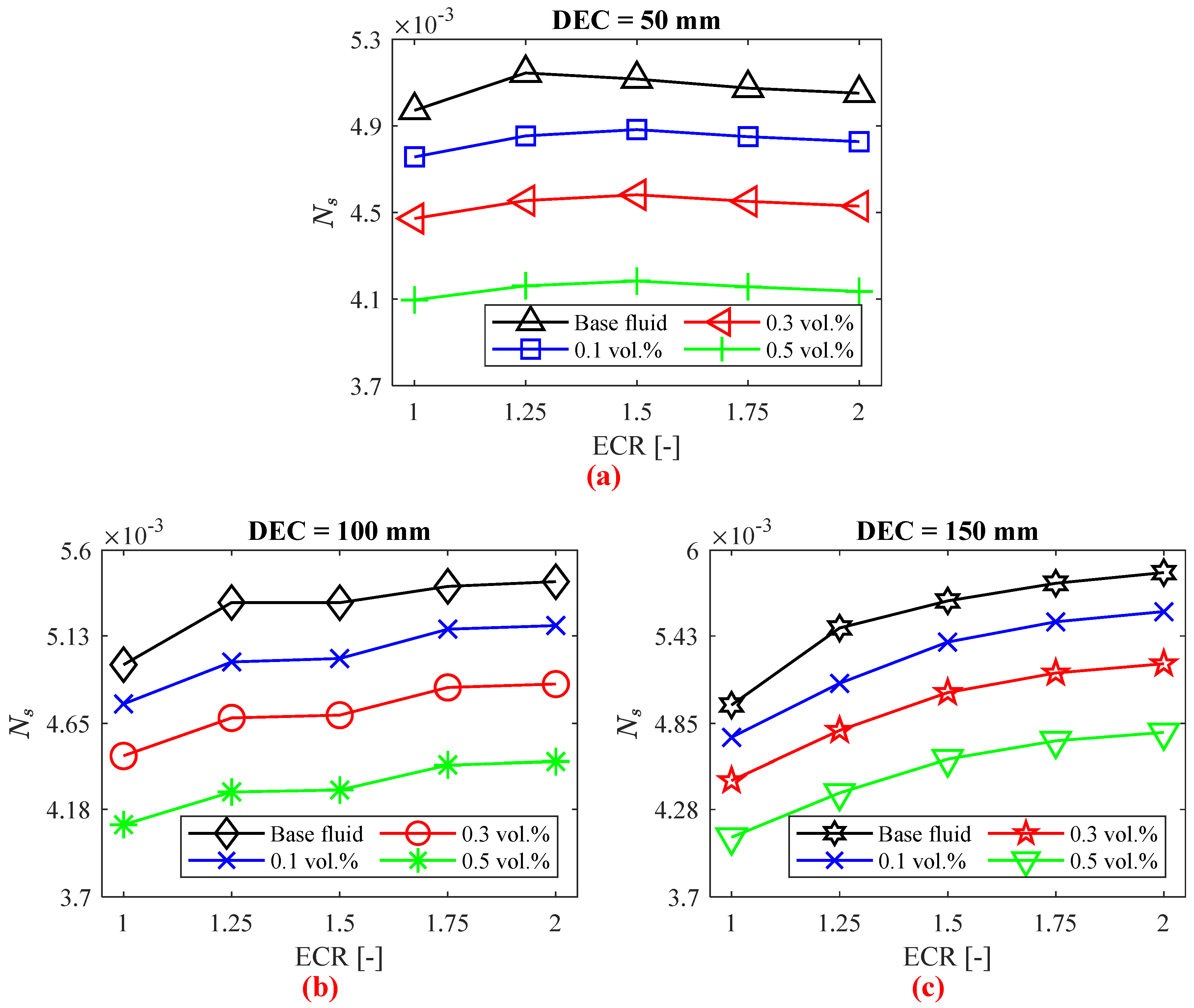
6.7. Entropy Generation Number
6.8. Thermal-Hydraulic Performance
7. Conclusions
- Model Validation: The numerical model demonstrated satisfactory accuracy in predicting thermal and hydraulic performance, matching well with experimental results and theoretical correlations.
- Impact of ECR: An increased ECR led to a reduction in the average convective heat transfer coefficient by up to 15.07% compared to a straight tube, with some improvements noted only for DEC = 50 mm.
- Effect of Nanofluids: Silver/water nanofluids improved the convective heat transfer coefficient by up to 24.90% at a 0.5% volumetric concentration and mitigated performance drops caused by higher ECR and DEC.
- Pressure Drop: The pressure drop increased with DEC and ECR, with reductions of up to 9.5% for DEC at 50 mm and ECR = 1.25. However, pressure drops increased with DEC for all cases.
- Viscosity and Pressure Drop: Nanoparticle addition increased coolant viscosity and pressure drop by up to 18.34%, though the effect of ECR on pressure drop was minimal.
- Exergy Efficiency: Exergy efficiency increased by up to 29.97% with higher silver nanoparticle concentrations.
- Overall Performance: Nanofluids generally outperformed the base fluid in thermal-hydraulic performance, with the most significant improvement (14.92%) observed for ECR = 1.5 and DEC = 100 mm with a 0.5% nanofluid concentration.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Nomenclature | |
| z | Axial distance [m] |
| h | Convective heat transfer coefficient [W/(m2·K)] |
| Entropy generation number [–] | |
| Exergy efficiency [%] | |
| Friction factor [–] | |
| Frictional entropy generation [W/K] | |
| Heat flux [W/m2] | |
| Heat transfer rate [W] | |
| D | Hydraulic diameter [m] |
| Kronecker delta | |
| Mass flow rate [kg/s] | |
| Nu | Nusselt number [–] |
| Pr | Prandlt number [-] |
| p | Pressure [Pa] |
| Pressure drop [Pa] | |
| Re | Reynolds number [–] |
| Specific heat [kJ/(kg·K)] | |
| T | Temperature [K] |
| Thermal entropy generation [W/K] | |
| Total entropy generation [W/K] | |
| L | Tube length [m] |
| u | Velocity [m/s] |
| Greek symbols | |
| Density [kg/m3] | |
| Dynamic viscosity [Pa·s] | |
| Thermal conductivity [W/(m·K)] | |
| Volumetric concentration [%] | |
| Abbreviations | |
| DEC | Distance between the contraction and expansion |
| ECR | Expansion–contraction Ratio |
| ER | Expansion Ratio |
| SST | Shear Stress Transport |
| Subscript | |
| ave | Average |
| bf | Base fluid |
| f | Fluid |
| in | Inlet |
| nf | Nanofluid |
| np | Nanoparticle |
| out | Outlet |
| w | Wall |
References
- Salman, S.; Talib, A.R.A.; Saadon, S.; Sultan, M.T.H. Hybrid Nanofluid Flow and Heat Transfer over Backward and Forward Steps: A Review. Powder Technol. 2020, 363, 448–472. [Google Scholar] [CrossRef]
- Kumar, A.; Yadav, S.K.; Kumar, V.; Kulkarni, A. A Comprehensive Exploration of Ejector Design, Operational Factors, Performance Metrics, and Practical Applications. J. Braz. Soc. Mech. Sci. Eng. 2024, 46, 39. [Google Scholar] [CrossRef]
- Li, H.; Lu, H.; Li, Q. Numerical investigations of the influences of valve spool structure on the eccentric jet flow characteristic in high-pressure angle valves. Energy 2024, 298, 131378. [Google Scholar] [CrossRef]
- Togun, H.; Sultan, H.S.; Hamidatou, S.; Mohammed, H.I.; Homod, R.Z.; Alhassan, M.S.; Dhabab, J.M.; Sadeq, A.M.; Yaseen, Z.M.; Deghoum, K.; et al. Comparative Study on Heat Transfer Improvement of Nanofluids Flow in Forward-Facing Reducing Channel with and without Novel Hybrid Ribs. Int. J. Therm. Sci. 2023, 193, 108543. [Google Scholar] [CrossRef]
- Cárdenas Contreras, E.M.; Oliveira, G.A.; Bandarra Filho, E.P. Experimental Analysis of the Thermohydraulic Performance of Graphene and Silver Nanofluids in Automotive Cooling Systems. Int. J. Heat Mass Transf. 2019, 132, 375–387. [Google Scholar] [CrossRef]
- Moreira, T.A.; Moreira, D.C.; Ribatski, G. Nanofluids for Heat Transfer Applications: A Review. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 303. [Google Scholar] [CrossRef]
- Togun, H.; Abu-Mulaweh, H.I.; Kazi, S.N.; Badarudin, A. Numerical Simulation of Heat Transfer and Separation Al2O3/Nanofluid Flow in Concentric Annular Pipe. Int. Commun. Heat Mass Transf. 2016, 71, 108–117. [Google Scholar] [CrossRef]
- Kimouche, A.; Mataoui, A.; Oztop, H.F.; Abu-Hamdeh, N. Analysis of Heat Transfer of Different Nanofluids Flow through an Abrupt Expansion Pipe. Appl. Therm. Eng. 2017, 112, 965–974. [Google Scholar] [CrossRef]
- Cárdenas Gómez, A.O.; Paz Alegrias, J.G.; Bandarra Filho, E.P. Experimental Analysis of the Thermal-Hydraulic Performance of Water Based Silver and SWCNT Nanofluids in Single-Phase Flow. Appl. Therm. Eng. 2017, 124, 1176–1188. [Google Scholar] [CrossRef]
- Nouri, D.; Pasandideh-Fard, M.; Javad Oboodi, M.; Mahian, O.; Sahin, A.Z. Entropy Generation Analysis of Nanofluid Flow over a Spherical Heat Source inside a Channel with Sudden Expansion and Contraction. Int. J. Heat Mass Transf. 2018, 116, 1036–1043. [Google Scholar] [CrossRef]
- Atashafrooz, M.; Sheikholeslami, M.; Sajjadi, H.; Amiri Delouei, A. Interaction Effects of an Inclined Magnetic Field and Nanofluid on Forced Convection Heat Transfer and Flow Irreversibility in a Duct with an Abrupt Contraction. J. Magn. Magn. Mater. 2019, 478, 216–226. [Google Scholar] [CrossRef]
- Danane, F.; Boudiaf, A.; Mahfoud, O.; Ouyahia, S.; Labsi, N.; Benkahla, Y.K. Effect of Backward Facing Step Shape on 3D Mixed Convection of Bingham Fluid. Int. J. Therm. Sci. 2020, 147, 106116. [Google Scholar] [CrossRef]
- Safaei, M.R.; Abdelghany Elkotb, M.; Alsharif, A.M.; Mansir, I.B.; Alamri, S.; Tirth, V.; Goodarzi, M. An Innovative Design of a High Strength and Low Weight Sudden Micro Expansion by Considering a Nanofluid: Electronic Cooling Application. Case Stud. Therm. Eng. 2021, 28, 101637. [Google Scholar] [CrossRef]
- Arabi, A.; Salhi, Y.; Zenati, Y.; Si-Ahmed, E.-K.; Legrand, J. Experimental Investigation of Sudden Expansion’s Influence on the Hydrodynamic Behavior of Different Sub-Regimes of Intermittent Flow. J. Pet. Sci. Eng. 2021, 205, 108834. [Google Scholar] [CrossRef]
- Der, O.; Bertola, V. An Experimental Investigation of Oil-Water Flow in a Serpentine Channel. Int. J. Multiph. Flow 2020, 129, 103327. [Google Scholar] [CrossRef]
- Kovalev, A.; Yagodnitsyna, A.; Bilsky, A. Plug Flow of Immiscible Liquids with Low Viscosity Ratio in Serpentine Microchannels. Chem. Eng. J. 2021, 417, 127933. [Google Scholar] [CrossRef]
- Auliano, D.; Auliano, M.; Næss, E. Towards a Better Understanding of Heat Transfer and Flow Mechanisms in a Cavity Channel with Numerical Simulations. Int. J. Therm. Sci. 2023, 193, 108531. [Google Scholar] [CrossRef]
- Auliano, D.; Auliano, M.; Næss, E. Near Wall Temperature Measurements and Turbulent Features in a Water Flow at Transition Reynolds Numbers in a Square Heated Asymmetric Cavity Channel. Therm. Sci. Eng. Prog. 2023, 37, 101541. [Google Scholar] [CrossRef]
- Iachachene, F.; Haddad, Z.; Arıcı, M.; Jamei, M.; Mataoui, A. Turbulent Forced Convective Flow in a Conical Diffuser: Hybrid and Single Nanofluids. Eng. Anal. Bound. Elem. 2023, 148, 205–219. [Google Scholar] [CrossRef]
- Moreira, T.A.; Fariñas Alvariño, P.; Cabezas-Gómez, L.; Ribatski, G. Experimental and Numerical Study of Slightly Loaded Water Alumina Nanofluids in the Developing Region of a 1.1 Mm in Diameter Pipe and Convective Enhancement Evaluation. Int. J. Heat Mass Transf. 2017, 115, 317–335. [Google Scholar] [CrossRef]
- Ali, A.M.; Angelino, M.; Rona, A. Numerical Analysis on the Thermal Performance of Microchannel Heat Sinks with Al2O3 Nanofluid and Various Fins. Appl. Therm. Eng. 2021, 198, 117458. [Google Scholar] [CrossRef]
- Ekiciler, R.; Çetinkaya, M.S.A. A Comparative Heat Transfer Study between Monotype and Hybrid Nanofluid in a Duct with Various Shapes of Ribs. Therm. Sci. Eng. Prog. 2021, 23, 100913. [Google Scholar] [CrossRef]
- Fluent, A. Ansys Fluent Theory Guide; ANSYS Inc.: Canonsburg, PA, USA, 2013; Volume 15317. [Google Scholar]
- do Nascimento, E.O.; de Freitas, E.A.; Lins, E.F.; Vaz, J.R.P. Performance Assessment of an Indalma Hydro-Turbine. SN Appl. Sci. 2020, 2, 2156. [Google Scholar] [CrossRef]
- Sadri, R.; Mallah, A.R.; Hosseini, M.; Ahmadi, G.; Kazi, S.N.; Dabbagh, A.; Yeong, C.H.; Ahmad, R.; Yaakup, N.A. CFD Modeling of Turbulent Convection Heat Transfer of Nanofluids Containing Green Functionalized Graphene Nanoplatelets Flowing in a Horizontal Tube: Comparison with Experimental Data. J. Mol. Liq. 2018, 269, 152–159. [Google Scholar] [CrossRef]
- Saffarian, M.R.; Moravej, M.; Doranehgard, M.H. Heat Transfer Enhancement in a Flat Plate Solar Collector with Different Flow Path Shapes Using Nanofluid. Renew. Energy 2020, 146, 2316–2329. [Google Scholar] [CrossRef]
- Roache, P.J. Quantification of Uncertainty in Computational Fluid Dynamics. Annu. Rev. Fluid Mech. 1997, 29, 123–160. [Google Scholar] [CrossRef]
- Wen, T.; Lu, L.; Zhang, S.; Zhong, H. Experimental Study and CFD Modelling on the Thermal and Flow Behavior of EG/Water ZnO Nanofluid in Multiport Mini Channels. Appl. Therm. Eng. 2021, 182, 116089. [Google Scholar] [CrossRef]
- do Nascimento, E.O.; Cardenas Contreras, E.M.; Cabezas-Gómez, L.; Bandarra Filho, E.P. Comparison of Experimental Results with Numerical Simulation of Thermal Performance in Car Radiator Using MWCNT/EG/Water Nanofluid. J. Braz. Soc. Mech. Sci. Eng. 2024, 46, 440. [Google Scholar] [CrossRef]
- Manca, O.; Nardini, S.; Ricci, D. A Numerical Study of Nanofluid Forced Convection in Ribbed Channels. Appl. Therm. Eng. 2012, 37, 280–292. [Google Scholar] [CrossRef]
- Petukhov, B.S. Heat Transfer and Friction in Turbulent Pipe Flow with Variable Physical Properties. Adv. Heat Transf. 1970, 6, 503–564. [Google Scholar]
- Gnielinski, V. Neue Gleichungen Für Den Wärme- Und Den Stoffübergang in Turbulent Durchströmten Rohren Und Kanälen. Forsch. Im Ingenieurwes 1975, 41, 8–16. [Google Scholar] [CrossRef]
- Dittus, F.W.; Boelter, L.M.K. Heat Transfer in Automobile Radiators of the Tubular Type. Int. Commun. Heat Mass Transf. 1985, 12, 3–22. [Google Scholar] [CrossRef]
- Blasius, H. Das Aehnlichkeitsgesetz bei Reibungsvorgängen in Flüssigkeiten. In Mitteilungen über Forschungsarbeiten auf dem Gebiete des Ingenieurwesens. Mitteilungen über Forschungsarbeiten auf dem Gebiete des Ingenieurwesens; Springer: Berlin/Heidelberg, Germany, 1913; Volume 131. [Google Scholar] [CrossRef]
- Xuan, Y.; Roetzel, W. Conceptions for Heat Transfer Correlation of Nanofluids. Int. J. Heat Mass Transf. 2000, 43, 3701–3707. [Google Scholar] [CrossRef]
- Pak, B.C.; Cho, Y.I. Hydrodynamic and Heat Transfer Study of Dispersed Fluids With Submicron Metallic Oxide Particle. Exp. Heat Transf. 1998, 11, 151–170. [Google Scholar] [CrossRef]
- Montazer, E.; Shafii, M.B.; Salami, E.; Muhamad, M.R.; Yarmand, H.; Gharehkhani, S.; Chowdhury, Z.Z.; Kazi, S.N.; Badarudin, A. Heat Transfer in Turbulent Nanofluids: Separation Flow Studies and Development of Novel Correlations. Adv. Powder Technol. 2020, 31, 3120–3133. [Google Scholar] [CrossRef]
- Ahmad, F.; Mahmud, S.; Ehsan, M.M.; Salehin, M. Numerical Assessment of Nanofluids in Corrugated Minichannels: Flow Phenomenon and Advanced Thermo-Hydrodynamic Analysis. Int. J. Thermofluids 2023, 20, 100449. [Google Scholar] [CrossRef]
- Amiri, A.; Shanbedi, M.; Rafieerad, A.R.; Rashidi, M.M.; Zaharinie, T.; Zubir, M.N.M.; Kazi, S.N.; Chew, B.T. Functionalization and Exfoliation of Graphite into Mono Layer Graphene for Improved Heat Dissipation. J. Taiwan Inst. Chem. Eng. 2017, 71, 480–493. [Google Scholar] [CrossRef]
- Montazer, E.; Yarmand, H.; Salami, E.; Muhamad, M.R.; Kazi, S.N.; Badarudin, A. A Brief Review Study of Flow Phenomena over a Backward-Facing Step and Its Optimization. Renew. Sustain. Energy Rev. 2018, 82, 994–1005. [Google Scholar] [CrossRef]
- Huminic, G.; Huminic, A. The Heat Transfer Performances and Entropy Generation Analysis of Hybrid Nanofluids in a Flattened Tube. Int. J. Heat Mass Transf. 2018, 119, 813–827. [Google Scholar] [CrossRef]
- Saleh, B.; Sundar, L.S. Entropy Generation and Exergy Efficiency Analysis of Ethylene Glycol-Water Based Nanodiamond + Fe3O4 Hybrid Nanofluids in a Circular Tube. Powder Technol. 2021, 380, 430–442. [Google Scholar] [CrossRef]
- Alklaibi, A.M.; Sundar, L.S.; Sousa, A.C.M. Experimental Analysis of Exergy Efficiency and Entropy Generation of Diamond/Water Nanofluids Flow in a Thermosyphon Flat Plate Solar Collector. Int. Commun. Heat Mass Transf. 2021, 120, 105057. [Google Scholar] [CrossRef]
- Hazbehian, M.; Mohammadiun, M.; Maddah, H.; Alizadeh, M. Analyses of Exergy Efficiency for Forced Convection Heat Transfer in a Tube with CNT Nanofluid under Laminar Flow Conditions. Heat Mass Transf. 2017, 53, 1503–1516. [Google Scholar] [CrossRef]
- Mazaheri, N.; Bahiraei, M.; Abdi Chaghakaboodi, H.; Moayedi, H. Analyzing Performance of a Ribbed Triple-Tube Heat Exchanger Operated with Graphene Nanoplatelets Nanofluid Based on Entropy Generation and Exergy Destruction. Int. Commun. Heat Mass Transf. 2019, 107, 55–67. [Google Scholar] [CrossRef]
- Samal, S.K.; Moharana, M.K. Thermo-Hydraulic and Entropy Generation Analysis of Recharging Microchannel Using Water-Based Graphene–Silver Hybrid Nanofluid. J. Therm. Anal. Calorim. 2021, 143, 4131–4148. [Google Scholar] [CrossRef]
- Ahammed, N.; Asirvatham, L.G.; Wongwises, S. Entropy Generation Analysis of Graphene–Alumina Hybrid Nanofluid in Multiport Minichannel Heat Exchanger Coupled with Thermoelectric Cooler. Int. J. Heat Mass Transf. 2016, 103, 1084–1097. [Google Scholar] [CrossRef]
- Syam Sundar, L. Experimental Study on the Thermophysical Properties, Heat Transfer, Thermal Entropy Generation and Exergy Efficiency of Turbulent Flow of ZrO2-Water Nanofluids. Alex. Eng. J. 2023, 65, 867–885. [Google Scholar] [CrossRef]
- Syam Sundar, L. Heat Transfer, Friction Factor and Exergy Efficiency Analysis of Nanodiamond-Fe3O4/Water Hybrid Nanofluids in a Tube with Twisted Tape Inserts. Ain Shams Eng. J. 2023, 14, 102068. [Google Scholar] [CrossRef]
- Mukherjee, S.; Poloju, V.; Mishra, P.C. Heat Transfer, Exergoeconomic Performance and Sustainability Impact of a Novel CuO + MgO + GO/Water Ternary Nanofluid. Appl. Therm. Eng. 2023, 235, 121391. [Google Scholar] [CrossRef]
- Sundar, L.S.; Shaik, F.; Sharma, K.V.; Punnaiah, V.; Sousa, A.C.M. The Second Law of Thermodynamic Analysis for Longitudinal Strip Inserted Nanodiamond-Fe3O4/Water Hybrid Nanofluids. Int. J. Therm. Sci. 2022, 181, 107721. [Google Scholar] [CrossRef]
- Syam Sundar, L.; Mesfin, S.; Venkata Ramana, E.; Said, Z.; Sousa, A.C.M. Experimental Investigation of Thermo-Physical Properties, Heat Transfer, Pumping Power, Entropy Generation, and Exergy Efficiency of Nanodiamond + Fe3O4/60:40% Water-Ethylene Glycol Hybrid Nanofluid Flow in a Tube. Therm. Sci. Eng. Prog. 2021, 21, 100799. [Google Scholar] [CrossRef]



| Parameter/Authorship | This Work | Ref. [9] | Difference |
|---|---|---|---|
| 14,064.71 [W/m·K] | 13,716.80 [W/m·K] | 2.54% | |
| 21.67 [kPa/m] | 20.84 [kPa/m] | 3.83% |
| Parameter | Value | Difference |
|---|---|---|
| Nuavg (CFD) | 155.55 [–] | – |
| Nuavg (Petukhov) | 153.91 [–] | 1.05 [%] |
| Nuavg (Gnielinski) | 149.67 [–] | 3.78 [%] |
| Nuavg (Dittus-Boelter) | 145.53 [–] | 6.44 [%] |
| f (CFD) | 0.02636 [–] | – |
| f (Petukhov) | 0.02615 [–] | 0.80 [%] |
| f (Blasius) | 0.02660 [–] | 0.91 [%] |
| Tout (CFD) | 20.60 [°C] | – |
| Tout (Theoretical) | 20.57 [°C] | 0.15 [%] |
| Fluid/Parameter | [J/(kg·K)] | [kg/m3] | [W/(m·K)] | [kg/(m·s)] |
|---|---|---|---|---|
| Water | 4183 | 998.20 | 0.5861 | 0.001002 |
| 0.1 | 4142 | 1007.7 | 0.6281 | 0.001018 |
| 0.3 | 4062 | 1026.7 | 0.6961 | 0.001040 |
| 0.5 | 3985 | 1045.7 | 0.8058 | 0.001066 |
| Authorship | Coolant | Concentration | Heat Flux | Reynolds Number | ECR | |
|---|---|---|---|---|---|---|
| [48] | ZrO2/water | 0 vol.% | – | ~19,700 | 1 | ~38% |
| [49] | Diamond-Fe3O4/water | 0 vol.% | – | ~19,900 | 1 | ~19% |
| [50] | CuO-MgO-GO/water | 0 vol.% | 5.36 kW/m2 | ~17,500 | 1 | ~18% |
| [51] | Diamond-Fe3O4/water | 0 vol.% | – | ~20,000 | 1 | ~19% |
| This Work | Ag/Water | 0 vol.% | 20 kW/m2 | 20011 | 1 | 41% |
| [49] | Diamond-Fe3O4/water | 0.1 vol.% | – | 20,900 | 1 | ~23% |
| [51] | Diamond-Fe3O4/water | 0.1 vol.% | – | ~19,500 | 1 | ~24% |
| This work | Ag/water | 0.1 vol.% | 20 kW/m2 | 20,011 | 1 | 44% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bandarra Filho, E.P.; do Nascimento, E.O.; Farooq, M.; Cabezas-Gómez, L. Numerical Investigation on Heat Transfer and Pressure Drop in Silver/Water Nanofluids Flowing Through Tubes with Variable Expansion–Contraction Ratios. Energies 2025, 18, 161. https://doi.org/10.3390/en18010161
Bandarra Filho EP, do Nascimento EO, Farooq M, Cabezas-Gómez L. Numerical Investigation on Heat Transfer and Pressure Drop in Silver/Water Nanofluids Flowing Through Tubes with Variable Expansion–Contraction Ratios. Energies. 2025; 18(1):161. https://doi.org/10.3390/en18010161
Chicago/Turabian StyleBandarra Filho, Enio Pedone, Erick Oliveira do Nascimento, Muhammad Farooq, and Luben Cabezas-Gómez. 2025. "Numerical Investigation on Heat Transfer and Pressure Drop in Silver/Water Nanofluids Flowing Through Tubes with Variable Expansion–Contraction Ratios" Energies 18, no. 1: 161. https://doi.org/10.3390/en18010161
APA StyleBandarra Filho, E. P., do Nascimento, E. O., Farooq, M., & Cabezas-Gómez, L. (2025). Numerical Investigation on Heat Transfer and Pressure Drop in Silver/Water Nanofluids Flowing Through Tubes with Variable Expansion–Contraction Ratios. Energies, 18(1), 161. https://doi.org/10.3390/en18010161







