Experimental Study on Two-Phase Countercurrent Flow Limitation in Horizontal Circular Pipes
Abstract
1. Introduction
| Authors | Test Fluid | Test Section | Conclusion |
|---|---|---|---|
| Wallis and Dobson (1973) [13] | Air–water | Rectangular channel: a = 0.0254, 0.089 m; b = 0.0254, 0.076–0.305 m | They presented a criterion for the occurrence of slug or plug flow in a horizontal rectangular channel. |
| Gardner (1983) [14] | Air–water | Circular pipe: D = 0.072 m | They developed a flooding criterion in horizontal countercurrent flow. |
| Bankoff et al. (1987) [15] | Steam–water | Rectangular channel: a = 0.095 m; b = 0.095 m | They developed a countercurrent flow regime map and explored the mechanism of hysteresis effects. |
| Ansari and Nariai (1989) [16] | Air–water | Rectangular channel: a = 0.05 m; b = 0.1 m | They observed three zones during slug initiation. They proposed that the short wavelength waves create slugs. |
| Wang and Kondo (1990) [3] | Air–water | Rectangular channel: a = 0.035 m; b = 0.02, 0.035, 0.05 m | They proposed an instability criterion including a viscous term. They observed various flow patterns under different void fractions. |
| Choi and No (1995) [17] | Air–water | Circular pipe: D = 0.04, 0.06, 0.07 m | They proposed two flooding mechanisms. They investigated the geometrical factors on flooding. |
| Chun et al. (2000) [18] | Steam–water, air–water | Circular pipe: D = 0.083 m | They studied the effect of steam condensation on CCFL. The gas flow rate required for the occurrence of CCFL for steam–water countercurrent flow is larger than that for air–water countercurrent flow. They found that the condensation effect on CCFL increases when the system pressure, the pipe diameter, or the subcooling is increased. |
| Gargallo (2005) [19] | Air–water | Rectangular channel: a = 0.11 m; b = 0.09 m | They observed hydraulic jump and flow reversal. They proposed that subcritical flow is necessary for the onset of flow reversal. |
| Wintterle et al. 2008 [20] | Air–water | Rectangular channel: a = 0.11 m; b = 0.09 m | They discussed the velocity fields, velocity fluctuations, and void fraction distributions in countercurrent supercritical flow. |
| Ma et al. (2020) [9] | Air–water | Circular pipe: D = 0.02, 0.04, 0.07, 0.1, 0.13 m | They studied the effect of diameter on CCFL characteristics and proposed an empirical correlation applied to small-diameter pipes. |
| Dhar et al. (2022) [21] | Air–water | Rectangular channel: a = 0.012 m; b = 0.05 m | They investigated the effect of hydraulic jump on flow regime transition. |
2. Experimental Setup
3. Results and Discussion
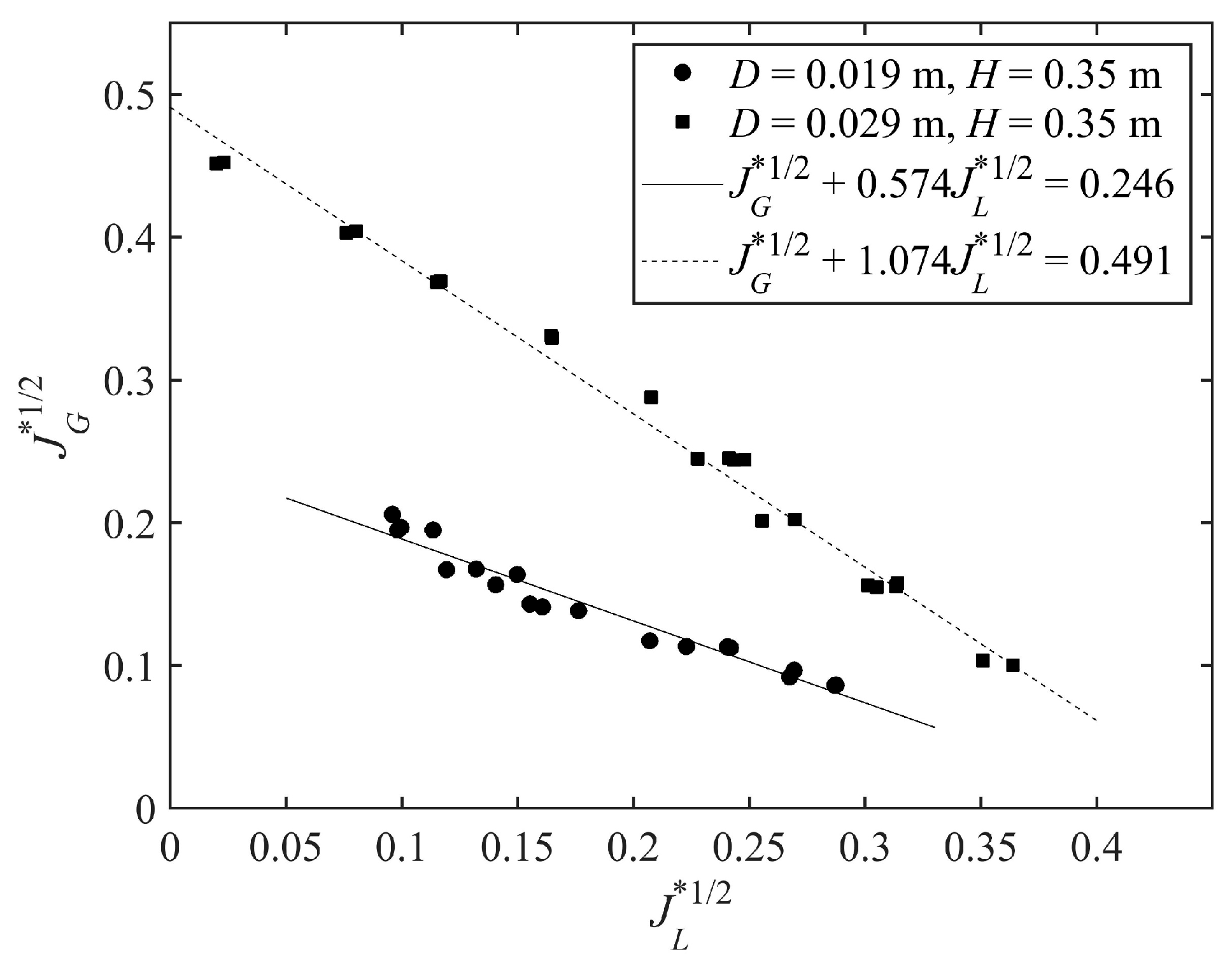
3.1. CCFL Characteristics
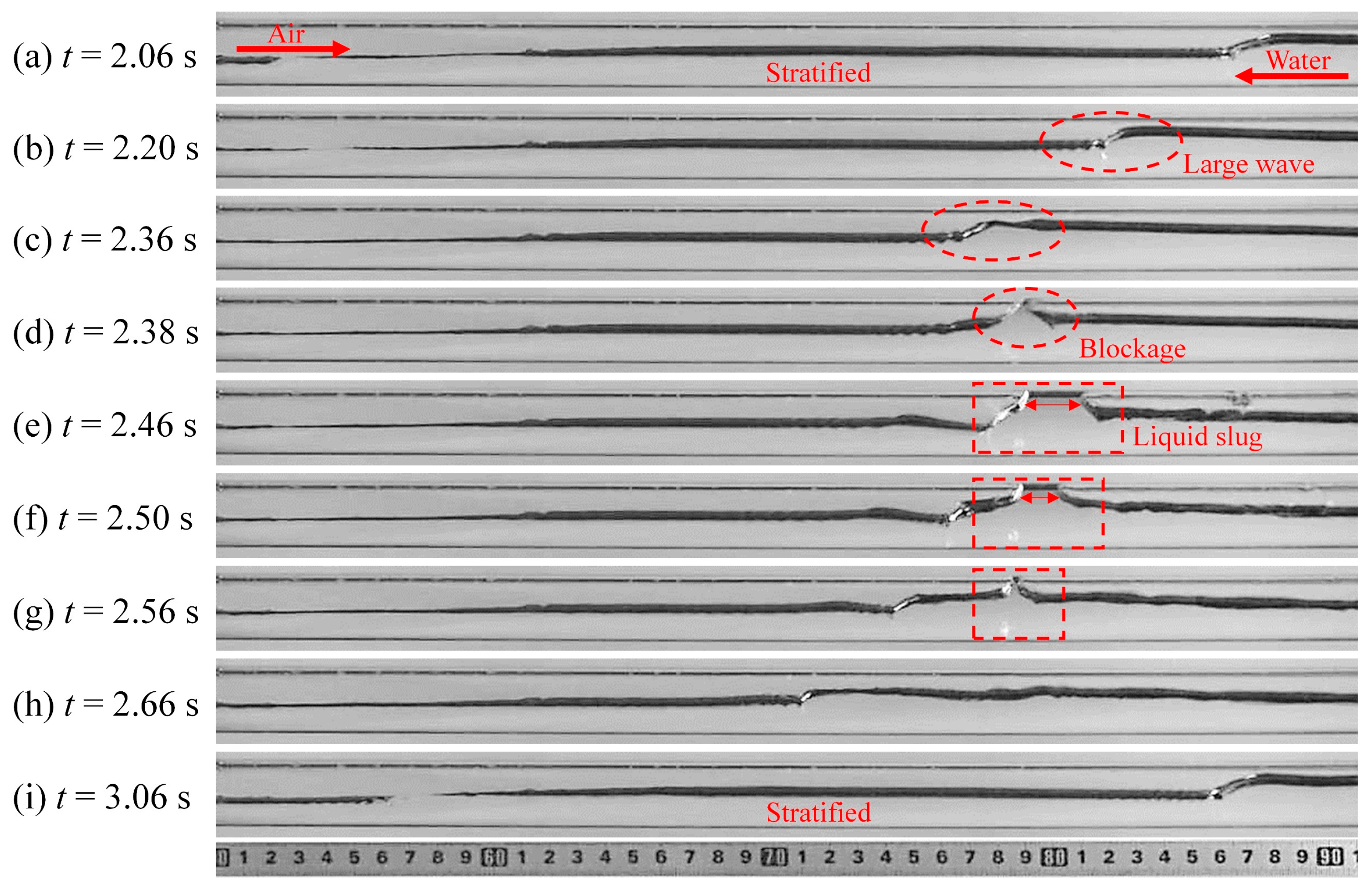
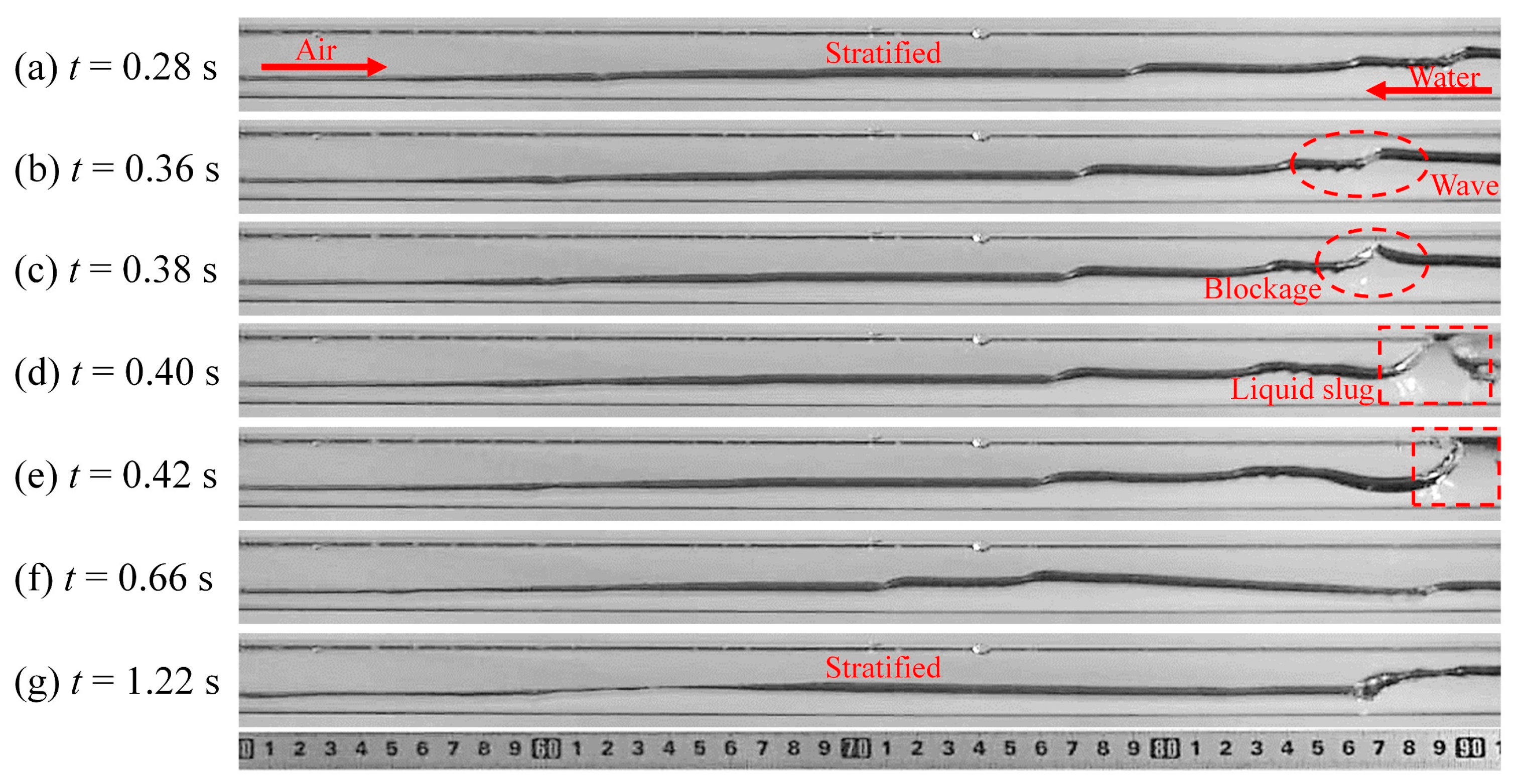
3.2. Flow Behaviors
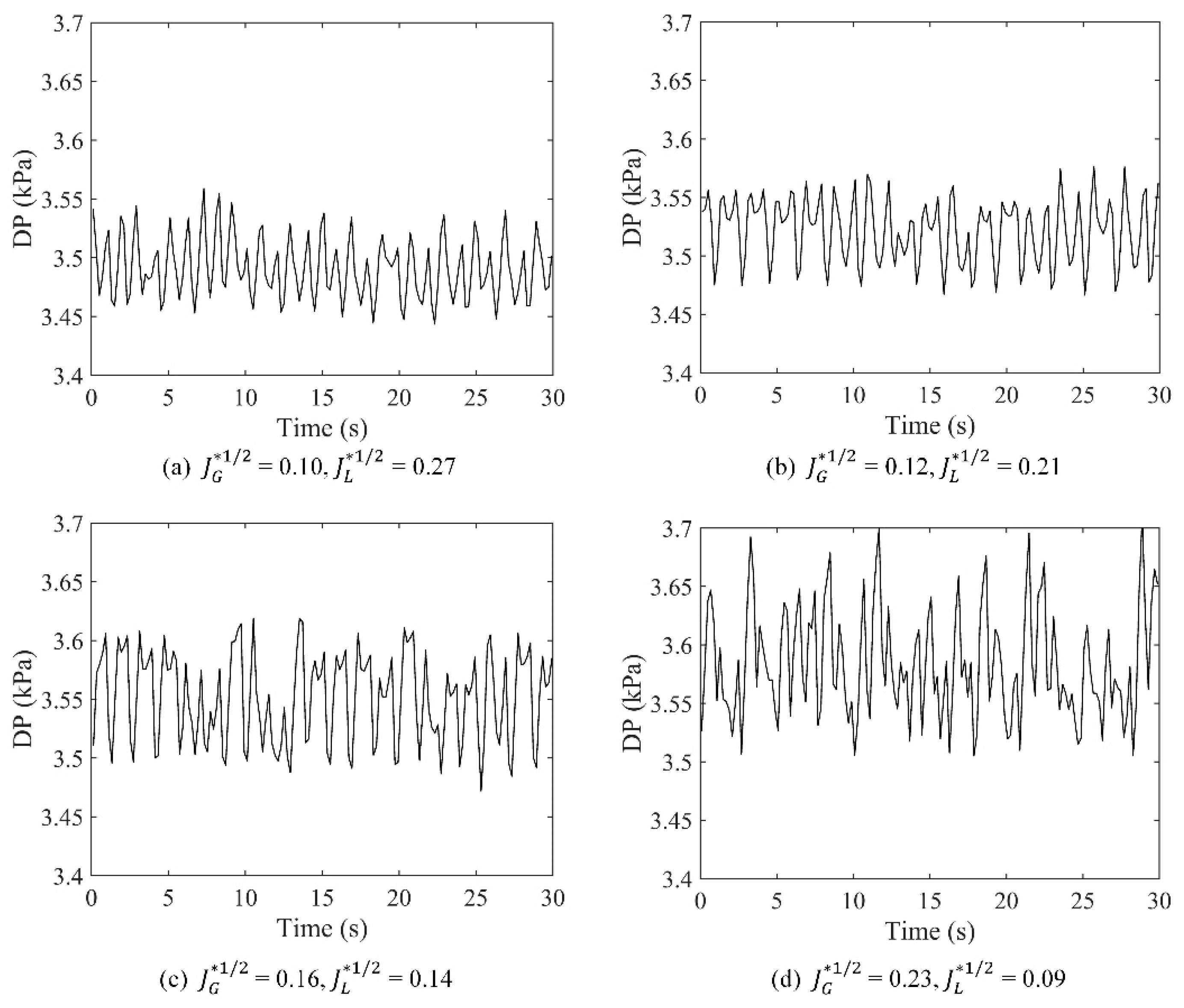
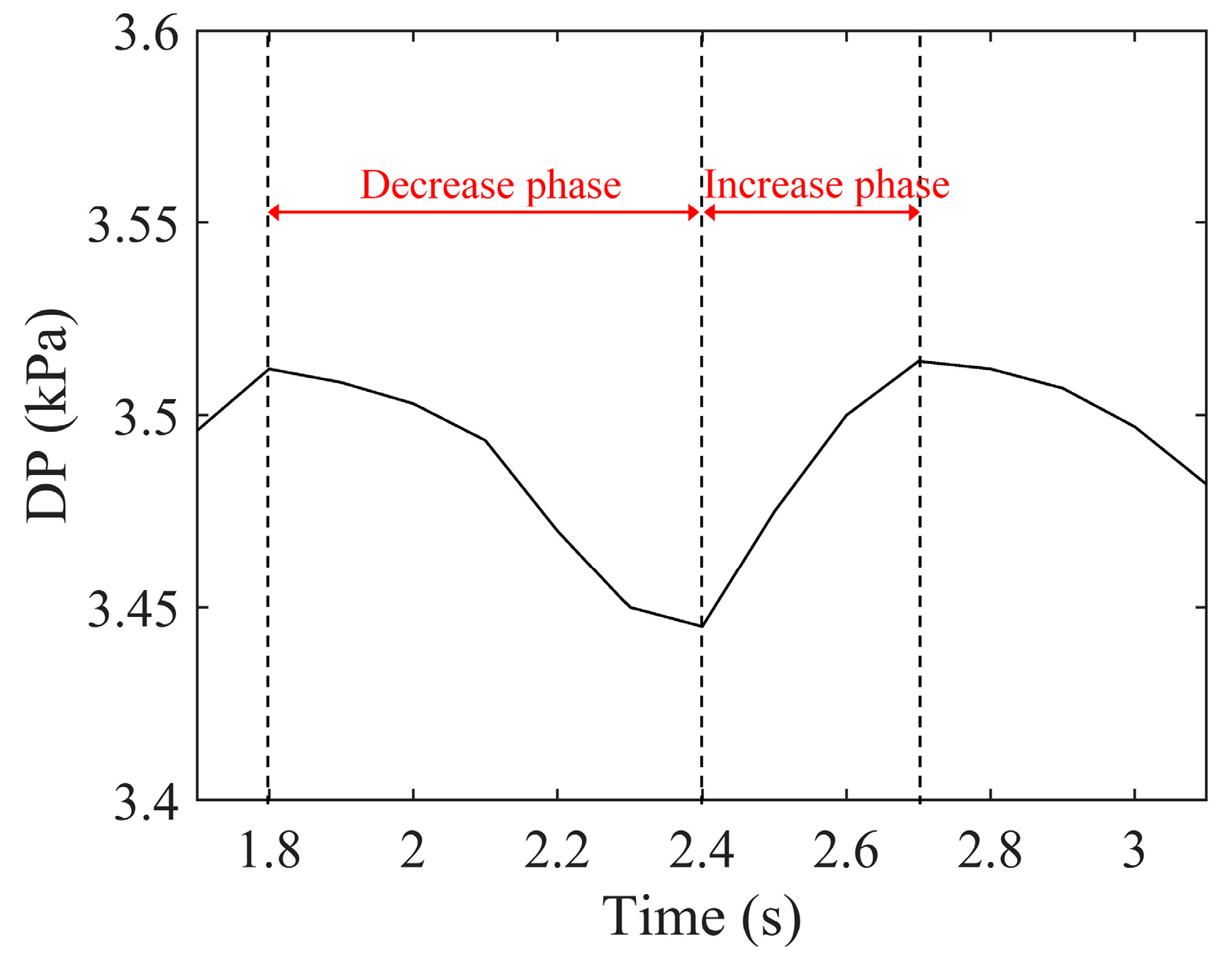
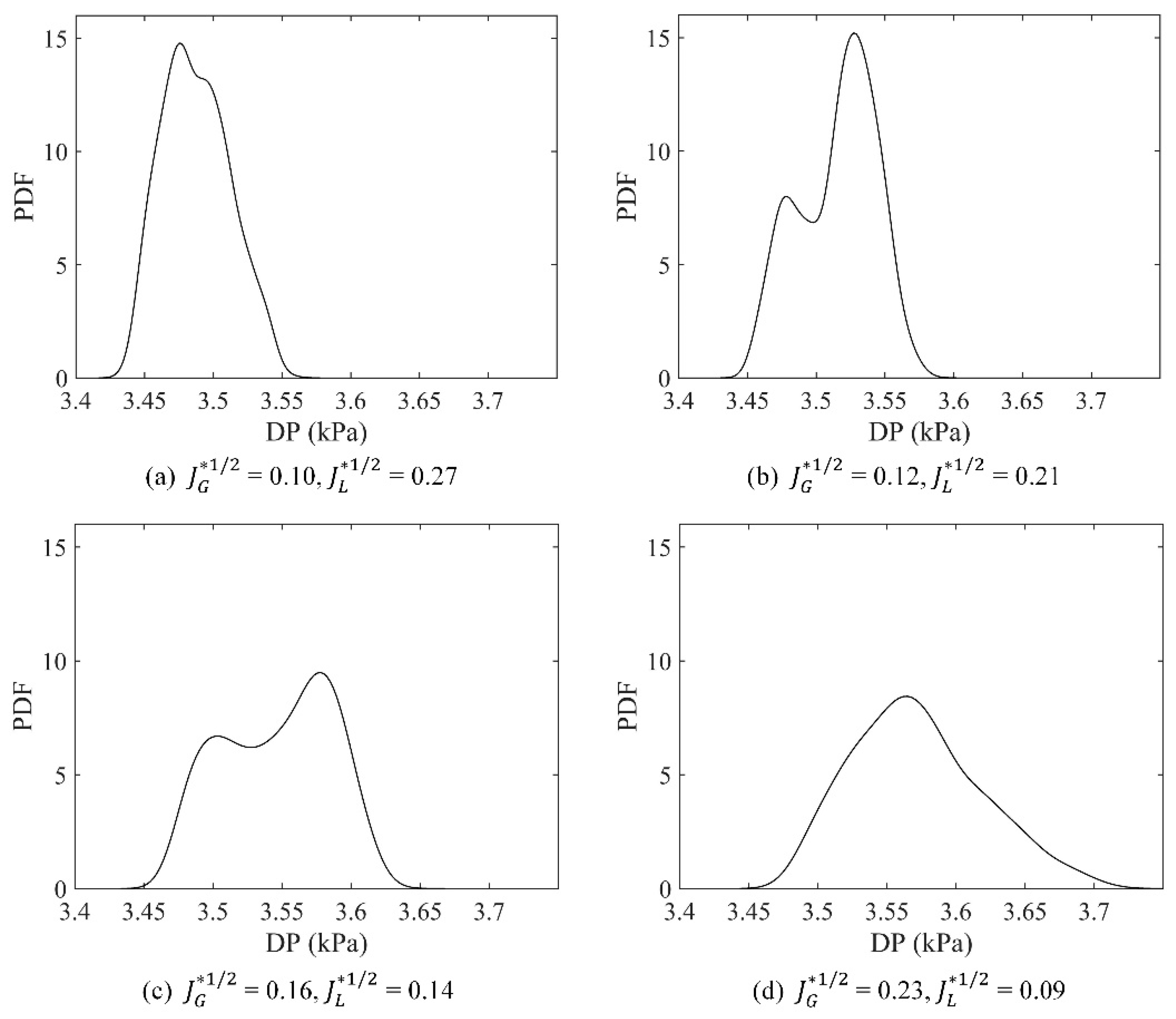
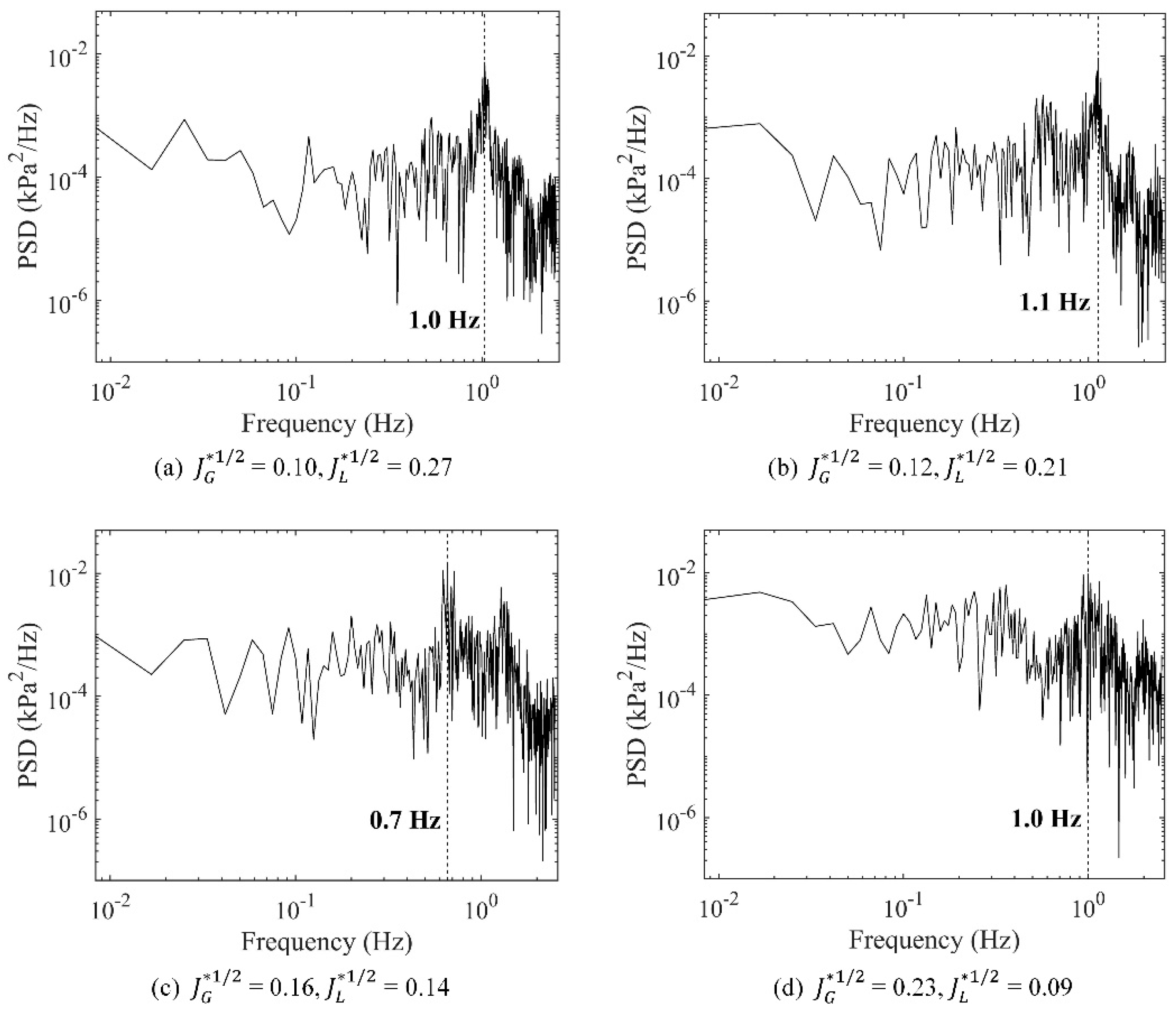
3.3. Pressure Signal Analysis
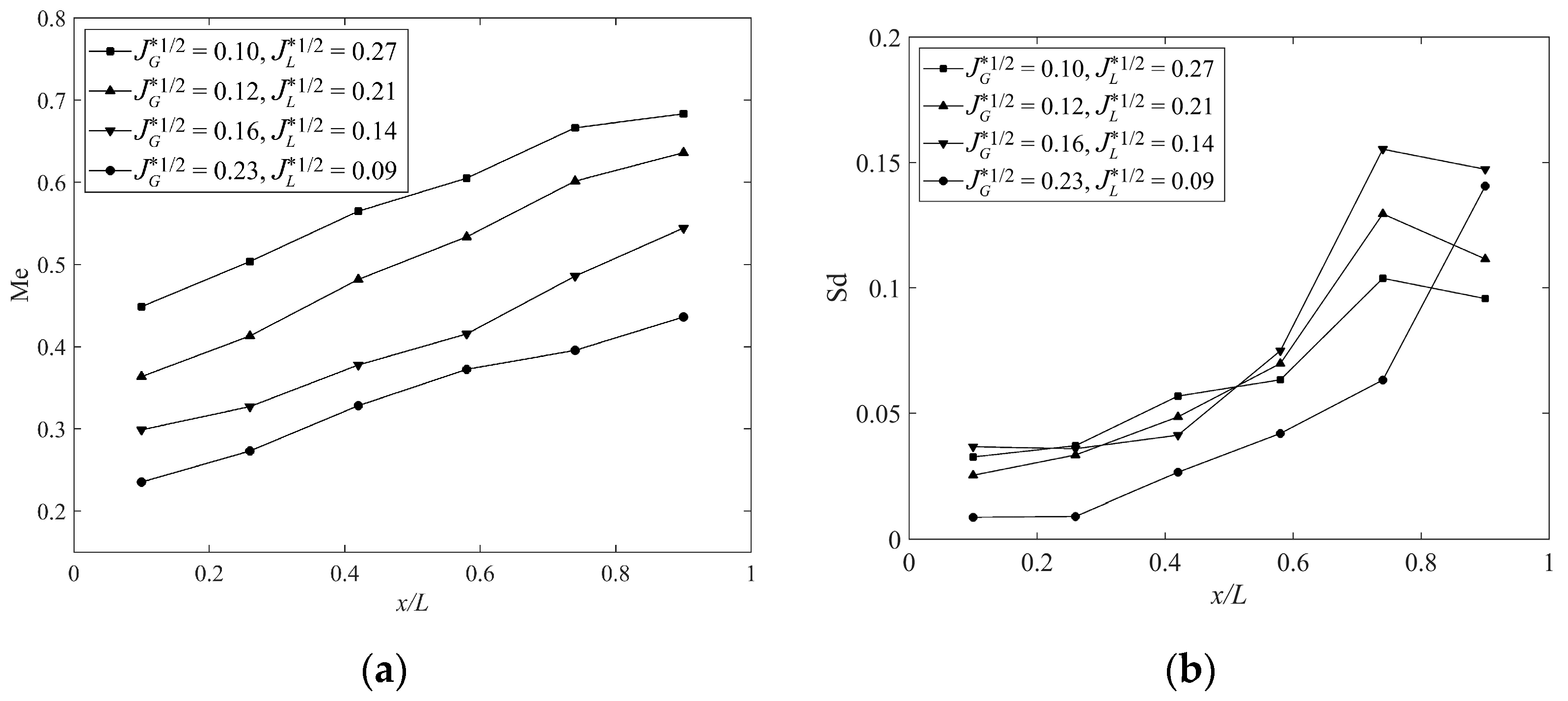
3.4. Liquid Holdup Data Analysis
4. Conclusions
- (1)
- The CCFL characteristics for horizontal pipes with small diameters can be well correlated using the dimensionless parameter . The CCFL characteristics are significantly affected by pipe diameter and are slightly affected by the water head above the horizontal pipe. The flooding liquid velocity increases as the pipe diameter increases. The ZLP point is higher for larger-diameter pipes.
- (2)
- During the CCFL experiment, the gas–liquid interface fluctuates within certain periods, and flow pattern transitions happen in the horizontal countercurrent flow. As the air flow rate increases, the occurrence location of the liquid slug appears to shift towards the water entrance, and the formation process of the liquid blockage is more severe, accompanied by the droplet entrainment from the liquid blockage.
- (3)
- For different flow rates, the average liquid holdup increases as the air flow rate decreases. The further away from the water entrance, the lower the average of liquid holdup. In addition, the dominant frequencies of the pressure signal and the liquid holdup are the same at the same flow rate.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| General Symbols | |
| a | width, m |
| b | height, m |
| D | inner diameter of pipe, m |
| g | acceleration of gravity, |
| H | water head above the horizontal pipe, m |
| j | superficial velocity, |
| dimensionless superficial velocity | |
| L | pipe length, m |
| Me | average |
| P | pixel distance |
| Sd | standard deviation |
| Greek alphabet | |
| void fraction | |
| viscosity, | |
| density, | |
| Superscript | |
| * | dimensionless |
| Subscript | |
| d | inner diameter of pipe |
| G | gas |
| h | water level height |
| K | gas/liquid |
| L | liquid |
| Abbreviations | |
| CCFL | countercurrent flow limitation |
| ECC | emergency core coolant |
| LOCA | loss of coolant accident |
| probability density function | |
| PSD | power spectral density |
| SBLOCA | small break loss of coolant accident |
| ZLP | zero liquid penetration |
References
- Al Issa, S.; Macian, R. A Review of CCFL Phenomenon. Ann. Nucl. Energy 2011, 38, 1795–1819. [Google Scholar] [CrossRef]
- Zhao, D.; Xu, C.; Wang, Z.; Zhu, X.; Li, Y.; Chi, X.; Wang, N. Countercurrent Flow Limitation in a Pipeline with an Orifice. Energies 2023, 16, 222. [Google Scholar] [CrossRef]
- Wang, H.; Kondo, S. A Study on the Stratified Horizontal Counter-Current Two-Phase Flow. Nucl. Eng. Des. 1990, 121, 45–52. [Google Scholar] [CrossRef]
- de Sampaio, P.A.B.; Faccini, J.L.H.; Su, J. Modelling of Stratified Gas-Liquid Two-Phase Flow in Horizontal Circular Pipes. Int. J. Heat Mass Transf. 2008, 51, 2752–2761. [Google Scholar] [CrossRef]
- Deendarlianto; Vallée, C.; Lucas, D.; Beyer, M.; Pietruske, H.; Carl, H. Experimental Study on the Air/Water Counter-Current Flow Limitation in a Model of the Hot Leg of a Pressurized Water Reactor. Nucl. Eng. Des. 2008, 238, 3389–3402. [Google Scholar] [CrossRef]
- Bankoff, S.G.; Lee, S.C. A Critical Review of the Flooding Literature. Multiph. Sci. Technol. 1986, 2, 95–180. [Google Scholar] [CrossRef]
- Pushkina, O.L.; Sorokin, Y.L. Breakdown of Liquid Film Motion in Vertical Tubes. Heat Transf. Sov. Res. 1969, 1, 56–64. [Google Scholar]
- Wallis, G.B.; Makkenchery, S. The Hanging Film Phenomenon in Vertical Annular Two-Phase Flow. J. Fluids Eng. 1974, 96, 297–298. [Google Scholar] [CrossRef][Green Version]
- Ma, Y.; Shao, J.; Lyu, J.; Peng, J. Experimental Study on the Effect of Diameter on Gas–Liquid CCFL Characteristics of Horizontal Circular Pipes. Nucl. Eng. Des. 2020, 364, 110645. [Google Scholar] [CrossRef]
- Drosos, E.I.P.; Paras, S.V.; Karabelas, A.J. Counter-Current Gas-Liquid Flow in a Vertical Narrow Channel-Liquid Film Characteristics and Flooding Phenomena. Int. J. Multiph. Flow 2006, 32, 51–81. [Google Scholar] [CrossRef]
- Liu, Y.; Upchurch, E.R.; Ozbayoglu, E.M. Experimental Study of Single Taylor Bubble Rising in Stagnant and Downward Flowing Non-Newtonian Fluids in Inclined Pipes. Energies 2021, 14, 578. [Google Scholar] [CrossRef]
- Liu, Y.; Mitchell, T.; Upchurch, E.R.; Ozbayoglu, E.M.; Baldino, S. Investigation of Taylor Bubble Dynamics in Annular Conduits with Counter-Current Flow. Int. J. Multiph. Flow 2024, 170, 104626. [Google Scholar] [CrossRef]
- Wallis, G.B.; Dodson, J.E. The Onset of Slugging in Horizontal Stratified Air-Water Flow. Int. J. Multiph. Flow 1973, 1, 173–193. [Google Scholar] [CrossRef]
- Gardner, G.C. Flooded Countercurrent Two-Phase Flow in Horizontal Tubes and Channels. Int. J. Multiph. Flow 1983, 9, 367–382. [Google Scholar] [CrossRef]
- Bankoff, S.G.; Lee, S.C. Flooding and Hysteresis Effects in Nearly-Horizontal Countercurrent Stratified Steam-Water Flow. Int. J. Heat Mass Transf. 1987, 30, 581–588. [Google Scholar] [CrossRef]
- Ansari, M.R.; Nariai, H. Experimental Investigation on Wave Initiation and Slugging of Air-Water Stratified Flow in Horizontal Duct. J. Nucl. Sci. Technol. 1989, 26, 681–688. [Google Scholar] [CrossRef]
- Choi, K.Y.; No, H.C. Experimental Studies of Flooding in Nearly Horizontal Pipes. Int. J. Multiph. Flow 1995, 21, 419–436. [Google Scholar] [CrossRef]
- Chun, M.H.; Yu, S.O. Effect of Steam Condensation on Countercurrent Flow Limiting in Nearly Horizontal Two-Phase Flow. Nucl. Eng. Des. 2000, 196, 201–217. [Google Scholar] [CrossRef]
- Gargallo, M.; Schulenberg, T.; Meyer, L.; Laurien, E. Counter-Current Flow Limitations during Hot Leg Injection in Pressurized Water Reactors. Nucl. Eng. Des. 2005, 235, 785–804. [Google Scholar] [CrossRef]
- Wintterle, T.; Laurien, E.; Stäbler, T.; Meyer, L.; Schulenberg, T. Experimental and Numerical Investigation of Counter-Current Stratified Flows in Horizontal Channels. Nucl. Eng. Des. 2008, 238, 627–636. [Google Scholar] [CrossRef]
- Dhar, M.; Ray, S.; Das, G.; Kumar Das, P. Hydraulic Jump Induced Flooding and Slugging in Stratified Gas-Liquid Flow—An Experimental Appraisal. Exp. Therm. Fluid Sci. 2022, 134, 110617. [Google Scholar] [CrossRef]
- Catrawedarma, I.G.N.B.; Deendarlianto; Indarto. Statistical Characterization of Flow Structure of Air–Water Two-Phase Flow in Airlift Pump–Bubble Generator System. Int. J. Multiph. Flow 2021, 138, 103596. [Google Scholar] [CrossRef]
- Wijayanta, S.; Indarto; Deendarlianto; Catrawedarma, I.G.N.B.; Hudaya, A.Z. Statistical Characterization of the Interfacial Behavior of the Sub-Regimes in Gas-Liquid Stratified Two-Phase Flow in a Horizontal Pipe. Flow Meas. Instrum. 2022, 83, 102107. [Google Scholar] [CrossRef]
- Fossa, M.; Guglielmini, G.; Marchitto, A. Two-Phase Flow Structure Close to Orifice Contractions during Horizontal Intermittent Flows. Int. Commun. Heat Mass Transf. 2006, 33, 698–708. [Google Scholar] [CrossRef]
- Rodrigues, R.L.P.; Cozin, C.; Naidek, B.P.; Marcelino Neto, M.A.; da Silva, M.J.; Morales, R.E.M. Statistical Features of the Flow Evolution in Horizontal Liquid-Gas Slug Flow. Exp. Therm. Fluid Sci. 2020, 119, 110203. [Google Scholar] [CrossRef]
- dos Reis, E.; Goldstein Leonardo, J. Characterization of Slug Flows in Horizontal Piping by Signal Analysis from a Capacitive Probe. Flow Meas. Instrum. 2010, 21, 347–355. [Google Scholar] [CrossRef]
- Montoya, G.A.; Deendarlianto; Lucas, D.; Höhne, T.; Vallée, C. Image-Processing-Based Study of the Interfacial Behavior of the Countercurrent Gas-Liquid Two-Phase Flow in a Hot Leg of a PWR. Sci. Technol. Nucl. Install. 2012, 2012, 209542. [Google Scholar] [CrossRef][Green Version]
- do Amaral, C.E.F.; Alves, R.F.; Da Silva, M.J.; Arruda, L.V.R.; Dorini, L.; Morales, R.E.M.; Pipa, D.R. Image Processing Techniques for High-Speed Videometry in Horizontal Two-Phase Slug Flows. Flow Meas. Instrum. 2013, 33, 257–264. [Google Scholar] [CrossRef]
- Badarudin, A.; Setyawan, A.; Dinaryanto, O.; Widyatama, A.; Indarto; Deendarlianto. Interfacial Behavior of the Air-Water Counter-Current Two-Phase Flow in a 1/30 Scale-down of Pressurized Water Reactor (PWR) Hot Leg. Ann. Nucl. Energy 2018, 116, 376–387. [Google Scholar] [CrossRef]
- Hudaya, A.Z.; Widyatama, A.; Dinaryanto, O.; Juwana, W.E.; Indarto; Deendarlianto. The Liquid Wave Characteristics during the Transportation of Air-Water Stratified Co-Current Two-Phase Flow in a Horizontal Pipe. Exp. Therm. Fluid Sci. 2019, 103, 304–317. [Google Scholar] [CrossRef]
- Zhu, X.; Xu, C.; Gu, M.; Tang, S.; Wang, N. Experimental Study on Air-Water Countercurrent Flow Limitation in Horizontal Pipes with Different Types of Obstructions. Prog. Nucl. Energy 2024, 169, 105087. [Google Scholar] [CrossRef]
- Deendarlianto; Höhne, T.; Lucas, D.; Vierow, K. Gas-Liquid Countercurrent Two-Phase Flow in a PWR Hot Leg: A Comprehensive Research Review. Nucl. Eng. Des. 2012, 243, 214–233. [Google Scholar] [CrossRef]
- Wallis, G.B. Flooding Velocities for Air and Water in Vertical Tubes; Atomic Energy Establishment: Harwell, UK, 1961.
- Ohnuki, A. Experimental Study of Counter-Current Two-Phase Flow in Horizontal Tube Connected to Inclined Riser. J. Nucl. Sci. Technol. 1986, 23, 219–232. [Google Scholar] [CrossRef][Green Version]
- Navarro, M.A. Study of Countercurrent Flow Limitation in a Horizontal Pipe Connected to an Inclined One. Nucl. Eng. Des. 2005, 235, 1139–1148. [Google Scholar] [CrossRef]
- Yu, J.; Zhang, D.; Shi, L.; Wang, Z.; Yan, S.; Dong, B.; Tian, W.; Su, G.H.; Qiu, S. Experimental Investigation of Air-Water CCFL in the Pressurizer Surge Line of AP1000. Nucl. Technol. 2016, 196, 614–640. [Google Scholar] [CrossRef]
- Yu, J.; Zhang, D.; Shi, L.; Wang, Z.; Tian, W.; Su, G.H.; Qiu, S.Z. Experimental Research on the Characteristics of Steam-Water Counter-Current Flow in the Pressurizer Surge Line Assembly. Exp. Therm. Fluid Sci. 2018, 96, 180–191. [Google Scholar] [CrossRef]
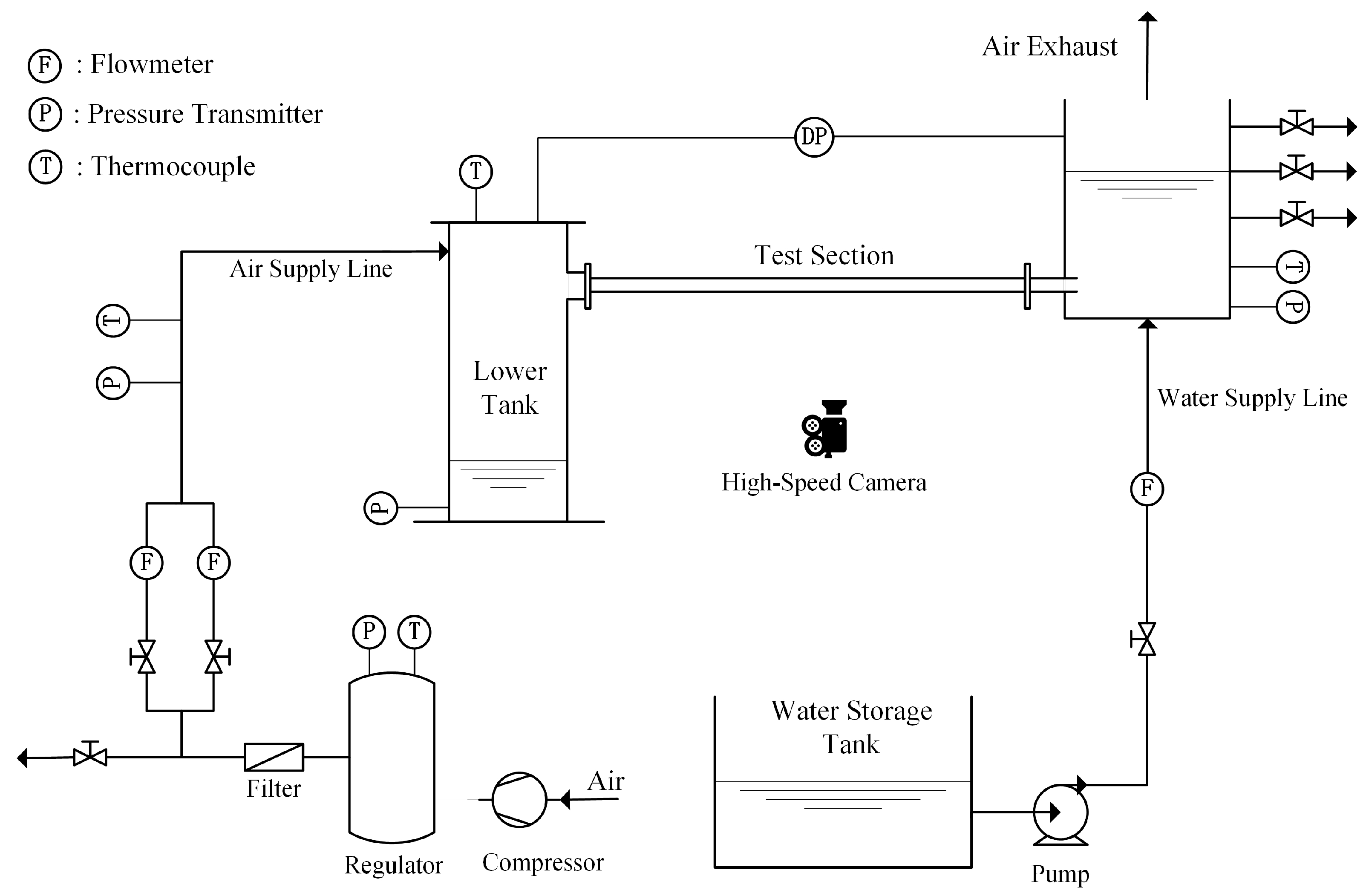





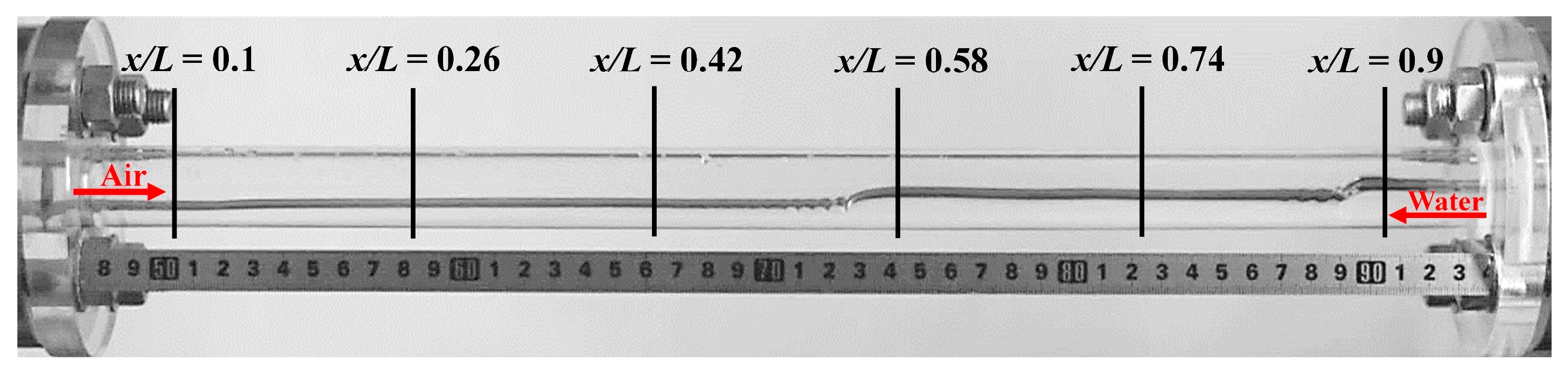

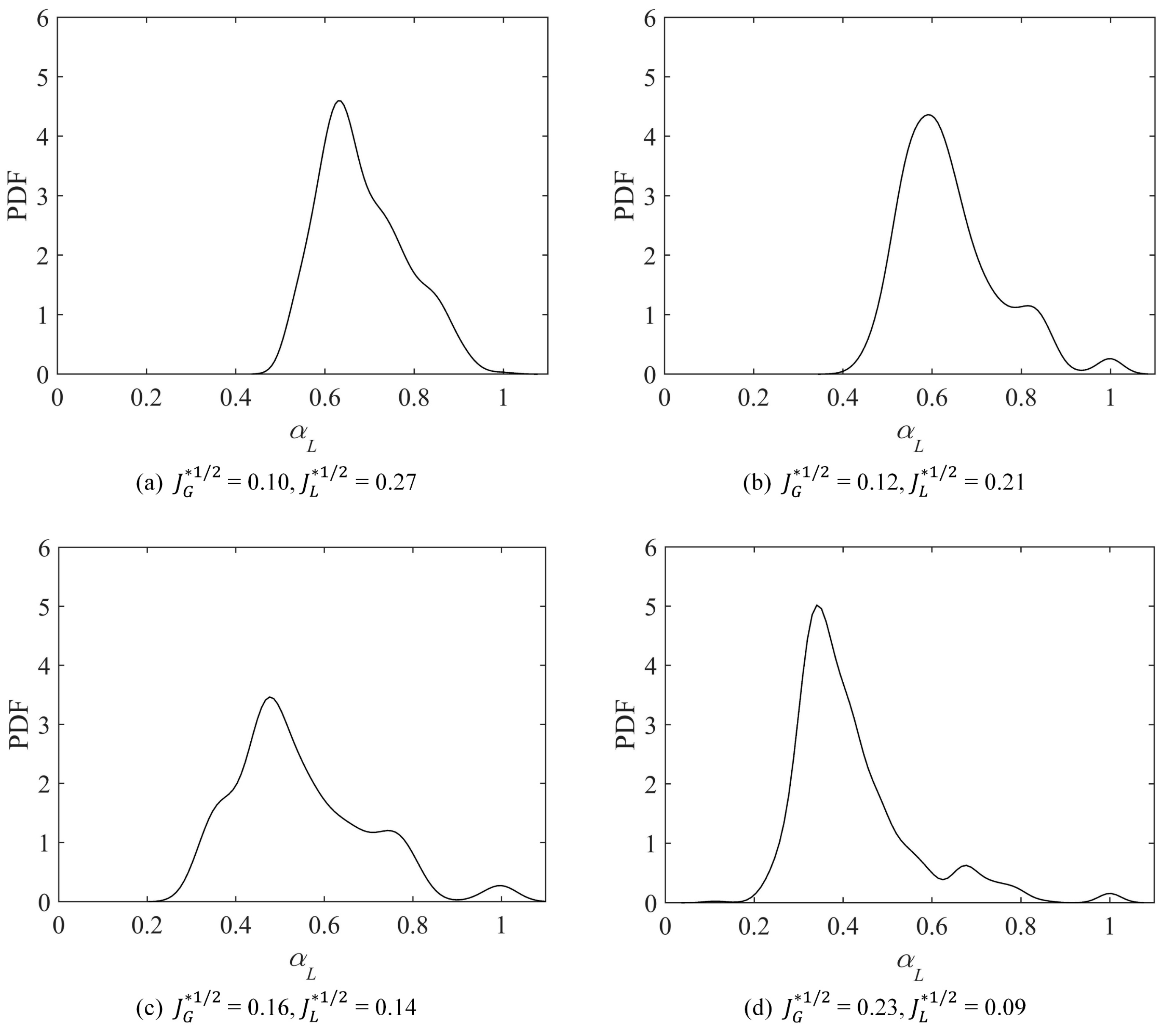
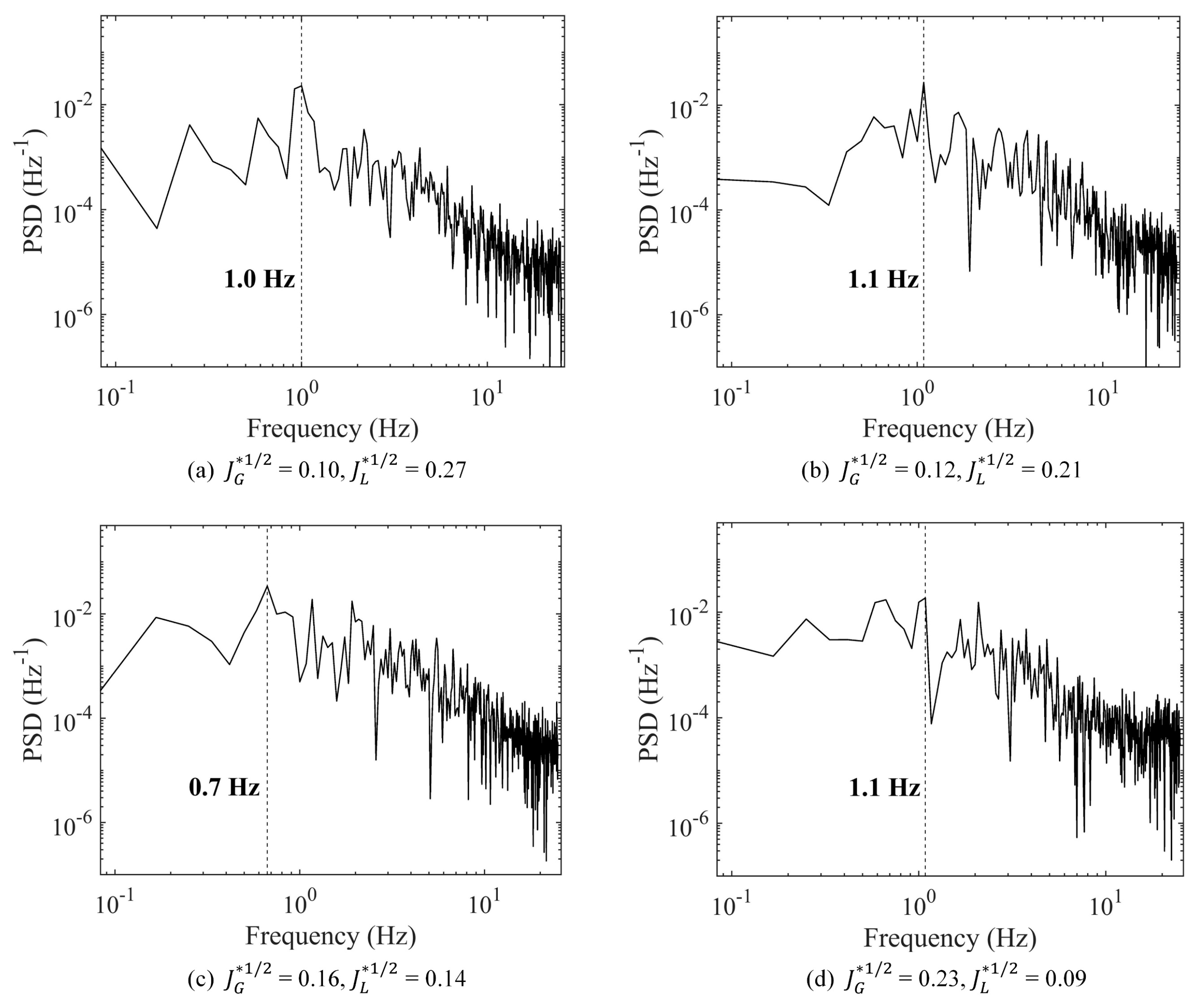
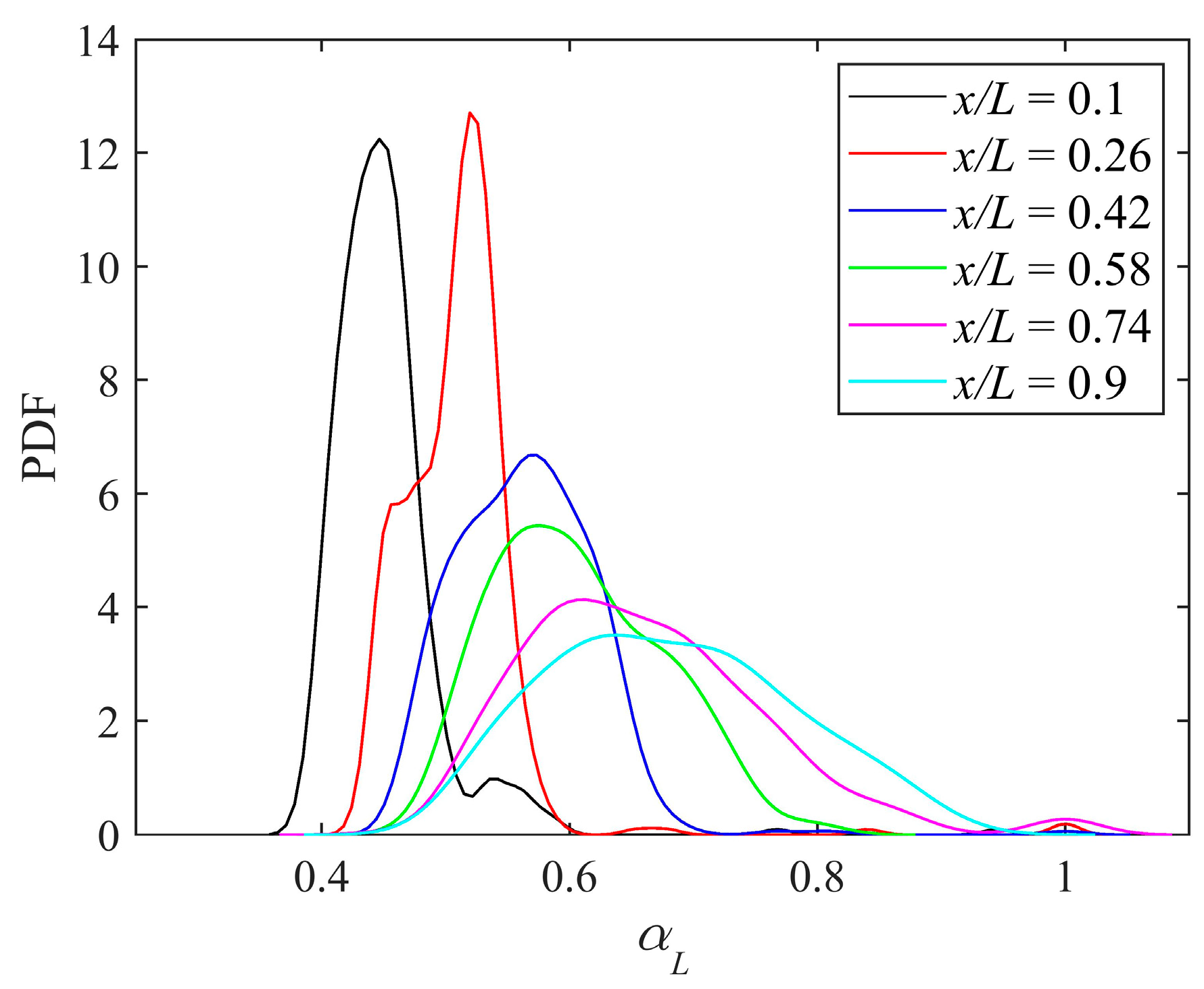
| Parameter | Values |
|---|---|
| L (m) | 0.5 |
| D (m) | 0.019, 0.029 |
| H (m) | 0.15, 0.35, 0.55 |
| (m/s) | 0.0929~0.5322 (0.019 m), 0.1549~3.1490 (0.029 m) |
| (m/s) | 0.0040~0.0357 (0.019 m), 0.0002~0.0706 (0.029 m) |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, X.; Xu, C.; Gu, M.; Wang, N. Experimental Study on Two-Phase Countercurrent Flow Limitation in Horizontal Circular Pipes. Energies 2024, 17, 2081. https://doi.org/10.3390/en17092081
Zhu X, Xu C, Gu M, Wang N. Experimental Study on Two-Phase Countercurrent Flow Limitation in Horizontal Circular Pipes. Energies. 2024; 17(9):2081. https://doi.org/10.3390/en17092081
Chicago/Turabian StyleZhu, Xixi, Chende Xu, Mingzhou Gu, and Naihua Wang. 2024. "Experimental Study on Two-Phase Countercurrent Flow Limitation in Horizontal Circular Pipes" Energies 17, no. 9: 2081. https://doi.org/10.3390/en17092081
APA StyleZhu, X., Xu, C., Gu, M., & Wang, N. (2024). Experimental Study on Two-Phase Countercurrent Flow Limitation in Horizontal Circular Pipes. Energies, 17(9), 2081. https://doi.org/10.3390/en17092081







