Optimally Splitting Solar Spectrums by Concentrating Solar Spectrums Splitter for Hydrogen Production via Solid Oxide Electrolysis Cell
Abstract
1. Introduction
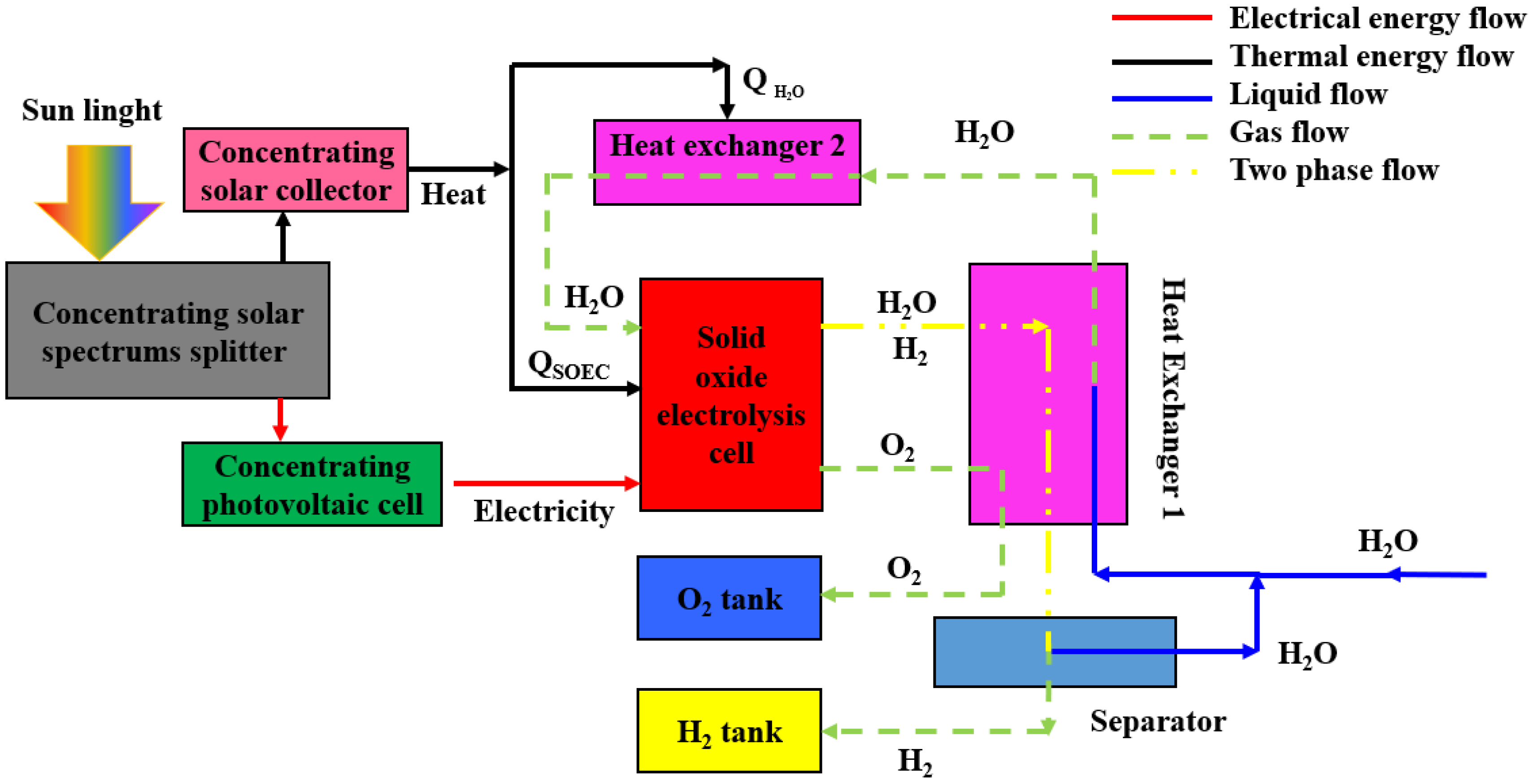
2. System Descriptions
- (1)
- The hybrid system operates continuously under stable and consistent conditions;
- (2)
- All wavelengths of sunlight entering the system are utilized [42];
- (3)
- Ideal gas properties are assumed for all the working gases [43];
- (4)
- The working fluids are considered homogeneous and continuous, and effects such as viscous heat dissipation, pressure drop, kinetic energy, and potential energy are neglected [44];
- (5)
- Negligible heat losses occur in the pipelines [45];
- (6)
- All electrochemical reactions in thermochemical cycles occur at the reaction temperature and with a pressure of 1 atm [46];
- (7)
- The increases in thermal conductance and electrical resistance resulting from the operation of the coupling system are negligible;
- (8)
- SOEC operates in isothermal mode [47];
- (9)
- Contact resistances are ignored [48].
2.1. Solid Oxide Electrolysis Cell
- (1)
- Activation overpotential
- (2)
- Concentration overpotential
- (3)
- Ohmic overpotential
2.2. Concentrating Solar Spectrums Splitter
2.2.1. Concentrating Photovoltaic
2.2.2. Concentrating Solar Collector
2.3. Solar Spectrums Splitting in the Hybrid System
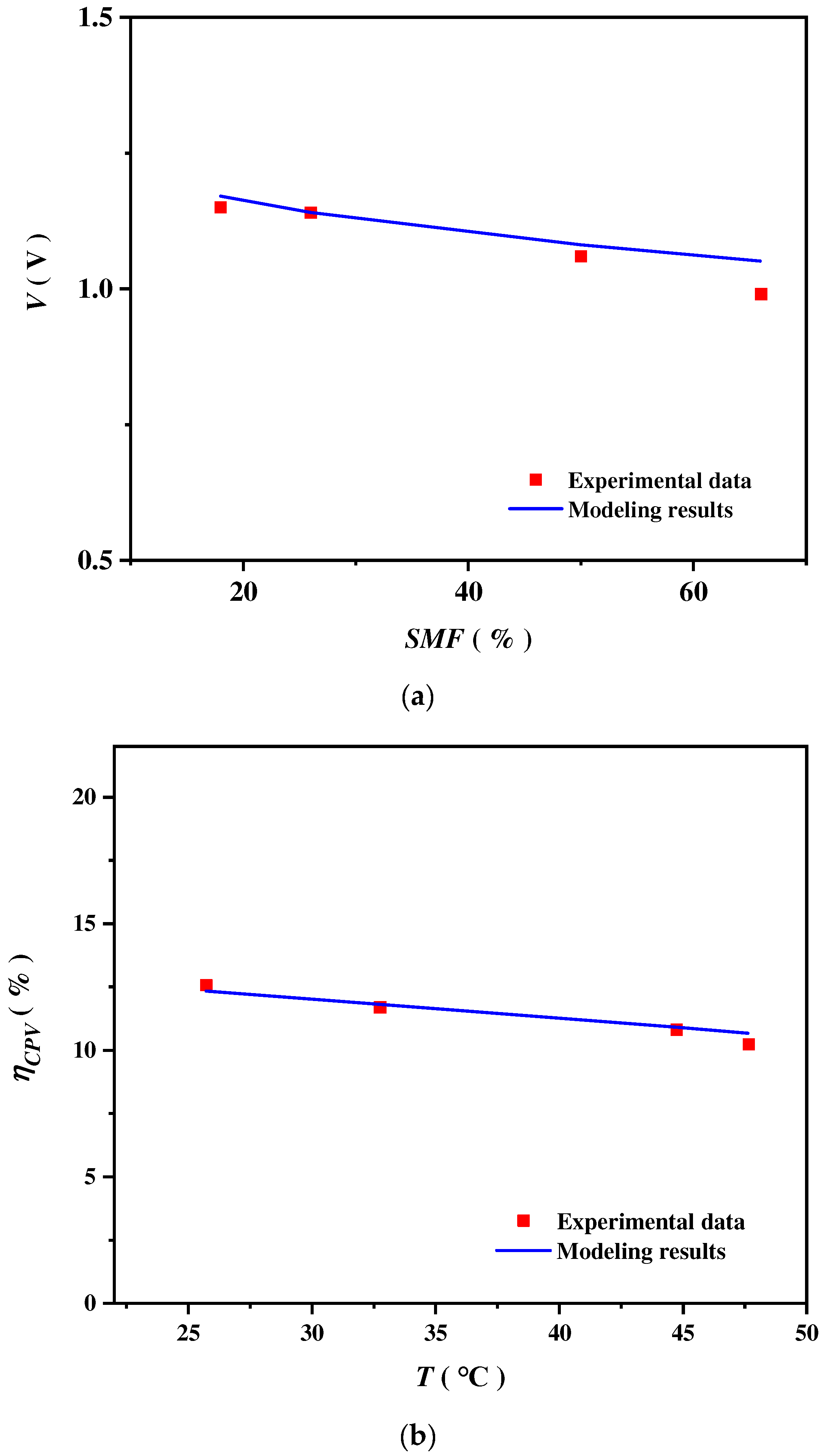
3. Model Validation
4. Results and Discussion
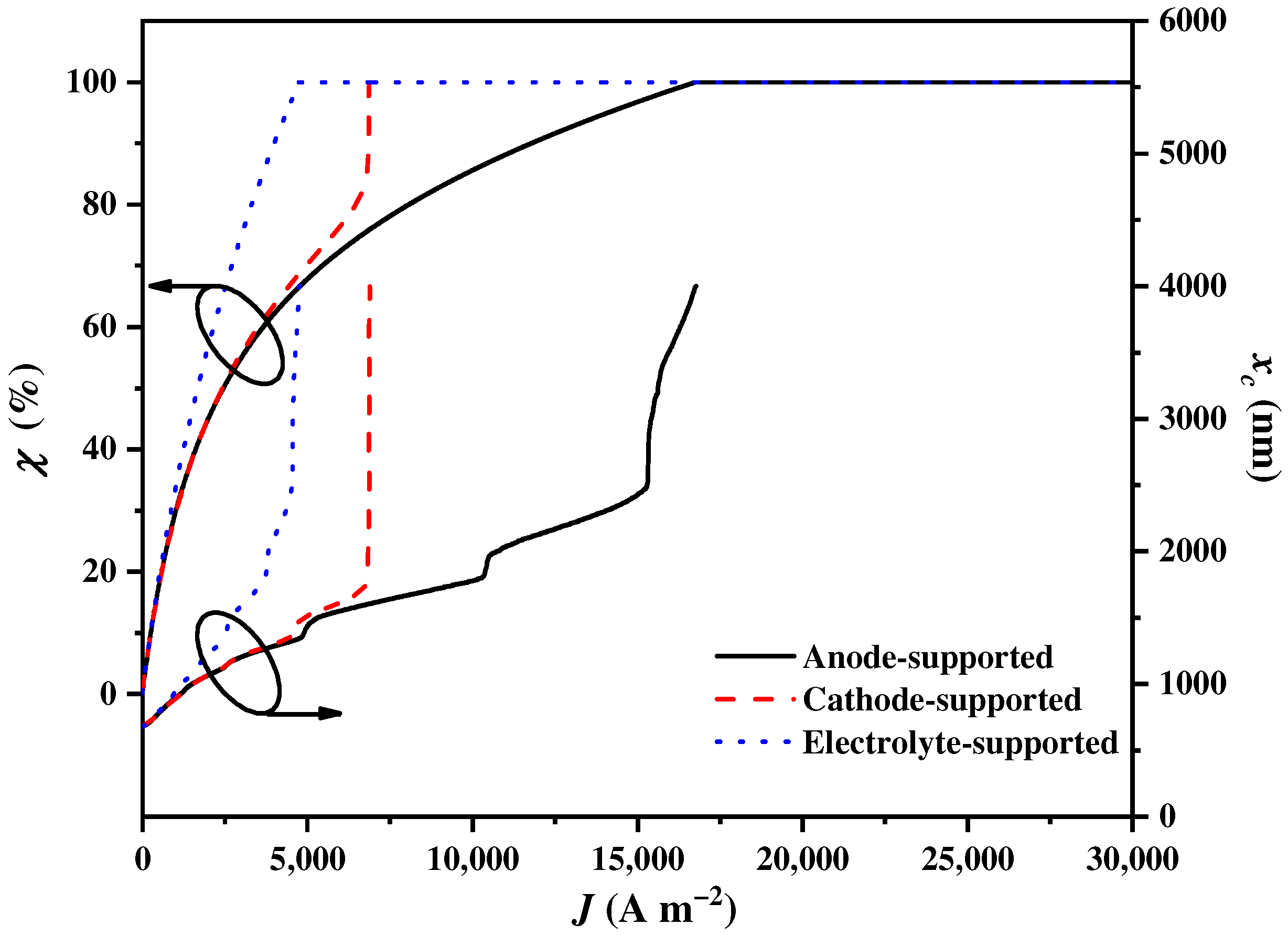
4.1. Influences of the SOEC Support Types
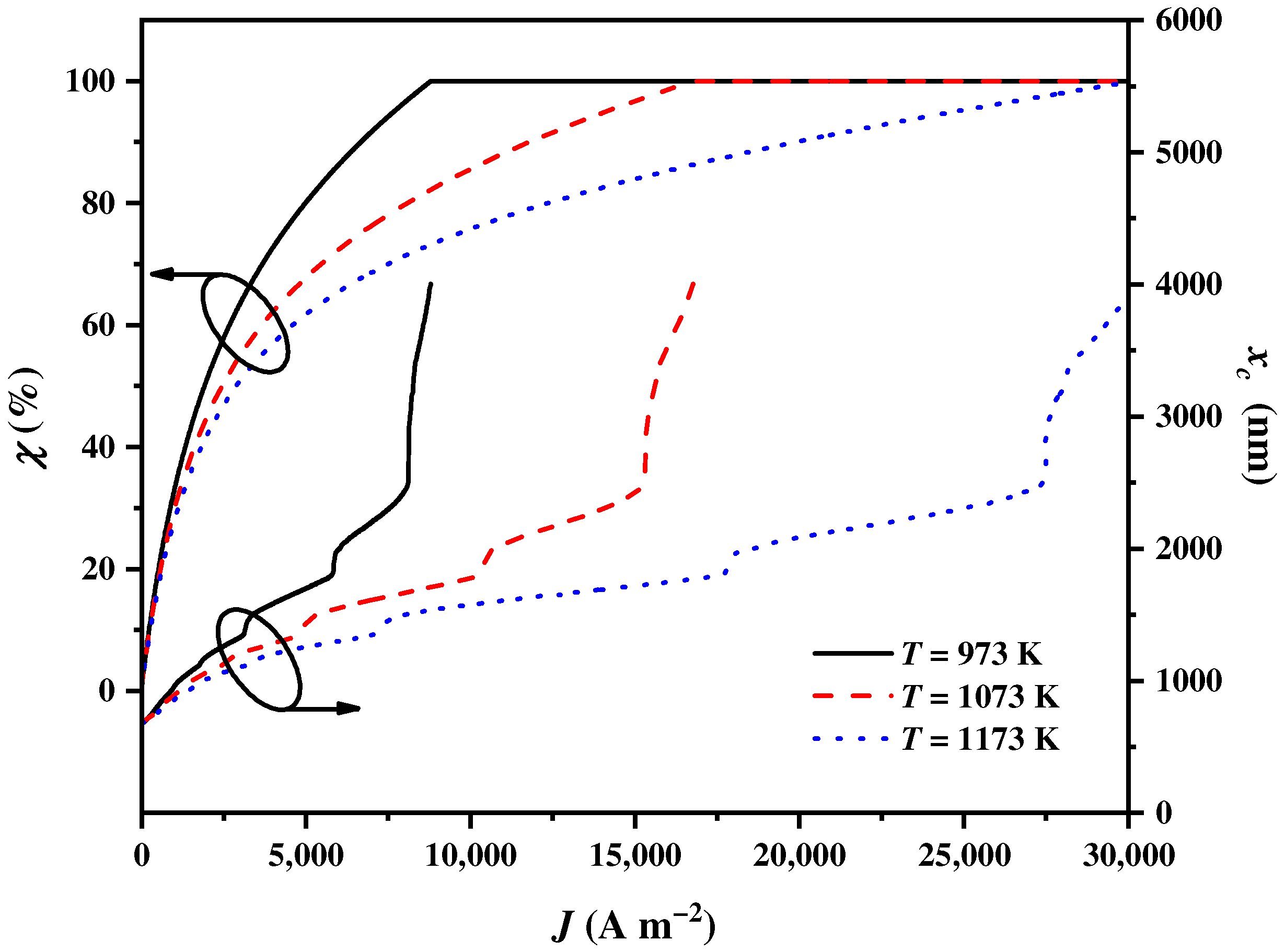
4.2. Influences of the SOEC Operating Temperature
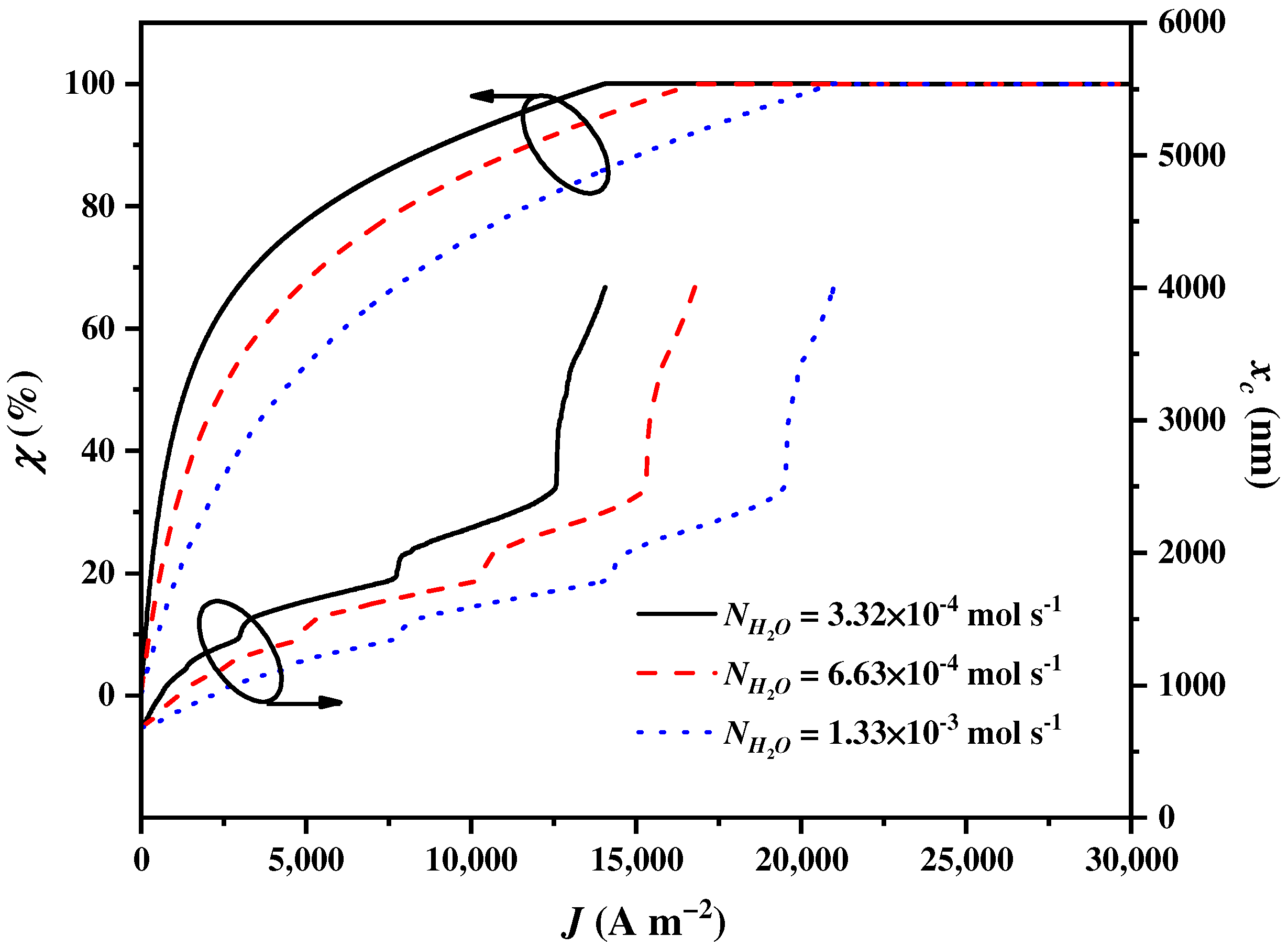
4.3. Influences of the SOEC Inlet Flow Rate of Water
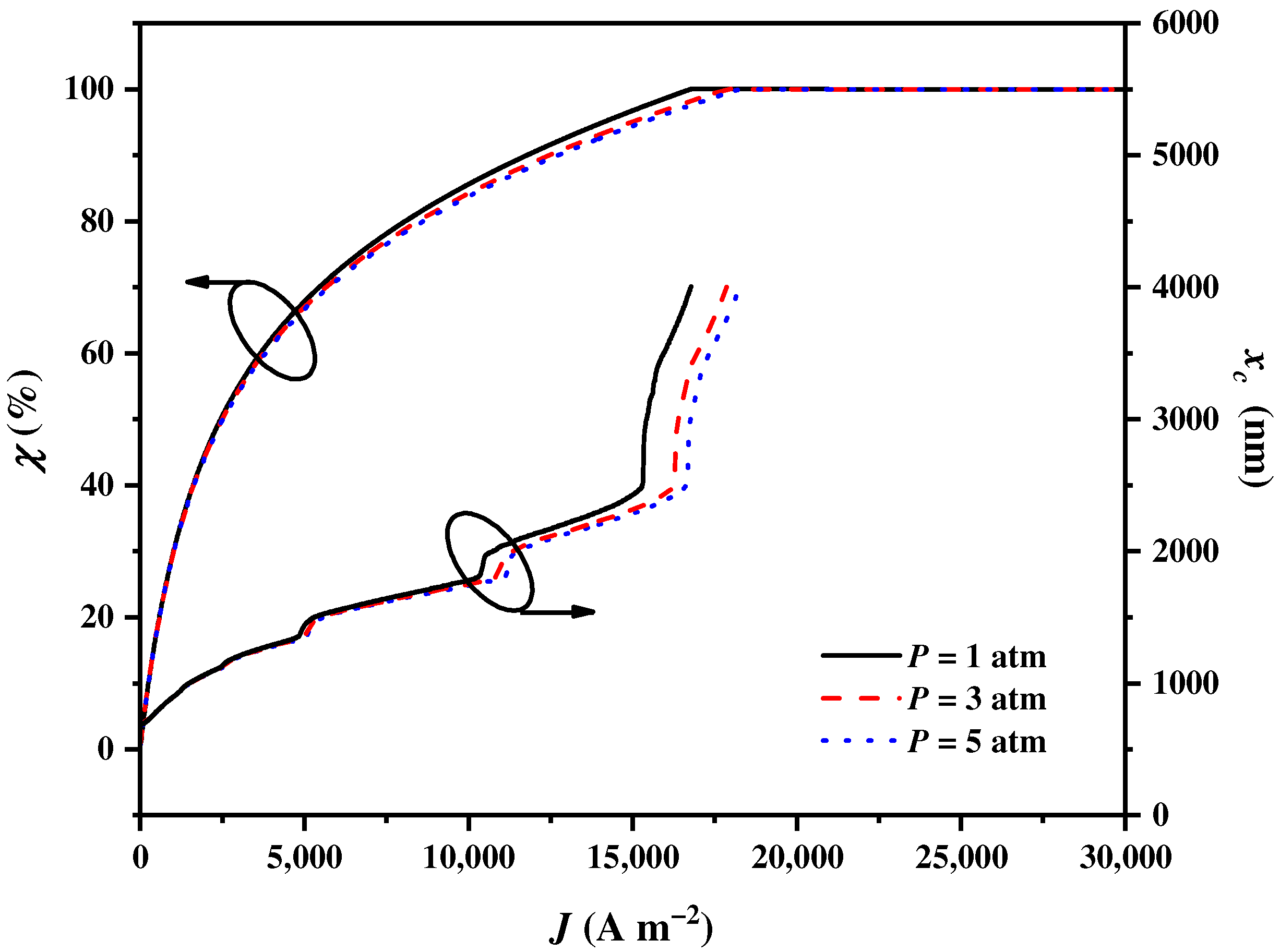
4.4. Influences of the SOEC Operating Pressure
4.5. Influences of the Effectiveness of Heat Exchangers
4.6. Influences of the CSC Operating Temperature
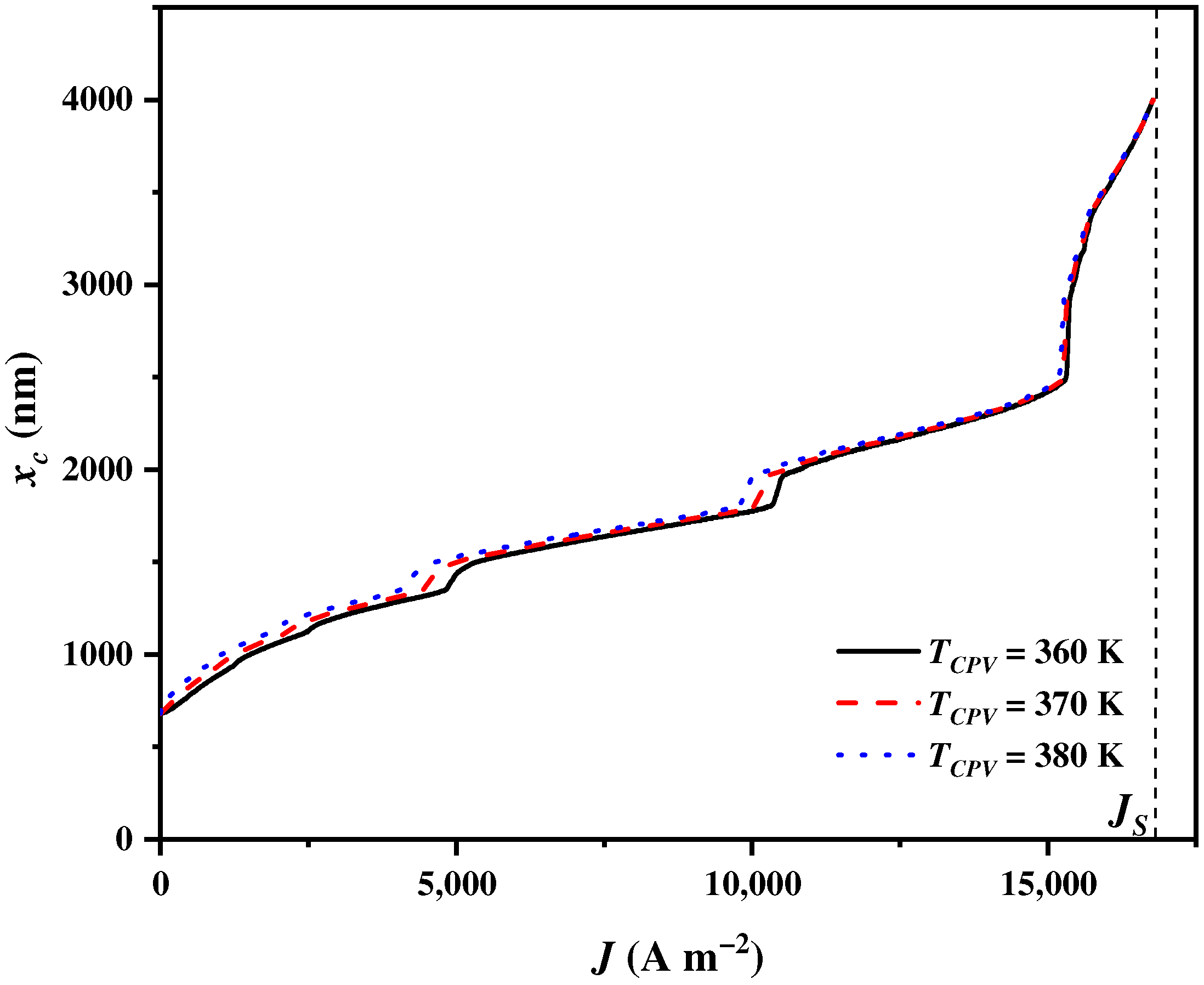
4.7. Influences of the CPV Operating Temperature
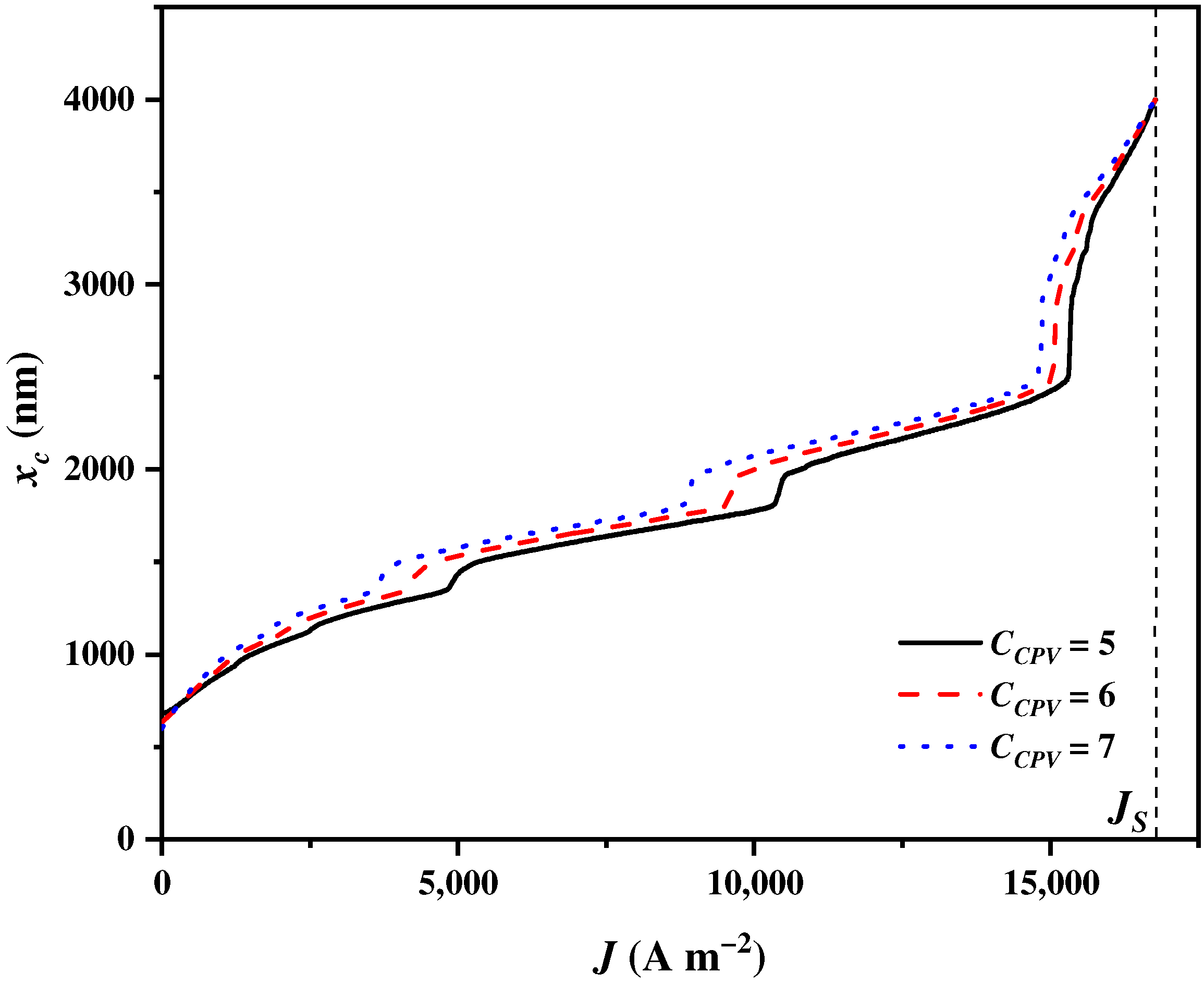
4.8. Influences of the CPV Optical Concentration Ratio
4.9. Influences of the CPV Electric Current
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Diode ideality factor | |
| Area of the concentrator | |
| Effective area of the CPV | |
| Effective surface area of the SOEC () | |
| Permeability () | |
| Optical concentration ratio of CPV | |
| Molar heat capacities of reactant/products () | |
| Effective diffusion coefficient of () | |
| Solar irradiance of AM1.5 direct spectrums () | |
| Solar irradiance of AM1.5 direct spectrum at () | |
| Equilibrium potential () | |
| Standard potential () | |
| Activation energy () | |
| Band-gap energy of semiconductor materials () | |
| Faraday constant () | |
| Gibbs energy () | |
| Solar radiation () | |
| Reference solar radiation () | |
| Enthalpy () | |
| Electric current through the SOEC () | |
| Diode reverse current () | |
| Output electric current of the CPV () | |
| Photocurrent of the CPV () | |
| Reverse saturation current at reference temperature () | |
| Short-circuit current of the CPV at reference temperature and solar radiation () | |
| Current density () | |
| Exchange current density () | |
| Boltzmann constant () | |
| Short-circuit current temperature coefficient | |
| Thickness () | |
| Latent heat of water under 1 bar () | |
| Number of moles | |
| Number of cells in parallel | |
| Number of cells in series | |
| Power output of CPV () | |
| Partial pressure of hydrogen () | |
| Partial pressure of steam () | |
| Partial pressure of oxygen () | |
| Input electric power of the SOEC () | |
| Charge of an electron () | |
| Heat () | |
| Energy received by the CPV () | |
| Energy received by the CSC () | |
| Heat required for heating water per unit time () | |
| Heat required for the SOSE per unit time () | |
| Total solar radiation energy () | |
| Gas constant () | |
| Intrinsic series resistance of the CPV () | |
| Entropy () | |
| Operating temperature of SOEC () | |
| Environment temperature () | |
| Boiling temperature of water () | |
| operating temperature of CPV () | |
| Temperature of the heat supplied by CSC () | |
| Surface temperature of the sun () | |
| Temperature of the heat supplied by the CSC or the SOEC () | |
| Potential () | |
| Output electric voltage of the CPV () | |
| Cut-off wavelength () | |
| Modeling results | |
| Experimental data | |
| Acronyms | |
| CPV | Concentrated photovoltaic |
| CSC | Concentrating solar collector |
| CSSS | Concentrating solar spectrums splitter |
| SMF | Steam molar fraction |
| SOEC | Solid oxide electrolysis cell |
| Greek symbols | |
| Balance parameter | |
| Rate of entropy () | |
| Pre-exponential factors () | |
| Energy efficiency | |
| Optical efficiency of the concentrator and filter | |
| Wavelength of sunlight () | |
| Dynamic viscosity () | |
| Rate of electrochemical reaction () | |
| Electric conductivity () | |
| Exergy efficiency | |
| Superscripts and subscripts | |
| Anode | |
| Activation | |
| Cathode | |
| Concentration | |
| Electrolyte | |
| Ohmic | |
References
- Acar, C.; Dincer, I. The potential role of hydrogen as a sustainable transportation fuel to combat global warming. Int. J. Hydrogen Energy 2020, 45, 3396–3406. [Google Scholar] [CrossRef]
- Asongu, S.A.; Agboola, M.O.; Alola, A.A.; Bekun, F.V. The criticality of growth, urbanization, electricity and fossil fuel consumption to environment sustainability in Africa. Sci. Total Environ. 2020, 712, 136376. [Google Scholar] [CrossRef] [PubMed]
- Lü, X.; Wu, Y.; Lian, J.; Zhang, Y.; Chen, C.; Wang, P.; Meng, L. Energy management of hybrid electric vehicles: A review of energy optimization of fuel cell hybrid power system based on genetic algorithm. Energy Convers. Manag. 2020, 205, 112474. [Google Scholar] [CrossRef]
- Cetinkaya, E.; Dincer, I.; Naterer, G.F. Life cycle assessment of various hydrogen production methods. Int. J. Hydrogen Energy 2012, 37, 2071–2080. [Google Scholar] [CrossRef]
- Balat, M. Potential importance of hydrogen as a future solution to environmental and transportation problems. Int. J. Hydrogen Energy 2008, 33, 4013–4029. [Google Scholar] [CrossRef]
- Muradov, N.Z.; Veziroğlu, T.N. “Green” path from fossil-based to hydrogen economy: An overview of carbon-neutral technologies. Int. J. Hydrogen Energy 2008, 33, 6804–6839. [Google Scholar] [CrossRef]
- Huang, H.; Lin, M. Dynamic behavior of solar thermochemical reactors for fuel generation: Modeling and control strategies. Energy Convers. Manag. 2022, 270, 116232. [Google Scholar] [CrossRef]
- Caliskan, H.; Dincer, I.; Hepbasli, A. Exergoeconomic and environmental impact analyses of a renewable energy based hydrogen production system. Int. J. Hydrogen Energy 2013, 38, 6104–6111. [Google Scholar] [CrossRef]
- Balthasar, W. Hydrogen production and technology: Today, tomorrow and beyond. Int. J. Hydrogen Energy 1984, 9, 649–668. [Google Scholar] [CrossRef]
- Lakhera, S.K.; Rajan, A.; Rugma, T.; Bernaurdshaw, N. A review on particulate photocatalytic hydrogen production system: Progress made in achieving high energy conversion efficiency and key challenges ahead. Renew. Sustain. Energy Rev. 2021, 152, 111694. [Google Scholar] [CrossRef]
- Erdemir, D.; Dincer, I. Development of solar-driven charging station integrated with hydrogen as an energy storage option. Energy Convers. Manag. 2022, 257, 115436. [Google Scholar] [CrossRef]
- Li, Y.; Wei, X.; Chen, L.; Shi, J. Electrocatalytic hydrogen production trilogy. Angew. Chem. Int. Ed. 2021, 60, 19550–19571. [Google Scholar] [CrossRef] [PubMed]
- Tong, W.; Forster, M.; Dionigi, F.; Dresp, S.; Sadeghi Erami, R.; Strasser, P.; Cowan, A.J.; Farràs, P. Electrolysis of low-grade and saline surface water. Nat. Energy 2020, 5, 367–377. [Google Scholar] [CrossRef]
- Kelly, N.A.; Gibson, T.L. Solar energy concentrating reactors for hydrogen production by photoelectrochemical water splitting. Int. J. Hydrogen Energy 2008, 33, 6420–6431. [Google Scholar] [CrossRef]
- Ngoh, S.K.; Njomo, D. An overview of hydrogen gas production from solar energy. Renew. Sustain. Energy Rev. 2012, 16, 6782–6792. [Google Scholar] [CrossRef]
- Satyapal, S.; Petrovic, J.; Read, C.; Thomas, G.; Ordaz, G. The US Department of Energy’s National Hydrogen Storage Project: Progress towards meeting hydrogen-powered vehicle requirements. Catal. Today 2007, 120, 246–256. [Google Scholar] [CrossRef]
- Udagawa, J.; Aguiar, P.; Brandon, N. Hydrogen production through steam electrolysis: Model-based steady state performance of a cathode-supported intermediate temperature solid oxide electrolysis cell. J. Power Sources 2007, 166, 127–136. [Google Scholar] [CrossRef]
- Demin, A.; Gorbova, E.; Tsiakaras, P. High temperature electrolyzer based on solid oxide co-ionic electrolyte: A theoretical model. J. Power Sources 2007, 171, 205–211. [Google Scholar] [CrossRef]
- Herring, J.S.; O’Brien, J.E.; Stoots, C.M.; Hawkes, G.; Hartvigsen, J.J.; Shahnam, M. Progress in high-temperature electrolysis for hydrogen production using planar SOFC technology. Int. J. Hydrogen Energy 2007, 32, 440–450. [Google Scholar] [CrossRef]
- Arashi, H.; Naito, H.; Miura, H. Hydrogen production from high-temperature steam electrolysis using solar energy. Int. J. Hydrogen Energy 1991, 16, 603–608. [Google Scholar] [CrossRef]
- Maka, A.O.; O’Donovan, T.S. A review of thermal load and performance characterisation of a high concentrating photovoltaic (HCPV) solar receiver assembly. Sol. Energy 2020, 206, 35–51. [Google Scholar] [CrossRef]
- Liu, J.; Liu, X.; Xin, J.; Zhang, Y.; Wen, L.; Liang, Q.; Miao, Z. Dual Function of the Third Component in Ternary Organic Solar Cells: Broaden the Spectrum and Optimize the Morphology. Small 2024, 2308863. [Google Scholar] [CrossRef]
- Zou, Y.; Qin, C.; Liu, H.; Zhang, B.; Wu, X. Concentrating photovoltaic systems: A review of temperature effects and components. J. Therm. Anal. Calorim. 2024, 149, 1301–1329. [Google Scholar] [CrossRef]
- Rajendran, D.R.; Ganapathy Sundaram, E.; Jawahar, P.; Sivakumar, V.; Mahian, O.; Bellos, E. Review on influencing parameters in the performance of concentrated solar power collector based on materials, heat transfer fluids and design. J. Therm. Anal. Calorim. 2020, 140, 33–51. [Google Scholar] [CrossRef]
- Mohammadi, A.; Mehrpooya, M. Techno-economic analysis of hydrogen production by solid oxide electrolyzer coupled with dish collector. Energy Convers. Manag. 2018, 173, 167–178. [Google Scholar] [CrossRef]
- Imenes, A.; Mills, D. Spectral beam splitting technology for increased conversion efficiency in solar concentrating systems: A review. Sol. Energy Mater. Sol. Cells 2004, 84, 19–69. [Google Scholar] [CrossRef]
- Joshi, A.S.; Dincer, I.; Reddy, B.V. Solar hydrogen production: A comparative performance assessment. Int. J. Hydrogen Energy 2011, 36, 11246–11257. [Google Scholar] [CrossRef]
- Edwards, J.; Badwal, S.; Duffy, G.; Lasich, J.; Ganakas, G. The application of solid state ionic technology for novel methods of energy generation and supply. Solid State Ion. 2002, 152, 843–852. [Google Scholar] [CrossRef]
- Mittelman, G.; Kribus, A. Innovative Solar Spectral Beam Splitting Concepts: Alternative Fuels Production. In Proceedings of the 35th European Photovoltaic Solar Energy Conference and Exhibition, Brussels, Belgium, 24–28 September 2018; pp. 1895–1898. [Google Scholar]
- Daneshpour, R.; Mehrpooya, M. Design and optimization of a combined solar thermophotovoltaic power generation and solid oxide electrolyser for hydrogen production. Energy Convers. Manag. 2018, 176, 274–286. [Google Scholar] [CrossRef]
- Kaleibari, S.S.; Yanping, Z.; Abanades, S. Solar-driven high temperature hydrogen production via integrated spectrally split concentrated photovoltaics (SSCPV) and solar power tower. Int. J. Hydrogen Energy 2019, 44, 2519–2532. [Google Scholar] [CrossRef]
- Gopalan, S.; Mosleh, M.; Hartvigsen, J.J.; McConnell, R.D. Analysis of self-sustaining recuperative solid oxide electrolysis systems. J. Power Sources 2008, 185, 1328–1333. [Google Scholar] [CrossRef]
- Lin, M.; Haussener, S. Techno-economic modeling and optimization of solar-driven high-temperature electrolysis systems. Sol. Energy 2017, 155, 1389–1402. [Google Scholar] [CrossRef]
- Thompson, J.R.; McConnell, R.D.; Mosleh, M. Cost Analysis of a Concentrator Photovoltaic Hydrogen Production System; National Renewable Energy Lab. (NREL): Golden, CO, USA, 2005.
- Mottaghizadeh, P.; Fardadi, M.; Jabbari, F.; Brouwer, J. Dynamics and control of a thermally self-sustaining energy storage system using integrated solid oxide cells for an islanded building. Int. J. Hydrogen Energy 2021, 46, 24891–24908. [Google Scholar] [CrossRef]
- Udagawa, J.; Aguiar, P.; Brandon, N. Hydrogen production through steam electrolysis: Control strategies for a cathode-supported intermediate temperature solid oxide electrolysis cell. J. Power Sources 2008, 180, 354–364. [Google Scholar] [CrossRef]
- Mahapatra, M.; Lu, K. Glass-based seals for solid oxide fuel and electrolyzer cells—A review. Mater. Sci. Eng. R Rep. 2010, 67, 65–85. [Google Scholar] [CrossRef]
- Liu, J.; Wang, J.; Tang, Y.; Jin, J.; Li, W. Solar photovoltaic–thermal hydrogen production system based on full-spectrum utilization. J. Clean. Prod. 2023, 430, 139340. [Google Scholar] [CrossRef]
- Baniasadi, E. Concurrent hydrogen and water production from brine water based on solar spectrum splitting: Process design and thermoeconomic analysis. Renew. Energy 2017, 102, 50–64. [Google Scholar] [CrossRef]
- Fang, J.; Yang, M.; Cui, L.; Yi, X.; Huo, H.; Wen, Z.; Liu, X. Efficient hydrogen production system with complementary utilization of methane and full-spectrum solar energy. Energy Convers. Manag. 2023, 283, 116951. [Google Scholar] [CrossRef]
- Zhang, H.; Lin, G.; Chen, J. Evaluation and calculation on the efficiency of a water electrolysis system for hydrogen production. Int. J. Hydrogen Energy 2010, 35, 10851–10858. [Google Scholar] [CrossRef]
- Guo, X.-Z.; Zhang, Y.-D.; Qin, D.; Luo, Y.-H.; Li, D.-M.; Pang, Y.-T.; Meng, Q.-B. Hybrid tandem solar cell for concurrently converting light and heat energy with utilization of full solar spectrum. J. Power Sources 2010, 195, 7684–7690. [Google Scholar] [CrossRef]
- Wang, C.; Chen, M.; Liu, M.; Yan, J. Dynamic modeling and parameter analysis study on reversible solid oxide cells during mode switching transient processes. Appl. Energy 2020, 263, 114601. [Google Scholar] [CrossRef]
- Zhao, Y.; Lu, M.; Li, Y.; Ge, M.; Xie, L.; Liu, L. Characteristics analysis of an exhaust thermoelectric generator system with heat transfer fluid circulation. Appl. Energy 2021, 304, 117896. [Google Scholar] [CrossRef]
- Akikur, R.; Saidur, R.; Ping, H.; Ullah, K. Performance analysis of a co-generation system using solar energy and SOFC technology. Energy Convers. Manag. 2014, 79, 415–430. [Google Scholar] [CrossRef]
- Özdemir, A.; Gamze, G. A comprehensive comparative energy and exergy analysis in solar based hydrogen production systems. Int. J. Hydrogen Energy 2022, 47, 12189–12203. [Google Scholar] [CrossRef]
- Yadav, D.; Banerjee, R. Economic assessment of hydrogen production from solar driven high-temperature steam electrolysis process. J. Clean. Prod. 2018, 183, 1131–1155. [Google Scholar] [CrossRef]
- Taheri, M.H.; Khani, L.; Mohammadpourfard, M.; Aminfar, H.; Akkurt, G.G. Multi-objective optimization of a novel supercritical CO2 cycle-based combined cycle for solar power tower plants integrated with SOFC and LNG cold energy and regasification. Int. J. Energy Res. 2022, 46, 12082–12107. [Google Scholar] [CrossRef]
- Udagawa, J.; Aguiar, P.; Brandon, N. Hydrogen production through steam electrolysis: Model-based dynamic behaviour of a cathode-supported intermediate temperature solid oxide electrolysis cell. J. Power Sources 2008, 180, 46–55. [Google Scholar] [CrossRef]
- Ni, M.; Leung, M.K.H.; Leung, D.Y.C. A modeling study on concentration overpotentials of a reversible solid oxide fuel cell. J. Power Sources 2006, 163, 460–466. [Google Scholar] [CrossRef]
- Iwahara, H. High temperature proton conducting oxides and their applications to solid electrolyte fuel cells and steam electrolyzer for hydrogen production. Solid State Ion. 1988, 28, 573–578. [Google Scholar] [CrossRef]
- Hino, R.; Haga, K.; Aita, H.; Sekita, K. 38. R&D on hydrogen production by high-temperature electrolysis of steam. Nucl. Eng. Des. 2004, 233, 363–375. [Google Scholar]
- Shin, Y.; Park, W.; Chang, J.; Park, J. Evaluation of the high temperature electrolysis of steam to produce hydrogen. Int. J. Hydrogen Energy 2007, 32, 1486–1491. [Google Scholar] [CrossRef]
- Ni, M.; Leung, M.K.; Leung, D.Y. An electrochemical model of a solid oxide steam electrolyzer for hydrogen production. Chem. Eng. Technol. Ind. Chem.-Plant Equip.-Process Eng.-Biotechnol. 2006, 29, 636–642. [Google Scholar] [CrossRef]
- Ni, M.; Leung, M.K.; Leung, D.Y. Energy and exergy analysis of hydrogen production by solid oxide steam electrolyzer plant. Int. J. Hydrogen Energy 2007, 32, 4648–4660. [Google Scholar] [CrossRef]
- Zhao, Y.; Sadhukhan, J.; Lanzini, A.; Brandon, N.; Shah, N. Optimal integration strategies for a syngas fuelled SOFC and gas turbine hybrid. J. Power Sources 2011, 196, 9516–9527. [Google Scholar] [CrossRef]
- Nam, J.H.; Jeon, D.H. A comprehensive micro-scale model for transport and reaction in intermediate temperature solid oxide fuel cells. Electrochim. Acta 2006, 51, 3446–3460. [Google Scholar] [CrossRef]
- Todd, B.; Young, J. Thermodynamic and transport properties of gases for use in solid oxide fuel cell modelling. J. Power Sources 2002, 110, 186–200. [Google Scholar] [CrossRef]
- Yakabe, H.; Hishinuma, M.; Uratani, M.; Matsuzaki, Y.; Yasuda, I. Evaluation and modeling of performance of anode-supported solid oxide fuel cell. J. Power Sources 2000, 86, 423–431. [Google Scholar] [CrossRef]
- Cai, Q.; Luna-Ortiz, E.; Adjiman, C.; Brandon, N. The Effects of Operating Conditions on the Performance of a Solid Oxide Steam Electrolyser: A Model-Based Study. Fuel Cells 2010, 10, 1114–1128. [Google Scholar] [CrossRef]
- Cai, Q.; Adjiman, C.S.; Brandon, N.P. Maximizing Hydrogen Production of A Solid Oxide Electrolyser Cell. In Proceedings of the 2012 International Conference on Clean and Green Energy, Singapore, 5–7 January 2012; pp. 72–77. [Google Scholar]
- Zhao, Y.; Ou, C.; Chen, J. A new analytical approach to model and evaluate the performance of a class of irreversible fuel cells. Int. J. Hydrogen Energy 2008, 33, 4161–4170. [Google Scholar] [CrossRef]
- Watowich, S.J.; Berry, R.S. Optimal current paths for model electrochemical systems. J. Phys. Chem. 1986, 90, 4624–4631. [Google Scholar] [CrossRef]
- Ni, M.; Leung, M.K.; Leung, D.Y. Technological development of hydrogen production by solid oxide electrolyzer cell (SOEC). Int. J. Hydrogen Energy 2008, 33, 2337–2354. [Google Scholar] [CrossRef]
- Doenitz, W.; Schmidberger, R.; Steinheil, E.; Streicher, R. Hydrogen production by high temperature electrolysis of water vapour. Int. J. Hydrogen Energy 1980, 5, 55–63. [Google Scholar] [CrossRef]
- Li, W.; Jin, J.; Wang, H.; Wei, X.; Ling, Y.; Hao, Y.; Pei, G.; Jin, H. Full-spectrum solar energy utilization integrating spectral splitting, photovoltaics and methane reforming. Energy Convers. Manag. 2018, 173, 602–612. [Google Scholar] [CrossRef]
- Wu, H.; Zhou, Z.; Shan, S. Optimal design principle of a cascading solar photovoltaic system with concentrating spectrum splitting and reshaping. Renew. Energy 2022, 197, 197–210. [Google Scholar] [CrossRef]
- Liao, T.; Lin, B.; Yang, Z. Performance characteristics of a low concentrated photovoltaic–thermoelectric hybrid power generation device. Int. J. Therm. Sci. 2014, 77, 158–164. [Google Scholar] [CrossRef]
- Le Pierrès, N.; Cosnier, M.; Luo, L.; Fraisse, G. Coupling of thermoelectric modules with a photovoltaic panel for air pre-heating and pre-cooling application; an annual simulation. Int. J. Energy Res. 2008, 32, 1316–1328. [Google Scholar] [CrossRef]
- Zhao, Q.; Zhang, H.; Hu, Z. Hybridizing photovoltaic cell with direct contact membrane distillation for electricity and freshwater cogeneration: Concept and performance evaluation. Desalination 2020, 496, 114701. [Google Scholar] [CrossRef]
- Ma, L.; Zhao, Q.; Zhang, H. Performance analysis of a new hybrid system composed of a concentrated photovoltaic cell and a two-stage thermoelectric generator. Sustain. Energy Grids Netw. 2021, 27, 100481. [Google Scholar] [CrossRef]
- Kalogirou, S.A. Solar thermal collectors and applications. Progress Energy Combust. Sci. 2004, 30, 231–295. [Google Scholar] [CrossRef]
- Basha, C.H.; Rani, C.; Odofin, S. A review on non-isolated inductor coupled DC-DC converter for photovoltaic grid-connected applications. Int. J. Renew. Energy Res. IJRER 2017, 7, 1570–1585. [Google Scholar]
- Harrag, A.; Titraoui, A.; Bahri, H.; Messalti, S. Photovoltaic pumping system-Comparative study analysis between direct and indirect coupling mode. AIP Conf. Proc. 2017, 1814, 020002. [Google Scholar]
- Dahbi, S.; Aboutni, R.; Aziz, A.; Benazzi, N.; Elhafyani, M.; Kassmi, K. Optimised hydrogen production by a photovoltaic-electrolysis system DC/DC converter and water flow controller. Int. J. Hydrogen Energy 2016, 41, 20858–20866. [Google Scholar] [CrossRef]
- Kelly, N.A.; Gibson, T.L.; Ouwerkerk, D.B. Generation of high-pressure hydrogen for fuel cell electric vehicles using photovoltaic-powered water electrolysis. Int. J. Hydrogen Energy 2011, 36, 15803–15825. [Google Scholar] [CrossRef]
- Kelly, N.A.; Gibson, T.L.; Cai, M.; Spearot, J.A.; Ouwerkerk, D.B. Development of a renewable hydrogen economy: Optimization of existing technologies. Int. J. Hydrogen Energy 2010, 35, 892–899. [Google Scholar] [CrossRef]
- Zhang, H.; Su, S.; Chen, X.; Lin, G.; Chen, J. Configuration design and performance optimum analysis of a solar-driven high temperature steam electrolysis system for hydrogen production. Int. J. Hydrogen Energy 2013, 38, 4298–4307. [Google Scholar] [CrossRef]
- Ni, M.; Leung, M.K.H.; Leung, D.Y.C. Parametric study of solid oxide steam electrolyzer for hydrogen production. Int. J. Hydrogen Energy 2007, 32, 2305–2313. [Google Scholar] [CrossRef]
- Momma, A.; Kato, T.; Kaga, Y.; Nagata, S. Polarization behavior of high temperature solid oxide electrolysis cells (SOEC). J. Ceram. Soc. Jpn. 1997, 105, 369–373. [Google Scholar] [CrossRef]
- Kong, C.; Xu, Z.; Yao, Q. Outdoor performance of a low-concentrated photovoltaic–thermal hybrid system with crystalline silicon solar cells. Appl. Energy 2013, 112, 618–625. [Google Scholar] [CrossRef]
- Wu, S.-Y.; Zhong, Z.-H.; Xiao, L.; Chen, Z.-L. Performance analysis on a novel photovoltaic-hydrophilic modified tubular seawater desalination (PV-HMTSD) system. Desalination 2021, 499, 114829. [Google Scholar] [CrossRef]
- Mahmoudinezhad, S.; Rezania, A.; Rosendahl, L.A. Behavior of hybrid concentrated photovoltaic-thermoelectric generator under variable solar radiation. Energy Convers. Manag. 2018, 164, 443–452. [Google Scholar] [CrossRef]
- Ma, L.; Zhao, Q.; Zhang, H.; Hou, S.; Zhao, J.; Wang, F.; Zhang, C.; Miao, H.; Yuan, J. Performance analysis of a concentrated photovoltaic cell-elastocaloric cooler hybrid system for power and cooling cogeneration. Energy 2022, 239, 122290. [Google Scholar] [CrossRef]
- Joshi, A.S.; Dincer, I.; Reddy, B.V. Effects of various parameters on energy and exergy efficiencies of a solar thermal hydrogen production system. Int. J. Hydrogen Energy 2016, 41, 7997–8007. [Google Scholar] [CrossRef]




| Parameter | Symbol | Value |
|---|---|---|
| Operating pressure | ||
| Partial pressure of hydrogen | ||
| Partial pressure of steam | ||
| Partial pressure of oxygen | ||
| Preexponential factor for anode exchanger current density | ||
| Preexponential factor for cathode exchanger current density | ||
| Activation energy for anode | ||
| Activation energy for cathode | ||
| Electrode porosity | ||
| Electrode tortuosity | ||
| Average pore radius | ||
| Electrolyte thickness | ||
| Electrolyte ionic conductivity | ||
| Cathode thickness | ||
| Cathode electric conductivity | ||
| Anode thickness | ||
| Anode electric conductivity | ||
| Efficiency of the heat exchangers | ||
| Operating temperature | ||
| Temperature of the CSC | ||
| Temperature of the environment | ||
| Flow rate of at SOEC inlet | ||
| Area of single-cell bipolar plates |
| Parameter | Symbol | Value |
|---|---|---|
| Short-circuit current | ||
| Diode ideality factor | ||
| Short-circuit current temperature coefficient | ||
| Reverse saturation current at reference temperature | ||
| Area of CPV | ||
| Number of strings in parallel | ||
| Number of cells in series | ||
| Intrinsic series resistance of the CPV | ||
| Reference temperature of CPV | ||
| Reference solar irradiation | ||
| Electric current | ||
| Optical concentration ratio | ||
| Operating temperature of CPV | ||
| Surface temperature of the sun | ||
| Elementary electron charge | ||
| Boltzmann constant |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lang, S.; Yuan, J.; Zhang, H. Optimally Splitting Solar Spectrums by Concentrating Solar Spectrums Splitter for Hydrogen Production via Solid Oxide Electrolysis Cell. Energies 2024, 17, 2067. https://doi.org/10.3390/en17092067
Lang S, Yuan J, Zhang H. Optimally Splitting Solar Spectrums by Concentrating Solar Spectrums Splitter for Hydrogen Production via Solid Oxide Electrolysis Cell. Energies. 2024; 17(9):2067. https://doi.org/10.3390/en17092067
Chicago/Turabian StyleLang, Shaocheng, Jinliang Yuan, and Houcheng Zhang. 2024. "Optimally Splitting Solar Spectrums by Concentrating Solar Spectrums Splitter for Hydrogen Production via Solid Oxide Electrolysis Cell" Energies 17, no. 9: 2067. https://doi.org/10.3390/en17092067
APA StyleLang, S., Yuan, J., & Zhang, H. (2024). Optimally Splitting Solar Spectrums by Concentrating Solar Spectrums Splitter for Hydrogen Production via Solid Oxide Electrolysis Cell. Energies, 17(9), 2067. https://doi.org/10.3390/en17092067








