Thermal Stress in Full-Size Solid Oxide Fuel Cell Stacks by Multi-Physics Modeling
Abstract
1. Introduction
- (1)
- Only gas flow within the stack is considered, aiming to optimize the stack structure and to improve gas distribution uniformity. On the other hand, only the average temperature field is considered in some cases, aiming to explore the distribution of temperature or stress distribution within the stack, without accounting for the electrochemical reactions.
- (2)
- Only the electrochemical reactions are considered but simplified by reducing the three-dimensional electrochemical reactions occurring within the electrodes into two-dimensional ones.
- (3)
- The full-size of the stack and the coupling of the multi-physics processes are considered, yet the stack size is relatively small and cannot fully reflect the characteristics appearing inside the industrial-sized stacks.
- (4)
- Large-sized stacks and multi-physics coupling are all considered, while the real geometric details may be omitted.
2. Modeling Methods and Validation
- (1)
- The gases in the model flow laminarly (based on the estimated Reynolds number) are ideal and incompressible.
- (2)
- The thermal radiation inside the SOFC is neglected.
- (3)
- Homogeneity is exhibited by the porous electrode.
- (4)
- The constant temperature of 800 °C is maintained by the external ambient.
- (5)
- The anode, electrolyte layer, and cathode materials are isotropic and linear elastic materials.
- (6)
- The thermophysical characteristics of all materials composing the SOFC stack remain constant, irrespective of the local temperature.
- (7)
- The anode, cathode, and electrolyte layer interfaces, along with the connecting body and sealing material, form a continuous structure that permits collective deformation without fracturing.
- (8)
- The external loads imposed during the preparation and assembly of the stack and the residual stresses in the material are negligible.
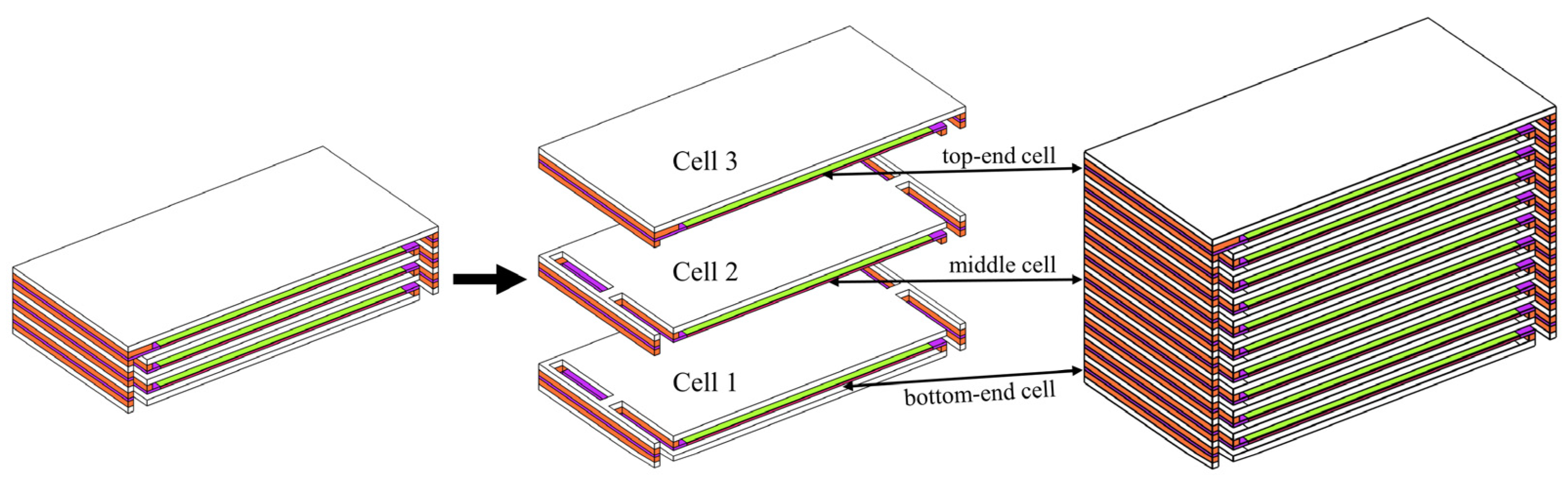
2.1. Stack Parameters and Geometric Model
2.2. Governing Equations
2.2.1. Electrochemical Model
2.2.2. Gas Flow and Momentum Equations
2.2.3. Gas Species Transport Equation
2.2.4. Heat Transfer Equation
2.2.5. Thermal Stress–Strain Relation and Failure Probability Analysis Method
2.3. Effective Properties of Porous Composite Materials
2.4. Boundary Conditions
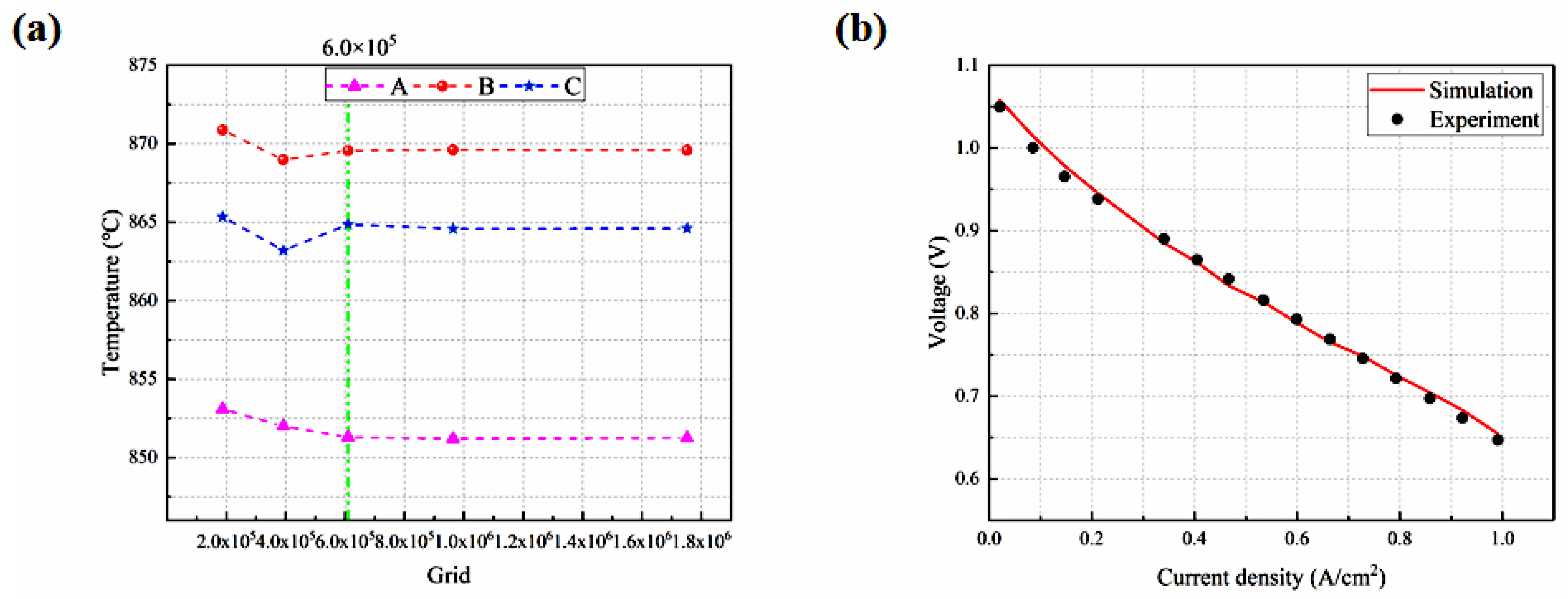
2.5. Grid Arrangement and Model Validation
3. Results and Discussion
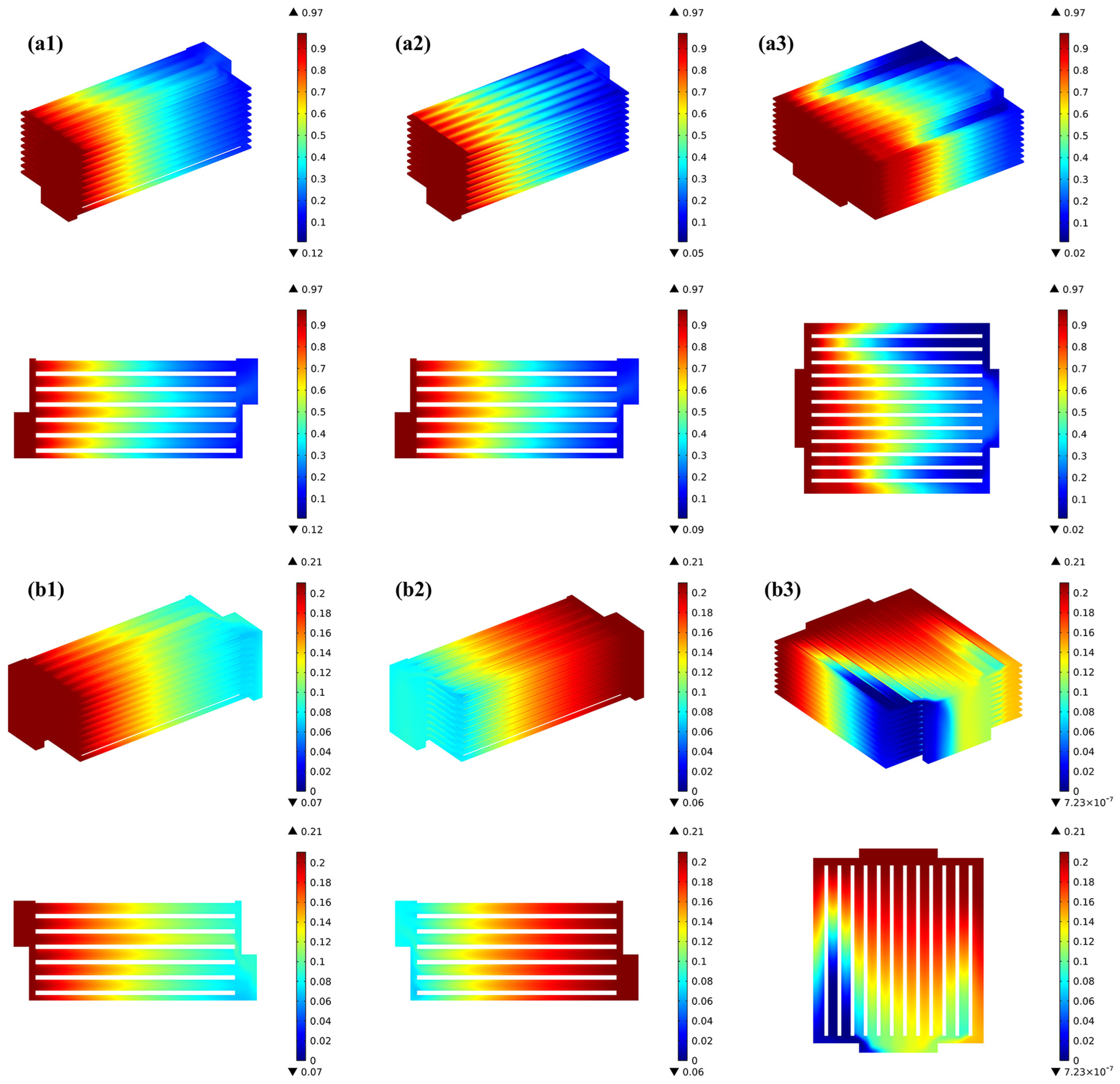
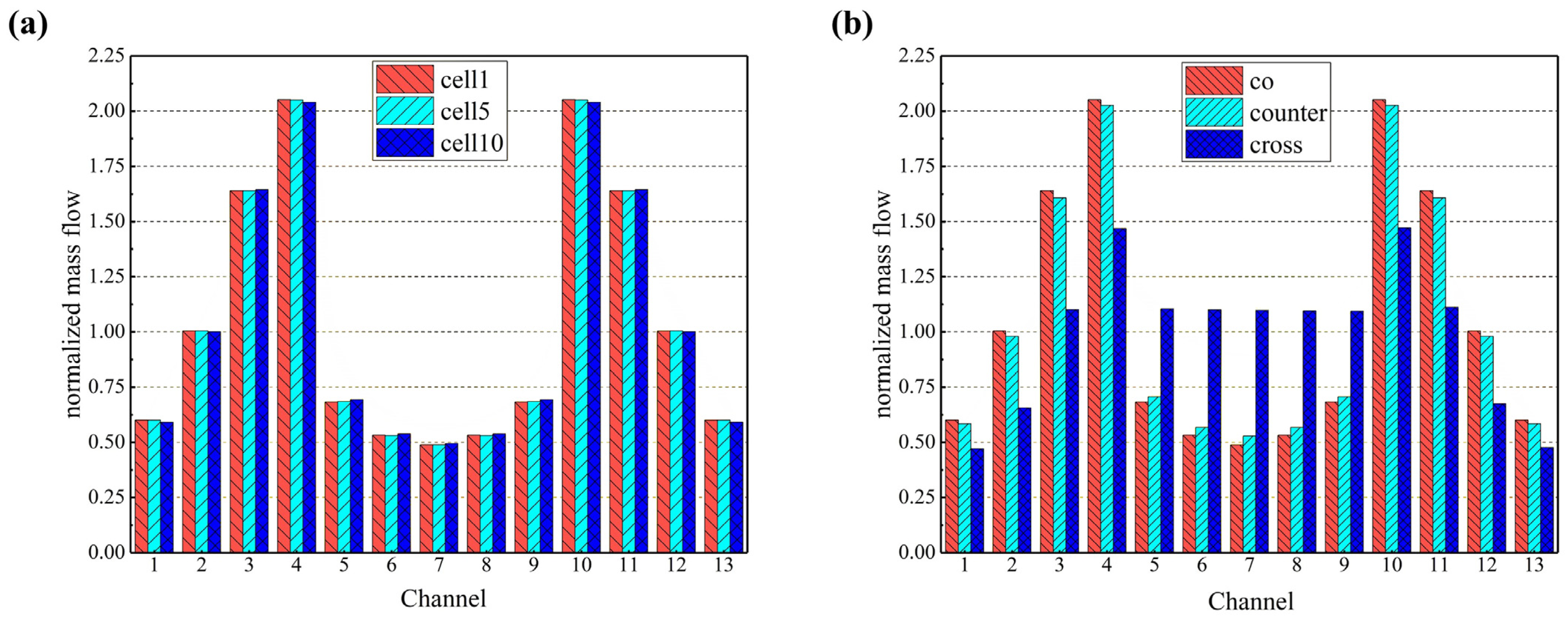
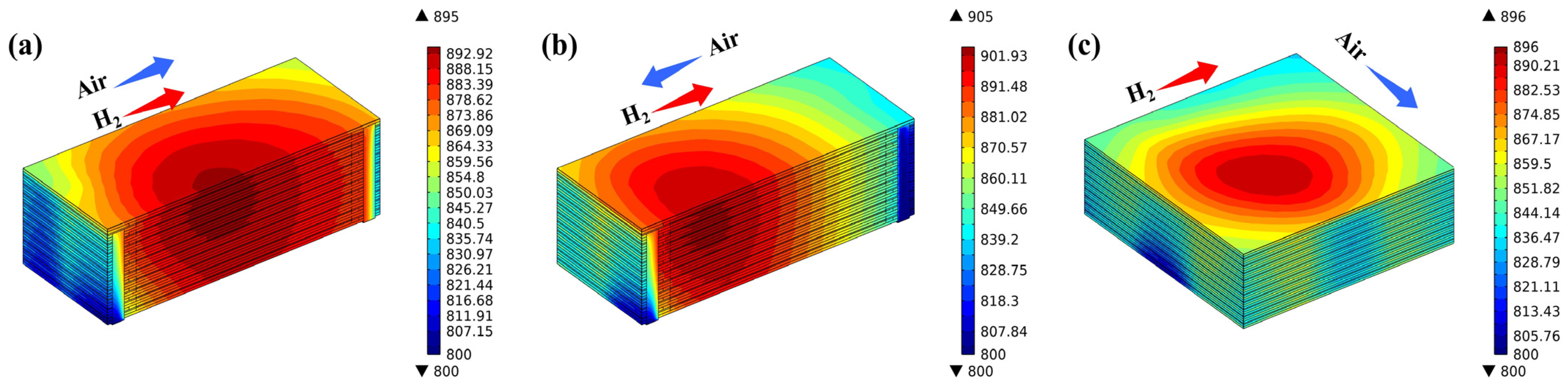
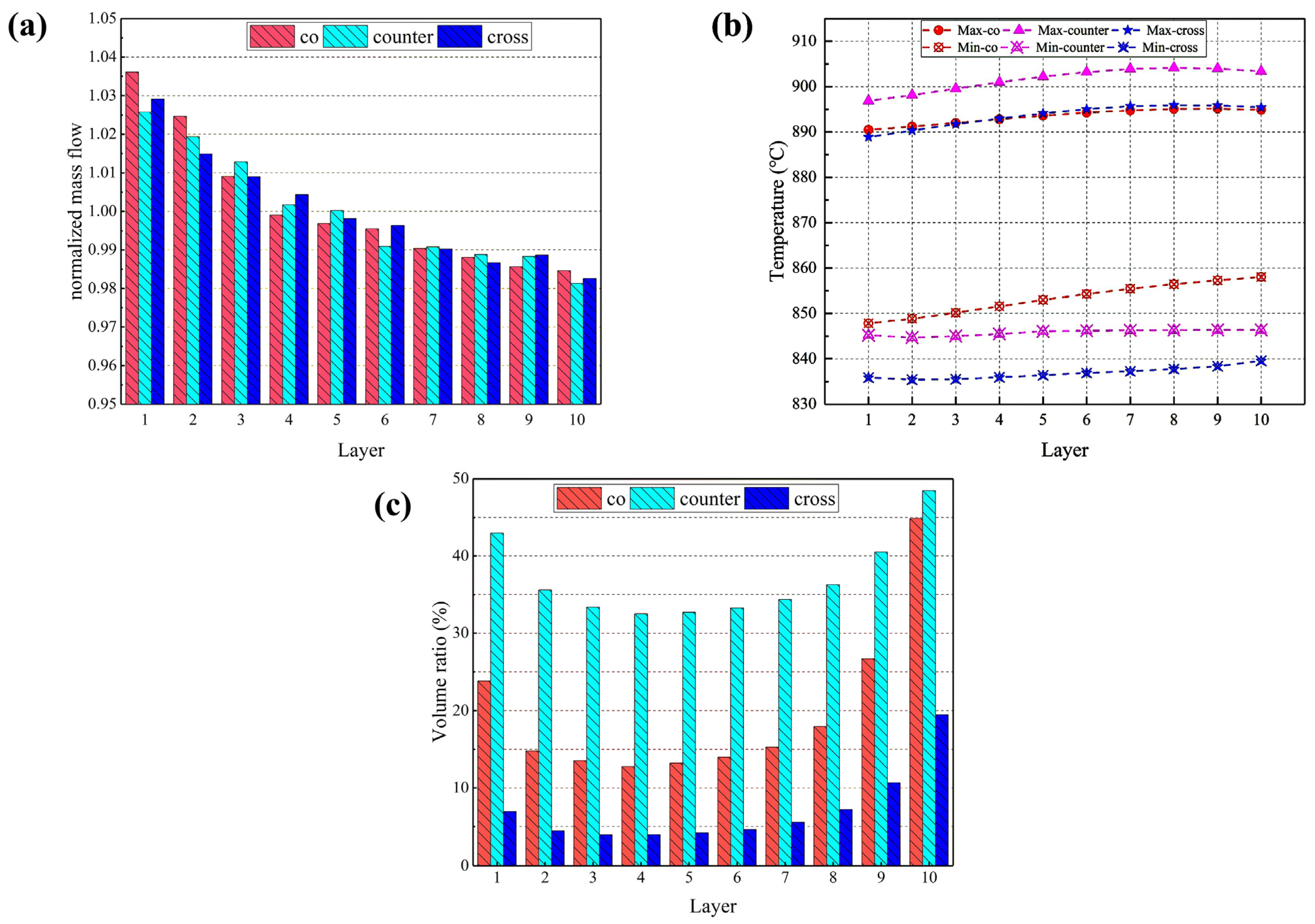
3.1. Effect of Flow Arrangement and Configurations
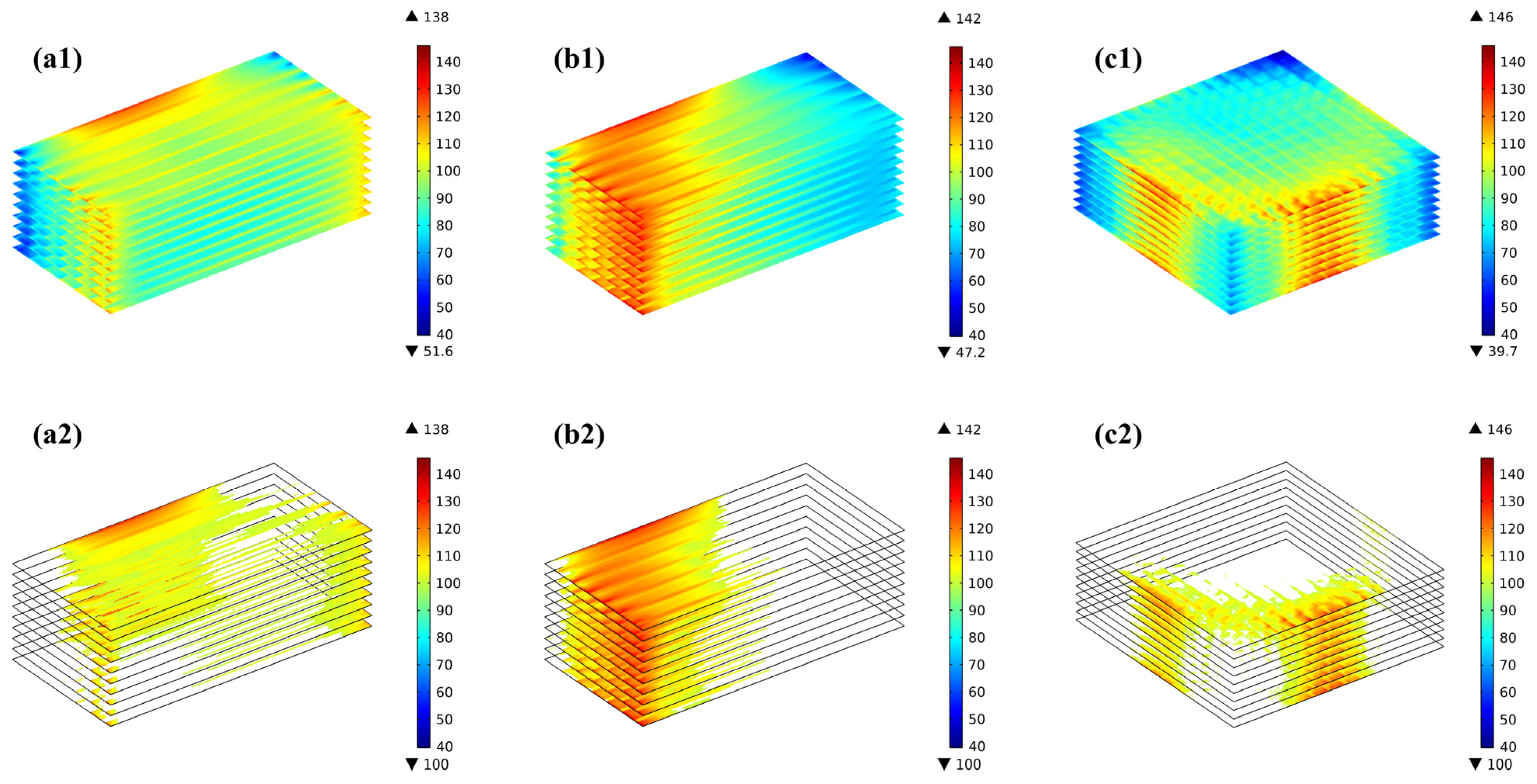
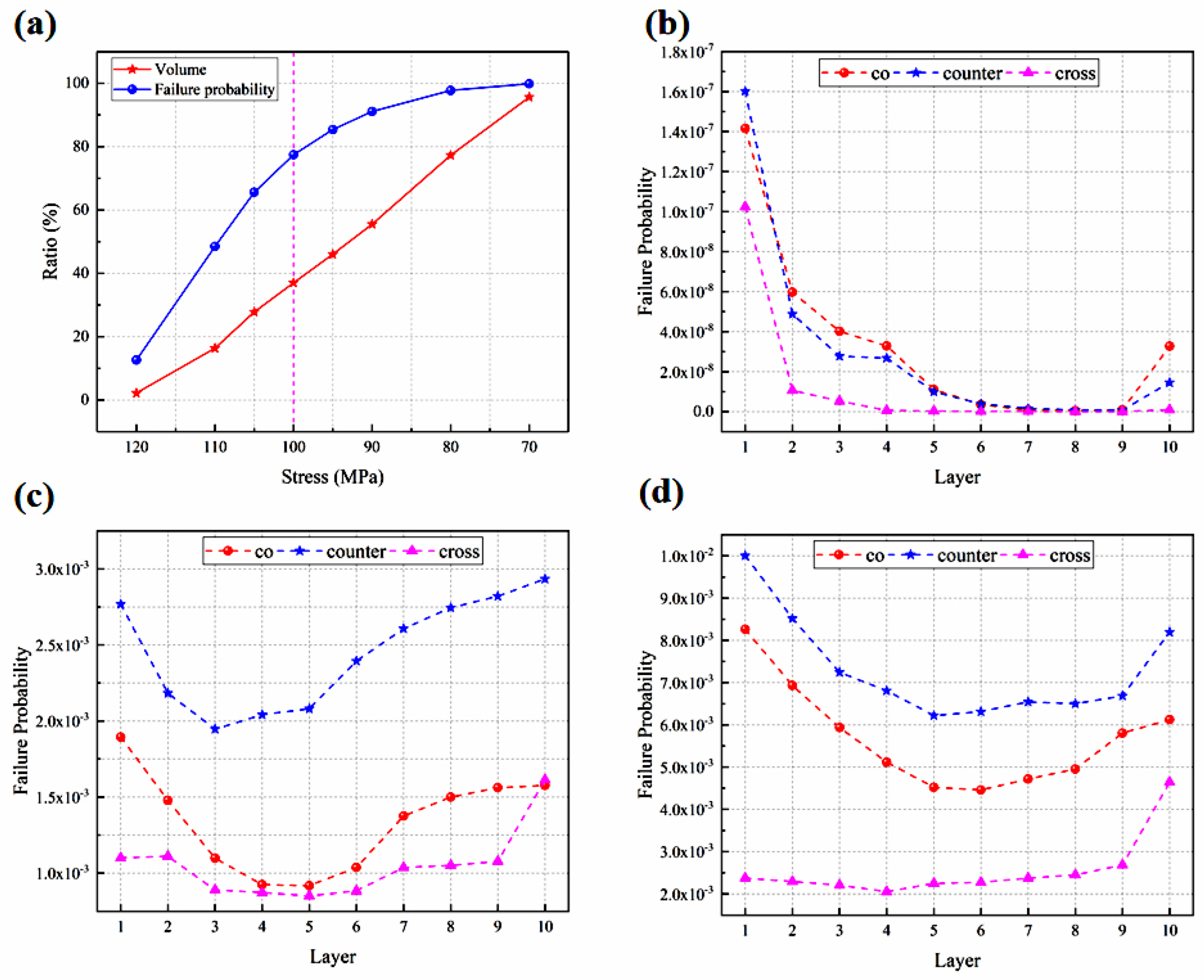
3.2. Failure Probability Analysis
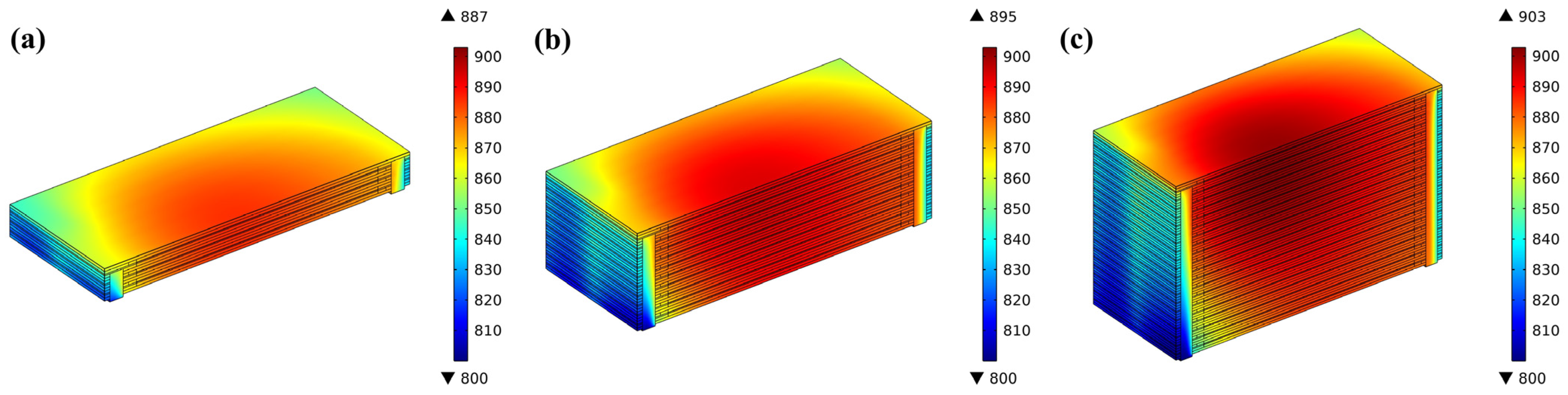
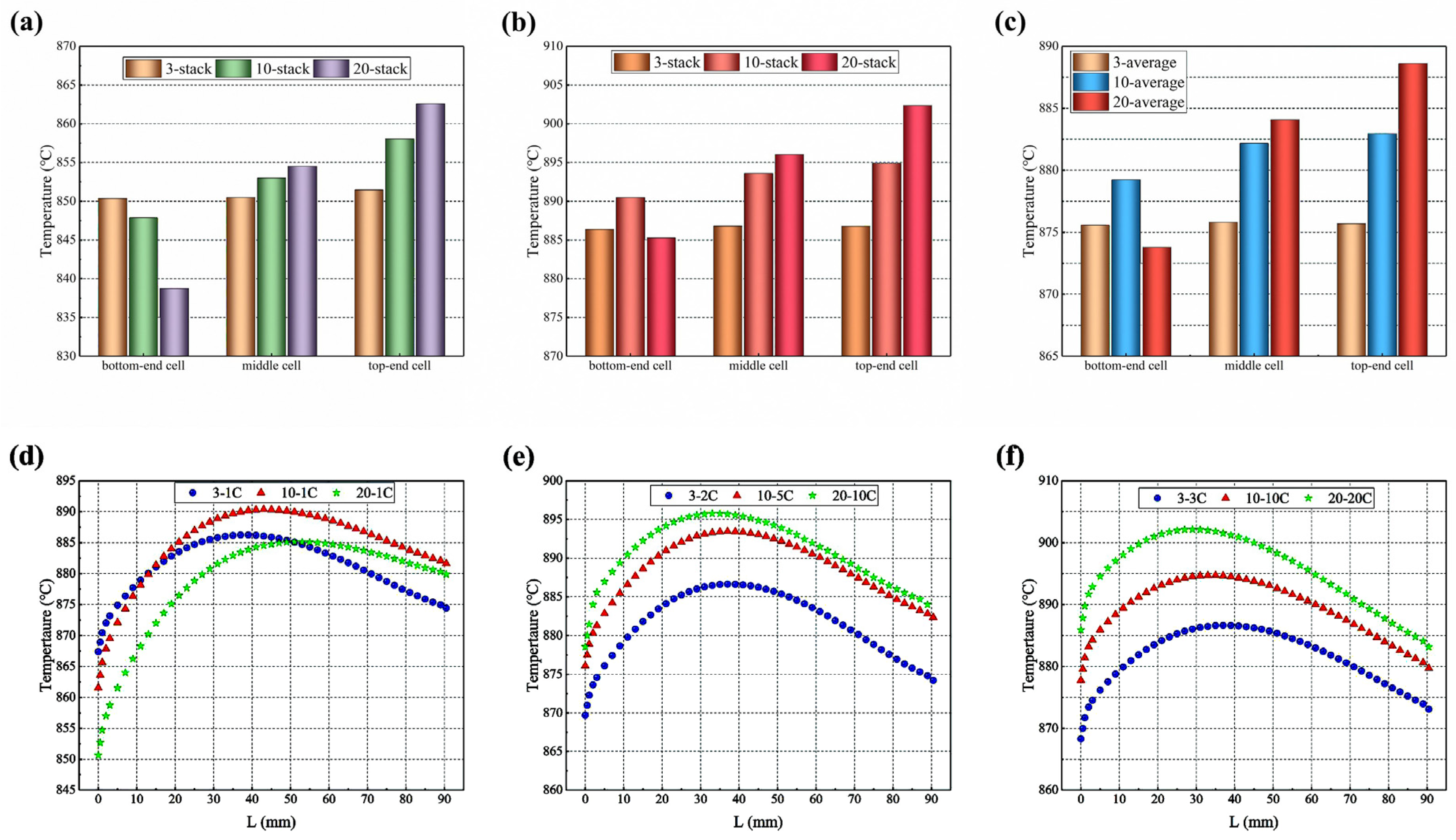
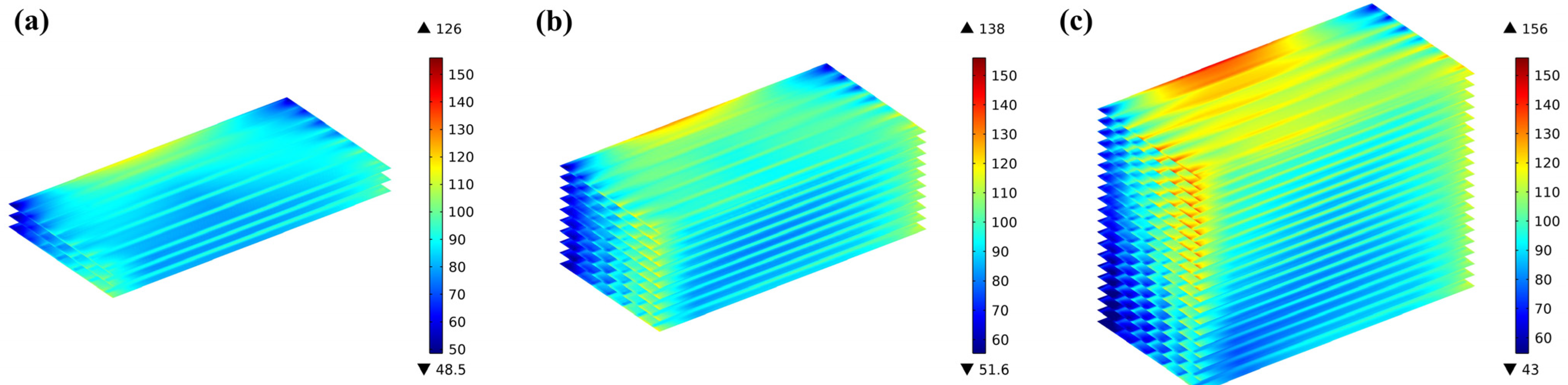
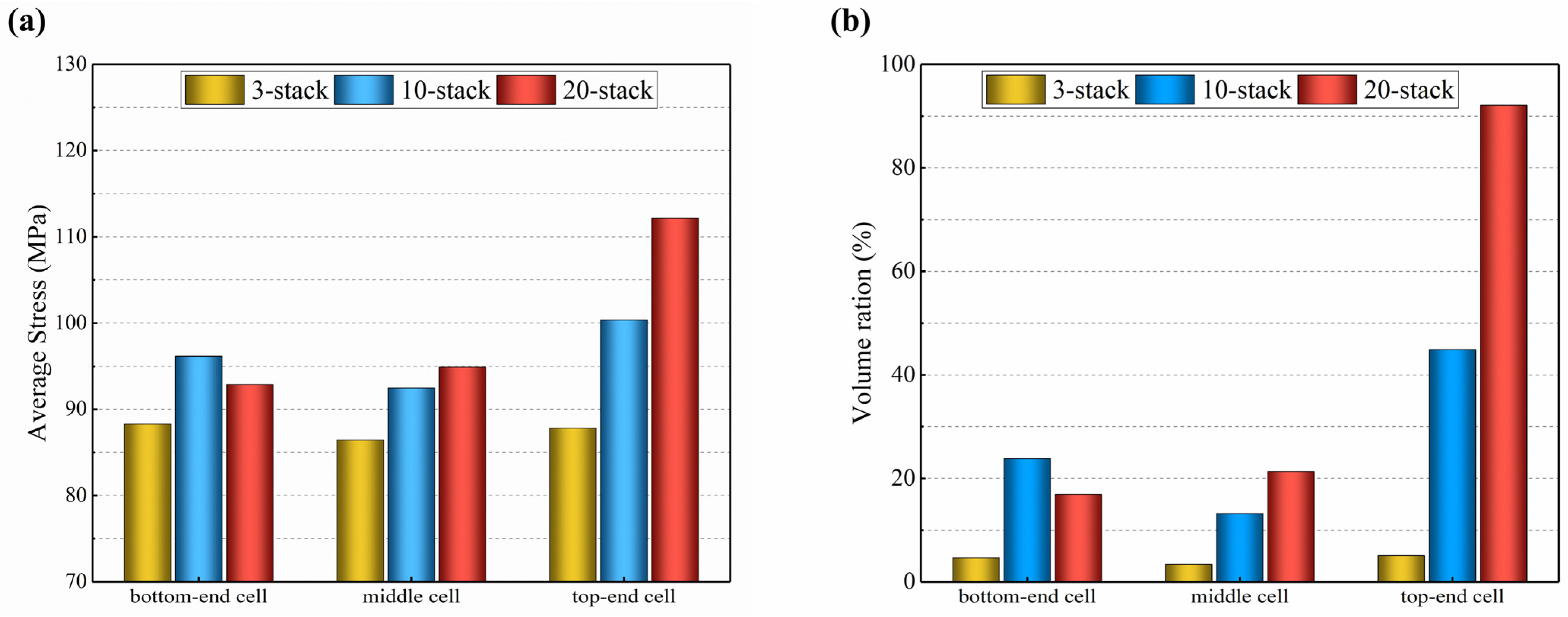
3.3. Comparative Study with Shorter and Longer Stacks
4. Conclusions
- (1)
- In identical situations, the counter-flow stack has the highest power output together with the greater forecasted thermal stress; the cross-flow stack has the lowest power output accompanied by the highest thermal stress. The electrolyte layer experiences greater thermal stress, and the volume ratio with the thermal stress beyond 100 MPa on the electrolyte layer is the highest in the counter-flow stack and the lowest in the cross-flow stack (the reduction in the volume ratio is about 30% in the cross-flow stack). The thermal stress distribution is primarily influenced by the stack air flow direction under the same conditions.
- (2)
- The highest and lowest temperatures vary similarly between the unit cells, and the highest temperature appears in the counter-flow stack, and the lowest temperature is slightly higher in the co-flow stack than in the other stacks. The HTSR on the electrolyte layer in each cells decreases first and then increases, reaching a maximum value in the 10th cell (the top-end cell).
- (3)
- The longer and shorter stacks have similar temperature and thermal stress distributions, but their difference between the unit cells of the longer stack is bigger than that predicted for the shorter stack. It would be inaccurate or even unreasonable if the findings and conclusions drawn for small-sized and shorter stacks are directly applied or extrapolated to large-sized longer stacks.
- (4)
- The probability of failure of the porous cathodes significantly exceeds that of the porous anodes and electrolyte layers; the probability of failure of the counter-flow stacks is the highest, particularly in the longer stack.
- (1)
- The accuracy of the model has been validated by comparing with experimental I-V curves, which needs further improvement. Future research could incorporate temperature distribution within the stack as the modeling validation.
- (2)
- The focus of this paper is on the distribution of thermal stress in the stack under steady-state operation. Future research could be extended to capture the transient operating performance of the stack with different stack configuration parameters and start–stop operations.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Guo, M.; Lin, Z. Long-term evolution of mechanical performance of solid oxide fuel cell stack and the underlying mechanism. Int. J. Hydrogen Energy 2021, 46, 23293–24304. [Google Scholar] [CrossRef]
- Yang, C.; Guo, R.; Jing, X.; Li, P.; Yuan, J.; Wu, Y. Degradation mechanism and modeling study on reversible solid oxide cell in dual-mode—A review. Int. J. Hydrogen Energy 2022, 47, 37895–37928. [Google Scholar] [CrossRef]
- Peksen, M. Numerical thermomechanical modelling of solid oxide fuel cells. Prog. Energy Combust. Sci. 2015, 48, 1–20. [Google Scholar] [CrossRef]
- Zhang, X.; Yu, S.; Wang, M.; Dong, S.; Parbey, J.; Li, T.; Andersson, M. Thermal stress analysis at the interface of cathode and electrolyte in solid oxide fuel cells. Int. Commun. Heat Mass Transf. 2020, 118, 104831–104840. [Google Scholar] [CrossRef]
- Zhang, S.; Peters, R.; Varghese, B.; Deja, R.; Kruse, N.; Beale, S.B.; Blum, L.; Peters, R.; Eichel, R.-A. Modeling of reversible solid oxide cell stacks with an open-source library. J. Electrochem. Soc. 2022, 169, 114501–114512. [Google Scholar] [CrossRef]
- Colombo, K.; Kharton, V. Reliability analysis of a multi-stack solid oxide fuel cell from a systems engineering. Chem. Eng. Sci. 2021, 238, 116571–116585. [Google Scholar] [CrossRef]
- Lin, C.-K.; Chen, T.-T.; Chyou, Y.-P.; Chiang, L.-K. Thermal stress analysis of a planar SOFC stack. J. Power Sources 2007, 164, 238–251. [Google Scholar] [CrossRef]
- Wang, K.; An, B.; Chu, Z.; Wang, Y.; Liu, Z. Evaluation of gas channel structures on cell performance and thermal stress in a solid oxide fuel cell. Energy Sources Part A 2021, 1–16. [Google Scholar] [CrossRef]
- Molla, T.T.; Kwok, K.; Frandsen, H.L. Modeling the mechanical integrity of generic solid oxide cell stack designs exposed to long-term operation. Fuel Cells 2018, 19, 96–109. [Google Scholar] [CrossRef]
- Nakajo, A.; Wuillemin, Z.; Favrat, D. Simulation of thermal stresses in anode-supported solid oxide fuel cell stacks. Part I: Probability of failure of the cells. J. Power Sources 2009, 193, 203–215. [Google Scholar] [CrossRef]
- Lin, C.-K.; Huang, L.-H.; Chiang, L.-K.; Chyou, Y.-P. Thermal stress analysis of planar solid oxide fuel cell stacks: Effects of sealing design. J. Power Sources 2009, 192, 515–524. [Google Scholar] [CrossRef]
- Zheng, J.; Xiao, L.; Wu, M.; Lang, S.; Zhang, Z.; Chen, M.; Yuan, J. Numerical analysis of thermal stress for a stack of planar solid oxide fuel cells. Energies 2022, 15, 343. [Google Scholar] [CrossRef]
- Xu, Q.; Guo, M.; Xia, L. Temperature Gradient Analyses of a Tubular Solid Oxide Fuel Cell Fueled by Methanol. Trans. Tianjin Univ. 2023, 29, 14–30. [Google Scholar] [CrossRef]
- Peksen, M. 3D thermomechanical behaviour of solid oxide fuel cells operating in different environments. Int. J. Hydrogen Energy 2013, 38, 13408–13418. [Google Scholar] [CrossRef]
- Yuan, P.; Liu, S.-F. Effect of air flow rate distribution and flowing direction on the thermal stress of a solid oxide fuel cell stack with cross-flow configuration. Int. J. Hydrogen Energy 2022, 47, 6799–6810. [Google Scholar] [CrossRef]
- Wang, X.; Hou, R. Effect of Fuel Utilization on Tri-reforming-SOFC Systems with Different Heat Management Strategies. J. Phys. Conf. Ser. 2023, 2520, 012007. [Google Scholar] [CrossRef]
- Navasa, M.; Miao, X.Y.; Frandsen, H.L. A fully-homogenized multiphysics model for a reversible solid oxide cell stack. Int. J. Hydrogen Energy 2019, 44, 23330–23347. [Google Scholar] [CrossRef]
- Rizvandi, O.B.; Miao, X.Y.; Frandsen, H.L. Multiscale modeling of degradation of full solid oxide fuel cell stacks. Int. J. Hydrogen Energy 2021, 46, 27709–27730. [Google Scholar] [CrossRef]
- Miao, X.Y.; Rizvandi, O.B.; Navasa, M.; Frandsen, H.L. Modelling of local mechanical failures in solid oxide cell stacks. Appl. Energy 2021, 293, 116901. [Google Scholar] [CrossRef]
- Li, Q.; Xu, Z.; Cheng, M.; Ma, Y.; Zhang, X.; Li, G. Transient thermomechanical analysis of a solid oxide fuel cell stack based on 3D multiphysical field model. Mod. Phys. Lett. B 2020, 34, 2050158–2050181. [Google Scholar] [CrossRef]
- Buttler, A.; Koltun, R.; Wolf, R.; Spliethoff, H. A detailed techno-economic analysis of heat integration in high temperature electrolysis for efficient hydrogen production. Int. J. Hydrogen Energy 2015, 40, 38–50. [Google Scholar] [CrossRef]
- Yang, F. Generalized Butler-Volmer relation on a curved electrode surface under the action of stress. Sci. China Phys. Mech. Astron. 2016, 59, 50–56. [Google Scholar] [CrossRef]
- Hernández-Pacheco, E.; Singh, D.; Hutton, P.N.; Patel, N.; Mann, M.D. A macro-level model for determining the performance characteristics of solid oxide fuel cells. J. Power Sources 2004, 138, 174–186. [Google Scholar] [CrossRef]
- Andersson, M.; Yuan, J.; Sundn, B. SOFC modeling considering electrochemical reactions at the active three phase boundaries. Int. J. Heat Mass Transf. 2012, 55, 773–788. [Google Scholar] [CrossRef]
- Cui, T.; Xiao, G.; Yan, H.; Zhang, Y.; Wang, J.Q. Numerical simulation and analysis of the thermal stresses of a planar solid oxide electrolysis cell. Int. J. Green Energy 2023, 16, 432–444. [Google Scholar] [CrossRef]
- Jiang, C.; Gu, Y.; Guan, W.; Zheng, J.; Ni, M.; Zhong, Z. 3D thermo-electro-chemo-mechanical coupled modeling of solid oxide fuel cell with double-sided cathodes. Int. J. Hydrogen Energy 2020, 45, 904–915. [Google Scholar] [CrossRef]
- Zhang, Z.; Yue, D.; Yang, G.; Chen, J.; Zheng, Y.; Miao, H.; Wang, W.; Yuan, J.; Huang, N. Three-dimensional CFD modeling of transport phenomena in multi-channel anode-supported planar SOFCs. Int. J. Heat Mass Transf. 2015, 84, 942–954. [Google Scholar] [CrossRef]
- Ai-Kah, S.; Chen, W. Finite element formulations of strain gradient theory for microstructures and the C0–1 patch test. Int. J. Numer. Methods Eng. 2004, 61, 433–454. [Google Scholar] [CrossRef]
- Frandsen, H.L.; Makowska, M.; Greco, F.; Chatzichristodoulou, C.; Ni, D.W.; Curran, D.J.; Strobl, M.; Kuhn, L.T.; Hendriksen, P.V. Accelerated creep in solid oxide fuel cell anode supports during reduction. J. Power Sources 2016, 323, 78–89. [Google Scholar] [CrossRef]
- Weibull, W. A statistical distribution function of wide applicability. J. Appl. Mech. 1951, 18, 293–297. [Google Scholar] [CrossRef]
- Frandsen, H.L. Weibull statistics effective area and volume in the ball-on-ring testing method. Mech. Mater. 2014, 73, 28–37. [Google Scholar] [CrossRef]
- Radovic, M.; Lara-Curzio, E. Mechanical properties of tape cast nickel-based anode materials for solid oxide fuel cells before and after reduction in hydrogen. Acta Mater. 2004, 52, 5747–5756. [Google Scholar] [CrossRef]
- Hsieh, C.-L.; Tuan, W.-H. Elastic and thermal expansion behavior of two-phase composites. Mater. Sci. Eng. A 2006, 425, 349–360. [Google Scholar] [CrossRef]
- Vaßen, R.; Czech, N.; Mallener, W.; Stamm, W.; Stover, D. Influence of impurity content and porosity of plasma-sprayed yttria-stabilized zirconia layers on the sintering behaviour. Surf. Coat. Technol. 2001, 141, 135–140. [Google Scholar] [CrossRef]
- Ramakrishnan, N.; Arunachalam, V.S. Effective elastic moduli of porous solids. J. Mater. Sci. 1990, 25, 3930–3937. [Google Scholar] [CrossRef]
- Nakajo, A.; Kuebler, J.; Faes, A.; Vogt, U.F.; Schindler, H.J.; Chiang, L.-K.; Modena, S.; Van herle, J.; Hocker, T. Compilation of mechanical properties for the structural analysis of solid oxide fuel cell stacks. Constitutive materials of anode-supported cells. Ceram. Int. 2012, 38, 3907–3927. [Google Scholar] [CrossRef]
- Banerjee, A.; Wang, Y.; Diercks, J.; Deutschmann, O. Hierarchical modeling of solid oxide cells and stacks producing syngas via H2O/CO2 Co-electrolysis for industrial applications. Appl. Energy 2018, 230, 996–1013. [Google Scholar] [CrossRef]
- Bi, W.; Chen, D.; Lin, Z. A key geometric parameter for the flow uniformity in planar solid oxide fuel cell stacks. Int. J. Hydrogen Energy 2009, 34, 3873–3884. [Google Scholar] [CrossRef]
- Hirose, H. Reliability of the Estimates of the Weibull Parameters. IEEJ Trans. Fundam. Mater. 1990, 110, 903–912. [Google Scholar] [CrossRef][Green Version]
- Balaban, H.S.; Haspert, K. Estimating Weibull parameters for a general class of devices from limited failure data. IEEE Trans. Reliab. 1972, R-21, 111–117. [Google Scholar] [CrossRef]
- Baklizi, A.; Ahmed, S.E. On the estimation of reliabilty function in a Weibull lifetime distribution. Statistics 2008, 42, 351–362. [Google Scholar] [CrossRef]
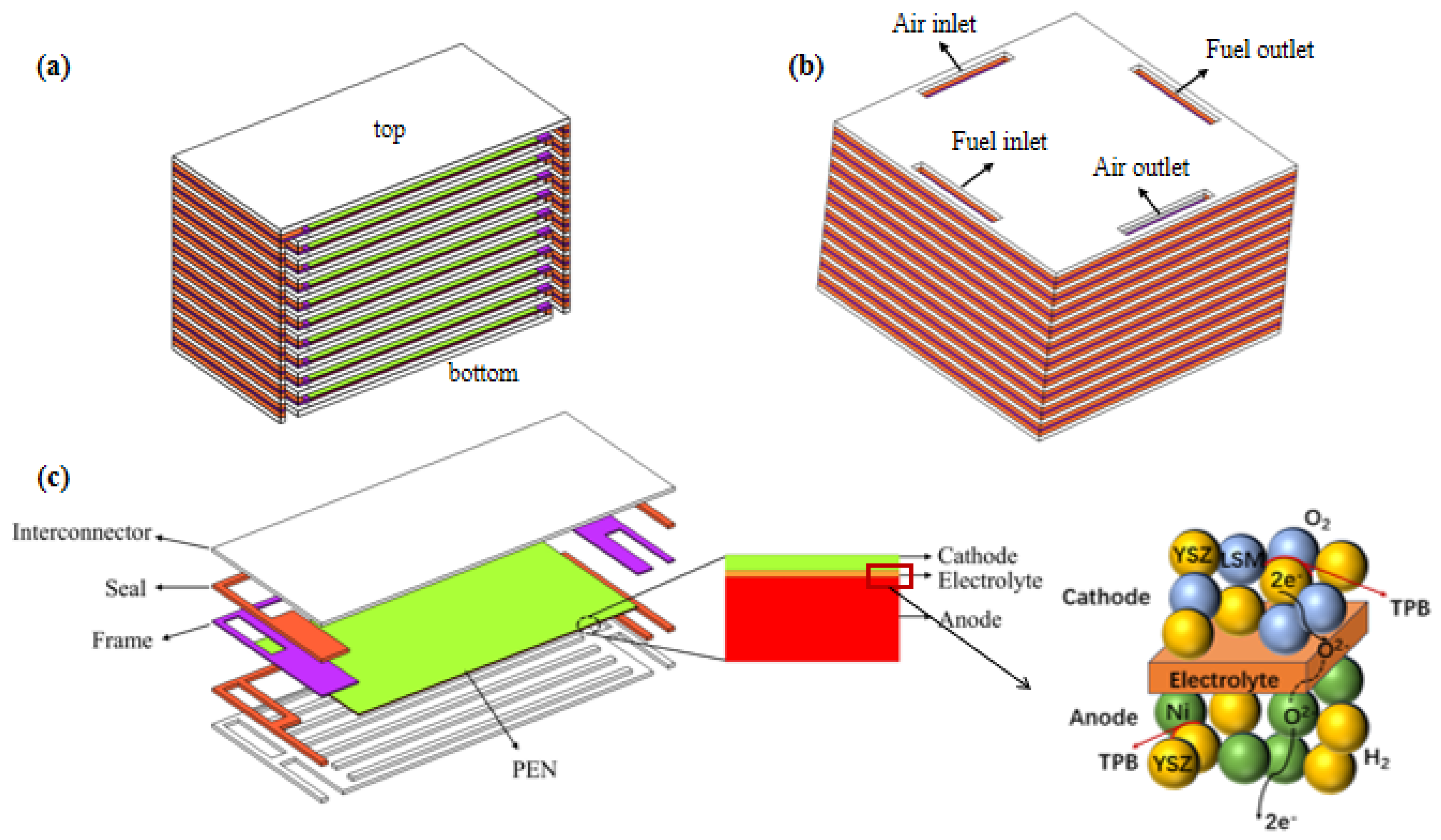


| Components | Value | Unit |
|---|---|---|
| Quantity of channels in each cell | 13 | - |
| Cell width | 91 | mm |
| Cell length | 91 | mm |
| Rib width | 2 | mm |
| Channel width | 5 | mm |
| Channel height | 1 | mm |
| Interconnector thickness | 1 | mm |
| Cathode thickness | 70 | μm |
| Electrolyte layer thickness | 20 | μm |
| Anode thickness | 380 | μm |
| Parameters | Anode | Cathode |
|---|---|---|
| θ (-) | 0.3 | 0.3 |
| τ (-) | 3 | 3 |
| Av (m−1) | 1.3 × 105 | 1.3 × 106 |
| Ai (Ωm−2) | 6.54 × 1011 | 2.35 × 1011 |
| Ea (kJ mol−1) | 140 | 137 |
| Components | Heat Capacity (J kg−1 K−1) | Thermal Conductivity (W m−1 K−1) | Porosity (-) | Permeability (m2) | Density (kg m−3) |
|---|---|---|---|---|---|
| Seal | 560 | 0.064 | - | - | 4010 |
| Cathode | 430 | 6 | 0.3 | 2 × 10−11 | 3030 |
| Electrolyte | 550 | 2.7 | - | - | 5160 |
| Anode | 450 | 11 | 0.3 | 2 × 10−11 | 3310 |
| Interconnector | 475 | 44.5 | - | - | 7860 |
| Components | (MPa) | M (-) |
|---|---|---|
| Anode | 128 | 13 |
| Electrolyte | 282 | 8 |
| Cathode | 75 | 4 |
| Components | Young’s Modulus (GPa) | Poisson’s Ratio (-) | CTE (10−6 K−1) |
|---|---|---|---|
| Anode | 76.57 | 0.28 | 14.24 |
| Electrolyte | 183 | 0.32 | 10.8 |
| Cathode | 35 | 0.25 | 11.7 |
| Seals | 0.019 | 0 | 13.9 |
| Interconnector | 60 | 0.3 | 15.5 |
| Boundary Conditions | Value | Unit |
|---|---|---|
| Cathode inlet gas | 21% O2, 79% N2 | - |
| Anode inlet gas | 97% H2, 3% H2O | - |
| Cathode intake flow rate | 4500 | sccm |
| Anode intake flow rate | 1500 | sccm |
| Environment temperature | 800 | °C |
| Convective heat transfer coefficient | 2 | W∙m−2∙K−1 |
| Surface emissivity | 0.3 | - |
| Stack Component | Element Type (Number of the Meshes Employed) |
|---|---|
| PEN | Hexahedron (124,488) |
| Interconnector | Hexahedron (66,832) |
| Seal | Hexahedron (10,836) |
| Frame | Tetrahedron (70,317), pyramid (8053) |
| Rib | Hexahedron (21,840) |
| Channel | Hexahedron (65,748) |
| Inlet and outlet pipeline | Tetrahedron (82,653), pyramid (3562) |
| Flow Pattern | Co-Stack | Counter-Stack | Cross-Stack | |
|---|---|---|---|---|
| Thermal Stress Range (MPa) | ||||
| >120 | 0.3% | 2.4% | 0.2% | |
| >110 | 3.5% | 11.1% | 1.5% | |
| >100 | 19.7% | 37.0% | 7.1% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Wu, M.; Xiao, L.; Wang, H.; Liu, Y.; Ou, D.; Yuan, J. Thermal Stress in Full-Size Solid Oxide Fuel Cell Stacks by Multi-Physics Modeling. Energies 2024, 17, 2025. https://doi.org/10.3390/en17092025
Zhang X, Wu M, Xiao L, Wang H, Liu Y, Ou D, Yuan J. Thermal Stress in Full-Size Solid Oxide Fuel Cell Stacks by Multi-Physics Modeling. Energies. 2024; 17(9):2025. https://doi.org/10.3390/en17092025
Chicago/Turabian StyleZhang, Xueping, Mingtao Wu, Liusheng Xiao, Hao Wang, Yingqi Liu, Dingrong Ou, and Jinliang Yuan. 2024. "Thermal Stress in Full-Size Solid Oxide Fuel Cell Stacks by Multi-Physics Modeling" Energies 17, no. 9: 2025. https://doi.org/10.3390/en17092025
APA StyleZhang, X., Wu, M., Xiao, L., Wang, H., Liu, Y., Ou, D., & Yuan, J. (2024). Thermal Stress in Full-Size Solid Oxide Fuel Cell Stacks by Multi-Physics Modeling. Energies, 17(9), 2025. https://doi.org/10.3390/en17092025







