The Mechanism of the Semi-Transparent Coverings Affecting the Power Generation Capacity of the Photovoltaic Module and Array
Abstract
1. Introduction
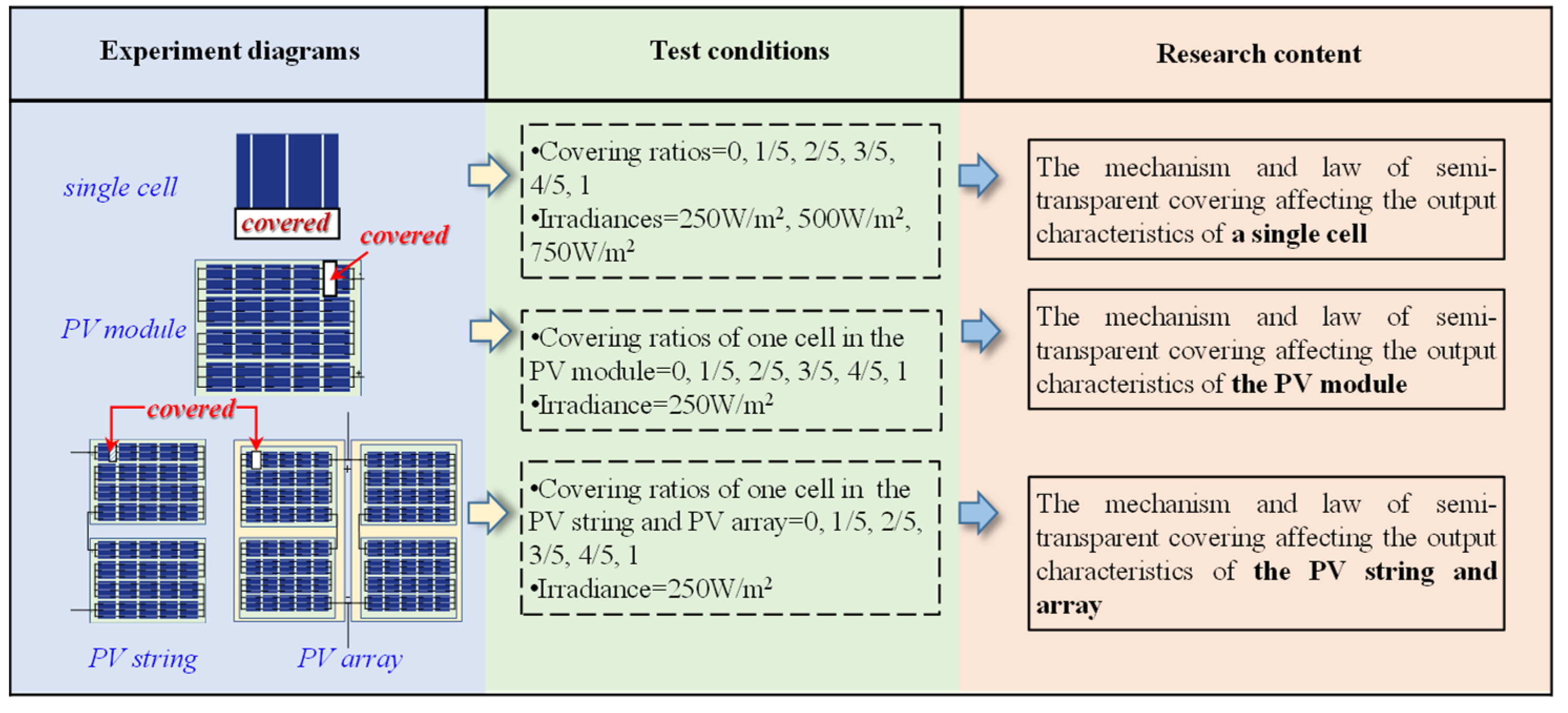
2. Research Processes and Methodologies
2.1. Experimental Design
2.2. Research Processes
3. Results and Discussion
3.1. Output of a Single Cell under Semi-Transparent Covering
3.2. Output of PV Module under Semi-Transparent Covering
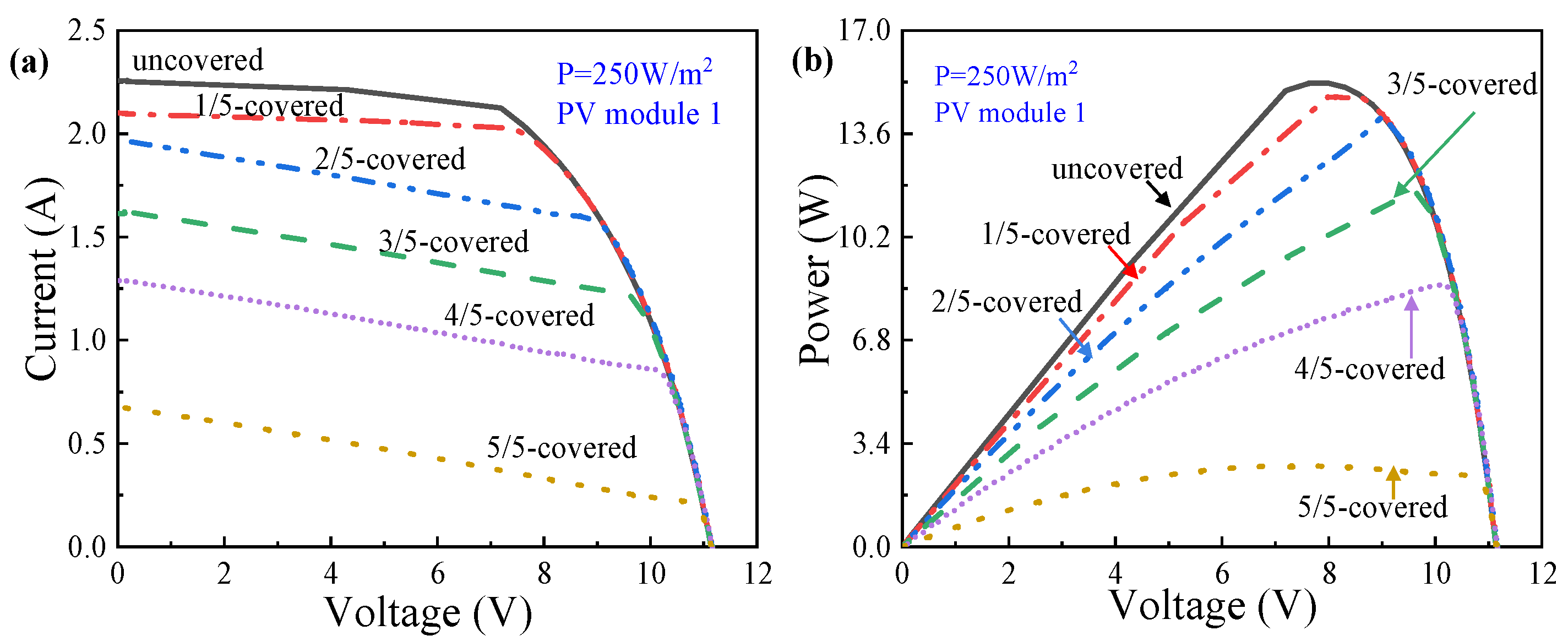
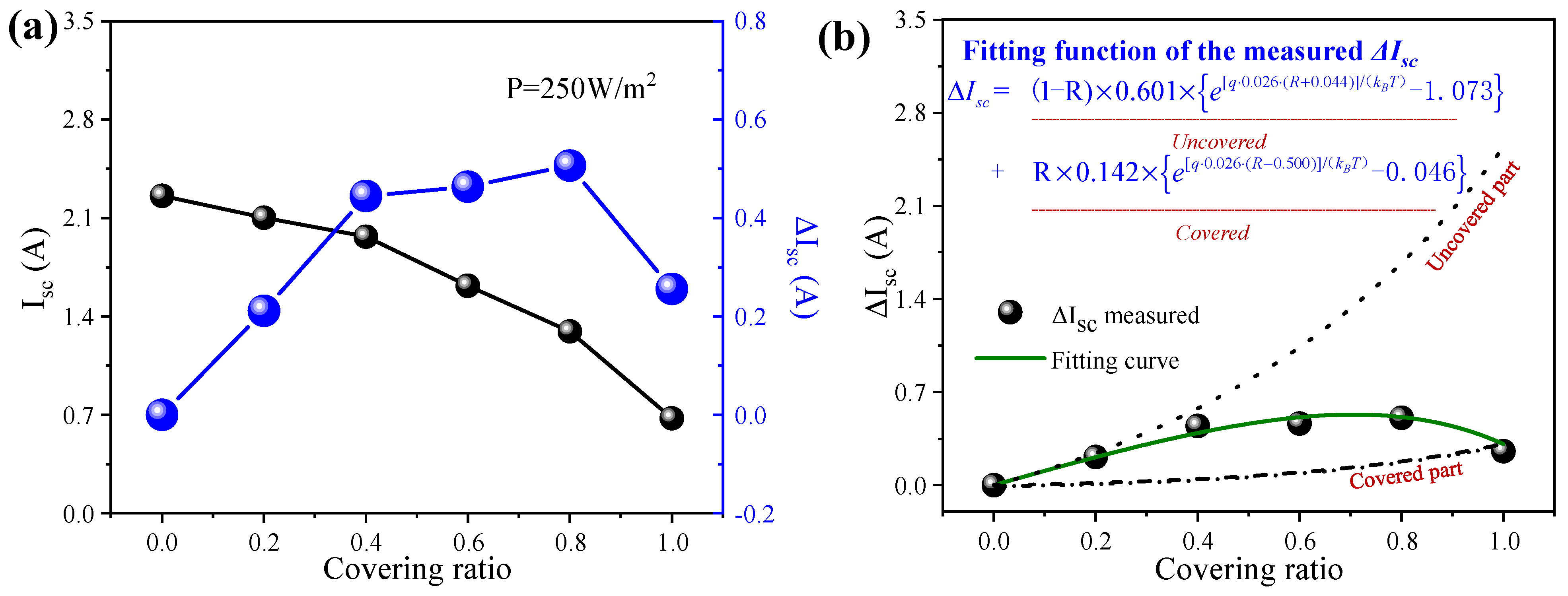
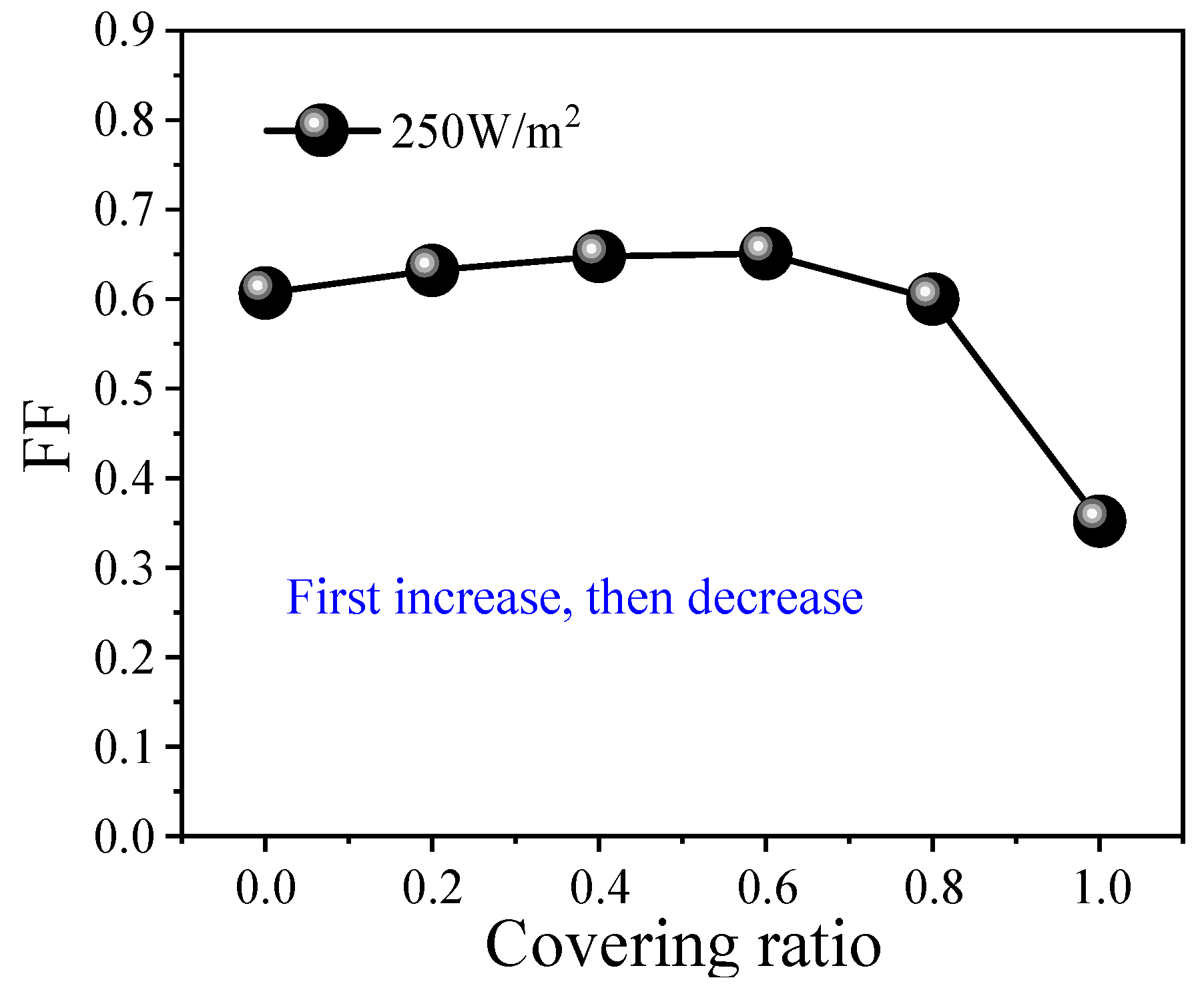
3.2.1. Output of the PV Module When One Cell Is Fully Covered
3.2.2. Impact of Covering Ratio on the Output of the PV Module
3.3. Output of PV String and Array under Semi-Transparent Covering
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- International Energy Agency. World Energy Outlook 2022; IEA: Paris, France, 2022. [Google Scholar]
- RTS Corporate. Forecasting PV Installed Capacity in Japan toward FY 2030; RTS Corporate: Tokyo, Japan, 2022. [Google Scholar]
- Global Energy Interconnection Development and Cooperation Organization. China’s Path Carbon Neutrality; Global Energy Interconnection Development and Cooperation Organization: Beijing, China, 2021. [Google Scholar]
- National Energy Administration of China. China’s Installed Capacity of Renewable Energy Exceeds That of Coal-Fired Power. Available online: http://www.nea.gov.cn/2023-08/04/c_1310735564.htm (accessed on 4 August 2023).
- National Energy Administration of China. Operation Status of Photovoltaic Power Generation Construction in the First Half of 2023. Available online: http://www.nea.gov.cn/2023-07/27/c_1310734298.htm (accessed on 27 July 2023).
- China Renewable Energy Engineering Institute. China Renewable Energy Development Report of 2022. Available online: http://www.creei.cn/userfiles/site/735bdbbfd56241a78ae2895f232e95f1.pdf (accessed on 1 July 2023).
- Ghosh, A. Potential of building integrated and attached/applied photovoltaic (BIPV/BAPV) for adaptive less energy-hungry building’s skin: A comprehensive review. J. Clean. Prod. 2020, 276, 123343. [Google Scholar] [CrossRef]
- Reddy, P.; Gupta, M.S.; Nundy, S.; Karthick, A.; Ghosh, A. Status of BIPV and BAPV system for less energy-hungry building in India—A review. Appl. Sci. 2020, 10, 2337. [Google Scholar] [CrossRef]
- Dos SantosÍ, P.D.; Rüther, R. The potential of building-integrated (BIPV) and building-applied photovoltaics (BAPV) in single-family, urban residences at low latitudes in Brazil. Energy Build. 2012, 50, 290–297. [Google Scholar] [CrossRef]
- Kumar, N.M.; Sudhakar, K.; Samykano, M. Performance comparison of BAPV and BIPV systems with c-Si, CIS and CdTe photovoltaic technologies under tropical weather conditions. Case Stud. Therm. Eng. 2019, 13, 100374. [Google Scholar]
- Toledo, O.M.; Filho, D.O.; Diniz, A.S.A.C. Distributed photovoltaic generation and energy storage systems: A review. Renew. Sustain. Energy Rev. 2010, 14, 506–511. [Google Scholar] [CrossRef]
- Sisodia, A.K.; Mathur, R.K. Impact of bird dropping deposition on solar photovoltaic module performance: A systematic study in Western Rajasthan. Environ. Sci. Pollut. Res. Int. 2019, 26, 31119–31132. [Google Scholar] [CrossRef] [PubMed]
- Ali, K.A.M.A. Performance Evaluation of Solar Photovoltaic Panels Under Bird Droppings Accumulation Using Thermography. MRS Energy Sustain. 2024, 11, 150–160. [Google Scholar]
- Ghosh, S.; Singh, S.K.; Yadav, V.K. Experimental investigation of hot-spot phenomenon in PV arrays under mismatch conditions. Sol. Energy 2023, 253, 219–230. [Google Scholar] [CrossRef]
- Sulaiman, S.A.; Hussain, H.H.; Leh, N.; Razali, M.S. Effects of dust on the performance of PV panels. World Acad. Sci. Eng. Technol. 2011, 58, 588–593. [Google Scholar]
- Fan, S.; Wang, Y.; Cao, S.; Sun, T.; Liu, P. A novel method for analyzing the effect of dust accumulation on energy efficiency loss in photovoltaic (PV) system. Energy 2021, 234, 121112. [Google Scholar] [CrossRef]
- Barbieri, F.; Rajakaruna, S.; Ghosh, A. Very short-term photovoltaic power forecasting with cloud modeling: A review. Renew. Sustain. Energy Rev. 2017, 75, 242–263. [Google Scholar] [CrossRef]
- Järvelä, M.; Lappalainen, K.; Valkealahti, S. Characteristics of the cloud enhancement phenomenon and PV power plants. Sol. Energy 2020, 196, 137–145. [Google Scholar] [CrossRef]
- Kern, E.C.; Gulachenski, E.M.; Kern, G.A. Cloud effects on distributed photovoltaic generation: Slow transients at the Gardner, Massachusetts photovoltaic experiment. IEEE Trans. Energy Convers. 1989, 4, 184–190. [Google Scholar] [CrossRef]
- Alonsogarcia, M.; Ruiz, J.; Chenlo, F. Experimental study of mismatch and shading effects in the I–V characteristic of a photovoltaic module. Sol. Energy Mater. Sol. Cells 2006, 90, 329–340. [Google Scholar] [CrossRef]
- Wu, Z.; Hu, Y.; Wen, J.X.; Zhou, F.; Ye, X. A review for solar panel fire accident prevention in large-scale PV applications. IEEE Access. 2020, 8, 132466–132480. [Google Scholar] [CrossRef]
- Mazziotti, L.; Cancelliere, P.; Paduano, G.; Setti, P.; Sassi, S. Fire risk related to the use of PV systems in building. EDP Sci. 2016, 46, 05001. [Google Scholar] [CrossRef]
- Quaschning, V.; Hanitsch, R. Numerical simulation of current-voltage characteristics of photovoltaic systems with shaded solar cells. Sol. Energy 1996, 56, 513–520. [Google Scholar] [CrossRef]
- Jung, T.H.; Ko, J.W.; Kang, G.H.; Ahn, H.K. Output characteristics of PV module considering partially reverse biased conditions. Sol. Energy 2013, 92, 214–220. [Google Scholar] [CrossRef]
- Wenham, S.R.; Green, M.A.; Watt, M.E.; Corkish, R.; Sproul, A. Applied Photovoltaics; Routledge: London, UK, 2013. [Google Scholar]
- Ross, R.G., Jr.; Smokler, M. Flat-Plate Solar Array Project: Final Report, Volume 6, Engineering Sciences and Reliability; Jet Propulsion Lab: Pasadena, CA, USA, 1986.


| Covering Ratio | P (W/m2) | Ideal Power (W) | Power (W) | Power Loss (W) | Loss Ratio | Theoretical Loss Ratio |
|---|---|---|---|---|---|---|
| 0 | 250 | 0.775 | - | - | 0% | |
| 500 | 1.507 | - | - | |||
| 750 | 2.159 | - | - | |||
| 1/5 | 250 | 0.775 | 0.664 | 0.111 | 14.32% | 16.29% |
| 500 | 1.507 | 1.248 | 0.259 | 17.19% | ||
| 750 | 2.159 | 1.848 | 0.311 | 14.40% | ||
| 2/5 | 250 | 0.775 | 0.519 | 0.256 | 33.03% | 32.58% |
| 500 | 1.507 | 1.037 | 0.470 | 31.19% | ||
| 750 | 2.159 | 1.507 | 0.652 | 30.20% | ||
| 3/5 | 250 | 0.775 | 0.388 | 0.387 | 49.94% | 48.87% |
| 500 | 1.507 | 0.772 | 0.735 | 48.77% | ||
| 750 | 2.159 | 1.117 | 1.042 | 48.26% | ||
| 4/5 | 250 | 0.775 | 0.276 | 0.499 | 64.39% | 65.16% |
| 500 | 1.507 | 0.548 | 0.959 | 63.64% | ||
| 750 | 2.159 | 0.797 | 1.362 | 63.08% | ||
| 1 | 250 | 0.775 | 0.154 | 0.621 | 80.13% | 81.45% |
| 500 | 1.507 | 0.324 | 1.183 | 78.50% | ||
| 750 | 2.159 | 0.459 | 1.700 | 78.74% |
| Covering Ratio | Isc (A) | Voc (V) | FF | Ideal Power (W) | Actual Power (W) | Power Loss (W) | Loss Ratio |
|---|---|---|---|---|---|---|---|
| 0 | 2.258 | 11.255 | 0.607 | 15.495 | 15.278 | 0.217 | 1.40% |
| 1/5 | 2.102 | 11.125 | 0.632 | 15.495 | 14.816 | 0.679 | 4.38% |
| 2/5 | 1.967 | 11.165 | 0.648 | 15.495 | 14.200 | 1.295 | 8.36% |
| 3/5 | 1.618 | 11.120 | 0.651 | 15.495 | 11.731 | 3.764 | 24.29% |
| 4/5 | 1.293 | 11.187 | 0.600 | 15.495 | 8.679 | 6.816 | 43.99% |
| 1 | 0.675 | 11.223 | 0.352 | 15.495 | 2.666 | 12.829 | 82.79% |
| Covering Ratio | Isc (A) | Voc (V) | FF | |||
|---|---|---|---|---|---|---|
| Module | String | Module | String | Module | String | |
| 0 | 2.258 | 2.221 | 11.255 | 22.040 | 0.601 | 0.607 |
| 1/5 | 2.102 | 2.184 | 11.125 | 22.039 | 0.634 | 0.612 |
| 2/5 | 1.967 | 2.109 | 11.165 | 22.037 | 0.647 | 0.612 |
| 3/5 | 1.618 | 2.286 | 11.120 | 22.034 | 0.652 | 0.519 |
| 4/5 | 1.293 | 1.465 | 11.187 | 22.033 | 0.600 | 0.389 |
| 1 | 0.675 | 1.460 | 11.223 | 22.030 | 0.352 | 0.181 |
| Covering Ratio | Isc (A) | Voc (V) | FF | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Array | String 12 | String 34 | Array | String 12 | String 34 | Array | String 12 | String 34 | |
| 0 | 4.450 | 2.221 | 2.150 | 22.103 | 22.040 | 22.244 | 0.642 | 0.607 | 0.686 |
| 1/5 | 4.419 | 2.184 | 2.150 | 22.102 | 22.039 | 22.244 | 0.638 | 0.612 | 0.686 |
| 2/5 | 4.297 | 2.109 | 2.150 | 22.099 | 22.037 | 22.244 | 0.629 | 0.612 | 0.686 |
| 3/5 | 4.073 | 2.286 | 2.150 | 22.097 | 22.034 | 22.244 | 0.594 | 0.519 | 0.686 |
| 4/5 | 3.910 | 1.465 | 2.150 | 22.088 | 22.033 | 22.244 | 0.560 | 0.389 | 0.686 |
| 1 | 3.814 | 1.460 | 2.150 | 22.085 | 22.030 | 22.244 | 0.477 | 0.181 | 0.686 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Liu, Z.; Liu, D.; Wang, Z.; Gong, Y.; Gao, W.; Liu, Y.; An, J.; Li, M. The Mechanism of the Semi-Transparent Coverings Affecting the Power Generation Capacity of the Photovoltaic Module and Array. Energies 2024, 17, 1601. https://doi.org/10.3390/en17071601
Li Y, Liu Z, Liu D, Wang Z, Gong Y, Gao W, Liu Y, An J, Li M. The Mechanism of the Semi-Transparent Coverings Affecting the Power Generation Capacity of the Photovoltaic Module and Array. Energies. 2024; 17(7):1601. https://doi.org/10.3390/en17071601
Chicago/Turabian StyleLi, Yingfeng, Zhihan Liu, Dongxue Liu, Zixuan Wang, Yongshuai Gong, Wenxiang Gao, Yingjian Liu, Jiayuan An, and Meicheng Li. 2024. "The Mechanism of the Semi-Transparent Coverings Affecting the Power Generation Capacity of the Photovoltaic Module and Array" Energies 17, no. 7: 1601. https://doi.org/10.3390/en17071601
APA StyleLi, Y., Liu, Z., Liu, D., Wang, Z., Gong, Y., Gao, W., Liu, Y., An, J., & Li, M. (2024). The Mechanism of the Semi-Transparent Coverings Affecting the Power Generation Capacity of the Photovoltaic Module and Array. Energies, 17(7), 1601. https://doi.org/10.3390/en17071601






