Research on Cogging Torque Reduction of Direct-Drive Type Dual-Rotor Radial Flux Permanent Magnet Synchronous Motor for Electric Propulsion Aircraft
Abstract
1. Introduction
2. Machine Topology and Design Considerations
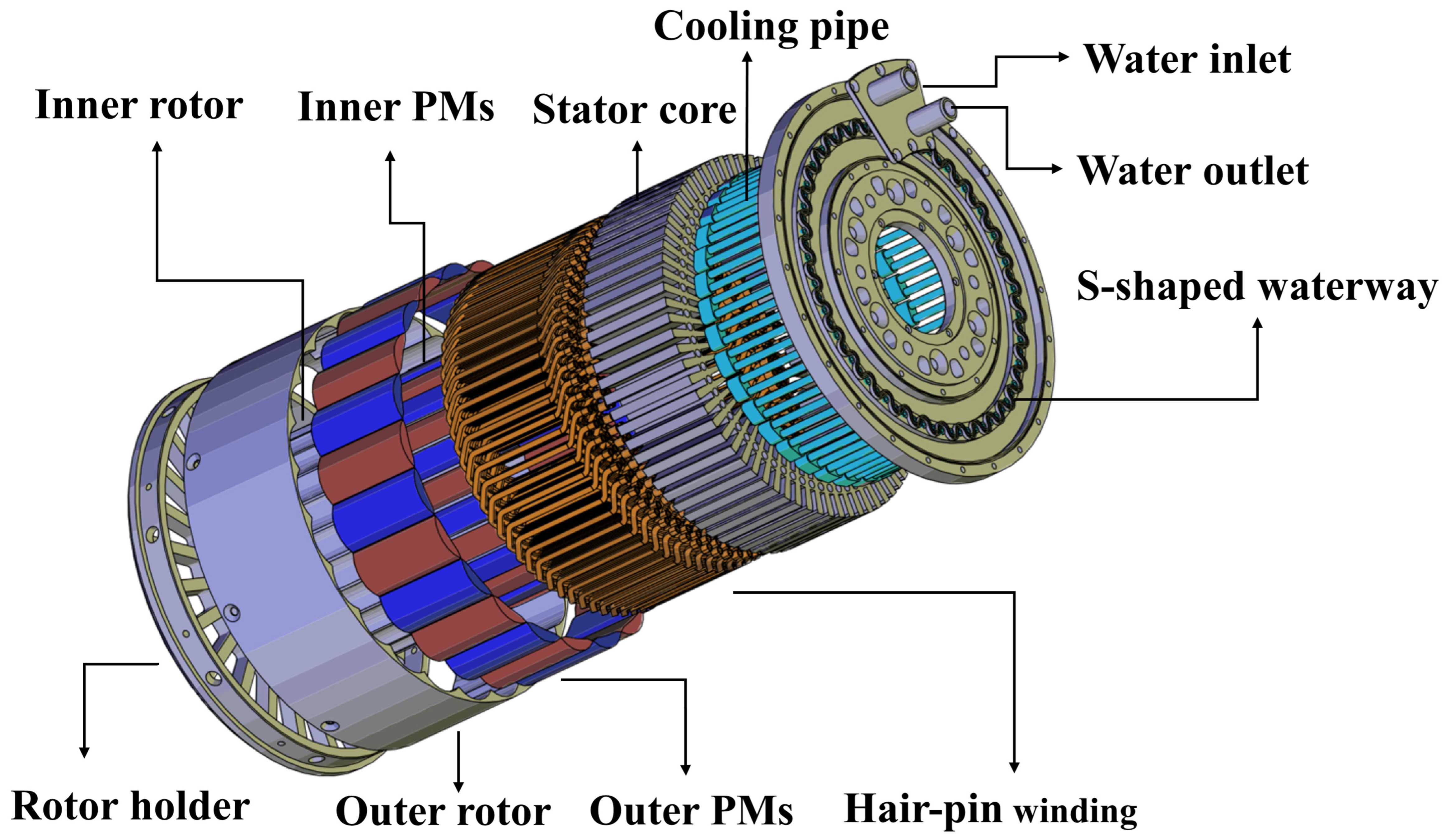
2.1. Machine Structure Design
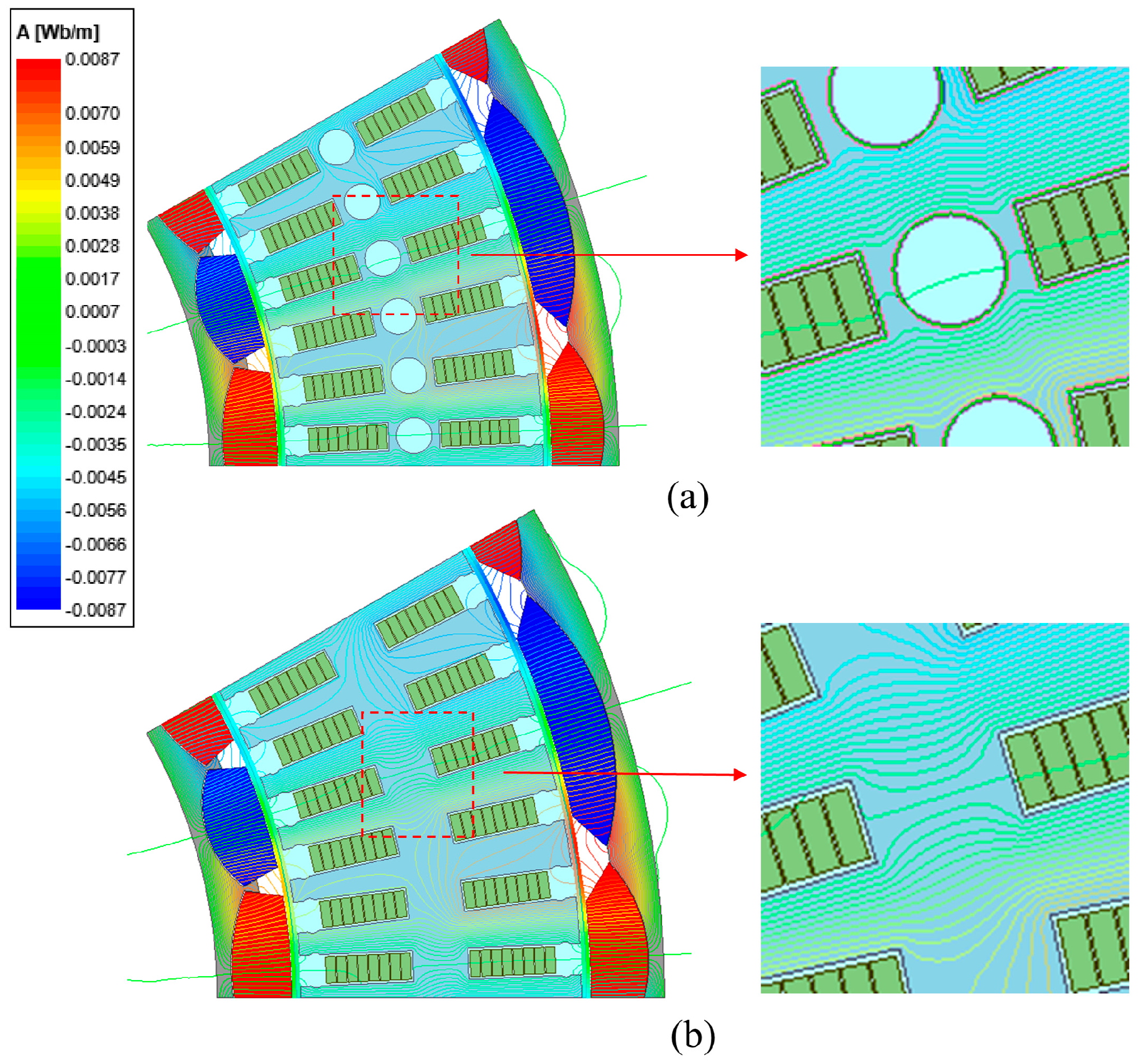
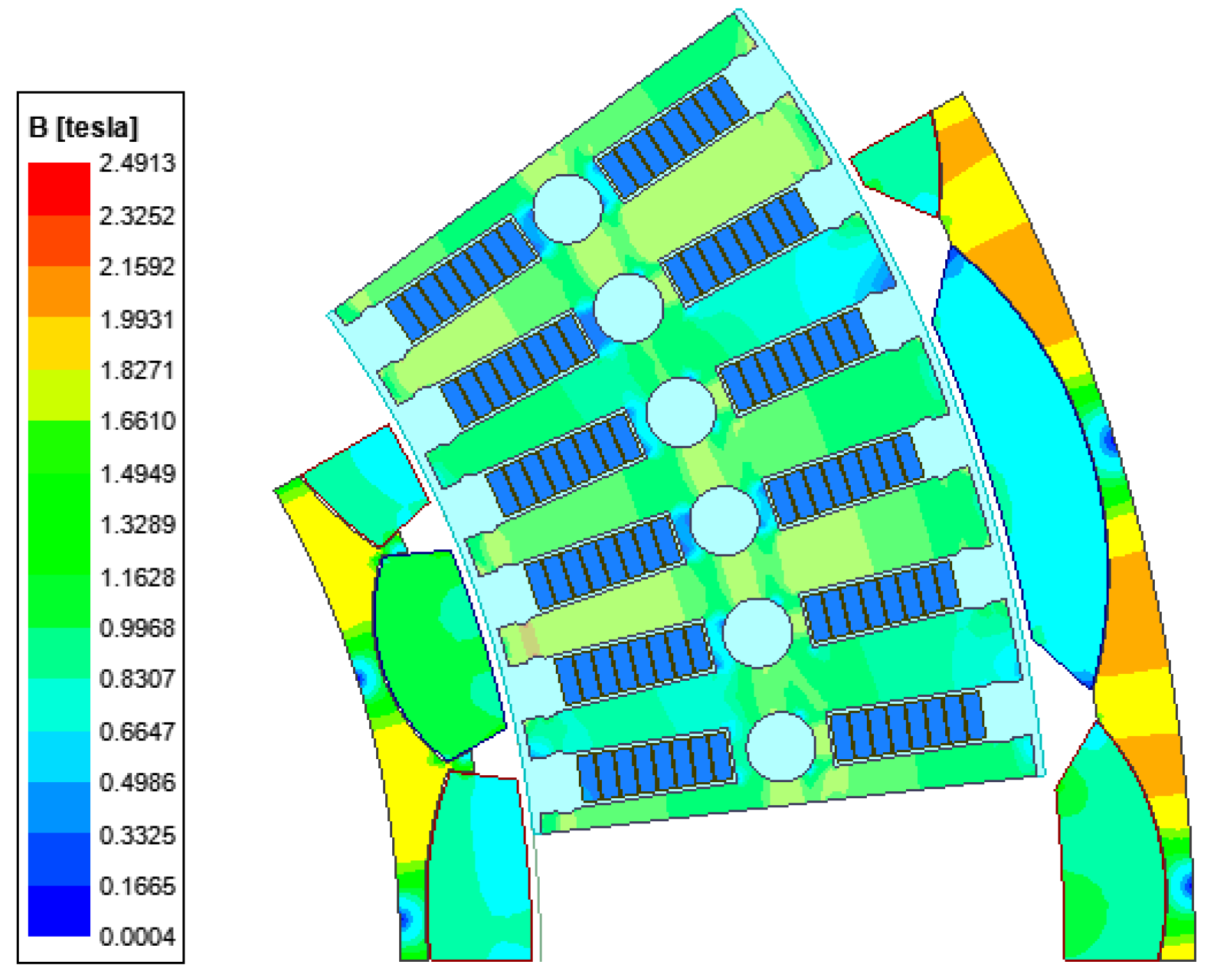
2.2. Magnet Circuit Design
3. Cogging Torque Reduction of DRPMSM
3.1. Permanent Magnet Shape Design
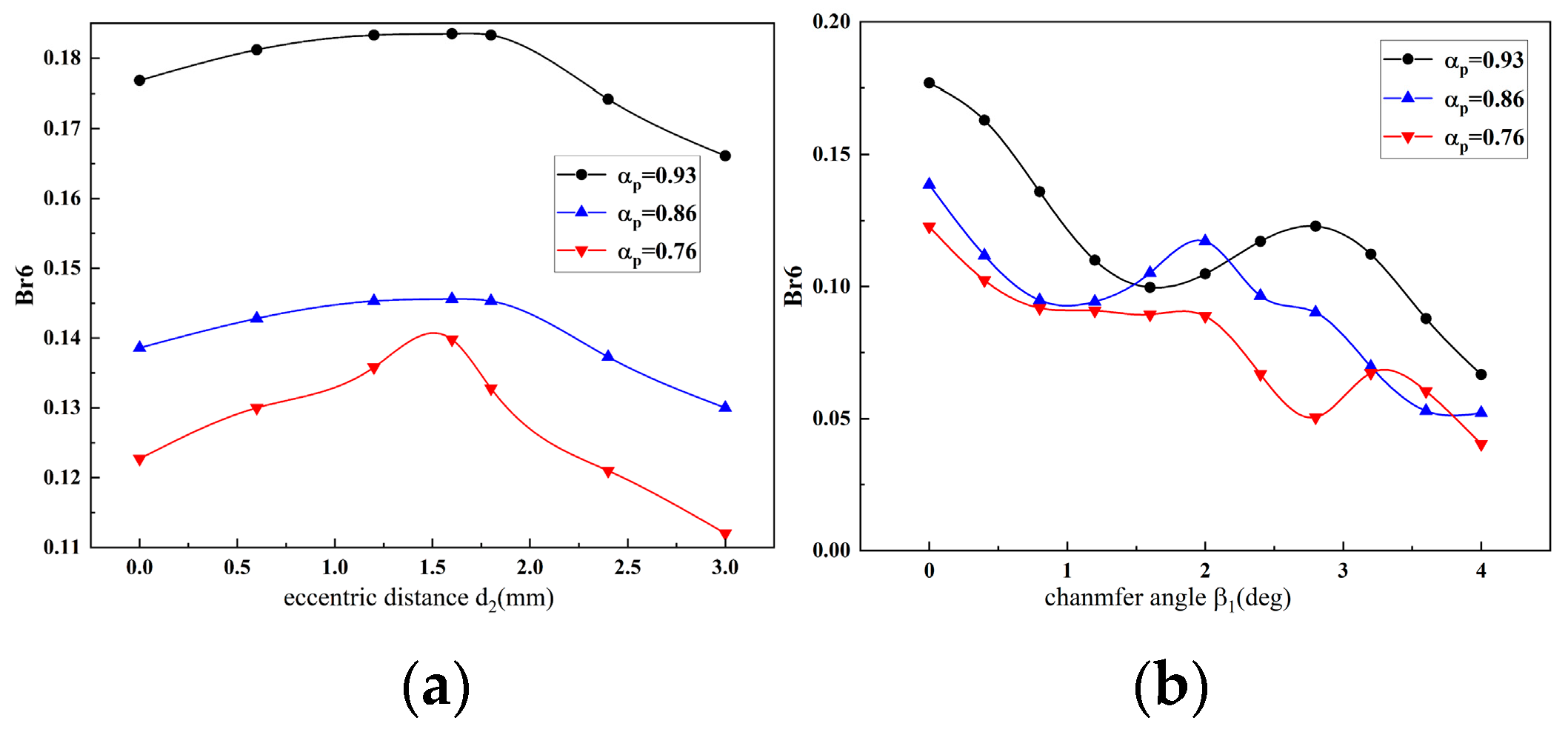
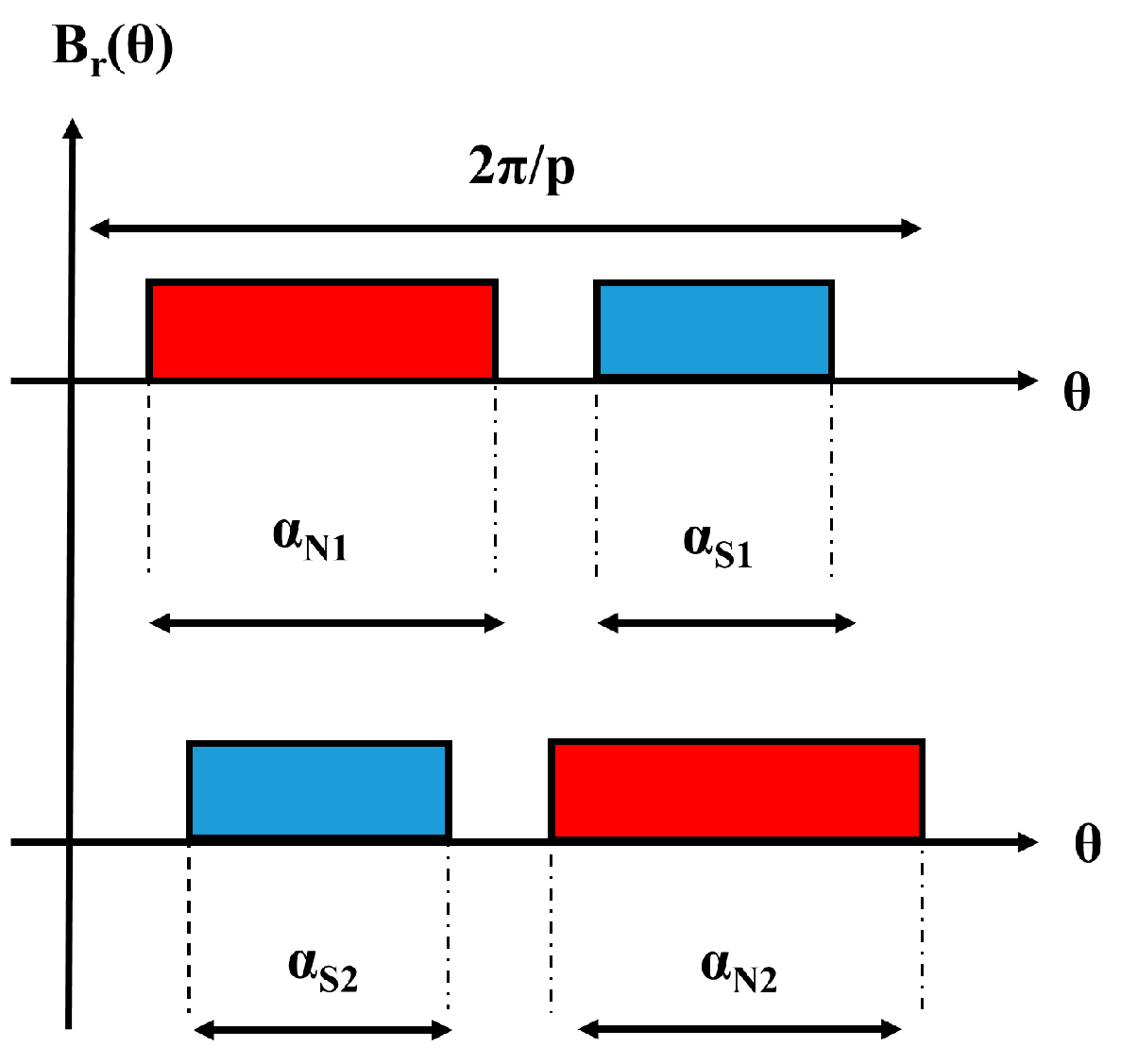
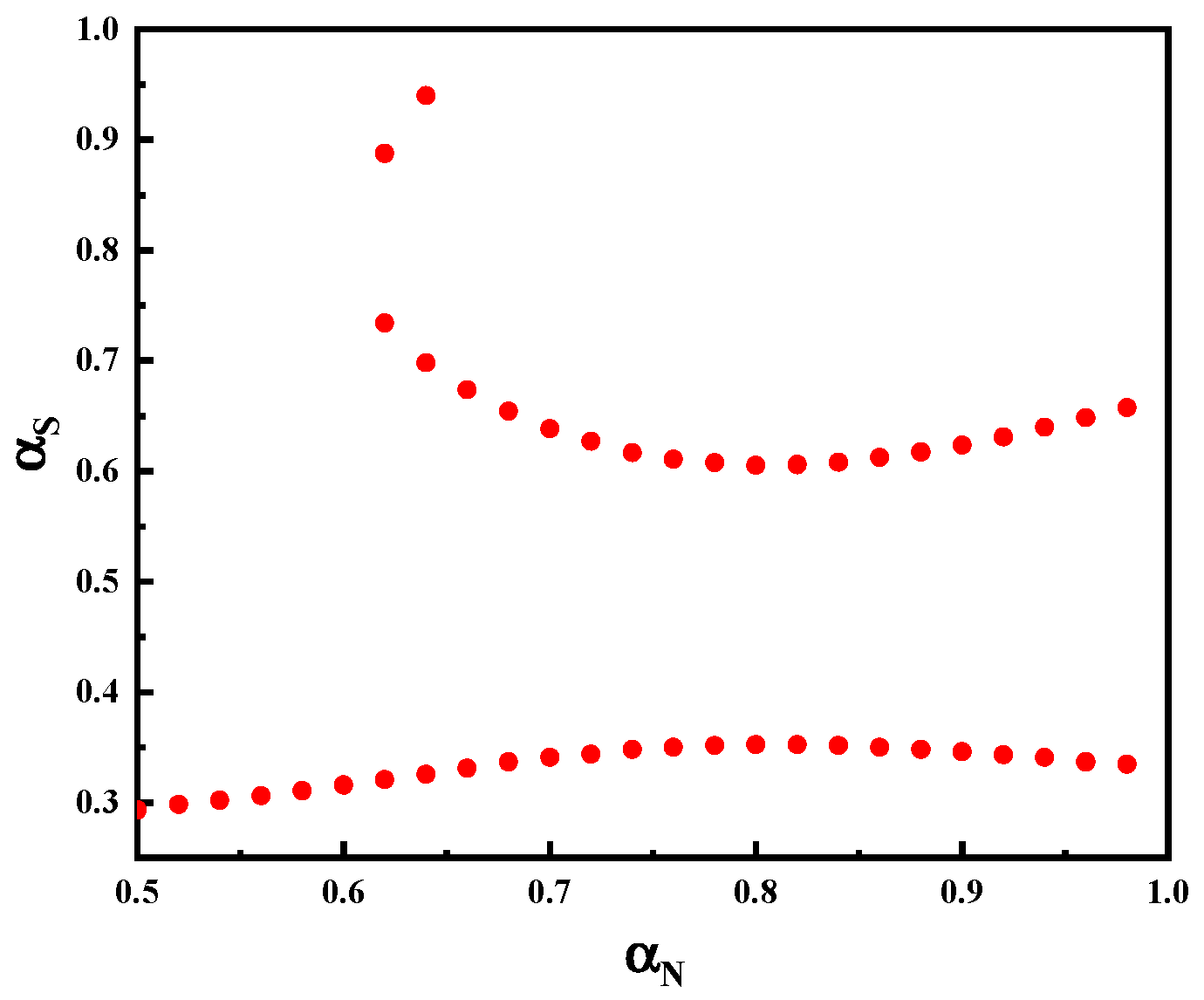
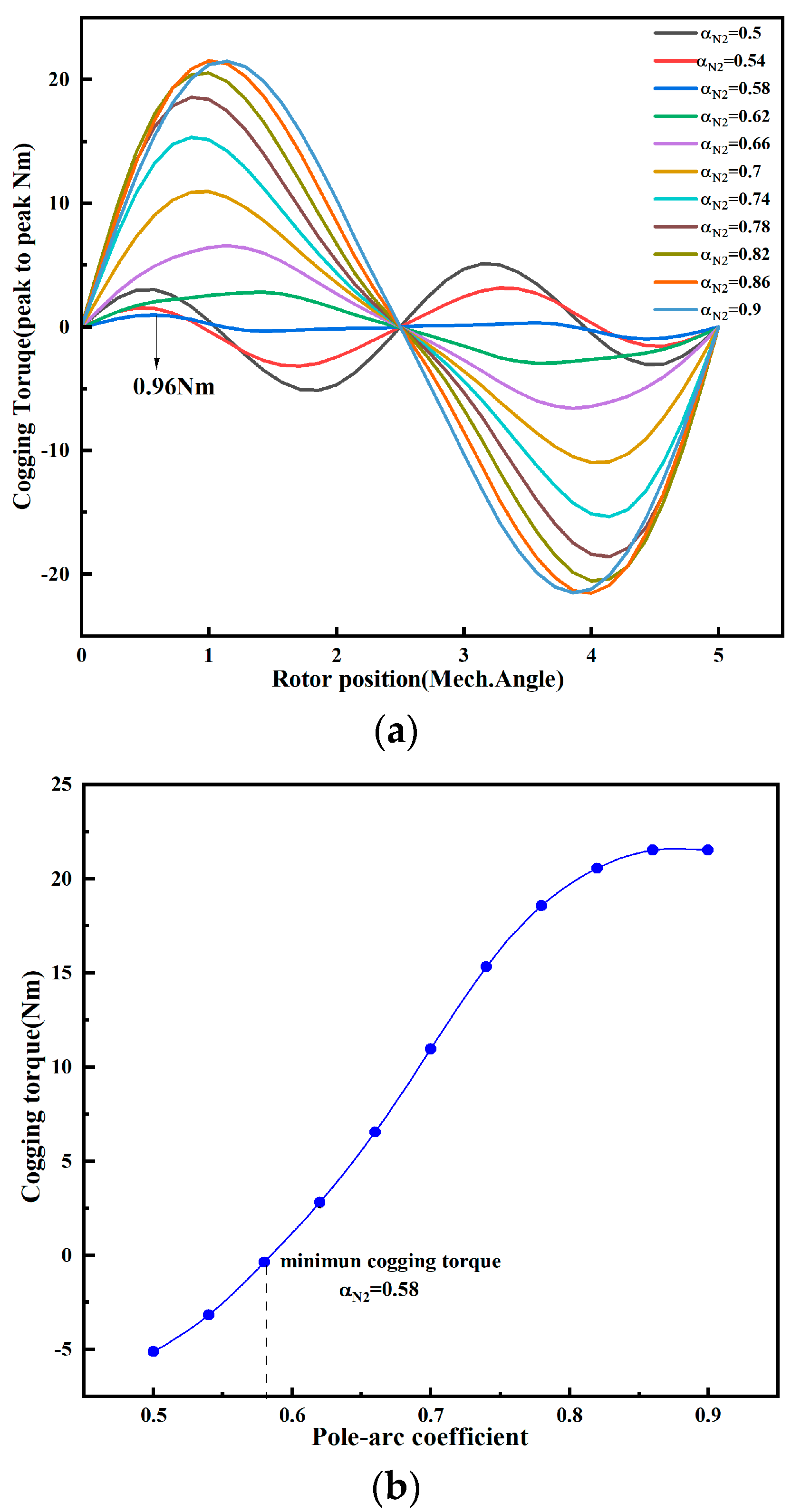
3.2. Pole-Arc Combination Design
- (1)
- Method one:
- (2)
- Method two:
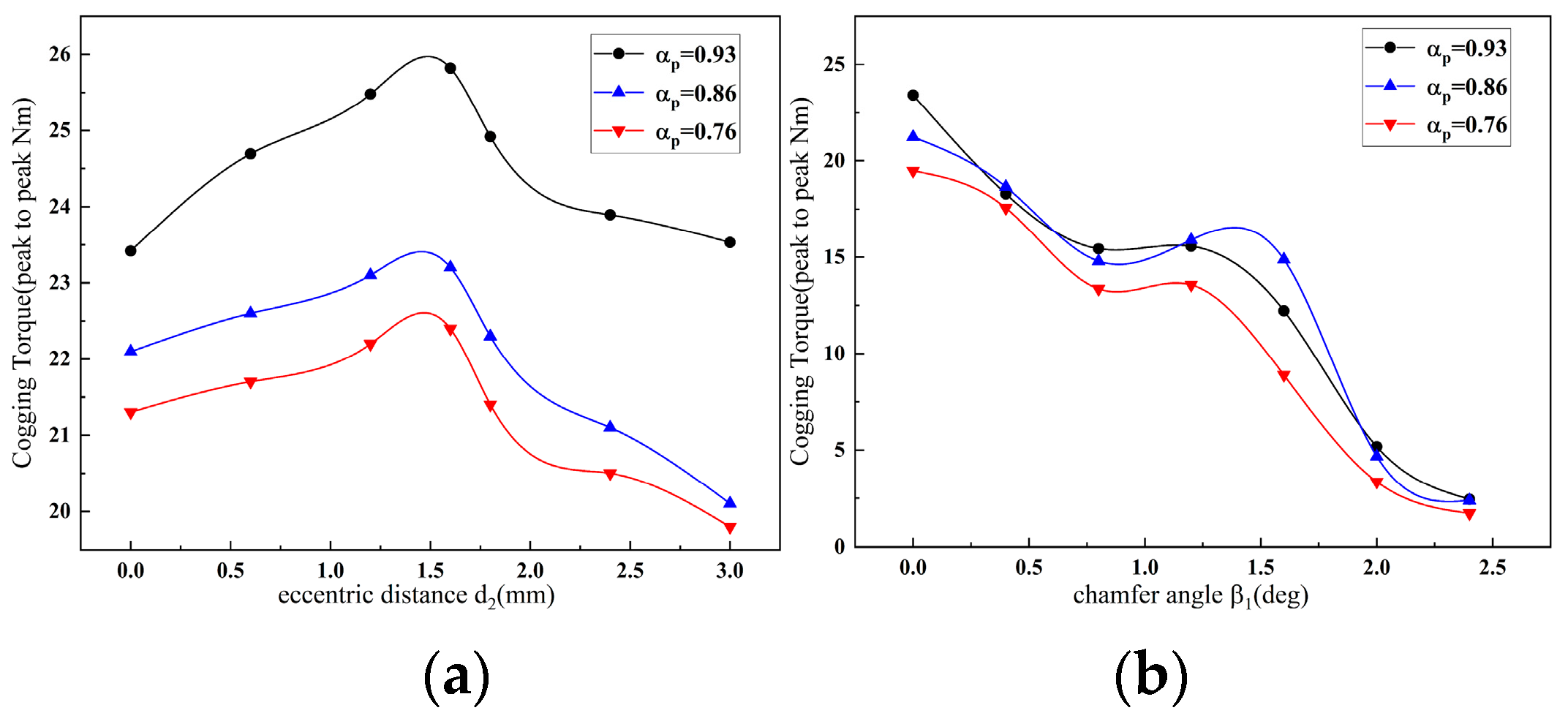
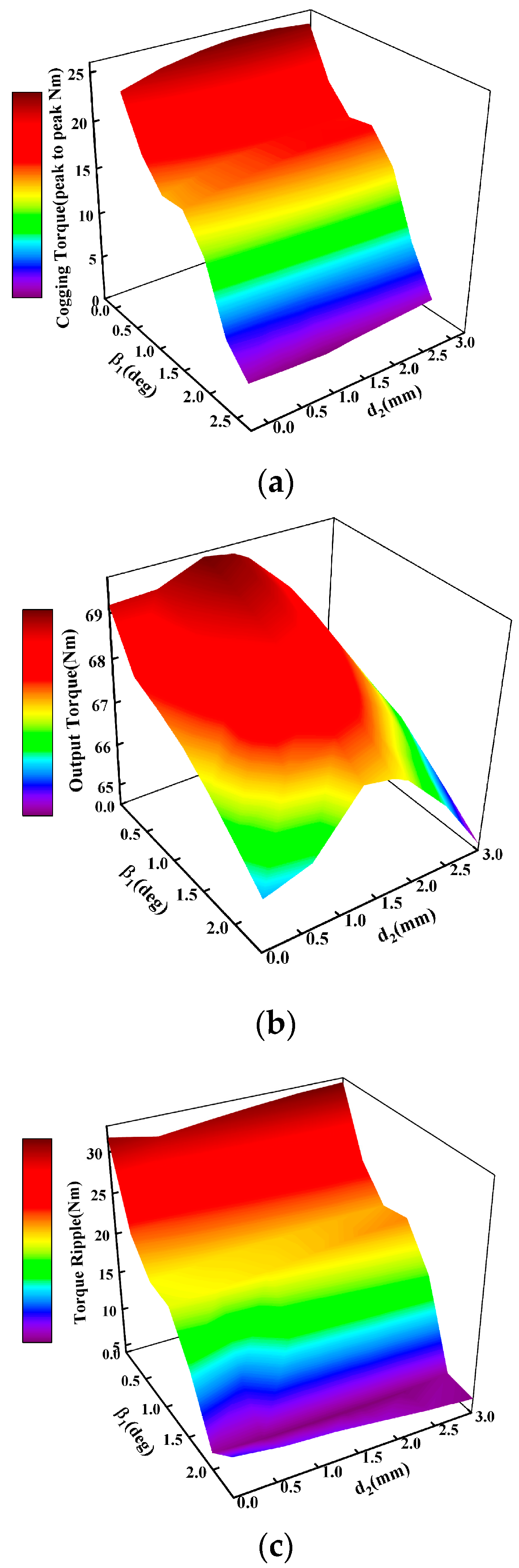
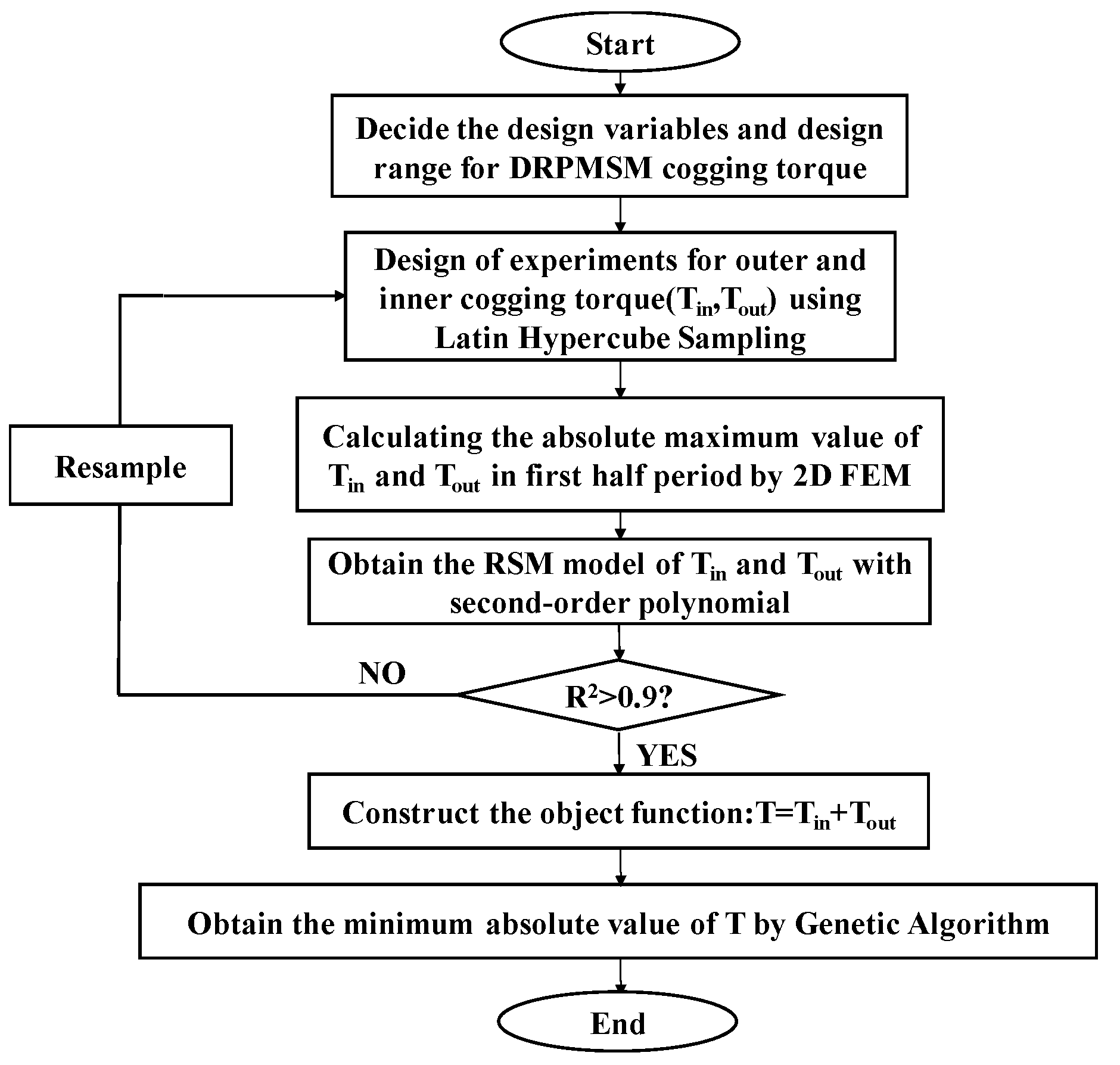
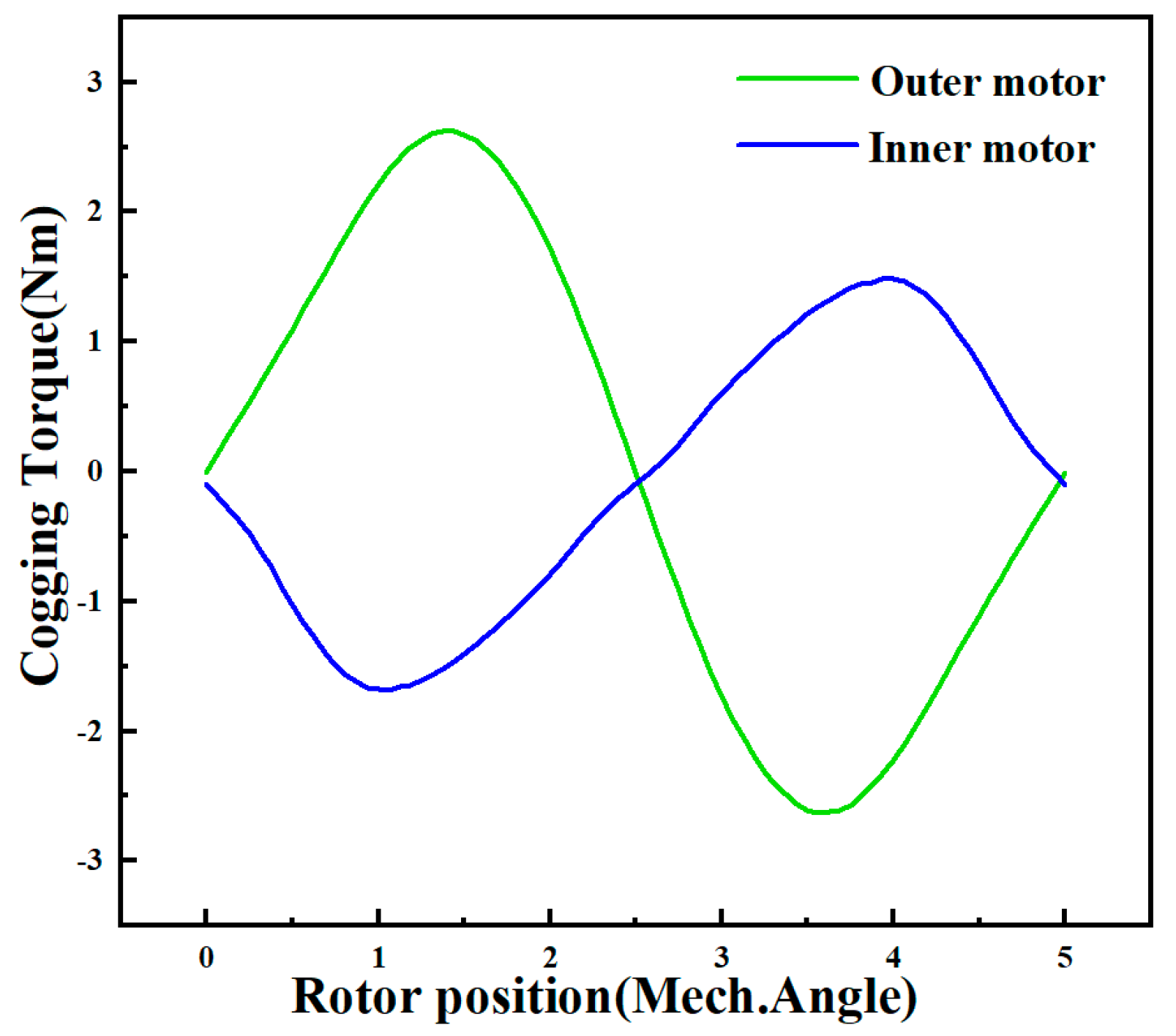
3.3. Cogging Torque Optimization
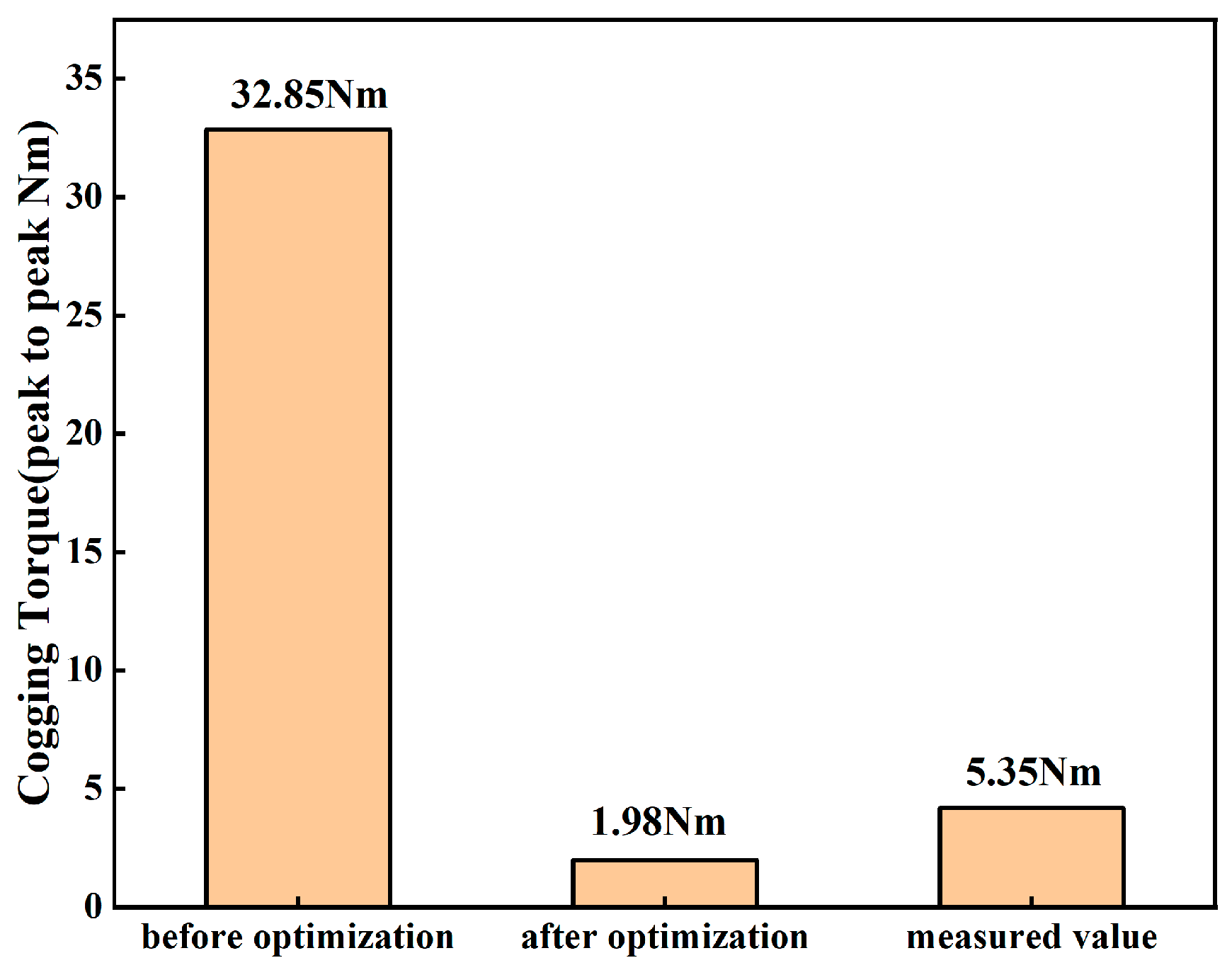
4. Experimental Validation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tabuchi, H. Worse than Anyone Expected’: Air Travel Emissionsvastly Outpace Predictions. The New York Times, 19 September 2019. [Google Scholar]
- Wheeler, P.W.; Sirimanna, T.S.; Bozhko, S.V.; Haran, K.S. Electric/Hybrid-Electric Aircraft Propulsion Systems. Proc. IEEE 2021, 109, 1115–1127. [Google Scholar] [CrossRef]
- Agrawal, S.; Banerjee, A.; Beach, R.F. Brushless Doubly-Fed Reluctance Machine Drive for Turbo-Electric Distributed Propulsion Systems. In Proceedings of the 2018 AIAA/IEEE Electric Aircraft Technologies Symposium (EATS), Cincinnati, OH, USA, 12 July 2018; pp. 1–17. [Google Scholar]
- Brown, G.V. Weights and Efficiencies of Electric Components of a Turboelectric Aircraft Propulsion System. In Proceedings of the Weights and Efficiencies of Electric Components of a Turboelectric Aircraft Propulsion System, Orlando, FL, USA, 4–7 January 2011. [Google Scholar]
- Kowalczyk, M.; Vezzini, A.; Grzesiak, L.M. Cylindrical double air gap motor for aerial applications. Przegląd Elektrotechniczny 2013, 2000, 150. [Google Scholar]
- Cao, W.; Mecrow, B.C.; Atkinson, G.J.; Bennett, J.W.; Atkinson, D.J. Overview of Electric Motor Technologies Used for More Electric Aircraft (MEA). IEEE Trans. Ind. Electron. 2012, 59, 3523–3531. [Google Scholar]
- Ganev, E. Selecting the Best Electric Machines for Electrical Power-Generation Systems: High-performance solutions for aerospace More electric architectures. IEEE Electrif. Mag. 2014, 2, 13–22. [Google Scholar] [CrossRef]
- Sayed, E.; Abdalmagid, M.; Pietrini, G.; Sa’adeh, N.-M.; Callegaro, A.D.; Goldstein, C.; Emadi, A. Review of Electric Machines in More-/Hybrid-/Turbo-Electric Aircraft. IEEE Trans. Transp. Electrif. 2021, 7, 2976–3005. [Google Scholar] [CrossRef]
- Chandel, D.; Reband, J.D.; Hall, D.K.; Balachandran, T.; Xiao, J.; Haran, K.S.; Greitzer, E.M. Fan and Motor Co-Optimization for a Distributed Electric Aircraft Propulsion System. IEEE Trans. Transp. Electrif. 2023, 9, 3579–3589. [Google Scholar] [CrossRef]
- Fan, Y.; Liang, P.; Liang, L.; Zhang, D.; Liu, W.; Zhang, X. Analytical model of no-load axial force for electric propulsion conical motor in electric aircraft. IET Electr. Power Appl. 2023, 18, 336–344. [Google Scholar] [CrossRef]
- Hebala, A.; Nuzzo, S.; Volpe, G.; Connor, P.H.; Giangrande, P.; Gerada, C.; Galea, M. Feasibility Design Study of High-Performance, High-Power-Density Propulsion Motor for Middle-Range Electric Aircraft. In Proceedings of the 2020 IEEE 29th International Symposium on Industrial Electronics (ISIE), Delft, The Netherlands, 17–19 June 2020; pp. 300–306. [Google Scholar]
- Jike, N.; Mitsuda, H.; Kojima, T.; Hazeyama, M. Design and Fabrication of Dual-Rotor Motors with Axially Extended Stator for Electrified Aircraft Propulsion. In Proceedings of the 2022 25th International Conference on Electrical Machines and Systems (ICEMS), Chiang Mai, Thailand, 29 November–2 December 2022; pp. 1–5. [Google Scholar]
- Talebi, D.; Gardner, M.C.; Sankarraman, S.V.; Daniar, A.; Toliyat, H.A. Electromagnetic Design Characterization of a Dual Rotor Axial Flux Motor for Electric Aircraft. IEEE Trans. Ind. Appl. 2021, 58, 7088–7098. [Google Scholar] [CrossRef]
- Wiley, C.; Talebi, D.; Sankarraman, S.V.; Gardner, M.C.; Benedict, M. Design of a Carbon Fiber Rotor in a Dual Rotor Axial Flux Motor for Electric Aircraft. In Proceedings of the 2022 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 9–13 October 2022; pp. 1–8. [Google Scholar]
- Deodhar, R.P.; Staton, D.A.; Jahns, T.M.; Miller, T.J.E. Prediction of cogging torque using the flux-MMF diagram technique. In Proceedings of the IAS ‘95. Conference Record of the 1995 IEEE Industry Applications Conference Thirtieth IAS Annual Meeting, Orlando, FL, USA, 8–12 October 1995; Volume 691, pp. 693–700. [Google Scholar]
- Le-Huy, H.; Perret, R.; Feuillet, R. Minimization of Torque Ripple in Brushless DC Motor Drives. IEEE Trans. Ind. Appl. 1986, IA-22, 748–755. [Google Scholar]
- Wanjiku, J.; Khan, M.A.; Barendse, P.S.; Pillay, P. Influence of Slot Openings and Tooth Profile on Cogging Torque in Axial-Flux PM Machines. IEEE Trans. Ind. Electron. 2015, 62, 7578–7589. [Google Scholar] [CrossRef]
- Carraro, E.; Bianchi, N.; Zhang, S.; Koch, M. Design and Performance Comparison of Fractional Slot Concentrated Winding Spoke Type Synchronous Motors with Different Slot-Pole Combinations. IEEE Trans. Ind. Appl. 2018, 54, 2276–2284. [Google Scholar] [CrossRef]
- Xia, C.; Chen, Z.; Shi, T.; Wang, H. Cogging Torque Modeling and Analyzing for Surface-Mounted Permanent Magnet Machines with Auxiliary Slots. IEEE Trans. Magn. 2013, 49, 5112–5123. [Google Scholar] [CrossRef]
- Xintong, J.; Jingwei, X.; Yong, L.; Yongping, L. Theoretical and Simulation Analysis of Influences of Stator Tooth Width on Cogging Torque of BLDC Motors. IEEE Trans. Magn. 2009, 45, 4601–4604. [Google Scholar] [CrossRef]
- Dosiek, L.; Pillay, P. Cogging Torque Reduction in Permanent Magnet Machines. IEEE Trans. Ind. Appl. 2006, 43, 1565–1571. [Google Scholar] [CrossRef]
- Hwang, S.-M.; Eom, J.-B.; Jung, Y.; Lee, D.-W.; Kang, B.-S. Various design techniques to reduce cogging torque by controlling energy variation in permanent magnet motors. IEEE Trans. Magn. 2001, 37, 2806–2809. [Google Scholar] [CrossRef]
- Ashabani, M.; Mohamed, Y.A.-R.I. Multiobjective Shape Optimization of Segmented Pole Permanent-Magnet Synchronous Machines with Improved Torque Characteristics. IEEE Trans. Magn. 2011, 47, 795–804. [Google Scholar] [CrossRef]
- Hwang, M.-h.; Lee, H.-S.; Yang, S.-H.; Lee, G.-S.; Han, J.-H.; Kim, D.-H.; Kim, H.-W.; Cha, H.R. Cogging Torque Reduction and Offset of Dual-Rotor Interior Permanent Magnet Motor in Electric Vehicle Traction Platforms. Energies 2019, 12, 1761. [Google Scholar] [CrossRef]
- Qu, R.; Lipo, T.A. Dual-rotor, radial-flux, toroidally-wound, permanent-magnet machines. In Proceedings of the Conference Record of the 2002 IEEE Industry Applications Conference. 37th IAS Annual Meeting (Cat. No.02CH37344), Pittsburgh, PA, USA, 15 October 2002; Volume 1282, pp. 1281–1288. [Google Scholar]
- Zhu, L.; Jiang, S.Z.; Zhu, Z.Q.; Chan, C. Analytical Methods for Minimizing Cogging Torque in Permanent-Magnet Machines. IEEE Trans. Magn. 2009, 45, 2023–2031. [Google Scholar] [CrossRef]



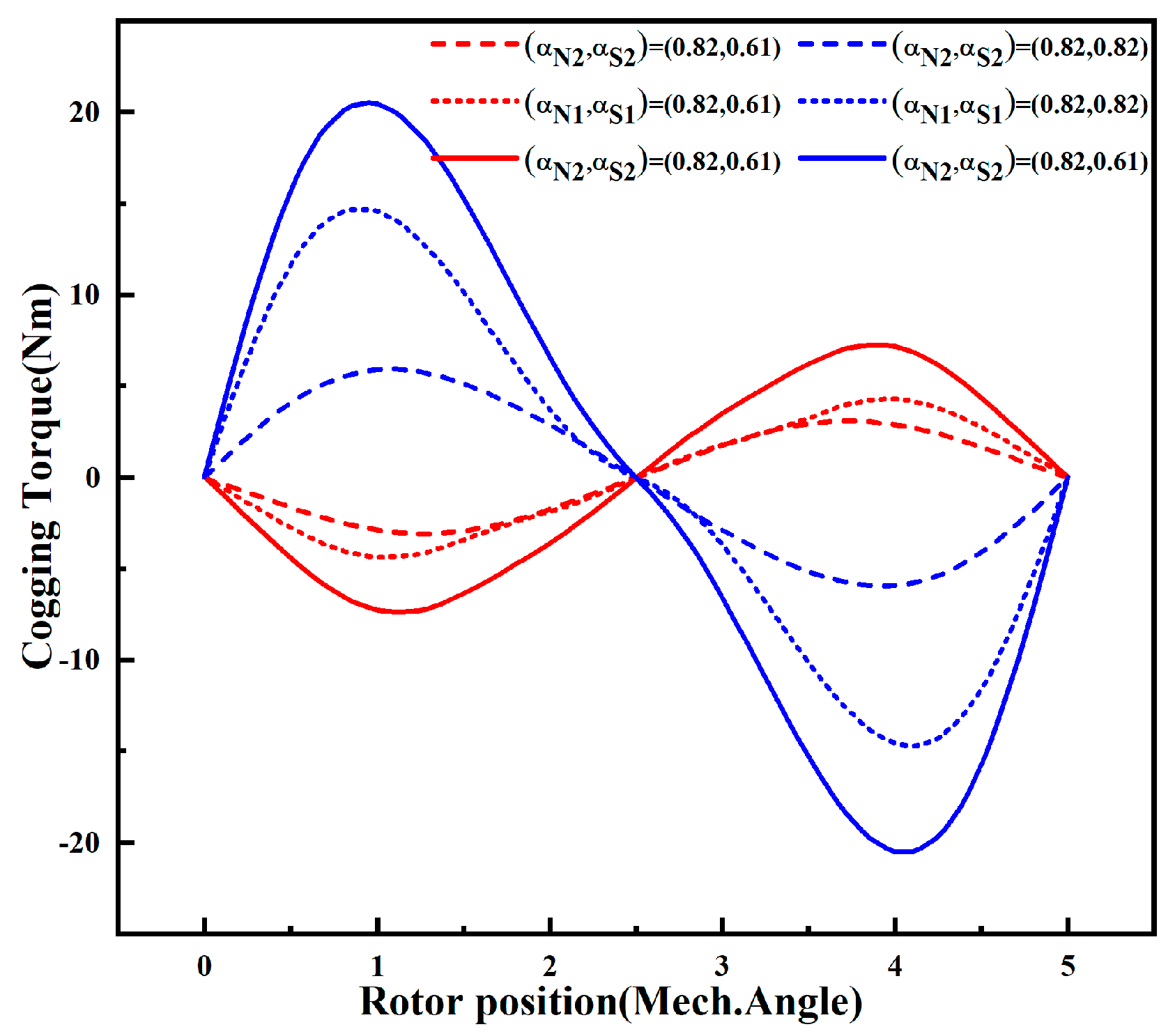
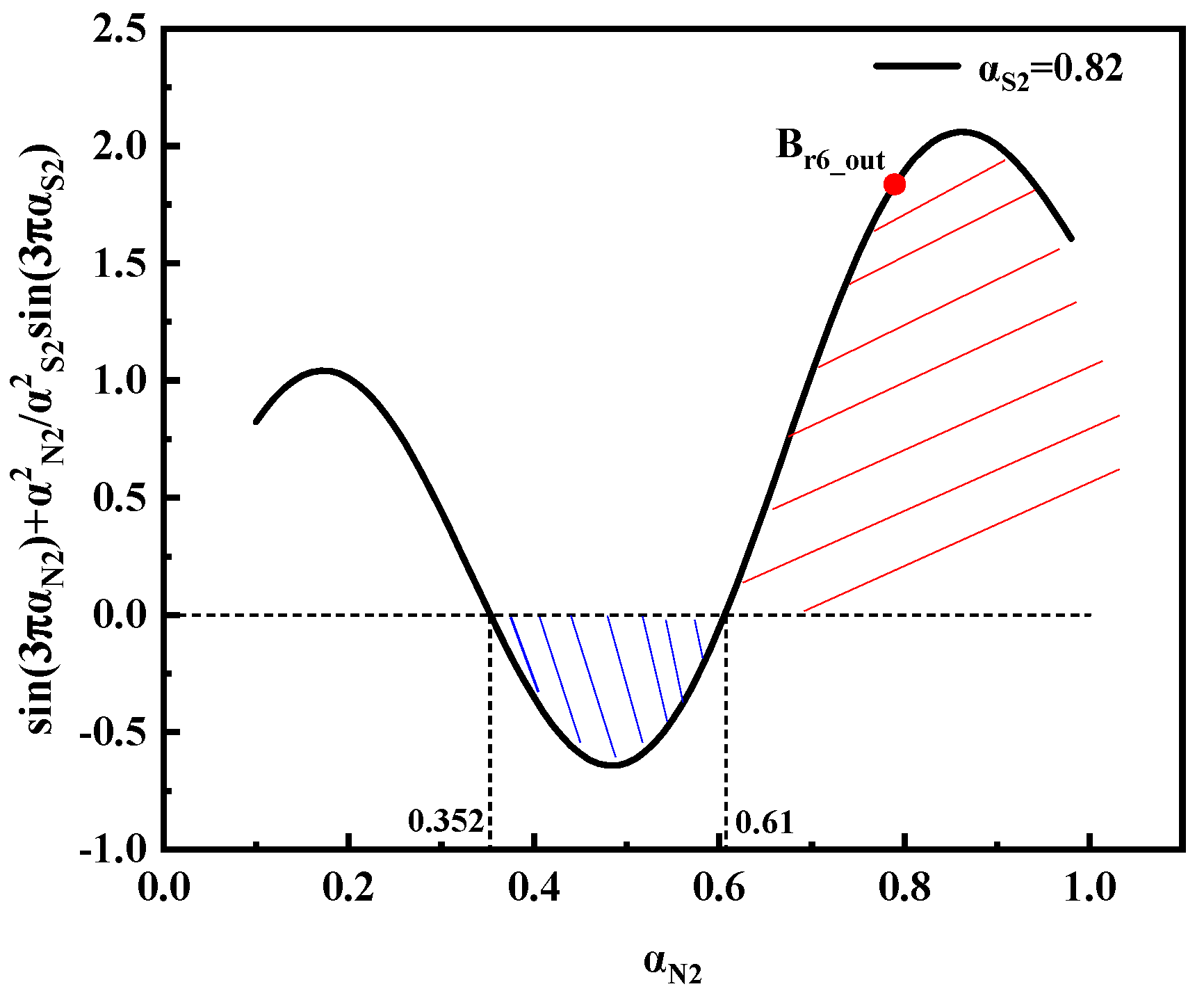
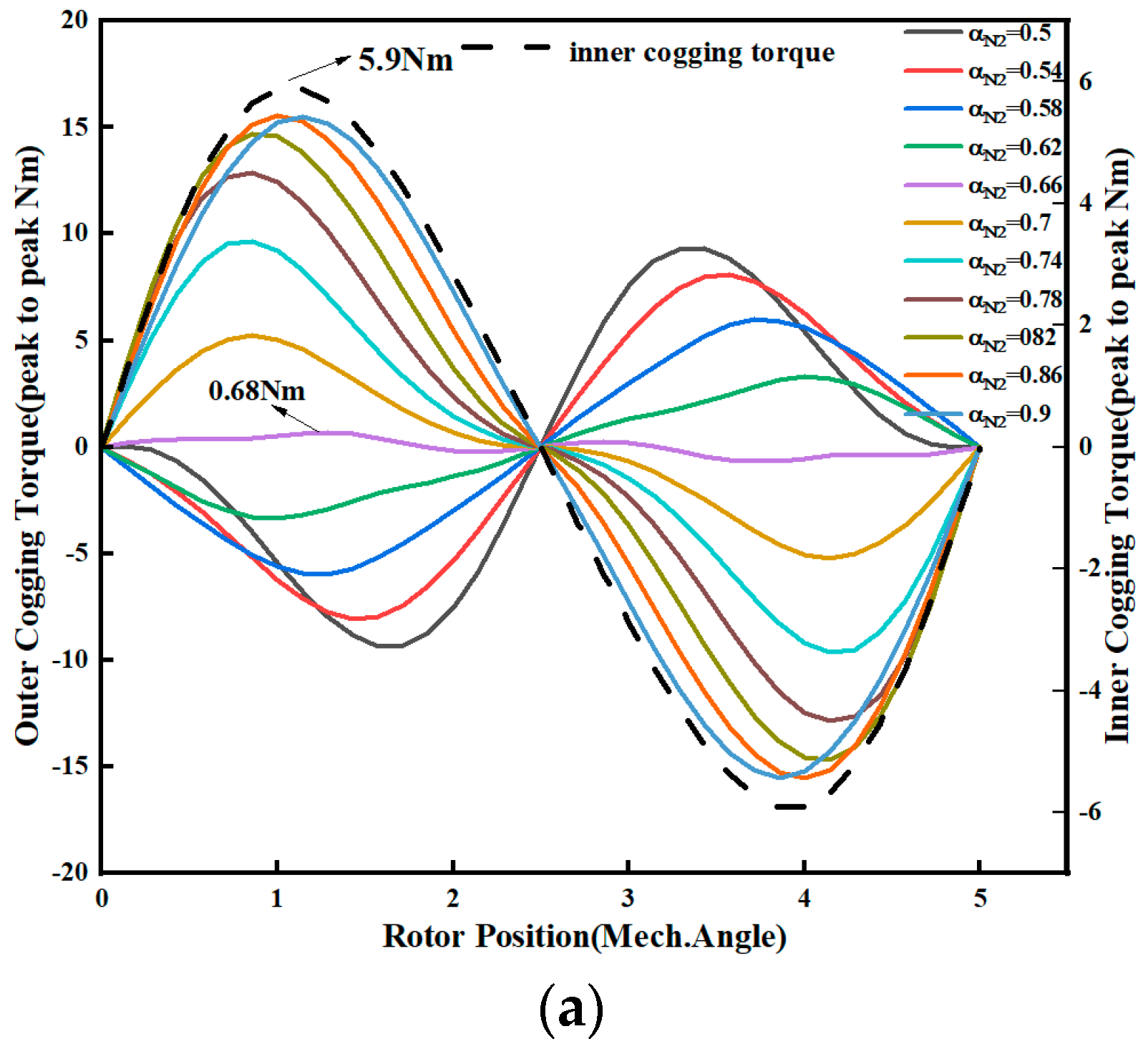
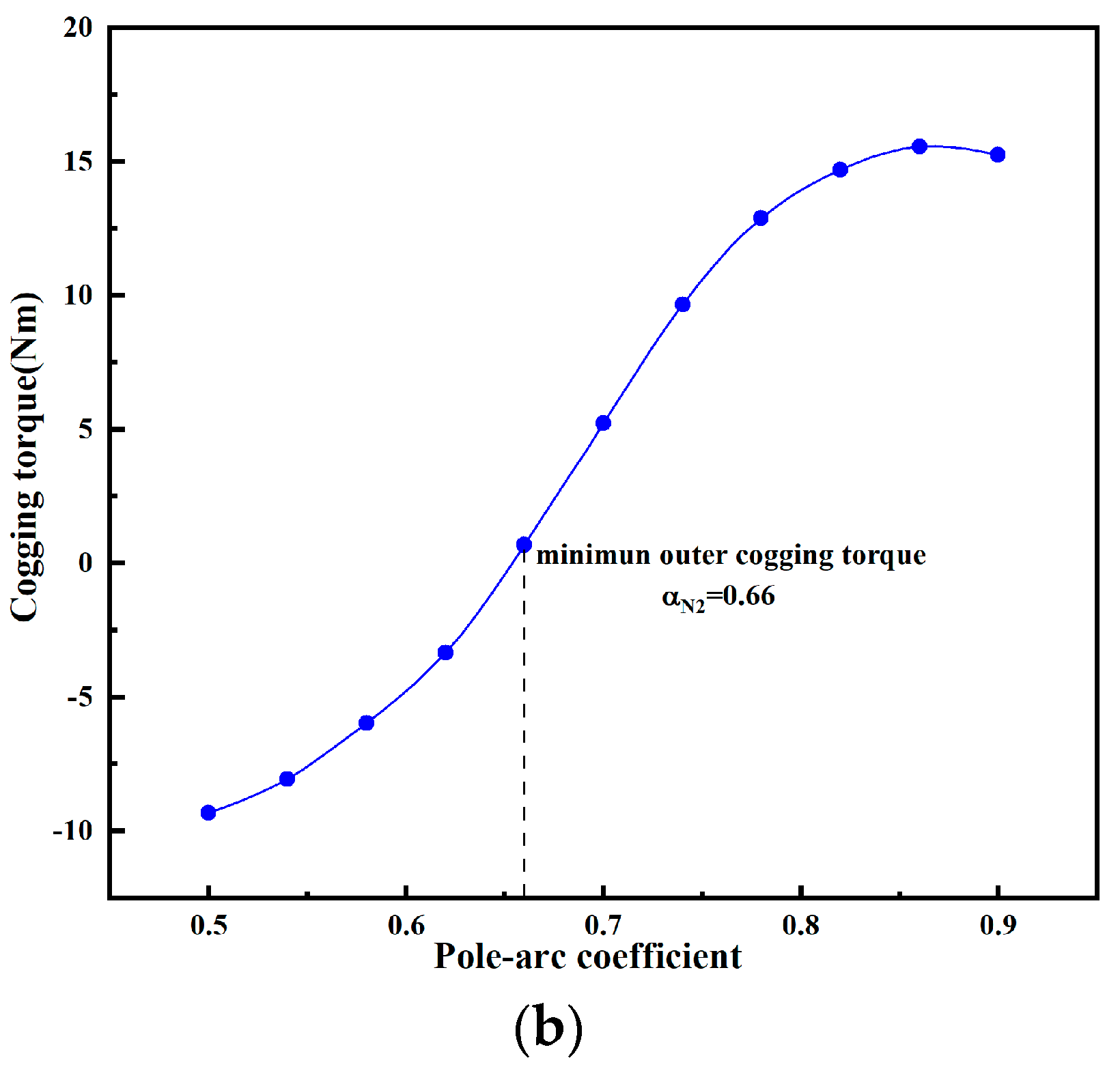


| Hole | No Hole | |
|---|---|---|
| stator core loss | 67.3 W | 77 W |
| average torque | 112.8 Nm | 111.2 Nm |
| torque ripple | 4.6% | 5% |
| Parameter | Initial | Optimized |
|---|---|---|
| α1 (deg) | 0 | 1.91 |
| d1 (mm) | 0 | 1.53 |
| αN1 | 0.86 | 0.67 |
| αS1 | 0.86 | 0.82 |
| β1 (deg) | 0 | 2.24 |
| d2 (mm) | 0 | 2.21 |
| αN2 | 0.86 | 0.86 |
| αS2 | 0.86 | 0.79 |
| Initial | Optimized | |
|---|---|---|
| Cogging torque | 32.85 Nm | 1.21 Nm |
| Average torque | 129.48 Nm | 115.91 Nm |
| Torque ripple | 32.1% | 2.9% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; Feng, M.; Lei, L.; Geng, Y.; Wang, J. Research on Cogging Torque Reduction of Direct-Drive Type Dual-Rotor Radial Flux Permanent Magnet Synchronous Motor for Electric Propulsion Aircraft. Energies 2024, 17, 1583. https://doi.org/10.3390/en17071583
Li H, Feng M, Lei L, Geng Y, Wang J. Research on Cogging Torque Reduction of Direct-Drive Type Dual-Rotor Radial Flux Permanent Magnet Synchronous Motor for Electric Propulsion Aircraft. Energies. 2024; 17(7):1583. https://doi.org/10.3390/en17071583
Chicago/Turabian StyleLi, Haomin, Minglu Feng, Li Lei, Yingsan Geng, and Jianhua Wang. 2024. "Research on Cogging Torque Reduction of Direct-Drive Type Dual-Rotor Radial Flux Permanent Magnet Synchronous Motor for Electric Propulsion Aircraft" Energies 17, no. 7: 1583. https://doi.org/10.3390/en17071583
APA StyleLi, H., Feng, M., Lei, L., Geng, Y., & Wang, J. (2024). Research on Cogging Torque Reduction of Direct-Drive Type Dual-Rotor Radial Flux Permanent Magnet Synchronous Motor for Electric Propulsion Aircraft. Energies, 17(7), 1583. https://doi.org/10.3390/en17071583






