A Review of the Modeling of Parabolic Trough Solar Collectors Coupled to Solar Receivers with Photovoltaic/Thermal Generation
Abstract
1. Introduction
2. Methodology of the State-of-the-Art Review
2.1. Previous State-of-the-Art Review Papers
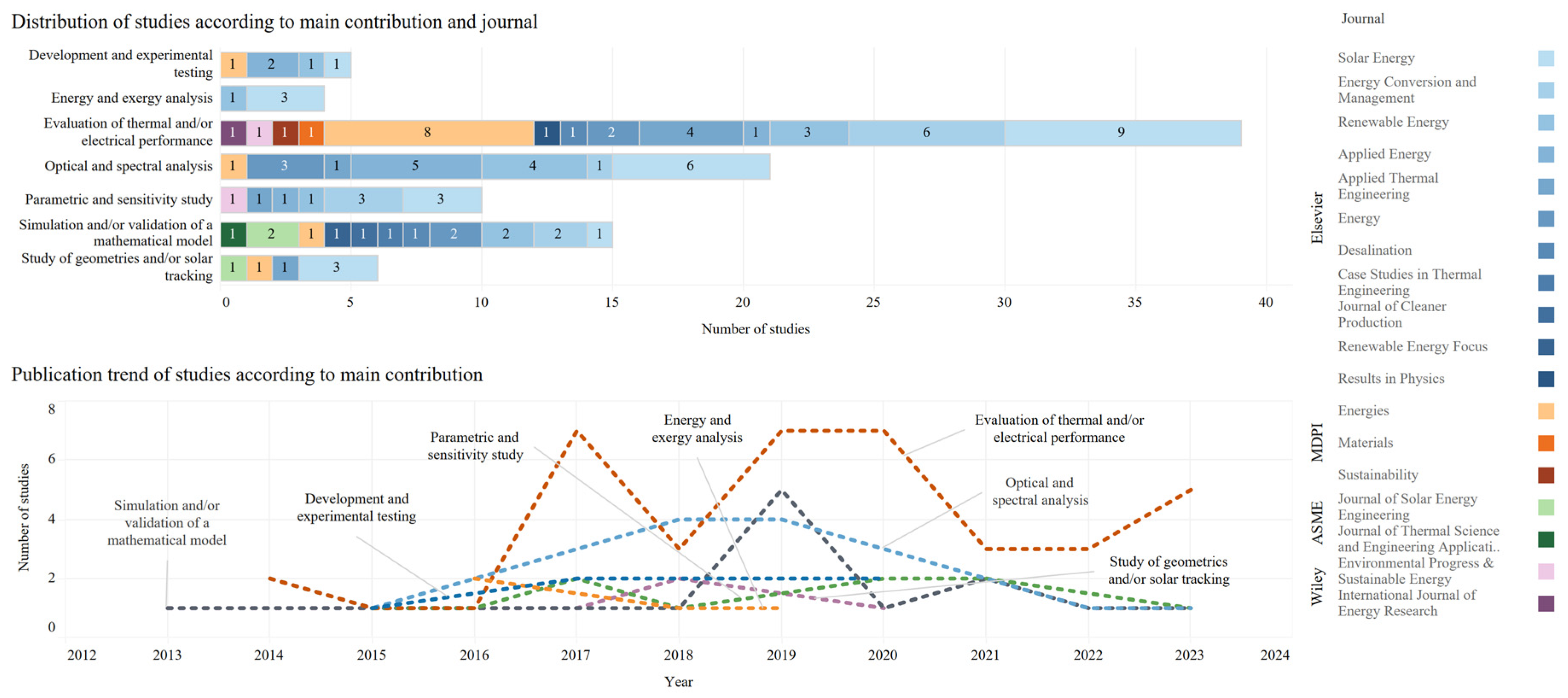
2.2. Review of Previous Studies
- PTC—PVT: Parabolic Trough Collector—PhotoVoltaic Thermal;
- CPC—PVT: Compound Parabolic Collector—PhotoVoltaic Thermal;
- CPV/T: Concentrated PhotoVoltaic Thermal;
- LCPV/T: Low Concentrating PhotoVoltaic Thermal;
- HCPV/T: High Concentrating PhotoVoltaic Thermal;
- PTES: Photovoltaic Thermal Electrical System;
- CHAPS: Combined Heat And Power Solar.
- Simulation and/or validation of a mathematical model;
- Evaluation of thermal and/or electrical performance;
- Optical and spectral analysis;
- Energy and exergy analysis;
- Parametric and sensitivity study;
- Development and experimental testing;
- Study of geometries and/or solar tracking.
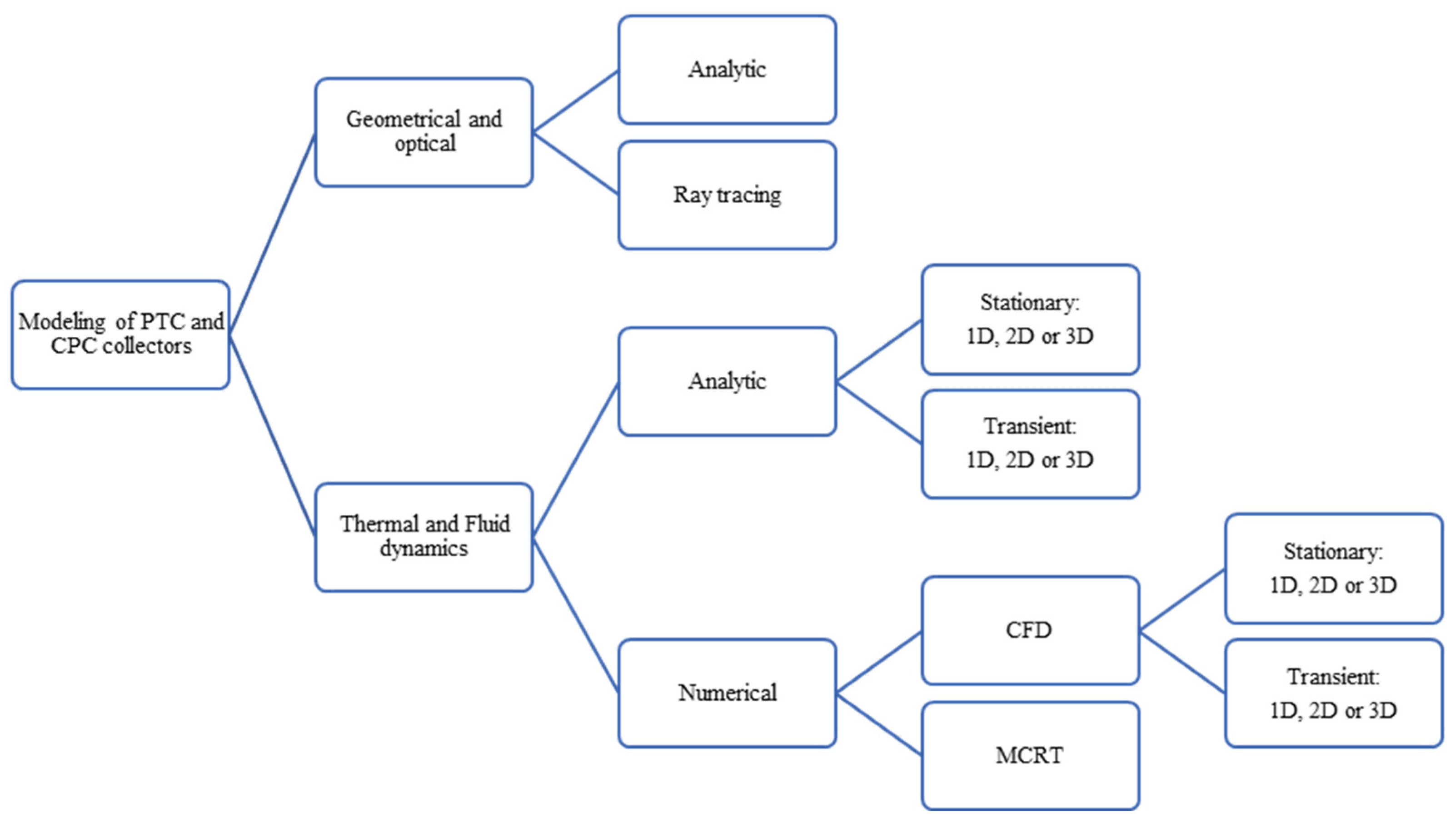
3. Fundamentals of PTCs and CPCs
3.1. Geometry for PTCs and CPCs
3.2. Optical Analysis for PTCs and CPCs
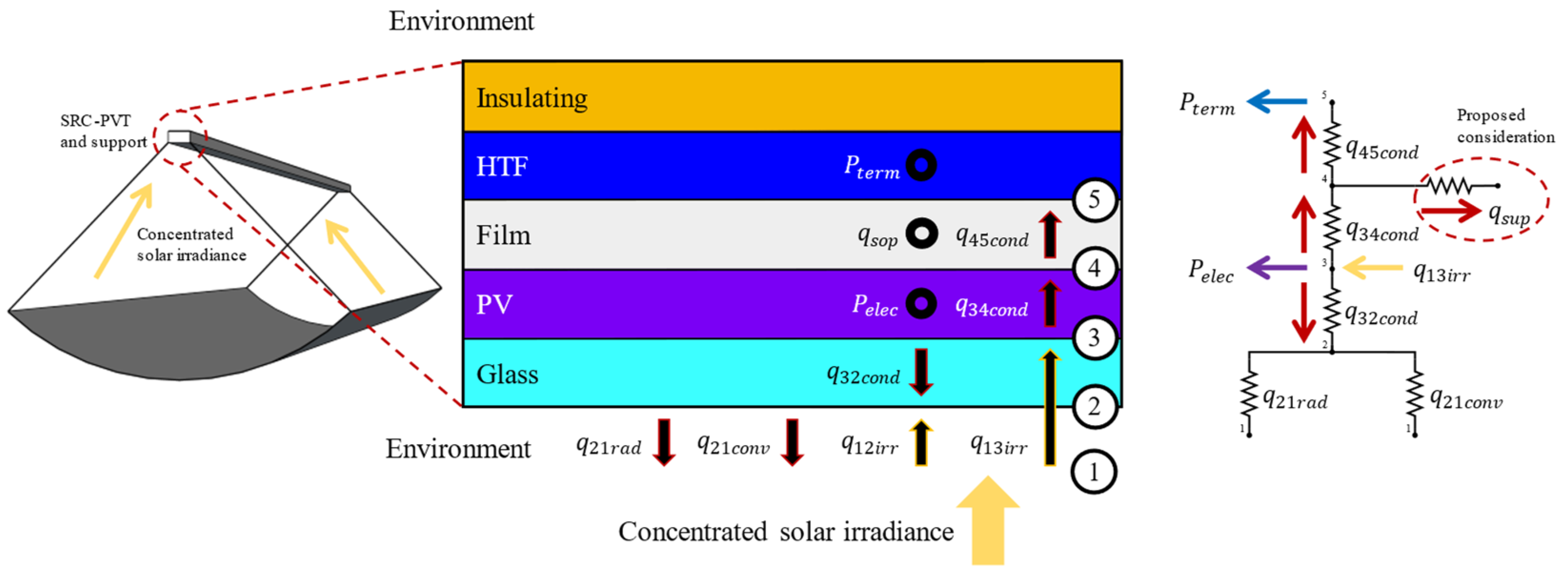
3.3. Thermal Resistance Analysis of the SRC-PVT
- Effective concentrated solar irradiance () is incident on the side of the glass exposed to the environment, while a heat flux emitted by the PV () is incident on the opposite side;
- A fraction of the incident irradiance on the crystal is converted into heat (), which is added to the heat flux from the PV (). Both of these are then emitted to the environment as radiation () and convection ();
- The irradiation passing through the crystal is incident on the PV () and is converted into electrical energy () due to the photoelectric effect;
- A fraction of the irradiance incident on the PV () is emitted as two heat fluxes, the first in the crystal direction () and the second through the film to the HTF ();
- The heat arriving at the film is again divided into two fluxes, the first in the HTF direction () and the second corresponding to the conduction heat losses in the supports ();
- The heat flow conducted through the PV and the film to the HTF () is extracted as thermal energy ().
- The energy balance is one-dimensional and steady-state. Uniformity is assumed for all temperatures, heat fluxes and thermodynamic properties. Furthermore, the insulation on the opposite side of the glass is assumed to prevent any heat loss;
- Effective concentrated solar irradiation refers to the amount of solar energy that remains following the consideration of optical and geometrical losses of the concentrator. The energy balance excludes terms that represent concentrator losses;
- The energy flux resulting from effective concentrated solar irradiation corresponds to the amount of light absorbed by the glass and PV (absorbance). This phenomenon is volumetric in nature, but for ease of analysis it is treated as a surface phenomenon under the assumption that the resulting error is relatively minor [119].
3.4. Description of the SRC-PVT Energy Balance Equations
3.4.1. Electrical Model of the PV
3.4.2. Thermal Model of the HTF
3.4.3. Model of the Conduction Heat Losses in the Supports
3.5. Modeling and Simulation Strategies
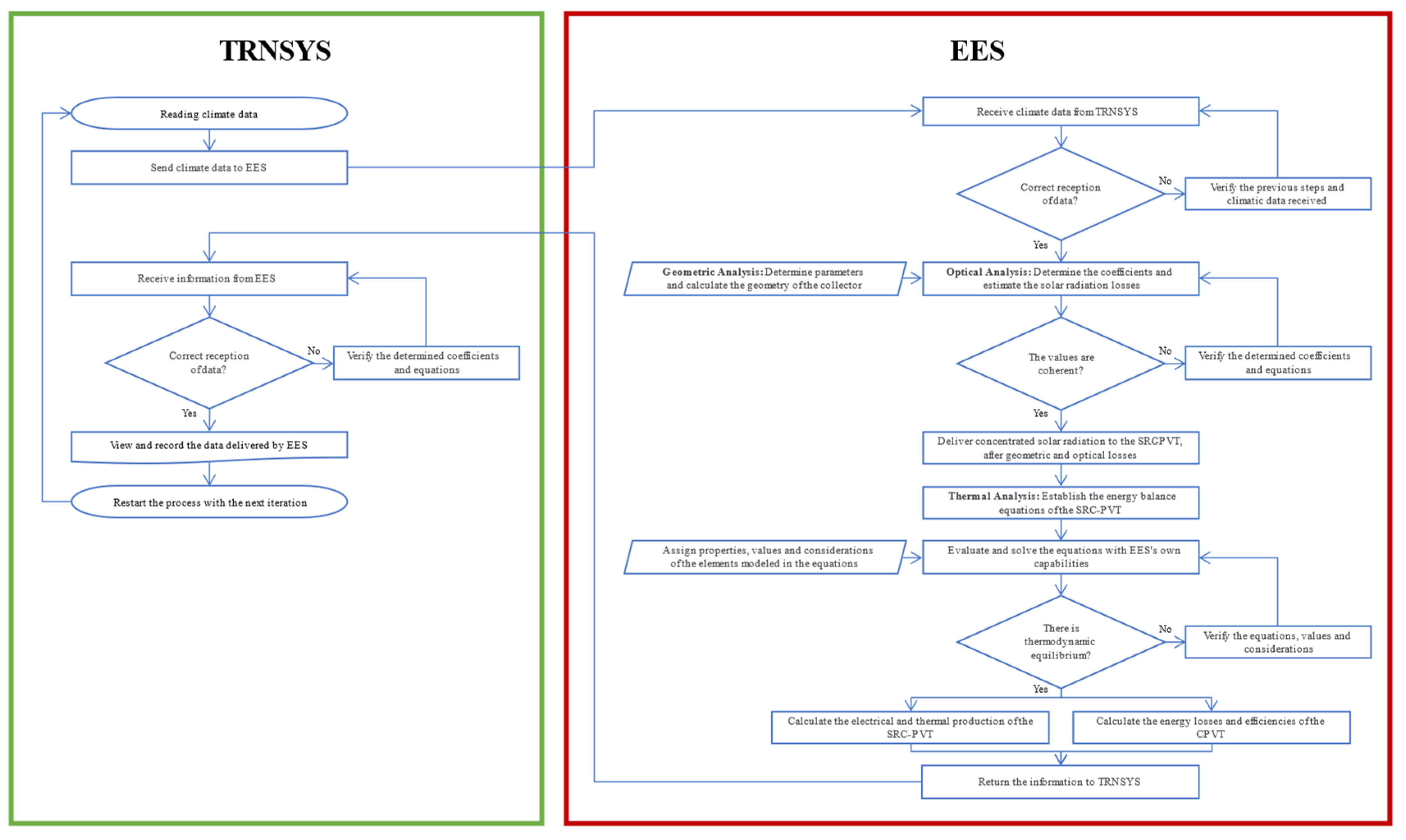
3.5.1. Simulation Software
3.5.2. Structure for Simulation Development
- TRNSYS is a software with a stage called “kernel” that reads and processes input files (e.g., climate files), solves iteratively, determines convergences and plots variables in conjunction with another stage, which is a library containing a set of mathematical models [124]. It allows the development of renewable energy systems through quasi-dynamic simulations (stationary models iterated several times). However, it does not have a model representing a PTC or CPC coupled to SRC-PVT.
- EES is software focused on solving systems of equations. It includes a database of highly accurate thermodynamic and transport properties for several substances [125]. This attribute facilitates the researcher in considering relevant aspects in their mathematical models. Hence, it is convenient to develop energy balance equations that represent the SRC-PVT in ESS and connect it to TRNSYS.
4. Review Studies Focusing on SRC-PVT Models
4.1. Geometry of SRC-PVTs
4.2. Thermal and Electrical Efficiency Equations
4.3. Parameters and Values for the Validation of Mathematical Models
5. Discussion and Conclusions
5.1. Geometry and Type of Collector
5.2. Solutions
5.3. Modeling
5.4. Strategies for Use
- The impact on the overall response of the SRC-PVT model when considering thermal conduction losses due to the SRC-PVT supports with the collector;
- The impact on collector field dimensioning and MED systems when using SRC-PVTs;
- Strategies and configurations that enable the SRC-PVT to achieve higher temperatures without compromising the electrical efficiency of the PV. These include the use of selective filters, nanofluids and dissipative fins.
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| CSP | Concentrated Solar Thermal Power |
| CPVT | Concentrated Photovoltaic Thermal |
| LCOE | Levelized Cost Of Energy |
| MED | Multi-Effect Distillation |
| PV | PhotoVoltaic cell |
| PVT | PhotoVoltaic Thermal |
| SRC | Solar Receiver Collector |
| HCE | Heat Collector Element |
| HTF | Heat Fluid Transfer |
| PTC | Parabolic Trough Collector |
| CPC | Compound Parabolic Collector |
| TEG | ThermoElectric Generation |
References
- Murdock, H.E.; Gibb, D.; Andre, T.; Sawin, J.L.; Brown, A.; Ranalder, L.; Andre, T.; Brown, A.; Collier, U.; Dent, C.; et al. Renewables 2021—Global Status Report; REN21 Secretariat: Paris, France, 2021; ISBN 978-3-948393-03-8. [Google Scholar]
- Ajbar, W.; Parrales, A.; Huicochea, A.; Hernández, J.A. Different ways to improve parabolic trough solar collectors’ performance over the last four decades and their applications: A comprehensive review. Renew. Sustain. Energy Rev. 2022, 156, 111947. [Google Scholar] [CrossRef]
- Islam, M.T.; Huda, N.; Abdullah, A.B.; Saidur, R. A comprehensive review of state-of-the-art concentrating solar power (CSP) technologies: Current status and research trends. Renew. Sustain. Energy Rev. 2018, 91, 987–1018. [Google Scholar] [CrossRef]
- Hachicha, A.A.; Yousef, B.A.A.; Said, Z.; Rodríguez, I. A review study on the modeling of high-temperature solar thermal collector systems. Renew. Sustain. Energy Rev. 2019, 112, 280–298. [Google Scholar] [CrossRef]
- Hasan, A.; Sarwar, J.; Shah, A.H. Concentrated photovoltaic: A review of thermal aspects, challenges and opportunities. Renew. Sustain. Energy Rev. 2018, 94, 835–852. [Google Scholar] [CrossRef]
- Tagle-Salazar, P.D. Parabolic trough Solar Collectors: A General Overview of Technology, Industrial Applications, Energy Market, Modeling, and Standards. Available online: https://www.degruyter.com/document/doi/10.1515/gps-2020-0059/html (accessed on 19 September 2023).
- Jaaz, A.H.; Hasan, H.A.; Sopian, K.; Ruslan, M.H.B.H.; Zaidi, S.H. Design and development of compound parabolic concentrating for photovoltaic solar collector: Review. Renew. Sustain. Energy Rev. 2017, 76, 1108–1121. [Google Scholar] [CrossRef]
- Herez, A.; El Hage, H.; Lemenand, T.; Ramadan, M.; Khaled, M. Review on photovoltaic/thermal hybrid solar collectors: Classifications, applications and new systems. Sol. Energy 2020, 207, 1321–1347. [Google Scholar] [CrossRef]
- Bayareh, M.; Usefian, A. Simulation of parabolic trough solar collectors using various discretization approaches: A review. Eng. Anal. Bound. Elem. 2023, 153, 126–137. [Google Scholar] [CrossRef]
- Singh, R.K.; Chandra, P. Parabolic trough solar collector: A review on geometrical interpretation, mathematical model, and thermal performance augmentation. Eng. Res. Express 2023, 5, 012003. [Google Scholar] [CrossRef]
- Masood, F.; Nor, N.B.M.; Elamvazuthi, I.; Saidur, R.; Alam, M.A.; Akhter, J.; Yusuf, M.; Ali, S.M.; Sattar, M.; Baba, M.; et al. The compound parabolic concentrators for solar photovoltaic applications: Opportunities and challenges. Energy Rep. 2022, 8, 13558–13584. [Google Scholar] [CrossRef]
- Malan, A.; Kumar, K.R. A comprehensive review on optical analysis of parabolic trough solar collector. Sustain. Energy Technol. Assess. 2021, 46, 101305. [Google Scholar] [CrossRef]
- Yılmaz, İ.H.; Mwesigye, A. Modeling, simulation and performance analysis of parabolic trough solar collectors: A comprehensive review. Appl. Energy 2018, 225, 135–174. [Google Scholar] [CrossRef]
- Azizi, M.; Tabatabaeekoloor, R.; Motevali, A.; Seyedi, S.R.M. Evaluation of mono and hybrid nano-fluids on energy and exergy parameters of a photovoltaic-thermal system equipped with an eccentric parabolic trough concentrator. Appl. Therm. Eng. 2023, 223, 119979. [Google Scholar] [CrossRef]
- Hou, Y.; Yu, X.; Yang, B.; Liu, S.; Qi, Y. Optical performance investigation on flat receiver for parabolic trough solar collector based on the MCRT method. Renew. Energy 2023, 202, 525–536. [Google Scholar] [CrossRef]
- Renno, C.; Perone, A.; D’Agostino, D.; Minichiello, F. Performance Evaluation of a Linear CPV/T System in Different Working Conditions. Energies 2023, 16, 2115. [Google Scholar] [CrossRef]
- Santana, J.P.; Rivera-Solorio, C.I.; Chew, J.W.; Tan, Y.Z.; Gijón-Rivera, M.; Acosta-Pazmiño, I. Performance Assessment of Coupled Concentrated Photovoltaic-Thermal and Vacuum Membrane Distillation (CPVT-VMD) System for Water Desalination. Energies 2023, 16, 1541. [Google Scholar] [CrossRef]
- Zheng, N.; Zhang, H.; Duan, L.; Wang, Q.; Bischi, A.; Desideri, U. Techno-economic analysis of a novel solar-driven PEMEC-SOFC-based multi-generation system coupled parabolic trough photovoltaic thermal collector and thermal energy storage. Appl. Energy 2023, 331, 120400. [Google Scholar] [CrossRef]
- Acosta-Pazmiño, I.P.; Rivera-Solorio, C.I.; Gijón-Rivera, M. Hybridization of a parabolic trough-based thermal plant for industrial heat and power generation. Renew. Energy 2022, 191, 961–973. [Google Scholar] [CrossRef]
- Dogahe, S.A.; Javaran, E.J.; Abdolzadeh, M. Energy and economic analysis of photovoltaic, concentrating photovoltaic, and combined concentrating photovoltaic/thermal-organic Rankine cycle power plants in Iran. Environ. Prog. Sustain. Energy 2022, 41, e13763. [Google Scholar] [CrossRef]
- Gorouh, H.A.; Salmanzadeh, M.; Nasseriyan, P.; Hayati, A.; Cabral, D.; Gomes, J.; Karlsson, B. Thermal modelling and experimental evaluation of a novel concentrating photovoltaic thermal collector (CPVT) with parabolic concentrator. Renew. Energy 2022, 181, 535–553. [Google Scholar] [CrossRef]
- Kurşun, B.; Ökten, K. Comprehensive energy, exergy, and economic analysis of the scenario of supplementing pumped thermal energy storage (PTES) with a concentrated photovoltaic thermal system. Energy Convers. Manag. 2022, 260, 115592. [Google Scholar] [CrossRef]
- Zhu, T.; Li, Q.; Yu, A. Analysis of the solar spectrum allocation in a spectral-splitting photovoltaic-thermochemical hybrid system. Sol. Energy 2022, 232, 63–72. [Google Scholar] [CrossRef]
- Cabral, D.; Gomes, J.; Hayati, A.; Karlsson, B. Experimental investigation of a CPVT collector coupled with a wedge PVT receiver. Sol. Energy 2021, 215, 335–345. [Google Scholar] [CrossRef]
- Herez, A.; El Hage, H.; Lemenand, T.; Ramadan, M.; Khaled, M. Parabolic trough Photovoltaic/Thermal hybrid system: Thermal modeling, case studies, and economic and environmental analyzes. Renew. Energy Focus 2021, 38, 9–21. [Google Scholar] [CrossRef]
- Herez, A.; El Hage, H.; Lemenand, T.; Ramadan, M.; Khaled, M. Parabolic trough photovoltaic/thermal hybrid system: Thermal modeling and parametric analysis. Renew. Energy 2021, 165, 224–236. [Google Scholar] [CrossRef]
- Hu, Y.; Zhang, L.; Zhang, H.; Lv, H.; Xu, C. Thermodynamic analysis of a spectral-splitting hybrid PV-thermal system with LiBr/H2O absorption heat transformer. Energy Convers. Manag. 2021, 249, 114868. [Google Scholar] [CrossRef]
- Renno, C.; Perone, A.; D’Agostino, D.; Minichiello, F. Experimental and Economic Analysis of a Concentrating Photovoltaic System Applied to Users of Increasing Size. Energies 2021, 14, 4968. [Google Scholar] [CrossRef]
- Zhang, J.J.; Qu, Z.G.; Wang, Q.; Zhang, J.F.; He, Y.L. Multiscale investigation of the plasmonic solar cell in the spectral splitting concentrating photovoltaic-thermal system. Energy Convers. Manag. 2021, 250, 114846. [Google Scholar] [CrossRef]
- Zima, W.; Cisek, P.; Cebula, A. Mathematical model of an innovative double U-tube sun-tracked PTC and its experimental verification. Energy 2021, 235, 121293. [Google Scholar] [CrossRef]
- Acosta-Pazmiño, I.; Rivera-Solorio, C.; Gijón-Rivera, M. Energetic and Economic Analyses of an LCPV/T Solar Hybrid Plant for a Sports Center Building in Mexico. Energies 2020, 13, 5681. [Google Scholar] [CrossRef]
- Alayi, R.; Kasaeian, A.; Atabi, F. Optical modeling and optimization of parabolic trough concentration photovoltaic/thermal system. Environ. Prog. Sustain. Energy 2020, 39, e13303. [Google Scholar] [CrossRef]
- Felsberger, R.; Buchroithner, A.; Gerl, B.; Wegleiter, H. Conversion and Testing of a Solar Thermal Parabolic trough Collector for CPV-T Application. Energies 2020, 13, 6142. [Google Scholar] [CrossRef]
- Gakkhar, N.; Soni, M.K.; Jakhar, S. Experimental and theoretical analysis of hybrid concentrated photovoltaic/thermal system using parabolic trough collector. Appl. Therm. Eng. 2020, 171, 115069. [Google Scholar] [CrossRef]
- Liang, H.; Wang, F.; Zhang, D.; Cheng, Z.; Zhang, C.; Lin, B.; Xu, H. Experimental investigation of cost-effective ZnO nanofluid based spectral splitting CPV/T system. Energy 2020, 194, 116913. [Google Scholar] [CrossRef]
- Khouya, A. Performance assessment of a heat pump and a concentrated photovoltaic thermal system during the wood drying process. Appl. Therm. Eng. 2020, 180, 115923. [Google Scholar] [CrossRef]
- Otanicar, T.P.; Wingert, R.; Orosz, M.; McPheeters, C. Concentrating photovoltaic retrofit for existing parabolic trough solar collectors: Design, experiments, and levelized cost of electricity. Appl. Energy 2020, 265, 114751. [Google Scholar] [CrossRef]
- Renno, C.; Petito, F.; D’Agostino, D.; Minichiello, F. Modeling of a CPV/T-ORC Combined System Adopted for an Industrial User. Energies 2020, 13, 3476. [Google Scholar] [CrossRef]
- Renno, C. Theoretical and Experimental Evaluation of the Working Fluid Temperature Levels in a CPV/T System. Energies 2020, 13, 3077. [Google Scholar] [CrossRef]
- Riahi, A.; Ali, A.B.H.; Fadhel, A.; Guizani, A.; Balghouthi, M. Performance investigation of a concentrating photovoltaic thermal hybrid solar system combined with thermoelectric generators. Energy Convers. Manag. 2020, 205, 112377. [Google Scholar] [CrossRef]
- Wang, G.; Yao, Y.; Lin, J.; Chen, Z.; Hu, P. Design and thermodynamic analysis of a novel solar CPV and thermal combined system utilizing spectral beam splitter. Renew. Energy 2020, 155, 1091–1102. [Google Scholar] [CrossRef]
- Wingert, R.; O’Hern, H.; Orosz, M.; Harikumar, P.; Roberts, K.; Otanicar, T. Spectral beam splitting retrofit for hybrid PV/T using existing parabolic trough power plants for enhanced power output. Sol. Energy 2020, 202, 1–9. [Google Scholar] [CrossRef]
- Adam, S.A.; Ju, X.; Zhang, Z.; El-Samie, M.M.A.; Xu, C. Theoretical investigation of different CPVT configurations based on liquid absorption spectral beam filter. Energy 2019, 189, 116259. [Google Scholar] [CrossRef]
- Alves, P.; Fernandes, J.F.P.; Torres, J.P.N.; Branco, P.J.C.; Fernandes, C.; Gomes, J. From Sweden to Portugal: The effect of very distinct climate zones on energy efficiency of a concentrating photovoltaic/thermal system (CPV/T). Sol. Energy 2019, 188, 96–110. [Google Scholar] [CrossRef]
- Karathanassis, I.K.; Papanicolaou, E.; Belessiotis, V.; Bergeles, G.C. Dynamic simulation and exergetic optimization of a Concentrating Photovoltaic/ Thermal (CPVT) system. Renew. Energy 2019, 135, 1035–1047. [Google Scholar] [CrossRef]
- Liew, N.J.Y.; Lee, H.-J. Numerical analysis of hybrid photovoltaic-thermal systems utilizing different spectral bandpass filters. Renew. Energy 2019, 144, 15–29. [Google Scholar] [CrossRef]
- Maatallah, T.; Youssef, W.B. Simulation and performance analysis of concentrating photovoltaic/thermal collector (CPV/T) with three-sided thermal insulation based on coupled optothermal model. Sol. Energy 2019, 181, 308–324. [Google Scholar] [CrossRef]
- Renno, C.; D’Agostino, D.; Minichiello, F.; Petito, F.; Balen, I. Performance analysis of a CPV/T-DC integrated system adopted for the energy requirements of a supermarket. Appl. Therm. Eng. 2019, 149, 231–248. [Google Scholar] [CrossRef]
- Renno, C.; Petito, F. Modelling of a linear focus concentrating photovoltaic and thermal system for different load scenarios of a residential user. Energy Convers. Manag. 2019, 188, 214–229. [Google Scholar] [CrossRef]
- Valizadeh, M.; Sarhaddi, F.; Adeli, M.M. Exergy performance assessment of a linear parabolic trough photovoltaic thermal collector. Renew. Energy 2019, 138, 1028–1041. [Google Scholar] [CrossRef]
- Wang, G.; Yao, Y.; Chen, Z.; Hu, P. Thermodynamic and optical analyses of a hybrid solar CPV/T system with high solar concentrating uniformity based on spectral beam splitting technology. Energy 2019, 166, 256–266. [Google Scholar] [CrossRef]
- Widyolar, B.; Jiang, L.; Ferry, J.; Winston, R.; Kirk, A.; Osowski, M.; Cygan, D.; Abbasi, H. Theoretical and experimental performance of a two-stage (50X) hybrid spectrum splitting solar collector tested to 600 °C. Appl. Energy 2019, 239, 514–525. [Google Scholar] [CrossRef]
- Youssef, W.B.; Maatallah, T.; Menezo, C.; Nasrallah, S.B. Modeling and optimization of a solar system based on concentrating photovoltaic/thermal collector. Sol. Energy 2018, 170, 301–313. [Google Scholar] [CrossRef]
- Youssef, W.B.; Maatallah, T.; Menezo, C.; Nasrallah, S.B. Assessment viability of a concentrating photovoltaic/thermal-energy cogeneration system (CPV/T) with storage for a textile industry application. Sol. Energy 2018, 159, 841–851. [Google Scholar] [CrossRef]
- Otanicar, T.; Dale, J.; Orosz, M.; Brekke, N.; DeJarnette, D.; Tunkara, E.; Roberts, K.; Harikumar, P. Experimental evaluation of a prototype hybrid CPV/T system utilizing a nanoparticle fluid absorber at elevated temperatures. Appl. Energy 2018, 228, 1531–1539. [Google Scholar] [CrossRef]
- Renno, C. Experimental and Theoretical Analysis of a Linear Focus CPV/T System for Cogeneration Purposes. Energies 2018, 11, 2960. [Google Scholar] [CrossRef]
- Widyolar, B.; Jiang, L.; Abdelhamid, M.; Winston, R. Design and modeling of a spectrum-splitting hybrid CSP-CPV parabolic trough using two-stage high concentration optics and dual junction InGaP/GaAs solar cells. Sol. Energy 2018, 165, 75–84. [Google Scholar] [CrossRef]
- Widyolar, B.; Jiang, L.; Winston, R. Spectral beam splitting in hybrid PV/T parabolic trough systems for power generation. Appl. Energy 2018, 209, 236–250. [Google Scholar] [CrossRef]
- An, W.; Li, J.; Ni, J.; Taylor, R.A.; Zhu, T. Analysis of a temperature dependent optical window for nanofluid-based spectral splitting in PV/T power generation applications. Energy Convers. Manag. 2017, 151, 23–31. [Google Scholar] [CrossRef]
- Karathanassis, I.K.; Papanicolaou, E.; Belessiotis, V.; Bergeles, G.C. Design and experimental evaluation of a parabolic-trough concentrating photovoltaic/thermal (CPVT) system with high-efficiency cooling. Renew. Energy 2017, 101, 467–483. [Google Scholar] [CrossRef]
- Mohsenzadeh, M.; Shafii, M.B.; Jafari Mosleh, H. A novel concentrating photovoltaic/thermal solar system combined with thermoelectric module in an integrated design. Renew. Energy 2017, 113, 822–834. [Google Scholar] [CrossRef]
- Srivastava, S.; Reddy, K.S. Simulation studies of thermal and electrical performance of solar linear parabolic trough concentrating photovoltaic system. Sol. Energy 2017, 149, 195–213. [Google Scholar] [CrossRef]
- Wang, G.; Chen, Z.; Hu, P. Design and experimental investigation of a Multi-segment plate concentrated photovoltaic solar energy system. Appl. Therm. Eng. 2017, 116, 147–152. [Google Scholar] [CrossRef]
- Widyolar, B.K.; Abdelhamid, M.; Jiang, L.; Winston, R.; Yablonovitch, E.; Scranton, G.; Cygan, D.; Abbasi, H.; Kozlov, A. Design, simulation and experimental characterization of a novel parabolic trough hybrid solar photovoltaic/thermal (PV/T) collector. Renew. Energy 2017, 101, 1379–1389. [Google Scholar] [CrossRef]
- Yazdanifard, F.; Ebrahimnia-Bajestan, E.; Ameri, M. Performance of a parabolic trough concentrating photovoltaic/thermal system: Effects of flow regime, design parameters, and using nanofluids. Energy Convers. Manag. 2017, 148, 1265–1277. [Google Scholar] [CrossRef]
- Brekke, N.; Otanicar, T.; DeJarnette, D.; Hari, P. A Parametric Investigation of a Concentrating Photovoltaic/Thermal System with Spectral Filtering Utilizing a Two-Dimensional Heat Transfer Model. J. Sol. Energy Eng. 2016, 138, 021007. [Google Scholar] [CrossRef]
- Stanley, C.; Mojiri, A.; Rahat, M.; Blakers, A.; Rosengarten, G. Performance testing of a spectral beam splitting hybrid PVT solar receiver for linear concentrators. Appl. Energy 2016, 168, 303–313. [Google Scholar] [CrossRef]
- Al-Nimr, M.A.; Dahdolan, M.-E. Modeling of a novel concentrated PV/T distillation system enhanced with a porous evaporator and an internal condenser. Sol. Energy 2015, 120, 593–602. [Google Scholar] [CrossRef]
- Barrau, J.; Perona, A.; Dollet, A.; Rosell, J. Outdoor test of a hybrid jet impingement/micro-channel cooling device for densely packed concentrated photovoltaic cells. Sol. Energy 2014, 107, 113–121. [Google Scholar] [CrossRef]
- Xu, Z.; Kleinstreuer, C. Computational Analysis of Nanofluid Cooling of High Concentration Photovoltaic Cells. J. Therm. Sci. Eng. Appl. 2014, 6, 031009. [Google Scholar] [CrossRef]
- Calise, F.; Palombo, A.; Vanoli, L. A finite-volume model of a parabolic trough photovoltaic/thermal collector: Energetic and exergetic analyses. Energy 2012, 46, 283–294. [Google Scholar] [CrossRef]
- Karathanassis, I.K.; Papanicolaou, E.; Belessiotis, V.; Bergeles, G.C. Multi-objective design optimization of a micro heat sink for Concentrating Photovoltaic/Thermal (CPVT) systems using a genetic algorithm. Appl. Therm. Eng. 2013, 59, 733–744. [Google Scholar] [CrossRef]
- Cabral, D.; Hayati, A.; Gomes, J.; Gorouh, H.A.; Nasseriyan, P.; Salmanzadeh, M. Experimental Electrical Assessment Evaluation of a Vertical n-PERT Half-Size Bifacial Solar Cell String Receiver on a Parabolic trough Solar Collector. Energies 2023, 16, 2007. [Google Scholar] [CrossRef]
- Korres, D.N.; Papingiotis, T.; Koronaki, I.; Tzivanidis, C. Thermal and Optical Analyses of a Hybrid Solar Photovoltaic/Thermal (PV/T) Collector with Asymmetric Reflector: Numerical Modeling and Validation with Experimental Results. Sustainability 2023, 15, 9932. [Google Scholar] [CrossRef]
- Saberi, Z.; Jarimi, H.; Jumali, M.H.H.; Suhendri, S.; Riffat, S.; Fudholi, A.; Razali, H.H.; Sopian, K. Performance assessment of double pass photovoltaic/thermal solar air collector using bifacial PV with CPC and mirror reflector under Malaysian climate. Case Stud. Therm. Eng. 2023, 44, 102811. [Google Scholar] [CrossRef]
- Li, G.; Lu, Y.; Xuan, Q.; Akhlaghi, Y.G.; Pei, G.; Ji, J.; Zhao, X. Small scale optimization in crystalline silicon solar cell on efficiency enhancement of low-concentrating photovoltaic cell. Sol. Energy 2020, 202, 316–325. [Google Scholar] [CrossRef]
- Li, G.; Lu, Y.; Xuan, Q.; Pei, G.; Ji, J.; Zhao, X. Performance analysis on a crystalline silicon photovoltaic cell under non-uniform illumination distribution with a high electrical efficiency. Sol. Energy 2020, 203, 275–283. [Google Scholar] [CrossRef]
- Li, G.; Lu, Y.; Shittu, S.; Zhao, X. Scale effect on electrical characteristics of CPC-PV. Energy 2020, 192, 116726. [Google Scholar] [CrossRef]
- Nasseriyan, P.; Afzali Gorouh, H.; Gomes, J.; Cabral, D.; Salmanzadeh, M.; Lehmann, T.; Hayati, A. Numerical and Experimental Study of an Asymmetric CPC-PVT Solar Collector. Energies 2020, 13, 1669. [Google Scholar] [CrossRef]
- Cabral, D.; Gomes, J.; Karlsson, B. Performance evaluation of non-uniform illumination on a transverse bifacial PVT receiver in combination with a CPC geometry. Sol. Energy 2019, 194, 696–708. [Google Scholar] [CrossRef]
- Chen, H.; Guo, X.; Zhang, H.; Liu, Y.; Liu, H.; Bu, Y. Experimental study on a flash tank integrated with low concentrating PV/T (FT-LCPVT) hybrid system for desalination. Appl. Therm. Eng. 2019, 159, 113874. [Google Scholar] [CrossRef]
- Chen, H.; Huang, J.; Zhang, H.; Liang, K.; Liu, H.; Liang, S. Experimental investigation of a novel low concentrating photovoltaic/thermal–thermoelectric generator hybrid system. Energy 2019, 166, 83–95. [Google Scholar] [CrossRef]
- Chen, H.; Liu, H.; Zhang, H.; Liang, K.; Guo, X.; Yang, B. Numerical simulation and experimental analysis of an LCPV/T system under real operating conditions. J. Clean. Prod. 2019, 209, 903–915. [Google Scholar] [CrossRef]
- Wang, Z.; Wei, J.; Zhang, G.; Xie, H.; Khalid, M. Design and performance study on a large-scale hybrid CPV/T system based on unsteady-state thermal model. Sol. Energy 2019, 177, 427–439. [Google Scholar] [CrossRef]
- Guo, X.; Zhang, H.; Chen, H.; Liang, K.; Huang, J.; Liu, H. Experimental and theoretical investigation on a hybrid LCPV/T solar still system. Desalination 2019, 468, 114063. [Google Scholar] [CrossRef]
- Yang, L.; Heng, Z.; Haiping, C.; Han, Y.; Fei, Y. Simulating and experimental research on a low-concentrating PV/T triple-generation system. Energy Convers. Manag. 2019, 199, 111942. [Google Scholar] [CrossRef]
- Cabral, D.; Karlsson, B.O. Electrical and thermal performance evaluation of symmetric truncated C-PVT trough solar collectors with vertical bifacial receivers. Sol. Energy 2018, 174, 683–690. [Google Scholar] [CrossRef]
- Gupta, V.S.; Singh, D.B.; Mishra, R.K.; Sharma, S.K.; Tiwari, G.N. Development of characteristic equations for PVT-CPC active solar distillation system. Desalination 2018, 445, 266–279. [Google Scholar] [CrossRef]
- Jaaz, A.H.; Sopian, K.; Gaaz, T.S. Study of the electrical and thermal performances of photovoltaic thermal collector-compound parabolic concentrated. Results Phys. 2018, 9, 500–510. [Google Scholar] [CrossRef]
- Tiwari, G.N.; Meraj, M.; Khan, M.E. Exergy analysis of N-photovoltaic thermal-compound parabolic concentrator (N-PVT-CPC) collector for constant collection temperature for vapor absorption refrigeration (VAR) system. Sol. Energy 2018, 173, 1032–1042. [Google Scholar] [CrossRef]
- Torres, J.P.N.; Fernandes, C.A.; Gomes, J.; Luc, B.; Carine, G.; Olsson, O.; Branco, P.C. Effect of Reflector Geometry in the Annual Received Radiation of Low Concentration Photovoltaic Systems. Energies 2018, 11, 1878. [Google Scholar] [CrossRef]
- Zhang, G.; Wei, J.; Xie, H.; Wang, Z.; Xi, Y.; Khalid, M. Performance investigation on a novel spectral splitting concentrating photovoltaic/thermal system based on direct absorption collection. Sol. Energy 2018, 163, 552–563. [Google Scholar] [CrossRef]
- Chen, H.; Zhang, H.; Bu, Y.; Liu, H.; Zhang, X. The performance analysis and evaluation of C-PV/T aided power generation system. Int. J. Energy Res. 2018, 42, 863–876. [Google Scholar] [CrossRef]
- Zhang, H.; Liu, H.; Chen, H.; Guo, X.; Liang, K.; Yao, P. Research on the Performance of Flat-Box Photovoltaic/Thermal Collector with Cooling Channels. J. Sol. Energy Eng. 2017, 140, 021002. [Google Scholar] [CrossRef]
- Jaaz, A.H.; Hasan, H.A.; Sopian, K.; Kadhum, A.A.H.; Gaaz, T.S.; Al-Amiery, A.A. Outdoor Performance Analysis of a Photovoltaic Thermal (PVT) Collector with Jet Impingement and Compound Parabolic Concentrator (CPC). Materials 2017, 10, 888. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.; Jia, Y.; Lin, Y.; Alva, G.; Fang, G. Numerical study of a novel miniature compound parabolic concentrating photovoltaic/thermal collector with microencapsulated phase change slurry. Energy Convers. Manag. 2017, 153, 106–114. [Google Scholar] [CrossRef]
- Proell, M.; Osgyan, P.; Karrer, H.; Brabec, C.J. Experimental efficiency of a low concentrating CPC PVT flat plate collector. Sol. Energy 2017, 147, 463–469. [Google Scholar] [CrossRef]
- Singh, D.B.; Tiwari, G.N. Performance analysis of basin type solar stills integrated with N identical photovoltaic thermal (PVT) compound parabolic concentrator (CPC) collectors: A comparative study. Sol. Energy 2017, 142, 144–158. [Google Scholar] [CrossRef]
- Tripathi, R.; Tiwari, G.N. Annual performance evaluation (energy and exergy) of fully covered concentrated photovoltaic thermal (PVT) water collector: An experimental validation. Sol. Energy 2017, 146, 180–190. [Google Scholar] [CrossRef]
- Tripathi, R.; Tiwari, G.N.; Dwivedi, V.K. Energy matrices evaluation and exergoeconomic analysis of series connected N partially covered (glass to glass PV module) concentrated-photovoltaic thermal collector: At constant flow rate mode. Energy Convers. Manag. 2017, 145, 353–370. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, H.; Han, Y.; Liu, H.; Li, M. Experimental and simulation studies on a novel compound parabolic concentrator. Renew. Energy 2017, 113, 784–794. [Google Scholar] [CrossRef]
- Atheaya, D.; Tiwari, A.; Tiwari, G.N. Exergy analysis of photovoltaic thermal (PVT) compound parabolic concentrator (CPC) for constant collection temperature mode. Sol. Energy 2016, 135, 222–231. [Google Scholar] [CrossRef]
- Proell, M.; Karrer, H.; Brabec, C.J.; Hauer, A. The influence of CPC reflectors on the electrical incidence angle modifier of c-Si cells in a PVT hybrid collector. Sol. Energy 2016, 126, 220–230. [Google Scholar] [CrossRef]
- Tripathi, R.; Tiwari, G.N.; Al-Helal, I.M. Thermal modelling of N partially covered photovoltaic thermal (PVT)—Compound parabolic concentrator (CPC) collectors connected in series. Sol. Energy 2016, 123, 174–184. [Google Scholar] [CrossRef]
- Tripathi, R.; Tiwari, G.N. Energetic and exergetic analysis of N partially covered photovoltaic thermal-compound parabolic concentrator (PVT-CPC) collectors connected in series. Sol. Energy 2016, 137, 441–451. [Google Scholar] [CrossRef]
- Vance, W.; Gustafson, M.; Huang, H.; Menart, J. Computational Study of a Fixed Orientation Photovoltaic Compound Parabolic Concentrator. J. Sol. Energy Eng. 2016, 139, 021002. [Google Scholar] [CrossRef]
- Yousef, M.S.; Rahman, A.K.A.; Ookawara, S. Performance investigation of low—Concentration photovoltaic systems under hot and arid conditions: Experimental and numerical results. Energy Convers. Manag. 2016, 128, 82–94. [Google Scholar] [CrossRef]
- Li, G.; Pei, G.; Ji, J.; Yang, M.; Su, Y.; Xu, N. Numerical and experimental study on a PV/T system with static miniature solar concentrator. Sol. Energy 2015, 120, 565–574. [Google Scholar] [CrossRef]
- Li, G.; Pei, G.; Ji, J.; Su, Y. Outdoor overall performance of a novel air-gap-lens-walled compound parabolic concentrator (ALCPC) incorporated with photovoltaic/thermal system. Appl. Energy 2015, 144, 214–223. [Google Scholar] [CrossRef]
- Zhou, H.; Su, Y.; Bottarelli, M.; Bortoloni, M.; Wu, S. Numerical Validation of a New Approach to Model Single Junction Low Concentration PV Cells under Non-Uniform Illumination. Energies 2015, 8, 4529–4548. [Google Scholar] [CrossRef]
- Bahaidarah, H.M.; Tanweer, B.; Gandhidasan, P.; Ibrahim, N.; Rehman, S. Experimental and numerical study on non-concentrating and symmetric unglazed compound parabolic photovoltaic concentration systems. Appl. Energy 2014, 136, 527–536. [Google Scholar] [CrossRef]
- Li, G.; Gang, P.; Su, Y.; Wang, Y.; Jie, J. Design and investigation of a novel lens-walled compound parabolic concentrator with air gap. Appl. Energy 2014, 125, 21–27. [Google Scholar] [CrossRef]
- Li, G.; Pei, G.; Yang, M.; Ji, J.; Su, Y. Optical evaluation of a novel static incorporated compound parabolic concentrator with photovoltaic/thermal system and preliminary experiment. Energy Convers. Manag. 2014, 85, 204–211. [Google Scholar] [CrossRef]
- Tiwari, G.N.; Meraj, M.; Khan, M.E.; Dwevedi, V.K. Effect of Series and Parallel Combination of Photovoltaic Thermal Collectors on the Performances of Integrated Active Solar Still. J. Therm. Sci. Eng. Appl. 2022, 14, 081006. [Google Scholar] [CrossRef]
- Tiwari, G.N.; Singh, R.K.; Sinha, A.S.K. Thermal and Electrical Analysis of N-PVT-FPC Hybrid Active Heating of Biogas Plant. J. Therm. Sci. Eng. Appl. 2023, 16, 011005. [Google Scholar] [CrossRef]
- Duffie, J.; Beckman, W. Solar Engineering of Thermal Processes; Wiley: New York, NY, USA, 2006. [Google Scholar]
- Kalogirou, S.A. Solar Energy Engineering: Processes and Systems. In EngineeringPro Collection; Elsevier Science: Burlington, MA, USA; San Diego, CA, USA; London, UK, 2009; Available online: https://books.google.com.mx/books?id=wYRqAAAAQBAJ&printsec=frontcover&hl=es&source=gbs_ge_summary_r&cad=0#v=onepage&q&f=false (accessed on 4 October 2023).
- Kalogirou, S.A. A detailed thermal model of a parabolic trough collector receiver. Energy 2012, 48, 298–306. [Google Scholar] [CrossRef]
- Forristall, R. Heat Transfer Analysis and Modeling of a Parabolic trough Solar Receiver Implemented in Engineering Equation Solver; NREL/TP-550-34169; National Renewable Energy Lab. (NREL): Golden, CO, USA, 2003. [CrossRef]
- Behar, O.; Khellaf, A.; Mohammedi, K. A novel parabolic trough solar collector model—Validation with experimental data and comparison to Engineering Equation Solver (EES). Energy Convers. Manag. 2015, 106, 268–281. [Google Scholar] [CrossRef]
- Incropera, F.P.; DeWitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer; Wiley: New York, NY, USA, 1996; Volume 6. [Google Scholar]
- Ji, X.; Li, M.; Lin, W.; Wang, W.; Wang, L.; Xi, J. Modeling and Characteristic Parameters Analysis of a trough Concentrating Photovoltaic/Thermal System with GaAs and Super Cell Arrays. Int. J. Photoenergy 2012, 2012, 782560. [Google Scholar] [CrossRef]
- Ju, X.; Wang, Z.; Flamant, G.; Li, P.; Zhao, W. Numerical analysis and optimization of a spectrum splitting concentration photovoltaic–thermoelectric hybrid system. Sol. Energy 2012, 86, 1941–1954. [Google Scholar] [CrossRef]
- Welcome|TRNSYS: Transient System Simulation Tool. Available online: http://www.trnsys.com/ (accessed on 4 October 2023).
- EES: Engineering Equation Solver|F-Chart Software: Engineering Software. Available online: https://fchartsoftware.com/ees/ (accessed on 4 October 2023).
- Bernardo, L.R.; Perers, B.; Håkansson, H.; Karlsson, B. Performance evaluation of low concentrating photovoltaic/thermal systems: A case study from Sweden. Sol. Energy 2011, 85, 1499–1510. [Google Scholar] [CrossRef]
- Othman, M.Y.H.; Yatim, B.; Sopian, K.; Bakar, M.N.A. Performance analysis of a double-pass photovoltaic/thermal (PV/T) solar collector with CPC and fins. Renew. Energy 2005, 30, 2005–2017. [Google Scholar] [CrossRef]
- Herez, A.; El Hage, H.; Lemenand, T.; Ramadan, M.; Khaled, M. Parabolic trough photovoltaic thermoelectric hybrid system: Thermal modeling, case studies and economic and environmental analyses. Sustain. Energy Technol. Assess. 2021, 47, 101368. [Google Scholar] [CrossRef]
- Herez, A.; Jaber, H.; El Hage, H.; Lemenand, T.; Chahine, K.; Ramadan, M.; Khaled, M. Parabolic trough photovoltaic thermoelectric hybrid system: Simulation model, parametric analysis, and practical recommendations. Int. J. Thermofluids 2023, 17, 100309. [Google Scholar] [CrossRef]
- Calise, F.; Vanoli, L. Parabolic trough Photovoltaic/Thermal Collectors: Design and Simulation Model. Energies 2012, 5, 4186–4208. [Google Scholar] [CrossRef]
- Renewable Power Generation Costs in 2022. Available online: https://www.irena.org/Publications/2023/Aug/Renewable-Power-Generation-Costs-in-2022 (accessed on 11 March 2024).
- Singh, P.; Ravindra, N.M. Temperature dependence of solar cell performance—An analysis. Sol. Energy Mater. Sol. Cells 2012, 101, 36–45. [Google Scholar] [CrossRef]
- Ghadamyari, M.; Nejad, A.A.; Mehregan, M.; Mohammadkhani, M. Thermodynamic and environmental analysis on a CCHP system based on MCFC-GT integrated with MED-TVC desalination system. Energy Rep. 2024, 11, 1925–1944. [Google Scholar] [CrossRef]



| Researcher—Year | Focus | Highlights |
|---|---|---|
| Bayareh—2023 [9] | Modeling and simulation of conventional PTC collectors. |
|
| Singh—2023 [10] | Modeling and simulation of conventional PTC collectors. |
|
| Masood—2022 [10] | Characteristics, analysis and improvement strategies for conventional CPC collectors and PVT systems. |
|
| Malan—2021 [11] | Mathematical analysis and optical modeling of conventional PTC collectors. |
|
| Herez—2020 [8] | Overview of solar collectors coupled to SRC-PVTs. |
|
| Yilmaz—2018 [12] | Modeling and simulation of conventional PTC collectors. |
|
| Year | Researcher | Journal | Study | Main Focus of Work | Modeling Considerations |
|---|---|---|---|---|---|
| 2023 [14] | Azizi | Applied Thermal Engineering | Experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2023 [15] | Hou | Renewable Energy | Theoretical | Optical and spectral analysis | N/A |
| 2023 [16] | Renno | Energies | Experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2023 [17] | Santana | Energies | Theoretical | Evaluation of thermal and/or electrical performance | N/A |
| 2023 [18] | Zheng | Applied Energy | Theoretical | Parametric and sensitivity study | Energy balance eq. |
| 2022 [19] | Acosta | Renewable Energy | Theoretical | Evaluation of thermal and/or electrical performance | Stationary |
| 2022 [20] | Dogahe | Environmental Progress and Sustainable Energy | Theoretical and experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2022 [21] | Gorouh | Renewable Energy | Theoretical and experimental | Simulation and/or validation of a mathematical model | Quasi-stationary, 1D |
| 2022 [22] | Kurşun | Energy Conversion and Management | Theoretical | Evaluation of thermal and/or electrical performance | Stationary |
| 2022 [23] | Zhu | Solar Energy | Theoretical and experimental | Optical and spectral analysis | N/A |
| 2021 [24] | Cabral | Solar Energy | Experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2021 [25] | Herez | Renewable Energy Focus | Theoretical | Simulation and/or validation of a mathematical model | Stationary, 1D |
| 2021 [26] | Herez | Renewable Energy | Theoretical | Parametric and sensitivity study | Stationary, 1D |
| 2021 [27] | Hu | Energy Conversion and Management | Theoretical | Parametric and sensitivity study | Stationary |
| 2021 [28] | Renno | Energies | Experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2021 [29] | Zhang | Energy Conversion and Management | Theoretical | Evaluation of thermal and/or electrical performance | Energy balance eq. |
| 2021 [30] | Zima | Energy | Theoretical and experimental | Simulation and/or validation of a mathematical model | Dynamic, 1D |
| 2020 [31] | Acosta | Energies | Theoretical | Evaluation of thermal and/or electrical performance | Stationary |
| 2020 [32] | Alayi | Environmental Progress and Sustainable Energy | Theoretical | Parametric and sensitivity study | N/A |
| 2020 [33] | Felsberger | Energies | Experimental | Development and experimental testing | N/A |
| 2020 [34] | Gakkhar | Applied Thermal Engineering | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Stationary, 1D |
| 2020 [35] | Huaxu | Energy | Experimental | Optical and spectral analysis | N/A |
| 2020 [36] | Khouya | Applied Thermal Engineering | Theoretical | Parametric and sensitivity study | Finite elements |
| 2020 [37] | Otanicar | Applied Energy | Theoretical and experimental | Development and experimental testing | Stationary |
| 2020 [38] | Renno | Energies | Theoretical | Simulation and/or validation of a mathematical model | Stationary, 1D |
| 2020 [39] | Renno | Energies | Theoretical and experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2020 [40] | Riahi | Energy Conversion and Management | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Dynamic |
| 2020 [41] | Wang | Renewable Energy | Theoretical | Optical and spectral analysis | N/A |
| 2020 [42] | Wingert | Solar Energy | Experimental | Optical and spectral analysis | N/A |
| 2019 [43] | Adam | Energy | Theoretical | Optical and spectral analysis | Stationary, 2D |
| 2019 [44] | Alves | Solar Energy | Theoretical | Evaluation of thermal and/or electrical performance | Stationary, 3D, Finite elements 3D |
| 2019 [45] | Karathanassis | Renewable Energy | Theoretical | Simulation and/or validation of a mathematical model | Dynamic |
| 2019 [46] | Liew | Renewable Energy | Theoretical | Evaluation of thermal and/or electrical performance | 1D, Energy balance eq. |
| 2019 [47] | Maatallah | Solar Energy | Theoretical | Simulation and/or validation of a mathematical model | Dynamic, 3D |
| 2019 [48] | Renno | Applied Thermal Engineering | Theoretical | Evaluation of thermal and/or electrical performance | Stationary |
| 2019 [49] | Renno | Energy Conversion and Management | Theoretical and experimental | Simulation and/or validation of a mathematical model | Stationary, 1D |
| 2019 [50] | Valizadeh | Renewable Energy | Theoretical | Energy and exergy analysis | Stationary, 1D |
| 2019 [51] | Wang | Energy | Theoretical | Optical and spectral analysis | Finite elements |
| 2019 [52] | Widyolar | Applied Energy | Theoretical and experimental | Optical and spectral analysis | N/A |
| 2018 [53] | Ben Youssef | Solar Energy | Theoretical and experimental | Parametric and sensitivity study | 2D, Finite elements |
| 2018 [54] | Ben Youssef | Solar Energy | Theoretical | Evaluation of thermal and/or electrical performance | Stationary |
| 2018 [55] | Otanicar | Applied Energy | Experimental | Optical and spectral analysis | N/A |
| 2018 [56] | Renno | Energies | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Stationary, 1D, Finite elements |
| 2018 [57] | Widyolar | Solar Energy | Theoretical | Optical and spectral analysis | N/A |
| 2018 [58] | Widyolar | Applied Energy | Theoretical | Optical and spectral analysis | N/A |
| 2017 [59] | An | Energy Conversion and Management | Theoretical and experimental | Parametric and sensitivity study | Stationary |
| 2017 [60] | Karathanassis | Renewable Energy | Experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2017 [61] | Mohsenzadeh | Renewable Energy | Experimental | Development and experimental testing | N/A |
| 2017 [62] | Srivastava | Solar Energy | Theoretical | Evaluation of thermal and/or electrical performance | Stationary, 3D, Finite elements |
| 2017 [63] | Wang | Applied Thermal Engineering | Theoretical and experimental | Optical and spectral analysis | N/A |
| 2017 [64] | Widyolar | Renewable Energy | Theoretical and experimental | Optical and spectral analysis | Finite elements |
| 2017 [65] | Yazdanifard | Energy Conversion and Management | Theoretical | Parametric and sensitivity study | Quasi-stationary, 1D |
| 2016 [66] | Brekke | Journal of Solar Energy Engineering | Theoretical | Simulation and/or validation of a mathematical model | 2D |
| 2016 [67] | Stanley | Applied Energy | Experimental | Optical and spectral analysis | N/A |
| 2015 [68] | Al-Nimr | Solar Energy | Theoretical | Parametric and sensitivity study | Stationary |
| 2014 [69] | Barrau | Solar Energy | Experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2014 [70] | Xu | Journal of Thermal Science and Engineering Applications | Theoretical | Simulation and/or validation of a mathematical model | Stationary, 2D, Finite elements |
| 2013 [71] | Calise | Energy | Theoretical | Simulation and/or validation of a mathematical model | Stationary |
| 2013 [72] | Karathanassis | Applied Thermal Engineering | Theoretical | Study of geometries and/or solar tracking | N/A |
| Year | Researcher | Journal | Study | Main Focus of Work | Modeling Considerations |
|---|---|---|---|---|---|
| 2023 [73] | Cabral | Energies | Theoretical and experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2023 [74] | Korres | Sustainability | Theoretical | Evaluation of thermal and/or electrical performance | Stationary, Finite elements |
| 2023 [75] | Saberi | Case Studies in Thermal Engineering | Theoretical and experimental | Simulation and/or validation of a mathematical model | Stationary |
| 2020 [76] | Li | Solar Energy | Experimental | Study of geometries and/or solar tracking | Differential eq. |
| 2020 [77] | Li | Solar Energy | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Continuity eq. |
| 2020 [78] | Li | Energy | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Dynamic, Continuity eq. |
| 2020 [79] | Nasseriyan | Energies | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Stationary, Finite elements, Differential eq. |
| 2019 [80] | Cabral | Solar Energy | Theoretical | Optical and spectral analysis | Quasi-dynamic |
| 2019 [81] | Haiping | Applied Thermal Engineering | Experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2019 [82] | Haiping | Energy | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Quasi-stationary |
| 2019 [83] | Haiping | Journal of Cleaner Production | Theoretical and experimental | Simulation and/or validation of a mathematical model | Stationary, 1D |
| 2019 [84] | Wang | Solar Energy | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Dynamic and Stationary |
| 2019 [85] | Xinxin | Desalination | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Stationary, 1D |
| 2019 [86] | Yang | Energy Conversion and Management | Theoretical and experimental | Simulation and/or validation of a mathematical model | Dynamic |
| 2018 [87] | Cabral | Solar Energy | Theoretical | Study of geometries and/or solar tracking | Stationary, 1D |
| 2018 [88] | Gupta | Desalination | Theoretical | Simulation and/or validation of a mathematical model | Quasi-stationary |
| 2018 [89] | Jaaz | Results in Physics | Experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2018 [90] | Tiwari | Solar Energy | Theoretical | Energy and exergy analysis | Quasi-stationary, 1D |
| 2018 [91] | Torres | Energies | Theoretical | Study of geometries and/or solar tracking | N/A |
| 2018 [92] | Zhang | Solar Energy | Theoretical and experimental | Optical and spectral analysis | Energy balance eq. |
| 2017 [93] | Haiping | International Journal of Energy Research | Theoretical and experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2017 [94] | Heng | Journal of Solar Energy Engineering | Theoretical and experimental | Simulation and/or validation of a mathematical model | 3D, Finite elements, Differential eq. |
| 2017 [95] | Jaaz | Materials | Experimental | Evaluation of thermal and/or electrical performance | N/A |
| 2017 [96] | Liu | Energy Conversion and Management | Theoretical | Evaluation of thermal and/or electrical performance | Stationary, 3D, Finite elements |
| 2017 [97] | Probell | Solar Energy | Experimental | Development and experimental testing | N/A |
| 2017 [98] | Singh | Solar Energy | Theoretical | Evaluation of thermal and/or electrical performance | Quasi-stationary, Energy balance eq. |
| 2017 [99] | Tripathi | Solar Energy | Theoretical and experimental | Study of geometries and/or solar tracking | Quasi-stationary, Energy balance eq. |
| 2017 [100] | Tripathi | Energy Conversion and Management | Theoretical | Evaluation of thermal and/or electrical performance | Quasi-stationary |
| 2017 [101] | Zhang | Renewable Energy | Theoretical and experimental | Optical and spectral analysis | Non uniformity eq. |
| 2016 [102] | Atheaya | Solar Energy | Theoretical | Energy and exergy analysis | Quasi-stationary |
| 2016 [103] | Probell | Solar Energy | Theoretical and experimental | Optical and spectral analysis | Finite elements |
| 2016 [104] | Tripathi | Solar Energy | Theoretical | Parametric and sensitivity study | Quasi-stationary, Energy balance eq. |
| 2016 [105] | Tripathi | Solar Energy | Theoretical | Energy and exergy analysis | Quasi-stationary, 1D, Energy balance eq. |
| 2016 [106] | Vance | Journal of Solar Energy Engineering | Theoretical | Study of geometries and/or solar tracking | N/A |
| 2016 [107] | Yousef | Energy Conversion and Management | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Quasi-stationary, Governing Eq. |
| 2015 [108] | Li | Solar Energy | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Dynamic |
| 2015 [109] | Li | Applied Energy | Theoretical and experimental | Development and experimental testing | N/A |
| 2015 [110] | Zhou | Energies | Theoretical | Optical and spectral analysis | Stationary, Finite elements |
| 2014 [111] | Bahaidarah | Applied Energy | Theoretical and experimental | Evaluation of thermal and/or electrical performance | Stationary, 1D, Energy balance eq. |
| 2014 [112] | Guiqiang | Applied Energy | Theoretical and experimental | Optical and spectral analysis | N/A |
| 2014 [113] | Li | Energy Conversion and Management | Theoretical and experimental | Optical and spectral analysis | N/A |
| Mode | Mechanism | Equation or Model | |
|---|---|---|---|
| Conduction | Diffusion of energy due to random molecular motion | (22) | |
| Convection | Diffusion of energy due to random molecular motion plus energy transfer due to global motion (advection) | (23) | |
| Radiation | Energy transfer by electromagnetic waves | (24) | |
| Researcher | Parameters | Equation or Model | |
|---|---|---|---|
| Herez [26] | = Electrical power of the PV. = Area of the PV. = PV concentration ratio. = Incidence angle modifier. = Solar radiation beam. = PV efficiency. = Optical efficiency. | (32) | |
| Karathanassis [45] | = Electrical power. = Electrical efficiency. = Radiation flux incident on the surface of the solar cells. = Aperture area. = Solar cell absorption. = Glass absorption. = Direct solar radiation flux. = Optical efficiency. = Glass transmittance. | (33) | |
| Maatallah [47] Ben Youssef [53,54] | = Electrical output power. = Concentration ratio. = Beam radiation. = PV area. = Optical efficiency. = Module efficiency. = Inverter efficiency. = PV efficiency. | (34) | |
| Alayi [32] Calise [71] | = Electrical power. = PVT concentration ratio. = PVT area. = Beam radiation. = Optical efficiency. = PV efficiency. = Electrical angle of incidence modifier. | (35) | |
| Yazdanifard [65] | = Beam radiation intensity. = Concentration ratio. = Reflection coefficient of the concentrator. = Intercept factor. = PV absorption coefficient. = Transmission coefficient of the glass cover. = Packing factor. = PV/T area. = Reference efficiency of solar cells. = Reference temperature coefficient. = PV temperature. = Reference temperature. | (36) | |
| Ji [122] | = PV electric power. = Photocurrent. = Diode saturation reverse current. = Current. = PV pieces. = Elemental charge. = Load voltage. = Series resistance. = Diode quality factor. = Boltzmann constant. = Average working temperature of the PV. | (37) | |
| Acosta [19,31] | = Electrical power. = Aperture area. = Collector heat removal factor. = Temperature coefficient. = Room temperature. = Intermediate temperature. = Collector heat loss coefficient. = Incident solar irradiance. = PV efficiency. = Reference PV efficiency. = Absorbance. | (38) | |
| Software | Main Characteristics | Simulation Method | Type of License |
|---|---|---|---|
| Tonatiuh 2.2.4 | Tailored for modeling sunlight behavior with solar collectors and concentrators | Ray tracing | Open Source |
| SolTrace 3.4 | Developed by NREL for analyzing solar concentrating systems like parabolic troughs, dish collectors and linear Fresnel systems | Ray tracing | Free |
| TracePro 23.3 | Versatile optical simulation software applicable to a wide range of applications, including solar collectors | Ray tracing | Commercial (paid license) |
| SolView 2021.0 | Allows visualization and analysis of solar ray paths in concentrating systems; evaluates shadows and losses | Ray tracing | Commercial (paid license) |
| Tracer 8.2.1 | Offers advanced optical modeling and analysis capabilities | Ray tracing | Commercial (paid license) |
| Zemax 24.1 | Commercial optical design and analysis software used for various optical systems, including solar collectors | Ray tracing | Commercial (paid license) |
| LightTools 9.1 | Another commercial optical design and analysis software suitable for simulating optical behavior of solar collectors | Ray tracing | Commercial (paid license) |
| RayTracing | Models light propagation through optical components | Ray tracing | Varies (open source and commercial) |
| SolarGIS 1.4.4 | Provides solar resource data and modeling tools, including tools for assessing solar concentration systems | Varies (depending on tool) | Commercial (paid license) |
| TASCSim V4.00 | Developed by Sandia National Laboratories for analyzing the optical and thermal performance of concentrating solar power (CSP) systems | Ray tracing and more | Free |
| OptisWorks | Software suite for optical simulation and virtual prototyping, applicable to optical analysis of solar collectors | Ray tracing | Commercial (paid license) |
| Software | Main Characteristics | Simulation Method | Type of License |
|---|---|---|---|
| TRNSYS 18.05 | Provides a wide range of components for system modeling | Numerical methods | Commercial (paid license) |
| RETScreen 9.1.0.24 | Developed for analyzing clean energy projects with modules for solar thermal collector simulations | Numerical methods | Free |
| SAM 2022.11.21 | Versatile tool for modeling the financial and energy performance of solar thermal systems including collectors | Numerical methods | Free |
| PVSyst 7.4 | Known for photovoltaic simulations; it also offers thermal modules for solar collectors | Numerical methods | Commercial (paid license) |
| HOMER | Optimizes and analyzes renewable energy systems, including solar thermal collectors | Energy balance analysis | Commercial (paid license) |
| RETScreen Expert eighth version | An advanced version of RETScreen with additional features for detailed solar thermal system simulations | Numerical methods | Free |
| Transol 3.2 | Specialized software for simulating solar thermal collectors and domestic hot water systems. Commonly used in the solar thermal industry | Numerical methods | Commercial (paid license) |
| EnergyPlus 8.4 | Building energy simulation software that can model solar thermal systems integrated into buildings | Numerical methods | Free |
| EES 10.2 | The software is a general equation solver used for a wide range of engineering and thermodynamic simulations | Numerical methods | Commercial (paid license) |
| Geometry of SRC-PTV | Example | Description |
|---|---|---|
| Simple Rectangular: Xinxin [85]; Haiping [83]; Xu [70]; Hou [15]; Maatallah [47]; Ben Youssef [54]. | 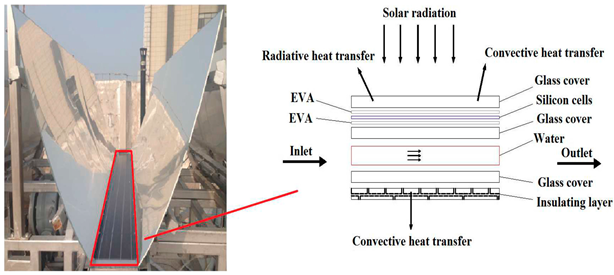 | It has a rectangular prism structure, with one of the larger rectangular sides mounted with a PV and situated at the focal point of a CPC or PTC. Behind the PV, a rectangular trough is installed for circulating the HTF. |
| Rectangular with cylindrical pipe: Yazdanifard [65]; Cabral [80]; Ji [122]. |  | It adopts the same geometry and trough as the SRC-PTV “Simple Rectangular”, but inside the trough there is a substrate that acts as a thermal insulator and one or more cylindrical absorber pipes in which the HTF circulates. |
| Rectangular with fins: Karathanassis [45]. | 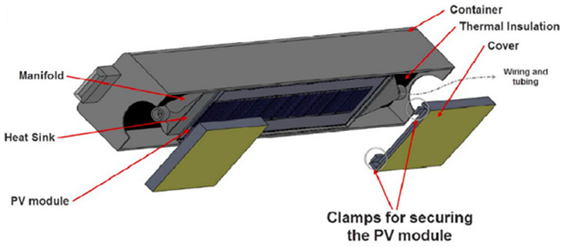 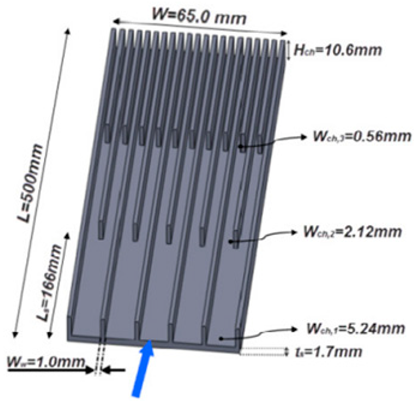 | The SRC-PTV “Simple Rectangular” has identical geometry and trough design to the one mentioned above, but inside the trough there are fins to enhance heat transfer between the HTF and the PV cell. |
| Triangular: Herez [25]; Calise [71]; Zheng [18]; Santana [17]; Mohsenzadeh [61]. | 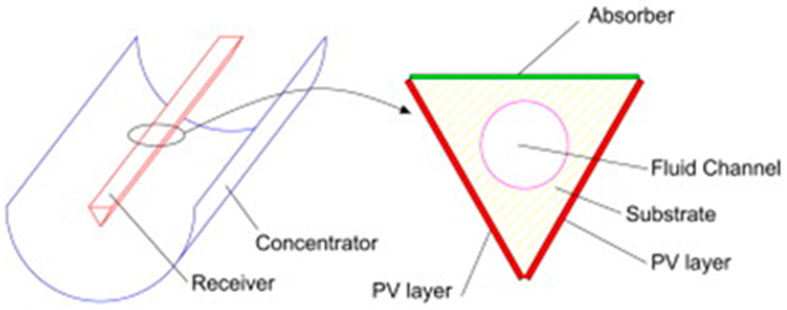 | It has a triangular prismatic structure in which the two lower sides are equipped with PVs and are oriented to the focal point of a PTC, while the upper side is equipped with a heat absorber. An absorber pipe is placed within of triangular prism, where the HTF circulates. |
| Wedge: Gorouh [21]; Cabral [24]. | 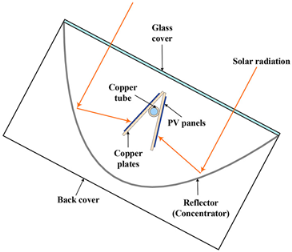 | The geometry of the trough is in the form of a “V” or wedge. An absorber tube is placed at the inner bottom of the trough, where the HTF circulates. PVs are mounted on the exterior sides of the trough. |
| Bifacial: Cabral [73,87]. | 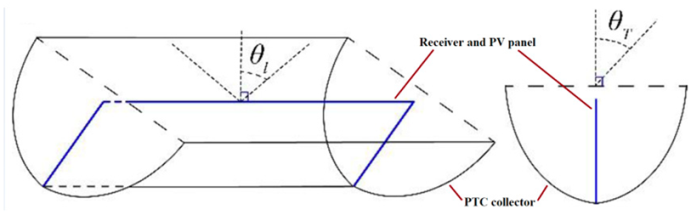 | It consists of two PVs positioned opposite each other and facing each side of a PTC or CPC. A series of pipes are placed between the backs of the PVs to circulate the HTF. |
| Cylindrical with internal CPC: Widyolar [64]. | 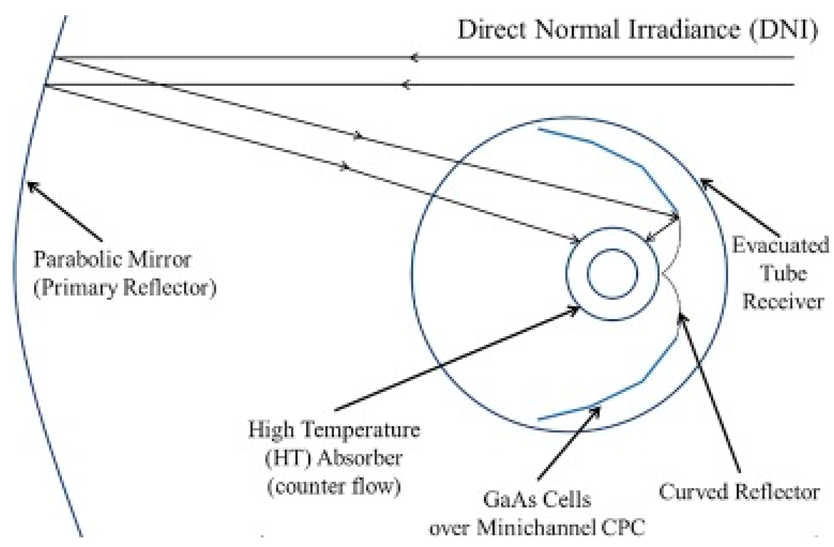 | It has cylindrical geometry that can adopt the dimensions of a conventional SRC-PTV for industrial thermal applications. It incorporates a CPC with PVs at its extremities and an absorber pipe with the HTF at its focal point. |
| Cylindrical with intermediate PV: Gakkhar [34]. | 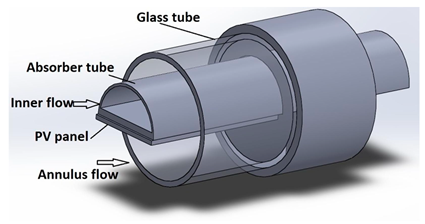 | It has cylindrical geometry that can adopt the dimensions of a conventional SRC-PTV for industrial thermal applications. The difference is that the absorber pipe in the center of the cylinder is replaced by a PV. The HTF circulates on the front and back of the PV. |
| Cylindrical with PVs on pipe: Srivastava [62]; Felsberger [33]. | 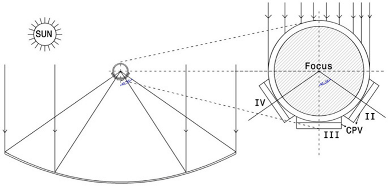 | It has cylindrical geometry that can adopt the dimensions of a conventional SRC-PTV for industrial thermal applications. Within the cylinder resides an absorber pipe surrounded by PVs. |
| Author | Electrical Efficiency Equation | Thermal Efficiency Equation | ||
|---|---|---|---|---|
| Herez [25,26] | (41) | (42) | ||
| Karathanassis [45,60,72] | (43) | (44) | ||
| Haiping [83] | (45) | (46) | ||
| Xinxin [85] | (47) | (48) | ||
| Xu [122] | (49) | (50) | ||
| Studies Included in the Review that Report Experimental Results | |
|---|---|
| PTC collectors | Felsberger [33] Gakkar [34] Otanicar [37] Mohsenzadeh [61] |
| CPC collectors | Probell [97] Tripathi [99] Li [76,78,108] Bahaidarah [111] |
| Studies not included in the review that report experimental results | |
| PTC collectors | Ji [122] Bernardo [126] |
| CPC collectors | Othman [127] |
| Component | Parameter | Value | Unity |
|---|---|---|---|
| PTC | Aperture area | 60 | m2 |
| Absorptance | 0.03 | - | |
| Emissivity | 0.30 | - | |
| Concentration ratio | 10 | - | |
| PV | Area | 6.0 | m2 |
| Thermal conductivity | 50 | W/mK | |
| Absorptance | 0.97 | - | |
| Emissivity | 0.20 | - | |
| Absorber | Area | 3.0 | m2 |
| Thermal conductivity | 205 | W/mK | |
| Absorptance | 0.90 | - | |
| Emissivity | 0.20 | - | |
| Substrate | Thermal conductivity | 250 | W/mK |
| TEG | TEG area | 0.0025 | m2 |
| Thermal conductivity | 1.4 | W/mK | |
| Absorptance | 0.4 | - | |
| Emissivity | 0.60 | - | |
| HTF pipeline | Pipe diameter | 0.06 | m |
| HTF specific heat | 4183 | J/kg K | |
| Mass flow rate | 0.15 | Kg/s | |
| IAM electrical coefficient | 0.28 | - | |
| IAM thermal coefficient | 0.14 | - | |
| Boundary conditions | Total radiation | 1000 | W/m2 |
| Beam radiation | 800 | W/m2 | |
| Sky temperature | 25 | °C | |
| Ambient temperature | 25 | °C | |
| Fluid inlet temperature | 25 | °C | |
| Air velocity | 5 | m/s | |
| Incident angle | 0 | ° |
| Parameter | Results from Thermal Model | Reference [130] | Deviation |
|---|---|---|---|
| Thermal power Total electrical power PV temperature | 177.9 W 20.9 W 94.75 °C | 176.3 W 19.6 W 90.75 °C | −0.9% −6.6% −1.1% |
| Parameter | Results from thermal model | Reference [61] | Deviation |
| HTF outlet temperature PV temperature Absorber temperature Substrate temperature | 77.7 °C 82.56 °C 78.8 °C 78.7 °C | 79.3 °C 82.3 °C 82.7 °C 80.5 °C | 0.45% −0.07% 1.10% 0.51% |
| Component | Parameter | Value | Unity |
|---|---|---|---|
| PTC | Concentration ratio | 15 | - |
| Reflection coefficient | 0.8 | - | |
| Intercept factor | 0.95 | - | |
| Glass cover | Thickness | 0.004 | m |
| Transmittance | 0.92 | - | |
| Absorptance | 0.04 | - | |
| PV | Super-thermal conductivity | 150.0 | (W m2)/°C |
| Super-absorbance | 0.80 | - | |
| Super-emittance | 0.35 | - | |
| Super-thickness | 0.30 | mm | |
| GaAs thermal conductivity | 55.0 | (W m2)/°C | |
| GaAs absorbance | 0.85 | - | |
| GaAs emittance | 0.30 | - | |
| GaAs thickness | 0.70 | mm | |
| Reference efficiency | 15 | % | |
| Reference temperature coefficient | −273.1455 | °C−1 | |
| Reference temperature | 24.85 | °C | |
| HTF | Inlet temperature | 24.85 | °C |
| Mass flow rate in laminar regime | 0.02 | kg s−1 | |
| Mass flow rate in turbulent regime | 0.12 | kg s−1 | |
| Boundary conditions | Wind velocity | 1.5 | m s−1 |
| Collector slope | 30 | ° | |
| Ambient temperature | 24.85 | °C | |
| Solar radiation intensity | 700 | W m−2 | |
| Packing factor | 0.9 | - | |
| Pump efficiency | 0.8 | - |
| PV Type | Parameter | Value | ||||
|---|---|---|---|---|---|---|
| Reference [122] | Model Result | Deviation | Reference [122] | Model Result | Deviation | |
| Substrate | 50.1 °C | 50.9 °C | 1.6% | 83.2 °C | 81.7 °C | −1.8% |
| 39.0 °C | 37.2 °C | −4.6% | 57.6 °C | 62.7 °C | 8.8% | |
| GaAs | 39.1 °C | 38.3 °C | −2.0% | 61.4 °C | 62.9 °C | 2.4% |
| 38.2 °C | 37.4 °C | −2.1% | 59.4 °C | 61.2 °C | 3.0% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chavarría-Domínguez, B.; De León-Aldaco, S.E.; Velázquez-Limón, N.; Ponce-Silva, M.; Aguilar-Jiménez, J.A.; Chavarría-Domínguez, F. A Review of the Modeling of Parabolic Trough Solar Collectors Coupled to Solar Receivers with Photovoltaic/Thermal Generation. Energies 2024, 17, 1582. https://doi.org/10.3390/en17071582
Chavarría-Domínguez B, De León-Aldaco SE, Velázquez-Limón N, Ponce-Silva M, Aguilar-Jiménez JA, Chavarría-Domínguez F. A Review of the Modeling of Parabolic Trough Solar Collectors Coupled to Solar Receivers with Photovoltaic/Thermal Generation. Energies. 2024; 17(7):1582. https://doi.org/10.3390/en17071582
Chicago/Turabian StyleChavarría-Domínguez, Benjamín, Susana Estefany De León-Aldaco, Nicolás Velázquez-Limón, Mario Ponce-Silva, Jesús Armando Aguilar-Jiménez, and Fernando Chavarría-Domínguez. 2024. "A Review of the Modeling of Parabolic Trough Solar Collectors Coupled to Solar Receivers with Photovoltaic/Thermal Generation" Energies 17, no. 7: 1582. https://doi.org/10.3390/en17071582
APA StyleChavarría-Domínguez, B., De León-Aldaco, S. E., Velázquez-Limón, N., Ponce-Silva, M., Aguilar-Jiménez, J. A., & Chavarría-Domínguez, F. (2024). A Review of the Modeling of Parabolic Trough Solar Collectors Coupled to Solar Receivers with Photovoltaic/Thermal Generation. Energies, 17(7), 1582. https://doi.org/10.3390/en17071582










