Ground Resistance Estimation for a DC Converter Station: Theory versus Experiment
Abstract
1. Introduction
| Ref. | Year | Experimental/Theoretical | Grid Geometry | Measurement Method | Calculation Method | Results | Soil Type |
|---|---|---|---|---|---|---|---|
| [14] | 2004 | Theoretical | Earthing grid with dimensions | - | Finite element method, COMSOL Multiphysics | Ground resistance and surface potential | Uniform, two-layer, and multilayer soils |
| [8] | 2018 | Theoretical | Earthing grid with dimensions | - | IEEE Std.80-2000 | Grid resistance, ground potential rise, mesh and step potential. | Multilayer soil |
| [9] | 2013 | Theoretical | Earthing grid with dimensions | - | IEEE Std.80-2000 | Grid resistance, mesh, and step potential. | Uniform and multilayer soil |
| [23] | 2014 | Experimental and theoretical | Earthing grid with dimensions | Wenner four-pole -method for soil resistivity measurements | CDEGS software | Ground resistance | Two-layer soil |
| [17] | 2023 | Theoretical | Rod dimension with radius , length | - | ANSYS software | Ground resistance | Nonuniform, homogeneous soil |
| [21] | 2023 | Theoretical | Earthing grid with unequal conductor spacing. | - | Modified Harris Hawks Optimizer | Ground resistance | Two-layer soil |
| [11] | 2022 | Theoretical | Earthing grid with dimensions depth depth | - | Boundary element method | Grid resistance, ground potential rise, mesh and step potential. | Uniform soil |
| [22] | 2022 | Experimental | Auxiliary circuits displaced vertically in the ground. | Grounding impedance measuring circuit using low-velocity propagation current | - | Transient impedance. | Uniform soil. |
| [15] | 2021 | Experimental and theoretical | Earthing grid with dimensions , and buried at . | Ground resistance tester | CDEGS software | Ground resistance, step and touch potential. | Uniform soil. |
| [39] | 2020 | Theoretical | Earthing grid with different shapes | - | Finite element method and genetic algorithm | Ground resistance, step and touch potential. | Multilayer soil |
| [25] | 2018 | Experimental and theoretical | Earthing grid with dimensions | Current simulation method with point source | Grid resistance, mesh and step potential. | Multilayer soils. | |
| [26] | 2012 | Theoretical | Earthing grid with dimensions | - | Current simulation method with point sources. | Ground resistance and earth surface potential. | Two-layer soil |
| [12] | 2013 | Theoretical | Earthing grid with dimensions | - | Current simulation method with point sources. | Ground resistance and earth surface potential. | Two-layer soil |
| [13] | 2013 | Theoretical | Earthing grid supported by vertical rods. and 75 m × 31.25 m | - | Current simulation with point sources. Software TOTBEM. | Ground resistance and earth surface potential | Homogeneous soil |
| [29] | 2017 | Experimental | Earthing grid with dimensions 200 m × 150, 200 m × 100 | Conventional fall-of-potential | - | Ground resistance | Nonhomogeneous soil |
| [19] | 2017 | Experimental and theoretical | Earthing grid with dimensions , provided with/without rods. | Wenner four-pole -method for soil resistivity measurements at selected sites. | Finite element method, CYMGRD Software | Ground resistance, touch, step and surface potential. | Wet and dry soil |
| [27] | 2019 | Experimental and theoretical | Earthing grid with rod and plate electrodes buried vertically. | Wenner four-pole -method for soil resistivity measurements at selected sites. | Finite element method, COMSOL Multiphysics | Ground resistance, surface potential. | Uniform soil |
| [24] | 2014 | Theoretical | Earthing grid with dimension | - | Finite element method, COMSOL Multiphysics | Ground resistance, surface potential. | Two-layer soil |
| [32] | 2011 | Experimental | Earthing grid with dimension | Four-pole method for soil resistivity and fall of potential method for measuring ground resistance. | Finite element method, COMSOL Multiphysics | Ground resistance, touch, step, and surface potential. | Two-layer soil |
| [30] | 2006 | Experimental and theoretical | S2emi-spherical of radius | Soil resistivity measurement by Wenner method and ground resistance using the fall-of-potential method | Finite-element method | Ground resistance, soil resistivity. | Uniform soil |
| [33] | 2004 | Theoretical | Earthing grid with dimensions | - | Extremal charges method | Earth surface potential | Multilayer, homogeneous soil |
| [31] | 1994 | Theoretical | Earthing grid with dimensions | Voltage and current measurements. | The method of images+ voltage and current measurements. | Ground resistance, touch, step and surface potential. | Uniform, two-layer soil |
| [28] | 1998 | Experimental and theoretical | Earthing grid with dimensions | fall-of-potential method | Simulation by current sources. | Ground resistance | Two-layer soil |
2. Research Gaps
3. Problem Formation
4. Method of Analysis
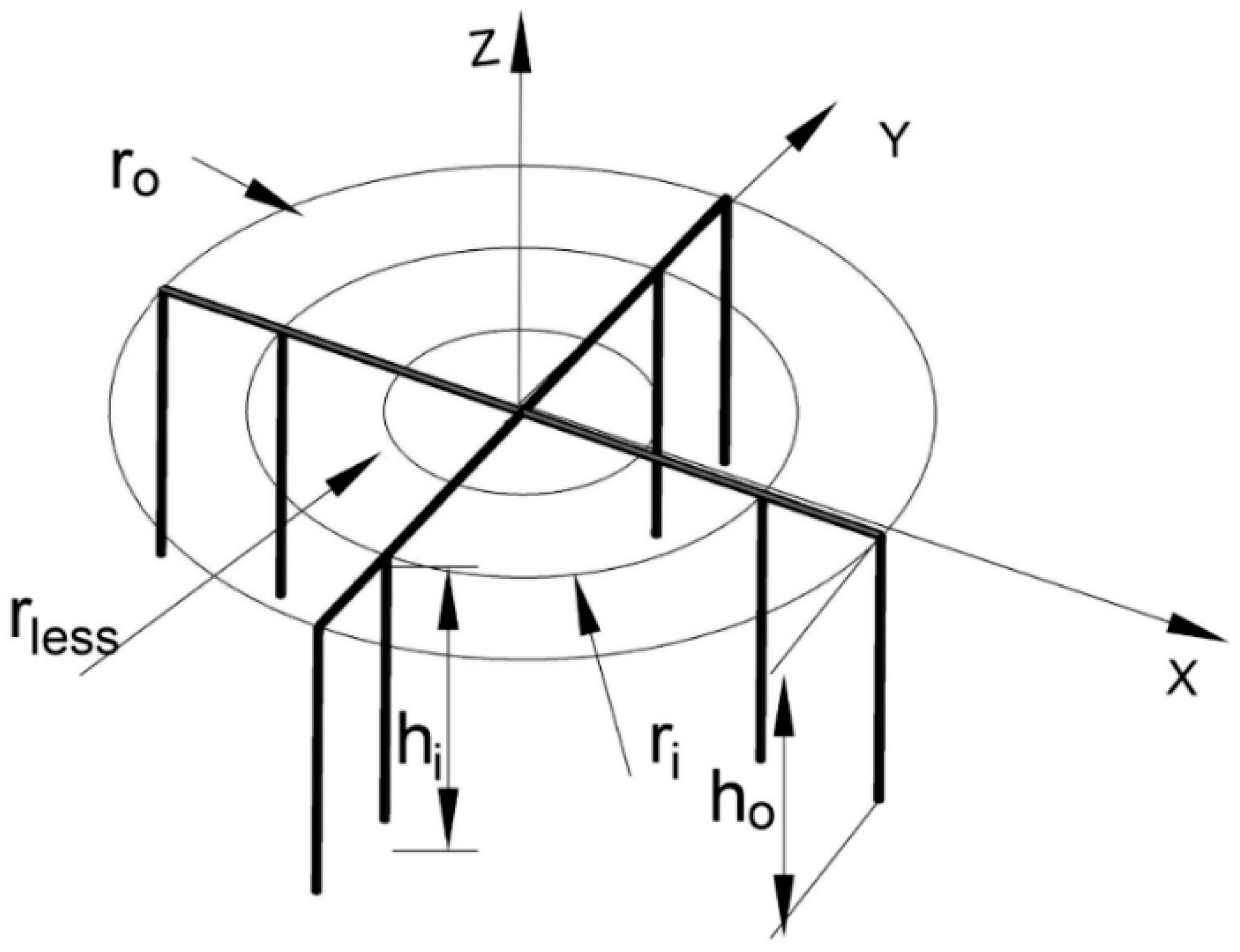
4.1. Geometry of the Grounding Scheme
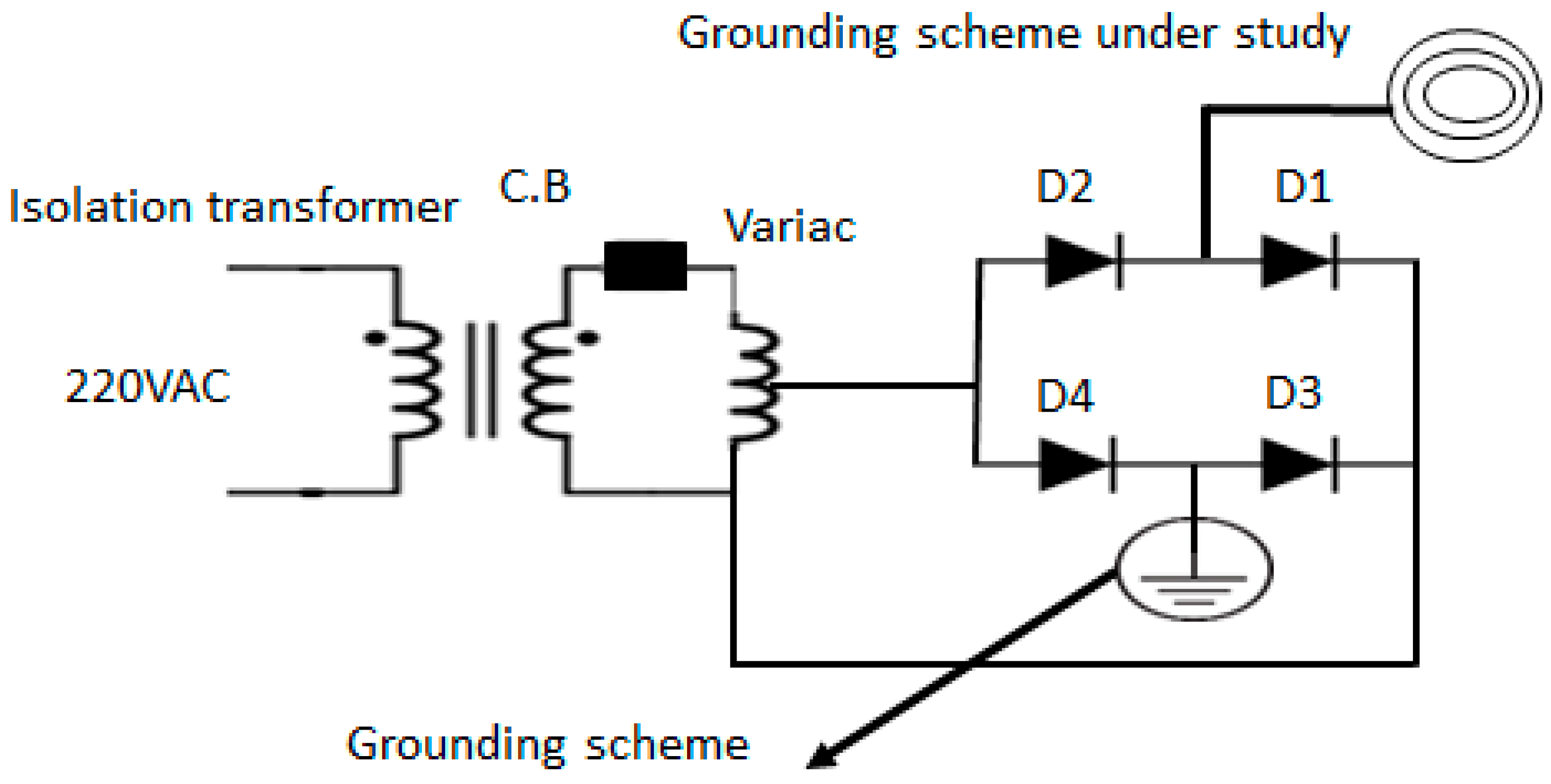
4.2. Simulation Technique
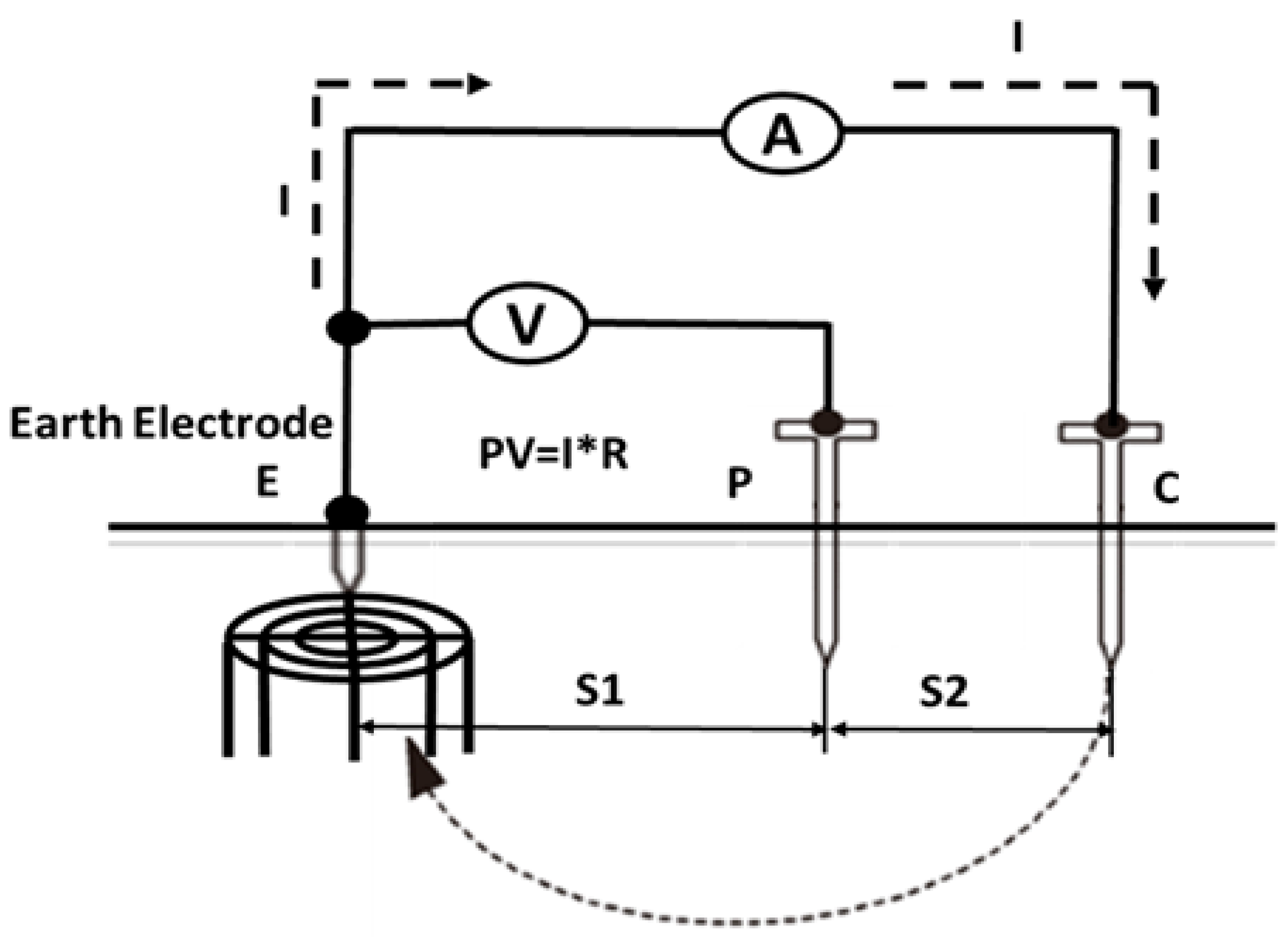
5. Experimental Setup and Technique
6. Results and Discussion
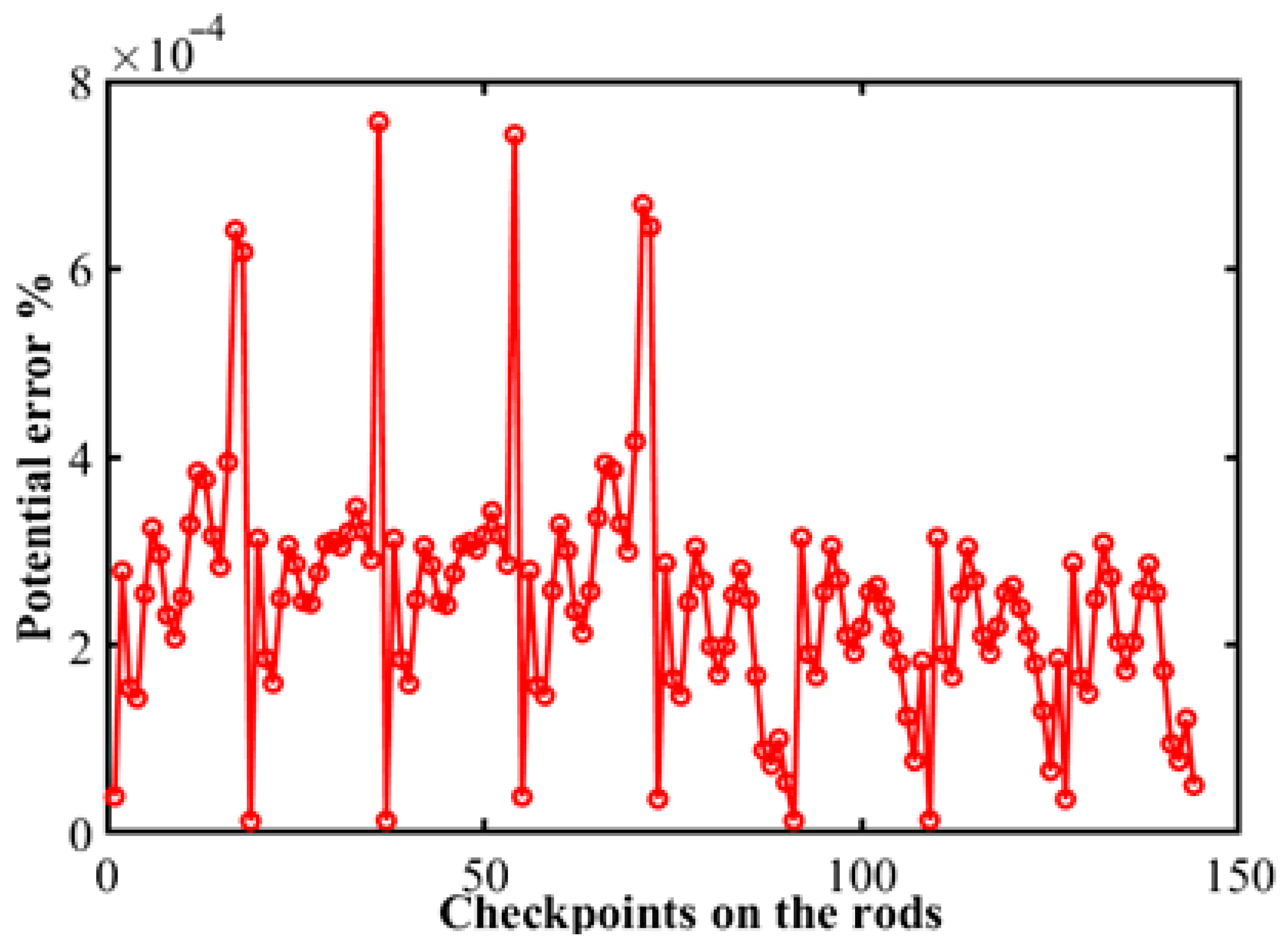
6.1. Accuracy of Simulation Technique
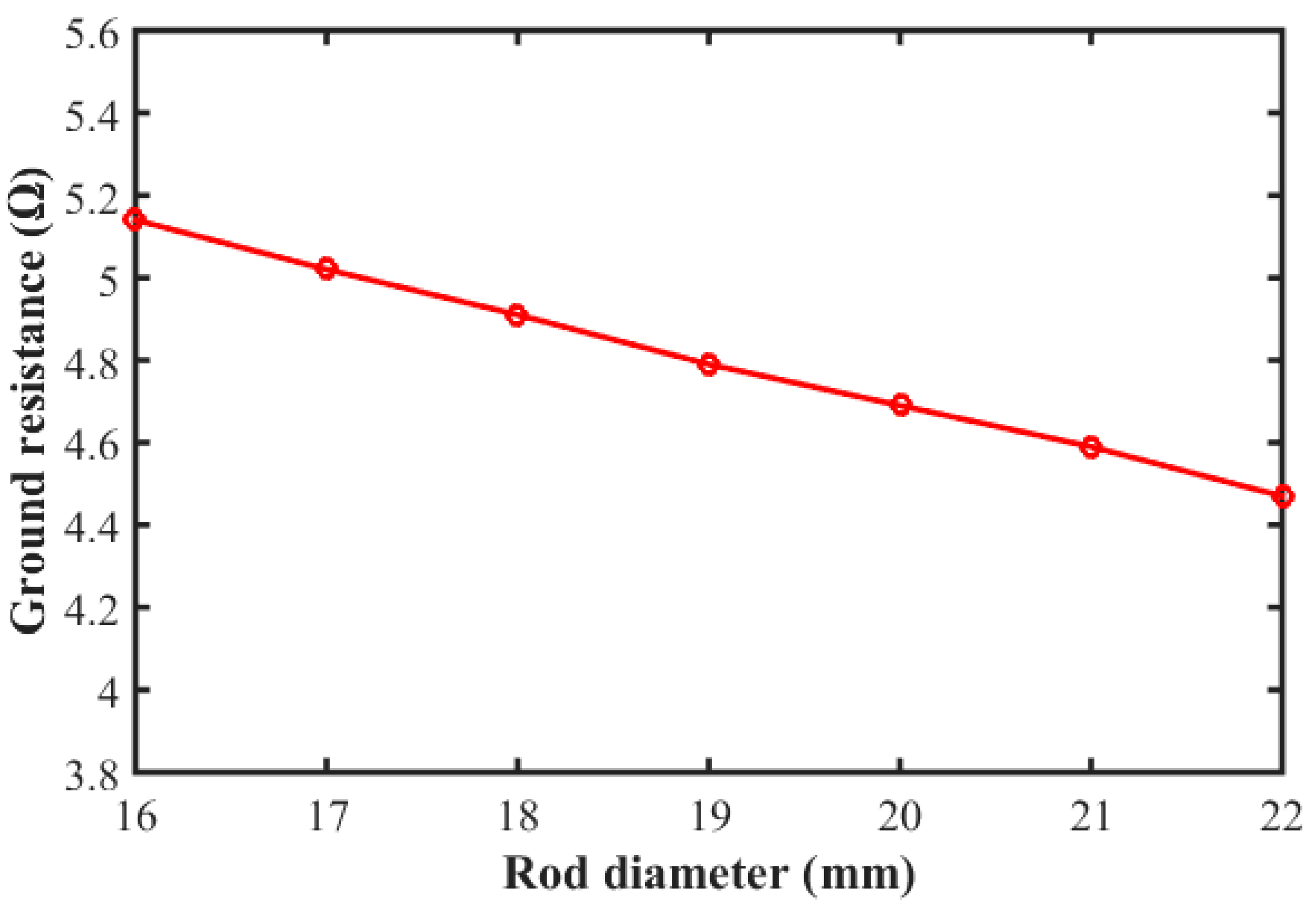
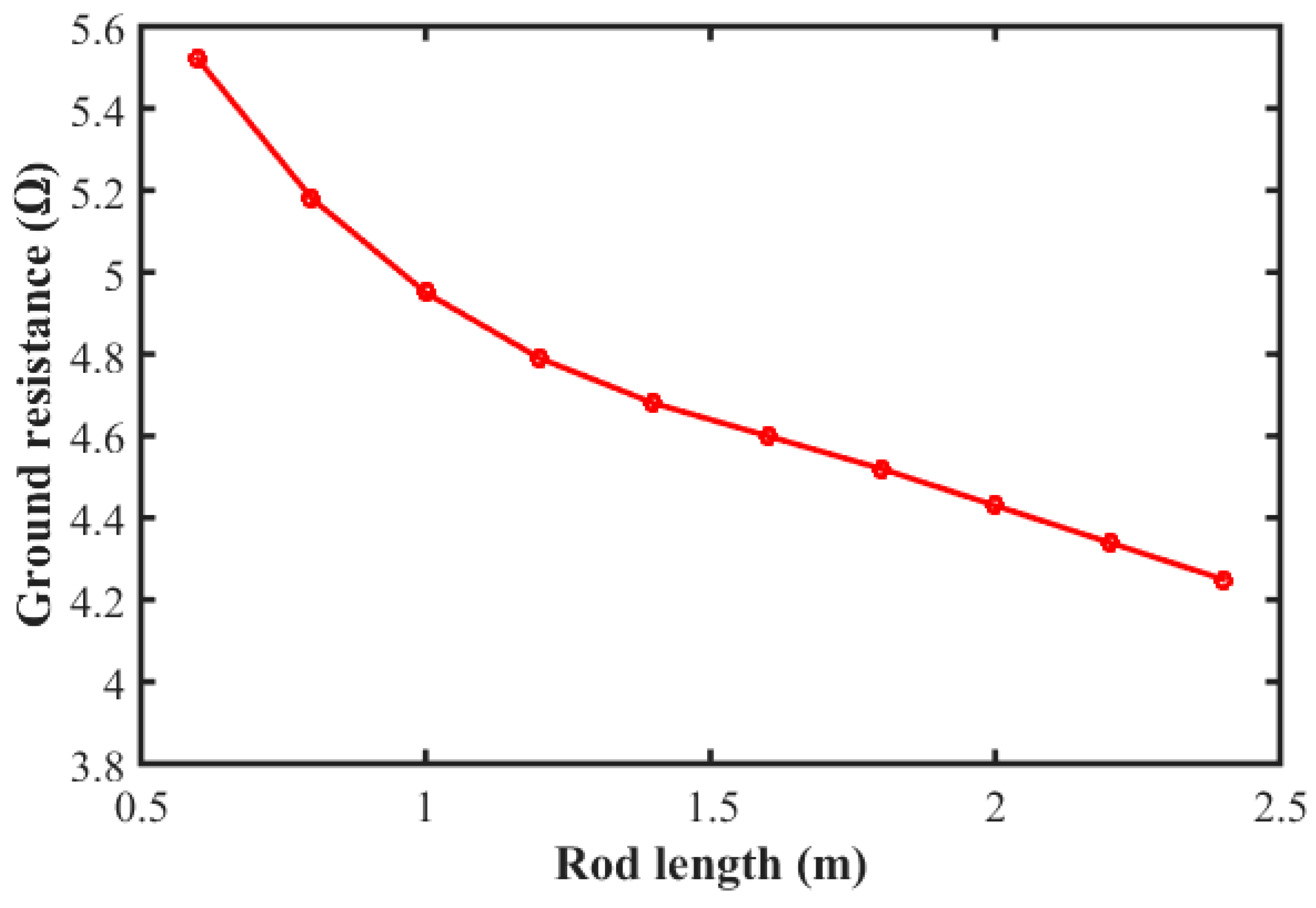
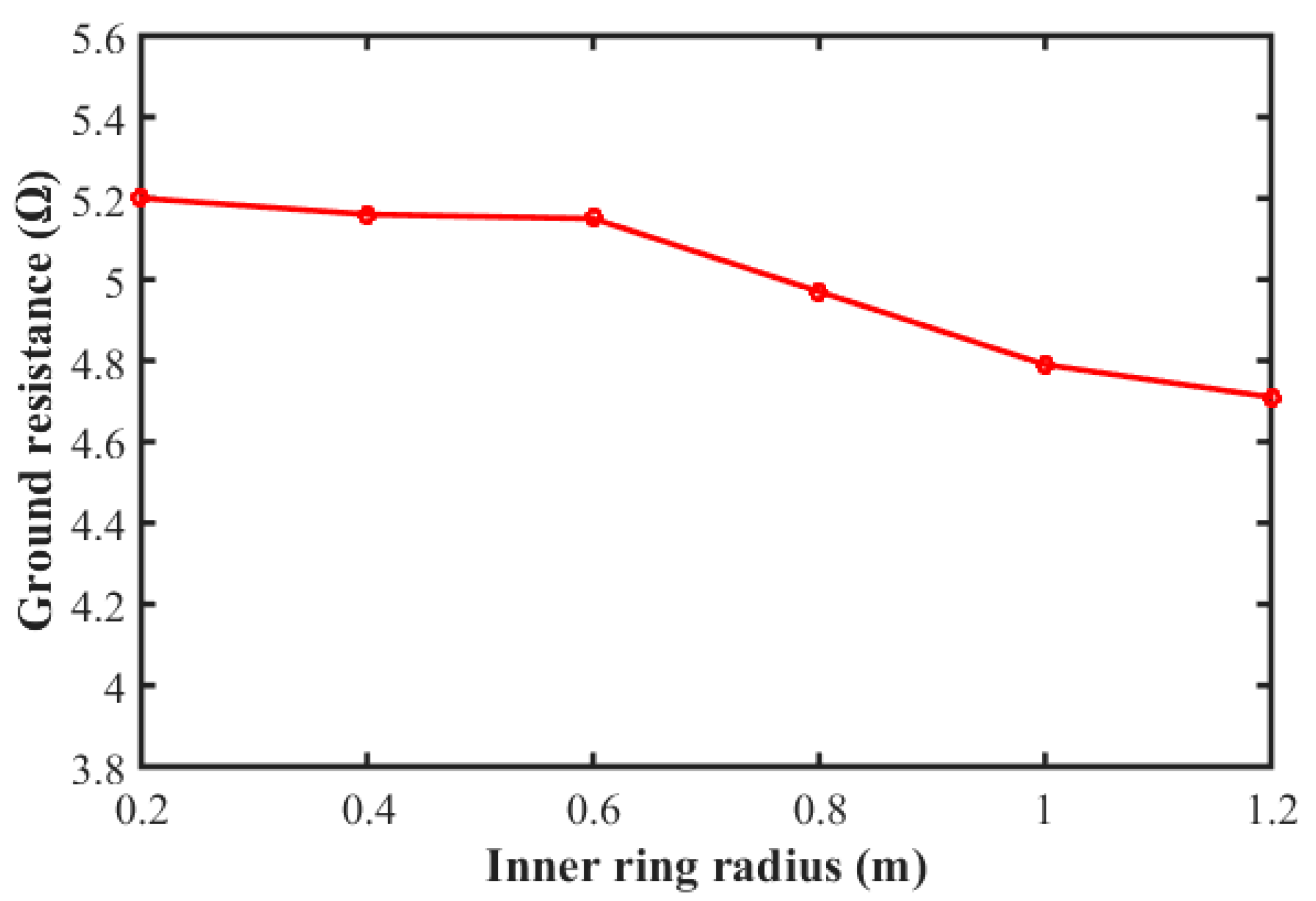
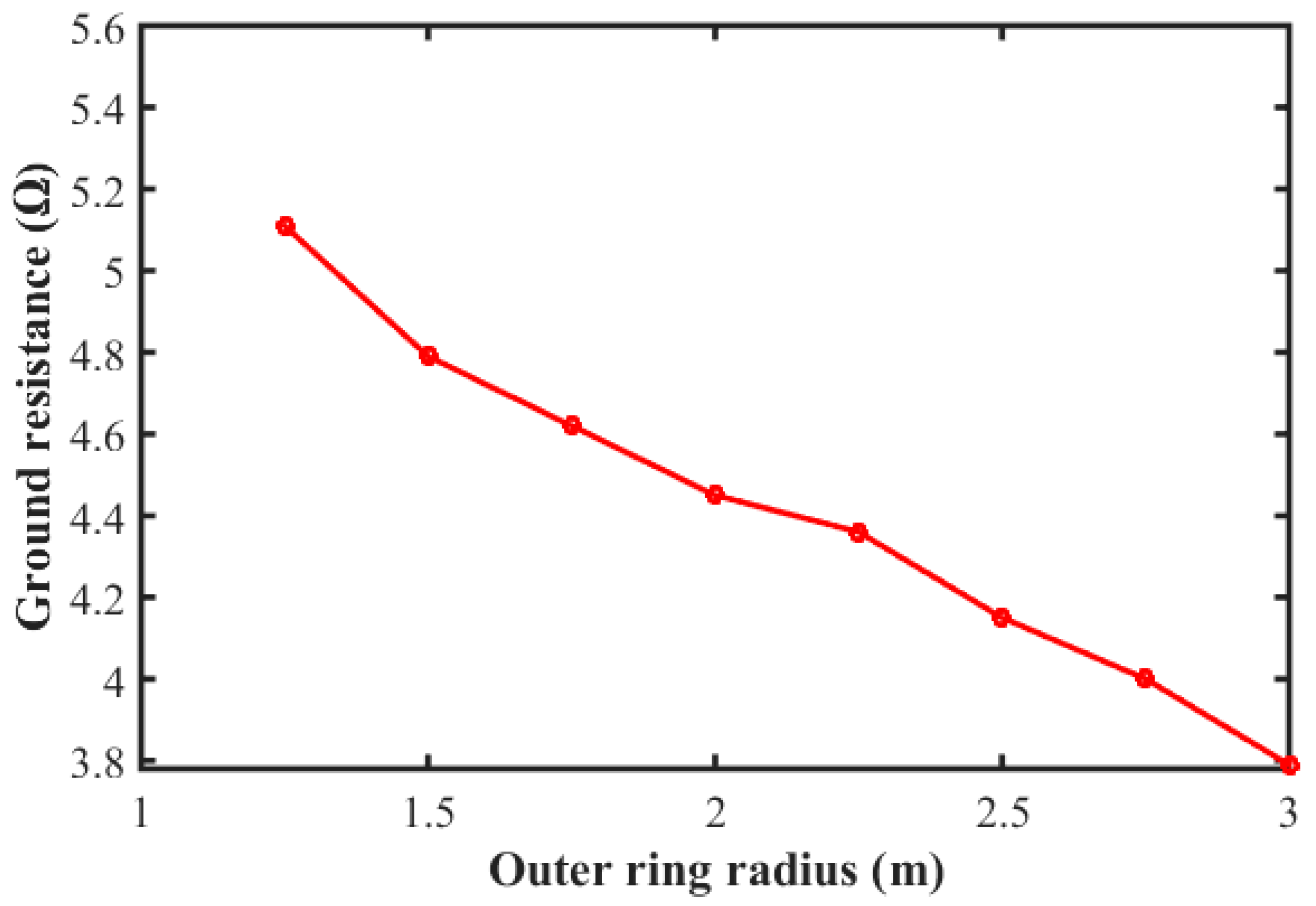
6.2. Ground Resistance as Influenced by Grounding-Scheme Parameters
6.3. Surface Potential
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Electrical capacitance | |
| Circuit breaker | |
| Current electrode | |
| CST | Charge simulation technique |
| Rectification diodes | |
| Grounding scheme | |
| HVDC | High-voltage direct current |
| Length of inner rod | |
| Length of outer rod | |
| Depth of rings forming the grounding scheme | |
| IEC | International electrotechnical commission |
| Conductor length | |
| Number of simulation charges | |
| Number of check points/Number of check points | |
| Potential coefficient | |
| Potential electrode | |
| Potential due to the simulation charges at the ith boundary point. | |
| Radius of inner ring | |
| Radius of outer ring | |
| Radius of rodless ring; | |
| Resistance of grounding scheme | |
| Rod radius | |
| Conductor radius | |
| Distance between grounding scheme and potential electrode | |
| Distance between current electrode and potential electrode | |
| Voltage Applied | |
| Soil resistivity | |
| Soil permittivity |
References
- Stockin, D.R. Grounding and Earthing Handbook, 1st ed.; McGraw-Hill’s: New York, NY, USA, 2014; ISBN 978-0-07-180065-5. [Google Scholar]
- Kimbark, E.W. Direct Current Transmission, 1st ed.; Wiley: New York, NY, USA, 1971. [Google Scholar]
- CIGRE Working Group. General Guidelines for HVDC Electrode Design; CIGRÉ: Paris, France, 2017; ISBN 9782858733781. [Google Scholar]
- Aljohani, T.M.; Alzahrani, A.M. The Operation of the GCCIA HVDC Project and Its Potential Impacts on the Electric Power Systems of the Region. Int. J. Electron. Electr. Eng. 2014, 2, 207–213. [Google Scholar] [CrossRef]
- IEC TS 60479-1; Effects of Current on Human Beings and Livestock—Part 1: General Aspects. International Electrotechnical Commission: Geneva, Switzerland, 2016.
- Meliopoulos, A.P.S. Power System Grounding and Transients: An Introduction, 1st ed.; Marcel Dekker Inc.: New York, NY, USA, 1988. [Google Scholar]
- Li, B.; Li, C.; Li, B.; Wen, W. Study on the distributed-parameter resistance earth model and potential distribution of the monopole-ground-return HVDC. Electr. Power Syst. Res. 2020, 187, 106478. [Google Scholar] [CrossRef]
- Pratama, R.A.; Hermawan; Facta, M. Analysis of Grounding System in 150 kV Kudus Substation. In Proceedings of the 2018 5th International Conference on Information Technology, Computer, and Electrical Engineering (ICITACEE), Semarang, Indonesia, 27–28 September 2018; pp. 245–250. [Google Scholar]
- Kushare, B.E.; Unde, M.G. Impact of seasonal variation of soil resistivity on safety of substation grounding system. In Proceedings of the Fifth International Conference on Advances in Recent Technologies in Communication and Computing (ARTCom 2013), Bangalore, India, 20–21 September 2013; Institution of Engineering and Technology: Lucknow, India, 2013; pp. 173–182. [Google Scholar]
- Singer, H.; Steinbigler, H.; Weiss, P. A Charge Simulation Method for the Calculation of High Voltage Fields. IEEE Trans. Power Appar. Syst. 1974, PAS-93, 1660–1668. [Google Scholar] [CrossRef]
- Guizán, R.; Colominas, I.; París, J.; Couceiro, I.; Navarrina, F. Numerical analysis and safety design of grounding systems in underground compact substations. Electr. Power Syst. Res. 2022, 203, 107627. [Google Scholar] [CrossRef]
- Ghoneim, S.S.M. Charge and Current Simulation Method with Boundary Element Method for Grounding System Calculations in Case of MultiLayer Soil. IOSR J. Eng. 2013, 03, 14–22. [Google Scholar] [CrossRef]
- Ghoneim, S.S.M. Analytical methods of earth surface potential calculation for grounding grids. Int. J. Eng. Comput. Sci. 2013, 13, 3–47. [Google Scholar]
- Güemes, J.A.; Hernando, F.E. Method for calculating the ground resistance of grounding grids using FEM. IEEE Trans. Power Deliv. 2004, 19, 595–600. [Google Scholar] [CrossRef]
- Cai, Y.; Ji, H.; Wang, D.; Lei, X.; Sun, Y.; Yuan, S. Optimal construction method and demonstration application of multi-in-one station grounding system. Glob. Energy Interconnect. 2021, 4, 520–530. [Google Scholar] [CrossRef]
- Freire, P.; Kalife, J.; Oliveira, G. Rio Madeira HVDC System: Commissioning of the Ground Electrode for Bipole 2 at Porto Velho. Appl. Sci. 2022, 12, 3279. [Google Scholar] [CrossRef]
- Kwon, H.; Kil, Y.; Kim, S. A method for grounding resistance calculation of vertical electrode. Electr. Power Syst. Res. 2023, 224, 109718. [Google Scholar] [CrossRef]
- Wen, X.; Jing, M.; Cai, H.; Zhang, Y.; Hu, S.; Teng, Y.; Liu, G.; Lan, L.; Lu, H. Temperature characteristics and influence of water-saturated soil resistivity on the HVDC grounding electrode temperature rise. Int. J. Electr. Power Energy Syst. 2020, 118, 105720. [Google Scholar] [CrossRef]
- Salam, M.A.; Rahman, Q.M.; Ang, S.P.; Wen, F. Soil resistivity and ground resistance for dry and wet soil. J. Mod. Power Syst. Clean Energy 2017, 5, 290–297. [Google Scholar] [CrossRef]
- Paulo, P.E.; Pereira, S.Y.; Padilha, A.L. Adjustment of a crustal geoelectric model from Commissioning data of HVDC ground electrodes: A case study from the Northeastern Paraná Basin, Brazil. J. Appl. Geophys. 2020, 182, 104186. [Google Scholar] [CrossRef]
- Bhosale, M.J.; Karandikar, P.B.; Kulkarni, N.R. An optimal design for grounding grid configuration with unequal conductor spacing. Adv. Eng. Softw. 2023, 176, 103367. [Google Scholar] [CrossRef]
- Guimarães, M.F.; Paulino, J.O.S.; Caetano, C.E.F.; Barbosa, C.F.; Boaventura, W.C. Grounding measurements using auxiliary circuits disposed vertically in the ground. Electr. Power Syst. Res. 2022, 213, 108727. [Google Scholar] [CrossRef]
- Buba, S.D.; Wan Ahmad, W.F.; Ab Kadir, M.Z.A.; Gomes, C.; Jasni, J.; Osman, M. Design of distribution substation earth grid in high resistivity soil using CDEGS. In Proceedings of the 2014 IEEE 8th International Power Engineering and Optimization Conference, PEOCO 2014, Langkawi, Malaysia, 24–25 March 2014; IEEE Computer Society: Washington, DC, USA, 2014; pp. 508–513. [Google Scholar]
- Trifunovic, J.; Kostic, M. Analysis of influence of imperfect contact between grounding electrodes and surrounding soil on electrical properties of grounding loops. Electr. Eng. 2014, 96, 255–265. [Google Scholar] [CrossRef]
- Mondal, D.; Dey, S.; Pradhan, A.K.; Das, S. Earthing grid designs for heterogeneous soil structures in hilly regions using current simulation method. IET Gener. Transm. Distrib. 2018, 12, 3021–3027. [Google Scholar] [CrossRef]
- Zaini, H.G.; Ghoneim, S.S. Earth surface potential and grounding resistance for grounding grid in two-layer model soil. In Proceedings of the 2012 IEEE International Conference on Power System Technology (POWERCON), Auckland, New Zealand, 30 October–2 November 2012; pp. 1–5. [Google Scholar]
- Colella, P.; Pons, E.; Tommasini, R.; Silvestre, M.L.D.; Sanseverino, E.R.; Zizzo, G. Fall of potential measurement of the earth resistance in urban environments: Accuracy evaluation. IEEE Trans. Ind. Appl. 2019, 55, 2337–2346. [Google Scholar] [CrossRef]
- Wang, C.-g.; Takasima, T.; Sakuta, T.; Tsubota, Y. Grounding resistance measurement using fall-of-potential method with potential probe located in opposite direction to the current probe. IEEE Trans. Power Deliv. 1998, 13, 1128–1135. [Google Scholar] [CrossRef]
- Yuan, T.; Bai, Y.; Sima, W.; Xian, C.; Peng, Q.; Guo, R. Grounding resistance measurement method based on the fall of potential curve test near current electrode. IEEE Trans. Power Deliv. 2017, 32, 2005–2012. [Google Scholar] [CrossRef]
- Güemes-Alonso, J.A.; Hernando-Fernández, F.E.; Rodríguez-Bona, F.; Ruiz-Moll, J.M. A practical approach for determining the ground resistance of grounding grids. IEEE Trans. Power Deliv. 2006, 21, 1261–1266. [Google Scholar] [CrossRef]
- Dawalibi, F.P.; Ma, J.; Southey, R.D. Behaviour of grounding systems in multilayer soils: A parametric analysis. IEEE Trans. Power Deliv. 1994, 9, 334–342. [Google Scholar] [CrossRef]
- Salam, M.A.; Jen, K.M.; Khan, M.A. Measurement and simulation of grounding resistance with two and four mesh grids. In Proceedings of the 2011 IEEE Ninth International Conference on Power Electronics and Drive Systems, Singapore, 5–8 December 2011; pp. 208–213. [Google Scholar]
- Bendito, E.; Carmona, Á.; Encinas, A.M.; Jiménez, M.J. The Extremal Charges Method in Grounding Grid Design. IEEE Trans. Power Deliv. 2004, 19, 118–123. [Google Scholar] [CrossRef]
- Li, W.; Pan, Z.; Lu, H.; Chen, X.; Zhang, L.; Wen, X. Influence of deep earth resistivity on HVDC ground-return currents distribution. IEEE Trans. Power Deliv. 2017, 32, 1844–1851. [Google Scholar] [CrossRef]
- Ma, C.; Zhao, S.; Sun, L.; Wang, L.; Zhang, G. The Simulation Study of DC Grounding Electrode Based on CDEGS and ANSYS. J. Phys. Conf. Ser. 2018, 1072, 012006. [Google Scholar] [CrossRef]
- Zhang, B.; Zeng, R.; He, J.; Zhao, J.; Li, X.; Wang, Q.; Cui, X. Numerical analysis of potential distribution between ground electrodes of HVDC system considering the effect of deep earth layers. IET Gener. Transm. Distrib. 2008, 2, 185–191. [Google Scholar] [CrossRef]
- Dan, Y.; Zhang, Z.; Zhao, H.; Li, Y.; Ye, H.; Deng, J. A novel segmented sampling numerical calculation method for grounding parameters in horizontally multilayered soil. Int. J. Electr. Power Energy Syst. 2021, 126, 106586. [Google Scholar] [CrossRef]
- Peiyu, H.; Peng, L.; Qingjun, P.; Min, C.; Hu, Y.; Bo, L. Calculation model for the earth potential of HVDC ground electrode based on image recognition of surface-layer soil moisture. Meas. Control 2020, 53, 1682–1693. [Google Scholar] [CrossRef]
- Sengar, K.P.; Chandrasekaran, K. Effects of cost optimised grid configuration on earthing system performance: A comparative assessment. IET Sci. Meas. Technol. 2020, 14, 610–620. [Google Scholar] [CrossRef]
- Zalhaf, A.S.; Ahmed, M.; Ookawara, S.; Abdel-Salam, M. A Simplified Model of Wind Turbine for Lightning Transient Analysis as Influenced by Structure of Grounding System. In Proceedings of the 2018 5th International Conference on Electric Power and Energy Conversion Systems (EPECS), Kitakyushu, Japan, 23–25 April 2018; pp. 1–6. [Google Scholar]
- Eaton, J.R. Electric Power Transmission Systems; Prentice-Hall: Lansing, MI, USA, 1972; ISBN 9780132473040. [Google Scholar]
- El-Hawary, H.H.; Abdel-Salam, M.; Hashem, A.A.R.; Turky, A.H.A. Inception Voltage of Burst Pulses, Onset Streamers, and Positive Glow in Short Rod-to-Plane Gaps. IEEE Trans. Plasma Sci. 2021, 49, 1763–1775. [Google Scholar] [CrossRef]
- Burden, R.L.; Faires, J.D. Numerical Analysis, 9th ed.; Brooks/Cole, Cengage Learning: Boston, MA, USA, 2011; ISBN 9780538733519. [Google Scholar]
- Bouderballa, A.; Zegnini, B.; Seghier, T. Determination of the resistance of a grounding system with application of finite element method. Prz. Elektrotechniczny 2021, 97, 5–11. [Google Scholar]
- Rüdenberg, R. Grounding principles and practice I—Fundamental considerations on ground currents. Electr. Eng. 1945, 64, 1–13. [Google Scholar] [CrossRef]
- Dwight, H.B. Calculation of Resistances to Ground. Trans. Am. Inst. Electr. Eng. 1936, 55, 1319–1328. [Google Scholar] [CrossRef]
- Liew, A.C.; Darveniza, M. Dynamic model of impulse characteristics of concentrated earths. Proc. Inst. Electr. Eng. 1974, 121, 123. [Google Scholar] [CrossRef]
- Dafalla, M.A.; AlFouzan, F.A. Influence of physical parameters and soil chemical composition on electrical resistivity: A guide for geotechnical soil profiles. Int. J. Electrochem. Sci. 2012, 7, 3191–3204. [Google Scholar] [CrossRef]





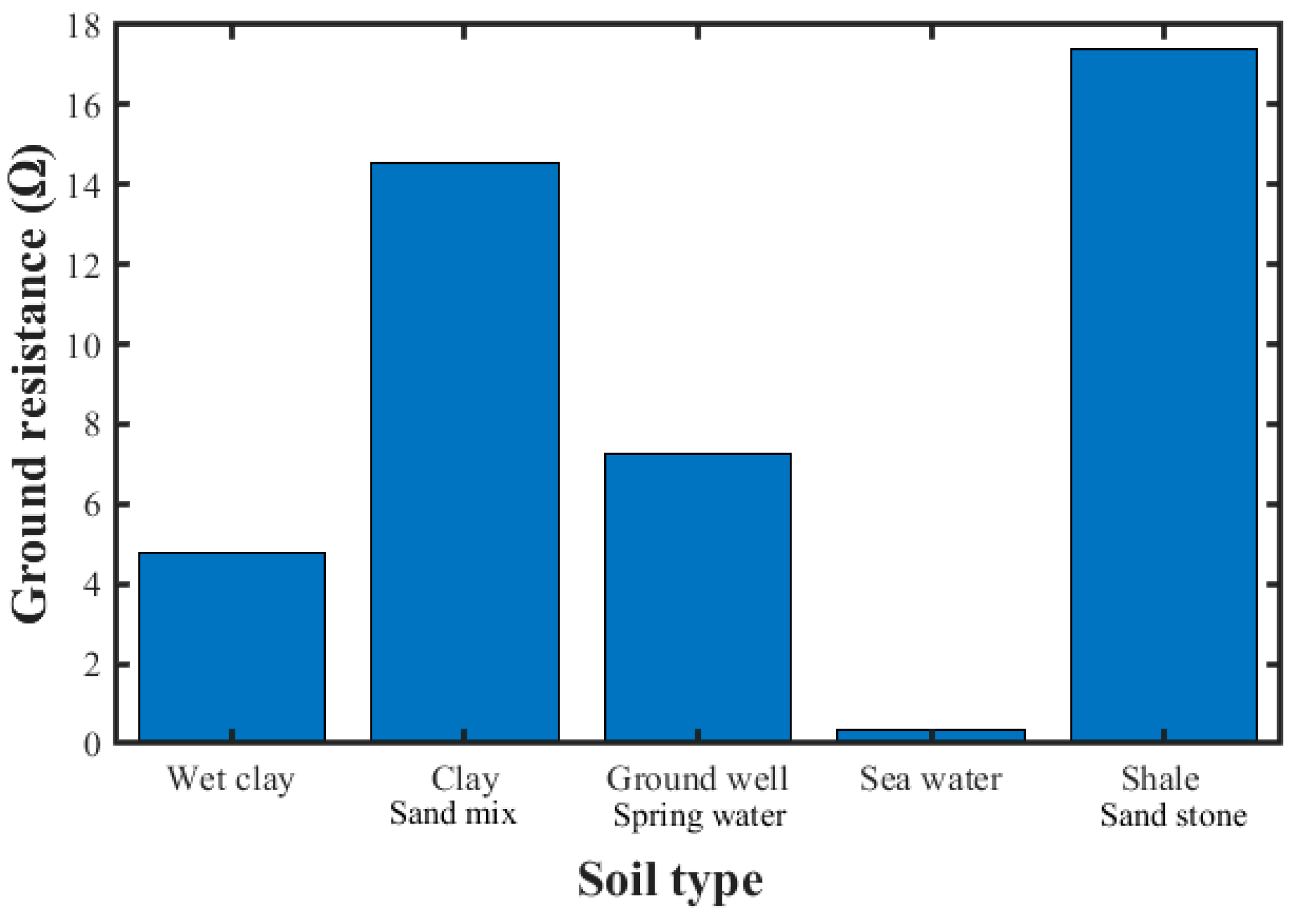
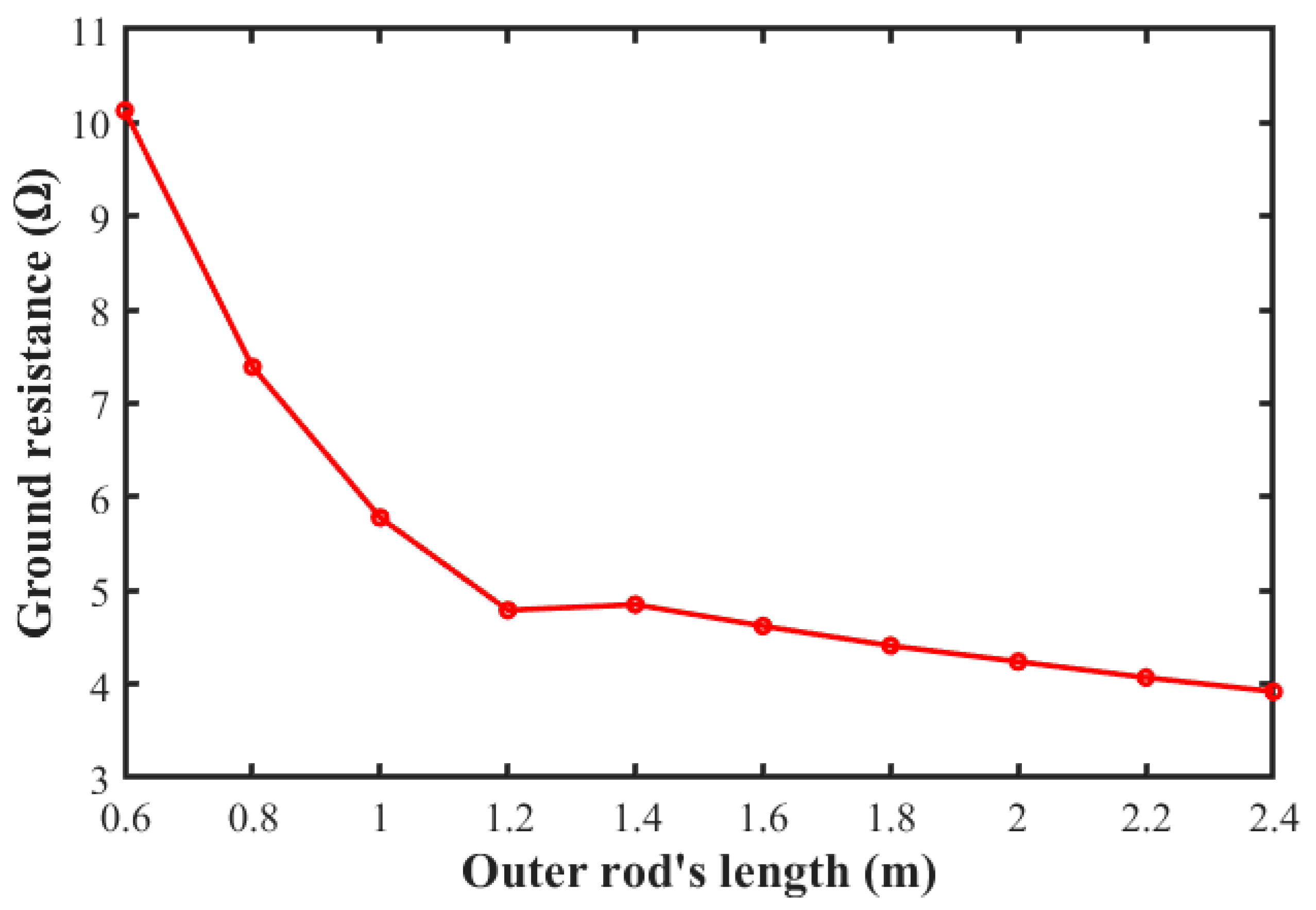

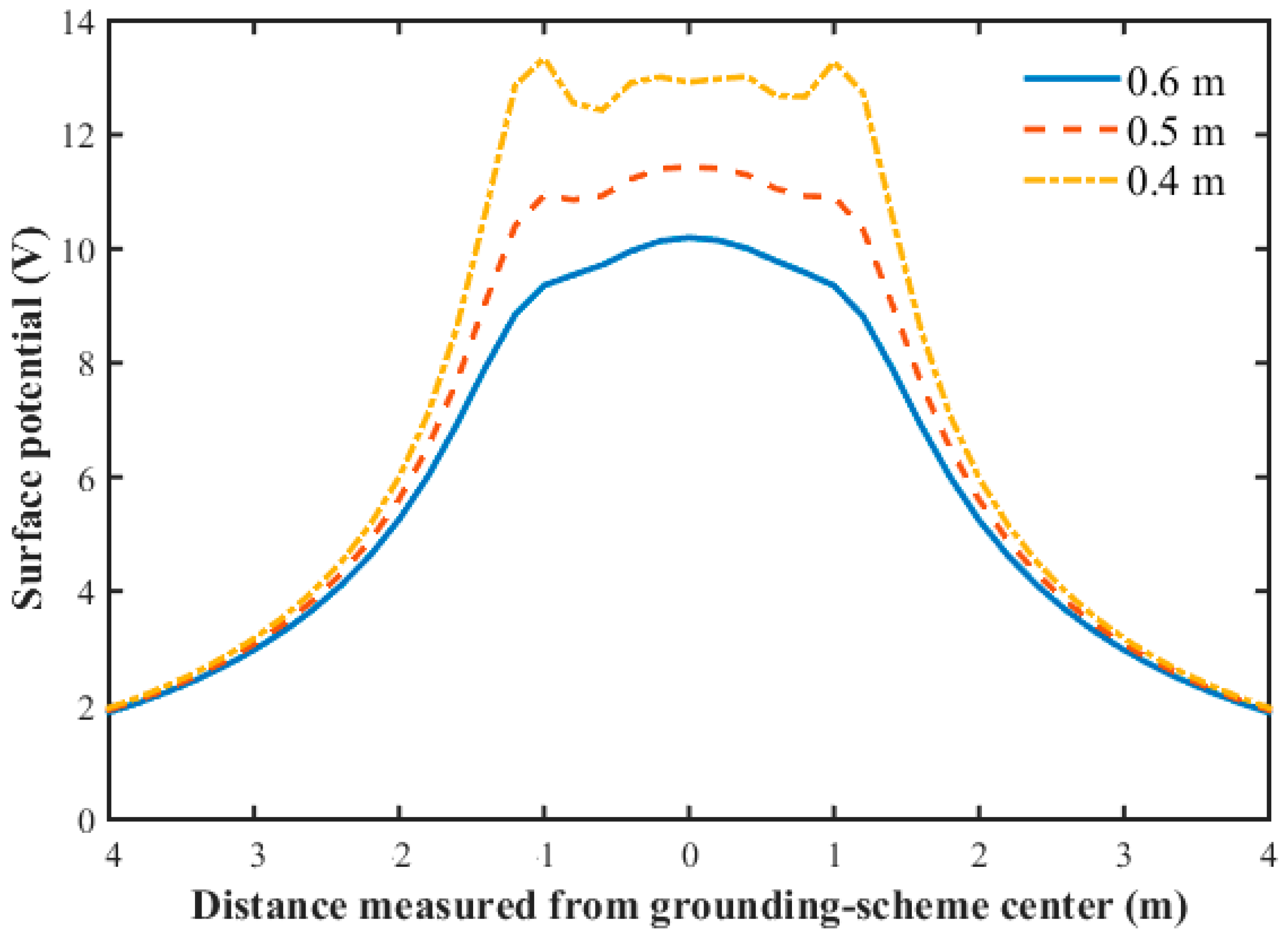
| Parameters of the Design | Value |
|---|---|
| Type of Soil | Usual Limits (Ω·m) | Selected Resistivity (Ω·m) |
|---|---|---|
| Sea water | 0.1–10 | |
| Wet clay | 8–70 | |
| Ground well and spring water | 10–150 | |
| Clay and sand mix. | 4–300 | |
| Shale, slates and sandstone | 10–1000 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akef, S.; El-Morshedy, A.M.K.; Samy, M.M.; Emam, A.M.; El-Hawary, H.H. Ground Resistance Estimation for a DC Converter Station: Theory versus Experiment. Energies 2024, 17, 765. https://doi.org/10.3390/en17040765
Akef S, El-Morshedy AMK, Samy MM, Emam AM, El-Hawary HH. Ground Resistance Estimation for a DC Converter Station: Theory versus Experiment. Energies. 2024; 17(4):765. https://doi.org/10.3390/en17040765
Chicago/Turabian StyleAkef, Samar, Ahdab M. K. El-Morshedy, Mohamed M. Samy, Ahmed M. Emam, and Hadeer H. El-Hawary. 2024. "Ground Resistance Estimation for a DC Converter Station: Theory versus Experiment" Energies 17, no. 4: 765. https://doi.org/10.3390/en17040765
APA StyleAkef, S., El-Morshedy, A. M. K., Samy, M. M., Emam, A. M., & El-Hawary, H. H. (2024). Ground Resistance Estimation for a DC Converter Station: Theory versus Experiment. Energies, 17(4), 765. https://doi.org/10.3390/en17040765








