Analysis of the Parity-Time Symmetry Model in the Receiver-Based Wireless Power Transfer
Abstract
:1. Introduction
2. Modeling of PT-Symmetric WPT System
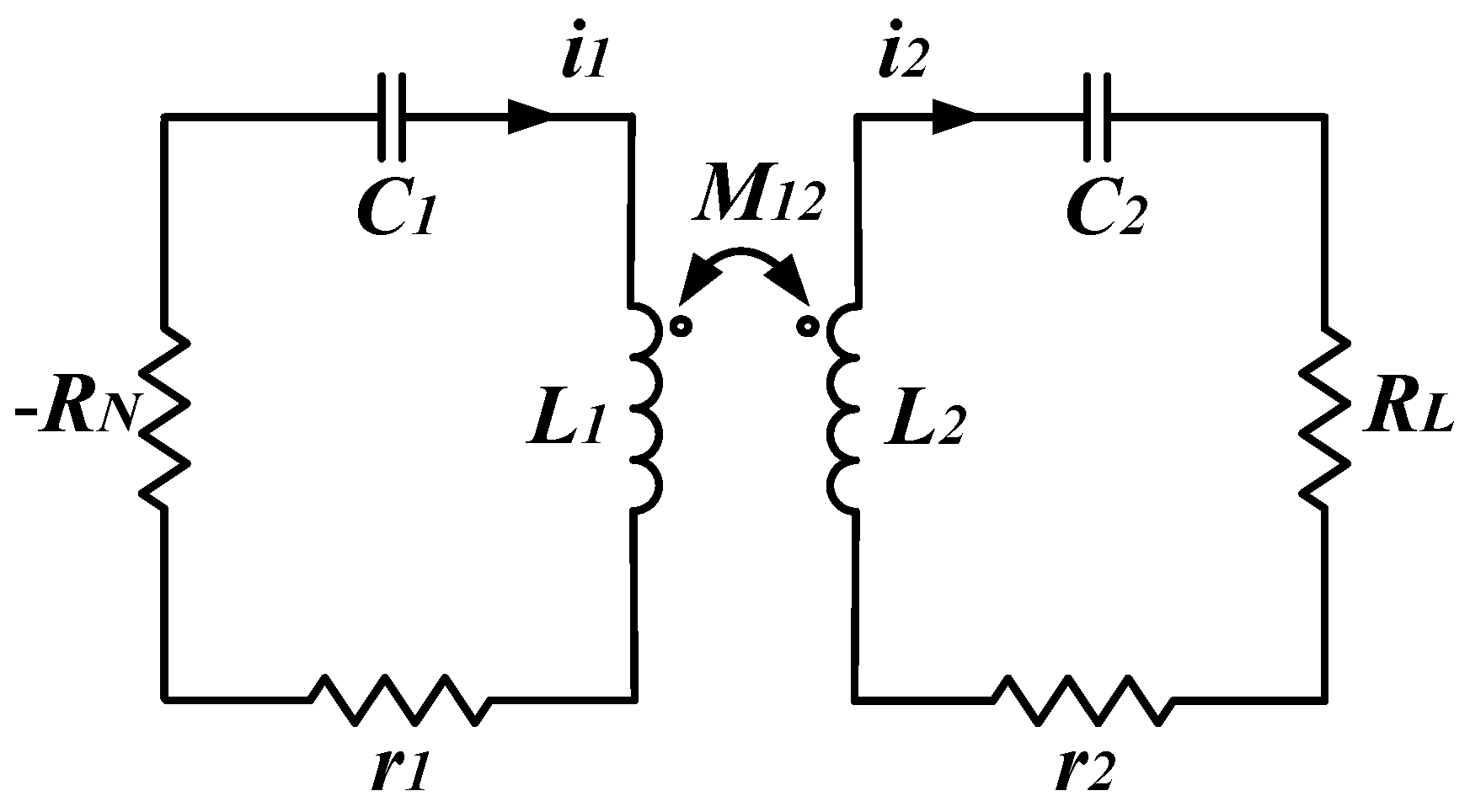
2.1. PT-Symmetric WPT Model
- 1.
- When ,
- 2.
- When ,
- 1.
- When
- 2.
- When
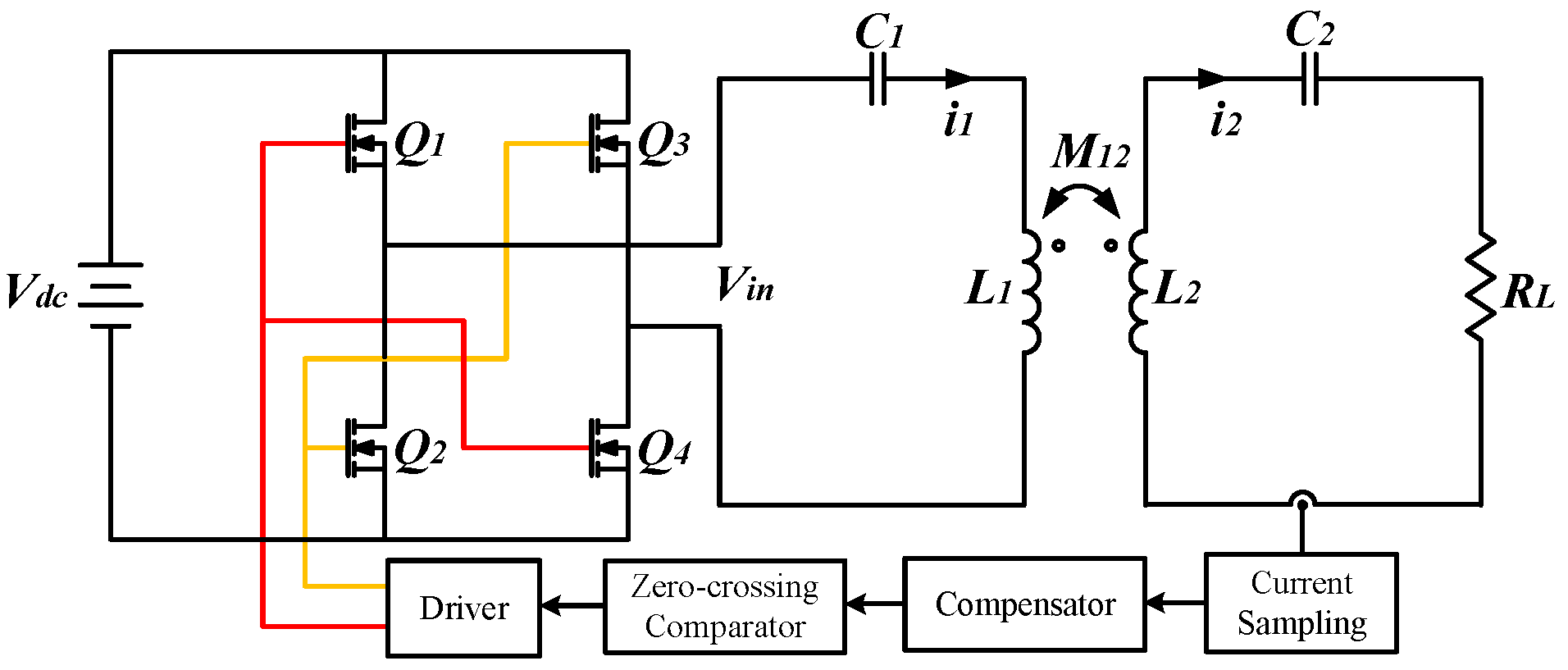
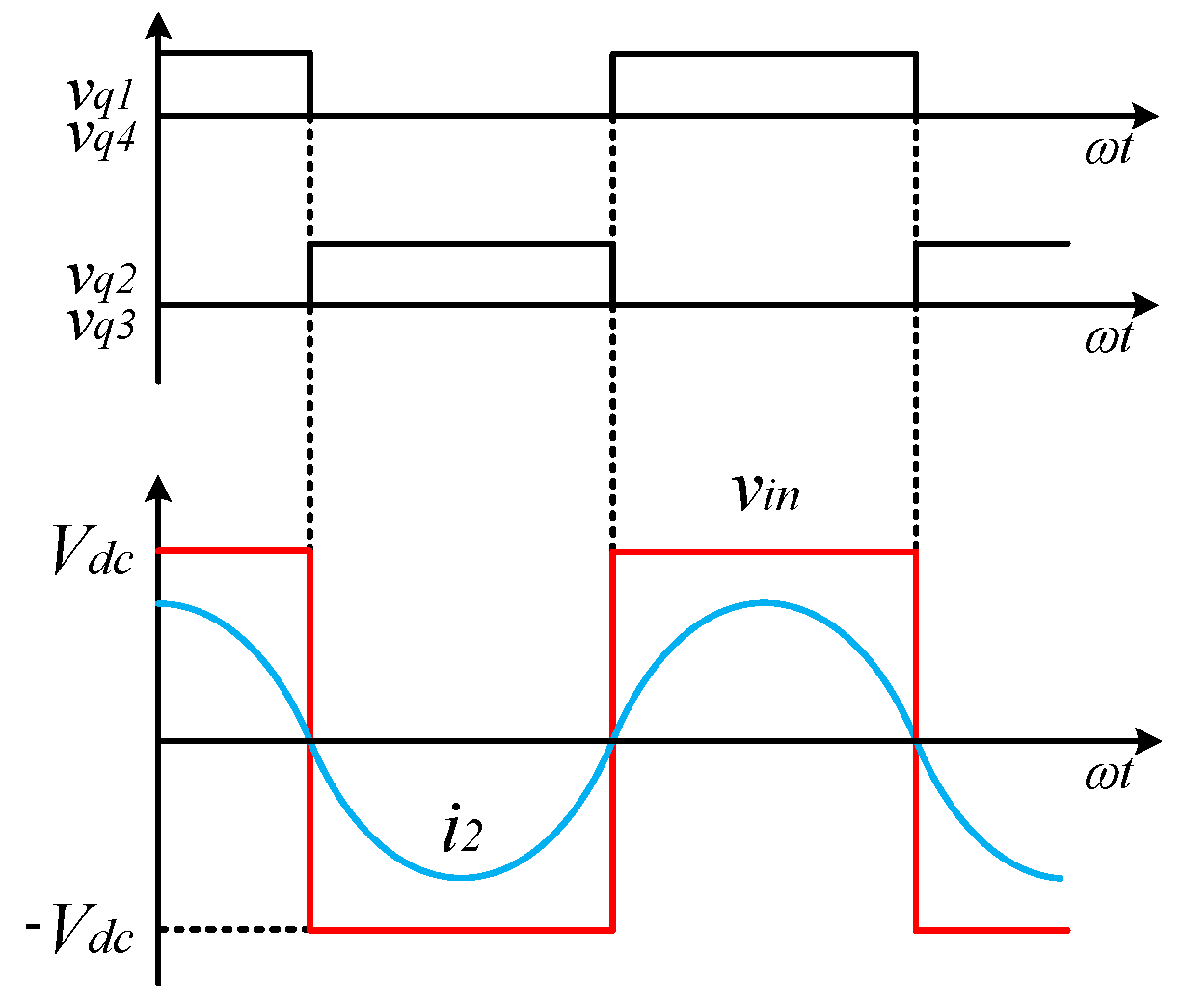
2.2. The Receiver-Based PT-Symmetric WPT Model
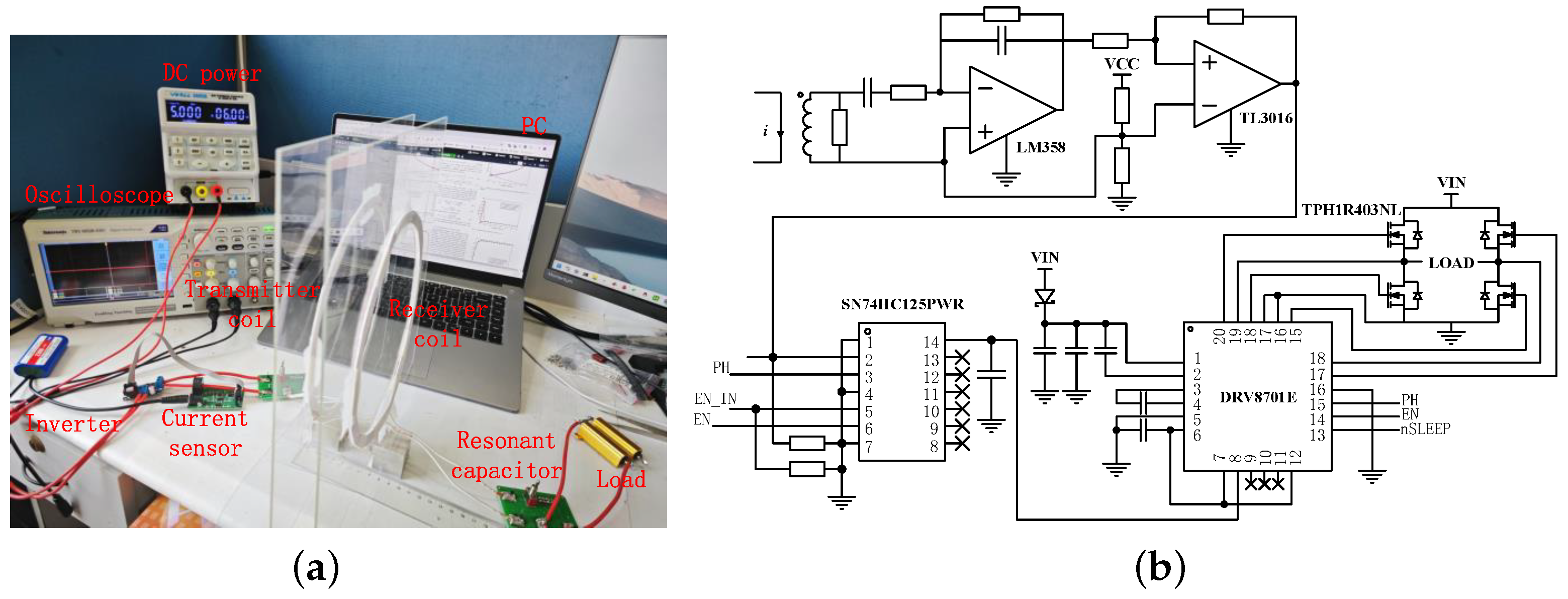
3. Experimental Verification
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lan, Y.; Jiang, J.; Yang, F.; Song, K.; Fan, F.; Sun, C.; Yang, G.; Zhang, B.; Lan, H. Research on Impedance Boundary and Tolerance Zone for Improving Wireless Charging Interoperability Evaluation of Electric Vehicles. IEEE Trans. Power Electron. 2024, 39, 12035–12040. [Google Scholar] [CrossRef]
- Pahlavan, S.; Shooshtari, M.; Jafarabadi Ashtiani, S. Star-Shaped Coils in the Transmitter Array for Receiver Rotation Tolerance in Free-Moving Wireless Power Transfer Applications. Energies 2022, 15, 8643. [Google Scholar] [CrossRef]
- Rüter, C.E.; Makris, K.G.; El-Ganainy, R.; Christodoulides, D.N.; Segev, M.; Kip, D. Observation of Parity–Time Symmetry in Optics. Nat. Phys. 2010, 6, 192–195. [Google Scholar] [CrossRef]
- Bender, C.M.; Boettcher, S. Real Spectra in Non-Hermitian Hamiltonians Having PT Symmetry. Phys. Rev. Lett. 1998, 80, 5243–5246. [Google Scholar] [CrossRef]
- El-Ganainy, R.; Makris, K.G.; Khajavikhan, M.; Musslimani, Z.H.; Rotter, S.; Christodoulides, D.N. Non-Hermitian Physics and PT Symmetry. Nat. Phys. 2018, 14, 11–19. [Google Scholar] [CrossRef]
- Krasnok, A.; Baranov, D.G.; Generalov, A.; Li, S.; Alù, A. Coherently Enhanced Wireless Power Transfer. Phys. Rev. Lett. 2018, 120, 143901. [Google Scholar] [CrossRef]
- Zhang, H.; Zhu, K.; Guo, Z.; Chen, Y.; Sun, Y.; Jiang, J.; Li, Y.; Yu, Z.; Chen, H. Robustness of Wireless Power Transfer Systems with Parity-Time Symmetry and Asymmetry. Energies 2023, 16, 4605. [Google Scholar] [CrossRef]
- Xie, Y.; Zhang, Z.; Lin, Y.; Feng, T.; Xu, Y. Magnetic Quasi-Bound State in the Continuum for Wireless Power Transfer. Phys. Rev. Appl. 2021, 15, 044024. [Google Scholar] [CrossRef]
- Zeng, C.; Guo, Z.; Zhu, K.; Fan, C.; Li, G.; Jiang, J.; Li, Y.; Jiang, H.; Yang, Y.; Sun, Y.; et al. Efficient and Stable Wireless Power Transfer Based on the Non-Hermitian Physics. Chin. Phys. B 2022, 31, 010307. [Google Scholar] [CrossRef]
- Assawaworrarit, S.; Yu, X.; Fan, S. Robust Wireless Power Transfer Using a Nonlinear Parity–Time-Symmetric Circuit. Nature 2017, 546, 387–390. [Google Scholar] [CrossRef]
- Ra’Di, Y.; Chowkwale, B.; Valagiannopoulos, C.; Liu, F.; Alu, A.; Simovski, C.R.; Tretyakov, S.A. On-Site Wireless Power Generation. IEEE Trans. Antennas Propag. 2018, 66, 4260–4268. [Google Scholar] [CrossRef]
- Schindler, J.; Lin, Z.; Lee, J.M.; Ramezani, H.; Ellis, F.M.; Kottos, T. PT-Symmetric Electronics. J. Phys. A Math. Theor. 2012, 45, 444029. [Google Scholar] [CrossRef]
- Zhou, J.; Zhang, B.; Xiao, W.; Qiu, D.; Chen, Y. Nonlinear Parity-Time-Symmetric Model for Constant Efficiency Wireless Power Transfer: Application to a Drone-in-Flight Wireless Charging Platform. IEEE Trans. Ind. Electron. 2019, 66, 4097–4107. [Google Scholar] [CrossRef]
- Assawaworrarit, S.; Fan, S. Robust and Efficient Wireless Power Transfer Using a Switch-Mode Implementation of a Nonlinear Parity–Time Symmetric Circuit. Nat. Electron. 2020, 3, 273–279. [Google Scholar] [CrossRef]
- Wei, Z.; Zhang, B. Transmission Range Extension of PT-Symmetry-Based Wireless Power Transfer System. IEEE Trans. Power Electron. 2021, 36, 11135–11147. [Google Scholar] [CrossRef]
- Zheng, W.; Xie, F.; Xiao, W.; Qiu, D.; Zhang, B. Plane-Omnidirectional Wireless Power Transfer System Based on Vector-Controlled Flux Linkage. IEEE Access 2021, 9, 105651–105666. [Google Scholar] [CrossRef]
- Gu, Y.; Chen, J.; Chang, S.; Zhang, Z. Constant Power Control Against M/R With Expanded PT-Symmetric Range for Wireless in-Flight Charging of Drones. IEEE Trans. Magn. 2023, 59, 1–6. [Google Scholar] [CrossRef]
- Qu, Y.; Zhang, B.; Gu, W.; Li, J.; Shu, X. Distance Extension of S-PS Wireless Power Transfer System Based on Parity-Time Symmetry. IEEE Trans. Circuits Syst. II 2023, 70, 2954–2958. [Google Scholar] [CrossRef]
- Zeng, C.; Sun, Y.; Li, G.; Li, Y.; Jiang, H.; Yang, Y.; Chen, H. High-Order Parity-Time Symmetric Model for Stable Three-Coil Wireless Power Transfer. Phys. Rev. Appl. 2020, 13, 034054. [Google Scholar] [CrossRef]
- Luo, C.; Qiu, D.; Lin, M.; Zhang, B. Circuit Model and Analysis of Multi-Load Wireless Power Transfer System Based on Parity-Time Symmetry. Energies 2020, 13, 3260. [Google Scholar] [CrossRef]
- Qu, Y.; Zhang, B.; Gu, W.; Shu, X. Wireless Power Transfer System With High-Order Compensation Network Based on Parity-Time-Symmetric Principle and Relay Coil. IEEE Trans. Power Electron. 2023, 38, 1314–1323. [Google Scholar] [CrossRef]
- Guo, Z.; Yang, F.; Zhang, H.; Wu, X.; Wu, Q.; Zhu, K.; Jiang, J.; Jiang, H.; Yang, Y.; Li, Y.; et al. Level Pinning of Anti-PT-Symmetric Circuits for Efficient Wireless Power Transfer. Natl. Sci. Rev. 2023, 11, nwad172. [Google Scholar] [CrossRef] [PubMed]
- Hao, X.; Yin, K.; Zou, J.; Wang, R.; Huang, Y.; Ma, X.; Dong, T. Frequency-Stable Robust Wireless Power Transfer Based on High-Order Pseudo-Hermitian Physics. Phys. Rev. Lett. 2023, 130, 077202. [Google Scholar] [CrossRef] [PubMed]
- Paul, D. Low-Voltage Power System Surge Overvoltage Protection. IEEE Trans. Ind. Appl. 2001, 37, 223–229. [Google Scholar] [CrossRef]
- Samaras, K.; Sandberg, C.; Saimas, C.; Koulaxouzidis, A. Electrical Surge Protection Devices for Industrial Facilities—A Tutorial Review. In Proceedings of the Record of Conference Papers Industry Applications Society 52nd Annual Petroleum and Chemical Industry Conference, Denver, CO, USA, 12–14 September 2005; pp. 165–175. [Google Scholar]
- Wang, C.S.; Covic, G.; Stielau, O. Power Transfer Capability and Bifurcation Phenomena of Loosely Coupled Inductive Power Transfer Systems. IEEE Trans. Ind. Electron. 2004, 51, 148–157. [Google Scholar] [CrossRef]
- Wu, J.; Li, K.; Zeng, J.; Hui, S.Y.R. On the Limitations of the Coupled Mode Theory and Parity-Time Symmetry for Near-Field Wireless Power Transfer Research. IEEE Trans. Power Electron. 2024, 39, 6433–6441. [Google Scholar] [CrossRef]
- Vu, V.-B.; Ramezani, A.; Triviño, A.; González-González, J.M.; Kadandani, N.B.; Dahidah, M.; Pickert, V.; Narimani, M.; Aguado, J. Operation of Inductive Charging Systems Under Misalignment Conditions: A Review for Electric Vehicles. IEEE Trans. Transp. Electrif. 2023, 9, 1857–1887. [Google Scholar] [CrossRef]
- Song, K.; Lan, Y.; Zhang, X.; Jiang, J.; Sun, C.; Yang, G.; Yang, F.; Lan, H. A Review on Interoperability of Wireless Charging Systems for Electric Vehicles. Energies 2023, 16, 1653. [Google Scholar] [CrossRef]

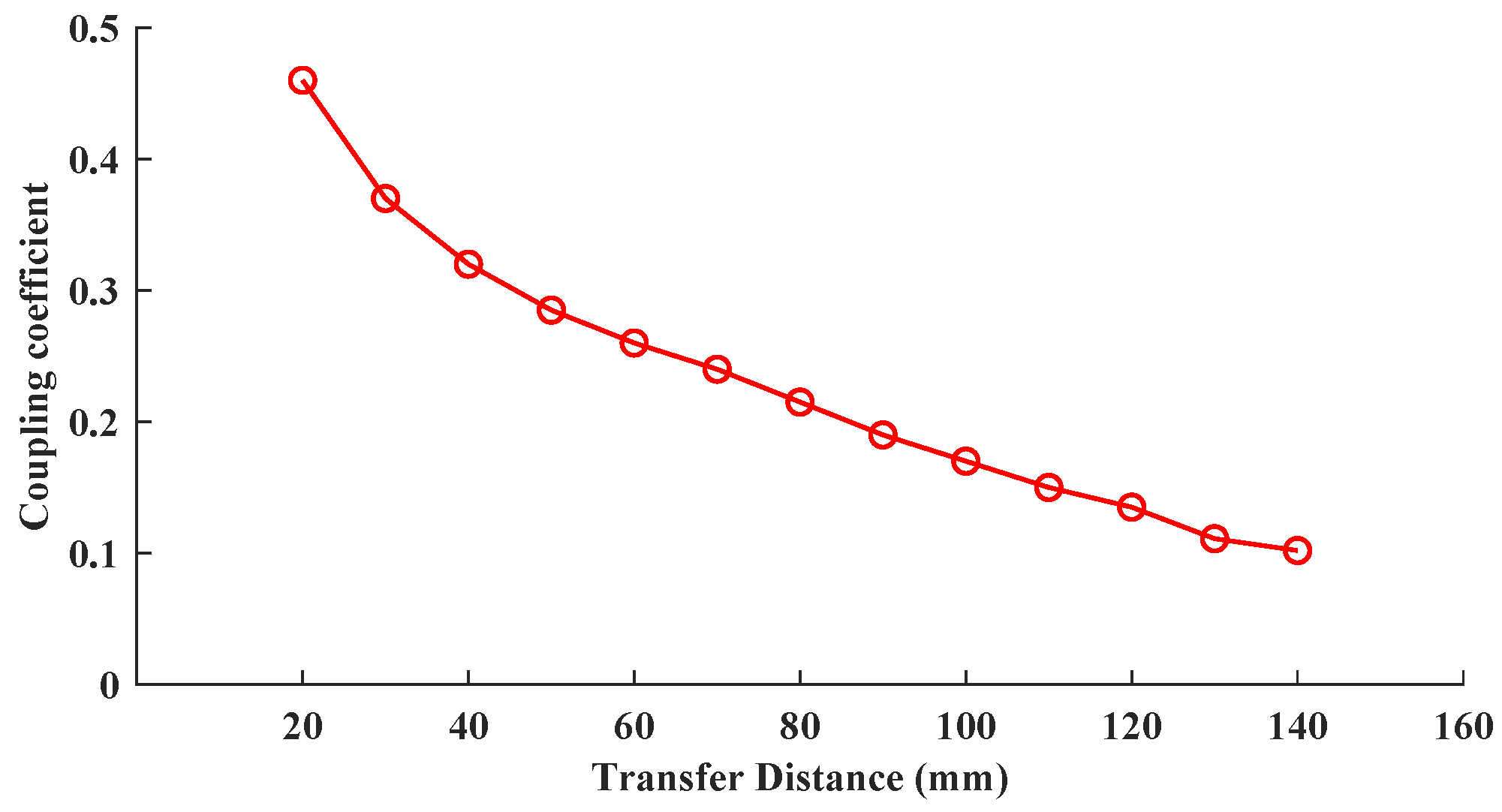
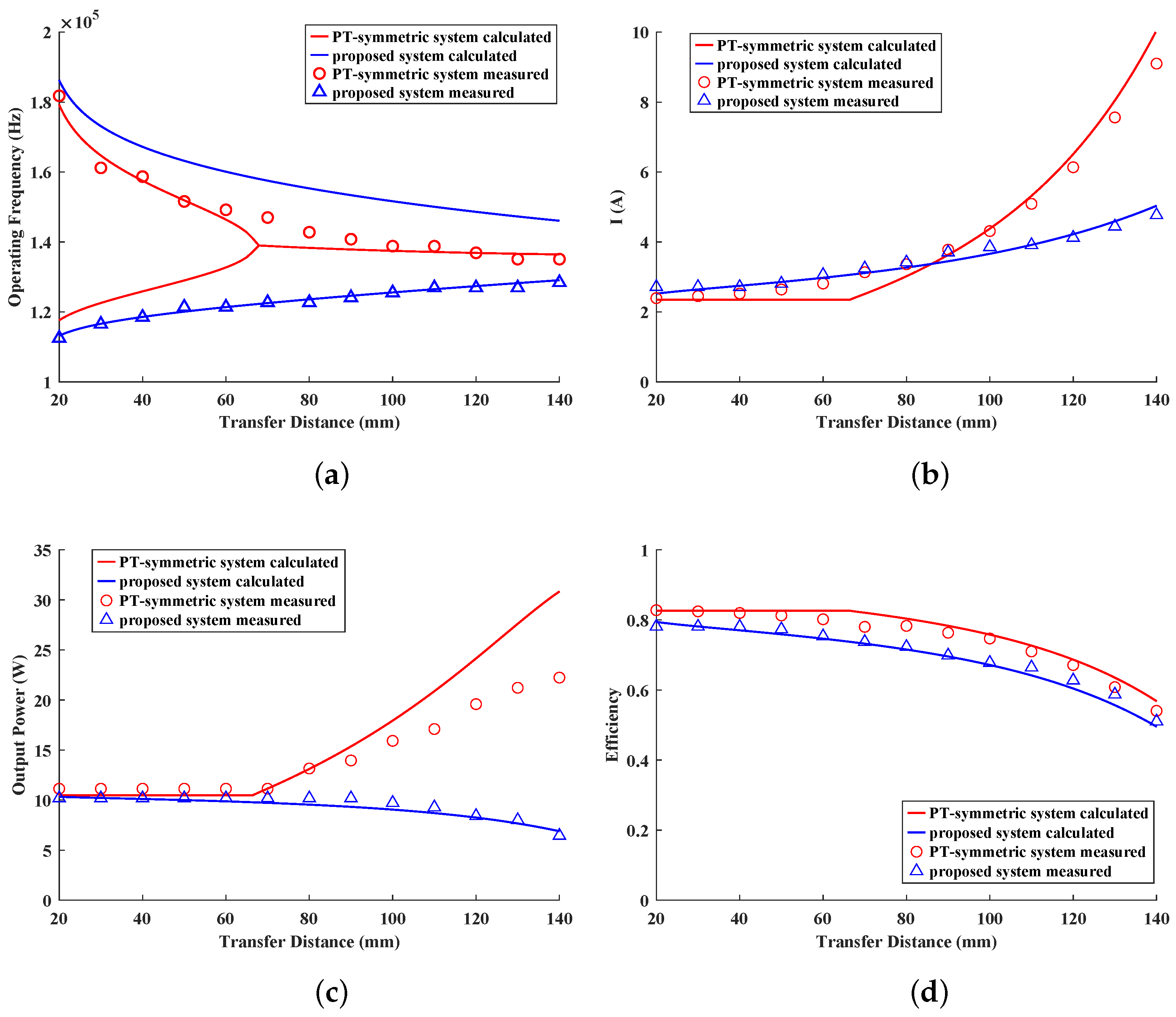
| Symbol | Note | Values |
|---|---|---|
| Transmitter inductance | 10.12 H | |
| Receiver inductance | 10.1 H | |
| Transmitter capacitance | 135.2 nF | |
| Receiver capacitance | 135.6 nF | |
| DC source voltage | 6 V | |
| Transmitter equivalent resistance | 221 m | |
| Receiver equivalent resistance | 213 m | |
| Equivalent load resistance | 2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, X.; Yan, W. Analysis of the Parity-Time Symmetry Model in the Receiver-Based Wireless Power Transfer. Energies 2024, 17, 6103. https://doi.org/10.3390/en17236103
Yan X, Yan W. Analysis of the Parity-Time Symmetry Model in the Receiver-Based Wireless Power Transfer. Energies. 2024; 17(23):6103. https://doi.org/10.3390/en17236103
Chicago/Turabian StyleYan, Xiaoxi, and Wen Yan. 2024. "Analysis of the Parity-Time Symmetry Model in the Receiver-Based Wireless Power Transfer" Energies 17, no. 23: 6103. https://doi.org/10.3390/en17236103
APA StyleYan, X., & Yan, W. (2024). Analysis of the Parity-Time Symmetry Model in the Receiver-Based Wireless Power Transfer. Energies, 17(23), 6103. https://doi.org/10.3390/en17236103





