Modeling and Numerical Investigations of Flowing N-Decane Partial Catalytic Steam Reforming at Supercritical Pressure
Abstract
1. Introduction
2. Experimental Principles and System
2.1. Experimental Principles
2.2. Experimental System
2.3. Catalyst Preparation
2.4. Uncertainty Analysis and Error Analysis
3. Numerical Model
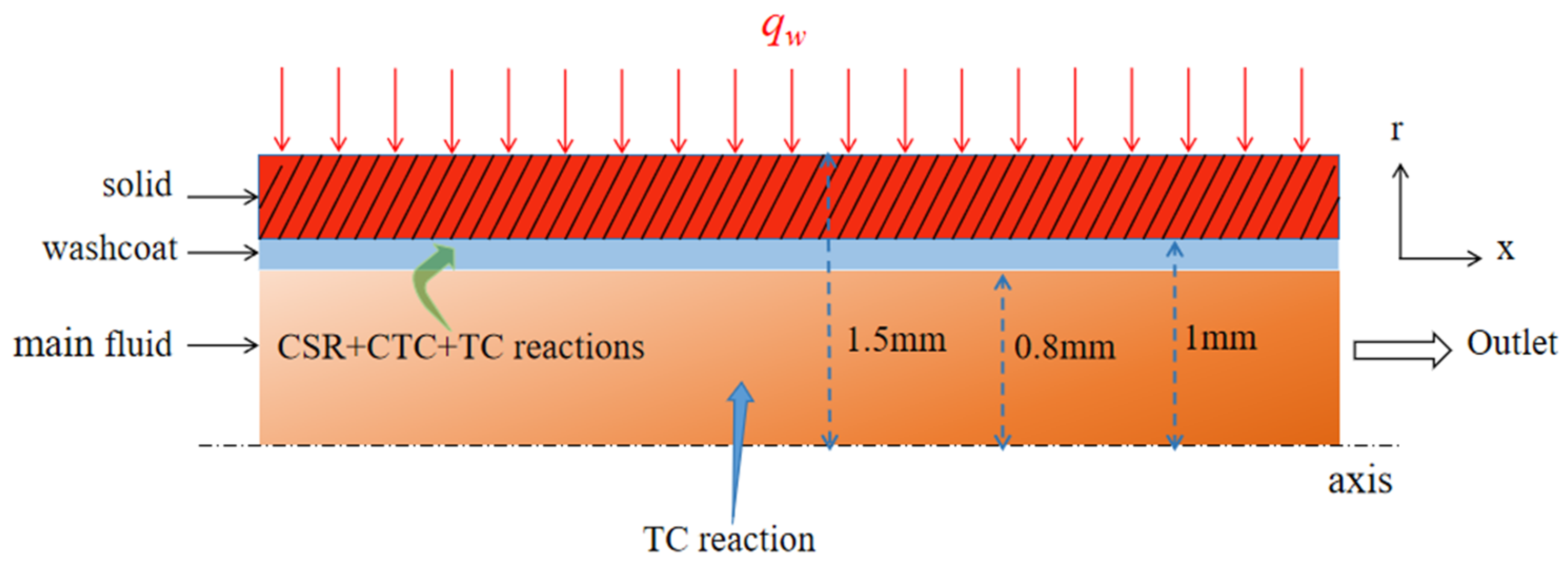
3.1. Governing Equations
3.2. Real Gas Properties
3.3. Computational Model and Solution Strategy
4. Chemical Reaction Model
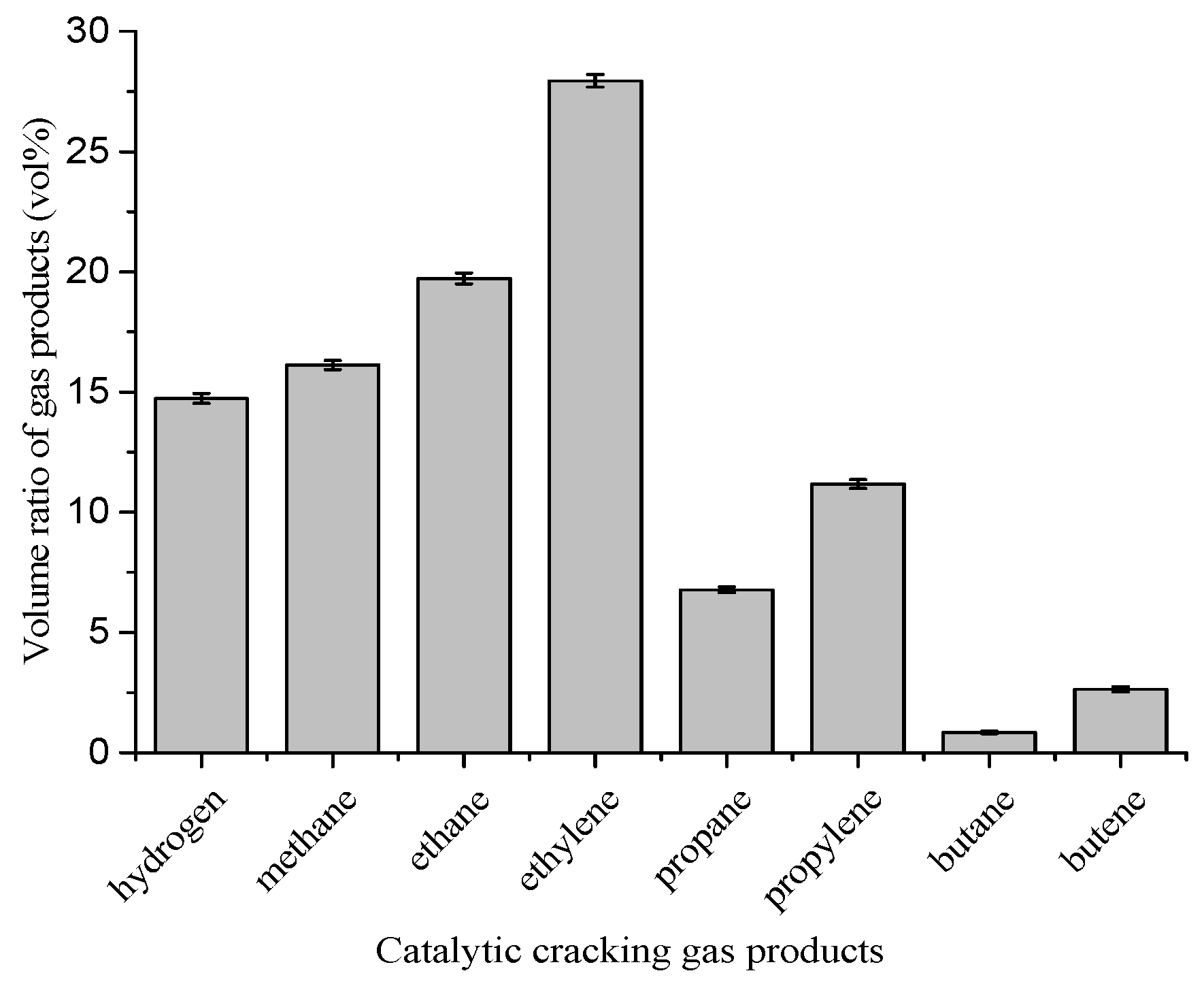
4.1. Product Distribution
0.122C5H12 + 0.211C5H10 + 0.077C6H14 + 0.318C6H12 + 0.072C7H16 + 0.274C7H14 + 0.0136C8H18 + 0.232C8H16 +
0.0042C9H20 + 0.0858C9H18
0.0048C4H10 + 0.2015C5H10 + 0.1167C5H12 + 0.3033C6H12 + 0.0735C6H14 + 0.2611C7H14 + 0.0684C7H16 +
0.2209C8H16 + 0.0130C8H18 + 0.0817C9H18 + 0.0040C9H20
4.2. PSO Algorithm Optimization Model
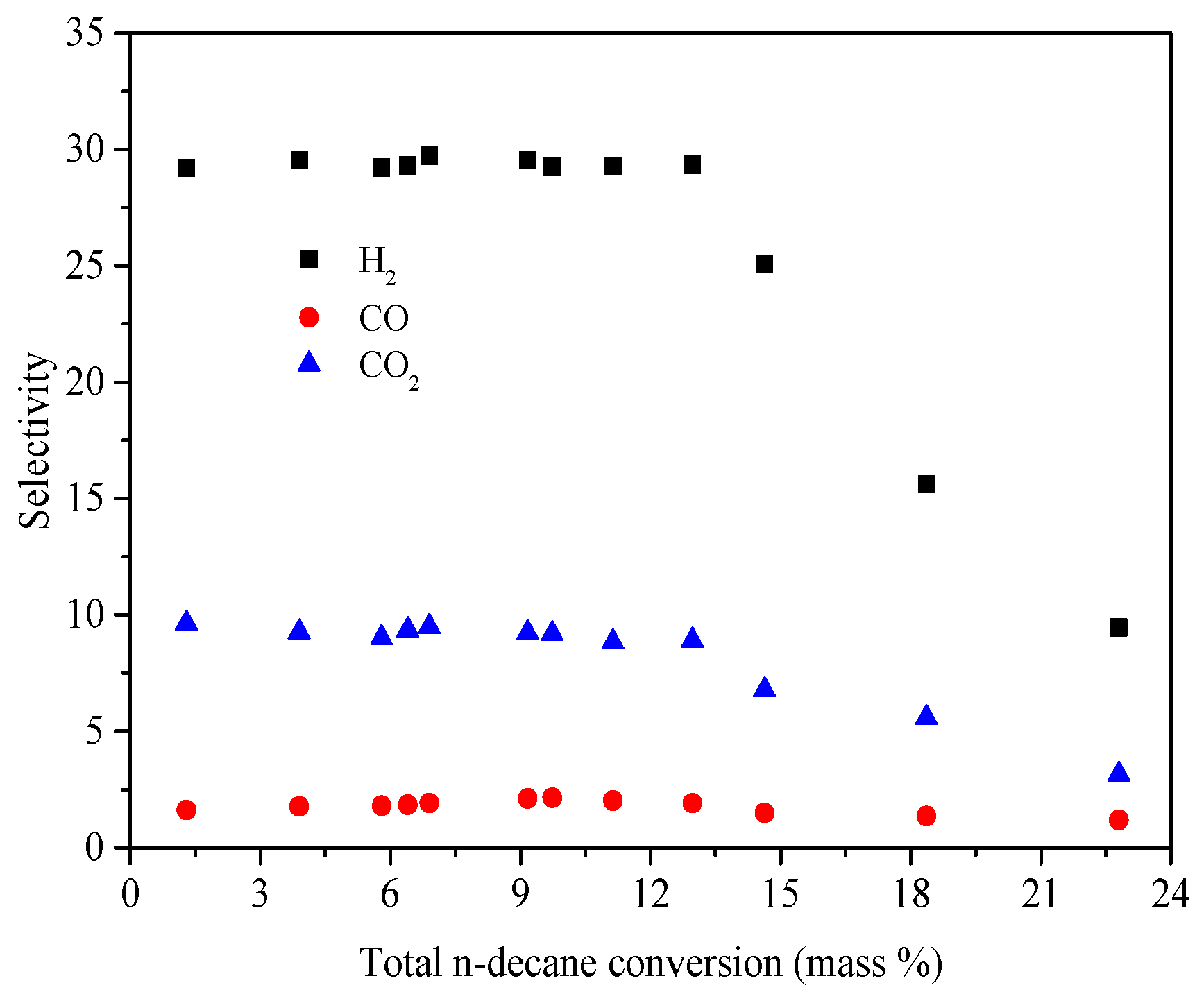
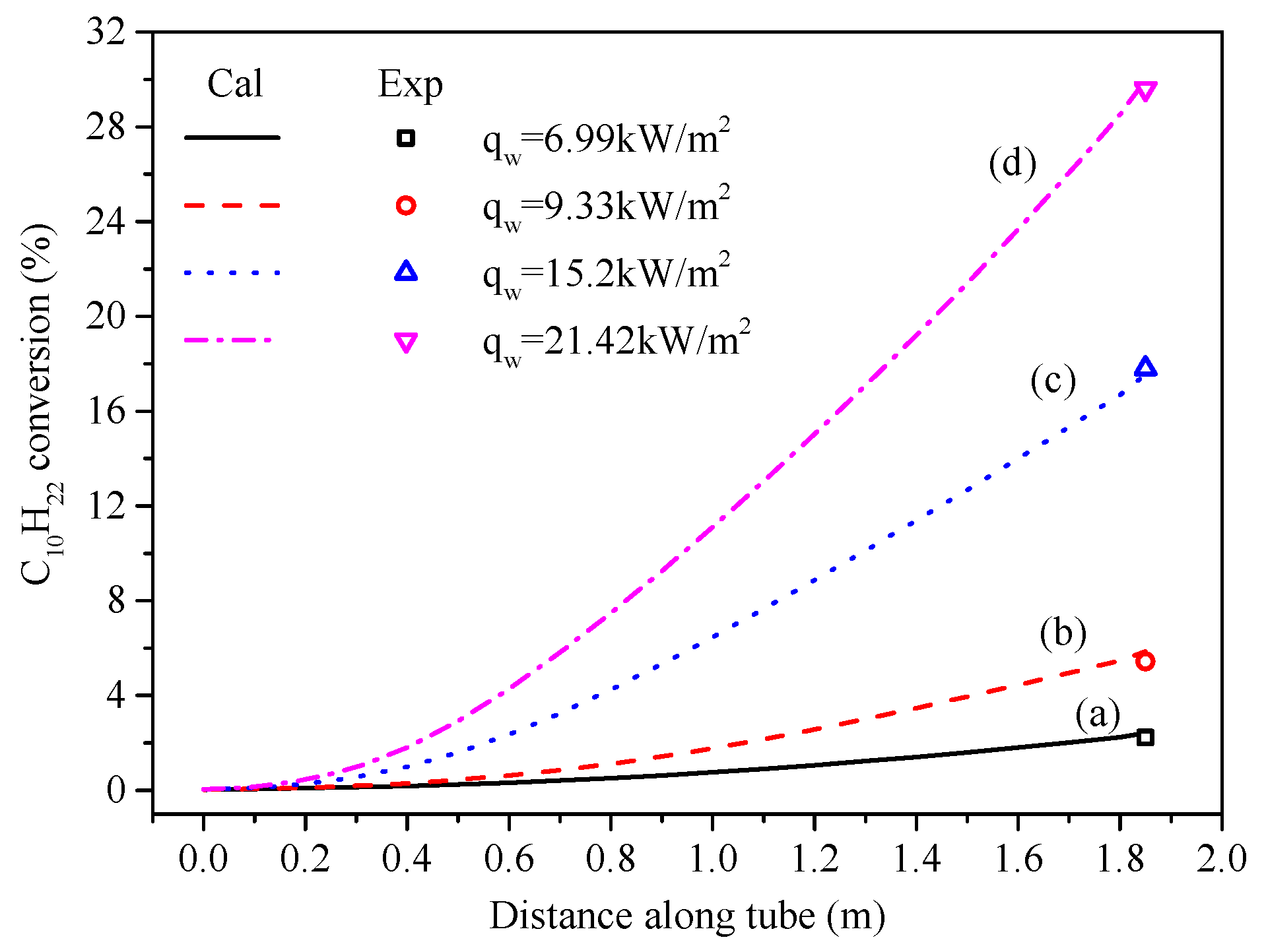
4.3. Validation of PSCR Reaction Models
5. Results and Discussion
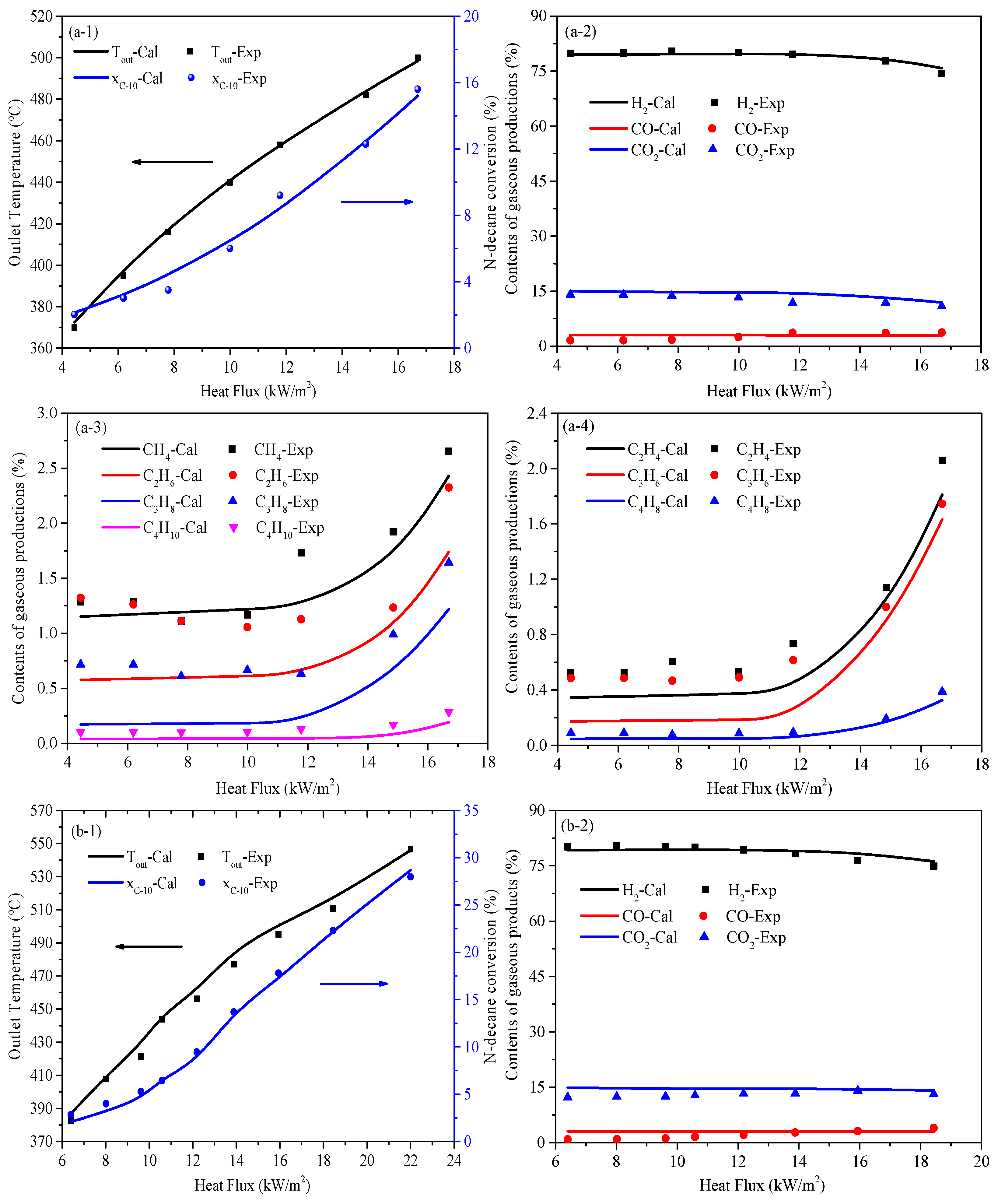
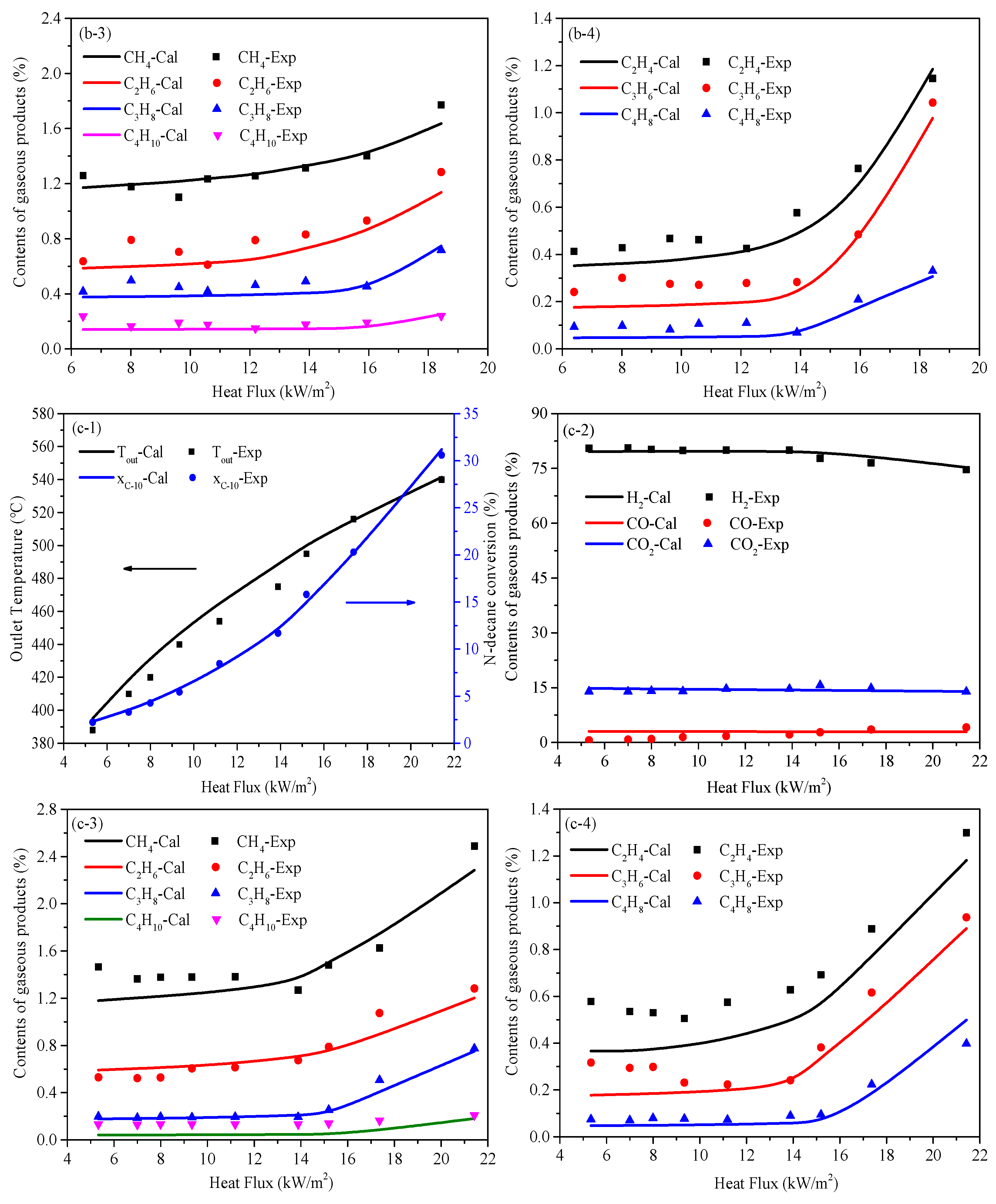
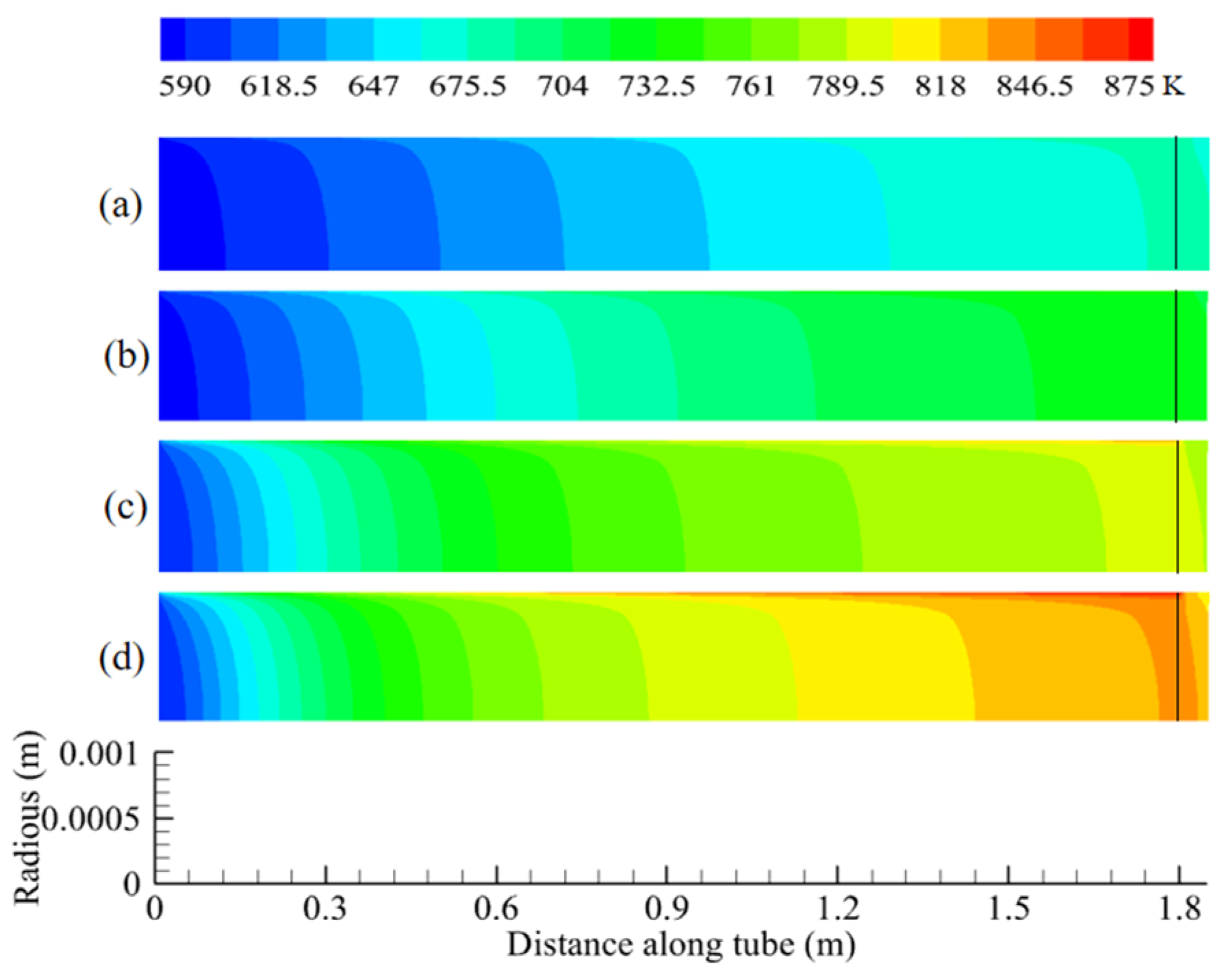
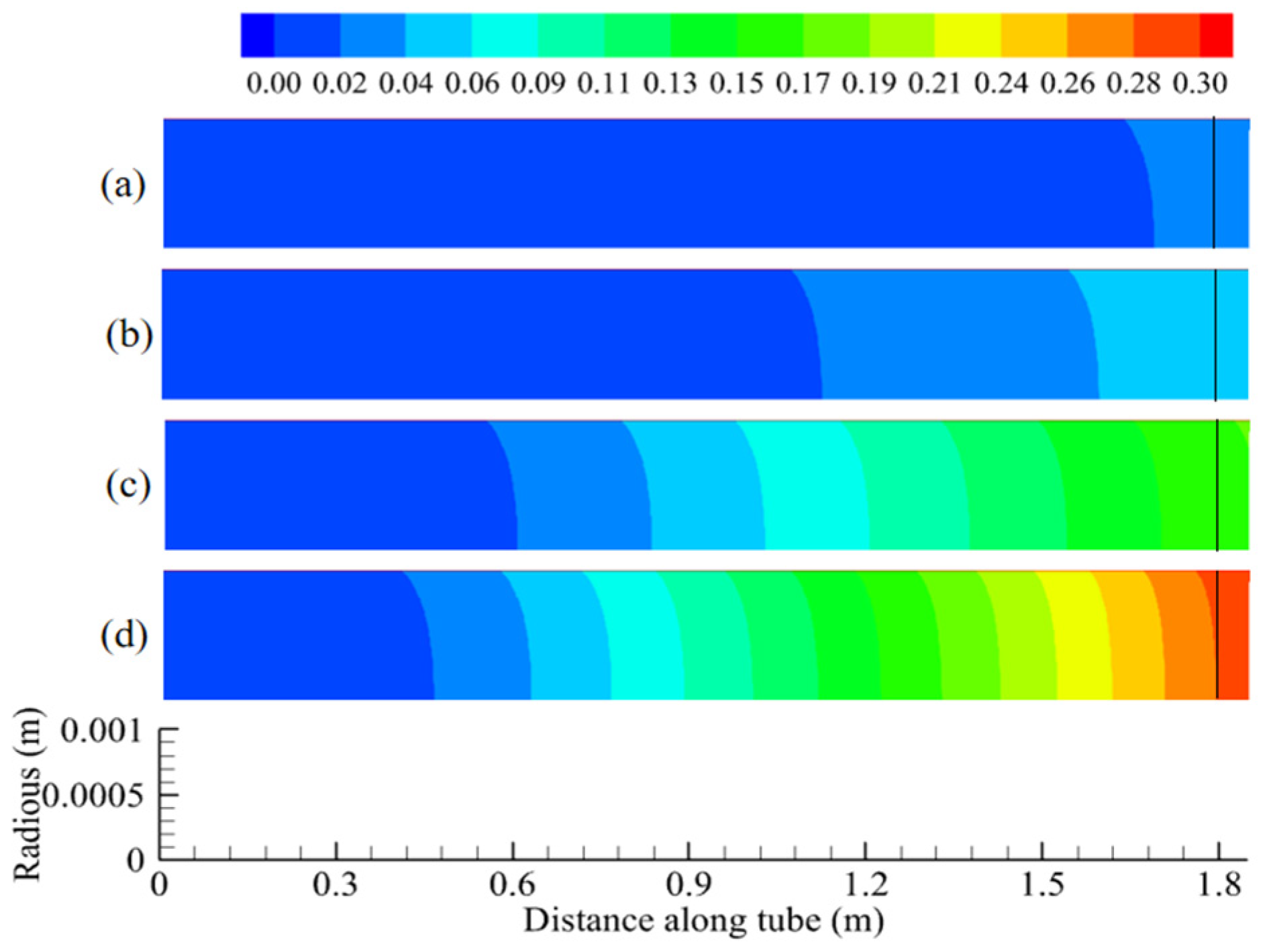
5.1. Temperature and Conversion of N-Decane Along Tube
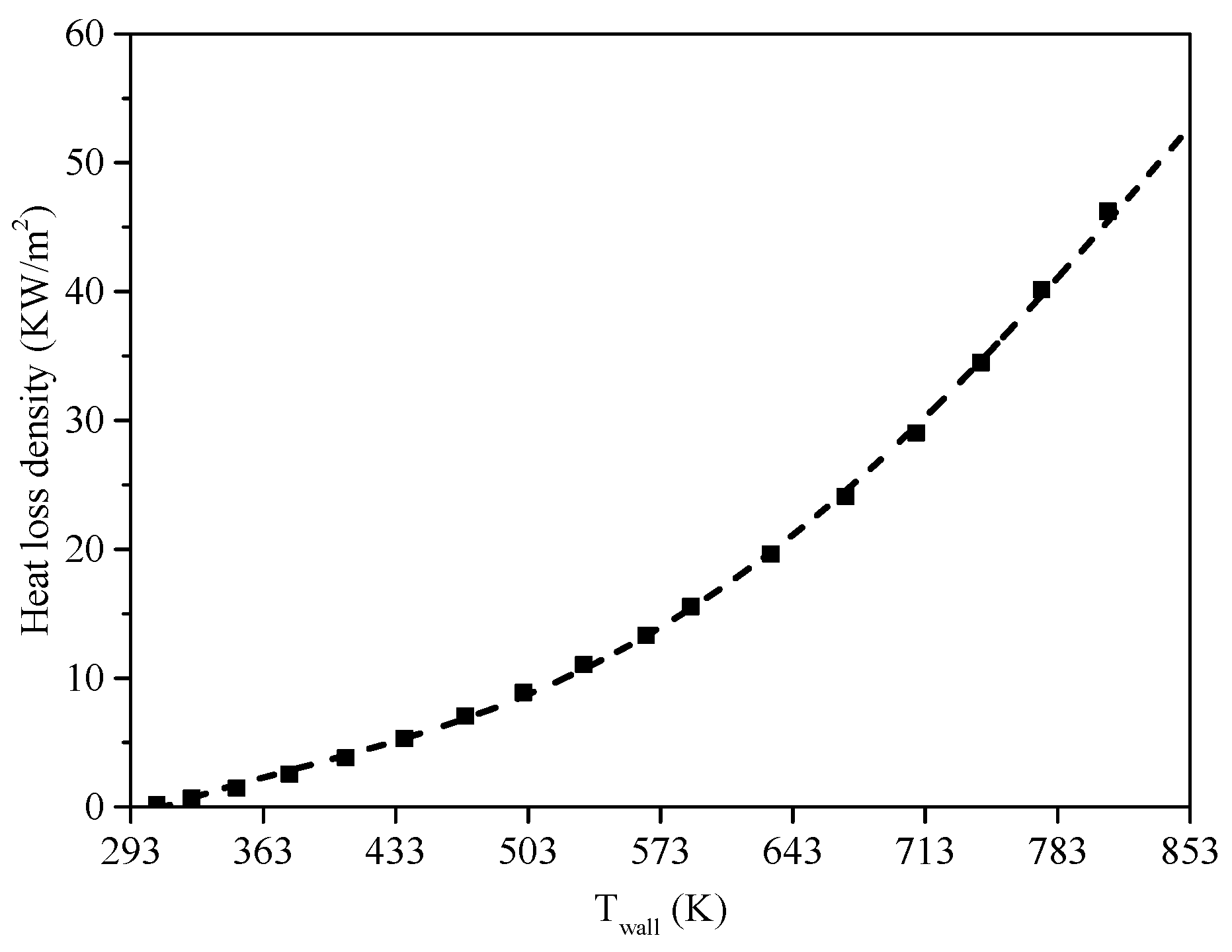
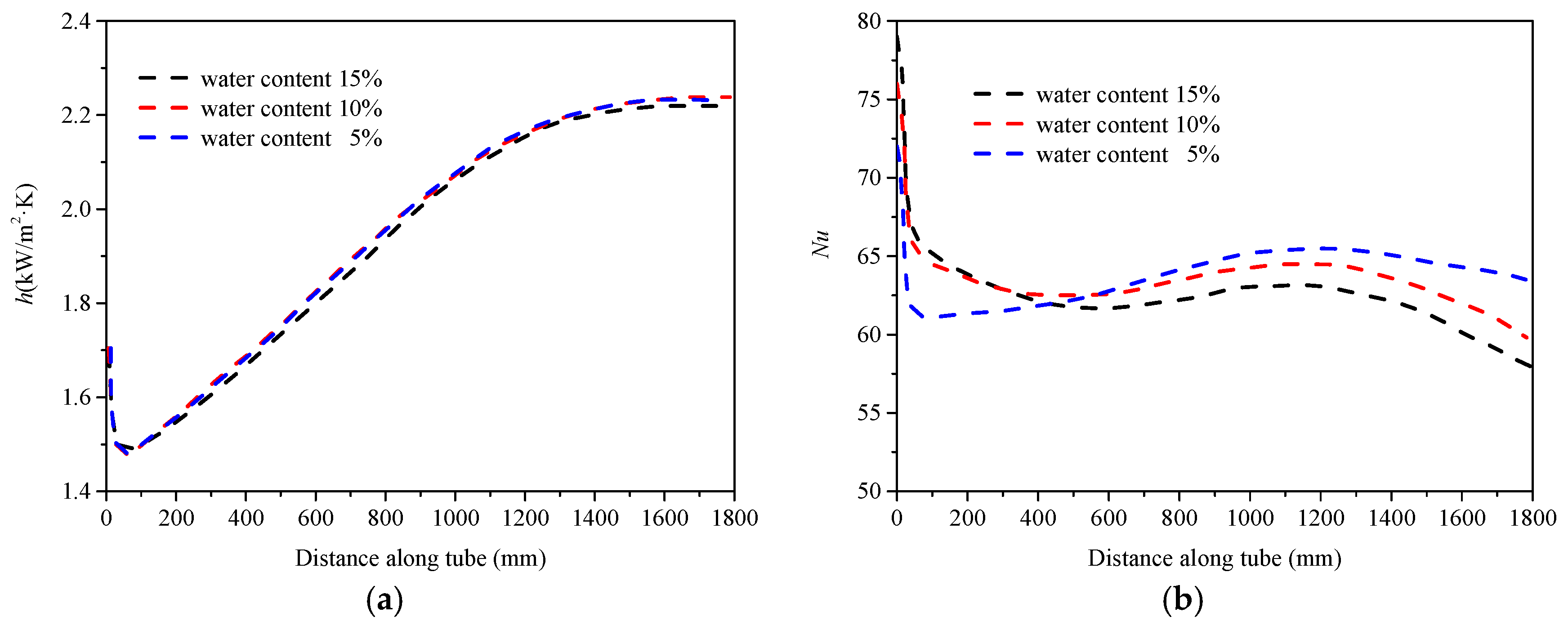
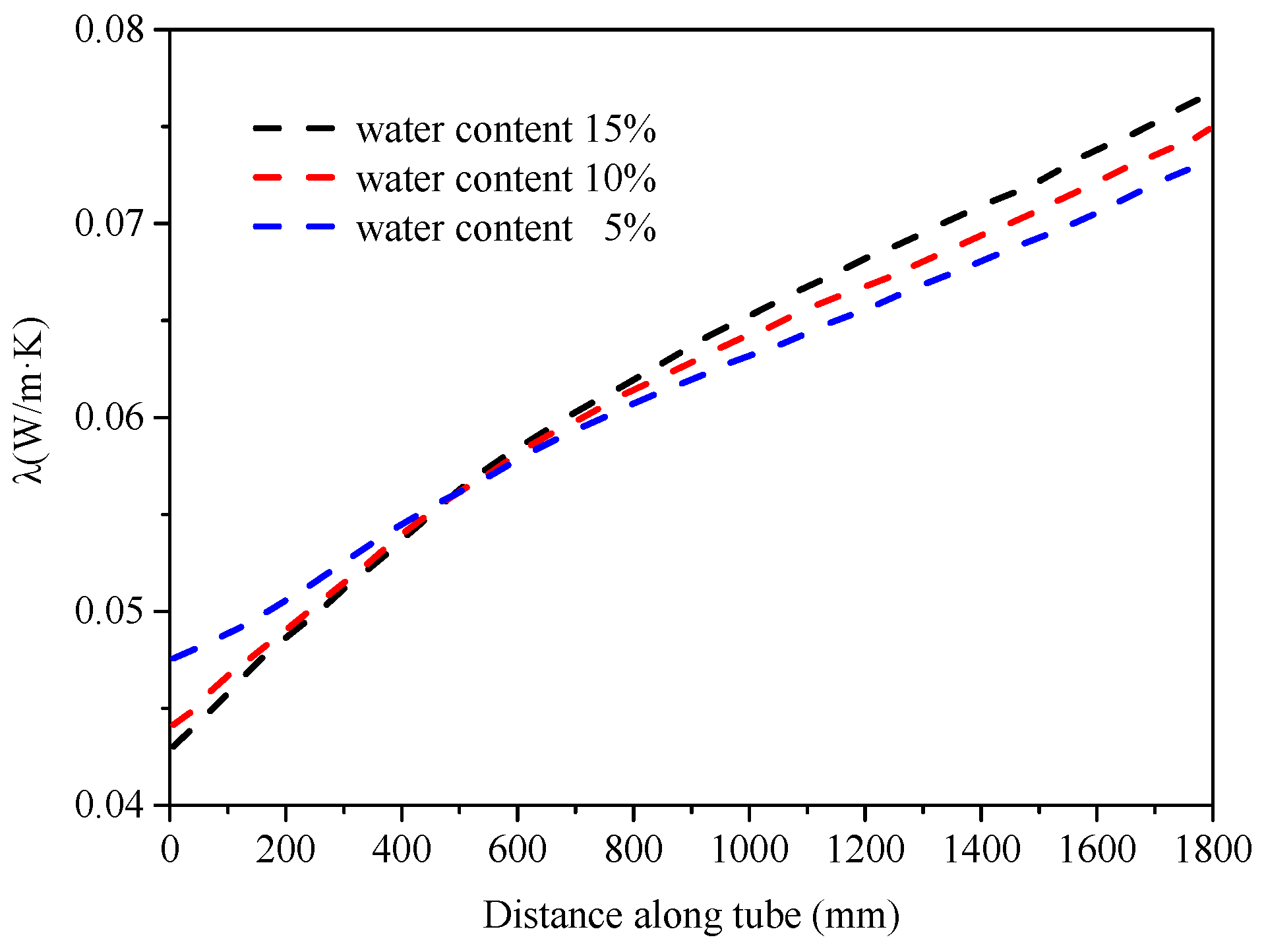
5.2. Heat Transfer Characteristics Along the Tube
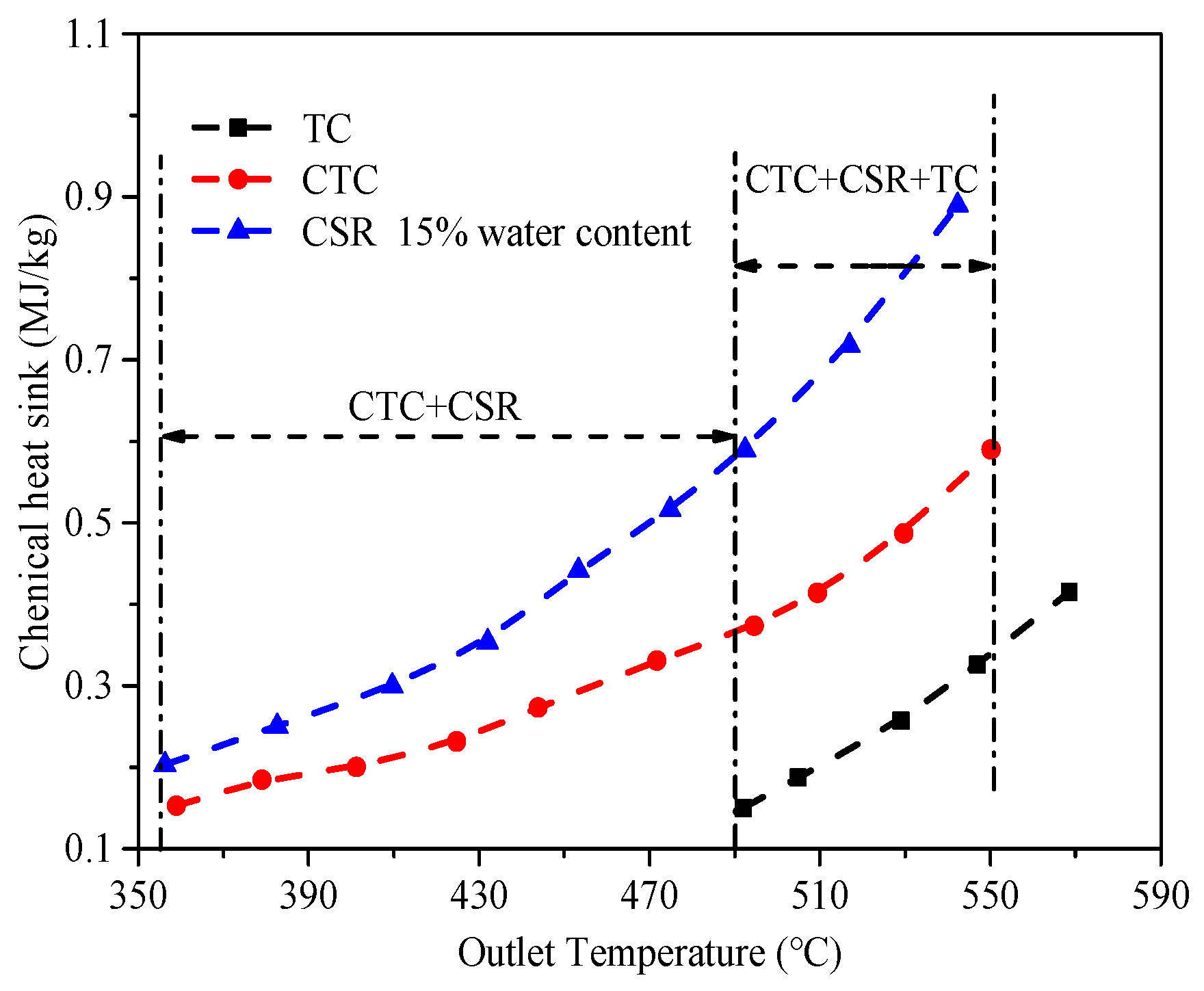
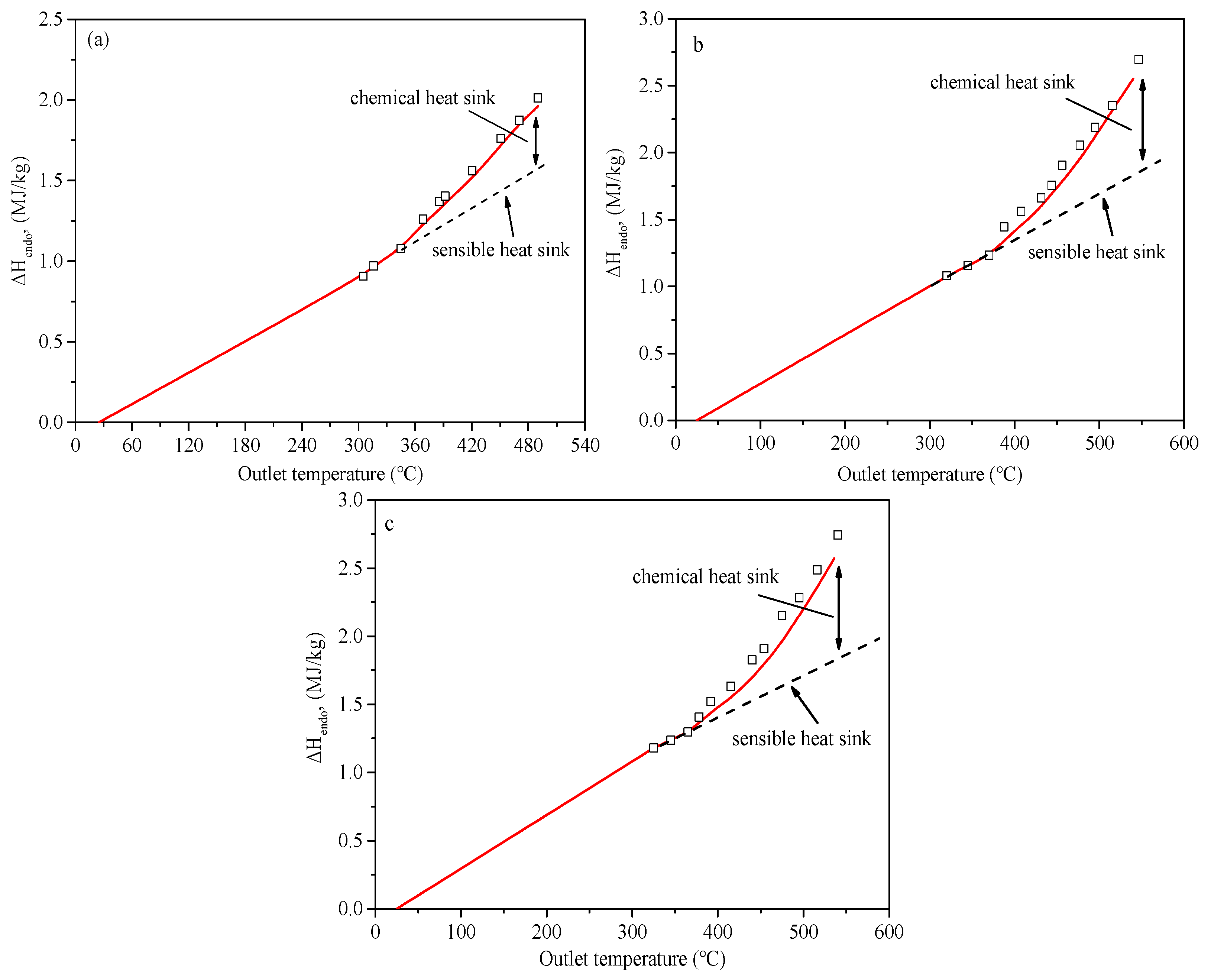
5.3. Heat Sink Distribution
6. Conclusions
- (1)
- During the regeneration cooling process, the H2 generated by the CSR reaction can improve the heat sink. The reaction products of CTC and TC include some liquid hydrocarbons, which have a weak improvement in endothermic capacity.
- (2)
- By comparing the experimental values of the main product content, outlet temperature, conversion rate, and heat sink with the corresponding simulated values, the results show that the proposed model is reliable for analyzing the RCSR reaction process and heat absorption capacity of regenerative cooling.
- (3)
- After the fuel flows into the connecting tube, the TC reaction continues, increasing the conversion rate of n-decane. The TC reaction of the connecting tube has a certain impact on the fuel conversion rate and temperature, which should be considered in the numerical calculation.
- (4)
- Unlike the convective heat transfer coefficient, the Nusselt number of a fluid is influenced by the thermal conductivity of the mixed fluid, exhibiting different patterns with changes in the water content.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Notation
| Nomenclature | |||
| A | pre-exponential factor | T | temperature, K |
| b | bulk fluid | thermal cracking | |
| catalytic steam reforming | V | volume, m3 | |
| catalytic thermal cracking | conversion, % | ||
| d | inner diameter of the tube, mm | y | yield of reaction product |
| Ea | activation energy, kJ/mol | Subscripts | |
| ΔHendo | heat sink, MJ/kg | g | gas phase |
| h | convective heat transfer coefficient, kW/(m2·K) | i | raw material component |
| washcoat thickness, mm | j | product components | |
| specific enthalpy, J/kg | l | liquid phase | |
| m | feed quality of raw materials, g | w | mass fraction |
| M | molar mass, kg/mol | wall | |
| Nu | Nusselt number | in | inside surface |
| partial catalytic steam reforming | out | outside surface | |
| q | heat flux, (kW/m2) | Greek Symbols | |
| r | radius, mm | μ | dynamic viscosity, N·s/m2 |
| R | molar gas constant, J/(K·mol) | ρ | density, kg/m3 |
| S | molar selectivity | λ | thermal conductivity, W/(m·K) |
References
- Pan, Y.; Zhang, H.; Zhang, C.; Wang, H.; Jing, K.; Wang, L.; Zhang, X.; Liu, G. Supercritical pyrolysis and coking of JP-10 in regenerative cooling channels. Energy Fuels 2020, 34, 1627–1638. [Google Scholar] [CrossRef]
- Feng, Y.; Zhang, H.; Zhang, D.; Chen, F.; Xu, X.; Qin, J.; Jiao, Y. The mechanism of ethanol blending on the variation of chemical heat sink in n-decane thermal cracking process. Fuel 2023, 353, 129204. [Google Scholar] [CrossRef]
- Huang, H.; Spadaccini, L.J.; Sobel, D.R. Fuel-Cooled Thermal Management for Advanced Aeroengines. J. Eng. Gas Turbines Power 2002, 126, 284–293. [Google Scholar] [CrossRef]
- Zheng, Q.; Xiao, Z.; Xu, J.; Pan, L.; Zhang, X.; Zhou, J. Catalytic steam reforming and heat sink of high-energy-density fuels: Correlation of reaction behaviors with molecular structures. Fuel 2021, 286, 119371. [Google Scholar] [CrossRef]
- Edwards, T. Cracking and Deposition Behavior of Supercritical Hydrocarbon Aviation Fuels. Combust. Sci. Technol. 2006, 178, 307–334. [Google Scholar] [CrossRef]
- Liu, G.; Wang, X.; Zhang, X. Pyrolytic depositions of hydrocarbon aviation fuels in regenerative cooling channels. J. Anal. Appl. Pyrolysis 2013, 104, 384–395. [Google Scholar] [CrossRef]
- Feng, Y.; Liu, Y.; Cao, Y.; Gong, K.; Liu, S.; Qin, J. Thermal management evaluation for advanced aero-engines using catalytic steam reforming of hydrocarbon fuels. Energy 2020, 193, 116738. [Google Scholar] [CrossRef]
- Li, J.; Shao, J.; Liu, C.; Rao, H.; Li, Z.; Li, X. Pyrolysis Mechanism of Hydrocarbon Fuels and Kinetic Modeling. Acta Chim Sinica 2010, 68, e45. [Google Scholar]
- Jiao, Y.; Li, J.; Wang, J.; Wang, J.; Zhu, Q.; Chen, Y.; Li, X. Experiment and kinetics simulation on the pyrolysis of n-decane. Acta Phys.-Chim. Sin. 2011, 27, 1061–1067. [Google Scholar]
- Zeng, M.; Yuan, W.; Wang, Y.; Zhou, W.; Zhang, L.; Qi, F.; Li, Y. Experimental and kinetic modeling study of pyrolysis and oxidation of n-decane. Combust. Flame 2014, 161, 1701–1715. [Google Scholar] [CrossRef]
- Jia, Z.; Huang, H.; Zhou, W. Experimental and Modeling Investigation of n-Decane Pyrolysis at Supercritical Pressures. Energy Fuels 2014, 28, 6019–6028. [Google Scholar] [CrossRef]
- Zhong, F.; Fan, X.; Yu, G.; Li, J. Thermal cracking of aviation kerosene for scramjet applications. Sci. China Ser. E Technol. Sci. 2009, 52, 2644–2652. [Google Scholar] [CrossRef]
- Ward, T.; Ervin, J.; Striebich, R.; Zabarnick, S. Simulations of flowing mildly-cracked normal alkanes incorporating proportional product distributions. J. Propuls. Power 2004, 20, 394–402. [Google Scholar] [CrossRef]
- Ward, T.; Ervin, J.; Zabarnick, S.; Shafer, L. Pressure effects on flowing mildly-cracked n-decane. J. Propuls. Power 2005, 21, 344–355. [Google Scholar] [CrossRef]
- Jiang, R.; Liu, G.; Zhang, X. Thermal Cracking of Hydrocarbon Aviation Fuels in Regenerative Cooling Microchannels. Energy Fuels 2013, 27, 2563–2577. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, Y.; Liang, C.; Chen, Y.; Zhang, Q.; Li, X. Molecular-level modeling investigation of n-decane pyrolysis at high temperature. J. Anal. Appl. Pyrolysis 2017, 128, 412–422. [Google Scholar] [CrossRef]
- Zhu, Y.; Liu, B.; Jiang, P. Experimental and Numerical Investigations on n-Decane Thermal Cracking at Supercritical Pressures in a Vertical Tube. Energy Fuels 2013, 28, 466–474. [Google Scholar] [CrossRef]
- Wang, P.; Zhang, X.; Shi, R.; Zhao, J.; Yuan, Z.; Zhang, T. Light-Driven Hydrogen Production from Steam Methane Reforming via Bimetallic Pd/Ni Catalysts Derived from Layered Double Hydroxide Nanosheets. Energy Fuels 2022, 36, 11627–11635. [Google Scholar] [CrossRef]
- Lu, M.; Xiong, Z.; Fang, K.; Li, X.; Li, J.; Li, T. Steam reforming of toluene over nickel catalysts supported on coal gangue ash. Renew. Energy 2020, 160, 385–395. [Google Scholar] [CrossRef]
- Habib, M.A.; Harale, A.; Paglieri, S.; Alrashed, F.S. Palladium-Alloy Membrane Reactors for Fuel Reforming and Hydrogen Production: A Review. Energy Fuels 2021, 35, 5558–5593. [Google Scholar] [CrossRef]
- Zhang, Z.; Qin, C.; Ou, Z.; Xia, H.; Ran, J.; Wu, C. Experimental and thermodynamic study on sorption-enhanced steam reforming of toluene for H2 production using the mixture of Ni/perovskite-CaO. Fuel 2021, 305, 121447. [Google Scholar] [CrossRef]
- Chen, F.; Cao, Y.; Feng, Y.; Wang, Z.; Zhang, D.; Qin, J.; He, X. Experimental study on the mechanism of water on heat sink variation in catalytic partial steam reforming of supercritical n-decane. Fuel 2024, 357, 129892. [Google Scholar] [CrossRef]
- Gong, K.; Zhang, Y.; Cao, Y.; Feng, Y.; Qin, J. Deep learning approach for predicting the flow field and heat transfer of supercritical hydrocarbon fuels. Int. J. Heat Mass Transf. 2024, 219, 124869. [Google Scholar] [CrossRef]
- Irani, M.; Alizadehddkhel, A.; Pour, A.N.; Hoseini, N.; Adinehnia, M. CFD modeling of hydrogen production using steam reforming of methane in monolith reactors: Surface or volume-base reaction model? Int. J. Hydrogen Energy 2011, 36, 15602–15610. [Google Scholar] [CrossRef]
- Zhang, Y.; Cao, Y.; Feng, Y.; Xu, S.; Wang, J.; Qin, J. A new modeling method to estimate the heat transfer characteristics of supercritical aviation kerosene RP-3 with pyrolysis. Chem. Eng. Sci. 2023, 267, 118324. [Google Scholar] [CrossRef]
- Lei, Z.; Liu, B.; Huang, Q.; He, K.; Bao, Z.; Zhu, Q.; Li, X. Thermal cracking characteristics of n-decane in the rectangular and circular tubes. Chin. J. Chem. Eng. 2019, 27, 2876–2883. [Google Scholar] [CrossRef]
- Jiang, P.; Wang, Y.; Zhu, Y. Differential global reaction model with variable stoichiometric coefficients for thermal cracking of n-decane at supercritical pressures. Energy Fuels 2019, 33, 7244–7256. [Google Scholar] [CrossRef]
- Jones, W.P.; Lindstedt, R.P. Global reaction schemes for hydrocarbon combustion. Combust. Flame 1988, 73, 233–249. [Google Scholar] [CrossRef]
- Wang, L.; Singh, C. Balancing risk and cost in fuzzy economic dispatch including wind power penetration based on particle swarm optimization. Electr. Power Syst. Res. 2008, 78, 1361–1368. [Google Scholar] [CrossRef]





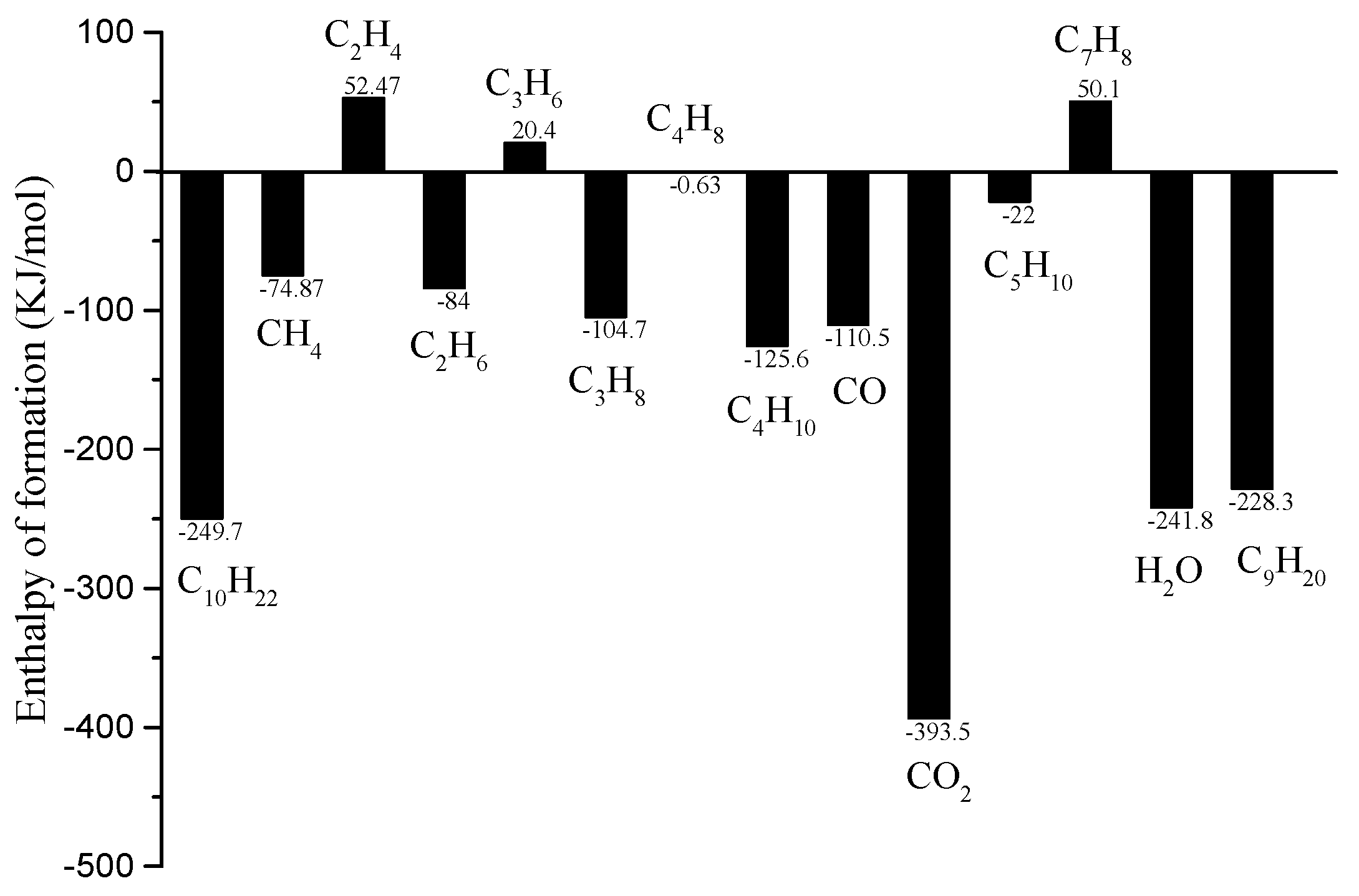
| Reaction | Ea (KJ/mol) | A |
|---|---|---|
| C10H22 + 18.3H2O → 29.3H2 + 1.7CO + 8.3CO2 (R1) | 87.09 | 1.6 × 105 |
| C10H22 → 4.623H2 + 0.2CH4 + 0.06C2H4 + 0.1C2H6 + 0.03C3H6 + 0.03C3H8 + 0.007C4H10 + 0.008C4H8 + 1.32C7H8 (R2) | 91.07 | 1.1 × 106 |
| C10H22 → 0.017H2 + 0.184CH4 + 0.198C2H4 + 0.1327C2H6 + 0.0566C3H6 + 0.0372C3H8 + 0.0135C4H8 + 0.0048C4H10 + 0.63626C5H10 + 0.6243C9H20 (R3) | 267.43 | 5.0 × 1016 |
| CH4 + H2O = CO + 3H2 (R4) | 125.5 | 3.0 × 108 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, F.; He, J.; Feng, Y.; Wang, Z. Modeling and Numerical Investigations of Flowing N-Decane Partial Catalytic Steam Reforming at Supercritical Pressure. Energies 2024, 17, 5215. https://doi.org/10.3390/en17205215
Chen F, He J, Feng Y, Wang Z. Modeling and Numerical Investigations of Flowing N-Decane Partial Catalytic Steam Reforming at Supercritical Pressure. Energies. 2024; 17(20):5215. https://doi.org/10.3390/en17205215
Chicago/Turabian StyleChen, Fuqiang, Junbo He, Yu Feng, and Zhenhua Wang. 2024. "Modeling and Numerical Investigations of Flowing N-Decane Partial Catalytic Steam Reforming at Supercritical Pressure" Energies 17, no. 20: 5215. https://doi.org/10.3390/en17205215
APA StyleChen, F., He, J., Feng, Y., & Wang, Z. (2024). Modeling and Numerical Investigations of Flowing N-Decane Partial Catalytic Steam Reforming at Supercritical Pressure. Energies, 17(20), 5215. https://doi.org/10.3390/en17205215






