Enhancing Efficiency and Reliability of Tidal Stream Energy Conversion through Swept-Blade Design
Abstract
1. Introduction
- (1)
- Which sweep design is more effective in improving the energy capture efficiency of TCT blades?
- (2)
- How does the sweep design affect the cavitation resistance of TCT blades?
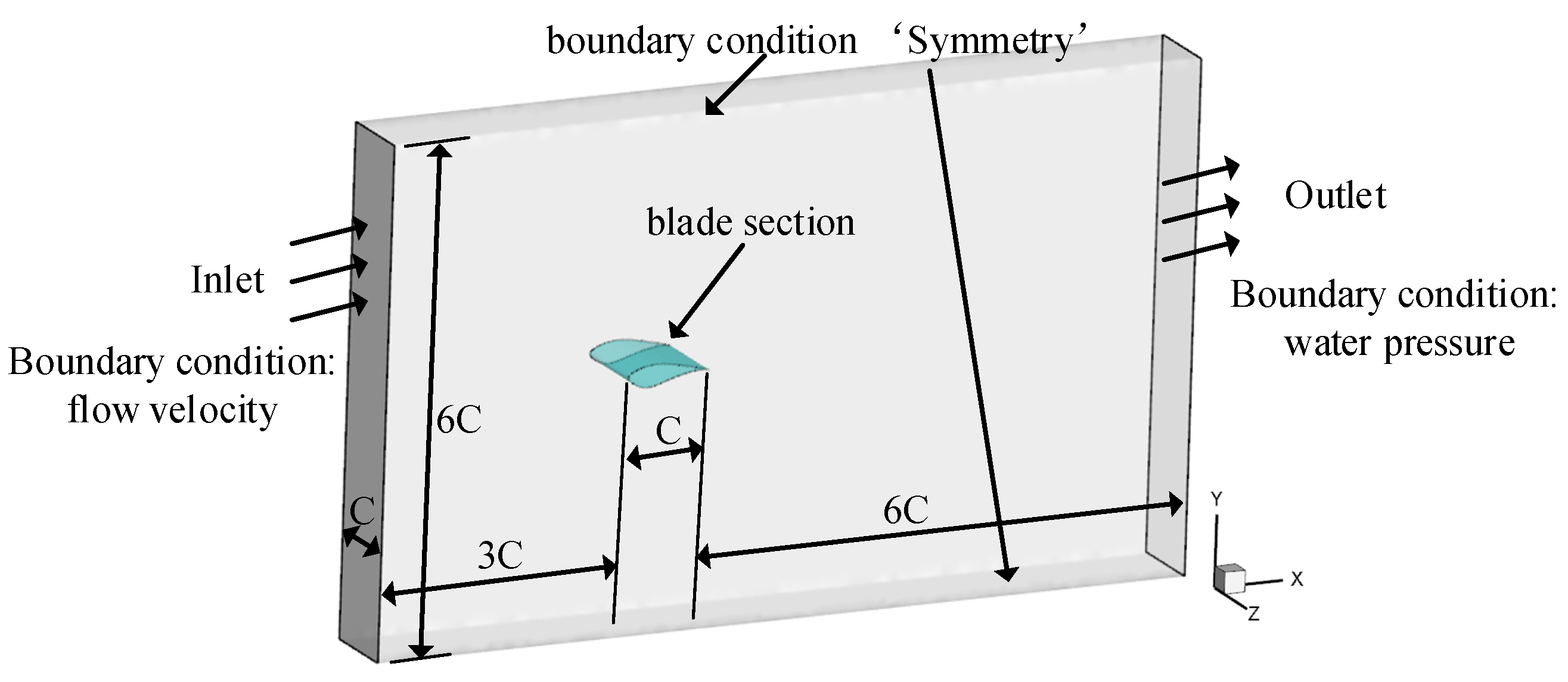
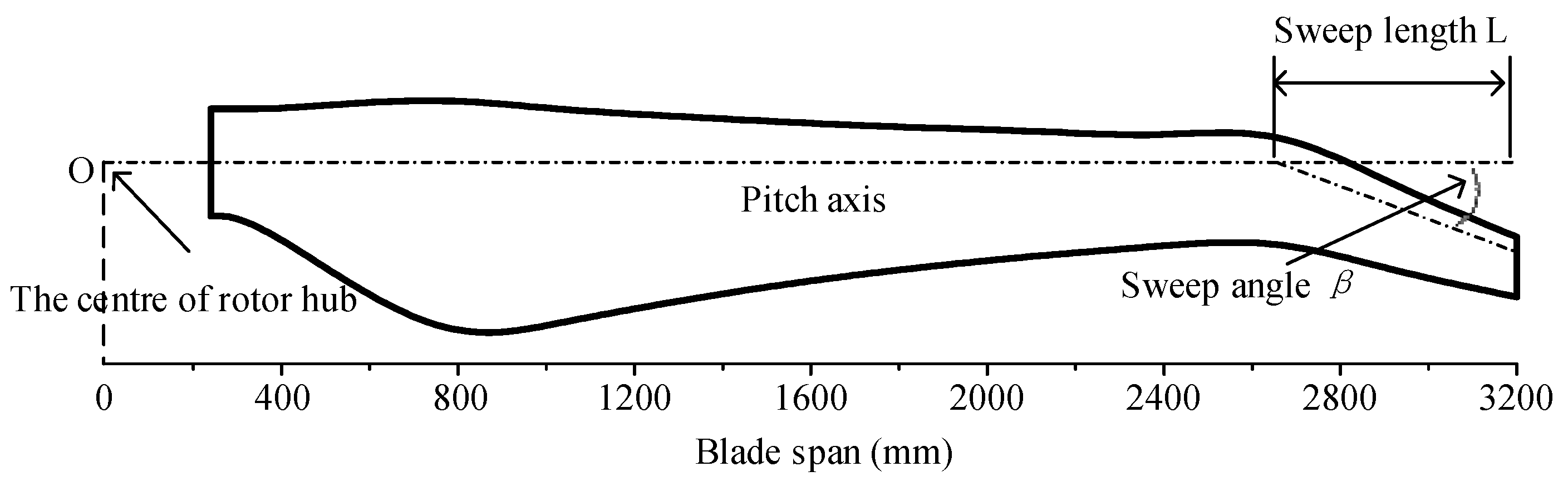
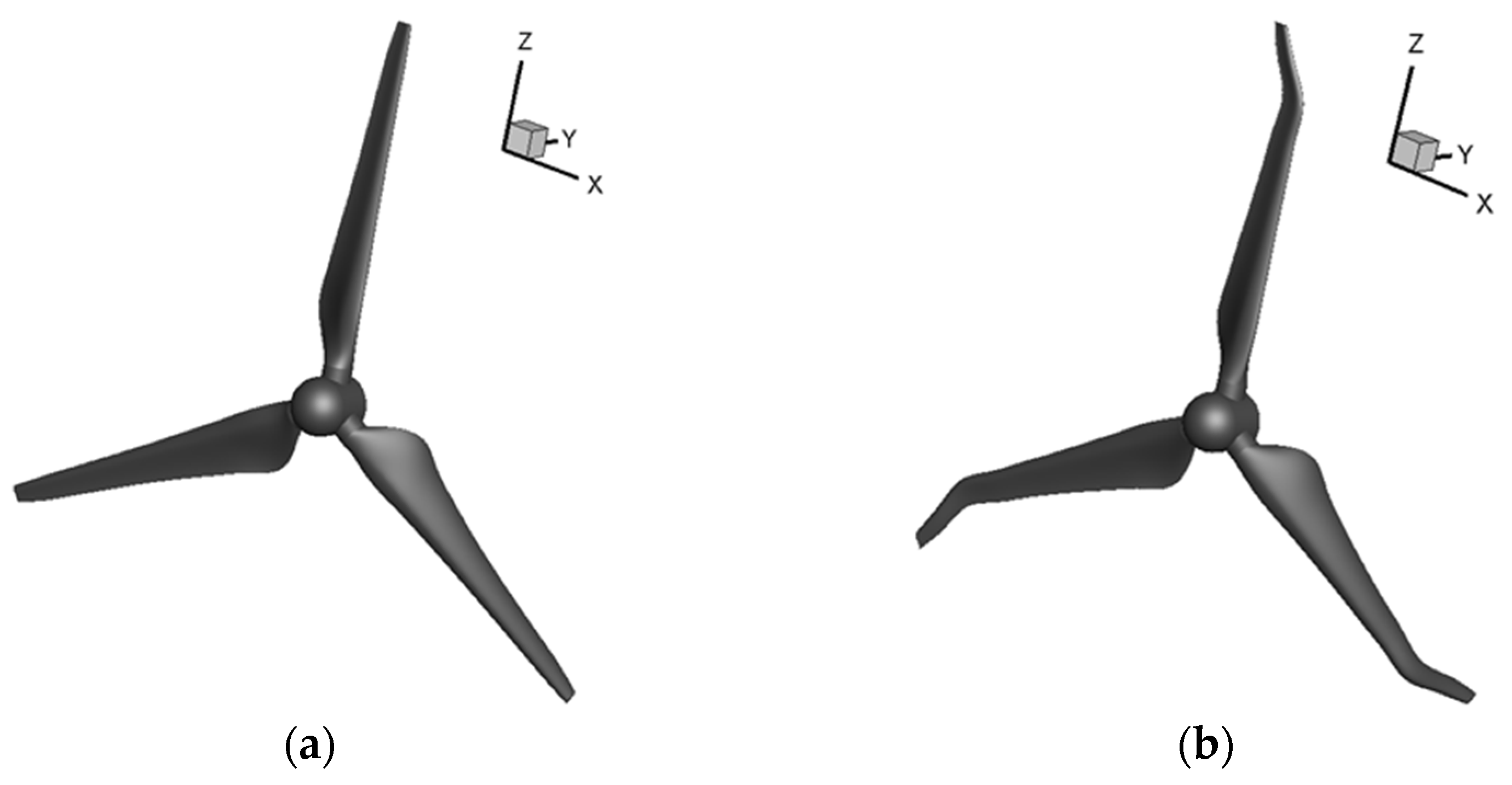
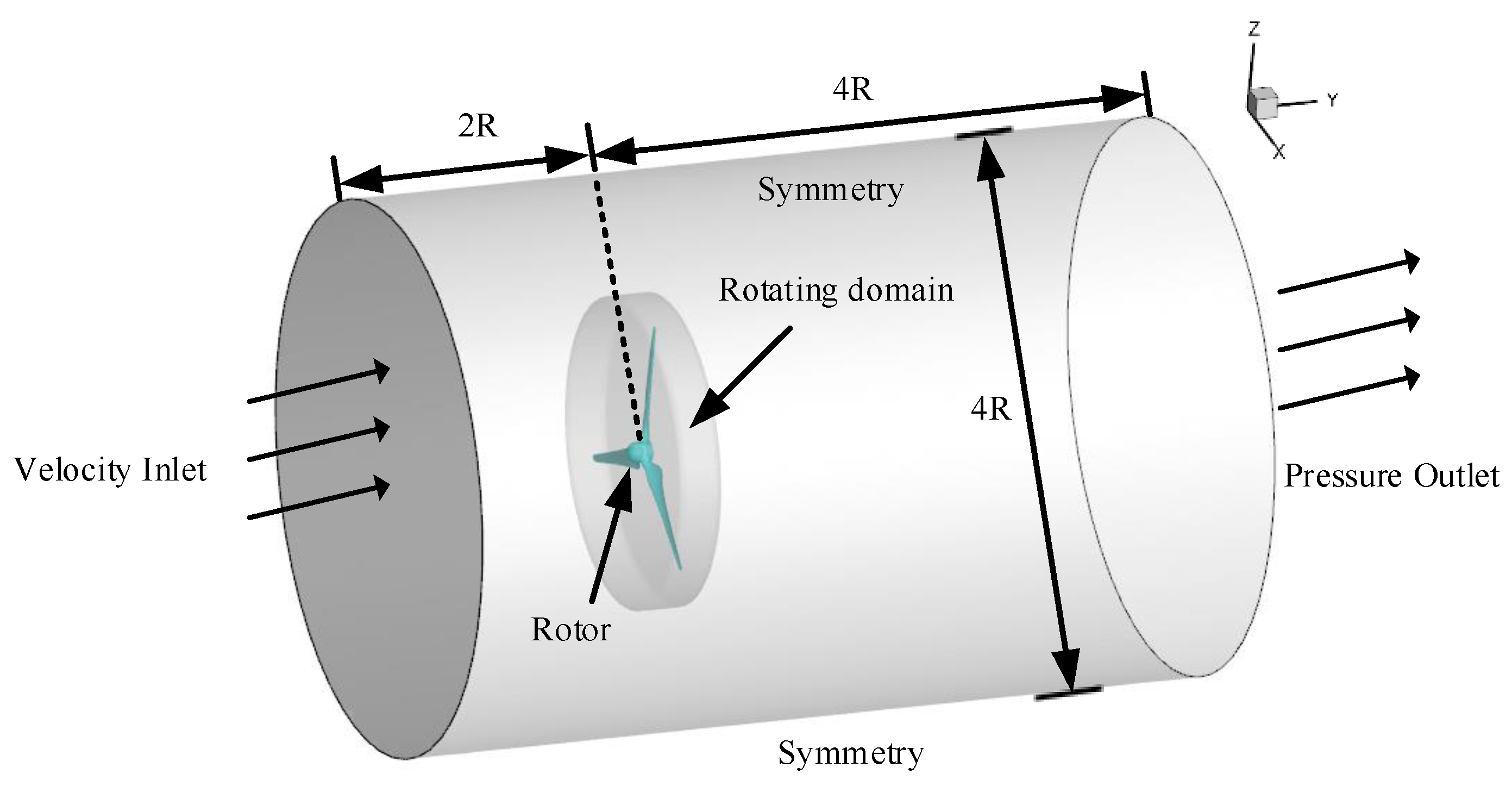
2. Numerical Model of a Swept-Blade TCT
- (1)
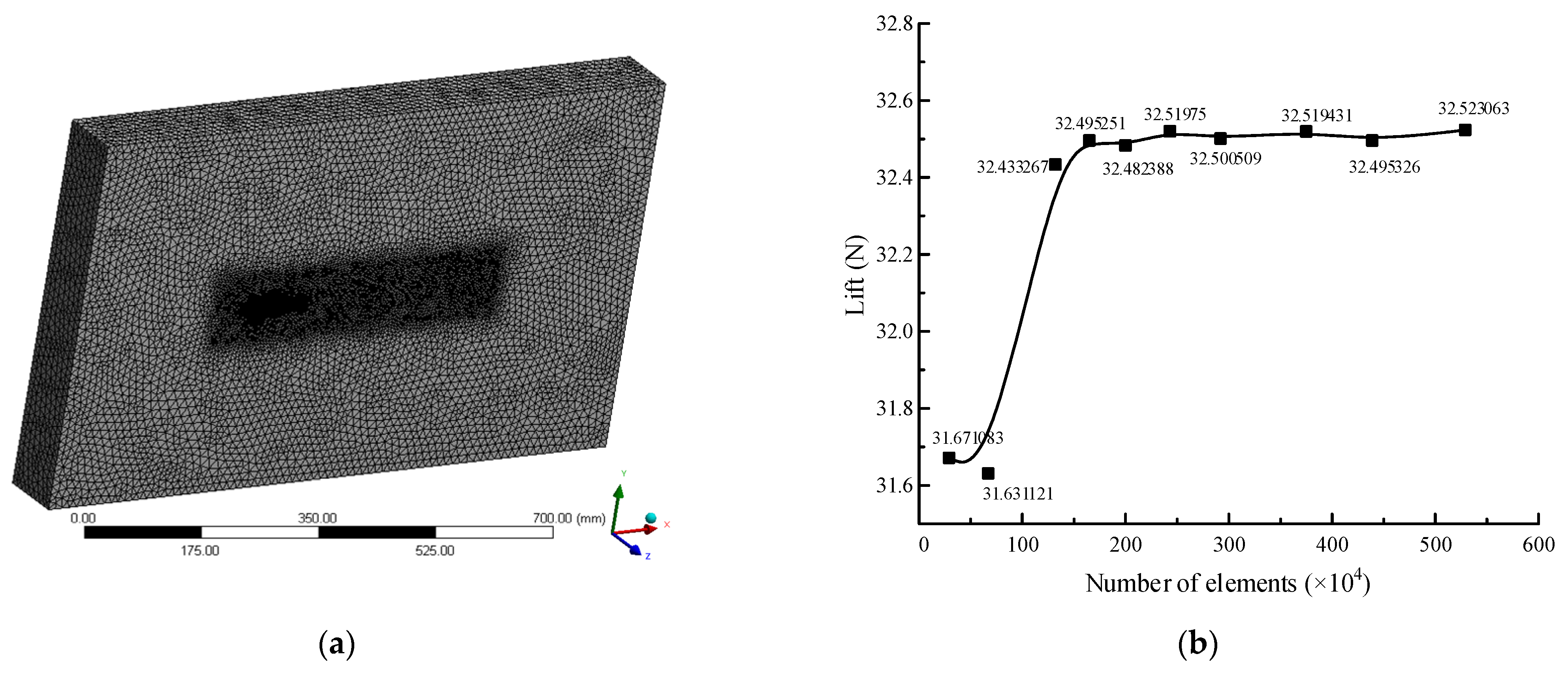
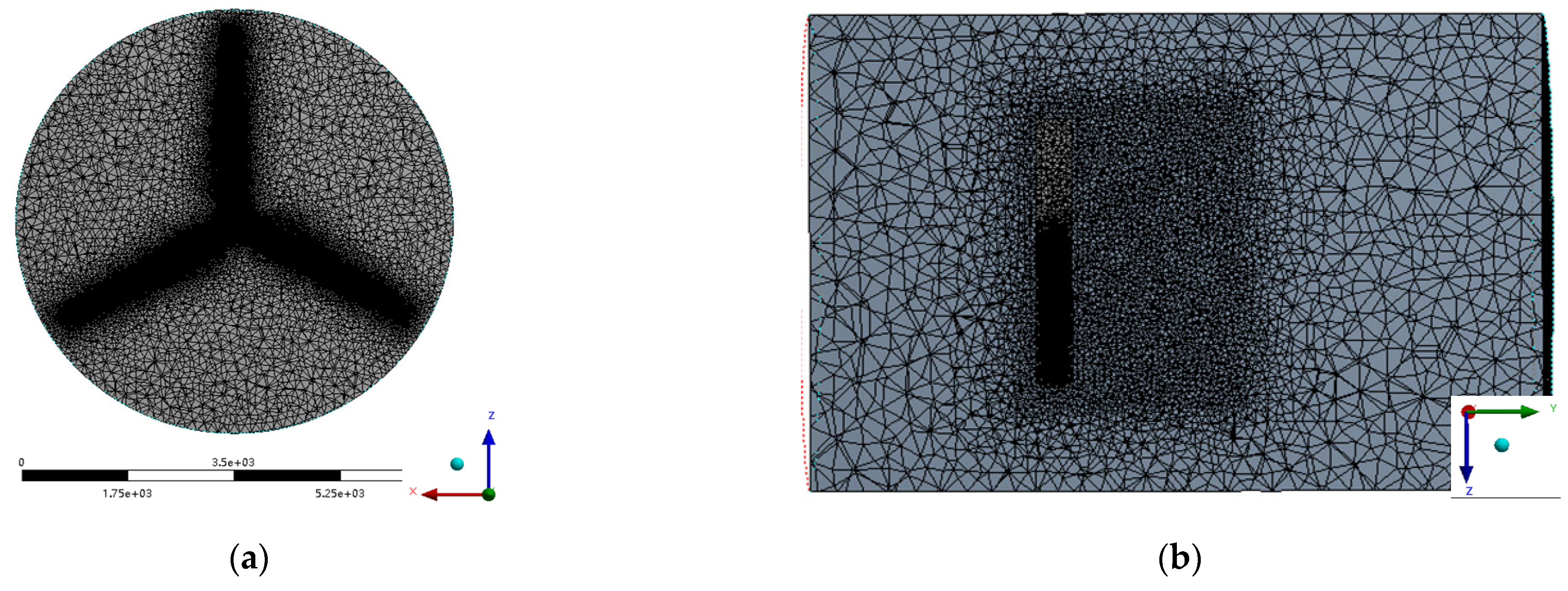
- ‘Face sizing’—Refers to the size of meshing elements on the blade surfaces. The higher the number of elements used for meshing, the smaller the face sizing. In this study, the initial size of the elements is set to 0.006 m. However, the size may be adjusted based on the number of elements;
- (2)
- ‘Body sizing’—A cylindrical region, with a diameter of 8 m and a height of 5.4 m, was defined to enclose the rotating domain. ‘Body sizing’, which is set to 0.18 m, refers to the size of the cells for meshing the cylindrical region. In the modelling, the body of influence control is utilized to create finer meshes in the cylindrical region;
- (3)
- ‘Inflation’—An inflation region is designed around the blade during the modelling process of this study. The inflation region consists of five layers. The thickness of the fifth inflation layer, representing the maximum thickness of the inflation layer, is set to 0.01 m. The thickness growth rate of these five layers in the direction of inflation is 1.2.
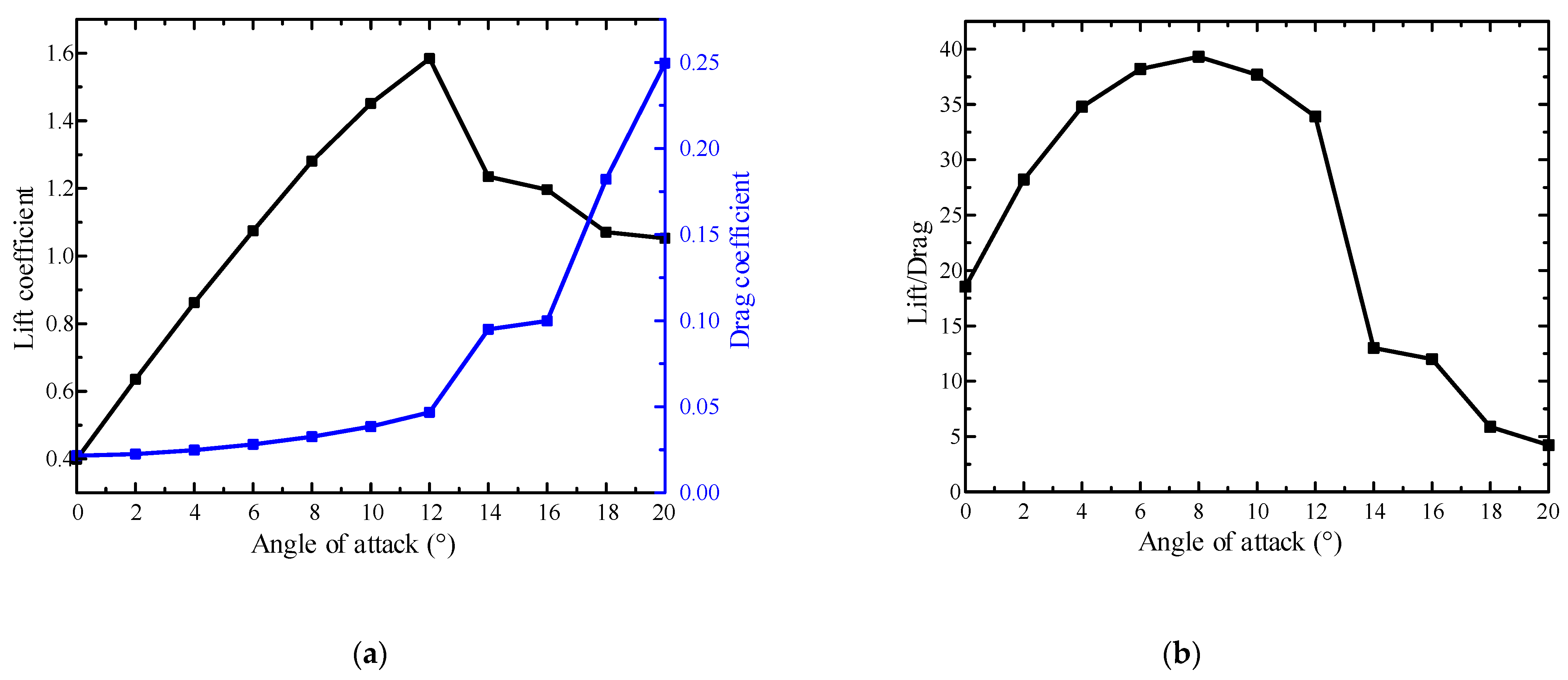
3. Numerical Study of Swept Blade Efficiency
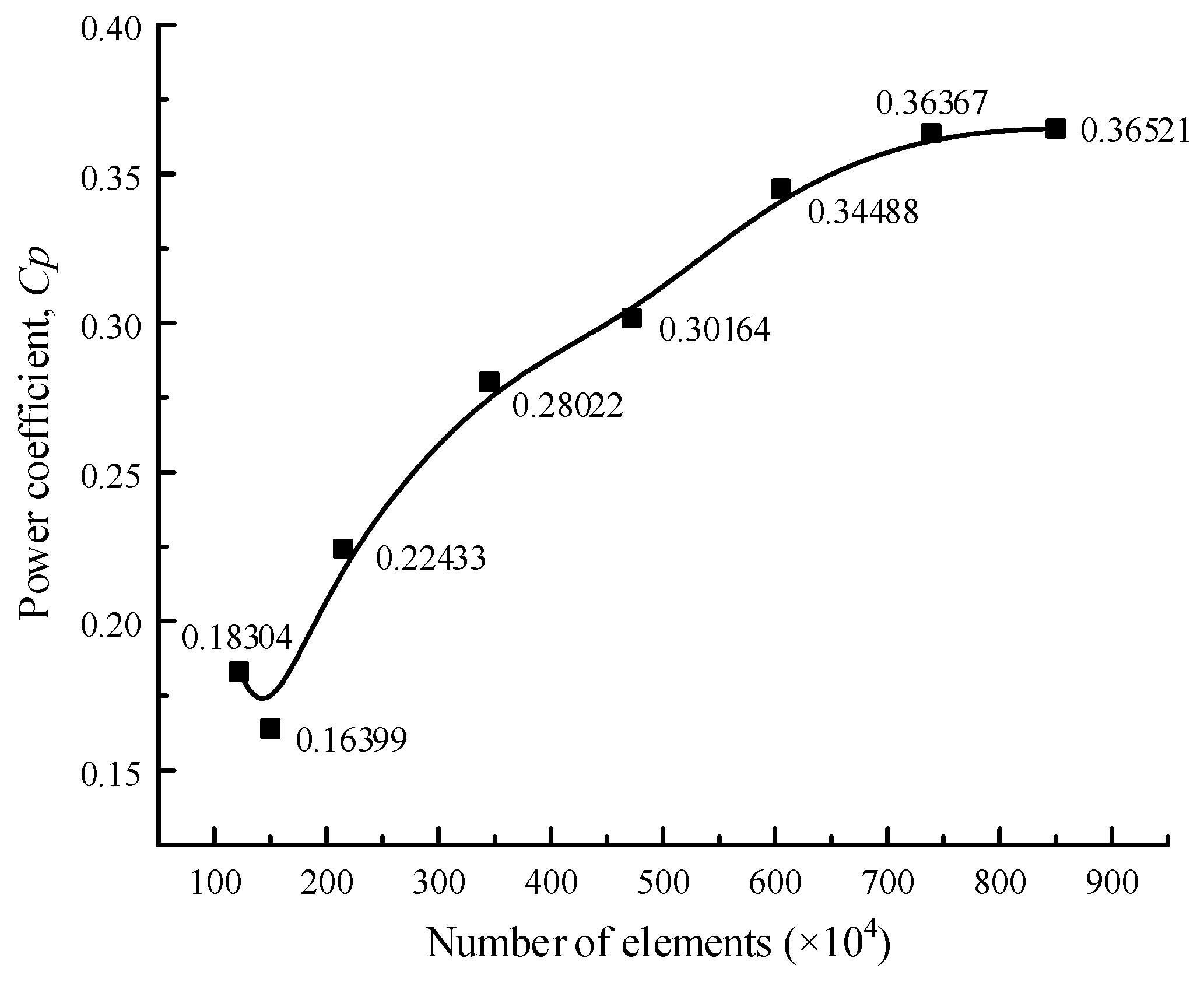
- The power coefficient of the TCT with conventional straight blades (i.e., when ) is 0.36521;
- Both the sweep length and sweep angle of the blade can influence the power coefficient of the TCT;
- The power coefficient of the swept-blade TCT increases first and then decreases with the increase in sweep angle . This suggests that not all sweep designs can enhance the efficiency of the TCT. The efficiency of the TCT can be increased only when an appropriate sweep design is adopted. If either the sweep length or the sweep angle is excessively large or small, the efficiency of the TCT will significantly decline, particularly in scenarios featuring a substantial sweep length;
- Under different sweep lengths L, the power coefficient reaches the different maximum values at different sweep angles . For example, when L = 0.272 m, reaches its maximum value of 0.4096 at ; when L = 0.544 m, reaches its maximum value of 0.41654 at ; when L = 1.088 m, reaches its maximum value of 0.40801 at ; and when L = 1.36 m, reaches its maximum value of 0.40733 at ;
- Among all the scenarios examined, the maximum power coefficient that the swept-blade TCT can achieve is 0.41654, which is 14% higher than that of the TCT using three conventional straight blades.
4. Numerical Study of Swept-Blade Cavitation Resistance
- (1)
- As expected, the calculated value of the cavitation number decreases gradually with the increase in TSR. This trend suggests that blade tip cavitation is more likely to occur at higher rotor speeds;
- (2)
- In all instances of TSRs examined, the cavitation number value for the swept blade consistently surpasses the corresponding value for the straight blade. This finding indicates that the swept blade exhibits a superior cavitation resistance capacity over the conventional straight blade when operating in harsh marine environments;
- (3)
- Finally, the increment in the cavitation number value achieved by the swept blade tends to increase with the increase in TSR. This suggests that as the rotor speed rises, the advantages of the swept blade in terms of anti-cavitation become more pronounced compared to the straight blade.
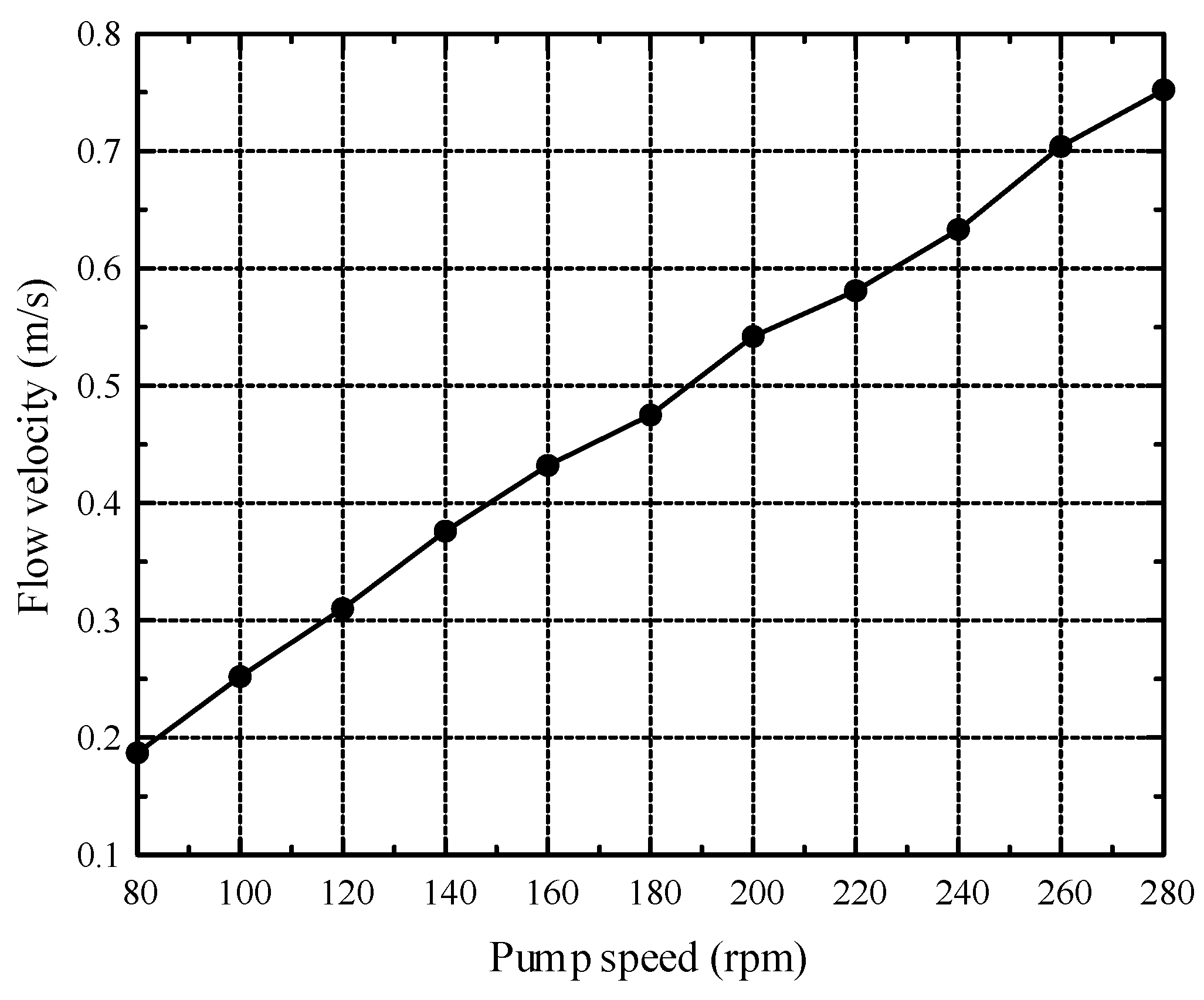
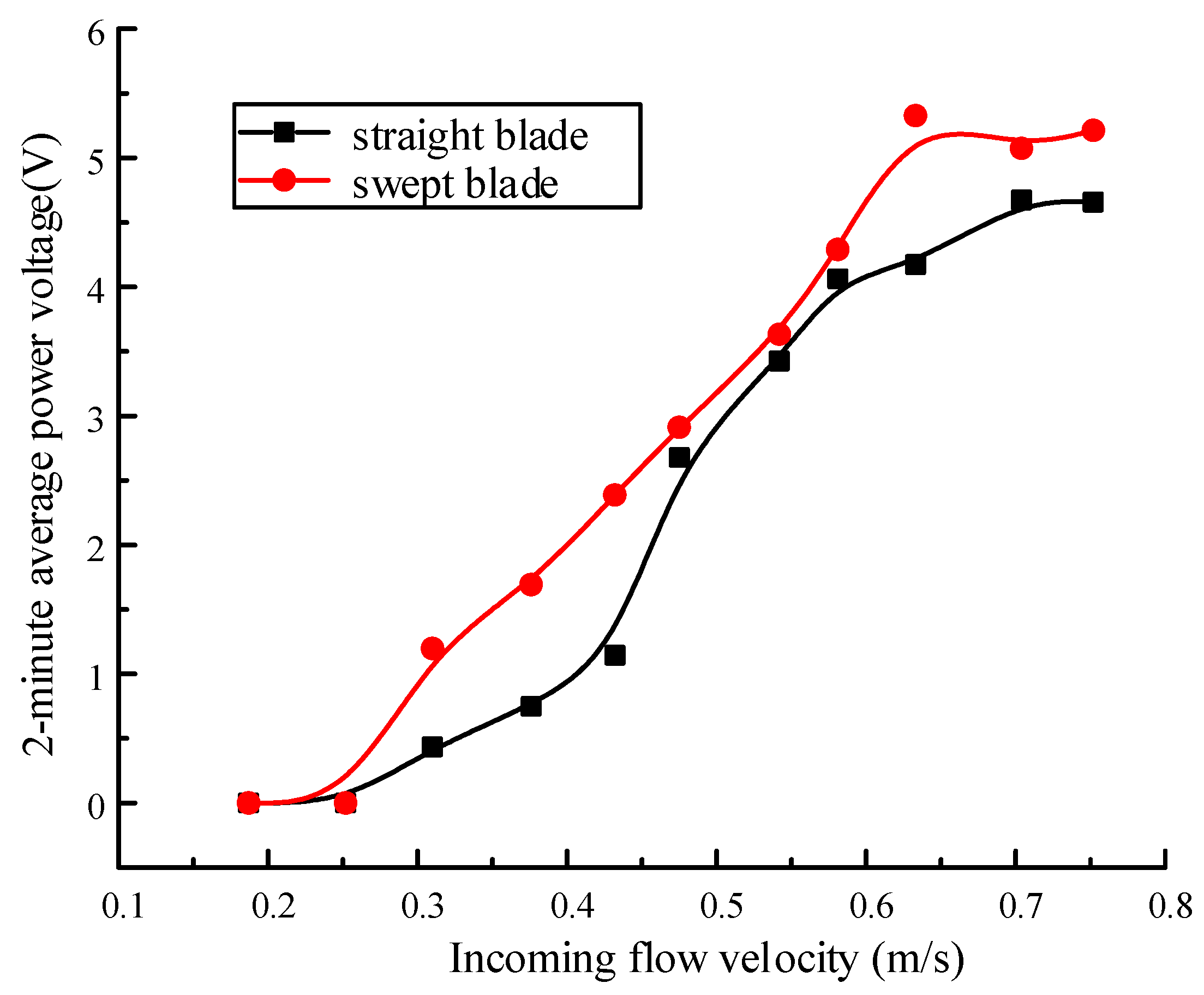
5. Verification Test
- (1)
- When the flow velocity is smaller than 0.252 m/s, neither the straight-blade rotor nor the swept-blade rotor rotates, indicating that both types of TCTs have a cut-in speed of about 0.252 m/s;
- (2)
- After reaching the cut-in speed, the rotors start to rotate and harness kinetic energy from tidal streams. Notably, across all corresponding flow velocities, the power voltages generated by the swept-blade TCT consistently surpass those generated by the straight-blade TCT. This suggests that the swept blade, thanks to its sweep design, exhibits a superior efficiency in capturing energy from tidal streams compared to the conventional straight blade.
6. Conclusions
- (1)
- Even at higher Reynolds numbers, the sweep design remains effective in enhancing the energy capture efficiency of the TCT blades. Both the sweep length and sweep angle of the blade play significant roles in influencing the power generation efficiency of the TCT. It is observed that under varying sweep lengths, the power coefficient attains its maximum value at different sweep angles. For the particular swept blade investigated in this study, the power coefficient reaches the highest at 0.41654 when the sweep length is 0.544 m and the sweep angle is , which is 14% higher than that of the TCT using conventional straight blades;
- (2)
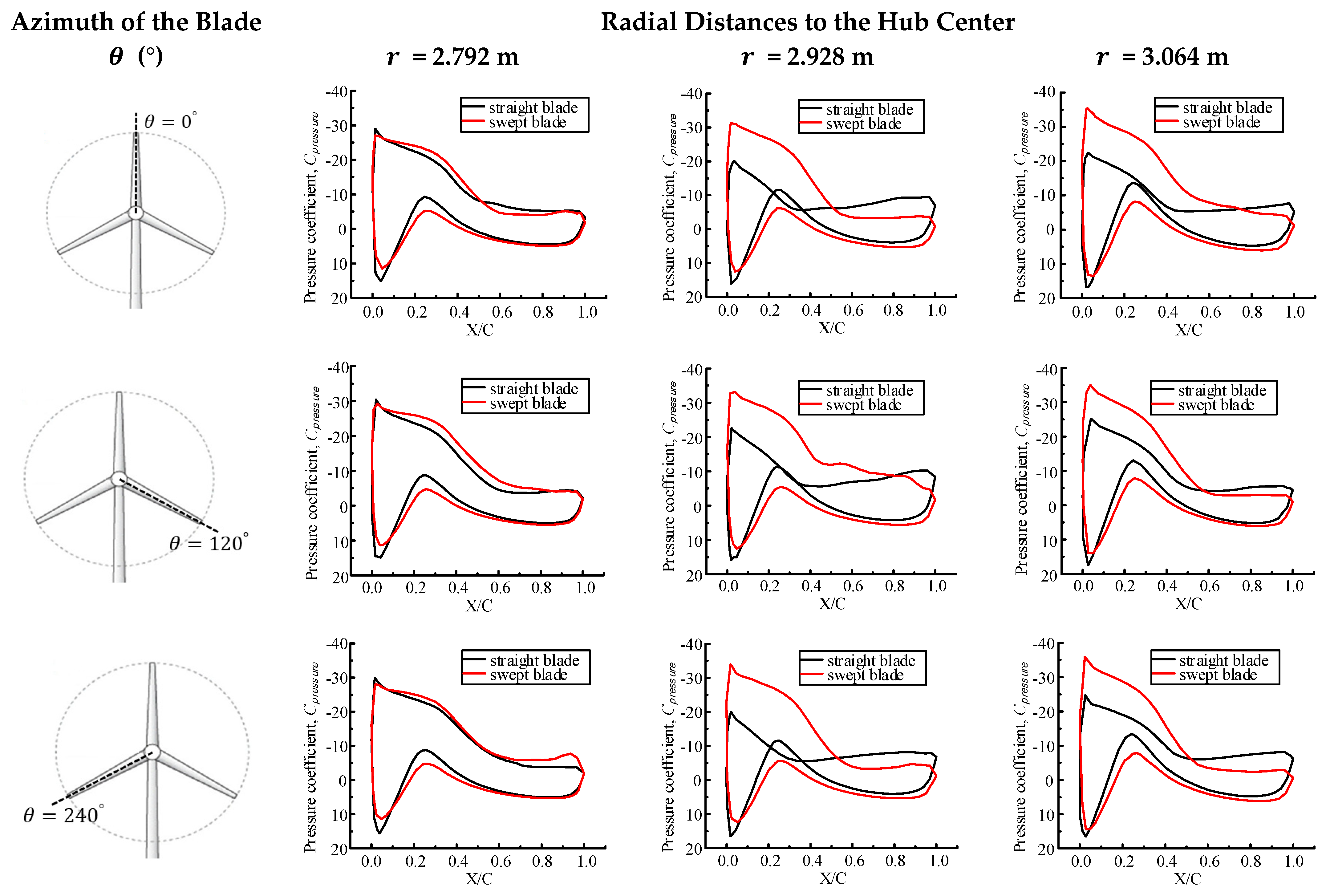
- The contribution of the sweep design to efficiency enhancement varies with the span locations and the azimuth positions of the blade, but its contribution will become more significant in the tip section of the blade;
- (3)
- The swept-blade TCT shows a higher power generation efficiency than the straight-blade TCT across a broad range of rotor speeds, spanning from 8 to 18 rpm. To be precise, with the swept blades, the power coefficient of the TCT can be improved by 5–17%, depending on the TSR of the TCT rotor;
- (4)
- Under all TSRs examined, the cavitation number values for the swept blade are always larger than those for the straight blade. This suggests that the swept blade exhibits a superior cavitation resistance capacity over the conventional straight blade when operating in harsh marine environments. Moreover, the rise in the cavitation number associated with the swept blade tends to amplify with an increase in TSR. This implies that as the rotor speed escalates, the benefits of the swept blade in terms of anti-cavitation characteristics become more evident when contrasted with those of the straight blade.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
| No. of Point | Coordinates | ||
|---|---|---|---|
| x | y | z | |
| 1 | 1 | 0 | 0 |
| 2 | 0.9966 | 0.003137 | 0 |
| 3 | 0.99314 | 0.004139 | 0 |
| 4 | 0.98961 | 0.005151 | 0 |
| 5 | 0.98601 | 0.006171 | 0 |
| 6 | 0.98235 | 0.007196 | 0 |
| 7 | 0.97863 | 0.008224 | 0 |
| 8 | 0.97484 | 0.009259 | 0 |
| 9 | 0.97098 | 0.010301 | 0 |
| 10 | 0.96706 | 0.011348 | 0 |
| 11 | 0.963069 | 0.012403 | 0 |
| 12 | 0.95902 | 0.013465 | 0 |
| 13 | 0.954901 | 0.014539 | 0 |
| 14 | 0.95072 | 0.015622 | 0 |
| 15 | 0.94647 | 0.016719 | 0 |
| 16 | 0.94216 | 0.017829 | 0 |
| 17 | 0.93778 | 0.018954 | 0 |
| 18 | 0.93333 | 0.020093 | 0 |
| 19 | 0.92882 | 0.021245 | 0 |
| 20 | 0.92425 | 0.02241 | 0 |
| 21 | 0.91961 | 0.02359 | 0 |
| 22 | 0.9149 | 0.024787 | 0 |
| 23 | 0.91013 | 0.025997 | 0 |
| 24 | 0.90529 | 0.027224 | 0 |
| 25 | 0.90039 | 0.028464 | 0 |
| 26 | 0.89542 | 0.029719 | 0 |
| 27 | 0.89039 | 0.030989 | 0 |
| 28 | 0.88529 | 0.032274 | 0 |
| 29 | 0.88013 | 0.033572 | 0 |
| 30 | 0.8749 | 0.034887 | 0 |
| 31 | 0.86961 | 0.036215 | 0 |
| 32 | 0.86425 | 0.037558 | 0 |
| 33 | 0.85882 | 0.038915 | 0 |
| 34 | 0.85333 | 0.040285 | 0 |
| 35 | 0.84778 | 0.041666 | 0 |
| 36 | 0.84216 | 0.04306 | 0 |
| 37 | 0.83647 | 0.044467 | 0 |
| 38 | 0.83072 | 0.045886 | 0 |
| 39 | 0.8249 | 0.047319 | 0 |
| 40 | 0.81902 | 0.048765 | 0 |
| 41 | 0.81307 | 0.050226 | 0 |
| 42 | 0.80706 | 0.051699 | 0 |
| 43 | 0.80098 | 0.053185 | 0 |
| 44 | 0.79484 | 0.054682 | 0 |
| 45 | 0.78863 | 0.056193 | 0 |
| 46 | 0.78235 | 0.057714 | 0 |
| 47 | 0.77601 | 0.059245 | 0 |
| 48 | 0.76961 | 0.060782 | 0 |
| 49 | 0.76314 | 0.062328 | 0 |
| 50 | 0.7566 | 0.063883 | 0 |
| 51 | 0.75 | 0.065444 | 0 |
| 52 | 0.74333 | 0.067014 | 0 |
| 53 | 0.73667 | 0.068573 | 0 |
| 54 | 0.73 | 0.070128 | 0 |
| 55 | 0.72333 | 0.071676 | 0 |
| 56 | 0.71667 | 0.073216 | 0 |
| 57 | 0.71 | 0.074751 | 0 |
| 58 | 0.70333 | 0.076276 | 0 |
| 59 | 0.69667 | 0.077791 | 0 |
| 60 | 0.69 | 0.079299 | 0 |
| 61 | 0.68333 | 0.080795 | 0 |
| 62 | 0.67667 | 0.082279 | 0 |
| 63 | 0.67 | 0.083754 | 0 |
| 64 | 0.66333 | 0.085217 | 0 |
| 65 | 0.65667 | 0.086666 | 0 |
| 66 | 0.65 | 0.088103 | 0 |
| 67 | 0.64333 | 0.089527 | 0 |
| 68 | 0.63667 | 0.090934 | 0 |
| 69 | 0.63 | 0.092328 | 0 |
| 70 | 0.62333 | 0.093708 | 0 |
| 71 | 0.61667 | 0.095071 | 0 |
| 72 | 0.61 | 0.096418 | 0 |
| 73 | 0.60333 | 0.097748 | 0 |
| 74 | 0.59667 | 0.099058 | 0 |
| 75 | 0.59 | 0.100353 | 0 |
| 76 | 0.58333 | 0.101632 | 0 |
| 77 | 0.57667 | 0.10289 | 0 |
| 78 | 0.57 | 0.10413 | 0 |
| 79 | 0.56333 | 0.105351 | 0 |
| 80 | 0.55667 | 0.106549 | 0 |
| 81 | 0.55 | 0.107728 | 0 |
| 82 | 0.54333 | 0.108883 | 0 |
| 83 | 0.53667 | 0.110014 | 0 |
| 84 | 0.53 | 0.111124 | 0 |
| 85 | 0.52333 | 0.112209 | 0 |
| 86 | 0.51667 | 0.113266 | 0 |
| 87 | 0.51 | 0.114297 | 0 |
| 88 | 0.50333 | 0.115302 | 0 |
| 89 | 0.49667 | 0.116279 | 0 |
| 90 | 0.49 | 0.117228 | 0 |
| 91 | 0.48333 | 0.118151 | 0 |
| 92 | 0.47667 | 0.119044 | 0 |
| 93 | 0.47 | 0.119909 | 0 |
| 94 | 0.46333 | 0.120742 | 0 |
| 95 | 0.45667 | 0.12154 | 0 |
| 96 | 0.45 | 0.122304 | 0 |
| 97 | 0.44333 | 0.12303 | 0 |
| 98 | 0.43667 | 0.123717 | 0 |
| 99 | 0.43 | 0.124364 | 0 |
| 100 | 0.42333 | 0.124971 | 0 |
| 101 | 0.41667 | 0.125536 | 0 |
| 102 | 0.409999 | 0.126062 | 0 |
| 103 | 0.40333 | 0.126545 | 0 |
| 104 | 0.39667 | 0.126985 | 0 |
| 105 | 0.39 | 0.127382 | 0 |
| 106 | 0.38333 | 0.127732 | 0 |
| 107 | 0.37667 | 0.128032 | 0 |
| 108 | 0.37 | 0.128276 | 0 |
| 109 | 0.36333 | 0.128462 | 0 |
| 110 | 0.35667 | 0.128582 | 0 |
| 111 | 0.35 | 0.128635 | 0 |
| 112 | 0.34333 | 0.128618 | 0 |
| 113 | 0.33667 | 0.128532 | 0 |
| 114 | 0.33 | 0.128374 | 0 |
| 115 | 0.32333 | 0.128147 | 0 |
| 116 | 0.31667 | 0.12785 | 0 |
| 117 | 0.31 | 0.127486 | 0 |
| 118 | 0.30333 | 0.127054 | 0 |
| 119 | 0.29667 | 0.12656 | 0 |
| 120 | 0.29 | 0.126 | 0 |
| 121 | 0.28333 | 0.125376 | 0 |
| 122 | 0.27667 | 0.124688 | 0 |
| 123 | 0.27 | 0.123936 | 0 |
| 124 | 0.26333 | 0.12312 | 0 |
| 125 | 0.25667 | 0.122242 | 0 |
| 126 | 0.2500001 | 0.121298 | 0 |
| 127 | 0.24342 | 0.120303 | 0 |
| 128 | 0.23693 | 0.119261 | 0 |
| 129 | 0.23053 | 0.118173 | 0 |
| 130 | 0.22421 | 0.117039 | 0 |
| 131 | 0.21798 | 0.115863 | 0 |
| 132 | 0.21184 | 0.114648 | 0 |
| 133 | 0.20579 | 0.113395 | 0 |
| 134 | 0.19982 | 0.112103 | 0 |
| 135 | 0.19395 | 0.110779 | 0 |
| 136 | 0.18816 | 0.109419 | 0 |
| 137 | 0.18245 | 0.108025 | 0 |
| 138 | 0.17684 | 0.106604 | 0 |
| 139 | 0.17131 | 0.105151 | 0 |
| 140 | 0.16587 | 0.103672 | 0 |
| 141 | 0.16052 | 0.102168 | 0 |
| 142 | 0.15526 | 0.100639 | 0 |
| 143 | 0.15008 | 0.099085 | 0 |
| 144 | 0.14499 | 0.097509 | 0 |
| 145 | 0.13999 | 0.095913 | 0 |
| 146 | 0.13508 | 0.094297 | 0 |
| 147 | 0.13026 | 0.092663 | 0 |
| 148 | 0.12552 | 0.091008 | 0 |
| 149 | 0.12087 | 0.089336 | 0 |
| 150 | 0.11631 | 0.087651 | 0 |
| 151 | 0.11183 | 0.085948 | 0 |
| 152 | 0.10745 | 0.084235 | 0 |
| 153 | 0.10315 | 0.082507 | 0 |
| 154 | 0.09893 | 0.080764 | 0 |
| 155 | 0.09481 | 0.079015 | 0 |
| 156 | 0.09077 | 0.077252 | 0 |
| 157 | 0.08683 | 0.075487 | 0 |
| 158 | 0.08297 | 0.07371 | 0 |
| 159 | 0.07919 | 0.071922 | 0 |
| 160 | 0.07551 | 0.070134 | 0 |
| 161 | 0.07191 | 0.068338 | 0 |
| 162 | 0.0684 | 0.066539 | 0 |
| 163 | 0.06498 | 0.064738 | 0 |
| 164 | 0.06164 | 0.062932 | 0 |
| 165 | 0.0584 | 0.061132 | 0 |
| 166 | 0.05524 | 0.059329 | 0 |
| 167 | 0.05217 | 0.057528 | 0 |
| 168 | 0.04918 | 0.055726 | 0 |
| 169 | 0.04629 | 0.053932 | 0 |
| 170 | 0.04348 | 0.052137 | 0 |
| 171 | 0.04076 | 0.050349 | 0 |
| 172 | 0.03812 | 0.048561 | 0 |
| 173 | 0.03558 | 0.046787 | 0 |
| 174 | 0.03312 | 0.045017 | 0 |
| 175 | 0.03075 | 0.043257 | 0 |
| 176 | 0.02847 | 0.041508 | 0 |
| 177 | 0.02627 | 0.039764 | 0 |
| 178 | 0.02417 | 0.03804 | 0 |
| 179 | 0.02215 | 0.036323 | 0 |
| 180 | 0.02022 | 0.034622 | 0 |
| 181 | 0.01837 | 0.032929 | 0 |
| 182 | 0.01662 | 0.031264 | 0 |
| 183 | 0.01495 | 0.029608 | 0 |
| 184 | 0.01337 | 0.027973 | 0 |
| 185 | 0.01187 | 0.026346 | 0 |
| 186 | 0.01047 | 0.024753 | 0 |
| 187 | 0.00915 | 0.023169 | 0 |
| 188 | 0.00792 | 0.021609 | 0 |
| 189 | 0.00678 | 0.020072 | 0 |
| 190 | 0.00572 | 0.018547 | 0 |
| 191 | 0.00476 | 0.017056 | 0 |
| 192 | 0.00388 | 0.015569 | 0 |
| 193 | 0.00309 | 0.014094 | 0 |
| 194 | 0.00238 | 0.012606 | 0 |
| 195 | 0.00177 | 0.011096 | 0 |
| 196 | 0.00124 | 0.009445 | 0 |
| 197 | 0.0008 | 0.007656 | 0 |
| 198 | 0.00044 | 0.005684 | 0 |
| 199 | 0.00018 | 0.003633 | 0 |
| 200 | 0 | 0 | 0 |
| 201 | 0.000245 | −0.002549 | 0 |
| 202 | 0.000678 | −0.004584 | 0 |
| 203 | 0.000925 | −0.005491 | 0 |
| 204 | 0.006381 | −0.01743 | 0 |
| 205 | 0.016792 | −0.031576 | 0 |
| 206 | 0.031367 | −0.046492 | 0 |
| 207 | 0.049641 | −0.061655 | 0 |
| 208 | 0.07124 | −0.076713 | 0 |
| 209 | 0.09561 | −0.091093 | 0 |
| 210 | 0.122438 | −0.104309 | 0 |
| 211 | 0.151203 | −0.115726 | 0 |
| 212 | 0.181669 | −0.124578 | 0 |
| 213 | 0.213672 | −0.130235 | 0 |
| 214 | 0.247139 | −0.13131 | 0 |
| 215 | 0.283942 | −0.127757 | 0 |
| 216 | 0.323782 | −0.120293 | 0 |
| 217 | 0.367326 | −0.109093 | 0 |
| 218 | 0.414593 | −0.095249 | 0 |
| 219 | 0.465255 | −0.07966 | 0 |
| 220 | 0.518814 | −0.063236 | 0 |
| 221 | 0.574574 | −0.046894 | 0 |
| 222 | 0.631638 | −0.03152 | 0 |
| 223 | 0.688908 | −0.017904 | 0 |
| 224 | 0.745125 | −0.006688 | 0 |
| 225 | 0.798908 | 0.001698 | 0 |
| 226 | 0.84883 | 0.00707 | 0 |
| 227 | 0.893492 | 0.009517 | 0 |
| 228 | 0.931609 | 0.009395 | 0 |
| 229 | 0.962086 | 0.007279 | 0 |
| 230 | 0.983819 | 0.003889 | 0 |
| 231 | 0.996132 | 0.001014 | 0 |
| 232 | 1 | 0 | 0 |
References
- Shalby, M.; A Salah, A.; A Matarneh, G.; Marashli, A.; Gommaa, M.R. An investigation of a 3D printed micro-wind turbine for residential power production. Int. J. Renew. Energy Dev. 2023, 12, 550–559. [Google Scholar] [CrossRef]
- Shalby, M.; Elhanafi, A.; Walker, P.; Dorrell, D.G.; Salah, A.; Gomaa, M.R. Experimental investigation of the small-scale fixed multi-chamber OWC Device. Chin. J. Mech. Eng. 2021, 34, 124. [Google Scholar] [CrossRef]
- Chen, H.; Tang, T.; Ait-Ahmed, N.; Benbouzid, M.E.H.; Machmoum, M.; Zaim, M.E.-H. Attraction, challenge and current status of marine current energy. IEEE Access 2018, 6, 12665–12685. [Google Scholar] [CrossRef]
- Wang, X.; Yang, W.; Qin, B.; Wei, K.; Ma, Y.; Zhang, D. Intelligent monitoring of photovoltaic panels based on infrared detection. Energy Rep. 2022, 8, 5005–5015. [Google Scholar] [CrossRef]
- Goundar, J.N.; Ahmed, M.R. Marine current energy resource assessment and design of a marine current turbine for Fiji. Renew. Energy 2014, 65, 14–22. [Google Scholar] [CrossRef]
- EnergyBC. Estimate of Global Potential Tidal Resources. Available online: http://www.energybc.ca/cache/tidal3/tidalreserves.html (accessed on 11 December 2023).
- Zhou, Z.; Benbouzid, M.; Charpentier, J.-F.; Scuiller, F.; Tang, T. Developments in large marine current turbine technologies–A review. Renew. Sustain. Energy Rev. 2017, 71, 852–858. [Google Scholar] [CrossRef]
- Betz, A. Introduction to the Theory of Flow Machines; Elsevier: Amsterdam, The Netherlands, 2014. [Google Scholar]
- Lanzafame, R.; Messina, M. Fluid dynamics wind turbine design: Critical analysis, optimization and application of BEM theory. Renew. Energy 2007, 32, 2291–2305. [Google Scholar] [CrossRef]
- Glauert, H. Aerodynamic Theory; Durand, W.F., Ed.; Chapter XI. Division, L. airplanes propellers, reprinted; Dover: New York, NY, USA, 1963; Volume 4, p. 191e5. [Google Scholar]
- Song, F.; Ni, Y.; Tan, Z. Optimization design, modeling and dynamic analysis for composite wind turbine blade. Procedia Eng. 2011, 16, 369–375. [Google Scholar] [CrossRef]
- Li, Y.; Wei, K.; Yang, W.; Wang, Q. Improving wind turbine blade based on multi-objective particle swarm optimization. Renew. Energy 2020, 161, 525–542. [Google Scholar] [CrossRef]
- Maalawi, K.Y.; Badawy MT, S. A direct method for evaluating performance of horizontal axis wind turbines. Renew. Sustain. Energy Rev. 2001, 5, 175–190. [Google Scholar] [CrossRef]
- Bavanish, B.; Thyagarajan, K. Optimization of power coefficient on a horizontal axis wind turbine using bem theory. Renew. Sustain. Energy Rev. 2013, 26, 169–182. [Google Scholar] [CrossRef]
- Siddiqui, N.A.; Asrar, W.; Sulaeman, E. Literature review: Biomimetic and conventional aircraft wing tips. Int. J. Aviat. Aeronaut. Aerosp. 2017, 4, 6. [Google Scholar] [CrossRef]
- Chen, K.; Yao, W.; Wei, J.; Gao, R.; Li, Y. Bionic coupling design and aerodynamic analysis of horizontal axis wind turbine blades. Energy Sci. Eng. 2021, 9, 1826–1838. [Google Scholar] [CrossRef]
- Gao, R.; Chen, K.; Li, Y.; Yao, W. Investigation on aerodynamic performance of wind turbine blades coupled with airfoil and herringbone groove structure. J. Renew. Sustain. Energy 2021, 13, 053301. [Google Scholar] [CrossRef]
- Lin, Y.; Chiu, P. Influence of leading-edge protuberances of fx63 airfoil for horizontal-axis wind turbine on power performance. Sustain. Energy Technol. Assess. 2020, 38, 100675. [Google Scholar] [CrossRef]
- Zhu, X.; Guo, Z.; Zhang, Y.; Song, X.; Cai, C.; Kamada, Y.; Maeda, T.; Li, Q. Numerical study of aerodynamic characteristics on a straight-bladed vertical axis wind turbine with bionic blades. Energy 2022, 239, 122453. [Google Scholar] [CrossRef]
- Ke, W.; Hashem, I.; Zhang, W.; Zhu, B. Influence of leading-edge tubercles on the aerodynamic performance of a horizontal-axis wind turbine: A numerical study. Energy 2022, 239, 122186. [Google Scholar] [CrossRef]
- Lv, J.; Yang, W.; Zhang, H.; Liao, D.; Ren, Z.; Chen, Q. A feasibility study to reduce infrasound emissions from existing wind turbine blades using a biomimetic technique. Energies 2021, 14, 4923. [Google Scholar] [CrossRef]
- Videler, J.; Stamhuis, E.; Povel, G. Leading-edge vortex lifts swifts. Science 2004, 306, 1960–1962. [Google Scholar] [CrossRef]
- Larwood, S.; Van Dam, C.P.; Schow, D. Design studies of swept wind turbine blades. Renew. Energy 2014, 71, 563–571. [Google Scholar] [CrossRef]
- Ikeda, T.; Tanaka, H.; Yoshimura, R.; Noda, R.; Fujii, T.; Liu, H. A robust biomimetic blade design for micro wind turbines. Renew. Energy 2018, 125, 155–165. [Google Scholar] [CrossRef]
- Hua, X.; Zhang, C.; Wei, J.; Hu, X.; Wei, H. Wind turbine bionic blade design and performance analysis. J. Vis. Commun. Image Represent. 2019, 60, 258–265. [Google Scholar] [CrossRef]
- Gözcü, O.; Kim, T.; Verelst, D.R.; McWilliam, M.K. Swept Blade Dynamic Investigations for a 100 kW Small Wind Turbine. Energies 2022, 15, 3005. [Google Scholar] [CrossRef]
- Fritz, E.K.; Ferreira, C.; Boorsma, K. An efficient blade sweep correction model for blade element momentum theory. Wind Energy 2022, 25, 1977–1994. [Google Scholar] [CrossRef]
- Shi, W.; Rosli, R.; Atlar, M.; Norman, R.; Wang, D.; Yang, W. Hydrodynamic performance evaluation of a tidal turbine with leading-edge tubercles. Ocean Eng. 2016, 117, 246–253. [Google Scholar] [CrossRef]
- Yang, W.; Alexandridis, T.; Tian, W. Numerical research of the effect of surface biomimetic features on the efficiency of tidal turbine blades. Energies 2018, 11, 1014. [Google Scholar] [CrossRef]
- Liu, S.; Zhang, J.; Sun, K.; Guo, Y.; Guan, D. Influence of swept blades on the performance and hydrodynamic characteristics of a bidirectional horizontal-axis tidal turbine. J. Mar. Sci. Eng. 2022, 10, 365. [Google Scholar] [CrossRef]
- Singh, P.; Choi, Y. Shape design and numerical analysis on a 1 MW tidal current turbine for the south-western coast of Korea. Renew. Energy 2014, 68, 485–493. [Google Scholar] [CrossRef]
- Sun, Z.; Li, D.; Mao, Y.; Feng, L.; Zhang, Y.; Liu, C. Anti-cavitation optimal design and experimental research on tidal turbines based on improved inverse BEM. Energy 2022, 239, 122263. [Google Scholar] [CrossRef]
- Kim, S.; Singh, P.; Hyum, B.; Lee, Y.; Choi, Y. A study on the floating bridge type horizontal axis tidal current turbine for energy independent islands in Korea. Renew. Energy 2017, 112, 35–43. [Google Scholar] [CrossRef]
- Omelyanyuk, M.; Ukolov, A.; Pakhlyan, I.; Bukharin, N.; El Hassan, M. Experimental and numerical study of cavitation number limitations for hydrodynamic cavitation inception prediction. Fluids 2022, 7, 198. [Google Scholar] [CrossRef]
- Picanço, H.P.; Kleber Ferreira de Lima, A.; Dias do Rio Vaz, D.A.T.; Lins, E.F.; Pinheiro Vaz, J.R. Cavitation inception on hydrokinetic turbine blades shrouded by diffuser. Sustainability 2022, 14, 7067. [Google Scholar] [CrossRef]


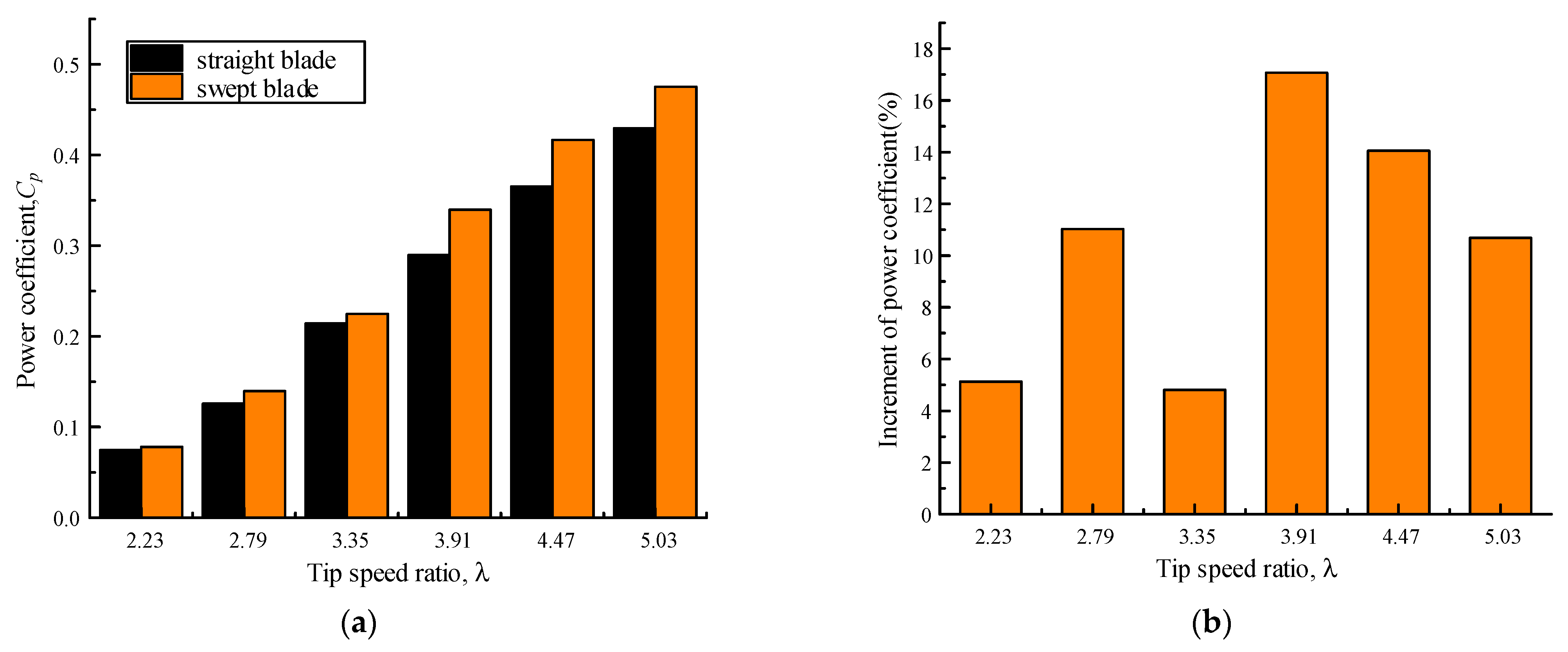



| Element No. | r/R | Twist Angle () | Span (m) | Chord Length (m) |
|---|---|---|---|---|
| 1 | 0.10625 | circular | 0.340 | 0.240 |
| 2 | 0.23500 | 19.0 | 0.752 | 0.552 |
| 3 | 0.32000 | 14.0 | 1.024 | 0.478 |
| 4 | 0.4050 | 10.0 | 1.296 | 0.412 |
| 5 | 0.4900 | 7.5 | 1.568 | 0.357 |
| 6 | 0.5750 | 5.5 | 1.840 | 0.313 |
| 7 | 0.6600 | 4.0 | 2.112 | 0.278 |
| 8 | 0.7450 | 2.5 | 2.384 | 0.249 |
| 9 | 0.8300 | 1.5 | 2.656 | 0.223 |
| 10 | 0.9150 | 0.4 | 2.928 | 0.194 |
| 11 | 1.0000 | 0.0 | 3.200 | 0.134 |
| Parameters | Value |
|---|---|
| Number of blades | 3 |
| Length of blades | 2.96 m |
| Rotor diameter | 6.4 m |
| Hub diameter | 0.48 m |
| Rotational speed | 17.9 rpm |
| Rated velocity of the water flow | 1.2 m/s |
| Rated power | 10 kW |
| Power coefficient | 0.4 |
| Rated tip speed ratio | 5 |
| Azimuth and Location | The Area Enclosed by the Pressure Coefficient Curve | Area Increment (%) | ||
|---|---|---|---|---|
| () | (m) | Straight Blade | Swept Blade | |
| 0 | 2.792 | 13.93257 | 15.24226 | 9.4002 |
| 2.928 | 9.62108 | 15.62383 | 62.3916 | |
| 3.064 | 10.11471 | 17.91881 | 77.1559 | |
| 120 | 2.792 | 14.47556 | 17.3614 | 19.9359 |
| 2.928 | 10.74689 | 19.29826 | 79.5706 | |
| 3.064 | 11.24235 | 16.53381 | 47.0672 | |
| 240 | 2.792 | 14.8018 | 17.12875 | 15.7207 |
| 2.928 | 9.18577 | 16.6376 | 81.1236 | |
| 3.064 | 11.85701 | 16.2461 | 37.0168 | |
| (m/s) | (rpm) | Tip Speed Ratio |
|---|---|---|
| 1.2 | 8 | 2.23 |
| 10 | 2.79 | |
| 12 | 3.35 | |
| 14 | 3.91 | |
| 16 | 4.47 | |
| 18 | 5.03 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, Y.; Yang, W.; Wei, K.; Chen, Y.; Zou, H. Enhancing Efficiency and Reliability of Tidal Stream Energy Conversion through Swept-Blade Design. Energies 2024, 17, 334. https://doi.org/10.3390/en17020334
Zheng Y, Yang W, Wei K, Chen Y, Zou H. Enhancing Efficiency and Reliability of Tidal Stream Energy Conversion through Swept-Blade Design. Energies. 2024; 17(2):334. https://doi.org/10.3390/en17020334
Chicago/Turabian StyleZheng, Yangyang, Wenxian Yang, Kexiang Wei, Yanling Chen, and Hongxiang Zou. 2024. "Enhancing Efficiency and Reliability of Tidal Stream Energy Conversion through Swept-Blade Design" Energies 17, no. 2: 334. https://doi.org/10.3390/en17020334
APA StyleZheng, Y., Yang, W., Wei, K., Chen, Y., & Zou, H. (2024). Enhancing Efficiency and Reliability of Tidal Stream Energy Conversion through Swept-Blade Design. Energies, 17(2), 334. https://doi.org/10.3390/en17020334








