Analysis of the Arc Quenching System of an Arrester Operation Based on a Flow Ultrasound Generator
Abstract
1. Introduction
2. Methods
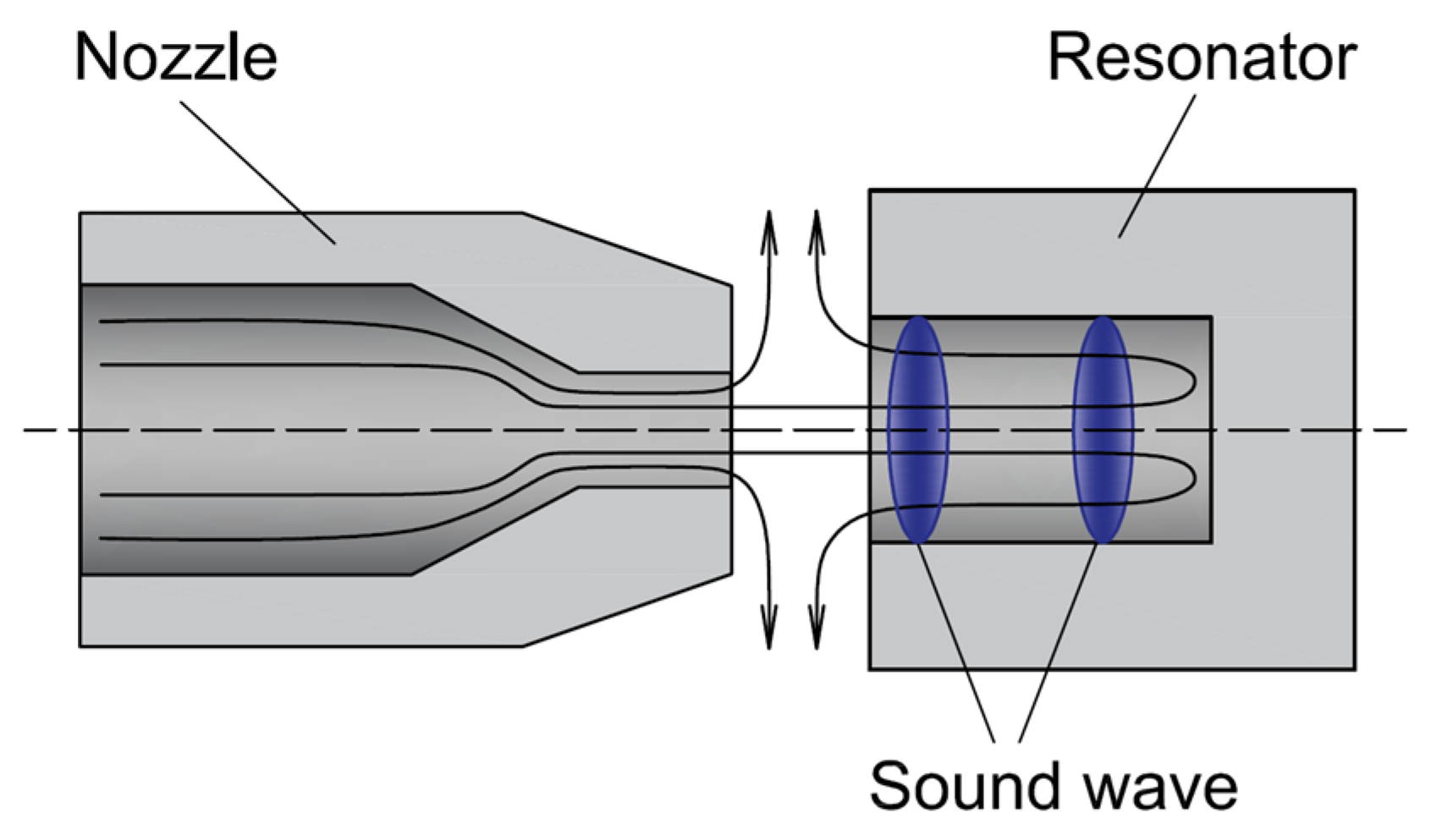
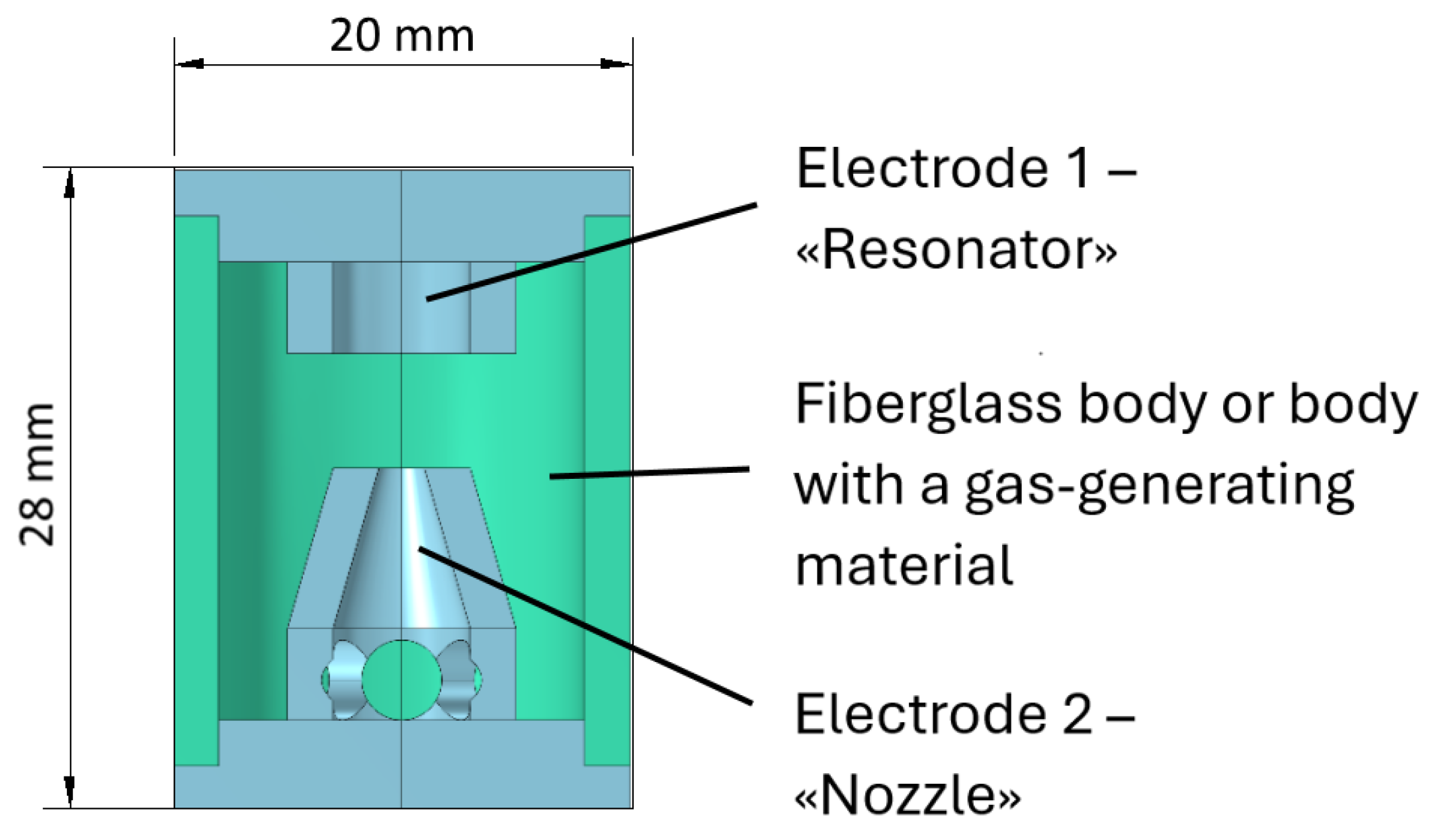
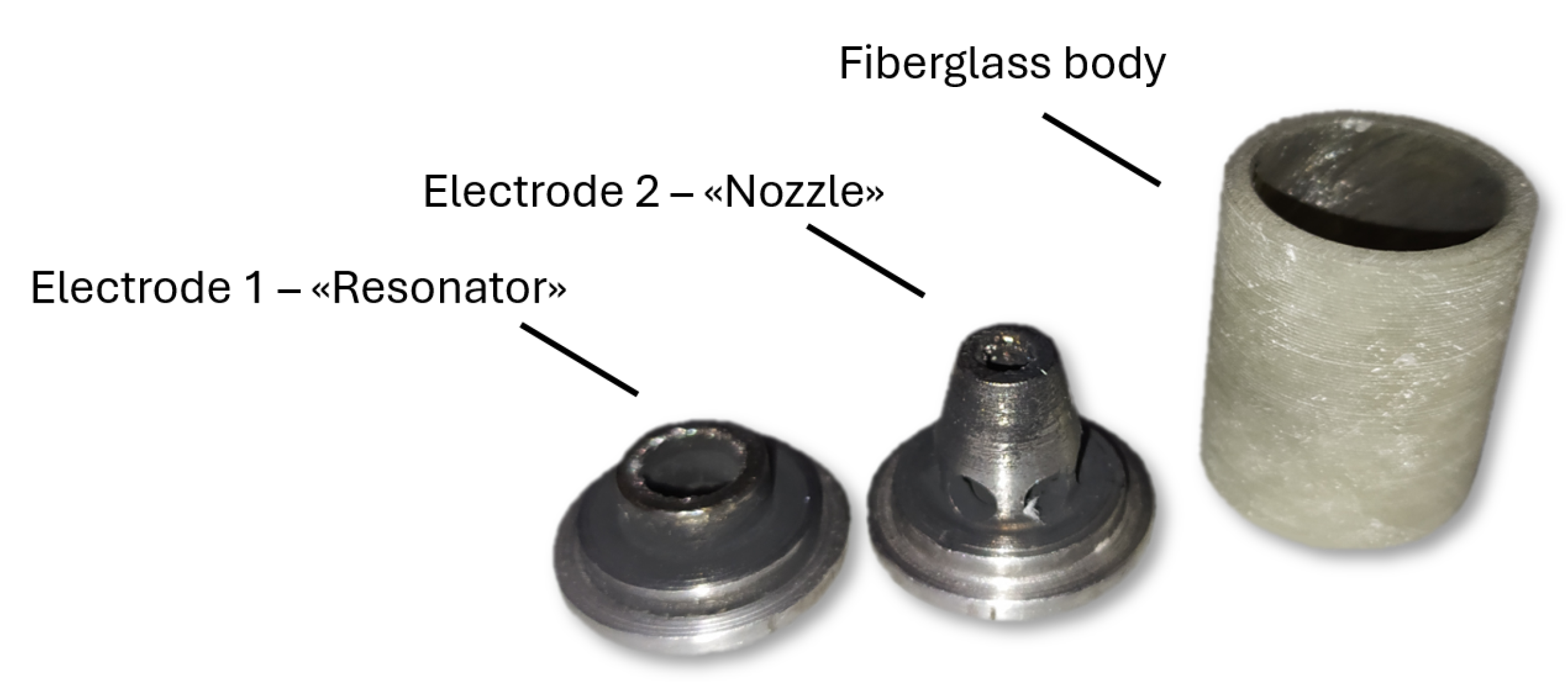
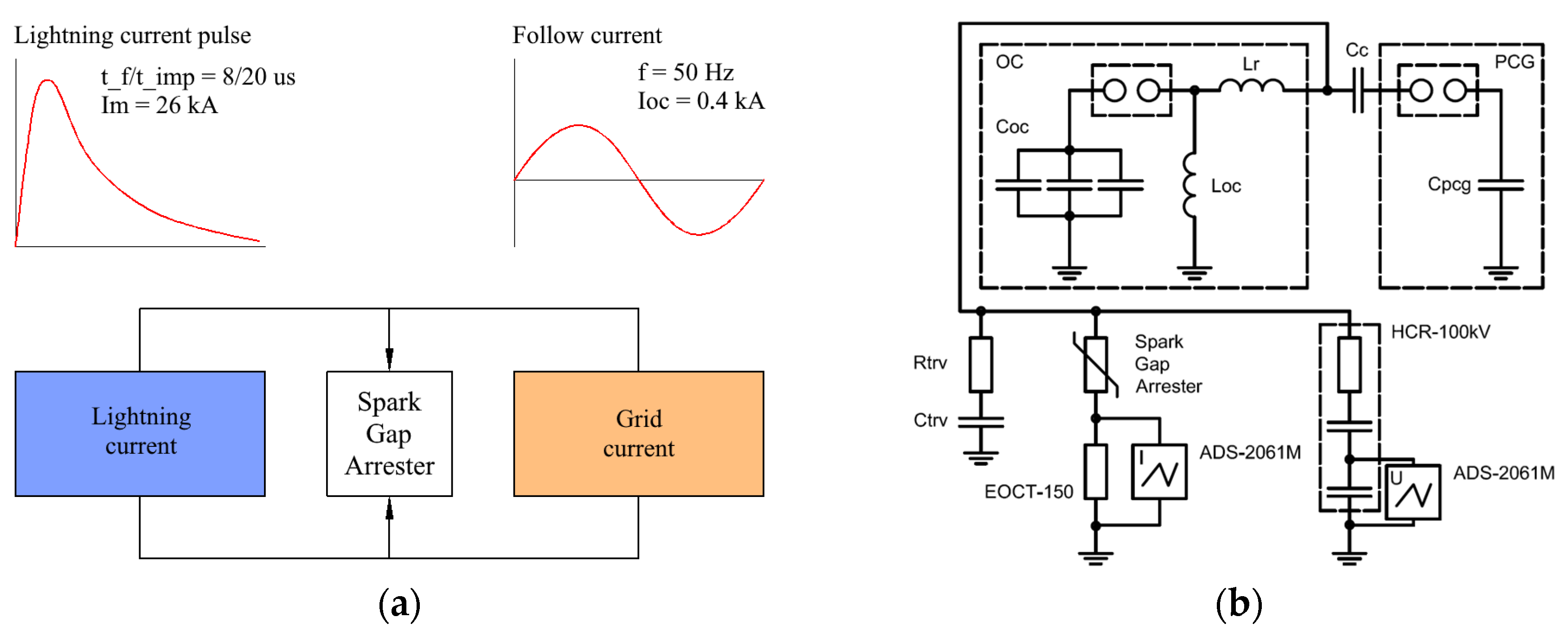
2.1. Experimental Investigation of a Spark Gap Arrester with the Design Features of Ultrasound Generator
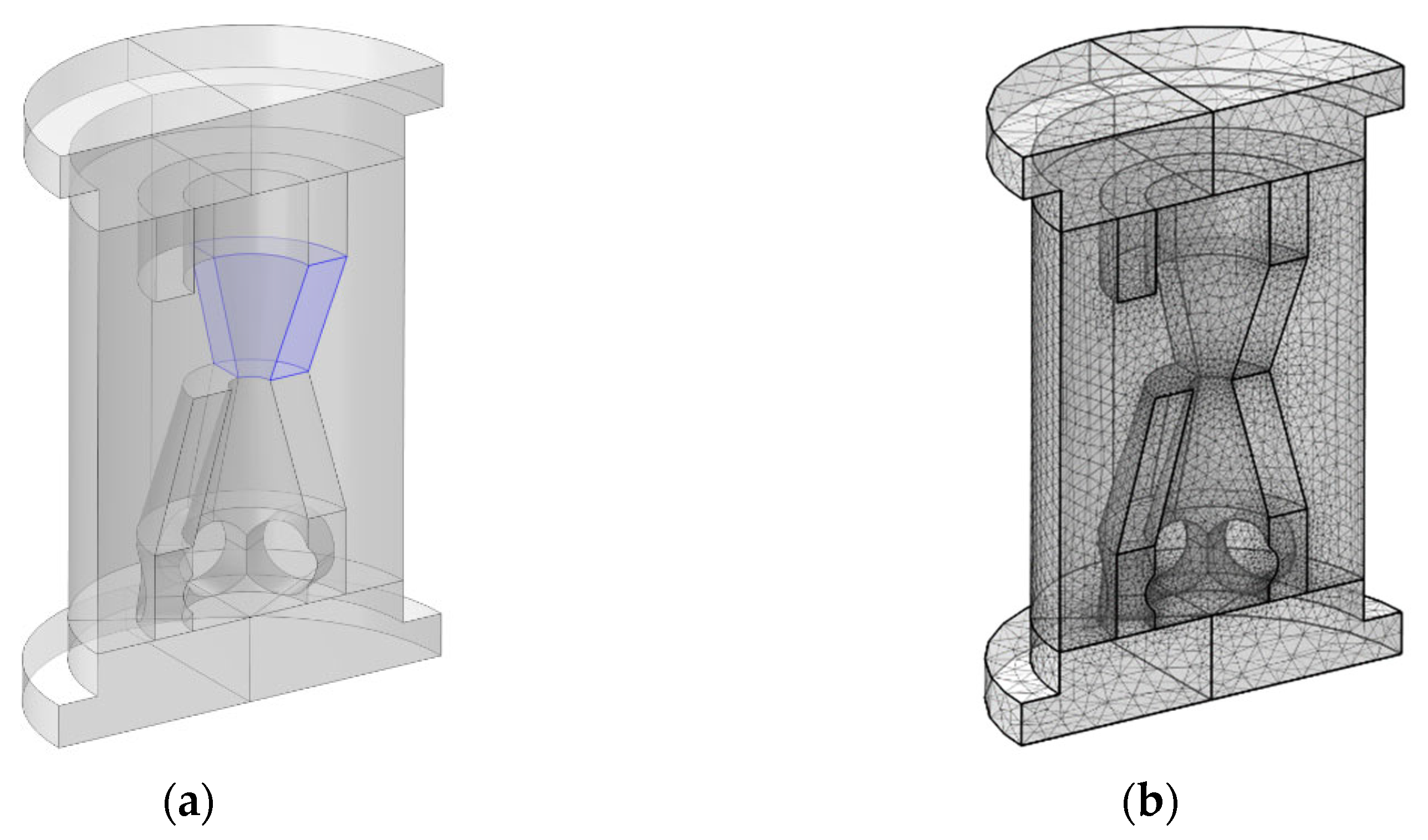
2.2. Numerical Modeling of the Spark Gap Arrester
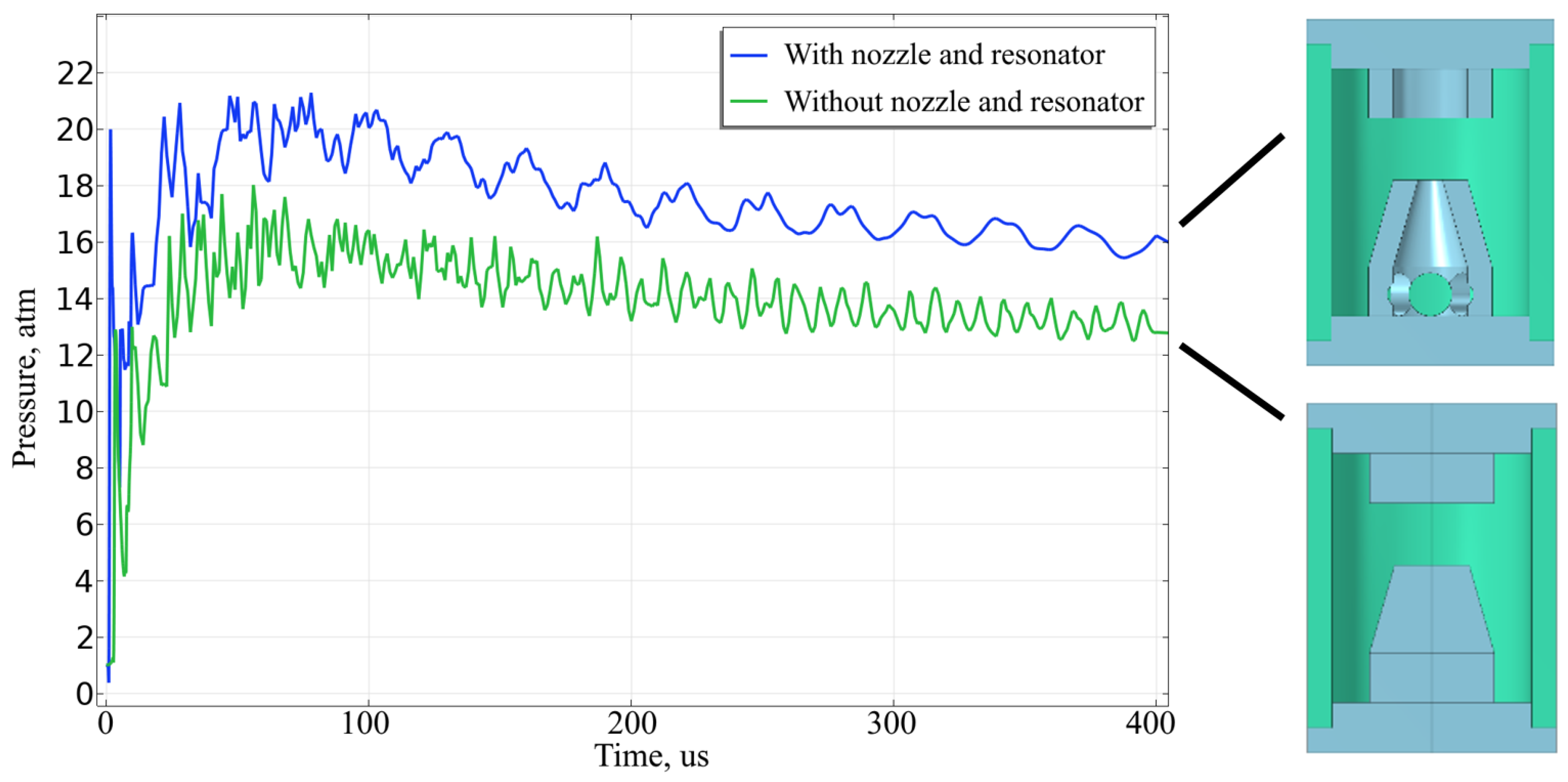
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Sadkhan, M.M. Application of surge arresters in low voltage network supply to reduce lightning overvoltages. In Proceedings of the Fifth International Engineering Conference on Developments in Civil & Computer Engineering Applications (IEC2019), Erbil, Iraq, 23–25 June 2019; pp. 74–78. [Google Scholar] [CrossRef]
- Refiasto, S.; Denov, B.; Suwarno, S.; Zoro, R. Installation of multi chamber arrester to improve lightning reliability performance of distribution lines system in oil and gas area. In Proceedings of the 3rd International Conference on High Voltage Engineering and Power Systems (ICH-VEPS), Bandung, Indonesia, 5–6 October 2023; pp. 258–262. [Google Scholar] [CrossRef]
- Wu, D.; Ji, Z.; Wang, J. Simulation and experimental analysis of multi-chamber arc-quenching arresters (MCAA) for 10 kV transmission lines. Energies 2021, 14, 6185. [Google Scholar] [CrossRef]
- Kuan, T.; Chew, K.; Chua, K. Surge Protection Device for Ex Application. Adv. Sci. Technol. Eng. Syst. J. 2020, 5, 768–780. [Google Scholar] [CrossRef]
- Podporkin, G.; Enkin, E.; Kalakutsky, E.; Pilshikov, V.; Sivaev, A. Lightning protection of overhead lines by multi-chamber arresters and insulator-arresters. In Proceedings of the 30th International Symposium on Lightning Protection, Cagliari, Italy, 13–17 September 2010; p. 7845759. [Google Scholar] [CrossRef]
- Kozakov, R.; Khakpour, A.; Gorchakov, S.; Uhrlandt, D.; Ivanov, D.; Murashov, I.; Podporkin, G.; Frolov, V. Investigation of a Multi-Chamber System for Lightning Protection at Overhead Power Lines. Plasma Phys. Technol. 2015, 2, 150–154. [Google Scholar]
- Pinchuk, M.E.; Budin, A.V.; Kumkova, I.I.; Chusov, A.N. Studying energy evolution in the discharge chamber of a multichamber lightning protection system. Tech. Phys. Lett. 2016, 42, 395–398. [Google Scholar] [CrossRef]
- Frolov, V.Y.; Ivanov, D.V.; Belsky, R.A. Increasing of operation security and of breaking capacity of surge arresters. In Proceedings of the IEEE Russia Section Young Researchers in Electrical and Electronic Engineering Conference (ElConRus 2017)f, St. Petersburg/Moscow, Russia, 1–3 February 2017; Volume 7910861, pp. 1520–1523. [Google Scholar] [CrossRef]
- Li, Z.; Fu, J.; Zhou, X.; Feng, Z.; Yao, Y.; Wang, J. Study on arc extinguishing characteristics of multiple series gap under long clearances. AIP Adv. 2022, 12, 055326. [Google Scholar] [CrossRef]
- Chusov, A.N.; Podporkin, G.V.; Chistyakov, A.S.; Apolinskiy, M.I.; Frolov, V.Y.; Ivanov, D.V. The application of gas-generating materials for increase of line lightning protection device efficiency. In Proceedings of the Seminar on Industrial Electronic Devices and Systems (IEDS), St. Petersburg, Russia, 27–28 November 2023; pp. 72–77. [Google Scholar] [CrossRef]
- Chistyakov, A.; Frolov, V.; Zaretskiy, N.; Chusov, A. The investigation of effect of a multi-chamber arrester mechanical properties on the efficiency of arc extinction. In Proceedings of the Conference of Young Researchers in Electrical and Electronic Engineering (ElCon), St. Petersburg, Russia, 29–31 January 2024; pp. 803–805. [Google Scholar] [CrossRef]
- Huang, S.; Wang, J.; Xu, Y.; Li, Z.; Yan, R.; Zhang, Q. The effect of a multi-fracture compression airflow arc-extinguishing structure interrupting the power frequency follow current. AIP Adv. 2020, 10, 035129. [Google Scholar] [CrossRef]
- Chusov, A.; Chystiakov, A.; Apolinskiy, M. Application of numerical simulations for improvement of line lightning protection device efficiency. In Proceedings of the 36th International Conference on Lightning Protections, Cape Town, South Africa, 2–7 October 2022; pp. 468–472. [Google Scholar] [CrossRef]
- Li, Z.; Wang, J.; Zhou, X.; Huang, S.; Xia, Z. Study on impulse quenching based multichamber arc quenching structure. AIP Adv. 2019, 9, 85104. [Google Scholar] [CrossRef]
- Podporkin, G.V.; Enkin, E.Y.; Kalakutsky, E.S.; Pilshikov, V.E.; Sivaev, A.D. Overhead lines lightning protection by multi-chamber arresters and insulator-arresters. IEEE Trans. Power Deliv. 2011, 26, 214–221. [Google Scholar] [CrossRef]
- Frolov, V.; Ivanov, D.; Murashov, I.; Sivaev, A. Mathematical simulation of processes in discharge chamber of multi-chamber system for lightning protection at overhead power lines. In Proceedings of the IEEE North West Russia Section Young Researchers in Electrical and Electronic Engineering Conference (ELCONRUSNW 2016), St. Petersburg, Russia, 2–3 February 2016; pp. 562–565. [Google Scholar] [CrossRef]
- Guo, Z.; Long, X.; Qian, Z.; Qiu, N. Three dimensional simulation of the arc inside an insulator-arrester with a multichamber system. AIP Adv. 2016, 6, 45117. [Google Scholar] [CrossRef]
- Frolov, V.Y.; Ivanov, D.V.; Podporkin, G.V.; Sivaev, A.D. Development of mathematical model of processes in multi-chamber arrester for identification of criteria of arc extinction. In Proceedings of the 2017 International Symposium on Lightning Protection (XIV SIPDA 2017), Natal, Brazil, 2–6 October 2017; pp. 240–243. [Google Scholar] [CrossRef]
- Chusov, A.; Rodikova, E.; Murmann, M.; Nordborg, H.; Fuchs, R. A review of progress towards simulation of arc quenching in lightning protection devices based on multi chamber systems. Plasma Phys. Technol. 2017, 4, 273–276. [Google Scholar] [CrossRef]
- Frolov, V.; Ivanov, D.; Sivaev, A. Simulation of arc extinction in discharge chambers of lightning protection equipment. In Proceedings of the 10th International Scientific Symposium on Electrical Power Engineering (ELEKTROENERGETIKA 2019), Stara Lesna, Slovakia, 16–18 September 2019; pp. 143–146. [Google Scholar]
- Frolov, V.; Ivanov, D.; Sivaev, A.; Chusov, A. Development of two-temperature mathematical model of processes in discharge chamber of multi-chamber arrester operating in conditions of mountain areas. Plasma Phys. Technol. 2019, 6, 135–139. [Google Scholar] [CrossRef]
- Liu, Y.; Wu, G.; Liu, K.; Guo, Y.; Zhang, X.; Shi, C. Study on the arc motion characteristics of multi-chamber arrester based on 3D model. IEEE Access 2020, 8, 90035–90041. [Google Scholar] [CrossRef]
- Frolov, V.; Ivanov, D.; Sivaev, A.; Chusov, A.; Chistyakov, A.; Rogozhina, A. Mathematical Model of Arc Quenching in the Adjacent Chambers of an RMKE-10 Multichamber Lightning Arrester. Elektrichestvo 2022, 7, 40–51. [Google Scholar] [CrossRef]
- Frolov, V.Y.; Ivanov, D.V.; Sivaev, A.D.; Chusov, A.N.; Botvinkin, I.D. Improved mathematical model of the operation of two adjacent chambers of the RMKE-10 multi-chamber arrester for lightning protection of overhead power lines. In Proceedings of the Seminar on Industrial Electronic Devices and Systems (IEDS), St. Petersburg, Russia, 27–28 November 2023; pp. 89–93. [Google Scholar] [CrossRef]
- Chusov, A.; Podporkin, G.; Pinchuk, M.; Ivanov, D.; Murashov, I.; Frolov, V. Development of a physical 2-D model for arc quenching chamber of lightning protection multi-chamber systems. In Proceedings of the 33rd International Conference on Lightning Protection (ICLP 2016 33), Estoril, Portugal, 25–30 September 2016; p. 7791509. [Google Scholar] [CrossRef]
- Murashov, I.V.; Frolov, V.Y.; Uhrlandt, D.; Gortschakow, S.; Ivanov, D.V.; Sivaev, A.D. Analysis of arc processes in multi-chamber arrester for lightning protection at high-voltage overhead power lines. Plasma Phys. Technol. 2017, 4, 124–128. [Google Scholar] [CrossRef]
- Chusov, A.; Pinchuk, M.E.; Budin, A.V. Investigation of altitude dependence of multi-chamber arrester quenching efficiency. In Proceedings of the 11th Asia-Pacific International Conference on Lightning (APL), Hong Kong, China, 12–14 June 2019. [Google Scholar] [CrossRef]
- Li, Z.; Wang, J.; Zhou, X.; Huang, S.; Yan, R.; Xia, Z. Influence of chamber structure on arc quenching in multigap system. High Volt. 2020, 5, 313–318. [Google Scholar] [CrossRef]
- Chusov, A.; Pinchuk, M.; Ivanov, D.; Frolov, V. Effects of high altitude on multi-chamber arrester performance. In Proceedings of the 35th International Conference on Lightning Protection (ICLP) and XVI International Symposium on Lightning Protection (SIPDA), Colombo, Sri Lanka, 20–26 September 2021. [Google Scholar] [CrossRef]
- Muermann, M.; Chusov, A.; Fuchs, R.; Nefedov, A.; Nordborg, H. Modeling and simulation of the current quenching behaviour of a line lightning protection device. J. Phys. D Appl. Phys. 2017, 50, 105203. [Google Scholar] [CrossRef]
- Sander, C. Entwicklung eines Simulationsmodells für Schaltlichtbögen in Überspannungsableitern. Ph.D. Dissertation, Technische Universität Braunschweig, Braunschweig, Germany, 2020. [Google Scholar]
- Zhang, Y.; Zhang, W.; Ji, J. Study of a Combined Surge Protective Device for a Relay Protection Circuit in a UHV Converter Station. IEEE Access 2020, 8, 159956–159964. [Google Scholar] [CrossRef]
- Meppelink, J.; Drilling, C.; Droldner, M.; Jordan, E.G.; Trinkwald, J. Lightning Arresters with Spark Gaps. Requirements and Future Trends of Development and Application. 2000. Available online: https://www.obo.com.tr/fileadmin/default/Downloads/FUNKENS2.PDF (accessed on 12 August 2024).
- Huo, J.; Selezneva, S.; Jacobs, L.; Cao, Y. Study of Wall Ablation on Low-Voltage Interruption: The Effect of Stefan Flow. J. Appl. Phys. 2019, 125, 213302. [Google Scholar] [CrossRef]
- Fievet, C.; Burrault, M.; Petit, P.; Chevrier, P.; Fleurier, C.; Andre, V. Optical diagnostics and numerical modelling of arc re-strikes in low-voltage circuit breakers. J. Phys. D Appl. Phys. 1997, 30, 2991. [Google Scholar] [CrossRef]
- Rümpler, C. Lichtbogensimulation für Niederspannungsschaltgeräte. Ph.D. Dissertation, Technische Universität Ilmenau, Ilmenau, Germany, 2009. [Google Scholar]
- Eichhoff, D.; Kurz, A.; Kozakov, R.; Gott, G.; Uhrlandt, D.; Schnettler, A. Study of an ablation-dominated arc in a model circuit breaker. J. Phys. D Appl. Phys. 2012, 45, 305204. [Google Scholar] [CrossRef]
- Fuchs, R.; Mürmann, M.; Nordborg, H. Towards an efficient arc simulation framework. Plasma Phys. Technol. 2017, 4, 79–82. [Google Scholar] [CrossRef]
- Rümpler, C.; Stammberger, H.; Zacharias, A. Low–voltage arc simulation with out–gassing polymers. In Proceedings of the2011 IEEE 57th Holm Conference on Electrical Contacts (Holm), Minneapolis, MN, USA, 11–14 September 2011. [Google Scholar] [CrossRef]
- Quéméneur, J.; Lu, J.; Gonzalez, J.; Freton, P. Arc motion in low voltage circuitbreaker (lvcb) experimental and theoretical approaches. Sci. Technol. 2018, 8, 35–45. [Google Scholar] [CrossRef]
- Ehrhardt, A.; Schreiter, S.; Strangfeld, U.; Rock, M. Encapsulated lightning current arrester with spark gap and deion chamber. In Proceedings of the 19th International Symposium on Physics of Switching Arc, Brno, Czech Republic, 5–9 September 2011. [Google Scholar]
- Fujimoto, T.; Ishii, T.; Enomoto, S.; Ishikawa, H. Study on the behavior of fluid acoustic device by numerical simulation. Trans. Jpn. Soc. Mech. Eng. 2023, 89, 1–13. [Google Scholar] [CrossRef]
- Rensong, C.; Guoqing, W.; Xiuhai, C.; Xinjun, G.; Junbo, J.; Yongquan, P. Numerical simulation on the acoustic characteristics of the Hartmann acoustic generator array. In Proceedings of the IEEE Advanced Information Technology, Electronic and Automation Control Conference (IAEAC 2015), Chongqing, China, 19–20 December 2015; pp. 446–450. [Google Scholar] [CrossRef]
- Balek, R.; Pekarek, S. Hartmann ultrasound generator combined with electrical discharge. In Proceedings of the IEEE International Ultrasonics Symposium, Rome, Italy, 20–23 September 2009; pp. 2615–2618. [Google Scholar] [CrossRef]
- D’Angola, A.; Colonna, G.; Gorse, C.; Capitelli, M. Thermodynamic and transport properties in equilibrium air plasmas in a wide pressure and temperature range. Eur. Phys. J. D 2007, 46, 129–150. [Google Scholar] [CrossRef]
- Capitelli, M.; Colonna, G.; D’Angola, A. Fundamental Aspects of Plasma Chemical Physics: Thermodynamics; Springer: New York, NY, USA, 2011. [Google Scholar]
- Capitelli, M.; Bruno, D.; Laricchiuta, A. Fundamental Aspects of Plasma Chemical Physics: Transport; Springer: New York, NY, USA, 2013. [Google Scholar]
- Nordborg, H.; Iordanidis, A.A. Self-Consistent Radiation Based Modelling of Electric Arcs: I. Efficient Radiation Approximations. J. Phys. D Appl. Phys. 2008, 41, 135205. [Google Scholar] [CrossRef]
- Petrenya, Y.K.; Frolov, V.Y.; Ivanov, D.V. Physical and Technical Aspects of Arc Plasma Energy Dissipation; Polytech-Press: St. Petersburg, Russia, 2023. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Apolinskiy, M.I.; Frolov, V.Y.; Sivaev, A.D.; Enkin, E.Y. Analysis of the Arc Quenching System of an Arrester Operation Based on a Flow Ultrasound Generator. Energies 2024, 17, 4975. https://doi.org/10.3390/en17194975
Apolinskiy MI, Frolov VY, Sivaev AD, Enkin EY. Analysis of the Arc Quenching System of an Arrester Operation Based on a Flow Ultrasound Generator. Energies. 2024; 17(19):4975. https://doi.org/10.3390/en17194975
Chicago/Turabian StyleApolinskiy, Matvey I., Vladimir Ya. Frolov, Alexander D. Sivaev, and Evgeniy Y. Enkin. 2024. "Analysis of the Arc Quenching System of an Arrester Operation Based on a Flow Ultrasound Generator" Energies 17, no. 19: 4975. https://doi.org/10.3390/en17194975
APA StyleApolinskiy, M. I., Frolov, V. Y., Sivaev, A. D., & Enkin, E. Y. (2024). Analysis of the Arc Quenching System of an Arrester Operation Based on a Flow Ultrasound Generator. Energies, 17(19), 4975. https://doi.org/10.3390/en17194975





