A Stability Control Method to Maintain Synchronization Stability of Wind Generation under Weak Grid
Abstract
1. Introduction
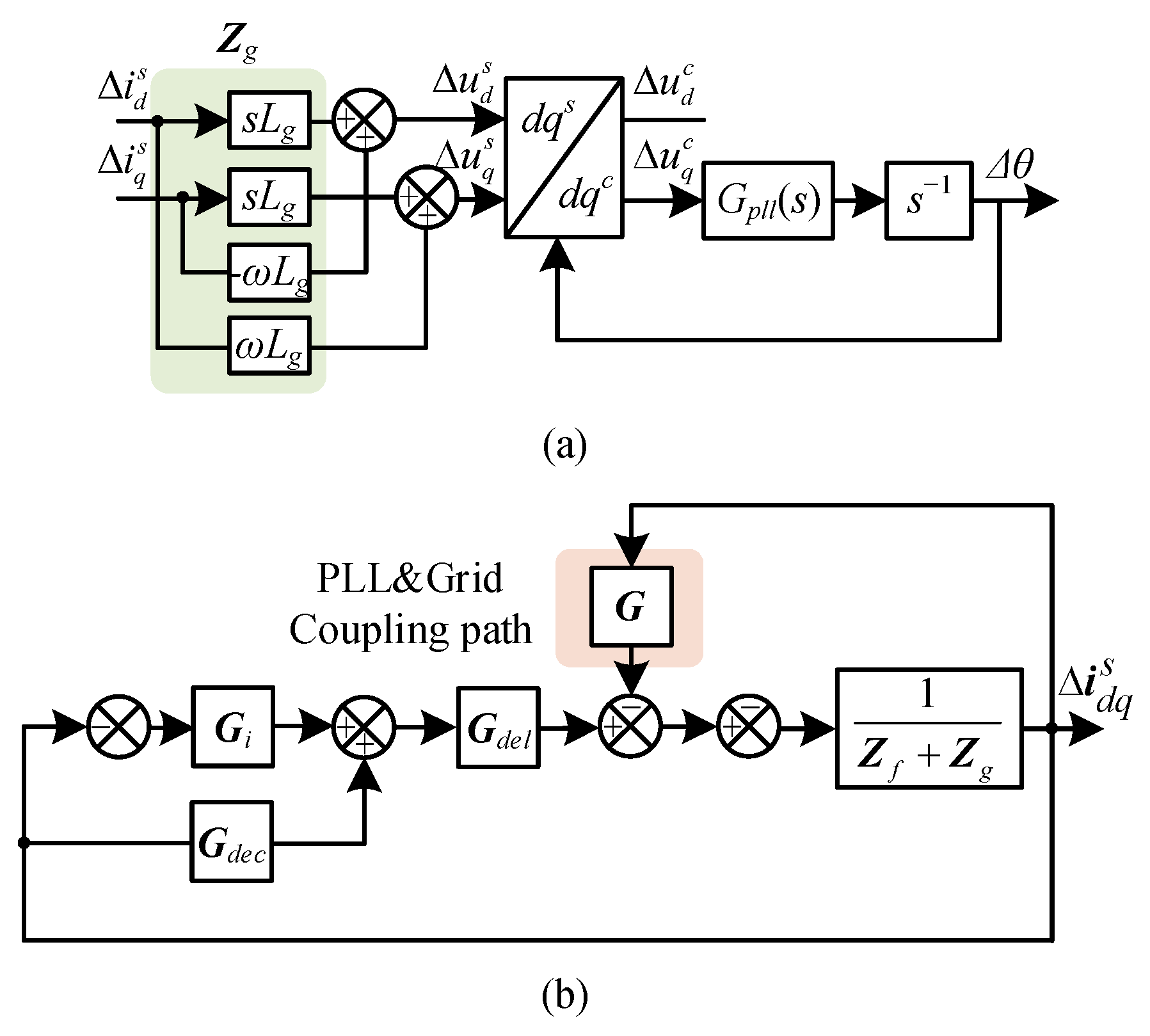
- (a)
- A clear exposition of the coupling mechanism among the PLL, weak grid, and current control is provided, laying the groundwork for forthcoming stability enhancement techniques.
- (b)
- An equivalent parallel resistance compensation method integrated into PLL is proposed to improve synchronization stability. It reshapes the qq-axis impedance and will not decrease the dynamic performance of PLL with a proper design of virtual resistance.
- (c)
- The compensation method demonstrates robustness against system parameter variations and grid impedance measurement errors.
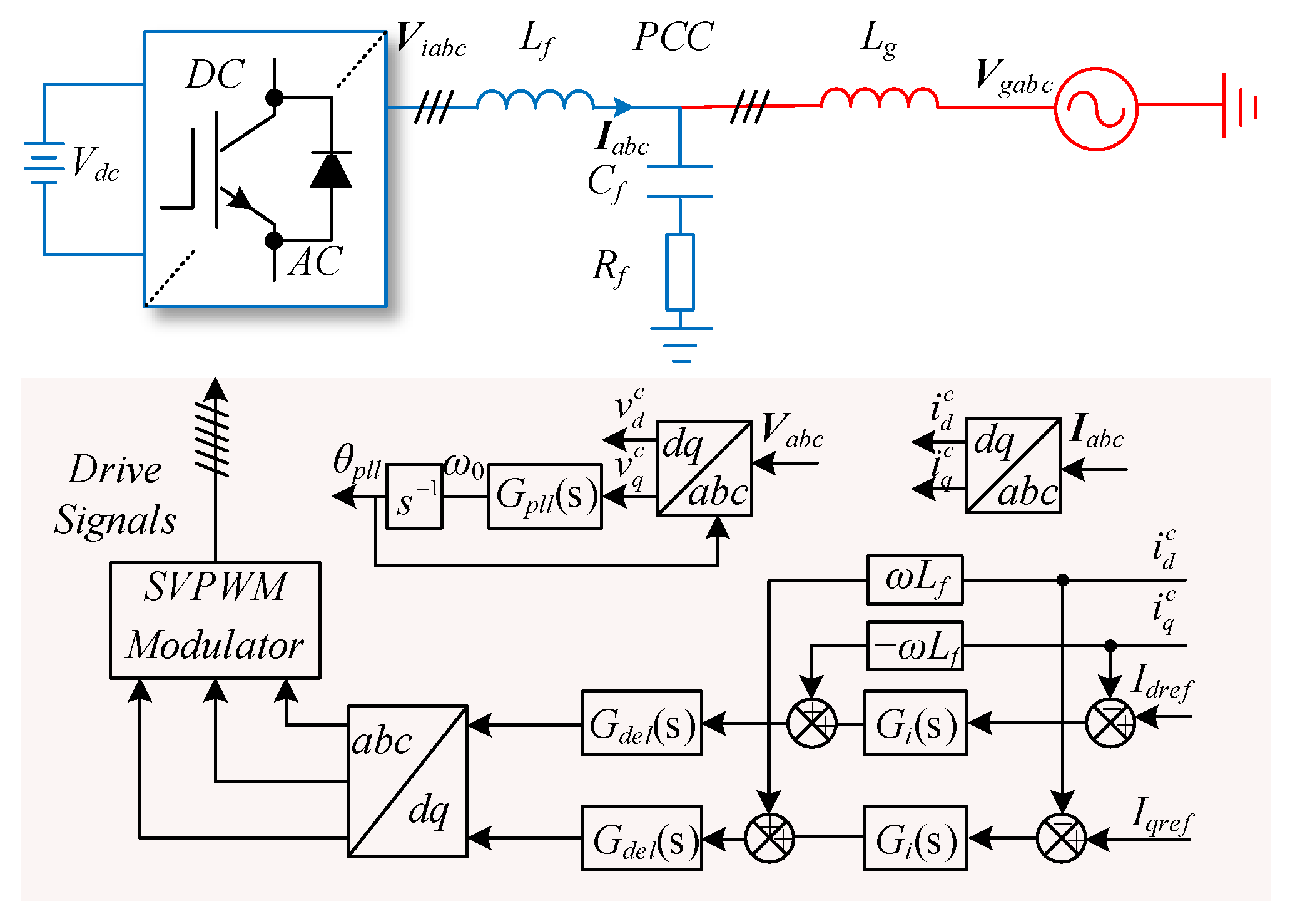
2. Impedance Model and Stability Analysis of the Grid-Connected System
2.1. Description and Model of System
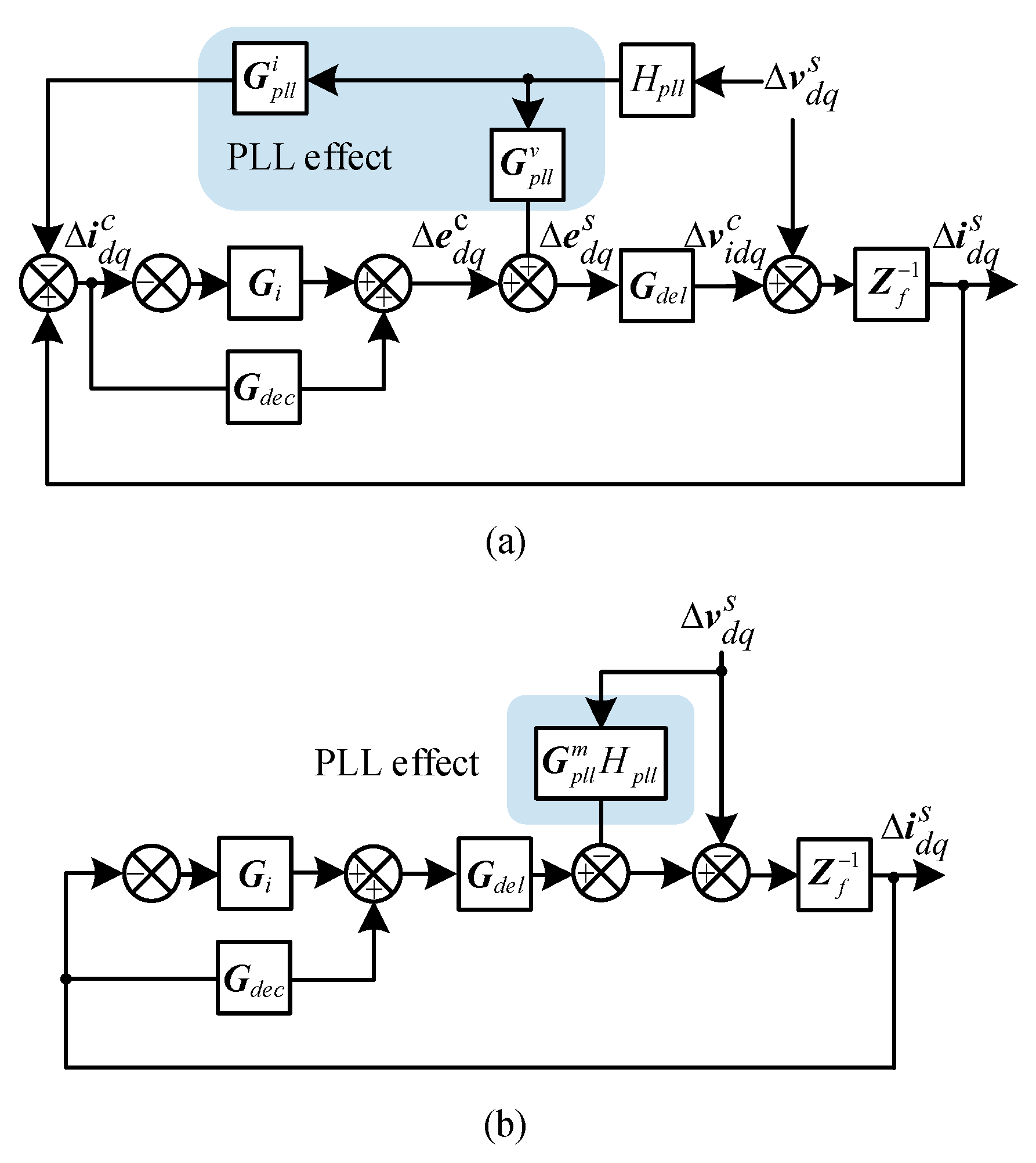
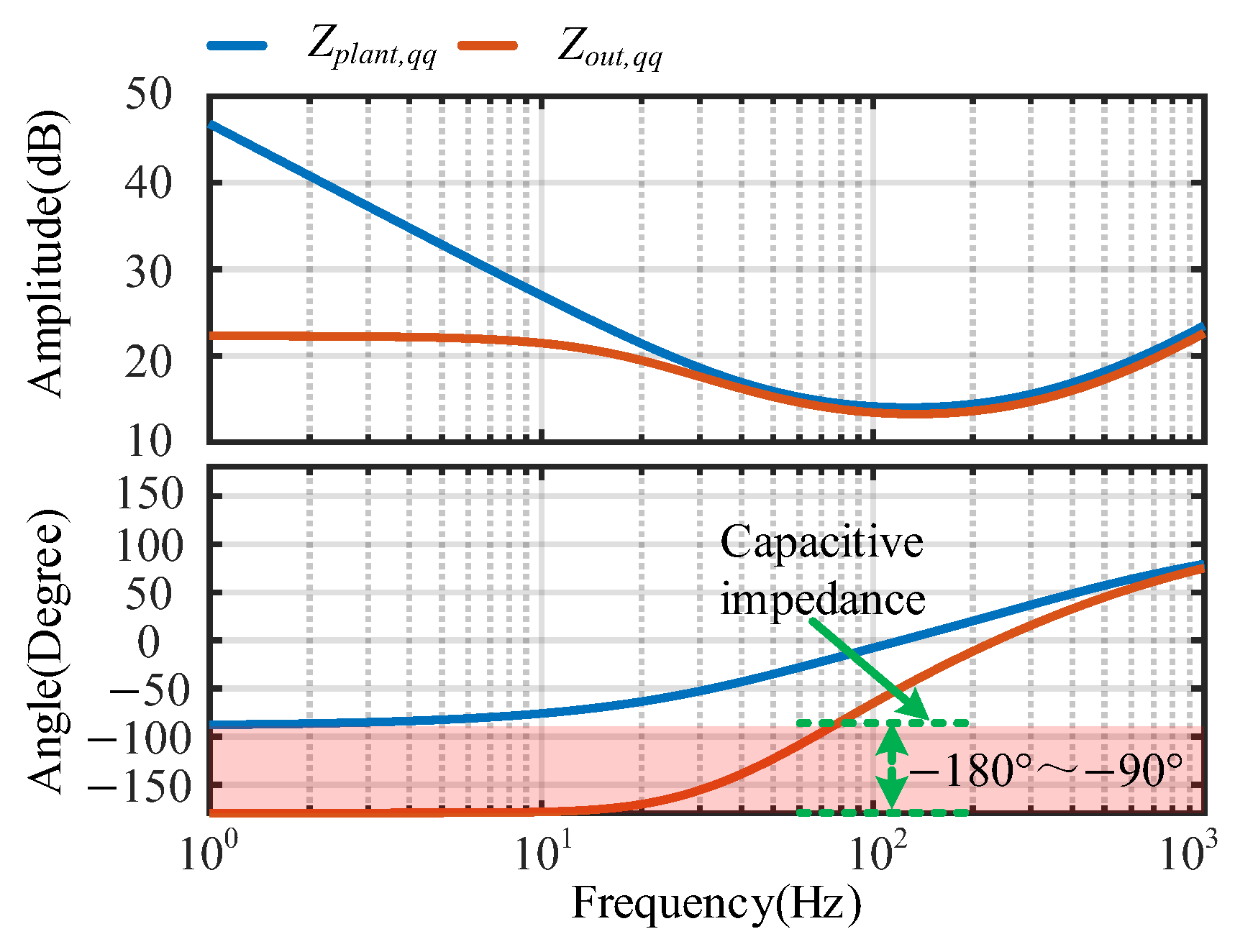
2.2. Stability and the Coupling Mechanism Analysis
3. Analysis and Design of Equivalent Parallel Compensation Method
3.1. Analysis of the Equivalent Parallel Compensation Method
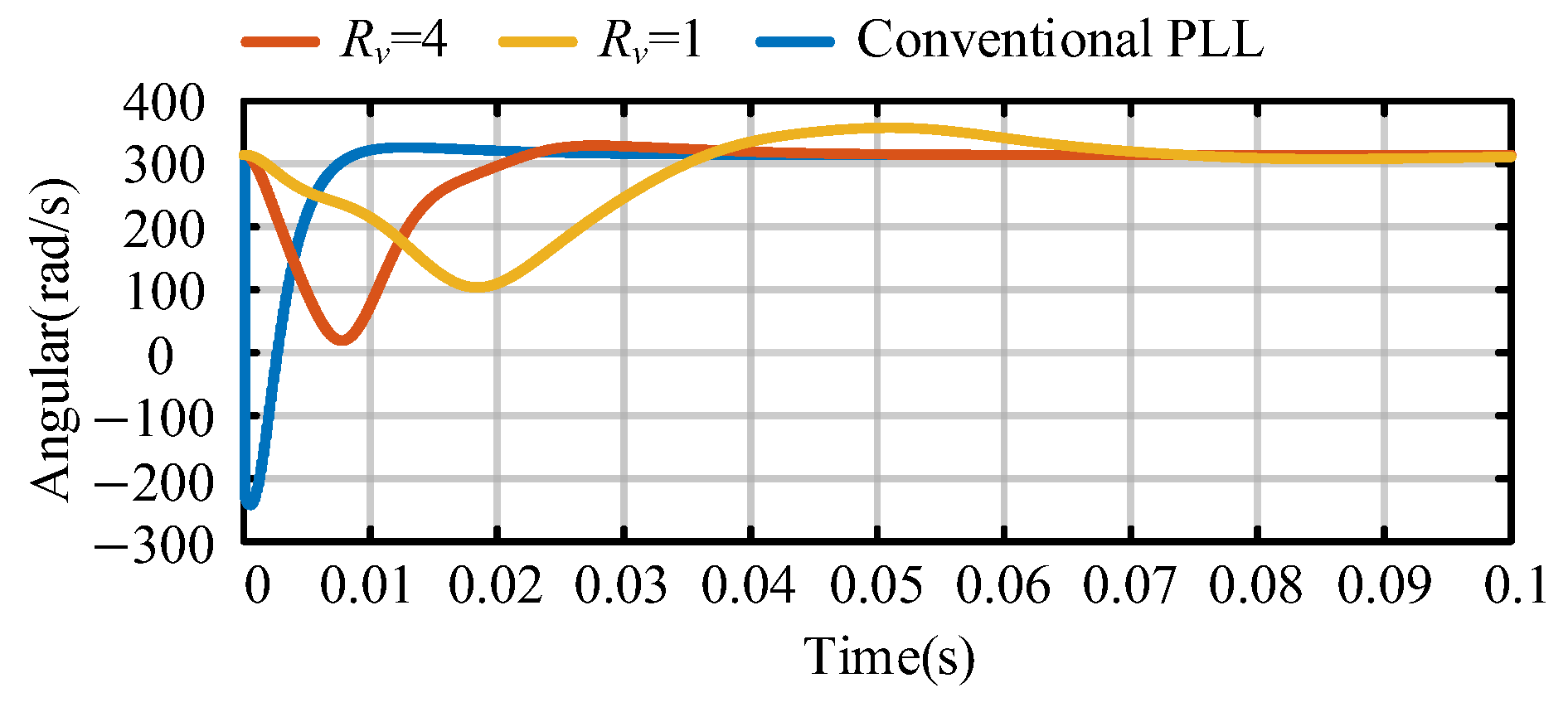
3.2. Dynamic Performance of Compensation Method
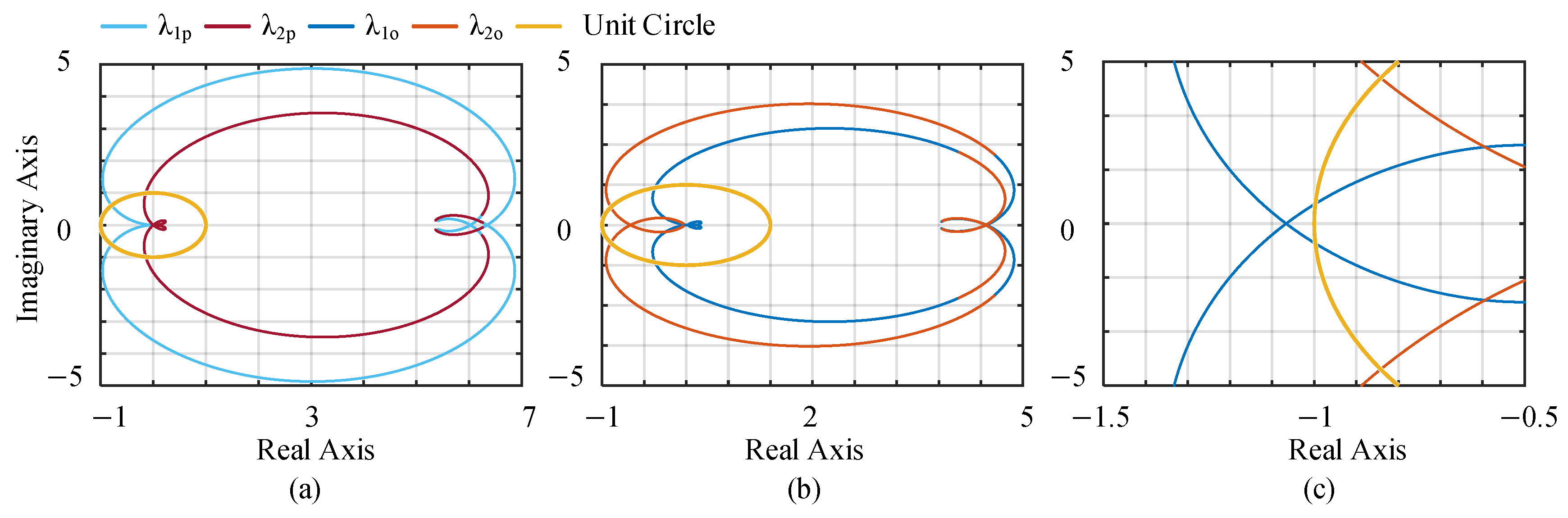
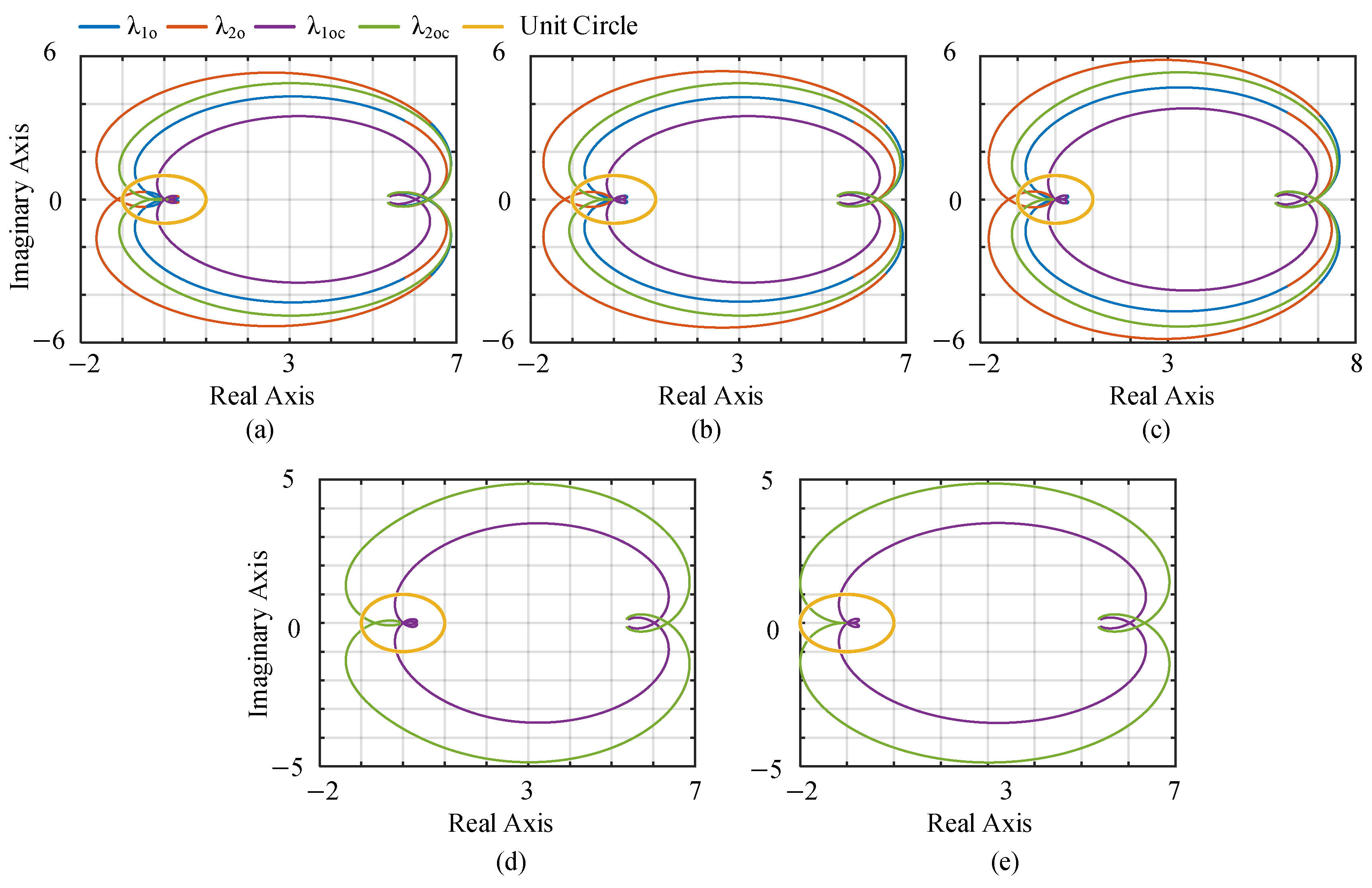
3.3. Design and Stability Analysis
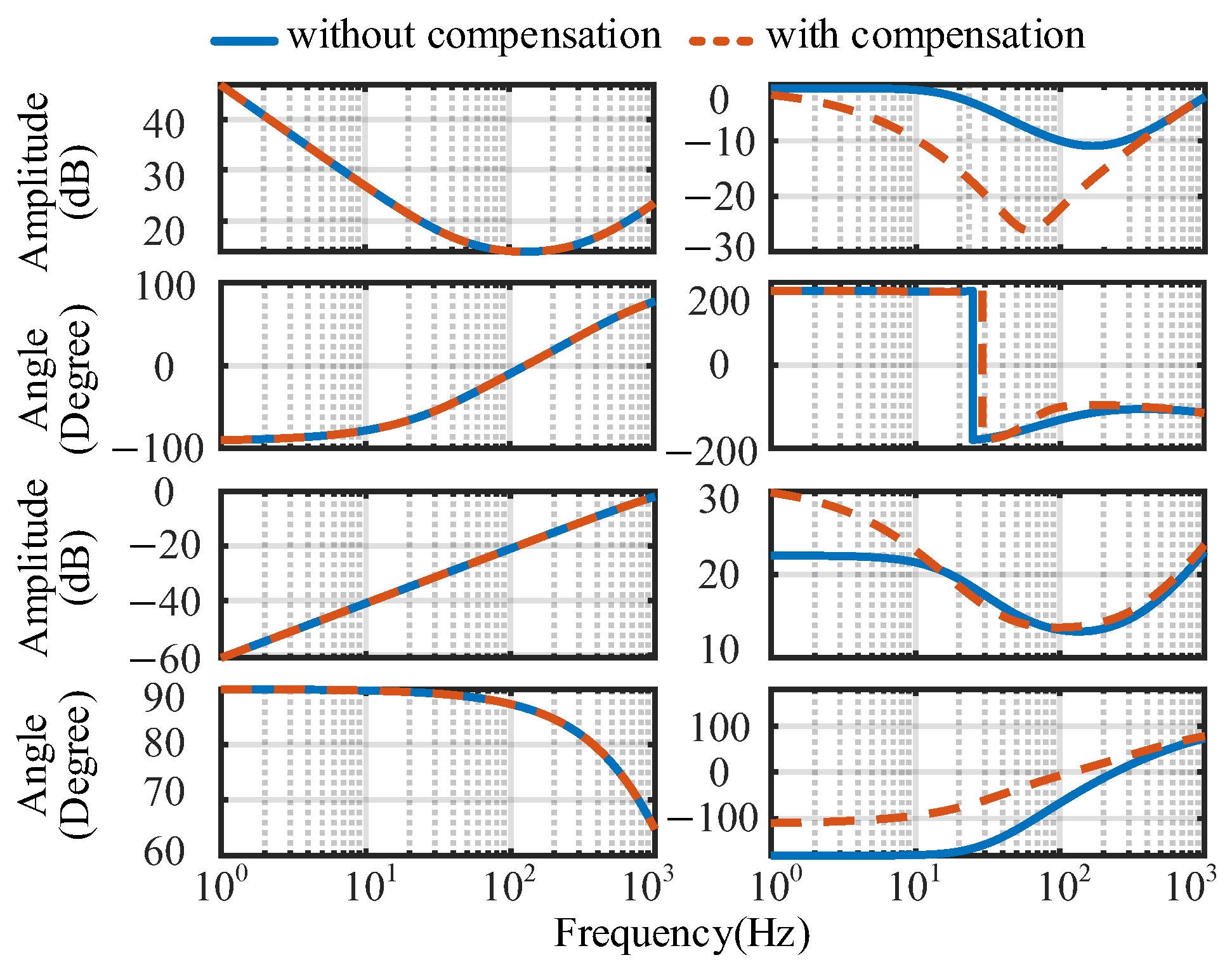
3.4. Robustness Analysis
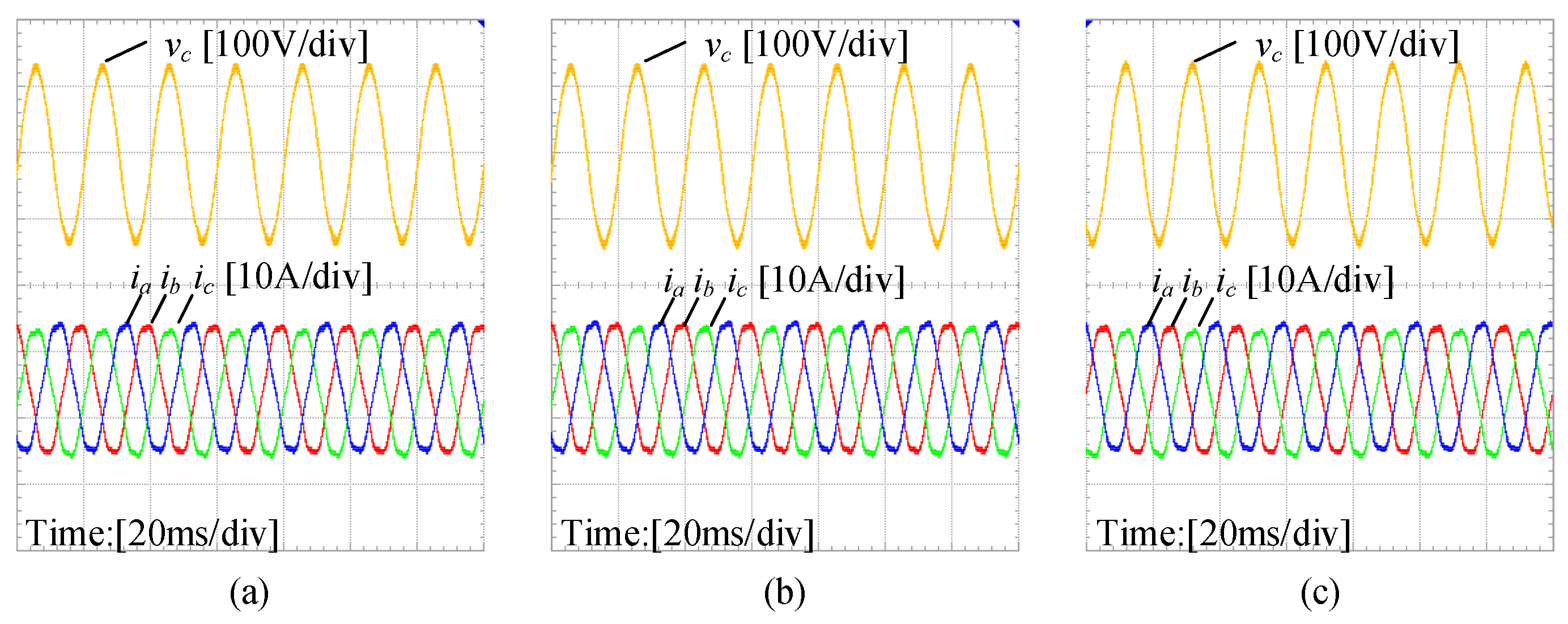
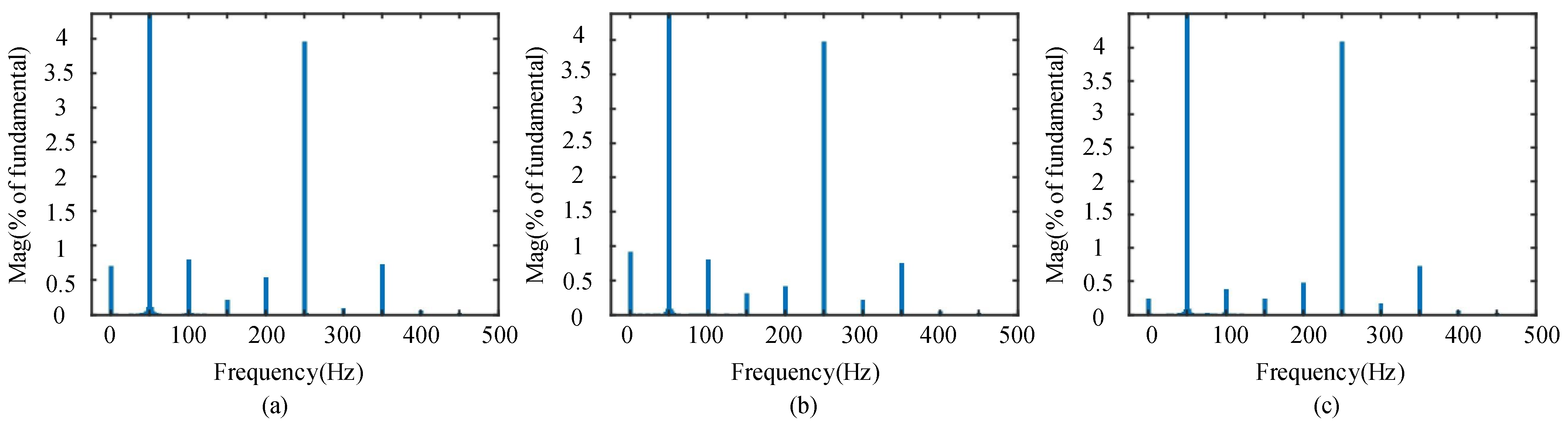
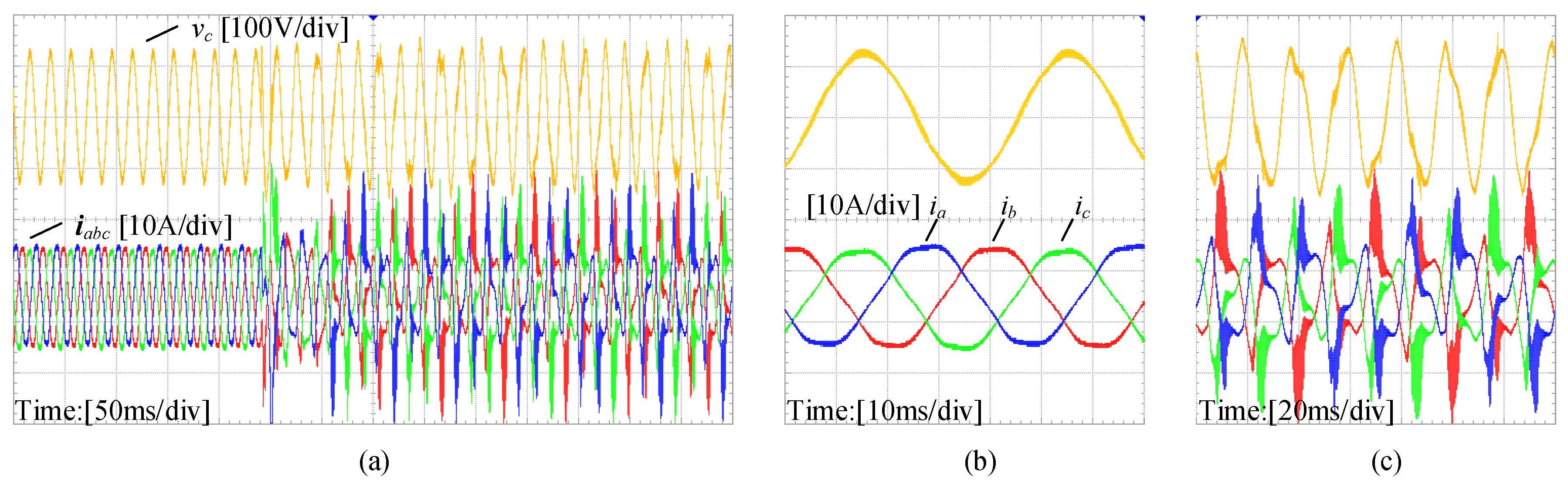
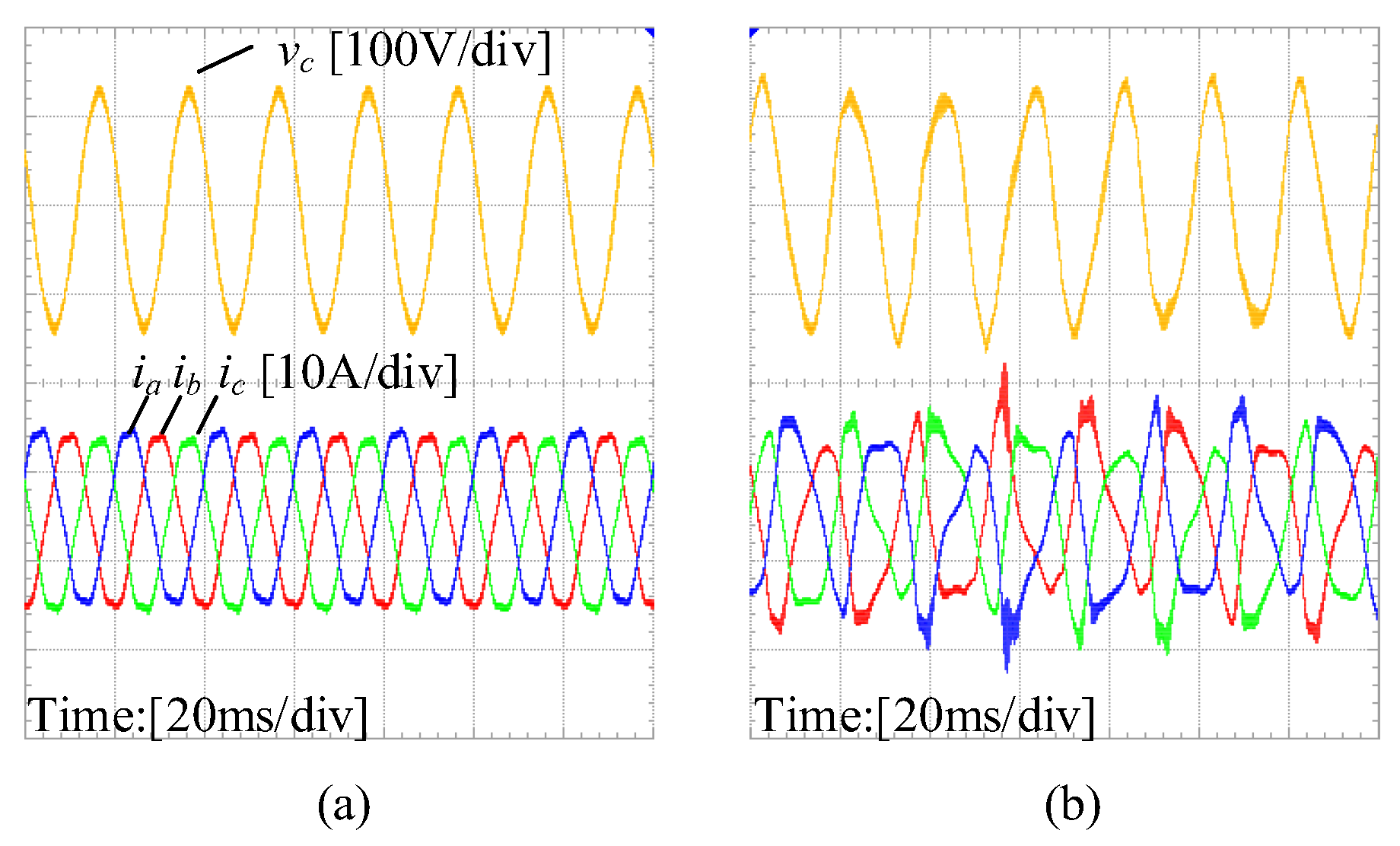
4. Experiment and Simulation Verification
5. Conclusions and Future Work
- (1)
- The instability mechanism of the grid-connected system can be deeply analyzed, which enables us to propose a more effective stability control method.
- (2)
- The effectiveness and implementation of the equivalent parallel resistance compensation method in scenarios such as multiple inverters should be explored.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Li, Y.; Fan, L.; Miao, Z. Stability Control for Wind in Weak Grids. IEEE Trans. Sustain. Energy 2019, 10, 2094–2103. [Google Scholar] [CrossRef]
- Ma, J.; Shen, Y.; Du, Y.; Liu, H.; Wang, J. Overview on Active Damping Technology of Wind Power Integrated System Adapting to Broadband Oscillation. Power Syst. Technol. 2021, 45, 1673–1686. [Google Scholar] [CrossRef]
- Sun, J. Impedance-Based Stability Criterion for Grid-Connected Inverters. IEEE Trans. Power Electron. 2011, 26, n3075–n3078. [Google Scholar] [CrossRef]
- Wen, B.; Boroyevich, D.; Burgos, R.; Mattavelli, P.; Shen, Z. Analysis of D-Q Small-Signal Impedance of Grid-Tied Inverters. IEEE Trans. Power Electron. 2016, 31, 675–687. [Google Scholar] [CrossRef]
- Tu, C.; Gao, J.; Xiao, F.; Guo, Q.; Jiang, F. Stability Analysis of the Grid-Connected Inverter Considering the Asymmetric Positive-Feedback Loops Introduced by the PLL in Weak Grids. IEEE Trans. Ind. Electron. 2022, 69, 5793–5802. [Google Scholar] [CrossRef]
- Gong, H.; Wang, X.; Harnefors, L. Rethinking Current Controller Design for PLL-Synchronized VSCs in Weak Grids. IEEE Trans. Power Electron. 2021, 37, 1369–1381. [Google Scholar] [CrossRef]
- Huang, L.; Xin, H.; Li, Z.; Ju, P.; Yuan, H.; Lan, Z.; Wang, Z. Grid-Synchronization Stability Analysis and Loop Shaping for PLL-Based Power Converters with Different Reactive Power Control. IEEE Trans. Smart Grid 2020, 11, 501–516. [Google Scholar] [CrossRef]
- Wu, M.; Zeng, J.; Ying, G.; Liu, J. A d-axis current error compensation method based on coupling mechanism for Grid-Connected inverters under weak grid. Int. J. Electr. Power Energy Syst. 2023, 151, 109189. [Google Scholar] [CrossRef]
- Shair, J.; Xie, X.; Li, H.; Zhao, W.; Liu, W. A grid-side multi-modal adaptive damping control of super-/sub-synchronous oscillations in type-4 wind farms connected to weak AC grid. Electr. Power Syst. Res. 2023, 215, 108963. [Google Scholar] [CrossRef]
- Fang, J.; Li, X.; Li, H.; Tang, Y. Stability Improvement for Three-Phase Grid-Connected Converters through Impedance Reshaping in Quadrature-Axis. IEEE Trans. Power Electron. 2018, 33, 8365–8375. [Google Scholar] [CrossRef]
- Li, G.; Chen, Y.; Luo, A.; Wang, Y. An Inertia Phase Locked Loop for Suppressing Sub-Synchronous Resonance of Renewable Energy Generation System under Weak Grid. IEEE Trans. Power Syst. 2021, 36, 4621–4631. [Google Scholar] [CrossRef]
- Yang, D.; Wang, X.; Liu, F.; Xin, K.; Liu, Y.; Blaabjerg, F. Symmetrical PLL for SISO Impedance Modeling and Enhanced Stability in Weak Grids. IEEE Trans. Power Electron. 2020, 35, 1473–1483. [Google Scholar] [CrossRef]
- Lin, X.; Wen, Y.; Yu, R.; Yu, J.; Wen, H. Improved Weak Grids Synchronization Unit for Passivity Enhancement of Grid-Connected Inverter. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 7084–7097. [Google Scholar] [CrossRef]
- Tao, R.; Wu, M.; Ying, G.; Tang, J.; An, R.; Wang, Q.; Liu, J. A phase compensation method combined with power correction for suppressing sub-synchronous resonance of wind generation under weak grid. Int. J. Electr. Power Energy Syst. 2023, 151, 109115. [Google Scholar] [CrossRef]
- Li, C.; Liu, W.; Liang, J.; Ding, X.; Cipcigan, L.M. Improved Grid Impedance Compensation for Phase-Locked Loop to Stabilize the Very-Weak-Grid Connection of VSIs. IEEE Trans. Power Deliv. 2022, 37, 3863–3872. [Google Scholar] [CrossRef]
- Duan, Z.; Meng, Y.; Wu, K.; Yang, Y.; Wang, X.; Wang, X. Back-electromotive-force observer (BEMF observer) based symmetrical PLL for grid synchronization stability enhancement under weak grid conditions. IET Gener. Transm. Distrib. 2022, 16, 4068–4079. [Google Scholar] [CrossRef]
- Mirmohammad, M.; Azad, S.P. Control and Stability of Grid-Forming Inverters: A Comprehensive Review. Energies 2024, 17, 3186. [Google Scholar] [CrossRef]
- Gui, Y.; Wang, X.; Blaabjerg, F. Vector Current Control Derived from Direct Power Control for Grid-Connected Inverters. IEEE Trans. Power Electron. 2019, 34, 9224–9235. [Google Scholar] [CrossRef]
- Gui, Y.; Wang, X.; Wu, H.; Blaabjerg, F. Voltage-Modulated Direct Power Control for a Weak Grid-Connected Voltage Source Inverters. IEEE Trans. Power Electron. 2019, 34, 11383–11395. [Google Scholar] [CrossRef]
- Li, M.; Zhang, X.; Yang, Y.; Cao, P. The Grid Impedance Adaptation Dual Mode Control Strategy in Weak Grid. In Proceedings of the 2018 International Power Electronics Conference (IPEC-Niigata 2018-ECCE Asia), Niigata, Japan, 20–24 May 2018; pp. 2973–2979. [Google Scholar] [CrossRef]
- Wang, S.; Cui, K.; Hao, P. Grid-Connected Inverter Grid Voltage Feedforward Control Strategy Based on Multi-Objective Constraint in Weak Grid. Energies 2024, 17, 3288. [Google Scholar] [CrossRef]
- Zhu, D.; Zhou, S.; Zou, X.; Kang, Y. Improved Design of PLL Controller for LCL-Type Grid-Connected Converter in Weak Grid. IEEE Trans. Power Electron. 2020, 35, 4715–4727. [Google Scholar] [CrossRef]
- Li, X.; Lin, H. A Design Method of Phase-Locked Loop for Grid-Connected Converters Considering the Influence of Current Loops in Weak Grid. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 2420–2429. [Google Scholar] [CrossRef]
- Li, Y.; Chen, J.; Wang, X.; Zhang, X.; Zhao, X. Dynamic Stability Study of Grid-Connected Inverter Based on Virtual Synchronizer under Weak Grid. Energies 2022, 15, 7091. [Google Scholar] [CrossRef]
- Piyasinghe, L.; Miao, Z.; Khazaei, J.; Fan, L. Impedance Model-Based SSR Analysis for TCSC Compensated Type-3 Wind Energy Delivery Systems. IEEE Trans. Sustain. Energy 2015, 6, 179–187. [Google Scholar] [CrossRef]
- Wang, X.; Harnefors, L.; Blaabjerg, F. Unified Impedance Model of Grid-Connected Voltage-Source Converters. IEEE Trans. Power Electron. 2018, 33, 1775–1787. [Google Scholar] [CrossRef]
- Lin, X.; Yu, J.; Yu, R.; Zhang, J.; Yan, Z.; Wen, H. Improving Small-Signal Stability of Grid-Connected Inverter Under Weak Grid by Decoupling Phase-Lock Loop and Grid Impedance. IEEE Trans. Ind. Electron. 2022, 69, 7040–7053. [Google Scholar] [CrossRef]





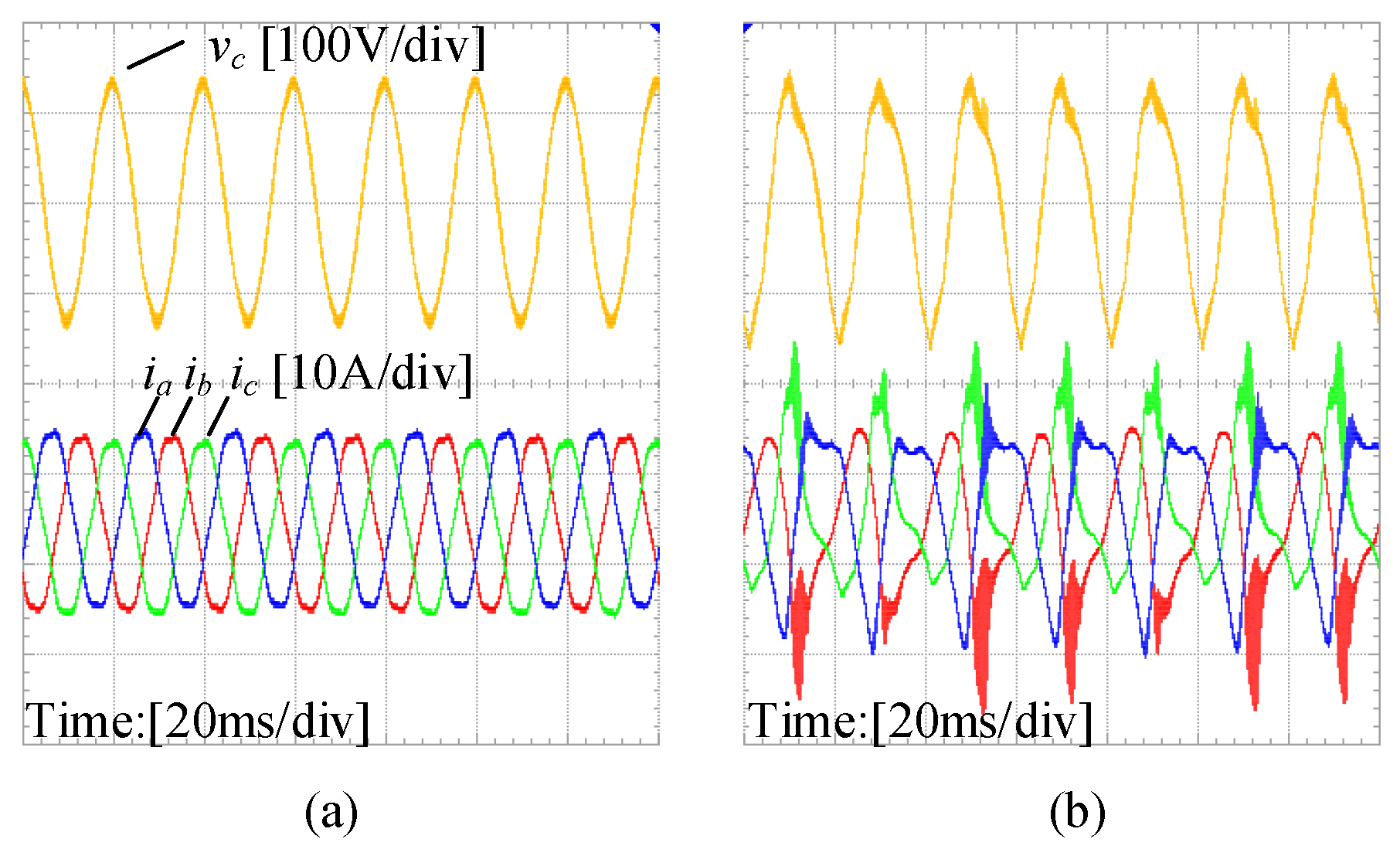
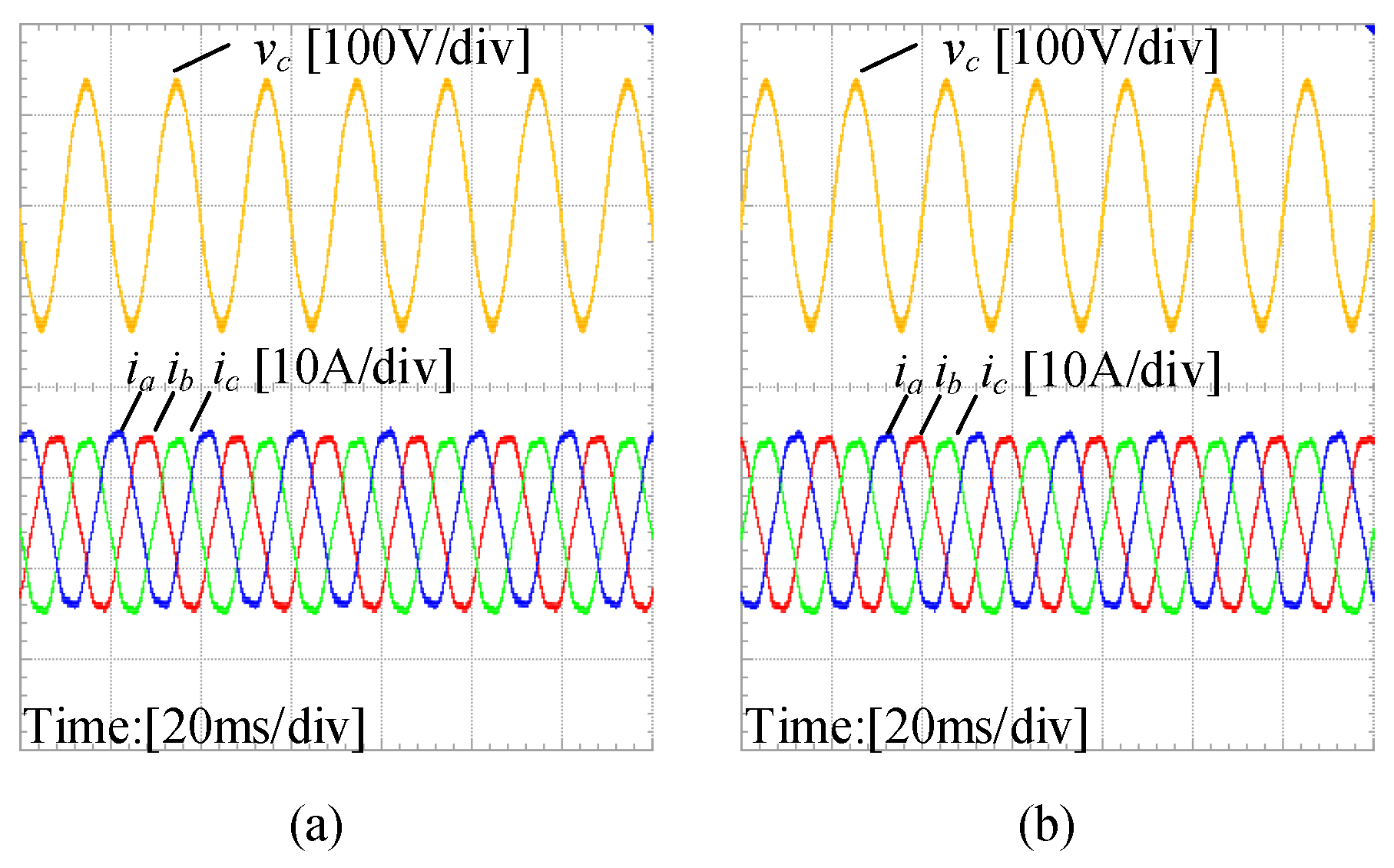

| Parameter | Values |
|---|---|
| DC-link voltage Vdc | 400 V |
| Grid voltage RMS value Vg | 130 V |
| Rated power Pn | 2 kW |
| Filter inductors Lf | 3 mH |
| Filter capacitors Cf | 20 μF |
| Damping resistors Rf | 10 Ω |
| Grid inductors Lg | 9/16 mH |
| Switching frequency fsw | 10 kHz |
| Sampling frequency fs | 10 kHz |
| Proportional gain of Gi kpi | 5.24 |
| Integral gain of Gi kii | 1370 |
| Proportional gain of Gpll kppll | 4.2 |
| Integral gain of Gpll kipll | 384 |
| Cases | Lmg (mH) | Lg (mH) | PLL Parameters | dq-Axis Current (A) |
|---|---|---|---|---|
| I | 16 | 16 | kppll = 4.2, kipll = 384 | Idref = 10, Iqref = 2 |
| II | 16 | 16 | kppll = 4.2, kipll = 484 | Idref = 10, Iqref = 0 |
| III | 16 | 17.5 | kppll = 4.2, kipll = 384 | Idref = 10, Iqref = 0 |
| IV | 4.8 (−70% Lg) | 16 | kppll = 4.2, kipll = 384 | Idref = 10, Iqref = 0 |
| V | 41.6 (+160% Lg) | 16 | kppll = 4.2, kipll = 384 | Idref = 10, Iqref = 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, M.; Zeng, J.; Ying, G.; Xu, J.; Yang, S.; Zhou, Y.; Liu, J. A Stability Control Method to Maintain Synchronization Stability of Wind Generation under Weak Grid. Energies 2024, 17, 4450. https://doi.org/10.3390/en17174450
Wu M, Zeng J, Ying G, Xu J, Yang S, Zhou Y, Liu J. A Stability Control Method to Maintain Synchronization Stability of Wind Generation under Weak Grid. Energies. 2024; 17(17):4450. https://doi.org/10.3390/en17174450
Chicago/Turabian StyleWu, Minhai, Jun Zeng, Gengning Ying, Jidong Xu, Shuangfei Yang, Yuebin Zhou, and Junfeng Liu. 2024. "A Stability Control Method to Maintain Synchronization Stability of Wind Generation under Weak Grid" Energies 17, no. 17: 4450. https://doi.org/10.3390/en17174450
APA StyleWu, M., Zeng, J., Ying, G., Xu, J., Yang, S., Zhou, Y., & Liu, J. (2024). A Stability Control Method to Maintain Synchronization Stability of Wind Generation under Weak Grid. Energies, 17(17), 4450. https://doi.org/10.3390/en17174450








