Performance Enhancement of H-Type Darrieus VAWT Using a Hybrid Method of Blade Pitch Angle Regulation
Abstract
1. Introduction
2. Solution Strategy
3. Materials and Methods
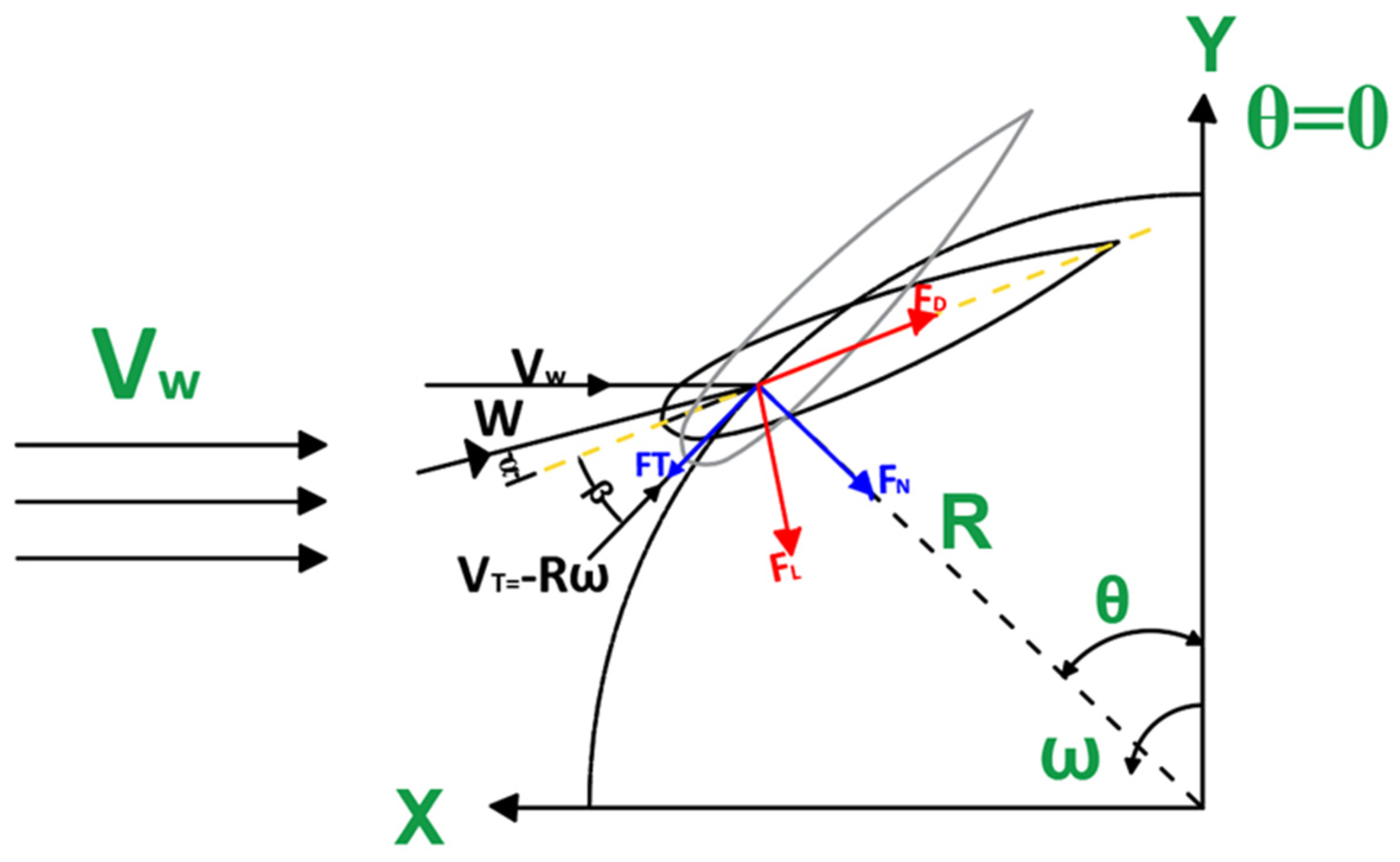
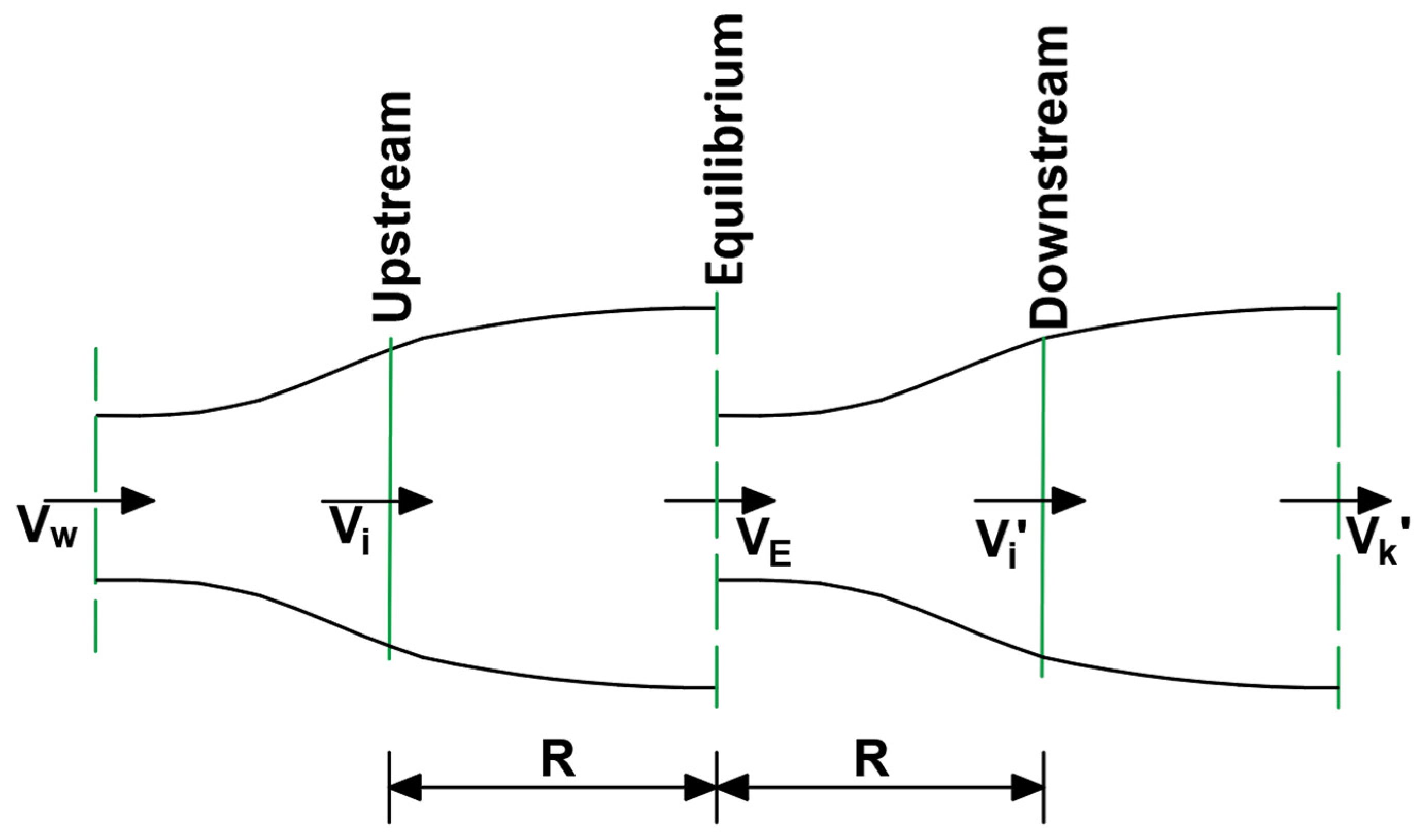
3.1. Double Multiple Streamtube Model
3.2. Numerical Modeling and Simulation
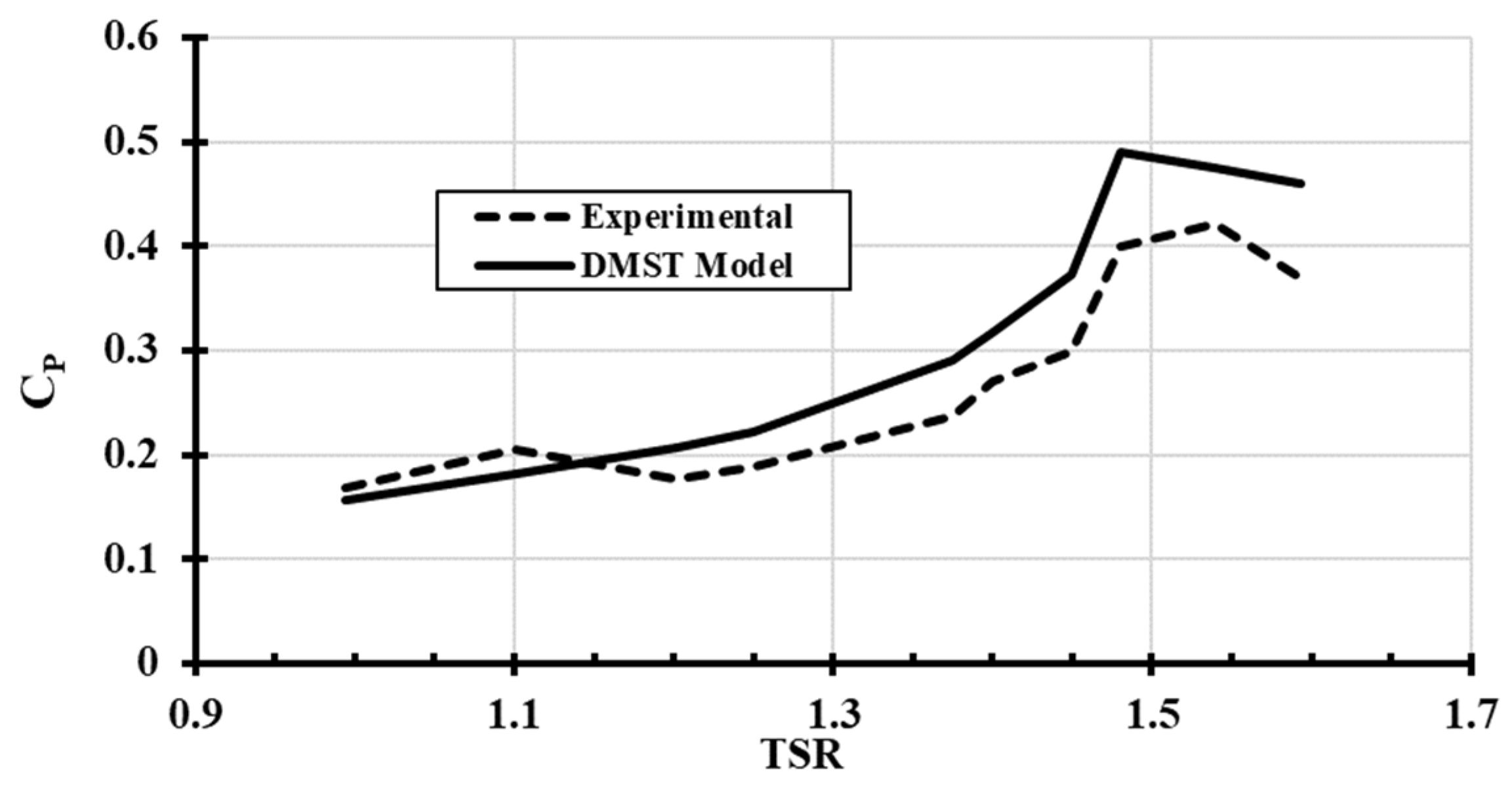
3.3. Experimental Validation
4. Results and Discussion
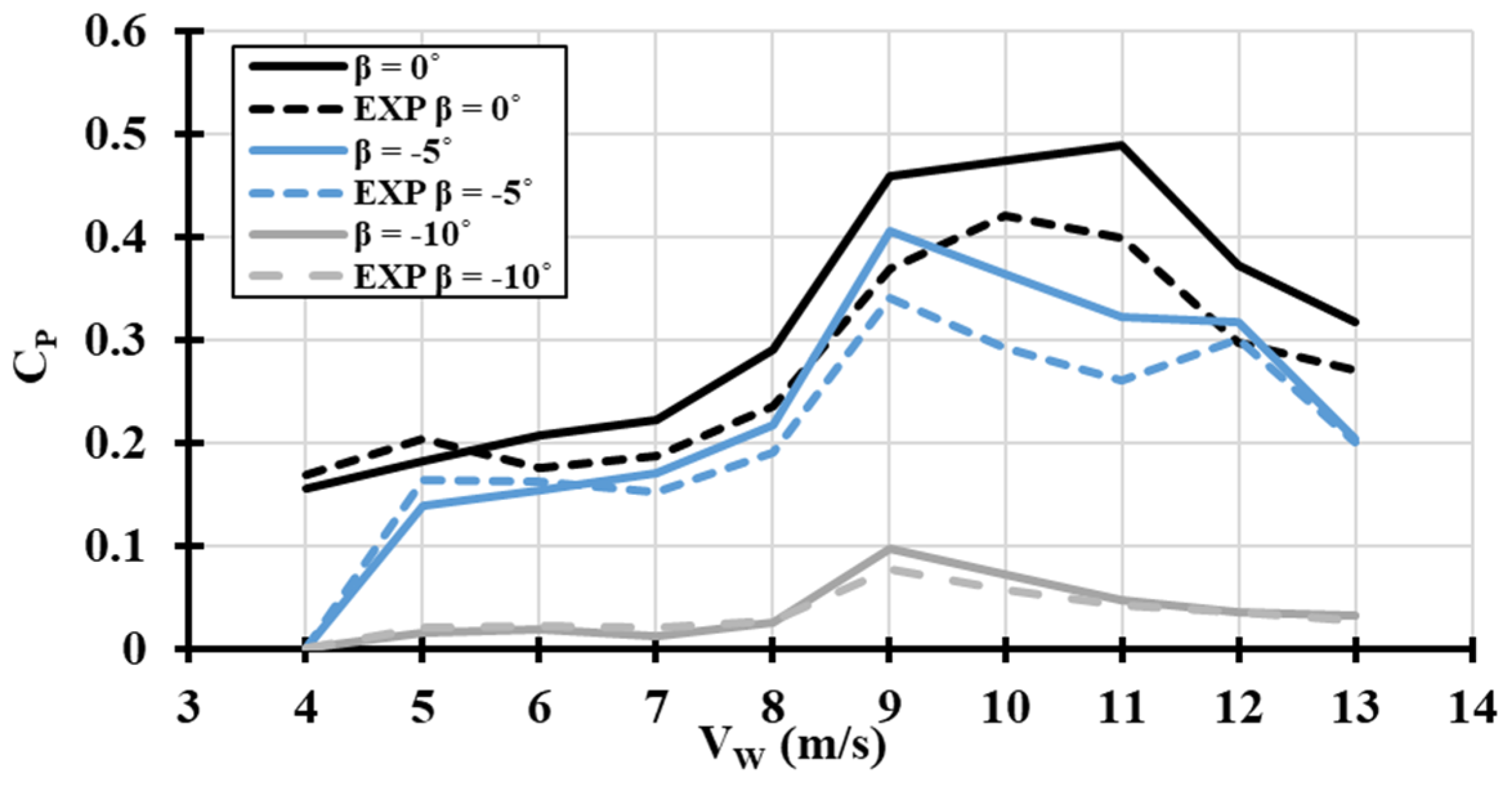
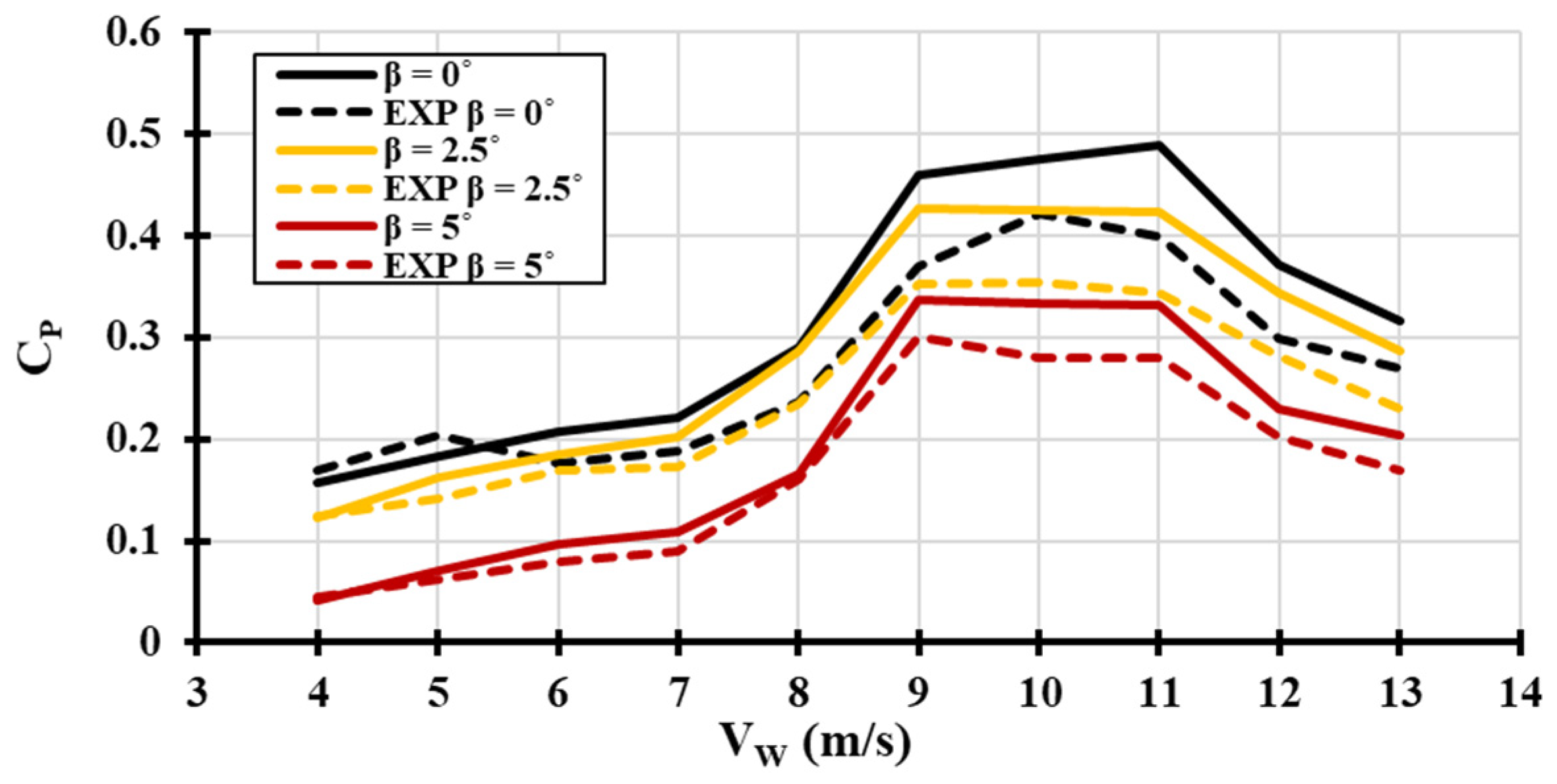
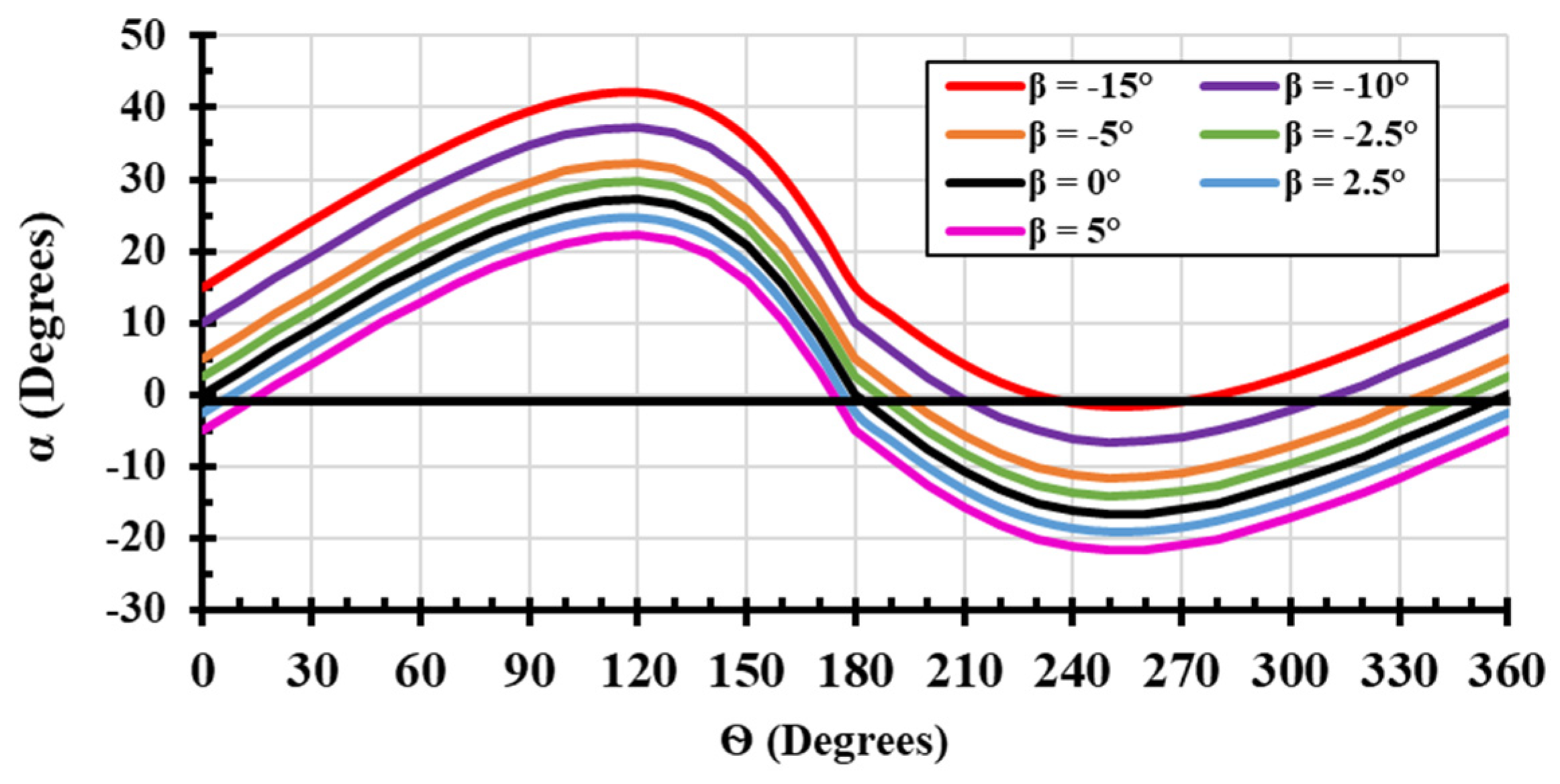
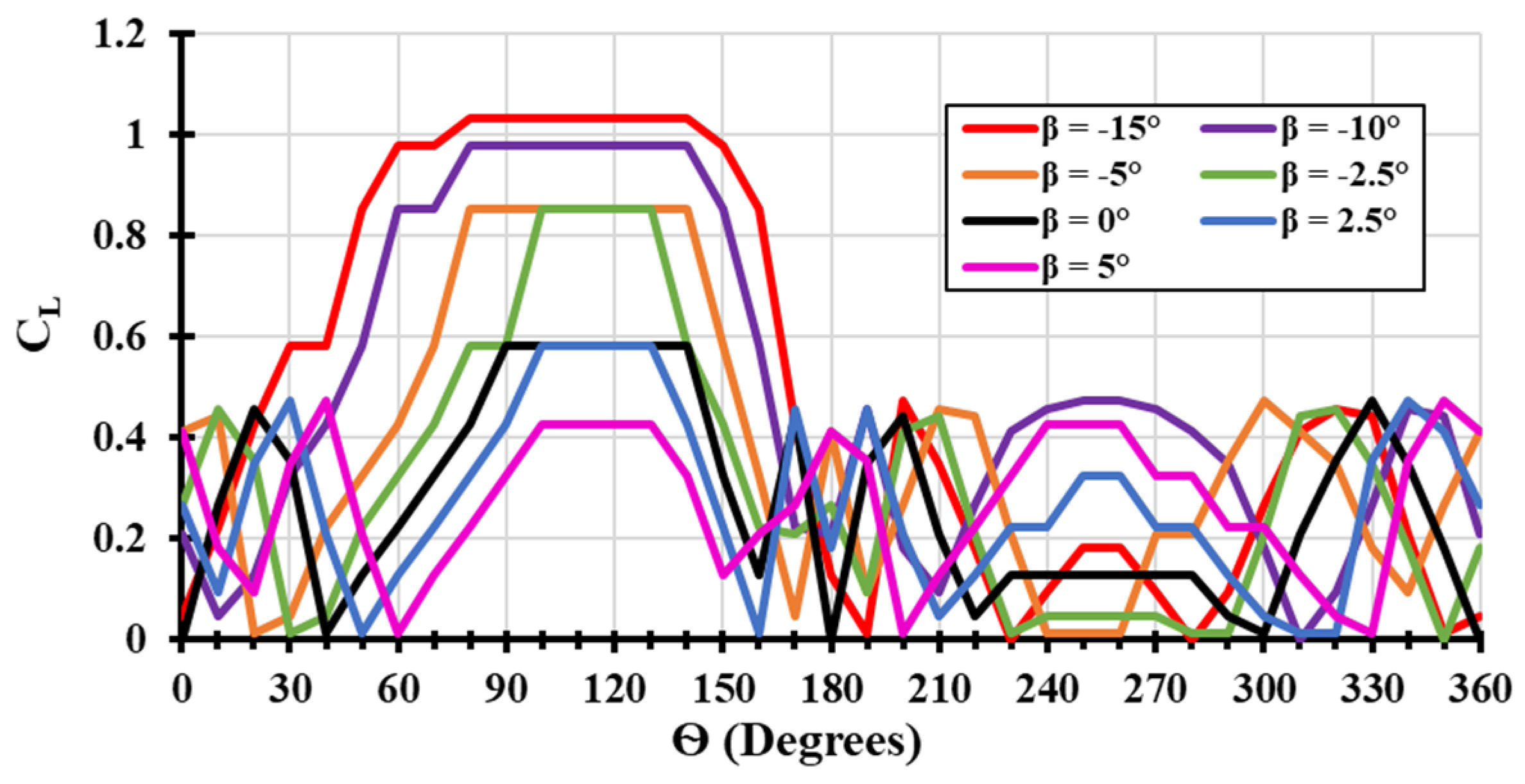
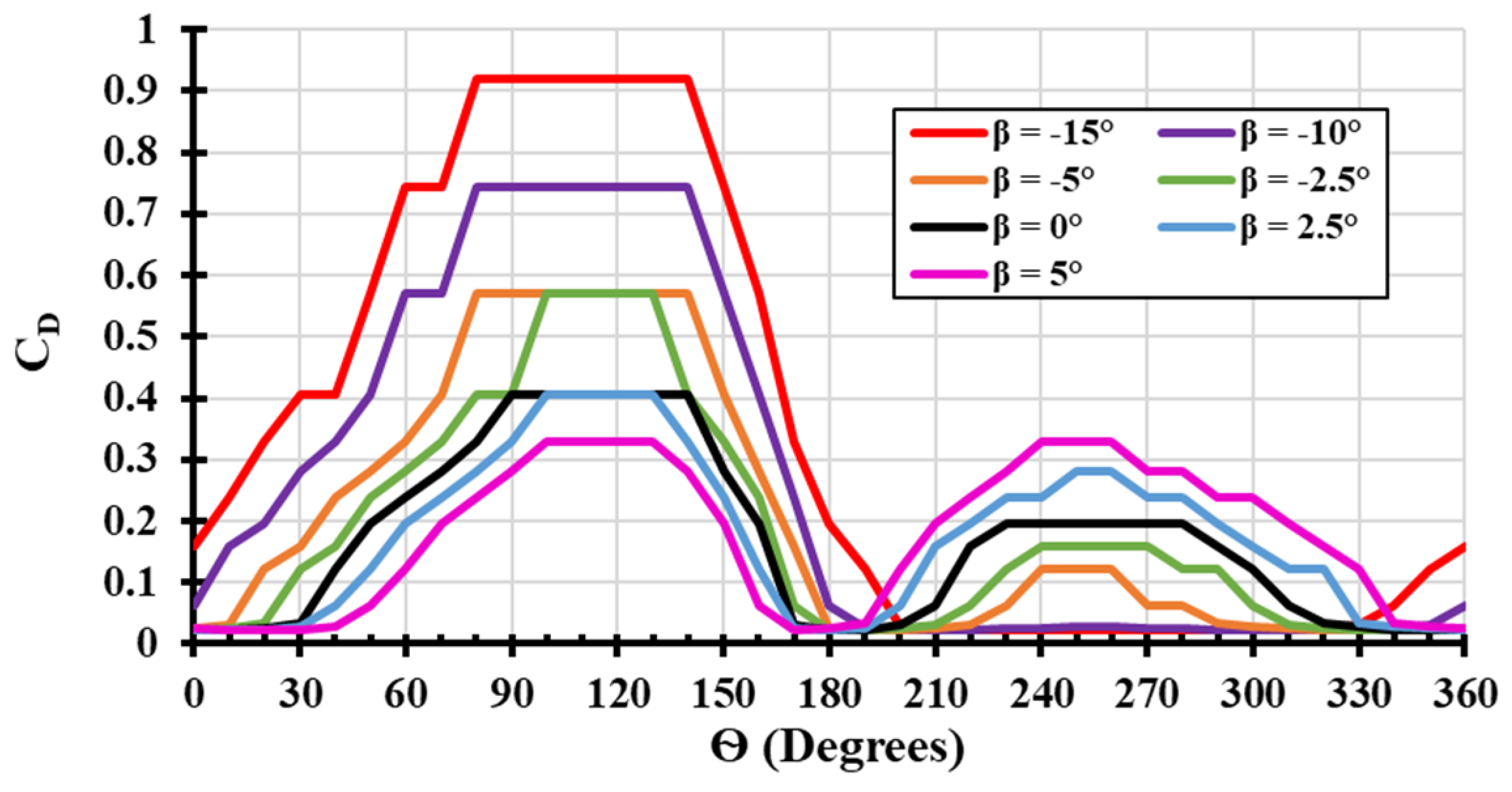
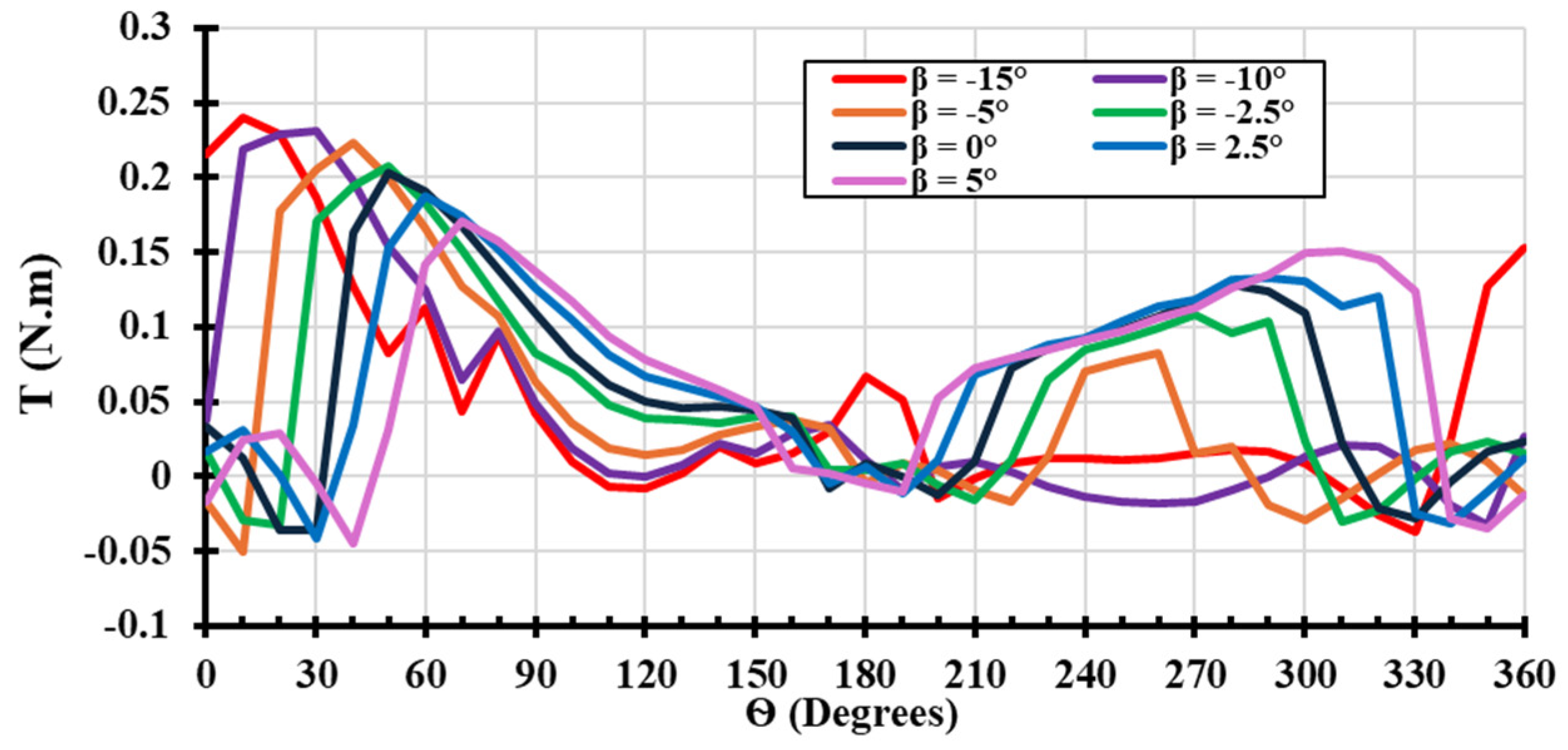
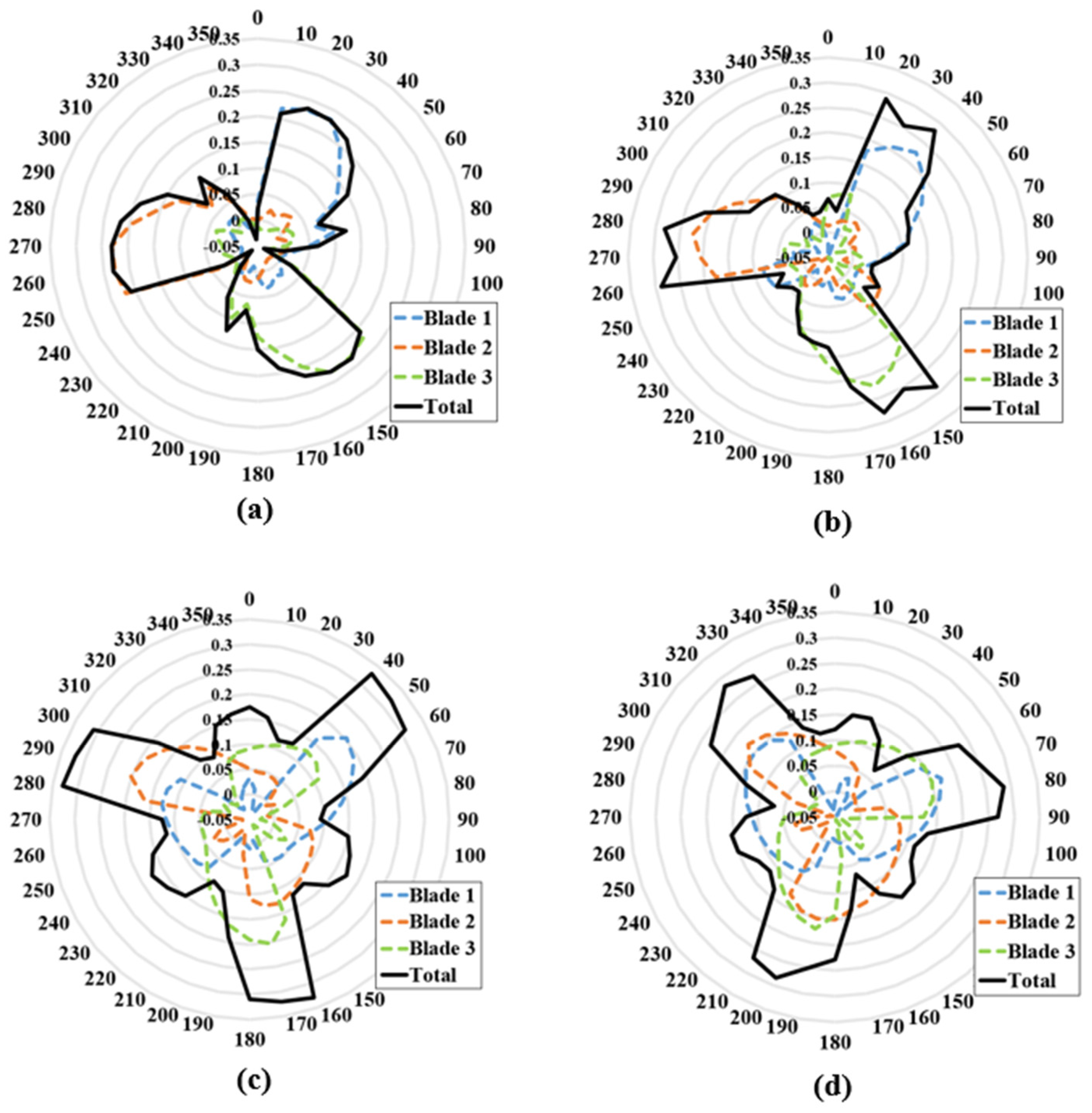
4.1. Impact of Blade Pitch Angle Regulation on H-Type Darrieus VAWT Aerodynamics
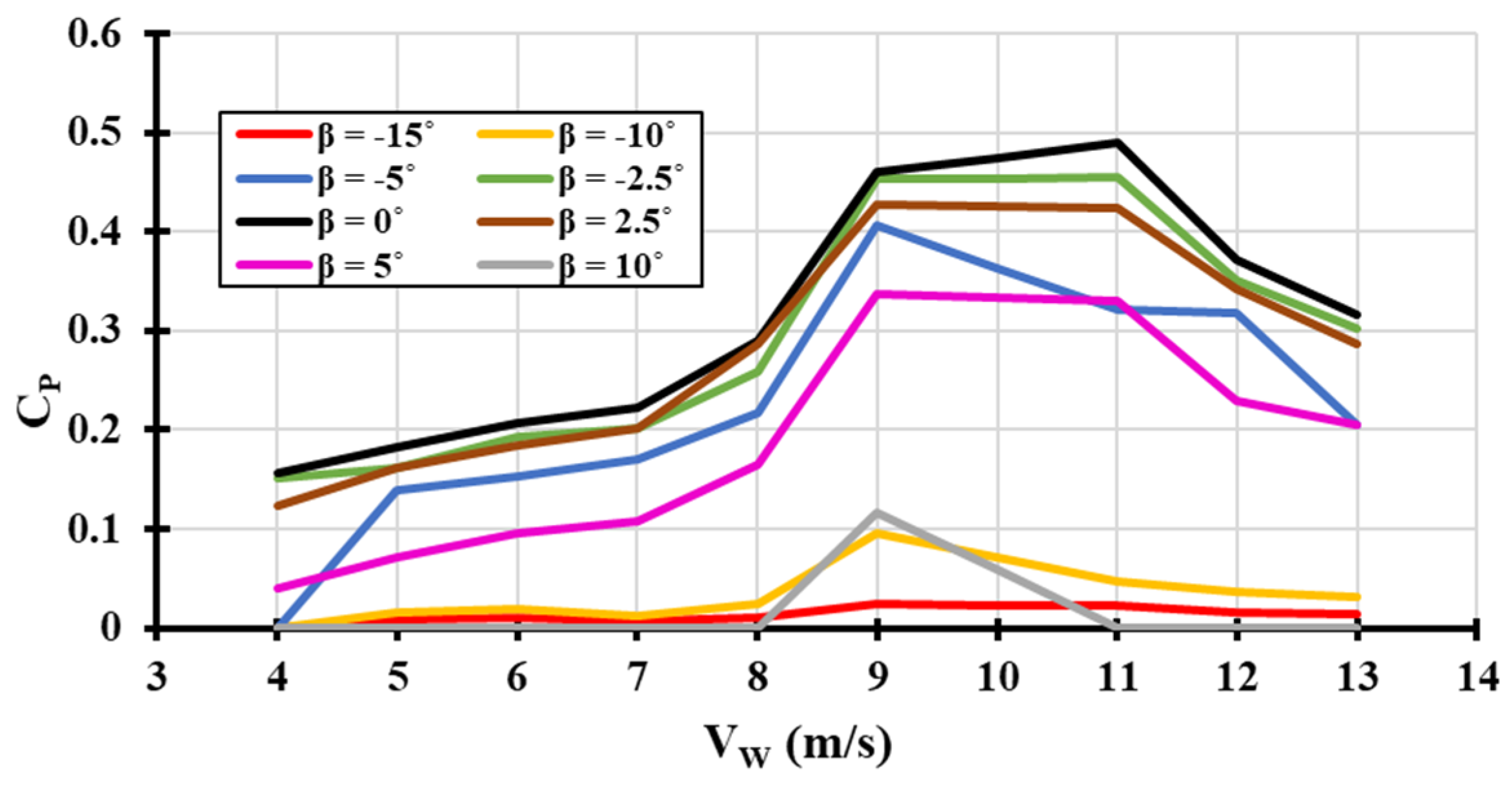
4.2. Effect of Blade Pitch Angle Regulation on H-Type Darrieus VAWT Performance
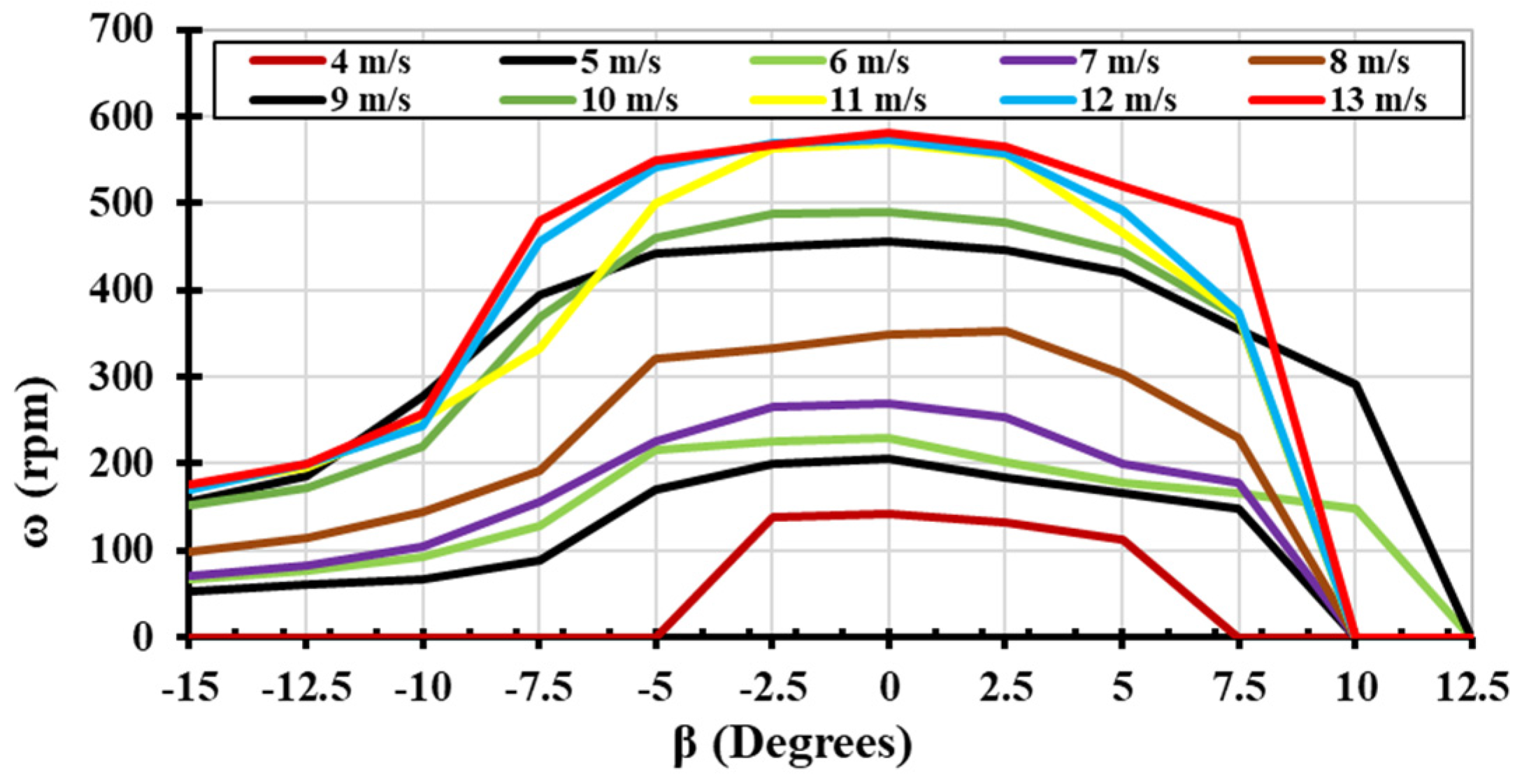
4.3. Self-Starting Behavior of H-Type Darrieus VAWT
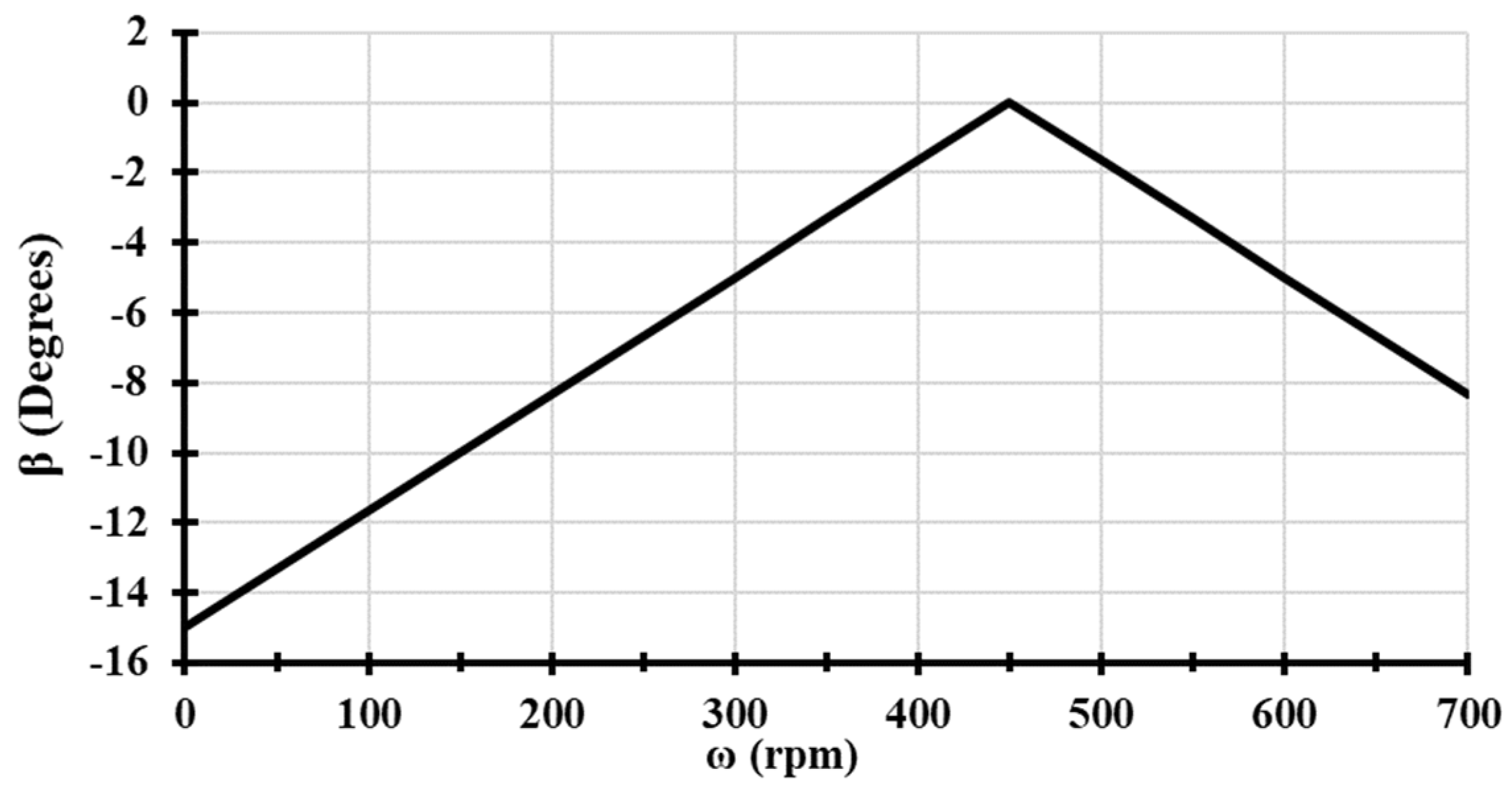
4.4. Hybrid Blade Pitch Angle Technique
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Didane, D.H.; Behery, M.R.; Al-Ghriybah, M.; Manshoor, B. Recent Progress in Design and Performance Analysis of Vertical-Axis Wind Turbines—A Comprehensive Review. Processes 2024, 12, 1094. [Google Scholar] [CrossRef]
- Acosta-López, J.G.; Blasetti, A.P.; Lopez-Zamora, S.; de Lasa, H. CFD Modeling of an H-Type Darrieus VAWT under High Winds: The Vorticity Index and the Imminent Vortex Separation Condition. Processes 2023, 11, 644. [Google Scholar] [CrossRef]
- Zereg, A.; Aksas, M.; Bouzaher, M.T.; Laghrouche, S.; Lebaal, N. Efficiency Improvement of Darrieus Wind Turbine Using Oscillating Gurney Flap. Fluids 2024, 9, 150. [Google Scholar] [CrossRef]
- Abdalrahman, G.; Daoud, M.A.; Melek, W.W.; Lien, F.-S.; Yee, E. Design and Implementation of an Intelligent Blade Pitch Control System and Stability Analysis for a Small Darrieus Vertical-Axis Wind Turbine. Energies 2022, 15, 235. [Google Scholar] [CrossRef]
- Kumar, R.; Raahemifar, K.; Fung, A.S. A critical review of vertical axis wind turbines for urban applications. Renew. Sustain. Energy Rev. 2018, 89, 281–291. [Google Scholar] [CrossRef]
- Du, L.; Ingram, G.; Dominy, R.G. A review of H-Darrieus wind turbine aerodynamic research. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 7590–7616. [Google Scholar] [CrossRef]
- Nizamani, Z.; Muhammad, A.; Ali, M.O.A.; Wahab, M.A.; Nakayama, A.; Ahmed, M.M. Renewable wind energy resources in offshore low wind speeds regions near the equator: A review. Ocean Eng. 2024, 311, 118834. [Google Scholar] [CrossRef]
- Gupta, A.; Abderrahmane, H.A.; Janajreh, I. Flow analysis and sensitivity study of vertical-axis wind turbine under variable pitching. Appl. Energy 2024, 358, 122648. [Google Scholar] [CrossRef]
- Sun, X.; Hao, T.; Zhang, J.; Dong, L.; Zhu, J. The performance increase of the wind-induced rotation VAWT by application of the passive variable pitching blade. Int. J. Low-Carbon Technol. 2022, 17, 1420–1434. [Google Scholar] [CrossRef]
- Zhao, Z.; Wang, R.; Shen, W.; Wang, T.; Xu, B.; Zheng, Y.; Qian, S. Variable pitch approach for performance improving of straight-bladed VAWT at rated tip speed ratio. Appl. Sci. 2018, 8, 957. [Google Scholar] [CrossRef]
- Yamada, T.; Kiwata, T.; Kita, T.; Hirai, M.; Komatsu, N.; Kono, T. Overspeed Control of a Variable-Pitch Vertical-Axis Wind Turbine by Means of Tail Vanes. J. Environ. Eng. 2012, 7, 39–52. [Google Scholar] [CrossRef]
- Miliket, T.A.; Ageze, M.B.; Tigabu, M.T. Aerodynamic performance enhancement and computational methods for H-Darrieus vertical axis wind turbines: Review. Int. J. Green Energy 2022, 19, 1428–1465. [Google Scholar] [CrossRef]
- Szczerba, Z.; Szczerba, P.; Szczerba, K.; Szumski, M.; Pytel, K. Wind Tunnel Experimental Study on the Efficiency of Vertical-Axis Wind Turbines via Analysis of Blade Pitch Angle Influence. Energies 2023, 16, 4903. [Google Scholar] [CrossRef]
- Hao, W.; Abdi, A.; Wang, G.; Wu, F. Study on the Pitch Angle Effect on the Power Coefficient and Blade Fatigue Load of a Vertical Axis Wind Turbine. Energies 2023, 16, 7279. [Google Scholar] [CrossRef]
- Castillo, O.C.; Andrade, V.R.; Rivas, J.J.R.; González, R.O. Comparison of Power Coefficients in Wind Turbines Considering the Tip Speed Ratio and Blade Pitch Angle. Energies 2023, 16, 2774. [Google Scholar] [CrossRef]
- Sagharichi, A.; Maghrebi, M.J.; ArabGolarcheh, A. Variable pitch blades: An approach for improving performance of Darrieus wind turbine. J. Renew. Sustain. Energy 2016, 8, 053305. [Google Scholar] [CrossRef]
- Liu, L.; Liu, C.; Zheng, X. Modeling, simulation, hardware implementation of a novel variable pitch control for H-type vertical axis wind turbine. J. Electr. Eng. 2015, 66, 264–269. [Google Scholar] [CrossRef]
- Zhao, Z.; Qian, S.; Shen, W.; Wang, T.; Xu, B.; Zheng, Y.; Wang, R. Study on variable pitch strategy in H-type wind turbine considering effect of small angle of attack. J. Renew. Sustain. Energy 2017, 9, 053302. [Google Scholar] [CrossRef]
- Arif, S.K.; Maree, I.E. Investigation on aerodynamic performance of H-type darrieus vertical axis wind turbine with different series airfoil shapes. Al-Qadisiyah J. Eng. Sci. 2023, 16, 121–126. [Google Scholar] [CrossRef]
- Islam, M.; Ting, D.S.-K.; Fartaj, A. Aerodynamic models for Darrieus-type straight-bladed vertical axis wind turbines. Renew. Sustain. Energy Rev. 2008, 12, 1087–1109. [Google Scholar] [CrossRef]
- Moutis, P.; Pastromas, S.; Hatziargyriou, N.D. Load-frequency control supported by variable speed variable pitch wind generators—From theory to testing. Eur. Wind Energy Conf. Exhib. EWEC 2013, 3, 1743–1748. [Google Scholar]
- Chougule, P.; Nielsen, S. Overview and Design of self-acting pitch control mechanism for vertical axis wind turbine using multi body simulation approach. J. Phys. Conf. Ser. 2014, 524, 012055. [Google Scholar] [CrossRef]
- Schönborn, A.; Chantzidakis, M. Development of a hydraulic control mechanism for cyclic pitch marine current turbines. Renew. Energy 2007, 32, 662–679. [Google Scholar] [CrossRef]
- Abdalrahman, G.; Melek, W.; Lien, F.-S. Pitch angle control for a small-scale Darrieus vertical axis wind turbine with straight blades (H-Type VAWT). Renew. Energy 2017, 114, 1353–1362. [Google Scholar] [CrossRef]
- Zhang, L.-X.; Liang, Y.-B.; Liu, X.-H.; Guo, J. Effect of blade pitch angle on aerodynamic performance of straight-bladed vertical axis wind turbine. J. Cent. South Univ. 2014, 21, 1417–1427. [Google Scholar] [CrossRef]
- Rezaeiha, A.; Kalkman, I.; Blocken, B. Effect of pitch angle on power performance and aerodynamics of a vertical axis wind turbine. Appl. Energy 2017, 197, 132–150. [Google Scholar] [CrossRef]
- Yang, Y.; Guo, Z.; Song, Q.; Zhang, Y.; Li, Q. Effect of blade pitch angle on the aerodynamic characteristics of a straight-bladed vertical axis wind turbine based on experiments and simulations. Energies 2018, 11, 1514. [Google Scholar] [CrossRef]
- Firdaus, R.; Kiwata, T.; Kono, T.; Nagao, K. Numerical and experimental studies of a small vertical-axis wind turbine with variable-pitch straight blades. J. Fluid Sci. Technol. 2015, 10, JFST0001. [Google Scholar] [CrossRef]
- Gerrie, C.; Islam, S.Z.; Gerrie, S.; Turner, N.; Asim, T. 3D CFD Modelling of Performance of a Vertical Axis Turbine. Energies 2023, 16, 1144. [Google Scholar] [CrossRef]
- Luo, Z.; Sun, Z.; Ma, F.; Qin, Y.; Ma, S. Power optimization for wind turbines based on stacking model and pitch angle adjustment. Energies 2020, 13, 4158. [Google Scholar] [CrossRef]
- Sarkar, R.; Julai, S.; Tong, C.W.; Uddin, M.; Romlie, M.; Shafiullah, G. Hybrid pitch angle controller approaches for stable wind turbine power under variable wind speed. Energies 2020, 13, 3622. [Google Scholar] [CrossRef]
- Templin, R.J. Aerodynamic Performance Theory for the NRC Vertical-Axis Wind Turbine; NASA STI/Recon Technical Report N76; National Aeronautical Establishment: Ottawa, ON, Canada, 1974. [Google Scholar]
- Wilson, R.E. LPBS, Machines Power Wind of Aerodynamics, Ntis Pb 238594; Oregon State University: Corvallis, OR, USA, 1974. [Google Scholar]
- Strickland, J.H. Darrieus Turbine: A Performance Prediction Model Using Multiple Streamtubes; Sandia Labs.: Albuquerque, NM, USA, 1975. [Google Scholar]
- Paraschivoiu, I.; Fraunie, P.; Beguier, C. Streamtube expansion effects on the Darrieus wind turbine. J. Propuls. Power 1985, 1, 150–155. [Google Scholar] [CrossRef]
- Pawsey, N. Development and Evaluation of Passive Variable-Pitch Vertical Axis Wind Turbines. Ph.D. Thesis, The University of New South Wales, Sydney, NSW, Australia, 2002. [Google Scholar]
- Shahriar, A.; Kumar, R.; Shoele, K. Vortex dynamics of axisymmetric cones at high angles of attack. Theor. Comput. Fluid Dyn. 2023, 37, 337–356. [Google Scholar] [CrossRef]
- Faure, T.M.; Leogrande, C. High angle-of-attack aerodynamics of a straight wing with finite span using a discrete vortex method. Phys. Fluids 2020, 32, 104109. [Google Scholar] [CrossRef]
- Sogukpinar, H. The effects of NACA 0012 airfoil modification on aerodynamic performance improvement and obtaining high lift coefficient and post-stall airfoil. AIP Conf. Proc. 2018, 1935, 020001. [Google Scholar]
- Hansen, M.O.L. Aerodynamics of Wind Turbines, 2nd ed.; Earthscan: London, UK, 2008. [Google Scholar]
- Torabi, F. Fundamentals of Wind Farm Aerodynamic Layout Design; Academic Press: Cambridge, MA, USA, 2022. [Google Scholar]
- Battisti, L. Design Options to Improve the Dynamic Behavior and the Control of Small H-Darrieus VAWTs. Appl. Sci. 2021, 11, 9222. [Google Scholar] [CrossRef]
- Robert, S.E.; Paul, K.C. Aerodynamic Characteristics of Seven Symmetrical Airfoil Sections through 180-Degree Angle of Attack for Use in Aerodynamic Analysis of Vertical Axis Wind Turbines. Sandia National Lab.: Albuquerque, NM, USA, 1981. [Google Scholar] [CrossRef]


| H-Type Darrieus VAWT Specification | Symbol | Value |
|---|---|---|
| Blade Profile | ------ | NACA0018 |
| Blade Shape | ------ | Straight |
| Number of blades | N | 3 |
| Rotor Radius (mm) | R | 300 |
| Blade height (mm) | H | 400 |
| Chord length (mm) | C | 250 |
| Solidity | σ | 0.191 |
| VW | β = −15° | β = −12.5° | β = −10° | β = −7.5° | β = −5° | β = −2.5° | β = 0° | β = 2.5° | β = 5° | β = 7.5° |
|---|---|---|---|---|---|---|---|---|---|---|
| 4 m/s | F-S | F-S | F-S | F-S | F-S | 100 | 110 | 110 | 120 | F-S |
| 5 m/s | 0 | 0 | 20 | 30 | 70 | 80 | 90 | 110 | 110 | 120 |
| 6 m/s | 0 | 0 | 0 | 0 | 20 | 50 | 60 | 100 | 110 | 120 |
| 7 m/s | 0 | 0 | 0 | 0 | 10 | 60 | 60 | 90 | 100 | 120 |
| 8 m/s | 0 | 0 | 0 | 0 | 0 | 0 | 20 | 80 | 100 | 110 |
| 9 m/s | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 110 |
| 10 m/s | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 110 |
| 11 m/s | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 110 |
| 12 m/s | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 100 |
| 13 m/s | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 100 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hammad, M.A.; Mahmoud, A.M.; Abdelrhman, A.M.; Sarip, S. Performance Enhancement of H-Type Darrieus VAWT Using a Hybrid Method of Blade Pitch Angle Regulation. Energies 2024, 17, 4044. https://doi.org/10.3390/en17164044
Hammad MA, Mahmoud AM, Abdelrhman AM, Sarip S. Performance Enhancement of H-Type Darrieus VAWT Using a Hybrid Method of Blade Pitch Angle Regulation. Energies. 2024; 17(16):4044. https://doi.org/10.3390/en17164044
Chicago/Turabian StyleHammad, Mahmood Abduljabbar, Abdelgadir Mohamed Mahmoud, Ahmed M. Abdelrhman, and Shamsul Sarip. 2024. "Performance Enhancement of H-Type Darrieus VAWT Using a Hybrid Method of Blade Pitch Angle Regulation" Energies 17, no. 16: 4044. https://doi.org/10.3390/en17164044
APA StyleHammad, M. A., Mahmoud, A. M., Abdelrhman, A. M., & Sarip, S. (2024). Performance Enhancement of H-Type Darrieus VAWT Using a Hybrid Method of Blade Pitch Angle Regulation. Energies, 17(16), 4044. https://doi.org/10.3390/en17164044






