Recent Developments in Supercritical CO2-Based Sustainable Power Generation Technologies
Abstract
1. Introduction
2. Relevance of Supercritical CO2 Cycles in Power Generation Systems
3. sCO2 Brayton Cycle
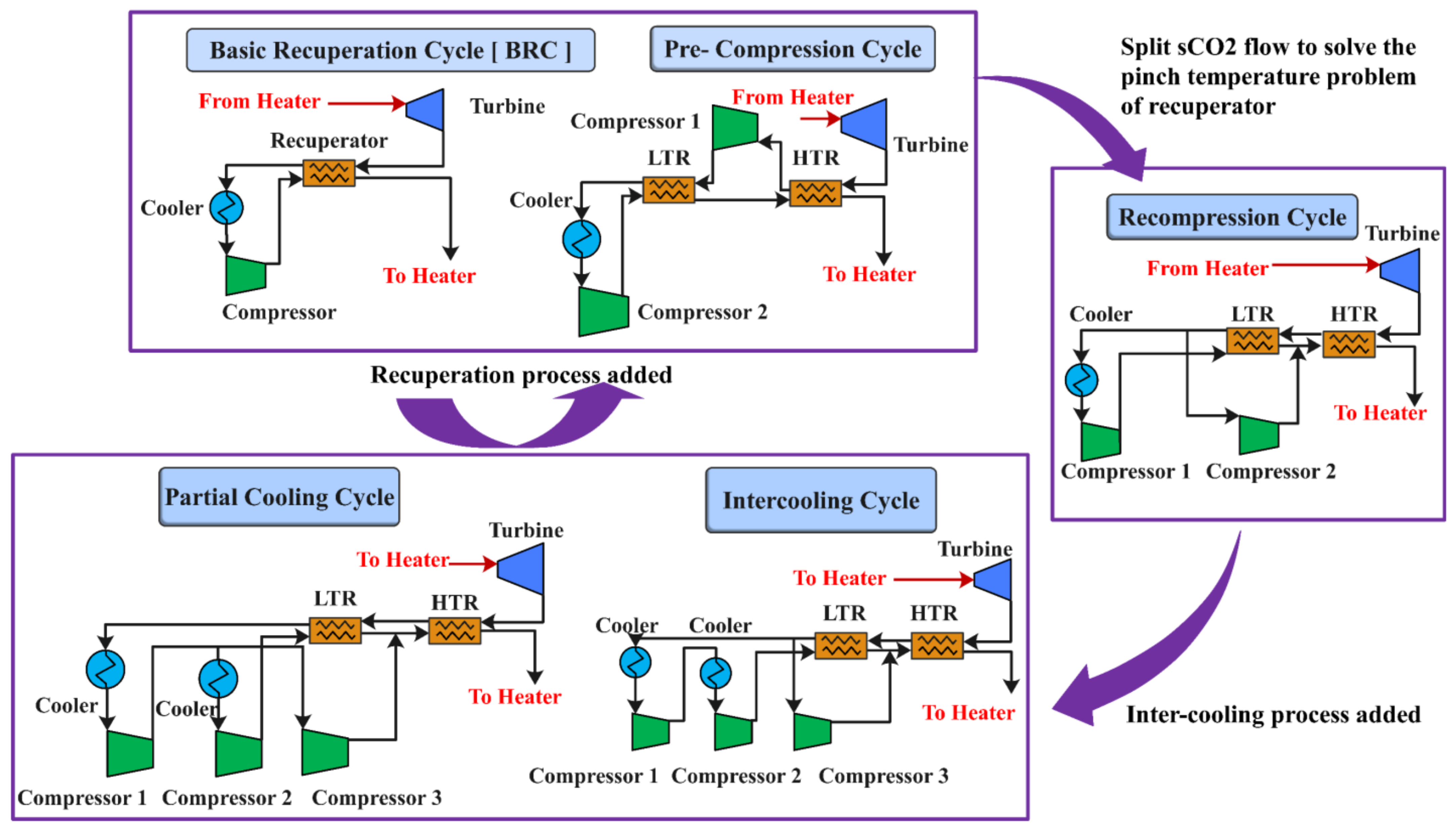
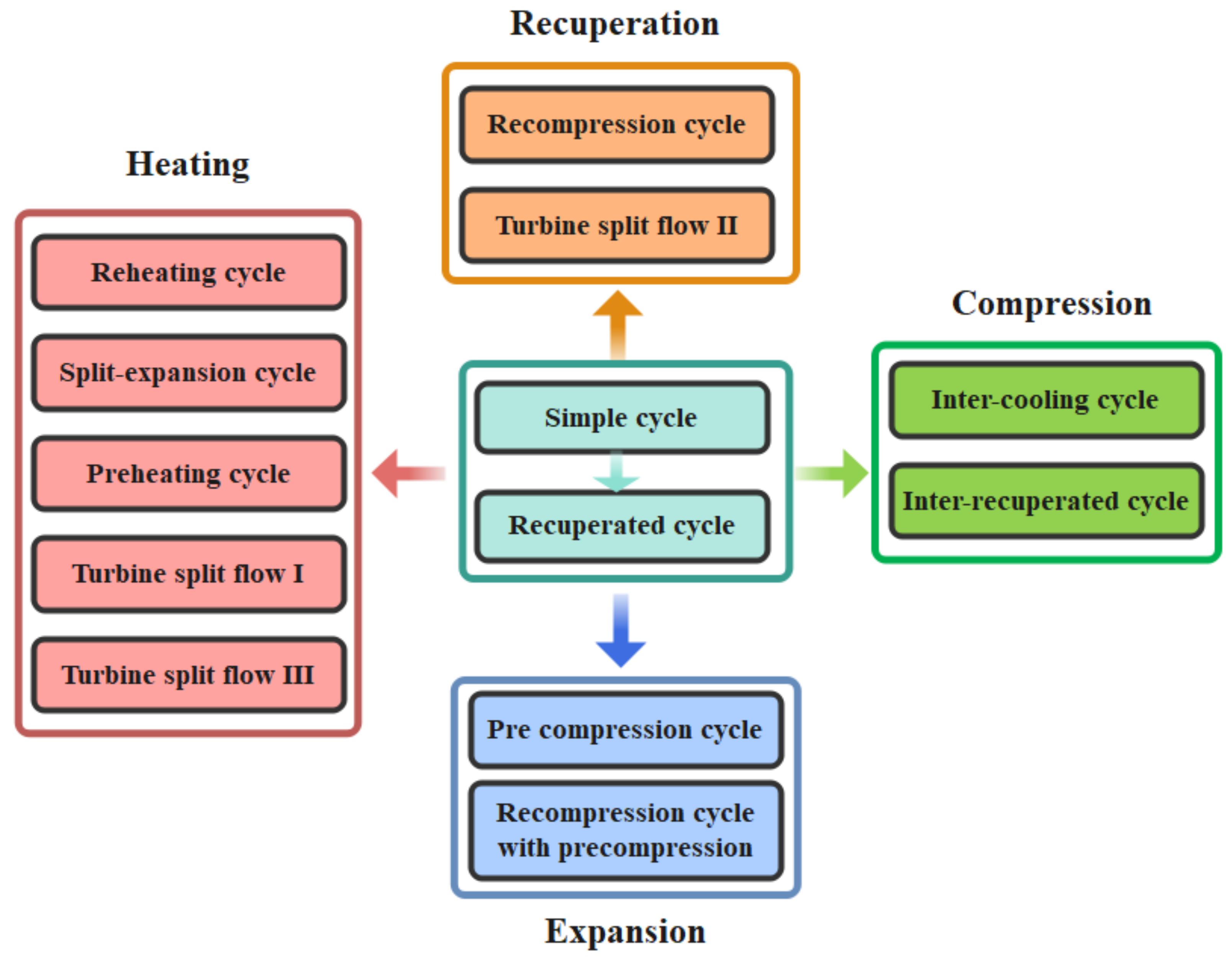
3.1. Classification of the sCO2 Brayton Cycles
3.2. Recent Advances in the sCO2 Brayton Cycle
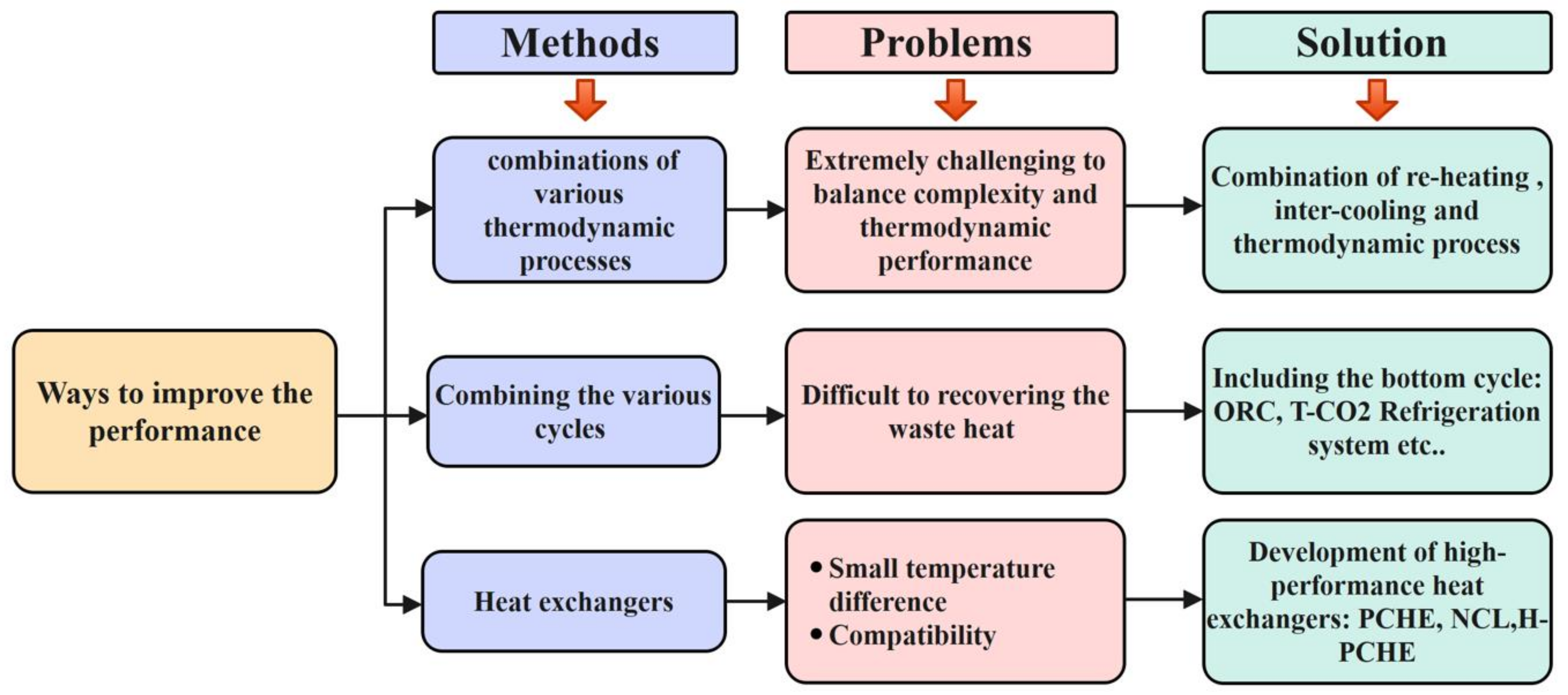
3.3. Methods to Enhance the Efficiency and Performance of the sCO2 Brayton Cycle
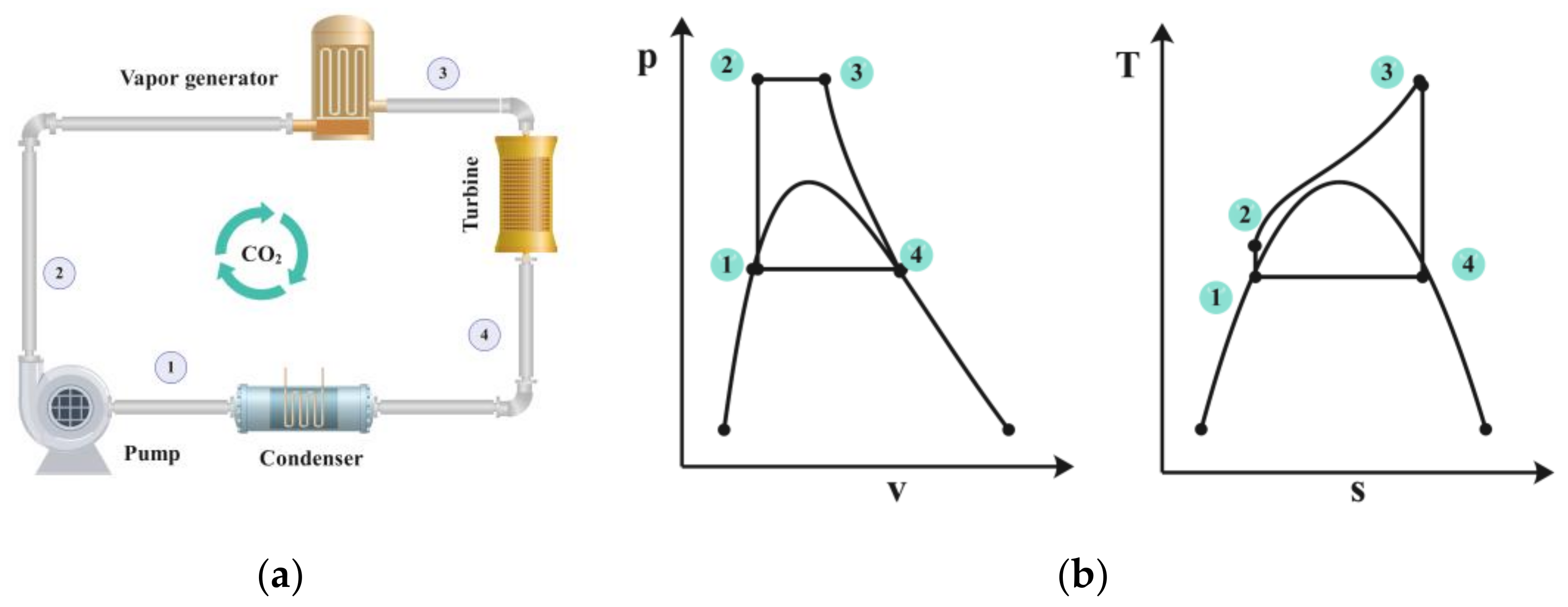
4. sCO2 Rankine Cycle
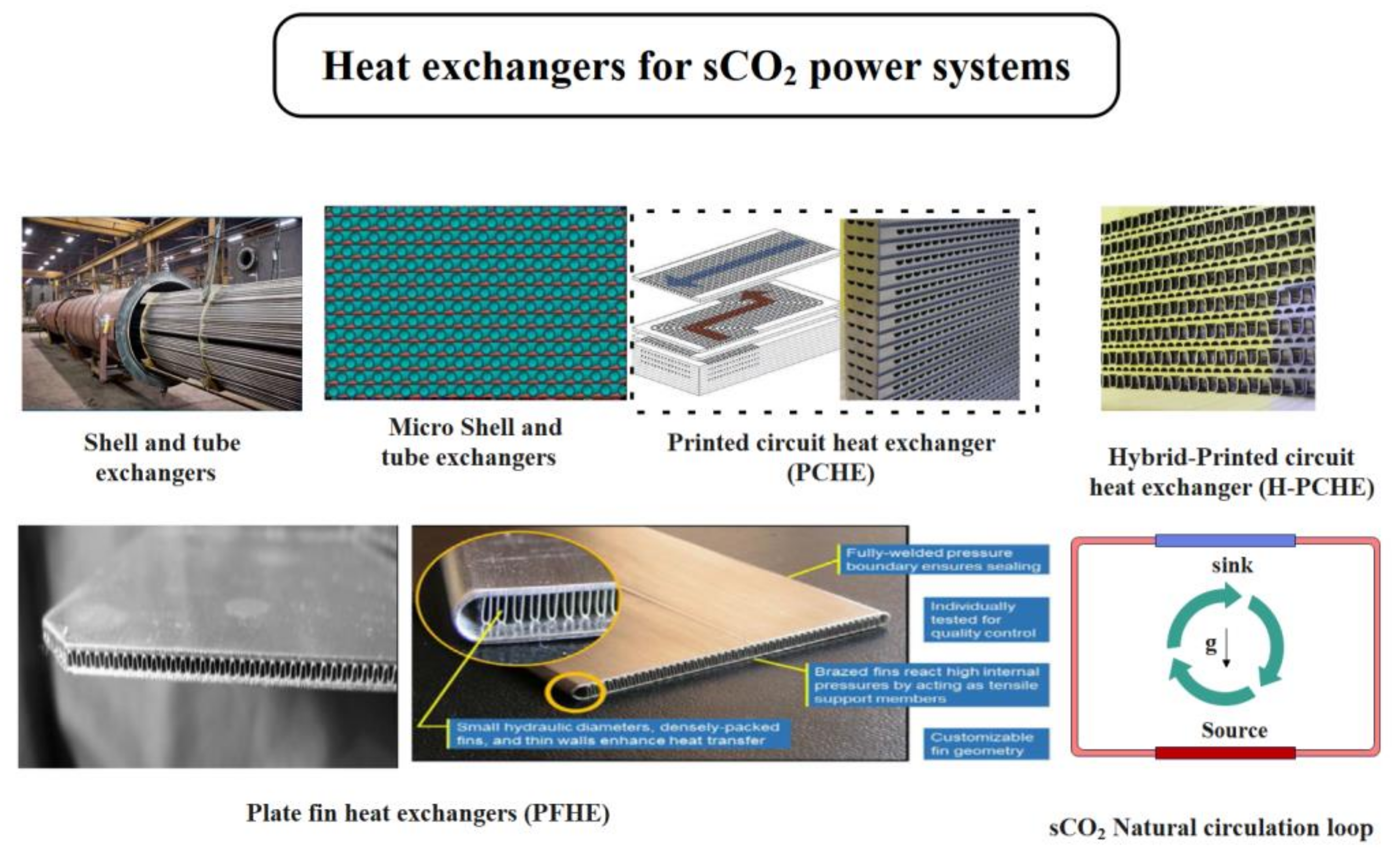
5. Heat Exchanger Technology for sCO2 Systems
6. sCO2 Cycle Application in Power Generation Industries
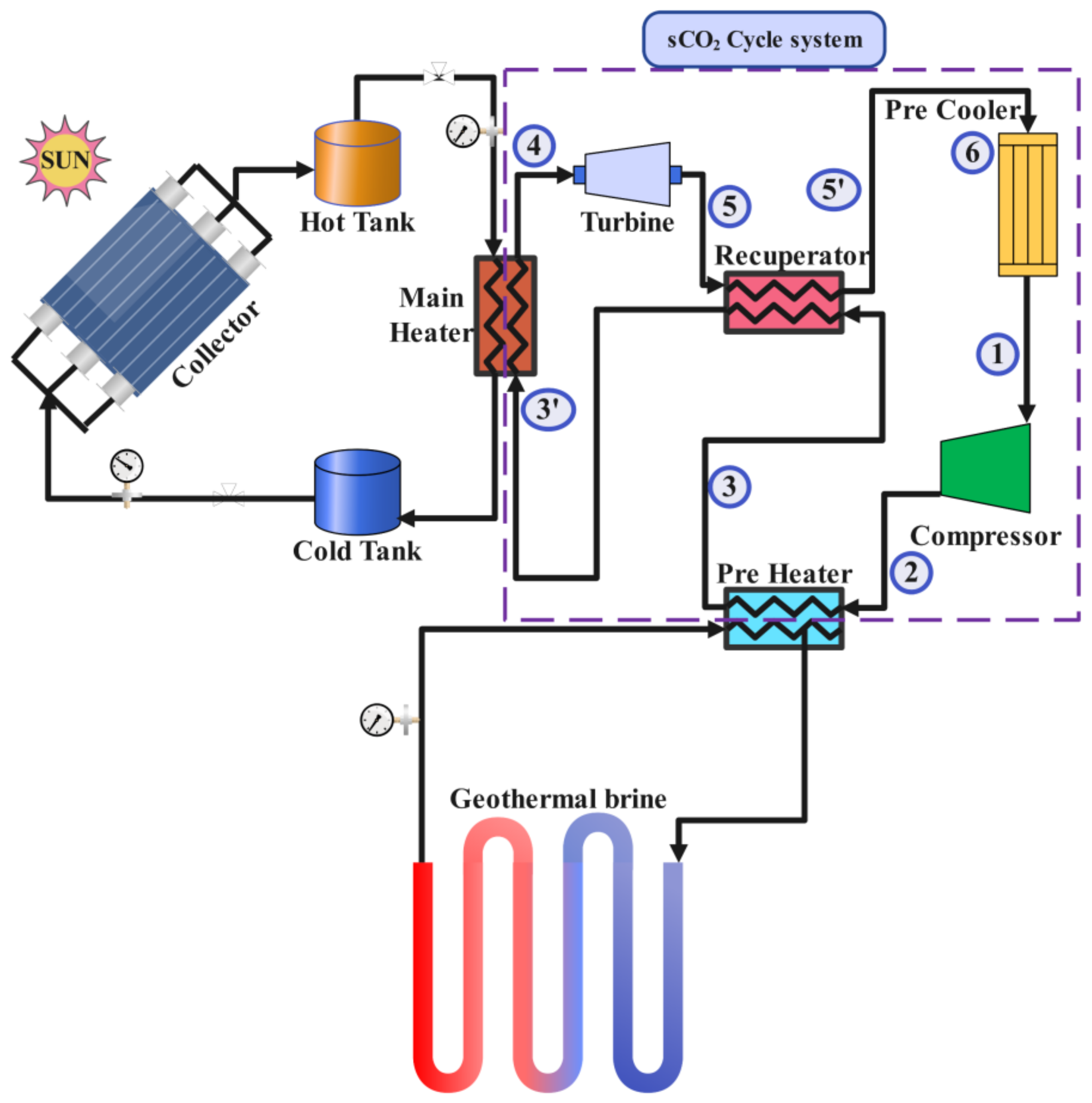
6.1. sCO2 Cycle in the Solar Power Industry
6.2. sCO2 Cycle in the Nuclear Industry
6.3. sCO2 Cycle in Miscellaneous Power Generation Industries
6.4. sCO2 Cycles Economic Analysis
7. Future Directions and Roadmap for the Commercialization of sCO2 Technologies
8. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- The Energy Institute. Statistical Review of World Energy 2023, 72nd ed.; The Energy Institute: London, UK, 2023. [Google Scholar]
- United Nations Sustainable Development Goals. Available online: https://sdgs.un.org/goals (accessed on 18 April 2024).
- Jradi, M.; Riffat, S. Tri-Generation Systems: Energy Policies, Prime Movers, Cooling Technologies, Configurations and Operation Strategies. Renew. Sustain. Energy Rev. 2014, 32, 396–415. [Google Scholar] [CrossRef]
- Abdelghafar, M.M.; Hassan, M.A.; Kayed, H. Comprehensive Analysis of Combined Power Cycles Driven by SCO2-Based Concentrated Solar Power: Energy, Exergy, and Exergoeconomic Perspectives. Energy Convers. Manag. 2024, 301, 118046. [Google Scholar] [CrossRef]
- Li, Q.; Erqi, E.; Qiu, Y.; Wang, J.; Zhang, Y. Conceptual Design of Novel He-SCO2 Brayton Cycles for Ultra-High-Temperature Concentrating Solar Power. Energy Convers. Manag. 2022, 260, 115618. [Google Scholar] [CrossRef]
- Luo, D.; Huang, D. Thermodynamic and Exergoeconomic Investigation of Various SCO2 Brayton Cycles for next Generation Nuclear Reactors. Energy Convers. Manag. 2020, 209, 112649. [Google Scholar] [CrossRef]
- Zhang, F.; Zhu, Y.; Li, C.; Jiang, P. Thermodynamic Optimization of Heat Transfer Process in Thermal Systems Using CO2 as the Working Fluid Based on Temperature Glide Matching. Energy 2018, 151, 376–386. [Google Scholar] [CrossRef]
- Xu, J.; Wang, X.; Sun, E.; Li, M. Economic Comparison between SCO2 Power Cycle and Water-Steam Rankine Cycle for Coal-Fired Power Generation System. Energy Convers. Manag. 2021, 238, 114150. [Google Scholar] [CrossRef]
- Yousef, M.S.; Santana, D. Thermodynamic and Exergoeconomic Optimization of a New Combined Cooling and Power System Based on Supercritical CO2 Recompression Brayton Cycle. Energy Convers. Manag. 2023, 295, 117592. [Google Scholar] [CrossRef]
- Yu, S.-C.; Chen, L.; Zhao, Y.; Li, H.-X.; Zhang, X.-R. A Brief Review Study of Various Thermodynamic Cycles for High Temperature Power Generation Systems. Energy Convers. Manag. 2015, 94, 68–83. [Google Scholar] [CrossRef]
- Siva Reddy, V.; Kaushik, S.C.; Tyagi, S.K. Exergetic Analysis of Solar Concentrator Aided Natural Gas Fired Combined Cycle Power Plant. Renew. Energy 2012, 39, 114–125. [Google Scholar] [CrossRef]
- Peng, S.; Hong, H.; Jin, H.; Wang, Z. An Integrated Solar Thermal Power System Using Intercooled Gas Turbine and Kalina Cycle. Energy 2012, 44, 732–740. [Google Scholar] [CrossRef]
- White, M.T.; Bianchi, G.; Chai, L.; Tassou, S.A.; Sayma, A.I. Review of Supercritical CO2 Technologies and Systems for Power Generation. Appl. Therm. Eng. 2021, 185, 116447. [Google Scholar] [CrossRef]
- Lemmon, E.W.; Bell, I.H.; Huber, M.L.; McLinden, M.O. NIST Standard Reference Database 23: Reference Fluid Thermodynamic and Transport Properties-REFPROP, Version 10.0. In Standard Reference Data Program; National Institute of Standards and Technology: Gaithersburg, MA, USA, 2018. [Google Scholar] [CrossRef]
- Ahn, Y.; Bae, S.J.; Kim, M.; Cho, S.K.; Baik, S.; Lee, J.I.; Cha, J.E. Review of Supercritical CO2 Power Cycle Technology and Current Status of Research and Development. Nucl. Eng. Technol. 2015, 47, 647–661. [Google Scholar] [CrossRef]
- Ming, Y.; Tian, R.; Zhao, F.; Luo, C.; Tan, S. Control Strategies and Transient Characteristics of a 5MWth Small Modular Supercritical CO2 Brayton-Cycle Reactor System. Appl. Therm. Eng. 2023, 235, 121302. [Google Scholar] [CrossRef]
- Manente, G.; Lazzaretto, A. Innovative Biomass to Power Conversion Systems Based on Cascaded Supercritical CO2 Brayton Cycles. Biomass Bioenergy 2014, 69, 155–168. [Google Scholar] [CrossRef]
- Guo, J.Q.; Li, M.J.; He, Y.L.; Jiang, T.; Ma, T.; Xu, J.L.; Cao, F. A Systematic Review of Supercritical Carbon Dioxide(S-CO2) Power Cycle for Energy Industries: Technologies, Key Issues, and Potential Prospects. Energy Convers. Manag. 2022, 258, 115437. [Google Scholar] [CrossRef]
- Wang, E.; Peng, N.; Zhang, M. System Design and Application of Supercritical and Transcritical CO2 Power Cycles: A Review. Front. Energy Res. 2021, 9, 723875. [Google Scholar] [CrossRef]
- Xu, J.; Sun, E.; Li, M.; Liu, H.; Zhu, B. Key Issues and Solution Strategies for Supercritical Carbon Dioxide Coal Fired Power Plant. Energy 2018, 157, 227–246. [Google Scholar] [CrossRef]
- Li, M.J.; Zhu, H.H.; Guo, J.Q.; Wang, K.; Tao, W.Q. The Development Technology and Applications of Supercritical CO2 Power Cycle in Nuclear Energy, Solar Energy and Other Energy Industries. Appl. Therm. Eng. 2017, 126, 255–275. [Google Scholar] [CrossRef]
- Fan, Y.H.; Tang, G.H.; Yang, D.L.; Li, X.L.; Wang, S.Q. Integration of S-CO2 Brayton Cycle and Coal-Fired Boiler: Thermal-Hydraulic Analysis and Design. Energy Convers. Manag. 2020, 225, 113452. [Google Scholar] [CrossRef]
- Zhou, A.; Li, X.-s.; Ren, X.-d.; Gu, C.w. w. Improvement Design and Analysis of a Supercritical CO2/Transcritical CO2 Combined Cycle for Offshore Gas Turbine Waste Heat Recovery. Energy 2020, 210, 118562. [Google Scholar] [CrossRef]
- Siddiqui, M.; Taimoor, A.; Almitani, K. Energy and Exergy Analysis of the S-CO2 Brayton Cycle Coupled with Bottoming Cycles. Processes 2018, 6, 153. [Google Scholar] [CrossRef]
- Wang, J.; Guo, Y.; Zhou, K.; Xia, J.; Li, Y.; Zhao, P.; Dai, Y. Design and Performance Analysis of Compressor and Turbine in Supercritical CO2 Power Cycle Based on System-Component Coupled Optimization. Energy Convers. Manag. 2020, 221, 113179. [Google Scholar] [CrossRef]
- Ma, Y.; Morosuk, T.; Liu, M.; Liu, J. Development and Comparison of Control Schemes for the Off-Design Operation of a Recompression Supercritical CO2 Cycle with an Intercooled Main Compressor. Energy 2020, 211, 119011. [Google Scholar] [CrossRef]
- Hu, H.; Jiang, Y.; Guo, C.; Liang, S. Thermodynamic and Exergy Analysis of a S-CO2 Brayton Cycle with Various of Cooling Modes. Energy Convers. Manag. 2020, 220, 113110. [Google Scholar] [CrossRef]
- Mecheri, M.; Le Moullec, Y. Supercritical CO2 Brayton Cycles for Coal-Fired Power Plants. Energy 2016, 103, 758–771. [Google Scholar] [CrossRef]
- Siddiqui, M.E.; Almitani, K.H. Energy Analysis of the S-CO2 Brayton Cycle with Improved Heat Regeneration. Processes 2018, 7, 3. [Google Scholar] [CrossRef]
- Siddiqui, M.E.; Almitani, K.H. Proposal and Thermodynamic Assessment of S-CO2 Brayton Cycle Layout for Improved Heat Recovery. Entropy 2020, 22, 305. [Google Scholar] [CrossRef]
- Li, H.; Xu, M.; Yan, X.; Li, J.; Su, W.; Wang, J.; Dai, Y. Preliminary Conceptual Exploration about Performance Improvement on Supercritical CO2 Power System via Integrating with Different Absorption Power Generation Systems. Energy Convers. Manag. 2018, 173, 219–232. [Google Scholar] [CrossRef]
- Guo, J.Q.; Li, M.J.; Xu, J.L.; Yan, J.J.; Ma, T. Energy, Exergy and Economic (3E) Evaluation and Conceptual Design of the 1000 MW Coal-Fired Power Plants Integrated with S-CO2 Brayton Cycles. Energy Convers. Manag. 2020, 211, 112713. [Google Scholar] [CrossRef]
- Sun, E.; Xu, J.; Li, M.; Liu, G.; Zhu, B. Connected-Top-Bottom-Cycle to Cascade Utilize Flue Gas Heat for Supercritical Carbon Dioxide Coal Fired Power Plant. Energy Convers. Manag. 2018, 172, 138–154. [Google Scholar] [CrossRef]
- Kim, Y.M.; Kim, C.G.; Favrat, D. Transcritical or Supercritical CO2 Cycles Using Both Low- and High-Temperature Heat Sources. Energy 2012, 43, 402–415. [Google Scholar] [CrossRef]
- Chen, Z.; Wang, Y.; Zhang, X. Energy and Exergy Analyses of S–CO2 Coal-Fired Power Plant with Reheating Processes. Energy 2020, 211, 118651. [Google Scholar] [CrossRef]
- Shi, L.; Shu, G.; Tian, H.; Huang, G.; Chen, T.; Li, X.; Li, D. Experimental Comparison between Four CO2-Based Transcritical Rankine Cycle (CTRC) Systems for Engine Waste Heat Recovery. Energy Convers. Manag. 2017, 150, 159–171. [Google Scholar] [CrossRef]
- Niu, X.D.; Yamaguchi, H.; Zhang, X.R.; Iwamoto, Y.; Hashitani, N. Experimental Study of Heat Transfer Characteristics of Supercritical CO2 Fluid in Collectors of Solar Rankine Cycle System. Appl. Therm. Eng. 2011, 31, 1279–1285. [Google Scholar] [CrossRef]
- Sarkar, J. Review and Future Trends of Supercritical CO2 Rankine Cycle for Low-Grade Heat Conversion. Renew. Sustain. Energy Rev. 2015, 48, 434–451. [Google Scholar] [CrossRef]
- Mohammadi, K.; Ellingwood, K.; Powell, K. A Novel Triple Power Cycle Featuring a Gas Turbine Cycle with Supercritical Carbon Dioxide and Organic Rankine Cycles: Thermoeconomic Analysis and Optimization. Energy Convers. Manag. 2020, 220, 113123. [Google Scholar] [CrossRef]
- Akbari, A.D.; Mahmoudi, S.M.S. Thermoeconomic Analysis & Optimization of the Combined Supercritical CO2 (Carbon Dioxide) Recompression Brayton/Organic Rankine Cycle. Energy 2014, 78, 501–512. [Google Scholar] [CrossRef]
- Ochoa, G.V.; Forero, J.D.; Rojas, J.P. Thermoeconomic Analysis of a Combined Supercritical CO2 Reheating under Different Configurations of Organic Rankine Cycle ORC as a Bottoming Cycle. Heliyon 2022, 8, e12230. [Google Scholar] [CrossRef]
- Karthikeyan, B.; Praveen Kumar, G. Thermoeconomic and Optimization Approaches for Integrating Cooling, Power, and Green Hydrogen Production in Dairy Plants with a Novel Solar-Biomass Cascade ORC System. Energy Convers. Manag. 2023, 295, 117645. [Google Scholar] [CrossRef]
- Bhuvaneshwaran, K.; Govindasamy, P.K. Technical Assessment of Novel Organic Rankine Cycle Driven Cascade Refrigeration System Using Environmental Friendly Refrigerants: 4E and Optimization Approaches. Environ. Sci. Pollut. Res. 2023, 30, 35096–35114. [Google Scholar] [CrossRef]
- Kim, I.H.; No, H.C. Thermal Hydraulic Performance Analysis of a Printed Circuit Heat Exchanger Using a Helium-Water Test Loop and Numerical Simulations. Appl. Therm. Eng. 2011, 31, 4064–4073. [Google Scholar] [CrossRef]
- Zhao, J.-W.; Zhao, R.; Nian, Y.-L.; Cheng, W.-L. Experimental Study of Supercritical CO2 in a Vertical Adaptive Flow Path Heat Exchanger. Appl. Therm. Eng. 2021, 188, 116597. [Google Scholar] [CrossRef]
- Cabeza, L.F.; de Gracia, A.; Fernández, A.I.; Farid, M.M. Supercritical CO2 as Heat Transfer Fluid: A Review. Appl. Therm. Eng. 2017, 125, 799–810. [Google Scholar] [CrossRef]
- Xu, X.Y.; Wang, Q.W.; Li, L.; Chen, Y.T.; Ma, T. Study on Thermal Resistance Distribution and Local Heat Transfer Enhancement Method for SCO2–Water Heat Exchange Process near Pseudo-Critical Temperature. Int. J. Heat Mass Transf. 2015, 82, 179–188. [Google Scholar] [CrossRef]
- Shi, H.-Y.; Li, M.-J.; Wang, W.-Q.; Qiu, Y.; Tao, W.-Q. Heat Transfer and Friction of Molten Salt and Supercritical CO2 Flowing in an Airfoil Channel of a Printed Circuit Heat Exchanger. Int. J. Heat Mass Transf. 2020, 150, 119006. [Google Scholar] [CrossRef]
- Seo, H.; Cha, J.E.; Kim, J.; Sah, I.; Kim, Y.-W. Design and Performance Analysis of a Supercritical Carbon Dioxide Heat Exchanger. Appl. Sci. 2020, 10, 4545. [Google Scholar] [CrossRef]
- Theologou, K.; Hofer, M.; Mertz, R.; Buck, M.; Laurien, E.; Starflinger, J. Experimental Investigation and Modelling of Steam-Heated Supercritical CO2 Compact Cross-Flow Heat Exchangers. Appl. Therm. Eng. 2021, 190, 116352. [Google Scholar] [CrossRef]
- Kim, I.H.; No, H.C. Physical Model Development and Optimal Design of PCHE for Intermediate Heat Exchangers in HTGRs. Nucl. Eng. Des. 2012, 243, 243–250. [Google Scholar] [CrossRef]
- Lee, S.M.; Kim, K.Y.; Kim, S.W. Multi-Objective Optimization of a Double-Faced Type Printed Circuit Heat Exchanger. Appl. Therm. Eng. 2013, 60, 44–50. [Google Scholar] [CrossRef]
- Cheng, K.; Zhou, J.; Huai, X.; Guo, J. Experimental Exergy Analysis of a Printed Circuit Heat Exchanger for Supercritical Carbon Dioxide Brayton Cycles. Appl. Therm. Eng. 2021, 192, 116882. [Google Scholar] [CrossRef]
- Ma, T.; Li, M.J.; Xu, J.L.; Cao, F. Thermodynamic Analysis and Performance Prediction on Dynamic Response Characteristic of PCHE in 1000 MW S-CO2 Coal Fired Power Plant. Energy 2019, 175, 123–138. [Google Scholar] [CrossRef]
- Meshram, A.; Jaiswal, A.K.; Khivsara, S.D.; Ortega, J.D.; Ho, C.; Bapat, R.; Dutta, P. Modeling and Analysis of a Printed Circuit Heat Exchanger for Supercritical CO2 Power Cycle Applications. Appl. Therm. Eng. 2016, 109, 861–870. [Google Scholar] [CrossRef]
- Baik, S.; Kim, S.G.; Lee, J.; Lee, J.I. Study on CO2—Water Printed Circuit Heat Exchanger Performance Operating under Various CO2 Phases for S-CO2 Power Cycle Application. Appl. Therm. Eng. 2017, 113, 1536–1546. [Google Scholar] [CrossRef]
- Gkountas, A.A.; Stamatelos, A.M.; Kalfas, A.I. Recuperators Investigation for High Temperature Supercritical Carbon Dioxide Power Generation Cycles. Appl. Therm. Eng. 2017, 125, 1094–1102. [Google Scholar] [CrossRef]
- Sullivan, S.D.; Kesseli, J.; Nash, J.; Farias, J.; Kesseli, D.; Caruso, W. High-Efficiency Low-Cost Solar Receiver for Use Ina a Supercritical CO2 Recompression Cycle. In High-Efficiency Low-Cost Solar Receiver for Use Ina a Supercritical CO2 Recompression Cycle; No. DOE-BRAYTON-0005799; Brayton Energy, LLC: Portsmouth, NH, USA, 2016. [Google Scholar] [CrossRef]
- Chordia, L.; Portnoff, M.A.; Green, E. High Temperature Heat Exchanger Design and Fabrication for Systems with Large Pressure Differentials; Thar Energy, LLC: Pittsburgh, PA, USA; Morgantown, WV, USA, 2017. [Google Scholar]
- Boopalan, V.; Kumar Arumugam, S.; Rajesh Kanna, P. Effect of Operating Regimes on the Heat Transfer and Buoyancy Characteristics of Supercritical CO2 Natural Circulation Loop—A Numerical Study. Therm. Sci. Eng. Prog. 2024, 50, 102579. [Google Scholar] [CrossRef]
- Boopalan, V.; Kumar Arumugam, S.; Rajesh Kanna, P. Numerical Investigation on the Impact of Circumferentially Varying Temperature on the Buoyancy and Heat Transfer Characteristics of Supercritical CO2 Natural Circulation Loop. Appl. Therm. Eng. 2024, 248, 123165. [Google Scholar] [CrossRef]
- Boopalan, V.; Kanna, P.R.; Arumugam, S.K. Numerical Investigation of Thermohydraulic Characteristics in Supercritical CO2 Natural Circulation Loop with Spatially Varying Temperature. Heat Transf. Eng. 2024, 45, 1–17. [Google Scholar] [CrossRef]
- Yamaguchi, H.; Sawada, N.; Suzuki, H.; Ueda, H.; Zhang, X.R. Preliminary Study on a Solar Water Heater Using Supercritical Carbon Dioxide as Working Fluid. J. Sol. Energy Eng. 2010, 132, 011010. [Google Scholar] [CrossRef]
- Atrens, A.D.; Gurgenci, H.; Rudolph, V. CO2 Thermosiphon for Competitive Geothermal Power Generation. Energy Fuels 2009, 23, 553–557. [Google Scholar] [CrossRef]
- Dimmick, G.R.; Chatoorgoon, V.; Khartabil, H.F.; Duffey, R.B. Natural-Convection Studies for Advanced CANDU Reactor Concepts. Nucl. Eng. Des. 2002, 215, 27–38. [Google Scholar] [CrossRef]
- Sinha, R.K.; Kakodkar, A. Design and Development of the AHWR—The Indian Thorium Fuelled Innovative Nuclear Reactor. Nucl. Eng. Des. 2006, 236, 683–700. [Google Scholar] [CrossRef]
- Kaga, S.; Nomura, T.; Seki, K.; Hirano, A. Development of Compact Inverter Refrigerating System Using R600a/CO2 by Thermo-Siphon. In Proceedings of the 8th Gustav Lorentzen Conference on Natural Working Fluids, Copenhagen, Denmark, 7–10 September 2008; pp. 3–4. [Google Scholar]
- Turchi, C.S.; Ma, Z.; Neises, T.W.; Wagner, M.J. Thermodynamic Study of Advanced Supercritical Carbon Dioxide Power Cycles for Concentrating Solar Power Systems. J. Sol. Energy Eng. 2013, 135, 041007. [Google Scholar] [CrossRef]
- Li, X.; Huang, H.; Zhao, W. A Supercritical or Transcritical Rankine Cycle with Ejector Using Low-Grade Heat. Energy Convers. Manag. 2014, 78, 551–558. [Google Scholar] [CrossRef]
- Singh, H.; Mishra, R.S. Energy- and Exergy-Based Performance Evaluation of Solar Powered Combined Cycle (Recompression Supercritical Carbon Dioxide Cycle/Organic Rankine Cycle). Clean Energy 2018, 2, 140–153. [Google Scholar] [CrossRef]
- Mohammadi, Z.; Fallah, M.; Mahmoudi, S.M.S. Advanced Exergy Analysis of Recompression Supercritical CO2 Cycle. Energy 2019, 178, 631–643. [Google Scholar] [CrossRef]
- Xu, C.; Xin, T.; Li, X.; Li, S.; Sun, Y.; Liu, W.; Yang, Y. A Thermodynamic Analysis of a Solar Hybrid Coal-Based Direct-Fired Supercritical Carbon Dioxide Power Cycle. Energy Convers. Manag. 2019, 196, 77–91. [Google Scholar] [CrossRef]
- Jose, J.; Parthasarathy, R.K.; Arumugam, S.K. Energy and Exergy Analysis of a Combined Cooling Heating and Power System with Regeneration. Sustainability 2023, 15, 13523. [Google Scholar] [CrossRef]
- Pérez-Pichel, G.D.; Linares, J.I.; Herranz, L.E.; Moratilla, B.Y. Thermal Analysis of Supercritical CO2 Power Cycles: Assessment of Their Suitability to the Forthcoming Sodium Fast Reactors. Nucl. Eng. Des. 2012, 250, 23–34. [Google Scholar] [CrossRef]
- Floyd, J.; Alpy, N.; Moisseytsev, A.; Haubensack, D.; Rodriguez, G.; Sienicki, J.; Avakian, G. A Numerical Investigation of the SCO2 Recompression Cycle Off-Design Behaviour, Coupled to a Sodium Cooled Fast Reactor, for Seasonal Variation in the Heat Sink Temperature. Nucl. Eng. Des. 2013, 260, 78–92. [Google Scholar] [CrossRef]
- Le Moullec, Y. Conceptual Study of a High Efficiency Coal-Fired Power Plant with CO2 Capture Using a Supercritical CO2 Brayton Cycle. Energy 2013, 49, 32–46. [Google Scholar] [CrossRef]
- Li, M.; Wang, J.; Li, S.; Wang, X.; He, W.; Dai, Y. Thermo-Economic Analysis and Comparison of a CO2 Transcritical Power Cycle and an Organic Rankine Cycle. Geothermics 2014, 50, 101–111. [Google Scholar] [CrossRef]
- Serrano, I.P.; Linares, J.I.; Cantizano, A.; Moratilla, B.Y. Enhanced Arrangement for Recuperators in Supercritical CO2 Brayton Power Cycle for Energy Conversion in Fusion Reactors. Fusion Eng. Des. 2014, 89, 1909–1912. [Google Scholar] [CrossRef]
- Baronci, A.; Messina, G.; McPhail, S.J.; Moreno, A. Numerical Investigation of a MCFC (Molten Carbonate Fuel Cell) System Hybridized with a Supercritical CO2 Brayton Cycle and Compared with a Bottoming Organic Rankine Cycle. Energy 2015, 93, 1063–1073. [Google Scholar] [CrossRef]
- Linares, J.I.; Herranz, L.E.; Fernández, I.; Cantizano, A.; Moratilla, B.Y. Supercritical CO2 Brayton Power Cycles for DEMO Fusion Reactor Based on Helium Cooled Lithium Lead Blanket. Appl. Therm. Eng. 2015, 76, 123–133. [Google Scholar] [CrossRef]
- Hu, L.; Chen, D.; Huang, Y.; Li, L.; Cao, Y.; Yuan, D.; Wang, J.; Pan, L. Investigation on the Performance of the Supercritical Brayton Cycle with CO2-Based Binary Mixture as Working Fluid for an Energy Transportation System of a Nuclear Reactor. Energy 2015, 89, 874–886. [Google Scholar] [CrossRef]
- Wang, M.; Zhao, P.; Wang, J.; Li, H.; Dai, Y. Conceptual Design and Parametric Study of Combined Carbon Dioxide/Organic Rankine Cycles. Appl. Therm. Eng. 2016, 103, 759–772. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, H.; Han, W.; Bai, W.; Yang, Y.; Yao, M.; Wang, Y. Improved Design of Supercritical CO2 Brayton Cycle for Coal-Fired Power Plant. Energy 2018, 155, 1–14. [Google Scholar] [CrossRef]
- Xia, M.; Yao, S.; Ying, C. Analysis and Multi-Objective Optimization of SOFC/GT/SCO2 Hybrid Power System Based on Thermodynamics and Economics. Appl. Therm. Eng. 2023, 232, 121033. [Google Scholar] [CrossRef]
- Hou, S.; Zhou, Y.; Yu, L.; Zhang, F.; Cao, S.; Wu, Y. Optimization of a Novel Cogeneration System Including a Gas Turbine, a Supercritical CO2 Recompression Cycle, a Steam Power Cycle and an Organic Rankine Cycle. Energy Convers. Manag. 2018, 172, 457–471. [Google Scholar] [CrossRef]
- Singh, H.; Mishra, R.S. Performance Analysis of Solar Parabolic Trough Collectors Driven Combined Supercritical CO2 and Organic Rankine Cycle. Eng. Sci. Technol. Int. J. 2018, 21, 451–464. [Google Scholar] [CrossRef]
- Song, J.; Li, X.-s.; Ren, X.-d.; Gu, C.-w. Performance Analysis and Parametric Optimization of Supercritical Carbon Dioxide (S-CO2) Cycle with Bottoming Organic Rankine Cycle (ORC). Energy 2018, 143, 406–416. [Google Scholar] [CrossRef]
- Park, J.H.; Park, H.S.; Kwon, J.G.; Kim, T.H.; Kim, M.H. Optimization and Thermodynamic Analysis of Supercritical CO2 Brayton Recompression Cycle for Various Small Modular Reactors. Energy 2018, 160, 520–535. [Google Scholar] [CrossRef]
- Li, M.J.; Jie, Y.J.; Zhu, H.H.; Qi, G.J.; Li, M.J. The Thermodynamic and Cost-Benefit-Analysis of Miniaturized Lead-Cooled Fast Reactor with Supercritical CO2 Power Cycle in the Commercial Market. Prog. Nucl. Energy 2018, 103, 135–150. [Google Scholar] [CrossRef]
- Weiland, N.T.; White, C.W. Techno-Economic Analysis of an Integrated Gasification Direct-Fired Supercritical CO2 Power Cycle. Fuel 2018, 212, 613–625. [Google Scholar] [CrossRef]
- Mishra, R.S.; Singh, H. Detailed Parametric Analysis of Solar Driven Supercritical CO2 Based Combined Cycle for Power Generation, Cooling and Heating Effect by Vapor Absorption Refrigeration as a Bottoming Cycle. Therm. Sci. Eng. Prog. 2018, 8, 397–410. [Google Scholar] [CrossRef]
- Ma, T.; Li, M.J.; Xu, J.L.; Ni, J.W.; Tao, W.Q.; Wang, L. Study of Dynamic Response Characteristics of S-CO2 Cycle in Coal-Fired Power Plants Based on Real-Time Micro-Grid Load and a Novel Synergistic Control Method with Variable Working Conditions. Energy Convers. Manag. 2022, 254, 115264. [Google Scholar] [CrossRef]
- Xiao, T.; Liu, C.; Liu, L.; Wang, S.; Tang, J. A Nuclear Driven Hybrid SCO2 Power Cycle/Membrane Distillation System for Water-Electricity Cogeneration. Energy Convers. Manag. 2022, 271, 116329. [Google Scholar] [CrossRef]
- Wang, G.; Dong, B.; Chen, Z. Design and Behaviour Estimate of a Novel Concentrated Solar-Driven Power and Desalination System Using S–CO2 Brayton Cycle and MSF Technology. Renew. Energy 2021, 176, 555–564. [Google Scholar] [CrossRef]
- Sun, E.; Xu, J.; Li, M.; Li, H.; Liu, C.; Xie, J. Synergetics: The Cooperative Phenomenon in Multi-Compressions S-CO2 Power Cycles. Energy Convers. Manag. X 2020, 7, 100042. [Google Scholar] [CrossRef]
- Ehsan, M.M.; Duniam, S.; Guan, Z.; Gurgenci, H.; Klimenko, A. Seasonal Variation on the Performance of the Dry Cooled Supercritical CO2 Recompression Cycle. Energy Convers. Manag. 2019, 197, 111865. [Google Scholar] [CrossRef]
- Li, H.; Zhang, Y.; Yao, M.; Yang, Y.; Han, W.; Bai, W. Design Assessment of a 5 MW Fossil-Fired Supercritical CO2 Power Cycle Pilot Loop. Energy 2019, 174, 792–804. [Google Scholar] [CrossRef]
- Wang, K.; He, Y.L. Thermodynamic Analysis and Optimization of a Molten Salt Solar Power Tower Integrated with a Recompression Supercritical CO2 Brayton Cycle Based on Integrated Modeling. Energy Convers. Manag. 2017, 135, 336–350. [Google Scholar] [CrossRef]
- González-Portillo, L.F.; Muñoz-Antón, J.; Martínez-Val, J.M. Supercritical Carbon Dioxide Cycles with Multi-Heating in Concentrating Solar Power Plants. Sol. Energy 2020, 207, 144–156. [Google Scholar] [CrossRef]
- Fan, G.; Li, H.; Du, Y.; Chen, K.; Zheng, S.; Dai, Y. Preliminary Design and Part-Load Performance Analysis of a Recompression Supercritical Carbon Dioxide Cycle Combined with a Transcritical Carbon Dioxide Cycle. Energy Convers. Manag. 2020, 212, 112758. [Google Scholar] [CrossRef]
- Li, M.J.; Xu, J.L.; Cao, F.; Guo, J.Q.; Tong, Z.X.; Zhu, H.H. The Investigation of Thermo-Economic Performance and Conceptual Design for the Miniaturized Lead-Cooled Fast Reactor Composing Supercritical CO2 Power Cycle. Energy 2019, 173, 174–195. [Google Scholar] [CrossRef]
- Song, J.; Wang, Y.; Wang, K.; Wang, J.; Markides, C.N. Combined Supercritical CO2 (SCO2) Cycle and Organic Rankine Cycle (ORC) System for Hybrid Solar and Geothermal Power Generation: Thermoeconomic Assessment of Various Configurations. Renew. Energy 2021, 174, 1020–1035. [Google Scholar] [CrossRef]
- Tafur-Escanta, P.; Valencia-Chapi, R.; López-Guillem, M.; Fierros-Peraza, O.; Muñoz-Antón, J. Electrical Energy Storage Using a Supercritical CO2 Heat Pump. Energy Rep. 2022, 8, 502–507. [Google Scholar] [CrossRef]
- Ryu, J.Y.; Ko, A.R.; Park, S.H.; Park, J.P. Thermo-Economic Assessment of Molten Carbonate Fuel Cell Hybrid System Combined between Individual SCO2 Power Cycle and District Heating. Appl. Therm. Eng. 2020, 169, 114911. [Google Scholar] [CrossRef]
- Goyal, K.; Singh, H.; Bhatia, R. Behaviour of Carbon Nanotubes-Cr2O3 Thermal Barrier Coatings in Actual Boiler. Surf. Eng. 2020, 36, 124–134. [Google Scholar] [CrossRef]
- Ramachandran, K.; Boopalan, V.; Bear, J.C.; Subramani, R. Multi-Walled Carbon Nanotubes (MWCNTs)-Reinforced Ceramic Nanocomposites for Aerospace Applications: A Review. J. Mater. Sci. 2021, 57, 3923–3953. [Google Scholar] [CrossRef]
- Ramachandran, K.; Ram Subramani, R.; Arunkumar, T.; Boopalan, V. Mechanical and Thermal Properties of Spark Plasma Sintered Alumina-MWCNTs Nanocomposites Prepared via Improvised Colloidal Route. Mater. Chem. Phys. 2021, 272, 125034. [Google Scholar] [CrossRef]
- Jiang, Y.; Zhan, L.; Tian, X.; Nie, C. Thermodynamic Performance Comparison and Optimization of SCO2 Brayton Cycle, TCO2 Brayton Cycle and TCO2 Rankine Cycle. J. Therm. Sci. 2023, 32, 611–627. [Google Scholar] [CrossRef]
- Yang, J.; Yang, Z.; Duan, Y. Design Optimization and Operating Performance of S-CO2 Brayton Cycle under Fluctuating Ambient Temperature and Diverse Power Demand Scenarios. J. Therm. Sci. 2024, 33, 190–206. [Google Scholar] [CrossRef]
- Marchionni, M.; Bianchi, G.; Tassou, S.A. Review of Supercritical Carbon Dioxide (SCO2) Technologies for High-Grade Waste Heat to Power Conversion. SN Appl. Sci. 2020, 2, 611. [Google Scholar] [CrossRef]
- Berthet Couso, G.; Barraza Vicencio, R.; Vasquez Padilla, R.; Soo Too, Y.C.; Pye, J. Dynamic Model of Supercritical CO2 Brayton Cycles Driven by Concentrated Solar Power. In Proceedings of the ASME 2017 11th International Conference on Energy Sustainability, Charlotte, NC, USA, 26–30 June 2017. [Google Scholar]
- Ming, Y.; Shen, H.; Cammi, A.; Zhao, F.; Tian, R. Modeling and Safety Analysis of Supercritical CO2 Brayton Cycle Reactor System under Loss of Coolant Accident (LOCA). Ann. Nucl. Energy 2024, 207, 110740. [Google Scholar] [CrossRef]
- Li, Z.; Tian, S.; Zhang, D.; Chang, C.; Zhang, Q.; Zhang, P. Optimization Study on Improving Energy Efficiency of Power Cycle System of Staged Coal Gasification Coupled with Supercritical Carbon Dioxide. Energy 2022, 239, 122168. [Google Scholar] [CrossRef]
- Thanganadar, D.; Asfand, F.; Patchigolla, K.; Turner, P. Techno-Economic Analysis of Supercritical Carbon Dioxide Cycle Integrated with Coal-Fired Power Plant. Energy Convers. Manag. 2021, 242, 114294. [Google Scholar] [CrossRef]
- Sun, R.; Liu, M.; Chen, X.; Yang, K.; Yan, J. Thermodynamic Optimization on Supercritical Carbon Dioxide Brayton Cycles to Achieve Combined Heat and Power Generation. Energy Convers. Manag. 2022, 251, 114929. [Google Scholar] [CrossRef]
- Thanganadar, D.; Asfand, F.; Patchigolla, K. Thermal Performance and Economic Analysis of Supercritical Carbon Dioxide Cycles in Combined Cycle Power Plant. Appl. Energy 2019, 255, 113836. [Google Scholar] [CrossRef]
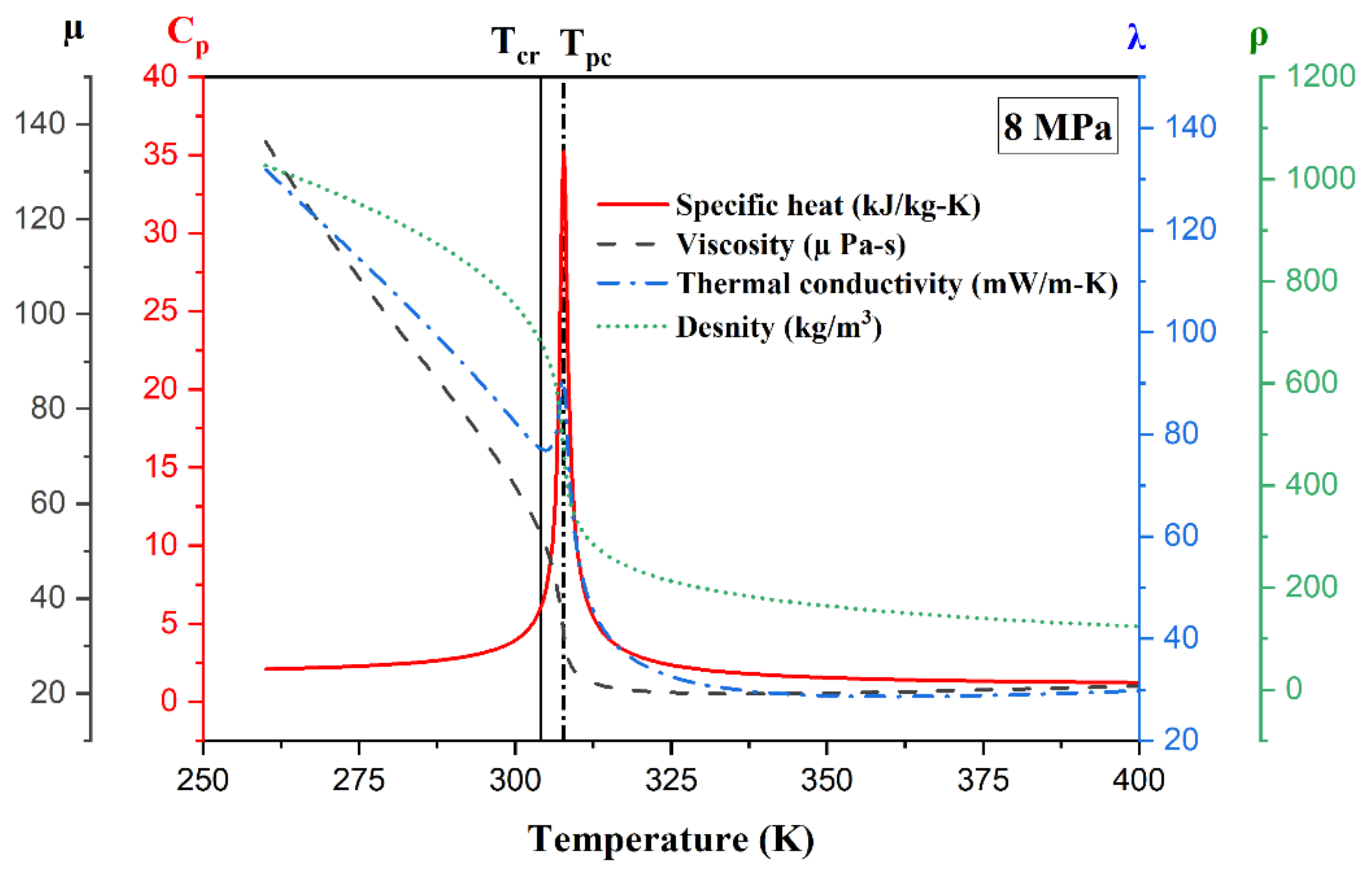
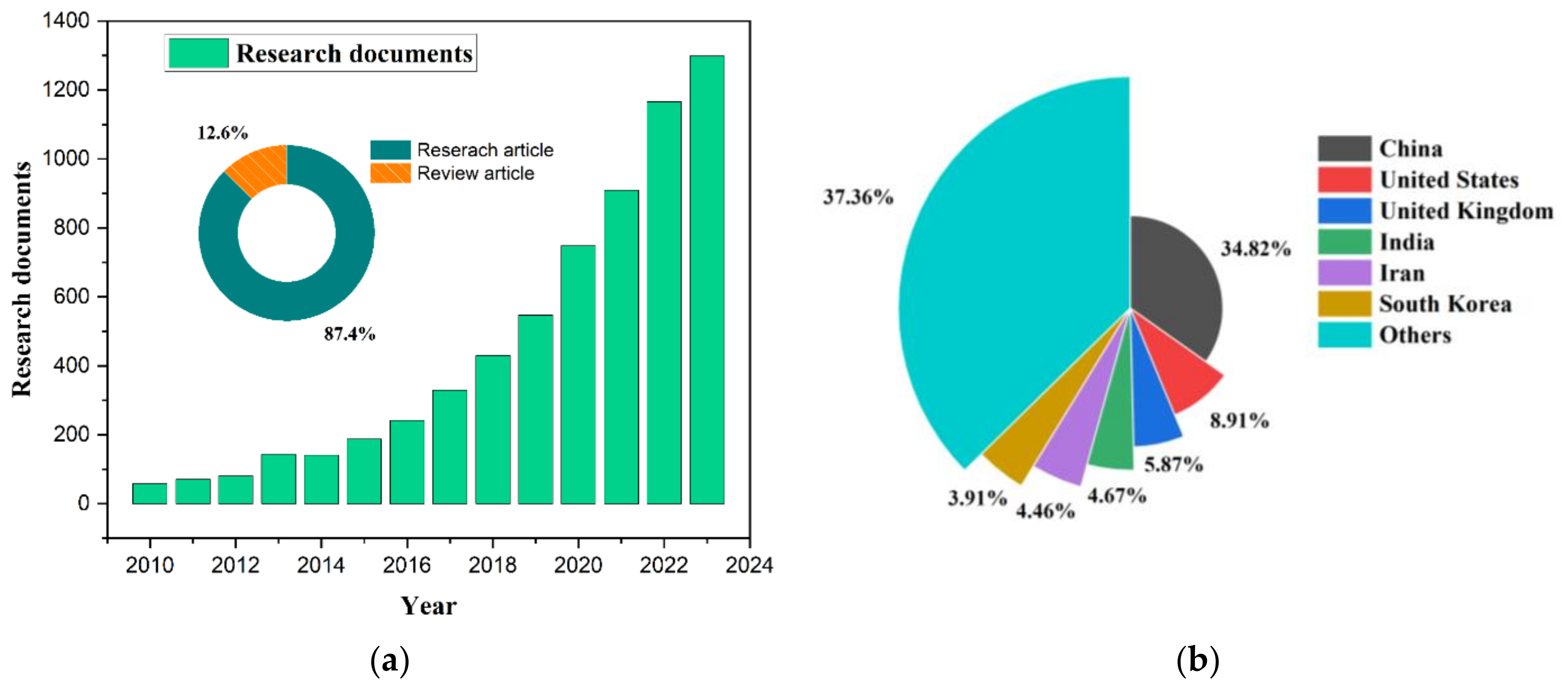
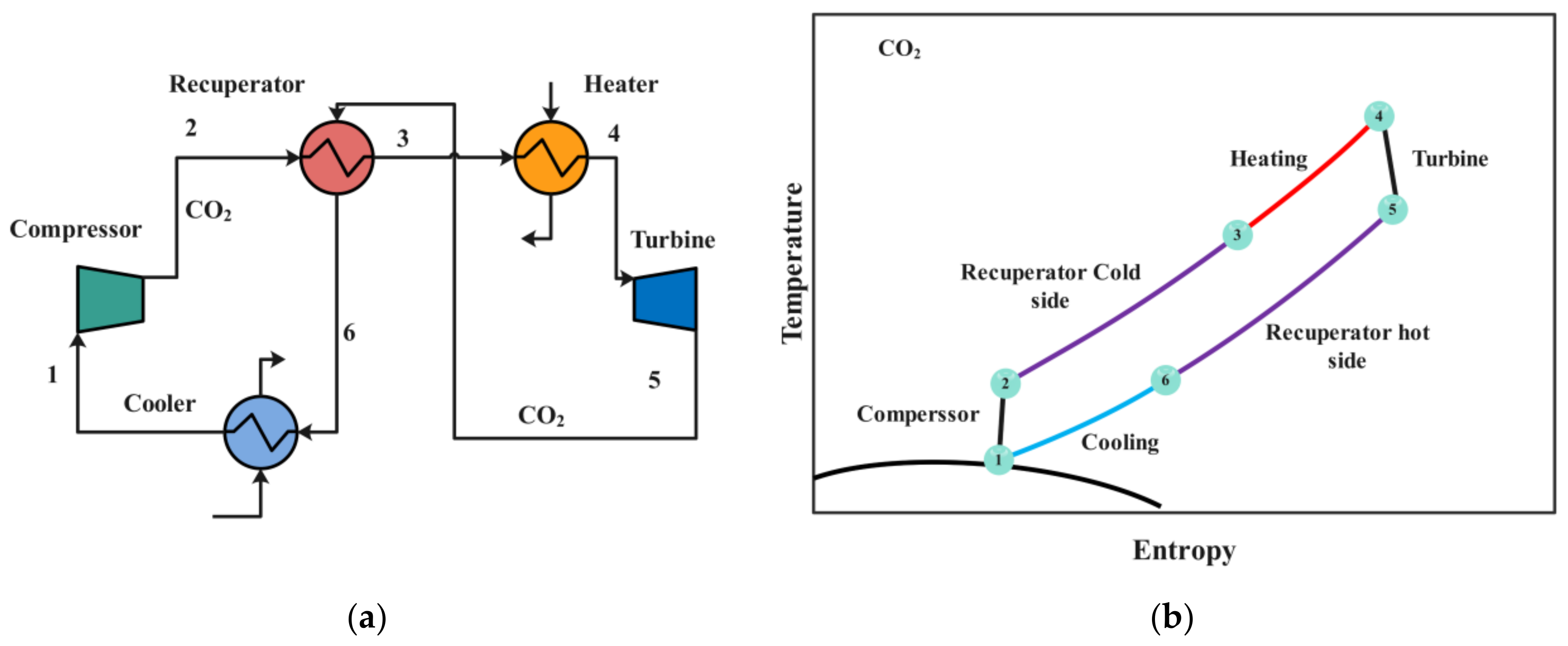



| Author(s) | sCO2 System Layout | First Law Analysis | Second Law Analysis | Economic Analysis |
|---|---|---|---|---|
| Pérez-Pichel et al. [74] | sCO2 + sodium fast reactor | 43.31% | - | - |
| Turchi et al. [68] | sCO2 + CSP | >50% | - | - |
| Floyd et al. [75] | sCO2 recompression + sodium fast reactor | 42.2% | - | - |
| Le Moullec [76] | sCO2 + coal-fired plant | 41.3% net plant efficiency (LHV) | - | Cost of electricity of 67 euro/MWh |
| Akbari et al. [40] | sCO2 Brayton cycle (SCRBC) recompression | 38.3% | 53.03% | 7819 $/h capital cost rate |
| sCO2/ORC different fluids | 41.85–42.16% | 57.95–58.38% | 7957–8568 $/h Capital cost rate ($/h) | |
| Manente et al. [17] | Cascaded sCO2 Brayton cycle (biomass—part flow topping cycle) | 36% | - | - |
| Li et al. [77] | tCO2 | 5.5% | 54.40% | Cost per net power output (CPP) −1750 $/W |
| tCO2 (IHX) | 6.1% | 51.09% | 1870 $/W | |
| Serrano et al. [78] | sCO2 Brayton + fusion reactor | 46.5% | - | - |
| Li et al. [69] | 1. Supercritical Rankine cycle 2. Transcritical Rankine cycle without/with ejector | 7.88% 8.18%/6.23% | - | - |
| Baronci et al. [79] | sCO2 + molten carbonate fuel cell | 56.25% (electrical efficiency) | - | - |
| Linares et al. [80] | sCO2 Brayton + fusion reactor | 40.59% (cycle efficiency) | - | - |
| Hu et al. [81] | sCO2 Brayton + recompression CO2 + He mixture CO2 + butane | 47.82% >41.5% >35% | - | - |
| Wang et al. [82] | sCO2 + ORC Scheme 1: Pcomp inlet = 6.61 MPa, Pcomp outlet = 20 MPa, Ppump outlet = 0.97 MPa, ΔTevapor.hot = 56 K Scheme 2: Pcomp inlet = 4.69 MPa, Pcomp outlet = 7.65 MPa, Ppump outlet = 0.43 MPa, ΔTevapor.hot = 29 K Scheme 3: Pcomp inlet = 5.305 MPa, Pcomp outlet = 7.65 MPa, Ppump outlet = 0.66 MPa, ΔTevapor.hot = 33 K Scheme 4: Pcomp inlet = 3.783 MPa, Ppump outlet = 3.491 MPa, ΔTevapor.hot = 37.1 K | 41.40% 40.48% 40.85% 42.85% | 56.96% 55.69% 56.21% 58.95% | - |
| Mecheri et al. [28] | sCO2 Brayton cycle for coal-fired powerplant (net cycle efficiency) Case 1: Simple regenerative cycle without recompression Case 2: Simple regenerative cycle without recompression with a bypass pipe Case 3: Single recompression + 2 recuperator + 2 compressor + 1 bypass pipe Case 4: Single recompression + 3 compressor + 3 recuperator + 1 bypass pipe | 41.9% 42% 46.8% 47.1% | - | - |
| Zhang et al. [83] | sCO2 Brayton cycle for coal-fired plant | 50.71% | - | - |
| Shi et al. [36] | B-CTRC R-CTRC P-CTRC PR-CTRC | 4.24% 5.52% 5.02% 7.19% | 8.84% 10.63% 12.38% 15.87% | - |
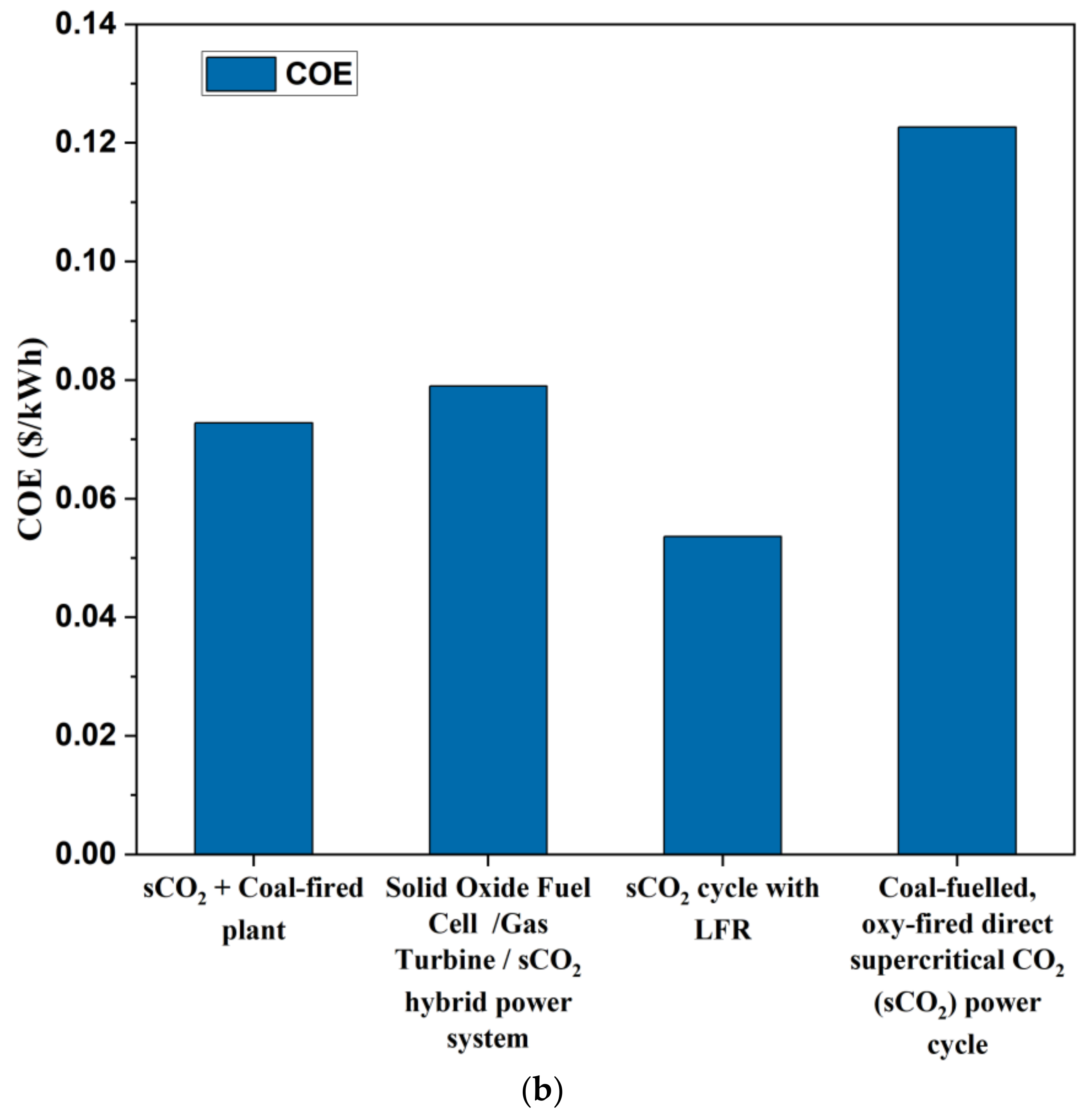
| Xia et al. [84] | Solid oxide fuel cell/gas turbine/sCO2 hybrid power system | 60.42% energy efficiency | 63.03% | 0.079$/kWh Electricity production cost |
| Sun et al. [33] | Coal-fired plant − top bottom cycle cascade (CTB recompression cycle) | 51.82% | - | - |
| Hou et al. [85] | Cogeneration system (gas turbine + sCO2 recompression cycle + steam power cycle + organic Rankine cycle) − Optimum | - | 69.33% | Total product unit cost = 10.77 $/GJ |
| Singh et al. [70] | Solar-powered combined cycle (Recompression sCO2 cycle + organic Rankine cycle) R 123 based R-sCO2 + ORC at various conditions | 63.86–85.83% | 35.57–47.82% | - |
| Singh et al. [86] | sCO2 & organic Rankine cycle (solar trough collector) organic working fluid R407c | 43.49% | 78.07% | |
| Song et al. [87] | sCO2 with bottoming organic Rankine cycle Organic working fluid R245fa | Standalone regenerative sCO2 = 16.4% sCO2 + ORC = 17.7% sCO2 + ORC with vaporize at high temperature = 18.1% Recompression sCO2 + ORC + precooler = 19.1% | - | - |
| Xu et al. [20] | sCO2 coal-fired plant (Anthracite coal) | Thermal efficiency = 51.22% Electricity efficiency = 48.37% | - | - |
| Jose J et al. [73] | sCO2 combined cooling, heating, and power system with regeneration | 37% improvement in energy efficiency | 0.6% reduction in exergy destruction rate | - |
| Park J et al. [88] | sCO2 Brayton recompression cycle for various small modular reactors | Pressurized water reactor = 30.6% Sodium cooled fast reactor = 46.38% High-temperature gas cooled reactor = 50.04% | - | - |
| Li et al. [89] | sCO2 cycle with LFR | Reheating recompression sCO2 Brayton cycle = 43.72% LFR with reheating recompression sCO2 Brayton cycle = 41.53% | - | Electricity production cost = 0.0536 $/kWh |
| Weiland et al. [90] | Coal-fueled, oxy-fired direct supercritical CO2 (sCO2) power cycle | Plant thermal efficiency = 40.6% | - | Cost of electricity (COE) = 122.7 $/MWh |
| Mishra et al. [91] | Combined sCO2 & VAR cycle (at max cycle temp. = 650 K) | 41.89% | 75.2% | - |
| Ma et al. [92] | sCO2 cycle in coal-fired plant | 1. Temperature major control method—37.42% 2. Mass flow rate major control method—34.57% 3. Novel synergetic control method—36.88% | - | - |
| Xiao et al. [93] | Nuclear-driven sCO2 block with membrane distillation block | Power block—48.18% Desalination block—37.10% | 67.82% | 1. LCOE = 0.0527 $/kWh 2. LCOW = 0.445 $/m3 |
| Xu et al. [8] | sCO2 power cycle for coal-fired power plant | 49.01% | - | LCOE = 60.56 $/MWh |
| Wang et al. [94] | Concentrated solar-driven power and desalination (CSPD) system using sCO2 Brayton cycle and multi-stage flash (MSF) | 36.6% | - | 1. LCOE of 0.059 $∙kW/h 2. LCOW = 1.15 $∙t−1 |
| Guo et al. [32] | sCO2 Brayton cycle integrated coal power plants | 47.69–49.09% | 47.69–50.55% | LCOE = 0.0397 $·(kWh)−1 |
| Wang et al. [25] | sCO2 power cycle | 19.47% | ||
| Sun et al. [95] | Multi-compressions sCO2 power cycle | 47.43% for recompression cycle 49.47% for tri compression cycle | ||
| Chen et al. [35] | sCO2 coal-fired power plant with reheating | 49.06% for double-reheat 48.72% for single reheat | 48.02% for double reheat 47.69% for single reheat | - |
| Mohammadi et al. [39] | Triple power cycle is suggested for waste heat recovery from turbine for driving the sCO2 recompression cycle and recuperative ORC | 52.1% | - | LCOE = $52.819/MWh |
| Mohammadi et al. [71] | Recompression sCO2 cycle | - | 1. 16.63% for real conditions 2. 17.13% for unavoidable conditions | - |
| Ehsan et al. [96] | Dry-cooled sCO2 recompression cycle | 50.9% | - | - |
| Li et al. [97] | Fossil-fired sCO2 power cycle pilot loop [recompression and reheat cycle with two split ratio] | 33.49% | - | - |
| Xu et al. [72] | Solar hybrid coal-based direct-fired supercritical carbon dioxide power cycle | 43.4% | 44.6% | - |
| sCO2 Power Technology | Technology Readiness Level | Comments |
|---|---|---|
| Brayton Power Cycle Systems | 7–8 | This technology has undergone sufficient development, and many such systems are already in operation [108,109]. |
| Waste Heat Recovery | 5–6 | Waste heat recovery systems using sCO2 have been exhaustively researched and pilot projects are being evaluated for further development [110]. |
| Solar Power Plants | 4–5 | Research is ongoing to improve the efficiency and for the smooth integration of sCO2 technology into concentrated solar power systems [111]. |
| Nuclear Power Plants | 3–4 | The nuclear power plants show huge potential in the application of sCO2 technologies. The research and development are still in earlier stages, where the feasibility and safety studies are underway [21,112]. |
| Fossil Fuel Power Plants | 4–5 | There are crucial challenges related to the integration of sCO2 technologies with the prevailing infrastructure, especially for coal and natural gas applications, and achieving reasonable efficiency [113,114]. |
| Combined Heat and Power (CHP) Systems | 3–4 | Research on CHP systems is in the early stages with the main focus on small-scale applications and improving the overall system efficiency [115,116]. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tamilarasan, S.K.; Jose, J.; Boopalan, V.; Chen, F.; Arumugam, S.K.; Ramachandran, J.C.; Parthasarathy, R.K.; Taler, D.; Sobota, T.; Taler, J. Recent Developments in Supercritical CO2-Based Sustainable Power Generation Technologies. Energies 2024, 17, 4019. https://doi.org/10.3390/en17164019
Tamilarasan SK, Jose J, Boopalan V, Chen F, Arumugam SK, Ramachandran JC, Parthasarathy RK, Taler D, Sobota T, Taler J. Recent Developments in Supercritical CO2-Based Sustainable Power Generation Technologies. Energies. 2024; 17(16):4019. https://doi.org/10.3390/en17164019
Chicago/Turabian StyleTamilarasan, Saravana Kumar, Jobel Jose, Vignesh Boopalan, Fei Chen, Senthil Kumar Arumugam, Jishnu Chandran Ramachandran, Rajesh Kanna Parthasarathy, Dawid Taler, Tomasz Sobota, and Jan Taler. 2024. "Recent Developments in Supercritical CO2-Based Sustainable Power Generation Technologies" Energies 17, no. 16: 4019. https://doi.org/10.3390/en17164019
APA StyleTamilarasan, S. K., Jose, J., Boopalan, V., Chen, F., Arumugam, S. K., Ramachandran, J. C., Parthasarathy, R. K., Taler, D., Sobota, T., & Taler, J. (2024). Recent Developments in Supercritical CO2-Based Sustainable Power Generation Technologies. Energies, 17(16), 4019. https://doi.org/10.3390/en17164019












