Mechanical Behavior of Sediment-Type High-Impurity Salt Cavern Gas Storage during Long-Term Operation
Abstract
1. Introduction
2. Numerical Model
2.1. Continuous–Discontinuous Coupling Theory
2.2. Model Establishment
- (1)
- Establish the cavern of sediment accumulation and generate a certain number of sediment particles. First, the PFC module is introduced into the FLAC software for coupling calculation. A certain gradation of sediment particles is generated in the cavern bottom regarding the particle parameters and assigned to the sediment particles (Table 1 and Table 2). Taking the brine buoyancy into account, the density of the sediment particles is set to the density of the solids minus the density of the brine [31]. Then, the sediment particles are accumulated by self-gravity in the cavern bottom.
- (2)
- Embed the excavated salt cavern model and the sediment particle accumulation in the same interface to achieve the coupled calculation of the continuous boundary of the surrounding rock and the discontinuous boundary of the particles. Then, delete the particles outside the boundary of the cavern and recalculate the stress redistribution after the cavern excavation.
- (3)
- Apply different pressures to the cavern walls. Since the cavern is filled with natural gas during operation, the upper part of the cavern is subject to gas pressure, and the lower part is subject to the combined action of brine and sediment pressure. Brine pressure varying with depth is applied to the cavern wall, and the sediment is modeled by the particle accumulation generated by the PFC 5.0 software.
- (4)
- Set the creep time to start the calculation. According to the creep parameters in the paper [12] and assigning them to the salt rock (Table 3), a combination of the viscoelastic Norton–Hoff model and Mohr–Coulomb elastoplastic model is used to calculate the coupled creep of the sediment particles in the cavern and the surrounding rock [32]. The timestep of the creep model is 5 × 10−6, while the timestep of the particle model is 1.0 × 10−4. When the creep of the surrounding rock is calculated 20 times, the displacement information is transmitted to the particles and the contact force changes between particles. Then, the contact force information is transmitted back to the surrounding rock.
- (5)
- To analyze the effects of the salt cavern’s sediment height, particle gradation, and operating pressure on the volume shrinkage of the cavern and the deformation of the surrounding rock, ten numerical simulation schemes were carried out (Table 4).


| Density /kg·m−3 | Effective Modulus /GPa | Normal Critical Damping Ratio | Damp | Initial Porosity | Friction Coefficient | Radius /m |
|---|---|---|---|---|---|---|
| 2450 | 10 | 0.7 | 0.7 | 0.4 | 0.5 | 0.5 |
| Normal Stiffness /MPa | Shear Stiffness /MPa | Damp Normal Ratio | Friction Coefficient |
|---|---|---|---|
| 100 | 100 | 0.5 | 0.1 |
| Lithology | Elastic Modulus /GPa | Poisson’s Ratio | Cohesion /MPa | Friction Angle /° | Tensile Strength /MPa |
|---|---|---|---|---|---|
| Salt rock | 5 | 0.3 | 2.0 | 30 | 0.6 |
| No. | Sediment Height /m | Operating pressure /MPa | Particle Gradation |
|---|---|---|---|
| 1 | 0 | 10 | Uniform distribution |
| 2 | 30 | 10 | Uniform distribution |
| 3 | 60 | 10 | Uniform distribution |
| 4 | 90 | 10 | Uniform distribution |
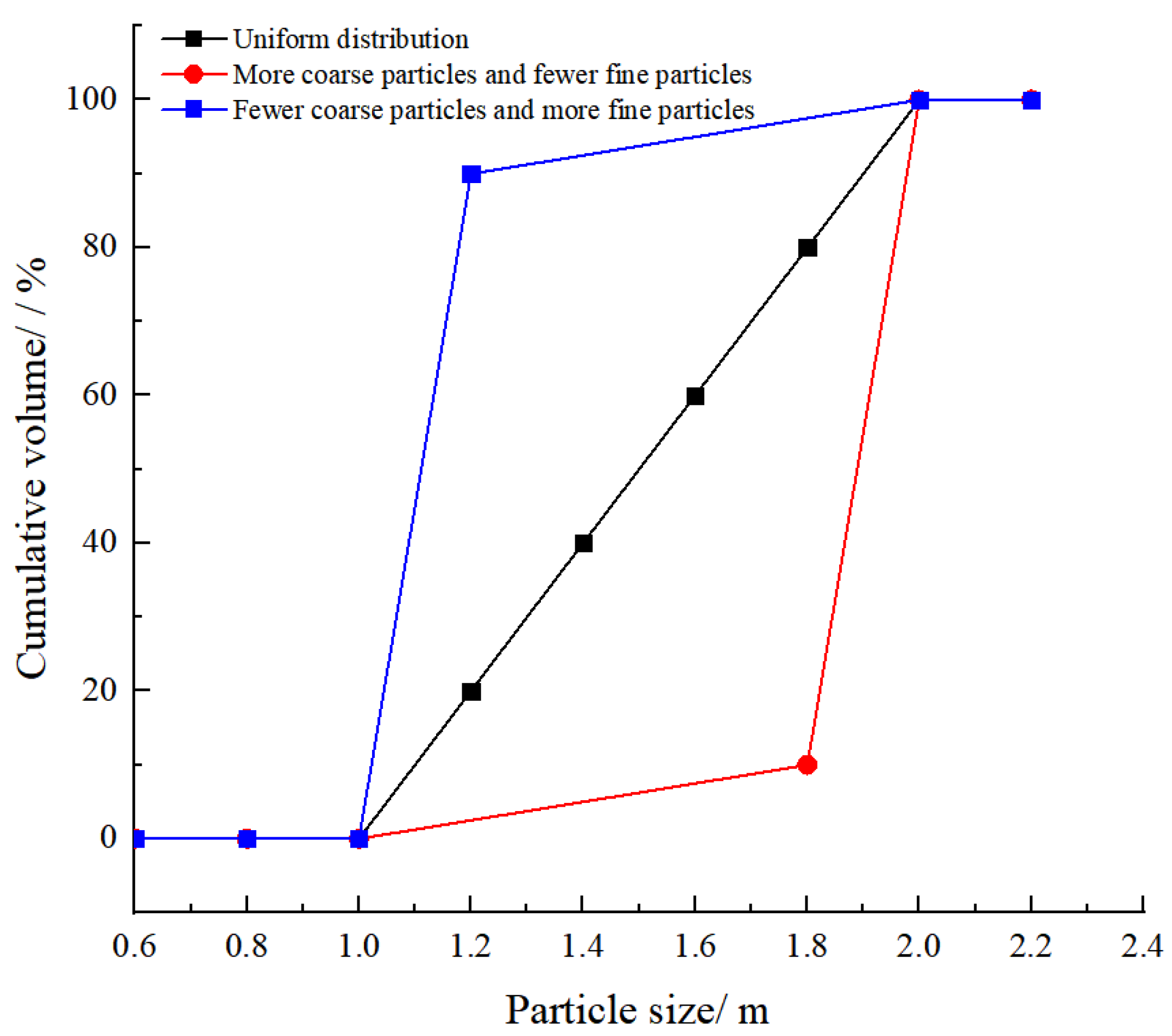
| 5 | 60 | 10 | More coarse particles and less fine particles |
| 6 | 60 | 10 | Less coarse particles and more fine particles |
| 7 | 60 | 4 | Uniform distribution |
| 8 | 60 | 6 | Uniform distribution |
| 9 | 60 | 8 | Uniform distribution |
3. Results and Analysis
3.1. Sediment Height
3.2. Particle Gradation
3.3. Operating Pressure
4. Discussion
5. Conclusions
- For the same particle gradation and operating pressure, a larger sediment height results in a smaller maximum displacement of the cavern wall and reduces volume shrinkage of the cavern, indicating a stronger inhibitory effect of the sediment particles on the surrounding rock. The self-weight effect of the accumulated particles enhances the support force on the cavern bottom, slows down the creep shrinkage at the cavern bottom, and shifts the maximum displacement location of the surrounding rock to the upper part of the cavern.
- At the same sediment height and operating pressure, a higher coarse particle content leads to smaller cavern volume shrinkage. However, the effect of particle gradation on volume shrinkage is minimal, primarily due to its limited impact on packing density. The simulation did not account for the stable force chain structure formed by irregularly shaped particles, indicating a need for further study on the influence of particle morphology on long-term operating stability.
- For the same particle gradation and sediment height, a higher operating pressure results in a smaller maximum displacement around the cavern and reduces the volume shrinkage of the cavern, causing a smaller maximum upward displacement of the sediment particles after extrusion, increasing gas storage space in the upper part of the cavern. Compared to scenarios without sediment in the salt cavern, the accumulation of sediment particles helps maintain cavern stability. Utilizing the sediment voids for gas storage does not compromise cavern stability and maximizes the use of the cavern mining space for gas storage.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yang, C.; Wang, T. Deep Underground Energy Storage: Aiming for Carbon Neutrality and Its Challenges. Engineering 2023, 29, 11–14. [Google Scholar] [CrossRef]
- Li, P.; Li, Y.; Shi, X.; Wei, X.; Yang, K.; Ma, H.; Yang, C.; Hu, W.; Xu, H. Pressure monitoring and deformation analysis of a brine-filled salt cavern—A case study of Jianghan, China. Int. J. Rock Mech. Min. Sci. 2024, 177, 105737. [Google Scholar] [CrossRef]
- Bérest, P.; Gharbi, H.; Brouard, B.; Brückner, D.; Devries, K.; Hévin, G.; Hofer, G.; Spiers, C.; Urai, J. Very Slow Creep Tests on Salt Samples. Rock Mech. Rock Eng. 2019, 52, 2917–2934. [Google Scholar] [CrossRef]
- Blanco-Martín, L.; Rouabhi, A.; Billiotte, J.; Hadj-Hassen, F.; Tessier, B.; Hévin, G.; Balland, C.; Hertz, E. Experimental and numerical investigation into rapid cooling of rock salt related to high frequency cycling of storage caverns. Int. J. Rock Mech. Min. Sci. 2018, 102, 120–130. [Google Scholar] [CrossRef]
- Li, P.; Li, Y.; Shi, X.; Ma, H.; Zhao, K.; Liang, X.; Wei, X.; Yang, C. Pore Structure and Brine Flow Simulation of Salt Cavern Sediments Based on X-ray Computed Tomography. Rock Mech. Rock Eng. 2024, 57, 115–130. [Google Scholar] [CrossRef]
- Liu, W.; Dong, Y.; Zhang, Z.; Li, L.; Jiang, D.; Fan, J.; Chen, J.; Zhang, X.; Wan, J.; Li, Z. Optimization of operating pressure of hydrogen storage salt cavern in bedded salt rock with multi-interlayers. Int. J. Hydrogen Energy 2024, 58, 974–986. [Google Scholar] [CrossRef]
- Li, J.L.; Tang, Y.; Shi, X.L.; Xu, W.J.; Yang, C.H. Modeling the construction of energy storage salt caverns in bedded salt. Appl. Energy 2019, 255, 113866. [Google Scholar] [CrossRef]
- Li, P.; Li, Y.; Shi, X.; Zhao, K.; Liu, X.; Ma, H.; Yang, C. Prediction method for calculating the porosity of insoluble sediments for salt cavern gas storage applications. Energy 2021, 221, 119815. [Google Scholar] [CrossRef]
- Lankof, L.; Nagy, S.; Polański, K.; Urbańczyk, K. Potential for Underground Storage of Liquid Fuels in Bedded Rock Salt Formations in Poland. Energies 2022, 15, 7005. [Google Scholar] [CrossRef]
- Li, P.; Li, Y.; Shi, X.; Xie, D.; Ma, H.; Yang, C.; Daemen, J.J.K. Experimental and theoretical research on the debrining process in sediments for a gas storage salt cavern. Geoenergy Sci. Eng. 2023, 225, 211667. [Google Scholar] [CrossRef]
- Li, P.; Li, Y.; Shi, X.; Zhao, K.; Liu, X.; Ma, H.; Yang, C. Compaction and restraining effects of insoluble sediments in underground energy storage salt caverns. Energy 2022, 249, 123752. [Google Scholar] [CrossRef]
- Shi, X.; Chen, Q.; Ma, H.; Li, Y.; Wang, T.; Zhang, C. Geomechanical investigation for abandoned salt caverns used for solid waste disposal. Bull. Eng. Geol. Environ. 2020, 80, 1205–1218. [Google Scholar] [CrossRef]
- Wang, T.T.; Li, J.J.; Jing, G.; Zhang, Q.Q.; Yang, C.H.; Daemen, J.J.K. Determination of the maximum allowable gas pressure for an underground gas storage salt cavern—A case study of Jintan, China. J. Rock Mech. Geotech. Eng. 2019, 11, 251–262. [Google Scholar] [CrossRef]
- Jong, C.d. Gas storage valuation and optimization. J. Nat. Gas Sci. Eng. 2015, 24, 365–378. [Google Scholar] [CrossRef]
- Portarapillo, M.; Di Benedetto, A. Risk Assessment of the Large-Scale Hydrogen Storage in Salt Caverns. Energies 2021, 14, 2856. [Google Scholar] [CrossRef]
- Chen, W.; Wu, G.; Dai, Y.; Yang, C. Stability analysis of abandoned salt caverns used for underground gas storage. Chin. J. Rock Mech. Eng. 2006, 25, 848–854. [Google Scholar]
- Liu, W.; Zhang, Z.X.; Fan, J.Y.; Jiang, D.Y.; Daemen, J.J.K. Research on the Stability and Treatments of Natural Gas Storage Caverns With Different Shapes in Bedded Salt Rocks. IEEE Access 2020, 8, 18995–19007. [Google Scholar] [CrossRef]
- Zhao, K.; Liu, Y.X.; Li, Y.P.; Ma, H.L.; Hou, W.; Yu, C.F.; Liu, H.L.; Feng, C.; Yang, C.H. Feasibility analysis of salt cavern gas storage in extremely deep formation: A case study in China. J. Energy Storage 2022, 47, 103649. [Google Scholar] [CrossRef]
- Li, J.L.; Shi, X.; Yang, C.; Li, Y.; Wang, T.; Ma, H. Mathematical model of salt cavern leaching for gas storage in high-insoluble salt formations. Sci. Rep. 2018, 8, 372. [Google Scholar] [CrossRef]
- Xue, T.; Yang, C.; Shi, X.; Hongling, M.; Liu, X. The formation mechanism of irregular salt caverns during solution mining for natural gas storage. Energy Sources Part A Recovery Util. Environ. Eff. 2020, 340, 1–17. [Google Scholar] [CrossRef]
- Li, P.; Li, Y.; Shi, X.; Zhao, A.; Liu, Y. Stability analysis of U-shaped horizontal salt cavern for underground natural gas storage. J. Energy Storage 2021, 38, 102541. [Google Scholar] [CrossRef]
- Chen, X.S.; Li, Y.P.; Shi, Y.F.; Yu, Y.; Jiang, Y.L.; Liu, Y.X.; Dong, J.L. Tightness and stability evaluation of salt cavern underground storage with a new fluid-solid coupling seepage model. J. Pet. Sci. Eng. 2021, 202, 108475. [Google Scholar] [CrossRef]
- Marketos, G.; Spiers, C.J.; Govers, R. Impact of rock salt creep law choice on subsidence calculations for hydrocarbon reservoirs overlain by evaporite caprocks. J. Geophys. Res. Solid Earth 2016, 121, 4249–4267. [Google Scholar] [CrossRef]
- Li, H.; Yang, C.; Ma, H.; Shi, X.; Zhang, H.; Dong, Z. A 3D grain-based creep model (3D-GBCM) for simulating long-term mechanical characteristic of rock salt. J. Pet. Sci. Eng. 2019, 185, 106672. [Google Scholar] [CrossRef]
- Sánchez, V.; Castro, R.L.; Palma, S. Gravity flow characterization of fine granular material for Block Caving. Int. J. Rock Mech. Min. Sci. 2019, 114, 24–32. [Google Scholar] [CrossRef]
- Cai, M.; Kaiser, P.K.; Morioka, H.; Minami, M.; Maejima, T.; Tasaka, Y.; Kurose, H. FLAC/PFC coupled numerical simulation of AE in large-scale underground excavations. Int. J. Rock Mech. Min. Sci. 2007, 44, 550–564. [Google Scholar] [CrossRef]
- Jia, M.; Yang, Y.; Liu, B.; Wu, S. PFC/FLAC coupled simulation of dynamic compaction in granular soils. Granul. Matter 2018, 20, 76. [Google Scholar] [CrossRef]
- Wang, T.T.; Yang, C.H.; Chen, J.S.; Daemen, J.J.K. Geomechanical investigation of roof failure of China’s first gas storage salt cavern. Eng. Geol. 2018, 243, 59–69. [Google Scholar] [CrossRef]
- Teófilo, F.A.F.; Poiate Junior, E.; Roehl, D.; Martha, L.F. A numerical approach for investigation of stress states induced by salt structures. Int. J. Rock Mech. Min. Sci. 2018, 106, 223–233. [Google Scholar] [CrossRef]
- Khaledi, K.; Mahmoudi, E.; Datcheva, M.; Schanz, T. Stability and serviceability of underground energy storage caverns in rock salt subjected to mechanical cyclic loading. Int. J. Rock Mech. Min. Sci. 2016, 86, 115–131. [Google Scholar] [CrossRef]
- Li, P.; Li, Y.; Shi, X.; Yang, K.; Wei, X.; Zhao, K.; Ma, H.; Yang, C. Theoretical and numerical simulation studies of the self-stabilization capability of salt cavern roofs. Comput. Geotech. 2023, 163, 105719. [Google Scholar] [CrossRef]
- Reedlunn, B.; Argüello, J.G.; Hansen, F.D. A reinvestigation into Munson’s model for room closure in bedded rock salt. Int. J. Rock Mech. Min. Sci. 2022, 151, 105007. [Google Scholar] [CrossRef]
- Yang, C.H.; Wang, T.T.; Li, Y.P.; Yang, H.J.; Li, J.J.; Qu, D.A.; Xu, B.C.; Yang, Y.; Daemen, J.J.K. Feasibility analysis of using abandoned salt caverns for large-scale underground energy storage in China. Appl. Energy 2015, 137, 467–481. [Google Scholar] [CrossRef]
- Mou, J.; Shang, H.; Ji, W.; Wan, J.; Xing, T.; Ma, H.; Peng, W. Feasibility Analysis of Compressed Air Energy Storage in Salt Caverns in the Yunying Area. Energies 2023, 16, 7171. [Google Scholar] [CrossRef]
- Yu, H.; Liu, Y.; Ma, H.; Zhao, K.; Liu, J. Pillar safety in shallow salt caverns by using numerical simulations. J. Energy Storage 2022, 55, 105881. [Google Scholar] [CrossRef]
- Shi, X.; Ma, H.; Zhang, Y. Advances of large-scale gas storage technology in existing salt caverns in high-insoluble salt formations. J. Shandong Univ. Sci. Technol. (Nat. Sci.) 2020, 39, 55–65. [Google Scholar]
- Wang, J.; Zhang, Q.; Song, Z.; Feng, S.; Zhang, Y. Nonlinear creep model of salt rock used for displacement prediction of salt cavern gas storage. J. Energy Storage 2022, 48, 103951. [Google Scholar] [CrossRef]
- Seyyedan, S.M.; Mirghasemi, A.A.; Mohammadi, S. Numerical simulation of direct shear test on granular materials composed of breakable angular particles: A DEM-XFEM approach. Powder Technol. 2021, 391, 450–466. [Google Scholar] [CrossRef]
- Liang, X.; Ma, H.; Cai, R.; Zhao, K.; Wang, X.; Zheng, Z.; Shi, X.; Yang, C. Study of Impact of Sediment on the Stability of Salt Cavern Underground Gas Storage. Energies 2023, 16, 7825. [Google Scholar] [CrossRef]
- Haque, E. Estimating bulk density of compacted grains in storage bins and modifications of Janssen’s load equations as affected by bulk density. Food Sci. Nutr. 2013, 1, 150–156. [Google Scholar] [CrossRef]
- Liang, X.; Ma, H.; Cai, R.; Zhao, K.; Zeng, Z.; Li, H.; Yang, C. Feasibility analysis of natural gas storage in the voids of sediment within salt cavern—A case study in China. Energy 2023, 285, 129340. [Google Scholar] [CrossRef]
- Wang, T.; Chai, G.; Cen, X.; Yang, J.; Daemen, J.J.K. Safe distance between debrining tubing inlet and sediment in a gas storage salt cavern. J. Pet. Sci. Eng. 2021, 196, 107707. [Google Scholar] [CrossRef]










Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Li, P.; Bai, W.; Lu, J.; Fu, X.; Fu, Y.; Shi, X. Mechanical Behavior of Sediment-Type High-Impurity Salt Cavern Gas Storage during Long-Term Operation. Energies 2024, 17, 3983. https://doi.org/10.3390/en17163983
Wang J, Li P, Bai W, Lu J, Fu X, Fu Y, Shi X. Mechanical Behavior of Sediment-Type High-Impurity Salt Cavern Gas Storage during Long-Term Operation. Energies. 2024; 17(16):3983. https://doi.org/10.3390/en17163983
Chicago/Turabian StyleWang, Jian, Peng Li, Weizheng Bai, Jun Lu, Xinghui Fu, Yaping Fu, and Xilin Shi. 2024. "Mechanical Behavior of Sediment-Type High-Impurity Salt Cavern Gas Storage during Long-Term Operation" Energies 17, no. 16: 3983. https://doi.org/10.3390/en17163983
APA StyleWang, J., Li, P., Bai, W., Lu, J., Fu, X., Fu, Y., & Shi, X. (2024). Mechanical Behavior of Sediment-Type High-Impurity Salt Cavern Gas Storage during Long-Term Operation. Energies, 17(16), 3983. https://doi.org/10.3390/en17163983







