1. Introduction
The performance limitations of silicon-based power devices are increasingly evident, moving the semiconductor industry towards alternative materials like silicon carbide (SiC) and gallium nitride (GaN). GaN, in particular, has gained significant traction due to its superior switching speed capabilities [
1,
2]. Traditional silicon power MOSFETs have faced challenges in balancing conduction and switching losses, as efforts to reduce on-resistance often result in increased parasitic capacitances, leading to higher switching losses [
3].
In Pulse-Width Modulation (PWM) motor drive applications, the adoption of GaN technology offers the potential to achieve higher switching frequencies, which in turn reduces torque ripple and improves the waveform quality of the motor current [
4]. In a motor drive powered by a voltage source inverter, the dead time is necessary to avoid cross-conduction [
5]. Unfortunately, dead time always causes the waveform distortion phenomenon in a motor drive, and dead time compensation strategies are required [
6]. Moreover, voltage source inverters used in these applications require dead time to prevent cross-conduction, introducing waveform distortion. This distortion originates from the inherent delays in switching devices and the characteristics of the devices themselves, such as turn-on and turn-off delays and reverse conduction voltage drop [
7].
GaN FETs are particularly attractive in power electronics due to their low on-resistance and ability to operate at very high frequencies [
8]. For low-voltage (V < 100 V) motor drives, GaN FET-based inverters have demonstrated advantages in reducing the size of passive components and minimizing motor current distortion and torque ripple. On the other hand, an advanced motor insulation layout and a deep investigation of the commutation transient in the inverter leg are required due to the dV/dt increase [
9].
Properly setting the dead time is crucial to minimize both reverse conduction [
10]. Numerous studies have aimed to optimize dead time settings, exploring solutions like gate driver ICs with adjustable or adaptive dead time capabilities. While some methods, such as programmable dead time settings, lack adaptability in real-world applications [
11], others have shown promise but often lack generality or theoretical underpinning [
12,
13]. Recent research has highlighted the importance of theoretically derived optimal dead time values, which have demonstrated improvements in efficiency [
14]. These research studies require a deep knowledge of the considered device behavior, depending on the operative conditions and the technology features [
15,
16].
This paper investigates the commutation transients of MOSFET and GaN FET devices during dead time for motor drive applications. Experimental tests are conducted in an inverter leg board controlling the phase current. Results reveal different switching behaviors depending on the working conditions. The energy exchanged between the high-side and low-side devices during commutations and the energy losses are estimated through a validated model of the system. The contribution to switching losses during hard-switching and soft-switching commutations and the differences between GaN FET and MOSFET results are distinguished and deeply investigated. Findings aim to provide insights and guidelines for optimizing dead time based on the specific technology for different operating conditions. Furthermore, an optimization strategy for the dead time related to the GaN FET in inverter leg application is presented and described.
2. GaN FET and MOSFET Commutation Transients in Motor Drive Application
The motor drive system used consists of a GaN FET-based inverter powering a 3-phase permanent magnet (PM) motor.
Figure 1 shows the system composed of the inverter and the electrical machine. The inverter is composed of three legs, one for each motor phase. The stator phase currents
,
,
are controlled by the high-side
and the low-side
using a Pulse-Width Modulation (PWM). The modulation works at the switching frequency
, significantly higher than the AC stator phase current frequency of the motor in order to ensure control stability. A dead time (
) is introduced between the devices’ commutation in which both driving signals are off-state. This
is set by the user to avoid shoot-through in the inverter leg [
17]. Nevertheless, the introduction of
creates voltage harmonic distortion affecting the phase current waveform [
18,
19].
In AC motor drive systems, each inverter leg operates with a sinusoidal phase current of various amplitudes. These currents are directed either from the inverter leg’s switching node to the motor phase or in the reverse direction.
Figure 1 shows that a current entering the stator phase is considered positive.
To study commutation transients in switching legs with MOSFET and GaN FET devices, we used two half-bridge experimental board PCBs. These boards only differed in device technology. This setup ensured consistent parasitic effects from the PCB, allowing a fair comparison. Nevertheless, the different packages of GaN FET and MOSFET cannot be removed. However, the choice of the technology leads to the use of the corresponding parasitic elements introduced by the case of the selected device [
20,
21]. Moreover, tests are carried out using equal operating conditions for both the GaN FET-based board and the MOSFET-based one.
The GaN FET board featured EPC2065 GaN FET, while the MOSFET one featured Onsemi FDMS2D5N08C. The device features are reported in
Table 1.
Figure 2a depicts the schematic of the inverter leg.
Figure 2b,c show the pictures of the GaN FET board and the MOSFET one, respectively.
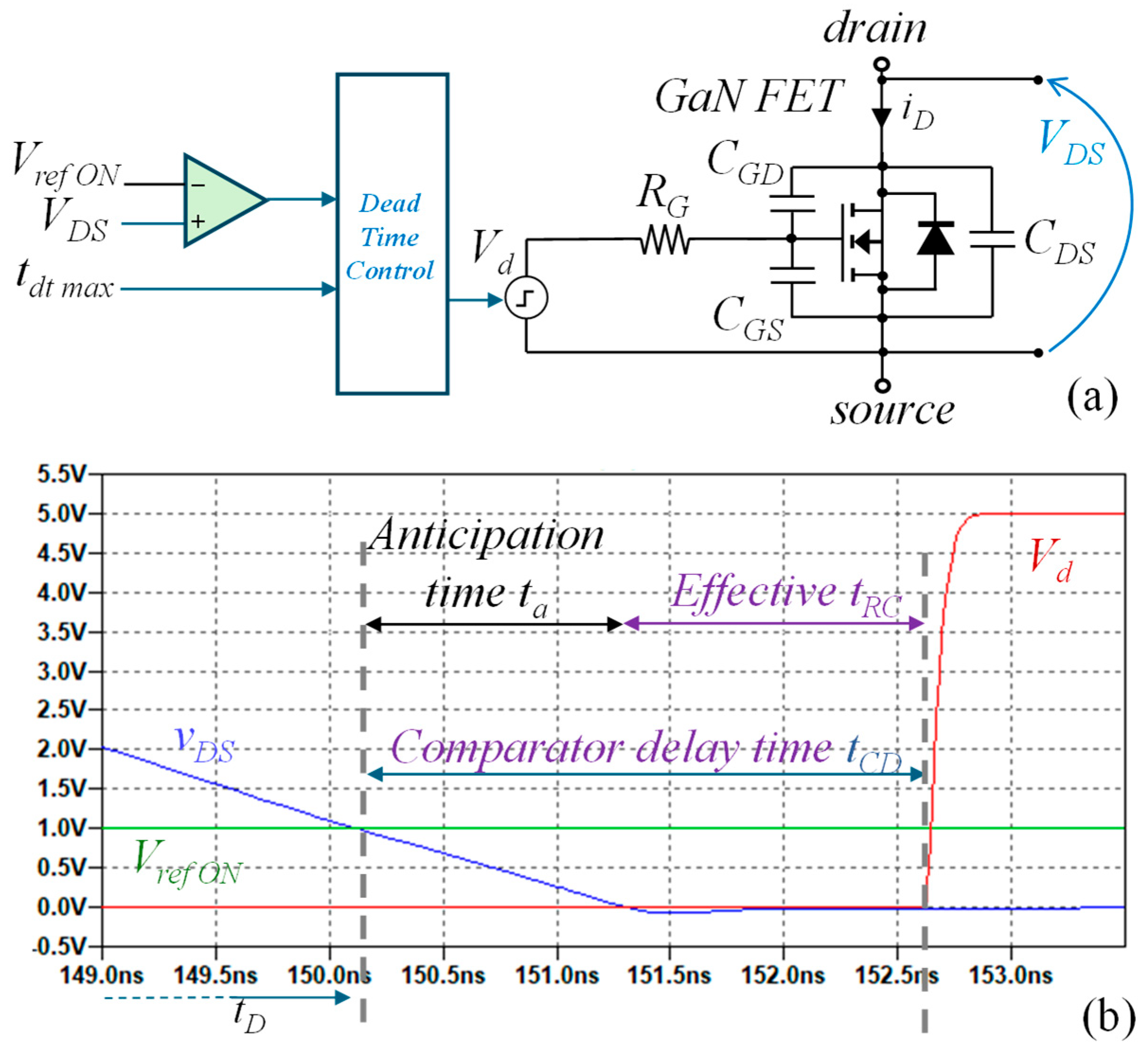
Precise measurement results are particularly challenging to obtain, especially when they aim to distinguish different events that happen in a short time (e.g., during the switching transient of WBG devices). Therefore, a dedicated experimental setup controlling the system variables is required [
22].
Testing occurred at an ambient temperature of 25 °C with a DC input voltage of
. A second inverter board is used to control the phase current
connecting an LCL filter to the half-bridge switching node (point a of
Figure 2a). An STM32H7 microcontroller generated PWM signals and controlled the phase current. The PWM operated at a
switching frequency with a duty cycle of 0.1 to reduce current ripple. This
is sufficient to ensure that the switching transient has been completed before a new switching. Despite the fact that WBG devices can operate at a higher switching frequency, the
selection does not affect the switching transient’s investigation [
23]. Additionally,
is a reasonable settlement for the MOSFET, which operates at a lower
than the GaN FET;
duration needs to be chosen long enough to prevent shoot-through and obtain hard-switching for commutations with low phase current [
24]. Furthermore, significant distortion effects due to the duration of the dead time need to be avoided [
25]. In the experimental test, both GaN FET and MOSFET boards have a dead time of
which is a good trade-off between the GaN FET and MOSFET requirements. During
, transitioning from high-side (
) turn-off to low-side (
) turn-on can cause zero-voltage transients at different current levels [
26].
Experimental tests carried out with
reveal that the MOSFET switching leg achieves zero voltage switching (ZVS) [
27] for currents
. The GaN FET achieves ZVS at lower
.
The voltage waveforms are measured using a digital scope featuring a bandwidth of 500 MHz, an output resistance of 10 MΩ, and an output capacitance of 10 pF.
The experimental setup of the controlled current-level system is shown in
Figure 3. It includes the half-bridge board under test, the power converter regulating
, and the STM32H7 microcontroller.
Tests are conducted for positive current values (exiting from the switching node and entering the converter regulating current) at . Two commutation characteristics for two transitions are analyzed:
High-Side Turn-Off, Low-Side Turn-On: This commutation features a negative voltage slew rate () as the switching node voltage () decreases;
Low-Side Turn-Off, High-Side Turn-On: This had a positive voltage slew rate () as the switching node voltage () increased to .
The experimental test result achieved in these two commutations for various amplitudes are each reported separately.
2.1. Commutation with and Positive
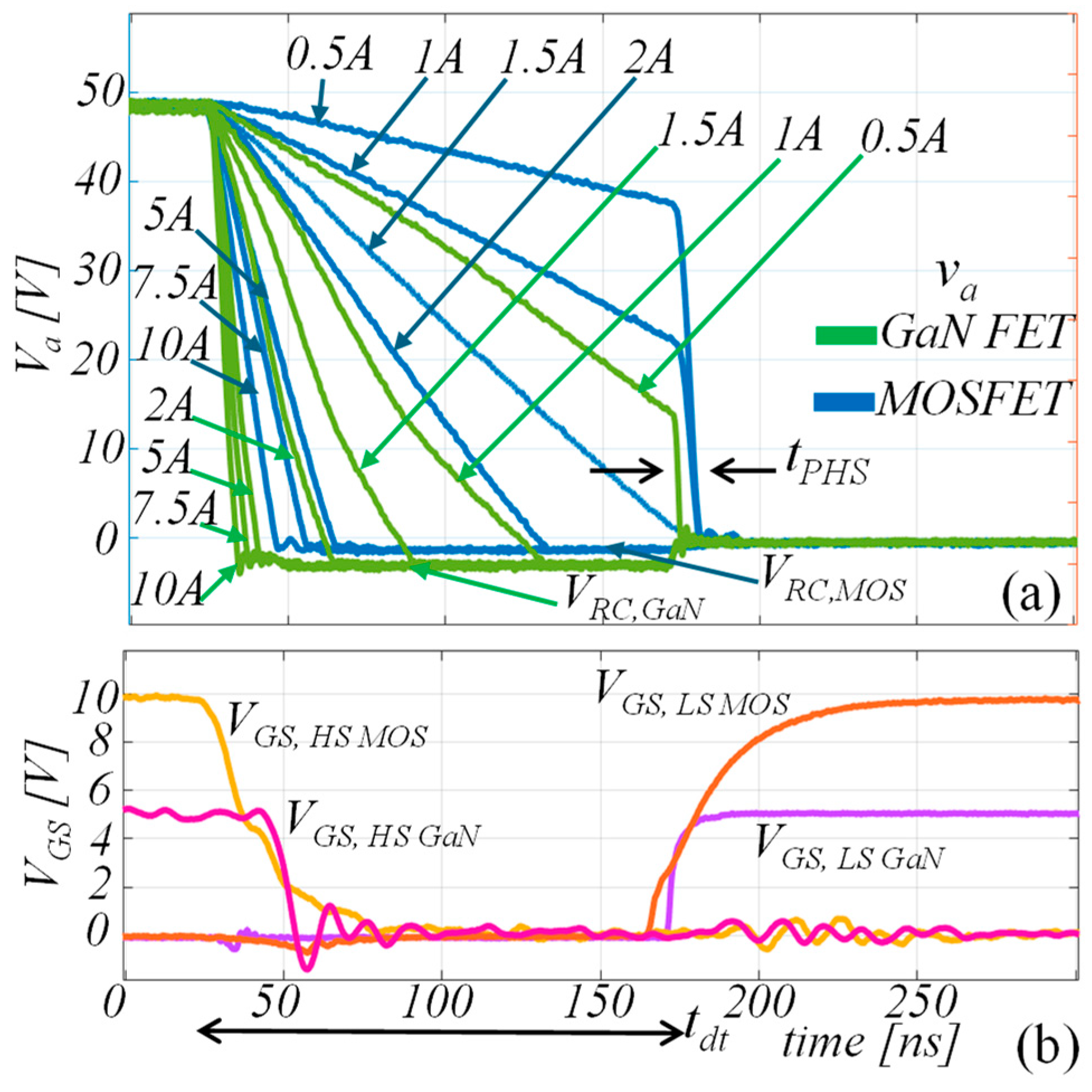
Figure 4 shows the voltage waveforms measured on the half-bridge boards using GaN FETs and MOSFETs.
Figure 4a displays the switching node voltage
, while
Figure 4b illustrates the gate-source voltages for the high-side device (
) and the low-side device (
). The
waveforms correspond to the current amplitudes
indicated by the arrows.
Depending on the length and the , three different switching events can happen for the switching node voltage:
Zero voltage switching (ZVS);
Voltage variation and partial hard switching (PHS);
Voltage fall transient and reverse conduction (RC).
When the high-side switch (
) turns off,
starts to fall. The rate of
decline is steeper with higher
due to the parasitic output capacitances of the devices (
) and the load [
28]. Since
is not constant with voltage, the dynamics of
can be described using an equivalent capacitance
. This
is derived as the average value resulting from the
slew rate (
) measured at different
amplitudes and considering the time (
) taken for
to fall to 0 V when
turns off. Integrating the constitutive equation of a capacitance (
), it is possible to calculate
as
ZVS occurs when the low-side switch (
) turns on exactly as
reaches 0 V. This is the condition in which
. As shown in
Figure 4, ZVS for MOSFET happens at a phase current amplitude of
, while for GaN FET, it occurs between
and
. The GaN FET has a lower
, compared to the MOSFET’s
. The lower
of the GaN FET results in a shorter
, enabling ZVS at lower
compared to the MOSFET.
When
is lower than the ZVS threshold, a PHS event follows the
transient. In this case,
turns on before
has fully dropped to 0 V. After
,
falls to
’s conduction value within the partial hard switching duration time (
), causing PHS losses.
Figure 4 shows MOSFET experiencing PHS at
and
, while the GaN FET exhibits PHS only at
.
In the cases of higher values than those required for ZVS, drops to 0 V before ends . Subsequently, operates in RC mode until turns on. The reverse conduction duration is . is negative at due to the activation of the body-diode in the MOSFET or the equivalent diode behavior in the GaN FET. The reverse conduction voltage is higher for GaN FET () than for MOSFET (), causing higher RC losses in the GaN FET than in the MOSFET. No losses follow as turns on.
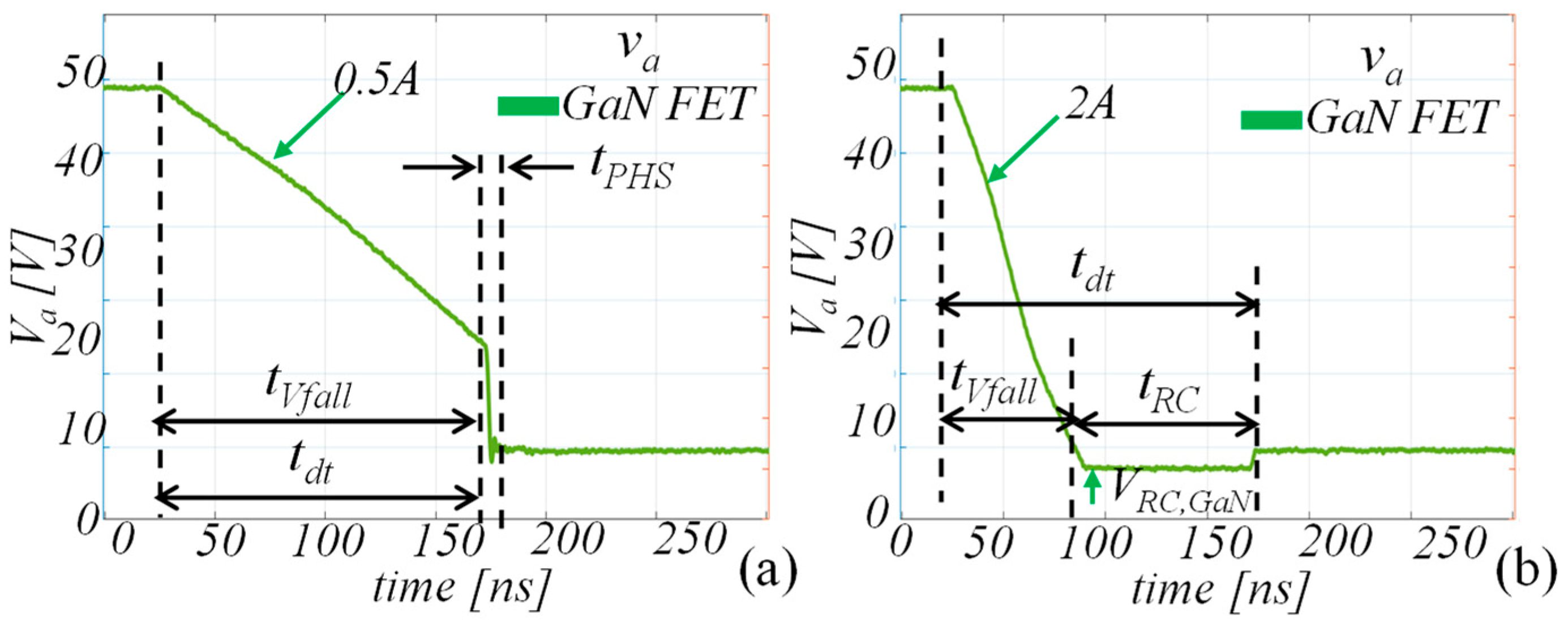
In
Figure 5a are highlighted
and
in the example of the PHS event achieved with the GaN FET with
.
Figure 5b indicates the time intervals of
and
relative to the
curves achieved with
for the GaN FET.
2.2. Commutation with and Positive
Figure 6 shows voltage waveforms with
for both the half-bridge board using GaN FETs and the one using MOSFETs at the same current variations as in
Figure 4.
Figure 6a presents the switching node voltage
, while
Figure 6b illustrates the gate-source voltages (
for the low-side device and
for the high-side device).
The phase voltage waveforms rise with no differences for all amplitudes used in the tests. The difference in is determined by the device technology. The parasitic capacitance of the device affects the voltage rise time . The reverse conduction (RC) phase begins when turns off and ends when turns on. During RC, due to the body-diode of the MOSFET or the equivalent diode in the GaN FET. A hard switching (HS) event follows the dead time, lasting a few nanoseconds, and is a dissipative process because of equals .
2.3. Commutation with and Negative
The results for negative current are dual to those with positive current. The switching event of
and
is characterized by an RC phenomenon lasting
. During RC,
reaches the value of
. Consequently, an HS event with a rapid
rising happens, and it elapses in lasting a few nanoseconds (
). All considerations made for
and
(
Section 2.2) are valid.
Figure 7 illustrates
measured on the boards with GaN FETs and MOSFET during the commutation with
when testing with
.
Figure 7a depicts the falling
waveforms, while
Figure 7b shows the gate-source voltages for
and
(
for the low-side device and
for the high-side device).
2.4. Commutation with and Negative
For
and
, the commutation dynamics are influenced by the parasitic capacitance. Depending on the amplitude of
, Zero Voltage Switching (ZVS), Partial Hard Switching (PHS), and Reverse Conduction (RC) events can occur. The same considerations discussed for
and
apply here. At higher
amplitudes, the voltage rise time (
) is shorter.
Figure 8 shows the voltages measured on GaN FET and MOSFET boards with
during commutation with
.
Figure 8a illustrates the rising
waveforms, while
Figure 8b presents the gate-source voltages for
and
(
and
, respectively).
3. GaN FET vs. MOSFET Commutation Energy Evaluation
The board used for experimental tests does not incorporate current sensing to measure the current of transistors in a half-bridge configuration. This is intentionally designed to prevent any impact on the switching board’s performance. Nevertheless, measuring transistor current is crucial for evaluating power trends and energy during commutations. To achieve the current waveform of devices, LTSpice simulations are used. The simulation models for MOSFET and GaN FET are sourced from the manufacturer’s official websites.
The simulated electrical circuit replicates the experimental setup (see
Figure 2a) and maintains the same operating conditions:
;
and duty-cycle 0.1. The half-bridge circuit model is validated by ensuring it produces waveforms consistent with those obtained in the experimental tests, as depicted in
Figure 4 and
Figure 6 [
29]. The phase currents exiting the switching node (positive
) in the simulations are
, emulating the experimental conditions.
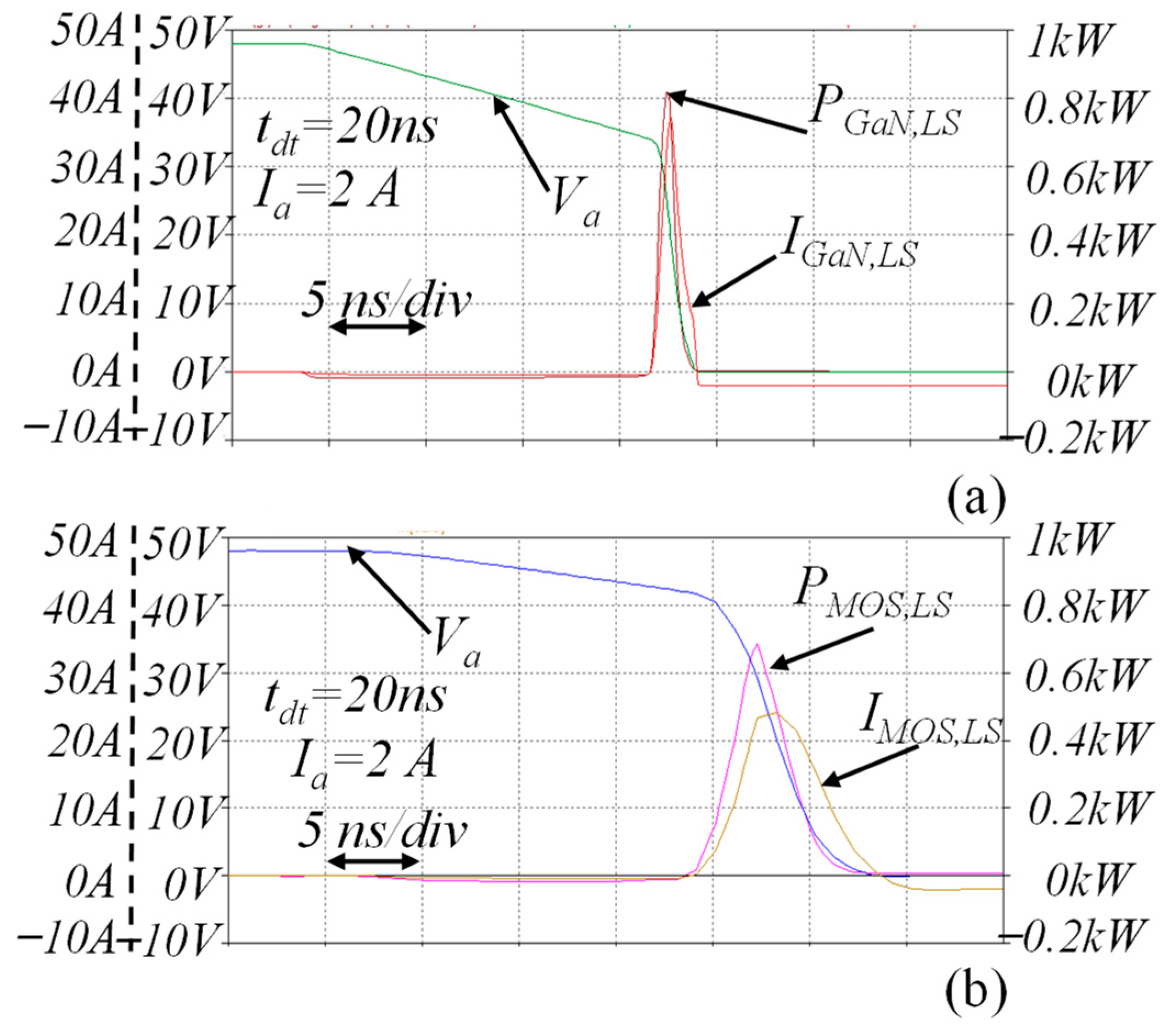
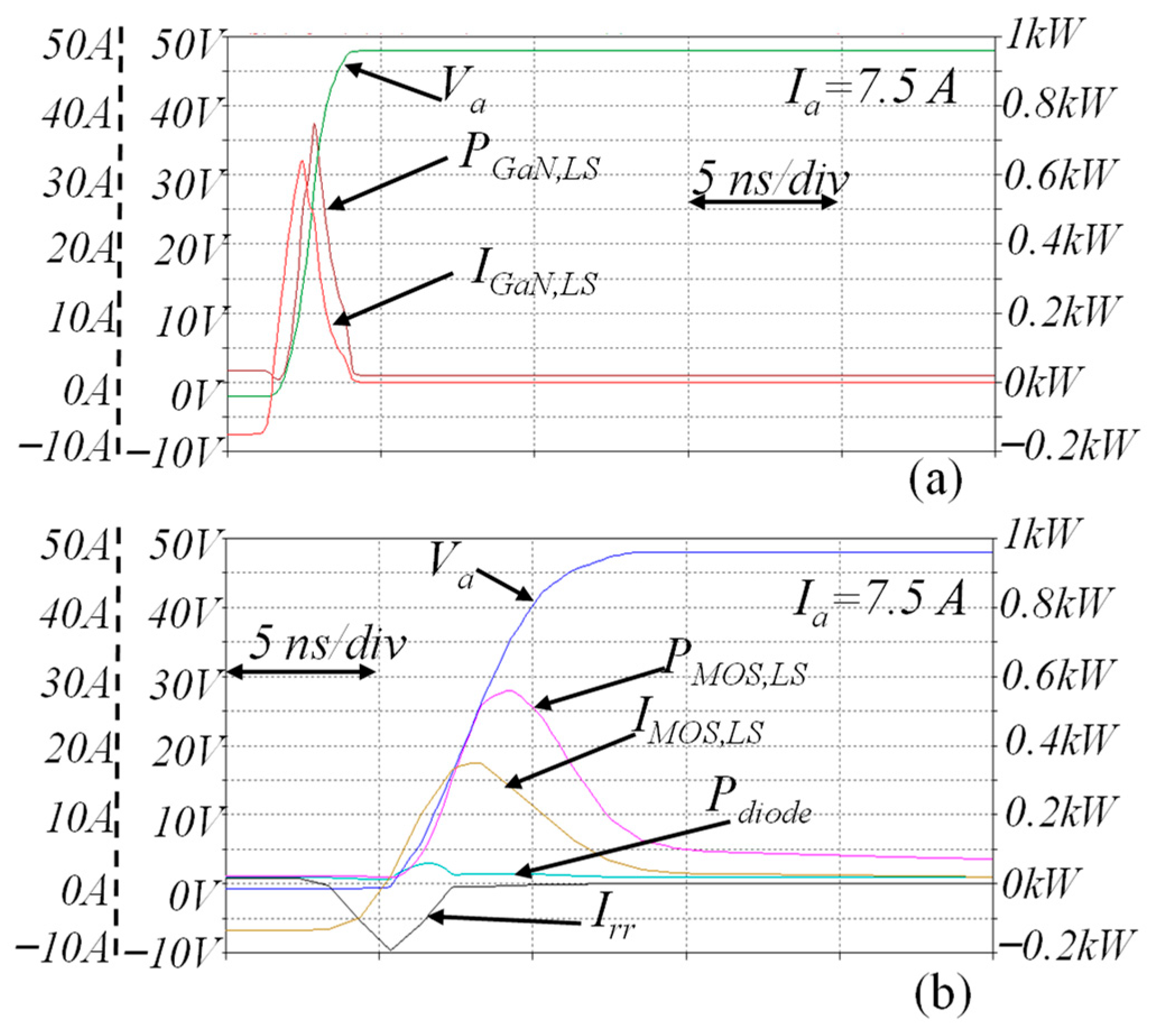
Simulations are performed twice with dead times and . These values are typical for the respective devices (20 ns for GaN FETs and 150 ns for MOSFETs) and are relevant for motor drive applications. The energy values computed from the simulations pertain to the low-side device ().
Figure 9 and
Figure 10 show the
waveforms of the current
, the phase voltage
and the power
during the commutation with
, load current of
and a dead time of
and with
respectively.
Figure 9a and
Figure 10a refer to the GaN FET, while
Figure 9b and
Figure 10b refer to the MOSFET.
Comparing the GaN FET waveforms of
Figure 9a and
Figure 10a with the MOSFET ones in
Figure 9b and
Figure 10b shows that the
and current variations last longer in the MOSFET than in the GaN FET. As a result, during the
fall the GaN FET power
has a peak comparable with those of the MOSFET
, but
lasts shorter. In the case of soft switching following the
fall in
Figure 9a,b, GaN FET features a higher reverse conduction voltage drop (
). The current flowing through the device operating in reverse conduction is
and it is equal for both the GaN FET or the MOSFET. Therefore, the higher
of the GaN FET leads to higher reverse conduction losses than the MOSFET [
30]. Due to the higher voltage drop of the GaN transistor during reverse conduction operation, the device in the third quadrant must work with reduced timing to optimize performance and losses. In the hard-switching event with
and
shown in
Figure 10a,b, the overall GaN FET hard switching losses are much lower than the MOSFET ones.
During the commutation exhibiting
(
turning off and
turning on) with a positive
, it is possible to distinguish between the
amplitudes that result in ZVS or RC and those that cause PHS. The energy of
during the voltage variation,
, is determined by the equation:
where
is the current through
and
is the time taken for the switching node variation
. The time
varies according to the
falling slew rate. If
drops to 0 V before the end of
, RC conditions appear. ZVS condition appears when
equals the maximum value of
if
reaches 0 V at the end of
. The PHS conditions occur if the theoretical voltage fall time
exceeds
. When
is followed by RC conditions lasting
, the energy losses during
are denoted as
and calculated as
where
is the RC time interval when
is negative and equal to
for the GaN FET or MOSFET. The upper integral limit at
is close to
and includes turn-on delays of
and the driving circuit’s propagation delay uncertainty.
If
does not drop to 0 V within
, the voltage variation during
is followed by PHS. The energy losses due to the PHS
are calculated as
where
is the duration of the PHS phenomenon, starting at the end of
and ending as
.
In the ZVS condition, and . is the only energy involved in the switching event.
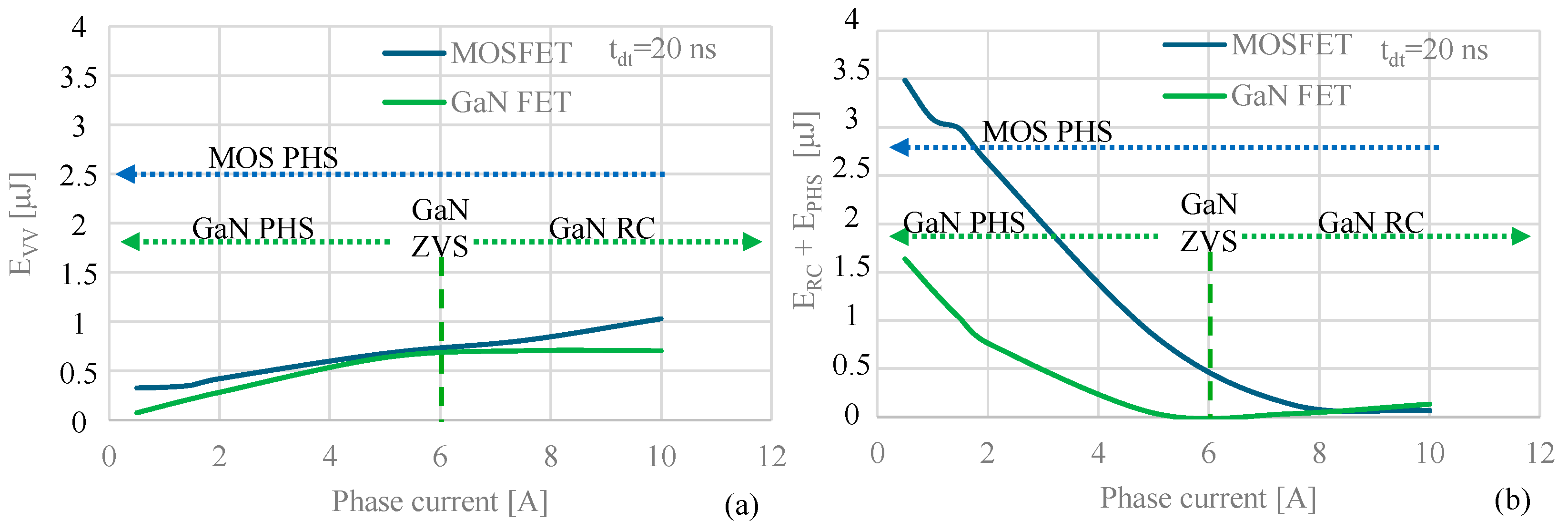
Figure 11 and
Figure 12 illustrate the energies involved during the
QHS turn-off and
QLS turn-on with positive
from 0.5 A to 10 A.
Figure 11 refers to results with
, while
Figure 12 refers to those achieved with
. In particular,
Figure 11a and
Figure 12a depict the energy
as a function of
. On the other hand,
Figure 11b and
Figure 12b show the energy losses due to RC (
) and PHS (
) as a function of
. ZVS is marked with a dashed line, while PHS and RC are marked with dotted lines. MOSFET curves are blue, and GaN FET curves are green.
GaN FET achieves ZVS at with and at with . Differently, the MOSFET achieves ZVS at with , while it does not achieve ZVS with for currents up to . RC losses occur for higher than the one causing ZVS, while PHS occurs for lower than the ZVS.
Commutation with starts with the initial conditions of of charged to and of discharged to nearly 0 V. Immediately after turning off , of discharges to 0 V and of charges to . During this, both devices are in the off-state, but the variation of causes an exchange of energy between them. The amount of exchanged varies depending on the switching event:
ZVS or RC: of discharges of until stabilizes at 0 V, while of charges of up to . is maximum () since of fully discharges using the charging energy of of . is calculated as
and looking at
Figure 11a and
Figure 12a,
for GaN FET and
for MOSFET.
PHS: of does not discharge completely because after the phase voltage is not null, . of charges of , and of does not fully charge to . GaN FET’s smaller results in a steeper fall and a shorter for exchanging . As a result, MOSFET features a greater quantity of losses than the GaN FET when using the same .
ZVS condition features zero energy losses
. Only
is involved due to the energy exchange between the switching leg devices’ output capacitances (
and
). Differently, PHS and RC are dissipative phenomena following
(
Figure 11b and
Figure 12b).
Considering the same , PHS energy losses is lower with long because turns on with a lower (closer to ZVS). Additionally, elapsed , GaN FET’s smaller results in a steeper fall than MOSFET, leading to lower for GaN FET. On the other hand, MOSFET features a lower voltage drop and corresponding losses than the GaN FET. increase proportionally with and whatever the technology is considered.
Figure 13 and
Figure 14 show the GaN FET and MOSFET waveforms of the
current
, the phase voltage
and the device power
during the commutation with
. Additionally,
Figure 13a and
Figure 14a show the GaN FET power
, while
Figure 13b and
Figure 14b depict the MOSFET reverse recovery current and the body-diode power, respectively. In particular,
Figure 13 refers to the case with
and
Figure 14 to
.
During commutation with ( turn off and turn on), the transient event starts with the of being discharged, and of being charged to . With positive , works in reverse conduction for all . At the end of , turns on with a drain-source voltage of , causing hard switching (HS). The device has a current peak and an almost instantaneous charge.
GaN FET waveforms in
Figure 13a and
Figure 14a exhibit negligible differences with the
level. Furthermore, GaN FET shows a faster dynamic than the MOSFET one of
Figure 13b and
Figure 14b because of the low GaN FET
. Additionally, MOSFET features a reverse recovery current
due to the body-diode which causes an increase of
in switching losses
. A higher
leads to a higher
peak and higher
, as shown comparing
Figure 13b and
Figure 14b. GaN FET does not feature reverse recovery current and corresponding losses. The
peak is due only to the charging
.
The reverse conduction energy
achieved by
both for GaN FET and MOSFET is illustrated in
Figure 15a and
Figure 16a as a function of
.
Figure 15b and
Figure 16b depict the hard switching energy losses (
) as a function of
.
Figure 15 refers to the case with
, while
Figure 16 refers to the one with
. GaN FET curves are depicted in green, while MOSFET curves are shown in blue.
The comparison of the GaN FET and MOSFET
curves in
Figure 15a and
Figure 16a demonstrates that GaN FET losses are higher than MOSFET during
due to higher
[
30]. Despite this,
losses are significantly low (up to 0.3 μJ for GaN FET and 0.1 μJ for MOSFET) considering
.
ERC increases linearly with
and the level of
.
Figure 15b and
Figure 16b show that HS energy losses
of GaN FET are significantly lower than the MOSFET ones. GaN FET features a
regardless of
and
. Differently, MOSFET, and
EHS has a minimum
for low
levels which persist according to
duration:
for
and
for
.
corresponds to the energy required to charge the
of
to
(no energy comes from
of
) and it is calculated as
where
is the constant equivalent value of
(which is a non-linear parameter with the voltage).
is not affected by
and closely matches the maximum energy capacity of
(
), as arises comparing in
Figure 12a and
Figure 16b.
When
level is higher,
of the MOSFET grows with the
amplitude, while the GaN FET one remains constant. The MOSFET
increase is due to the additional reverse recovery charge
in the MOSFET’s P-N junction, which grows with longer
and higher reverse recovery current
[
31,
32]. Conversely, the GaN FET maintains a constant
due to the absence of a P-N junction, thereby featuring
[
33]. In general,
significantly exceeds
, particularly at
. When considering both energy dissipation components, the GaN FET exhibits reduced energy losses during the switching transient, especially with a shorter dead time (
).