Geometrical Aspects of the Optics of Linear Fresnel Concentrators: A Review
Abstract
1. Introduction
2. System Description
2.1. A Semantic Clarification
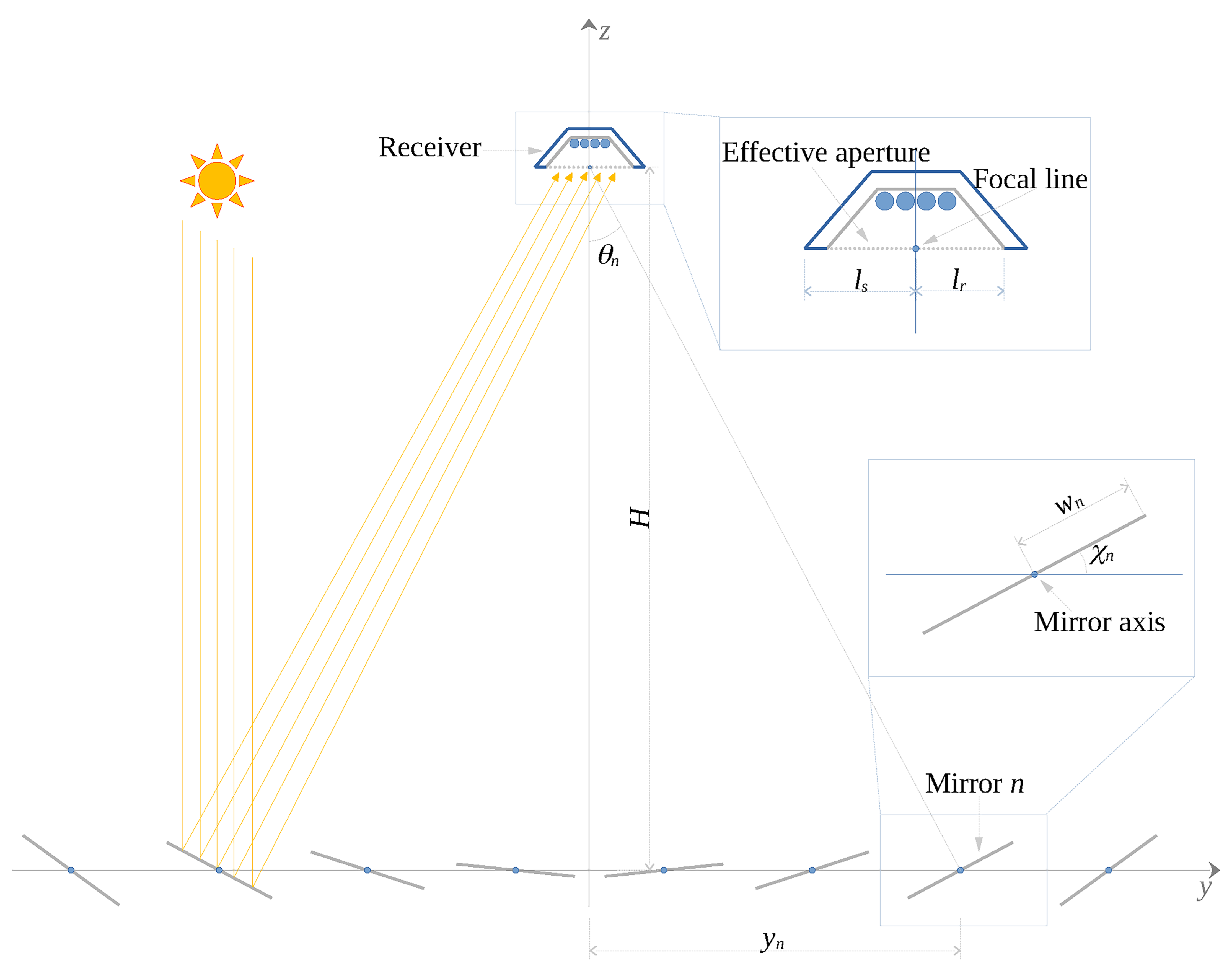
2.2. Geometrical Description of the Mirror Field
- is the standard Azimuth angle of the local frame x-axis;
- is the inclination of the focal line w.r.t. the horizontal plane, considered positive if the local x-axis points downwards;
- is obtainable from the inclination of the local frame y-axis w.r.t. the horizontal plane (, considered positive if local y-axis points upwards) by the relation .
- all the mirrors have a common aiming line;
- the mirror axes lie on a common plane;
- each mirror is mounted symmetrically w.r.t. its axis and rotates around its central point.
2.3. Incidence Angles and Tracking
- the formula is universal, in the sense that it is correct everywhere is well defined (i.e., when ), and it gives correct angles and signs also when . But if one limits the considered cases to , the simpler formula (without the sign function) can be used:saving the computation of a trigonometric function.
- formulas for , both in (6) and in (7) versions are not fully explicit, since must be computed before . Fully explicit formulas for from can be obtained substituting with (note that the sign is always correct, since ). Note however, that and are usually computed together, so (6) (or (7)) can be applied.
2.4. Receiver
- A tubular receiver without a secondary reflector: the target is the tube itself, which presents the same section view from every direction. Such a configuration favors the mirror farther from the axis, allowing a higher width ratio for the plant, but the absence of a secondary is a strong disadvantage for tracking.
- A triangular receiver [6], in which the mirrors with aim at an effective target that is flat and inclined to face more favorably this half of the mirror field, and the opposite happens for mirrors with . In fact, from the optical point of view, the two halves of the mirror field can be considered as two independent LFRs, reducing the analysis to the next case;
- A more general case with a flat, but inclined effective target, e.g., for EW-oriented plants: triangular receivers are then just a couple of symmetrical, non-superimposing plants of this kind;
- A flat vertical target, such as the vertical rack of Dewar tubes discussed in [14], can be considered a degenerate triangular receiver.
3. Methodology for Ray-Tracing Simulations
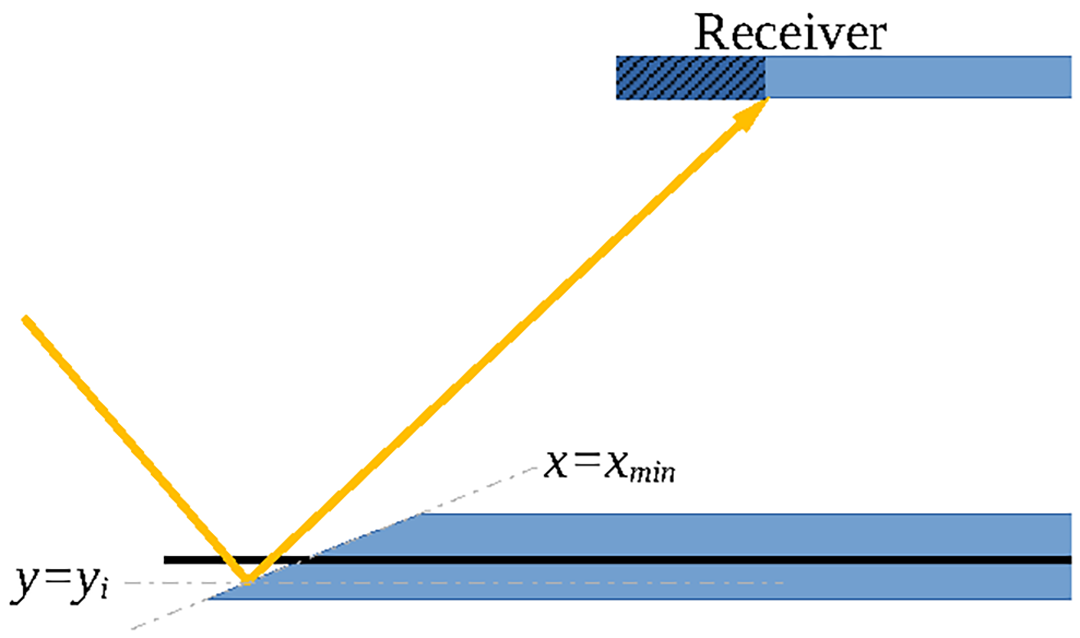
3.1. Receiver Shadow
3.2. Selecting Which Mirror Is Hit, and Computing the Incidence Point
3.3. Reflected Ray
3.4. Is the Reflected Ray Blocked?
3.5. Does the Ray Hit the Receiver?
3.6. Sum of the Contribution of All the Rays
- the reflectivity of the mirror it hits;
- the cosine factor.
- In the plane, choose a horizontal segment of length L above the receiver (), making sure that the radiation coming from this region will “cover” entirely the mirror field;
- From this segment, shoot a sample of rays with a uniform distribution of the starting coordinate y (the starting x coordinate can be set to 0), and with an angular distribution of the rays that reproduces the solar divergence around according to the chosen sun profile. Both the starting points and the directions can be chosen randomly (Monte Carlo ray tracing) or distributed on a uniform grid. The first option is safer (no systematic errors due to the discretization) but significantly slower;
- Shoot each ray of the sample, as illustrated: if it hits the receiver, add the quantity to the “absorbed” energy;
- Normalize the absorbed energy multiplying by the quantity : here is the local Zenith of the sun, that is, the incidence angle of the radiation on the mirror plane, and it accounts for the cosine factor. The following relation holds:The result is the power that enters the receiver per length unit, for a unitary DNI. The procedure actually involves an approximation, since the rays coming from within the sun radiation cone are considered equivalent, neglecting the cosine effect of peripherical rays when they contribute to the DNI; it is clearly a negligible effect (the cosine of the solar angular size differs by about from 1).
4. Quasi-Analytical Analysis
4.1. Collimated Radiation
- are shadowed by the receiver;
- are shadowed by other mirrors;
- reflect radiation that is blocked by adjacent mirrors;
- reflect radiation that does not hit the target.
4.1.1. Receiver Shadow
- or : the mirror n is not shadowed by the receiver.
- and : the mirror region from to is shadowed.
- and : the mirror region from to is shadowed.
- : the mirror region from to is shadowed.
- and : the entire mirror is shadowed (and the analysis for mirror n can terminate here).
4.1.2. Mirror Shadowing
4.1.3. Blocking
- First check: if , the mirror cannot suffer from blocking and the analysis stops here.
- Second check: if the line connecting the rightmost point of the mirror to the rightmost point of the receiver is not blocked by mirror , the mirror cannot suffer from blocking (rays directed to the target cannot be blocked). The line has equationand the plane of mirror is . After a bit of computation, the coordinate of intersection on the mirror can be obtained:If , blocking is not present and the analysis stops here.
- Third check: if the rightmost ray reflected from mirror n is not blocked by mirror , the mirror cannot suffer blocking (rays reflected by mirror n cannot be blocked). The local coordinate on mirror of the intersection isThis equation makes use of the equation for the reflected ray projected on section (see below), and of the simplified Formula (17).If blocking is not present.
- First check: if , no blocking.
- Second check: if the line connecting the leftmost point of the mirror to the leftmost point of the receiver is not blocked by mirror , the mirror cannot suffer from blocking. The to consider isIf , blocking is not present and the analysis stops here.
- Third check: if the leftmost ray reflected from mirror n is not blocked by mirror , the mirror cannot suffer from blocking. The local coordinate of intersection isIf blocking is not present.
- If none of the above conditions is verified, the inactive part of mirror n can be obtained from the equationand the part of the mirror n from to is inactive since the reflected rays are blocked.
4.1.4. In-Target Reflected Beam
- find all the solutions of both equations , in the interval ;
- if k solutions are found, put the solutions in order on the interval , dividing the interval in segments;
- for each of the segments, compute a probing value with belonging to the segment, in order to check if the segment is active (the ray hits the receiver) or not (the ray does not hit the receiver).
4.1.5. Sum of the Contributions of All Mirrors
4.2. Solar Divergence, Slope and Tracking Errors
4.2.1. Pillbox Sun
- Obtain the tracking angles for each mirror using (8);
- Compute the M integration nodes of (50) and the corresponding values of ;
- For each , compute the function applying the procedure described in this section, i.e., compute the active part for each mirror n, and sum the contribution of all the mirrors applying (47);
4.2.2. Tracking and Slope Errors
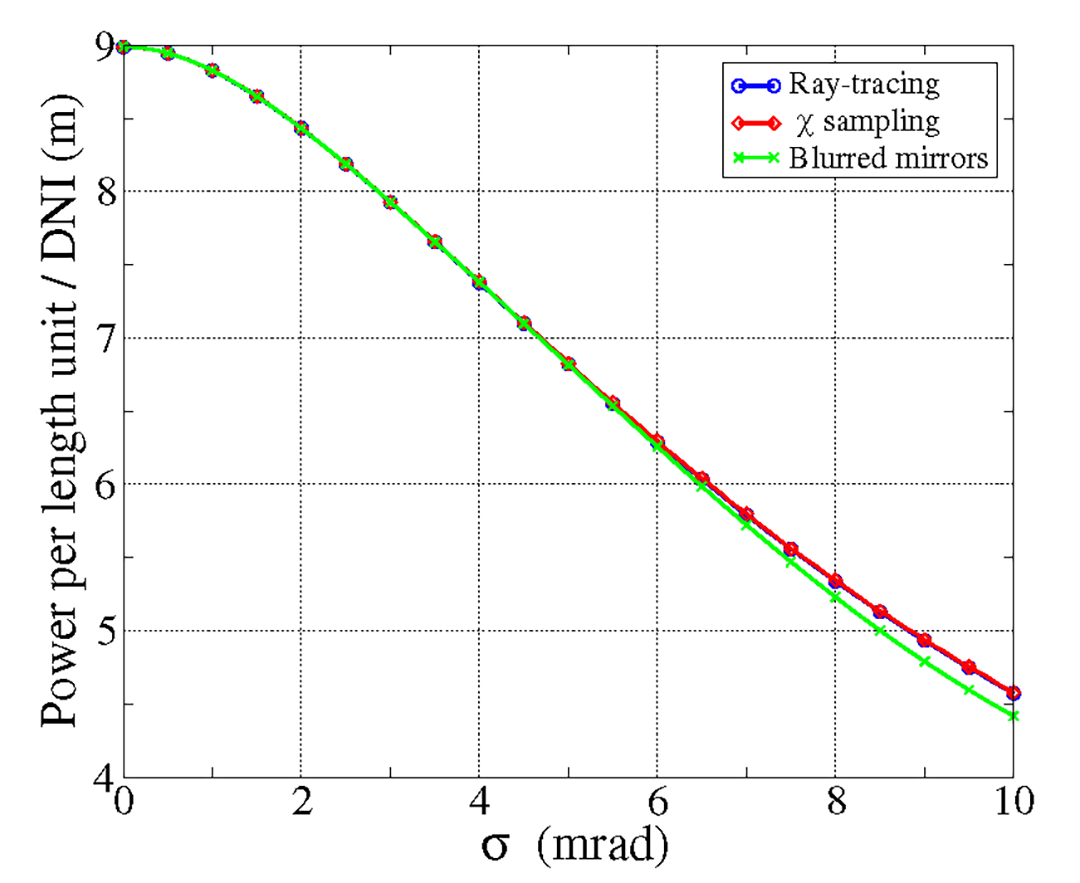
- Sampling of the : repeat the computation for a perfect system on a suitable large sample of different sets of , introducing a random deviation of the angles. The set of must be chosen with a standard deviation that is the combined standard deviation of transversal slope errors and random tracking errors. Unfortunately, a quite large sample is required to obtain accurate results.
- Blurred mirrors: perform the computation as for a perfect system, but when computing for the mirror n takes the average on a Gaussian distribution of , with a standard deviation corresponding to the combination of random tracking and transversal slope errors.
Sampling of
Blurred Mirrors
5. Comparison and Possible Integrations between Ray-Tracing and Quasi-Analytical Methods
- The projection of the sun profile on (i.e., the approximation within the solar disk), and the following application of (49) and of the (50) quadrature rule, can also be adopted in ray-tracing, significantly reducing the number of rays that are necessary to reproduce the solar divergence (of course, the analysis of longitudinal slope errors is not possible in this case);
- Relations for the inactive part of a mirror can be used to exclude from ray-tracing analysis the rays that fall within these parts (when computing inactive parts, the extremal rays of the solar cone must be considered).
6. End Effects
- a quick estimation, which is useful to correct the radiation collected by a receiver string by subtracting end effects, but does not supply information on the radiation collected in a given receiver section;
- a method for integrating the end effects analysis in the ray-tracing approach, slightly modifying the procedure described in Section 3;
- a method for integrating the end effects analysis in the quasi-analytical approach presented in Section 4, modifying the computation of for collimated radiation.
6.1. Quick Estimation
- all the rays have the same ;
- for a mirror n, the “representative ray” directed from , to , (from the center of the mirror to the center of the receiver) is chosen to compute the x shift of the radiation;
- mirror defects are neglected.
6.2. End Effects in Ray-Tracing
6.3. End Effects in the Quasi-Analytical Method
- Find the maximum distance , in the section , between a point of mirror n and a point of the receiver (, );
- Find the minimum distance , in the section , between a point of mirror n and a point of the receiver (, );
- Case : if , then mirror n is free from end effects;
- Case : if or , then mirror n is completely inactive due to end effects;
- Case : if , then mirror n is free from end effects;
- Case : if or , then mirror n is completely inactive due to end effects.
- and : the minimum distance is between the right edge of the receiver and the left edge of the mirror;
- and : the minimum distance is between the right edge of the receiver and the left edge of the mirror;
- and : the minimum distance is between the left edge of the receiver and the right edge of the mirror;
- and : the minimum distance is between the left edge of the receiver and the right edge of the mirror.
- Compute the two projections of the two edges of the receiver (at ) on the plane containing the mirror n.
- If lies within the mirror width, compute the distance between the right edge of the receiver () and : this is a possible minimum distance.
- If lies within the mirror width, compute the distance between the left edge of the receiver () and : this is a possible minimum distance.
- Compute the two projections of the two edges of the mirror n on the plane containing the receiver (these will simply be , ).
- If lies within the receiver width, compute the distance between the right edge of the mirror and (that is, ): this is a possible minimum distance.
- If lies within the receiver width, compute the distance between the left edge of the mirror () and (that is, ): this is a possible minimum distance.
- Compute the four distances between the two edges of the receiver and the two edges of the mirror (left-left, left-right, right-left, right-right): these are four possible minimum distances.
- Among the possible minimum distances found so far (of which there will be a number between 4 and 8), choose the shortest one.
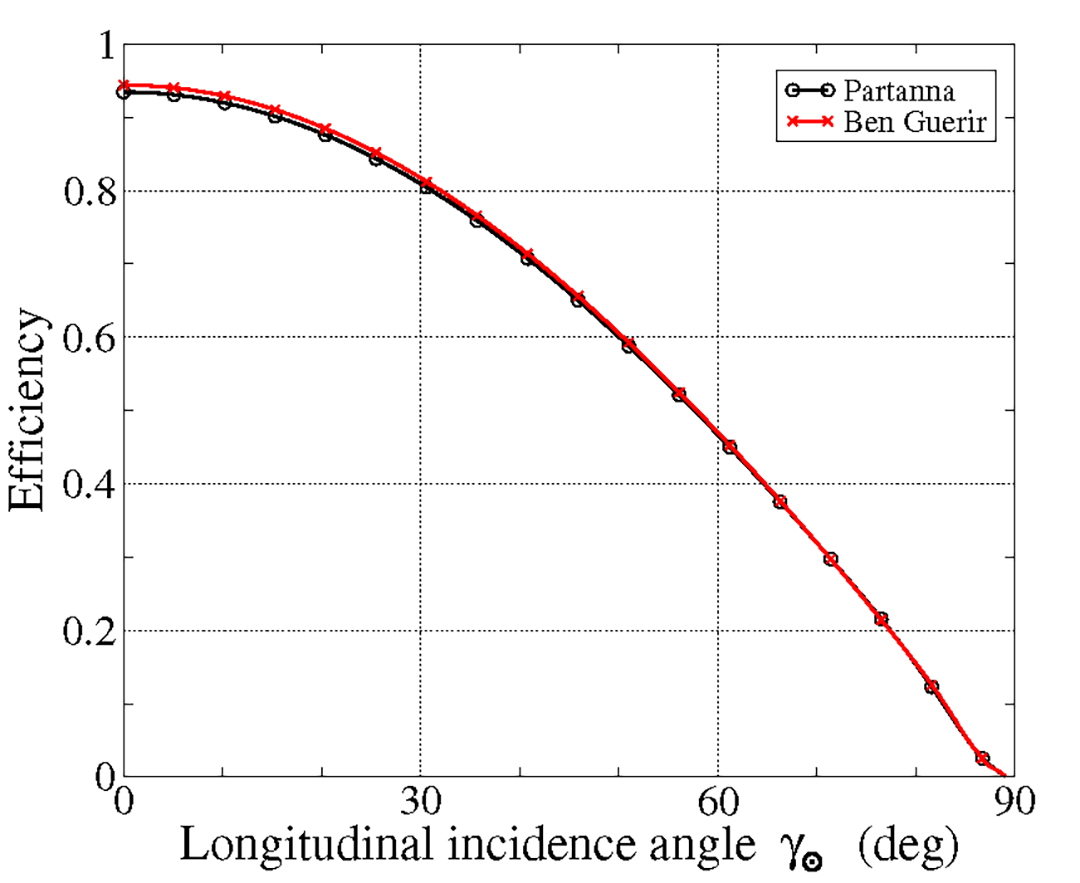
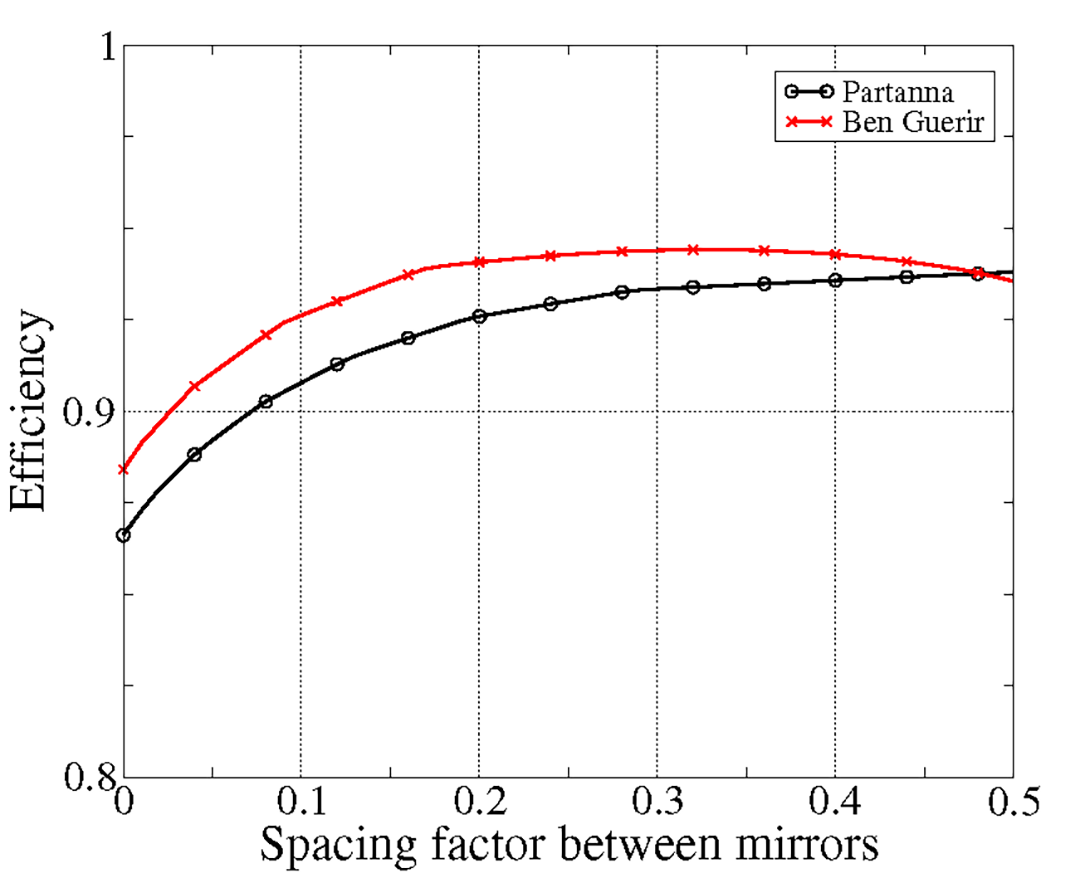
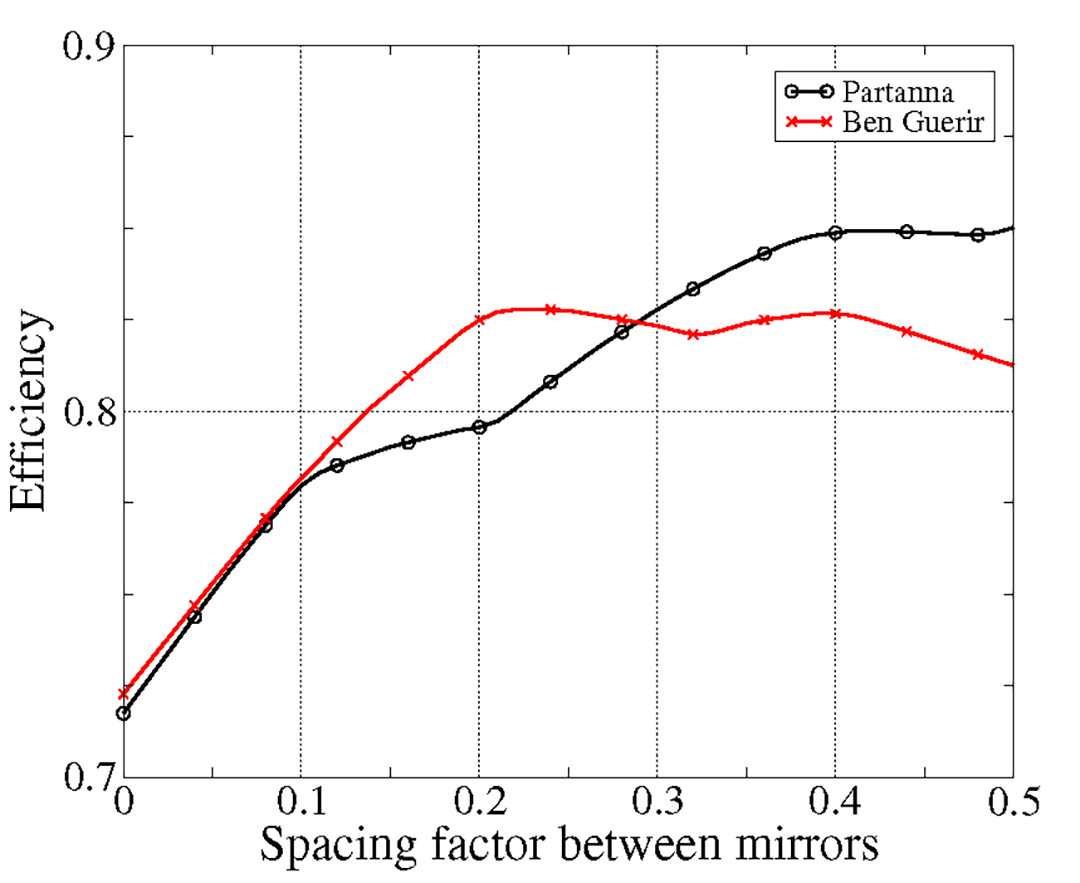
7. Examples of Simulations
8. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations and Symbols
| CFD | Computational Fluid Dynamics |
| CPV | Concentrating Photovoltaic |
| DNI | Direct Normal Irradiation |
| GAX | Generator-absorber heat exchange |
| IAM | Incidence Angle Modifier |
| LFR | Linear Fresnel concentrator |
| PTC | Parabolic Trough Collector |
| PV | Photovoltaic |
| PV/T | Photovoltaic/Thermal |
| SEV | South-East-Vertical reference frame |
| a | Sun angular radius |
| Width of the active part of the nth mirror | |
| , | Min./max. distance between the receiver and the mirror n, in projection |
| Local coordinate on the nth mirror in section | |
| of projection of receiver shadow | |
| of intersection for blocking | |
| of intersection between a ray and a mirror | |
| of projection of other mirrors’ shadow | |
| Inverse of focal length of the nth mirror | |
| H | Height of the LFR receiver (in local frame) |
| Incident ray versor | |
| Collected power for length unit, for collimated radiation | |
| Semi-width of the effective receiver aperture | |
| Semi-width of the effective receiver shadowing plane | |
| L | Width of the origin of rays for ray-tracing |
| M | Number of integration nodes |
| N | Number of primary mirrors |
| n | Progressive number identifying a mirror () |
| Versor orthogonal to the mirror | |
| x component of | |
| Number of shot rays in ray-tracing | |
| Projections of the two edges of the receiver () on the plane containing a mirror | |
| Weights associated to integration nodes | |
| Projections of the two edges of a mirror on the plane | |
| Reflected ray versor | |
| x component of | |
| y component of | |
| z component of | |
| Correction factor to a mirror contribution for end effects | |
| Global correction factor for end effects, for all the mirror field (i: different approximations) | |
| Initial fraction of inactive mirror for end effects | |
| Final fraction of inactive mirror for end effects | |
| Standard integration nodes | |
| Semi-width of the nth mirror | |
| Starting x of a ray in ray-tracing | |
| x coord. of a section of the receiver (when considering end effects) | |
| x coord. at the receiver of a ray reflected from | |
| x coord. at the receiver of a ray reflected from | |
| , | The mirror field extends in x direction between the two |
| , | The receiver extends in x direction between the two |
| y coord. of projection of receiver shadow | |
| Starting y of a ray in ray-tracing | |
| y of intersection for blocking | |
| y of intersection with the receiver plane of a ray reflected at | |
| y of intersection between a ray and a mirror | |
| y coord. of the axis of the nth mirror | |
| y of intercept between a ray and the shadowing plane | |
| y of intercept between a reflected ray and the receiver | |
| Starting z of a ray in ray-tracing | |
| z of intersection between a ray and a mirror | |
| Transversal incidence angle | |
| of the Sun center | |
| Integration nodes in | |
| Local Azimuth (in frame) | |
| Global Azimuth (in SEV frame) | |
| Longitudinal incidence angle, or simply incidence angle | |
| of the Sun center | |
| Random deviation of due to tracking error | |
| Longitudinal incidence angle (alternative definition) | |
| of the Sun center | |
| Optical efficiency of the receiver | |
| Auxiliary angle (see Figure 1) | |
| Auxiliary angle | |
| Mirror reflectivity | |
| Standard deviation of slope + tracking errors | |
| Local Zenith (in frame) | |
| Local Zenith of the Sun center | |
| Global Zenith (in SEV frame) | |
| Inclination of the nth mirror | |
| Incidence angle on the plane of mirror n | |
| Azimuth of the focal line in SEV frame | |
| Inclination of the focal line w.r.t horizontal plane | |
| Third rotation angle | |
| Inclination of local y-axis w.r.t. horizontal plane |
References
- Francia, G. Pilot plants of solar steam generating stations. Sol. Energy 1968, 12, 51–64. [Google Scholar] [CrossRef]
- Canio, D.G.D.; Treytl, W.J.; Jur, F.A.; Watson, C.D. Line Focus Solar Thermal Central Receiver Research Study. Final Report, April 30, 1977–March 30, 1979; Technical Report DOE/ET/20426-1; U.S. Department of Energy: Washington, DC, USA, 1979. [Google Scholar]
- Singh, R.; Mathur, S.; Kandpal, T. Some geometrical design aspects of a linear fresnel reflector concentrator. Int. J. Energy Res. 1980, 4, 59–67. [Google Scholar] [CrossRef]
- Singhal, A.; Singh, R.; Kandpal, T.; Mathur, S. Geometrical concentration characteristics of a linear Fresnel reflector using a fin receiver. Opt. Appl. 1982, 12, 373. [Google Scholar]
- Singhal, A.; Sharma, M.; Negi, B.; Mathur, S. Performance testing of a linear Fresnel reflector. Int. J. Energy Res. 1986, 10, 39–46. [Google Scholar] [CrossRef]
- Goswami, R.; Negi, B.; Sehgal, H.; Sootha, G. Optical designs and concentration characteristics of a linear Fresnel reflector solar concentrator with a triangular absorber. Sol. Energy Mater. 1990, 21, 237–251. [Google Scholar] [CrossRef]
- Negi, B.; Kandpal, T.; Mathur, S. Designs and performance characteristics of a linear fresnel reflector solar concentrator with a flat vertical absorber. Sol. Wind Technol. 1990, 7, 379–392. [Google Scholar] [CrossRef]
- Mathur, S.; Kandpal, T.; Negi, B. Optical design and concentration characteristics of linear Fresnel reflector solar concentrators–I. Mirror elements of varying width. Energy Convers. Manag. 1991, 31, 205–219. [Google Scholar] [CrossRef]
- Mathur, S.; Kandpal, T.; Negi, B. Optical design and concentration characteristics of linear Fresnel reflector solar concentrators–II. Mirror elements of equal width. Energy Convers. Manag. 1991, 31, 221–232. [Google Scholar] [CrossRef]
- Sootha, G.; Negi, B. A comparative study of optical designs and solar flux concentrating characteristics of a linear fresnel reflector solar concentrator with tubular absorber. Sol. Energy Mater. Sol. Cells 1994, 32, 169–186. [Google Scholar] [CrossRef]
- Negi, B.; Mathur, S.; Kandpal, T. Optical and thermal performance evaluation of a linear fresnel reflector solar concentrator. Sol. Wind Technol. 1989, 6, 589–593. [Google Scholar] [CrossRef]
- Gordon, J.M.; Ries, H. Tailored edge-ray concentrators as ideal second stages for Fresnel reflectors. Appl. Opt. 1993, 32, 2243–2251. [Google Scholar] [CrossRef]
- Feuermann, D.; Gordon, J.M. Analysis of a Two-Stage Linear Fresnel Reflector Solar Concentrator. J. Sol. Energy Eng. 1991, 113, 272–279. [Google Scholar] [CrossRef]
- Mills, D.R.; Morrison, G.L. Compact Linear Fresnel Reflector solar thermal powerplants. Sol. Energy 2000, 68, 263–283. [Google Scholar] [CrossRef]
- Morin, G.; Platzer, W.; Eck, M.; Uhlig, R.; Häberle, A.; Berger, M.; Zarza, E. Road map towards the demonstration of a linear Fresnel collector using single tube receiver. In Proceedings of the 13th International Symposium on Concentrated Solar Power and Chemical Energy Technologies, Seville, Spain, 20–24 June 2006. [Google Scholar]
- Abbas, R.; Muñoz-Antón, J.; Valdés, M.; Martínez-Val, J. High concentration linear Fresnel reflectors. Energy Convers. Manag. 2013, 72, 60–68. [Google Scholar] [CrossRef]
- Pino, F.; Caro, R.; Rosa, F.; Guerra, J. Experimental validation of an optical and thermal model of a linear Fresnel collector system. Appl. Therm. Eng. 2013, 50, 1463–1471. [Google Scholar] [CrossRef]
- Montes, M.J.; Rubbia, C.; Abbas, R.; Martínez-Val, J.M. A comparative analysis of configurations of linear Fresnel collectors for concentrating solar power. Energy 2014, 73, 192–203. [Google Scholar] [CrossRef]
- Hofer, A.; Büchner, D.; Kramer, K.; Fahr, S.; Heimsath, A.; Platzer, W.; Scholl, S. Comparison of Two Different (Quasi-) Dynamic Testing Methods for the Performance Evaluation of a Linear Fresnel Process Heat Collector. Energy Procedia 2015, 69, 84–95. [Google Scholar] [CrossRef]
- Beltagy, H.; Semmar, D.; Lehaut, C.; Said, N. Theoretical and experimental performance analysis of a Fresnel type solar concentrator. Renew. Energy 2017, 101, 782–793. [Google Scholar] [CrossRef]
- Bellos, E.; Tzivanidis, C.; Papadopoulos, A. Daily, monthly and yearly performance of a linear Fresnel reflector. Sol. Energy 2018, 173, 517–529. [Google Scholar] [CrossRef]
- Rungasamy, A.; Craig, K.; Meyer, J. Comparative study of the optical and economic performance of etendue-conserving compact linear Fresnel reflector concepts. Sol. Energy 2019, 181, 95–107. [Google Scholar] [CrossRef]
- Babu, M.; Sekhar Babu, P.; Raj, S.S.; Saravanan, S. Theoretical design, material study and material selection for compact linear Fresnel reflector concentrating system. Mater. Today Proc. 2021, 45, 1671–1678. [Google Scholar] [CrossRef]
- Platzer, W.J.; Mills, D.; Gardner, W. Chapter 6—Linear Fresnel Collector (LFC) solar thermal technology. In Concentrating Solar Power Technology, 2nd ed.; Lovegrove, K., Stein, W., Eds.; Woodhead Publishing Series in Energy; Woodhead Publishing: Sawston, UK, 2021; pp. 165–217. [Google Scholar] [CrossRef]
- Zhu, G.; Wendelin, T.; Wagner, M.J.; Kutscher, C. History, current state, and future of linear Fresnel concentrating solar collectors. Sol. Energy 2014, 103, 639–652. [Google Scholar] [CrossRef]
- Bellos, E. Progress in the design and the applications of linear Fresnel reflectors—A critical review. Therm. Sci. Eng. Prog. 2019, 10, 112–137. [Google Scholar] [CrossRef]
- Rungasamy, A.; Craig, K.; Meyer, J. A review of linear Fresnel primary optical design methodologies. Sol. Energy 2021, 224, 833–854. [Google Scholar] [CrossRef]
- Hangweirer, M.; Höller, R.; Schneider, H. Design and analysis of a novel concentrated photovoltaic–thermal receiver concept. Jpn. J. Appl. Phys. 2015, 54, 08KE01. [Google Scholar] [CrossRef]
- Lucio, C.; Behar, O.; Dally, B. Techno-Economic Assessment of CPVT Spectral Splitting Technology: A Case Study on Saudi Arabia. Energies 2023, 16, 5392. [Google Scholar] [CrossRef]
- Boito, P.; Grena, R. Application of a fixed-receiver Linear Fresnel Reflector in concentrating photovoltaics. Sol. Energy 2021, 215, 198–205. [Google Scholar] [CrossRef]
- Dey, C. Heat transfer aspects of an elevated linear absorber. Sol. Energy 2004, 76, 243–249. [Google Scholar] [CrossRef]
- Singh, P.L.; Sarviya, R.; Bhagoria, J. Thermal performance of linear Fresnel reflecting solar concentrator with trapezoidal cavity absorbers. Appl. Energy 2010, 87, 541–550. [Google Scholar] [CrossRef]
- Facão, J.; Oliveira, A.C. Numerical simulation of a trapezoidal cavity receiver for a linear Fresnel solar collector concentrator. Renew. Energy 2011, 36, 90–96. [Google Scholar] [CrossRef]
- Abbas, R.; Muñoz, J.; Martínez-Val, J. Steady-state thermal analysis of an innovative receiver for linear Fresnel reflectors. Appl. Energy 2012, 92, 503–515. [Google Scholar] [CrossRef]
- Natarajan, S.K.; Reddy, K.; Mallick, T.K. Heat loss characteristics of trapezoidal cavity receiver for solar linear concentrating system. Appl. Energy 2012, 93, 523–531. [Google Scholar] [CrossRef]
- Moghimi, M.; Craig, K.; Meyer, J. Optimization of a trapezoidal cavity absorber for the Linear Fresnel Reflector. Sol. Energy 2015, 119, 343–361. [Google Scholar] [CrossRef]
- Qiu, Y.; He, Y.L.; Wu, M.; Zheng, Z.J. A comprehensive model for optical and thermal characterization of a linear Fresnel solar reflector with a trapezoidal cavity receiver. Renew. Energy 2016, 97, 129–144. [Google Scholar] [CrossRef]
- Montes, M.J.; Barbero, R.; Abbas, R.; Rovira, A. Performance model and thermal comparison of different alternatives for the Fresnel single-tube receiver. Appl. Therm. Eng. 2016, 104, 162–175. [Google Scholar] [CrossRef]
- Montes, M.J.; Abbas, R.; Muñoz, M.; Muñoz-Antón, J.; Martínez-Val, J.M. Advances in the linear Fresnel single-tube receivers: Hybrid loops with non-evacuated and evacuated receivers. Energy Convers. Manag. 2017, 149, 318–333. [Google Scholar] [CrossRef]
- Montanet, E.; Rodat, S.; Falcoz, Q.; Roget, F. Experimental and Numerical Evaluation of Solar Receiver Heat Losses of a Commercial 9 MWe Linear Fresnel Power Plant. Energies 2023, 16, 7912. [Google Scholar] [CrossRef]
- Lin, M.; Sumathy, K.; Dai, Y.; Wang, R.; Chen, Y. Experimental and theoretical analysis on a linear Fresnel reflector solar collector prototype with V-shaped cavity receiver. Appl. Therm. Eng. 2013, 51, 963–972. [Google Scholar] [CrossRef]
- Pardellas, A.; Fortuny Ayuso, P.; Bayón, L.; Barbón, A. A New Two-Foci V-Trough Concentrator for Small-Scale Linear Fresnel Reflectors. Energies 2023, 16, 1597. [Google Scholar] [CrossRef]
- Moghimi, M.; Craig, K.; Meyer, J. A novel computational approach to combine the optical and thermal modelling of Linear Fresnel Collectors using the finite volume method. Sol. Energy 2015, 116, 407–427. [Google Scholar] [CrossRef]
- Moghimi, M.; Rungasamy, A.; Craig, K.; Meyer, J. Introducing CFD in the optical simulation of linear Fresnel collectors. AIP Conf. Proc. 2016, 1734, 020015. [Google Scholar]
- López-Núñez, O.A.; Alfaro-Ayala, J.A.; Jaramillo, O.; Ramírez-Minguela, J.; Castro, J.C.; Damian-Ascencio, C.E.; Cano-Andrade, S. A numerical analysis of the energy and entropy generation rate in a Linear Fresnel Reflector using computational fluid dynamics. Renew. Energy 2020, 146, 1083–1100. [Google Scholar] [CrossRef]
- Alcalde-Morales, S.; Valenzuela, L.; Serrano-Aguilera, J. Heat losses in a trapezoidal cavity receiver of a linear Fresnel collector: A CFD approach. Heliyon 2023, 9, e18692. [Google Scholar] [CrossRef] [PubMed]
- Grena, R.; Tarquini, P. Solar linear Fresnel collector using molten nitrates as heat transfer fluid. Energy 2011, 36, 1048–1056. [Google Scholar] [CrossRef]
- Morin, G.; Karl, M.; Mertins, M.; Selig, M. Molten Salt as a Heat Transfer Fluid in a Linear Fresnel Collector—Commercial Application Backed by Demonstration. Energy Procedia 2015, 69, 689–698. [Google Scholar] [CrossRef]
- Qiu, Y.; He, Y.L.; Cheng, Z.D.; Wang, K. Study on optical and thermal performance of a linear Fresnel solar reflector using molten salt as HTF with MCRT and FVM methods. Appl. Energy 2015, 146, 162–173. [Google Scholar] [CrossRef]
- Bachelier, C.; Stieglitz, R. Design and optimization of linear Fresnel power plants based on the direct molten salt concept. Sol. Energy 2017, 152, 171–192. [Google Scholar] [CrossRef]
- Xu, G.; Song, G.; Zhu, X.; Gao, W.; Li, H.; Quan, Y. Performance evaluation of a direct vapor generation supercritical ORC system driven by linear Fresnel reflector solar concentrator. Appl. Therm. Eng. 2015, 80, 196–204. [Google Scholar] [CrossRef]
- Ghodbane, M.; Boumeddane, B.; Said, Z.; Bellos, E. A numerical simulation of a linear Fresnel solar reflector directed to produce steam for the power plant. J. Clean. Prod. 2019, 231, 494–508. [Google Scholar] [CrossRef]
- Marugán-Cruz, C.; Serrano, D.; Gómez-Hernández, J.; Sánchez-Delgado, S. Solar multiple optimization of a DSG linear Fresnel power plant. Energy Convers. Manag. 2019, 184, 571–580. [Google Scholar] [CrossRef]
- Sepúlveda, F.J.; Miranda, M.T.; Montero, I.; Arranz, J.I.; Lozano, F.J.; Matamoros, M.; Rodríguez, P. Analysis of Potential Use of Linear Fresnel Collector for Direct Steam Generation in Industries of the Southwest of Europe. Energies 2019, 12, 4049. [Google Scholar] [CrossRef]
- Said, Z.; Ghodbane, M.; Sundar, L.S.; Tiwari, A.K.; Sheikholeslami, M.; Boumeddane, B. Heat transfer, entropy generation, economic and environmental analyses of linear fresnel reflector using novel rGO-Co3O4 hybrid nanofluids. Renew. Energy 2021, 165, 420–437. [Google Scholar] [CrossRef]
- Nixon, J.; Davies, P. Cost-exergy optimization of linear Fresnel reflectors. Sol. Energy 2012, 86, 147–156. [Google Scholar] [CrossRef]
- Nixon, J.; Dey, P.; Davies, P. Design of a novel solar thermal collector using a multi-criteria decision-making methodology. J. Clean. Prod. 2013, 59, 150–159. [Google Scholar] [CrossRef]
- Filali Baba, Y.; Ajdad, H.; Al Mers, A.; Bouatem, A.; Bououlid Idrissi, B.; El Alj, S. Preliminary cost-effectiveness assessment of a Linear Fresnel Concentrator: Case studies. Case Stud. Therm. Eng. 2020, 22, 100730. [Google Scholar] [CrossRef]
- Ghodbane, M.; Bellos, E.; Said, Z.; Boumeddane, B.; Khechekhouche, A.; Sheikholeslami, M.; Ali, Z.M. Energy, Financial, and Environmental Investigation of a Direct Steam Production Power Plant Driven by Linear Fresnel Solar Reflectors. J. Sol. Energy Eng. 2020, 143, 021008. [Google Scholar] [CrossRef]
- González-Mora, E.; Durán García, M.D. Methodology for an Opto-Geometric Optimization of a Linear Fresnel Reflector for Direct Steam Generation. Energies 2020, 13, 355. [Google Scholar] [CrossRef]
- González-Mora, E.; Durán-García, M.D. Energy and Exergy (2E) Analysis of an Optimized Solar Field of Linear Fresnel Reflectors for a Conceptual Direct Steam Generation Power Plant. Energies 2021, 14, 4234. [Google Scholar] [CrossRef]
- Ordóñez, F.; Flores, E.; Soria, R. Comprehensive analysis of the variables influencing the techno-economic optimization of medium temperature linear Fresnel collectors. Energy Rep. 2021, 7, 5747–5761. [Google Scholar] [CrossRef]
- Askari, I.B.; Ameri, M. Techno economic feasibility analysis of Linear Fresnel solar field as thermal source of the MED/TVC desalination system. Desalination 2016, 394, 1–17. [Google Scholar] [CrossRef]
- Serag-Eldin, M.A. Thermal design of a roof-mounted CLFR collection system for a desert absorption chiller. Int. J. Sustain. Energy 2014, 33, 506–524. [Google Scholar] [CrossRef]
- Velázquez, N.; García-Valladares, O.; Sauceda, D.; Beltrán, R. Numerical simulation of a Linear Fresnel Reflector Concentrator used as direct generator in a Solar-GAX cycle. Energy Convers. Manag. 2010, 51, 434–445. [Google Scholar] [CrossRef]
- Duan, Z.; An, W. Promote optical performance of linear Fresnel micro-concentrator by an offset-axis mirror layout in building-integrated PV/T application. Renew. Energy 2022, 200, 1047–1058. [Google Scholar] [CrossRef]
- Marefati, M.; Mehrpooya, M.; Mousavi, S.A. Introducing an integrated SOFC, linear Fresnel solar field, Stirling engine and steam turbine combined cooling, heating and power process. Int. J. Hydrogen Energy 2019, 44, 30256–30279. [Google Scholar] [CrossRef]
- Marefati, M.; Mehrpooya, M. Introducing and investigation of a combined molten carbonate fuel cell, thermoelectric generator, linear fresnel solar reflector and power turbine combined heating and power process. J. Clean. Prod. 2019, 240, 118247. [Google Scholar] [CrossRef]
- Barbón, A.; Barbón, N.; Bayón, L.; Otero, J. Theoretical elements for the design of a small scale Linear Fresnel Reflector: Frontal and lateral views. Sol. Energy 2016, 132, 188–202. [Google Scholar] [CrossRef]
- Barbón, A.; Barbón, N.; Bayón, L.; Sánchez-Rodríguez, J. Parametric study of the small scale linear Fresnel reflector. Renew. Energy 2018, 116, 64–74. [Google Scholar] [CrossRef]
- Barbón, A.; Bayón-Cueli, C.; Bayón, L.; Rodríguez, L. Investigating the influence of longitudinal tilt angles on the performance of small scale linear Fresnel reflectors for urban applications. Renew. Energy 2019, 143, 1581–1593. [Google Scholar] [CrossRef]
- Barbón, A.; Bayón-Cueli, C.; Bayón, L.; Ayuso, P.F. Influence of solar tracking error on the performance of a small-scale linear Fresnel reflector. Renew. Energy 2020, 162, 43–54. [Google Scholar] [CrossRef]
- Bayón-Cueli, C.; Barbón, A.; Bayón, L.; Barbón, N. A cost-energy based methodology for small-scale linear Fresnel reflectors on flat roofs of urban buildings. Renew. Energy 2020, 146, 944–959. [Google Scholar] [CrossRef]
- Barbón, A.; Fernández-Rubiera, J.; Martínez-Valledor, L.; Pérez-Fernández, A.; Bayón, L. Design and construction of a solar tracking system for small-scale linear Fresnel reflector with three movements. Appl. Energy 2021, 285, 116477. [Google Scholar] [CrossRef]
- Nixon, J.D.; Davies, P.A. Construction and Experimental Study of an Elevation Linear Fresnel Reflector. J. Sol. Energy Eng. 2016, 138, 031001. [Google Scholar] [CrossRef]
- Taramona, S.; Ángel González-Gómez, P.; Briongos, J.V.; Gómez-Hernández, J. Designing a flat beam-down linear Fresnel reflector. Renew. Energy 2022, 187, 484–499. [Google Scholar] [CrossRef]
- Yang, M.; Zhu, Y.; Taylor, R.A. End losses minimization of linear Fresnel reflectors with a simple, two-axis mechanical tracking system. Energy Convers. Manag. 2018, 161, 284–293. [Google Scholar] [CrossRef]
- Camacho, E.F.; Gallego, A.J.; Sanchez, A.J.; Berenguel, M. Incremental State-Space Model Predictive Control of a Fresnel Solar Collector Field. Energies 2019, 12, 3. [Google Scholar] [CrossRef]
- Montenon, A.C.; Meligy, R. Control Strategies Applied to a Heat Transfer Loop of a Linear Fresnel Collector. Energies 2022, 15, 3338. [Google Scholar] [CrossRef]
- Ruiz-Moreno, S.; Gallego, A.J.; Sanchez, A.J.; Camacho, E.F. Fault Detection and Isolation Based on Deep Learning for a Fresnel Collector Field. IFAC-PapersOnLine 2022, 55, 563–568. [Google Scholar] [CrossRef]
- Pulido-Iparraguirre, D.; Valenzuela, L.; Fernández-Reche, J.; Galindo, J.; Rodríguez, J. Design, Manufacturing and Characterization of Linear Fresnel Reflector’s Facets. Energies 2019, 12, 2795. [Google Scholar] [CrossRef]
- Hoshi, A.; Mills, D.R.; Bittar, A.; Saitoh, T.S. Screening of high melting point phase change materials (PCM) in solar thermal concentrating technology based on CLFR. Sol. Energy 2005, 79, 332–339. [Google Scholar] [CrossRef]
- Barale, G.; Heimsath, A.; Nitz, P.; Toro, A. Optical design of a linear Fresnel collector for Sicily. In Proceedings of the 16th SolarPACES International Symposium, Perpignan, France, 21–24 September 2010. [Google Scholar]
- Montenon, A.C.; Tsekouras, P.; Tzivanidis, C.; Bibron, M.; Papanicolas, C. Thermo-optical modelling of the linear Fresnel collector at the Cyprus institute. AIP Conf. Proc. 2019, 2126, 100004. [Google Scholar]
- Gharbi, N.E.; Derbal, H.; Bouaichaoui, S.; Said, N. A comparative study between parabolic trough collector and linear Fresnel reflector technologies. Energy Procedia 2011, 6, 565–572. [Google Scholar] [CrossRef]
- Morin, G.; Dersch, J.; Platzer, W.; Eck, M.; Häberle, A. Comparison of Linear Fresnel and Parabolic Trough Collector power plants. Sol. Energy 2012, 86, 1–12. [Google Scholar] [CrossRef]
- Giostri, A.; Binotti, M.; Silva, P.; Macchi, E.; Manzolini, G. Comparison of Two Linear Collectors in Solar Thermal Plants: Parabolic Trough Versus Fresnel. J. Sol. Energy Eng. 2013, 135, 011001. [Google Scholar] [CrossRef]
- Cau, G.; Cocco, D. Comparison of Medium-size Concentrating Solar Power Plants based on Parabolic Trough and Linear Fresnel Collectors. Energy Procedia 2014, 45, 101–110. [Google Scholar] [CrossRef]
- Schenk, H.; Hirsch, T.; Fabian Feldhoff, J.; Wittmann, M. Energetic Comparison of Linear Fresnel and Parabolic Trough Collector Systems. J. Sol. Energy Eng. 2014, 136, 041015. [Google Scholar] [CrossRef]
- Sait, H.H.; Martinez-Val, J.M.; Abbas, R.; Munoz-Anton, J. Fresnel-based modular solar fields for performance/cost optimization in solar thermal power plants: A comparison with parabolic trough collectors. Appl. Energy 2015, 141, 175–189. [Google Scholar] [CrossRef]
- Abbas, R.; Valdés, M.; Montes, M.; Martínez-Val, J. Design of an innovative linear Fresnel collector by means of optical performance optimization: A comparison with parabolic trough collectors for different latitudes. Sol. Energy 2017, 153, 459–470. [Google Scholar] [CrossRef]
- Sun, J.; Zhang, Z.; Wang, L.; Zhang, Z.; Wei, J. Comprehensive review of line-focus concentrating solar thermal technologies: Parabolic trough collector (PTC) vs linear Fresnel reflector (LFR). J. Therm. Sci. 2020, 29, 1097–1124. [Google Scholar] [CrossRef]
- Kincaid, N.; Mungas, G.; Kramer, N.; Wagner, M.; Zhu, G. An optical performance comparison of three concentrating solar power collector designs in linear Fresnel, parabolic trough, and central receiver. Appl. Energy 2018, 231, 1109–1121. [Google Scholar] [CrossRef]
- Häberle, A.; Zahler, C.; Lerchenmüller, H.; Mertins, M.; Wittwer, C.; Trieb, F.; Dersch, J. The Solarmundo line focussing Fresnel collector. Optical and thermal performance and cost calculations. In Proceedings of the 2002 SolarPACES International Symposium, Zurich, Switzerland, 4–6 September 2002. [Google Scholar]
- Bernhard, R.; Laabs, H.J.; de Lalaing, J.; Eck, M.; Eickhoff, M.; Feldhoff, J.F.; Heimsath, A.; Hülsey, H.; Morin, G. Linear Fresnel collector demonstration on the PSA. Part I—Design, construction and quality control. In Proceedings of the 14th International SolarPACES Conference, Las Vegas, NV, USA, 4–7 March 2008. [Google Scholar]
- Bernhard, R.; Hein, S.; de LaLaing, J.; Eck, M.; Eickhoff, M.; Pfänder, M.; Morin, G.; Häberle, A. Linear Fresnel collector demonstration on the PSA. Part II—Commissioning and first performance tests. In Proceedings of the 14th International SolarPACES Conference, Las Vegas, NV, USA, 4–7 March 2008. [Google Scholar]
- Bernhard, R.; de Lalaing, J.; andMarkus Eck, R.K.; Eickhoff, M.; Pottler, K.; Morin, G.; Heimsath, A.; Georg, A.; Häberle, A. Linear Fresnel collector demonstration on the PSA—Operation and investigation. In Proceedings of the 15th International SolarPACES Conference, Berlin, Germany, 15–18 September 2009. [Google Scholar]
- Conlon, W.M.; Johnson, P.; Hanson, R. Superheated Steam From CLFR Solar Steam Generators. In Proceedings of the ASME 2011 Power Conference, Denver, CO, USA, 12–14 July 2011. [Google Scholar] [CrossRef]
- Falchetta, M.; Mazzei, D.; Russo, V.; Campanella, V.A.; Floridia, V.; Schiavo, B.; Venezia, L.; Brunatto, C.; Orlando, R. The Partanna project: A first of a kind plant based on molten salts in LFR collectors. AIP Conf. Proc. 2020, 2303, 040001. [Google Scholar]
- El Alj, S.; Al Mers, A.; Merroun, O.; Bouatem, A.; Boutammachte, N.; Ajdad, H.; Benyakhlef, S.; Filali Baba, Y. Optical modeling and analysis of the first Moroccan linear fresnel solar collector prototype. ASME J. Sol. Energy Eng. 2017, 139, 041009. [Google Scholar] [CrossRef]
- Said, Z.; Ghodbane, M.; Hachicha, A.A.; Boumeddane, B. Optical performance assessment of a small experimental prototype of linear Fresnel reflector. Case Stud. Therm. Eng. 2019, 16, 100541. [Google Scholar] [CrossRef]
- Miranda, M.; Larra, D.; Montero, I.; Sepúlveda, F.; Arranz, J. Prototype plant for indirect low-pressure steam generation with Fresnel solar collectors: Sizing and commissioning tests. Energy Convers. Manag. X 2024, 21, 100513. [Google Scholar] [CrossRef]
- Morin, G.; Dersch, J.; Eck, M.; Häberle, A.; Platzer, W. Comparison of Linear Fresnel and Parabolic Trough Collector Systems-Influence of Linear Fresnel Collector Design Variations on Break Even Cost. In Proceedings of the 15th SolarPACES International Symposium, Berlin, Germany, 15–18 September 2009. [Google Scholar]
- Mahan, J.R. The Monte Carlo Ray-Trace Method in Radiation Heat Transfer and Applied Optics; John Wiley & Sons: Hoboken, NJ, USA, 2019. [Google Scholar]
- Heimsath, A.; Bern, G.; van Rooyen, D.; Nitz, P. Quantifying Optical Loss Factors of Small Linear Concentrating Collectors for Process Heat Application. Energy Procedia 2014, 48, 77–86. [Google Scholar] [CrossRef]
- Osório, T.; Horta, P.; Larcher, M.; Pujol-Nadal, R.; Hertel, J.; Van Rooyen, D.W.; Heimsath, A.; Schneider, S.; Benitez, D.; Frein, A.; et al. Ray-tracing software comparison for linear focusing solar collectors. AIP Conf. Proc. 2016, 1734, 020017. [Google Scholar]
- Fossa, M.; Boccalatte, A.; Memme, S. Solar Fresnel modelling, geometry enhancement and 3D ray tracing analysis devoted to different energy efficiency definitions and applied to a real facility. Sol. Energy 2021, 216, 75–89. [Google Scholar] [CrossRef]
- Memme, S.; Fossa, M. Ray tracing analysis of linear Fresnel concentrators and the effect of plant azimuth on their optical efficiency. Renew. Energy 2023, 216, 119121. [Google Scholar] [CrossRef]
- Zhu, G. Development of an analytical optical method for linear Fresnel collectors. Sol. Energy 2013, 94, 240–252. [Google Scholar] [CrossRef]
- Abbas, R.; Martínez-Val, J. A comprehensive optical characterization of linear Fresnel collectors by means of an analytic study. Appl. Energy 2017, 185, 1136–1151. [Google Scholar] [CrossRef]
- Eddhibi, F.; Amara, M.B.; Balghouthi, M.; Qoaider, L.; Guizani, A. Analytic optical design of a Linear Fresnel solar collector with variable parameters. J. Mater. Environ. Sci. 2017, 8, 4068–4084. [Google Scholar]
- Santos, A.V.; Canavarro, D.; Horta, P.; Collares-Pereira, M. An analytical method for the optical analysis of Linear Fresnel Reflectors with a flat receiver. Sol. Energy 2021, 227, 203–216. [Google Scholar] [CrossRef]
- Chaves, J.; Collares-Pereira, M. Etendue-matched two-stage concentrators with multiple receivers. Sol. Energy 2010, 84, 196–207. [Google Scholar] [CrossRef]
- Abbas, R.; Martínez-Val, J. Analytic optical design of linear Fresnel collectors with variable widths and shifts of mirrors. Renew. Energy 2015, 75, 81–92. [Google Scholar] [CrossRef]
- Sharma, V.; Nayak, J.K.; Kedare, S.B. Effects of shading and blocking in linear Fresnel reflector field. Sol. Energy 2015, 113, 114–138. [Google Scholar] [CrossRef]
- Sharma, V.; Nayak, J.K.; Kedare, S.B. Comparison of line focusing solar concentrator fields considering shading and blocking. Sol. Energy 2015, 122, 924–939. [Google Scholar] [CrossRef]
- Sharma, V.; Khanna, S.; Nayak, J.K.; Kedare, S.B. Effects of shading and blocking in compact linear fresnel reflector field. Energy 2016, 94, 633–653. [Google Scholar] [CrossRef]
- Abbas, R.; Montes, M.; Piera, M.; Martínez-Val, J. Solar radiation concentration features in Linear Fresnel Reflector arrays. Energy Convers. Manag. 2012, 54, 133–144. [Google Scholar] [CrossRef]
- Santos, A.V.; Canavarro, D.; Horta, P.; Collares-Pereira, M. On the comparison of parabolical and cylindrical primary mirrors for linear Fresnel solar concentrators. Renew. Energy 2023, 218, 119380. [Google Scholar] [CrossRef]
- Benyakhlef, S.; Al Mers, A.; Merroun, O.; Bouatem, A.; Boutammachte, N.; El Alj, S.; Ajdad, H.; Erregueragui, Z.; Zemmouri, E. Impact of heliostat curvature on optical performance of Linear Fresnel solar concentrators. Renew. Energy 2016, 89, 463–474. [Google Scholar] [CrossRef]
- El Amine, M.; Sallaou, M. Integration of mechanical deformation and optical losses in the design of linear Fresnel solar collectors. Int. J. Interact. Des. Manuf. (IJIDeM) 2019, 13, 831–840. [Google Scholar] [CrossRef]
- He, Y.L.; Wang, K.; Qiu, Y.; Du, B.C.; Liang, Q.; Du, S. Review of the solar flux distribution in concentrated solar power: Non-uniform features, challenges, and solutions. Appl. Therm. Eng. 2019, 149, 448–474. [Google Scholar] [CrossRef]
- Qiu, Y.; Li, M.J.; Wang, K.; Liu, Z.B.; Xue, X.D. Aiming strategy optimization for uniform flux distribution in the receiver of a linear Fresnel solar reflector using a multi-objective genetic algorithm. Appl. Energy 2017, 205, 1394–1407. [Google Scholar] [CrossRef]
- Walker, G.; Von Backström, T.; Gauché, P. A method of increasing collector aperture in linear Fresnel solar concentrators at high zenith angles. In Proceedings of the 1st Annual Southern African Solar Energy Conference, Stellenbosch, South Africa, 21–23 May 2012; pp. 21–23. [Google Scholar]
- Hongn, M.; Larsen, S.F.; Gea, M.; Altamirano, M. Least square based method for the estimation of the optical end loss of linear Fresnel concentrators. Sol. Energy 2015, 111, 264–276. [Google Scholar] [CrossRef]
- Lv, H.; Chen, K.; Chen, P.; Wang, X.; Wen, L. Optical Efficiency Analysis of Primary Mirror in LFR-CSP. J. Phys. Conf. Ser. 2022, 2254, 012030. [Google Scholar] [CrossRef]
- Nakhaei Zadeh, A.; Ameri, M.; Shojaei, A.; Baniasad Askari, I. Optical Efficiency of Linear Fresnel Reflectors in Fixed, Variable and Optimal Distance between Mirrors: Theoretical and Experimental Studies. Int. J. Eng. 2024, 37, 283–297. [Google Scholar] [CrossRef]
- Zhu, J.; Huang, H. Design and thermal performances of Semi-Parabolic Linear Fresnel Reflector solar concentration collector. Energy Convers. Manag. 2014, 77, 733–737. [Google Scholar] [CrossRef]
- Zhu, Y.; Shi, J.; Li, Y.; Wang, L.; Huang, Q.; Xu, G. Design and experimental investigation of a stretched parabolic linear Fresnel reflector collecting system. Energy Convers. Manag. 2016, 126, 89–98. [Google Scholar] [CrossRef]
- Zhu, Y.; Shi, J.; Li, Y.; Wang, L.; Huang, Q.; Xu, G. Design and thermal performances of a scalable linear Fresnel reflector solar system. Energy Convers. Manag. 2017, 146, 174–181. [Google Scholar] [CrossRef]
- Zhu, J.; Chen, Z. Optical design of compact linear fresnel reflector systems. Sol. Energy Mater. Sol. Cells 2018, 176, 239–250. [Google Scholar] [CrossRef]
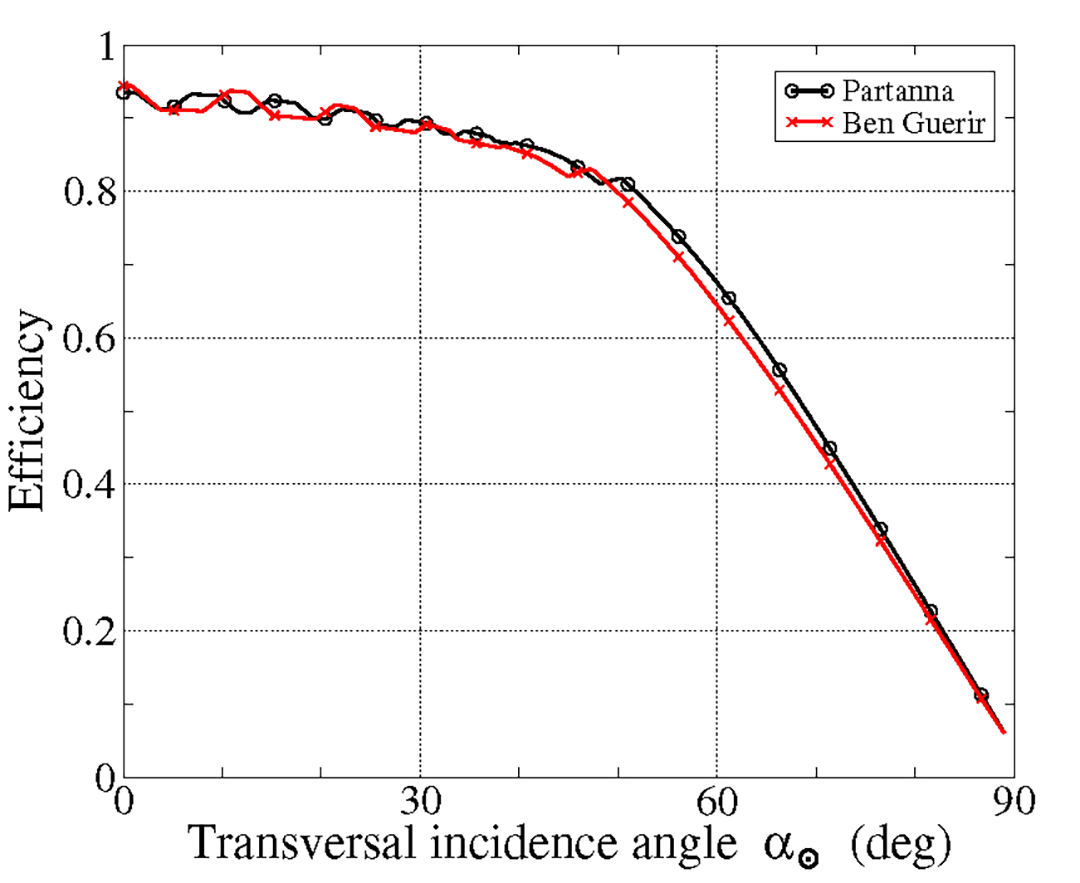
- Bellos, E.; Tzivanidis, C. Development of analytical expressions for the incident angle modifiers of a linear Fresnel reflector. Sol. Energy 2018, 173, 769–779. [Google Scholar] [CrossRef]
- Boccalatte, A.; Fossa, M.; Ménézo, C. Calculation of the incidence angle modifier of a Linear Fresnel Collector: The proposed declination and zenith angle model compared to the biaxial factored approach. Renew. Energy 2022, 185, 123–138. [Google Scholar] [CrossRef]
- Memme, S.; Fossa, M. A novel approach for incidence angle modifier calculation of arbitrarily oriented linear Fresnel collectors: Theory, simulations and case studies. Renew. Energy 2024, 222, 119857. [Google Scholar] [CrossRef]
- Boito, P.; Grena, R. Optimization of the geometry of Fresnel linear collectors. Sol. Energy 2016, 135, 479–486. [Google Scholar] [CrossRef]
- Cheng, Z.D.; Zhao, X.R.; He, Y.L.; Qiu, Y. A novel optical optimization model for linear Fresnel reflector concentrators. Renew. Energy 2018, 129, 486–499. [Google Scholar] [CrossRef]
- Ajdad, H.; Filali Baba, Y.; Al Mers, A.; Merroun, O.; Bouatem, A.; Boutammachte, N. Particle swarm optimization algorithm for optical-geometric optimization of linear Fresnel solar concentrators. Renew. Energy 2019, 130, 992–1001. [Google Scholar] [CrossRef]
- Pulido-Iparraguirre, D.; Valenzuela, L.; Serrano-Aguilera, J.J.; Fernández-García, A. Optimized design of a Linear Fresnel reflector for solar process heat applications. Renew. Energy 2019, 131, 1089–1106. [Google Scholar] [CrossRef]
- López-Núñez, O.A.; Alfaro-Ayala, J.A.; Ramírez-Minguela, J.; Belman-Flores, J.; Jaramillo, O. Optimization of a Linear Fresnel Reflector Applying Computational Fluid Dynamics, Entropy Generation Rate and Evolutionary Programming. Renew. Energy 2020, 152, 698–712. [Google Scholar] [CrossRef]
- Ma, J.; Wang, C.L.; Zhou, Y.; Wang, R.D. Optimized design of a linear Fresnel collector with a compound parabolic secondary reflector. Renew. Energy 2021, 171, 141–148. [Google Scholar] [CrossRef]
- Ahmadpour, A.; Dejamkhooy, A.; Shayeghi, H. Optimization and modelling of linear Fresnel reflector solar concentrator using various methods based on Monte Carlo Ray–Trace. Sol. Energy 2022, 245, 67–79. [Google Scholar] [CrossRef]
- Boito, P.; Grena, R. Optimal focal length of primary mirrors in Fresnel linear collectors. Sol. Energy 2017, 155, 1313–1318. [Google Scholar] [CrossRef]
- Santos, A.V.; Canavarro, D.; Horta, P.; Collares-Pereira, M. Assessment of the optimal curvature radius of linear Fresnel primary mirrors. Sol. Energy 2024, 270, 112376. [Google Scholar] [CrossRef]
- Bellos, E.; Tzivanidis, C.; Papadopoulos, A. Secondary concentrator optimization of a linear Fresnel reflector using Bezier polynomial parametrization. Sol. Energy 2018, 171, 716–727. [Google Scholar] [CrossRef]
- Vouros, A.; Mathioulakis, E.; Papanicolaou, E.; Belessiotis, V. On the optimal shape of secondary reflectors for linear Fresnel collectors. Renew. Energy 2019, 143, 1454–1464. [Google Scholar] [CrossRef]
- Rosell, J.; Vallverdú, X.; Lechón, M.; Ibáñez, M. Design and simulation of a low concentrating photovoltaic/thermal system. Energy Convers. Manag. 2005, 46, 3034–3046. [Google Scholar] [CrossRef]
- Liu, Y.; Hu, P.; Zhang, Q.; Chen, Z. Thermodynamic and optical analysis for a CPV/T hybrid system with beam splitter and fully tracked linear Fresnel reflector concentrator utilizing sloped panels. Sol. Energy 2014, 103, 191–199. [Google Scholar] [CrossRef]
- Gomaa, M.R.; Mustafa, R.J.; Rezk, H. An experimental implementation and testing of a concentrated hybrid photovoltaic/thermal system with monocrystalline solar cells using linear Fresnel reflected mirrors. Int. J. Energy Res. 2019, 43, 8660–8673. [Google Scholar] [CrossRef]
- Wang, G.; Wang, F.; Shen, F.; Jiang, T.; Chen, Z.; Hu, P. Experimental and optical performances of a solar CPV device using a linear Fresnel reflector concentrator. Renew. Energy 2020, 146, 2351–2361. [Google Scholar] [CrossRef]
- McIntire, W.R. Factored approximations for biaxial incident angle modifiers. Sol. Energy 1982, 29, 315–322. [Google Scholar] [CrossRef]
- Hertel, J.D.; Martinez-Moll, V.; Pujol-Nadal, R. Estimation of the influence of different incidence angle modifier models on the biaxial factorization approach. Energy Convers. Manag. 2015, 106, 249–259. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; US Government Printing Office: Washington, DC, USA, 1968; Volume 55.
- Michalsky, J.J. The Astronomical Almanac’s algorithm for approximate solar position (1950–2050). Sol. Energy 1988, 40, 227–235. [Google Scholar] [CrossRef]
- Grena, R. Five new algorithms for the computation of sun position from 2010 to 2110. Sol. Energy 2012, 86, 1323–1337. [Google Scholar] [CrossRef]



| Strategy | = 0 mrad | = 2 mrad | = 5 mrad |
|---|---|---|---|
| Quasi-analytical (no errors) | 1 | — | — |
| Ray-tracing | 4104 | 14,693 | 31,269 |
| Quasi-analytical ( sampling) | — | 6144 | 10,614 |
| Quasi-analytical (blurred mirrors) | — | 11.1 | 8.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grena, R. Geometrical Aspects of the Optics of Linear Fresnel Concentrators: A Review. Energies 2024, 17, 3564. https://doi.org/10.3390/en17143564
Grena R. Geometrical Aspects of the Optics of Linear Fresnel Concentrators: A Review. Energies. 2024; 17(14):3564. https://doi.org/10.3390/en17143564
Chicago/Turabian StyleGrena, Roberto. 2024. "Geometrical Aspects of the Optics of Linear Fresnel Concentrators: A Review" Energies 17, no. 14: 3564. https://doi.org/10.3390/en17143564
APA StyleGrena, R. (2024). Geometrical Aspects of the Optics of Linear Fresnel Concentrators: A Review. Energies, 17(14), 3564. https://doi.org/10.3390/en17143564







