Research on the Design of a MIMO Management System for Lithium-Ion Batteries Based on Radiation–Conductivity–Convection Coupled Thermal Analysis
Abstract
1. Introduction
2. Radiation–Conduction–Convection Coupled Model for Lithium-Ion Battery Packs
2.1. Battery Pack Mathematical Model
2.2. Battery Pack Physical Model
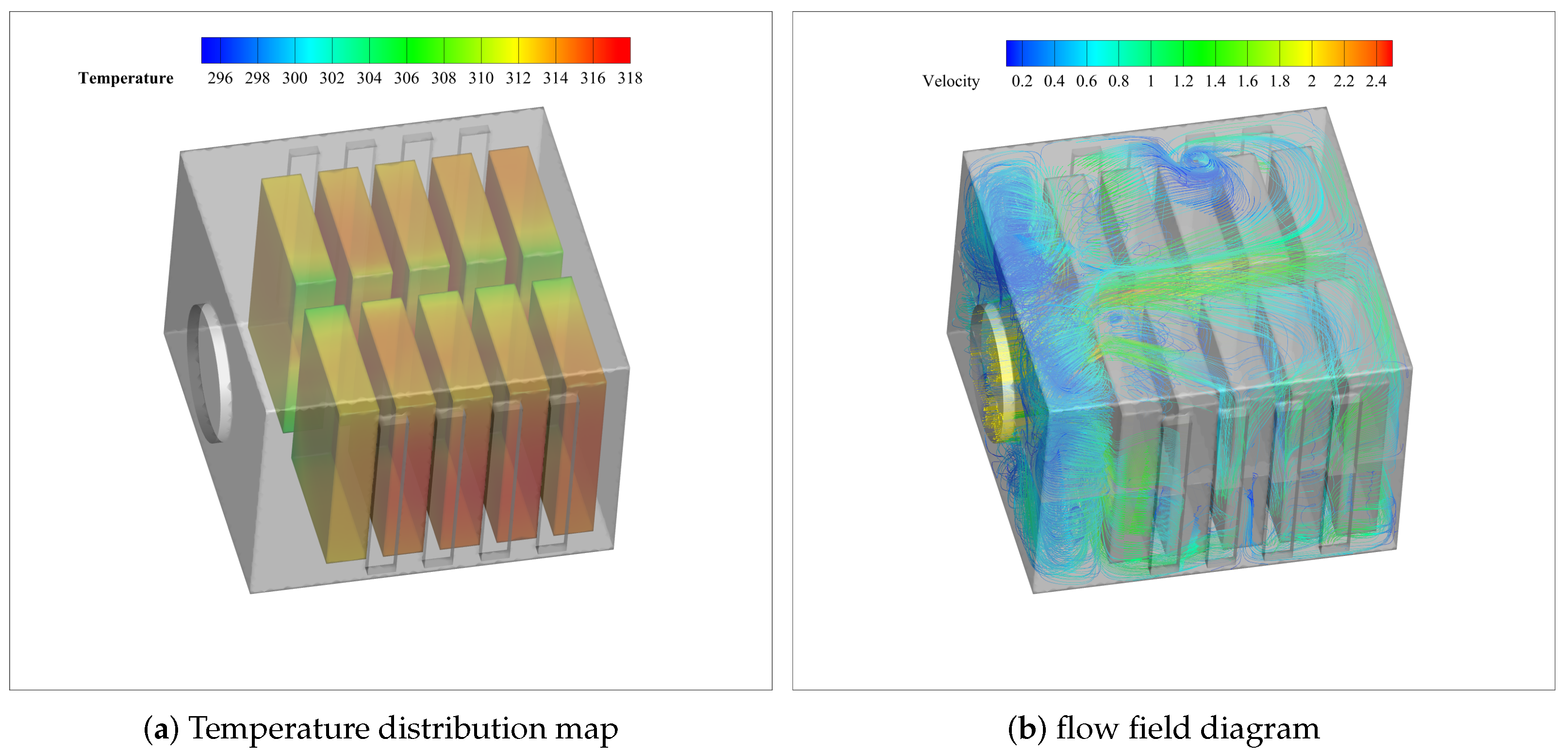
2.3. Original Model Simulation Results
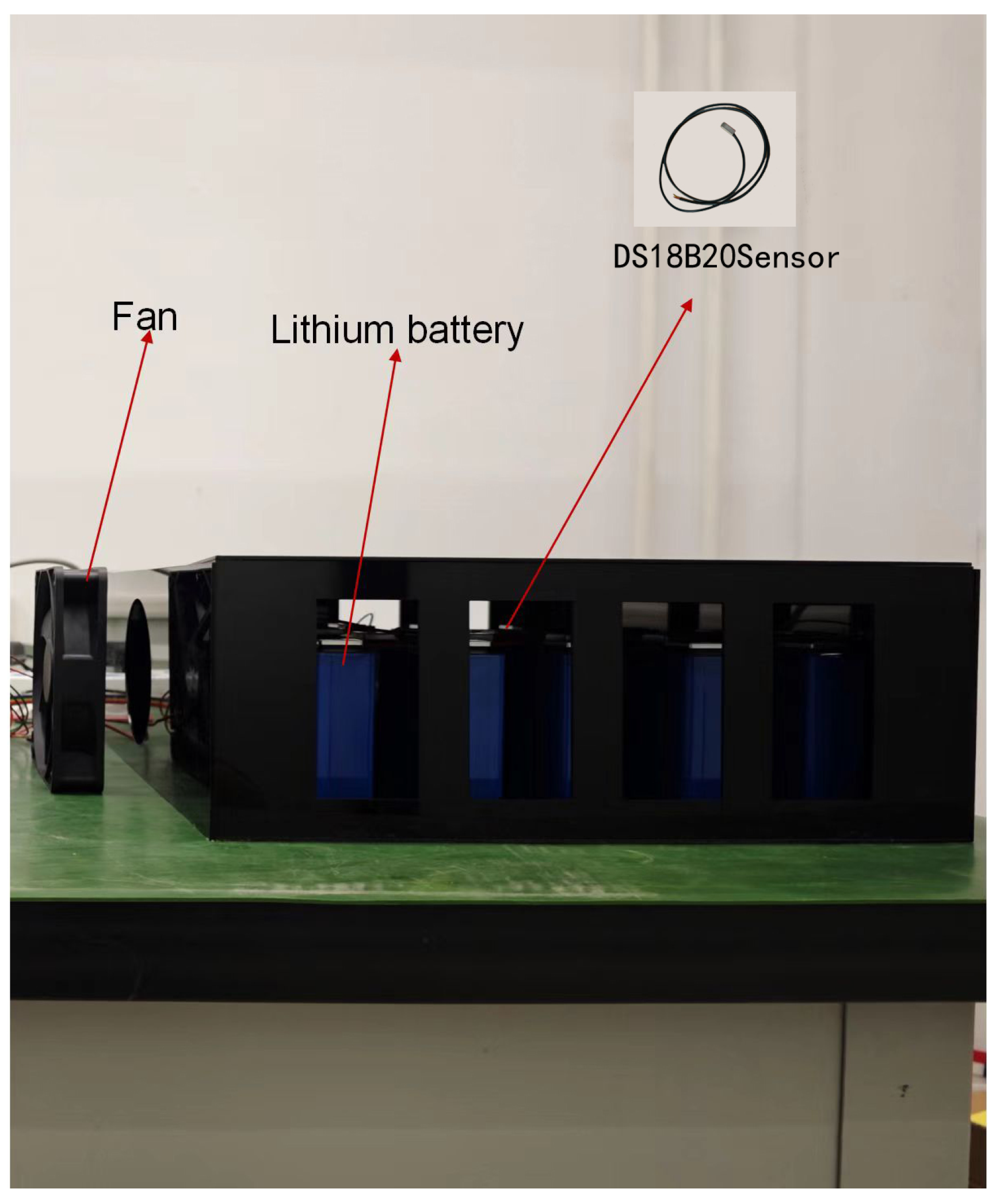
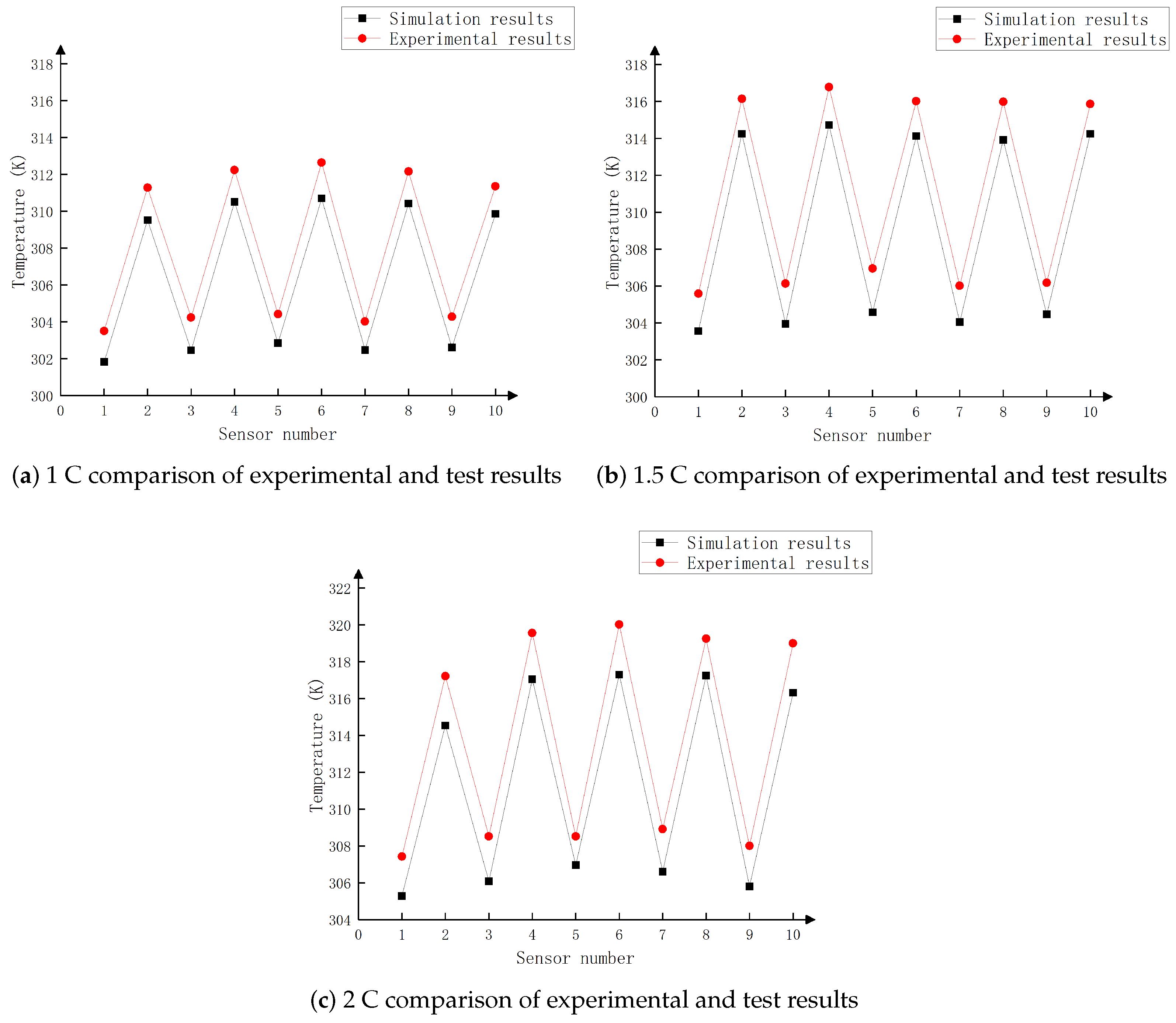
2.4. Experimental Results of the Original Model
3. Optimization of Air Cooling Heat Dissipation in Battery Packs
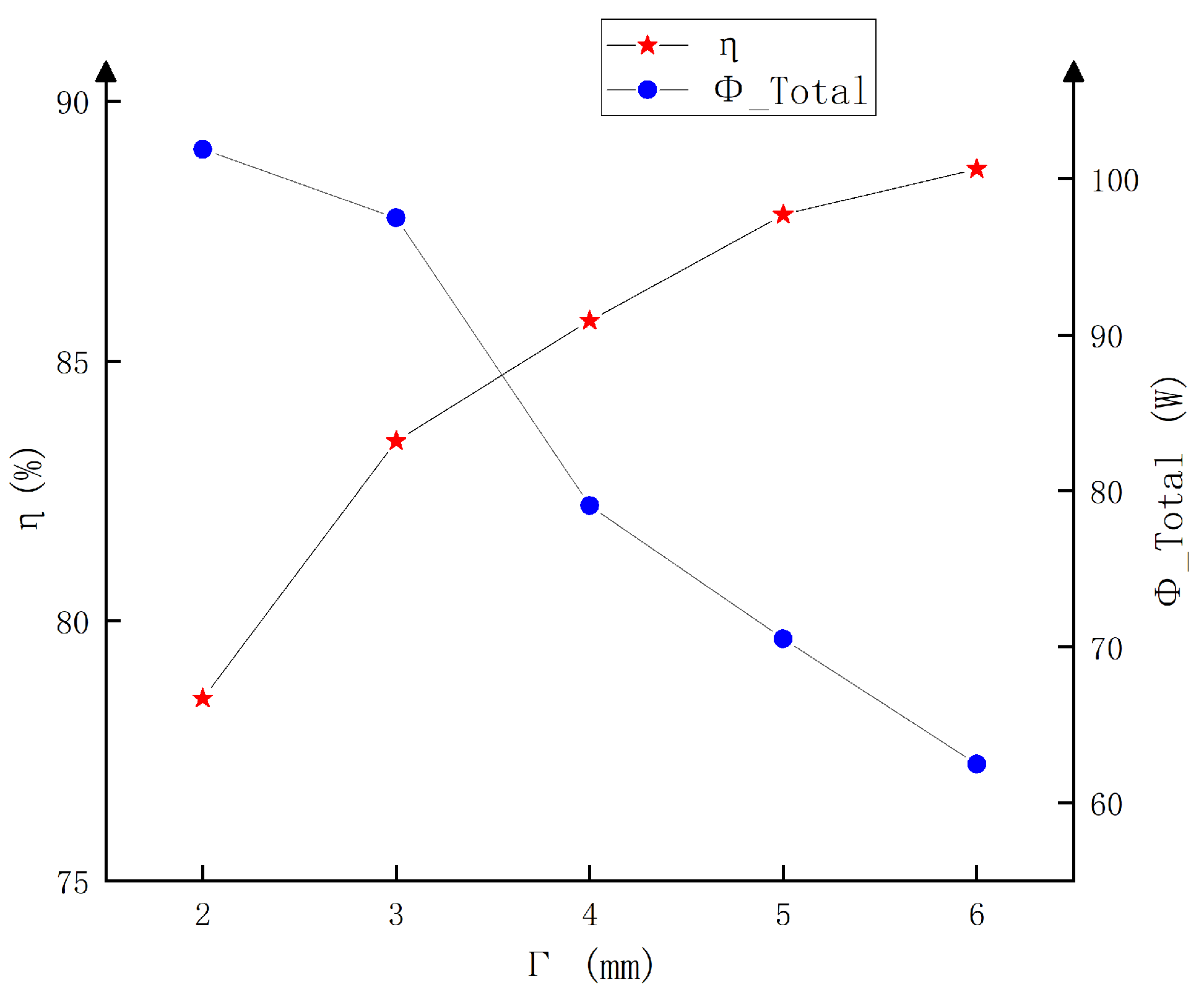
3.1. Analysis of Heat Transfer Performance of Batteries with Additional Heat Dissipation Ribs
3.2. Analysis of Heat Transfer Performance of Applied Thermal Coatings
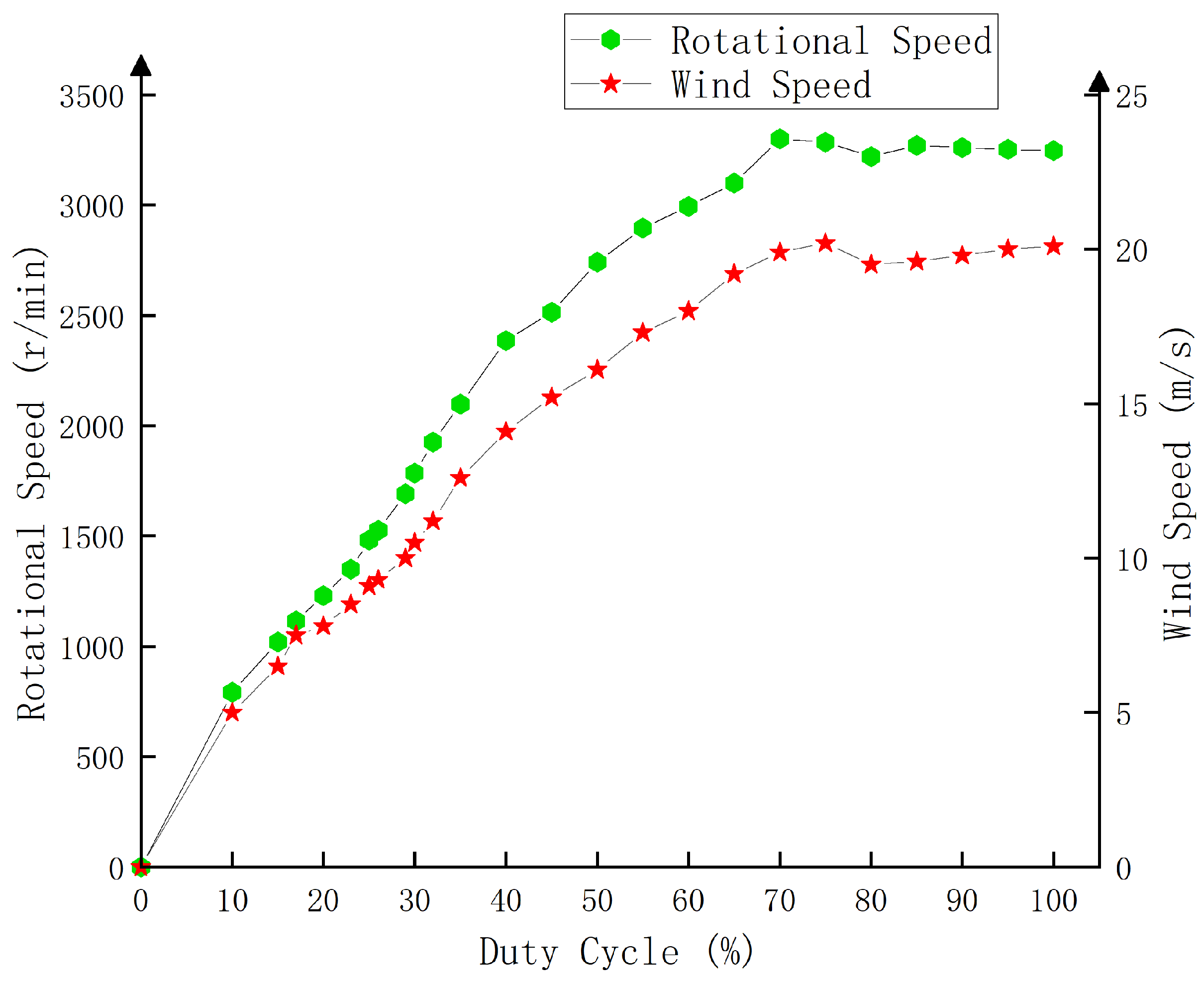
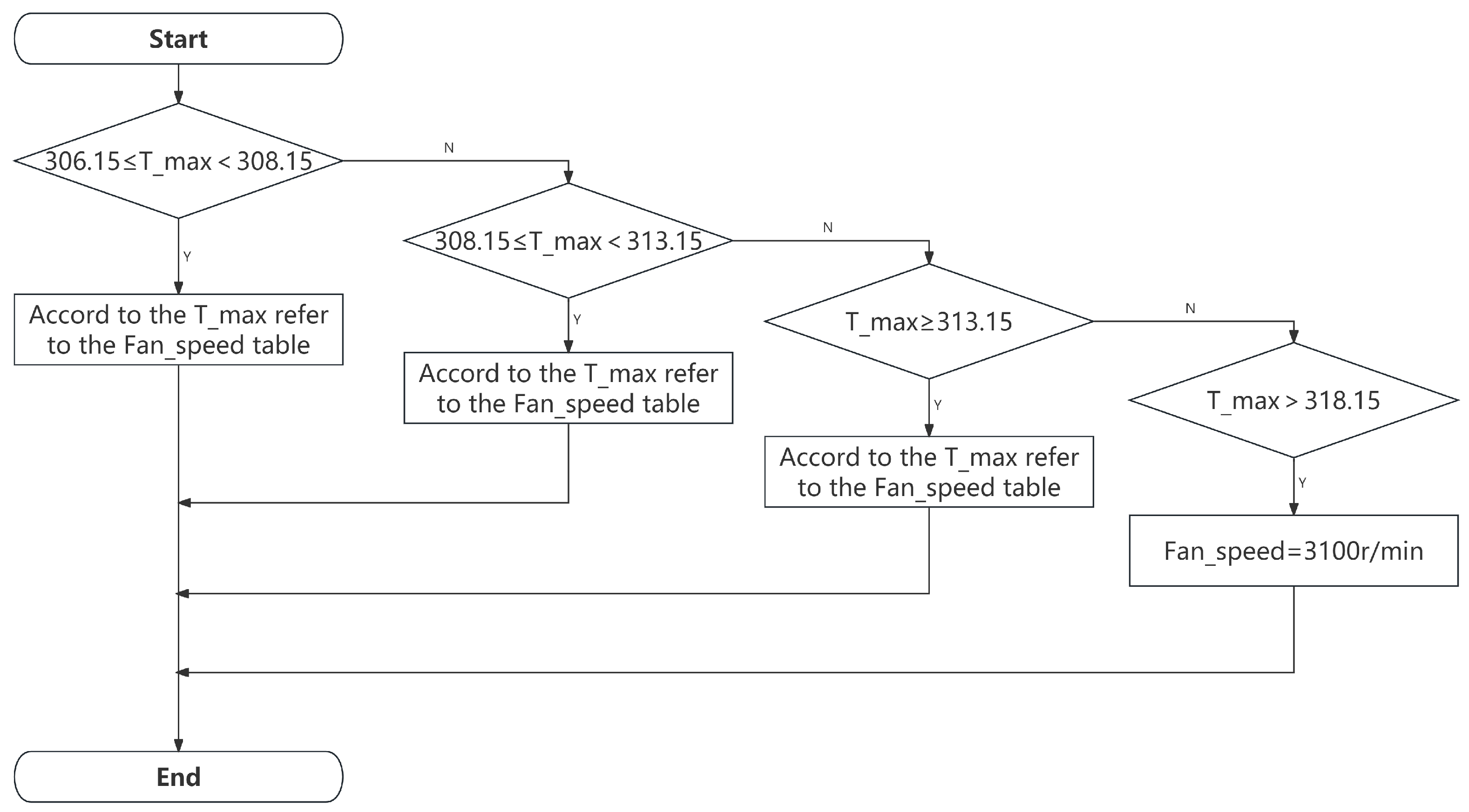
3.3. Analysis of Battery Heat Transfer Performance with Varying Fan Speeds
4. Overall Optimization Experiment Analysis
5. Conclusions
- Comprehensive Heat Transfer Model Establishment: This study has established, for the first time, a heat transfer model for lithium-ion battery packs that encompasses radiation, conduction, and convection. Through theoretical analysis and simulation calculations, this model provides a solid theoretical foundation for subsequent simulation experiments and practical applications. However, due to the assumptions made in the modeling of the heat source, there is an error between the simulation results and the experimental results. In order to reduce the error, the model can be considered to be optimized in two aspects: the internal resistance and the dynamic simulation of the heat source. By collecting experimental data under different conditions, an internal resistance model that changes dynamically with temperature and state of charge (SOC) is introduced. Meanwhile, the heat source should be a variable that changes with internal resistance and current; the battery model is calibrated and optimized using machine learning techniques. Machine learning methods are used to analyze large amounts of experimental data; discover complex nonlinear relationships between internal resistance, temperature, and SOC; and optimize heat source modeling. Predictive models are established to dynamically adjust the simulation parameters to make them closer to the experimental conditions.
- Diversified optimization measures for cooling performance: The study improved the cooling performance of the system in all aspects by adding heat dissipation ribs, applying heat dissipation coatings and adjusting the fan speed. When the thickness of heat dissipation ribs is 4 mm, the total heat dissipation rate efficiency reaches 86.2%. Compared with the battery pack without any optimization, the maximum temperature is reduced by 1.98 K, and the maximum temperature difference is reduced by 1.94 K. When the thickness of heat dissipation coating is 260 m, the radiative rate is 0.92. Compared with the battery pack without any optimization, the maximum temperature is reduced by 1.4 K. The higher the fan rotation speed is, the better the heat dissipation effect is. The combined application of these optimization measures significantly reduces the operating temperature of the lithium battery, extends the cycle life of the battery, and improves the stability of the system.
- Development of a temperature management system based on the MIMO control strategy: In this study, a temperature management system based on the MIMO control strategy is developed to achieve a dynamic balance of temperature and optimization of energy consumption. The application of this system not only improves the heat dissipation efficiency and ensures that the lithium-ion battery operates within the safe temperature limit of 318.15 K, thus extending the battery’s cycle life and enhancing its stability, but also significantly reduces the risk of thermal runaway. The problem of excessive temperature difference between different battery modules is solved and the output capacity of the system is improved.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kumar, R.; Goel, V. A study on thermal management system of lithium-ion batteries for electrical vehicles: A critical review. J. Energy Storage 2023, 71, 108025. [Google Scholar] [CrossRef]
- Zhou, S.; Song, Z.; Zhao, Y. Analysis of the thermal effect of a lithium iron phosphate battery cell and module. Energy Sci. Eng. 2021, 9, 661–675. [Google Scholar] [CrossRef]
- Akbarzadeh, M.; Kalogiannis, T.; Jaguemont, J.; Jin, L.; Behi, H.; Karimi, D.; Beheshti, H.; Mierlo, J.V.; Berecibar, M. A comparative study between air cooling and liquid cooling thermal management systems for a high-energy lithium-ion battery module. Appl. Therm. Eng. 2021, 198, 117503. [Google Scholar] [CrossRef]
- Tuğan, V.; Yardımcı, U. Numerical study for battery thermal management system improvement with air channel in electric vehicles. J. Energy Storage 2023, 72, 108515. [Google Scholar] [CrossRef]
- Xu, Z.; Yu, G.; Zhang, T.; Wang, R. Cooling performance of battery pack as affected by inlet position and inlet air velocity in electric vehicle. Case Stud. Therm. Eng. 2022, 39, 102382. [Google Scholar] [CrossRef]
- Yang, W.; Wang, Y.; Guo, F.; Bai, Y.; Liu, X. Optimization study of air-cooled stagger-arranged battery pack with reverse-layered airflow. J. Energy Storage 2022, 55, 105524. [Google Scholar] [CrossRef]
- Li, H.; Xiao, X.; Wang, Y.; Lian, C.; Li, Q.; Wang, Z. Performance investigation of a battery thermal management system with microencapsulated phase change material suspension. Appl. Therm. Eng. 2020, 180, 115795. [Google Scholar] [CrossRef]
- Basu, S.; Hariharan, K.S.; Kolake, S.M.; Song, T.; Sohn, D.K.; Yeo, T. Coupled electrochemical thermal modelling of a novel Li-ion battery pack thermal management system. Appl. Energy 2016, 181, 1–13. [Google Scholar] [CrossRef]
- Deng, T.; Zhang, G.; Ran, Y. Study on thermal management of rectangular Li-ion battery with serpentine-channel cold plate. Int. J. Heat Mass Transf. 2018, 125, 143–152. [Google Scholar] [CrossRef]
- E, J.; Han, D.; Qiu, A.; Zhu, H.; Deng, Y.; Chen, J.; Zhao, X.; Zuo, W.; Wang, H.; Chen, J.; et al. Orthogonal experimental design of liquid-cooling structure on the cooling effect of a liquid-cooled battery thermal management system. Appl. Therm. Eng. 2018, 132, 508–520. [Google Scholar]
- Wu, C.; Wang, Z.; Bao, Y.; Zhao, J.; Rao, Z. Investigation on the performance enhancement of baffled cold plate based battery thermal management system. J. Energy Storage 2021, 41, 102882. [Google Scholar] [CrossRef]
- He, P.; Lu, H.; Fan, Y.; Ruan, H.; Wang, C.; Zhu, Y. Numerical investigation on a lithium-ion battery thermal management system utilizing a double-layered I-shaped channel liquid cooling plate exchanger. Int. J. Therm. Sci. 2023, 187, 108200. [Google Scholar] [CrossRef]
- Zhao, D.; An, C.; Lei, Z. Liquid cooling plate with drop-shaped deflectors based on Coanda Effect-For Li-ion battery thermal management. J. Energy Storage 2023, 70, 108014. [Google Scholar] [CrossRef]
- Yang, H.; Wang, Z.; Li, M.; Ren, F.; Feng, Y. A manifold channel liquid cooling system with low-cost and high temperature uniformity for lithium-ion battery pack thermal management. Therm. Sci. Eng. Prog. 2023, 41, 101857. [Google Scholar] [CrossRef]
- Zeng, W.; Ma, C.; Hu, S.; Li, S.; Zhang, Y. The performance investigation and optimization of reciprocating flow applied for liquid-cooling-based battery thermal management system. Energy Convers. Manag. 2023, 292, 117378. [Google Scholar] [CrossRef]
- Xu, S.; Su, Y.; Wang, W. Numerical Simulation Study on the Thermal Characteristics of Lithium-ion Battery Packs in Electric Vehicles. Sci. Technol. Eng. 2018, 18, 55–61. [Google Scholar]
- Liu, X.; Zou, T.; Hou, H.; Xu, Z.; Wang, X. Numerical Study on the Air-cooled Temperature Field of Lithium-ion Batteries and Modules Based on Fluent. Softw. Guide 2020, 19, 5–10. [Google Scholar]
- Li, M.; Zang, M.; Li, C.; Dai, H. Optimization of the Air-cooled Heat Dissipation Structure of Lithium-ion Battery Packs. Battery 2020, 50, 266–270. [Google Scholar]
- Yang, K.; Pei, H.; Zhu, X.; Zou, Y.; Wang, J.; Shi, H. Thermal Design Research and Optimization of a Specific Type of Containerized Energy Storage Battery Module. Energy Storage Sci. Technol. 2020, 9, 1858. [Google Scholar]
- Huang, X.; Dai, H.; Hu, L.; Zhu, J.; Huang, X.; Yang, Z.; Li, C. Thermal Simulation of Power Battery Packs Based on COMSOL Multiphysics. Automot. Appl. Technol. 2022, 47, 1–9. [Google Scholar]
- Mahamud, R.; Park, C. Reciprocating air flow for Li-ion battery thermal management to improve temperature uniformity. J. Power Sources 2011, 196, 5685–5696. [Google Scholar] [CrossRef]
- Fan, L.; Khodadadi, J.M.; Pesaran, A.A. A parametric study on thermal management of an air-cooled lithium-ion battery module for plug-in hybrid electric vehicles. J. Power Sources 2013, 238, 301–312. [Google Scholar] [CrossRef]
- Park, H. A design of air flow configuration for cooling lithium ion battery in hybrid electric vehicles. J. Power Sources 2013, 239, 30–36. [Google Scholar] [CrossRef]
- Yang, T.; Yang, N.; Zhang, X.; Li, G. Investigation of the thermal performance of axial-flow air cooling for the lithium-ion battery pack. Int. J. Therm. Sci. 2016, 108, 132–144. [Google Scholar] [CrossRef]
- Lv, C.; Zhang, S.; Zhu, S.; Song, Y.; Ge, Y. Thermal simulation analysis and optimization of forced air cooling system for energy storage lithium-ion battery pack. Power Syst. Prot. Control 2021, 49, 48–55. [Google Scholar]
- Liu, Z.; Wang, Y.; Zhang, J.; Liu, Z. Shortcut computation for the thermal management of a large air-cooled battery pack. Appl. Therm. Eng. 2014, 66, 445–452. [Google Scholar] [CrossRef]
- Chen, S.C.; Wan, C.C.; Wang, Y.Y. Thermal analysis of lithium-ion batteries. J. Power Sources 2005, 140, 111–124. [Google Scholar] [CrossRef]
- Mevawalla, A.; Shabeer, Y.; Tran, M.K.; Panchal, S.; Fowler, M.; Fraser, R. Thermal modelling utilizing multiple experimentally measurable parameters. Batteries 2022, 8, 147. [Google Scholar] [CrossRef]
- Xie, Y.; Li, W.; Hu, X.; Tran, M.-K.; Panchal, S.; Fowler, M.; Liu, K. Coestimation of SOC and three-dimensional SOT for lithium-ion batteries based on distributed spatial–temporal online correction. IEEE Trans. Ind. Electron. 2022, 70, 5937–5948. [Google Scholar] [CrossRef]
- Geng, D.; Hu, Y. Structural design of CPU cooler. J. Shenyang Univ. Technol. 2011, 30, 82–85. [Google Scholar]
- Lee, J.; Rew, K.; Lee, C.; Kim, D.; Kim, M. A study on heatsink fin wave optimization for climate control seat. J. Mech. Sci. Technol. 2017, 31, 1489–1495. [Google Scholar] [CrossRef]
- Yin, H.; Yin, Z. Numerical calculation of the effect of thermal conductivity of anticorrosive coatings on the heat dissipation capacity of chip radiators. Sci. Technol. Eng. 2020, 20, 5142–5148. [Google Scholar]
- Serrano, D.; Sánchez-Delgado, S.; Pérez-Álvarez, R.; Acosta-Iborra, A. Experimental determination of the convection heat transfer coefficient in an eccentric annular duct. Exp. Therm. Fluid Sci. 2022, 136, 110664. [Google Scholar] [CrossRef]



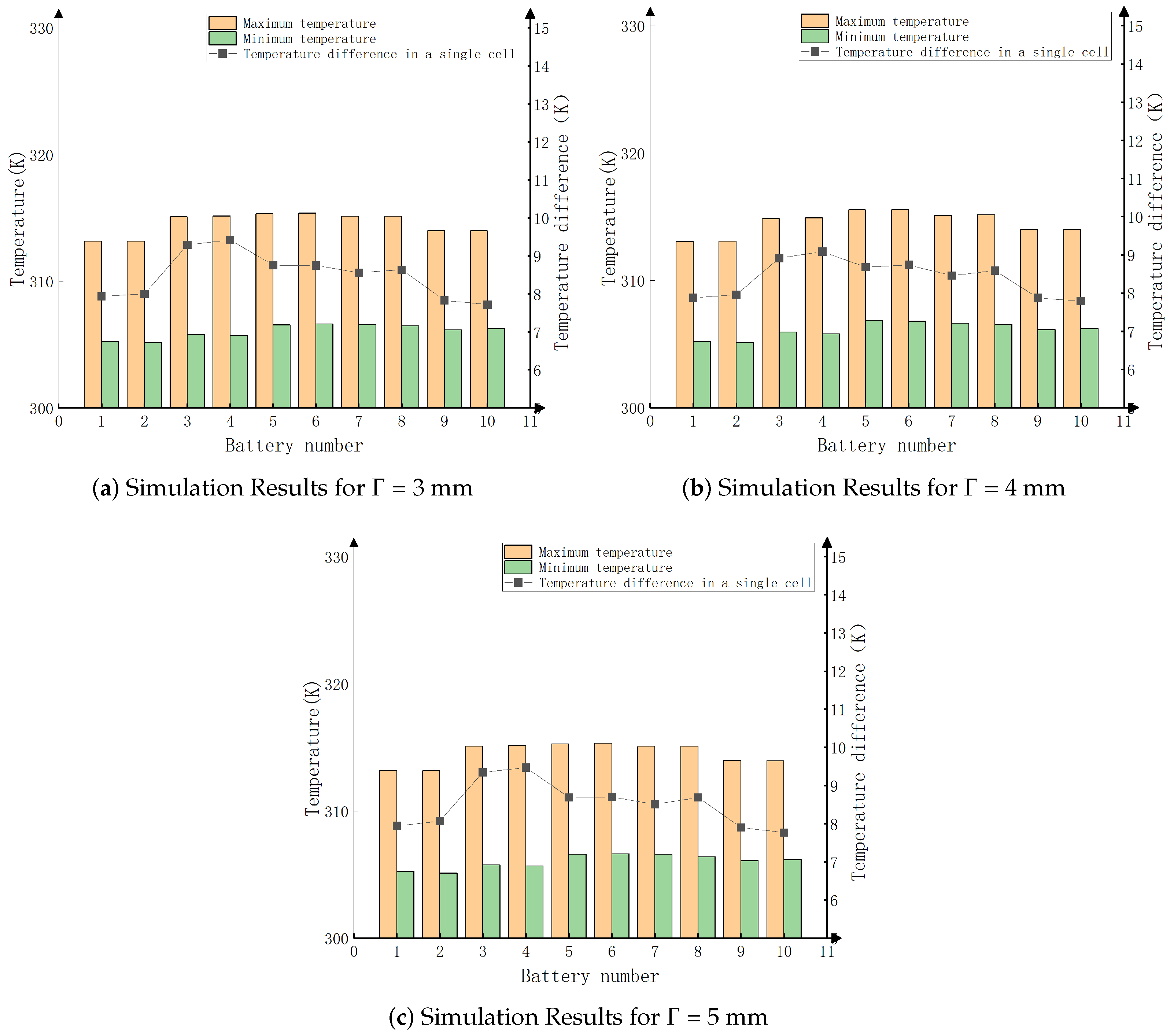

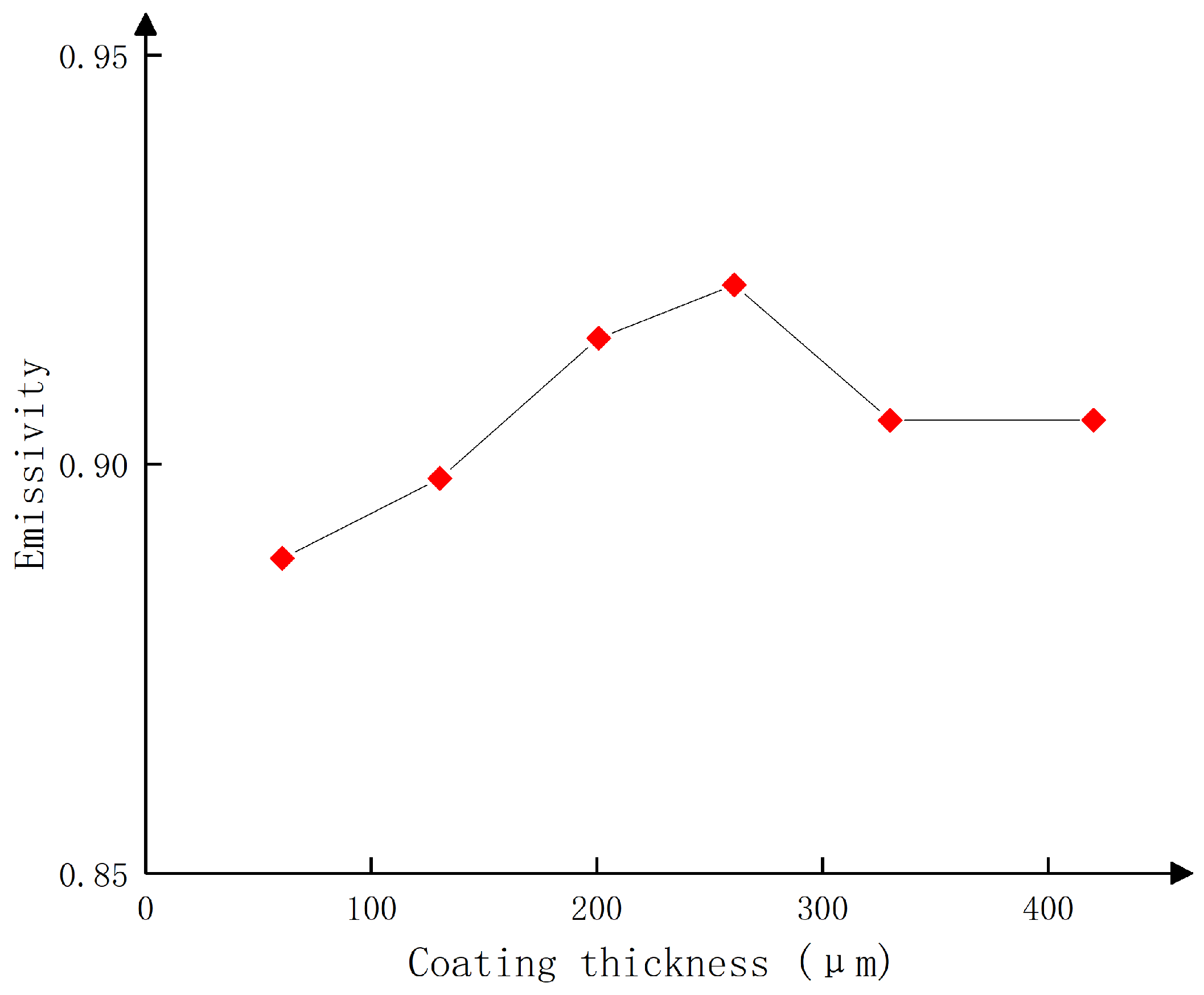
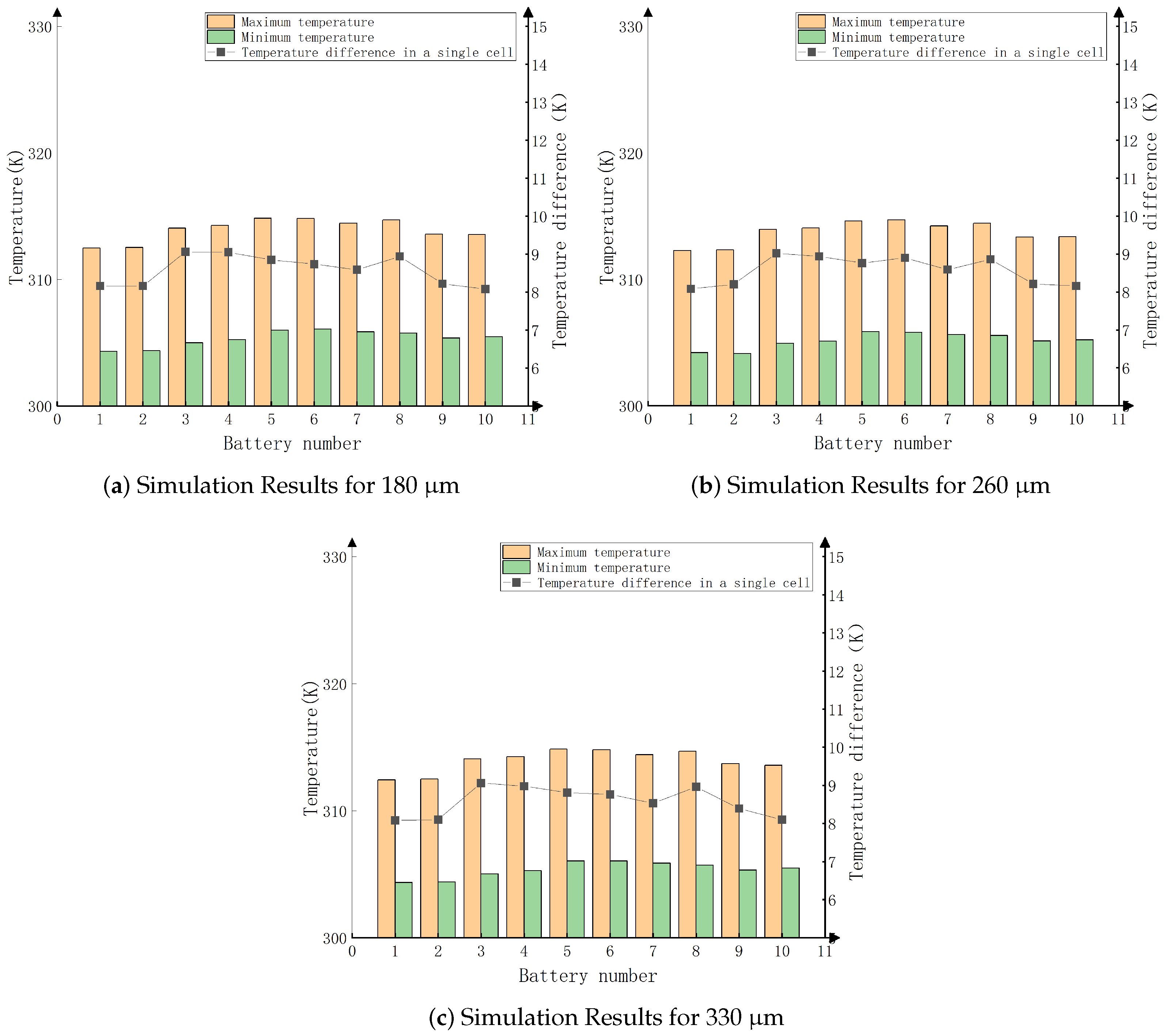



| Parameter | Numerical Value |
|---|---|
| Length × Width × Height (mm) | 135 × 30 × 180 |
| Nominal capacity (Ah) | 50 |
| Nominal voltage (V) | 3.2 |
| End-of-charge voltage (V) | 3.65 |
| Cutoff voltage during discharge (V) | 2.5 |
| Maximum charge and discharge C-rate (C) | 2 |
| Operating temperature under charging conditions (K) | 273.15∼318.15 |
| Operating temperature under discharge conditions (K) | 253.15∼328.15 |
| Temperature/(K) | Fan Speed (r/min) | Duty Cycle (%) |
|---|---|---|
| 306.15 | 792 | 10 |
| 308.15 | 1120 | 17 |
| 313.15 | 2741 | 50 |
| 318.15 | 3100 | 67 |
| Operating Condition | Ambient Temperature/(K) | Battery Pack | Fan Speed (r/min) | Maximum Temperature/(K) | Minimum Temperature/(K) | Temperature Difference/(K) |
|---|---|---|---|---|---|---|
| 1 | 293.15 | A | 3100 | 305.61 | 298.12 | 7.49 |
| 2 | 296.15 | B | 3100 | 309.55 | 300.99 | 8.56 |
| 3 | 298.15 | C | 3100 | 312.87 | 302.23 | 10.64 |
| 4 | 293.15 | A | ※ | 310.64 | 306.66 | 3.98 |
| 5 | 296.15 | B | 312.17 | 308.02 | 4.15 | |
| 6 | 298.15 | C | 313.97 | 309.23 | 4.74 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Q.; Yan, L.; Yang, L.; Wang, J. Research on the Design of a MIMO Management System for Lithium-Ion Batteries Based on Radiation–Conductivity–Convection Coupled Thermal Analysis. Energies 2024, 17, 3504. https://doi.org/10.3390/en17143504
Wang Q, Yan L, Yang L, Wang J. Research on the Design of a MIMO Management System for Lithium-Ion Batteries Based on Radiation–Conductivity–Convection Coupled Thermal Analysis. Energies. 2024; 17(14):3504. https://doi.org/10.3390/en17143504
Chicago/Turabian StyleWang, Qian, Linbin Yan, Lushi Yang, and Jianxiao Wang. 2024. "Research on the Design of a MIMO Management System for Lithium-Ion Batteries Based on Radiation–Conductivity–Convection Coupled Thermal Analysis" Energies 17, no. 14: 3504. https://doi.org/10.3390/en17143504
APA StyleWang, Q., Yan, L., Yang, L., & Wang, J. (2024). Research on the Design of a MIMO Management System for Lithium-Ion Batteries Based on Radiation–Conductivity–Convection Coupled Thermal Analysis. Energies, 17(14), 3504. https://doi.org/10.3390/en17143504





