Novel Fault Protection Method for Flexible DC Power Systems
Abstract
1. Introduction
2. Fault Analysis of Flexible DC Power System
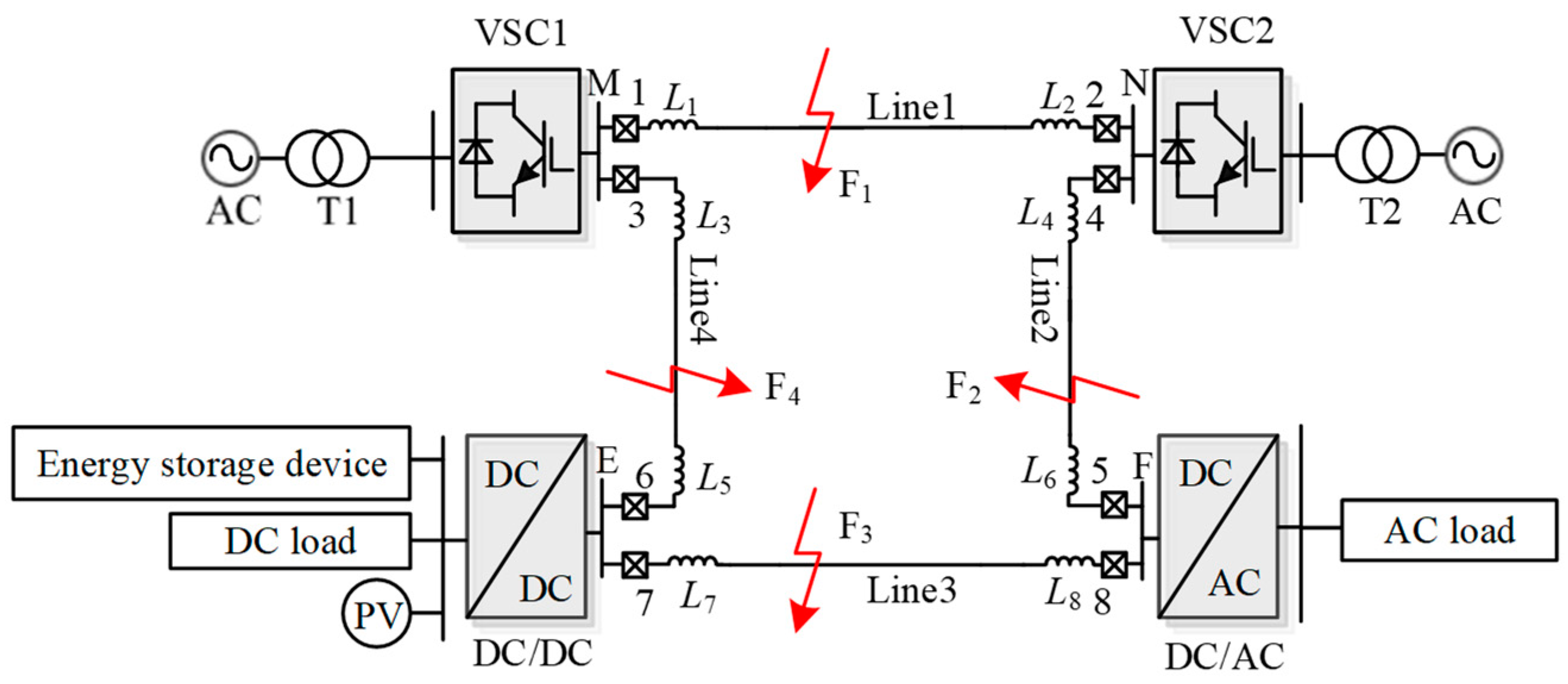
2.1. Topology of Flexible DC Power System
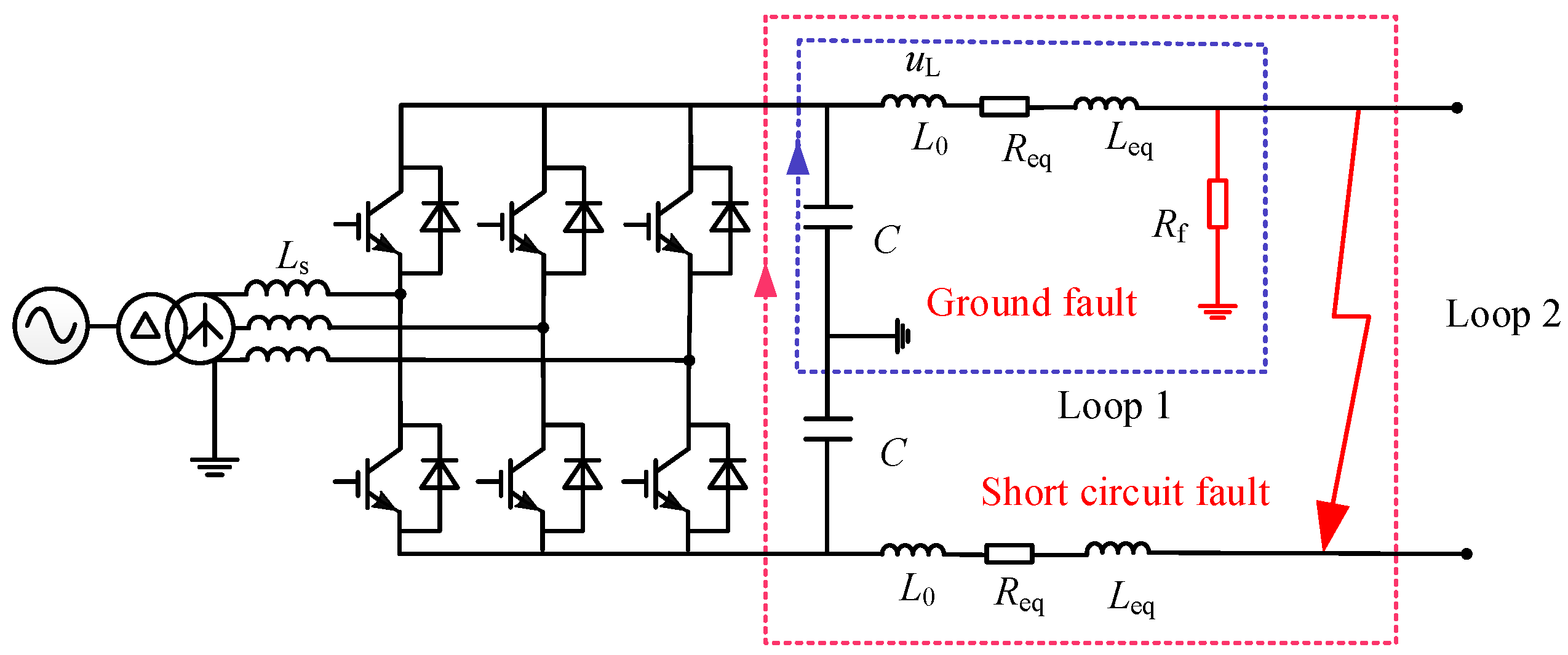
2.2. Fault Analysis
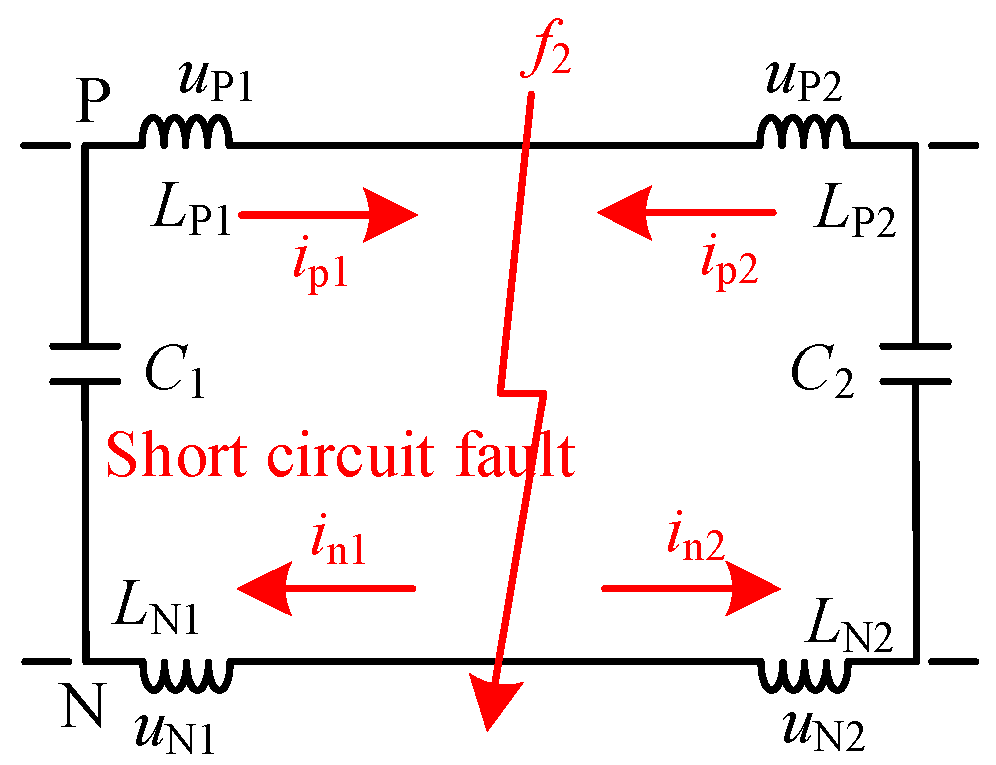
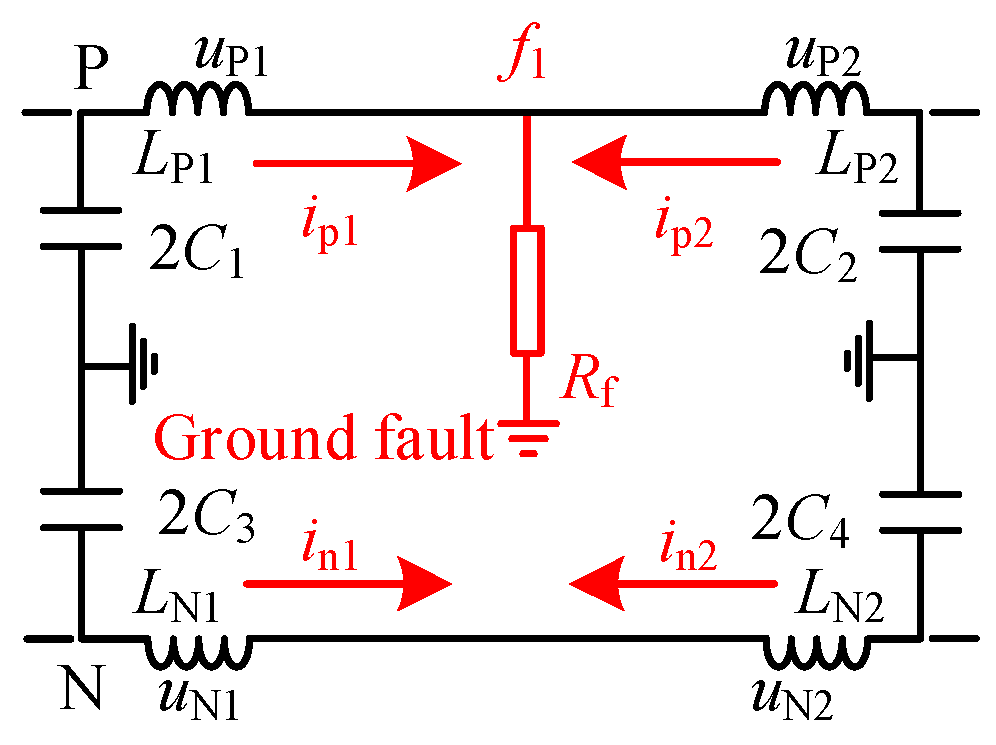
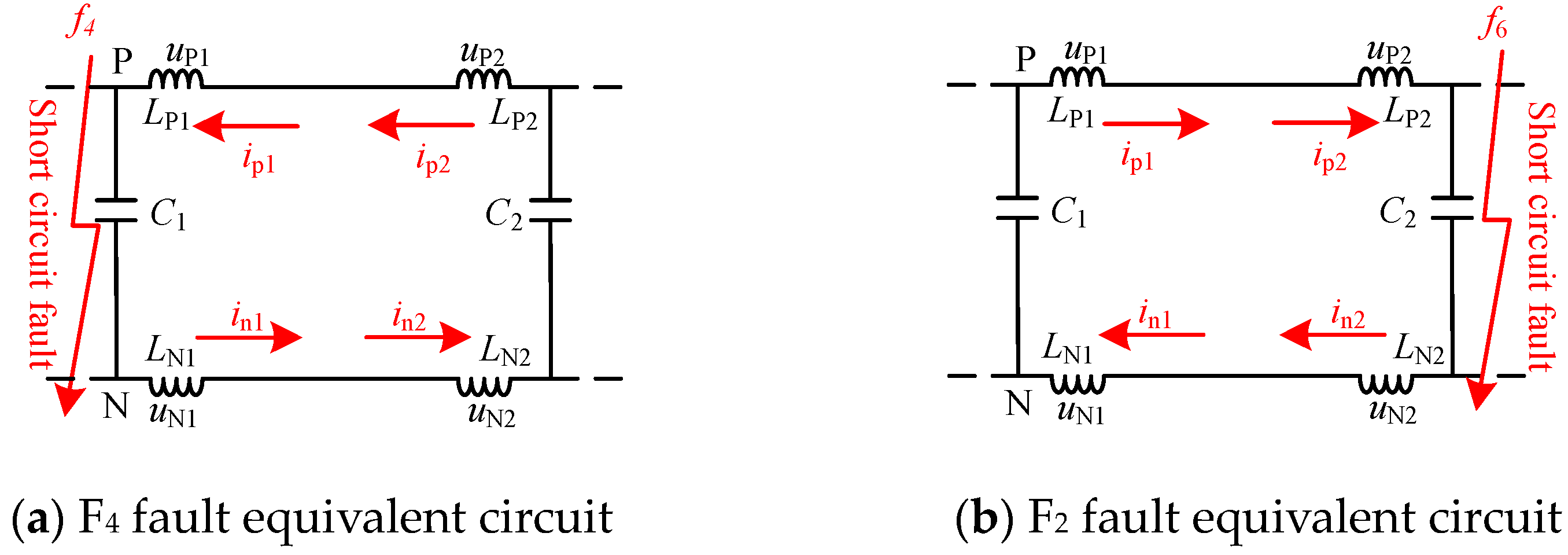
2.2.1. Internal Fault Analysis
2.2.2. External Fault Analysis
3. Principle of Breakage Protection
3.1. Principle of Cosine Similarity
3.2. Fault Detection Criterion
3.3. Broken Pole Selection and Fault Identification
3.4. Breakage Protection Flow Chart
4. Simulation Results
4.1. Simulation Results of Internal Fault
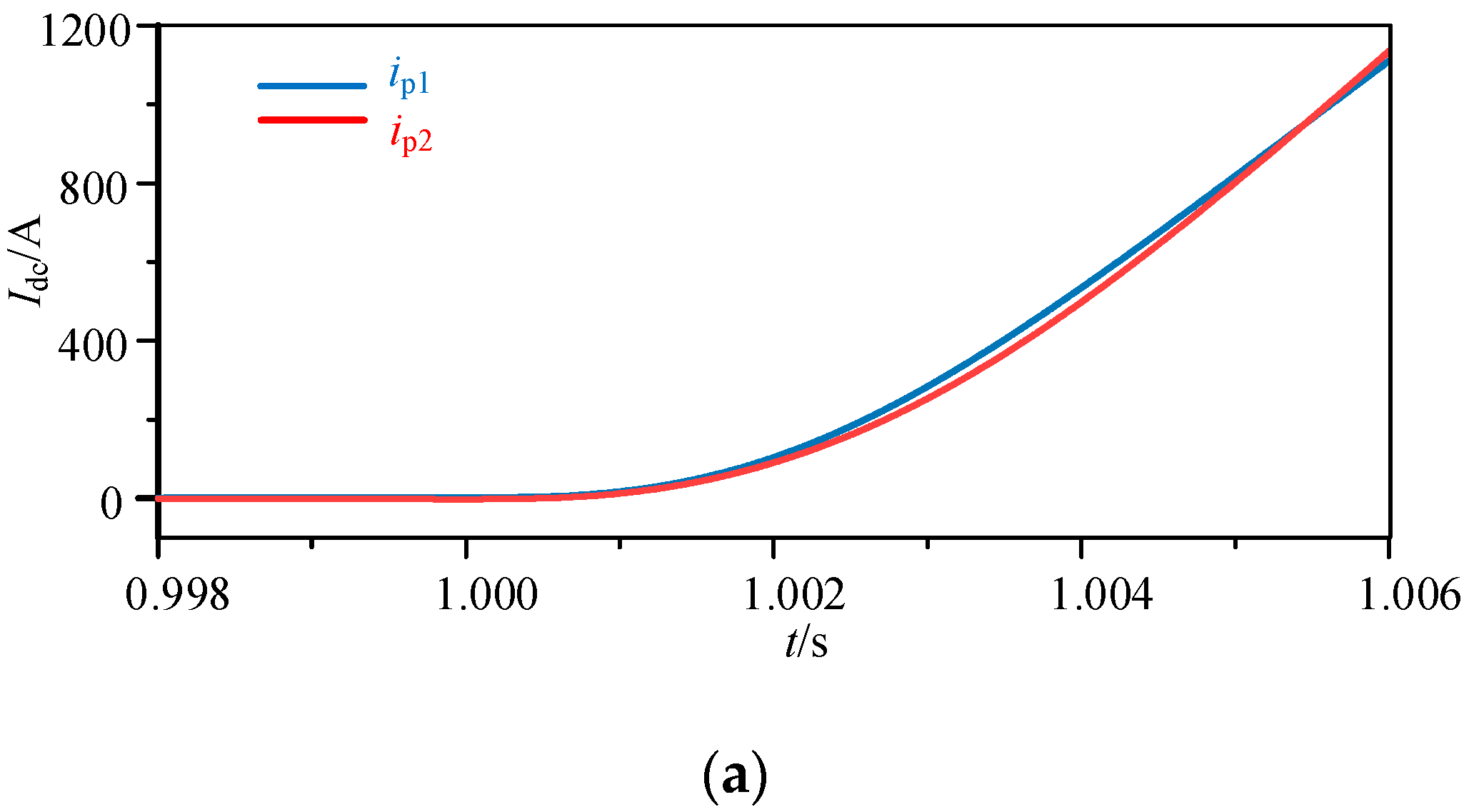
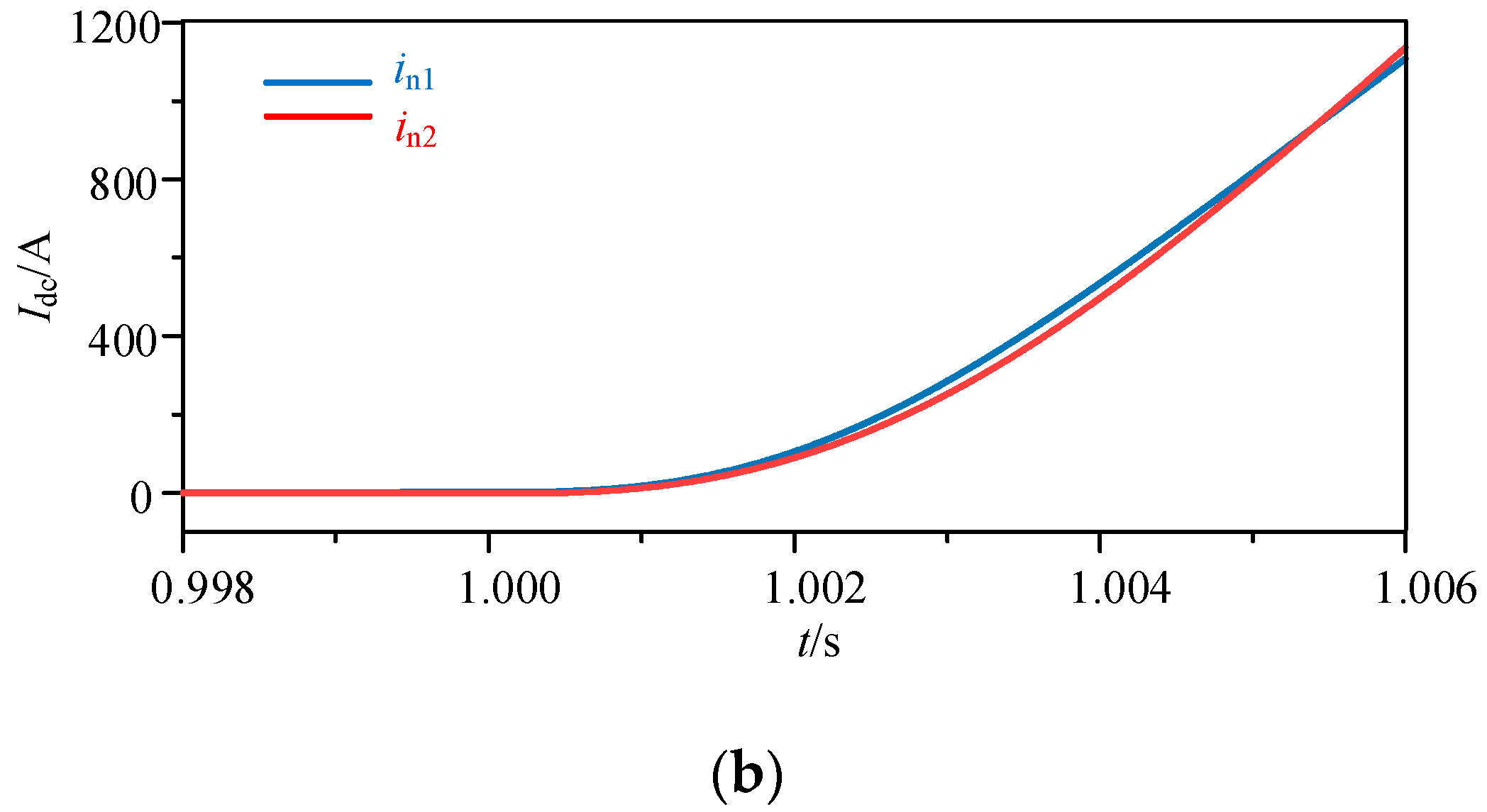
4.1.1. Internal Line-to-Line Short-Circuit Fault
4.1.2. Internal Single Line-to-Ground Fault
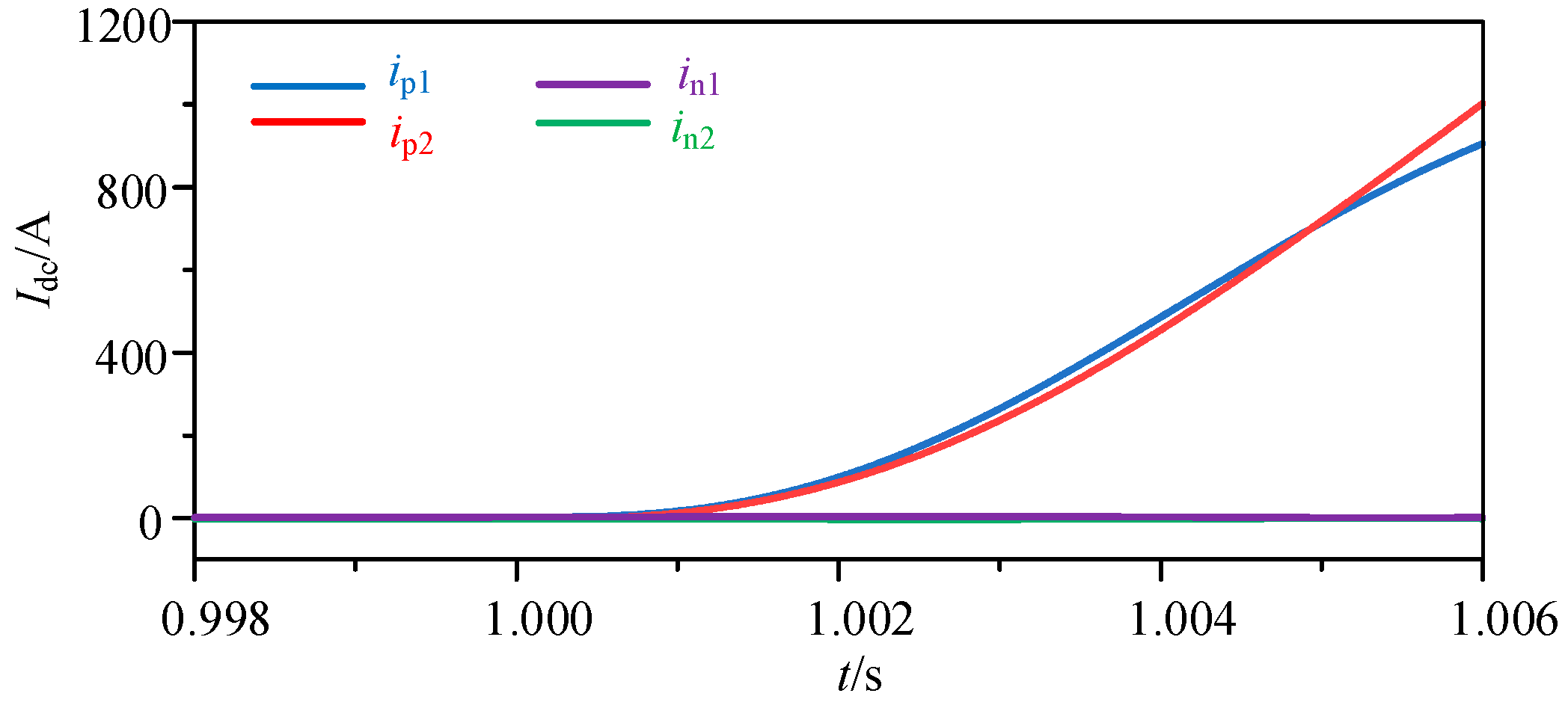
4.2. Simulation Results of External Fault
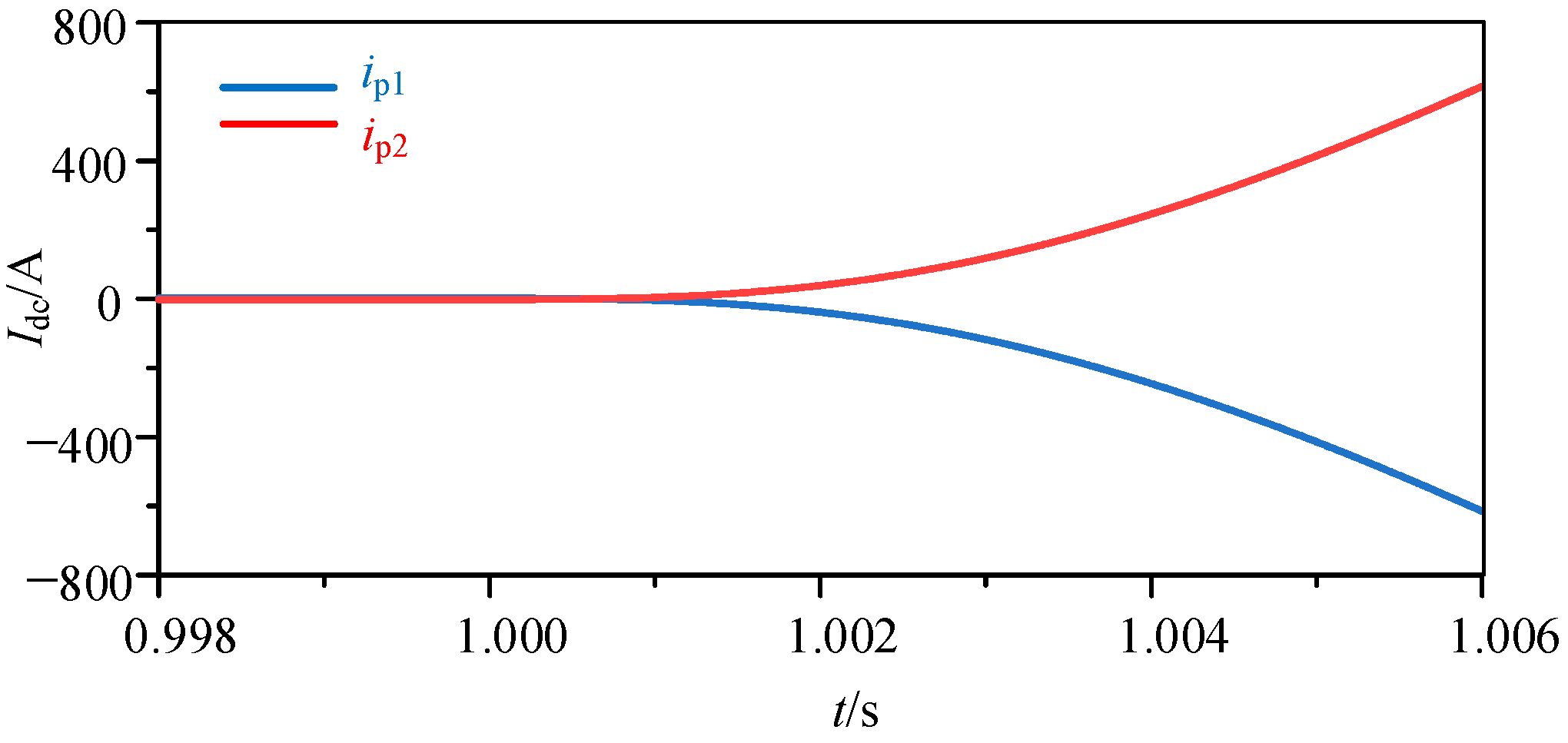
4.3. Influences of Fault Resistance
4.4. Influences of Communication Delays
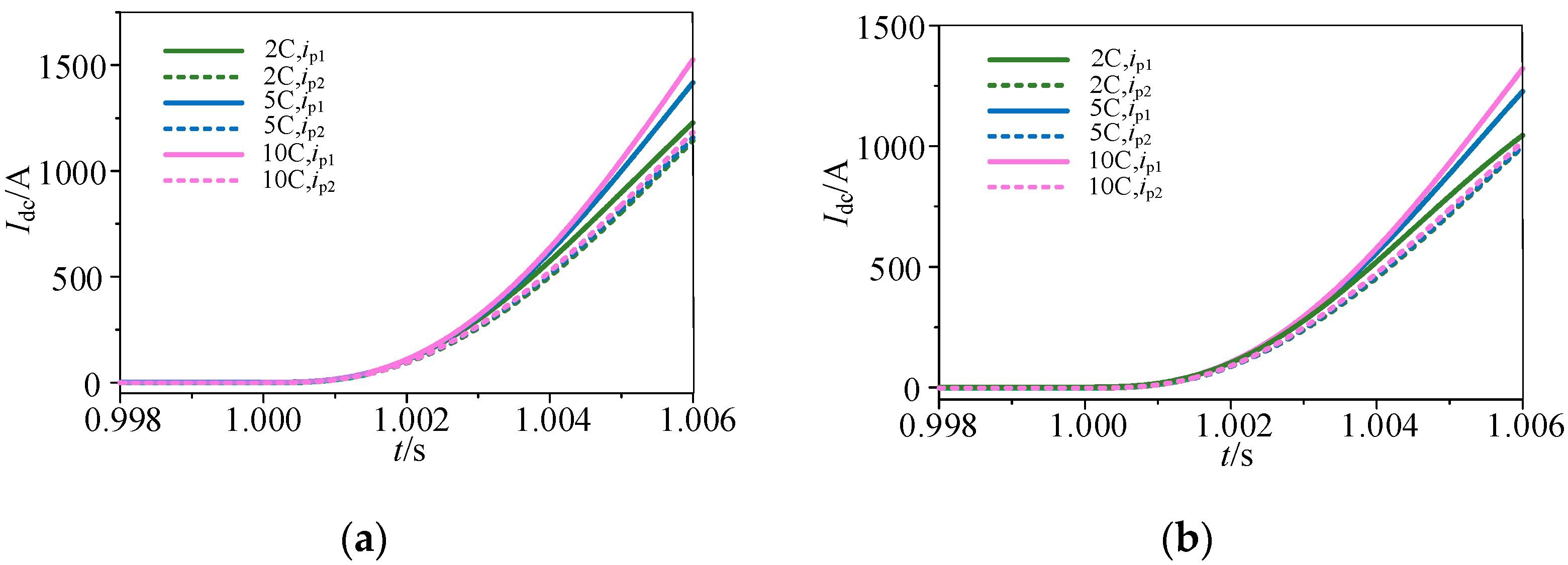
4.5. Influences of Distributed Capacitance
4.6. Influences of Noise
4.7. Comparison of Protection Schemes
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, Y.; Yang, L.; Wu, Q.; Chen, S.; Yu, Z.; Guan, Y.; Zhang, Y. A hybrid isolated bidirectional DC/DC solid-state transformer for DC distribution network. IEEE Access 2021, 9, 159059–159070. [Google Scholar] [CrossRef]
- Liu, Y.; Guan, L.; Chen, J.; Shi, G.; Zheng, J.; Wei, T.; Guo, F. Over-current mechanism and suppression strategy of DC transformer in multiterminal interconnected flexible DC distribution network. IEEE Syst. J. 2023, 17, 1785–1796. [Google Scholar] [CrossRef]
- Zheng, T.; Lv, W.; Wu, Q.; Li, R.; Liu, X.; Zhang, C.; Xu, L. An integrated control and protection scheme based on FBSM-MMC active current limiting strategy for DC distribution network. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 9, 2632–2642. [Google Scholar] [CrossRef]
- Shuai, Z.; He, D.; Xiong, Z.; Lei, Z.; John Shen, Z. Comparative study of short-circuit fault characteristics for VSC-based DC distribution networks with different distributed generators. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 528–540. [Google Scholar] [CrossRef]
- Li, M.; Jia, K.; Bi, T.; Zhu, R.; Wang, C.; Yang, Q. Full-current-based directional pilot protection for VSC-DC distribution systems. IET Generation. Transm. Distrib. 2019, 13, 3713–3724. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, S.; Wang, Z.; Zhu, L.; Guan, Y.; Zhang, G.; Yang, L.; Zhang, Y. A Multiple modular isolated DC/DC converter with bidirectional fault handling and efficient energy conversion for DC distribution network. IEEE Trans. Power Electron. 2020, 35, 11502–11517. [Google Scholar] [CrossRef]
- Dambhare, S.; Soma, S.A.; Chandorkar, M.C. Adaptive current differential protection schemes for transmission-line protection. IEEE Trans. Power Deliv. 2009, 24, 1832–1841. [Google Scholar] [CrossRef]
- Sneath, J.; Rajapakse, A.D. Fault detection and interruption in an earthed HVDC grid using ROCOV and hybrid DC breakers. IEEE Trans. Power Deliv. 2014, 31, 973–981. [Google Scholar] [CrossRef]
- Zhang, C.; Song, G.; Dong, X. A novel traveling wave protection method for DC transmission lines using current fitting. IEEE Trans. Power Deliv. 2019, 35, 2980–2991. [Google Scholar] [CrossRef]
- Meghwani, A.; Srivastava, S.C.; Chakrabarti, S. A non-unit protection scheme for DC microgrid based on local measurements. IEEE Trans. Power Deliv. 2016, 32, 172–181. [Google Scholar] [CrossRef]
- Zhou, J.; Zhao, C.; Li, C.; Xu, J.; An, T. Boundary protection scheme of multi-terminal flexible DC power grid based on DC reactor voltage. Autom. Electr. Power Syst. 2017, 41, 89–94. [Google Scholar]
- Zheng, T.; Wu, Q.; Lü, W.; Wang, X.; Cao, H. Research on protection scheme of flexible DC distribution grids based on DC current zero-crossing characteristics. Power Syst. Technol. 2020, 44, 1806–1815. [Google Scholar]
- Li, M.; Jia, K.; Zhang, Q.; Liu, Y.; Bi, T. Directional pilot protection for flexible DC distribution network based on directional characteristics of instantaneous current. Autom. Electr. Power Syst. 2019, 43, 116–122. [Google Scholar]
- Zhang, S.; Zou, G.; Huang, Q.; Xu, B.; Li, J. Single-ended line protection for MMC-MTDC grids. IET Gener. Transm. Distrib. 2019, 13, 4331–4338. [Google Scholar] [CrossRef]
- Jia, K.; Wang, C.; Bi, T.; Feng, T.; Zhu, R. Transient current correlation based protection for DC distribution system. IEEE Trans. Ind. Electron. 2019, 67, 9927–9936. [Google Scholar] [CrossRef]
- Li, R.; Xu, L.; Yao, L. DC fault detection and location in meshed multiterminal HVDC systems based on DC reactor voltage change rate. IEEE Trans. Power Deliv. 2016, 32, 1516–1526. [Google Scholar] [CrossRef]
- Li, S.; Chen, W.; Yin, X.; Chen, D.; Teng, Y. A Novel integrated protection for VSC-HVDC transmission line based on current limiting reactor power. IEEE Trans. Power Deliv. 2020, 35, 226–233. [Google Scholar] [CrossRef]
- Bi, T.; Yang, B.; Jia, K.; Zheng, L.; Liu, Q.; Yang, Q. Review on renewable energy source fault characteristics analysis. CSEE J. Power Energy Syst. 2022, 8, 963–972. [Google Scholar]
- Li, Z.; Duan, J.; Lu, W.; Du, X.; Yang, W.; Tu, S. Non-permanent Pole-to-pole fault restoration strategy for flexible DC distribution network. J. Mod. Power Syst. Clean Energy 2021, 9, 1339–1351. [Google Scholar] [CrossRef]
- Fei, K.; Song, Z.; Zhiguo, H.; Baohui, Z. Development of a novel protection device for bipolar HVDC transmission Lines. IEEE Trans. Power Deliv. 2014, 29, 2270–2278. [Google Scholar]
- Li, B.; Liao, K.; Zhu, Y.; He, Z. Single-terminal protection of DC distribution network based on current-limiting reactor voltage. High Volt. Technol. 2023, 2, 1–23. [Google Scholar]



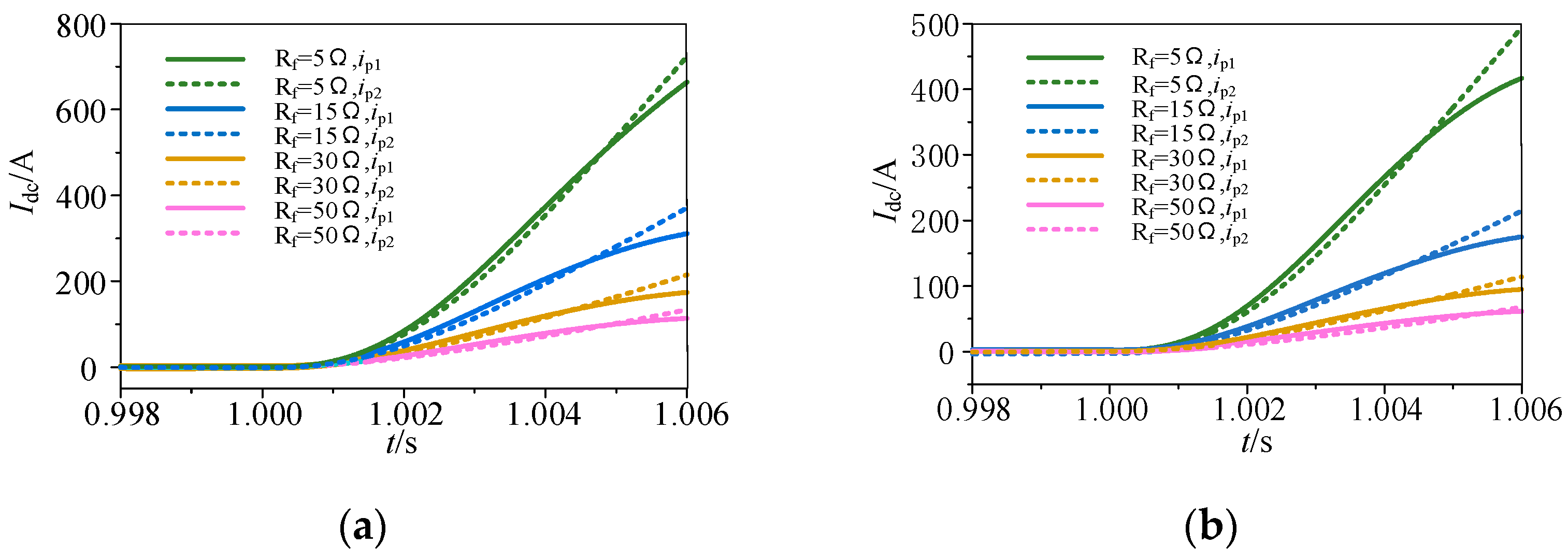
| Simulation Parameters | Simulation Values |
|---|---|
| DC bus voltage | ±10 kV |
| DC line resistance per unit length | 0.0139 Ω/km |
| DC line inductance per unit length | 0.159 mH/km |
| DC line length | 10 km |
| DC bus capacitor | 4500 μF |
| The Location of the Fault | Fault Resistance/Ω | Similarity |
|---|---|---|
| internal line-to-line short-circuit fault | 5 | 0.998 |
| 15 | 0.994 | |
| 30 | 0.982 | |
| 50 | 0.978 | |
| internal single line-to-ground fault | 5 | 0.999 |
| 15 | 0.997 | |
| 30 | 0.995 | |
| 50 | 0.989 |
| The Location of the Fault | Communication Delays/ms | Similarity |
|---|---|---|
| internal line-to-line short-circuit fault | 0.1 | 0.987 |
| 0.2 | 0.975 | |
| 0.3 | 0.953 | |
| internal single line-to-ground fault | 0.1 | 0.997 |
| 0.2 | 0.984 | |
| 0.3 | 0.972 |
| The Location of the Fault | Distributed Capacitance/μF | Similarity |
|---|---|---|
| internal line-to-line short-circuit fault | 9000 | 0.998 |
| 22,500 | 0.996 | |
| 45,000 | 0.993 | |
| internal single line-to-ground fault | 9000 | 0.999 |
| 22,500 | 0.998 | |
| 45,000 | 0.995 |
| The Location of the Fault | Signal to Noise Ratio/dB | Similarity |
|---|---|---|
| internal line-to-line short-circuit fault | 20 | 0.997 |
| 30 | 0.995 | |
| 40 | 0.993 | |
| internal single line-to-ground fault | 20 | 0.996 |
| 30 | 0.992 | |
| 40 | 0.991 |
| Protection Schemes | Effect of Transition Resistance | Effect of Noise (20 dB) | Applicable Fault Type | Other Determinations |
|---|---|---|---|---|
| Reference [11] | Affects the quickness | √ | line-to-line short-circuit fault | × |
| Reference [12] | Affects the quickness | √ | line-to-line short-circuit fault | × |
| This study | √ | √ | line-to-line short-circuit fault and single line-to-ground fault | × |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, G.; Duan, Q.; Zhao, C.; Wang, H.; Sha, G.; Gao, J.; Li, Y.; Zhou, S. Novel Fault Protection Method for Flexible DC Power Systems. Energies 2024, 17, 3446. https://doi.org/10.3390/en17143446
Chen G, Duan Q, Zhao C, Wang H, Sha G, Gao J, Li Y, Zhou S. Novel Fault Protection Method for Flexible DC Power Systems. Energies. 2024; 17(14):3446. https://doi.org/10.3390/en17143446
Chicago/Turabian StyleChen, Genqi, Qing Duan, Caihong Zhao, Haoqing Wang, Guanglin Sha, Jian Gao, Yong Li, and Shuiliang Zhou. 2024. "Novel Fault Protection Method for Flexible DC Power Systems" Energies 17, no. 14: 3446. https://doi.org/10.3390/en17143446
APA StyleChen, G., Duan, Q., Zhao, C., Wang, H., Sha, G., Gao, J., Li, Y., & Zhou, S. (2024). Novel Fault Protection Method for Flexible DC Power Systems. Energies, 17(14), 3446. https://doi.org/10.3390/en17143446






