Numerical Research on Leakage Characteristics of Pure Hydrogen/Hydrogen-Blended Natural Gas in Medium- and Low-Pressure Buried Pipelines
Abstract
1. Introduction
2. Methods
2.1. Physical Model
2.2. Mathematical Models
2.2.1. Governing Equations
2.2.2. Initial and Boundary Conditions
2.3. Simulation Scenarios
2.4. Mesh Independency and Model Validation
3. Results and Discussion
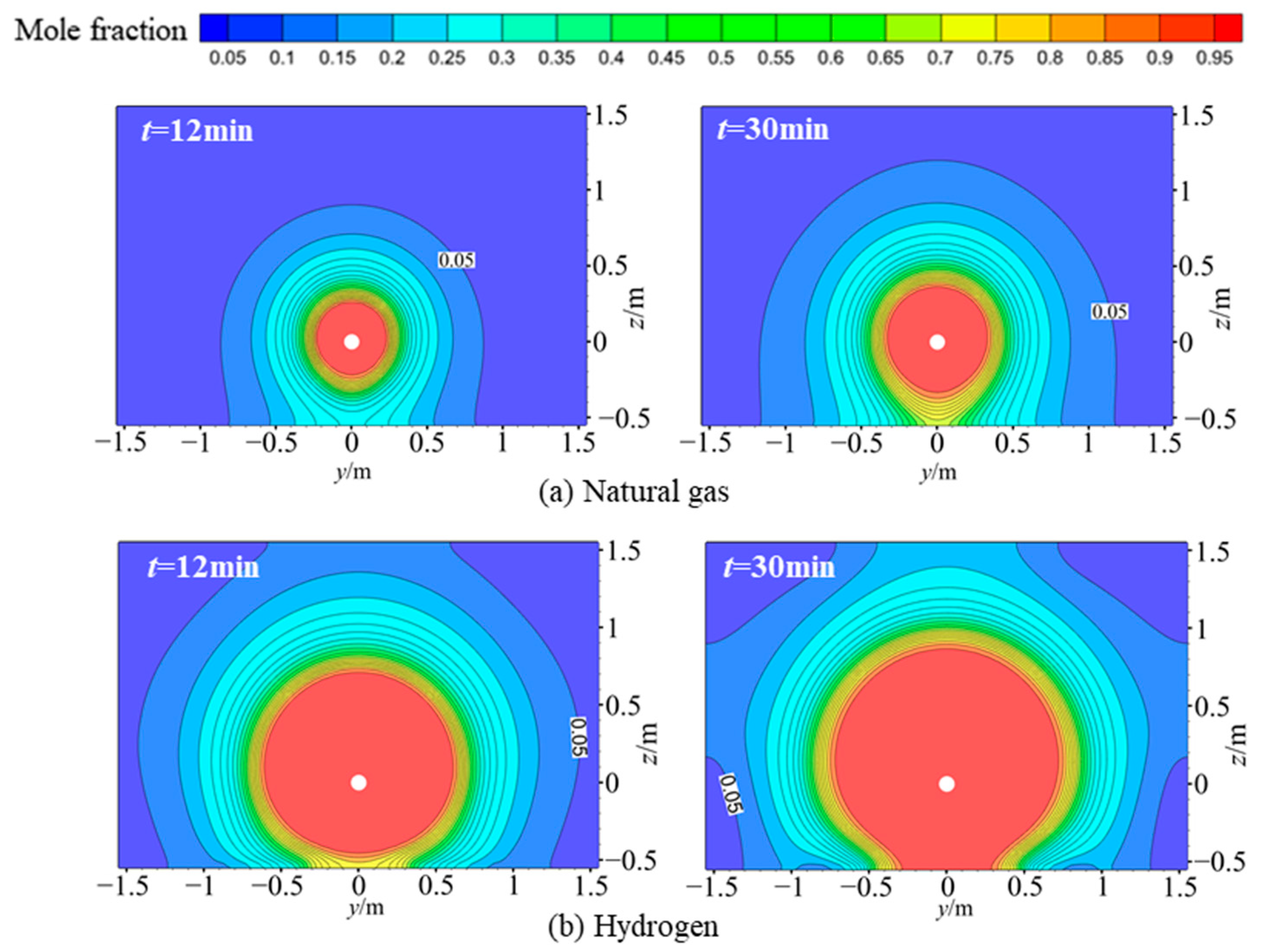
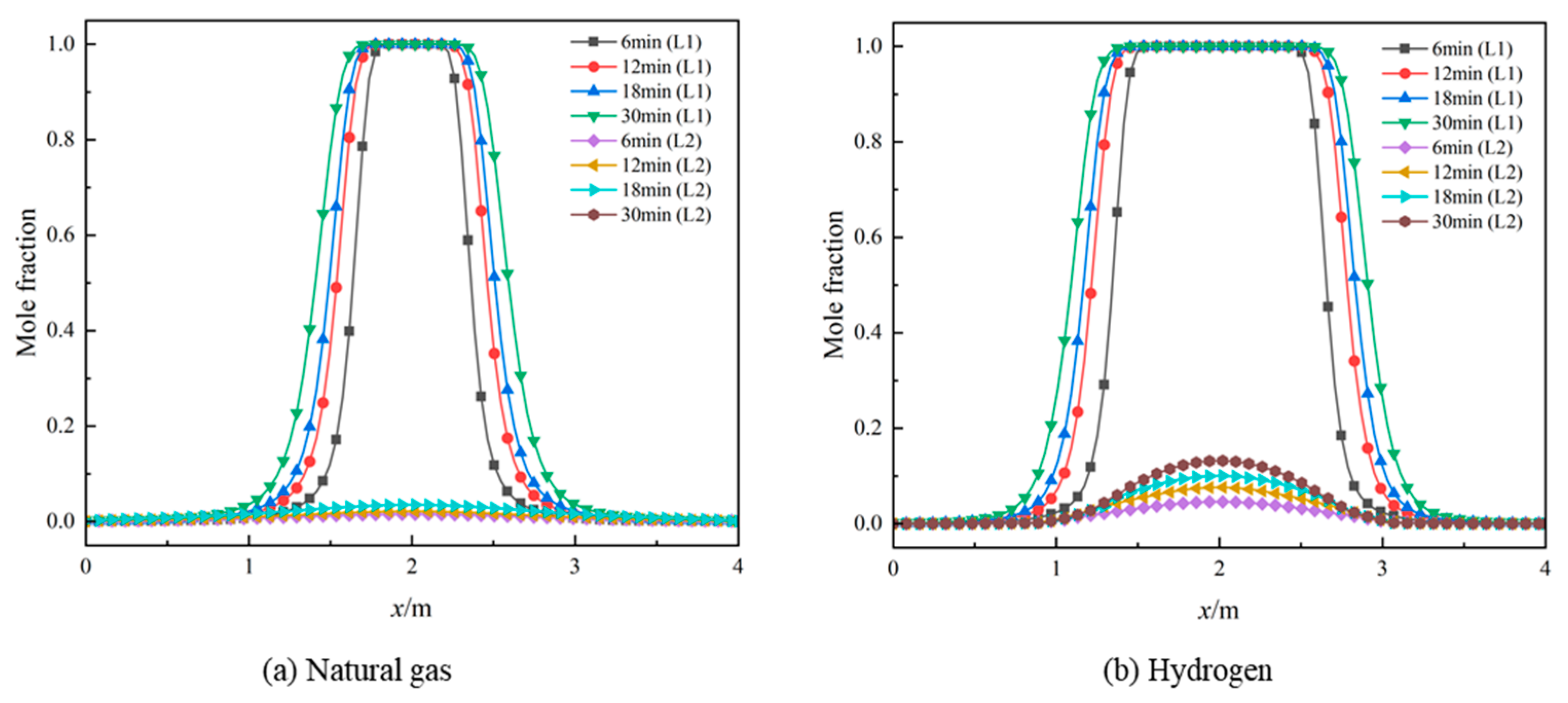
3.1. Leakage and Diffusion Characteristics
3.2. Impact Factor Analysis
3.2.1. Influence of Leakage Pressure
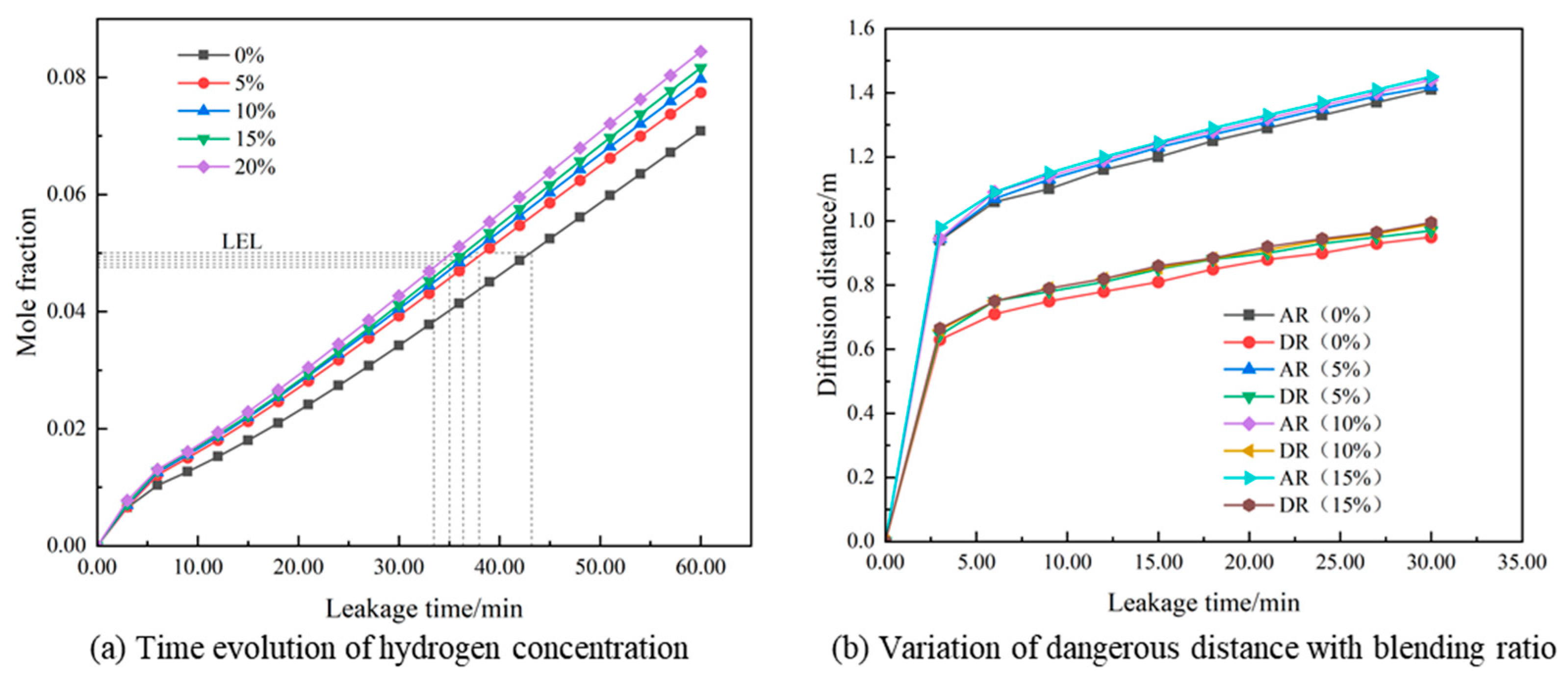
3.2.2. Influence of Hydrogen Blending Ratio
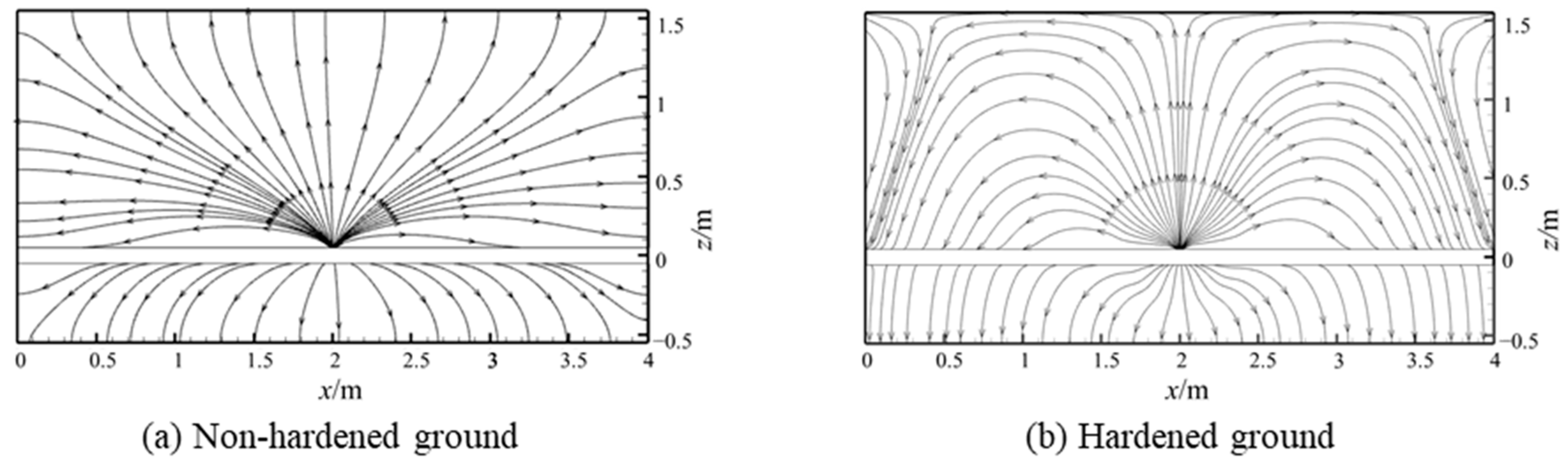
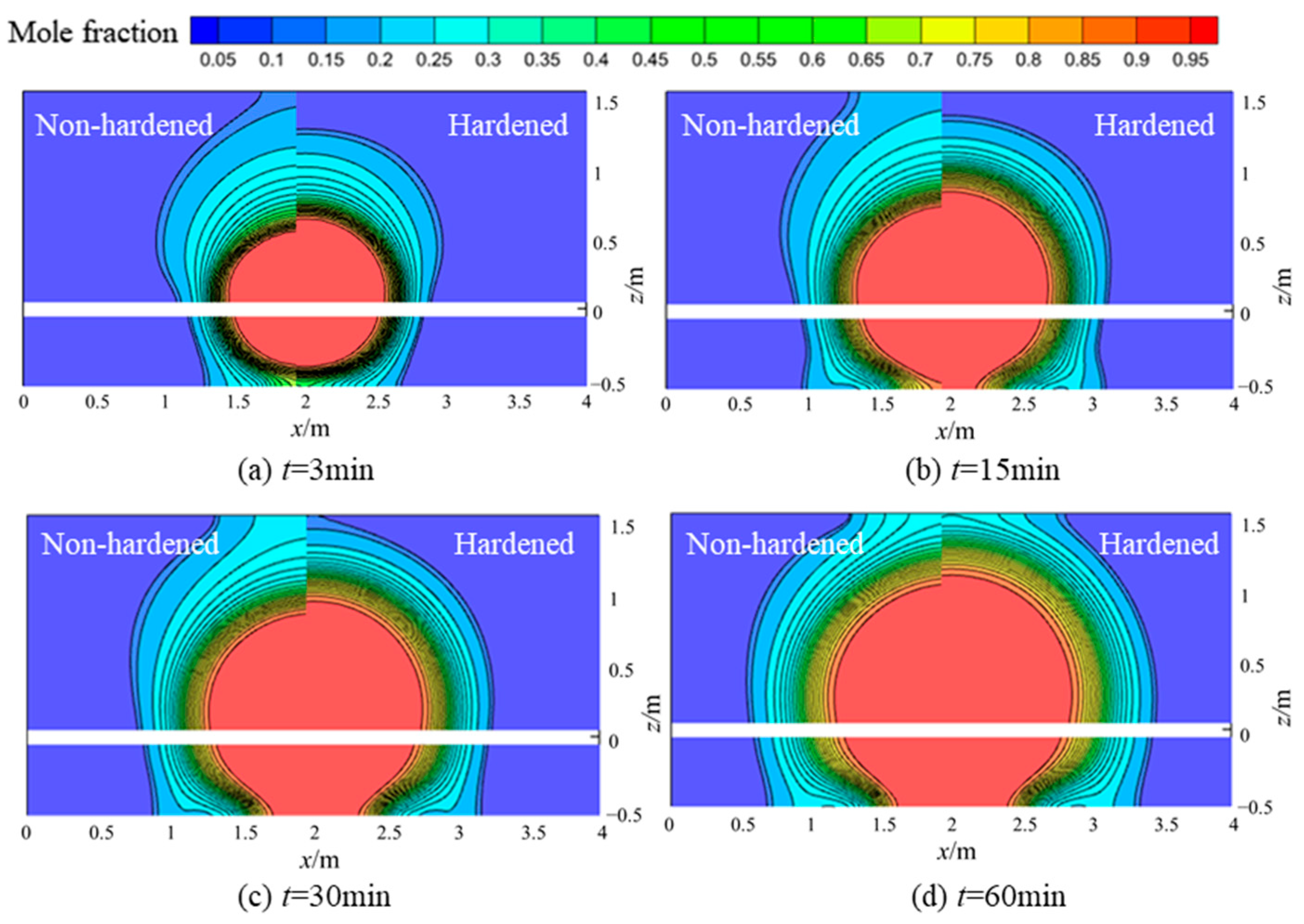
3.2.3. Influence of Ground Hardening Condition
3.2.4. Influence of Leakage Hole Diameter
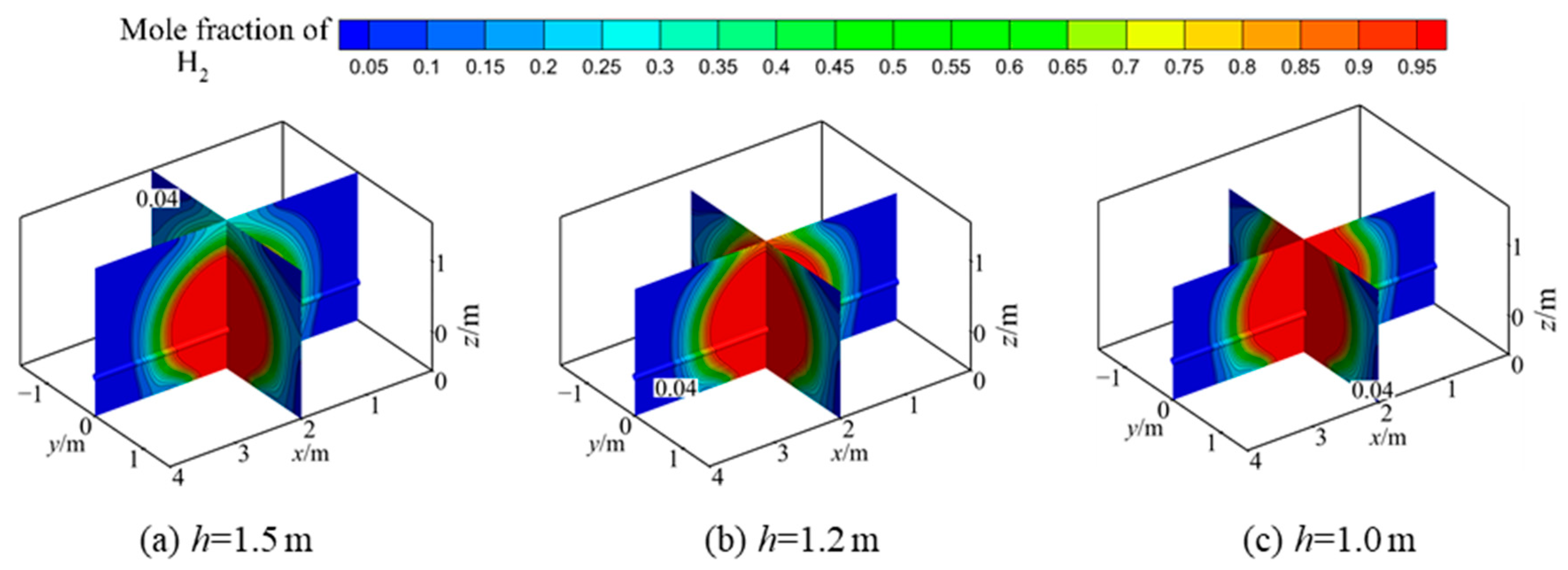
3.2.5. Influence of Pipeline Burial Depth
3.3. Leakage Diagnosis of Hydrogen-Blended Natural Gas
4. Conclusions
- Compared to natural gas, hydrogen exhibits faster pressure and velocity decay. At any given moment, the diffusion range of hydrogen is significantly larger than that of natural gas. Similar to natural gas, hydrogen diffusion in soil can be categorized into three stages: rapid growth, slow growth, and stabilization. The rapid growth stage of hydrogen lasts longer than that of natural gas. Hydrogen diffuses rapidly in soil, with the time for hydrogen reaching the explosive lower limit at the ground surface approximately eight-times faster than natural gas.
- The addition of hydrogen significantly advances the first danger time of gas leakage. As the hydrogen blending ratio increases from 0% to 5%, 10%, 15%, and 20%, the first danger time advances by 5.23 min, 1.55 min, 1.37 min, and 1.57, respectively. Adding hydrogen not only reduces the explosive lower limit of natural gas but also decreases the density and viscosity of natural gas, facilitating gas diffusion. With an increase in the hydrogen blending ratio, the gas velocity gradually decreases.
- The operating pressure, leakage hole diameter, and pipeline burial depth exert distinct levels of influence on the FDT and various danger distances, with the leakage hole diameter being the most influential factor on the FDT. When the leakage hole diameter decreases to a certain size, it effectively delays the arrival of hydrogen at its explosive lower limit at the ground surface.
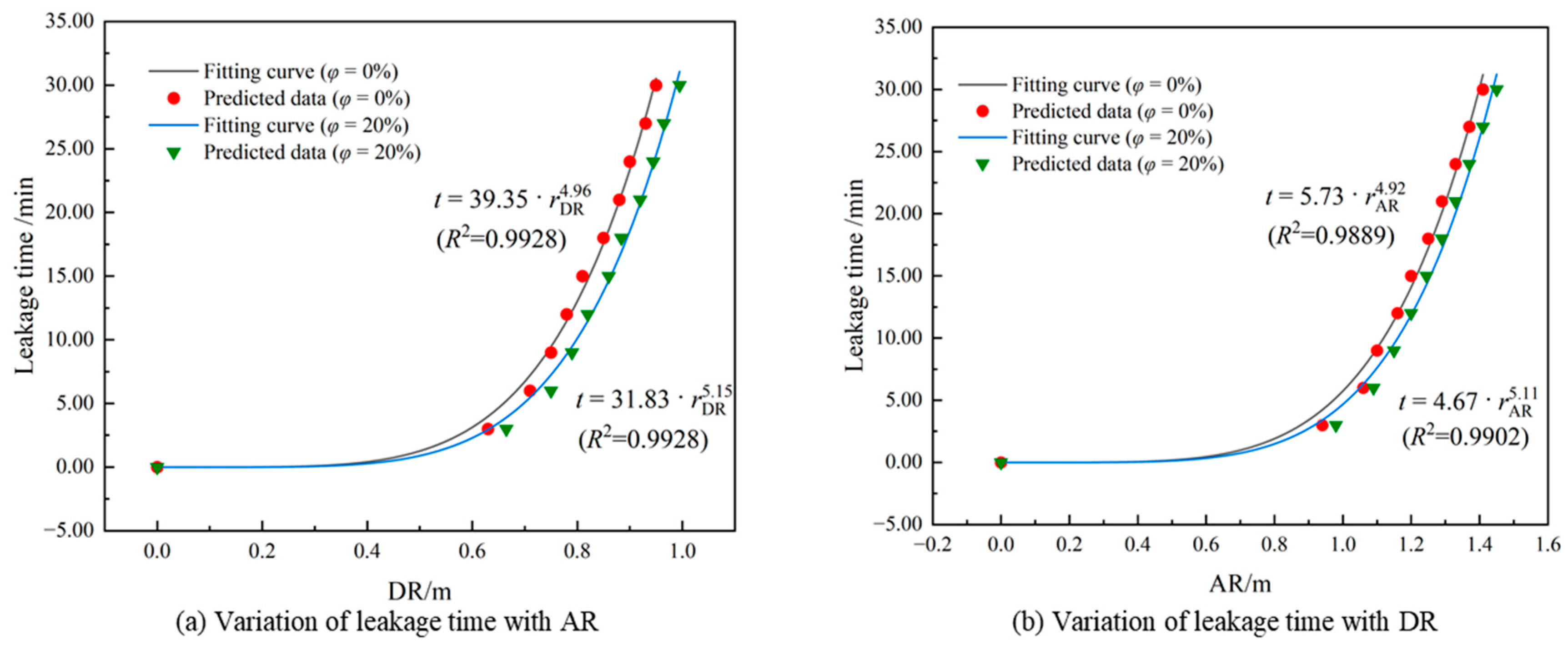
- To enhance the practical application of our findings, we performed a comprehensive risk assessment based on simulation data. Hydrogen-blended natural gas reaches the first-level alarm value more quickly than pure natural gas due to its lower alarm threshold and higher diffusion speed. Consequently, using the alarm radius as an evaluation indicator is recommended for practical gas leakage monitoring. Additionally, we established the relationship between gas leakage time and leakage distance, which can guide the installation of gas sensors and predict the time of gas leakage.
- As temperature changes can significantly impact the diffusion behavior and hazard area of leaking gases, future work will incorporate the effect of ground temperature variations to provide a more comprehensive understanding of gas distribution in the soil. Additionally, future research will consider the mechanisms of how leakage holes are produced in the pipeline structure to enhance the understanding of pipeline integrity and safety.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Groll, M. Can Climate Change Be Avoided? Vision of a Hydrogen-Electricity Energy Economy. Energy 2023, 264, 126029. [Google Scholar] [CrossRef]
- Faye, O.; Szpunar, J.; Eduok, U. A Critical Review on the Current Technologies for the Generation, Storage, and Transportation of Hydrogen. Int. J. Hydrogen Energy 2022, 47, 13771–13802. [Google Scholar] [CrossRef]
- Li, H.; Cao, X.; Liu, Y.; Shao, Y.; Nan, Z.; Teng, L.; Peng, W.; Bian, J. Safety of Hydrogen Storage and Transportation: An Overview on Mechanisms, Techniques, and Challenges. Energy Rep. 2022, 8, 6258–6269. [Google Scholar] [CrossRef]
- Abbas, A.J.; Hassani, H.; Burby, M.; John, I.J. An Investigation into the Volumetric Flow Rate Requirement of Hydrogen Transportation in Existing Natural Gas Pipelines and Its Safety Implications. Gases 2021, 1, 156–179. [Google Scholar] [CrossRef]
- Penev, M.; Zuboy, J.; Hunter, C. Economic Analysis of a High-Pressure Urban Pipeline Concept (HyLine) for Delivering Hydrogen to Retail Fueling Stations. Transp. Res. Part D Transp. Environ. 2019, 77, 92–105. [Google Scholar] [CrossRef]
- Ohaeri, E.; Eduok, U.; Szpunar, J. Hydrogen Related Degradation in Pipeline Steel: A Review. Int. J. Hydrogen Energy 2018, 43, 14584–14617. [Google Scholar] [CrossRef]
- Zhang, H.; Zhao, J.; Li, J.; Yu, B.; Wang, J.; Lyu, R.; Xi, Q. Research Progress on Corrosion and Hydrogen Embrittlement in Hydrogen–Natural Gas Pipeline Transportation. Nat. Gas Ind. B 2023, 10, 570–582. [Google Scholar] [CrossRef]
- Zvirko, O.; Tsyrulnyk, O.; Lipiec, S.; Dzioba, I. Evaluation of Corrosion, Mechanical Properties and Hydrogen Embrittlement of Casing Pipe Steels with Different Microstructure. Materials 2021, 14, 7860. [Google Scholar] [CrossRef] [PubMed]
- Papavinasam, S.; Winston Revie, R.; I. Friesen, W.; Doiron, A.; Panneerselvan, T. Review of Models to Predict Internal Pitting Corrosion Of Oil and Gas Pipelines. Corros. Rev. 2006, 24, 173–230. [Google Scholar] [CrossRef]
- Chaves, I.A.; Melchers, R.E. Pitting corrosion in pipeline steel weld zones. Corros. Sci. 2011, 53, 4026–4032. [Google Scholar] [CrossRef]
- Tang, C.; Zhang, Y.; Huang, Z. Progress in Combustion Investigations of Hydrogen Enriched Hydrocarbons. Renew. Sustain. Energy Rev. 2014, 30, 195–216. [Google Scholar] [CrossRef]
- Xiao, H.; Duan, Q.; Sun, J. Premixed Flame Propagation in Hydrogen Explosions. Renew. Sustain. Energy Rev. 2018, 81, 1988–2001. [Google Scholar] [CrossRef]
- Cirrone, D.; Makarov, D.; Friedrich, A.; Grune, J.; Takeno, K.; Molkov, V. Blast Wave Generated by Delayed Ignition of Under-Expanded Hydrogen Free Jet at Ambient and Cryogenic Temperatures. Hydrogen 2022, 3, 433–449. [Google Scholar] [CrossRef]
- Bu, F.; Chen, S.; Liu, Y.; Guan, B.; Wang, X.; Shi, Z.; Hao, G. CFD Analysis and Calculation Models Establishment of Leakage of Natural Gas Pipeline Considering Real Buried Environment. Energy Rep. 2022, 8, 3789–3808. [Google Scholar] [CrossRef]
- Parvini, M.; Gharagouzlou, E. Gas Leakage Consequence Modeling for Buried Gas Pipelines. J. Loss Prev. Process Ind. 2015, 37, 110–118. [Google Scholar] [CrossRef]
- Okamoto, H.; Gomi, Y.; Akagi, H. Movement Characteristics of Hydrogen Gas within Compacted Soil and Its Detection. Doboku Gakkai Ronbunshuu F 2010, 66, 146–157. [Google Scholar] [CrossRef][Green Version]
- Liu, C.; Liao, Y.; Liang, J.; Cui, Z.; Li, Y. Quantifying Methane Release and Dispersion Estimations for Buried Natural Gas Pipeline Leakages. Process Saf. Environ. Prot. 2021, 146, 552–563. [Google Scholar] [CrossRef]
- Bezaatpour, J.; Fatehifar, E.; Rasoulzadeh, A. CFD Investigation of Natural Gas Leakage and Propagation from Buried Pipeline for Anisotropic and Partially Saturated Multilayer Soil. J. Clean. Prod. 2020, 277, 123940. [Google Scholar] [CrossRef]
- Zhu, J.; Pan, J.; Zhang, Y.; Li, Y.; Li, H.; Feng, H.; Chen, D.; Kou, Y.; Yang, R. Leakage and Diffusion Behavior of a Buried Pipeline of Hydrogen-Blended Natural Gas. Int. J. Hydrogen Energy 2023, 48, 11592–11610. [Google Scholar] [CrossRef]
- Wang, L.; Chen, J.; Ma, T.; Ma, R.; Bao, Y.; Fan, Z. Numerical Study of Leakage Characteristics of Hydrogen-Blended Natural Gas in Buried Pipelines. Int. J. Hydrogen Energy 2024, 49, 1166–1179. [Google Scholar] [CrossRef]
- Zhang, Y.; Yang, Y.; Wu, F.; Li, Q.; Wang, J.; Liu, H.; Che, D.; Huang, Z. Numerical Investigation on Pinhole Leakage and Diffusion Characteristics of Medium-Pressure Buried Hydrogen Pipeline. Int. J. Hydrogen Energy 2024, 51, 807–817. [Google Scholar] [CrossRef]
- Li, Y.; Wang, Z.; Shang, Z. Analysis and Prediction of Hydrogen-Blended Natural Gas Diffusion from Various Pipeline Leakage Sources Based on CFD and ANN Approach. Int. J. Hydrogen Energy 2024, 53, 535–549. [Google Scholar] [CrossRef]
- Zhu, J.; Wang, S.; Pan, J.; Lv, H.; Zhang, Y.; Han, H.; Liu, C.; Duo, Z.; Li, Y. Experimental Study on Leakage Temperature Field of Hydrogen Blending into Natural Gas Buried Pipeline. Appl. Energy 2024, 359, 122656. [Google Scholar] [CrossRef]
- Li, Y.; Qian, X.; Zhang, S.; Sheng, J.; Hou, L.; Yuan, M. Assessment of Gas Explosion Risk in Underground Spaces Adjacent to a Gas Pipeline. Tunn. Undergr. Space Technol. 2023, 131, 104785. [Google Scholar] [CrossRef]
- GB50494-2009; Technical Code for City Gas. China Planning Press: Beijing, China, 2008.
- ISO 4437-5:2014; Plastics Piping Systems for the Supply of Gaseous Fuels—Polyethylene (PE)—Part 5: Fitness for Purpose of the System. ISO: Geneva, Switzerland, 2014. Available online: https://www.iso.org/standard/59131.html (accessed on 31 January 2014).
- EN 12007-2:2012; Gas Supply Systems—Pipelines for Maximum Operating Pressure up to and Including 16 bar—Part 2: Specific Functional Requirements for Polyethylene (PE) Pipelines. CEN: Brussels, Belgium, 2012. Available online: https://www.evs.ee/en/evs-en-12007-2-2012 (accessed on 1 December 2012).
- Luo, J.H.; Zheng, M.; Zhao, X.W.; Huo, C.Y.; Yang, L. Simplified Expression for Estimating Release Rate of Hazardous Gas from a Hole on High-Pressure Pipelines. J. Loss Prev. Process Ind. 2006, 19, 362–366. [Google Scholar] [CrossRef]
- Montiel, H.; Vilchez, J.; Casal, J.; Arnaldos, J. Mathematical Modelling of Accidental Gas Releases. J. Hazard. Mater. 1998, 59, 211–233. [Google Scholar] [CrossRef]
- Strelets, M. Detached Eddy Simulation of Massively Separated Flows. In Proceedings of the 39th Aerospace Sciences Meeting and Exhibit, Reston, VA, USA, 8 January 2001. [Google Scholar]
- Liu, Y.; Zheng, J. Numerical Simulation of the Diffusion of Natural Gas Due to Pipeline Failure. In Proceedings of the ASME 2007 Pressure Vessels and Piping Conference, San Antonio, TX, USA, 22–26 July 2007; Volume 7: Operations, Applications and Components, pp. 319–325. [Google Scholar]
- Wu, L.; Qiao, L.; Fan, J.; Wen, J.; Zhang, Y.; Jar, B. Investigation on Natural Gas Leakage and Diffusion Characteristics Based on CFD. Gas Sci. Eng. 2024, 123, 205238. [Google Scholar] [CrossRef]
- Lu, H.; Guo, B.; Chen, X.; Yao, J.; Liu, B. Numerical Investigation on Leakage and Diffusion Characteristics of Buried Hydrogen-Blended Natural Gas Pipelines. Int. J. Hydrogen Energy 2024, 59, 1491–1506. [Google Scholar] [CrossRef]
- Yan, Y.; Dong, X.; Li, J. Experimental Study of Methane Diffusion in Soil for an Underground Gas Pipe Leak. J. Nat. Gas Sci. Eng. 2015, 27, 82–89. [Google Scholar] [CrossRef]
- GB/T50493-2019; Standard for Design of Combustible Gas and Toxic Gas Detection and Alarm for Petrochemical Industry. China Planning Press: Beijing, China, 2019. Available online: https://www.codeofchina.com/standard/GBT50493-2019.html (accessed on 1 January 2020).
- Mashuga, C.V.; Crowl, D.A. Derivation of Le Chatelier’s Mixing Rule for Flammable Limits. Process Saf. Prog. 2000, 19, 112–117. [Google Scholar] [CrossRef]
- European Gas Pipeline Incident Data Group. 2011 Annual Report. 2011. Available online: https://www.annualreports.com/HostedData (accessed on 1 December 2014).


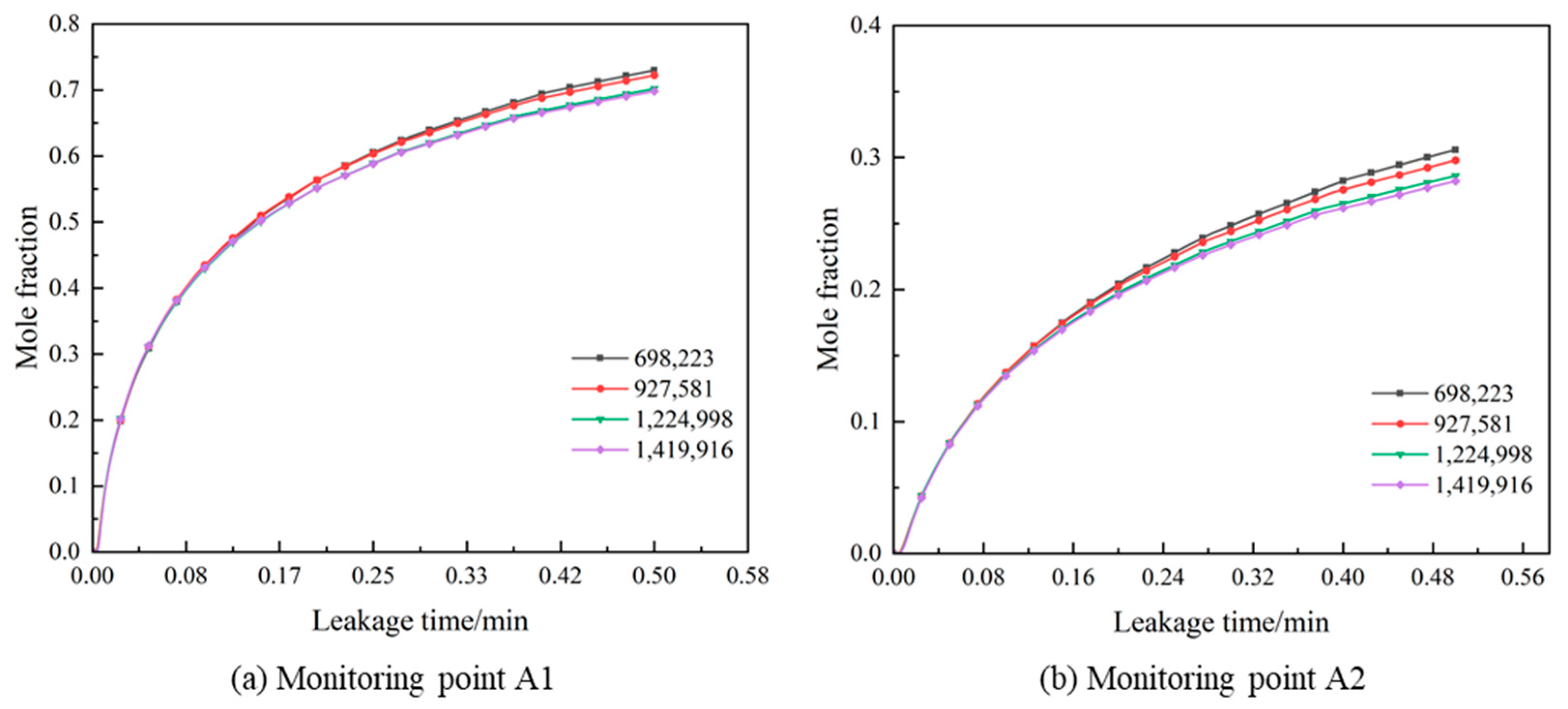

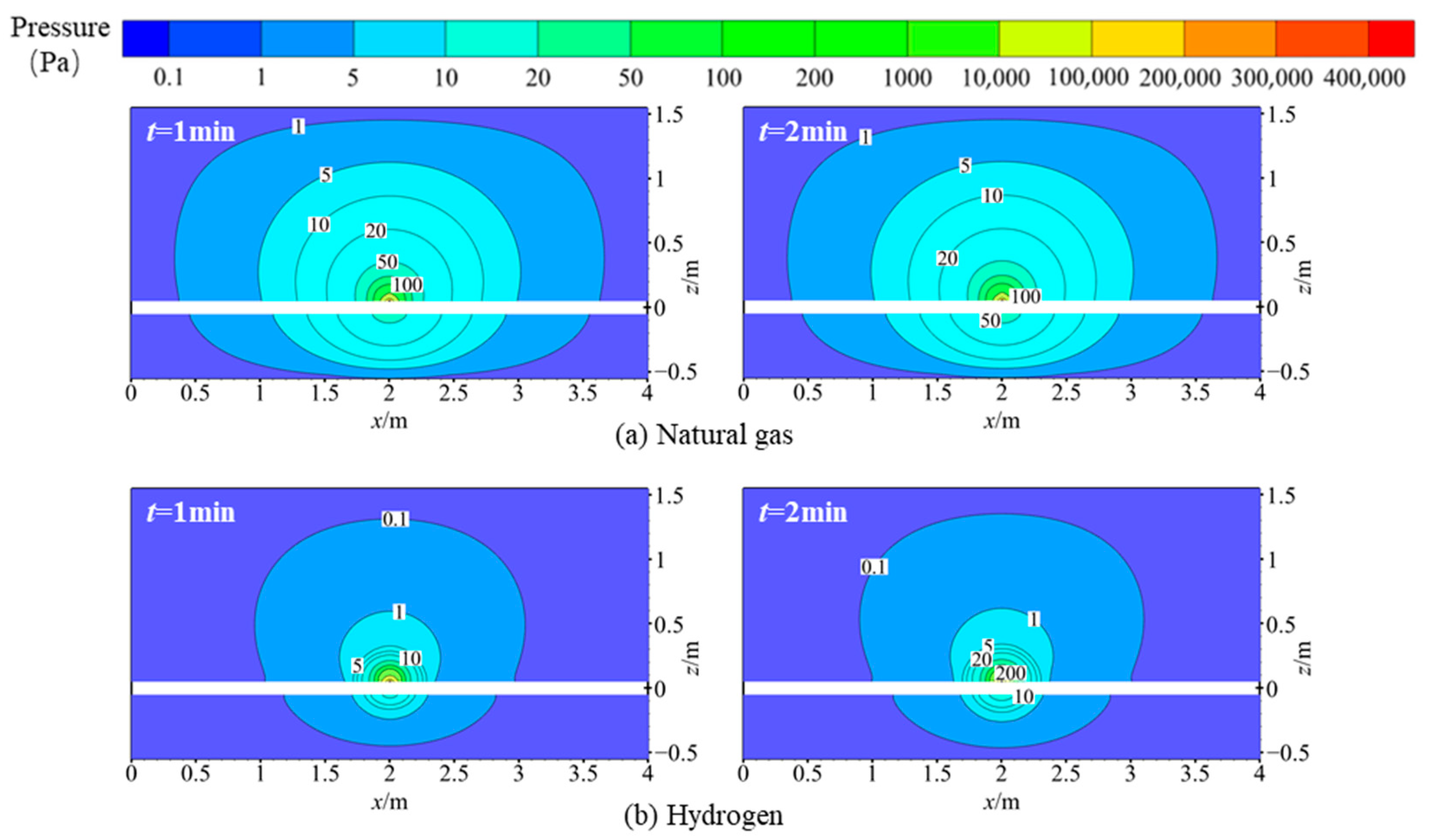
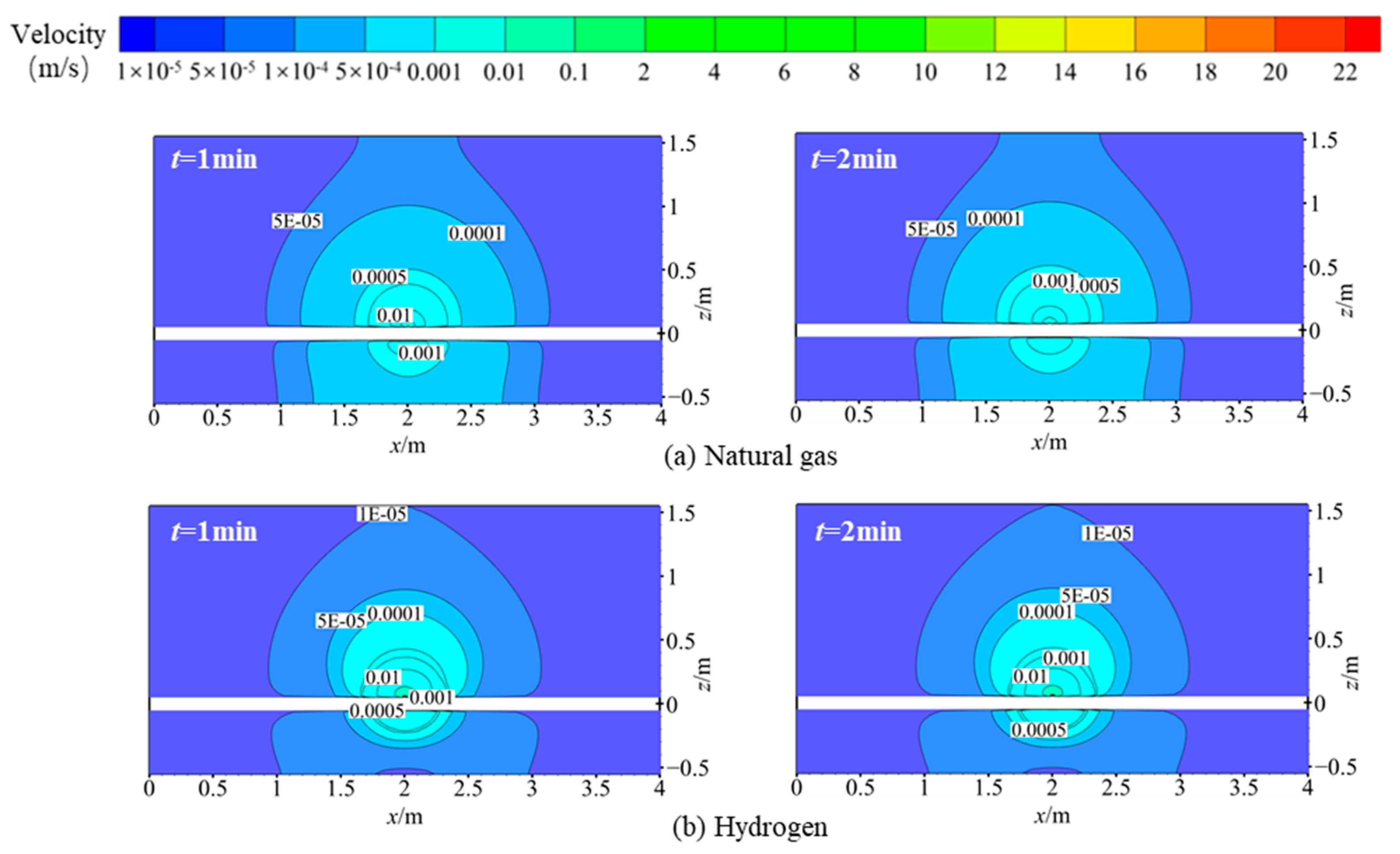
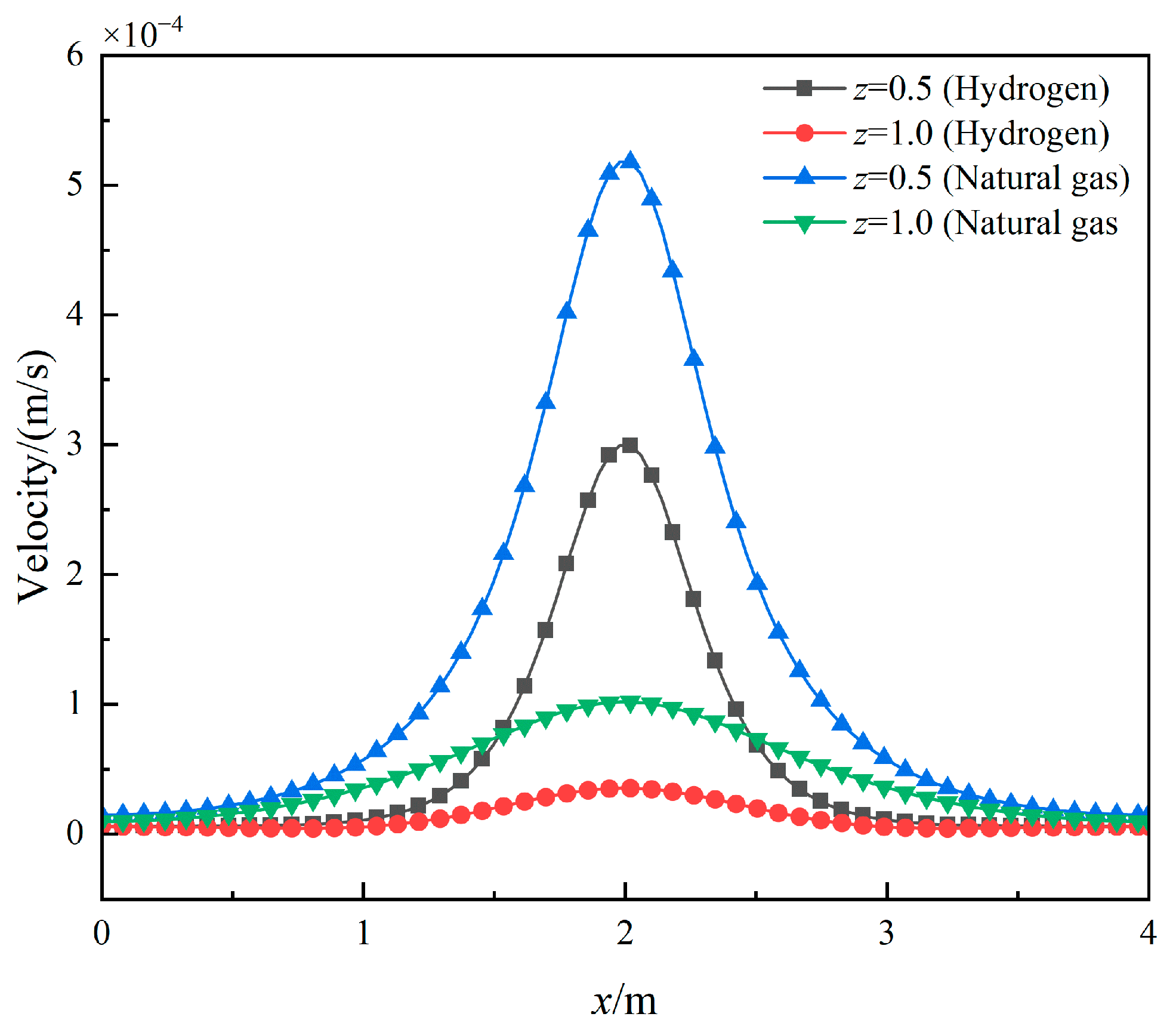







| Monitoring Points | Location | Monitoring Lines | Location |
|---|---|---|---|
| P1 | (2, 0, 0.5) | L1 | (x, 0, 0.05) |
| P2 | (2, 0, 1) | L2 | (x, 0, 1.55) |
| P3 | (2, 0, 1.55) | - | |
| P4 | (1.5, 0, 1) | ||
| P5 | (2.5, 0, 1) | ||
| P6 | (2, 0.5, 1) | ||
| Boundary | Type | Values |
|---|---|---|
| Leakage hole | Pressure inlet | 0.4 MPa |
| Lateral surface of pipe | Wall | No slip |
| Lateral surface of soil | Pressure outlet | 0.1 MPa |
| Bottom surface of soil | Pressure outlet | 0.1 MPa |
| Ground surface | Pressure outlet/Wall | 0.1 MPa/No slip |
| Scenario | p (MPa) | φ | d (m) | h (m) | Ground Hardening |
|---|---|---|---|---|---|
| 1 | 0.4 | 0 | 0.02 | 1.5 | No |
| 2 | 0.3 | 0 | 0.02 | 1.5 | No |
| 3 | 0.2 | 0 | 0.02 | 1.5 | No |
| 4 | 0.4 | 5% | 0.02 | 1.5 | No |
| 5 | 0.4 | 10% | 0.02 | 1.5 | No |
| 6 | 0.4 | 15% | 0.02 | 1.5 | No |
| 7 | 0.4 | 20% | 0.02 | 1.5 | No |
| 8 | 0.4 | 0 | 0.01 | 1.5 | No |
| 9 | 0.4 | 0 | 0.03 | 1.5 | No |
| 10 | 0.4 | 0 | 0.02 | 1.2 | No |
| 11 | 0.4 | 0 | 0.02 | 1.0 | No |
| 12 | 0.4 | 0 | 0.02 | 1.5 | Yes |
| Hydrogen Blending Ratio | LEL |
|---|---|
| 0 | 5% |
| 5% | 4.9383% |
| 10% | 4.878% |
| 15% | 4.8193% |
| 20% | 4.7619% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Xie, B.; Gong, L. Numerical Research on Leakage Characteristics of Pure Hydrogen/Hydrogen-Blended Natural Gas in Medium- and Low-Pressure Buried Pipelines. Energies 2024, 17, 2951. https://doi.org/10.3390/en17122951
Li J, Xie B, Gong L. Numerical Research on Leakage Characteristics of Pure Hydrogen/Hydrogen-Blended Natural Gas in Medium- and Low-Pressure Buried Pipelines. Energies. 2024; 17(12):2951. https://doi.org/10.3390/en17122951
Chicago/Turabian StyleLi, Jiadong, Bingchuan Xie, and Liang Gong. 2024. "Numerical Research on Leakage Characteristics of Pure Hydrogen/Hydrogen-Blended Natural Gas in Medium- and Low-Pressure Buried Pipelines" Energies 17, no. 12: 2951. https://doi.org/10.3390/en17122951
APA StyleLi, J., Xie, B., & Gong, L. (2024). Numerical Research on Leakage Characteristics of Pure Hydrogen/Hydrogen-Blended Natural Gas in Medium- and Low-Pressure Buried Pipelines. Energies, 17(12), 2951. https://doi.org/10.3390/en17122951






