Enhancing Heat Transfer in Mini-Scale Liquid-Cooled Heat Sinks by Flow Oscillation—A Numerical Analysis
Abstract
1. Introduction
2. Methodology
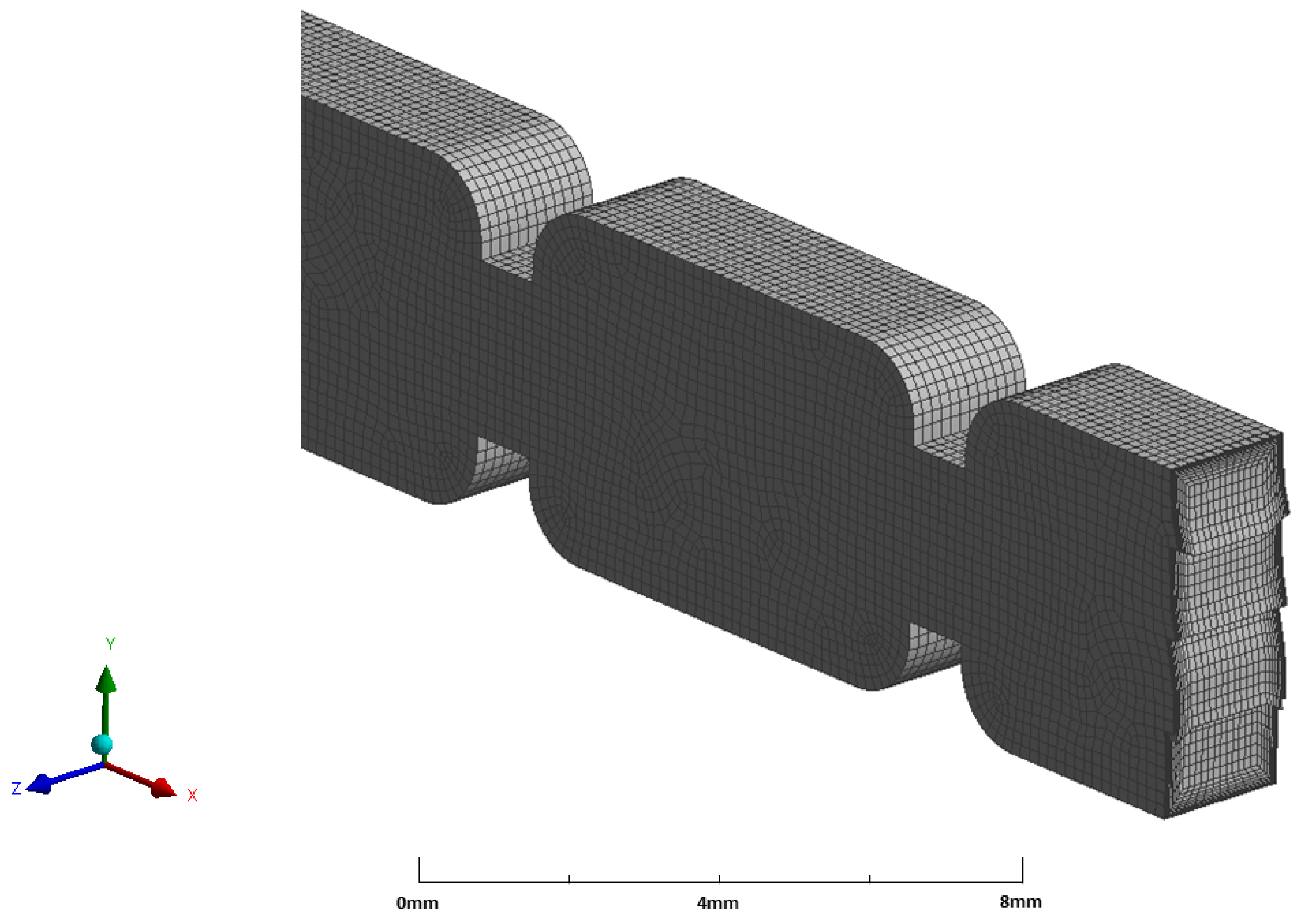
2.1. Geometry, Mesh and Model
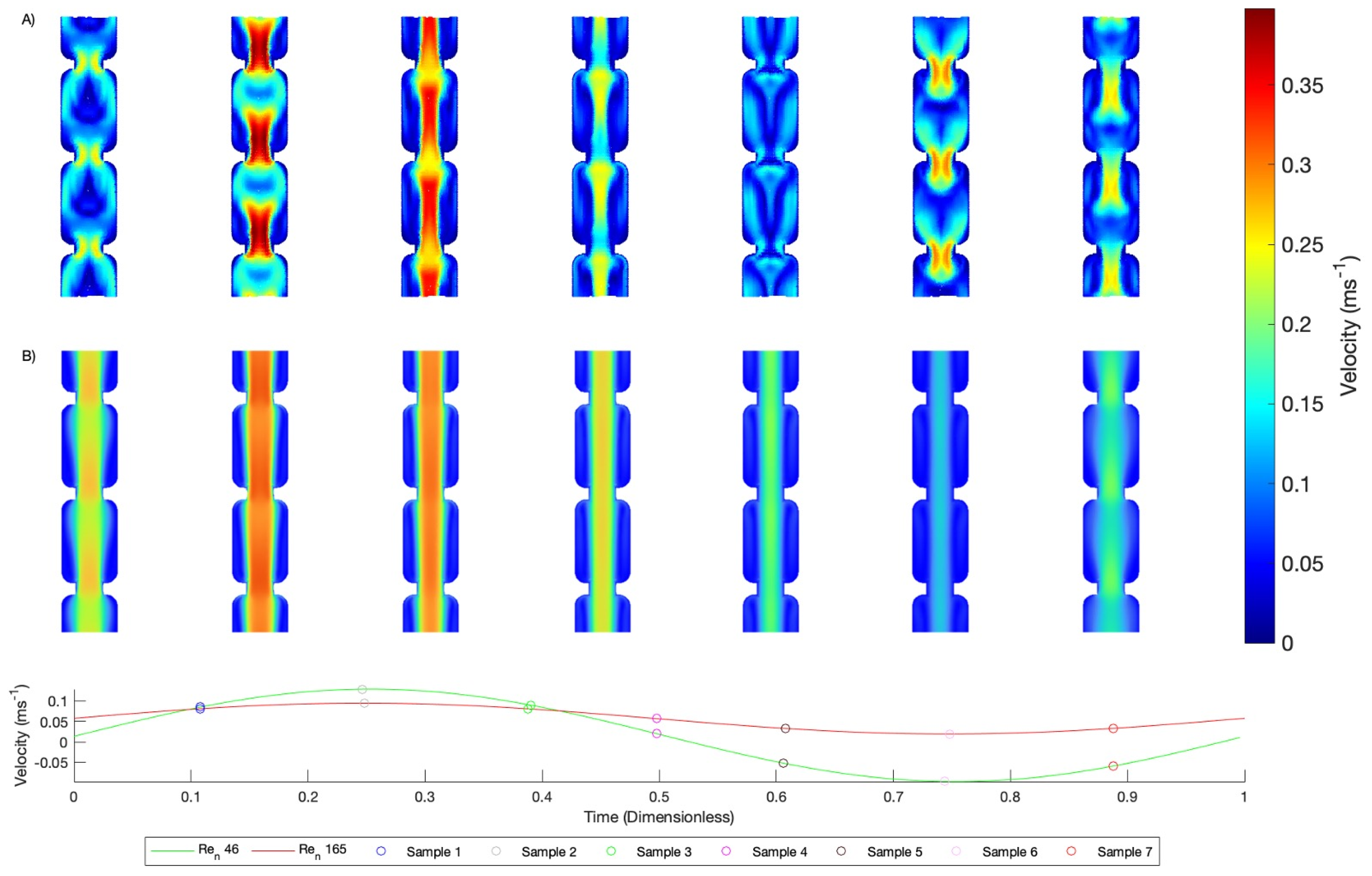
2.2. Data Analysis
3. Results and Discussion
3.1. Grid Independence Study
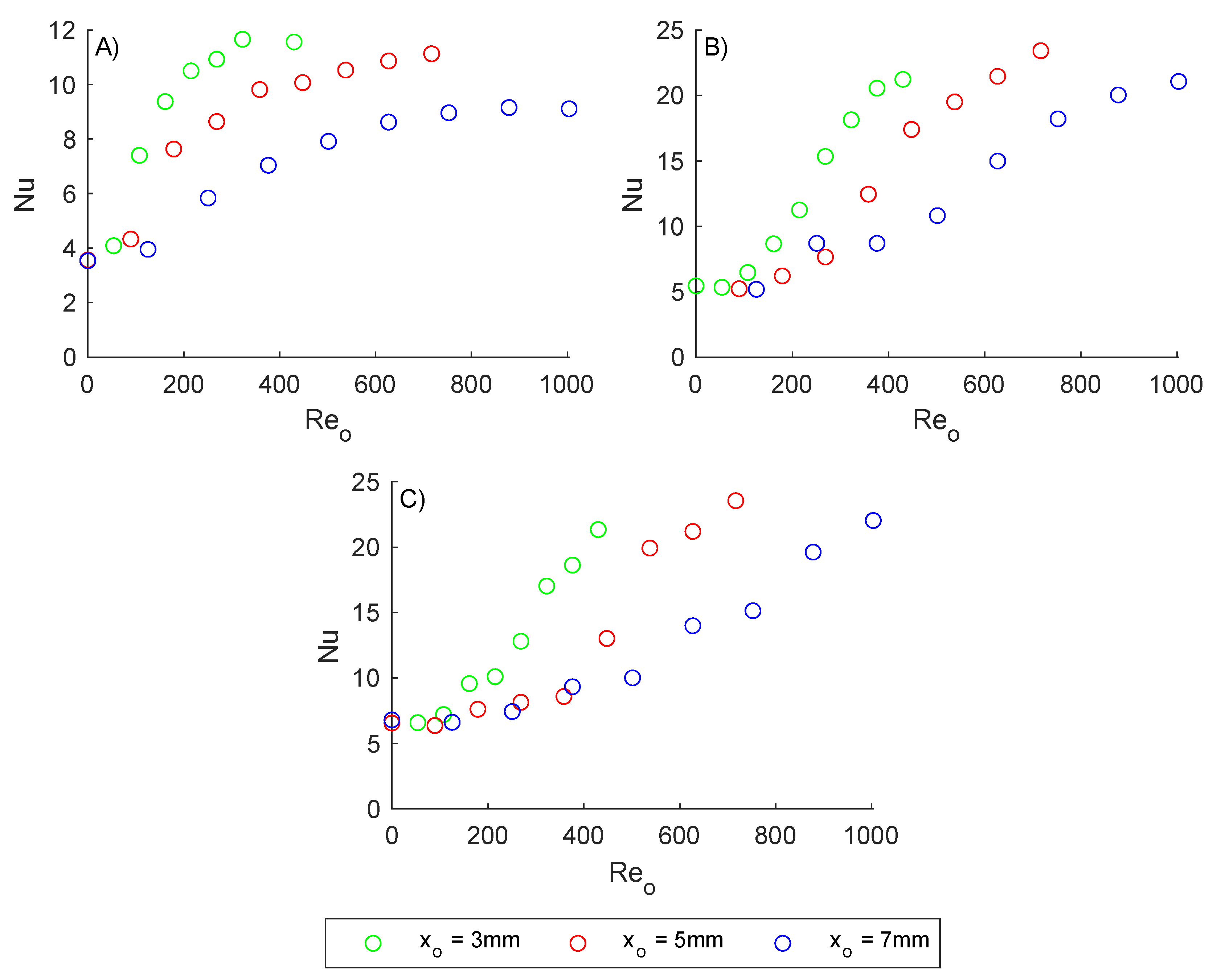
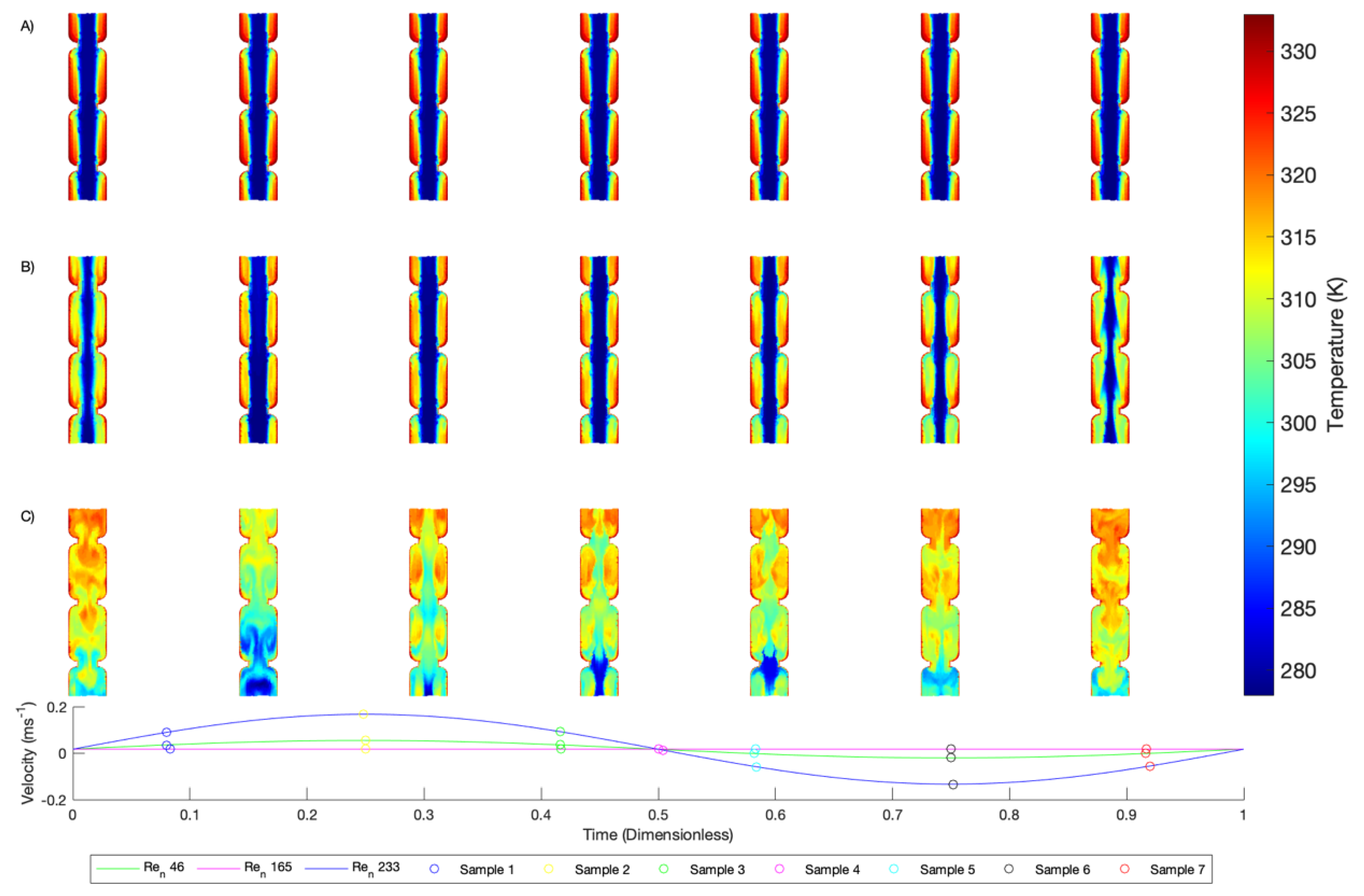
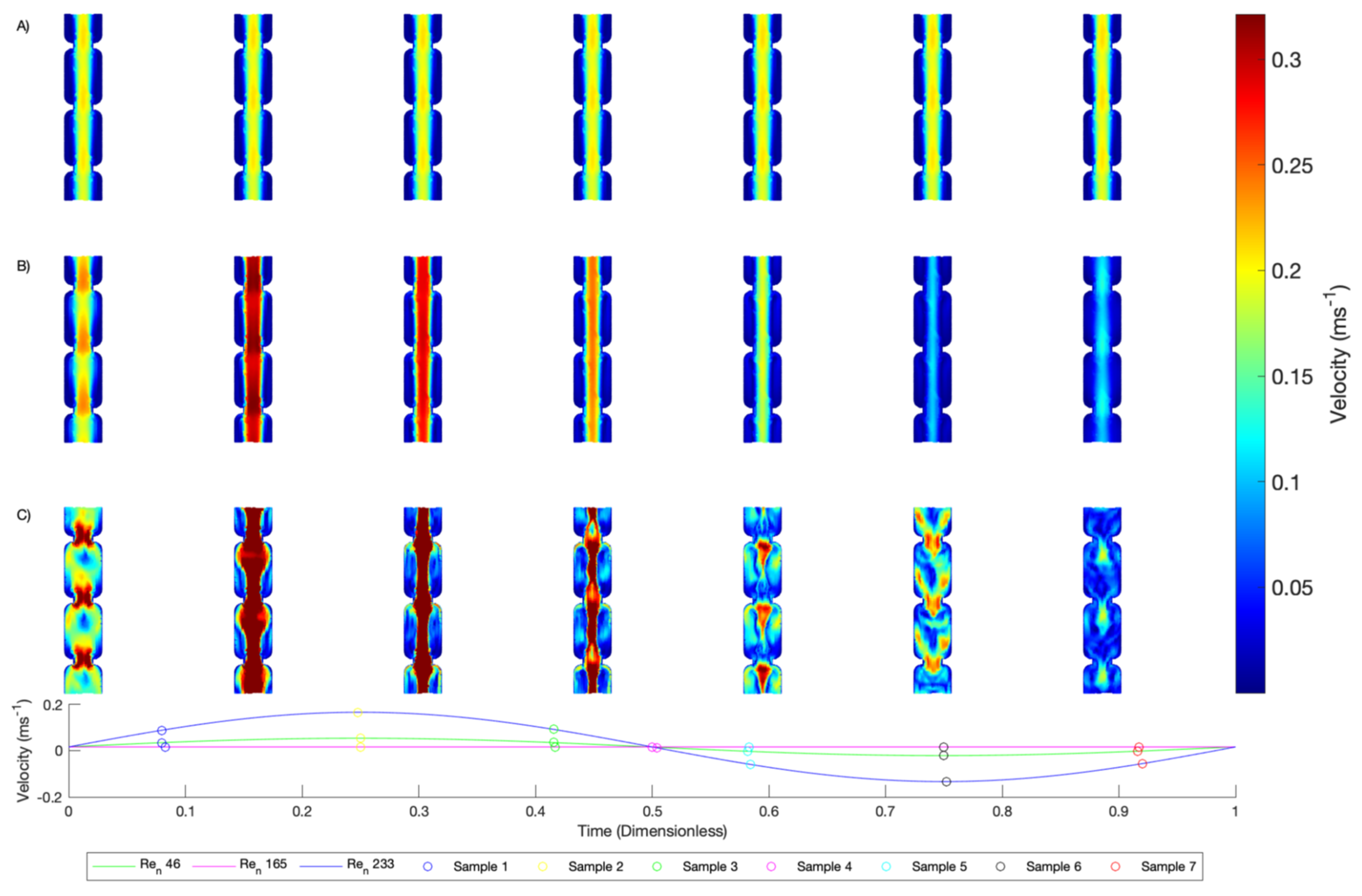
3.2. Heat Transfer Enhancement
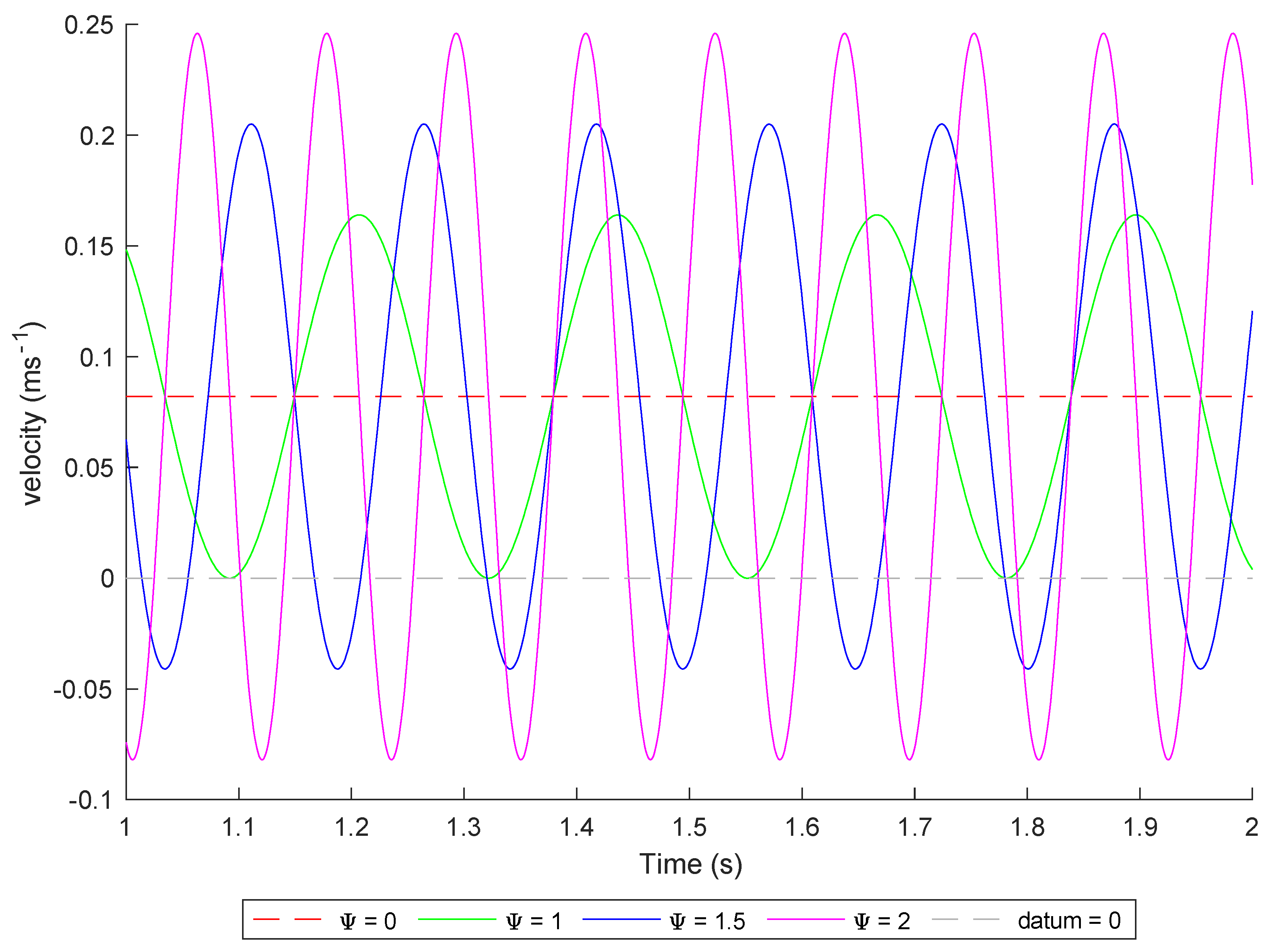
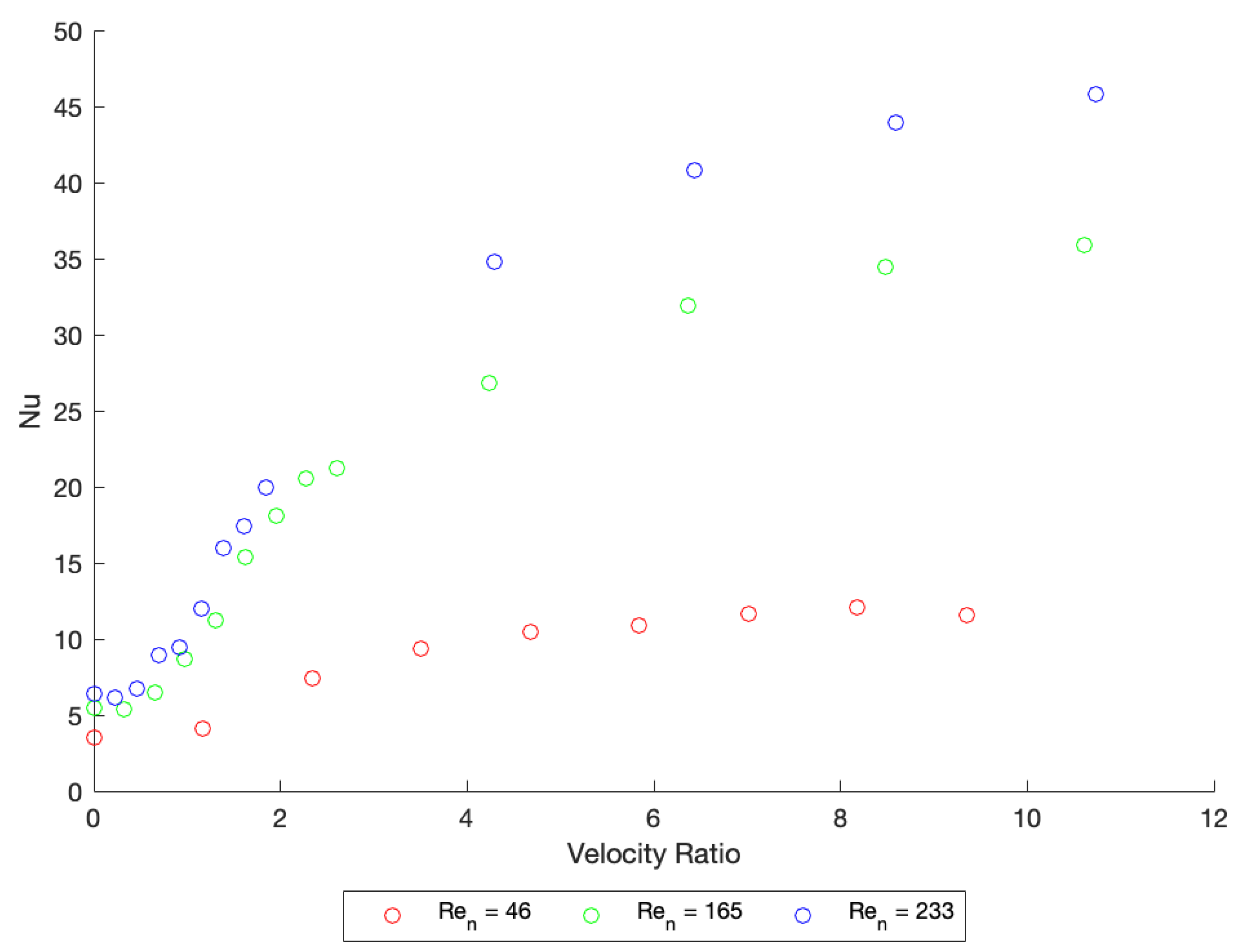
3.3. Effect of Velocity Ratio
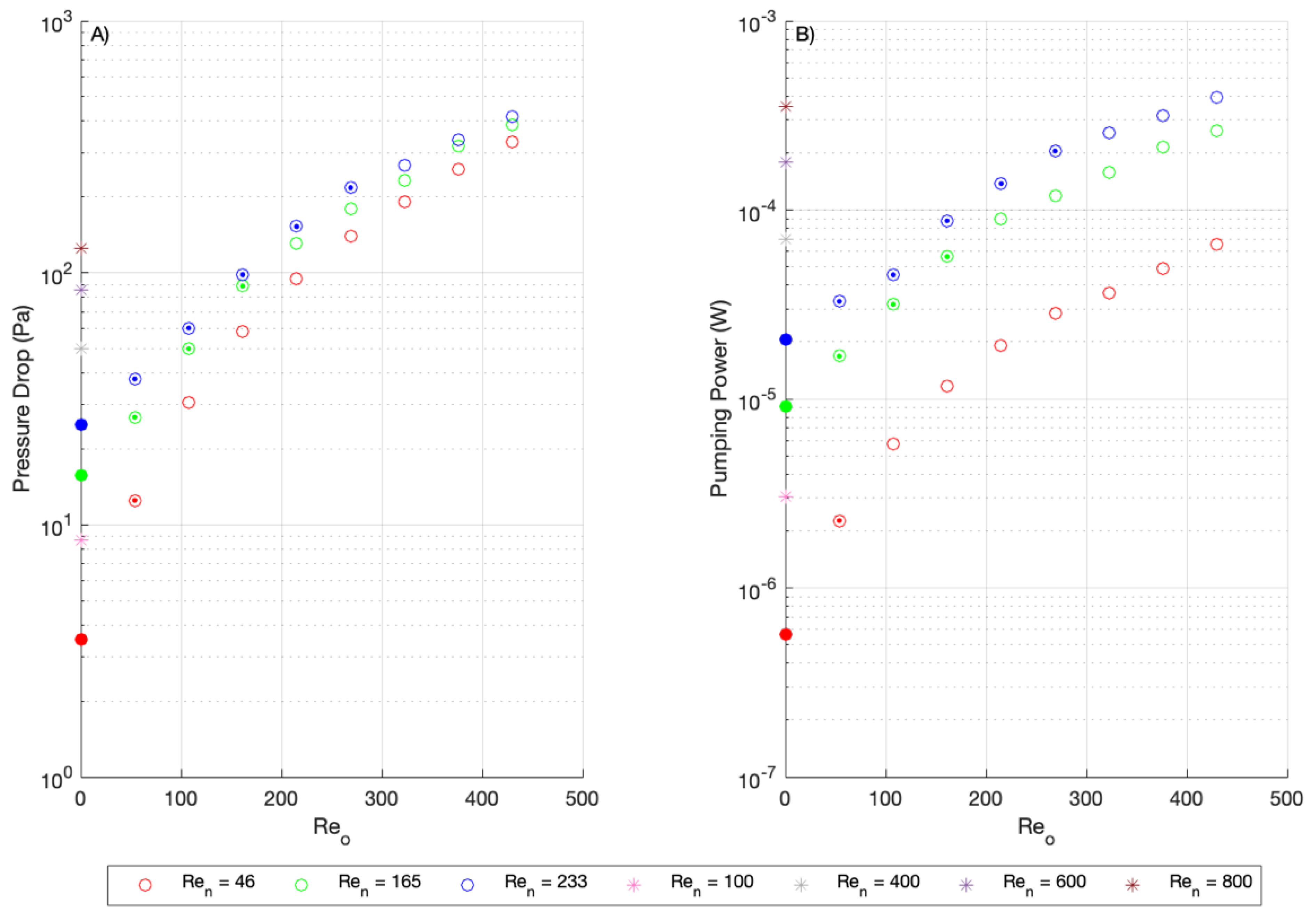
3.4. Power Requirements for Oscillatory Flow
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, Z.; Wang, X.; Yan, Y. A review of the state-of-the-art in electronic cooling. e-Prime-Adv. Electr. Eng. Electron. Energy 2021, 1, 100009. [Google Scholar] [CrossRef]
- Moore, S.K. The Transistor of 2047: What will the device be like on its 100th anniversary? IEEE Spectr. 2022, 59, 38–39. [Google Scholar] [CrossRef]
- Qu, W.; Mudawar, I. Analysis of three-dimensional heat transfer in micro-channel heat sinks. Int. J. Heat Mass Transf. 2002, 45, 3973–3985. [Google Scholar] [CrossRef]
- Marzougui, M.; Hammami, M.; Ben Maad, R. Experimental study on thermal performance of heat sinks: The effect of hydraulic diameter and geometric shape. Heat Mass Transf. 2015, 52, 2091–2100. [Google Scholar] [CrossRef]
- Liu, X.; Yu, J. Numerical study on performances of mini-channel heat sinks with non-uniform inlets. Appl. Therm. Eng. 2016, 93, 856–864. [Google Scholar] [CrossRef]
- Wei, M.; Boutin, G.; Fan, Y.; Luo, L. Numerical and experimental investigation on the realization of target flow distribution among parallel mini-channels. Chem. Eng. Res. Des. 2016, 113, 74–84. [Google Scholar] [CrossRef]
- Anbumeenakshi, C.; Thansekhar, M. Experimental investigation of header shape and inlet configuration on flow maldistribution in microchannel. Exp. Therm. Fluid Sci. 2016, 75, 156–161. [Google Scholar] [CrossRef]
- Mu, Y.-T.; Chen, L.; He, Y.-L.; Tao, W.-Q. Numerical study on temperature uniformity in a novel mini-channel heat sink with different flow field configurations. Int. J. Heat Mass Transf. 2015, 85, 147–157. [Google Scholar] [CrossRef]
- Xu, M.; Lu, H.; Gong, L.; Chai, J.C.; Duan, X. Parametric numerical study of the flow and heat transfer in microchannel with dimples. Int. Commun. Heat Mass Transf. 2016, 76, 348–357. [Google Scholar] [CrossRef]
- Khoshvaght-Aliabadi, M.; Sahamiyan, M.; Hesampour, M.; Sartipzadeh, O. Experimental study on cooling performance of sinusoidal–wavy minichannel heat sink. Appl. Therm. Eng. 2016, 92, 50–61. [Google Scholar] [CrossRef]
- Jadhav, S.V.; Kale, S.M.; Kashid, D.T.; Kakade, S.S.; Gavali, S.R.; Shinde, S.D. Performance Analysis of Heat Sink with Different Microchannel Orientations. In Techno-Societal 2018; Springer International Publishing: Cham, Switzerland, 2020. [Google Scholar]
- Duangthongsuk, W.; Wongwises, S. A comparison of the thermal and hydraulic performances between miniature pin fin heat sink and microchannel heat sink with zigzag flow channel together with using nanofluids. Heat Mass Transf. 2018, 54, 3265–3274. [Google Scholar] [CrossRef]
- Scott, A.W. Cooling of Electronic Equipment; John Wiley & Sons: Hoboken, NJ, USA, 1974. [Google Scholar]
- Meybodi, M.K.; Daryasafar, A.; Koochi, M.M.; Moghadasi, J.; Meybodi, R.B.; Ghahfarokhi, A.K. A novel correlation approach for viscosity prediction of water based nanofluids of Al2O3, TiO2, SiO2 and CuO. J. Taiwan Inst. Chem. Eng. 2016, 58, 19–27. [Google Scholar] [CrossRef]
- Nada, S.A.; El-Zoheiry, R.M.; Elsharnoby, M.; Osman, O.S. Enhancing the thermal performance of different flow configuration minichannel heat sink using Al2O3 and CuO-water nanofluids for electronic cooling: An experimental assessment. Int. J. Therm. Sci. 2022, 181, 107767. [Google Scholar] [CrossRef]
- Alkasmoul, F.S.; Al-Asadi, M.T.; Myers, T.G.; Thompson, H.M.; Wilson, M.C.T. A practical evaluation of the performance of Al2O3-water, TiO2-water and CuO-water nanofluids for convective cooling. Int. J. Heat Mass Transf. 2018, 126, 639–651. [Google Scholar] [CrossRef]
- Al-Neama, A.F.; Kapur, N.; Summers, J.; Thompson, H.M. Thermal management of GaN HEMT devices using serpentine minichannel heat sinks. Appl. Therm. Eng. 2018, 140, 622–636. [Google Scholar] [CrossRef]
- Al-Neama, A.F.; Khatir, Z.; Kapur, N.; Summers, J.; Thompson, H.M. An experimental and numerical investigation of chevron fin structures in serpentine minichannel heat sinks. Int. J. Heat Mass Transf. 2018, 120, 1213–1228. [Google Scholar] [CrossRef]
- Mackley, M.; Stonestreet, P. Heat transfer and associated energy dissipation for oscillatory flow in baffled tubes. Chem. Eng. Sci. 1995, 50, 2211–2224. [Google Scholar] [CrossRef]
- Ahmed, S.M.; Law, R.; Phan, A.N.; Harvey, A.P. Thermal performance of meso-scale oscillatory baffled reactors. Chem. Eng. Process.-Process. Intensif. 2018, 132, 25–33. [Google Scholar] [CrossRef]
- Law, R.; Ahmed, S.M.; Tang, N.; Phan, A.N.; Harvey, A.P. Development of a more robust correlation for predicting heat transfer performance in oscillatory baffled reactors. Chem. Eng. Process.-Process. Intensif. 2018, 125, 133–138. [Google Scholar] [CrossRef]
- McDonough, J.; Ahmed, S.; Phan, A.; Harvey, A. A study of the flow structures generated by oscillating flows in a helical baffled tube. Chem. Eng. Sci. 2017, 171, 160–178. [Google Scholar] [CrossRef]
- Phan, A.N.; Harvey, A. Development and evaluation of novel designs of continuous mesoscale oscillatory baffled reactors. Chem. Eng. J. 2010, 159, 212–219. [Google Scholar] [CrossRef]
- Stonestreet, P.; Harvey, A. A Mixing-Based Design Methodology for Continuous Oscillatory Flow Reactors. Chem. Eng. Res. Des. 2002, 80, 31–44. [Google Scholar] [CrossRef]
- Phan, A.N.; Harvey, A.P. Characterisation of mesoscale oscillatory helical baffled reactor—Experimental approach. Chem. Eng. J. 2012, 180, 229–236. [Google Scholar] [CrossRef]
- Muñoz-Cámara, J.; Crespí-Llorens, D.; Solano, J.; Vicente, P. Effect of three-orifice baffles orientation on the flow and thermal–hydraulic performance: Experimental analysis for net and oscillatory flows. Appl. Therm. Eng. 2024, 236, 121566. [Google Scholar] [CrossRef]
- Gonzalez-Juarez, D.; Herrero-Martin, R.; Solano, J.P. Enhanced heat transfer and power dissipation in oscillatory-flow tubes with circular-orifice baffles: A numerical study. Appl. Therm. Eng. 2018, 141, 494–502. [Google Scholar] [CrossRef]
- Solano, J.; Herrero, R.; Espín, S.; Phan, A.; Harvey, A. Numerical study of the flow pattern and heat transfer enhancement in oscillatory baffled reactors with helical coil inserts. Chem. Eng. Res. Des. 2012, 90, 732–742. [Google Scholar] [CrossRef]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method; Pearson Education Limited: London, UK, 2007. [Google Scholar]
- McDonough, J.; Ahmed, S.; Phan, A.; Harvey, A. The development of helical vortex pairs in oscillatory flows—A numerical and experimental study. Chem. Eng. Process.-Process. Intensif. 2019, 143, 107588. [Google Scholar] [CrossRef]
- Nogueira, X.; Taylor, B.J.; Gomez, H.; Colominas, I.; Mackley, M.R. Experimental and computational modeling of oscillatory flow within a baffled tube containing periodic-tri-orifice baffle geometries. Comput. Chem. Eng. 2013, 49, 107588. [Google Scholar] [CrossRef]
- Wang, J.; Li, Y.; Liu, X.; Shen, C.; Zhang, H.; Xiong, K. Recent active thermal management technologies for the development of energy-optimized aerospace vehicles in China. Chin. J. Aeronaut. 2020, 34, 1–27. [Google Scholar] [CrossRef]
- McDonough, J.; Oates, M.; Law, R.; Harvey, A. Micromixing in oscillatory baffled flows. Chem. Eng. J. 2018, 361, 508–518. [Google Scholar] [CrossRef]
- Munoz-Camara, J.; Crespi-Llorens, D.; Solano, J.P.; Vicente, P.G. Experimental analysis of flow pattern and heat transfer in circular-orifice baffled tubes. Int. J. Heat Mass Transf. 2020, 147, 118914. [Google Scholar] [CrossRef]
- Muñoz-Cámara, J.; Crespí-Llorens, D.; Solano, J.; Vicente, P. Baffled tubes with superimposed oscillatory flow: Experimental study of the fluid mixing and heat transfer at low net Reynolds numbers. Exp. Therm. Fluid Sci. 2020, 123, 110324. [Google Scholar] [CrossRef]
- Phan, A.N.; Harvey, A.P.; Rawcliffe, M. Continuous screening of base-catalysed biodiesel production using new designs of mesoscale oscillatory baffled reactors. Fuel Process. Technol. 2011, 92, 1560–1567. [Google Scholar] [CrossRef]
- Howes, T.; Mackley, M.; Roberts, E. The simulation of chaotic mixing and dispersion for periodic flows in baffled channels. Chem. Eng. Sci. 1991, 46, 1669–1677. [Google Scholar] [CrossRef]
- Muñoz-Cámara, J.; Solano, J.; Pérez-García, J. Non-dimensional analysis of experimental pressure drop and energy dissipation measurements in Oscillatory Baffled Reactors. Chem. Eng. Sci. 2022, 262, 118030. [Google Scholar] [CrossRef]
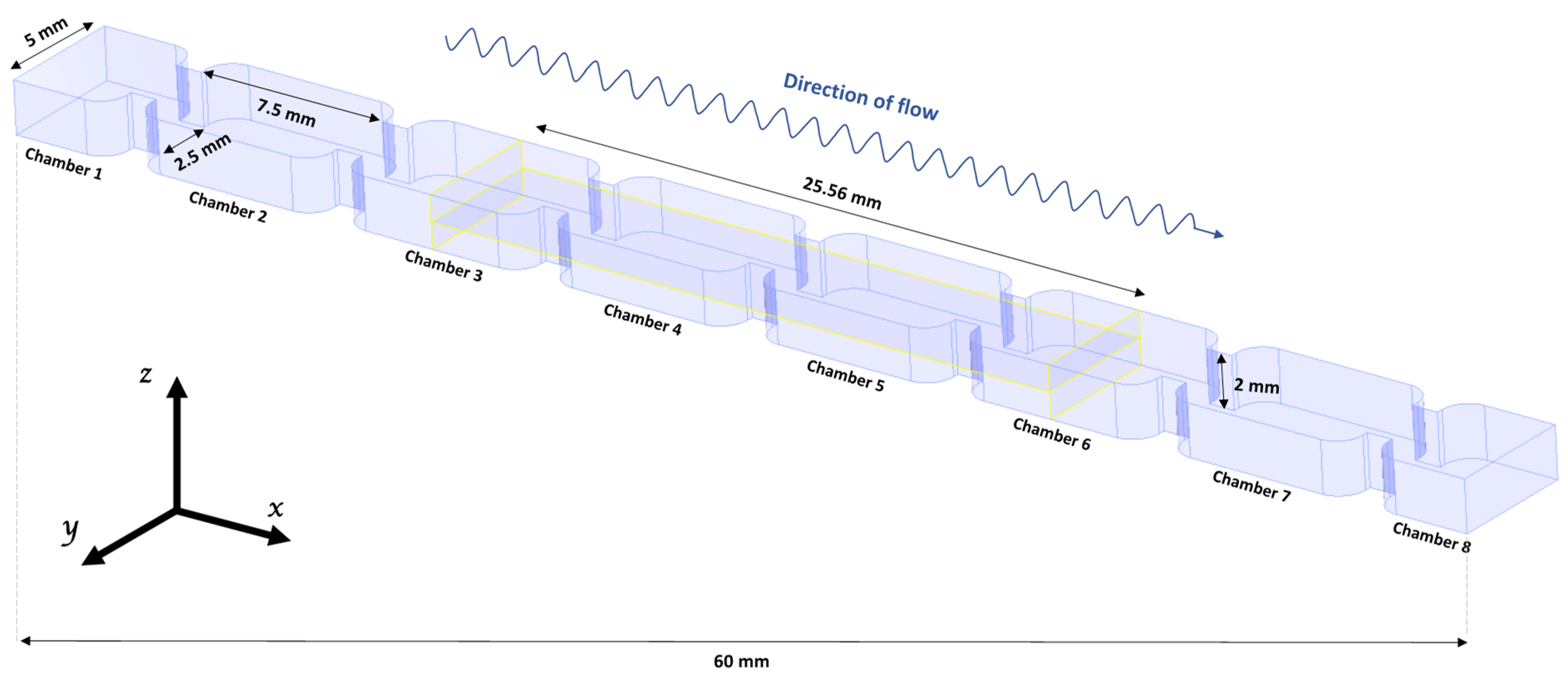




| Inlet Fluid Temperature (°C) | 5 |
| Wall Temperature (°C) | 60 |
| Initial System Temperature (°C) | 5 |
| Working Fluid | Water |
| Mesh Number | Inflation Layers | Element Size (mm) | Channel Volume (mm3) | Number of Elements | Elements per mm3 |
|---|---|---|---|---|---|
| 1 | 0 | 0.28 | 552.28 | 30,056 | 54.42 |
| 2 | 5 | 0.75 | 552.28 | 43,068 | 77.98 |
| 3 | 10 | 0.3 | 552.28 | 128,362 | 232.42 |
| 4 | 10 | 0.18 | 552.28 | 389,504 | 705.27 |
| 5 | 10 | 0.15 | 552.28 | 570,812 | 1033.56 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hockaday, J.; Law, R. Enhancing Heat Transfer in Mini-Scale Liquid-Cooled Heat Sinks by Flow Oscillation—A Numerical Analysis. Energies 2024, 17, 2459. https://doi.org/10.3390/en17112459
Hockaday J, Law R. Enhancing Heat Transfer in Mini-Scale Liquid-Cooled Heat Sinks by Flow Oscillation—A Numerical Analysis. Energies. 2024; 17(11):2459. https://doi.org/10.3390/en17112459
Chicago/Turabian StyleHockaday, James, and Richard Law. 2024. "Enhancing Heat Transfer in Mini-Scale Liquid-Cooled Heat Sinks by Flow Oscillation—A Numerical Analysis" Energies 17, no. 11: 2459. https://doi.org/10.3390/en17112459
APA StyleHockaday, J., & Law, R. (2024). Enhancing Heat Transfer in Mini-Scale Liquid-Cooled Heat Sinks by Flow Oscillation—A Numerical Analysis. Energies, 17(11), 2459. https://doi.org/10.3390/en17112459






