Impact of K-H Instability on NO_x Emissions in N₂O Thermal Decomposition Using Premixed CH₄ Co-Flow Flames and Electric Furnace

Abstract

This study systematically investigates the formation of NO_x in the thermal decomposition of N₂O, focusing on the impact of Kelvin–Helmholtz (K-H) instability in combustion environments. Using premixed CH₄ co-flow flames and an electric furnace as distinct heat sources, we explored NO_x emission dynamics under varying conditions, including reaction temperature, residence time, and N₂O dilution rates (X_N2O). Our findings demonstrate that diluting N₂O around a premixed flame increases flame length and decreases flame propagation velocity, inducing K-H instability. This instability was quantitatively characterized using Richardson and Strouhal numbers, highlighting N₂O’s role in augmenting oxygen supply within the flame and significantly altering flame dynamics. The study reveals that higher X_N2O consistently led to increased NO formation independently of nozzle exit velocity (u_jet) or co-flow rate, emphasizing the influence of N₂O concentration on NO production. In scenarios without K-H instability, particularly at lower u_jet, an exponential rise in NO₂ formation rates was observed, due to the reduced residence time of N₂O near the flame surface, limiting pyrolysis effectiveness. Conversely, at higher u_jet where K-H instability occurs, the formation rate of NO₂ drastically decreased. This suggests that K-H instability is crucial in optimizing N₂O decomposition for minimal NO_x production.

1. Introduction

Since the establishment of the United Nations Framework Convention on Climate Change (UNFCCC) in 1992, the world has united to confront the escalating issue of global warming. The International Conference on Parallel Processing (ICPP) recently advocated for a substantial reduction in global greenhouse gas emissions, targeting a minimum 45% decrease by 2030 relative to 2010 levels. This initiative aims to cap the global average temperature increase to 1.5 degrees Celsius by the year 2100. In response, leading global entities, including the European Union (EU), the United States, China, and Japan, have established their own specific emissions reduction goals. In line with these efforts, South Korea declared its 2030 National Greenhouse Gas Reduction Target in October 2020, seeking to achieve a 37% reduction from “business as usual” (BAU) trajectories [1].

Greenhouse gases refer to specific gases that absorb or reflect infrared radiation emanating from the Earth’s surface into space, thereby raising the Earth’s surface temperature. According to the Kyoto Protocol, major anthropogenic greenhouse gases such as carbon dioxide (CO₂), methane (CH₄), nitrous oxide (N₂O), hydrofluorocarbons (HFCs), perfluorocarbons (PFCs), and sulfur hexafluoride (SF₆) are subject to regulation [2]. Among these, N₂O ranks third in emission volume following CO₂ and CH₄ and possesses a global warming potential 310 times greater than that of CO₂ [3,4,5,6]. N₂O, the most chemically stable of these gases, has an atmospheric life span of approximately 150 years, enabling it to reach the stratosphere with minimal degradation. In the stratosphere, N₂O undergoes photodissociation or reacts with atomic oxygen (O), forming nitric oxide (NO), which subsequently participates in a catalytic cycle of ozone (O₃) depletion [7,8]. The reaction pathway is as follows:

N₂O + O → 2NO,

(1)

NO + O₃ → NO₂ + O₂,

(2)

NO₂ + O → NO + O₂.

(3)

In addressing the challenge of reducing N₂O emissions, thermal decomposition emerges as a particularly promising approach among various technologies [9,10,11,12,13,14,15]. This process is governed by a series of critical chemical reactions, crucial for understanding the N₂O thermal decomposition mechanism:

N₂O (+M) ⇌ N₂ + O (+M),

(4)

N₂O + O ⇌ N₂ + O₂,

(5)

N₂O + O ⇌ 2NO,

(6)

NO₂ + O ⇌ NO + O₂,

(7)

NO + O + M ⇌ NO₂ + M,

(8)

NO₂ + O ⇌ NO + O₂.

(9)

In these equations, “M” signifies a third body that aids in the decomposition process. The predominance of reactions (4) and (5) at elevated temperatures plays a significant role in effectively diminishing N₂O concentrations. However, a crucial aspect of this mechanism is the potential formation of NO_x, as delineated by reactions (6) to (9). This underlines the importance of maintaining controlled environmental conditions to suppress NO_x generation during the N₂O decomposition. Addressing these complexities is essential for the effective reduction of N₂O, especially given the intricate interplay of variables in the reaction environment.

Recent studies have underscored the profound influence of temperature on the decomposition efficiency of nitrous oxide (N₂O). For instance, research indicates that a rise in temperature from 820 °C to 880 °C leads to a significant reduction in N₂O emissions [16]. In the realm of oxy-combustion, an increased oxygen concentration has been identified as a crucial factor in enhancing the breakdown of N₂O [17]. The core reaction in N₂O decomposition is a third-body reaction, where gases like N₂, O₂, CO₂, and H₂O act as catalysts, accelerating the process [18]. This reaction has shown increased efficiency under diverse atmospheric conditions, especially in CO₂ and N₂ environments [19,20]. These findings highlight the critical role of atmospheric composition in optimizing N₂O decomposition, particularly at higher temperatures. However, there remains a notable gap in the literature regarding the quantitative analysis of NO_x production from the N₂O thermal decomposition process, particularly concerning reactions (6) to (9). This gap signifies a pressing need for further research to understand and quantify NO_x emissions arising from N₂O decomposition under various conditions.

Meanwhile, thermal decomposition processes are primarily categorized by their heat sources: electric furnaces [9,10,11], plasma [12,13], and combustion [14,15]. Although using an electric furnace as the heat source offers operational simplicity and lower technical demands, it incurs higher maintenance costs. On the other hand, plasma-based decomposition, while maintaining a lower overall temperature of the fluid medium, can generate localized reaction temperatures as high as 10,000 °C, leading to a significant production of thermal NO_x [21]. However, combustion as a heat source is distinguished in terms of processing efficiency, power requirements, operability, and maintenance costs, especially in sectors other than NO_x emissions, thus positioning it as the most effective technology for N₂O reduction. Recent research [10,22,23] underscores the impact of the mixture’s residence time and reaction temperature on the emission characteristics of N₂O and NO_x, highlighting the necessity for detailed analytical data on nitrogen oxide reaction processes in the practical implementation of high-temperature thermal decomposition technology.

In the case of using combustion as a heat source, the intrinsic combustion instability [24,25,26,27,28,29,30,31] of flames presents challenges for stable thermal energy supply. This is particularly problematic when considering the decomposition of N₂O, as such instabilities negatively impact the efficiency and stability of the reaction. Among these, Kelvin–Helmholtz (K-H) instability, commonly found in jet flames, induces significant changes in the combustion field due to its large amplitude. This type of instability originates from convective disturbances at the laminar flame front and is known to generate oscillating frequencies between 10 Hz and 20 Hz, influenced by varying burner sizes, flow rates, and fuel compositions [26,27,28,29]. Research by Chen et al. [30] employed the Mie scattering technique to elucidate the vortex structures surrounding flickering diffusion flames. Their analysis suggests that buoyancy within hot combustion gases amplifies axial flow velocities, which in turn leads to the formation of shear layers between the flame and surrounding air, complicating consistent control over the N₂O decomposition reaction. To overcome these issues, development of controllable burner technologies and flame stabilization mechanisms is necessary.

When using an electric furnace as the heat source, unlike combustion reactions, there are limitations for commercial applications due to its higher maintenance costs and inability to handle large volumes of gas. However, an electric furnace offers advantages such as ensuring uniform temperature of reactants and precise residence times. It also allows experiments across various temperature ranges, which is why it was commonly adopted as experimental equipment in prior research [32] that utilized thermal decomposition. Therefore, an electric furnace can be considered a highly useful tool for validating reaction mechanisms derived from N₂O thermal decomposition processes that employ combustion as the heat source.

In this study, we aim to focus on combustion as a heat source among various N₂O treatment technologies utilizing thermal decomposition, with a particular interest in the relationship between the decomposition rate of N₂O and NO_x emissions in the presence or absence of combustion instability. Specifically, our primary objective is to establish a correlation between the emissions of NO and NO₂ within combustion zones where K-H instability occurs. Additionally, to support our hypothesis regarding NOx emissions under conditions of K-H instability, we plan to conduct parallel analyses using an electric furnace as an alternative heat source, examining the trends of NO and NO₂ emissions in relation to reaction temperature. This approach is expected to provide empirical evidence supporting the connection between K-H instability and NO_x emissions.

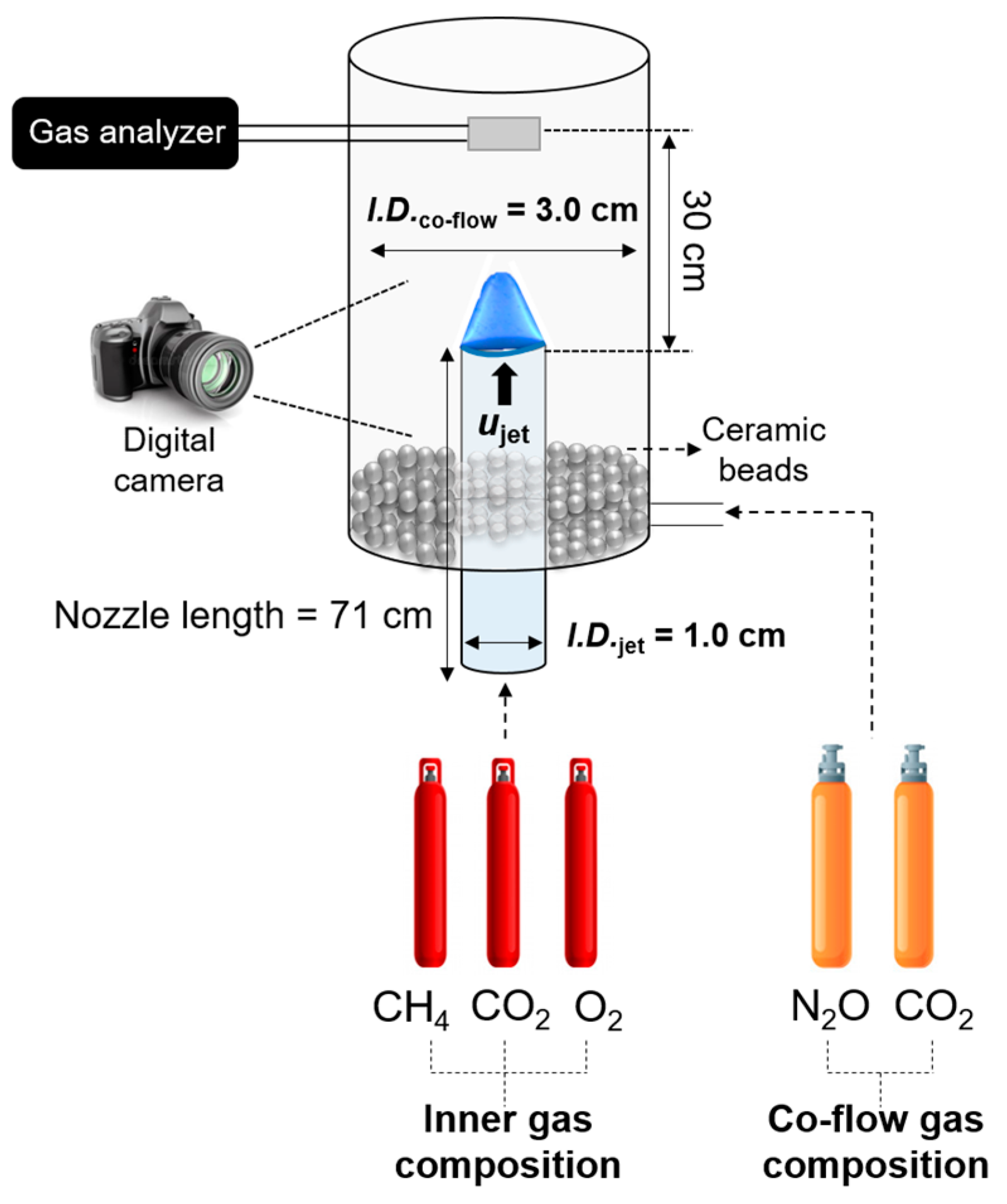
2. Experimental Methodology and Conditions

In our study of N₂O thermal decomposition, we have employed two distinct heat sources: premixed CH₄ Co-Flow Flames and an electric furnace.

Premixed CH₄ Co-Flow Flames: As depicted in Figure 1, our experimental setup is designed to explore the thermal decomposition characteristics of N₂O using a premixed flame. The co-flow burner employed in our experiment has internal and external diameters measuring 1 cm and 3 cm, respectively. The internal nozzle, extending to a length of 71 cm, is constructed of stainless steel to ensure a stable premixed gas flow. The co-flow tube, fashioned from acrylic, allows for internal observation. The central internal nozzle, acting as the primary heat source, facilitates high-temperature decomposition of N₂O, injected through the co-axial tube, establishing flame attachment.

The premixed gas mixture, detailed in

Table 1. Tested gas composition.

CH4 [%]	O2 [%]	CO2 [%]	$\mathit{\Phi }$	Tb [K]
10	19	71	1	1762

, comprises 10% CH₄, 19% O₂, and the balance of 71% CO₂ as a diluent. We set Φ = 1, aiming to prevent any additional reaction of unburned oxygen with N₂O in the downstream flame, which could potentially result in NO_x formation. To assess the N₂O reduction effect in relation to flame size, we varied the nozzle exit velocity (u_jet) at 55, 55, and 60 cm/s. The co-axial flow, incorporating CO₂ as the primary gas, diluted N₂O to concentrations of 0, 20%, 25%, and 30%. Additionally, to evaluate residence time efficiency, co-flow rates were established at 0.84, 0.88, and 0.92 L/min.

For gas supply control, a mass flow controller, calibrated by a gas calibrator, was utilized. Combustion gases emitted from the downstream flame were analyzed for concentrations of NO, NO₂, O₂, and CO₂ using a gas analyzer (ECOM, MK 6000+). Here, the gas analyzer was installed 30 cm downstream from the nozzle. The contribution to NO_x emissions, as measured by the gas analyzer, was attributed exclusively to the N₂O injected via the co-axial flow, as all gases supplied to the reactor were devoid of nitrogen. Flame imagery was captured at 30 fps using a digital camcorder (Sony, HDR-PJ675).

Electric furnace: In addition to experiments using combustion reactions as the heat source, our study also adopted an electric furnace as a means of thermal decomposition of N₂O. This approach was taken to introduce an electric furnace that ensures uniform reaction temperatures and precise residence times of reactants, thereby revalidating inferences about NO_x emission characteristics derived from the N₂O decomposition process in combustion reactions.

Figure 2 shows the setup, featuring an 850 mm-long, 10 mm-inner diameter alumina tube within the electric furnace. Internal coils, positioned at 100 mm intervals around the tube, create a 200 mm high-temperature pyrolysis zone from the center towards both inlet and outlet. This setup allows precise temperature control using internal coils and adjustable gas flow for reaction time calculations. Additionally, to suppress heat loss from the pyrolysis zone to the outside, the tube is wrapped with ceramic fiber. The length of this insulation section is 350 mm, which is greater than the 200 mm high-temperature pyrolysis zone. Five reaction temperatures (1100 K to 1500 K) and three residence times (1, 2, and 4 s) were selected. The rationale behind these choices is discussed in Section 3.4.1.

The experimental gas mixture simulated ambient conditions: N₂ (71.1%), O₂ (18.9%), and N₂O (100,000 ppm). Gases entered the reactor via a mass flow controller (MFC) and effluent gas was analyzed using combustion gas analysis systems: a TESTO 350 MARITIME and Fourier-transform infrared gas analyzer (I1801-E, Midac, Redford, MI, USA) at the Eco-friendly Shipbuilding Core Research Support Center. The nitrogen oxides’ measurable range is NO₂ up to ~500 ppm and NO up to ~3000 ppm. Expecting effluent gas NO_x concentrations to exceed this range, we cooled and diluted it using Argon (Ar) in a dilution tube equipped with ceramic beads and mesh for uniform gas flow. Silica gel removed moisture, ensuring dry measurement conditions.

Each residence time’s gas flow rate was calculated using Equation (10):

$$Q=\frac{\pi \times {\left(\frac{D}{2}\right)}^2\times L}{t}$$

(10)

where Q is the flow rate, D the alumina tube’s inner diameter, L its length, and t the residence time. Charles’s law was applied to maintain constant residence time across different reaction temperatures:

$$\frac{Q_1}{T_1}=\frac{Q_2}{T_2}$$

(11)

where Q₁ is the input flow rate, T₁ the input temperature, Q₂ the flow rate at each residence time, and T₂ the reaction temperature.

3. Results and Discussion

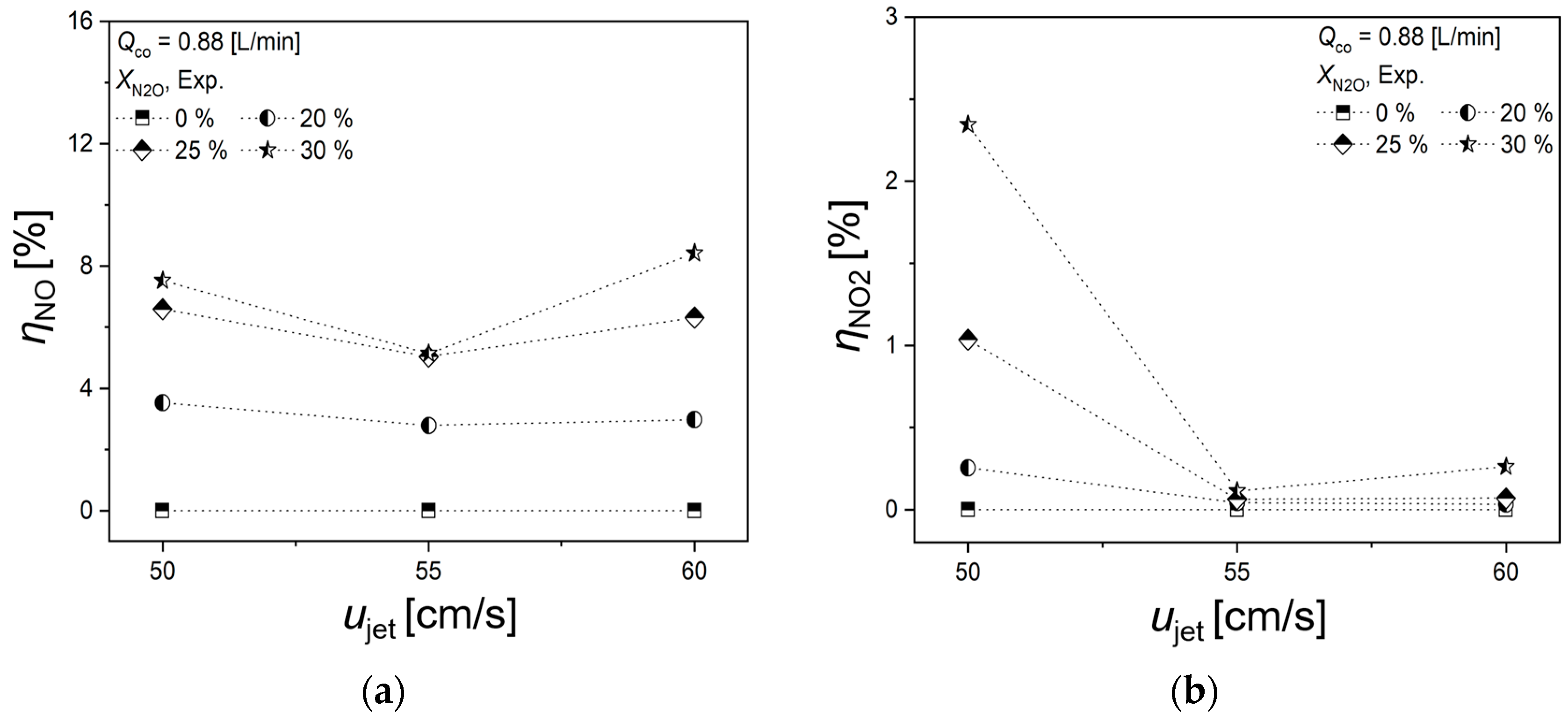
3.1. Effects of Nozzle Exit Velocity: Premixed CH₄ Co-Flow Flames

Figure 3a depicts flame images as a function of u_jet with varying N₂O dilution rates (X_N2O) injected into the co-flow, and the flame images were captured using a digital camera. The co-flow rate used in Figure 3a was fixed at 0.88 L/min. As evident from the figure, an increase in X_N2O and u_jet results in the elongation of the flame length.

In the context of premixed flames, the location of the flame front is typically determined by the point at which the flame propagation velocity (S_f) aligns with u_jet [33]. For the methane premixed gas utilized in our experiment, the theoretical laminar burning velocity (S_L) is approximately 4 cm/s. To form an attached flame, u_jet needs to be maintained above this threshold. Practically, as the premixed gas is ejected from the nozzle, its velocity diminishes due to momentum loss. Consequently, the flame is positioned at the point where the flame propagation velocity and the flow velocity reach equilibrium, resulting in the formation of a triangular combustion reaction zone. If u_jet falls below the flame propagation velocity, flashback can occur, a phenomenon characteristic of premixed flame propagation dynamics.

Figure 3b illustrates the flame propagation velocity as a function of u_jet and X_N2O. Consistent with premixed flame behavior, we observe that longer flame lengths correspond to slower flame propagation velocities. The methodology for calculating flame propagation velocity is illustrated in the top right of Figure 3b, with the corresponding equation provided as follows:

S_f = u_jet × sin α

(12)

Even when maintaining consistent nozzle exit velocities in premixed flames, an increase in the X_N2O leads to longer flame lengths and slower flame propagation velocities, as shown in Figure 3b. This behavior is attributed to the impact of N₂O reduction, which facilitates the generation of additional oxygen atoms.

In premixed flames, typically, there is only a singular premixed flame branch, in contrast to diffusion flames, which exhibit a triple-flame structure (comprising rich premixed, lean premixed, and diffusion flames) [26,27]. However, in scenarios where the external environment contains oxidizers, the diffusion flame’s tail region can manifest downstream of the premixed flame [34]. This occurs due to the diffusion-driven mass transfer process near the combustion reaction zone. Consequently, with the supply of N₂O into the co-flow, additional oxygen atoms are produced through Equations (4) and (5). As a result, the local equivalence ratio near the flame tip drops below unity. This delay in the flame propagation velocity extends the flame length [34].

Figure 4 illustrates the variation in NO and NO₂ formation rates with respect to u_jet, and the co-flow rate is 0.88 L/min. The formation rates of NO and NO₂ were derived through the following equations.

$${\eta }_{\mathrm{N}\mathrm{O}}=\frac{\mathrm{N}{\mathrm{O}}_{out}}{2{\mathrm{N}}_2{\mathrm{O}}_{in}}\times 100\%$$

(13)

$${\eta }_{\mathrm{N}{\mathrm{O}}_2}=\frac{\mathrm{N}{\mathrm{O}}_{2out}}{2{\mathrm{N}}_2{\mathrm{O}}_{in}}\times 100\%$$

(14)

Equations (13) and (14) represent the conversion ratios of NO and NO₂ according to the N₂O injection rate, where “in” and “out” denote the combustion chamber inlet and outlet, respectively.

Upon analyzing Figure 4a, it is noted that the rate of NO generation remains largely unchanged across various u_jet, with its dependence primarily on X_N2O. Particularly for X_N2O = 25% and 30%, there is an insignificant variation in the NO formation rates. In contrast, the NO₂ formation rate exhibits a distinct behavior: at u_jet = 55 and 60 cm/s, the NO₂ production is minimal, whereas at u_jet = 50 cm/s, the NO₂ formation rate escalates in correlation with X_N2O. However, when comparing the NO and NO₂ formation rates, it is apparent that the production of NO is substantially higher.

The relatively lower NO₂ formation rate, compared to NO, is attributed to the rapid formation of thermal NO_x in high-temperature fluid media, typically above 1300 °C [10,21]. The produced NO_x predominantly consists of NO, as NO₂ tends to decompose into NO and O₂ at temperatures above 650 °C, as illustrated by the reaction:

2NO₂ → 2NO + O₂

(15)

Therefore, when N₂O, injected into the co-flow, interacts with the high-temperature flame surface, it decomposes into nitrogen molecules and oxygen atoms. If these decomposed species linger in the high-temperature environment, they further break down into NO and nitrogen atoms, thereby augmenting NO production. As shown in Figure 4, an increase in N₂O injection rate leads to a higher concentration of N₂O interacting with the high-temperature flame surface, which, in turn, escalates the NO generation rate.

Moreover, it is observed that at X_N2O = 25% and 30%, the N₂O reduction due to heat release from the premixed flame reaches a saturation point. Consequently, this indicates the presence of a threshold in X_N2O, beyond which the NO conversion rate reaches saturation, leading to minimal differences in NO formation rates at these higher dilution levels.

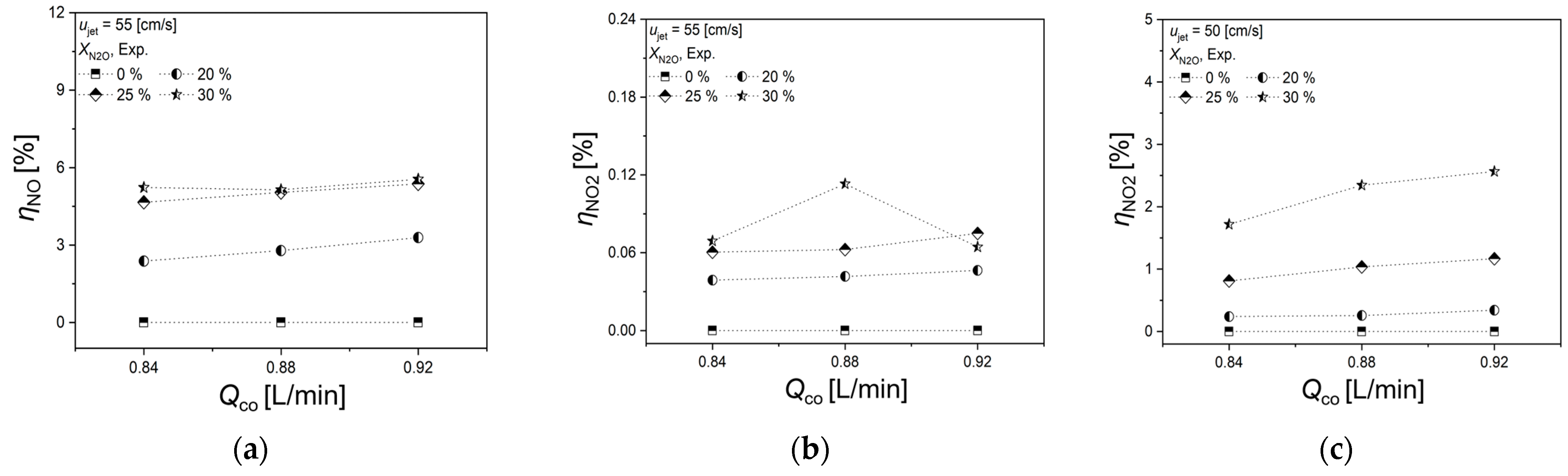
3.2. Effects of Co-Flow Rate: Premixed CH₄ Co-Flow Flames

Figure 5a,b illustrate the variation in NO and NO₂ formation rates in the N₂O reduction process, particularly at u_jet = 55 cm/s, and its dependence on the co-flow rate. Figure 5a shows that the NO formation rate increases consistently with X_N2O, regardless of the co-flow rate. Significantly, it is observed that at X_N2O exceeding 25%, the NO formation rate attains a saturation point, resulting in consistent formation rates at these elevated dilution levels.

Similarly, Figure 5b demonstrates that the NO₂ formation rate also increases with X_N2O, irrespective of the co-flow rate. Contrasting with the general trend, the highest NO₂ generation is observed at X_N2O = 30% and Q_co = 0.88 L/min. However, given that the NO₂ formation rate remains below 0.15% consistently, this increase may not be statistically significant. Finally, Figure 5c specifically examines the scenario at u_jet = 50 cm/s, which exhibited the highest NO₂ formation rate. Here, the NO₂ formation rate remains below 1% for all conditions, except at X_N2O = 30%, where it shows an increasing trend with the rise in co-flow rate. A more detailed analysis of the NO₂ formation rate, as inferred from Figure 5c, will be discussed in the subsequent sections.

In summary, from the experimental findings discussed in Section 3.1 and Section 3.2, it is evident that the NO formation rate increases with the X_N2O, regardless u_jet or Q_co. However, for NO₂ generation, except in the case of u_jet = 50 cm/s, the production of NO₂ is generally minimal.

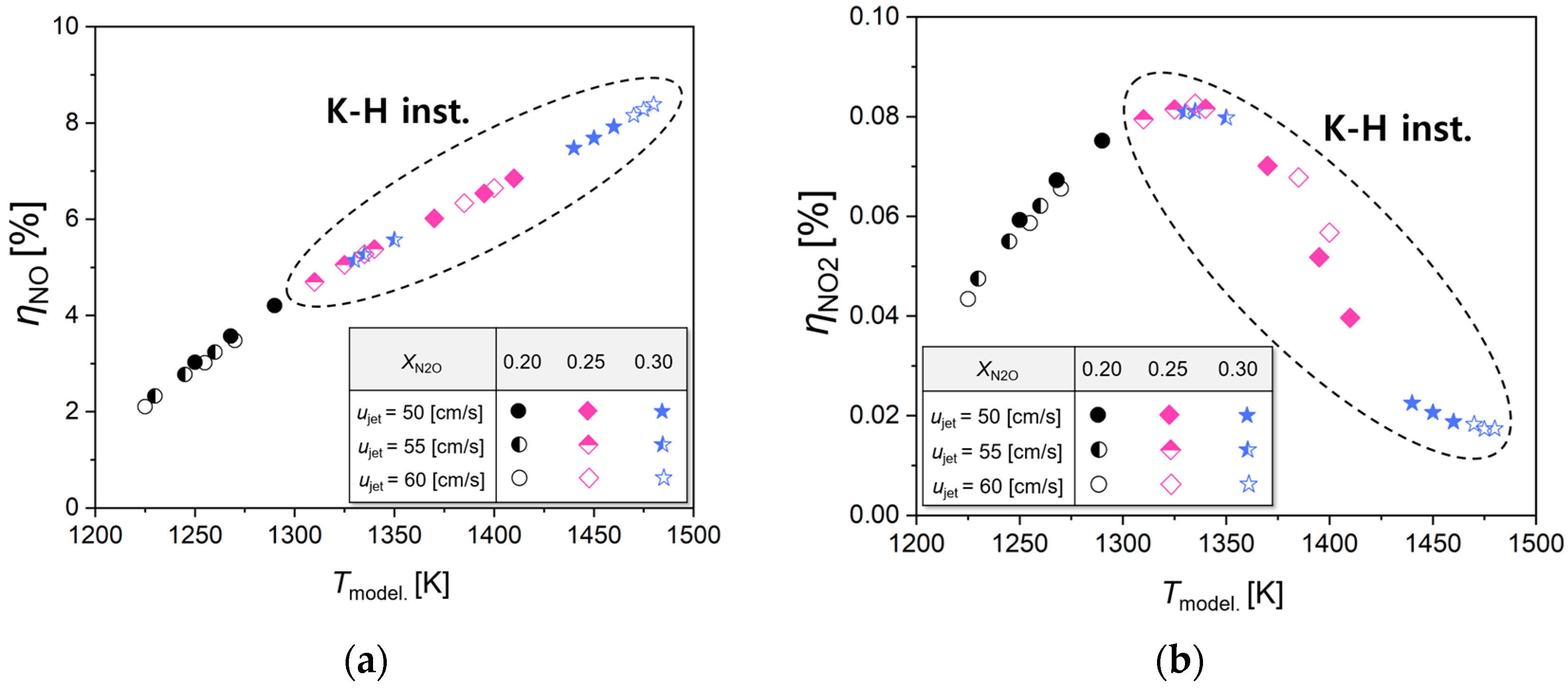
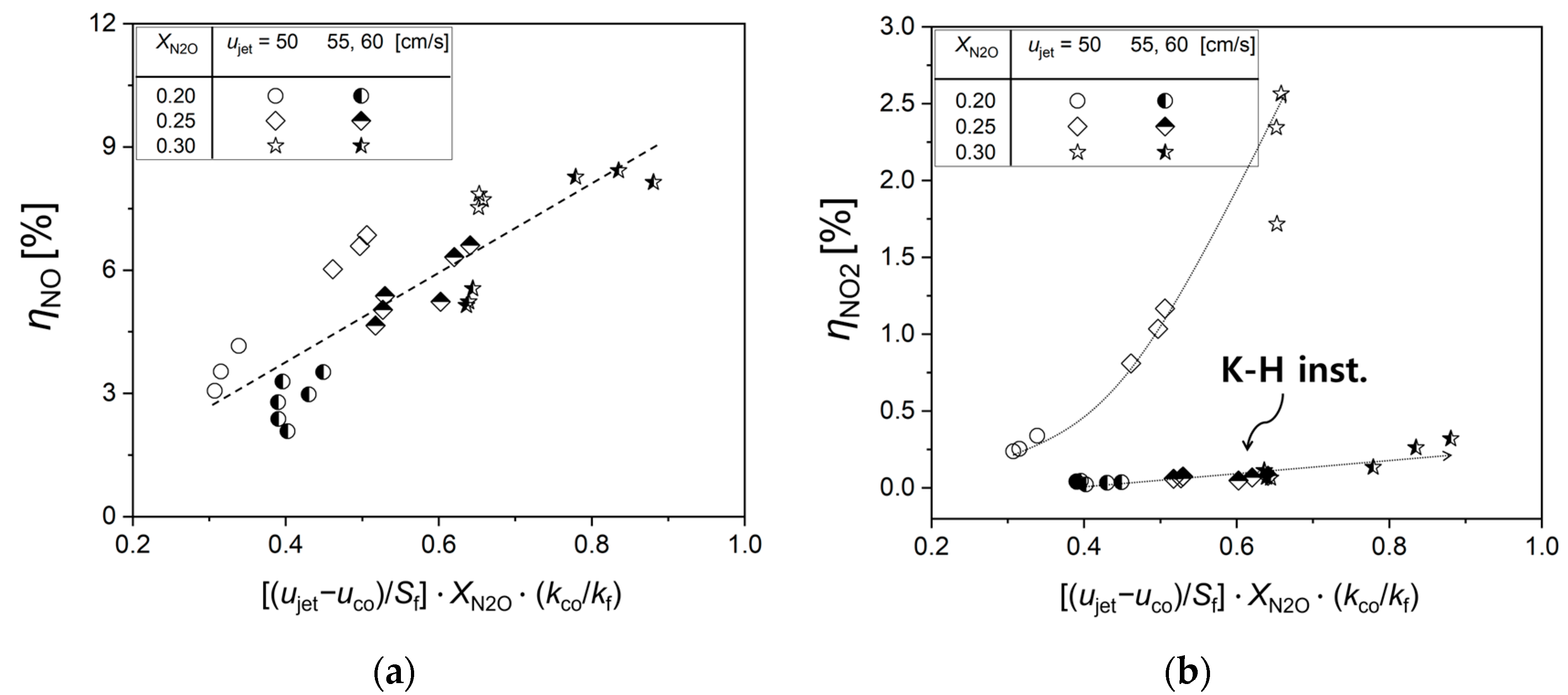
3.3. Correlation between NO_x Formation Rate and K-H Instability: Premixed CH₄ Co-Flow Flames

Figure 6 presents the conversion of NO and NO₂ emission quantities to ppm for all conducted experimental cases to provide a relative comparison in the N₂O reduction process. The figure exhibits two distinct trends. Firstly, at nozzle exit velocities of 55 and 60 cm/s, the NO₂ emission quantity is negligible, irrespective of the NO emission quantity. On the other hand, at u_jet = 50 cm/s, it is observed that the NO₂ emission quantity proportionally increases in a square root function with the increase in NO emission quantity.

For a detailed understanding of the NO₂ formation mechanism at u_jet = 50 cm/s, flame dynamic behaviors were observed by recording with a digital camcorder. The observation revealed that, unlike at u_jet = 55 and 60 cm/s, consistent oscillations near the flame tip were observed at u_jet = 50 cm/s. Figure 7 presents the fast Fourier transform (FFT) analysis results for flame flickering at u_jet = 60 cm/s. With a derived oscillation frequency of 12.65 Hz at u_jet = 55 cm/s, Q_co = 0.88 L/min and X_N2O = 0.25%, it is attributed to the influence of K-H instability due to flame flickering.

K-H instability is known for convectional flow instability resulting from density differences between burned and the surrounding gases [24,25,26,27]. The combustion field is particularly prone to K-H instability as the density of burned gas in flames is typically six to seven times smaller than that of the unburned gas. According to previous studies on K-H instability, the characteristic oscillation frequency is in the range of 10–15 Hz [26,27]. Therefore, in the N₂O reduction process, the presence of K-H instability within the flame leads to instability in the co-flow field supplying N₂O to the flame surface. The high-temperature heat released from the flame causes accumulation near the nozzle due to recirculation, suppressing the NO₂ formation rate, which corresponds to relatively lower-temperature oxidation reaction.

Furthermore, according to previous research [9,35] on the influence of residence time in high-temperature flow fields, an increase in residence time in the mixing chamber within a high-temperature flow field is reported to decrease the optimal temperature for N₂O reduction. In this study, when K-H instability occurs in the chosen flame as the heat source, a flow structure resembling a vortex is formed near the flame surface. This formation increases the residence time of the surrounding N₂O, and the vortex-shaped flow enhances convective heat transfer from the flame to the surrounding medium. Consequently, the NO₂ generation rate corresponding to the relatively lower-temperature reactions naturally decreases. Therefore, selecting u_jet that induces K-H instability to form a recirculation structure in the surrounding medium is considered effective not only for N₂O reduction efficiency but also for reducing NO₂ generation.

Most previous studies [26,27,31,36,37] on K-H instability have focused on characterizing it using the Richardson number (Ri), which represents the ratio of buoyancy to shear flow, and the Strouhal number (St), which describes the characteristics of oscillating flow field. While the constants of the relational equation vary depending on the gas composition, mixing conditions, and the structure of the burner, it has been consistently observed that St is exponentially proportional to the Ri. Therefore, in this study, FFT analysis was conducted under u_jet = 55 and 60 cm/s, where K-H instability was observed. Figure 8a displays frequency changes according to the co-flow rate, with frequencies ranging from 7.5 to 12.5 Hz. Figure 8b, based on the derived frequencies, applies the Ri and St as suggested in previous studies, illustrating their correlation. Here, “f” represents frequency, “L_f” the flame length, “u_co” the co-flow velocity, “ρ_co” the density of the co-flow, “ρ_b” the density of burned gas, and “g” the gravitational acceleration. As shown, similar to prior studies, St is found to be proportional to Ri.

Here, we aim to physically evaluate the contributions of each variable that composes Ri in K-H instability. Firstly, the difference in density between the surrounding gas and the burned gas, represented as ρ_co − ρ_b, indicates that an increase in this density difference intensifies K-H instability. Additionally, the momentum of the jet, represented as u_jet − u_co, is included in the denominator of Ri as a square. This implies that at lower velocity differences, K-H instability is dominated by buoyancy, but at higher velocity differences, it is influenced by both the buoyancy and momentum of the jet, in line with previous research findings [36,37].

3.4. NO_x Emission Characteristics in Non-Combustion: Electric Furnace

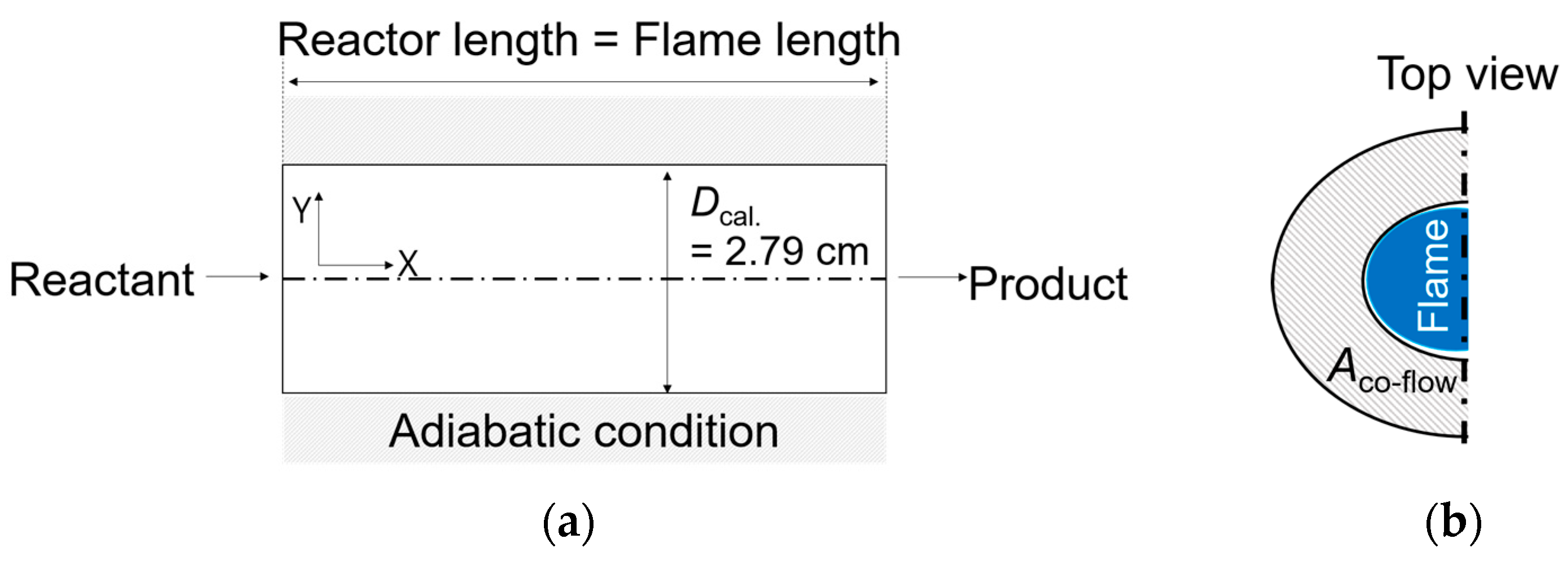
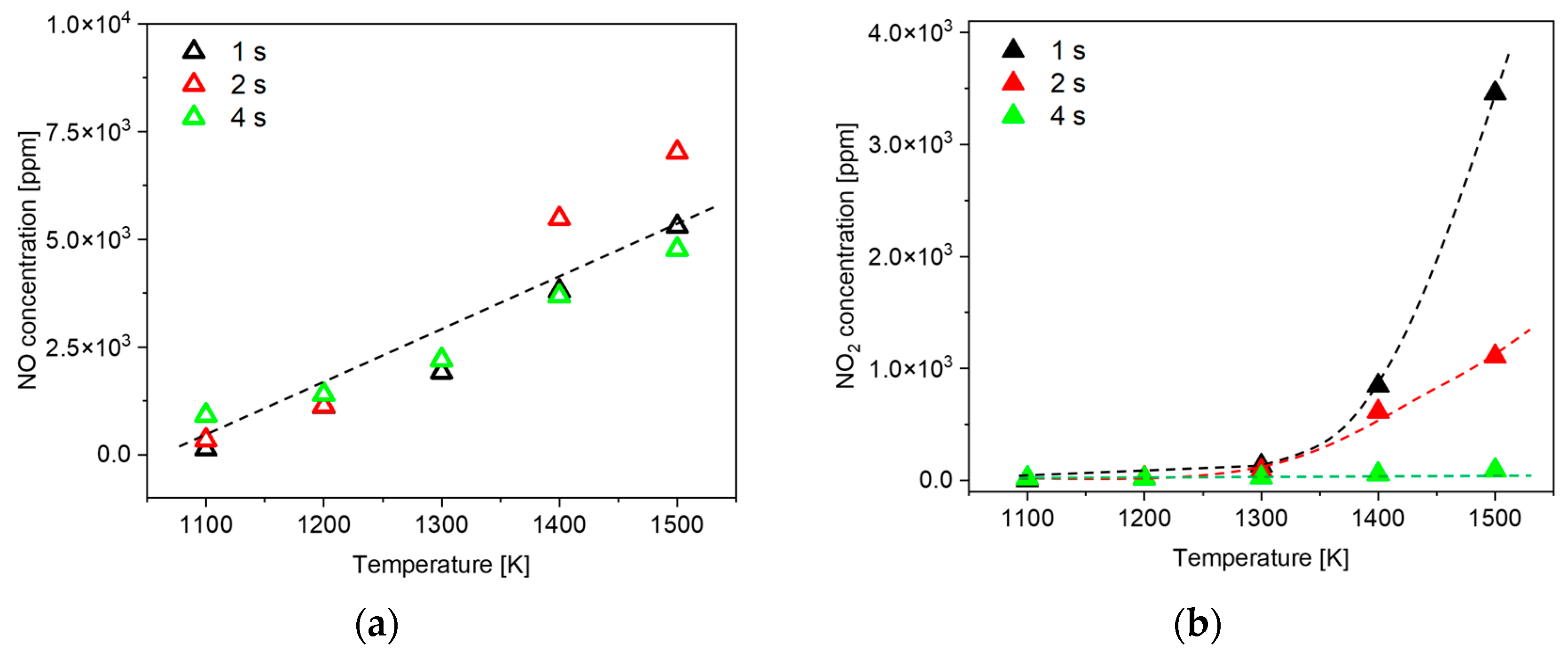
3.4.1. Determining Reaction Temperatures

In the context of our study, as elaborated in Section 3.3, the occurrence of K-H instability enhances the residence time within the recirculation zone, which subsequently increases the NO formation rate while diminishing the NO₂ formation rate. This section is dedicated to examining the formation of NO_x as a result of N₂O reduction in an electric furnace, distinctively absent of combustion reactions. Firstly, a numerical analysis simulating the co-flow flame was conducted to set a reaction temperature in the electric furnace similar to that in the combustion experiments. The numerical analysis utilized a one-dimensional reaction structure analysis model, the Plug Flow Reactor (PFR), integrated into the ANSYS Chemkin-Pro 2022 software [38], and calculations were performed using the GRI 3.0 reaction mechanism [39].

Figure 9a presents the schematic for the computational domain. The top view of the simulated coaxial burner, depicted in Figure 9b, shows N₂O and diluents being emitted from the area labeled “A_co-flow,” which forms an annulus region. For PFR model application, a circle with a diameter of D_cal = 2.79 cm, corresponding to this annulus area, was chosen. The reactor’s length was set to align with the observed flame length. The reactants, mirroring the composition used in the co-flow flame experiment, were a mixture of CO₂ as the primary gas, diluted N₂O to concentrations of 0, 20%, 25%, and 30%, and the co-flow rates were maintained at 0.84, 0.88, and 0.92 L/min. The reactor wall was maintained under adiabatic conditions to simulate realistic thermal behavior. The primary objective of this calculation was to ascertain the reaction temperature for N₂O decomposition within the co-flow flame setup. This was achieved by aligning the experimental NO emission data with the calculated NO values; the corresponding wall temperature at this intersection was then established as the reaction temperature, “T_model,” for N₂O decomposition.

Figure 10 displays the transformation rates of NO and NO₂ at varying calculated reaction temperatures. It is observed that NO emissions escalate with an increase in reaction temperatures, while the emission of NO₂ reaches its zenith around 1325 K, subsequently diminishing as the reaction temperature further elevates. Importantly, the reduction in NO₂ emissions aligns with the presence of K-H instability. Delving into the reaction coefficients from the GRI 3.0 reaction mechanism employed in our computations reveals that the activation energy for the conversion of NO to NO₂ in reaction Equation (8) is 0 cal/mol, whereas for the reverse process from NO₂ to NO in reaction Equation (9), it is −240 cal/mol [39]. This implies that the oxidation of NO₂ to NO occurs more rapidly than its reduction from NO to NO₂, suggesting a predominant conversion of NOx to NO, especially under extended residence times prompted by K-H instability. We aim to corroborate this inference with experiments conducted in an electric furnace, as detailed in the next section.

3.4.2. Effects of Reaction Temperature and Residence Time

In this section, we conducted experiments using an electric furnace as the heat source, adopting reaction temperatures derived from simulations in Section 3.4.1. This approach aimed to validate our hypothesis, discussed in Section 3.3, where the characteristics of NO_x emissions vary with residence time. In the experiments utilizing the electric furnace, the gas mixture consisted of 71.1% N₂, 18.9% O₂, and a specific concentration of N₂O (100,000 ppm). The selected reaction temperatures ranged from 1100 K to 1500 K, increasing in increments of 100 K, covering the range deduced from Figure 10. To match the NO_x emission levels observed in the co-flow flame experiments (as shown in Figure 6), residence times of 1, 2, and 4 s were determined through iterative testing. Figure 11a,b display the concentrations of NO and NO₂ produced due to N₂O decomposition within the electric furnace. Remarkably, the generation of NO shows a linear increase with escalating reaction temperatures, exhibiting consistent results regardless of residence time, mirroring findings from the co-flow flame tests. In contrast, the NO₂ output demonstrates an exponential escalation at a residence time of 1 s, with reaction temperatures rising; however, it becomes significantly minimal at a residence time of 4 s. These results corroborate the observations from co-flow flame experiments and validate our hypothesis: K-H instability leads to diminished NO₂ emissions as residence time increases during the N₂O decomposition process.

3.5. Comprehensive Characterization of NO_x Emissions in the N₂O Thermal Decomposition Process

Here, we focus on elucidating the interplay between K-H instability and the emission characteristics of NO_x in the N₂O thermal decomposition process. Figure 12 elucidates the relationship between effective thermal conductivity and the formation rates of NO and NO₂. We propose a novel approach to define effective thermal conductivity, $K_{eff.}$, as a pivotal parameter influencing NO_x emissions in co-flow flame scenarios. This approach is represented by a formula expressed in terms of a dimensionless number:

$$K_{eff.}=\left[\frac{u_{\mathrm{j}\mathrm{e}\mathrm{t}}-u_{\mathrm{c}\mathrm{o}}}{S_f}\right]·X_{\mathrm{N}2\mathrm{O}}·\frac{k_{\mathrm{c}\mathrm{o}}}{k_f}$$

(16)

In this formula, the first term embodies a dimensionless velocity factor, represents the co-flow shear layer ($u_{\mathrm{j}\mathrm{e}\mathrm{t}}-u_{\mathrm{c}\mathrm{o}}$), normalized by the flame propagation velocity ($S_f$), which is related to K-H instability (refer to Figure 8b). The second term, representing the N₂O dilution concentration ($X_{\mathrm{N}2\mathrm{O}}$), provides the eigenvalue for the NO_x formation rates (refer to Figure 4 and Figure 5). The third term, representing normalized thermal conductivity, symbolizes the ratio of the thermal conductivity of the burned gases, which exerts the most dominant influence on N₂O thermal decomposition, to the thermal conductivity of the co-flow gas derived from the reaction temperatures established in Section 3.4.1. An increase in normalized thermal conductivity enhances heat transfer from the burned gas to the co-flow gas, thereby increasing the NO_x conversion rate (refer to Figure 11).

Figure 12a reveals that an elevation in effective thermal conductivity linearly escalates the NO formation rate. Contrastingly, the NO₂ formation rate exhibits two distinct trends contingent on the occurrence of K-H instability. In scenarios where K-H instability is not present, as at u_jet = 50 cm/s, an increment in effective thermal conductivity triggers an exponential rise in NO₂ formation rate. On the contrary, at u_jet = 55 and 60 cm/s, where K-H instability is present, the NO₂ formation rate experiences a linear, albeit gradual, increase. Below are the respective fitting equations for each scenario, with “R” indicating the correlation coefficient:

$${\eta }_{\mathrm{N}\mathrm{O}}=10.82·K_{eff.} (R=0.75),$$

(17)

$${\eta }_{\mathrm{N}\mathrm{O}2}=0.13·e^{4.70{·K}_{eff.}} (R=0.95),$$

(18)

$${\eta }_{\mathrm{N}\mathrm{O}2}=0.44·K_{eff.} (R=0.72).$$

(19)

Drawing from the detailed outcomes of this research, our findings are expected to significantly inform the development and operational strategies of combustion systems tailored for N₂O reduction processes. This entails designing and managing these systems to optimize their functionality while substantially lowering NO_x emissions. Particularly when adopting combustion as the heat source, the intrinsic combustion instability is inherently challenging to eliminate, thereby emphasizing that the recirculation structure of the flow field induced by combustion instability can modify the residence time of the reactants. Therefore, if the results of this study are applied from an engineering perspective, it can be inferred that using the instability characteristics represented by the Ri and St numbers proposed in our paper, the intensity of combustion instability can be quantitatively assessed. Subsequently, through $K_{eff.}$, the emission rates of NO and NO₂ in the burner can be predicted. This aligns with our objective of enhancing the efficiency of N₂O decomposition mechanisms in a manner that concurrently addresses the environmental concerns associated with NO_x pollutants.

4. Conclusions

This comprehensive study has systematically explored the intricacies of NO_x formation in the N₂O thermal decomposition process, specifically examining the impact of Kelvin–Helmholtz (K-H) instability in a combustion environment. Utilizing both premixed CH₄ co-flow flames and an electric furnace as distinct heat sources, we have investigated the dynamics of NO_x emissions under various operational conditions, including reaction temperature, residence time, and N₂O dilution rates. The key findings derived from this comprehensive analysis are as follows.

(1)

Dynamic flame behavior: Diluting N₂O around a premixed flame leads to an increase in flame length and a decrease in flame propagation velocity, along with the induction of K-H instability. The observed K-H instability within the study was quantitatively characterized using the Richardson number and the Strouhal number. This demonstrates the crucial role of N₂O in augmenting oxygen supply within the flame and significantly altering the dynamics of flame behavior.

(2)

NO formation and N₂O dilution rates: Higher N₂O dilution rates consistently resulted in increased NO formation, independently of the nozzle exit velocity or co-flow rate. This underscores the significant impact of N₂O concentration on NO production in thermal decomposition processes.

(3)

NO₂ formation and K-H instability: In scenarios devoid of K-H instability, particularly at lower nozzle exit velocities (u_jet = 50 cm/s), we observed an exponential increase in NO₂ formation rates, attributable to the reduced residence time of N₂O near the flame surface, which in turn limits pyrolysis effectiveness. Conversely, at higher nozzle exit velocities, where K-H instability is suppressed, NO₂ formation rates exhibited a more gradual linear increase, suggesting that the occurrence of K-H instability is a critical factor in optimizing the N₂O decomposition process for minimal NO_x production.

(4)

Impact of effective thermal conductivity: A novel approach to define effective thermal conductivity showed that increases in this parameter linearly increased the NO formation rate. In contrast, the NO₂ formation rate varied depending on the presence or absence of K-H instability, demonstrating the critical role of thermal dynamics in NO_x emissions.

This research provides a comprehensive understanding of the factors influencing NO_x formation in the thermal decomposition of N₂O, particularly when combustion reactions are employed as the heat source. It highlights the inevitable impact of combustion instability on NO_x formation, offering crucial insights for the development of more efficient and environmentally friendly burners for N₂O reduction.