Potential Analysis and Feasibility Study on the Hydrothermal Utilization of Rivers—Using Marburg on the Lahn River as Case Study
Abstract
:1. Introduction
- : Thermal output in kW;
- : Density of water in kg/m3;
- : Specific heat capacity in kJ/(kg∙K);
- : Discharge of the river in m3/s;
- : Difference between original and changed water temperature in K.
- The macroscopic level, where the heat potential of the river section is considered (potential analysis);
- The microscopic level, where the usability of the river heat at the individual site is concretized (feasibility study);
- The economic level, where the feasibility of the supply targets is assessed on the heat sink side.
2. Materials and Methods
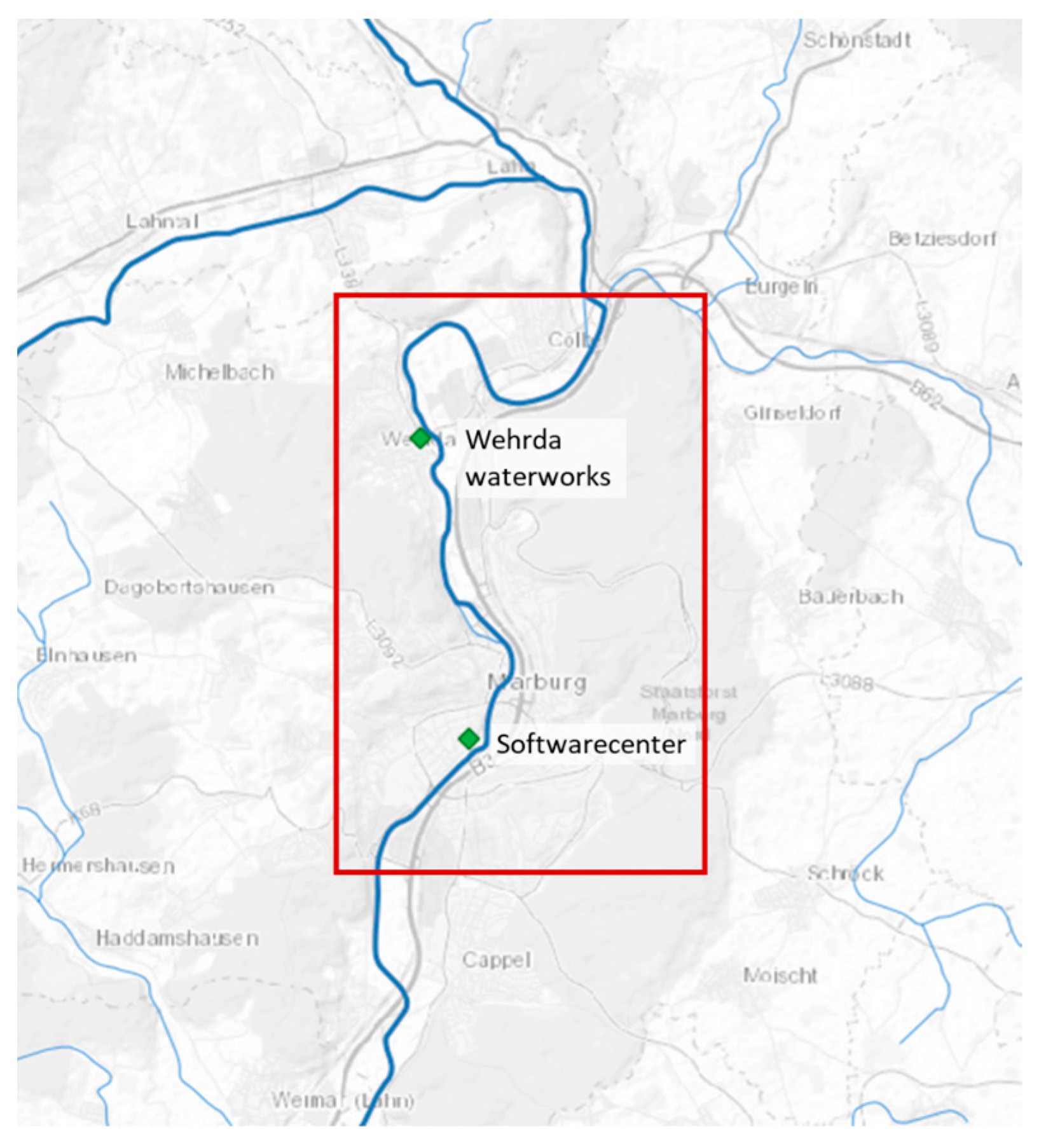
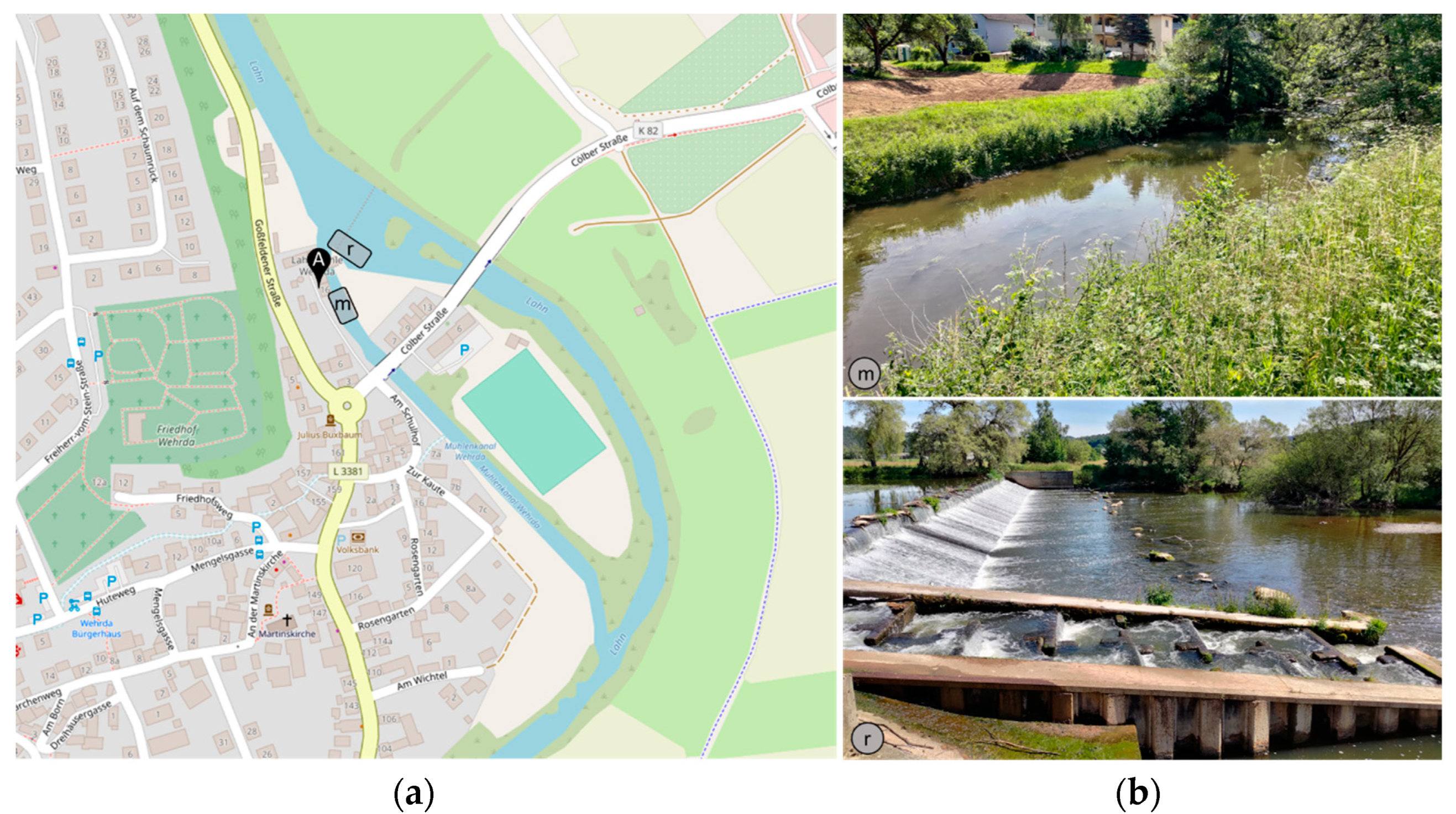
2.1. Case Example
2.2. Data Procurement
2.3. Statistical and Further Analysis Methods
2.3.1. Averaging the Measurement Data
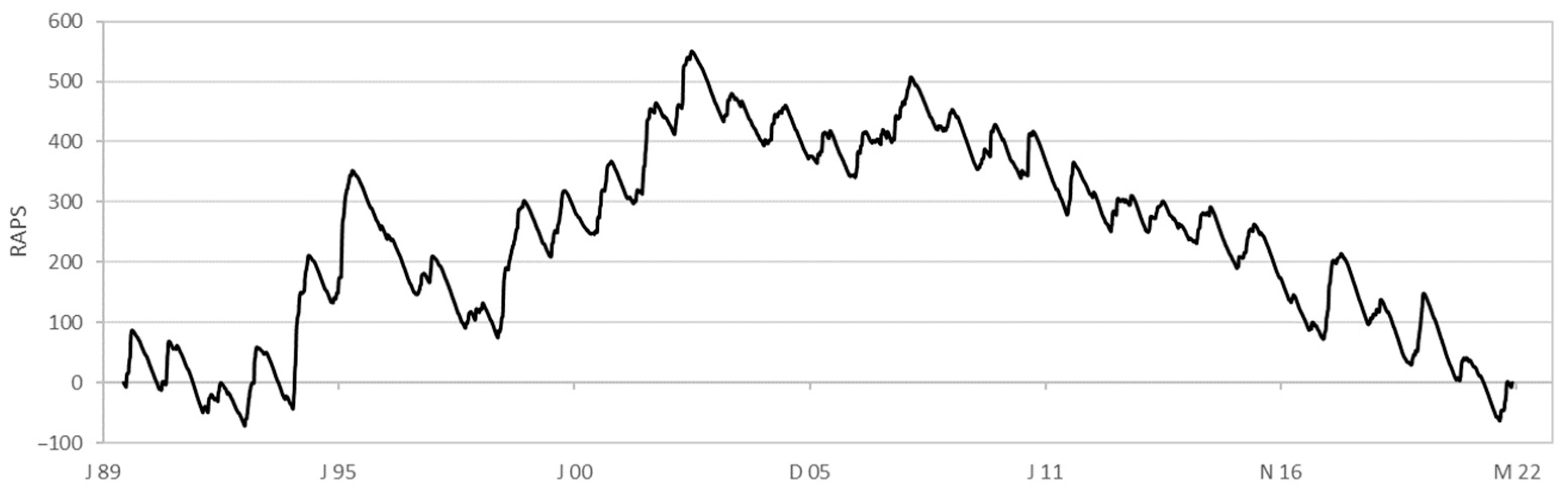
2.3.2. RAPS Method
- : RAPS value of current summation;
- : Value of time series at time point j;
- : Sample mean;
- : Standard derivation;
- : Counter limit of current summation.
2.3.3. Cross-Correlation
2.3.4. Duration Curve (Underrun Days)
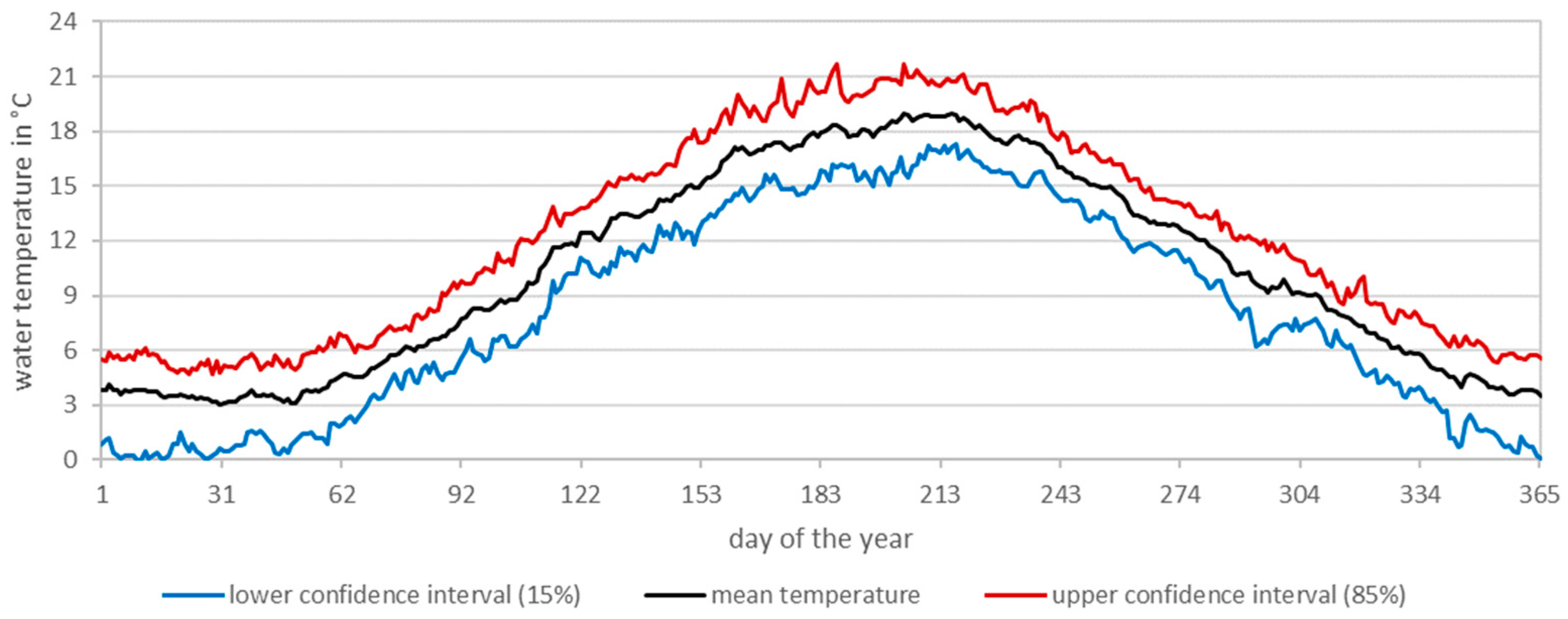
2.3.5. Typical Year
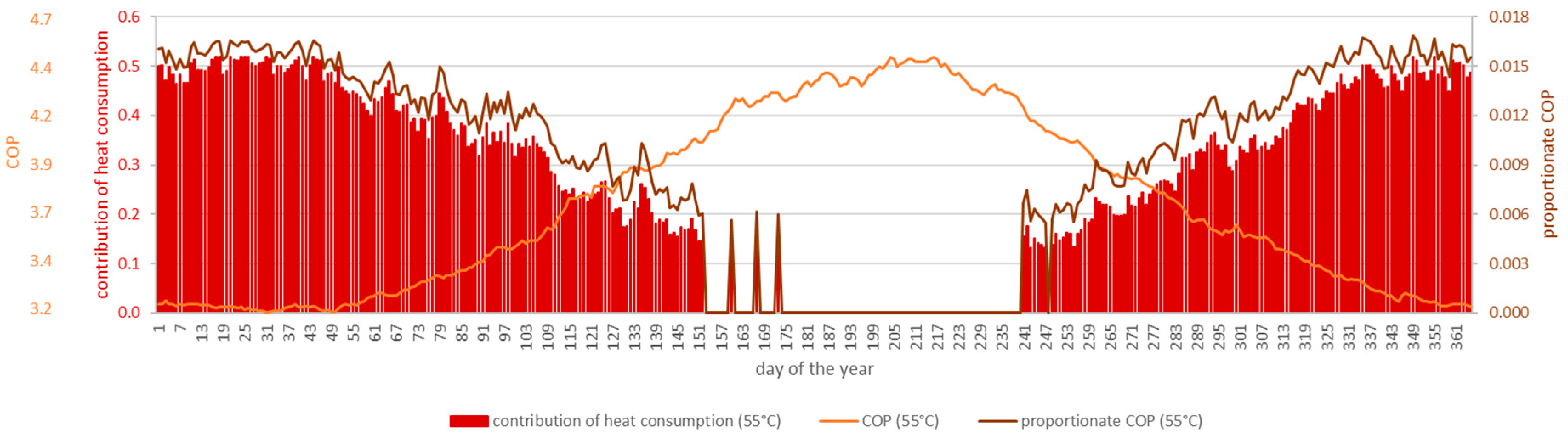
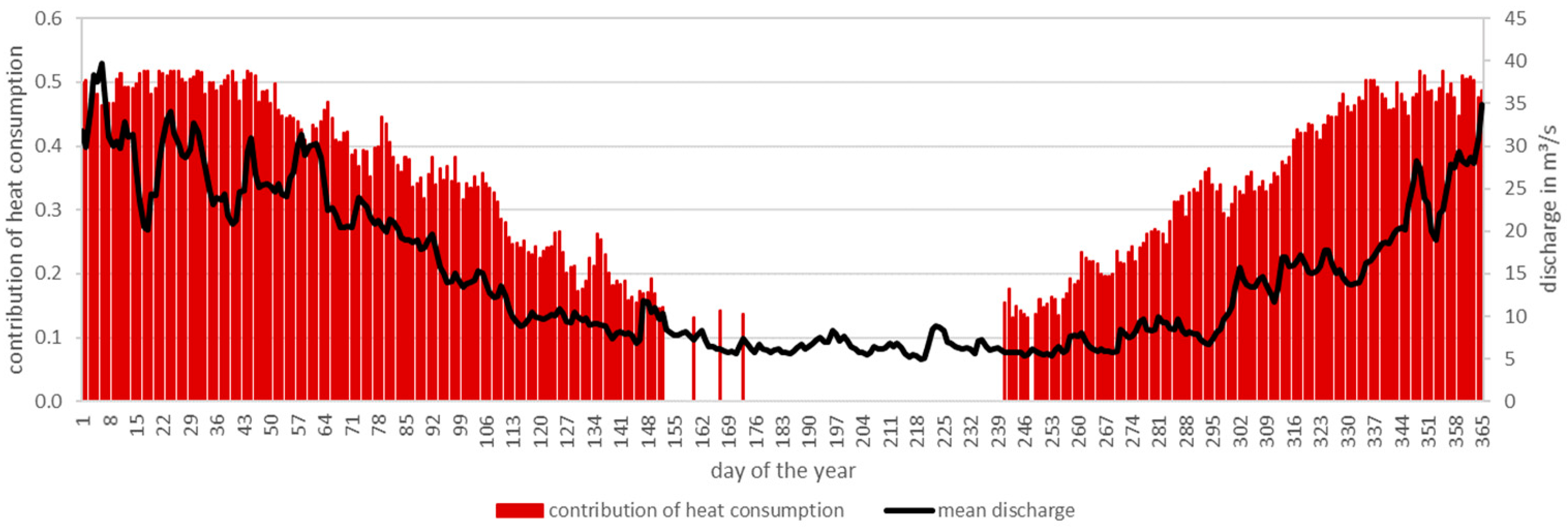
2.3.6. Contribution of Heat Consumption
3. Conducting the Potential Analysis and Feasibility Study
3.1. Water Temperature (Macroscopic)
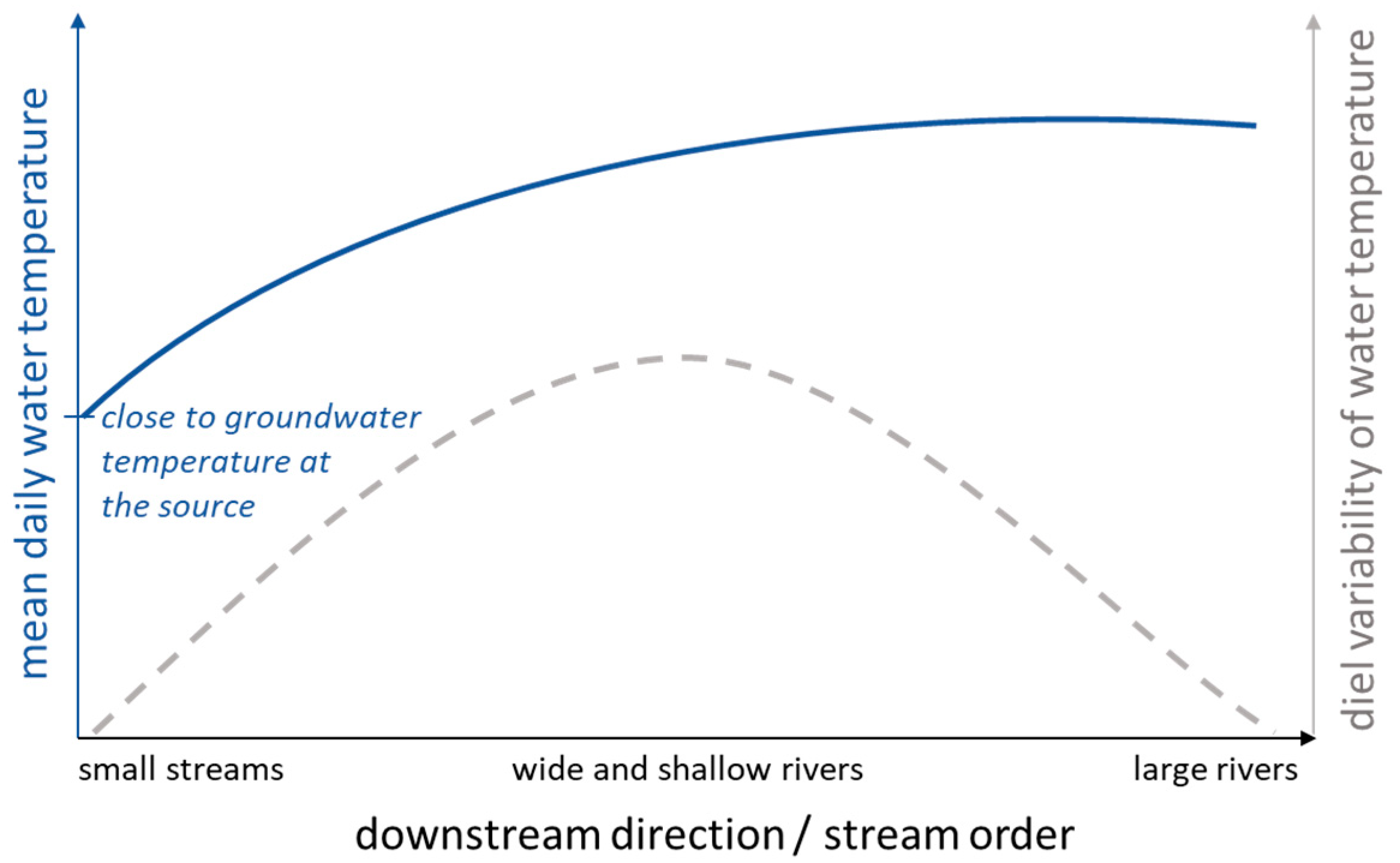
3.1.1. Characteristics: General Changes
3.1.2. Characteristics: Thermal Discharge
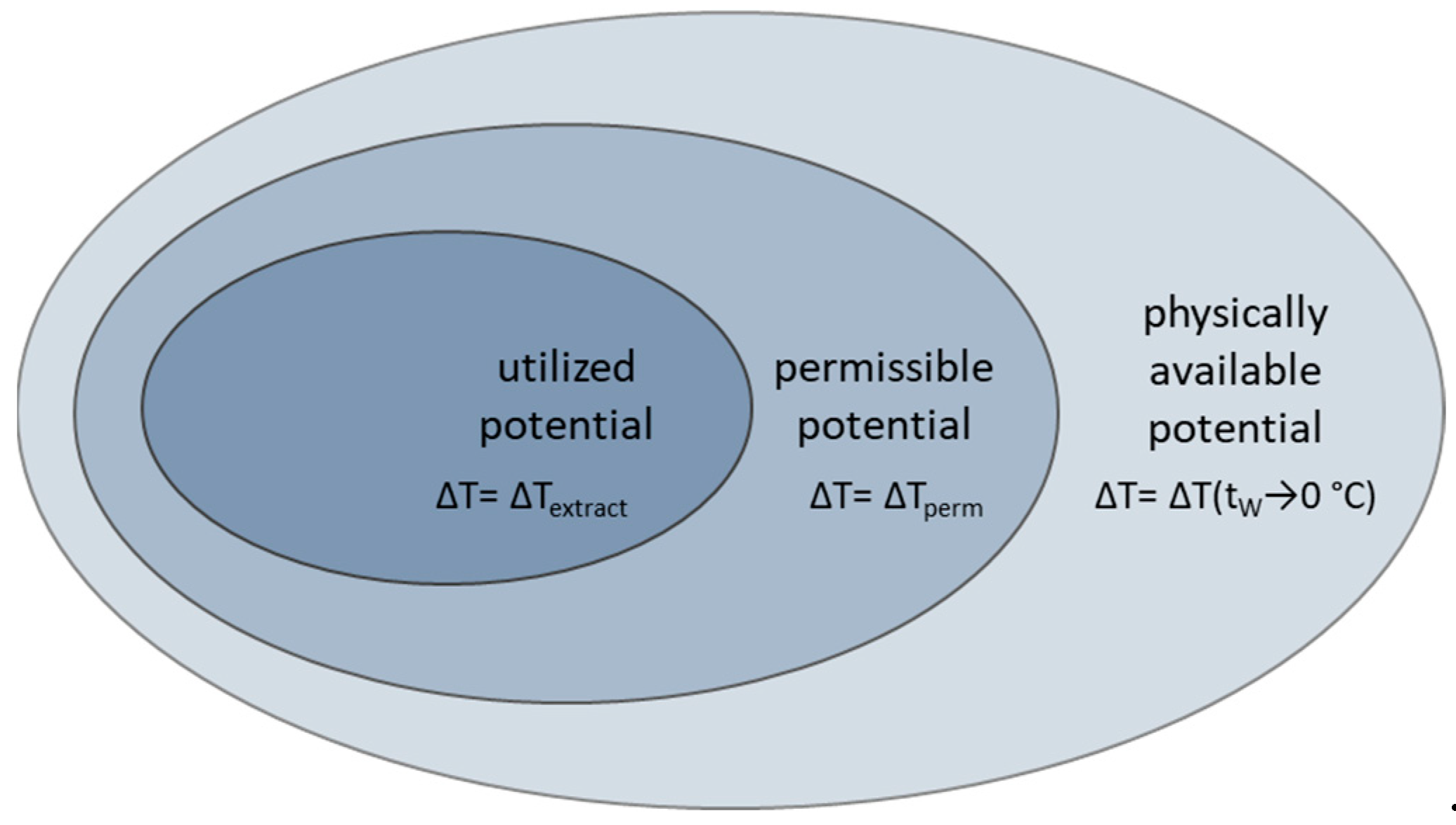
3.1.3. Preliminary Considerations: Ecological and Technical Limit Values
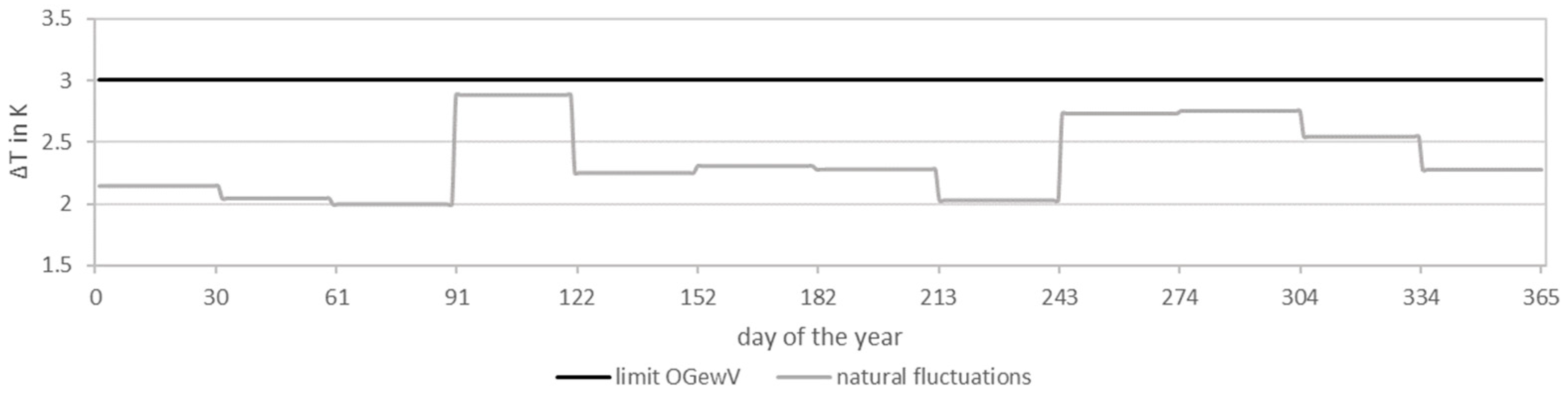
- In Switzerland, the thermal energy use of lakes is established, and changes in water temperature are enshrined in law. The Regulation of Water Protection (Gewässerschutzverordnung, GSchV) allows a maximum temperature change of 3 K in rivers due to heat input or extraction and 1.5 K in the trout region (see GSchV Annex 2, Chapter 1.2, Sentence 4). Several potential studies are based on the limit values [2,8,11]. However, the limit values can be tightened due to the requirement that the water quality should be such that the temperature conditions are close to natural (see GSchV Annex 1, Chapter 1, Sentence 3a) and due to the indication that water discharges must not change the temperature conditions of the water body in such a way that its self-purification capacity is reduced or the water quality is no longer conducive for the biotic communities typical of the water body to thrive (see GSchV Annex 2, Chapter 1.2, Sentence 3).
- In the United Kingdom, the UK Technical Advisory Group on the Water Framework Directive (WFD UK TAG) is based on the European Water Framework Directive, but it deviates from the maximum temperature increase or decrease of 1 K recommended here (see Table 5). The recommendations of the WFD UK TAG were applied as part of the creation of a national heat map [7].
- In Germany, the Regulation of Surface Waters (Oberflächengewässerverordnung, OGewV) sets limits for warming in water bodies, depending on the fish region, in order to ensure good water body status (see Table 6). To estimate a maximum temperature reduction, the maximum temperature increase prescribed in the OGewV can be assumed as the difference for cooling. As temperature increases are considered to be ecologically more serious than temperature decreases, the approach is considered justifiable at this point and is recommended by the Deggendorf Water Management Office [10], among others. To determine the river heat potential for district heating, a study by the IÖW assumed a general temperature change of 1 K [9], possibly to avoid uncertainties in the large-scale potential assessment.
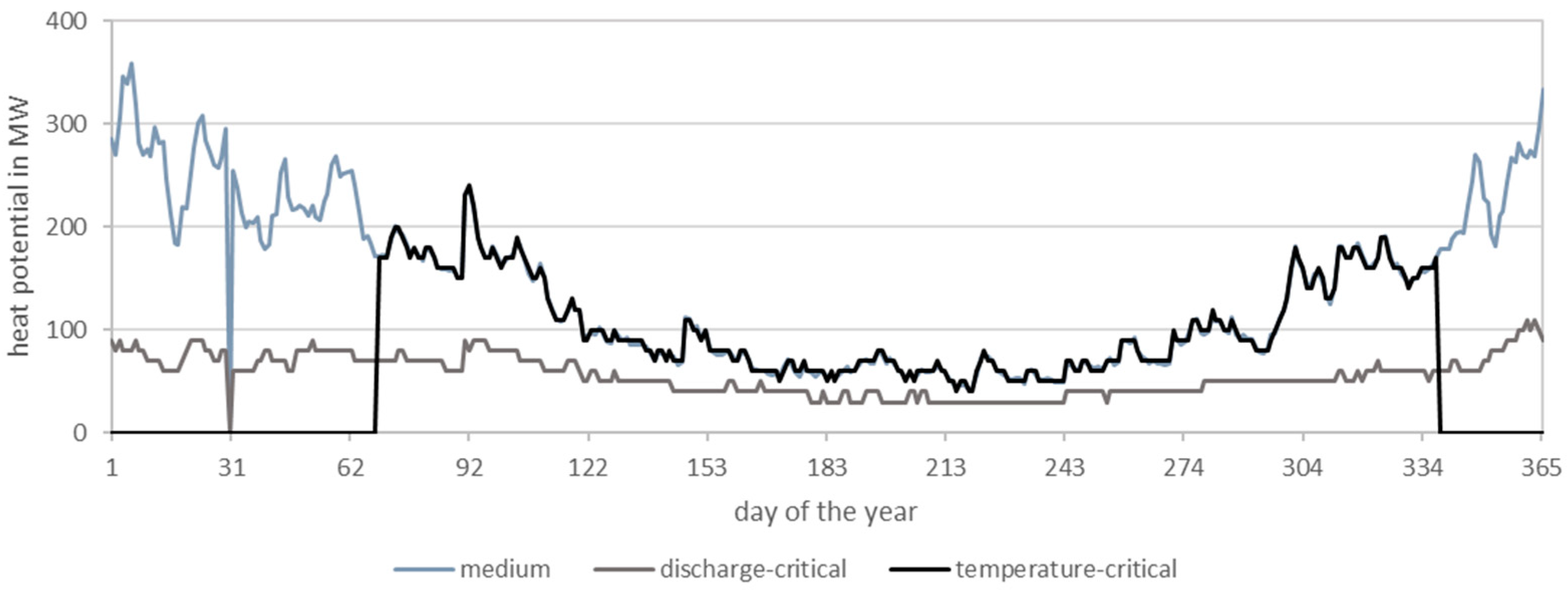
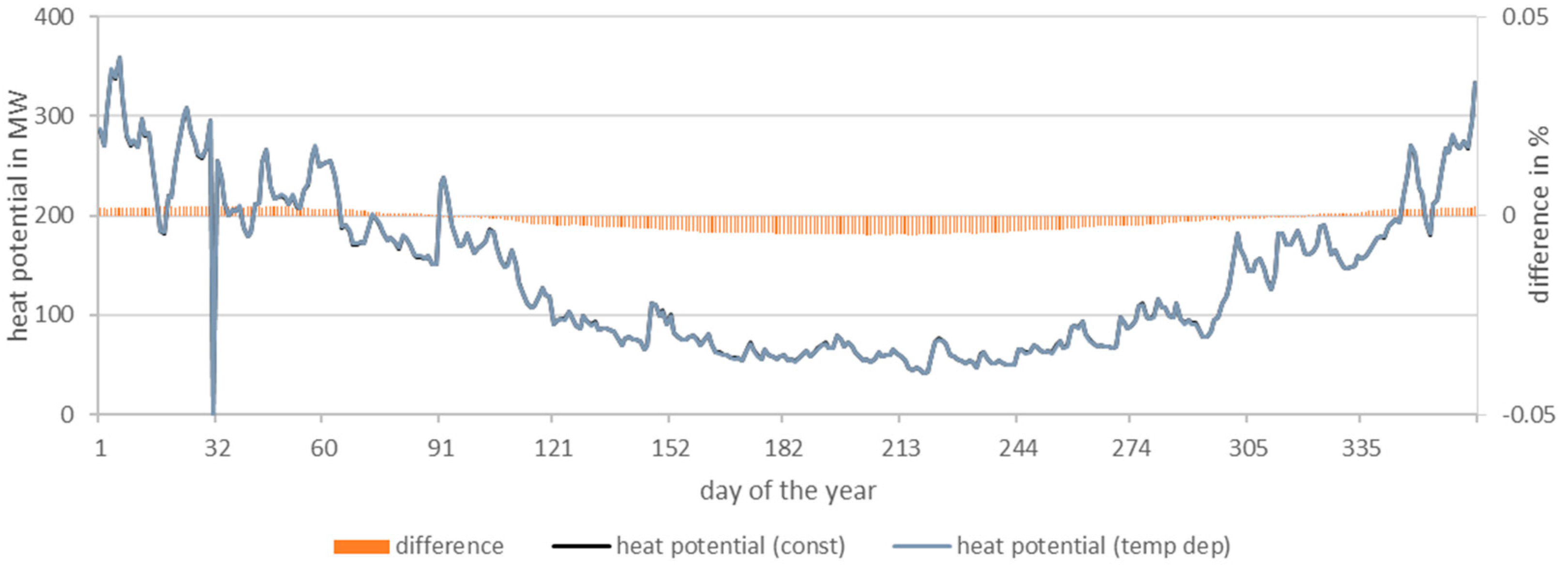
3.1.4. Analysis Parameters: Underrun Days
3.1.5. Analysis Parameters: Hydrothermal Coverage
3.1.6. Preliminary Considerations: Thermal Efficiency
3.1.7. Analysis Parameters: Performance Factors (COP, SPF, SCOP)
- : Coefficient of performance;
- : Useful heat output in W;
- : Electrical power of heat pump in W;
- : Grade for heat source;
- : Temperature of heat sink in K;
- : Temperature of heat source in K.
3.2. Discharge (Macroscopic)
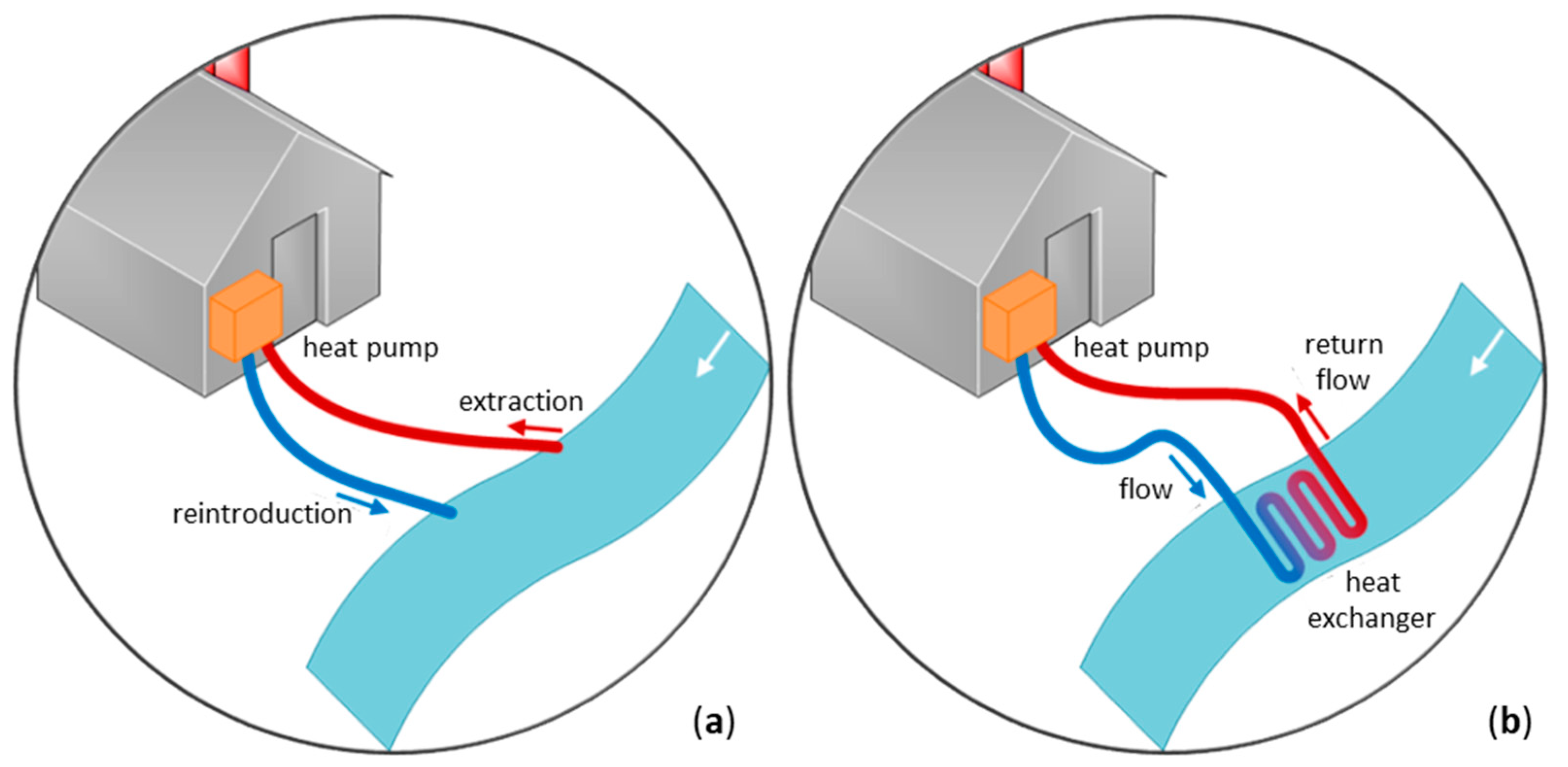
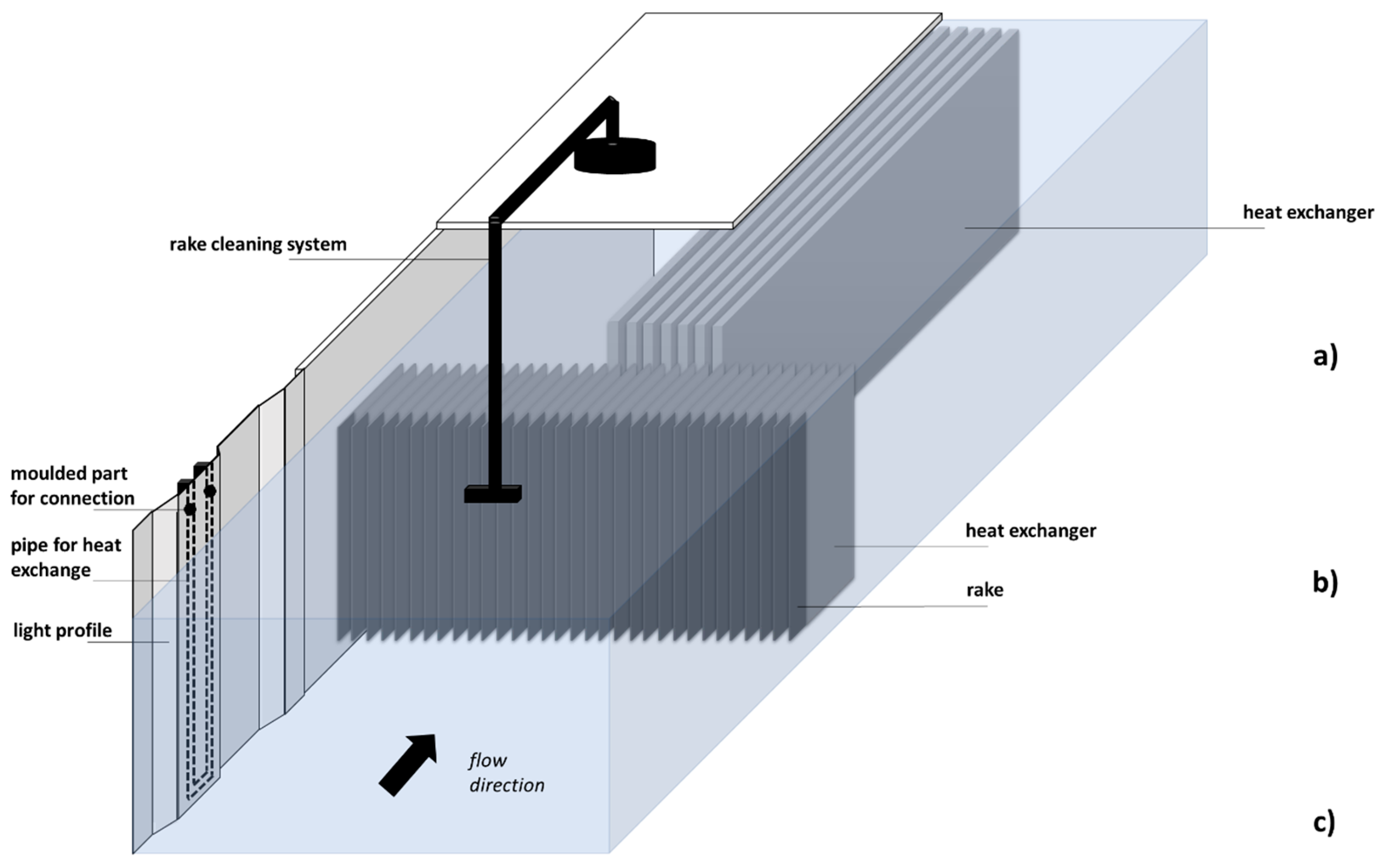
- Open heat extraction system: Up to 20% of the discharge can be extracted from the water body by means of suction extraction [39]. For higher extraction rates, separate extraction structures in the form of side, front, or bottom extraction make sense.
- Closed heat extraction system: If the geometric conditions and water depths are known, then the discharge can be used to determine the flow velocity, which influences the efficiency of the closed heat extraction system and biofilm formation.
3.3. Water Depth (Vertical)
- : Minimum water depth in river in m;
- : Distance to water surface in m;
- : Height of heat extraction system in m;
- : Distance to river bed in m;
- : Distance because of air-drawing vortices in m;
- : Diameter of the suction pipe in m;
- : Height of heat exchanger in m;
- : Distance to river bed in m, heat exchanger specific.
- How an increase in water depths could be achieved (e.g., via ground sills);
- Whether bank filtration should be installed;
- Whether the safety distances can be reduced.
3.4. River Geometry (Horizontal)
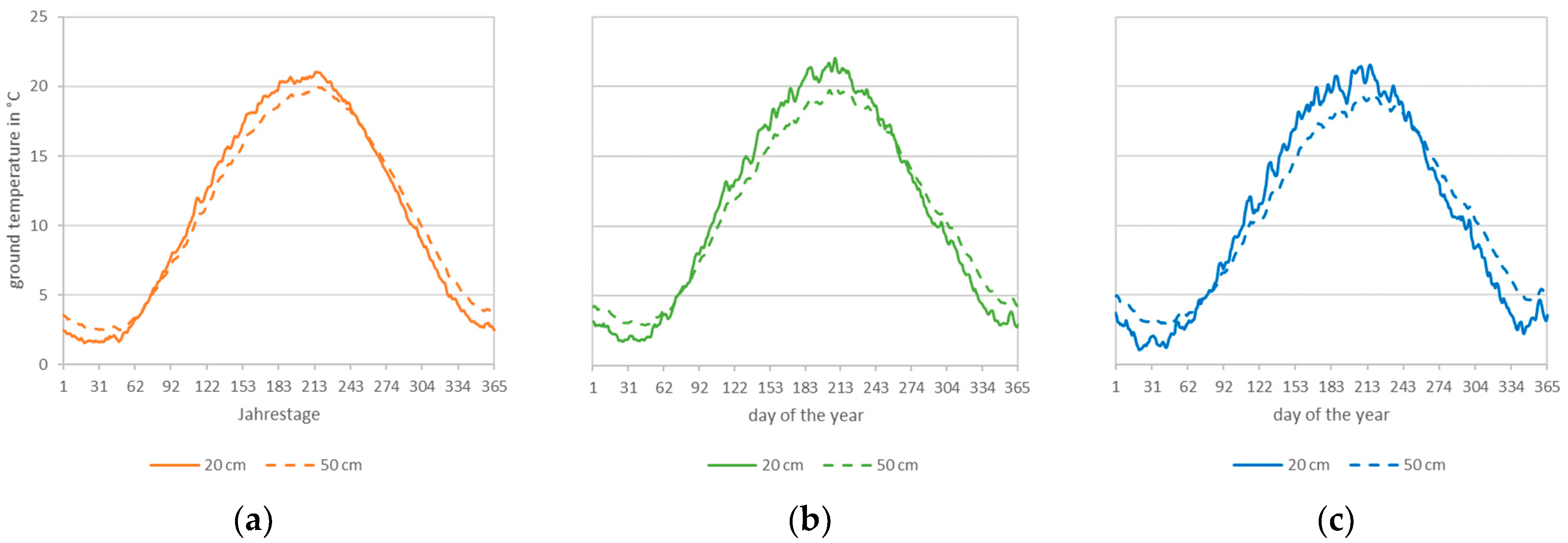
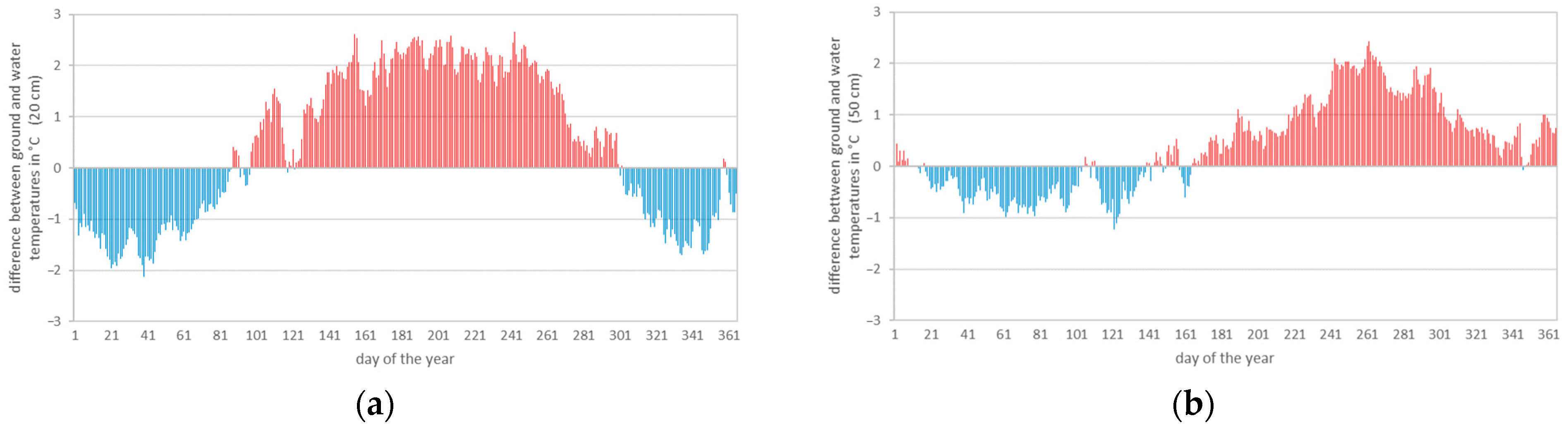
3.5. Distance to the Connection, Ground Temperature
- Thermal efficiency (heat losses or gains in the pipes);
- Electricity costs (pressure losses in the pipes);
- Material costs (pipes, insulation material if necessary);
- Construction costs (laying the pipes).
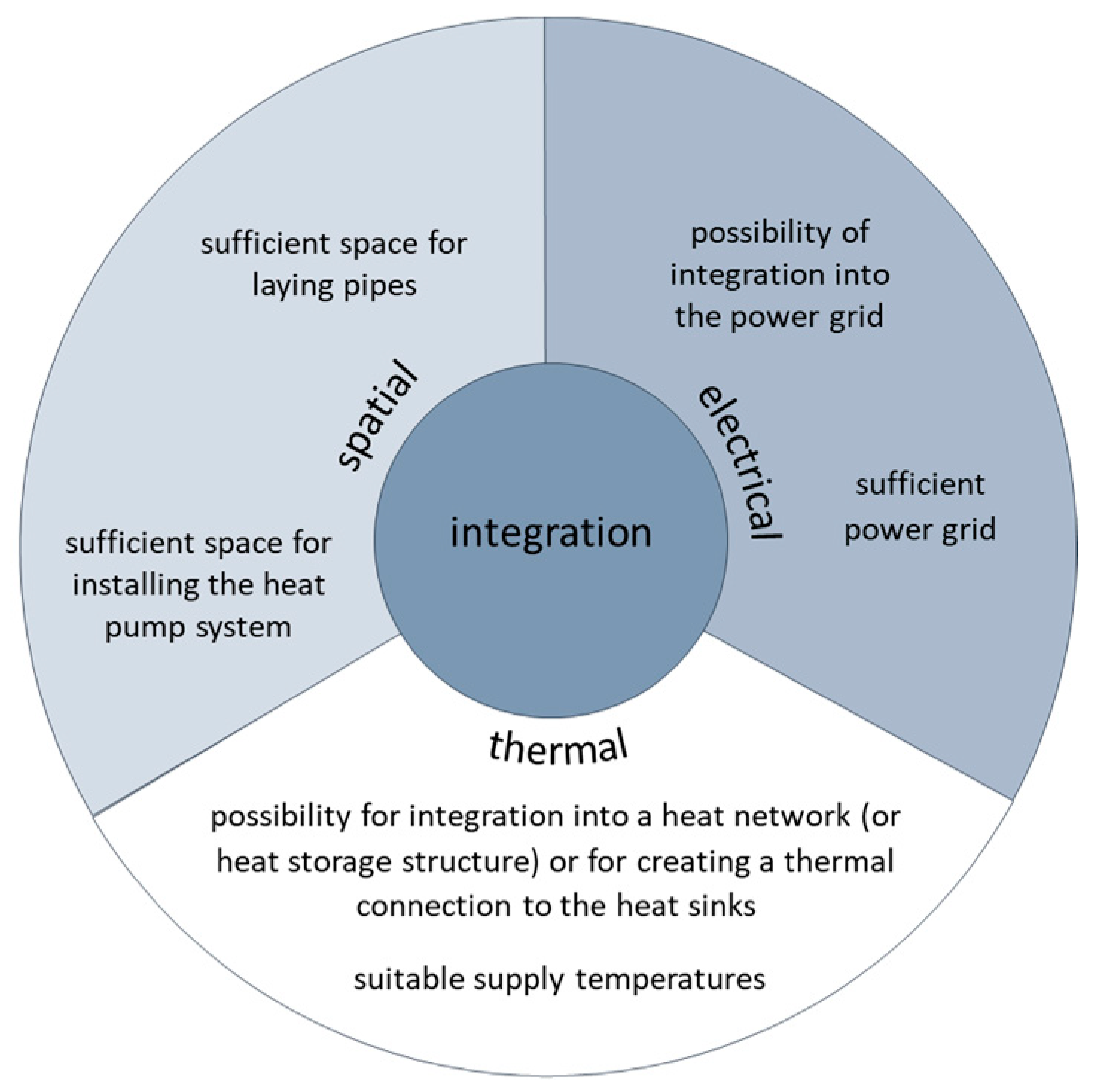
3.6. Existing Infrastructure
3.7. Flow Velocity, Turbulence
3.8. Water Quality
3.9. Balancing of Alternatives
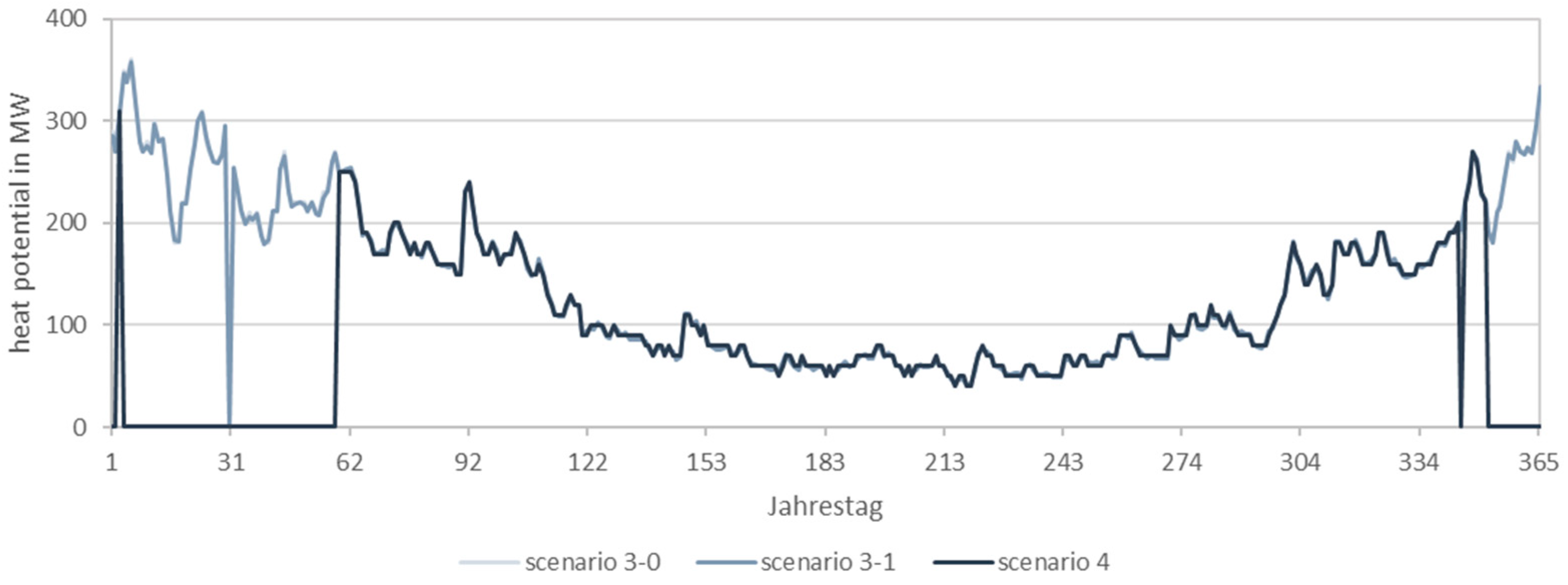
3.10. Heat Potential and Heat Demand
4. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Marotz, G. Der Einsatz von Wärmepumpen in fließenden Gewässern—Wasserwirtschaftliche und betriebliche Aspekte. Wasserwirtschaft 1977, 67, 376–381. [Google Scholar]
- Gaudard, A.; Schmid, M.; Wüest, A. Thermische Nutzung von Seen und Flüssen: Potenzial der Schweizer Oberflächengewässer. Aqua Gas 2018, 2, 26–33. [Google Scholar]
- Schwinghammer, F. Thermische Nutzung von Oberflächengewässern. Master’s Thesis, Albert-Ludwigs-Universität Freiburg, Freiburg, Germany, 2012. [Google Scholar]
- Borchardt, S. Wärmetechnische Nutzung von Fließgewässern. J. Arbeitsschutz Umw. 2018, 1, 21–24. [Google Scholar]
- Schmid, M.; Gaudard, A. Potenzial des Walensees für Wärme- und Kältenutzung; Amt für Wasser und Energie des Kantons St. Gallen: St. Gallen, Switzerland, 2019. [Google Scholar]
- Gaudard, A. Wärme- und Kältenutzung aus Brienzer-, Thuner- und Bielersee: Abschätzung des Potenzials und Beeinflussung der Seeökosysteme; Eawag: Kastanienbaum, Switzerland, 2016. [Google Scholar]
- Department of Energy & Climate Change. National Heat Map: Water Source Heat Map Layer; Department of Energy & Climate Change: London, UK, 2015. [Google Scholar]
- Anton, S. Thermische Nutzung Rhein: Schlussbericht Potentialstudie; Eichler + Pauli: Bern, Germany, 2016. [Google Scholar]
- Dunkelberg, E.; Deisböck, A.; Herrmann, B.; Hirschl, B.; Mitzinger, T.; Röder, J.; Salecki, S.; Thier, P.; Wassermann, T. Fernwärme Klimaneutral Transformieren: Eine Bewertung der Handlungsoptionen am Beispiel Berlin Nord-Neukölln; Schriftenreihe des Institut für Ökologische Wirtschaftsforschung 218/20; Institut für Ökologische Wirtschaftsforschung: Berlin, Germany, 2020. [Google Scholar]
- Wasserwirtschaftsamt Deggendorf. Wärmetauscher in Oberirdischen Gewässern: Wasserwirtschaftliche Betrachtung; Wasserwirtschaftsamt Deggendorf: Deggendorf, Germany, 2011. [Google Scholar]
- Gaudard, A.; Wüest, A.; Schmid, M. Using lakes and rivers for extraction and disposal of heat: Estimate of regional potentials. Renew. Energy 2019, 134, 330–342. [Google Scholar] [CrossRef]
- Marotz, G.W. Model Tests to Investigate Water-Circulation in Heat Pumps. In Proceedings of the 17th Congress of the International Association for Hydraulic Research, Baden-Baden, Germany, 14–19 August 1977; pp. 291–297. [Google Scholar]
- Maniak, U. Hydrologie und Wasserwirtschaft: Eine Einführung für Ingenieure, 7th ed.; Springer Vieweg: Berlin, Germany, 2016. [Google Scholar] [CrossRef]
- Deutsche Vereinigung für Wasserwirtschaft, Abwasser und Abfall e.V. (DVWK). Statistische Analyse von Hochwasserabflüssen. Merkblätter zur Wasserwirtschaft; Deutsche Vereinigung für Wasserwirtschaft, Abwasser und Abfall e.V. (DVWK): Hennef (Sieg), Germany, 1999. [Google Scholar]
- Linke, C. Leitlinien zur Interpretation Regionaler Klimamodelldaten des Bund-Länder-Fachgesprächs “Interpretation Regionaler Klimamodelldaten”; Landesamt für Umwelt Brandenburg: Potsdam, Germany, 2023. [Google Scholar]
- Kos, Z.; Durin, B.; Dogancic, D.; Kranjcic, N. Hydro-Energy Suitability of Rivers Regarding Their Hydrological and Hydrogeological Characteristics. Water 2021, 13, 1777. [Google Scholar] [CrossRef]
- Garbrecht, J.; Fernandez, G.P. Visualization of Trends and Fluctuations in Climatic Records. Wasser Resour. Bull. Am. Water Resour. Assoc. 1994, 30, 2. [Google Scholar]
- Caissie, D. The thermal regime of rivers: A review. Freshw. Biol. 2006, 51, 1389–1406. [Google Scholar] [CrossRef]
- van Vliet, M.; Franssen, W.; Yearsley, J.R.; Ludwig, F.; Haddeland, I.; Lettenmaier, D.P.; Kabat, P. Global river discharge and water temperature under climate change. Glob. Environ. Chang. 2013, 23, 450–464. [Google Scholar] [CrossRef]
- Orr, H.G.; Simpson, G.L.; Des Clers, S.; Watts, G.; Hughes, M.; Hannaford, J.; Dunbar, M.J.; Laizé, C.; Wilby, R.L.; Battarbee, R.W.; et al. Detecting changing river temperatures in England and Wales. Hydrol. Process. 2015, 29, 752–766. [Google Scholar] [CrossRef]
- European Environment Agency. Climate change, impacts and vulnerability in Europe 2016: An indicator-based report. Publications Office of the European Union. EEA Rep. 2017, 1. [Google Scholar] [CrossRef]
- Bay, O. Kalibrierung und Validierung Eines Wasserhaushalts- und Wärmemodells zur Simulation von Fließgewässertemperaturen. Master‘s Thesis, Technische Universität Darmstadt, Darmstadt, Germany, 2023. [Google Scholar] [CrossRef]
- Lange, J. Wärmelast Rhein: Studie; BUND: Mainz, Germany, 2009. [Google Scholar]
- WFD UK TAG. UK Environmental Standards and Conditions (Phase 2); UK Technical Advisory Group on the Water Framework Directive: Wallscope, Edingburgh, 2008. [Google Scholar]
- KLIWA. Klimawandel in Süddeutschland. Veränderungen von Meteorologischen und Hydrologischen Kenngrößen: Monitoringbericht 2021; Landesamt für Umwelt Rheinland-Pfalz: Mainz, Germany, 2021. [Google Scholar]
- Güttinger, H. Wärmepumpen an Oberflächengewässern: Ökologische Probleme und Einsatzmöglichkeiten in der Schweiz; Schriftenreihe des Bundesamtes für Energiewirtschaft: Bern, Switzerland, 1981. [Google Scholar]
- AGFW. Praxisleitfaden Großwärmepumpen; AGFW: Frankfurt, Germany, 2020. [Google Scholar]
- Kim, Y.; Yu, K.-H. Constructing a Database of Reference Hydrothermal Sources for a Zero-Energy Building Certification Rating in South Korea and Analyzing the Renewable Energy Self-Sufficiency Rate Achieved by Water-Source Heat Pumps. Energies 2023, 16, 543. [Google Scholar] [CrossRef]
- Ministerium für Umwelt, Landwirtschaft, Natur- und Verbraucherschutz des Landes Nordrhein-Westfalen (MULNV NRW). Bewirtschaftungsplan 2022–2027 für die Nordrhein-Westfälischen Anteile von Rhein, Weser, Ems und Maas; MULNV NRW: Düsseldorf, Germany, 2021. [Google Scholar]
- Kammer, H. Thermische Seewassernutzung in Deutschland: Bestandsanalyse, Potential und Hemmnisse Seewasserbetriebener Wärmepumpen; Springer Vieweg: Wiesbaden, Germany, 2017. [Google Scholar] [CrossRef]
- Koppmann, D. Untersuchung der Thermischen Aktivierung von Stahlspundwänden. Ph.D. Dissertation, Aachen University, Aachen, Germany, 2021. [Google Scholar]
- DWD. Wetter- und Klimalexikon, Klima. 2022. Available online: https://www.dwd.de/DE/service/lexikon/Functions/glossar.html?lv2=101334&lv3=101462 (accessed on 15 November 2023).
- Zogg, M. Zertifikatslehrgang ETH in Angewandten Erdwissenschaften: Geothermie—Die Energie des 21. Jahrhunderts; ETH Zürich: Oberburg, Switzerland, 2009. [Google Scholar]
- Verein Deutscher Ingenieure. Berechnung der Jahresarbeitszahl von Wärmepumpenanlagen: Elektrowärmepumpen zur Raumheizung und Trinkwassererwärmung; Beuth: Berlin, Germany, 2019. [Google Scholar]
- Ehrbar, M.P. Prüfungen im Testzentrum schaffen Vertrauen: Rückblick, Ausblick und Vergleich mit Feldbetrieb. In FAWA, Feldanalyse von Wärmepumpen-Anlagen; Rognon, F., Ed.; Bundesamt für Energie: Bern, Switzerland, 2004; pp. 27–38. [Google Scholar]
- ISE. Feldmessung Wärmepumpen im Gebäudebestand: Abschlussbericht; Fraunhofer Institut Solare Energiesysteme: Freiburg, Germany, 2010. [Google Scholar]
- Rognon, F. (Ed.) FAWA: Feldanalyse von Wärmepumpen-Anlagen; Bundesamt für Energie: Bern, Switzerland, 2004. [Google Scholar]
- BAFA. Wärmepumpe: Grundwissen zum Marktanreizprogramm; Bundesamt für Wirtschaft und Ausfuhrkontrolle: Eschborn, Germany, 2019. [Google Scholar]
- Scheuerlein, H. Die Wasserentnahme aus Geschiebeführenden Flüssen; Ernst Verlag: Berlin, Germany, 1984. [Google Scholar]
- Pardé, M. Fleuves et Riviéres; Colin: Paris, France, 1964. [Google Scholar]
- Deutsches Institut für Normung. Künstlich Angelegte Löschwasserteiche: Artificially Constructed Water Ponds for Fire Fighting; Beuth: Berlin, Germany, 2019; DIN 1420. [Google Scholar]
- Peterson, A.W.; Bouthillier, P.H.; Charbonneau, A.L.; Yaremko, E.K. River Intake Structures. In Pumping Station Design for the Practical Engineer: Water; Sank, R.L., Reh, C.W., Eds.; Montana State University: Bozeman, MT, USA, 1981. [Google Scholar]
- KSB SE & Co. KGaA. Auslegung von Kreiselpumpen: KSB Know-How; KSB SE & Co. KGaA: Frankenthal, Germany, 2019. [Google Scholar]
- Burlingame, R.S. Other Intake Structures. In Pumping Station Design for the Practical Engineer: Water; Sank, R.L., Reh, C.W., Eds.; Montana State University: Bozeman, MT, USA, 1981. [Google Scholar]
- Brede, H.; Koppe, B. SynTHERM: Untersuchung der synergetischen Nutzbarkeit der kinetischen und thermischen Energie von Oberflächengewässerkörpern an Wasserkraftanlagen-Standorten. In Mitteilungen, Heft 156. Tagungsband; Technische Universität Darmstadt: Darmstadt, Germany, 2018; pp. 112–118. [Google Scholar]
- Hansen, G.M. Experimental Testing and Analysis of Surface Water Heat Exchangers. Master’s Thesis, Oklahoma State University, Oklahoma, OK, USA, 2011. [Google Scholar]
- Valor Partners Oy. Suuret Lämpöpumput Kaukolämpöjärjestelmässä; Energiateollisuus: Helsinki, Finland, 2016. [Google Scholar]
- LAWA. Grundlagen für die Beurteilungen von Kühlwassereinleitungen in Gewässer, 4th ed.; Erich Schmidt Verlag: Berlin Germany, 2008. [Google Scholar]
- Dötsch, C.; Taschenberger, J.; Schönberg, I. Leitfaden Nahwärme; Fraunhofer IRB: Stuttgart, Germany, 1998. [Google Scholar]
- Kwon, Y.; Bae, S.; Nam, Y. Development of Design Method for River Water Source Heat Pump System Using an Optimization Algorithm. Energies 2022, 15, 4019. [Google Scholar] [CrossRef]
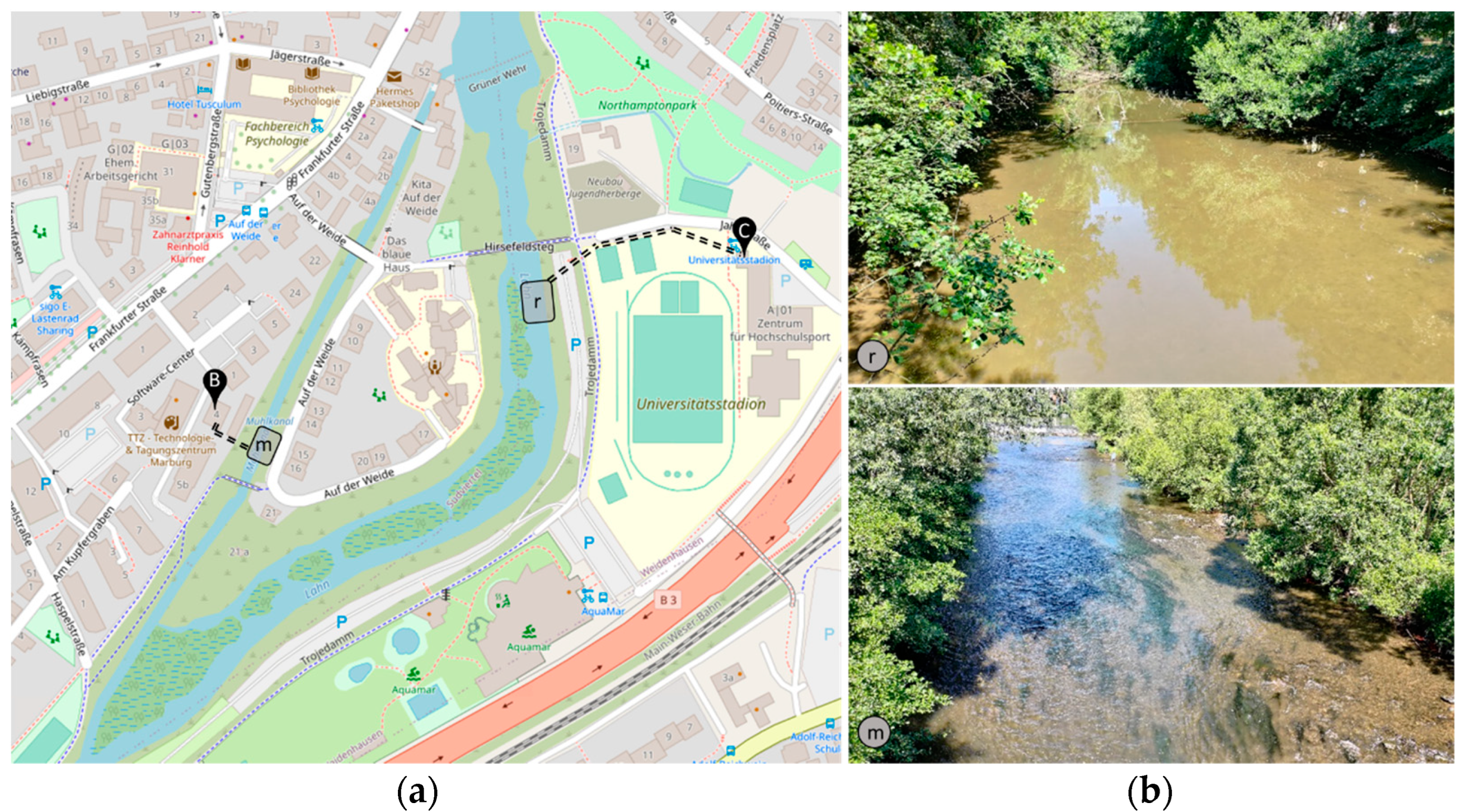
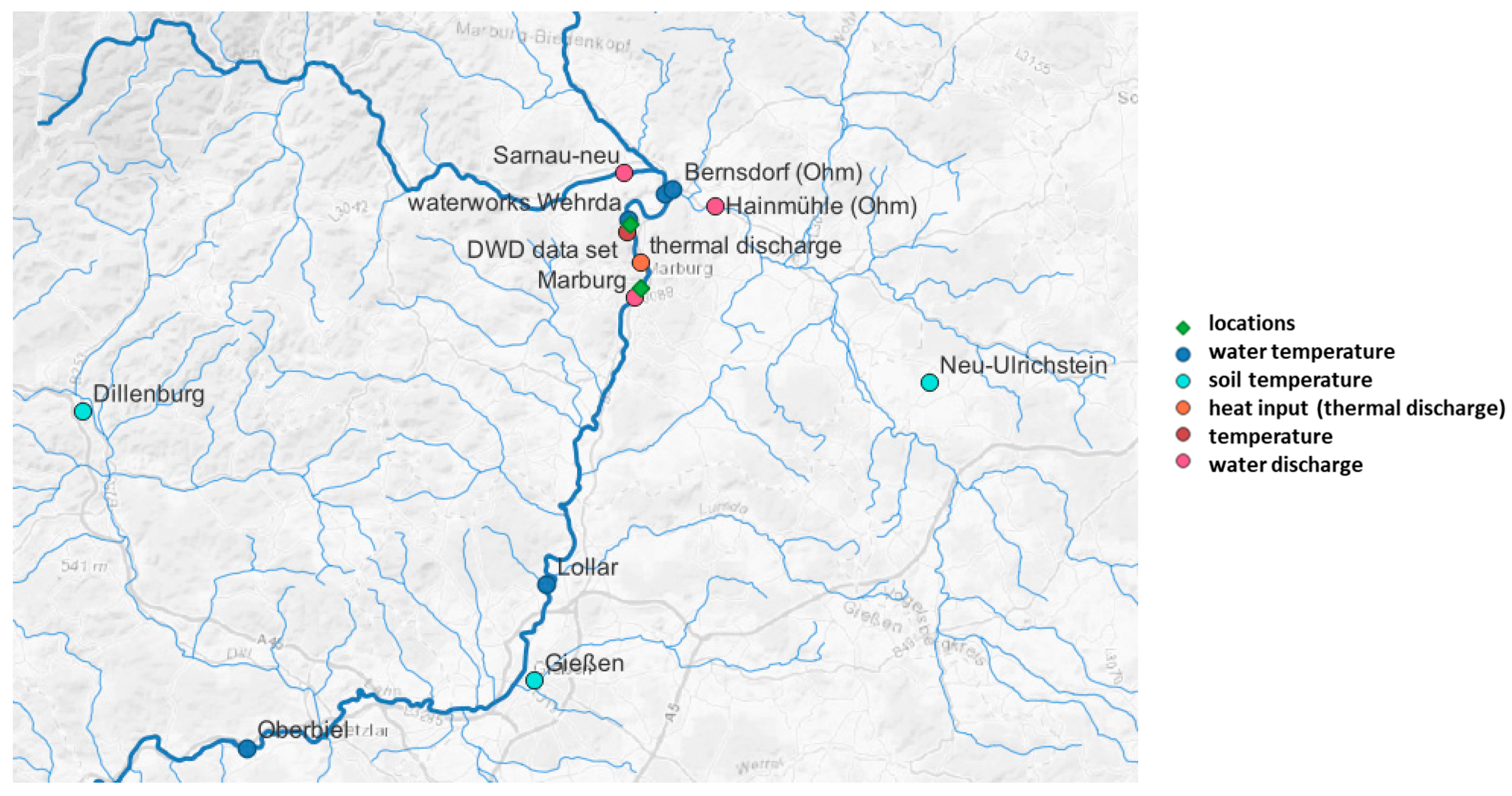
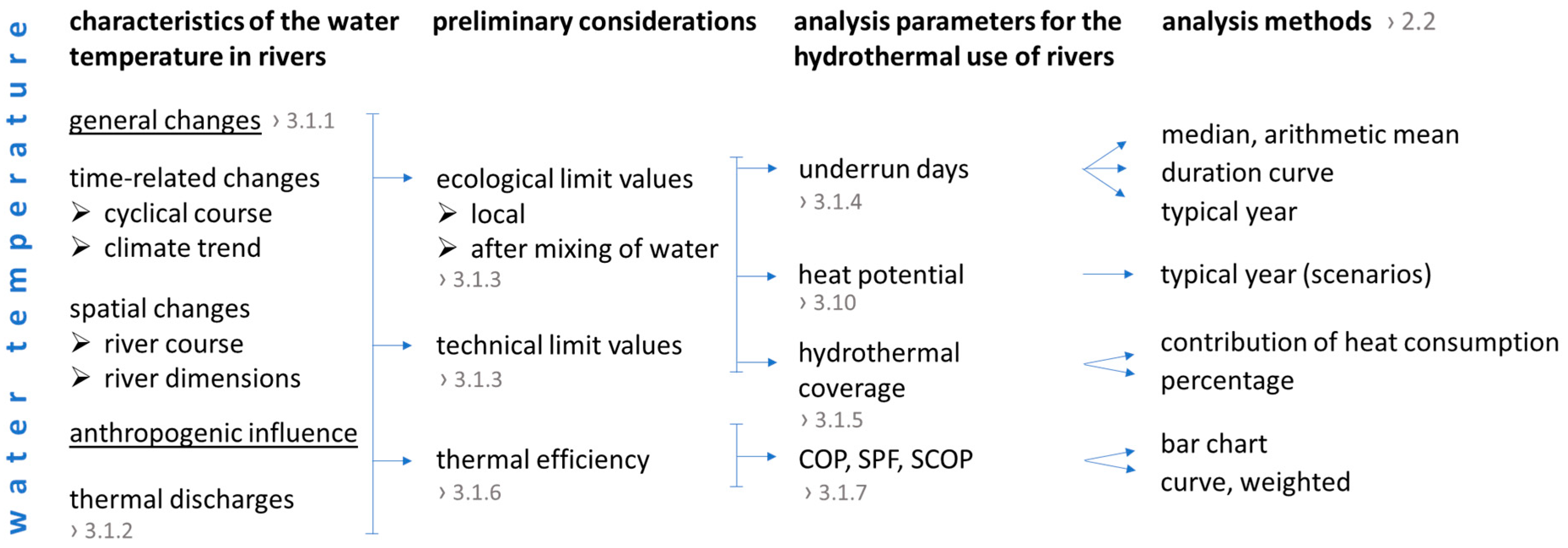
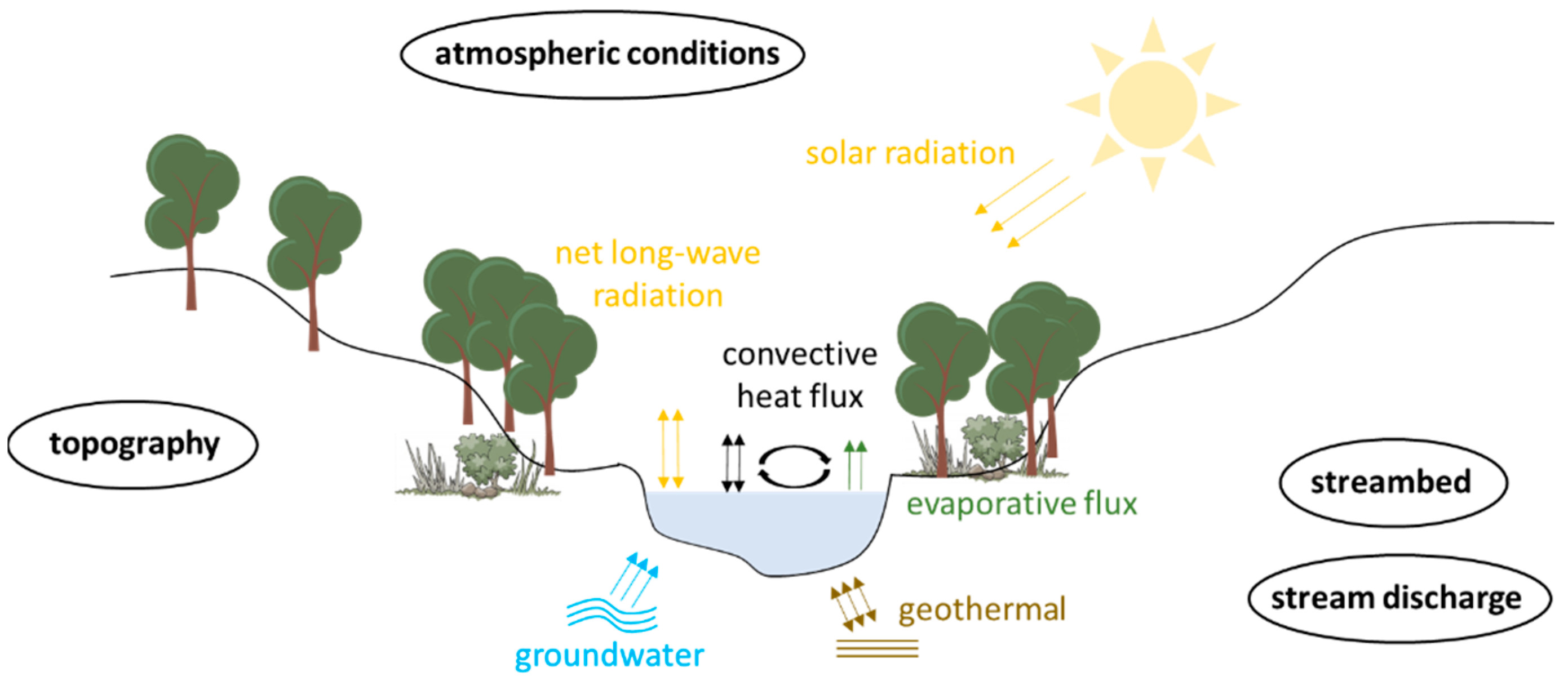









| Parameters, data | Significance for hydrothermal use of rivers |
| Macroscopic level: thermal potential of the river section (potential analysis) | |
| Water temperature | Thermal availability (technical feasibility, water protection) Thermal efficiency (COP) |
| Discharge | Possible heat quantity |
| Microscopic level: concretization at the site (feasibility study) | |
| Water depth | Space (vertical) for installing the heat extraction system: compliance with safety distances to the river bed and water level |
| River geometry (horizontal) | Space (horizontal) for installing the heat extraction system, if necessary: compliance with distance to other uses |
| Distance to the connection Ground temperature | Heat losses or gains via transport pipes |
| Existing infrastructure | Integration in heat storage structure/heat network possible? Feasibility through laying of pipes or having empty pipes already Feasibility through integration into the power grid |
| Flow velocity/turbulence | Mixing of temperature flag (Closed system:) Efficiency of heat transfer, biofouling |
| Water quality | Cleaning intervals Precautions to protect against solids |
| Economic level on the heat sink side: Is the implementation worthwhile? | |
| Heat demand | Hydrothermal coverage sufficient? |
| Balancing of alternatives | Is a possibly expensive, innovative implementation of hydrothermal energy worthwhile because it is better than its alternatives in monetary and climatic terms? |
| Measuring Point | Available Data Frequency | Data Volume (Time Period) | Origin |
|---|---|---|---|
| Water temperature | |||
| Bernsdorf (Ohm) | Monthly samples | 159 days (2008 to 2020) | HLNUG 1 |
| Bernsdorf (Lahn) | Monthly samples | 147 days (2008 to 2019) | HLNUG |
| Lollar | Monthly samples | 163 days (2008 to 2021) | HLNUG |
| Oberbiel | Daily averages | 11 years (2011 to 2022) | HLNUG |
| Wehrda waterworks | Daily samples, in morning | 26 years (1994 to 2021) | Stadtwerke Marburg |
| Wastewater temperature and discharge (thermal discharge as an influence on water temperature) | |||
| Location of thermal discharge | Three monthly samples | 24 days (2016 to 2021) | Originator (self-control report) |
| Discharge | |||
| Gauge Marburg | Daily averages | 32 Jahre (1990 to 2021) | HLNUG |
| Gauge Hainmühle | Not considered due to uncertain data collection | HLNUG | |
| Gauge Sarnau-new | Not considered due to uncertain data collection | HLNUG | |
| Water depth | |||
| Gauge Marburg | Daily averages | 32 years (1990 to 2021) | HLNUG |
| Wehrda waterworks | Daily samples | 28 years (1994 to 2022) | Stadtwerke Marburg |
| Ground temperature (at depth of 5 cm, 10 cm, 20 cm, 50 cm) | |||
| Dillenburg | Daily averages | 18.5 years (2004 to 2022) | DWD |
| Gießen | Daily averages | 72 years (1951 to 2022) | DWD |
| Neu-Ulrichstein | Daily averages | 12 years (2011 to 2022) | DWD |
| Air temperature | |||
| Measuring point: 50.8346° N; 8.7578° E (WGS84) | Hourly values | 18 years (1995 to 2012) | DWD (test reference years) |
| Heat consumption | |||
| Software center heat network | Annual duration curve (hourly) and monthly values | 3 years (2013 to 2015) | Stadtwerke Marburg |
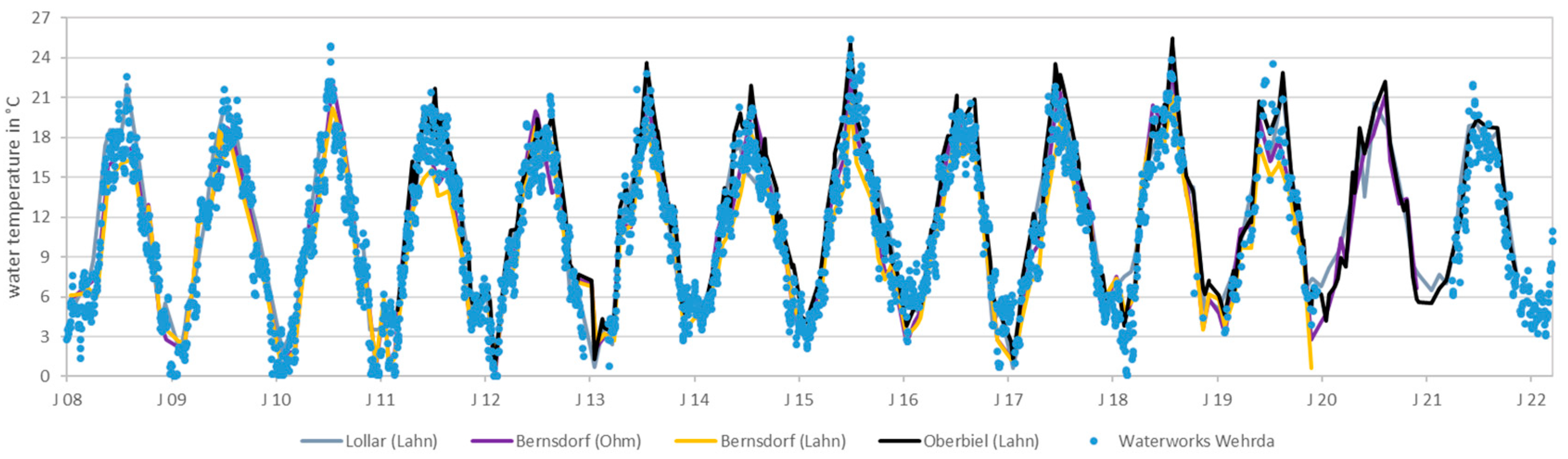
| Measuring Station | t0.5 in °C | Distance in km | Temperature Increase in K/km | ||
|---|---|---|---|---|---|
| Partial Route | From Bernsdorf | Partial Route | From Bernsdorf | ||
| Bernsdorf/Lahn | 9.7 | - | - | - | - |
| Wehrda waterworks | 10.6 (tm) | 5.5 | 5.5 | 0.16 | 0.16 |
| Lollar | 11.5 | 30.6 | 36.1 | 0.03 | 0.05 |
| Oberbiel | 12.0 | 31.7 | 37.8 | 0.02 | 0.06 |
| Wastewater Discharge | Lahn River | ||||||
|---|---|---|---|---|---|---|---|
| Qwaste m3/s | Twaste °C | TLahn °C | ϱW kg/m3 | cp,W kJ/kgK | ΔT K | kW | |
| Scenario medium | 2.2 | 26.4 | 10.6 | 998.5 | 4.2 | 15.8 | 40 |
| Scenario limit value | 20.0 | 34.0 | 10.6 | 997.8 | 4.2 | 22.8 | 530 |
| Scenario limit value, extreme | 20.0 | 34.0 | 0 | 998.8 | 4.2 | 34.0 | 790 |
| Ecological Status for the Water Framework Directive | ||||
|---|---|---|---|---|
| High | Good | Moderate | Poor | |
| tmax for cold water [°C] (annual 98-percentiles) 1 | 20 | 23 | 28 | 30 |
| tmax for warm water [°C] (annual 98-percentiles) 1 | 25 | 28 | 30 | 32 |
| Temperature uplift and drop values [ΔT in K] | 2 | 3 | 3 | 3 |
| Fish Community | |||||||
|---|---|---|---|---|---|---|---|
| Sa-ER | Sa-MR | Sa-MR | Cyp-R | EP | MP | HP | |
| tmax summer (April to November) [°C] | 20 | 20 | 21.5 | 23 | 25 | 28 | 28 |
| Max. increase in summer [ΔT in K] | 1.5 | 1.5 | 1.5 | 2 | 3 | 3 | 3 |
| tmax winter (December to March) [°C] | 8 | 10 | 10 | 10 | 10 | 10 | 10 |
| Max. increase in winter [ΔT in K] | 1 | 1.5 | 1.5 | 2 | 3 | 3 | 3 |
| Critical Temperature in °C | 2 | 3 | 4 |
|---|---|---|---|
| Underrun days | 22 ± 12 | 37 ± 13 | 56 ± 17 |
| Percentage of underrun days in relation to 279 heating days (15 °C heating limit temperature) | 8 ± 4 | 13 ± 5 | 20 ± 6 |
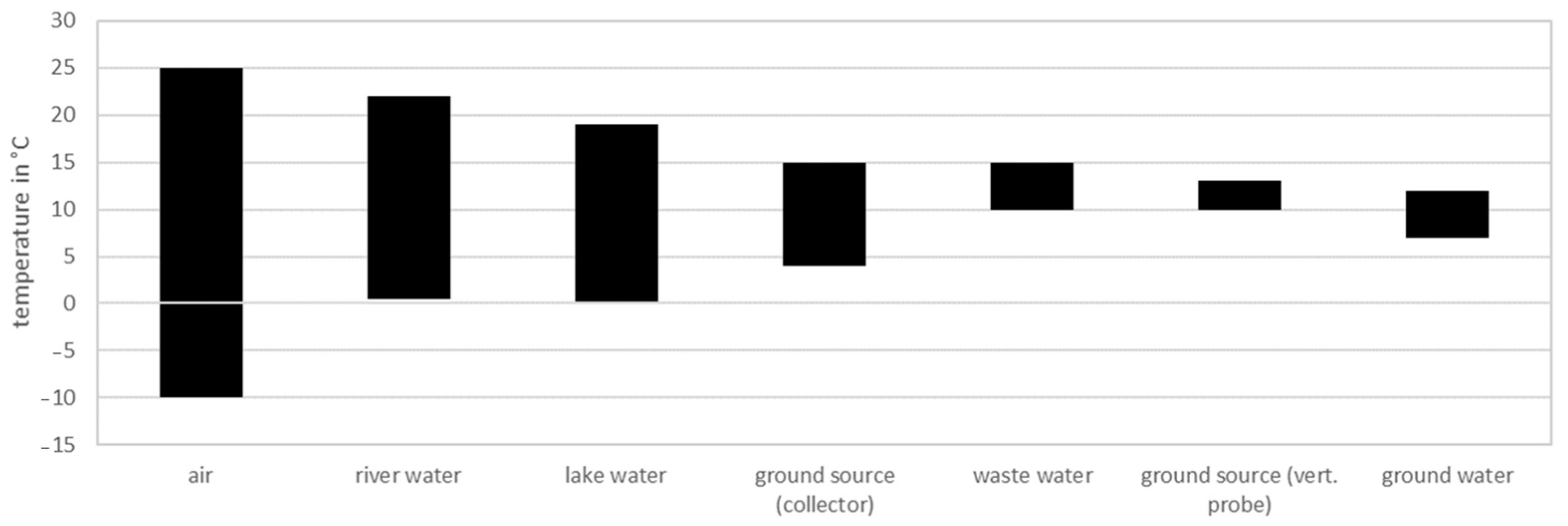
| Heat Source | |
|---|---|
| Air | 0.40 |
| Ground source (vertical probes) | 0.45 |
| Water (ground water, river, lake) | 0.50 |
| VTob = 65 °C | Assumption: heating in old buildings |
| VThw = 55 °C | If both hot water and space heating are provided by the heat pump, then the supply temperature is at least 55 °C. The analysis of the coefficient of performance is first carried out using this temperature and then compared with the two alternative supply temperatures of 45 °C and 65 °C. |
| VTls = 45 °C | Assumption: large-surface heating systems such as panel heating |
| Lahn (Marburg GER) | Danube (Heilbronn, Ulm GER) [3] | Landwehr Canal (Berlin GER) [9] | Rhine (CH) [8] | Thames, Trent (UK) [7] | |
|---|---|---|---|---|---|
| River scale | Mean discharges between 5 m3/s to 40 m3/s | MNQ (Heilbronn) = 30 m3/s) MNQ (Ulm) = 50 m3/s | Qconst = 2.2 m3/s | Mean discharges between 500 m3/s und 1500 m3/s | Qn95 (Thames) = 15 m3/s to 30 m3/s Qn95 (Trent) = 30 m3/s to 50 m3/s |
| Conditions of heat extraction | Discharges in the lower confidence interval 2 K to 3 K temperature change in water body (standard derivation) 1 °C minimum temperature after heat extraction | 20% of MNQ 1 K temperature in the water body 2 °C minimum temperature after heat extraction | Constant discharge in the millrace 1 K temperature change in the water body | Minimum daily mean discharge per month for the last 77 years 3 K temperature change in the water body 2 °C minimum temperature after heat extraction | Winter Q95 (i.e., discharge exceeded 95% of the time in winter) 3 K temperature change in the water body 0 °C minimum temperature after heat extraction |
| Heat potential | 30 MW (summer) to 100 MW (winter) | Donau/Heilbronn 70 MW (summer) to 120 MW (winter) Donau/Ulm 70 MW (summer) to 200 MW (winter) | 9.2 MW | 4000 MW (summer) to 9000 MW (winter) | Thames 25 MW to 100 MW Trent >100 MW |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gappisch, J.; Borchardt, S.; Lehmann, B. Potential Analysis and Feasibility Study on the Hydrothermal Utilization of Rivers—Using Marburg on the Lahn River as Case Study. Energies 2024, 17, 36. https://doi.org/10.3390/en17010036
Gappisch J, Borchardt S, Lehmann B. Potential Analysis and Feasibility Study on the Hydrothermal Utilization of Rivers—Using Marburg on the Lahn River as Case Study. Energies. 2024; 17(1):36. https://doi.org/10.3390/en17010036
Chicago/Turabian StyleGappisch, Jessika, Steve Borchardt, and Boris Lehmann. 2024. "Potential Analysis and Feasibility Study on the Hydrothermal Utilization of Rivers—Using Marburg on the Lahn River as Case Study" Energies 17, no. 1: 36. https://doi.org/10.3390/en17010036
APA StyleGappisch, J., Borchardt, S., & Lehmann, B. (2024). Potential Analysis and Feasibility Study on the Hydrothermal Utilization of Rivers—Using Marburg on the Lahn River as Case Study. Energies, 17(1), 36. https://doi.org/10.3390/en17010036






