Performance Enhancement of the Basic Solar Chimney Power Plant Integrated with an Adsorption Cooling System with Heat Recovery from the Condenser
Abstract
:1. Introduction
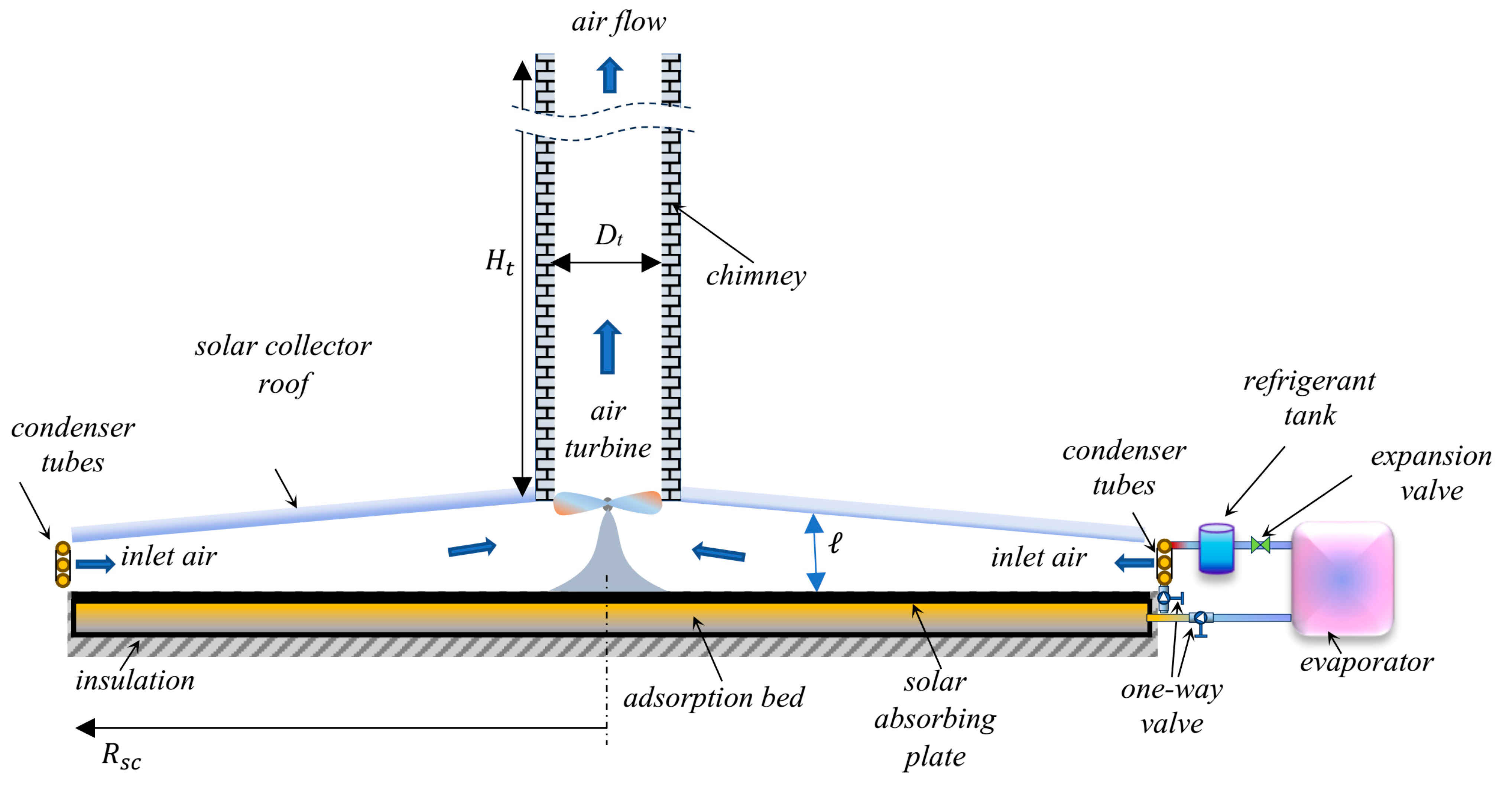
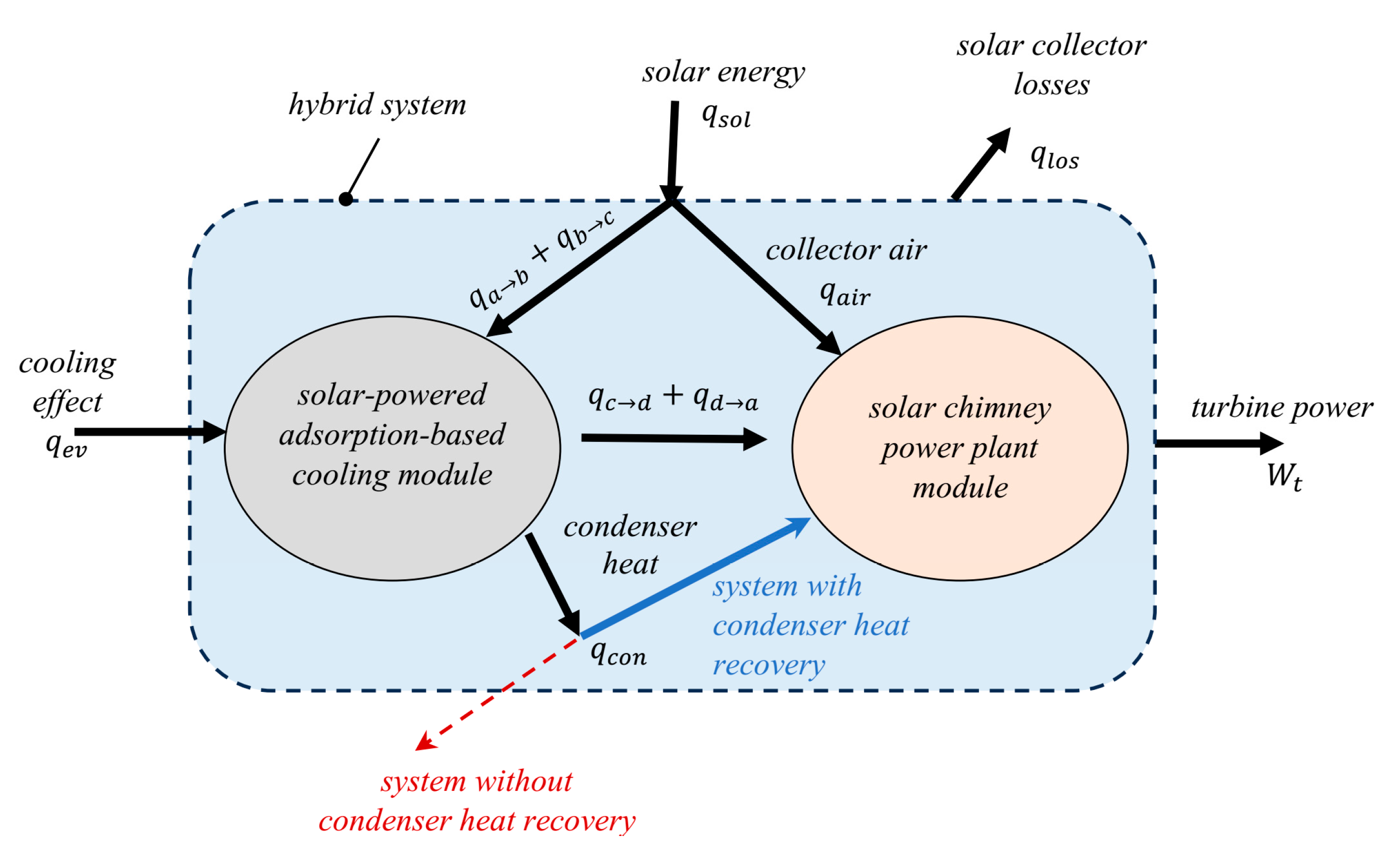
2. The Modified System Configuration and Operation
2.1. The Physical Model of the Modified System
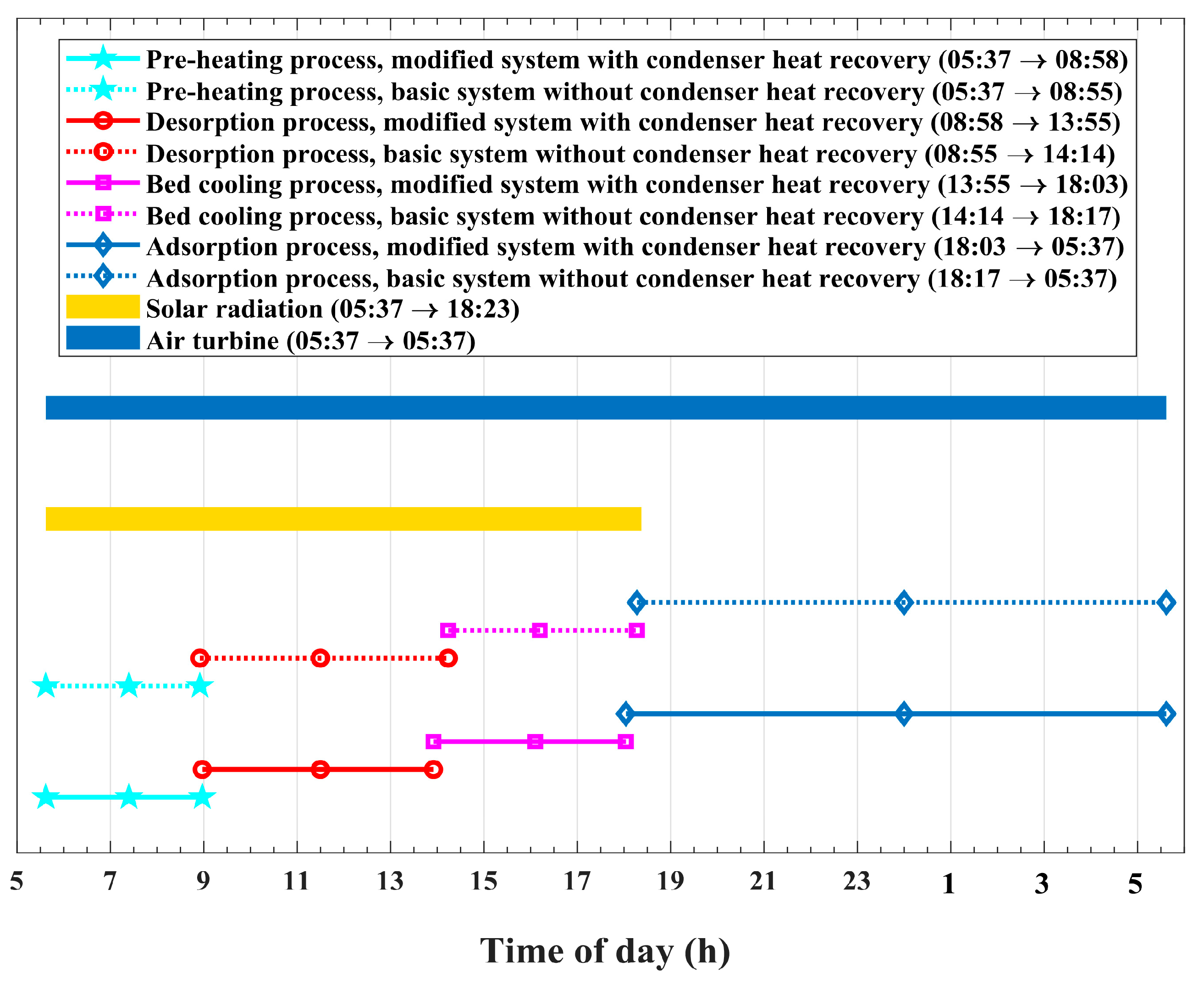
2.2. The Operation of the Modified System
3. The Mathematical Model
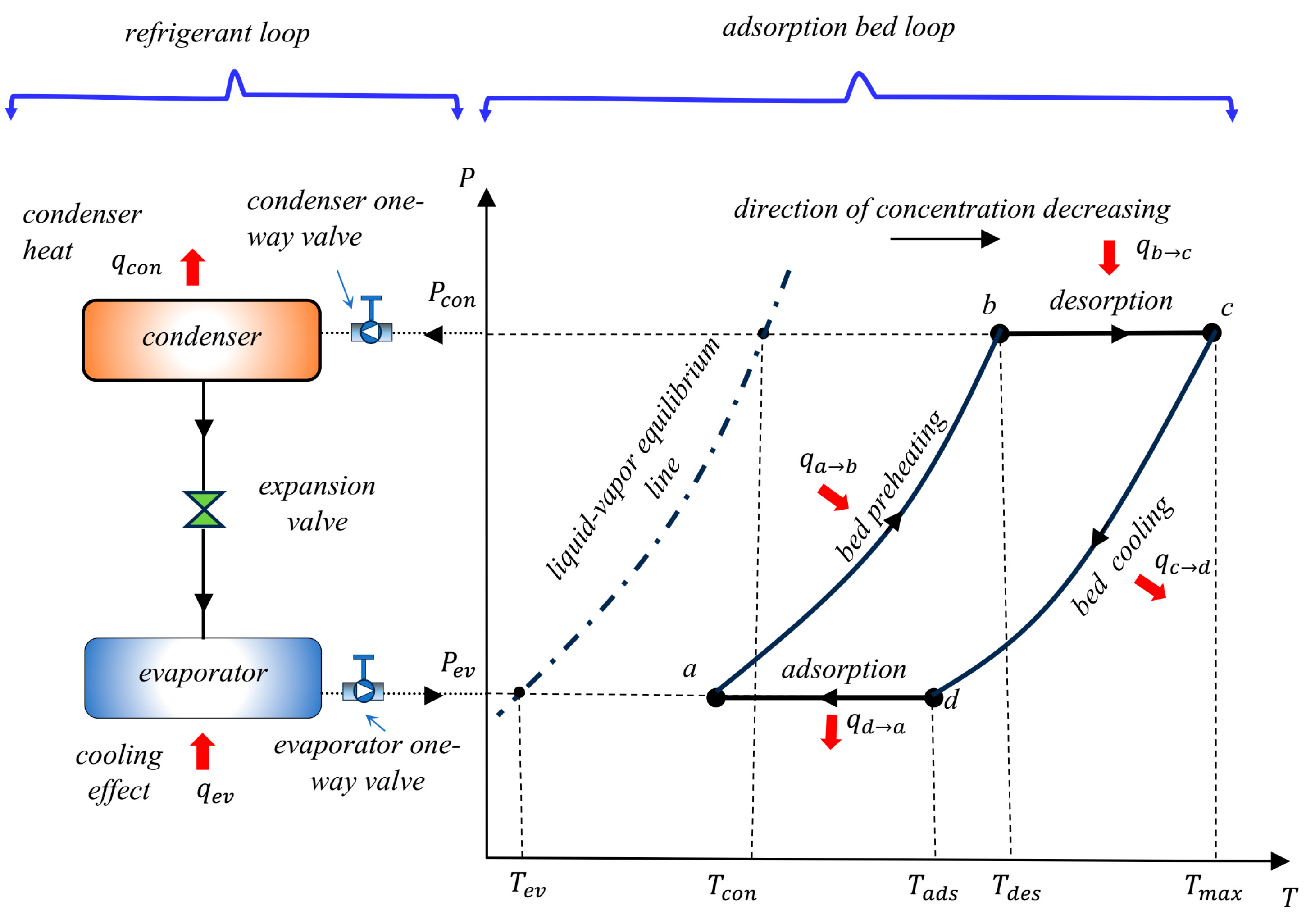
3.1. Mathematical Modeling of the Adsorption Reactor
3.1.1. The Refrigerant Equation of Conservation of Mass
3.1.2. The Refrigerant Equation of Conservation of Energy
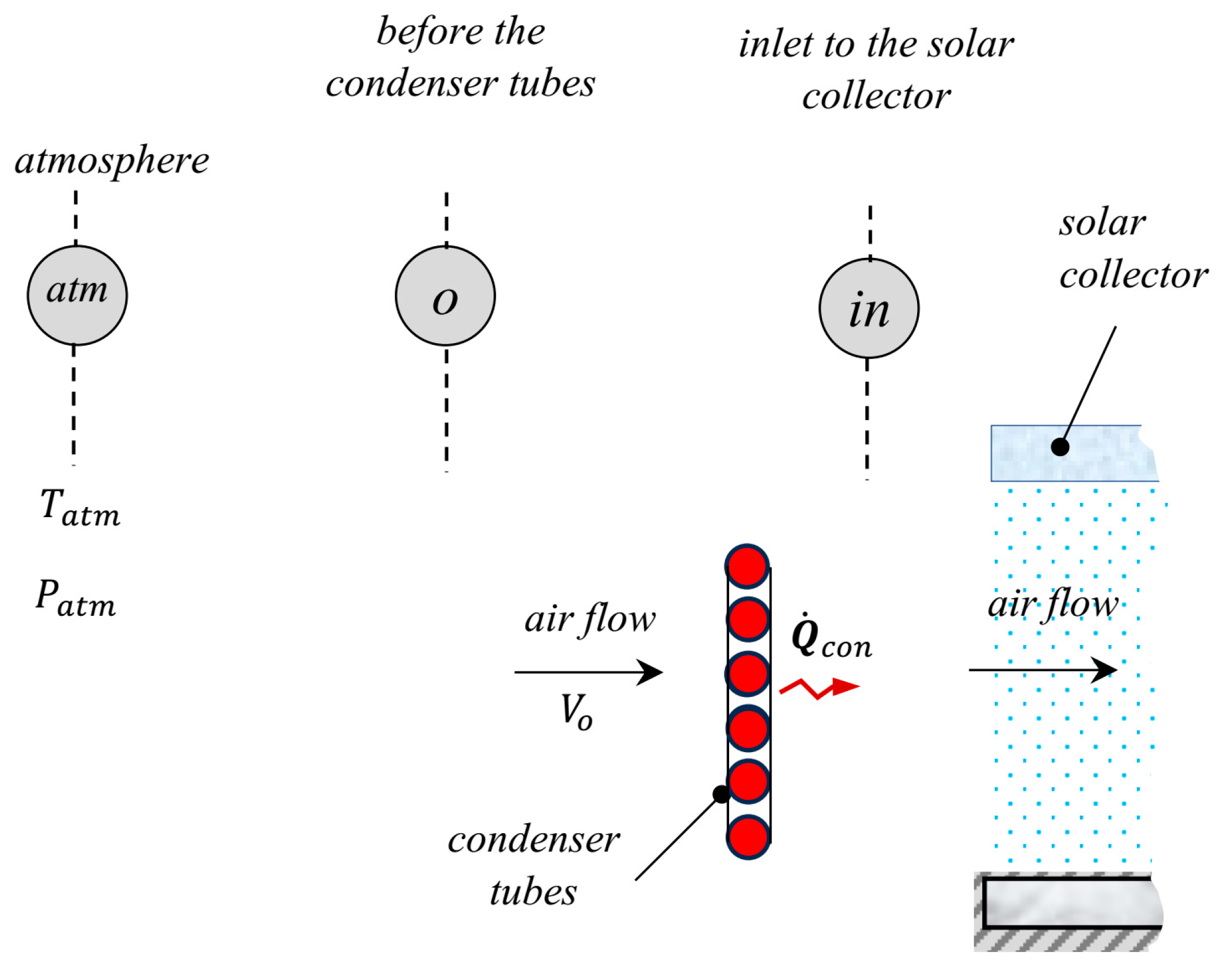
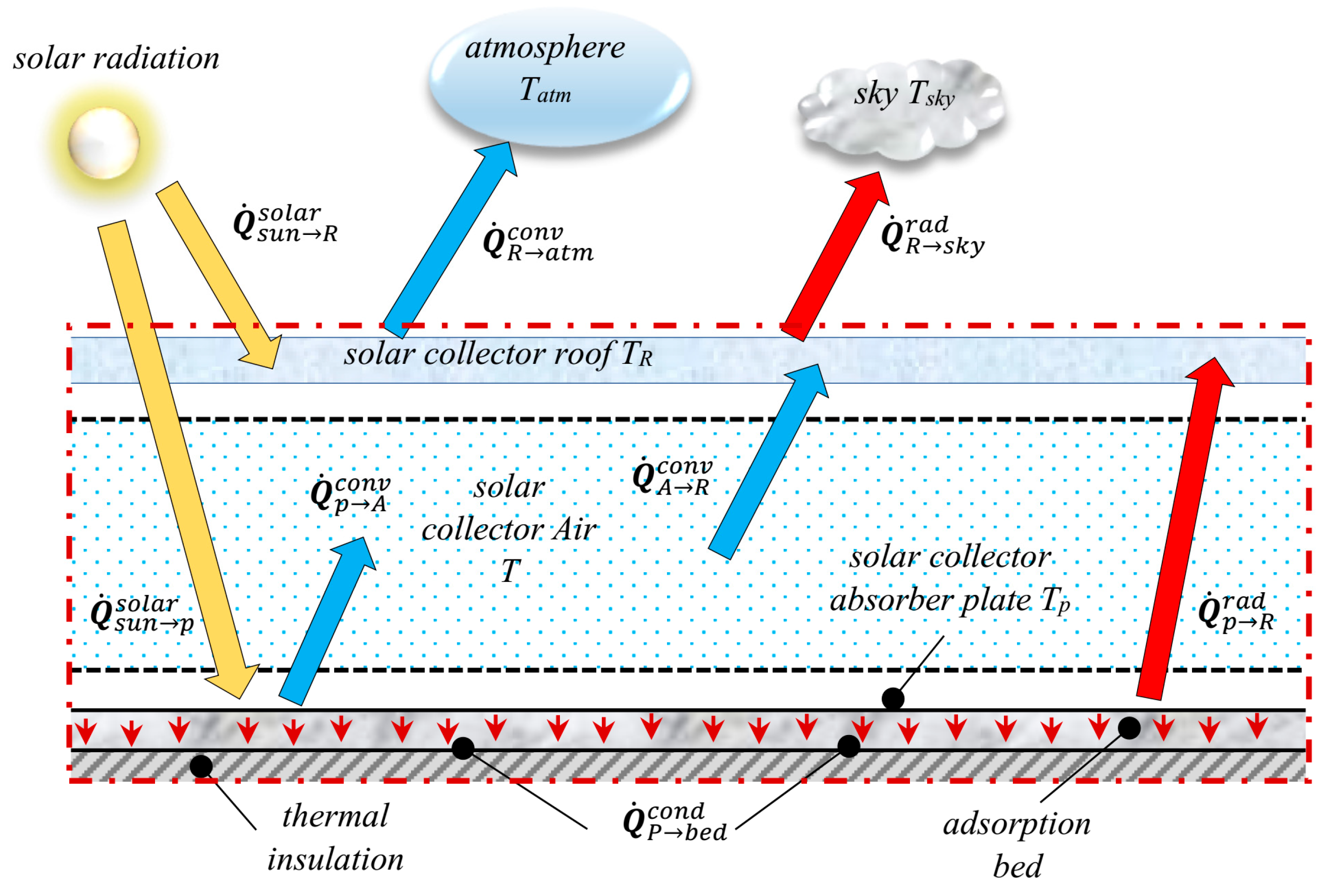
3.2. Mathematical Modeling of the Solar Collector
4. The Climatic Data and the Values of the Simulation Parameters
5. The Numerical Model and the Solution Assumptions
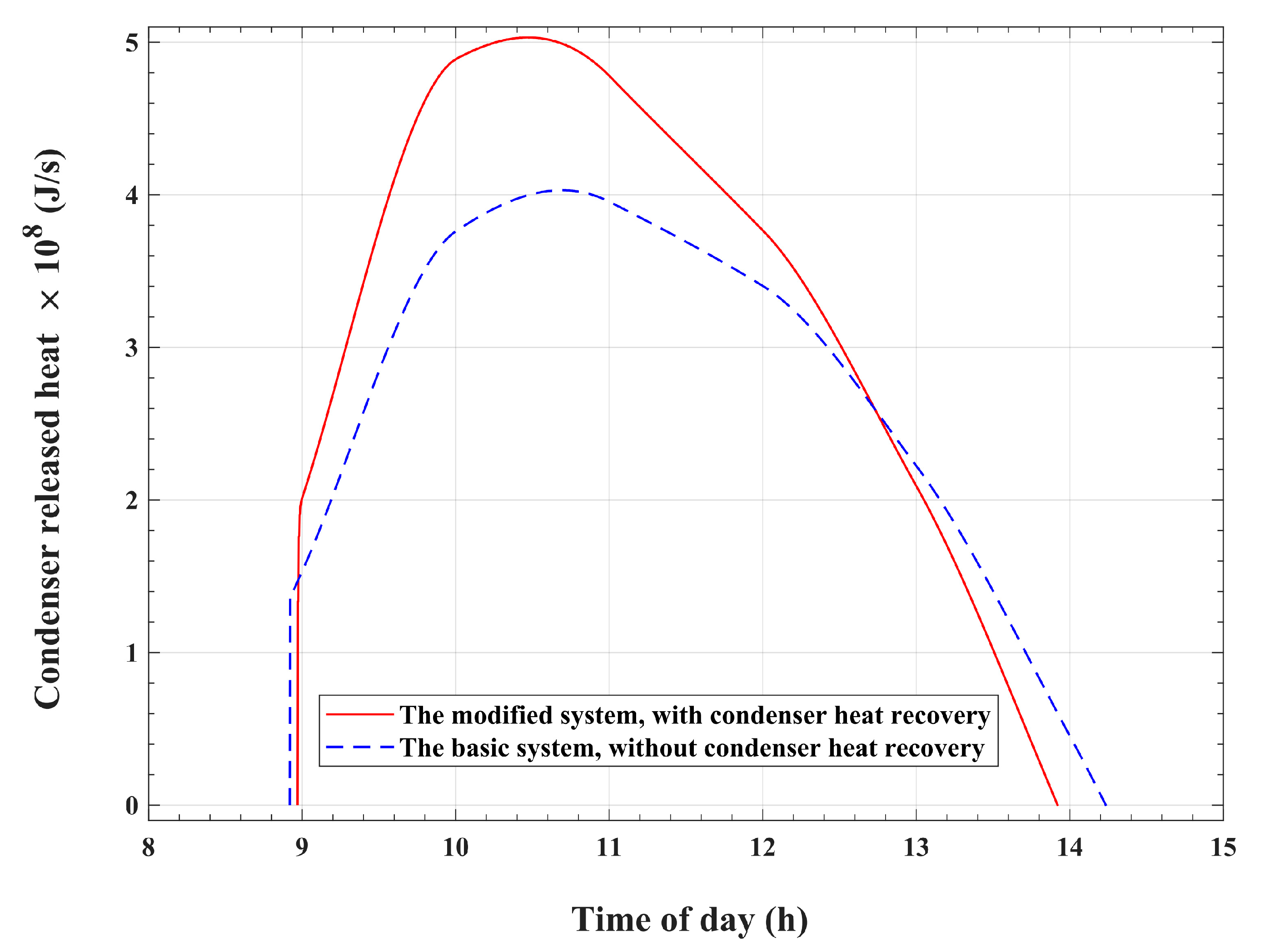
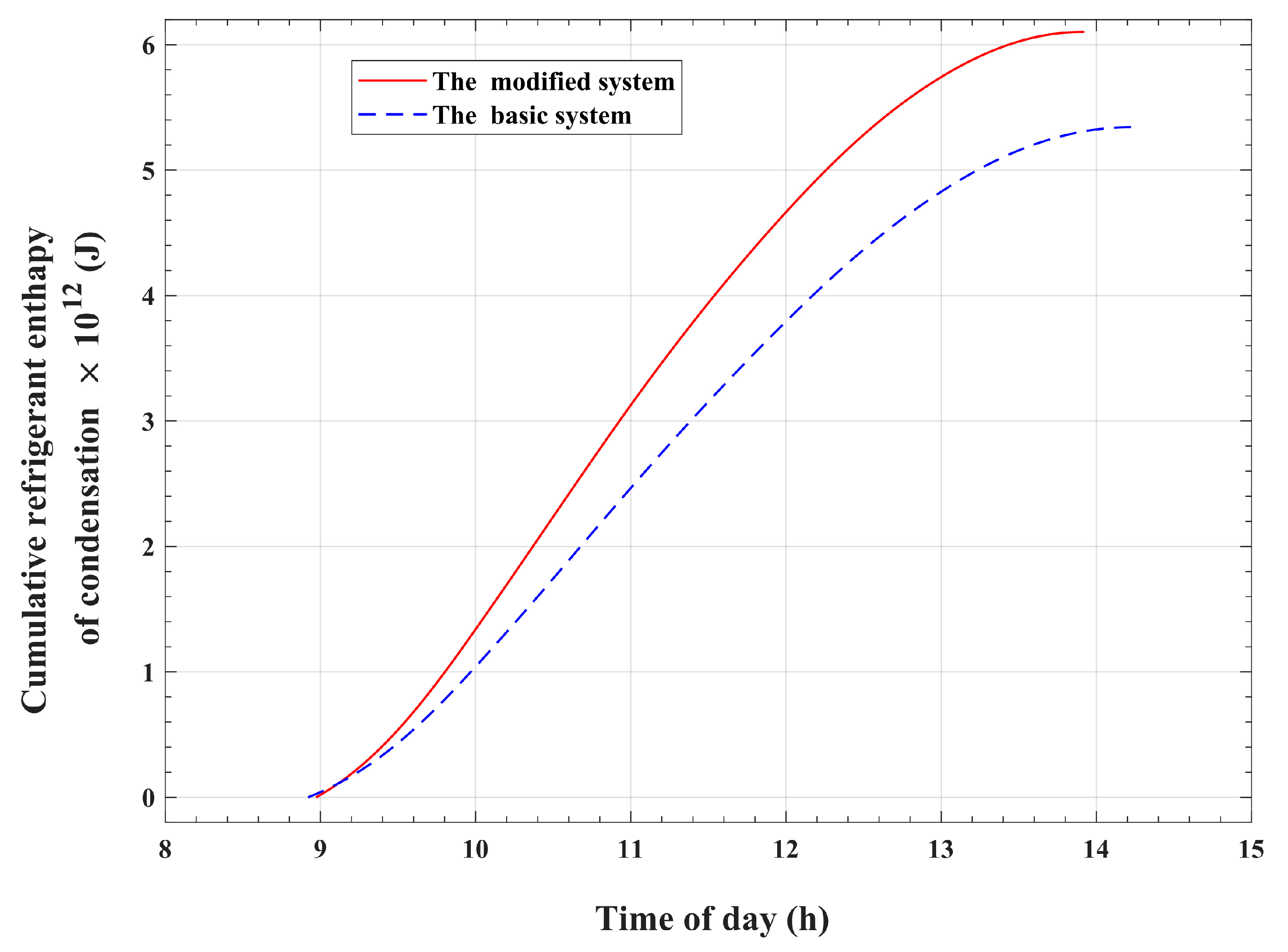
- Once the refrigerant vapor desorbs from the bed, it condenses inside the condenser tubes.
- All the heat of condensation is transferred to the air stream that crosses the condenser tubes and moves towards the entrance of the solar collector without any thermal loss.
- The thermodynamic properties of water are extracted from the tabulated data.
- One-dimensional heat transfer in the radial direction is considered.
- The sorption bed has perfect thermal insulation from the bottom as well as from side edges to prevent loss of heat to the ground.
- The sorption reactor is modeled as a lumped system that has uniform pressure and temperature distributions.
- Heat capacity effects of the roof and the absorber plate are ignored.
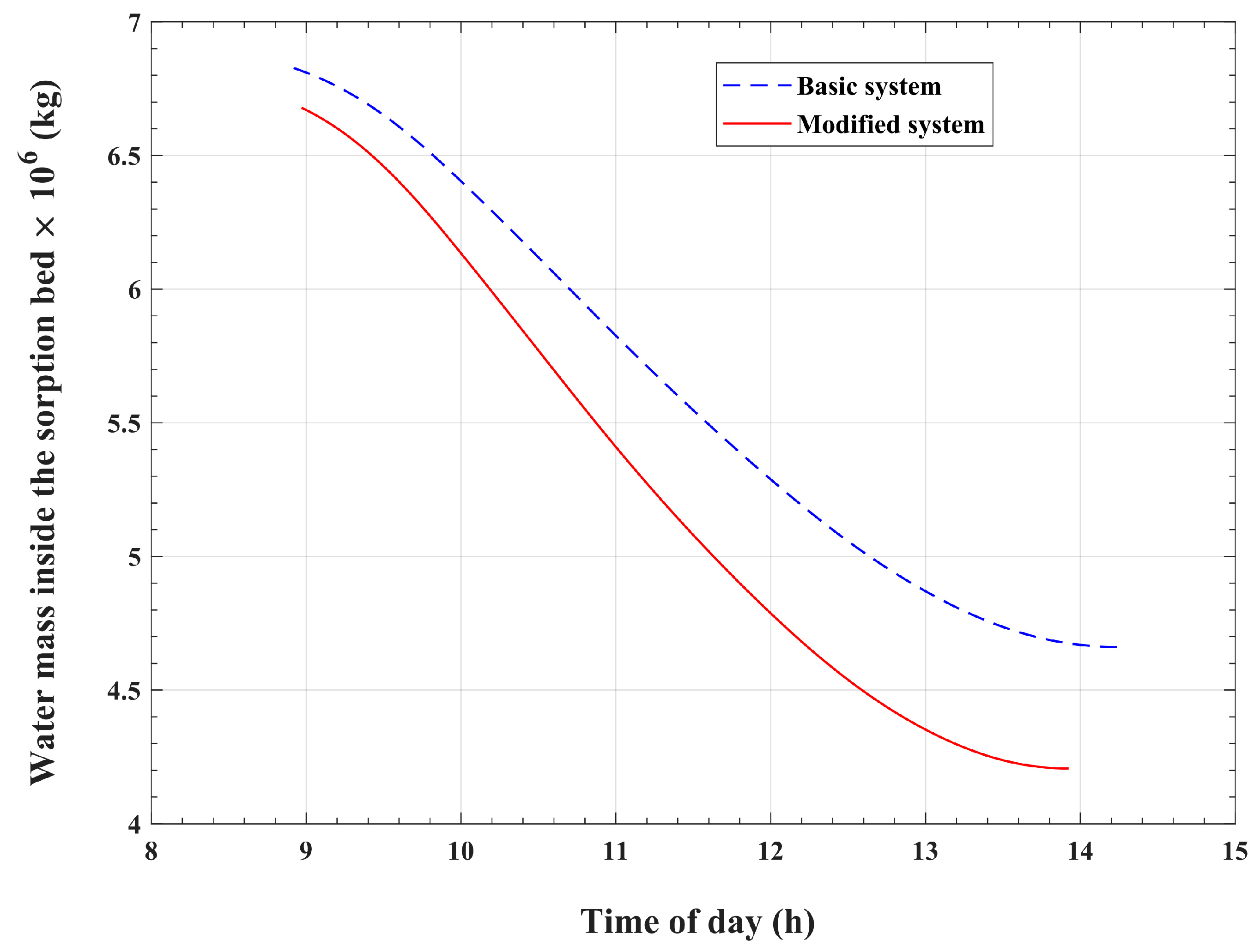
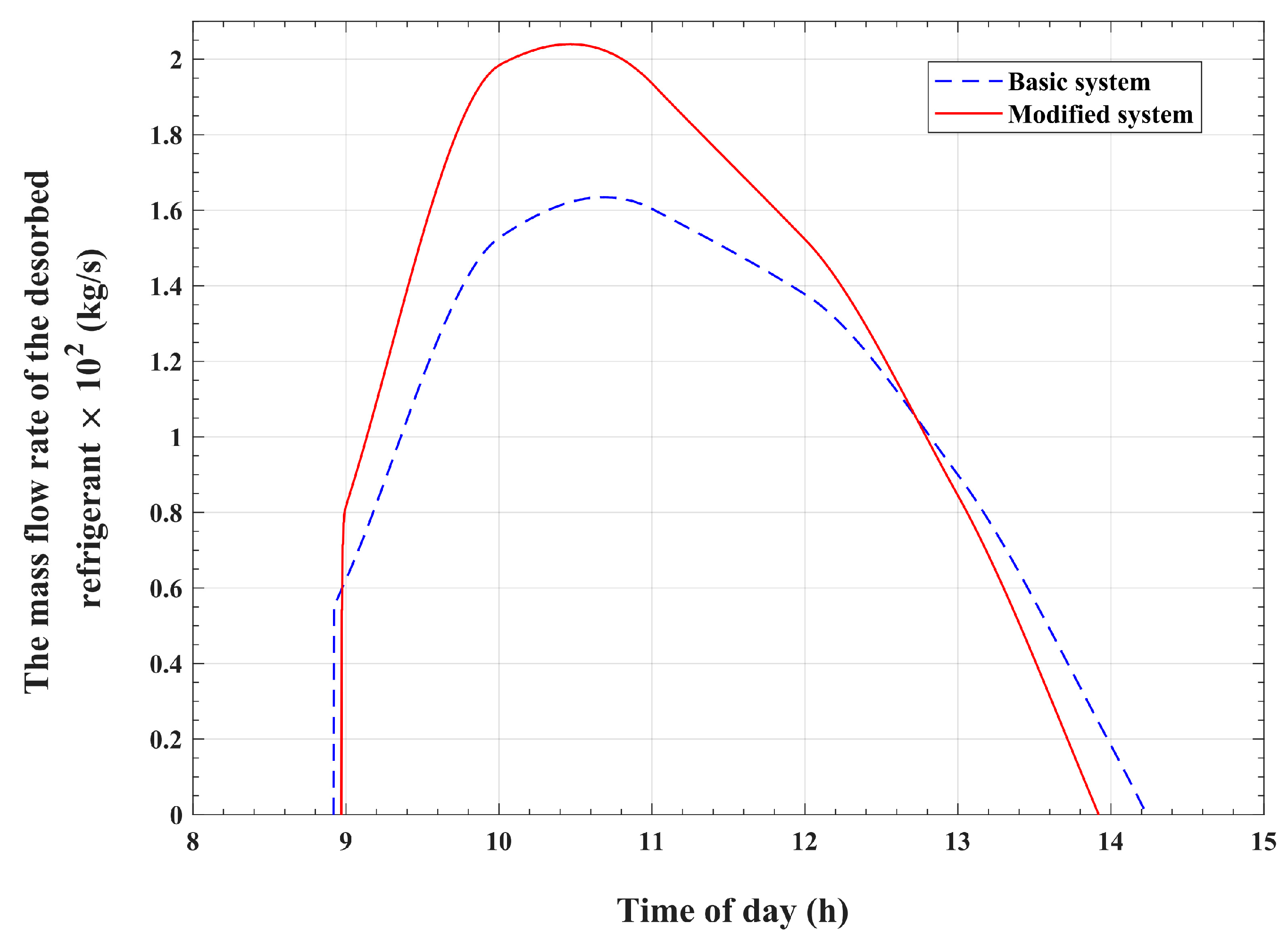
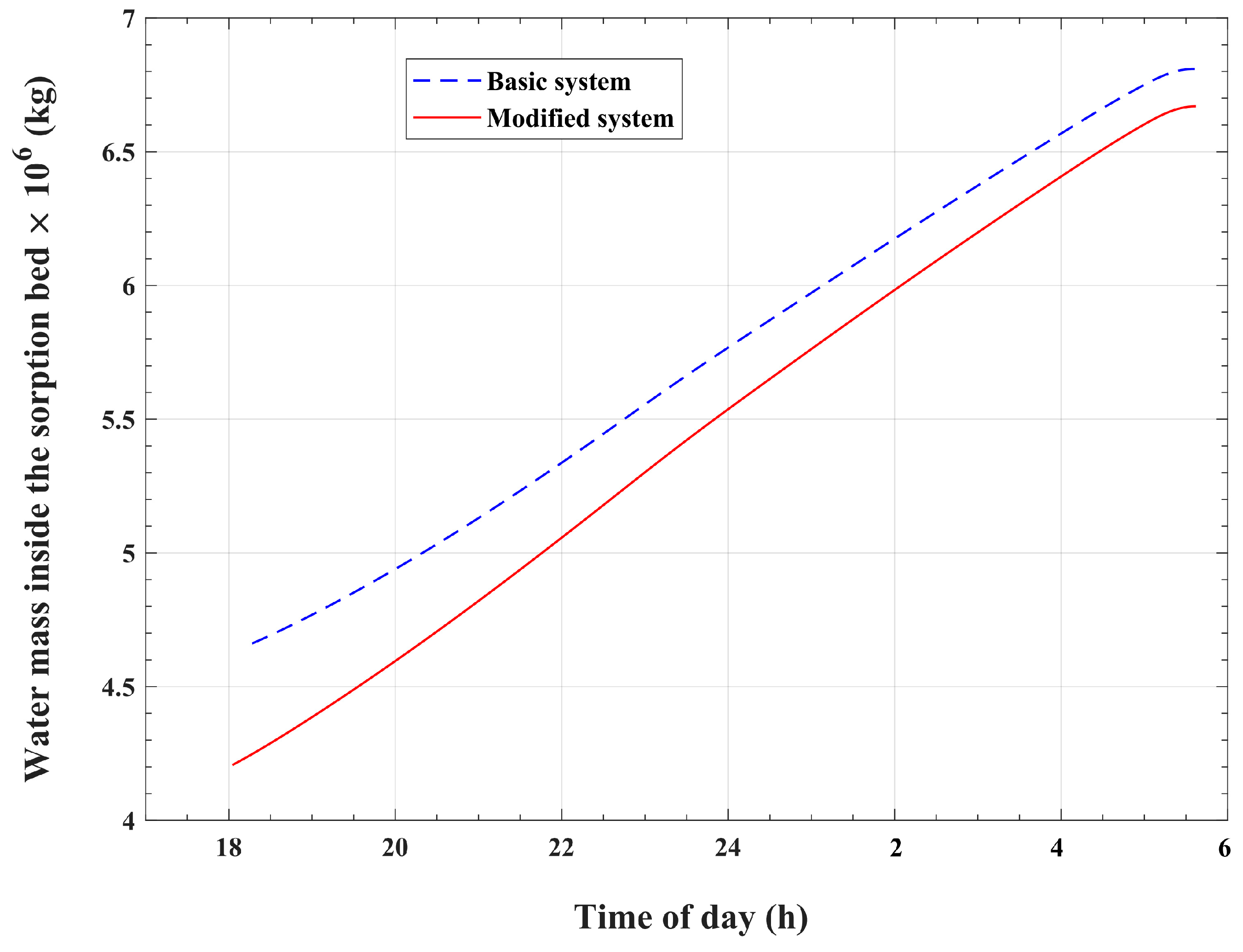
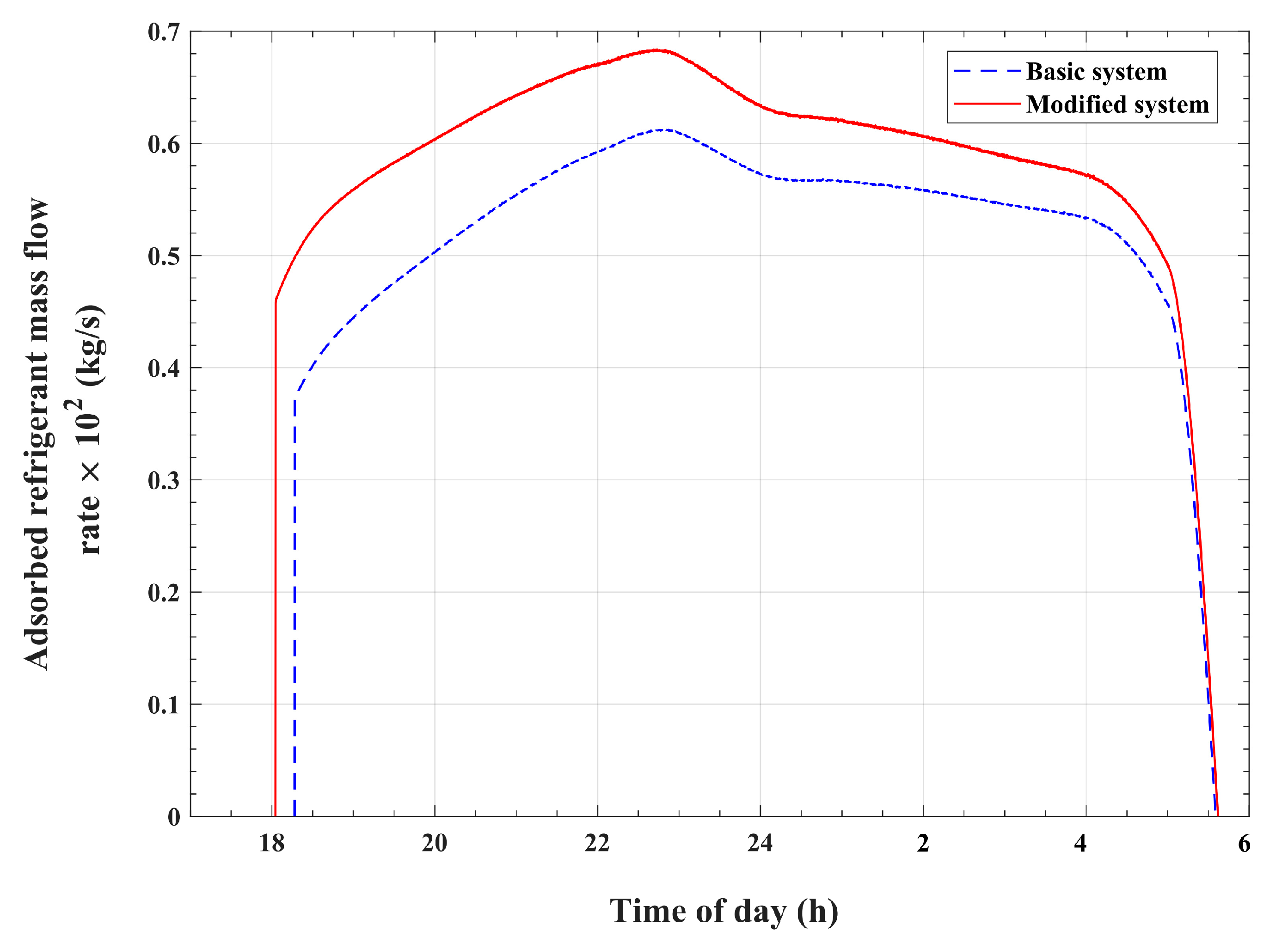
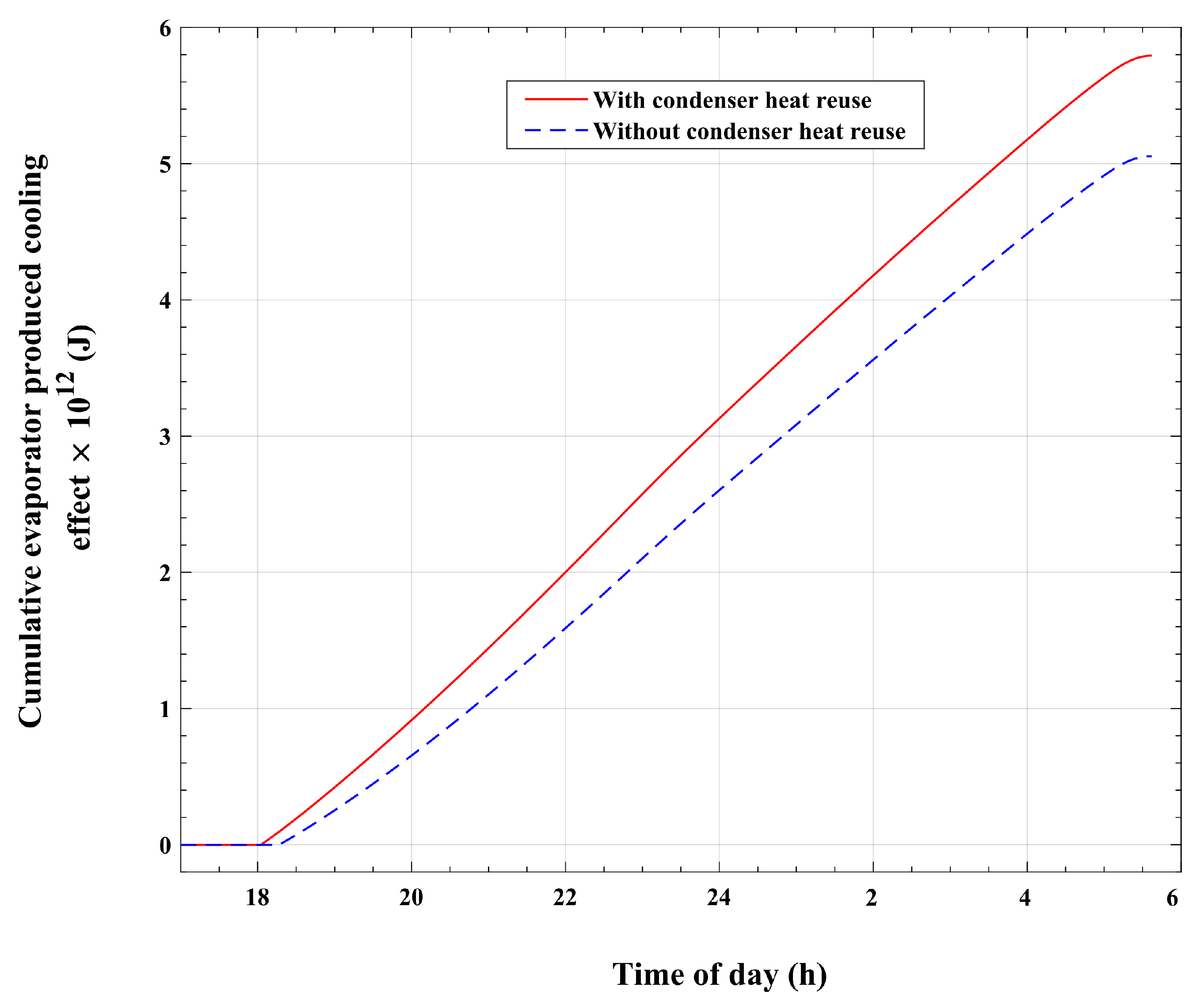
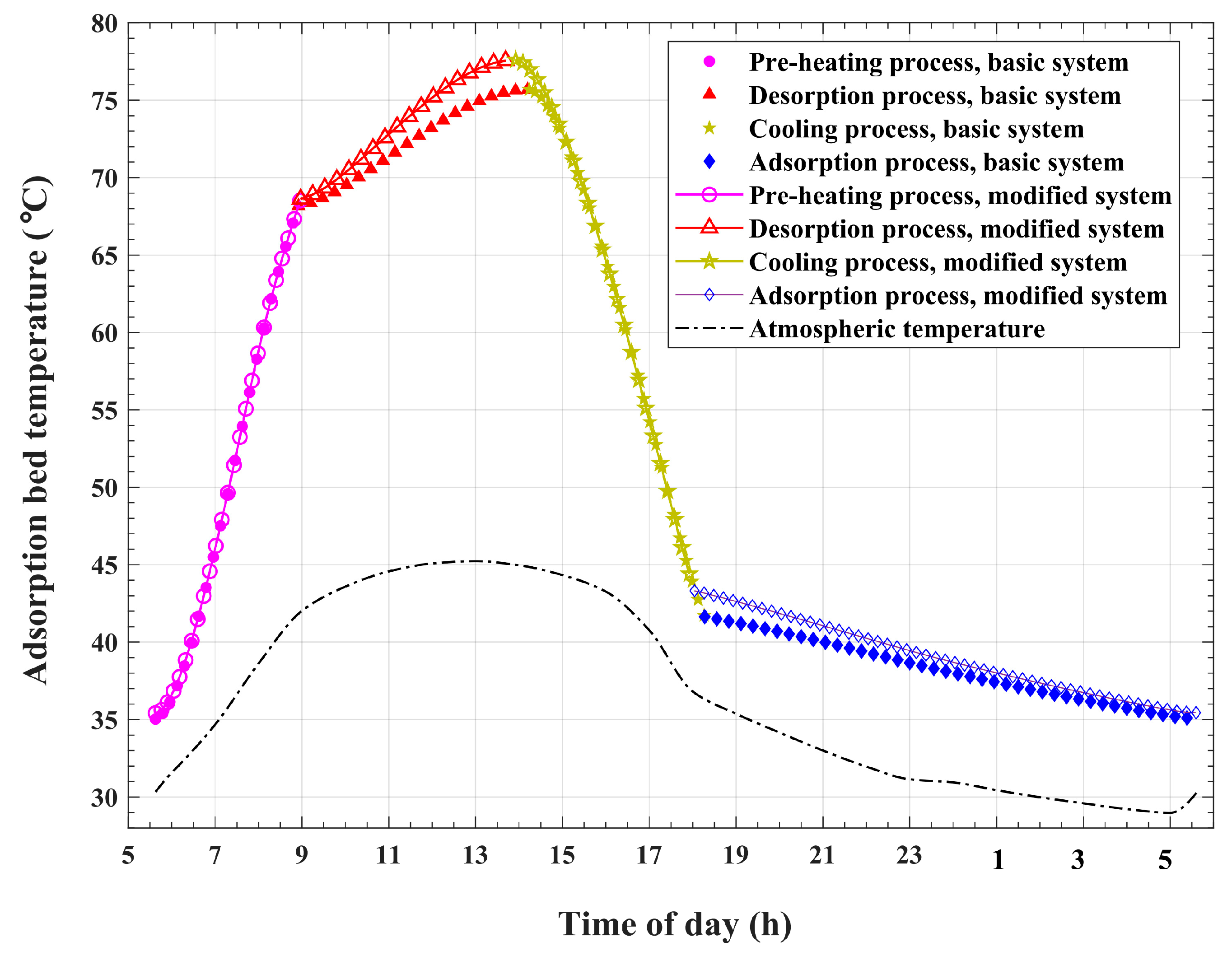
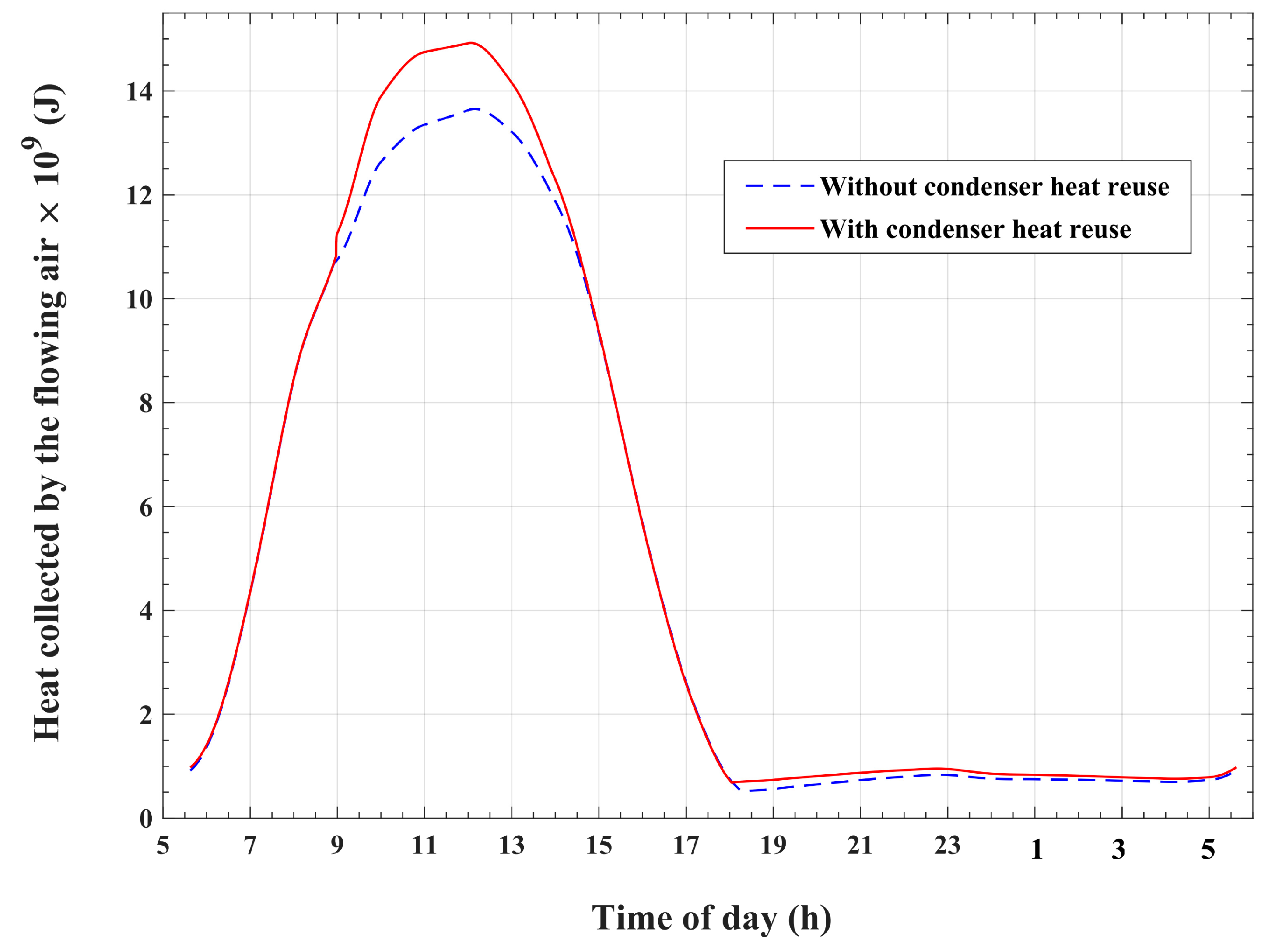
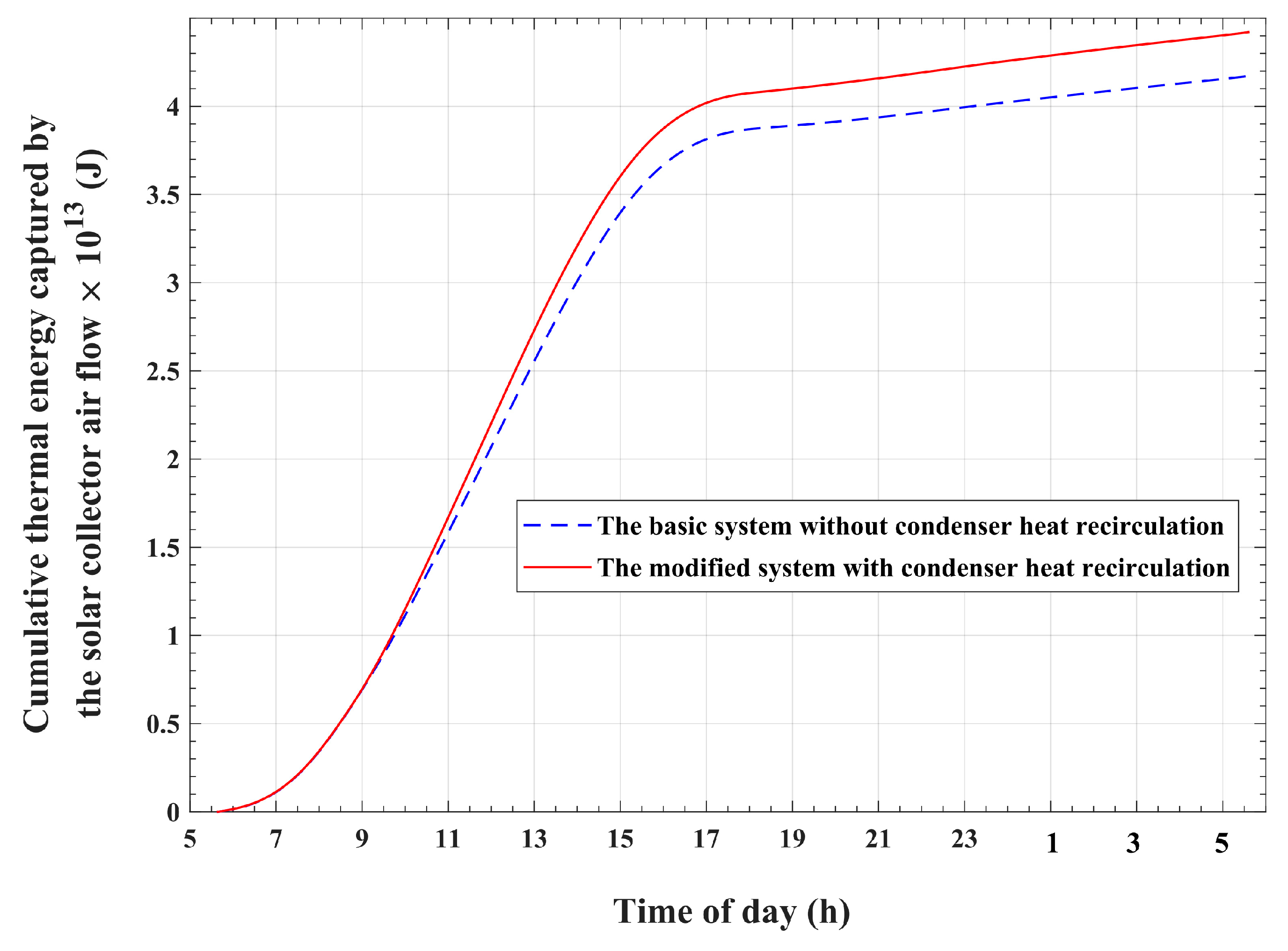
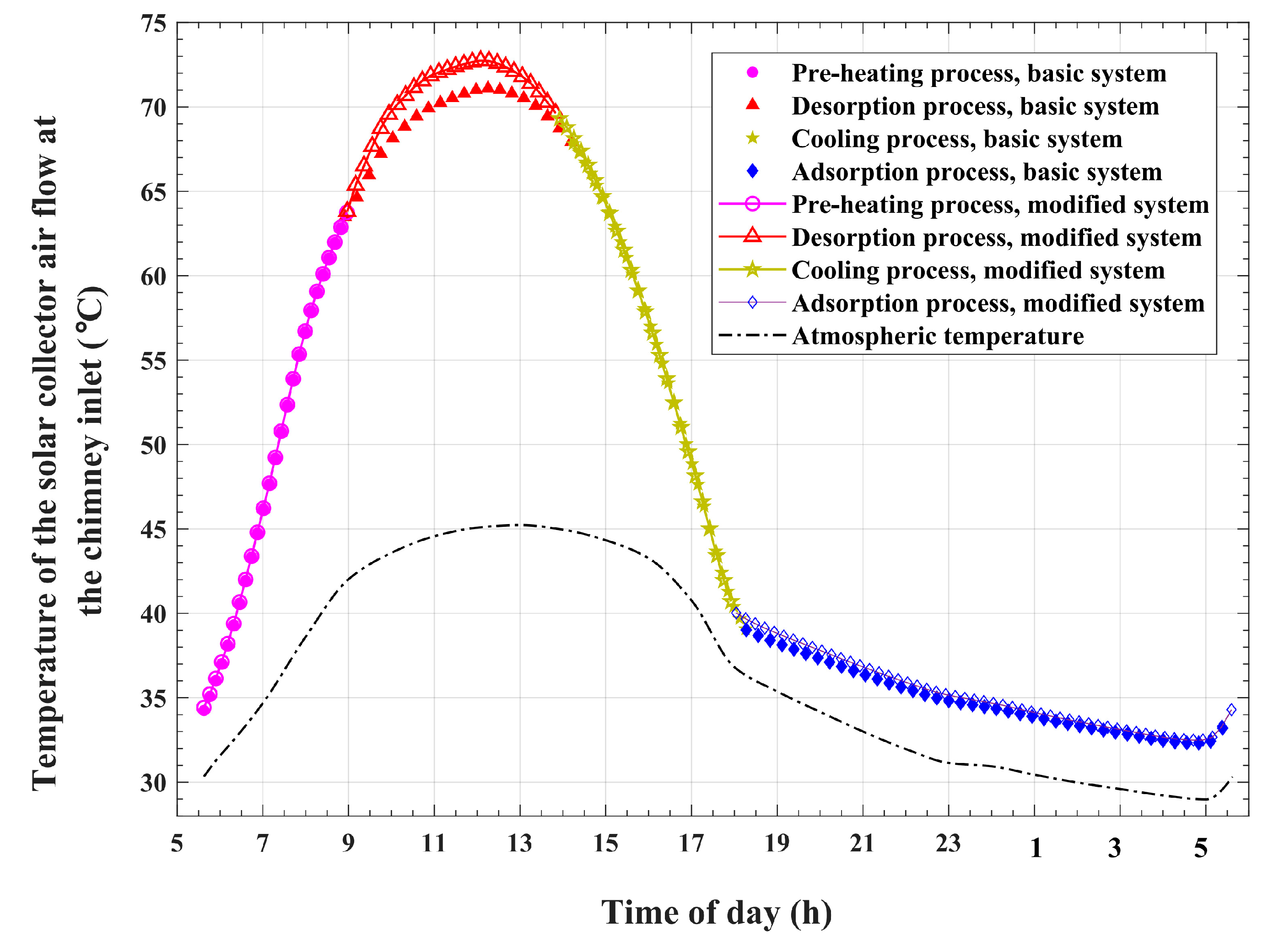
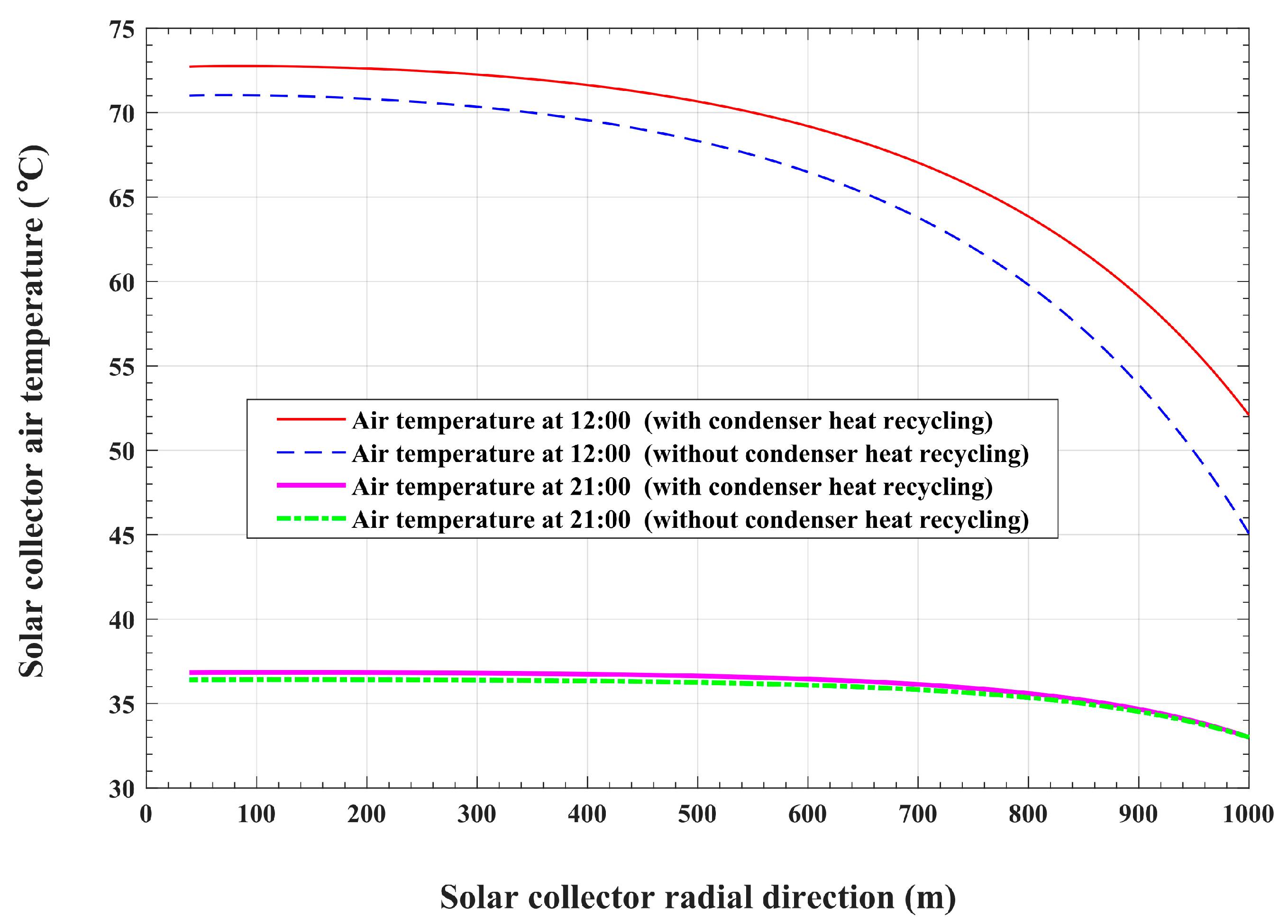
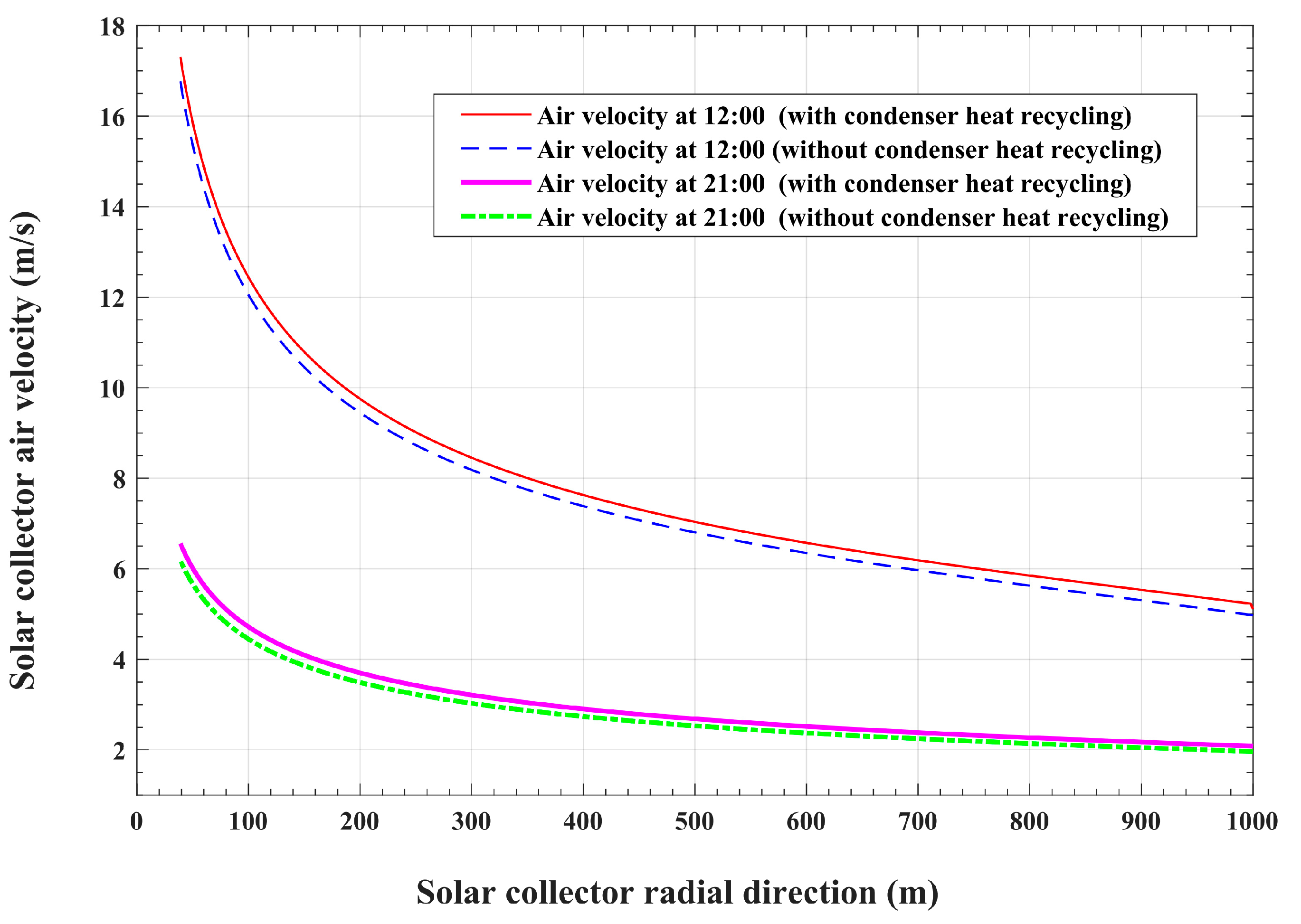
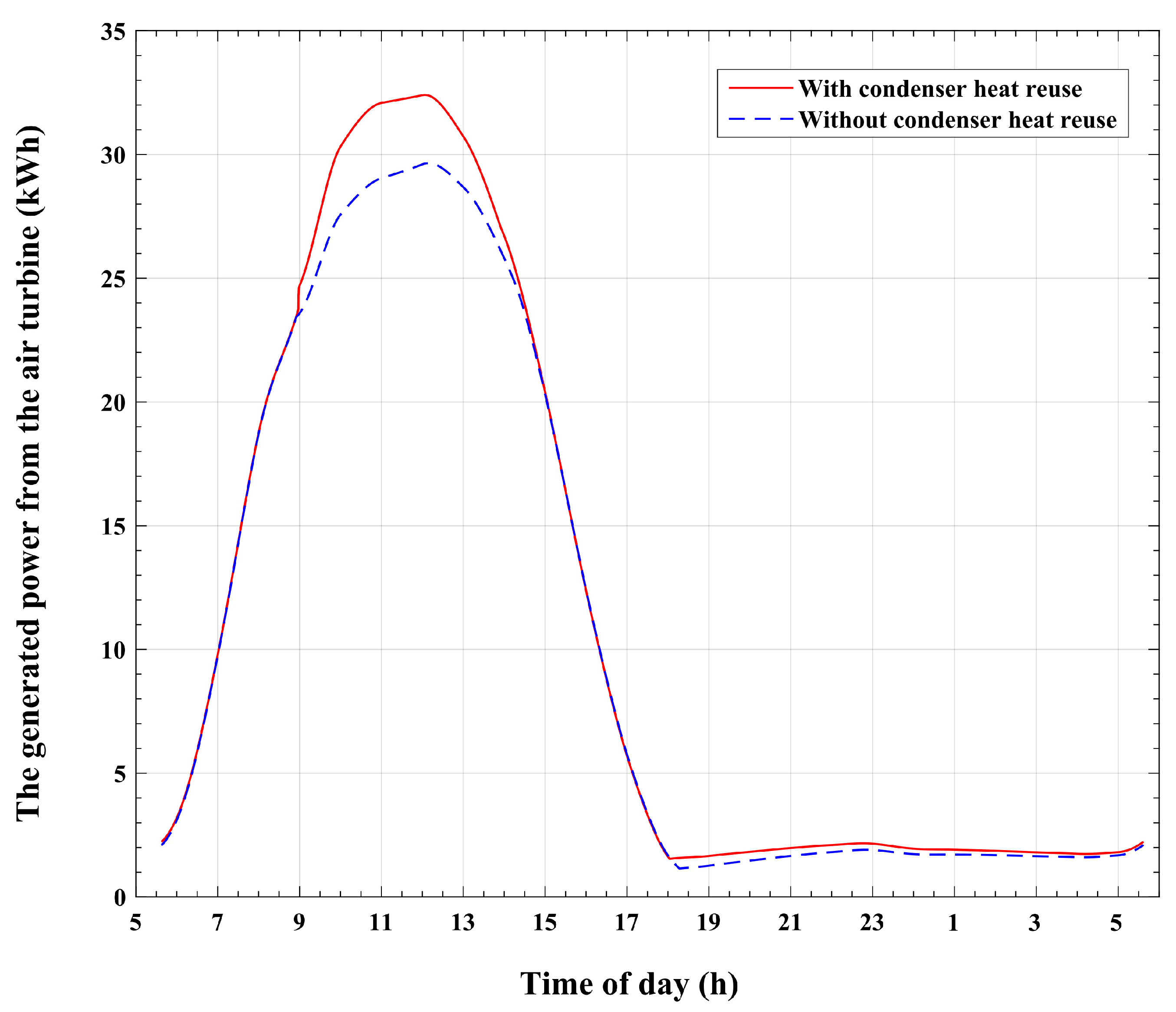
6. Results and Discussion
7. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ali, M.H.; Kurjak, Z.; Beke, J. Modelling and Simulation of Solar Chimney Power Plants in Hot and Arid Regions Using Experimental Weather Conditions. Int. J. Thermofluids 2023, 20, 100434. [Google Scholar] [CrossRef]
- Kumar Mandal, D.; Biswas, N.; Manna, N.K.; Benim, A.C. Impact of Chimney Divergence and Sloped Absorber on Energy Efficacy of a Solar Chimney Power Plant (SCPP). Ain Shams Eng. J. 2023, 15, 102390. [Google Scholar] [CrossRef]
- Hassan, H.Z. Transient Analysis of a Solar Chimney Power Plant Integrated with a Solid-Sorption Cooling System for Combined Power and Chilled Water Production. Energies 2022, 15, 6793. [Google Scholar] [CrossRef]
- Ghafoor, A.; Munir, A. Worldwide Overview of Solar Thermal Cooling Technologies. Renew. Sustain. Energy Rev. 2015, 43, 763–774. [Google Scholar] [CrossRef]
- Hassan, H.Z.; Mohamad, A.A.; Al-Ansary, H.A.; Alyousef, Y.M. Dynamic Analysis of the CTAR (Constant Temperature Adsorption Refrigeration) Cycle. Energy 2014, 77, 852–858. [Google Scholar] [CrossRef]
- Hassan, H.Z.; Atteia, G.E. Controlled Strategy for the Cooling Effect of the Solar-Driven Constant-Temperature Solid Sorption System. Int. Rev. Mech. Eng. (IREME) 2021, 15, 464–474. [Google Scholar] [CrossRef]
- Aristov, Y.I. Adsorptive Conversion of Ultralow-Temperature Heat: Thermodynamic Issues. Energy 2021, 236, 121892. [Google Scholar] [CrossRef]
- Chauhan, P.R.; Kaushik, S.C.; Tyagi, S.K. A Review on Thermal Performance Enhancement of Green Cooling System Using Different Adsorbent/Refrigerant Pairs. Energy Convers. Manag. X 2022, 14, 100225. [Google Scholar] [CrossRef]
- Hassan, H.Z. Sensitivity Analysis of the CO-SAR Machine. Int. Rev. Mech. Eng. (IREME) 2015, 9, 21–30. [Google Scholar] [CrossRef]
- Hassan, H.Z. Assessment of the Adsorber Surface Density Influence on the Performance and Operation of the Intermittent SAR System. Energy Convers. Manag. 2015, 94, 19–27. [Google Scholar] [CrossRef]
- Dias, J.M.S.; Costa, V.A.F. Adsorption Cooler Design, Dynamic Modeling, and Performance Analyses. Clean Technol. 2022, 4, 1152–1161. [Google Scholar] [CrossRef]
- Chauhan, P.R.; Kaushik, S.C.; Tyagi, S.K. Current Status and Technological Advancements in Adsorption Refrigeration Systems: A Review. Renew. Sustain. Energy Rev. 2022, 154, 111808. [Google Scholar] [CrossRef]
- Sah, R.P.; Choudhury, B.; Das, R.K. A Review on Low Grade Heat Powered Adsorption Cooling Systems for Ice Production. Renew. Sustain. Energy Rev. 2016, 62, 109–120. [Google Scholar] [CrossRef]
- Makahleh, F.M.; Badran, A.A.; Attar, H.; Amer, A.; Al-Maaitah, A.A. Modeling and Simulation of a Two-Stage Air Cooled Adsorption Chiller with Heat Recovery Part I: Physical and Mathematical Performance Model. Appl. Sci. 2022, 12, 6542. [Google Scholar] [CrossRef]
- Makahleh, F.M.; Badran, A.A.; Attar, H.; Amer, A.; Al-Maaitah, A.A. Modeling and Simulation of a Two-Stage Air-Cooled Adsorption Chiller with Heat Recovery Part II: Parametric Study. Appl. Sci. 2022, 12, 5156. [Google Scholar] [CrossRef]
- Carotenuto, A.; Figaj, R.D.; Vanoli, L. A Novel Solar-Geothermal District Heating, Cooling and Domestic Hot Water System: Dynamic Simulation and Energy-Economic Analysis. Energy 2017, 141, 2652–2669. [Google Scholar] [CrossRef]
- Hamdy, M.; Askalany, A.A.; Harby, K.; Kora, N. An Overview on Adsorption Cooling Systems Powered by Waste Heat from Internal Combustion Engine. Renew. Sustain. Energy Rev. 2015, 51, 1223–1234. [Google Scholar] [CrossRef]
- Golparvar, B.; Niazmand, H. Adsorption Cooling Systems for Heavy Trucks A/C Applications Driven by Exhaust and Coolant Waste Heats. Appl. Therm. Eng. 2018, 135, 158–169. [Google Scholar] [CrossRef]
- Lu, Z. Comprehensive Investigation of Cooling, Heating, and Power Generation Performance in Adsorption Systems Using Compound Adsorbents: Experimental and Computational Analysis. Sustainability 2023, 15, 15202. [Google Scholar] [CrossRef]
- Li, T.X.; Wang, R.Z.; Wang, L.W.; Lu, Z.S.; Chen, C.J. Performance Study of a High Efficient Multifunction Heat Pipe Type Adsorption Ice Making System with Novel Mass and Heat Recovery Processes. Int. J. Therm. Sci. 2007, 46, 1267–1274. [Google Scholar] [CrossRef]
- Mostafa, M.; Ezzeldien, M.; Attalla, M.; Ghazaly, N.M.; Alrowaili, Z.A.; Hasaneen, M.F.; Shmroukh, A.N. Comparison of Different Adsorption Pairs Based on Zeotropic and Azeotropic Mixture Refrigerants for Solar Adsorption Ice Maker. Environ. Sci. Pollut. Res. 2021, 28, 41479–41491. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.W.; Wu, J.Y.; Wang, R.Z.; Xu, Y.X.; Wang, S.G. Experimental Study of a Solidified Activated Carbon-Methanol Adsorption Ice Maker. Appl. Therm. Eng. 2003, 23, 1453–1462. [Google Scholar] [CrossRef]
- Dawoud, B. A Hybrid Solar-Assisted Adsorption Cooling Unit for Vaccine Storage. Renew. Energy 2007, 32, 947–964. [Google Scholar] [CrossRef]
- Kasi, P.; Cheralathan, M. Review of Cascade Refrigeration Systems for Vaccine Storage. J. Phys. Conf. Ser. 2021, 2054, 012041. [Google Scholar] [CrossRef]
- Tamainot-Telto, Z.; Metcalf, S.J.; Yande, N.N. Adsorption Solar Air Conditioning System for Singapore Climate. Energies 2022, 15, 6537. [Google Scholar] [CrossRef]
- Sumathy, K.; Yong, L.; Steinhagen, H.M.; Kerskes, H. Performance Analysis of a Modified Two-Bed Solar-Adsorption Air-Conditioning System. Int. J. Energy Res. 2009, 33, 675–686. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, R. Experimental and Simulation Analysis of Low Temperature Heat Sources Driven Adsorption Air Conditioning, Refrigeration, Integrating Ammonia, and Organic Expanding Power Generation. Int. J. Energy Res. 2018, 42, 4157–4169. [Google Scholar] [CrossRef]
- Palomba, V.; Varvagiannis, E.; Karellas, S.; Frazzica, A. Hybrid Adsorption-Compression Systems for Air Conditioning in Efficient Buildings: Design through Validated Dynamic Models. Energies 2019, 12, 1161. [Google Scholar] [CrossRef]
- Jiangzhou, S.; Wang, R.Z.; Lu, Y.Z.; Xu, Y.X.; Wu, J.Y. Experimental Investigations on Adsorption Air-Conditioner Used in Internal-Combustion Locomotive Driver-Cabin. Appl. Therm. Eng. 2002, 22, 1153–1162. [Google Scholar] [CrossRef]
- Missaoui, K.; Gabsi, S.; Frikha, N.; Kheiri, A.; El Ganaoui, M. Indigenous Fruits and Vegetables Storage Using Continuous Adsorption Refrigeration: A System Modelling. AIP Conf. Proc. 2021, 2345, 020011. [Google Scholar] [CrossRef]
- Aneke, M.; Agnew, B.; Underwood, C.; Menkiti, M. Thermodynamic Analysis of Alternative Refrigeration Cycles Driven from Waste Heat in a Food Processing Application. Int. J. Refrig. 2012, 35, 1349–1358. [Google Scholar] [CrossRef]
- Sur, A.; Sah, R.P.; Pandya, S. Milk Storage System for Remote Areas Using Solar Thermal Energy and Adsorption Cooling. Mater. Today Proc. 2020, 28, 1764–1770. [Google Scholar] [CrossRef]
- Biswas, N.; Mandal, D.K.; Bose, S.; Manna, N.K.; Benim, A.C. Experimental Treatment of Solar Chimney Power Plant—A Comprehensive Review. Energies 2023, 16, 6134. [Google Scholar] [CrossRef]
- Patel, S.K.; Prasad, D.; Ahmed, M.R. Computational Studies on the Effect of Geometric Parameters on the Performance of a Solar Chimney Power Plant. Energy Convers. Manag. 2014, 77, 424–431. [Google Scholar] [CrossRef]
- Haaf, W. Solar Chimneys Part II: Preliminary Test Results from the Manzanares Pilot Plant. Int. J. Sustain. Energy 2007, 2, 141–161. [Google Scholar] [CrossRef]
- Saad, M.; Ahmed, N.; Mahmood, M.; Sajid, M.B. Performance Enhancement of Solar Updraft Tower Plant Using Parabolic Chimney Profile Configurations: A Numerical Analysis. Energy Rep. 2022, 8, 4661–4671. [Google Scholar] [CrossRef]
- Cuce, E.; Cuce, P.M.; Carlucci, S.; Sen, H.; Kumarasamy, S.; Hasanuzzaman, M.; Daneshazarian, R. Solar Chimney Power Plants: A Review of the Concepts, Designs and Performances. Sustainability 2022, 14, 1450. [Google Scholar] [CrossRef]
- Gholamalizadeh, E.; Chung, J.D. A Comparative Study of CFD Models of a Real Wind Turbine in Solar Chimney Power Plants. Energies 2017, 10, 1674. [Google Scholar] [CrossRef]
- Danook, S.H.; Al-Bonsrulah, H.A.Z.; Hashim, I.; Veeman, D. Cfd Simulation of a 3d Solar Chimney Integrated with an Axial Turbine for Power Generation. Energies 2021, 14, 5771. [Google Scholar] [CrossRef]
- Caicedo, P.; Wood, D.; Johansen, C. Radial Turbine Design for Solar Chimney Power Plants. Energies 2021, 14, 674. [Google Scholar] [CrossRef]
- Wang, H.; Chen, J.; Dai, P.; Zhang, F.; Li, Q. Simulation and Experimental Study of the Influence of the Baffles on Solar Chimney Power Plant System. Processes 2021, 9, 902. [Google Scholar] [CrossRef]
- Ganguli, A.A.; Deshpande, S.S.; Pandit, A.B. Cfd Simulations for Performance Enhancement of a Solar Chimney Power Plant (Scpp) and Techno-economic Feasibility for a 5 Mw Scpp in an Indian Context. Energies 2021, 14, 3342. [Google Scholar] [CrossRef]
- Ahmed, M.R.; Patel, S.K. Computational and Experimental Studies on Solar Chimney Power Plants for Power Generation in Pacific Island Countries. Energy Convers. Manag. 2017, 149, 61–78. [Google Scholar] [CrossRef]
- Torabi, M.R.; Hosseini, M.; Akbari, O.A.; Afrouzi, H.H.; Toghraie, D.; Kashani, A.; Alizadeh, A. Investigation the Performance of Solar Chimney Power Plant for Improving the Efficiency and Increasing the Outlet Power of Turbines Using Computational Fluid Dynamics. Energy Rep. 2021, 7, 4555–4565. [Google Scholar] [CrossRef]
- Abdelsalam, E.; Kafiah, F.; Almomani, F.; Tawalbeh, M.; Kiswani, S.; Khasawneh, A.; Ibrahim, D.; Alkasrawi, M. An Innovative Design of a Solar Double-Chimney Power Plant for Electricity Generation. Energies 2021, 14, 6235. [Google Scholar] [CrossRef]
- Gholamalizadeh, E.; Kim, M.H. Multi-Objective Optimization of a Solar Chimney Power Plant with Inclined Collector Roof Using Genetic Algorithm. Energies 2016, 9, 971. [Google Scholar] [CrossRef]
- Keshari, S.R.; Chandramohan, V.P.; Das, P. A 3D Numerical Study to Evaluate Optimum Collector Inclination Angle of Manzanares Solar Updraft Tower Power Plant. Sol. Energy 2021, 226, 455–467. [Google Scholar] [CrossRef]
- Kebabsa, H.; Said Lounici, M.; Daimallah, A. Numerical Investigation of a Novel Tower Solar Chimney Concept. Energy 2021, 214, 119048. [Google Scholar] [CrossRef]
- Ming, T.; Liu, W.; Xu, G. Analytical and Numerical Investigation of the Solar Chimney Power Plant Systems. Int. J. Energy Res. 2006, 30, 861–873. [Google Scholar] [CrossRef]
- Kasaeian, A.; Mahmoudi, A.R.; Astaraei, F.R.; Hejab, A. 3D Simulation of Solar Chimney Power Plant Considering Turbine Blades. Energy Convers. Manag. 2017, 147, 55–65. [Google Scholar] [CrossRef]
- Yapıcı, E.Ö.; Ayli, E.; Nsaif, O. Numerical Investigation on the Performance of a Small Scale Solar Chimney Power Plant for Different Geometrical Parameters. J. Clean Prod. 2020, 276, 122908. [Google Scholar] [CrossRef]
- Hu, S.; Leung, D.Y.C.; Chan, J.C.Y. Impact of the Geometry of Divergent Chimneys on the Power Output of a Solar Chimney Power Plant. Energy 2017, 120, 1–11. [Google Scholar] [CrossRef]
- Milani Shirvan, K.; Mirzakhanlari, S.; Mamourian, M.; Kalogirou, S.A. Optimization of Effective Parameters on Solar Updraft Tower to Achieve Potential Maximum Power Output: A Sensitivity Analysis and Numerical Simulation. Appl. Energy 2017, 195, 725–737. [Google Scholar] [CrossRef]
- Ghalamchi, M.; Kasaeian, A.; Ghalamchi, M.; Mirzahosseini, A.H. An Experimental Study on the Thermal Performance of a Solar Chimney with Different Dimensional Parameters. Renew. Energy 2016, 91, 477–483. [Google Scholar] [CrossRef]
- Xu, Y.; Zhou, X. Performance of Divergent-Chimney Solar Power Plants. Sol. Energy 2018, 170, 379–387. [Google Scholar] [CrossRef]
- Hu, S.; Leung, D.Y.C.; Chen, M.Z.Q.; Chan, J.C.Y. Effect of Guide Wall on the Potential of a Solar Chimney Power Plant. Renew. Energy 2016, 96, 209–219. [Google Scholar] [CrossRef]
- Hassan, A.; Ali, M.; Waqas, A. Numerical Investigation on Performance of Solar Chimney Power Plant by Varying Collector Slope and Chimney Diverging Angle. Energy 2018, 142, 411–425. [Google Scholar] [CrossRef]
- Sundararaj, M.; Rajamurugu, N.; Anbarasi, J.; Yaknesh, S.; Sathyamurthy, R. Parametric Optimization of Novel Solar Chimney Power Plant Using Response Surface Methodology. Results Eng. 2022, 16, 100633. [Google Scholar] [CrossRef]
- Ayadi, A.; Driss, Z.; Bouabidi, A.; Abid, M.S. Experimental and Numerical Study of the Impact of the Collector Roof Inclination on the Performance of a Solar Chimney Power Plant. Energy Build 2017, 139, 263–276. [Google Scholar] [CrossRef]
- Mandal, D.K.; Biswas, N.; Barman, A.; Chakraborty, R.; Manna, N.K. A Novel Design of Absorber Surface of Solar Chimney Power Plant (SCPP): Thermal Assessment, Exergy and Regression Analysis. Sustain. Energy Technol. Assess. 2023, 56, 103039. [Google Scholar] [CrossRef]
- Al-Kayiem, H.H.; Aja, O.C. Historic and Recent Progress in Solar Chimney Power Plant Enhancing Technologies. Renew. Sustain. Energy Rev. 2016, 58, 1269–1292. [Google Scholar] [CrossRef]
- Cao, F.; Li, H.; Ma, Q.; Zhao, L. Design and Simulation of a Geothermal-Solar Combined Chimney Power Plant. Energy Convers. Manag. 2014, 84, 186–195. [Google Scholar] [CrossRef]
- Mokrani, O.B.E.K.; Ouahrani, M.R.; Sellami, M.H.; Segni, L. Experimental Investigations of Hybrid: Geothermal Water/Solar Chimney Power Plant. Energy Sources Part A Recovery Util. Environ. Eff. 2020, 1–18. [Google Scholar] [CrossRef]
- Abdelsalam, E.; Kafiah, F.; Alkasrawi, M.; Al-Hinti, I.; Azzam, A. Economic Study of Solar Chimney Power-Water Distillation Plant (SCPWDP). Energies 2020, 13, 2789. [Google Scholar] [CrossRef]
- Abdelsalam, E.; Almomani, F.; Kafiah, F.; Almaitta, E.; Tawalbeh, M.; Khasawneh, A.; Habash, D.; Omar, A.; Alkasrawi, M. A New Sustainable and Novel Hybrid Solar Chimney Power Plant Design for Power Generation and Seawater Desalination. Sustainability 2021, 13, 12100. [Google Scholar] [CrossRef]
- Alkasrawi, M.; Abdelsalam, E.; Alnawafah, H.; Almomani, F.; Tawalbeh, M.; Mousa, A. Integration of Solar Chimney Power Plant with Photovoltaic for Co-Cooling, Power Production, and Water Desalination. Processes 2021, 9, 2155. [Google Scholar] [CrossRef]
- Maia, C.B.; Silva, F.V.M.; Oliveira, V.L.C.; Kazmerski, L.L. An Overview of the Use of Solar Chimneys for Desalination. Sol. Energy 2019, 183, 83–95. [Google Scholar] [CrossRef]
- Lizcano, J.C.O.; Haghighi, Z.; Wapperom, S.; Ferreira, C.I.; Isabella, O.; Dobbelsteen, v.d.A.; Zeman, M. Photovoltaic Chimney: Thermal Modeling and Concept Demonstration for Integration in Buildings. Prog. Photovolt. Res. Appl. 2020, 28, 465–482. [Google Scholar] [CrossRef]
- Abdelsalam, E.; Almomani, F.; Ibrahim, S.; Kafiah, F.; Jamjoum, M.; Alkasrawi, M. A Novel Design of a Hybrid Solar Double-Chimney Power Plant for Generating Electricity and Distilled Water. Sustainability 2023, 15, 2729. [Google Scholar] [CrossRef]
- Habibollahzade, A.; Houshfar, E.; Ashjaee, M.; Behzadi, A.; Gholamian, E.; Mehdizadeh, H. Enhanced Power Generation through Integrated Renewable Energy Plants: Solar Chimney and Waste-to-Energy. Energy Convers. Manag. 2018, 166, 48–63. [Google Scholar] [CrossRef]
- Akbarzadeh, A.; Johnson, P.; Singh, R. Examining Potential Benefits of Combining a Chimney with a Salinity Gradient Solar Pond for Production of Power in Salt Affected Areas. Sol. Energy 2009, 83, 1345–1359. [Google Scholar] [CrossRef]
- Aliaga, D.M.; Feick, R.; Brooks, W.K.; Mery, M.; Gers, R.; Levi, J.F.; Romero, C.P. Modified Solar Chimney Configuration with a Heat Exchanger: Experiment and CFD Simulation. Therm. Sci. Eng. Prog. 2021, 22, 100850. [Google Scholar] [CrossRef]
- Zou, Z.; He, S. Modeling and Characteristics Analysis of Hybrid Cooling-Tower-Solar-Chimney System. Energy Convers. Manag. 2015, 95, 59–68. [Google Scholar] [CrossRef]
- Zandian, A.; Ashjaee, M. The Thermal Efficiency Improvement of a Steam Rankine Cycle by Innovative Design of a Hybrid Cooling Tower and a Solar Chimney Concept. Renew. Energy 2013, 51, 465–473. [Google Scholar] [CrossRef]
- Habibollahzade, A.; Houshfar, E.; Ashjaee, M.; Ekradi, K. Continuous Power Generation through a Novel Solar/Geothermal Chimney System: Technical/Cost Analyses and Multi-Objective Particle Swarm Optimization. J. Clean Prod. 2021, 283, 465–473. [Google Scholar] [CrossRef]
- Schlaich, J.; Bergermann, R.; Schiel, W.; Weinrebe, G. Design of Commercial Solar Updraft Tower Systems-Utilization of Solar Induced Convective Flows for Power Generation. J. Sol. Energy Eng. Trans. ASME 2005, 127, 117–124. [Google Scholar] [CrossRef]
- Fadaei, N.; Kasaeian, A.; Akbarzadeh, A.; Hashemabadi, S.H. Experimental Investigation of Solar Chimney with Phase Change Material (PCM). Renew. Energy 2018, 123, 26–35. [Google Scholar] [CrossRef]
- Omara, A.A.M.; Mohammed, H.A.; Al Rikabi, I.J.; Abuelnour, M.A.; Abuelnuor, A.A.A. Performance Improvement of Solar Chimneys Using Phase Change Materials: A Review. Sol. Energy 2021, 228, 68–88. [Google Scholar] [CrossRef]
- Guo, P.; Wang, Y.; Meng, Q.; Li, J. Experimental Study on an Indoor Scale Solar Chimney Setup in an Artificial Environment Simulation Laboratory. Appl. Therm. Eng. 2016, 107, 818–826. [Google Scholar] [CrossRef]
- Kasaeian, A.; Sharifi, S.; Yan, W.M. Novel Achievements in the Development of Solar Ponds: A Review. Sol. Energy 2018, 174, 189–206. [Google Scholar] [CrossRef]
- Fathi, N.; McDaniel, P.; Aleyasin, S.S.; Robinson, M.; Vorobieff, P.; Rodriguez, S.; Oliveira, C. de Efficiency Enhancement of Solar Chimney Power Plant by Use of Waste Heat from Nuclear Power Plant. J. Clean Prod. 2018, 180, 407–416. [Google Scholar] [CrossRef]
- Djimli, S.; Chaker, A.; Ajib, S.; Habka, M. Studying the Possibility of a Combined Hybrid Solar Chimney Power Plant with a Gas Turbine. Environ. Prog. Sustain. Energy 2018, 37, 1160–1168. [Google Scholar] [CrossRef]
- Shahdost, B.M.; Astaraei, F.R.; Ebrahimi-Moghadam, A.; Ahmadi, M.H. Experimental and Numerical Investigations of a Novel Chimney System for Power Generation Using the Combination of Fossil Fuel Power Plant Exhaust Gases and Ambient Air. Energy Sci. Eng. 2019, 7, 764–776. [Google Scholar] [CrossRef]
- Hassan, H.Z. Performance Evaluation of a Continuous Operation Adsorption Chiller Powered by Solar Energy Using Silica Gel and Water as the Working Pair. Energies 2014, 7, 6382–6400. [Google Scholar] [CrossRef]
- Hassan, H.Z. Effect of Parameters Variation on the Performance of Adsorption Based Cooling Systems. Int. Rev. Mech. Eng. (IREME) 2013, 7, 24–37. [Google Scholar]
- Çengel, Y.A.; Ghajar, A.J. Heat and Mass Transfer: Fundamentals & Applications, 6th ed.; McGraw-Hill Education: New York, NY, USA, 2020; ISBN 0073398195. [Google Scholar]
- Swinbank, W.C. Long-Wave Radiation from Clear Skies. Q. J. R. Meteorol. Soc. 1963, 89, 339–348. [Google Scholar] [CrossRef]
- Saraf, G.R.; Hamad, F.A.W. Optimum Tilt Angle for a Flat Plate Solar Collector. Energy Convers. Manag. 1988, 28, 185–191. [Google Scholar] [CrossRef]
- Koonsrisuk, A.; Chitsomboon, T. Mathematical Modeling of Solar Chimney Power Plants. Energy 2013, 51, 314–322. [Google Scholar] [CrossRef]
- Kiwan, S.; Al-Nimr, M.; Salim, I. A Hybrid Solar Chimney/Photovoltaic Thermal System for Direct Electric Power Production and Water Distillation. Sustain. Energy Technol. Assess. 2020, 38, 100680. [Google Scholar] [CrossRef]
- POWER|Data Access Viewer. Available online: https://power.larc.nasa.gov/data-access-viewer/ (accessed on 27 October 2023).


| 0 | ||
| 1 | ||
| 2 | ||
| 3 |
| Time | Solar Radiation (W/m2) | Ambient Temperature (°C) | Wind Velocity (m/s) |
|---|---|---|---|
| 05:00 | 9.5 | 28.98 | 3.38 |
| 06:00 | 129.34 | 31.58 | 3.44 |
| 07:00 | 346.77 | 34.69 | 4.71 |
| 08:00 | 579.27 | 38.63 | 4.84 |
| 09:00 | 714.28 | 42.03 | 6.22 |
| 10:00 | 914.2 | 43.59 | 6.59 |
| 11:00 | 969.55 | 44.57 | 6.92 |
| 12:00 | 967.54 | 45.08 | 7.07 |
| 13:00 | 898.96 | 45.23 | 7.16 |
| 14:00 | 753.34 | 44.96 | 7.25 |
| 15:00 | 553.74 | 44.33 | 7.27 |
| 16:00 | 320.73 | 43.26 | 7.06 |
| 17:00 | 115.34 | 40.76 | 4.83 |
| 18:00 | 6.92 | 36.81 | 3.94 |
| 19:00 | 0 | 35.37 | 4.02 |
| 20:00 | 0 | 34.16 | 3.92 |
| 21:00 | 0 | 33 | 3.73 |
| 22:00 | 0 | 31.96 | 3.5 |
| 23:00 | 0 | 31.14 | 3.2 |
| 24:00 | 0 | 30.94 | 3.63 |
| 01:00 | 0 | 30.44 | 3.58 |
| 02:00 | 0 | 29.98 | 3.52 |
| 03:00 | 0 | 29.59 | 3.47 |
| 04:00 | 0 | 29.22 | 3.43 |
| 05:00 | 9.5 | 28.98 | 3.38 |
| 06:00 | 129.34 | 31.58 | 3.44 |
| Symbol | Parameter | Value |
|---|---|---|
| Solar collector | ||
| Collector radius at entrance | 1000 m | |
| Exponent for the solar collector roof profile | 0.65 | |
| Entrance height solar collector | 1.5 m | |
| Height of the chimney | 500 m | |
| Diameter of the chimney | 60 m | |
| Emissivity of the solar collector transparent cover | 0.85 | |
| Turbine efficiency | 0.85 | |
| Plate emissivity | 0.05 | |
| Adsorption bed | ||
| Silica gel specific heat | 921 J·kg−1·K−1 | |
| Silica gel thermal conductivity | 0.198 W·m−1·K−1 | |
| Particle density of silica gel | 700 kg·m−3 | |
| Porosity of the adsorption bed | 0.4 | |
| Adsorption bed thickness | 2.0 cm | |
| Evaporator temperature | 10 °C | |
| Refrigerant condensation temperature | 40 °C |
| Component | Modified System | Basic System |
|---|---|---|
| Thermal energy absorbed by the reactor | ||
| J | ||
| Sum | ||
| Thermal energy ejected by the reactor | ||
| Sum | ||
| Enthalpy of condensation | ||
| Cooling effect at the evaporator | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hassan, H.Z. Performance Enhancement of the Basic Solar Chimney Power Plant Integrated with an Adsorption Cooling System with Heat Recovery from the Condenser. Energies 2024, 17, 136. https://doi.org/10.3390/en17010136
Hassan HZ. Performance Enhancement of the Basic Solar Chimney Power Plant Integrated with an Adsorption Cooling System with Heat Recovery from the Condenser. Energies. 2024; 17(1):136. https://doi.org/10.3390/en17010136
Chicago/Turabian StyleHassan, Hassan Zohair. 2024. "Performance Enhancement of the Basic Solar Chimney Power Plant Integrated with an Adsorption Cooling System with Heat Recovery from the Condenser" Energies 17, no. 1: 136. https://doi.org/10.3390/en17010136
APA StyleHassan, H. Z. (2024). Performance Enhancement of the Basic Solar Chimney Power Plant Integrated with an Adsorption Cooling System with Heat Recovery from the Condenser. Energies, 17(1), 136. https://doi.org/10.3390/en17010136






