Energy, Exergy and Thermoeconomic Analyses on Hydrogen Production Systems Using High-Temperature Gas-Cooled and Water-Cooled Nuclear Reactors

Abstract

The use of nuclear energy is inevitable to reduce the dependence on fossil fuels in the energy sector. High-temperature gas-cooled reactors (HTGRs) are considered as a system suitable for the purpose of reducing the use of fossil fuels. Furthermore, eco-friendly mass production of hydrogen is crucial because hydrogen is emerging as a next-generation energy carrier. The unit cost of hydrogen production by the levelized cost of energy (LCOE) method varies widely depending on the energy source and system configuration. In this study, energy, exergy, and thermoeconomic analyses were performed on the hydrogen production system using the HTGR and high-temperature water-cooled nuclear reactor (HTWR) to calculate reasonable unit cost of the hydrogen produced using a thermoeconomic method called modified production structure analysis (MOPSA). A flowsheet analysis was performed to confirm the energy conservation in each component. The electricity generated from the 600 MW HTGR system was used to produce 1.28 kmol/s of hydrogen by electrolysis to split hot water vapor. Meanwhile, 515 MW of heat from the 600 MW HTWR was used to produce 8.10 kmol/s of hydrogen through steam reforming, and 83.6 MW of electricity produced by the steam turbine was used for grid power. The estimated unit cost of hydrogen from HTGR is approximately USD 35.6/GJ with an initial investment cost of USD 2.6 billion. If the unit cost of natural gas is USD 10/GJ, and the carbon tax is USD 0.08/kg of carbon dioxide, the unit cost of hydrogen produced from HTWR is approximately USD 13.92/GJ with initial investment of USD 2.32 billion. The unit cost of the hydrogen produced in the scaled-down plant was also considered.

1. Introduction

The accelerated use of fossil fuels for economic development not only leads to their depletion but also severely affects the environment. The use of nuclear energy [1,2,3] is inevitable to reduce the dependence on fossil fuels in the energy sector. Jaszczur et al. [1] examined sulfur–iodine (S-I) and copper–chlorine (Cu-Cl) cycles using high-temperature Onuclear reactors. A four-step Cu-Cl cycle was used to produce hydrogen using a supercritical water-cooled reactor [2]. Milewski et al. [3] investigate two types of high temperature electrolyzers for hydrogen production using nuclear power plants. The high-temperature gas-cooled reactor (HTGR), which is the basis of small modular reactors (SMR) that requires a special design for the reaction core [4], is evaluated as a suitable system for the purpose of reducing the use of fossil fuel [5,6]. The reactor’s inert gas cooling and heat-resistant structure enable it to reach core exit temperatures of up to 950 °C [5] compared to other predictable reactors.

The HTGR is well suited for numerous thermal process applications [1]. One of these is the mass production of hydrogen using electricity and heat obtained from the HTGR. The reason why eco-friendly mass production of hydrogen is crucial is because hydrogen is emerging as a next-generation energy carrier. Hydrogen can be used in various approaches, including stationary power generation using fuel cells and transportation power devices such as automobiles.

Based on the conceptual design of the hydrogen production system by steam reforming of methane gas [7,8,9] or water decomposition by high temperature electrolysis [9] with HTGR, exergetic and thermoeconomic analyses of hydrogen production systems have been reported. Kim et al. [10] used the steam reforming of methane gas to produce hydrogen using heat from high-temperature nuclear power plants cooled with helium gas (HTGR) or water (HTWR). In the HTGR, 217.6 MW of electricity from the Brayton cycle was connected to the grid system, and 83.7 MW of heat was used to produce 1.12 kmol of hydrogen. However, in the HTWR, 502 MW of heat produced 10.0 kg/s of hydrogen, and 83.6 MW of power from the steam turbine was connected to the grid system. The unit costs of hydrogen produced by the HTGR and HTWR were USD 0.81/kg and USD 0.73/kg, respectively. The initial investment costs used in their study were USD 1642 million (USD 2740/kW) for an HTGR plant and USD 1478 million (USD 2460/kW) for an HTWR plant, with a uranium fuel cost of 2.33 USD/GJ. Bolden [9] performed an exergy analysis on an HTGR system that used electricity from a Brayton cycle to decompose water to produce hydrogen. They calculated the amount of exergy and cost flows at each state point.

Water electrolysis using renewable energy sources (RESs) with low carbon footprint, such as solar [11,12,13] and wind [14], is considered to be a sustainable hydrogen production method. However, the unit cost of renewable-based hydrogen production is inevitably high because of substantial capital expenditure. In terms of low cost and carbon footprint, methane pyrolysis through carbon capture and storage has been reported to have one of the lowest levelized costs of hydrogen (LCOH) at USD 2.01/kg [15]. Recent studies using life cycle assessment methods have shown that high-temperature electrolysis is cleaner than the S-I cycle [16].

Recently, Lee et al. [17] reported that 300 MW of electricity and 68 MW of heat were input to produce hydrogen at a rate of 2.33 kg/s in a solid oxide electrolytic cell using an HTGR system: the calculated unit cost of hydrogen was approximately USD 5.0/kg, which is similar to the LCOH of USD4.95/kg obtained by Al-Qahtani et al. [15]. The initial investment cost of HTGR covered by Lee et al. [16] was approximately USD 1120 million. However, Wang et al. [18] and Ozbilen et al. [19] used various reactor systems to obtain a more economical LCOH. The unit cost of hydrogen production by the levelized cost of energy (LCOE) method varies widely depending on the energy source. For nuclear energy, the unit cost of hydrogen ranges from USD 2.18/kg to USD 5.46/kg [12].

Exergy analysis [20,21] enables estimation of the lost work rate in each component of a thermal system, while the thermoeconomic analysis provides a tool to properly estimate the unit cost of system’s products. Modified production structure analysis (MOPSA) [22] combines exergy and thermoeconomic analyzes to calculate the production cost of each component and the lost cost flow due to entropy production rate [22]. In MOPSA, the entropy generation rate was calculated from the second law of thermodynamics.

In this study the high-temperature water electrolysis method proposed by the Idaho Research Institute in the United States [5] and the steam reforming method of methane gas in a water-cooled reactor proposed by the Electric Power Research Institute [8] were used as models for hydrogen production. Assuming the states at the inlet and outlet of each device of the configured system, the state was confirmed through the first law of thermodynamics and exergy analysis. Finally, a thermoeconomics analysis using MOPSA was performed using the input and output conditions of each of these devices to obtain a more reasonable unit cost of hydrogen than that of the LCOE method. The unit cost of hydrogen produced by electrolysis with an HTGR is approximately USD 4.27/kg, whereas the unit cost of hydrogen produced by steam reforming with an HTWR is approximately USD 1.67/kg. Detailed exergetic and thermoeconomic analyses using MOPSA provide a reliable unit cost of hydrogen for the system depending on the initial investments and fuel cost.

This paper is organized as follows. A description of HTGR and HTWR systems is in Section 2 where steam decomposition and steam reforming reactions are also discussed.

Section 3 and Section 4 deal with the general exergy-balance equation and the exergy cost-balance equation, respectively, which constitute the basic equations of the MOPSA method. Therefore, the exergy balance equation for each component and the corresponding exergy cost balance equation are formulated in these sections. Section 5 presents calculation results and discussions, and Section 6 concludes.

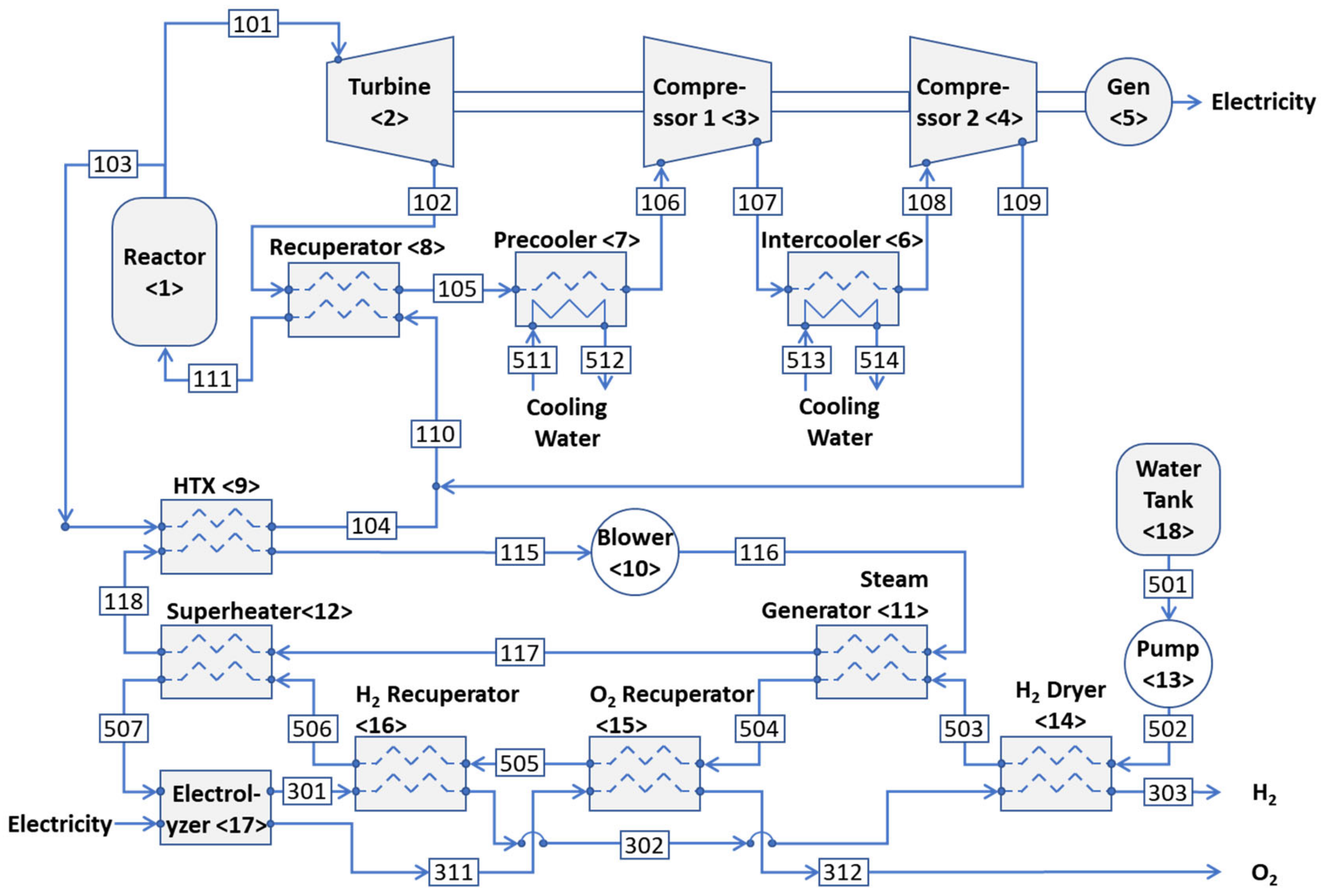
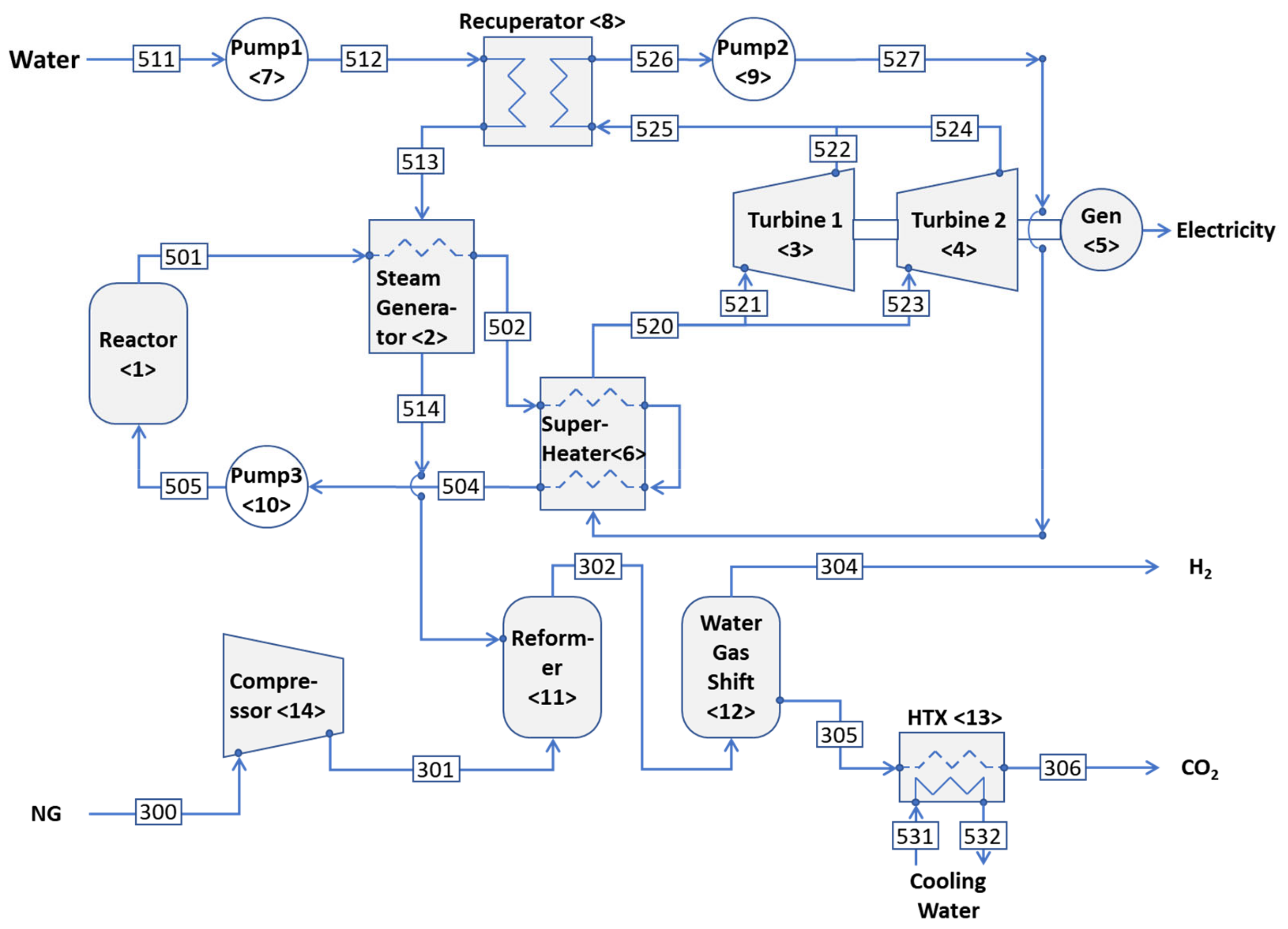
2. System Description

2.1. HTGR with the Brayton Cycle

In the case shown in Figure 1, the reactor is cooled with helium gas whose exit temperature from the reactor core is 850 °C, and its mass flow rate is 320 kg/s. A portion of the helium gas steam (278 kg/s) drives the turbine <2> and heats the helium gas, which returned to the recuperator <8>. The helium gas was cooled by a water flow in the pre-cooler <7> before entering the compressor <3>. The helium gas was cooled again by another water stream in the intercooler <6> before entering the compressor <4>. The pressure drop that occurs in the turbine is recovered by compressors <3> and <4>. The helium gas from compressor <4> mixes with the helium gas from heat exchanger <9> and returns to the reactor.

Figure 1. Schematic of a hydrogen production plant with the gas turbine (GT)-high-temperature gas-cooled reactor (HTGR) system. GEN: Generator, HTX: Heat exchanger.

Heat exchanger <9> provides heat for converting water to water vapor through a secondary loop of helium gas circulated by blower <10>. The water from the water tank is pressurized by the pump <13>, converted into steam in the steam generator <11>, and heated in the oxygen recuperator <15> and the hydrogen recuperator <16>. Subsequently, the water vapor heated again in the recuperator <12> enters the electrolytic cell <17>. In the electrolyzer <17>, water vapor is decomposed by electrical energy supplied from the generator <5>.

The chemical formula for steam electrolysis is:

$$H_2+O_2/2=H_{2}O \mathsf{\Delta }{H}_{293.2,H_{2}O}^o=241.8 kJ/mole,$$

(1)

For this process to occur, 241,826 kJ of energy, which is the lower heating value of water, is required. The amount of water vapor that can be electrolyzed is determined by the following law of energy conservation for the electrolyzer:

$${\dot{Q}}_{electrolyser}=\dot{H}(301)+\dot{H}(311)-\dot{H}(507)-{\dot{E}}_{x,<5>}^W+{\dot{n}}_{H_{2}O}(507)\cdot \Delta H_{293.2,H_{2}O}^o,$$

(2)

Considering a certain amount of heat loss in the electrolyzer, the value given in Equation (2) must be negative and the amount of water vapor decomposed in the electrolyzer can be determined using this equation.

2.2. HTWR with Steam Reforming

To convert the reactor energy into heat energy, it is recommended to cool the reactor with water. As shown in Figure 2, the HTWR system contains three water streams. The primary water steam from reactor <1> passes through steam generator <2> and exchanges heat with the secondary water stream heated in recuperator <8>. The secondary water stream from the pump 1 <7> passes through the steam generator and becomes hot steam before entering reformer <17>. Meanwhile the third water stream converted to steam in the superheater <6> by the first water stream operates turbines <3> and <4> and enters the recuperator <8> to transfer heat to the second water stream. Steam flows into reformer <11> and is mixed with methane gas introduced from compressor <14> to reform the methane gas. The CO₂ gas stream from the water–gas shift reactor <13> was cooled by a water stream. The reforming reaction of methane can be written as [23]:

$$C_n{H}_m+2n{H}_{2}O\to nC{O}_2+\left(2n+\frac{1}{2}m\right)H_2,\mathsf{\Delta }{H}_{298K}^o=162.0 kJ/mole$$

(3)

$$C_n{H}_m+n{H}_{2}O\to nCO+\left(n+\frac{1}{2}m\right)H_2,\mathsf{\Delta }{H}_{298K}^o=206.2 kJ/mole$$

(4)

Figure 2. Schematic of a hydrogen production plant with the ST-HTGR system.

The reactions in Equations (3) and (4) are endothermic: therefore, the heat required for the reforming process is supplied by the inflow of high-temperature steam. Since the natural gas entering the reformer is composed of CH₄, C₂H₆, C₃H₈, C₄H₁₀, etc., the reaction equation should be written considering these components. The overall reaction between hydrocarbons and steam in the reformer when the reaction in Equation (3) occurs f times and the reaction in Equation (4) occurs (l–f) times is presented below [24]. The smaller the value of f, the more heat the reforming reaction uses because the reaction given in Equation (3) occurs.

$$\begin{gathered} \dot{a}{C}_{{\alpha }_j}{H}_{{\beta }_j}+\dot{b}{C}_{{\alpha }_k}{H}_{{\beta }_k}+\dot{c}{C}_{{\alpha }_l}{H}_{{\beta }_l}+\dot{d}{C}_{{\alpha }_m}{H}_{{\beta }_m}+{\dot{n}}_w{H}_2 \\ f{\dot{n}}_{\alpha t}C{O}_2+\left(1-f\right){\dot{n}}_{\alpha t}CO+\left[\left(1+f\right){\dot{n}}_{\alpha t}+\frac{1}{2}{\dot{n}}_{\beta t}\right]H_2 \\ +\left[{\dot{n}}_w-(1+f){\dot{n}}_{\alpha t}\right]H_{2}O+(1-{\eta }_{CR})\dot{a}{C}_{{\alpha }_j}{H}_{{\beta }_j} \end{gathered}$$

(5)

In Equation (5), the molar flow rate of carbon and the hydrogen atomic weights of the hydrocarbons including methane are given as follows:

$$C:\dot{a}{\eta }_{CR}{\alpha }_j+\dot{b}{\alpha }_k+\dot{c}{\alpha }_l+\dot{d}{\alpha }_m={\dot{n}}_{\alpha t},$$

(6)

$$H:\dot{a}{\eta }_{CR}{\beta }_j+\dot{b}{\beta }_k+\dot{c}{\beta }_l+\dot{d}{\beta }_m={\dot{n}}_{\beta t}$$

(7)

In Equation (5), n_w is the number of moles of steam introduced into the reformer and η_CR is the reformer efficiency. As shown in Equation (5), the number of moles of water vapor entering the reformer must be greater than (1 + f) n_αt. The enthalpy of the combustion for the reforming reaction is given by:

$$\begin{gathered} {\overline{h}}_{RP,RF}=\sum _{product}{n}_e({\overline{h}}_f^0+\Delta \overline{h}{)}_o-\sum _{reactants}{n}_i({\overline{h}}_f^0+\Delta \overline{h}{)}_i \\ =LHV\_RFOM+\sum _{product}{n}_o(\Delta \overline{h}{)}_o-\sum _{reactants}{n}_i(\Delta \overline{h}{)}_i \end{gathered}$$

(8)

where LHV_RFOM in Equation (8) is given as follows:

$$\begin{gathered} LHV\_RFOM=f{n}_{\alpha t}({\overline{h}}_f^o{)}_{C{O}_2}+(1-f)n_{\alpha t}({\overline{h}}_f^o{)}_{CO}-(1+f)n_{\alpha t}({\overline{h}}_f^o{)}_{H_{2}O}-{\eta }_{CR}a({\overline{h}}_f^o{)}_{C_{\alpha j}{H}_{\beta j}} \\ -b({\overline{h}}_f^o{)}_{C_{\alpha k}{H}_{\beta k}}-c({\overline{h}}_f^o{)}_{C_{\alpha l}{H}_{\beta l}}-d({\overline{h}}_f^o{)}_{C_{\alpha m}{H}_{\beta m}} \end{gathered}$$

(9)

As the chemical reactions in the reformer are endothermic, LHV_RFOM has a positive value. The molar flow rate of hydrocarbons, including methane, into the reformer is determined by the law of energy conservation. Considering the heat loss in the reformer, the values in Equation (10) must be negative.

$${\dot{Q}}_{RFOM}=LHV\_RFOM+H(302)-H(301)-H(514)$$

(10)

Reformed gases, such as water vapor from the reformer, enter the water–gas shift reactor and react with the carbon monoxide (CO) generated in the reformer from CO₂ and H₂ gas. The chemical reaction is given as:

$$CO(g)+H_{2}O(g)\to C{O}_2(g)+H_2(g),\mathsf{\Delta }{H}_{298K}^o=-44.2 kJ/mole$$

(11)

Therefore, the chemical reaction equation in the water–gas shift reactor can be written as:

$$\begin{gathered} f{\dot{n}}_{\alpha t}C{O}_2+\left(1-f\right){\dot{n}}_{\alpha t}CO+\left[\left(1+f\right){\dot{n}}_{\alpha t}+\frac{1}{2}{\dot{n}}_{\beta t}\right]H_2+ \\ \left[{\dot{n}}_w-\left(1+f\right){\dot{n}}_{\alpha t}\right]H_{2}O+\left(1-{\eta }_{CR}\right)\dot{a}{C}_{{\alpha }_j}{H}_{{\beta }_j} \\ \to \dot{p}CO+\left({\dot{n}}_{\alpha t}-\dot{p}\right)C{O}_2+\left[2{\dot{n}}_{\alpha t}-\dot{p}+\frac{1}{2}{\dot{n}}_{\beta t}\right]H_2 \\ +\left[{\dot{n}}_w-2{\dot{n}}_{\alpha t}+\dot{p}\right]H_{2}O+(1-{\eta }_{CR})\dot{a}{C}_{{\alpha }_j}{H}_{{\beta }_j} \end{gathered}$$

(12)

where p is the molar flow rate of CO gas due to incomplete combustion. The enthalpy of combustion for the reaction given in Equation (12) is expressed as:

$$\begin{gathered} {{\overline{h}}_{RP,WGS}=\sum _{product}{n}_e({\overline{h}}_f^o+\Delta \overline{h})-\sum _{reactants}{n}_i({\overline{h}}_f^o+{\Delta \overline{h}}_i)}_i \\ =\mathrm{LHV}\_\mathrm{WGS}+\sum _{produts}{n}_o(\Delta \overline{h}{)}_o-\sum _{reactants}{n}_i(\Delta \overline{h}{)}_i \end{gathered}$$

(13)

where LHV_WGS is the amount of heat released in the WGS reaction.

$$LHV\_\mathit{WGS}=\left[p-(1-f)n_{\alpha t}\right]\left[{\left({\overline{h}}_f^o\right)}_{CO}-{\left({\overline{h}}_f^o\right)}_{C{O}_2}+{\left({\overline{h}}_f^o\right)}_{H_{2}O}\right]$$

(14)

The first law of thermodynamics in a water–gas shift reactor is given by:

$${\dot{Q}}_{WGS}=LHV\_WGS+H(304)-H(302)$$

(15)

Considering heat loss in the water–gas shift reactor, Equation (15) should be negative.

3. Exergy Analysis of HTGR/HTWR Systems

3.1. Mass and Energy Conservation, and the Exergy-Balance Equation

For each device, the following mass and energy conservation equations are satisfied.

Mass conservation:

$${\displaystyle \sum _{in}{\dot{m}}_i}={\displaystyle \sum _{out}{\dot{m}}_i}$$

(16)

Energy conservation:

$${\dot{Q}}_{cv}+{\displaystyle \sum _{in}{\dot{H}}_i}={\displaystyle \sum _{out}{\dot{H}}_i}+{\dot{W}}_{cv}$$

(17)

In Equation (17), ${\dot{H}}_i={\dot{m}}_i{h}_i$, where h is the enthalpy per mass, ${\dot{Q}}_i$ is the heat transfer rate, and ${\dot{W}}_i$ is the work rate.

From the first and second laws of thermodynamics, the following exergy balance equations for the flow of materials flowing in and out of each device were derived [20].

$${\dot{E}}_x^{CHE}+({\displaystyle \sum _{inlet}{\dot{E}}_x^T}-{\displaystyle \sum _{outlet}{\dot{E}}_x^T})+({\displaystyle \sum _{inlet}{\dot{E}}_x^P}-{\displaystyle \sum _{outlet}{\dot{E}}_x^P})+T_o({\displaystyle \sum _{inlet}{\dot{S}}_i}-{\displaystyle \sum _{outlet}{\dot{S}}_i}+{\dot{Q}}_{cv}/T_o)={\dot{E}}_x^W$$

(18)

where the superscripts CHE and W represent the chemical and work exergies, respectively, and the superscripts T and P represent the thermal exergy and mechanical exergy, respectively. The fourth term from the left is a negative value for the lost work rate owing to entropy generation. The lost work rate, ${\dot{E}}_x^D$, is given as [25]:

$${\dot{E}}_x^D=T_o{\dot{S}}_{gen}=T_o({\displaystyle \sum _{outlet}{\dot{S}}_i}-{\displaystyle \sum _{inlet}{\dot{S}}_i}-{\dot{Q}}_{cv}/T_o)$$

(19)

The exergy flow is expressed as:

$${\dot{E}}_x=\dot{m}{e}_x$$

(20)

The exergy per unit mass ($e_x$) can be expressed by dividing it into thermal exergy related to the temperature of the material flow and mechanical exergy related to the pressure as follows [26]:

$$e_x=e_x^T+e_x^P$$

(21)

The exergy for the material flow can be defined as follows based on the reference temperature (T_ref) and reference pressure (P_ref):

$$e_x=h(T,P)-h_{ref}(T_{ref},P_{ref})-T_o\left[s(T,P)-s_{ref}(T_{ref},P_{ref})\right]$$

(22)

In general, the exergy per unit mass given in Equation (22) can be described as follows based on the environmental temperature and pressure when the kinetic energy and potential energy of the material flow are ignored:

$$e_x=h(T,P)-h_o(T_o,P_o)-T_o\left[s(T,P)-s_o(T_o,P_o)\right]$$

(23)

The thermal exergy and mechanical exergies expressed in Equation (21) can be defined as:

$$e_x^T=\left[h(T,P)-h(T_{ref},P)\right]-T_o\left[s(T,P)-s(T_{ref},P)\right]$$

(24)

$$e_x^P=\left[h(T_{ref},P)-h_{ref}(T_{ref},P_{ref})\right]-T_o\left[s(T_{ref},P)-s_{ref}(T_{ref},P_{ref})\right]$$

(25)

The reference temperatures and reference pressures used in Equations (24) and (25) represent the ambient temperature and pressure, respectively.

3.2. Exergy-Balance Equations for the HTGR System

Applying the exergy balance expressed in Equation (18) for each device in the HTGR system yields the following exergy-balance equation: In E_x,_abc, which represents the flow of exergy entering and exiting each device, the subscript abc is expressed as a number and a indicates the type of material. Specifically, 1 represents the flow of helium gas, 3 represents oxygen or hydrogen gas, and 5 represents water flow. Among the numbers, bc represents the number of entrances and exits of each device.

1.

Reactor <1>:

$${\dot{Q}}^{UR}+\left({\dot{E}}_{x,111}^T-{\dot{E}}_{x,101}^T-{\dot{E}}_{x,103}^T\right)+\left({\dot{E}}_{x,111}^P-{\dot{E}}_{x,101}^P-{\dot{E}}_{x,103}^P\right)+T_0\left({\dot{S}}_{111}-{\dot{S}}_{101}-{\dot{S}}_{103}+\frac{{\dot{Q}}_{<1>}}{T_0}\right)=0$$

(26)

where the superscripts T and P denote the thermal exergy and mechanical exergy of the gas flow, respectively. In addition, ${\dot{Q}}^{UR}$ is the amount of heat produced in a nuclear reactor.

2.

Gas Turbine <2>:

$$\left({\dot{E}}_{x,101}^T-{\dot{E}}_{x,102}^T\right)+\left({\dot{E}}_{x,101}^P-{\dot{E}}_{x,102}^P\right)+T_0\left({\dot{S}}_{101}-{\dot{S}}_{102}+\frac{{\dot{Q}}_{<2>}}{T_0}\right)={\dot{E}}_{x,<2>}^W$$

(27)

3.

Compressor 1 <3>:

$$\left({\dot{E}}_{x,106}^T-{\dot{E}}_{x,107}^T\right)+\left({\dot{E}}_{x,106}^P-{\dot{E}}_{x,107}^P\right)+T_0\left({\dot{S}}_{106}-{\dot{S}}_{107}+\frac{{\dot{Q}}_{<3>}}{T_0}\right)={\dot{E}}_{x,<3>}^W$$

(28)

4.

Compressor 2 <4>:

$$\left({\dot{E}}_{x,108}^T-{\dot{E}}_{x,109}^T\right)+\left({\dot{E}}_{x,108}^P-{\dot{E}}_{x,109}^P\right)+T_0\left({\dot{S}}_{108}-{\dot{S}}_{109}+\frac{{\dot{Q}}_{<4>}}{T_0}\right)={\dot{E}}_{x,<4>}^W$$

(29)

5.

Generator <5>:

$$-\left({\dot{E}}_{x,<2>}^W+{\dot{E}}_{x,<3>}^W+{\dot{E}}_{x,<4>}^W\right)+{\dot{E}}_{x,<5>}^D+{\dot{E}}_{x,<5>}^W=0$$

(30)

In Equation (30), the first term is the work produced by the turbine plus the work supplied to compressors <1> and <2>, or the work entering the generator; the second term is the work lost in the generator; and the third term is the electricity produced by the generator.

6.

Intercooler <6>:

$$\begin{array}{l}\left({\dot{E}}_{x,107}^T-{\dot{E}}_{x,108}^T\right)+\left({\dot{E}}_{x,107}^P-{\dot{E}}_{x,108}^P\right)+\left({\dot{E}}_{x,513}^{DT}-{\dot{E}}_{x,514}^{DT}\right)+\left({\dot{E}}_{x,513}^{DP}-{\dot{E}}_{x,514}^{DP}\right)\\ +T_0\left({\dot{S}}_{107}-{\dot{S}}_{108}+{\dot{S}}_{513}-{\dot{S}}_{514}+\frac{{\dot{Q}}_{<6>}}{T_0}\right)={\dot{E}}_{x,<6>}^W\end{array}$$

(31)

7.

Precooler <7>:

$$\begin{array}{l}\left({\dot{E}}_{x,105}^T-{\dot{E}}_{x,106}^T\right)+\left({\dot{E}}_{x,105}^P-{\dot{E}}_{x,106}^P\right)+\left({\dot{E}}_{x,511}^{DT}-{\dot{E}}_{x,512}^{DT}\right)+\left({\dot{E}}_{x,511}^{DP}-{\dot{E}}_{x,512}^{DP}\right)+\\ T_0\left({\dot{S}}_{105}-{\dot{S}}_{106}+{\dot{S}}_{511}-{\dot{S}}_{512}+\frac{{\dot{Q}}_{<7>}}{T_0}\right)={\dot{E}}_{x,<7>}^W\end{array}$$

(32)

8.

Recuperator <8>:

$$\begin{array}{l}\left({\dot{E}}_{x,102}^T-{\dot{E}}_{x,105}^T\right)+\left({\dot{E}}_{x,110}^T-{\dot{E}}_{x,111}^T\right)+\left({\dot{E}}_{x,102}^P-{\dot{E}}_{x,105}^P\right)+\left({\dot{E}}_{x,110}^P-{\dot{E}}_{x,111}^P\right)+\\ T_0\left({\dot{S}}_{102}-{\dot{S}}_{105}+{\dot{S}}_{110}-{\dot{S}}_{111}+\frac{{\dot{Q}}_{<8>}}{T_0}\right)=0\end{array}$$

(33)

9.

Heat exchanger <9>:

$$\begin{array}{l}\left({\dot{E}}_{x,103}^T-{\dot{E}}_{x,104}^T\right)+\left({\dot{E}}_{x,118}^T-{\dot{E}}_{x,115}^T\right)+\left({\dot{E}}_{x,103}^P-{\dot{E}}_{x,104}^P\right)+\left({\dot{E}}_{x,118}^P-{\dot{E}}_{x,115}^P\right)+\\ T_0\left({\dot{S}}_{103}-{\dot{S}}_{104}+{\dot{S}}_{118}-{\dot{S}}_{115}+\frac{{\dot{Q}}_{<9>}}{T_0}\right)=0\end{array}$$

(34)

10.

Blower <10>:

$$\left({\dot{E}}_{x,115}^T-{\dot{E}}_{x,116}^T\right)+\left({\dot{E}}_{x,115}^P-{\dot{E}}_{x,116}^P\right)+T_0\left({\dot{S}}_{115}-{\dot{S}}_{116}+\frac{{\dot{Q}}_{<10>}}{T_0}\right)={\dot{E}}_{x,<10>}^W$$

(35)

11.

Steam generator <11>:

$$\begin{array}{l}\left({\dot{E}}_{x,116}^T-{\dot{E}}_{x,117}^T\right)+\left({\dot{E}}_{x,503}^{DT}-{\dot{E}}_{x,504}^{DT}\right)+\left({\dot{E}}_{x,116}^P-{\dot{E}}_{x,117}^P\right)+\left({\dot{E}}_{x,503}^{DP}-{\dot{E}}_{x,504}^{DP}\right)+\\ T_0\left({\dot{S}}_{116}-{\dot{S}}_{117}+{\dot{S}}_{503}-{\dot{S}}_{504}+\frac{{\dot{Q}}_{<11>}}{T_0}\right)=0\end{array}$$

(36)

In Equation (36), the superscripts DT and DP denote the thermal and mechanical exergies of the water or steam flow, respectively.

12.

Superheater <12>:

$$\begin{array}{l}\left({\dot{E}}_{x,117}^T-{\dot{E}}_{x,118}^T\right)+\left({\dot{E}}_{x,506}^{DT}-{\dot{E}}_{x,507}^{DT}\right)+\left({\dot{E}}_{x,117}^P-{\dot{E}}_{x,118}^P\right)+\left({\dot{E}}_{x,506}^{DP}-{\dot{E}}_{x,507}^{DP}\right)+\\ T_0\left({\dot{S}}_{117}-{\dot{S}}_{118}+{\dot{S}}_{506}-{\dot{S}}_{507}+\frac{{\dot{Q}}_{<12>}}{T_0}\right)=0\end{array}$$

(37)

13.

Pump <13>:

$$\left({\dot{E}}_{x,501}^{DT}-{\dot{E}}_{x,502}^{DT}\right)+\left({\dot{E}}_{x,501}^{DP}-{\dot{E}}_{x,502}^{DP}\right)+T_0\left({\dot{S}}_{501}-{\dot{S}}_{502}+\frac{{\dot{Q}}_{<13>}}{T_0}\right)={\dot{E}}_{x,<13>}^W$$

(38)

14.

Hydrogen dryer <14>:

$$\begin{array}{l}\left({\dot{E}}_{x,302}^T-{\dot{E}}_{x,303}^T\right)+\left({\dot{E}}_{x,502}^{DT}-{\dot{E}}_{x,503}^{DT}\right)+\left({\dot{E}}_{x,302}^P-{\dot{E}}_{x,303}^P\right)+\left({\dot{E}}_{x,502}^{DP}-{\dot{E}}_{x,503}^{DP}\right)+\\ T_0\left({\dot{S}}_{302}-{\dot{S}}_{303}+{\dot{S}}_{502}-{\dot{S}}_{503}+\frac{{\dot{Q}}_{<13>}}{T_0}\right)=0\end{array}$$

(39)

15.

Oxygen recuperator <15>:

$$\begin{array}{l}\left({\dot{E}}_{x,311}^T-{\dot{E}}_{x,312}^T\right)+\left({\dot{E}}_{x,504}^{DT}-{\dot{E}}_{x,505}^{DT}\right)+\left({\dot{E}}_{x,311}^P-{\dot{E}}_{x,312}^P\right)+\left({\dot{E}}_{x,504}^{DP}-{\dot{E}}_{x,505}^{DP}\right)+\\ T_0\left({\dot{S}}_{311}-{\dot{S}}_{312}+{\dot{S}}_{504}-{\dot{S}}_{505}+\frac{{\dot{Q}}_{<15>}}{T_0}\right)=0\end{array}$$

(40)

16.

Hydrogen recuperator <16>:

$$\begin{array}{l}\left({\dot{E}}_{x,301}^T-{\dot{E}}_{x,302}^T\right)+\left({\dot{E}}_{x,505}^{DT}-{\dot{E}}_{x,506}^{DT}\right)+\left({\dot{E}}_{x,301}^P-{\dot{E}}_{x,302}^P\right)+\left({\dot{E}}_{x,505}^{DP}-{\dot{E}}_{x,506}^{DP}\right)+\\ T_0\left({\dot{S}}_{301}-{\dot{S}}_{302}+{\dot{S}}_{505}-{\dot{S}}_{506}+\frac{{\dot{Q}}_{<16>}}{T_0}\right)=0\end{array}$$

(41)

17.

Electrolyzer <17>:

$$\begin{array}{l}-{\dot{E}}_{x,<5>}^W+\left({\dot{E}}_{x,<10>}^W+{\dot{E}}_{x,<13>}^W\right)+\left({\dot{E}}_{x,507}^{DT}-{\dot{E}}_{x,301}^T-{\dot{E}}_{x,311}^T\right)+\left({\dot{E}}_{x,507}^{DP}-{\dot{E}}_{x,301}^P-{\dot{E}}_{x,311}^P\right)\\ +T_0\left({\dot{S}}_{507}-{\dot{S}}_{301}-{\dot{S}}_{311}+\frac{{\dot{Q}}_{<17>}}{T_0}\right)=0\end{array}$$

(42)

3.3. Exergy-Balance Equations for the HTWR System

In the case of an HTGR, if the exergy-balance equation given in Equation (18) is used to establish the exergy-balance equation for each component of the HTWR, it is as follows:

1.

Reactor <1>:

$${\dot{Q}}^{UR}+\left({\dot{E}}_{x,505}^{DT}-{\dot{E}}_{x,501}^{DT}\right)+\left({\dot{E}}_{x,505}^{DP}-{\dot{E}}_{x,501}^{DP}\right)+T_0\left({\dot{S}}_{505}-{\dot{S}}_{501}+\frac{{\dot{Q}}_{<1>}}{T_0}\right)=0$$

(43)

2.

Steam generator <2>:

$$\begin{array}{l}\left({\dot{E}}_{x,501}^{DT}-{\dot{E}}_{x,502}^{DT}\right)+\left({\dot{E}}_{x,513}^{DT}-{\dot{E}}_{x,514}^{DT}\right)+\left({\dot{E}}_{x,501}^{DP}-{\dot{E}}_{x,502}^{DP}\right)+\left({\dot{E}}_{x,513}^{DP}-{\dot{E}}_{x,514}^{DP}\right)+\\ T_0\left({\dot{S}}_{501}-{\dot{S}}_{502}+{\dot{S}}_{513}-{\dot{S}}_{514}+\frac{{\dot{Q}}_{<2>}}{T_0}\right)=0\end{array}$$

(44)

3.

Turbine 1<3>:

$$\left({\dot{E}}_{x,521}^{DT}-{\dot{E}}_{x,522}^{DT}\right)+\left({\dot{E}}_{x,521}^{DP}-{\dot{E}}_{x,522}^{DP}\right)+T_0\left({\dot{S}}_{521}-{\dot{S}}_{522}+\frac{{\dot{Q}}_{<3>}}{T_0}\right)={\dot{E}}_{x,<3>}^W$$

(45)

4.

Turbine 2 <4>:

$$\left({\dot{E}}_{x,523}^{DT}-{\dot{E}}_{x,524}^{DT}\right)+\left({\dot{E}}_{x,523}^{DP}-{\dot{E}}_{x,524}^{DP}\right)+T_0\left({\dot{S}}_{523}-{\dot{S}}_{524}+\frac{{\dot{Q}}_{<4>}}{T_0}\right)={\dot{E}}_{x,<4>}^W$$

(46)

Notably, turbine 2 receives relatively less steam than that of turbine 1.

5.

Generator <5>:

$$-\left({\dot{E}}_{x,<3>}^W+{\dot{E}}_{x,<4>}^W\right)+{\dot{E}}_{x,<5>}^D+{\dot{E}}_{x,<5>}^W=0$$

(47)

In Equation (47), the first term is the work produced by the turbines <3> and <4>, the second term is the work lost by the generator, and the third term is the electricity produced by the generator.

6.

Superheater <6>:

$$\begin{array}{l}\left({\dot{E}}_{x,502}^{DT}-{\dot{E}}_{x,504}^{DT}\right)+\left({\dot{E}}_{x,527}^{DT}-{\dot{E}}_{x,520}^{DT}\right)+\left({\dot{E}}_{x,502}^{DP}-{\dot{E}}_{x,504}^{DP}\right)+\left({\dot{E}}_{x,527}^{DP}-{\dot{E}}_{x,520}^{DP}\right)+\\ T_0\left({\dot{S}}_{502}-{\dot{S}}_{504}+{\dot{S}}_{527}-{\dot{S}}_{520}+\frac{{\dot{Q}}_{<6>}}{T_0}\right)=0\end{array}$$

(48)

7.

Pump 1 <7>:

$$\left({\dot{E}}_{x,511}^{DT}-{\dot{E}}_{x,512}^{DT}\right)+\left({\dot{E}}_{x,511}^{DP}-{\dot{E}}_{x,512}^{DP}\right)+T_0\left({\dot{S}}_{511}-{\dot{S}}_{512}+\frac{{\dot{Q}}_{<7>}}{T_0}\right)=E_{x,<7>}^W$$

(49)

8.

Recuperator <8>:

$$\begin{array}{l}\left({\dot{E}}_{x,512}^{DT}-{\dot{E}}_{x,513}^{DT}\right)+\left({\dot{E}}_{x,525}^{DT}-{\dot{E}}_{x,526}^{DT}\right)+\left({\dot{E}}_{x,512}^{DP}-{\dot{E}}_{x,513}^{DP}\right)+\left({\dot{E}}_{x,525}^{DP}-{\dot{E}}_{x,526}^{DP}\right)+\\ T_0\left({\dot{S}}_{512}-{\dot{S}}_{513}+{\dot{S}}_{525}-{\dot{S}}_{526}+\frac{{\dot{Q}}_{<8>}}{T_0}\right)=0\end{array}$$

(50)

9.

Pump 2 <9>:

$$\left({\dot{E}}_{x,526}^{DT}-{\dot{E}}_{x,527}^{DT}\right)+\left({\dot{E}}_{x,526}^{DP}-{\dot{E}}_{x,527}^{DP}\right)+T_0\left({\dot{S}}_{526}-{\dot{S}}_{527}+\frac{{\dot{Q}}_{<9>}}{T_0}\right)=E_{x,<9>}^W$$

(51)

10.

Pump 3 <10>:

$$\left({\dot{E}}_{x,504}^{DT}-{\dot{E}}_{x,505}^{DT}\right)+\left({\dot{E}}_{x,504}^{DP}-{\dot{E}}_{x,505}^{DP}\right)+T_0\left({\dot{S}}_{504}-{\dot{S}}_{505}+\frac{{\dot{Q}}_{<10>}}{T_0}\right)=E_{x,<10>}^W$$

(52)

11.

Reformer <11>:

$$\begin{array}{l}-{\dot{E}}_{x,<5>}^W+\left({\dot{E}}_{x,<7>}^W+{\dot{E}}_{x,<9>}^W+{\dot{E}}_{x,<10>}^W+{\dot{E}}_{x,<14>}^W\right)+\left({\dot{E}}_{x,301}^T+{\dot{E}}_{x,514}^{DT}-{\dot{E}}_{x,302}^T\right)+\\ \left({\dot{E}}_{x,301}^P+{\dot{E}}_{x,514}^{DP}-{\dot{E}}_{x,302}^P\right)+T_0\left({\dot{S}}_{301}+{\dot{S}}_{514}-{\dot{S}}_{302}+\frac{{\dot{Q}}_{<11>}}{T_0}\right)=0\end{array}$$

(53)

12.

Water–gas shift reactor <12>:

$$\left({\dot{E}}_{x,302}^T-{\dot{E}}_{x,304}^T-{\dot{E}}_{x,305}^T\right)+\left({\dot{E}}_{x,302}^P-{\dot{E}}_{x,304}^P-{\dot{E}}_{x,305}^P\right)+T_0\left({\dot{S}}_{302}-{\dot{S}}_{304}-{\dot{S}}_{305}+\frac{{\dot{Q}}_{<12>}}{T_0}\right)=0$$

(54)

13.

Heat exchanger <13>:

$$\begin{array}{l}\left({\dot{E}}_{x,305}^T-{\dot{E}}_{x,306}^T\right)+\left({\dot{E}}_{x,305}^P-{\dot{E}}_{x,306}^P\right)+\left({\dot{E}}_{x,531}^{DT}-{\dot{E}}_{x,532}^{DT}\right)+\left({\dot{E}}_{x,531}^{DP}-{\dot{E}}_{x,532}^{DP}\right)+\\ T_0\left({\dot{S}}_{305}-{\dot{S}}_{306}+{\dot{S}}_{531}-{\dot{S}}_{532}+\frac{{\dot{Q}}_{<13>}}{T_0}\right)={\dot{E}}_{x,<13>}^W\end{array}$$

(55)

14.

Compressor <14>:

$$\left({\dot{E}}_{x,300}^T-{\dot{E}}_{x,301}^T\right)+\left({\dot{E}}_{x,300}^P-{\dot{E}}_{x,301}^P\right)+T_0\left({\dot{S}}_{300}-{\dot{S}}_{301}+\frac{{\dot{Q}}_{<14>}}{T_0}\right)=E_{x,<14>}^W$$

(56)

The electricity required for the pumps and compressors is produced by the generator. The pressure of the gas exiting the compressor must be equal to the pressure of the steam entering the reformer.

4. Thermoeconomic Analysis of HTGR/HTWR Systems

4.1. Exergy Cost-Balance Equations

Modified Productive Structure Analysis (MOPSA) [22], a thermoeconomics method assigns different unit costs according to the exergy types to the exergy-balance equation given in Equation (18) to obtain the exergy cost-balance equation. To rephrase, if C_O is assigned to the unit cost of fuel, C_T to the unit cost of thermal exergy, C_P to the unit cost of mechanical exergy, C_S to the unit cost of lost work, and C_W to the unit cost of work or electricity, the following general exergy cost-balance equation can be obtained from Equation (18):

$$\begin{array}{l}{\dot{E}}_x^{CHE}{C}_0+({\displaystyle \sum _{inlet}{\dot{E}}_{x,i}^T}-{\displaystyle \sum _{outlet}{\dot{E}}_{x,i}^T})C_T+({\displaystyle \sum _{inlet}{\dot{E}}_{x,i}^P}-{\displaystyle \sum _{outlet}{\dot{E}}_{x,i}^P})C_P+T_0({\displaystyle \sum _{inlet}{\dot{S}}_i}-{\displaystyle \sum _{outlet}{\dot{S}}_j+{\dot{Q}}_{cv}}/T_o)C_s\\ +{\dot{Z}}_k={\dot{E}}_x^W{C}_W,\end{array}$$

(57)

where ${\dot{Z}}_i$ is the flow of capital cost per unit time including the initial purchase cost and operating cost of the i-th device and can be obtained using the following equation [27]

$${\dot{Z}}_i=\frac{{\varphi }_i\cdot {\dot{C}}_i}{{\delta }_i}$$

(58)

where ${\varphi }_i$ and ${\delta }_i$ are the maintenance factor and the annual operating hours, respectively. In contrast, ${\dot{C}}_i$ is the initial purchase cost of the equipment converted into an annual equivalent cost and is given as follows:

$${\dot{C}}_i=\left[C_i-SV\cdot PWF(i,n)\right]\cdot CRF(i,n)$$

(59)

where C_i is the initial purchase cost of the equipment, SV is the depreciation cost after n years of equipment life, and PWF and CRF are the present worth factor and capital recovery factors, respectively.

The equations obtained by applying the exergy cost-balance equation given in Equation (57) for each device in the HTGR system shown in Figure 1 are as follows: A new unit cost may be assigned to the unit cost of a product that represents the characteristics of the device. For example, gas turbine <1> is a device that produces power; therefore, a new unit cost of work is given to the power term C_2W and is expressed in Gothic font. The number 2 in this expression represents the number of the device.

4.2. Exergy Cost-Balance Equations for the HTGR System

1.

Reactor <1>:

$$\begin{array}{l}{\dot{Q}}^{UR}{C}_{UR}+\left({\dot{E}}_{x,111}^T-{\dot{E}}_{x,101}^T-{\dot{E}}_{x,103}^T\right)C_{1T}+\left({\dot{E}}_{x,111}^P-{\dot{E}}_{x,101}^P-{\dot{E}}_{x,103}^P\right)C_P\\ +T_0\left({\dot{S}}_{111}-{\dot{S}}_{101}-{\dot{S}}_{103}+\frac{{\dot{Q}}_{<1>}}{T_0}\right)C_S+{\dot{Z}}_{<1>}=0\end{array}$$

(60)

Superscript UR of the first term in Equation (60) is the unit cost of uranium.

2.

Gas Turbine <2>:

$$\left({\dot{E}}_{x,101}^T-{\dot{E}}_{x,102}^T\right)C_T+\left({\dot{E}}_{x,101}^P-{\dot{E}}_{x,102}^P\right)C_P+T_0\left({\dot{S}}_{101}-{\dot{S}}_{102}+\frac{{\dot{Q}}_{<2>}}{T_0}\right)C_S+{\dot{Z}}_{<2>}={\dot{E}}_{x,<2>}^W{C}_{2W}$$

(61)

3.

Compressor 1 <3>:

$$\left({\dot{E}}_{x,106}^T-{\dot{E}}_{x,107}^T\right)C_T+\left({\dot{E}}_{x,106}^P-{\dot{E}}_{x,107}^P\right)C_{3P}+T_0\left({\dot{S}}_{106}-{\dot{S}}_{107}+\frac{{\dot{Q}}_{<3>}}{T_0}\right)C_S+{\dot{Z}}_{<3>}={\dot{E}}_{x,<3>}^W{C}_W$$

(62)

4.

Compressor 2 <4>:

$$\left({\dot{E}}_{x,108}^T-{\dot{E}}_{x,109}^T\right)C_T+\left({\dot{E}}_{x,108}^P-{\dot{E}}_{x,109}^P\right)C_{4P}+T_0\left({\dot{S}}_{108}-{\dot{S}}_{109}+\frac{{\dot{Q}}_{<4>}}{T_0}\right)C_S+{\dot{Z}}_{<4>}={\dot{E}}_{x,<4>}^W{C}_W$$

(63)

5.

Generator <5>:

$${\dot{E}}_{x,<2>}^W{C}_{2W}+\left({\dot{E}}_{x,<3>}^W+{\dot{E}}_{x,<4>}^W\right)C_W-{\dot{E}}_{x,<5>}^D{C}_S+{\dot{Z}}_{<5>}={\dot{E}}_{x,<5>}^W{C}_{5E}$$

(64)

6.

Intercooler <6>:

$$\begin{array}{l}\left({\dot{E}}_{x,107}^T-{\dot{E}}_{x,108}^T\right)C_{6T}+\left({\dot{E}}_{x,107}^P-{\dot{E}}_{x,108}^P\right)C_P+\left({\dot{E}}_{x,513}^{DT}-{\dot{E}}_{x,514}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,513}^{DP}-{\dot{E}}_{x,514}^{DP}\right)C_{DP}\\ +T_0\left({\dot{S}}_{107}-{\dot{S}}_{108}+{\dot{S}}_{513}-{\dot{S}}_{514}+\frac{{\dot{Q}}_{<6>}}{T_0}\right)C_S+{\dot{Z}}_{<6>}=0\end{array}$$

(65)

7.

Precooler <7>:

$$\begin{array}{l}\left({\dot{E}}_{x,105}^T-{\dot{E}}_{x,106}^T\right)C_{7T}+\left({\dot{E}}_{x,105}^P-{\dot{E}}_{x,106}^P\right)C_P+\left({\dot{E}}_{x,511}^{DT}-{\dot{E}}_{x,512}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,511}^{DP}-{\dot{E}}_{x,512}^{DP}\right)C_{DP}\\ +T_0\left({\dot{S}}_{105}-{\dot{S}}_{106}+{\dot{S}}_{511}-{\dot{S}}_{512}+\frac{{\dot{Q}}_{<7>}}{T_0}\right)C_S+{\dot{Z}}_{<7>}=0\end{array}$$

(66)

8.

Recuperator <8>:

$$\begin{array}{l}\left[\left({\dot{E}}_{x,102}^T-{\dot{E}}_{x,105}^T\right)+\left({\dot{E}}_{x,110}^T-{\dot{E}}_{x,111}^T\right)\right]C_{8T}+\left[\left({\dot{E}}_{x,102}^P-{\dot{E}}_{x,105}^P\right)+\left({\dot{E}}_{x,110}^P-{\dot{E}}_{x,111}^P\right)\right]C_P+\\ T_0\left({\dot{S}}_{101}-{\dot{S}}_{105}+{\dot{S}}_{110}-{\dot{S}}_{111}+\frac{{\dot{Q}}_{<8>}}{T_0}\right)C_S+{\dot{Z}}_{<8>}=0\end{array}$$

(67)

9.

Heat exchanger <9>:

$$\begin{array}{l}\left[\left({\dot{E}}_{x,103}^T-{\dot{E}}_{x,104}^T\right)+\left({\dot{E}}_{x,118}^T-{\dot{E}}_{x,115}^T\right)\right]C_{9T}+\left[\left({\dot{E}}_{x,103}^P-{\dot{E}}_{x,104}^P\right)+\left({\dot{E}}_{x,118}^P-{\dot{E}}_{x,115}^P\right)\right]C_P+\\ T_0\left({\dot{S}}_{103}-{\dot{S}}_{104}+{\dot{S}}_{118}-{\dot{S}}_{115}+\frac{{\dot{Q}}_{<9>}}{T_0}\right)C_S+{\dot{Z}}_{<9>}=0\end{array}$$

(68)

10.

Blower <10>:

$$\left({\dot{E}}_{x,115}^T-{\dot{E}}_{x,116}^T\right)C_T+\left({\dot{E}}_{x,115}^P-{\dot{E}}_{x,116}^P\right)C_{10P}+T_0\left({\dot{S}}_{115}-{\dot{S}}_{116}+\frac{{\dot{Q}}_{<10>}}{T_0}\right)C_S+{\dot{Z}}_{<10>}={\dot{E}}_{x,<10>}^W{C}_W$$

(69)

11.

Steam generator <11>:

$$\begin{array}{l}\left({\dot{E}}_{x,116}^T-{\dot{E}}_{x,117}^T\right)C_T+\left({\dot{E}}_{x,503}^{DT}-{\dot{E}}_{x,504}^{DT}\right)C_{11DT}+\left({\dot{E}}_{x,116}^P-{\dot{E}}_{x,117}^P\right)C_P+\left({\dot{E}}_{x,503}^{DP}-{\dot{E}}_{x,504}^{DP}\right)C_{DP}+\\ T_0\left({\dot{S}}_{116}-{\dot{S}}_{117}+{\dot{S}}_{503}-{\dot{S}}_{504}+\frac{{\dot{Q}}_{<11>}}{T_0}\right)C_S+{\dot{Z}}_{<11>}=0\end{array}$$

(70)

12.

Superheater <12>:

$$\begin{array}{l}\left({\dot{E}}_{x,117}^T-{\dot{E}}_{x,118}^T\right)C_T+\left({\dot{E}}_{x,506}^{DT}-{\dot{E}}_{x,507}^{DT}\right)C_{12DT}+\left({\dot{E}}_{x,117}^P-{\dot{E}}_{x,118}^P\right)C_P+\left({\dot{E}}_{x,506}^{DP}-{\dot{E}}_{x,507}^{DP}\right)C_{DP}+\\ T_0\left({\dot{S}}_{117}-{\dot{S}}_{118}+{\dot{S}}_{506}-{\dot{S}}_{507}+\frac{{\dot{Q}}_{<12>}}{T_0}\right)C_S+{\dot{Z}}_{<12>}=0\end{array}$$

(71)

13.

Pump <13>:

$$\left({\dot{E}}_{x,501}^{DT}-{\dot{E}}_{x,502}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,501}^{DP}-{\dot{E}}_{x,502}^{DP}\right)C_{13DP}+T_0\left({\dot{S}}_{501}-{\dot{S}}_{502}+\frac{{\dot{Q}}_{<13>}}{T_0}\right)C_S+{\dot{Z}}_{<13>}={\dot{E}}_{x,<13>}^W$$

(72)

14.

Hydrogen dryer <14>:

$$\begin{array}{l}\left({\dot{E}}_{x,302}^T-{\dot{E}}_{x,303}^T\right)C_T+\left({\dot{E}}_{x,502}^{DT}-{\dot{E}}_{x,503}^{DT}\right)C_{14DT}+\left({\dot{E}}_{x,302}^P-{\dot{E}}_{x,303}^P\right)C_P+\left({\dot{E}}_{x,502}^{DP}-{\dot{E}}_{x,503}^{DP}\right)C_{DP}+\\ T_0\left({\dot{S}}_{302}-{\dot{S}}_{303}+{\dot{S}}_{502}-{\dot{S}}_{503}+\frac{{\dot{Q}}_{<13>}}{T_0}\right)C_S+{\dot{Z}}_{<14>}=0\end{array}$$

(73)

15.

Oxygen recuperator <15>:

$$\begin{array}{l}\left({\dot{E}}_{x,311}^T-{\dot{E}}_{x,312}^T\right)C_T+\left({\dot{E}}_{x,504}^{DT}-{\dot{E}}_{x,505}^{DT}\right)C_{15DT}+\left({\dot{E}}_{x,311}^P-{\dot{E}}_{x,312}^P\right)C_P+\left({\dot{E}}_{x,504}^{DP}-{\dot{E}}_{x,505}^{DP}\right)C_{DP}+\\ T_0\left({\dot{S}}_{311}-{\dot{S}}_{312}+{\dot{S}}_{504}-{\dot{S}}_{505}+\frac{{\dot{Q}}_{<15>}}{T_0}\right)C_S+{\dot{Z}}_{<15>}=0\end{array}$$

(74)

16.

Hydrogen recuperator <16>:

$$\begin{array}{l}\left({\dot{E}}_{x,301}^T-{\dot{E}}_{x,302}^T\right)C_T+\left({\dot{E}}_{x,505}^{DT}-{\dot{E}}_{x,506}^{DT}\right)C_{16DT}+\left({\dot{E}}_{x,301}^P-{\dot{E}}_{x,302}^P\right)C_P+\left({\dot{E}}_{x,505}^{DP}-{\dot{E}}_{x,506}^{DP}\right)C_{DP}+\\ T_0\left({\dot{S}}_{301}-{\dot{S}}_{302}+{\dot{S}}_{505}-{\dot{S}}_{506}+\frac{{\dot{Q}}_{<16>}}{T_0}\right)C_S+{\dot{Z}}_{<16>}=0\end{array}$$

(75)

17.

Electrolyzer <17>:

$$\begin{array}{l}-{\dot{E}}_{x,<5>}^W{C}_{5E}+\left({\dot{E}}_{x,<10>}^W+{\dot{E}}_{x,<13>}^W\right)C_W+{\dot{E}}_{x,507}^{DT}{C}_{DT}-\left({\dot{E}}_{x,301}^T+{\dot{E}}_{x,311}^T\right)C_T+{\dot{E}}_{x,507}^{DP}{C}_{DP}\\ -\left({\dot{E}}_{x,301}^P+{\dot{E}}_{x,311}^P\right)C_P+T_0\left({\dot{S}}_{507}-{\dot{S}}_{301}-{\dot{S}}_{311}+\frac{{\dot{Q}}_{<17>}}{T_0}\right)C_S-{\dot{n}}_{H2}\cdot En{g}_{H2}\cdot C_{17H2}+{\dot{Z}}_{<17>}=0\end{array}$$

(76)

In Equation (76), ${\dot{n}}_{H2}$ and $En{g}_{H2}$ are the molar flow rate of hydrogen and the energy per kmol of hydrogen, respectively.

As shown above, 17 cost-balance equations were obtained from the 17 components. However, the unknowns shown in the cost-balance equation described above are C_1T, C_2W, C_3P, C_4P, C_5E, C_6T, C_7T, C_8T, C_9T, C_10P, C_11DT, C_12DT, C_13DP, C_14DT, C_15DT, C_16DT, C_17H2, C_T, C_P, C_DT, C_DP, C_W, and C_S. As there were twenty-three unknowns, six additional auxiliary equations were required to determine them. Six additional equations can be obtained for the thermal and mechanical exergy junctions for helium flow, thermal and mechanical exergy junctions for water flow, work exergy junction, and boundary condition, which are as follows:

Thermal exergy junction for helium flow:

$$\begin{array}{l}\left[\left({\dot{E}}_{x,110}^T-{\dot{E}}_{x,101}^T-{\dot{E}}_{x,103}^T\right)+\left({\dot{E}}_{x,107}^T-{\dot{E}}_{x,108}^T\right)+\left({\dot{E}}_{x,102}^T-{\dot{E}}_{x,106}^T\right)+\left({\dot{E}}_{x,103}^T-{\dot{E}}_{x,104}^T\right)+\left({\dot{E}}_{x,118}^T-{\dot{E}}_{x,115}^T\right)\right]C_T\\ =\left({\dot{E}}_{x,111}^T-{\dot{E}}_{x,101}^T-{\dot{E}}_{x,103}^T\right)C_{1T}+\left({\dot{E}}_{x,107}^T-{\dot{E}}_{x,108}^T\right)C_{6T}+\left({\dot{E}}_{x,105}^T-{\dot{E}}_{x,106}^T\right)C_{7T}\\ \left[\left({\dot{E}}_{x,110}^T-{\dot{E}}_{x,111}^T\right)+\left({\dot{E}}_{x,102}^T-{\dot{E}}_{x,105}^T\right)\right]{\mathrm{C}}_{8\mathrm{T}}+\left[\left({\dot{E}}_{x,103}^T-{\dot{E}}_{x,104}^T\right)+\left({\dot{E}}_{x,118}^T-{\dot{E}}_{x,115}^T\right)\right]{\mathrm{C}}_{9\mathrm{T}}\end{array}$$

(77)

Mechanical exergy junction for helium flow:

$$\begin{array}{l}\left[\left({\dot{E}}_{x,106}^P-{\dot{E}}_{x,107}^P\right)+\left({\dot{E}}_{x,108}^P-{\dot{E}}_{x,109}^P\right)+\left({\dot{E}}_{x,115}^P-{\dot{E}}_{x,116}^P\right)\right]C_P=\left({\dot{E}}_{x,106}^P-{\dot{E}}_{x,107}^P\right){\mathrm{C}}_{3\mathrm{P}}\\ +\left({\dot{E}}_{x,108}^P-{\dot{E}}_{x,109}^P\right){\mathrm{C}}_{4\mathrm{P}}+\left({\dot{E}}_{x,115}^P-{\dot{E}}_{x,116}^P\right)C_{10P}\end{array}$$

(78)

Thermal exergy junction for water flow:

$$\begin{array}{l}\left({\dot{E}}_{x,502}^{DT}-{\dot{E}}_{x,507}^{DT}\right)C_{DT}\\ =\left({\dot{E}}_{x,503}^{DT}-{\dot{E}}_{x,504}^{DT}\right)C_{11DT}+\left({\dot{E}}_{x,506}^{DT}-{\dot{E}}_{x,507}^{DT}\right)C_{12DT}+\left({\dot{E}}_{x,502}^{DT}-{\dot{E}}_{x,503}^{DT}\right)C_{14DT}\\ +\left({\dot{E}}_{x,504}^{DT}-{\dot{E}}_{x,505}^{DT}\right)C_{15DT}+\left({\dot{E}}_{x,505}^{DT}-{\dot{E}}_{x,506}^{DT}\right)C_{16DT}\end{array}$$

(79)

Mechanical exergy junction for water flow:

$$\left({\dot{E}}_{x,501}^{DP}-{\dot{E}}_{x,502}^{DP}\right)C_{DP}=\left({\dot{E}}_{x,501}^P-{\dot{E}}_{x,502}^P\right){\mathrm{C}}_{13\mathrm{D}\mathrm{P}}$$

(80)

Work exergy junction:

$${\dot{E}}_{x,<2>}^W{C}_W={\dot{E}}_{x,<2>}^W{\mathrm{C}}_{2\mathrm{W}}$$

(81)

System boundary:

$$\begin{array}{l}\left({\dot{E}}_{x,512}^{DT}-{\dot{E}}_{x,511}^{DT}+{\dot{E}}_{x,514}^{DT}-{\dot{E}}_{x,513}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,512}^{DP}-{\dot{E}}_{x,511}^{DP}+{\dot{E}}_{x,514}^{DP}-{\dot{E}}_{x,513}^{DP}\right)C_{DP}\\ -\left({\dot{E}}_{x,303}^T+{\dot{E}}_{x,312}^T\right)C_T-\left({\dot{E}}_{x,303}^P+{\dot{E}}_{x,312}^P\right)C_P+T_0\left({\dot{S}}_{512}-{\dot{S}}_{511}+{\dot{S}}_{514}-{\dot{S}}_{513}+{\dot{S}}_{303}+{\dot{S}}_{312}\right)C_S=0\end{array}$$

(82)

4.3. Exergy Cost-Balance Equations for the HTWR System

In the case of the HTGR, the equations obtained by applying the exergy cost-balance equation given in Equation (53) for each device of the HTWR in Figure 2 are as follows:

1.

Reactor <1>:

$${\dot{Q}}^{UR}{C}_{UR}+\left({\dot{E}}_{x,505}^{DT}-{\dot{E}}_{x,501}^{DT}\right)C_{1DT}+\left({\dot{E}}_{x,505}^{DP}-{\dot{E}}_{x,501}^{DP}\right)C_{DP}+T_0\left({\dot{S}}_{505}-{\dot{S}}_{501}+\frac{{\dot{Q}}_{<1>}}{T_0}\right)C_S+{\dot{Z}}_{<1>}=0$$

(83)

2.

Steam generator <2>:

$$\begin{array}{l}\left[\left({\dot{E}}_{x,501}^{DT}-{\dot{E}}_{x,502}^{DT}\right)+\left({\dot{E}}_{x,513}^{DT}-{\dot{E}}_{x,514}^{DT}\right)\right]C_{2DT}+\left[\left({\dot{E}}_{x,501}^{DP}-{\dot{E}}_{x,502}^{DP}\right)+\left({\dot{E}}_{x,513}^{DP}-{\dot{E}}_{x,514}^{DP}\right)\right]C_{DP}+\\ T_0\left({\dot{S}}_{501}-{\dot{S}}_{502}+{\dot{S}}_{513}-{\dot{S}}_{514}+\frac{{\dot{Q}}_{<2>}}{T_0}\right)C_S+{\dot{Z}}_{<2>}=0\end{array}$$

(84)

3.

Turbine 1 <3>:

$$\left({\dot{E}}_{x,521}^{DT}-{\dot{E}}_{x,522}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,521}^{DP}-{\dot{E}}_{x,522}^{DP}\right)C_{DP}+T_0\left({\dot{S}}_{521}-{\dot{S}}_{522}+\frac{{\dot{Q}}_{<3>}}{T_0}\right)C_S+{\dot{Z}}_{<3>}={\dot{E}}_{x,<3>}^W{C}_{3W}$$

(85)

4.

Turbine 2 <4>:

$$\left({\dot{E}}_{x,523}^{DT}-{\dot{E}}_{x,524}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,523}^{DP}-{\dot{E}}_{x,524}^{DP}\right)C_{DP}+T_0\left({\dot{S}}_{523}-{\dot{S}}_{524}+\frac{{\dot{Q}}_{<4>}}{T_0}\right)C_S+{\dot{Z}}_{<4>}={\dot{E}}_{x,<4>}^W{C}_{4W}$$

(86)

5.

Generator <5>:

$$\left({\dot{E}}_{x,<3>}^W+{\dot{E}}_{x,<4>}^W\right)C_W-{\dot{E}}_{x,<5>}^D{C}_S+{\dot{Z}}_{<5>}={\dot{E}}_{x,<5>}^W{C}_{5E}$$

(87)

6.

Superheater <6>:

$$\begin{array}{l}\left[\left({\dot{E}}_{x,502}^{DT}-{\dot{E}}_{x,504}^{DT}\right)+\left({\dot{E}}_{x,527}^{DT}-{\dot{E}}_{x,520}^{DT}\right)\right]C_{6DT}+\left[\left({\dot{E}}_{x,502}^{DP}-{\dot{E}}_{x,504}^{DP}\right)+\left({\dot{E}}_{x,527}^{DP}-{\dot{E}}_{x,520}^{DP}\right)\right]C_{DP}+\\ T_0\left({\dot{S}}_{502}-{\dot{S}}_{504}+{\dot{S}}_{527}-{\dot{S}}_{520}+\frac{{\dot{Q}}_{<6>}}{T_0}\right)C_S+{\dot{Z}}_{<6>}=0\end{array}$$

(88)

7.

Pump 1 <7>:

$$\left({\dot{E}}_{x,511}^{DT}-{\dot{E}}_{x,512}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,511}^{DP}-{\dot{E}}_{x,512}^{DP}\right)C_{7DP}+T_0\left({\dot{S}}_{511}-{\dot{S}}_{512}+\frac{{\dot{Q}}_{<7>}}{T_0}\right)C_S+{\dot{Z}}_{<7>}=E_{x,<7>}^W{C}_W$$

(89)

8.

Recuperator <8>:

$$\begin{array}{l}\left[\left({\dot{E}}_{x,512}^{DT}-{\dot{E}}_{x,513}^{DT}\right)+\left({\dot{E}}_{x,525}^{DT}-{\dot{E}}_{x,526}^{DT}\right)\right]C_{8DT}+\left[\left({\dot{E}}_{x,512}^{DP}-{\dot{E}}_{x,513}^{DP}\right)+\left({\dot{E}}_{x,525}^{DP}-{\dot{E}}_{x,526}^{DP}\right)\right]C_{DP}+\\ T_0\left({\dot{S}}_{512}-{\dot{S}}_{513}+{\dot{S}}_{525}-{\dot{S}}_{526}+\frac{{\dot{Q}}_{<8>}}{T_0}\right)C_S+{\dot{Z}}_{<8>}=0\end{array}$$

(90)

9.

Pump 2 <9>:

$$\left({\dot{E}}_{x,526}^{DT}-{\dot{E}}_{x,527}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,526}^{DP}-{\dot{E}}_{x,527}^{DP}\right)C_{9DP}+T_0\left({\dot{S}}_{526}-{\dot{S}}_{527}+\frac{{\dot{Q}}_{<9>}}{T_0}\right)C_S+{\dot{Z}}_{<9>}=E_{x,<9>}^W{C}_W$$

(91)

10.

Pump 3 <10>:

$$\left({\dot{E}}_{x,504}^{DT}-{\dot{E}}_{x,505}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,504}^{DP}-{\dot{E}}_{x,505}^{DP}\right)C_{10DP}+T_0\left({\dot{S}}_{504}-{\dot{S}}_{505}+\frac{{\dot{Q}}_{<10>}}{T_0}\right)C_S+{\dot{Z}}_{<10>}=E_{x,<10>}^W{C}_W$$

(92)

11.

Reformer <11>:

$$\begin{array}{l}-{\dot{E}}_{x<5>}^W{C}_{5E}+\left({\dot{E}}_{x<7>}^W+{\dot{E}}_{x<9>}^W+{\dot{E}}_{x<10>}^W{\dot{E}}_{x<14>}^W\right)C_W+{\dot{E}}_{x,514}^{DT}{C}_{DT}+\left({\dot{E}}_{x,301}^T-{\dot{E}}_{x,302}^T\right)C_T+{\dot{E}}_{x,514}^{DP}{C}_{DP}\\ +\left({\dot{E}}_{x,301}^P-{\dot{E}}_{x,302}^P\right)C_P+T_0\left({\dot{S}}_{301}+{\dot{S}}_{514}-{\dot{S}}_{302}+\frac{{\dot{Q}}_{<11>}}{T_0}\right)C_S-{\dot{n}}_{H2}\cdot Ene{r}_{H2}\cdot C_{11H2}+{\dot{Z}}_{<11>}=0\end{array}$$

(93)

12.

Water-gas shift reactor <12>:

$$\begin{array}{l}\left({\dot{E}}_{x,302}^T-{\dot{E}}_{x,304}^T-{\dot{E}}_{x,305}^T\right)C_{12T}+\left({\dot{E}}_{x,302}^P-{\dot{E}}_{x,304}^P-{\dot{E}}_{x,305}^P\right)C_P+T_0\left({\dot{S}}_{302}-{\dot{S}}_{303}+{\dot{S}}_{302}-{\dot{S}}_{303}+\frac{{\dot{Q}}_{<12>}}{T_0}\right)C_S\\ +{\dot{m}}_{CO2}\cdot C_{m,CO2}+{\dot{Z}}_{<12>}=0\end{array}$$

(94)

In Equation (94), ${\dot{m}}_{CO2}$ and $C_{m,CO2}$ are the mass flow rate of CO₂ gas and the carbon tax imposed per kg of CO₂ gas, respectively.

Heat exchanger <13>:

$$\begin{array}{l}\left({\dot{E}}_{x,305}^T-{\dot{E}}_{x,306}^T\right)C_{13T}+\left({\dot{E}}_{x,305}^P-{\dot{E}}_{x,306}^P\right)C_P+\left({\dot{E}}_{x,531}^{DT}-{\dot{E}}_{x,532}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,531}^{DP}-{\dot{E}}_{x,532}^{DP}\right)C_{DP}\\ +T_0\left({\dot{S}}_{305}-{\dot{S}}_{306}+{\dot{S}}_{531}-{\dot{S}}_{532}+\frac{{\dot{Q}}_{<13>}}{T_0}\right)C_S+{\dot{Z}}_{<13>}=0\end{array}$$

(95)

14.

Compressor <14>:

$$\left({\dot{E}}_{x,300}^T-{\dot{E}}_{x,301}^T\right)C_T+\left({\dot{E}}_{x,300}^P-{\dot{E}}_{x,301}^P\right)C_{14P}+T_0\left({\dot{S}}_{300}-{\dot{S}}_{301}+\frac{{\dot{Q}}_{<14>}}{T_0}\right)C_S+{\dot{Z}}_{<14>}=E_{x,<14>}^W{C}_W$$

(96)

As shown above, 14 cost-balance equations were obtained from the 14 components. However, the unknowns shown in the cost-balance equations described above are C_1DT, C_2DT, C_3W, C_4W, C_5E, C_6DT, C_7DP, C_8DT, C_9DP, C_10DP, C_11H2, C_12T, C_13T, C_14P, C_T, C_P, C_DT, C_DP, C_W, and C_S, which are 20 unknowns. Therefore, six additional auxiliary equations are required to determine the unknowns. Six additional equations can be obtained for the thermal and mechanical exergy junctions for water flow, thermal and mechanical exergy junctions for gas flow, work exergy junction, and boundary conditions, which are as follows:

Thermal exergy junction for water flow:

$$\begin{array}{l}\left[\left({\dot{E}}_{x,505}^{DT}-{\dot{E}}_{x,504}^{DT}\right)+\left({\dot{E}}_{x,512}^{DT}-{\dot{E}}_{x,514}^{DT}\right)+\left({\dot{E}}_{x,527}^{DT}-{\dot{E}}_{x,520}^{DT}\right)+\left({\dot{E}}_{x,525}^{DT}-{\dot{E}}_{x,526}^{DT}\right)\right]C_{DT}\\ =\left({\dot{E}}_{x,505}^{DT}-{\dot{E}}_{x,501}^{DT}\right)C_{1DT}+\left[\left({\dot{E}}_{x,501}^{DT}-{\dot{E}}_{x,502}^{DT}\right)+\left({\dot{E}}_{x,513}^{DT}-{\dot{E}}_{x,514}^{DT}\right)\right]{\mathrm{C}}_{2\mathrm{D}\mathrm{T}}\\ +\left[\left({\dot{E}}_{x,502}^{DT}-{\dot{E}}_{x,504}^{DT}\right)+\left({\dot{E}}_{x,527}^{DT}-{\dot{E}}_{x,520}^{DT}\right)\right]{\mathrm{C}}_{6\mathrm{D}\mathrm{T}}+\left[\left({\dot{E}}_{x,512}^{DT}-{\dot{E}}_{x,513}^{DT}\right)+\left({\dot{E}}_{x,525}^{DT}-{\dot{E}}_{x,526}^{DT}\right)\right]{\mathrm{C}}_{8\mathrm{D}\mathrm{T}}\end{array}$$

(97)

Mechanical exergy junction for water flow:

$$\begin{array}{l}\left[\left({\dot{E}}_{x,511}^{DP}-{\dot{E}}_{x,512}^{DP}\right)+\left({\dot{E}}_{x,526}^{DP}-{\dot{E}}_{x,527}^{DP}\right)+\left({\dot{E}}_{x,504}^{DP}-{\dot{E}}_{x,505}^{DP}\right)\right]C_{DP}=\left({\dot{E}}_{x,511}^{DP}-{\dot{E}}_{x,512}^{DP}\right){\mathrm{C}}_{7\mathrm{D}\mathrm{P}}\\ +\left({\dot{E}}_{x,526}^{DP}-{\dot{E}}_{x,527}^{DP}\right){\mathrm{C}}_{9\mathrm{D}\mathrm{P}}+\left({\dot{E}}_{x,504}^{DP}-{\dot{E}}_{x,505}^{DP}\right)C_{10DP}\end{array}$$

(98)

Thermal exergy junction for gas flow:

$$\left[\left({\dot{E}}_{x,302}^T-{\dot{E}}_{x,303}^T\right)+\left({\dot{E}}_{x,305}^T-{\dot{E}}_{x,306}^T\right)\right]C_T=\left({\dot{E}}_{x,302}^T-{\dot{E}}_{x,303}^T\right)C_{12T}+\left({\dot{E}}_{x,305}^T-{\dot{E}}_{x,306}^T\right)C_{13T}$$

(99)

Mechanical exergy junction for gas flow:

$$\left({\dot{E}}_{x,300}^P-{\dot{E}}_{x,301}^P\right)C_P=\left({\dot{E}}_{x,300}^P-{\dot{E}}_{x,301}^P\right){\mathrm{C}}_{14\mathrm{P}}$$

(100)

Work exergy junction:

$$\left({\dot{E}}_{x,<3>}^W+{\dot{E}}_{x,<4>}^W\right)C_W={\dot{E}}_{x,<3>}^W{\mathrm{C}}_{3\mathrm{W}}+{\dot{E}}_{x,<4>}^W{\mathrm{C}}_{4\mathrm{W}}$$

(101)

System boundary:

$$\begin{array}{l}\left({\dot{E}}_{x,511}^{DT}+{\dot{E}}_{x,531}^{DT}-{\dot{E}}_{x,532}^{DT}\right)C_{DT}+\left({\dot{E}}_{x,511}^{DP}+{\dot{E}}_{x,531}^{DP}-{\dot{E}}_{x,532}^{DP}\right)C_{DP}+\left({\dot{E}}_{x,300}^T-{\dot{E}}_{x,304}^T-{\dot{E}}_{x,306}^T\right)C_T+\\ \left({\dot{E}}_{x,300}^P-{\dot{E}}_{x,304}^P-{\dot{E}}_{x,306}^P\right)C_P+T_0\left({\dot{S}}_{511}+{\dot{S}}_{531}-{\dot{S}}_{532}+{\dot{S}}_{300}-{\dot{S}}_{306}\right)C_S=0\end{array}$$

(102)

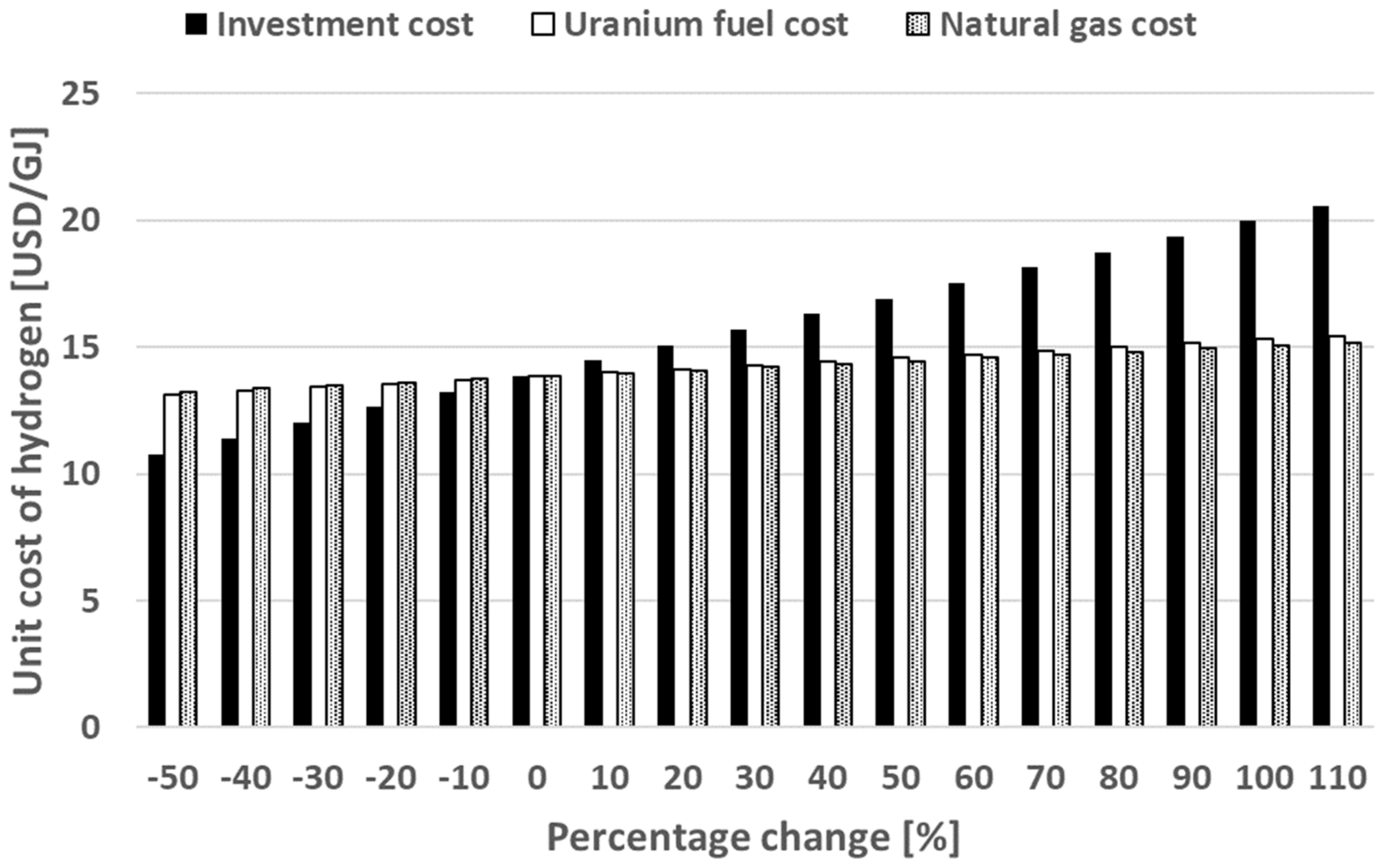
5. Results and Discussion

5.1. HTGR System

Table 1 shows the enthalpy, entropy, and exergy flow rates calculated using thermodynamic properties, such as the molar flow rate (kmol/s), temperature, and pressure, at the state points of the HTGR system. The preliminary design of the HTGR system at the Idaho Nuclear Research Laboratory [5] was referenced to determine the temperature and pressure at the critical state points. The flow rate of the working fluid was determined by trial and error to establish an energy conservation law for each device. The helium gas at 850 °C and 278 kg/s from the reactor enters the turbine <2> to produce work, and the helium at 40 kg/s enters the heat exchanger <9> and supplies heat to the gas circulating in the secondary helium loop whose mass flow rate is 23.0 kg/s. The molar flow rate of the water vapor entering the state point 527 was 1.28 kmol/s. The costs of the materials consumed in the HTGR and HTWR systems were assumed to be USD 3.5/GJ for uranium and USD 10.0/GJ for natural gas.

Table 1. Estimation of hydrogen production cost using a small modular reactor (SMR) (Reference [13]).

Account	Cost Per Year ($M/year)	Cost Flow Rate (USD/s)	Percent of Total
SMR plant capital charges	181.2	5.74	36.1
SMR plant O&M costs	37.4	1.19	7.5
H2 plant capital charges	135.3	4.29	26.9
H2 plant O&M costs	76.6	2.43	15.3
Nuclear fuel cost	71.2	2.26	14.2
Total	501.7	15.91	100.

If the gas temperature of helium exiting the nuclear reactor is 950 °C, the turbine can produce more electricity and the temperature of the steam entering the electrolyzer can be slightly higher, such that additional water vapor can be electrolyzed. However, even if the temperature of the water exiting the reactor is lowered and the temperature of the steam entering the electrolytic cell is lowered, this is not a significant problem. This is because the amount of water decomposed in the electrolytic cell is primarily determined by the amount of electricity supplied.

Table 2 shows the enthalpy, entropy, and exergy flow rates calculated using thermodynamic properties such as the molar flow rates, temperature and pressure at the state points of the HTGR system. Table 3 shows energy conservation figures for each device in the HTGR system. To rephrase, the amount of heat introduced into each device is the sum of the enthalpy flow out of the device, the enthalpy flow into the device, and the work performed by the device. In particular, in electrolyzer <17>, the amount of water vapor introduced into the device is determined by the amount of electricity input from the generator. From Table 2, the mass flow rate of water vapor entering the electrolyzer is approximately 23 kg/s, producing 1.277 kmol of hydrogen and 0.64 kmol of oxygen per second.

Table 2. Property values and enthalpy, entropy, and exergy flow rates at various state points in the GT-HTGR system.

States	$\dot{\mathit{n}}$ (kmole/s)	T (°C)	P (kPa)	H (kJ/s)	S (kJ/s-K)	Ex (kJ/s)
101	69.493	850.05	7100.0	1,191,862.03	−539.32	1,352,659.75
102	69.493	531.49	2600.0	731,678.16	−400.70	863,073.59
103	9.9990	850.05	7100.0	171,490.94	−77.60	194,627.30
104	9.9990	577.05	7100.0	114,746.47	−135.48	155,139.90
105	69.493	470.00	2550.0	642,844.23	−544.33	805,136.53
106	69.493	27.05	2500.0	2961.43	−1842.34	552,255.01
107	69.493	109.26	4300.0	121,721.01	−1806.04	660,190.72
108	69.493	27.05	4250.0	2961.43	−2148.93	643,665.80
109	69.493	103.56	7100.0	113,487.96	−2117.48	744,814.38
110	79.492	440.0	7100.0	685,766.34	−1367.53	1,093,496.89
111	79.492	488.05	7100.0	765,166.47	−1259.79	1,140,772.31
115	5.8244	800.0	450.0	93,833.64	54.64	50,523.54
116	5.8244	981.38	650.0	115,794.29	83.97	90,759.09
117	5.8244	750.35	600.0	87,822.23	63.20	6897.60
118	5.8244	566.85	500.0	64,394.09	46.66	50,482.80
301	1.2767	536.85	250.0	19,072.03	37.15	19,072.03
302	1.2767	476.85	200.0	16,801.64	34.24	16,801.64
303	1.2767	57.0	150.0	1179.74	3.76	1179.74
311	0.6384	536.85	250.0	10,396.94	20.07	10,396.94
312	0.6384	266.85	200.0	4733.97	11.58	4733.97
501	1.2767	27.05	101.0	2608.77	9.10	0.67
502	1.2767	27.07	600.0	2621.24	9.10	12.19
503	1.2767	75.0	550.0	7229.99	23.35	373.68
504	1.2767	389.85	500.0	74,771.08	178.55	21,641.04
505	1.2767	440.0	450.0	77,205.65	183.20	2268.81
506	1.2767	509.85	400.0	80,637.86	189.04	24,380.97
507	1.2767	686.85	350.0	89,627.93	200.79	29,865.72
511	238.69	25.0	101.20	450,887.36	1578.01	−0.54
512	238.69	51.0	150.	918,287.15	3080.37	19,470.79
513	44.4074	25.0	101.20	83,886.02	293.58	−0.10
514	44.4074	53.0	150.	177,534.48	593.67	4178.01

Table 3. Energy balance for each component in the GT-HTGR system shown in Figure 1.

Component	Work (kJ/s)	Heat (kJ/s)	Enthalpy in (kJ/s)	Enthalpy Out (kJ/s)
Reactor<1>	0.0	−598,186.50	−765,166.47	1,363,352.97
Turbine<2>	460,183.87	0.0	−119,1862.03	731,678.16
Compressor 1<3>	−118,759.58		−2961.43	121,721.01
Compressor 2<4>	−110,526.54		−2961.43	113,487.96
Pump<13>	−12.47		−2608.77	2621.24
Blow<10>	−21,960.66		−93,833.64	115,794.29
Intercooler<6>		118,759.58	−121,721.01	2961.43
recooler<7>		639,882.81	−642,844.23	2961.43
Recuperator<8>		9433.80	−1,417,444.50	1,408,010.70
HTX<9>		27,304.93	−235,885.03	208,580.10
Steam generator<11>		−39,569.02	−123,024.28	162,593.31
Superheater<12>		14,438.07	−168,460.09	154,022.01
H2 Dryer<14>		11,013.15	−19,422.88	8409.73
O2 Recuperator<15>		80,434.05	−85,168.02	4733.97
H2 Recuperator<16>		−1161.82	−96,277.68	97,439.50
Electrolyzer<17>	−111,355.22	17,1514.18	−89,627.93	29,468.97

Table 4 lists the exergy flow of each component. The sum of the exergy flows for each component was zero, indicating that the exergy-balance equation was satisfied. From Table 4, turbine <2> produces 460.2 MW of work. Of this, 118.8 MW is supplied to compressor 1 <3> and 110.5 MW is supplied to compressor 2 <4>. The remainder of the work input into the generator produces electricity. Assuming that the efficiency of the generator is 95%, it supplies approximately 219 MW of electricity to the electrolyzer. It was assumed that the isentropic efficiency of the turbine was 85% and that the isentropic efficiency of the compressor was 84–85%. However, in the electrolyzer, 265 MW of energy is required to decompose 1.277 kmol of water vapor (state point 507) into hydrogen and oxygen. Therefore, the remaining energy, except for the electric energy input into the electrolyzer should be obtained from incoming water vapor. As shown in Table 2, this energy is approximately 54 MW, which is the difference between the enthalpy of the gas flowing into the electrolyzer and that of the gas flowing out. From these calculations, the temperature of the water vapor entering the electrolyzer does not significantly affect the electrolysis of water.

Table 4. Exergy balance for each component in the HTGR system.

	${\dot{\mathit{E}}}_{(\mathit{k})}^{\mathit{W}}$ (kW)	${\dot{\mathit{E}}}_{\mathit{x}}^{\mathit{T}}$ (kW)	${\dot{\mathit{E}}}_{\mathit{x}}^{\mathit{P}}$ (kW)	Irreversibility Rate (kW)
Reactor	−600,000.0 *	406,514.75		193,485.25
Turbine	460,183.87	−316,527.62	−173,058.54	29,402.30
Compressor 1	−118,759.58	14,510.06	93,425.65	10,823.87
Compressor 2	−110,526.54	12,744.32	88,404.27	9377.95
Intercooler		−10,371.10	−1975.71	12,346.81
Precooler		−230,209.24	−3200.94	233,410.19
Recuperator		−7316.51	−3345.13	10,661.64
HTX		−19,322.62	−1521.23	20,843.85
Steam generator		643.72	−1156.84	513.12
Super heater		−10,377.47	−2633.58	13,011.05
H2 Dryer		−15,259.25	−1.15	15,260.40
O2 Recuperator		−27,294.82	−9.19	27,304.02
H2 Recuperator		−577.08	−1.15	578.23
Pump	−12.47	0.01	11.51	0.95
Blow	−21,960.66	16,323.40	5309.35	327.92
Electrolyzer	−197,379.73	−393.02	−5.74	308,734.95

* Heat.

Table 5 lists the initial investment costs and cost flows per unit rate for each component in the HTGR system. Assuming that the total initial investment of the HTGR power plant is USD 2588.0 million, the construction cost is equivalent to USD 4300/kW, and the cost flow including maintenance fees and repair costs is approximately USD 9.91/s. When calculating the maintenance cost, it was assumed that the annual operation time was 5000 h, the life of the equipment was 30 years, the annual interest was 5%, and there was no depreciation cost after 30 years. From this table, the cost flow rate of the HTGR system used in this study was 62% compared to the cost flow rate of 15.91 USD/s of the system considered by the Idaho Nuclear Institute, USA. However, the initial investment cost considered in this study is twice that of the HTGR system considered by Kim et al. [10].

Table 5. Initial investments, annualized costs, and corresponding monetary flow rates of each component in the GT−HTGR system.

Component	Initial Investment Cost	Annualized Cost (USD × 106/yr)	Monetary Flow Rate
	(USD × 106)		(USD/s)
Reactor	757.69	49.29	2.9026
Turbine	275.54	17.92	1.0555
Compressor 1	92.00	5.98	0.3524
Compressor 2	69.00	4.49	0.2643
Generator	23.00	1.50	0.0811
Intercooler	18.40	1.20	0.0705
Precooler	18.40	1.20	0.0705
Recuperator	27.60	1.80	0.1057
HTX	18.40	1.20	0.0705
Blower	13.80	0.90	0.0529
Steam generator	27.60	1.80	0.1057
Superheater	27.60	1.80	0.1057
Pump	13.80	0.90	0.0529
H2 Dryer	18.40	1.20	0.0705
O2 recuperator	18.40	1.20	0.0705
H2 recuperator	18.40	1.20	0.0705
Electrolyzer	1150.00	74.8	4.4054
Total	2588.03	168.38	9.9072

Table 6 lists the cost flow for each exergy in each component obtained by solving the 23 exergy cost-balance equations from Equations (60)–(82). Since the sum of the exergy cost streams for each device was zero, the exergy cost-balance was correct. As shown in Table 6, the loss cost has a negative value, which can be regarded as the investment cost of the device. As shown in Table 6, because the cost flow of electricity from the generator is 3.889 USD/s and the electricity produced from the generator is approximately 230.9 MW, the unit cost of electricity produced from the generator is approximately USD 16.84/GJ (=USD 0.061/kWh). The more accurate unit costs of the primary product for each unit are shown in Table 7. For example, the unit cost of the work produced by a turbine (C_2W) is USD 16.49/GJ and the unit cost of electricity produced by a generator (C_5E) is USD 17.7/GJ (USD 0.064/kWh). In addition, the unit cost of hydrogen (C_17H2) produced in the electrolyzer is USD35.7/GJ, which corresponds to USD 4.28/(hydrogen per kg). Since the unit cost of electricity produced by an HTGR is cheaper than that of any other energy source [11], the unit cost of hydrogen production by electrolyzing water with electricity from an HTGR is substantially lower than that of any other system. The total lost cost flow due to entropy generation is approximately USD 0.0157/s, which is less than 0.7% of the total investment cost flow USD 12.0/s.

Table 6. Cost flow rates of various exergies, lost cost, and investment cost flow rates of each component in the GT-HTGR system. Unit is USD/s.

Component	Thermal	Mechan	Work	Lost Cost	Investment
Reactor	5.0347			−0.0321	−5.0026
Turbine	−2.3670	−4.1435	7.5709	−0.0049	−1.0555
Compressor 1	0.1085	2.1996	−1.9358	−0.0018	−0.3524
Compressor 2	0.0953	1.9890	−1.8184	−0.0016	−0.2643
Generator		−3.7987	3.8887	−0.0019	−0.0881
Intercooler	−0.0591	0.1323	−0.0006	−0.0021	−0.0705
Precooler	−0.7760	0.8887	−0.0035	−0.0388	−0.0705
Recuperator	0.1876	−0.0801		−0.0018	−0.1057
HTX	0.1104	−0.0364		−0.0035	−0.0705
Blower	0.1221	0.2922	−0.3613	−0.0001	−0.0529
Steam generator	0.1388	−0.0330		−0.0001	−0.1057
Super heater	0.1762	−0.0683		−0.0022	−0.1057
Pump		0.0531	−0.0002	0.0	−0.0529
H2 Dryer	0.0783	−0.0053		−0.0025	−0.0705
O2 recuperator	0.1174	−0.0424		−0.0045	−0.0705
H2 recuperator	0.0759	−0.0053		−0.0001	−0.0705
Electrolyzer *	−4.0878	−0.0264	−3.5272	0.0184	−4.4054

* In the electrolyzer, a cost flow rate of 12.0283 USD/s must be added for hydrogen production.

Table 7. Unit cost of various exergies in the cost-balance equations for the HTGR system. Unit is USD/GJ.

C1T	C2W	C3P	C4P	C5E	C6T	C7T	C8T
12.39	16.45	23.54	22.50	17.73	45.23	14.25	−25.64
C9T	C10P	C11DT	C12DT	C13DP	C14DT	C15DT	C16T
−5.71	55.02	13.78	53.75	4611.3	538.15	−7.39	54.85
C17H2	CT	CP	CDT	CDP	CW	Cs
35.65	7.48	23.94	144.29	4611.3	16.45	−0.17

Figure 3 shows the change in the unit cost of hydrogen as a percentage change in the investment and uranium fuel costs. If the initial investment cost of HTGR increases by 50%, the unit cost of electricity and hydrogen production increases by 27% (USD 22.5/GJ = USD 0.081/kWh) and 45% (USD 51.7/GJ = USD 6.2/kg hydrogen), respectively. If the unit cost of uranium fuel increases by 50% from USD 3.5/GJ to USD 5.25/GJ, the unit cost of hydrogen production increases by 9.6% (USD 39.0/GJ). This result indicates that the unit cost of hydrogen in this system is highly dependent on the investment cost as shown in Figure 3.

Figure 3. Change in unit cost of hydrogen according to a percentage change in investment and uranium fuel costs.

5.2. HTWR System

Table 8 shows the enthalpy, entropy, and exergy flow rates calculated using thermodynamic properties such as the molar flow rate (kmol/s), temperature, and pressure at the state points of the HTWR system. The preliminary design of the HTWR system at the Electric Power Research Institute [8] was used to determine the temperature and pressure at critical state points. The flow rate of the working fluid was determined through trial and error to establish the law of energy conservation for each component. However, unlike Kim et al. [10], the turbine was designed as a system that operates by an independent loop without using water from the nuclear reactor to reduce radiative contamination to the greatest extent possible. In the HTWR system, the molar flow rate of steam entering the reformer (state point 514) is 7.2162 kmol/s (130 kg/s), and the flow rate of natural gas (state point 301) is 2.2 kmol/s.

Table 8. Property values and enthalpy, entropy, and exergy flow rates at various state points in the HTGR system.

States	$\dot{\mathit{n}}$ (kmole/s)	T (°C)	P (kPa)	H (kJ/s)	S (kJ/s−K)	Ex (kJ/s)
300	2.2000	25.05	101.300	4.19	7.36	−2190.32
301	2.2000	202.56	600.000	17,127.67	51.96	1636.31
302	13.6103	426.85	400.000	179,864.52	503.83	29,648.05
304	8.0958	326.85	200.000	79,685.41	253.49	4106.74
305	5.5145	326.85	200.000	63,097.87	185.70	7732.72
306	2.6036	25.05	101.300	4.77	9.59	−2855.32
501	37.1912	850.05	6700.000	2,847,520.14	5155.82	1,313,422.84
502	37.1912	646.85	6650.000	2,520,159.30	4849.22	1,077,418.12
504	37.1912	429.85	6450.000	2,175,795.72	4430.93	857,768.44
505	37.1912	426.85	6850.000	2,166,436.58	4400.45	857,495.57
511	7.2162	25.05	101.200	13,658.65	47.80	−0.01
512	7.2162	25.06	680.000	13,733.69	47.80	75.44
513	7.2162	150.05	700.000	82,225.49	239.45	11,426.97
514	7.2162	726.85	620.000	518,229.92	1112.55	187,116.32
520	8.3264	599.85	5800.000	548,627.72	1077.47	228,064.92
521	6.6611	600.00	5800.000	438,944.23	862.02	182,479.62
522	6.6611	310.22	700.000	369,741.37	880.40	107,796.78
523	1.6653	600.00	5800.000	109,736.06	215.51	45,619.91
524	1.6653	310.22	700.000	92,435.34	220.10	26,949.20
525	8.3264	310.22	700.000	462,176.71	1100.50	134,745.98
526	8.3264	159.85	650.000	101,226.40	291.14	15,106.90
527	8.3264	159.86	6100.00	101,714.99	290.19	15,878.27
531	29.4199	25.05	101.30	55,685.29	194.87	0.0
532	29.4199	51.85	150.0	115,067.90	385.48	2553.24

As described above, the mass flow rate of water flowing through pump 1<7>, that is, the amount of water vapor flowing into reformer <11> and the flow rate of natural gas flowing into the compressor, are determined by the energy conservation law for the reformer. From Table 8, the molar flow rate of water flowing through pump 1 was 7.216 kmol/s (=130.0 kg/s), and the city gas introduced through the compressor <14> was approximately 2.3 kmol/s. In this case, the heat required for the reformer is obtained from the steam entering the reformer. Table 9 lists the energy conservation figures for each device in the HTWR system. In the reforming process, 304 MW of enthalpy is consumed from the input enthalpy of 535 MW. The mass flow rate of steam to the reformer is determined from Equation (10).

Table 9. Energy balance for each component in the HTWR system shown in Figure 2.

Component	Work (kJ/s)	Heat (kJ/s)	Enthalpy In (kJ/s)	Enthalpy Out (kJ/s)
Reactor<1>		−681,083.56	−2,166,436.58	2,847,520.14
Steam generator<2>		−108,643.58	−2,929,745.63	3,038,389.22
Superheater<3>		−102,549.15	−2,621,874.29	2,724,423.45
Pump 3<10>	−338.38	9697.53	−2,175,795.72	2,166,436.58
Turbine 1<3>	69,202.86		−438,944.23	369,741.37
Turbine 2<4>	17,300.71		−109,736.06	92,435.34
Pump 1<7>	−95.01	19.96	−13,658.65	13,733.69
Recuperator<8>		292,458.51	−475,910.40	183,451.89
Pump 2<9>	−1032.20	543.61	−101,226.40	101,715.0
Compressor<14>	−17,123.48		−4.19	17,127.67
Reformer<11>		596,063.09	−535,357.59	179,864.52
Water-gas shift <12>		28,528.20	−179,864.52	142,783.28
HTX<13>	−26.08	45,997.94	−63,097.87	17,126.00

Table 10 lists the exergy flow of each component. The sum of the exergy flows for each component is zero, indicating that the exergy-balance equation is satisfied. From the table, the work produced by turbine 1<3> is 69.2 MW, and the work produced by turbine 2<4> is approximately 17.3 MW. With the work produced by these turbines, the generator produces 82.2 MW of electricity to supply electricity for the compressor and pump, constituting a surplus of 19.0 MW. The surplus electricity provides the electricity required for the system.

Table 10. Exergy balance for each component in the HTWR system.

	${\dot{\mathit{E}}}_{(\mathit{k})}^{\mathit{W}}$ (kW)	${\dot{\mathit{E}}}_{\mathit{x}}^{\mathit{T}}$ (kW)	${\dot{\mathit{E}}}_{\mathit{x}}^{\mathit{P}}$ (kW)	Irreversibility Rate (kW),
Reactor	−600,000.0 *	455,792.28	135.00	144,072.72
Steam generator		−60,035.96	−279.41	60,315.37
Superheater		−7284.03	88.99	7195.04
Pump 3	−338.38	−540.85	267.98	611.25
Turbine 1	69,202.86	−74,069.96	−612.88	5479.98
Turbine 2	17,300.71	−18,499.48	−171.23	1370.00
Pump 1	−95.01	0.0010	75.45	19.56
Recuperator		−108,282.63	−4.91	108,287.54
Pump 2	−1032.20	−47.26	818.63	260.83
Compressor	−17,123.48	−5874.58	9701.21	13,296.85
Reformer		−195,665.27	36,560.69	−81,465.44
Water-gas shift	−8553.05	5577.55	−23,886.14	26,361.64
HTX	−26.07	4090.15	−9269.62	5205.54

* Heat input.

Table 11 lists the initial investment costs and cost flows per unit rate for each component in the HTWR system. Assuming that the total initial investment of the HTWR plant is USD 2417.9 million, the construction cost is equivalent to USD 4000/kW, and the cost flow including maintenance and repair costs is approximately USD 8.88/s. When calculating the maintenance cost, it was assumed that the annual operation time was 5000 h, the life of the equipment was 30 years, the annual interest is 5%, and there was no depreciation cost after 30 years.

Table 11. Initial investments, annualized costs, and corresponding monetary flow rates of each component in the ST-HTWR system.

Component	Initial Investment Cost	Annualized Cost (USD × 106/yr)	Monetary Flow Rate
	(USD × 106)		(USD/s)
Reactor	747.5	48.63	2.8635
Steam generator	18.4	1.20	0.0705
Turbine 1	138.0	8.98	0.5287
Turbine 2	121.9	7.93	0.4670
Generator	20.7	1.35	0.0793
Superheater	12.42	0.81	0.0476
Pump 1	8.28	0.54	0.0317
Recuperator	12.42	0.81	0.0476
Pump 2	12.42	0.81	0.0476
Pump 3	28.98	1.89	0.1110
Reformer	575.0	37.4	2.2027
Water-gas shift	575.0	37.4	2.2027
HTX	12.42	0.81	0.0476
Compressor	34.5	2.24	0.1322
Total	2317.94	150.8	8.880

Table 12 lists the cost flow for each exergy in each component obtained by solving the 20 exergy cost-balance equations from Equations (83)–(102). Since the sum of the exergy cost streams for each device was zero, the exergy cost-balance was correct. As shown in Table 12, the lost cost has a negative value, indicating that the amount of cost loss occurring in the component is the investment cost for the device. As listed in Table 12, the total amount of work produced by the two turbines is 86.5 MW. If the efficiency of the generator is 95%, the electricity produced by the generator is approximately 82.2 MW. The more accurate unit costs for the primary product for each component are presented in Table 13. For example, the unit costs of work produced by turbines 1<3> and turbine 2<4> is USD 40.5/GJ and USD 60.0/GJ, respectively, and the unit cost of electricity produced by the generator (C_5E) is USD 48.1/GJ (=USD 0.173/kWh). In addition, the unit cost of the hydrogen (C_12H2) produced in the electrolyzer is USD 13.92/GJ, which corresponds to USD 1.67/(hydrogen per kg). In this calculation, a carbon tax of USD 0.08/(kg of CO₂) was imposed on carbon dioxide, leaving a state point of 306. The unit cost of hydrogen produced at the HTWR plant by steam reforming was twice as high as that of hydrogen obtained by Kim et al. [9]. This is because the initial investment and uranium fuel costs in this study were nearly double those reported by Kim et al. [10]. The total lost cost flow due to entropy generation for HTWR is approximately USD2.5/s, which is approximately 12.0% of the total investment cost flow USD 20.0/s.

Table 12. Cost flow rates of various exergies, lost cost, and investment cost flow rates of each component in the ST-HTWR system. Unit is USD/s.

Component	Thermal	Mechan	Work	Lost Cost	Investment
Reactor	6.153	0.032		−1.222	−4.9635
Steam generator	0.649	−0.067		−0.511	−0.0705
Turbine 1	−2.077	−0.147	2.799	−0.046	−0.5287
Turbine 2	−0.519	−0.041	1.038	−0.012	−0.4670
Generator	3.954 *		−3.838	−0.037	−0.0793
Superheater	0.087	0.021		−0.061	−0.0476
Pump 1		0.036	−0.004		−0.0317
Recuperator	0.967	−0.001		−0.918	−0.0476
Pump 2	−0.001	0.097	−0.046	−0.002	−0.0476
Pump 3	−0.015	0.146	−0.015	−0.005	−0.1110
Reformer	−18.016	24.401	−3.129	0.691	−3.9470
Water-gas shift	5.186	−15.590	20.204 **	−0.224	−9.5773
HTX	6.283	−6.197	0.001	−0.044	−0.0476
Compressor	−5.462	6.467	−0.760	−0.113	−0.1312

* Electricity input, ** Cost flow rate.

Table 13. Unit cost of various exergies in the cost-balance equations for the ST-HTWR system. Unit is USD/GJ.

C1DT	C2DT	C3W	C4W	C5E	C6DT	C7DP	C8DT
13.50	−10.81	40.45	60.02	48.11	−11.97	478.47	−8.93
C9DP	C10DP	C11BT	C12H2	C13BP	C14BP	C15DT	C16DP
118.38	546.2	1482.2	13.92	3975.2	666.62	28.04	240.42
CT	CP	CW	CS
929.85	666.6	44.36	−8.48

As shown in Table 8, the molar flow rate of hydrogen exiting to state point 304 is 8.0958 kmol/s: thus, the HTWR system produces 6.3-fold more hydrogen than the HTGR system does. However, the unit cost of hydrogen production is only 38% lower than that of HTGR because the HTWR system not only requires natural gas as a fuel but also imposes a carbon tax on carbon dioxide emitted. If a carbon tax is not imposed on carbon dioxide, the unit cost of hydrogen would be USD 8.77/GJ, which corresponds to a unit cost of USD 1.1/kg.

Figure 4 shows the change in the unit cost of hydrogen as a percentage change in investment, uranium fuel, and natural gas costs. If the initial investment cost of HTWR increases by 50%, the unit cost of hydrogen production increases by 22% (USD 16.9/GJ). A 50% increase in the uranium fuel increases the unit cost of hydrogen production by 5.2% (USD 14.6/GJ). If the unit cost of natural gas increases by 50%, from USD 10.0/GJ to USD 15.0/GJ, the unit cost of the hydrogen production increases by 4.3% (USD 14.5/GJ). In contrast, the initial investment cost of the HTWR plant decreases by 50%, and the unit cost of hydrogen decreases to USD 10.79/GJ. The unit cost of hydrogen produced in this system is not highly dependent on the unit cost of the uranium fuel and natural gas, as shown in Figure 4.

Figure 4. Change in unit cost of hydrogen according to a percentage change in investment, uranium fuel, and natural gas costs.

5.3. Scaled-Down of the Plant

Small module reactors (SMRs) [28] have capacities below 300 MW. The development of a 1-MW SMR for hydrogen production is currently planned. The unit cost of hydrogen production was calculated when the scale of the 600 MW HTGR plant or 600 MW HTWR plant used in this study was scaled down to 1/10 or 1/100. This can be achieved by reducing the flow rate of the working fluid and initial investment. Table 14 lists the calculated hydrogen production rate and corresponding unit costs of hydrogen produced by the 60 MW and 6 MW HTGR plants and the 60 MW and 6 MW HTWR plants. The hydrogen production rate in scaled-down reactors decreases proportionally with reactor capacity: however, the unit cost of hydrogen produced in the scaled-down reactors does not change. The slight decrease in the hydrogen unit cost in the scaled-down HTWR plant was due to the reduced CO₂ production in that plant.

Table 14. Hydrogen production rate and corresponding unit cost of hydrogen scaled-down HTGR and HTWR reactors.

Plant	Hydrogen Production Rate	Unit Cost of Hydrogen
	(kg/s)	(USD/GJ)
HTGR 60-MW	0.257	35.6
HTWR 60-MW	1.628	13.8
HTGR 6-MW	0.0258	35.6
HTWR 6-MW	0.159	13.4

6. Conclusions

In this study, to obtain a reasonable unit price of hydrogen produced from HTGR and HTWR, energy, exergy, and thermoeconomic analysis was performed. In the flowsheet analysis, the temperature, pressure, and flow rate of the working fluid at the critical state point of the system were determined such that the energy conservation law was established for each component of the system. A thermoeconomic method called MOPSA (Modified Production Structure Method) provides the appropriate unit cost of the product and the loss cost flow of each component due to entropy generation. When the reactor was cooled with helium (HTGR) or water (HTWR), the temperature of the working fluid exiting the reactor was assumed to be 850 °C and the size of the reactor was set to 600 MW.

For the 600 MW HTGR system, electricity is produced using the Brayton cycle and hydrogen is produced by decomposing high-temperature water vapor using this electricity, resulting in 1.28 kmol (2.57 kg) of hydrogen per second and 0.6384 kmol (20.43 kg) of oxygen. When the initial investment amount of the system is USD 2.6 billion, the unit cost of hydrogen production is USD 35.6/GJ (=USD 4.28/kg) thanks to the low unit cost of power produced by the system at USD 0.064/kWh.

For the 60 MW HTWR system, water with a temperature of 850 °C and a mass flow rate of 670 kg/s exiting a nuclear reactor is converted into water vapor at a temperature of 650 °C through heat exchange and injected into the reformer. Hydrogen is produced through a reforming reaction with natural gas at a temperature of 200 °C and a molar flow rate of 2.2 kmol/s entering the reformer. The maximum hydrogen production possible in this system is 8.33 kmol/s (16.74 kg/s), which is 6.6-fold more hydrogen than the HTGR system, but also emits carbon dioxide with a molar flow rate of 2.60 kmol/s. With the initial investment being USD 2318 million for the HTWR system, the unit cost of natural gas is USD 10.0/GJ, the carbon tax is USD 0.08 per kg of carbon dioxide, and the unit cost of hydrogen produced is USD 13.85/GJ (USD1.66/kg)), which is substantially more economical than the current selling price of hydrogen, which is USD 3.7/kg.

A more detailed study of the electrolysis system is needed to achieve a reasonable unit cost of hydrogen from HTGR. Additional research is also needed on the addition of CO₂ removal plants to HTWR systems with steam reforming. Currently, different types of SMRs are being developed in many countries. In the near future, cheaper hydrogen could be produced through SMR.