Study on Flow and Heat Transfer Characteristics in Lamilloy Structure with Different Configurations of Internal Minichannels
Abstract
:1. Introduction
2. Numerical Methods and Validation
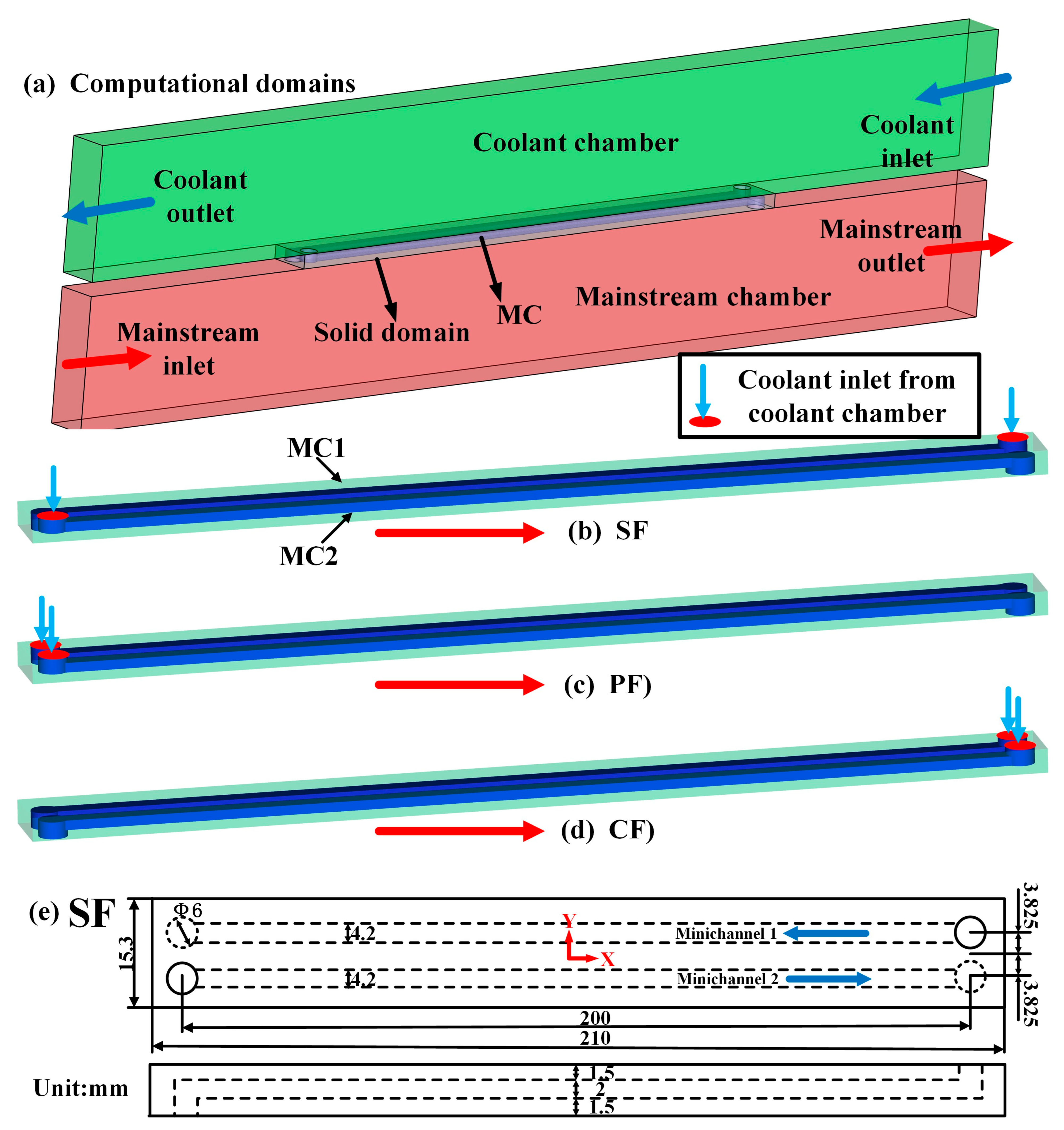
2.1. Physical Model
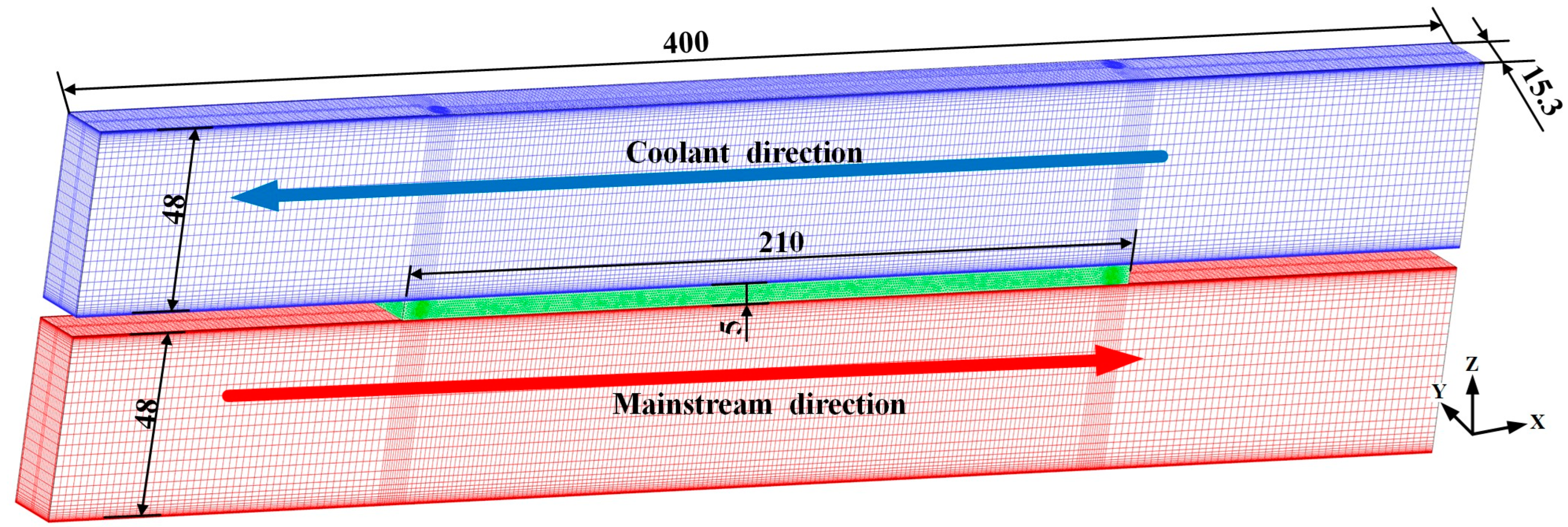
2.2. Computational Domain and Boundary Conditions
2.3. Turbulence Model Validation
2.4. Data Reduction
3. Results and Discussion
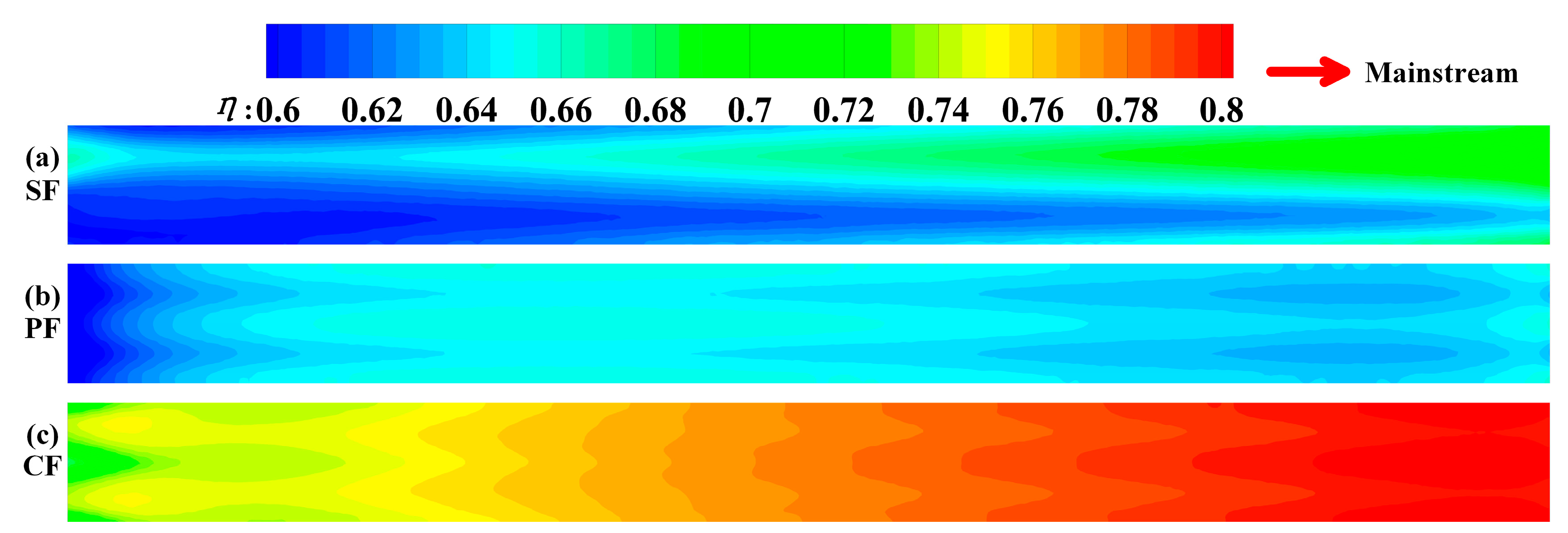
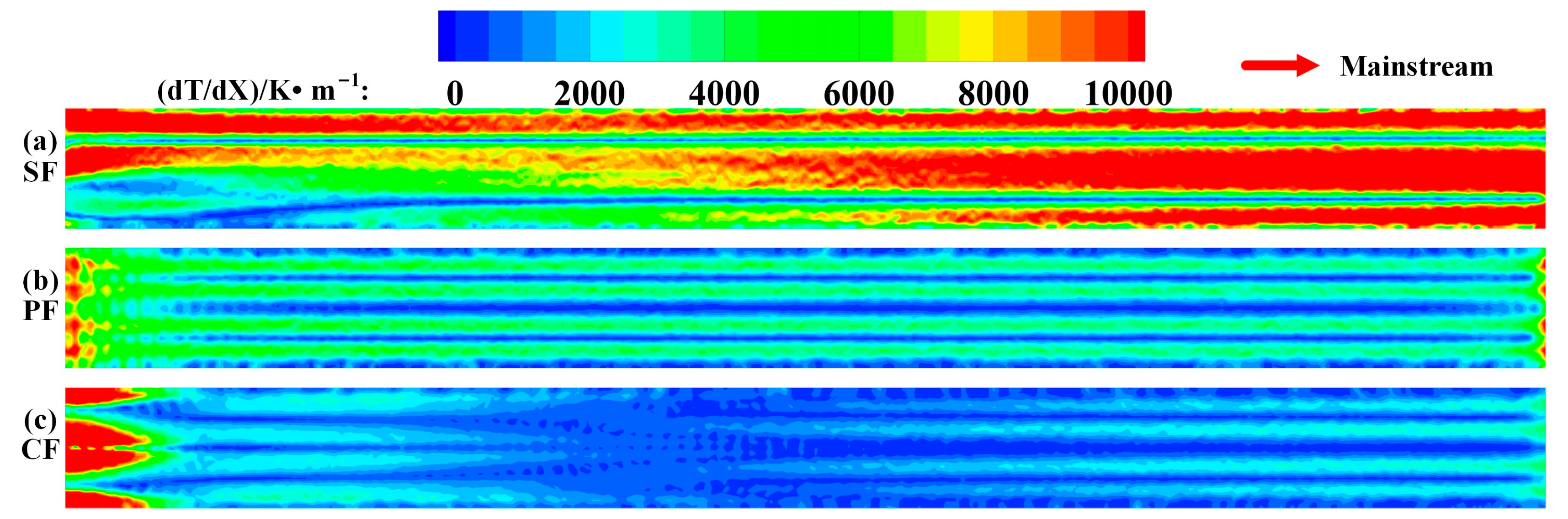
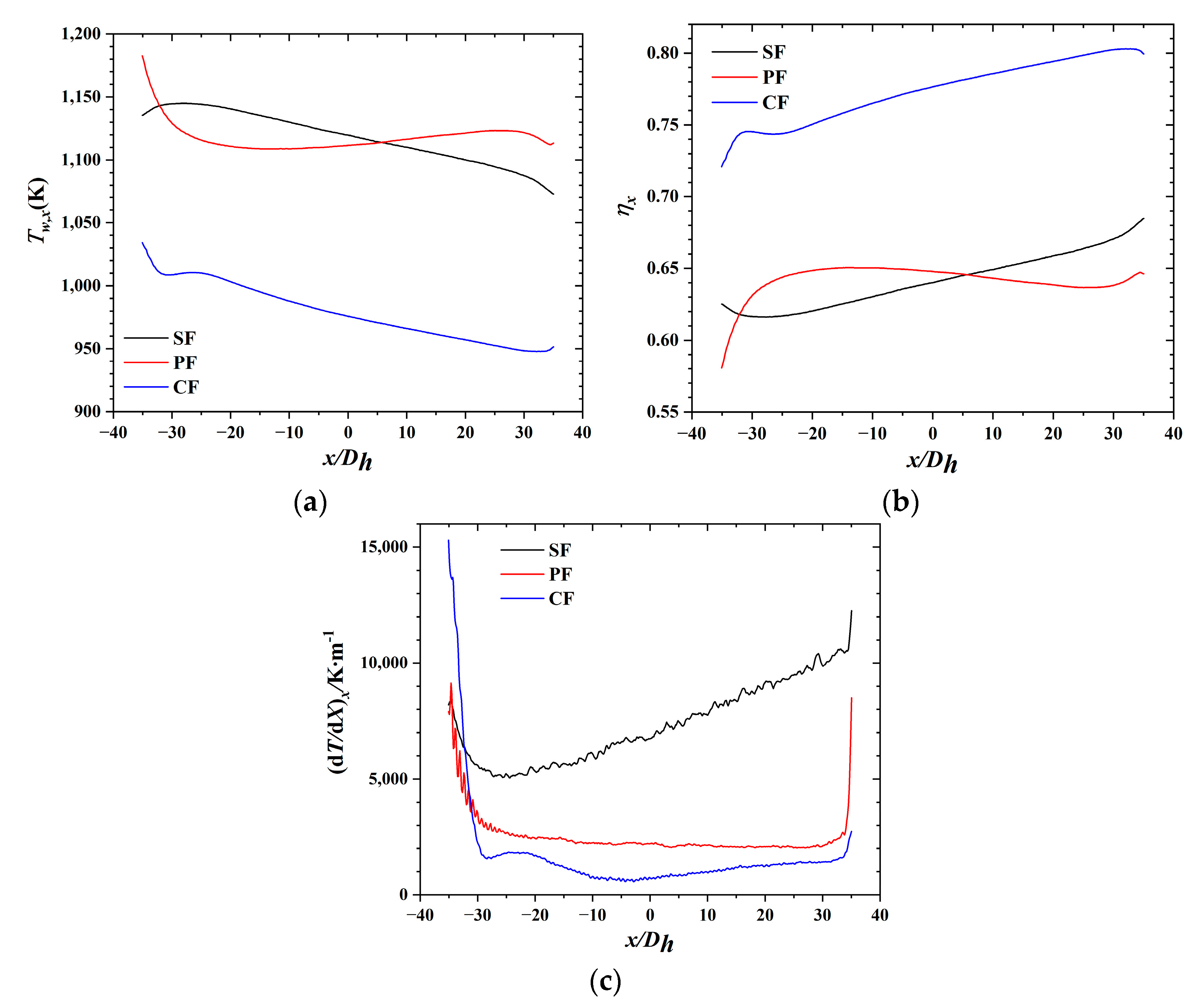
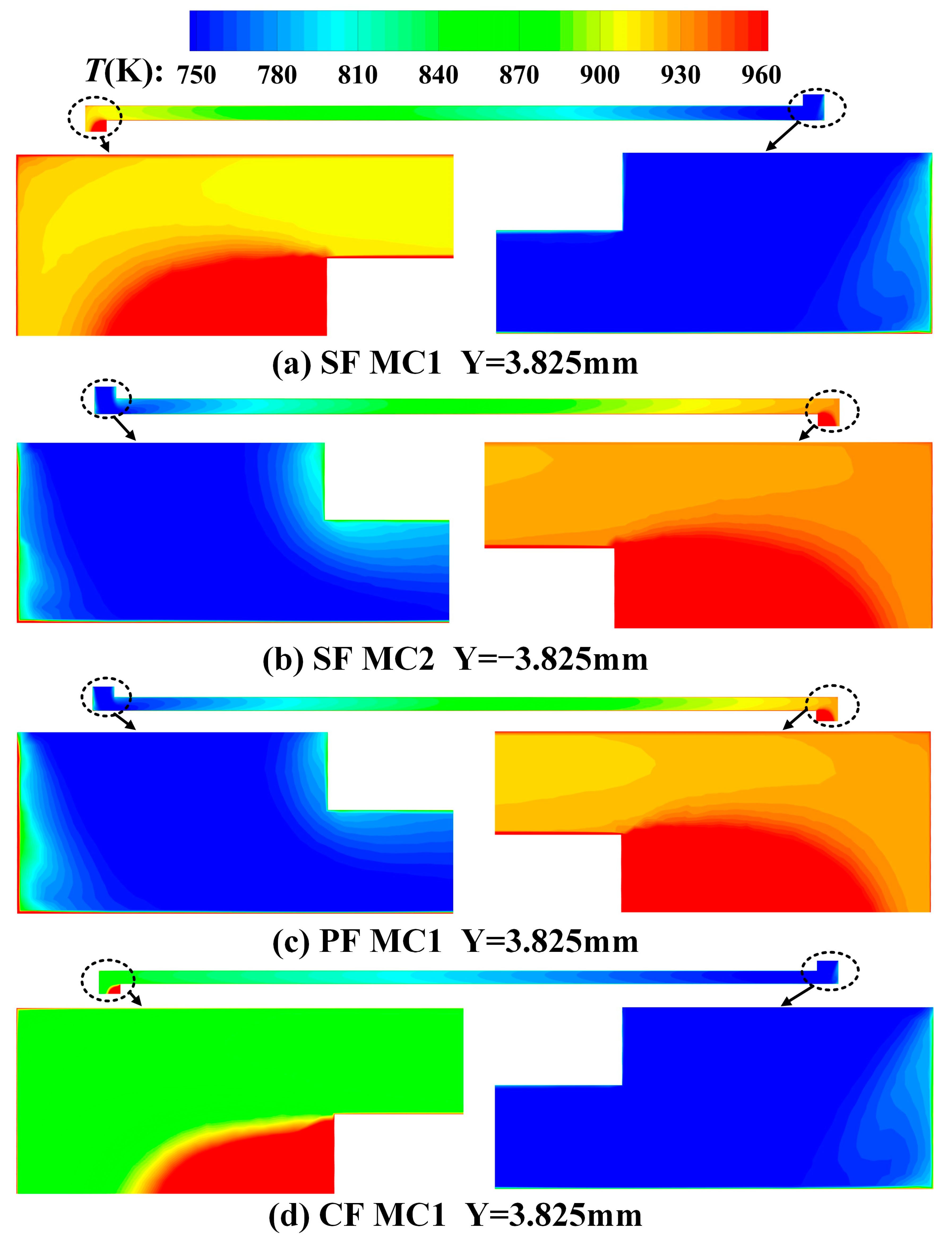
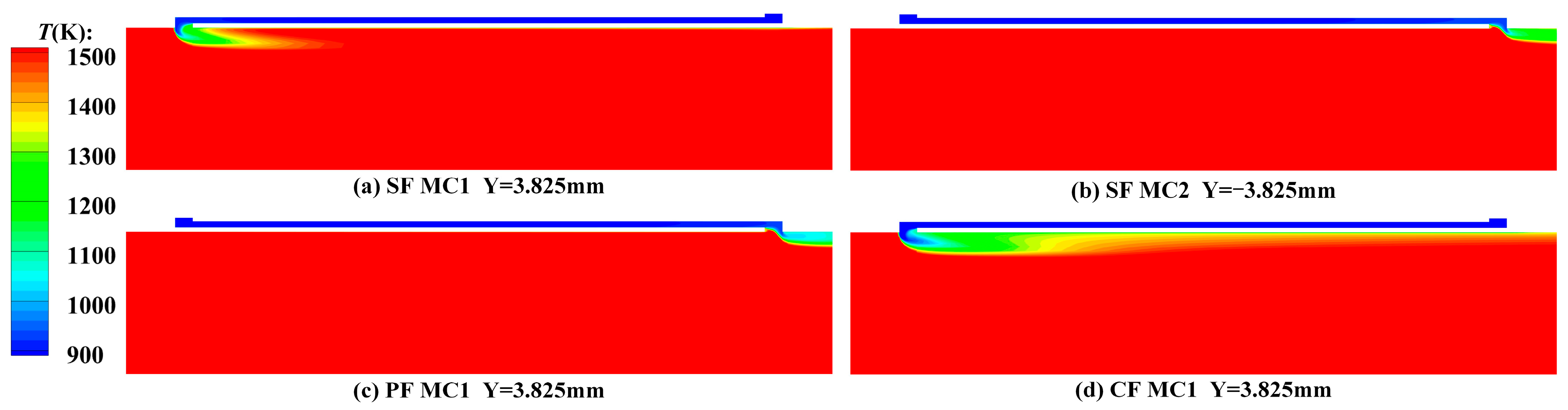
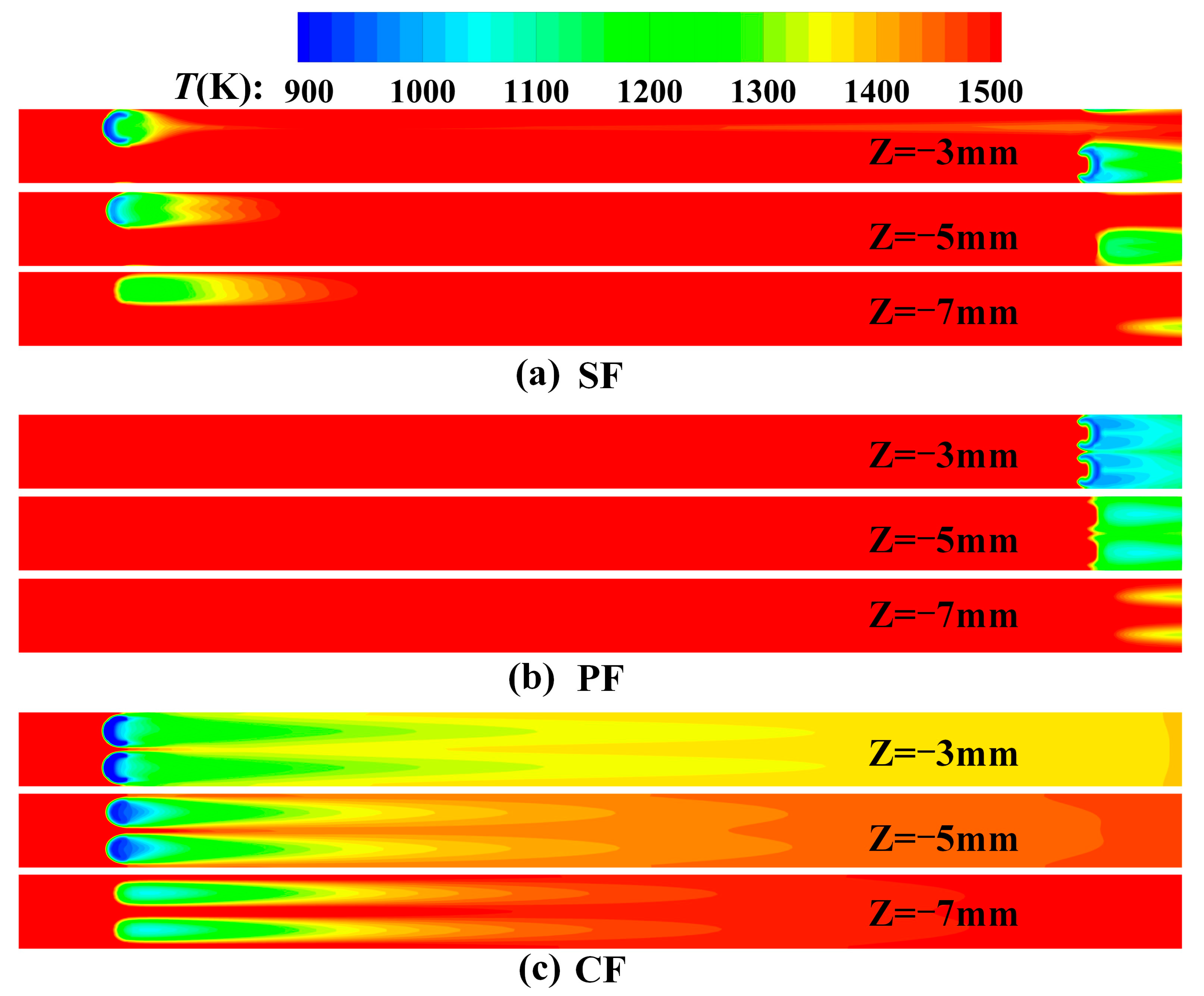
3.1. Heat Transfer Characteristic
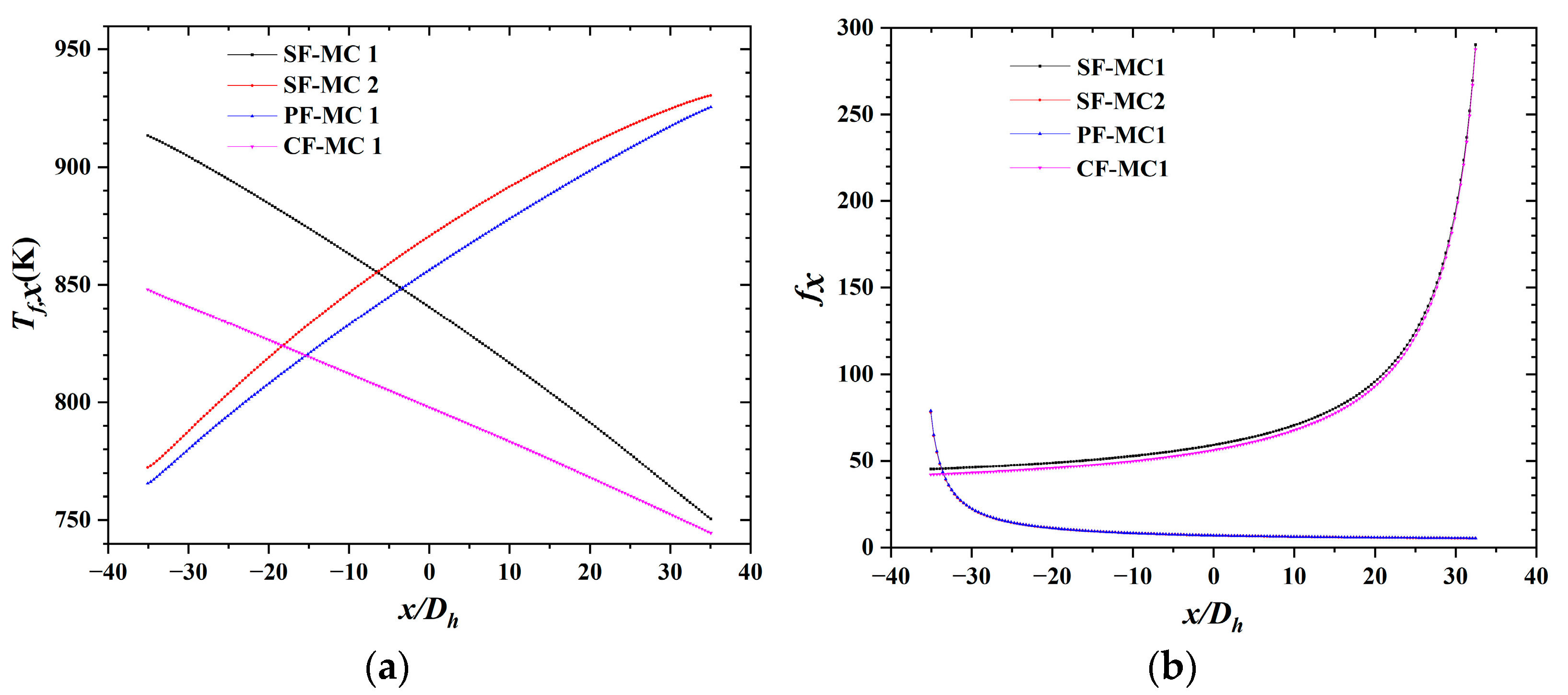
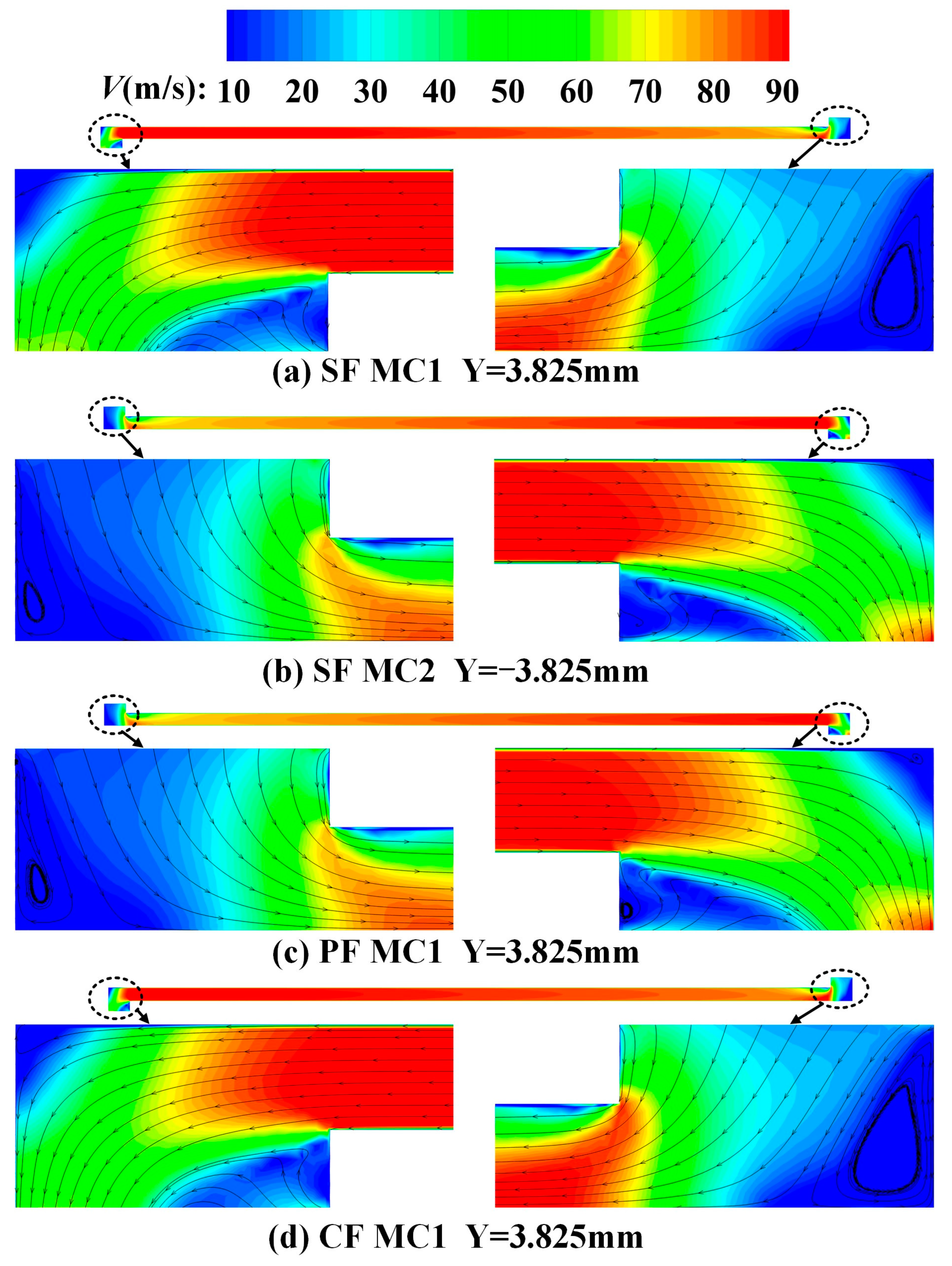
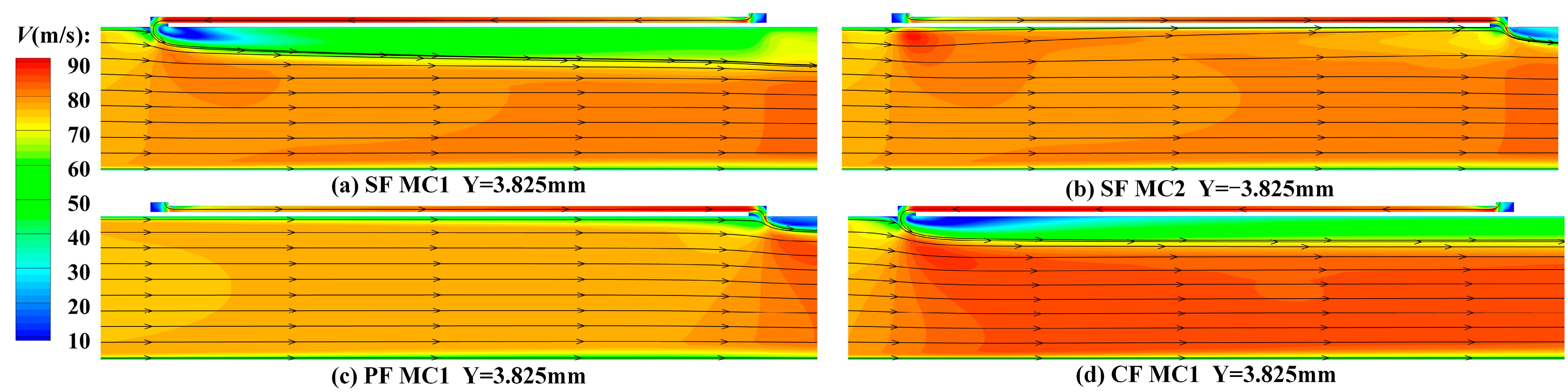
3.2. Flow Characteristic
4. Conclusions
- For the SF structure, the η at downstream of the SF structure is higher due to the impingement cooling of MC1; but at the same time, this deteriorates the cooling effect of the region at MC2. Therefore, this structure has the worst cooling effect and temperature uniformity.
- The ηave of the CF structure increased by 20.6% and 20.4%, and the decreased by 76.9% and 32.8%, compared with the SF structure and the PF structure, respectively. Namely, the CF structure possesses the best cooling effect and temperature uniformity.
- The coolant ejected from the MC1 of the SF structure completely blocks the direct contact between the mainstream and the lamilloy, while transferring more from the mainstream to the MC2 region.
- Film cooling at the two outlet of the CF structure transfers most of the mainstream to the lower position, which further improves the cooling effect of the two MCs.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Biot number | |
| Specific heat capacity (J/kg K) | |
| Friction coefficient | |
| Heat transfer coefficient (W/m2 K) | |
| Mass flow ratio of coolant-to-mainstream | |
| Mass flow ratio of coolant-to-mainstream | |
| Mass flow rate of coolant (kg/s) | |
| Mass flow rate of mainstream (kg/s) | |
| Nusselt number | |
| Reynolds number | |
| Temperature ratio of mainstream-to-coolant | |
| Overall temperature of lamilloy (K) | |
| Temperature of coolant (K) | |
| Temperature of mainstream (K) | |
| Temperature ratio of coolant-to-mainstream | |
| Pressure drop (Pa) | |
| Overall cooling effectiveness | |
| Average overall cooling effectiveness | |
| Thermal conductivity of air (W/m K) | |
| Thermal conductivity of 314 (W/m K) | |
| Density of air (kg/) | |
| Dynamic viscosity (Pa·s) | |
| inclination angles () |
References
- Leger, B.; Miron, P.; Emidio, J.M. Geometric and aero-thermal influences on multiholed plate temperature application on combustor wall. Int. J. Heat Mass Transf. 2003, 46, 1215–1222. [Google Scholar] [CrossRef]
- Gustafsson, K.M.B.; Johansson, T.G. An experimental study of surface temperature distribution on effusion-cooled plates. J. Eng. Gas Turbines Power 2001, 123, 308–316. [Google Scholar] [CrossRef]
- Wang, J.; Hu, Z.; Du, C.; Tian, L.; Baleta, J. Numerical study of effusion cooling of a gas turbine combustor liner. Fuel 2021, 294, 120578. [Google Scholar] [CrossRef]
- Bunker, R.S. Film Cooling Effectiveness Due to Discrete Holes Within a Transverse Surface Slo. In Proceedings of the ASME Turbo Expo 2002, Amsterdam, The Netherlands, 3–6 June 2002; Volume 36088, pp. 129–138. [Google Scholar]
- Vishal, V.; Sriraam, J.; Subhash, K.; Kishore, V.R.; Srikrishnan, A.R.; Balajee, R.; Suresh, B. Studies on effusion cooling: Impact of geometric parameters on cooling effectiveness and coolant consumption. Aerosp. Sci. Technol. 2018, 77, 58–66. [Google Scholar]
- Kusterer, K.; Bohn, D.; Sugimoto, T.; Tanaka, R. Double-Jet Ejection of Cooling Air for Improved Film Cooling. J. Turbomach. 2007, 129, 809–815. [Google Scholar] [CrossRef]
- Zhou, J.; Wang, X.; Li, J.; Lu, H. CFD analysis of mist/air film cooling on a flat plate with different hole types. Numer. Heat Transf. Part A Appl. 2017, 71, 1123–1140. [Google Scholar] [CrossRef]
- Kiyici, F.; Topal, A.; Hepkaya, E.; Inanli, S. Numerical Investigation of Gas Turbine Combustor Liner Film Cooling Slots. In Proceedings of the ASME Turbo Expo 2018 Turbomachinery Technical Conference and Exposition GT2018, Oslo, Norway, 11–15 June 2018. [Google Scholar]
- Lee, H.-W.; Park, J.J.; Le, J.S. Flow visualization and film cooling effectiveness measurements around shaped holes with compound angle orientations. Int. J. Heat Mass Transf. 2002, 45, 145–156. [Google Scholar] [CrossRef]
- Zhang, X.D.; Liu, J.J.; An, B.T. The influences of element layout and coolant ejection angle on overall cooling effectiveness of laminated cooling configuration. Int. J. Heat Mass Transf. 2016, 101, 988–991. [Google Scholar] [CrossRef]
- Liu, K. Heat transfer characteristics of triple-stage impingement designs and their application for industrial gas turbine combustor liner cooling. Int. J. Heat Mass Transf. 2021, 172, 121174. [Google Scholar] [CrossRef]
- Yoko, M.J.; Sun, X.; van der Spuy, S.J.; Sethi, V. Multi-fidelity modelling of an impingement/effusion cooled gas turbine combustor liner. Appl. Therm. Eng. 2021, 185, 116318. [Google Scholar] [CrossRef]
- Zhang, J.; Han, H.Z.; Li, Z.R.; Zhong, H.G. Effect of pin-fin forms on flow and cooling characteristics of three-layer porous laminate. Appl. Therm. Eng. 2021, 194, 117084. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, C.; Song, L.; Li, J. Influence of Biot number and geometric parameters on the overall cooling effectiveness of double wall structure with pins. Appl. Therm. Eng. 2021, 198, 117439. [Google Scholar] [CrossRef]
- Zhang, X.D.; Liu, J.J.; An, B.T. The investigations of slot film outflow used on the laminated cooling configuration. Int. J. Heat Mass Transf. 2019, 141, 1078–1086. [Google Scholar] [CrossRef]
- Wang, G.; Niu, D.; Xie, F.; Wang, Y.; Zhao, X.; Ding, G. Experimental and numerical investigation of a microchannel heat sink (MCHS) with micro-scale ribs and grooves for chip cooling. Appl. Therm. Eng. 2015, 85, 61–70. [Google Scholar] [CrossRef]
- Deng, D.; Xie, Y.; Chen, L.; Pi, G.; Huang, Y. Experimental investigation on thermal and combustion performance of a combustor with microchannel cooling. Energy 2019, 181, 954–963. [Google Scholar] [CrossRef]
- Chen, Z.; Feng, Z.; Zhang, Q.; Zhang, J.; Guo, F. Effects of regular triangular prisms on thermal and hydraulic characteristics in a minichannel heat sink. Int. J. Heat Mass Transf. 2022, 188, 122583. [Google Scholar] [CrossRef]
- Moradikazerouni, A.; Afrand, M.; Alsarraf, J.; Mahian, O.; Wongwises, S.; Tran, M.-D. Comparison of the effect of five different entrance channel shapes of a micro-channel heat sink in forced convection with application to cooling a supercomputer circuit board. Appl. Therm. Eng. 2019, 150, 1078–1089. [Google Scholar] [CrossRef]
- You, J.; Feng, H.; Chen, L.; Xie, Z.; Xia, S. Constructal design and experimental validation of a non-uniform heat generating body with rectangular cross-section and parallel circular cooling channels. Int. J. Heat Mass Transf. 2020, 148, 119028. [Google Scholar] [CrossRef]
- Osman, O.S.; El-Zoheiry, R.M.; Elsharnoby, M.; Nada, S.A. Performance enhancement and comprehensive experimental comparative study of cold plate cooling of electronic servers using different configurations of mini-channels flow. Alex. Eng. J. 2021, 60, 4451–4459. [Google Scholar] [CrossRef]
- Kanargi, B.; Lee, P.S.; Yap, C. A numerical and experimental investigation of heat transfer and fluid flow characteristics of an air-cooled oblique-finned heat sink. Int. J. Heat Mass Transf. 2018, 116, 393–416. [Google Scholar] [CrossRef]
- Radwan, A.; Ahmed, M. The influence of microchannel heat sink configurations on the performance of low concentrator photovoltaic systems. Appl. Energy 2017, 206, 594–611. [Google Scholar] [CrossRef]
- Chiu, H.-C.; Jang, J.-H.; Yeh, H.-W.; Wu, M.-S. The heat transfer characteristics of liquid cooling heatsink containing microchannels. Int. J. Heat Mass Transf. 2011, 54, 34–42. [Google Scholar] [CrossRef]
- Yu, C.; Park, J.; Youn, J.R.; Song, Y.S. Integration of form-stable phase change material into pyroelectric energy harvesting system. Appl. Energy 2022, 307, 118212. [Google Scholar] [CrossRef]
- Yang, T.; Sun, Y.; Xu, L.; Yang, X.; Duan, D.; Xi, L.; Gao, J.; Li, Y. Flow and heat transfer characteristics in cooling lamilloy with internal MCs. Int. J. Heat Mass Transf. 2022, 201, 123645. [Google Scholar] [CrossRef]
- Kemerli, U.; Kahveci, K. Conjugate forced convective heat transfer in a sandwich panel with a Kagome truss core: The effects of strut length and diameter. Appl. Therm. Eng. 2020, 167, 114794. [Google Scholar] [CrossRef]
- Sutherland, W. LII. The viscosity of gases and molecular force. Lond. Edinb. Dublin Philos. Mag. J. Sci. 2009, 36, 507–531. [Google Scholar] [CrossRef]
- Li, Y.; Xie, G.; Zhang, Y.; Ferla, P.; Sunden, B. Flow Characteristics and Heat Transfer of Supercritical n-decane in Novel Nested Channels for Scramjet Regenerative Cooling. Int. J. Heat Mass Transf. 2021, 167, 120836. [Google Scholar] [CrossRef]
- Lee, S.M.; Kim, K.Y. Comparative study on performance of a zigzag printed circuit heat exchanger with various channel shapes and configurations. Heat Mass Transf. 2013, 49, 1021–1028. [Google Scholar] [CrossRef]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Wei, W.; Jian, P.; Jianhua, W.; Weilong, W.; Ming, W. An experimental investigation on cooling characteristics of a vane laminated end-wall with axial-row layout of film-holes. Appl. Therm. Eng. 2019, 148, 953–962. [Google Scholar]



| Parameters | Values |
|---|---|
| Coolant inlet temperature | 740 K |
| Coolant inlet velocity | 5 m/s |
| Coolant outlet pressure | 2.3 MPa |
| Mainstream inlet temperature | 1795 K |
| Mainstream inlet velocity | 75 m/s |
| Mainstream outlet pressure | 2.1965 MPa |
| /K | ηave | /K·m−1 | |
|---|---|---|---|
| SF | 1118.415 | 0.641 | 7351.468 |
| PF | 1117.968 | 0.642 | 2529.664 |
| CF | 978.989 | 0.773 | 1698.985 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, T.; Zhang, X.; Chang, Z.; Xu, L.; Xi, L.; Gao, J.; Kou, W.; Jing, X. Study on Flow and Heat Transfer Characteristics in Lamilloy Structure with Different Configurations of Internal Minichannels. Energies 2023, 16, 8058. https://doi.org/10.3390/en16248058
Yang T, Zhang X, Chang Z, Xu L, Xi L, Gao J, Kou W, Jing X. Study on Flow and Heat Transfer Characteristics in Lamilloy Structure with Different Configurations of Internal Minichannels. Energies. 2023; 16(24):8058. https://doi.org/10.3390/en16248058
Chicago/Turabian StyleYang, Tao, Xiaoming Zhang, Zhenyuan Chang, Liang Xu, Lei Xi, Jianmin Gao, Wei Kou, and Xiaochun Jing. 2023. "Study on Flow and Heat Transfer Characteristics in Lamilloy Structure with Different Configurations of Internal Minichannels" Energies 16, no. 24: 8058. https://doi.org/10.3390/en16248058
APA StyleYang, T., Zhang, X., Chang, Z., Xu, L., Xi, L., Gao, J., Kou, W., & Jing, X. (2023). Study on Flow and Heat Transfer Characteristics in Lamilloy Structure with Different Configurations of Internal Minichannels. Energies, 16(24), 8058. https://doi.org/10.3390/en16248058







