Thermodynamic Properties of a Gas–Liquid–Solid System during the CO2 Geological Storage and Utilization Process: A Review
Abstract
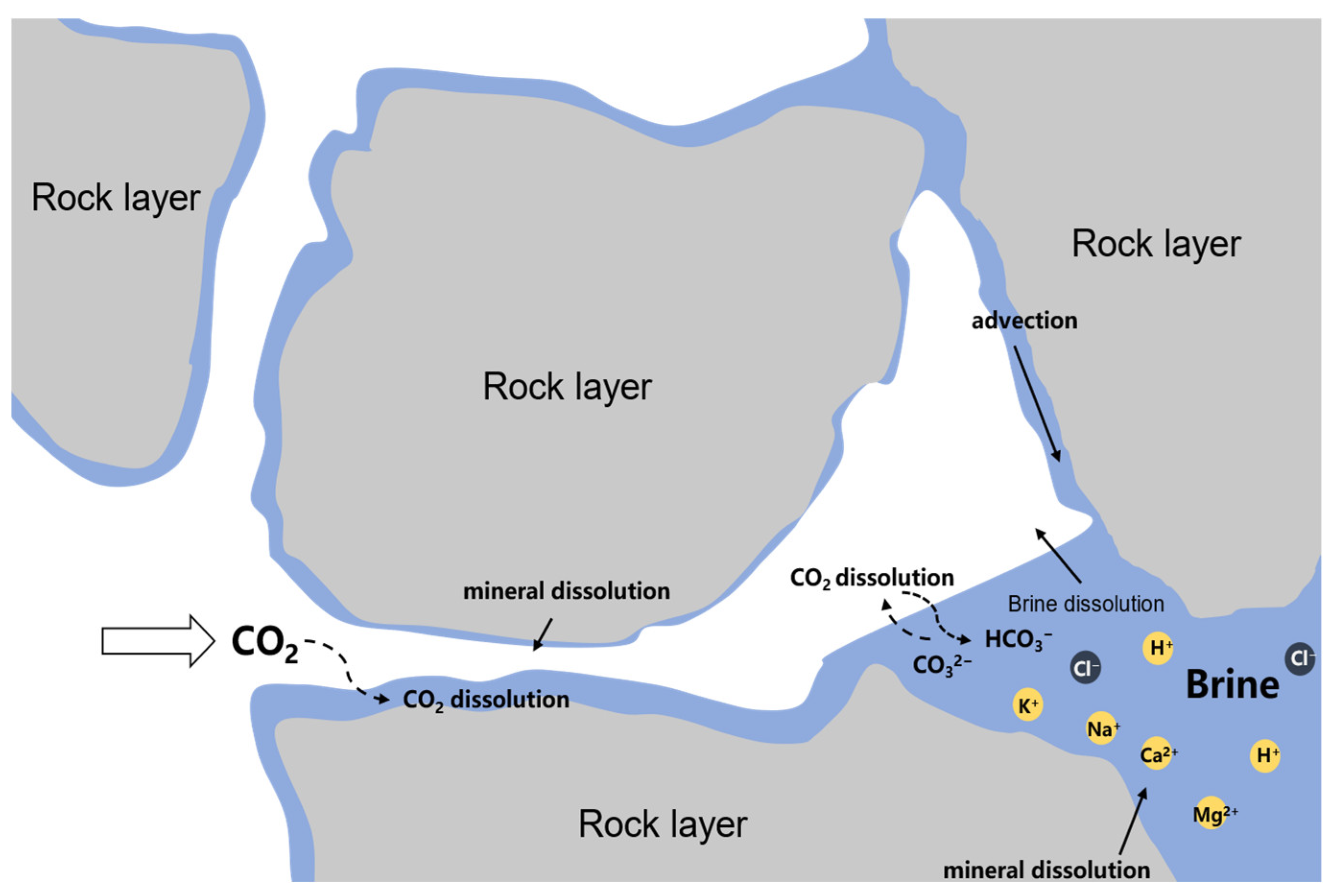
:1. Introduction
2. Thermodynamic Properties
2.1. Interfacial Tension between CO2 and Water/Brine
- Different methods exist to measure the IFT between gases and liquids. Among these, the pendent drop method, which is based on the axisymmetric drop shape analysis (ADSA) technique, is widely used because of its high accuracy and efficiency.
- An accurate measurement of the IFT in CO2 reservoir brines at the evaluated high-temperature and pressure ranges corresponding to actual reservoir conditions relies on the integrity of the experimental apparatus and approach.
- Because of the temperature and pressure limitations of the desired experimental apparatus, a prediction model for the IFT between CO2 and reservoir brines in accordance with the actual reservoir conditions covering a wide range of temperatures, pressures, ionic types, and strengths is essential.
- The results indicate that the IFT of the CO2-brine binary system depends on the temperature, pressure, and molality. However, the pressure has a limited influence on the CO2-brine IFT after the pseudo-plateau has been reached.
2.2. Wettability of the CO2-Water/Brine-Rock System
| Method | Analysis Mode | Resources | Schematic |
|---|---|---|---|
| Sessile drop | Experimental, manual/automatic analysis | Bikkina et al. (2011) [84] Iglauer et al. (2014) [85] Liu et al. (2015) [71] Mutailipu et al. (2019) [32] |  |
| Captive bubble | Experimental, manual/automatic analysis | Chiquet et al. (2007) [86] Broseta et al. (2012) [87] Farokhpoor et al. (2013) [88] Shojai Kaveh et al. (2016) [89] |  |
| Sessile drop/Captive bubble on a titled plate | Experimental, manual/automatic analysis | Saraji et al. (2013) [62] Arif et al. (2016) [73] Arif et al. (2017) [77] |  |
| Capillary meniscus analysis | Experimental, image analysis | Li et al. (2014) [90] Hobeike et al. (2017) [91] Al-Zaidi et al. (2018) [92] |  |
| 2D micromodel | Injected fluid imaged via microscope/analyzed using ImageJ | Chalbaud et al. (2009) [13] Kim et al. (2012) [93] Zhao et al. (2016) [82] |  |
| 3D μ-CT imaging | CT-scan under dynamic condition/image analyzed using an algorithm | Andrew et al. (2013) [94] Lv et al. (2017) [95] Iglauer et al. (2018) [96] |  |
| Molecular dynamics (MD)simulation | High power computing/numerical solution of force field algorithm | Iglauer et al. (2012) [97] Liang et al. (2017) [98] Wang et al. (2022) [99] |  |
- Conventional methods in terms of the contact angle and tomographic imaging measurements have serious limitations.
- The disparity in the CA results in the reported data can be attributed to the differences in the experimental procedures, substrates, roughness, and cleaning procedures used for the substrate surfaces.
- The effects of the ionic strength and ionic type on the CAs of different substrates need to be further investigated to verify the effects of salinity on CAs.
- Alternation in the wettability of the caprock in the presence of supercritical CO2 has been observed, and a molecular dynamics method should be implemented to simulate this phenomenon at the molecular level.
2.3. The Mutual Solubility between CO2 and Water/Brine
| References | Aqueous Phase | m/ (mol·kg−1) | Temperature T/K | Pressure p/MPa |
|---|---|---|---|---|
| Bamberger et al. (2000) [112] | H2O | 0 | 323.2–353.1 | 4–14.2 |
| Servio et al. (2001) [141] | H2O | 0 | 278.05–283.15 | 2–3.7 |
| Anderson et al. (2002) [125] | H2O | 0 | 274.15–288.15 | 0.07–2.2 |
| Kiepe et al. (2002) [129] | H2O | 0 | 313.2–393.17 | 0.09–10 |
| Valtz et al. (2004) [126] | H2O | 0 | 278.2–318.2 | 0–8 |
| Chapoy et al. (2004) [108] | H2O | 0 | 274.14–351.31 | 0.19–7.31 |
| Iwai et al. (2004) [136] | H2O | 0 | 313.2 | 15 |
| Zhang et al. (2005) [142] | H2O | 0 | 304–313 | 1–4.6 |
| Dalmolin et al. (2006) [127] | H2O | 0 | 288–323 | 0.1–0.5 |
| Qin et al. (2008) [143] | H2O | 0 | 323.6–324.1 | 0.03–0.05 |
| Pereira et al. (2009) [102] | H2O | 0 | 298.2–323.2 | 0.05–0.6 |
| Martin et al. (2009) [144] | H2O | 0 | 353–393 | 10–30 |
| Han et al. (2009) [145] | H2O | 0 | 313.2–343.2 | 4.3–17.3 |
| Dell’Era et al. (2010) [146] | H2O | 0 | 298.48–298.63 | 0.2–0.8 |
| Ferrentino et al. (2010) [147] | H2O | 0 | 308.15–323.15 | 5.5–15 |
| Ruffine et al. (2010) [148] | H2O | 0 | 333 | 4.8–11.5 |
| Liu et al. (2011) [149] | H2O | 0 | 308.15–323.15 | 2–16 |
| Tabasinejad et al. (2011) [150] | H2O | 0 | 422.98–478.35 | 3.9–103.4 |
| Schüler et al. (2012) [151] | H2O | 0 | 308–333 | 0.101 |
| Liu et al. (2012) [152] | H2O | 0 | 308.15–318.15 | 8–16 |
| Hou et al. (2013) [17] | H2O | 0 | 298.15–448.15 | 1–18 |
| Serpa et al. (2013) [153] | H2O | 0 | 298–323 | 0.1–0.4 |
| Wang et al. (2013) [154] | H2O | 0 | 313.15–373.15 | 9–17.6 |
| Tong et al. (2013) [155] | H2O | 0 | 374.1–323.2 | 7.2–27.3 |
| Jiang et al. (2014) [156] | H2O | 0 | 333.15–373.15 | 8–18 |
| Bastami et al. (2014) [157] | H2O | 0 | 328.15–375.15 | 6.8–20.7 |
| Al Ghafri et al. (2014) [158] | H2O | 0 | 323.15 | 2–18.7 |
| Krotov et al. (2014) [159] | H2O | 0 | 353.3 | 0.6–9.7 |
| Meyer et al. (2015) [160] | H2O | 0 | 283.15–333.19 | 0–5 |
| Muromachi et al. (2015) [161] | H2O | 0 | 286.15–298.15 | 0.2–4.0 |
| Tang et al. (2015) [128] | H2O | 0 | 308.15–468.15 | 7.9–41 |
| Foltran et al. (2015) [162] | H2O | 0 | 313.15 | 8–12.5 |
| Rumpf et al. (1994) [133] | NaCl | 4–6 | 313–433 | 0.5–9.6 |
| Kiepe et al. (2002) [98,129] | NaCl | 0.5–4.3 | 313–352 | 0.1–10 |
| Bando et al. (2003) [163] | H2O/NaCl | 0–0.55 | 303–333 | 10–20 |
| Kiepe et al. (2002) [98,129] | KCl | 0.5–4.0 | 313–353 | 0.1–10.5 |
| Hou et al. (2013) [17] | NaCl/KCl | 2.5–4.0 | 323–423 | 2.6–18.2 |
| Tong et al. (2013) [155] | Complex brine | 1–5 | 310–425 | 1.2–34.9 |
| Hoballah et al. (2017) [130] | NaHCO3 | 0.8 | 348–398 | 5–50 |
- Empirical equations and estimation models for CO2 + H2O systems have been widely implemented to calculate the solubility of water-saturated CO2; however, they have limitations in estimating the solubility of H2O in compressed CO2.
- There are still too many parameters in the Duan et al. model, and most importantly, it is not intended to compute the solubility of H2O in a compressed CO2 gas phase and does not distinguish between ions of the same charge.
- Although the Krichevsky–Kasarnovsky (KK) approach provides a reasonably good representation of the data, it either fails to fit the data or yields an unphysically negative slope at higher temperatures.
- The well-developed Spycher and Pruess model is widely used to develop a more reliable and simplified model for determining the mutual solubility between CO2 and brines containing different ionic species and of different strengths.
- In the Spycher and Pruess model, different coefficients and parameters have been developed for temperatures above 373 K and below 373 K, such as the Margules expression and equilibrium constant K, which have no thermodynamic meaning. Furthermore, the activity coefficients used to determine the effects of different chloride salts in the brine system were the same. The interaction parameter Kij, based on the EoS and mixing rules, can still be correlated with the modified Peng–Robinson and the mixing rules of Panagiotopoulos and Reid.
2.4. pH of CO2-Saturated Brine
- Very limited research is available for the pH of gas-saturated aqueous solutions under CO2 saline aquifer storage conditions, although it is considered to be one of the most important parameters of reservoir fluids.
- In general, electrometric or optical methods are used to measure the pH of CO2-saturated brine or water. On the basis of the literature review, it can be concluded that the electrometric method is often used to measure the pH of CO2-saturated aqueous solutions covering a wide range of temperatures and pressures (pressures of up to 35 MPa) due to its reliability and stability under reservoir conditions.
- Geochemical simulators based on the Pitzer model offer a convenient route for predicting the pH of complex brines with dissolved CO2. However, additional validation is required for brines other than NaCl (aq).
- The pH and solubility of gas-saturated aqueous solutions can be evaluated via geochemical simulators such as PHREEQC. However, there are limited studies available in the literature to investigate the thermodynamic relations between the pH and solubility of gas-saturated aqueous. The mechanisms of dissolution and acidizing during the CO2 saline aquifer storage process need to be studied systematically, and thermodynamics relations between the pH and solubility of gas-saturated aqueous solutions need to be clarified.
- Given the lack of experimental data, it is important to measure the pH of CO2-saturated brines other than NaCl (aq) over wide ranges of temperatures, pressures, and molality. The range of investigated conditions should include those relevant to CO2 storage in deep-saline aquifers. The results of these experiments were compared with calculations based on the Pitzer model to validate the geochemical simulators based on the Pitzer model.
3. Influence of Interface Properties on the Pore-Scale Multi-Phase Flow
3.1. Core Flooding Experiment and Modelling
3.2. Effects of Wettability
3.3. Effects of Interphase Properties
4. Conclusions
- (1)
- A simplified IFT prediction model with thermodynamic significance and high integrity for predicting IFTs when a pseudo-plateau is reached needs to be developed in future work to cover a wide range of temperatures and pressures corresponding to CO2 geological storage conditions.
- (2)
- Further laboratory and modelling research on the wettability alternations of caprock in terms of the molecular dynamics and simplified equations of state is required, and the thermodynamic relations between interphase properties need to be elaborated in particular.
- (3)
- The main methods for modelling the mutual solubility of liquid or gas phases include EoS, which is usually based on φ-φ or γ-φ equations. Until very recently, limited research has been available on the simultaneous calculation of the mutual solubility of the CO2-rich phase and the H2O-rich phase with high integrity for the application of the CO2 saline aquifer storage scheme. Furthermore, interaction mechanism coupling with multi-factors associated with the gas–liquid–solid interface properties and the dissolution and acidification process need to be explored in future work.
- (4)
- Very limited research is available on the pH of gas-saturated aqueous solutions under CO2 saline aquifer storage conditions. Given the lack of experimental data, it is important to measure the pH of CO2-saturated brines other than NaCl (aq) over wide ranges of temperature, pressure, and molality relevant to CO2 storage in deep-saline aquifers in future work.
- (5)
- Multi-dimensional and multi-scale gas–liquid flow visualization experiments, as well as pore-scale seepage numerical modeling coupling with multi-parameters, need to be carried out. The variation and the interaction mechanism of pore seepage features caused by the physical and chemical interactions of the multi-phase interface properties need to be clarified in future work to provide a theoretical base for and technical guidance on the achievement of the CCUS project with high integrity and efficiency.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Peridas, G.; Mordick Schmidt, B. The role of carbon capture and storage in the race to carbon neutrality. Electr. J. 2021, 34, 106996. [Google Scholar] [CrossRef]
- Zhao, K.; Jia, C.; Li, Z.; Du, X.; Wang, Y.; Li, J.; Yao, Z.; Yao, J. Recent Advances and Future Perspectives in Carbon Capture, Transportation, Utilization, and Storage (CCTUS) Technologies: A Comprehensive Review. Fuel 2023, 351, 128913. [Google Scholar] [CrossRef]
- Golomb, D.; Pennell, S. Ocean sequestration of carbon dioxide (CO2). In Developments and Innovation in Carbon Dioxide (CO2) Capture and Storage Technology; Woodhead Publishing: Sawston, UK, 2010; Volume 2, pp. 304–323. [Google Scholar]
- Bello, A.; Ivanova, A.; Cheremisin, A. A Comprehensive Review of the Role of CO2 Foam EOR in the Reduction of Carbon Footprint in the Petroleum Industry. Energies 2023, 16, 1167. [Google Scholar] [CrossRef]
- Cai, B.F.; Li, Q.; Zhang, X.; Cao, C.; Cao, L.B.; Chen, W.H. China Carbon Dioxide Capture, Utilization, and Storage (CCUS) Annual Report (2021)—Research Path of China CCUS; China 21st Century Agenda Management Center: Beijing, China, 2021. [Google Scholar]
- Cai, B.; Li, Q.; Liu, G.; Liu, L.; Jin, T.; Shi, H. Environmental concern-based site screening of carbon dioxide geological storage in China. Sci. Rep. 2017, 7, 7598. [Google Scholar] [CrossRef] [PubMed]
- Krevor, S.; Blunt, M.J.; Benson, S.M.; Pentland, C.H.; Reynolds, C.; Al-Menhali, A.; Niu, B. Capillary trapping for geologic carbon dioxide storage—From pore scale physics to field scale implications. Int. J. Greenh. Gas Control 2015, 40, 221–237. [Google Scholar] [CrossRef]
- Bradshaw, J.; Bachu, S.; Bonijoly, D.; Burruss, R.; Holloway, S.; Christensen, N.P.; Mathiassen, O.M. CO2 storage capacity estimation: Issues and development of standards. Int. J. Greenh. Gas Control 2007, 1, 62–68. [Google Scholar] [CrossRef]
- Afolayan, B.; Mackay, E.; Opuwari, M. Dynamic modeling of geological carbon storage in an oil reservoir, Bredasdorp Basin, South Africa. Sci. Rep. 2023, 13, 16573. [Google Scholar] [CrossRef]
- Sminchak, J.; Neeraj, G.; Charles, B.; Perry, B. Issues Related to Seismic Activity Induced by the Injection of CO2 in Deep Saline Aquifers; National Energy Technology Laboratory: Pittsburgh, PA, USA; Morgantown, WV, USA, 2001. [Google Scholar]
- Macminn, C.W.; Szulczewski, M.L.; Juanes, R. CO2 migration in saline aquifers. Part 1. Capillary trapping under slope and groundwater flow. J. Fluid Mech. 2010, 662, 329–351. [Google Scholar] [CrossRef]
- Teng, Y.; Jiang, L.; Liu, Y.; Wang, D.; Song, Y. MRI study on CO2 capillary trap and drainage behavior in sandstone cores under geological storage temperature and pressure. Int. J. Heat Mass Transf. 2018, 119, 678–687. [Google Scholar] [CrossRef]
- Chalbaud, C.; Robin, M.; Lombard, J.; Martin, F.; Egermann, P.; Bertin, H. Interfacial tension measurements and wettability evaluation for geological CO2 storage. Adv. Water Resour. 2009, 32, 98–109. [Google Scholar] [CrossRef]
- Teng, Y.; Wang, P.; Jiang, L.; Liu, Y.; Song, Y.; Wei, Y. An experimental study of density-driven convection of fluid pairs with viscosity contrast in porous media. Int. J. Heat Mass Transf. 2020, 152, 119514. [Google Scholar] [CrossRef]
- Irfan, M.F.; Bisson, T.M.; Bobicki, E.; Arguelles-Vivas, F.; Xu, Z.; Liu, Q.; Babadagli, T. CO2 storage in saline aquifers by dissolution and residual trapping under supercritical conditions: An experimental investigation. Colloids Surf. A Physicochem. Eng. Asp. 2018, 548, 37–45. [Google Scholar] [CrossRef]
- Rosenbauer, R.J.; Thomas, B. Carbon dioxide (CO2) sequestration in deep saline aquifers and formations. In Developments and Innovation in Carbon Dioxide (CO2) Capture and Storage Technology; Woodhead Publishing: Sawston, UK, 2010; Volume 2, pp. 57–103. [Google Scholar]
- Hou, S.-X.; Maitland, G.C.; Trusler, J.P.M. Phase equilibria of (CO2 + H2O + NaCl) and (CO2 + H2O + KCl): Measurements and modeling. J. Supercrit. Fluids 2013, 78, 78–88. [Google Scholar] [CrossRef]
- Al-Bazali, T.; Zhang, J.; Chenevert, M.E.; Sharma, M. Measurement of the Sealing Capacity of Shale Caprocks; SPE Annual Technical Conference and Exhibition: Houston, TX, USA, 2005. [Google Scholar] [CrossRef]
- Kaszuba, J.P.; Janecky, D.R.; Snow, M.G. Carbon dioxide reaction processes in a model brine aquifer at 200 °C and 200 bars: Implications for geologic sequestration of carbon. Appl. Geochem. 2003, 18, 1065–1080. [Google Scholar] [CrossRef]
- Druckenmiller, M.L.; Maroto-Valer, M.M. Carbon sequestration using brine of adjusted pH to form mineral carbonates. Fuel Process. Technol. 2005, 86, 1599–1614. [Google Scholar] [CrossRef]
- Morse, J.W.; Arvidson, R.S. The dissolution kinetics of major sedimentary carbonate minerals. Earth Sci. Rev. 2002, 58, 51–84. [Google Scholar] [CrossRef]
- Alkhaldi, M.H.; Nasr-El-Din, H.A.; Sarma, H.K. Kinetics of the Reaction of Citric Acid with Calcite. SPE J. 2010, 15, 704–713. [Google Scholar] [CrossRef]
- Mutailipu, M.; Liu, Y.; Song, Y.; Trusler, J.P.M. The pH of CO2–saturated aqueous KCl solutions at temperatures between 298 K and 423 K at pressures up to 13.5 MPa. Chem. Eng. Sci. 2021, 234, 116434. [Google Scholar] [CrossRef]
- Saadatpoor, E.; Bryant, S.L.; Sepehrnoori, K. New trapping mechanism in carbon sequestration. Transp. Porous Media 2010, 82, 3–17. [Google Scholar] [CrossRef]
- Krevor, S.C.M.; Pini, R.; Li, B.; Benson, S.M. Capillary heterogeneity trapping of CO2 in a sandstone rock at reservoir conditions. Geophys. Res. Lett. 2011, 38, L15401. [Google Scholar] [CrossRef]
- Luhmann, A.J.; Kong, X.Z.; Tutolo, B.M.; Ding, K.; Saar, M.O.; Seyfried, W.E., Jr. Permeability reduction produced by grain reorganization and accumulation of exsolved CO2 during geologic carbon sequestration: A new CO2 trapping mechanism. Environ. Sci. Technol. 2012, 47, 242–251. [Google Scholar] [CrossRef] [PubMed]
- Trusler, J.P.M. Thermophysical Properties and Phase Behavior of Fluids for Application in Carbon Capture and Storage Processes. Annu. Rev. Chem. Biomol. Eng. 2017, 8, 381–402. [Google Scholar] [CrossRef] [PubMed]
- Ebigbo, A.; Class, H.; Helmig, R. CO2 leakage through an abandoned well: Problem-oriented benchmarks. Comput. Geosci. 2007, 11, 103–115. [Google Scholar] [CrossRef]
- Chadwick, A.; Arts, R.J.; Bernstone, C.; May, F.; Thibeau, S.; Zweigel, P. Best practice for the storage of CO2 in saline aquifers. Br. Geol. Surv. Occas. Publ. 2008, 14, 267. [Google Scholar]
- Espinoza, D.N.; Kim, S.H.; Santamarina, J.C. CO2 geological storage—Geotechnical implications. KSCE J. Civ. Eng. 2011, 15, 707–719. [Google Scholar] [CrossRef]
- Amshoff, P.; Weger, T.; Ostertag-Henning, C. Dissolution kinetics of CO2 and CO2-SO2 mixtures in water and brine at geological storage conditions of 16 MPa and 333 K. Int. J. Greenh. Gas Control 2018, 79, 173–180. [Google Scholar] [CrossRef]
- Mutailipu, M.; Liu, Y.; Jiang, L.; Zhang, Y. Measurement and estimation of CO2–brine interfacial tension and rock wettability under CO2 sub- and super-critical conditions. J. Colloid Interface Sci. 2019, 534, 605–617. [Google Scholar] [CrossRef]
- Jho, C.; Nealon, D.; Shogbola, S.; King, A.D. Effect of pressure on the surface tension of water: Adsorption of hydrocarbon gases and carbon dioxide on water at temperatures between 0 and 50 °C. J. Colloid Interface Sci. 1978, 65, 141–154. [Google Scholar] [CrossRef]
- Hebach, A.; Oberhof, A.; Dahmen, N.; Kögel, A.; Ederer, H.; Dinjus, E. Interfacial tension at elevated pressures measurements and correlations in the water + carbon dioxide system. J. Chem. Eng. Data 2002, 47, 1540–1546. [Google Scholar] [CrossRef]
- Kuang, N.; Yang, S.; Yuan, Z.; Wang, M.; Zhang, Z.; Zhang, X.; Wang, M.; Zhang, Y.; Li, S.; Wu, J. Study on oil and gas amphiphilic surfactants promoting the miscibility of CO2 and crude oil. ACS Omega 2021, 6, 27170–27182. [Google Scholar] [CrossRef]
- Chun, B.S.; Wilkinson, G.T. Interfacial Tension in High-Pressure Carbon Dioxide Mixtures. Ind. Eng. Chem. Res. 1995, 34, 4371–4377. [Google Scholar] [CrossRef]
- Akutsu, T.; Yamaji, Y.; Yamaguchi, H.; Watanabe, M.; Smith, R.L., Jr.; Inomata, H. Interfacial tension between water and high pressure CO2 in the presence ofhydrocarbon surfactants. Fluid Phase Equilibria 2007, 157, 163–168. [Google Scholar] [CrossRef]
- Kvamme, B.; Kuznetsova, T.; Hebach, A.; Oberhof, A.; Lunde, E. Measurements and modelling of interfacial tension for water + carbon dioxide systems at elevated pressures. Comput. Mater. Sci. 2007, 38, 506–513. [Google Scholar] [CrossRef]
- Georgiadis, A.; Llovell, F.; Bismarck, A.; Blas, F.J.; Galindo, A.; Maitland, G.C.; Trusler, J.P.M.; Jackson, G. Interfacial tension measurements and modelling of (carbon dioxide + n-alkane) and (carbon dioxide + water) binary mixtures at elevated pressures and temperatures. J. Supercrit. Fluids 2010, 55, 743–754. [Google Scholar] [CrossRef]
- Li, X.S.; Boek, E.; Maitland, G.C.; Trusler, J.P.M. Interfacial Tension of (Brines + CO2): (0.864 NaCl + 0.136 KCl) at Temperatures between (298 and 448) K, Pressures between (2 and 50) MPa, and Total Molalities of (1 to 5) mol.kg(−1). J. Chem. Eng. Data 2012, 57, 1078–1088. [Google Scholar] [CrossRef]
- Aggelopoulos, C.; Robin, M.; Vizika, O. Interfacial tension between CO2 and brine (NaCl + CaCl2) at elevated pressures and temperatures: The additive effect of different salts. Adv. Water Resour. 2011, 34, 505–511. [Google Scholar] [CrossRef]
- Nielsen, L.C.; Bourg, I.C.; Sposito, G. Predicting CO2-water interfacial tension under pressure and temperature conditions of geologic CO2 storage. Geochim. Cosmochim. Acta 2012, 81, 28–38. [Google Scholar] [CrossRef]
- da Rocha, S.R.P.; Johnston, K.P.; Westacott, R.E.; Rossky, P.J. Molecular structure of the water-supercritical CO2 interface. J. Phys. Chem. B 2001, 105, 12092–12104. [Google Scholar] [CrossRef]
- Kuznetsova, T.; Kvamme, B. Thermodynamic properties and interfacial tension of a model water-carbon dioxide system. Phys. Chem. Chem. Phys. 2002, 4, 937–941. [Google Scholar] [CrossRef]
- Li, Z.; Wang, S.; Li, S.; Liu, W.; Li, B.; Lv, Q.-C. Accurate Determination of the CO2−Brine Interfacial Tension Using Graphical Alternating Conditional Expectation. Energy Fuels 2014, 28, 624–635. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Feng, Q.H.; Wang, S.H.; Zhang, X.M.; Wang, S.L. Estimation of CO2-brine interfacial tension using an artificial neural network. J. Supercrit. Fluids 2016, 107, 31–37. [Google Scholar] [CrossRef]
- Partovi, M.; Mosalanezhad, M.; Lotfi, S.; Barati-Harooni, A.; Najafi-Marghmaleki, A.; Mohammadi, A.H. On the estimation of CO2-brine interfacial tension. J. Mol. Liq. 2017, 243, 265–272. [Google Scholar] [CrossRef]
- Liu, X.; Mutailipu, M.; Zhao, J.; Liu, Y. Comparative Analysis of Four Neural Network Models on the Estimation of CO2–Brine Interfacial Tension. ACS Omega 2021, 6, 4282–4288. [Google Scholar] [CrossRef] [PubMed]
- Song, Y.; Sung, W.; Jang, Y.; Jung, W. Application of an artificial neural network in predicting the effectiveness of trapping mechanisms on CO2 sequestration in saline aquifers. Int. J. Greenh. Gas Control 2020, 98, 103042. [Google Scholar] [CrossRef]
- Chow, Y.T.F.; Eriksen, D.K.; Galindo, A.; Haslam, A.J.; Jackson, G.; Maitland, G.C.; Trusler, J.P.M. Interfacial tensions of systems comprising water, carbon dioxide and diluent gases at high pressures: Experimental measurements and modelling with SAFT-VR Mie and square-gradient theory. Fluid Phase Equilibria 2016, 407, 159–176. [Google Scholar] [CrossRef]
- Chapman, W.G.; Gubbins, K.E.; Jackson, G.; Radosz, M. SAFT: Equation-of-state solution model for associating fluids. Fluid Phase Equilibria 1989, 52, 31–38. [Google Scholar] [CrossRef]
- Walter, G.; Chapman, K.E.G.; Jackson, G.; Radosz, M. New Reference Equation of State for Associating Liquids. Ind. Eng. Chem. Res. 1990, 29, 1709–1721. [Google Scholar]
- Müller, E.A.; Gubbins, K.E. Molecular-Based Equations of State for Associating Fluids: A Review of SAFT and Related Approaches. Ind. Eng. Chem. Res. 2001, 40, 2193–2211. [Google Scholar] [CrossRef]
- Economou, I.G. Statistical Associating Fluid Theory: A Successful Model for the Calculation of Thermodynamic and Phase Equilibrium Properties of Complex Fluid Mixtures. Ind. Eng. Chem. Res. 2002, 41, 953–962. [Google Scholar] [CrossRef]
- Paricaud, P.; Galindo, A.; Jackson, G. Recent advances in the use of the SAFT approach in describing electrolytes, interfaces, liquid crystals and polymers. Fluid Phase Equilibria 2002, 194–197, 87–96. [Google Scholar] [CrossRef]
- Tan, S.P.; Adidharma, H.; Radosz, M. Recent Advances and Applications of Statistical Associating Fluid Theory. Ind. Eng. Chem. Res. 2008, 47, 8063–8082. [Google Scholar] [CrossRef]
- McCabe, C.; Galindo, A. Chapter 8 SAFT Associating Fluids and Fluid Mixtures. In Applied Thermodynamics of Fluids; The Royal Society of Chemistry: London, UK, 2010; pp. 215–279. [Google Scholar] [CrossRef]
- Jerauld, G.R.; Korrani, A.K.N. Revised Correlation for Accurate Estimation of CO2-Brine Interfacial Tension at Reservoir Conditions. In Proceedings of the SPE Improved Oil Recovery Conference, Virtual, 25–29 April 2022. [Google Scholar] [CrossRef]
- Kashefi, K. Measurement and Modelling of Interfacial Tension and Viscosity of Reservoir Fluids; Heriot-Watt University: Edinburgh, UK, 2012. [Google Scholar]
- Chiquet, P.; Daridon, J.-L.; Broseta, D.; Thibeau, S. CO2/water interfacial tensions under pressure and temperature conditions of CO2 geological storage. Energy Convers. Manag. 2007, 48, 736–744. [Google Scholar] [CrossRef]
- Bikkina, P.K.; Shoham, O.; Uppaluri, R. Equilibrated Interfacial Tension Data of the CO2–Water System at High Pressures and Moderate Temperatures. J. Chem. Eng. Data 2011, 56, 3725–3733. [Google Scholar] [CrossRef]
- Saraji, S.; Goual, L.; Piri, M.; Plancher, H. Wettability of Supercritical Carbon Dioxide/Water/Quartz Systems: Simultaneous Measurement of Contact Angle and Interfacial Tension at Reservoir Conditions. Langmuir 2013, 29, 6856–6866. [Google Scholar] [CrossRef]
- Khosharay, S.; Varaminian, F. Experimental and modeling investigation on surface tension and surface properties of (CH4 + H2O), (C2H6 + H2O), (CO2 + H2O) and (C3H8 + H2O) from 284.15 K to 312.15 K and pressures up to 60 bar. Int. J. Refrig. 2014, 47, 26–35. [Google Scholar] [CrossRef]
- Pereira, L.M.C.; Chapoy, A.; Burgass, R.; Oliveira, M.B.; Coutinho, J.A.P.; Tohidi, B. Study of the impact of high temperatures and pressures on the equilibrium densities and interfacial tension of the carbon dioxide/water system. J. Chem. Thermodyn. 2016, 93, 404–415. [Google Scholar] [CrossRef]
- Liu, Y.; Li, H.A.; Okuno, R. Measurements and Modeling of Interfacial Tension for CO2/CH4/Brine Systems under Reservoir Conditions. Ind. Eng. Chem. Res. 2016, 55, 12358–12375. [Google Scholar] [CrossRef]
- Sutjiadi-Sia, Y.; Jaeger, P.; Eggers, R. Interfacial phenomena of aqueous systems in dense carbon dioxide. J. Supercrit. Fluids 2008, 46, 272–279. [Google Scholar] [CrossRef]
- Chow, Y.T.F.; Maitland, G.C.; Trusler, J.P.M. Martin Interfacial tensions of the (CO2 + N2 + H2O) system at temperatures of (298 to 448) K and pressures up to 40 MPa. J. Chem. Thermodyn. 2016, 93, 392–403. [Google Scholar] [CrossRef]
- Yang, D.; Tontiwachwuthikul, P.; Gu, Y. Interfacial Interactions between Reservoir Brine and CO2 at High Pressures and Elevated Temperatures. Energy Fuels 2005, 19, 216. [Google Scholar] [CrossRef]
- Chalbaud, C.A.; Robin, M.; Egermann, P. Interfacial tension data and correlations of brine-CO2 systems under reservoir conditions. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 24–27 September 2006; Society of Petroleum Engineers: Houston, TX, USA, 2006. [Google Scholar]
- Bachu, S.; Bennion, D.B. Interfacial Tension between CO2, Freshwater, and Brine in the Range of Pressure from (2 to 27) MPa, Temperature from (20 to 125) degrees C, and Water Salinity from (0 to 334 000) mg·L−1. J. Chem. Eng. Data 2009, 54, 765–775. [Google Scholar] [CrossRef]
- Liu, Y.; Mutailipu, M.; Jiang, L.L.; Zhao, J.F.; Song, Y.C.; Chen, L.Y. Interfacial tension and contact angle measurements for the evaluation of CO2-brine two-phase flow characteristics in porous media. Environ. Prog. Sustain. Energy 2015, 34, 1756–1762. [Google Scholar] [CrossRef]
- Liu, Y.; Tang, J.; Wang, M.; Wang, Q.; Tong, J.; Zhao, J.; Song, Y. Measurement of interfacial tension of CO2 and NaCl aqueous solution over wide temperature, pressure, and salinity ranges. J. Chem. Eng. Data 2017, 62, 1036–1046. [Google Scholar] [CrossRef]
- Arif, M.; Al-Yaseri, A.Z.; Barifcani, A.; Lebedev, M.; Iglauer, S. Impact of pressure and temperature on CO2-brine-mica contact angles and CO2-brine interfacial tension: Implications for carbon geo-sequestration. J. Colloid Interface Sci. 2016, 462, 208–215. [Google Scholar] [CrossRef] [PubMed]
- Pereira, L.M.C.; Chapoy, A.; Burgass, R.; Tohidi, B. Interfacial tension of CO2 + brine systems: Experiments and predictive modelling. Adv. Water Resour. 2017, 103, 64–75. [Google Scholar] [CrossRef]
- Yekeen, N.; Padmanabhan, E.; Abdulelah, H.; Irfan, S.A.; Okunade, O.A.; Khan, J.A.; Negash, B.M. CO2/brine interfacial tension and rock wettability at reservoir conditions: A critical review of previous studies and case study of black shale from Malaysian formation. J. Pet. Sci. Eng. 2021, 196, 107673. [Google Scholar] [CrossRef]
- Aggelopoulos, C.A.; Robin, M.; Perfetti, E.; Vizika, O. CO2/CaCl2 solution interfacial tensions under CO2 geological storage conditions: Influence of cation valence on interfacial tension. Adv. Water Resour. 2010, 33, 691–697. [Google Scholar] [CrossRef]
- Arif, M.; Jones, F.; Barifcani, A.; Iglauer, S. Electrochemical investigation of the effect of temperature, salinity and salt type on brine/mineral interfacial properties. Int. J. Greenh. Gas Control 2017, 59, 136–147. [Google Scholar] [CrossRef]
- Wang, H.; Luo, M.; Lun, Z.; Lv, C.; Zhao, C.H.; Zhao, Q.-N.; Lang, D. Determination and Comparison of Interfacial Interactions between CO2, Crude Oil, and Brine at Reservoir Conditions. Energy Fuels 2018, 32, 8187–8192. [Google Scholar] [CrossRef]
- Amooie, M.A.; Hemmati-Sarapardeh, A.; Karan, K.; Husein, M.M.; Soltanian, M.R.; Dabir, B. Data-driven modeling of interfacial tension in impure CO2-brine systems with implications for geological carbon storage. Int. J. Greenh. Gas Control 2019, 90, 102811. [Google Scholar] [CrossRef]
- Mutailipu, M. Gas-Liquid-Solid Interface Properties and Their Effect on the Seepage Characteristics under CO2 Geological Storage Conditions. Ph.D. Thesis, Dalian University of Technology, Dalian, China, 2020. [Google Scholar]
- Blunt, M.J. (Ed.) Multiphase Flow in Permeable Media: A Pore-Scale Perspective. In Multiphase Flow in Permeable Media: A Pore-Scale Perspective; Cambridge University Press: Cambridge, UK, 2017; pp. i–iv. [Google Scholar]
- Zhao, B.; MacMinn, C.W.; Juanes, R. Wettability control on multiphase flow in patterned microfluidics. Proc. Natl. Acad. Sci. USA 2016, 113, 10251–10256. [Google Scholar] [CrossRef] [PubMed]
- Baban, A.; Al-Yaseri, A.; Keshavarz, A.; Amin, R.; Iglauer, S. CO2–brine–sandstone wettability evaluation at reservoir conditions via Nuclear Magnetic Resonance measurements. Int. J. Greenh. Gas Control 2021, 111, 103435. [Google Scholar] [CrossRef]
- Bikkina, P.K. Contact angle measurements of CO2–water–quartz/calcite systems in the perspective of carbon sequestration. Int. J. Greenh. Gas Control 2011, 5, 1259–1271. [Google Scholar] [CrossRef]
- Iglauer, S.; Salamah, A.; Sarmadivaleh, M.; Liu, K.; Phan, C. Contamination of silica surfaces: Impact on water–CO2–quartz and glass contact angle measurements. Int. J. Greenh. Gas Control 2014, 22, 325–328. [Google Scholar] [CrossRef]
- Chiquet, P.; Broseta, D.; Thibeau, S. Wettability alteration of caprock minerals by carbon dioxide. Geofluids 2007, 7, 112–122. [Google Scholar] [CrossRef]
- Broseta, D.; Tonnet, N.; Shah, V. Are rocks still water-wet in the presence of dense CO2 or H2S? Geofluids 2012, 12, 280–294. [Google Scholar] [CrossRef]
- Farokhpoor, R.; Bjørkvik, B.J.A.; Lindeberg, E.; Torsæter, O. Wettability behaviour of CO2 at storage conditions. Int. J. Greenh. Gas Control 2013, 12, 18–25. [Google Scholar] [CrossRef]
- Shojai Kaveh, N.; Barnhoorn, A.; Wolf, K.H. Wettability evaluation of silty shale caprocks for CO2 storage. Int. J. Greenh. Gas Control 2016, 49, 425–435. [Google Scholar] [CrossRef]
- Li, X.; Fan, X.; Brandani, S. Difference in pore contact angle and the contact angle measured on a flat surface and in an open space. Chem. Eng. Sci. 2014, 117, 137–145. [Google Scholar] [CrossRef]
- Hobeika, N.; Bouriat, P.; Touil, A.; Broseta, D.; Brown, R.; Dubessy, J. Help from a Hindrance: Using Astigmatism in Round Capillaries to Study Contact Angles and Wetting Layers. Langmuir 2017, 33, 5179–5187. [Google Scholar] [CrossRef]
- Al-Zaidi, E.; Fan, X. Effect of aqueous electrolyte concentration and valency on contact angle on flat glass surfaces and inside capillary glass tubes. Colloids Surf. A Physicochem. Eng. Asp. 2018, 543, 1–8. [Google Scholar] [CrossRef]
- Kim, Y.; Wan, J.; Kneafsey, T.J.; Tokunaga, T.K. Dewetting of Silica Surfaces upon Reactions with Supercritical CO2 and Brine: Pore-Scale Studies in Micromodels. Environ. Sci. Technol. 2012, 46, 4228–4235. [Google Scholar] [CrossRef] [PubMed]
- Andrew, M.; Bijeljic, B.; Blunt, M.J. Pore-scale imaging of geological carbon dioxide storage under in situ conditions. Geophys. Res. Lett. 2013, 40, 3915–3918. [Google Scholar] [CrossRef]
- Lv, P.; Liu, Y.; Wang, Z.; Liu, S.; Jiang, L.; Chen, J.; Song, Y. In Situ Local Contact Angle Measurement in a CO2–Brine–Sand System Using Microfocused X-ray CT. Langmuir 2017, 33, 3358–3366. [Google Scholar] [CrossRef] [PubMed]
- Iglauer, S.; Lebedev, M. High pressure-elevated temperature X-ray micro-computed tomography for subsurface applications. Adv. Colloid Interface Sci. 2018, 256, 393–410. [Google Scholar] [CrossRef]
- Iglauer, S.; Mathew, M.S.; Bresme, F. Molecular dynamics computations of brine–CO2 interfacial tensions and brine–CO2–quartz contact angles and their effects on structural and residual trapping mechanisms in carbon geo-sequestration. J. Colloid Interface Sci. 2012, 386, 405–414. [Google Scholar] [CrossRef]
- Liang, Y.; Tsuji, S.; Jia, J.; Tsuji, T.; Matsuoka, T. Modeling CO2-Water-Mineral Wettability and Mineralization for Carbon Geosequestration. Acc. Chem. Res. 2017, 50, 1530–1540. [Google Scholar] [CrossRef]
- Wang, Z.; Yu, C.; Zhao, J.; Guo, P.; Liu, H. Molecular dynamics simulation for quantitative characterization of wettability transition on silica surface. J. Mater. Res. Technol. 2022, 19, 4371–4380. [Google Scholar] [CrossRef]
- Arif, M.; Abu-Khamsin, S.A.; Iglauer, S. Wettability of rock/CO2/brine and rock/oil/CO2-enriched-brine systems: Critical parametric analysis and future outlook. Adv. Colloid Interface Sci. 2019, 268, 91–113. [Google Scholar] [CrossRef]
- Lv, P.; Liu, Y.; Jiang, L.; Song, Y.; Wu, B.; Zhao, J.; Zhang, Y. Experimental determination of wettability and heterogeneity effect on CO2 distribution in porous media. Greenh. Gases Sci. Technol. 2016, 6, 401–415. [Google Scholar] [CrossRef]
- Siqueira Campos, C.E.P.; Villardi, H.G.D.A.; Pessoa, F.L.P.; Uller, A.M.C. Solubility of Carbon Dioxide in Water and Hexadecane: Experimental Measurement and Thermodynamic Modeling. J. Chem. Eng. Data 2009, 54, 2881–2886. [Google Scholar] [CrossRef]
- Scott, R.; Konynenburg, P.V. Static properties of solutions. Van der Waals and related models for hydrocarbon mixtures. Discuss. Faraday Soc. 1970, 49, 10. [Google Scholar] [CrossRef]
- Crovetto, R. Evaluation of Solubility Data of the System CO2-H2O from 273 K to the Critical Point of Water. J. Phys. Chem. Ref. Data 1991, 20, 575–589. [Google Scholar] [CrossRef]
- Carroll, J.; Slupsky, J.D.; Mather, A.E. The Solubility of Carbon Dioxide in Water at Low Pressure. J. Phys. Chem. Ref. Data 1991, 20, 1201–1209. [Google Scholar] [CrossRef]
- Sun, X.; Wang, Z.; Li, H.; He, H.; Sun, B. A simple model for the prediction of mutual solubility in CO2-brine system at geological conditions. Desalination 2021, 504, 114972. [Google Scholar] [CrossRef]
- Diamond, L.W.; Akinfiev, N.N. Solubility of CO2 in water from −1.5 to 100 °C and from 0.1 to 100 MPa: Evaluation of literature data and thermodynamic modelling. Fluid Phase Equilibria 2003, 208, 265–290. [Google Scholar] [CrossRef]
- Chapoy, A.; Mohammadi, A.H.; Chareton, A.; Tohidi, B.; Richon, D. Measurement and Modeling of Gas Solubility and Literature Review of the Properties for the Carbon Dioxide—Water System. Ind. Eng. Chem. Res. 2004, 43, 1794–1802. [Google Scholar] [CrossRef]
- King, M.B.; Mubarak, A.; Kim, J.D.; Bott, T.R. The mutual solubilities of water with supercritical and liquid carbon dioxides. J. Supercrit. Fluids 1992, 5, 296–302. [Google Scholar] [CrossRef]
- Shyu, G.; Hanif, N.S.M.; Hall, K.; Eubank, P.T. Carbon dioxide-water phase equilibria results from the Wong-San dler combining rules. Fluid Phase Equilibria 1997, 130, 73–85. [Google Scholar] [CrossRef]
- Duan, Z.; Møller, N.; Weare, J.H. An equation of state for the CH4-CO2-H2O system: II. Mixtures from 50 to 1000 °C and 0 to 1000 bars. Geochim. Cosmochim. Acta 1992, 56, 2619–2631. [Google Scholar] [CrossRef]
- Bamberger, A.; Sieder, G.; Maurer, G. High-pressure (vapor + liquid) equilibrium in binary mixtures of (carbon dioxide + water or acetic acid) at temperatures from 313 to 353 K. J. Supercrit. Fluids 2000, 17, 97–100. [Google Scholar] [CrossRef]
- Hu, J.; Duan, Z.; Zhu, C.; Chou, I.-M. PVTx properties of the CO2-H2O and CO2-H2O-NaCl systems below 647 K: Assessment of experimental data and thermodynamic models. Chem. Geol. 2007, 238, 249–267. [Google Scholar] [CrossRef]
- Coan, C.R.; King, A.D. Solubility of water in compressed carbon dioxide, nitrous oxide, and ethane: Evidence for hydration of carbon dioxide and nitrous oxide in the gas phase. J. Am. Chem. Soc. 1971, 93, 1857–1862. [Google Scholar]
- Carroll, J.J.; Mather, A.E. The system carbon dioxide-water and the Krichevsky-Kasarnovsky equation. J. Solut. Chem. 1992, 21, 607–621. [Google Scholar] [CrossRef]
- Orbey, H.; Sandler, S.I. Cubic Equations of State and Their Mixing Rules; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Bermejo, M.D.; Martín, A.; Florusse, L.J.; Peters, C.J.; Cocero, M.J. The influence of Na2SO4 on the CO2 solubility in water at high pressure. Fluid Phase Equilibria 2005, 238, 220–228. [Google Scholar] [CrossRef]
- Duan, Z.; Møller, N.; Weare, H. Equation of state for the NaCl-H2O-CO2 system: Prediction of phase equilibria and volu metric properties. Geochem. Cosmochem. Acta 1995, 59, 2869–2882. [Google Scholar] [CrossRef]
- Duan, Z.; Sun, R. An improved model calculating CO2 solubility in pure water and aqueous NaCl solutions from 273 to 533 K and from 0 to 2000 bar. Chem. Geol. 2003, 193, 257–271. [Google Scholar] [CrossRef]
- Yan, Y.Z.; Chen, C.C. Thermodynamic modeling of CO2 solubility in aqueous solutions of NaCl and Na2SO4. J. Supercrit. Fluids 2010, 55, 623–634. [Google Scholar] [CrossRef]
- Springer, R.D.; Wang, Z.; Anderko, A.; Wang, P.; Felmy, A.R. A thermodynamic model for predicting mineral reactivity in supercritical carbon dioxide: I. Phase behavior of carbon dioxide–water–chloride salt systems across the H2O-rich to the CO2-rich regions. Chem. Geol. 2012, 322/323, 151–171. [Google Scholar] [CrossRef]
- Duan, Z.; Sun, R.; Zhu, C.; Chou, I.M. An improved model for the calculation of CO2 solubility in aqueous solutions containing Na+, K+, Ca2+, Mg2+, Cl−, and SO42−. Mar. Chem. 2006, 98, 131–139. [Google Scholar] [CrossRef]
- Ratnakar, R.R.; Venkatraman, A.; Kalra, A.; Dindoruk, B. On the prediction of gas solubility in brine solutions with single or mixed salts: Applications to gas injection and CO2 capture/sequestration. J. Nat. Gas Sci. Eng. 2020, 81, 103450. [Google Scholar] [CrossRef]
- Zhao, H.; Dilmore, R.; Allen, D.E.; Hedges, S.W.; Soong, Y.; Lvov, S.N. Measurement and modeling of CO2 solubility in natural and synthetic formation brines for CO2 sequestration. Environ. Sci. Technol. 2015, 49, 1972–1980. [Google Scholar] [CrossRef] [PubMed]
- Anderson, G.K. Solubility of Carbon Dioxide in Water under Incipient Clathrate Formation Conditions. J. Chem. Eng. Data 2002, 47, 219–222. [Google Scholar] [CrossRef]
- Valtz, A.; Chapoy, A.; Coquelet, C.; Paricaud, P.; Richon, D. Vapour–liquid equilibria in the carbon dioxide–water system, measurement and modelling from 278.2 to 318.2 K. Fluid Phase Equilibria 2004, 226, 333–344. [Google Scholar] [CrossRef]
- Dalmolin, I.; Skovroinski, E.; Biasi, A.; Corazza, M.L.; Dariva, C.; Oliveira, J.V. Solubility of carbon dioxide in binary and ternary mixtures with ethanol and water. Fluid Phase Equilibria 2006, 245, 193–200. [Google Scholar] [CrossRef]
- Tang, Y.; Bian, X.; Du, Z.; Wang, C. Measurement and prediction model of carbon dioxide solubility in aqueous solutions containing bicarbonate anion. Fluid Phase Equilibria 2015, 386, 56–64. [Google Scholar] [CrossRef]
- Kiepe, J.; Horstmann, S.; Fischer, K.; Gmehling, J. Experimental determination and prediction of gas solubility data for CO2 + H2O mixtures containing NaCl or KCl at temperatures between 313 and 393 K and pressures up to 10 MPa. Ind. Eng. Chem. Res. 2002, 41, 4393–4398. [Google Scholar] [CrossRef]
- Hoballah, R. On the Solubility of Acid and Sour Gases in Water and Brines under Reservoir Conditions. Ph.D. Thesis, Imperial College London, London, UK, 2017. [Google Scholar]
- Spycher, N.; Pruess, K.; Ennis-King, J. CO2-H2O mixtures in the geological sequestration of CO2. I. Assessment and calculation of mutual solubilities from 12 to 100 °C and up to 600 bar. Geochim. Cosmochim. Acta 2003, 67, 3015–3031. [Google Scholar] [CrossRef]
- Spycher, N.; Pruess, K. CO2-H2O mixtures in the geological sequestration of CO2. II. Partitioning in chloride brines at 12–100 °C and up to 600 bar. Geochim. Cosmochim. Acta 2005, 69, 3309–3320. [Google Scholar] [CrossRef]
- Rumpf, B.; Nicolaisen, H.; Öcal, C.; Maurer, G. Solubility of carbon dioxide in aqueous solutions of sodium chloride: Experimental results and correlation. J. Solut. Chem. 1994, 23, 431–448. [Google Scholar] [CrossRef]
- Spycher, N.; Pruess, K. A phase-partitioning model for CO2–brine mixtures at elevated temperatures and pressures: Application to CO2-enhanced geothermal systems. Transp. Porous Media 2010, 82, 173–196. [Google Scholar] [CrossRef]
- Redlich, O.; Kwong, J.N.S. On the Thermodynamics of Solutions. V. An Equation of State. Fugacities of Gaseous Solutions. Chem. Rev. 1949, 44, 233–244. [Google Scholar] [CrossRef]
- Iwai, Y.; Uno, M.; Nagano, H.; Arai, Y. Measurement of solubilities of palmitic acid in supercritical carbon dioxide and entrainer effect of water by FTIR spectroscopy. J. Supercrit. Fluids 2004, 28, 193–200. [Google Scholar] [CrossRef]
- Peng, D.Y.; Robinson, D.B. A New Two-Constant Equation of State. Ind. Eng. Chem. Res. 1976, 15, 59–64. [Google Scholar] [CrossRef]
- Valderrama, J.O. The state of the cubic equations of state. Ind. Eng. Chem. Res. 2003, 42, 1603–1618. [Google Scholar] [CrossRef]
- Assael, M.J.; Trusler, J.P.M.; Tsolakis, T.F. Thermophysical Properties of Fluids: An Introduction to Their Prediction; Imperial College Press: London, UK, 1996. [Google Scholar]
- Panagiotopoulos, A.Z.; Reid, R.C. New Mixing Rules for Cubic Equations of State for Highly Polar Asymmetric Mixtures; ACS Symposium Series; American Chemical Society: Washington, DC, USA, 1986; pp. 571–582. [Google Scholar] [CrossRef]
- Servio, P.; Englezos, P. Effect of temperature and pressure on the solubility of carbon dioxide in water in the presence of gas hydrate. Fluid Phase Equilibria 2001, 190, 127–134. [Google Scholar] [CrossRef]
- Zhang, G.; Wu, Y.; Ma, P.; Wu, G.; Li, D. Measurement and correlation of solubility of carbon monoxide and other gases solubility in phenol. Huagong Xuebao J. Chem. Ind. Eng. 2005, 56, 2039–2045. [Google Scholar]
- Qin, J.; Rosenbauer, R.J.; Duan, Z. Experimental measurements of vapor–liquid equilibria of the H2O + CO2 + CH4 ternary system. J. Chem. Eng. Data 2008, 53, 1246–1249. [Google Scholar] [CrossRef]
- Martín, Á.; Pham, H.M.; Kilzer, A.; Kareth, S.; Weidner, E. Phase equilibria of carbon dioxide + poly ethylene glycol + water mixtures at high pressure: Measurements and modelling. Fluid Phase Equilibria 2009, 286, 162–169. [Google Scholar] [CrossRef]
- Han, J.M.; Shin, H.Y.; Min, B.-M.; Han, K.-H.; Cho, A. Measurement and correlation of high pressure phase behavior of carbon dioxide + water system. J. Ind. Eng. Chem. 2009, 15, 212–216. [Google Scholar] [CrossRef]
- Dell’Era, C.; Uusi-Kyyny, P.; Pokki, J.-P.; Pakkanen, M.; Alopaeus, V. Solubility of carbon dioxide in aqueous solutions of diisopropanolamine and methyldiethanolamine. Fluid Phase Equilibria 2010, 293, 101–109. [Google Scholar] [CrossRef]
- Ferrentino, G.; Barletta, D.; Balaban, M.O.; Ferrari, G.; Poletto, M. Measurement and prediction of CO2 solubility in sodium phosphate monobasic solutions for food treatment with high pressure carbon dioxide. J. Supercrit. Fluids 2010, 52, 142–150. [Google Scholar] [CrossRef]
- Ruffine, L.; Trusler, J.P.M. Phase behaviour of mixed-gas hydrate systems containing carbon dioxide. J. Chem. Thermodyn. 2010, 42, 605–611. [Google Scholar] [CrossRef]
- Liu, Y.; Hou, M.; Yang, G.; Han, B. Solubility of CO2 in aqueous solutions of NaCl, KCl, CaCl2 and their mixed salts at different temperatures and pressures. J. Supercrit. Fluids 2011, 56, 125–129. [Google Scholar] [CrossRef]
- Tabasinejad, F.; Moore, R.G.; Mehta, S.A.; Fraassen, K.C.V.; Barzin, Y.; Rushing, J.A.; Newsham, K.E. Water Solubility in Supercritical Methane, Nitrogen, and Carbon Dioxide: Measurement and Modeling from 422 to 483 K and Pressures from 3.6 to 134 MPa. Ind. Eng. Chem. Res. 2011, 50, 4029–4041. [Google Scholar] [CrossRef]
- Schüler, N.; Hecht, K.; Kraut, M.; Dittmeyer, R. On the Solubility of Carbon Dioxide in Binary Water–Methanol Mixtures. J. Chem. Eng. Data 2012, 57, 2304–2308. [Google Scholar] [CrossRef]
- Liu, Y.; Hou, M.; Ning, H.; Yang, D.; Yang, G.; Han, B. Phase equilibria of CO2 + N2 + H2O and N2 + CO2 + H2O + NaCl + KCl + CaCl2 systems at different temperatures and pressures. J. Chem. Eng. Data 2012, 57, 1928–1932. [Google Scholar] [CrossRef]
- Serpa, F.S.; Vidal, R.S.; Filho, J.H.B.A.; Nascimento, J.F.D.; Ciambelli, J.R.P.; Figueiredo, C.M.S.; Salazar-Banda, G.R.; Santos, A.F.; Fortuny, M.; Franceschi, E.; et al. Solubility of carbon dioxide in ethane-1,2-diol–water mixtures. J. Chem. Eng. Data 2013, 58, 3464–3469. [Google Scholar] [CrossRef]
- Wang, Z.; Felmy, A.R.; Thompson, C.J.; Loring, J.S.; Joly, A.G.; Rosso, K.M.; Schaef, H.T.; Dixon, D.A. Near-infrared spectroscopic investigation of water in supercritical CO2 and the effect of CaCl2. Fluid Phase Equilibria 2013, 338, 155–163. [Google Scholar] [CrossRef]
- Tong, D.; Trusler, M.; Vega-Maza, D. Solubility of CO2 in aqueous solutions of CaCl2 or MgCl2 and in a synthetic formation brine at temperatures up to 423 K and pressures up to 40 MPa. J. Chem. Eng. Data 2013, 58, 2116–2124. [Google Scholar] [CrossRef]
- Jiang, C.Y.; Wu, J.F.; Sun, Z.J.; Pan, Q.M. Solubility of water in supercritical CO2. Huaxue Gongcheng Xi’an 2014, 42, 42–47. [Google Scholar]
- Bastami, A.; Allahgholi, M.; Pourafshary, P. Experimental and modelling study of the solubility of CO2 in various CaCl2 solutions at different temperatures and pressures. Pet. Sci. 2014, 11, 569–577. [Google Scholar] [CrossRef]
- Al Ghafri, S.Z.S.; Forte, E.; Galindo, A.; Maitland, G.C.; Trusler, J.P.M. Experimental and Modeling Study of the Phase Behavior of (Heptane + Carbon Dioxide + Water) Mixtures. J. Chem. Eng. Data 2015, 60, 3670–3681. [Google Scholar] [CrossRef]
- Krotov, D.G. Further Development of the group contribution equation of state VTPR for description of electrolyte and polymer systems. Ph.D. Thesis, Universität Oldenburg, Oldenburg, Germany, 2014. [Google Scholar]
- Meyer, C.W.; Harvey, A.H. Dew-point measurements for water in compressed carbon dioxide. AIChE J. 2015, 61, 2913–2925. [Google Scholar] [CrossRef]
- Muromachi, S.; Shijima, A.; Miyamoto, H.; Ohmura, R. Experimental measurements of carbon dioxide solubility in aqueous tetra-n-butylammonium bromide solutions. J. Chem. Thermodyn. 2015, 85, 94–100. [Google Scholar] [CrossRef]
- Foltran, S.; Vosper, M.E.; Suleiman, N.B.; Wriglesworth, A.; Ke, J.; Drage, T.C.; Poliakoff, M.; George, M.W. Understanding the solubility of water in carbon capture and storage mixtures: An FTIR spectroscopic study of H2O + CO2 + N2 ternary mixtures. Int. J. Greenh. Gas Control 2015, 35, 131–137. [Google Scholar] [CrossRef]
- Bando, S.; Takemura, F.; Nishio, M.; Hihara, E.; Akai, M. Solubility of CO2 in Aqueous Solutions of NaCl at (30 to 60) °C and (10 to 20) MPa. J. Chem. Eng. Data 2003, 48, 576–579. [Google Scholar] [CrossRef]
- Marion, G.M.; Millero, F.J.; Camões, M.F.; Spitzer, P.; Feistel, R.; Chen, C.T.A. pH of seawater. Mar. Chem. 2011, 126, 89–96. [Google Scholar] [CrossRef]
- Byrne, R.H.; Breland, J.A. High precision multiwavelength pH determinations in seawater using cresol red. Deep. Sea Res. Part A Oceanogr. Res. Pap. 1989, 36, 803–810. [Google Scholar] [CrossRef]
- Clayton, T.D.; Byrne, R.H. Spectrophotometric seawater pH measurements: Total hydrogen ion concentration scale calibration of m-cresol purple and at-sea results. Deep. Sea Res. Part I Oceanogr. Res. Pap. 1993, 40, 2115–2129. [Google Scholar] [CrossRef]
- Robert-Baldo, G.L.; Morris, M.J.; Byrne, R.H. Spectrophotometric determination of seawater pH using phenol red. Anal. Chem. 1985, 57, 2564–2567. [Google Scholar] [CrossRef]
- Meyssami, B.; Balaban, M.O.; Teixeira, A.A. Prediction of pH in Model Systems Pressurized with Carbon Dioxide. Biotechnol. Prog. 1992, 8, 149–154. [Google Scholar] [CrossRef]
- Toews, K.L.; Shroll, R.M.; Wai, C.M.; Smart, N.G. pH-Defining Equilibrium between Water and Supercritical CO2. Influence on SFE of Organics and Metal Chelates. Anal. Chem. 1995, 67, 4040–4043. [Google Scholar] [CrossRef]
- Parton, T.; Spilimbergo, S.; Elvassore, N.; Bertucco, A. UV–VIS spectroscopy for the determination of diffusion coefficient and pH in aqueous solutions/sc-CO2 systems. In Proceedings of the 4th International Symposium on High Pressure Process Technology and Chemical Engineering, Venice, Italy, 22–25 September 2002; pp. 447–452. [Google Scholar]
- Rosenqvist, J.; Kilpatrick, A.D.; Yardley, B.W.D. Solubility of carbon dioxide in aqueous fluids and mineral suspensions at 294 K and subcritical pressures. Appl. Geochem. 2012, 27, 1610–1614. [Google Scholar] [CrossRef]
- Peng, C.; Crawshaw, J.P.; Maitland, G.C.; Trusler, J.P.M.; Vega-Maza, D. The pH of CO2-saturated water at temperatures between 308 K and 423 K at pressures up to 15 MPa. J. Supercrit. Fluids 2013, 82, 129–137. [Google Scholar] [CrossRef]
- Kimuro, B.; Kusayanagi, T.; Yamaguchi, F.; Ohtsubo, K.; Morishita, M. Basic experimental results of liquid CO2 injection into the deep ocean. IEEE Trans. Energy Convers. 1994, 9, 732–735. [Google Scholar] [CrossRef]
- Crolet, J.; Bonis, M. pH measurements in aqueous CO2 solutions under high pressure and temperature. Corrosion 1983, 39, 39–46. [Google Scholar] [CrossRef]
- Schaef, H.T.; McGrail, B.P.; Marti, P.F. Direct Measurements of pH and Dissolved CO2 Concentrations in H2O-CO2-NaCl Mixtures to Supercritical Conditions. In Proceedings of the Carbon Sequestration Second Annual Conference, Alexandria, VA, USA, 5–8 May 2003. [Google Scholar]
- Schaef, T.H.; McGrail, P.B. Direct measurements of pH and dissolved CO2 in H2O-CO2 brine mixtures to supercritical conditions. In Greenhouse Gas Control Technologies 7; Elsevier Science Ltd.: Oxford, UK, 2005; pp. 2169–2173. [Google Scholar] [CrossRef]
- Hinds, G.; Cooling, P.; Wain, A.; Zhou, S.; Turnbull, A. Technical Note: Measurement of pH in Concentrated Brines. Corrosion 2009, 65, 635–638. [Google Scholar] [CrossRef]
- Millero, F.J.; DiTrolio, B.R.; Suarez, A.F.; Lando, G. Spectroscopic measurements of the pH in NaCl brines. Geochim. Cosmochim. Acta 2009, 73, 3109–3114. [Google Scholar] [CrossRef]
- Shao, H.; Thompson, C.J.; Cantrell, K.J. Evaluation of experimentally measured and model-calculated pH for rock–brine–CO2 systems under geologic CO2 sequestration conditions. Chem. Geol. 2013, 359, 116–124. [Google Scholar] [CrossRef]
- Truche, L.; Bazarkina, E.F.; Berger, G.; Caumon, M.-C.; Bessaque, G.; Dubessy, J. Direct measurement of CO2 solubility and pH in NaCl hydrothermal solutions by combining in-situ potentiometry and Raman spectroscopy up to 280 °C and 150 bar. Geochim. Cosmochim. Acta 2016, 177, 238–253. [Google Scholar] [CrossRef]
- Haghi, R.K.; Chapoy, A.; Peirera, L.M.C.; Yang, J.; Tohidi, B. pH of CO2 saturated water and CO2 saturated brines: Experimental measurements and modelling. Int. J. Greenh. Gas Control 2017, 66, 190–203. [Google Scholar] [CrossRef]
- Li, X.; Peng, C.; Crawshaw, J.P.; Maitland, G.C.; Trusler, J.P.M. The pH of CO2-saturated aqueous NaCl and NaHCO3 solutions at temperatures between 308 K and 373 K at pressures up to 15 MPa. Fluid Phase Equilibria 2018, 458, 253–263. [Google Scholar] [CrossRef]
- Hyde, A.M.; Zultanski, S.L.; Waldman, J.H.; Zhong, Y.L.; Shevlin, M.; Peng, F. General principles and strategies for salting-out informed by the Hofmeister series. Org. Process Res. Dev. 2017, 21, 1355–1370. [Google Scholar] [CrossRef]
- Stefánsson, A.; Bénézeth, P.; Schott, J. Carbonic acid ionization and the stability of sodium bicarbonate and carbonate ion pairs to 200 °C—A potentiometric and spectrophotometric study. Geochim. Cosmochim. Acta 2013, 120, 600–611. [Google Scholar] [CrossRef]
- Mohammadian, E.; Hadavimoghaddam, F.; Kheirollahi, M.; Jafari, M.; Chenlu, X.; Liu, B. Probing Solubility and pH of CO2 in aqueous solutions: Implications for CO2 injection into oceans. J. CO2 Util. 2023, 71, 102463. [Google Scholar]
- Parkhurst, D.L.; Appelo, C.A.J. Description of input and examples for PHREEQC version 3: A computer program for speciation, batch-reaction, one-dimensional transport, and inverse geochemical calculations. In U.S. Geological Survey Techniques and Methods, Book 6; U.S. Geological Survey: Denver, CO, USA, 2013; p. 519. [Google Scholar]
- Wolery, T.J. EQ3NR: A Computer Program for Geochemical Aqueous Speciation-Solubility Calculations User’s Guide and Documentation; U.S. Department of Energy Office of Scientific and Technical Information: Oak Ridge, TN, USA, 1983; p. 202. [Google Scholar]
- Pitzer, K.S. Thermodynamics of electrolytes. I. Theoretical basis and general equations. J. Phys. Chem. 1973, 77, 268–277. [Google Scholar] [CrossRef]
- Plummer, L.N.; Parkhurst, D.L.; Fleming, G.W.; Dunkle, S.A. A Computer Program Incorporating Pitzer’s Equations for Calculation of Geochemical Reactions in Brines; U.S. Geological Survey: Denver, CO, USA, 1988. [Google Scholar]
- Pitzer, K.S. (Ed.) Activity Coefficients in Electrolyte Solutions; CRC Press: Boca Raton, FL, USA, 1991. [Google Scholar]
- MacInnes, D.A. The Activities of the Ions of Strong Electrolytes. J. Am. Chem. Soc. 1919, 41, 1086–1092. [Google Scholar] [CrossRef]
- Bennion, B.; Bachu, S. Drainage and imbibition relative permeability relationships for supercritical CO2/Brine and H2S/Brine systems in intergranular sandstone, carbonate, shale, and anhydrite rock. SPE Reserv. Eval. Eng. 2008, 11, 487–496. [Google Scholar] [CrossRef]
- Perrin, J.C.; Benson, S. An Experimental Study on the Influence of Sub-Core Scale Heterogeneities on CO2 Distribution in Reservoir Rocks. Transp. Porous Media 2010, 82, 93–109. [Google Scholar] [CrossRef]
- Krevor, S.C.M.; Pini, R.; Zuo, L.; Benson, S.M. Relative permeability and trapping of CO2 and water in sandstone rocks at reservoir conditions. Water Resour. Res. 2012, 48, 16. [Google Scholar] [CrossRef]
- Perrin, J.-C.; Michael, K.; Chia-Wei, K.; Ljuba, M.; Ethan, C.; Sally, M., B. Core-scale experimental study of relative permeability properties of CO2 and brine in reservoir rocks. Energy Procedia 2009, 1, 3515–3522. [Google Scholar] [CrossRef]
- Farokhpoor, R.; Lindeberg, E.G.B.; Torsaeter, O.; Mork, M.B.; Mork, A. Permeability and relative permeability measurements for CO2-brine system at reservoir conditions in low permeable sandstones in Svalbard. Greenh. Gases 2014, 4, 36–52. [Google Scholar] [CrossRef]
- Sun, Y.; Li, Q.; Yang, D.; Liu, X. Laboratory core flooding experimental systems for CO2 geosequestration: An updated review over the past decade. J. Rock Mech. Geotech. Eng. 2016, 8, 113–126. [Google Scholar] [CrossRef]
- Kuo, C.-W.; Benson, S.M. Numerical and analytical study of effects of small scale heterogeneity on CO2/brine multiphase flow system in horizontal corefloods. Adv. Water Resour. 2015, 79, 1–17. [Google Scholar] [CrossRef]
- Wang, S.; Jiang, L.; Cheng, Z.; Liu, Y.; Zhao, J.; Song, Y. Experimental study on the CO2-decane displacement front behavior in high permeability sand evaluated by magnetic resonance imaging. Energy 2021, 217, 119433. [Google Scholar] [CrossRef]
- Wang, S.; Cheng, Z.; Jiang, L.; Song, Y.; Liu, Y. Quantitative study of density-driven convection mass transfer in porous media by MRI. J. Hydrol. 2021, 594, 125941. [Google Scholar] [CrossRef]
- Chen, L.Y.; Liu, Y.; Mutailipu, M. Based on the Pore Network Model of CO2-Water-Quartz System Two-Phase Flow Characteristics Study. Adv. Mater. Res. 2014, 962–965, 1289–1292. [Google Scholar] [CrossRef]
- Dong, H.; Fjeldstad, S.; Alberts, L.; Roth, S.; Bakke, S.; Øren, P.-E.; Numerical Rocks, A. Pore network modelling on carbonate: A comparative study of different micro-CT network extraction methods. In Proceedings of the International Symposium of the Society of Core Analysts, Abu Dhabi, United Arab Emirates, 29 October–2 November 2008; pp. 22–29. [Google Scholar]
- Dong, H.; Blunt, M. Pore-network extraction from micro-computerized-tomography images. Phys. Rev. E 2009, 80, 036307. [Google Scholar] [CrossRef]
- Valvatne, P.H.; Blunt, M.J. Predictive pore-scale modeling of two-phase flow in mixed wet media. Water Resour. Res. 2004, 40, W07406. [Google Scholar] [CrossRef]
- Zhao, X.C.; Blunt, M.J.; Yao, J. Pore-scale modeling: Effects of wettability on waterflood oil recovery. J. Pet. Sci. Eng. 2010, 71, 169–178. [Google Scholar] [CrossRef]
- Gunstensen, A.K.; Rothman, D.H.; Zaleski, S.; Zanetti, G. Lattice Boltzmann model of immiscible fluids. Phys. Rev. A 1991, 43, 4320–4327. [Google Scholar] [CrossRef] [PubMed]
- Shan, X.; Chen, H. Lattice Boltzmann model for simulating flows with multiple phases and components. Phys. Rev. E 1993, 47, 1815–1819. [Google Scholar] [CrossRef] [PubMed]
- Wang, M.; Wang, J.; Pan, N.; Chen, S. Mesoscopic predictions of the effective thermal conductivity for microscale random porous media. Phys. Rev. E 2007, 75, 036702. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.; Chen, L.; Kang, Q.; Rahman, S.S. Apparent permeability prediction of organic shale with generalized lattice Boltzmann model considering surface diffusion effect. Fuel 2016, 181, 478–490. [Google Scholar] [CrossRef]
- Lautenschlaeger, M.P.; Weinmiller, J.; Kellers, B.; Danner, T.; Latz, A. Homogenized lattice Boltzmann model for simulating multi-phase flows in heterogeneous porous media. Adv. Water Resour. 2022, 170, 104320. [Google Scholar] [CrossRef]
- Blunt, M.J.; Bijeljic, B.; Dong, H.; Gharbi, O.; Iglauer, S.; Mostaghimi, P.; Paluszny, A.; Pentland, C. Pore-scale imaging and modelling. Adv. Water Resour. 2012, 51, 197–216. [Google Scholar] [CrossRef]
- Alhammadi, A.M.; AlRatrout, A.; Singh, K.; Bijeljic, B.; Blunt, M.J. In situ characterization of mixed-wettability in a reservoir rock at subsurface conditions. Sci. Rep. 2017, 7, 10753. [Google Scholar] [CrossRef]
- Morrow, N.R. Wettability and its effect on oil recovery. J. Pet. Technol. 1990, 42, 1476–1484. [Google Scholar] [CrossRef]
- Landry, C.; Karpyn, Z.; Ayala, O. Relative permeability of homogenous-wet and mixed-wet porous media as determined by pore-scale lattice Boltzmann modeling. Water Resour. Res. 2014, 50, 3672–3689. [Google Scholar] [CrossRef]
- Hwang, S.I.; Lee, K.P.; Lee, D.S.; Powers, S.E. Effects of fractional wettability on capillary pressure–saturation–relative permeability relations of two-fluid systems. Adv. Water Resour. 2006, 29, 212–226. [Google Scholar] [CrossRef]
- Guo, R.; Dalton, L.; Crandall, D.; McClure, J.; Wang, H.; Li, Z.; Chen, C. Role of heterogeneous surface wettability on dynamic immiscible displacement, capillary pressure, and relative permeability in a CO2-water-rock system. Adv. Water Resour. 2022, 165, 104226. [Google Scholar] [CrossRef]
- Mahadevan, J. Comments on the paper titled “Contact angle measurements of CO2–water-quartz/calcite systems in the perspective of carbon sequestration”: A case of contamination? Int. J. Greenh. Gas Control 2012, 7, 261–262. [Google Scholar] [CrossRef]
- Wang, J.; Zhao, J.; Zhang, Y.; Wang, D.; Li, Y.; Song, Y. Analysis of the influence of wettability on permeability in hydrate-bearing porous media using pore network models combined with computed tomography. J. Nat. Gas Sci. Eng. 2015, 26, 1372–1379. [Google Scholar] [CrossRef]
- Wang, J.; Zhao, J.; Zhang, Y.; Wang, D.; Li, Y.; Song, Y. Analysis of the effect of particle size on permeability in hydrate-bearing porous media using pore network models combined with CT. Fuel 2016, 163, 34–40. [Google Scholar] [CrossRef]
- Mutailipu, M.; Liu, Y.; Wu, B.; Song, Y.; Wang, D.; Ai, L. Gas-Water Two Phase Flow Simulation Based on Pore Network Model for Reservoir Rocks. Energy Procedia 2017, 142, 3214–3219. [Google Scholar] [CrossRef]
- Mutailipu, M.; Liu, Y.; Wu, B. Simulation of wettability effects on gas-water flow in porous media. In Proceedings of the 16th International Heat Transfer Conference, Beijing, China, 10–15 August 2018. [Google Scholar]
- Mhriay, M.; Ying, T.; Peng, L.; Yu, L.; Yong, S. Numerical simulation of gas-water flow in heterogeneous wetting porous media. In Proceedings of the 4th Green House Gas Technology, Melbourne, Australia, 21–25 October 2018. [Google Scholar]
- Gu, S.J.; Lee, J.; Ki, S.; Huh, D.G.; Park, C.H. Effects of viscosity ratio, interfacial tension and flow rate on hysteric relative permeability of CO2/brine systems. Energy Oxf. 2017, 133, 62–69. [Google Scholar]
- Iyi, D.; Balogun, Y.; Oyeneyin, B.; Faisal, N. A numerical study of the effects of temperature and injection velocity on oil-water relative permeability for enhanced oil recovery. Int. J. Heat Mass Transf. 2022, 191, 122863. [Google Scholar] [CrossRef]
- Reis, P.; Carvalho, M.S. Pore-Scale Compositional Modeling of Gas-Condensate Flow: Effects of Interfacial Tension and Flow Velocity on Relative Permeability. J. Pet. Sci. Eng. 2021, 202, 108454. [Google Scholar] [CrossRef]
- Henderson, G.D.; Danesh, A.; Tehrani, D.H.; Peden, J.M. The effect of velocity and interfacial tension on relative permeability of gas condensate fluids in the wellbore region. J. Pet. Sci. Eng. 1997, 17, 265–273. [Google Scholar] [CrossRef]
- Balogun, Y.; Iyi, D.; Faisal, N.; Oyeneyin, B.; Oluyemi, G.; Mahon, R. Experimental investigation of the effect of temperature on two-phase oil-water relative permeability. J. Pet. Sci. Eng. 2021, 203, 108645. [Google Scholar] [CrossRef]
- Esmaeili, S.; Modaresghazani, J.; Sarma, H.; Harding, T.G.; Maini, B.B.J.F. Effect of temperature on relative permeability–Role of viscosity ratio. Fuel 2020, 278, 118318. [Google Scholar] [CrossRef]
- Bennion, B.; Bachu, S. Relative Permeability Characteristics for Supercritical CO2 Displacing Water in a Variety of Potential Sequestration Zones in the Western Canada Sedimentary Basin. In Proceedings of the SPE Annual Technical Conference and Exhibition, Dallas, TX, USA, 9–12 October 2005. [Google Scholar]
- Bennion, D.B.; Bachu, S. Dependence on Temperature, Pressure, and Salinity of the IFT and Relative Permeability Displacement Characteristics of CO2 Injected in Deep Saline Aquifers. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 24–27 September 2006. [Google Scholar]
- Babadagli, T. Analysis of oil recovery by spontaneous imbibition of surfactant solution. Oil Gas Sci. Technol. 2005, 60, 697–710. [Google Scholar] [CrossRef]
- Mohammad, J.; Javad, A. Investigating the effect of interfacial tension and contact angle on capillary pressure curve, using free energy Lattice Boltzmann Method. J. Nat. Gas Sci. Eng. 2016, 35, 1146–1157. [Google Scholar]
- Iyi, D.; Balogun, Y.; Oyeneyin, B.; Faisal, N. Numerical Modelling of the Effect of Wettability, Interfacial Tension and Temperature on Oil Recovery at Pore-Scale level. J. Pet. Sci. Eng. 2021, 201, 108453. [Google Scholar] [CrossRef]

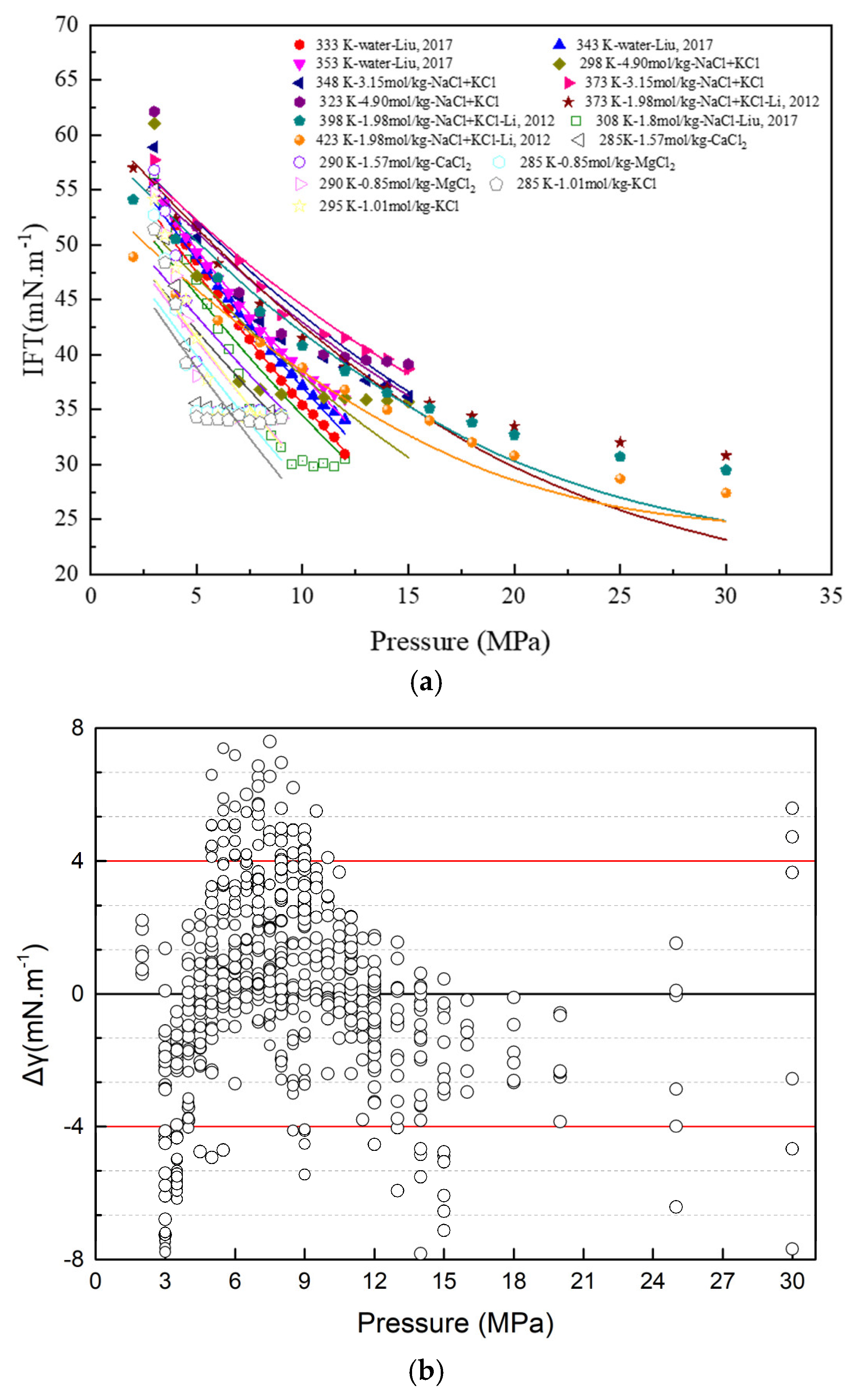
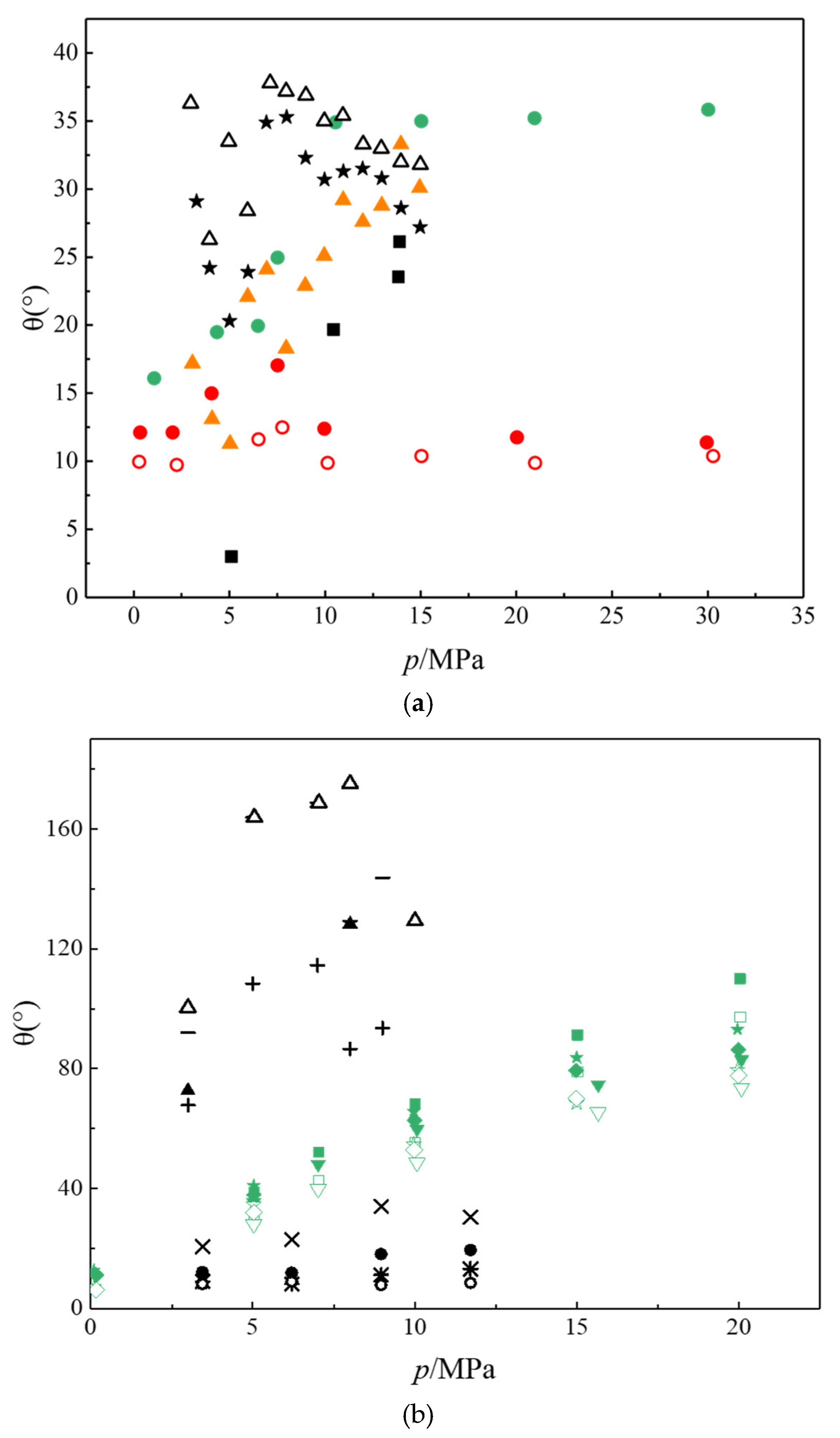

| References | Solutions | Method | T/K | p/MPa |
|---|---|---|---|---|
| Jho et al. (1978) [33] | H2O | CT | 284–318 | 0–6 |
| Chun et al. (1995) [36] | H2O/methanol | CT | 278–344 | 0.1–19 |
| Hebach et al. (2002) [34] | H2O | PD | 278–333 | 0.1–20 |
| Akutsu et al. (2007) [37] | H2O | PD | 298–318 | 7.5–17 |
| Kavame et al. (2007) [38] | H2O | PD | 278–335 | 0.1–20 |
| Chiquet et al. (2007) [60] | H2O | PD | 308–383 | 0–50 |
| Georgiadis et al. (2010) [39] | H2O/Alkane | PD/SAFT-VR/DG | 298–374 | 1–60 |
| Bikkina et al. (2011) [61] | H2O | PD | 298–333 | 1.4–21 |
| Li et al. (2012) [40] | H2O | PD | 335–448 | 0–50 |
| Saraji et al. (2013) [62] | H2O | PD | 308–333 | 3–10.5 |
| Khosharay et al. (2014) [63] | Complex system | PD | 284–313 | 0–6 |
| Pereira et al. (2016) [64] | H2O | PD | 298–469 | 0–69 |
| Liu et al. (2016) [65] | CH4/CO2-H2O | PD | 299–346 | 0–30 |
| Rocha et al. (2001) [43] | H2O | MD | 318–338 | 20–28 |
| Kuznetsov et al. (2002) [44] | H2O | MD | 285–298 | 9–30 |
| Sutjiadi et al. (2008) [66] | H2O/alcohol | PD | 313 | 0–27 |
| Nielsen et al. (2012) [42] | H2O | MD | 298–383 | 0–40 |
| Chow et al. (2016) [67] | Complex system | PD/EE | 298–448 | 0–40 |
| Chow et al. (2016) [50] | Complex system | SAFT-VR+SGT | 298–473 | 0–60 |
| Zhang et al. (2016) [46] | H2O | ANN | 278–448 | 0.1–61 |
| References | Solutions | Molalities | Method | T/K | p/MPa |
|---|---|---|---|---|---|
| Yang et al. (2005) [68] | Complex brine | 4.27 g·L−1 | PD | 300–331 | 0–30 |
| Chalbaud et al. (2006) [69] | NaCl (aq) | 0–150 g·L−1 | PD | 300–373 | 4–26 |
| Pierre et al. (2007) [60] | NaCl (aq) | 0–20 g·L−1 | PD | 308–383 | 5–45 |
| Bachu et al. (2009) [70] | NaCl (aq) | 0–334 g·L−1 | PD | 293–398 | 2–27 |
| Chalbaud et al. (2009) [13] | NaCl (aq) | 0.085–2.75 mol·kg−1 | PD | 300.15–373.2 | 4–25 |
| Li et al. (2012) [40] | Complex brine | 0.98–4.95 mol·kg−1 | PD | 298–448 | 2–50 |
| Liu et al. (2015) [71] | NaCl (aq) | 0.1–1.0 mol·L−1 | PD | 300–313 | 3–9 |
| Liu et al. (2017) [72] | NaCl (aq) | 0–1.80 mol·kg−1 | PD | 300–353 | 3–12 |
| Arif et al. (2016) [73] | NaCl (aq) | 25 wt % | PD | 308–343 | 0–20 |
| Pereira et al. (2017) [74] | NaCl (aq) | 0.98–1.98 mol·kg−1 | PD/DGT | 300–423 | 0–70 |
| Yekeen et al. (2021) [75] | NaCl (aq) | 0–7 wt % | PD | 353–453 | 8–22 |
| Aggelopoulos et al. (2010) [41] | Complex brine | 5–300 g·L−1 | PD | 300–373 | 5–25 |
| Aggelopoulos et al. (2011) [76] | CaCl2 (aq) | 0.045–1.50 mol·kg−1 | PD | 300–373 | 5–25 |
| Li et al. (2012) [40] | Complex brine | 2–5 mol·kg−1 | PD | 343.15–423.15 | 2–50 |
| Liu et al. (2017) [72] | Complex brine | 0.045–1.8 mol·kg−1 | PD | 284–353 | 2–12 |
| Arif et al. (2017) [77] | Complex brine | 0–30 wt % | PD | 323 | 0.1–20 |
| Wang et al. (2018) [78] | Complex brine | 21.46 g·L−1 | PD | 370.65 | 0.1–33.9 |
| Mutailipu et al. (2019) [32] | Complex brine | 0.98–4.95 mol·kg−1 | PD | 298–423 | 2–15 |
| Li et al. (2014) [45] | H2O/brine | 0–1 mol·kg−1 | GAC | 278–403 | 0–50 |
| Zhang et al. (2016) [46] | Complex brine | 0–5 mol·kg−1 | ANN | 278–448 | 0.1–61 |
| Partovi et al. (2017) [47] | Complex brine | 0–5 mol·kg−1 | ANN | 278–448 | 0.1–61 |
| Amooie et al. (2019) [79] | Complex brine | 0–4.087 mol·kg−1 | ML | 290–420 | 5–25 |
| Parameter | a1 | a2 | a3 | b1 | b2 | b3 | b4 | b5 | b6 |
|---|---|---|---|---|---|---|---|---|---|
| Coefficient | 3.672 | 1.376 | −3.232 | −165.524 | −3.663 | 382.276 | 1.760 | 13.217 | −160.054 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mutailipu, M.; Xue, Q.; Li, T.; Yang, Y.; Xue, F. Thermodynamic Properties of a Gas–Liquid–Solid System during the CO2 Geological Storage and Utilization Process: A Review. Energies 2023, 16, 7374. https://doi.org/10.3390/en16217374
Mutailipu M, Xue Q, Li T, Yang Y, Xue F. Thermodynamic Properties of a Gas–Liquid–Solid System during the CO2 Geological Storage and Utilization Process: A Review. Energies. 2023; 16(21):7374. https://doi.org/10.3390/en16217374
Chicago/Turabian StyleMutailipu, Meiheriayi, Qingnan Xue, Tao Li, Yande Yang, and Fusheng Xue. 2023. "Thermodynamic Properties of a Gas–Liquid–Solid System during the CO2 Geological Storage and Utilization Process: A Review" Energies 16, no. 21: 7374. https://doi.org/10.3390/en16217374
APA StyleMutailipu, M., Xue, Q., Li, T., Yang, Y., & Xue, F. (2023). Thermodynamic Properties of a Gas–Liquid–Solid System during the CO2 Geological Storage and Utilization Process: A Review. Energies, 16(21), 7374. https://doi.org/10.3390/en16217374







