Evaluation of Voltage Variations Arising from the Increase in Photovoltaic Insertion in the Transmission Network: Case Study Coremas-PB Power Plant
Abstract
:1. Introduction
2. Voltage Regulation Problems
2.1. Steady-State Voltage Variations
2.2. Modeling of Electrical Parameters in a Contingency Situation
3. Electric Network Modeling
3.1. Modeling of the Photovoltaic System in ANAREDE Software
3.2. Transformer Modeling
3.3. Transmission Line
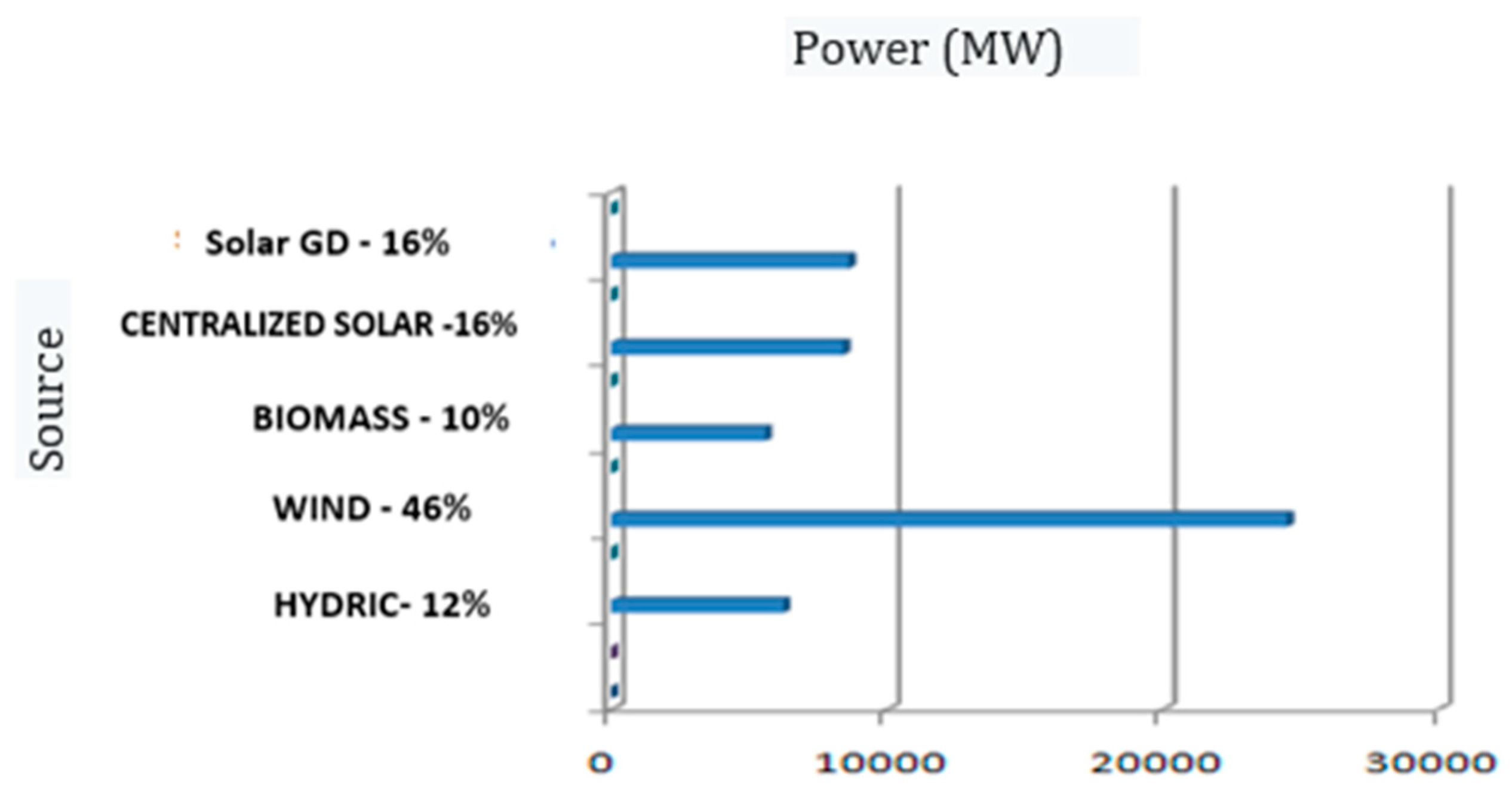
3.4. System Description
4. Methodology
4.1. Description of Scenarios and Results
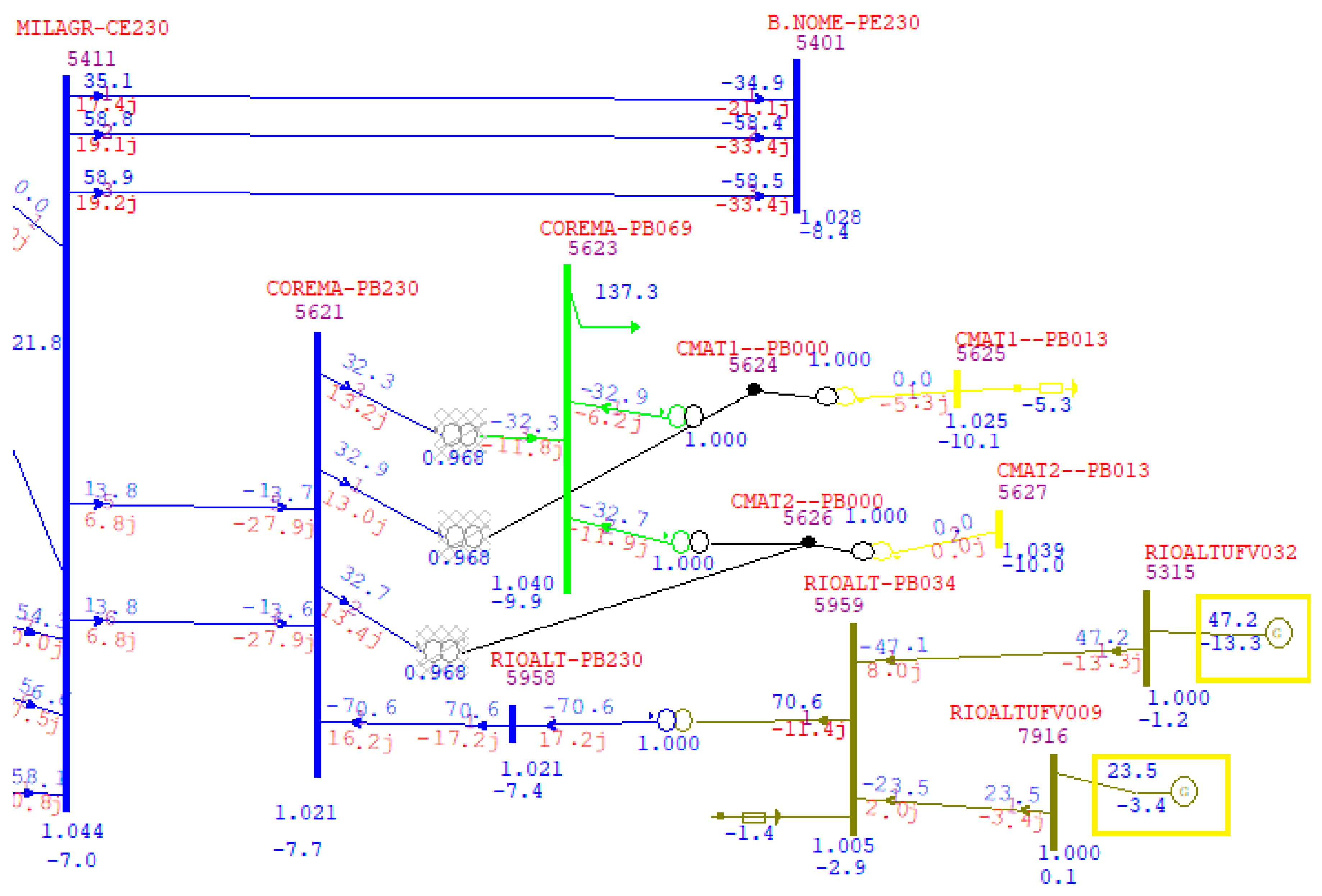
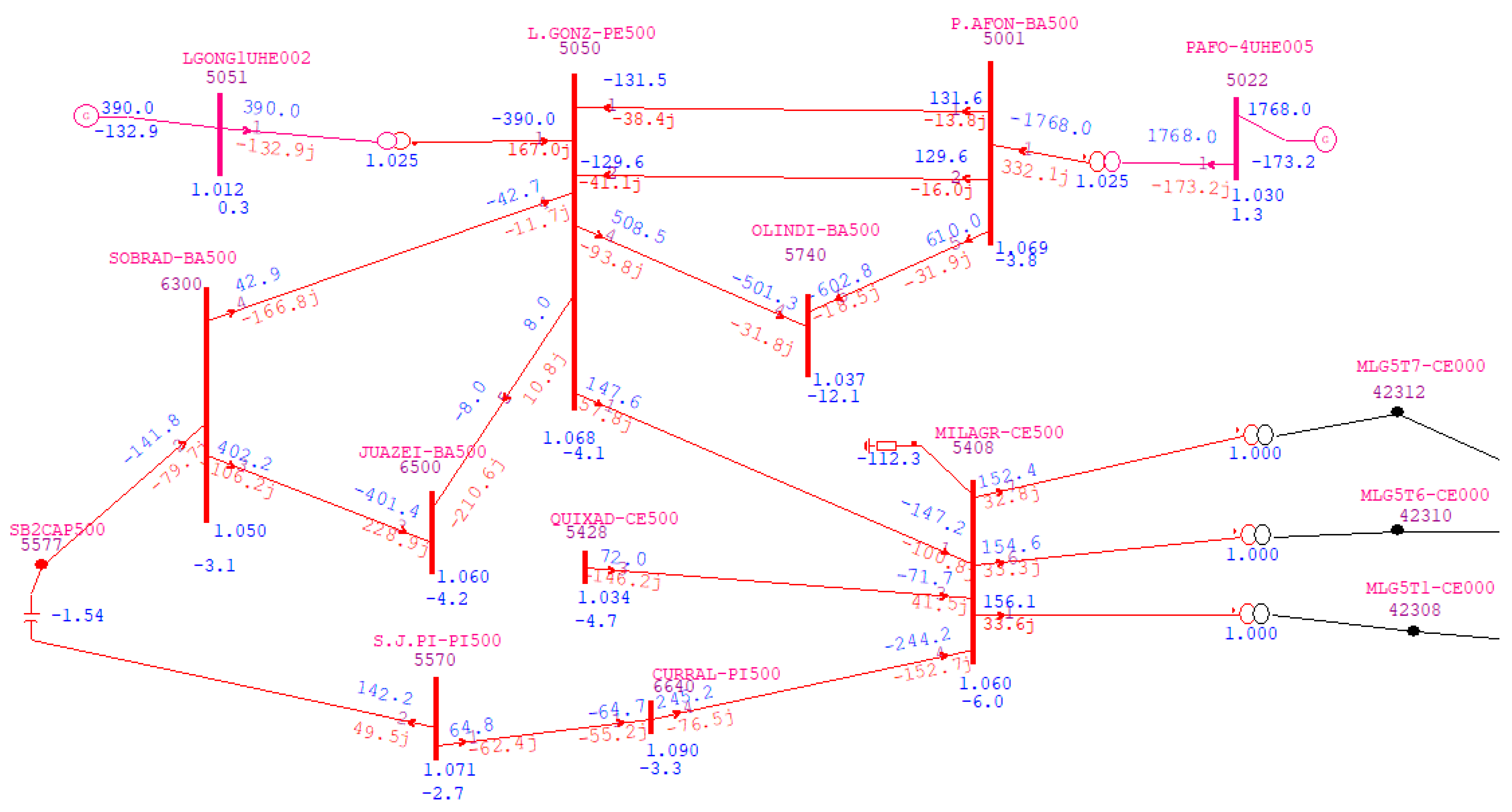
4.1.1. Scenario 1 (Baseline)—Actual Transmission System with Current Photovoltaic Generation
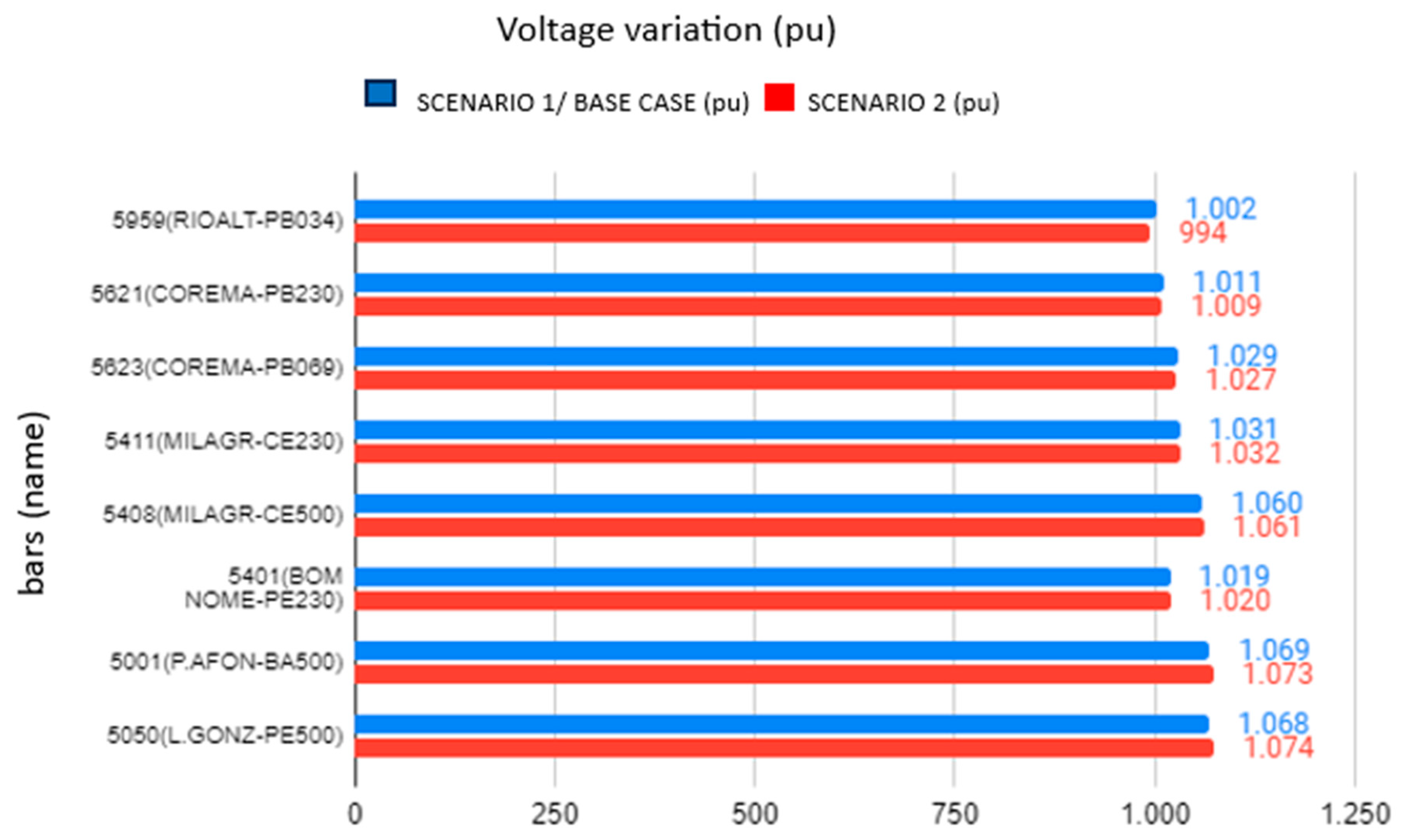
4.1.2. Scenario 2—Actual Transmission System with Photovoltaic Generation with 50% of the Expected Increase in Active Power Generation
- ➢
- 5408 (MILAGR-CE500)—5411 (MILAGR-CE230),
- ➢
- 5621 (COREMA-PB230)—5623 (COREMA-PB069).
- ➢
- 5621 (COREMA-PB230);
- ➢
- 5411 (MILAGR-CE230);
- ➢
- 5401 (BOM NOME-PE230);
- ➢
- 5959 (RIOALT-PB034);
- ➢
- 5623 (COREMA-PB069);
- ➢
- 5408 (MILAGR-CE500), which are buses close to the PV connection and in the buses;
- ➢
- 5050 (L.GONZ-PE500) and 5001 (P.AFON-BA500), which are buses close to the conventional generation (hydroelectric).
4.1.3. Scenario 3—Actual Transmission System with Photovoltaic Generation with 100% of the Expected Increase in Active Power Generation
- ➢
- 5408 (MILAGR-CE500)—5411 (MILAGR-CE230),
- ➢
- 5621 (COREMA-PB230)—5623 (COREMA-PB069).
4.1.4. Scenario 4—Contingency Analysis with Minimum PV Generation
- ➢
- 5621 (COREMA-PB230);
- ➢
- 5411 (MILAGR-CE230);
- ➢
- 5401 (BOM NOME-PE230);
- ➢
- 5959 (RIOALT-PB034);
- ➢
- 5623 (COREMA-PB069);
- ➢
- 5408 (MILAGR-CE500), which are buses closer to the PV connection; and on the bars:
- ➢
- 5050 (L.GONZ-PE500) and 5001 (P.AFON-BA500), which are buses closer to conventional hydroelectric generation.
4.1.5. Scenario 5—Contingency Analysis with PV Generation under Conditions of 100% PV Generation Increment
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Remund, J.; Ramirez, L.; Wilbert, S.; Blanc, P.; Lorenz, E.; Köhler, C.; Paristech, M. Solar Resource for High Penetration and Large-Scale Applications. In Proceedings of the 33rd European Photovoltaic Solar Energy Conference and Exhibition PVSEC, Amsterdam, The Netherlands, 25–29 September 2017; p. 40. [Google Scholar]
- IEA PVPS Reporting Countries, Becquerel Institute (BE). For the Non-IEA PVPS Countries: Izumi Kaizuka (RTS; Corporation), Arnulf Jäger-WALDAU (EU-JRC), Jose Donoso (UNEF). Snapshot of Global PV Markets 2023. [S.l: s.n.]. 2023. Available online: https://iea-pvps.org/wp-content/uploads/2023/04/IEA_PVPS_Snapshot_2023.pdf (accessed on 13 February 2023).
- ANEEL—Agência Nacional de Energia Elétrica. 2023. Available online: https://www.gov.br/aneel/pt-br/assuntos/noticias/2023/primeiro-semestre-de-2023-tem-crescimento-de-5-1-gw-na-matriz-eletrica-brasileira (accessed on 13 June 2023).
- ANEEL. Atlas de Energia Elétrica do Brasil, 3rd ed.; Agência Nacional de Energia Elétrica: Brasília, Brazil, 2008. Available online: https://www.fisica.net/energia/atlas_de_energia_eletrica_do_brasul_3a-ed.pdf (accessed on 13 December 2021).
- Pereira, E.B.; Martins, F.R.; Gonçalves, A.R.; Costa, R.S.; Lima, F.L.; Rüther, R.; Abreu, S.L.; Tiepolo, G.M.; Pereira, S.V.; Souza, J.G. Atlas Brasileiro de Energiaslar, 2nd ed.; Instituto Nacional de Pesquisas Espaciais (INPE): São José, Brazil, 2017.
- Pinho, J.T.; Galdino, M.A. Manual de Engenharia para Sistemas Fotovoltaicos. Rio de Janeiro: CEPEL/CRESESB. 2014. Available online: http://www.cresesb.cepel.br/publicacoes/download/Manual_de_Engenharia_FV_2014.pdf (accessed on 20 December 2021).
- Ruschel, C.S.; Santucci, A.; Oliveira, B. Evolução das Características Técnicas dos Projetos Fotovoltaicos nos Leilões de Energia do Mercado Regulado. 2014. Available online: https://anaiscbens.emnuvens.com.br/cbens/article/download/981/981 (accessed on 13 December 2022).
- Villalva, M.G. Energia Solar Fotovoltaica: Conceitos e Aplicações, 2nd ed.; Saraiva: São Paulo, Brazil, 2015. [Google Scholar]
- EPE. Plano Nacional de energia 2050. Ministério de Minas e Energia. Empresa de Pesquisa Energética. Energética. Brasília: MME/EPE. 2022. Available online: https://www.gov.br/mme/pt-br/assuntos/secretarias/sntep/publicacoes/plano-nacional-de-energia/plano-nacional-de-energia-2050 (accessed on 22 February 2022).
- Stevenson, W.D., Jr. Elementos de Análise de Sistemas de Potência; McGraw-Hill Education do Brasil: São Paulo, Brazil, 1978. [Google Scholar]
- EPE. Plano Decenal de Expansão de Energia 2029. Ministério de Minas e Energia. Empresa de Pesquisa Energética. Brasília: MME/EPE. 2020. Available online: https://www.epe.gov.br/sites-pt/publicacoes-dados-abertos/publicacoes/Documents/PDE%202029.pdf (accessed on 1 January 2021).
- Kumar, D.S.; Sharma, A.; Srinivasan, D.; Reindl, T. Stability implications of bulk power net works with large scale PVs. Energy 2019, 187, 115927. [Google Scholar] [CrossRef]
- Denholm, P.; O’Connell, M.; Brinkman, G.; Jorgenson, J. Overgeneration from Solar Energy in California: A Field Guide to the Duck Chart; Technical Report, November, 2015; Office of Energy Efficiency & Renewable Energy: Golden, CO, USA, 2015.
- Matarucco, R.R. Método da Continuação Aplicado na Análise de Contingência de Linhas de Transmissão. Ph.D. Thesis, Universidade Estadual Paulista, São Paulo, Brazil, 2010. [Google Scholar]
- ONS. Balanço de Energia. Available online: https://www.ons.org.br/paginas/energia-agora/balanco-de-energia (accessed on 22 December 2022).
- Zhang, Y.; Mensah-bonsu, C.; Walke, P.; Arora, S.; Pierce, J. Transient over-voltages in high voltage grid-connected PV solar interconnection. In Proceedings of the IEEE PES General Meeting, Minneapolis, MN, USA, 25–29 July 2010; p. 1e6. [Google Scholar]
- Martins, T.U. Estudos Elétricos para Conexão à Rede Básica de Uma Central Geradora Solar Fotovoltaica Utilizando a Ferramenta Computacional Anarede; Monografia (Graduação em Engenharia Elétrica); Universidade Federal do Ceará: Fortaleza, Brazil, 2019; p. 19. [Google Scholar]
- Luque, A.; Hegedus, S. (Eds.) PV Systems. In Handbook of Photovoltaic Science and Engineering, 2nd ed.; Wiley: Hoboken, NJ, USA, 2012. [Google Scholar]
- Torres, I.C.; Chigueru, T.; Negreiros, G.F. Impacto da Instalação Massiva de Sistemas FV Distríbuidos no Nível de Tensão da Rede de Distribuição Elétrica de Baixa Tensão. VIII Congresso Brasileiro de Energia Solar. Fortaleza, Ceará. Available online: http://www.abens.org.br/CBENS2020/home/ (accessed on 20 January 2022).
- Resende, J.W. Introdução a Compensação Reativa: Apostila da Disciplina Carga Elétricas não Convencionais na Rede Elétrica: Uberlândia; Universidade Federal de Uberlândia: Uberlândia, Brazil, 2020. [Google Scholar]
- Paludo, J.A. Avaliação dos Impactos de Elevados Níveis de Penetração da Geração Fotovoltaica no Desempenho de Sistemas de Distribuição de Energia Elétrica em Regime Permanente. Master’s Thesis, Escola de Engenharia de São Carlos da Universidade de São Paulo, São Carlos, Brazil, 2014. [Google Scholar]
- ANEEL—Agência Nacional de Energia Elétrica. Procedimentos de Rede, Módulo 2—Critérios e Requisitos, Submódulo 2.3 -Premissas, Critérios e Metodologia para estudos elétricos, Revisão 12. Resolução normativa nº 903/2020. 2021; pp. 1–35. [Google Scholar]
- CHESF. Subestação Coremas. Dados fornecidos pela empresa. 2021. Available online: https://www.chesf.com.br/Pages/default.aspx (accessed on 22 December 2022).
- Family Search. Coremas, Paraíba: Genealogia. Available online: https://www.familysearch.org/wiki/pt/Coremas,_Para%C3%ADba_-_Genealogia (accessed on 13 December 2021).
- Google Earth. Coremas. 2020. Available online: https://earth.google.com/web (accessed on 13 December 2021).
- ONS. Procedimentos de Rede. Operador Nacional do Sistema. 2020. Available online: https://www.ons.org.br/paginas/sobre-o-ons/procedimentos-de-rede/vigentes (accessed on 22 December 2021).
- Oliveira, E.H.G.D. Análise Estática de Contingências do Sistema de Transmissão de 230 kV do Acre / Rondônia. 2019. Available online: https://bdm.unb.br/bitstream/10483/24373/1/2019_EduardoHenriqueGomesDeOliveira_tcc.pdf (accessed on 18 December 2020).
- Monticelli, A.J. Fluxo de Carga em Redes de Energiaelétrica; Edgard Blücher: São Paulo, Brazil, 1983. [Google Scholar]






| Rated Operating Voltage | Normal Operating Condition | Operation Condition under Contingency | ||
|---|---|---|---|---|
| (kV) | (kV) | (pu) | (kV) | (pu) |
| <230 | ------- | 0.95 to 1.05 | ------- | 0.90 to 1.05 |
| 230 | 218 to 242 | 0.95 to 1.05 | 207 to 242 | 0.90 to 1.05 |
| 345 | 328 to 362 | 0.95 to 1.05 | 311 to 362 | 0.90 to 1.05 |
| 440 | 418 to 460 | 0.95 to 1.046 | 396 to 460 | 0.95 to 1.046 |
| 500 | 500 to 550 | 1.00 to 1.10 | 475 to 550 | 0.95 to 1.10 |
| 525 | 500 to 550 | 0.95 to 1.048 | 475 to 550 | 0.90 to 1.048 |
| 765 | 690 to 800 | 0.90 to 1.046 | 690 to 800 | 0.90 to 1.046 |
| Transformer | R (%) | X (%) | B (Mvar) | Capacity (Mva) | Transformation Ratio |
|---|---|---|---|---|---|
| 5408 (MILAGR-CE500)–5411 (MILAGR-CE230) | 0 | 0.096 | 0 | 600 | 500/230 kV |
| 5621 (COREMA-PB230)–5623 (COREMA-PB069) | 0 | 13.19 | 0 | 100 | 230/69 kV |
| Transmission Line | R | X | B | Capacity |
|---|---|---|---|---|
| (%) | (%) | (MVAr) | (MVA) | |
| 5428 (QUIXAD-CE500)–5408 (MILAGR-CE500) | 0.26 | 2.97 | 399.02 | 2070 |
| 5050 (L.GONZ-PE500)–5740 (OLINDI-BA500) | 0.31 | 3.1 | 324.34 | 1306 |
| 5001 (P.AFONS-BA500)–5740 (OLINDI-BA500) | 0.21 | 2.68 | 272.76 | 2165 |
| 6300 (SOBRAD-BA500)–5050 (L.GONZ-PE500) | 0.29 | 3.99 | 411.44 | 2165 |
| 5050 (L.GONZ-PE500)–5408 (MILAGR-CE500) | 0.21 | 2.57 | 343.26 | 2186 |
| 6500 (JUAZEI-BA500)–5050 (L.GONZ-PE500) | 0.25 | 3.2 | 327.32 | 1732 |
| 6640 (CURRAL-PI500)–5408 (MILAGR-CE500) | 0.155 | 2.231 | 356.98 | 1992 |
| 5570 (S.J.PI-PI500–6640 (CURRAL-PI500) | 0.134 | 1.92 | 279.9 | 1992 |
| 5577 (SJ-SB2CAP500)–6300 (SOBRAD-BA500) | 0.153 | 2.272 | 327.32 | 1732 |
| 6300 (SOBRAD-BA500)–6500 (JUAZEI-BA500) | 0.04 | 0.48 | 47.043 | 2165 |
| 6500 (JUAZEI-BA500)–5050 (L.GONZ-PE500) | 0.25 | 3.2 | 327.78 | 2165 |
| 5411 (MILAGR-CE230)–5621 (COREMA-PB230) | 2.2 | 11.64 | 20.3 | 291 |
| 5411 (MILAGR-CE230)–5401 (BOM NOME-PE230 | 1.09 | 4.83 | 25.02 | 400 |
| 5621 (COREMA-PB230)–5958 (RIOALT-PB230) | 0.1466 | 0.7 | 1.287 | 210 |
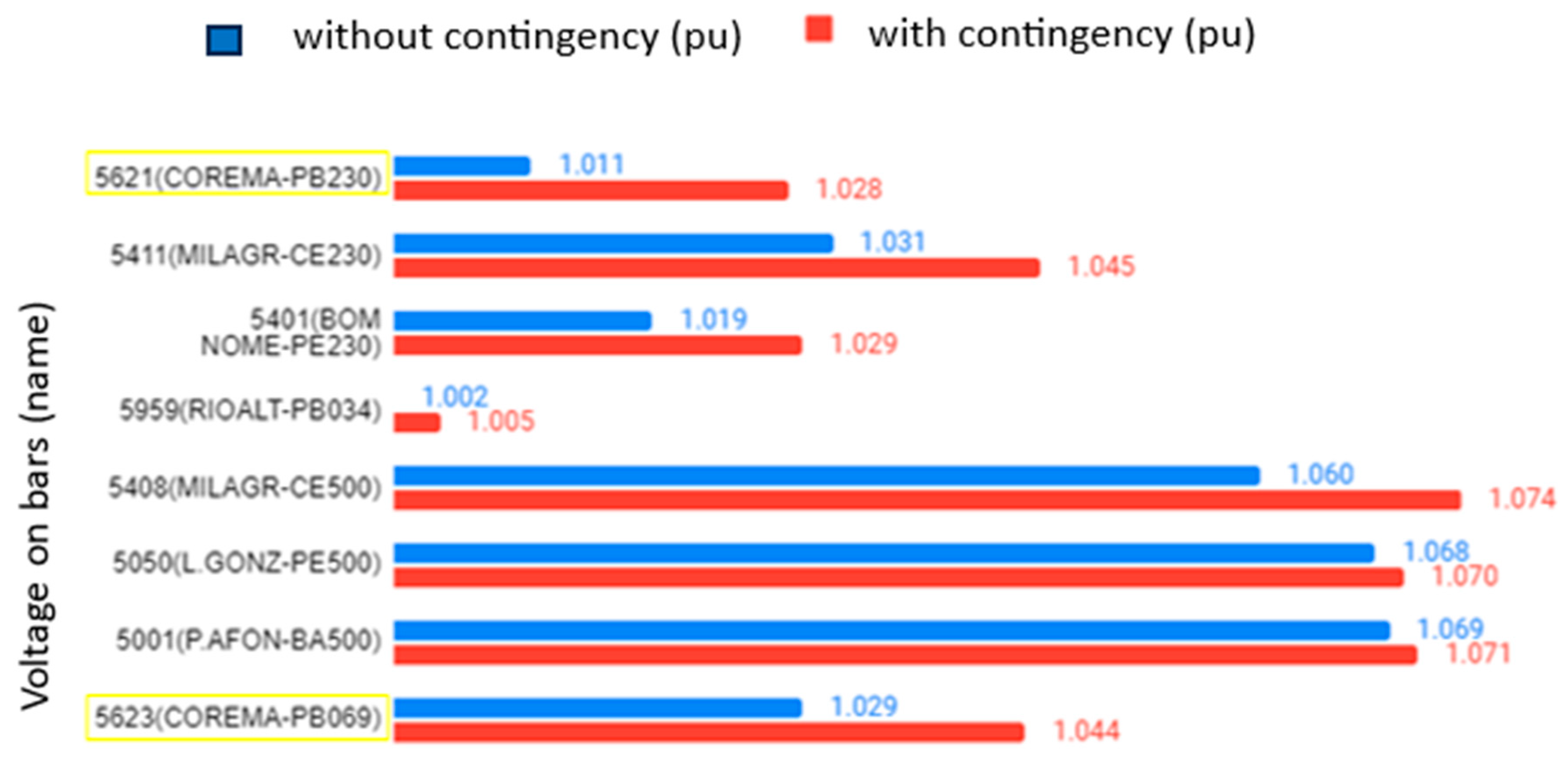
| Bars | Module | Angle | Generation | Load | Static C. | Shunt | Type | Status | ||
|---|---|---|---|---|---|---|---|---|---|---|
| (pu) | (°) | (MW) | (MVAR) | (MW) | (MVAR) | (MVAR) | (MVAR) | |||
| 5621 (COREMA-PB230) | 1.011 | −7.7 | 0 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5411 (MILAGR-CE230) | 1.031 | −7 | 0 | 0 | 0 | 0 | −46.3 | −21.3 | PQ | On |
| 5401 (BOM NOME-PE230) | 1.019 | −8.4 | 0 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5959 (RIOALT-PB034) | 1.002 | −2.8 | 0 | 0 | 0 | 0 | 0 | −1.4 | PQ | On |
| 5408 (MILAGR-CE500) | 1.060 | −6 | 0 | 0 | 0 | 0 | 0 | −112.3 | PQ | On |
| 5050 (L.GONZ-PE500) | 1.068 | −4.1 | 0 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5001 (P.AFON-BA500) | 1.069 | −3.8 | 0 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5623 (COREMA-PB069) | 1.029 | −10 | 0 | 0 | 137.3 | 29.9 | 0 | 0 | PQ | On |
| Buses and PV Generators | Module | Angle | Generation | Load | Shunt | Type | Status | ||
|---|---|---|---|---|---|---|---|---|---|
| (pu) | (°) | (MW) | (MVAR) | (MW) | (MVAR) | (MVAR) | |||
| 5621 (COREMA-PB230) | 1.09 | −11.3 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5411 (MILAGR-CE230) | 1.032 | −13.9 | 0 | 0 | 0 | 0 | −21.3 | PQ | On |
| 5401 (BOM NOME-PE230) | 1.020 | −15.7 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5959 (RIOALT-PB034) | 0.994 | 0.6 | 0 | 0 | 0 | 0 | −1.4 | PQ | On |
| 5408 (MILAGR-CE500) | 1.061 | −13 | 0 | 0 | 0 | 0 | −112.3 | PQ | On |
| 5050 (L.GONZ-PE500) | 1.074 | −12.3 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5001 (P.AFON-BA500) | 1.073 | −11.9 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5623 (COREMA-PB069) | 1.027 | −13.6 | 0 | 0 | 137.3 | 29.9 | 0 | PQ | On |
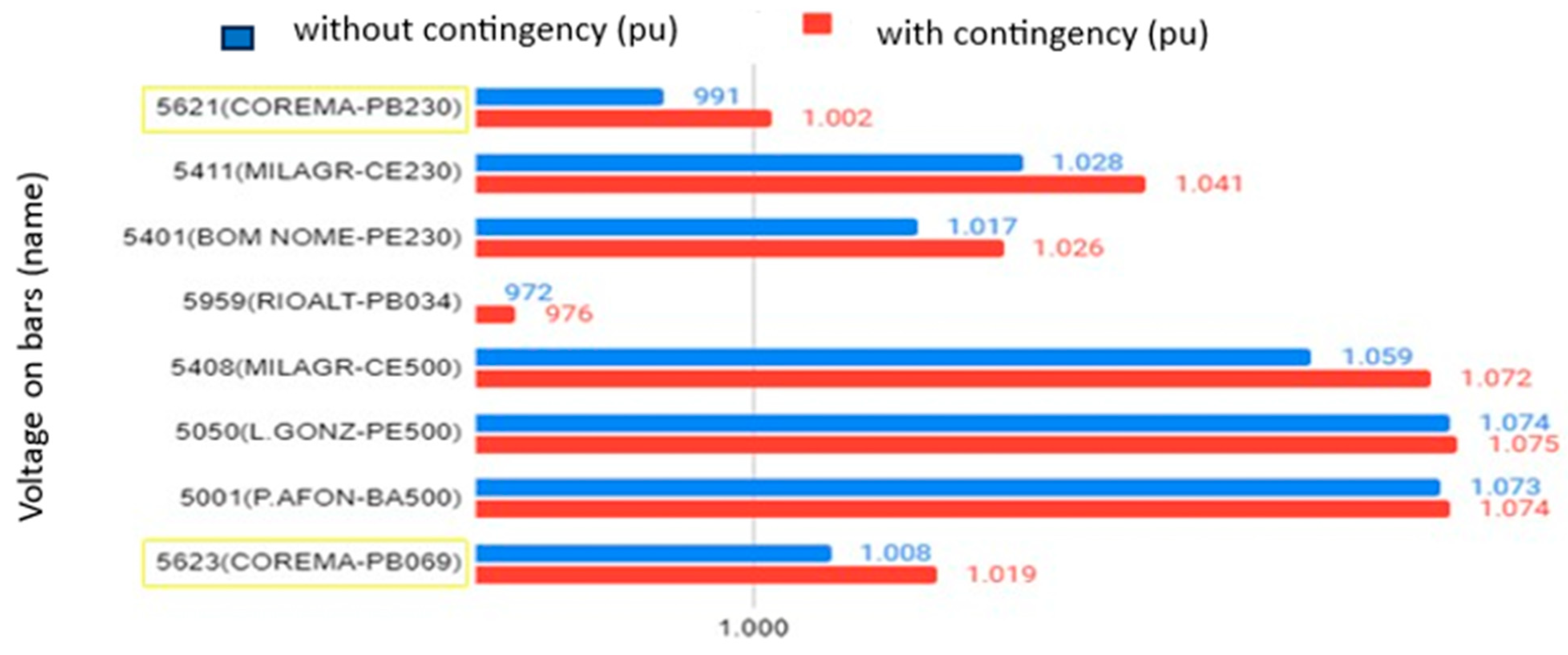
| Buses | Module | Angle | Generation | Load | Shunt | Type | Status | ||
|---|---|---|---|---|---|---|---|---|---|
| (pu) | (°) | (MW) | (MVAR) | (MW) | (MVAR) | (MVAR) | |||
| 5621 (COREMA-PB230) | 0.991 | −4.7 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5411 (MILAGR-CE230) | 1.028 | −10.9 | 0 | 0 | 0 | 0 | −21.1 | PQ | On |
| 5401 (BOM NOME-PE230) | 1.017 | −12.8 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5959 (RIOALT-PB034) | 0.972 | 15.3 | 0 | 0 | 0 | 0 | −1.3 | PQ | On |
| 5408 (MILAGR-CE500) | 1.059 | −10.2 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5050 (L.GONZ-PE500) | 1.074 | −9.9 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5001 (P.AFON-BA500) | 1.073 | −9.4 | 0 | 0 | 0 | 0 | 0 | PQ | On |
| 5623 (COREMA-PB069) | 1.008 | −7.1 | 0 | 0 | 137.3 | 29.9 | 0 | PQ | On |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lobo, F.X.; de Negreiros, G.F.; Torres, I.C.; Tiba, C. Evaluation of Voltage Variations Arising from the Increase in Photovoltaic Insertion in the Transmission Network: Case Study Coremas-PB Power Plant. Energies 2023, 16, 7310. https://doi.org/10.3390/en16217310
Lobo FX, de Negreiros GF, Torres IC, Tiba C. Evaluation of Voltage Variations Arising from the Increase in Photovoltaic Insertion in the Transmission Network: Case Study Coremas-PB Power Plant. Energies. 2023; 16(21):7310. https://doi.org/10.3390/en16217310
Chicago/Turabian StyleLobo, Fábio Xavier, Gustavo Fernandes de Negreiros, Igor Cavalcante Torres, and Chigueru Tiba. 2023. "Evaluation of Voltage Variations Arising from the Increase in Photovoltaic Insertion in the Transmission Network: Case Study Coremas-PB Power Plant" Energies 16, no. 21: 7310. https://doi.org/10.3390/en16217310
APA StyleLobo, F. X., de Negreiros, G. F., Torres, I. C., & Tiba, C. (2023). Evaluation of Voltage Variations Arising from the Increase in Photovoltaic Insertion in the Transmission Network: Case Study Coremas-PB Power Plant. Energies, 16(21), 7310. https://doi.org/10.3390/en16217310








