Numerical Study on Cavitating Flow-Induced Pressure Fluctuations in a Gerotor Pump
Abstract
:1. Introduction
2. Computational Objects and Numerical Simulation Methods
2.1. Computational Model
2.2. Meshing and Boundary Condition Settings
2.3. Monitoring Points Setting
2.4. Control Equation and Calculation Method
3. Analysis of Calculation Results
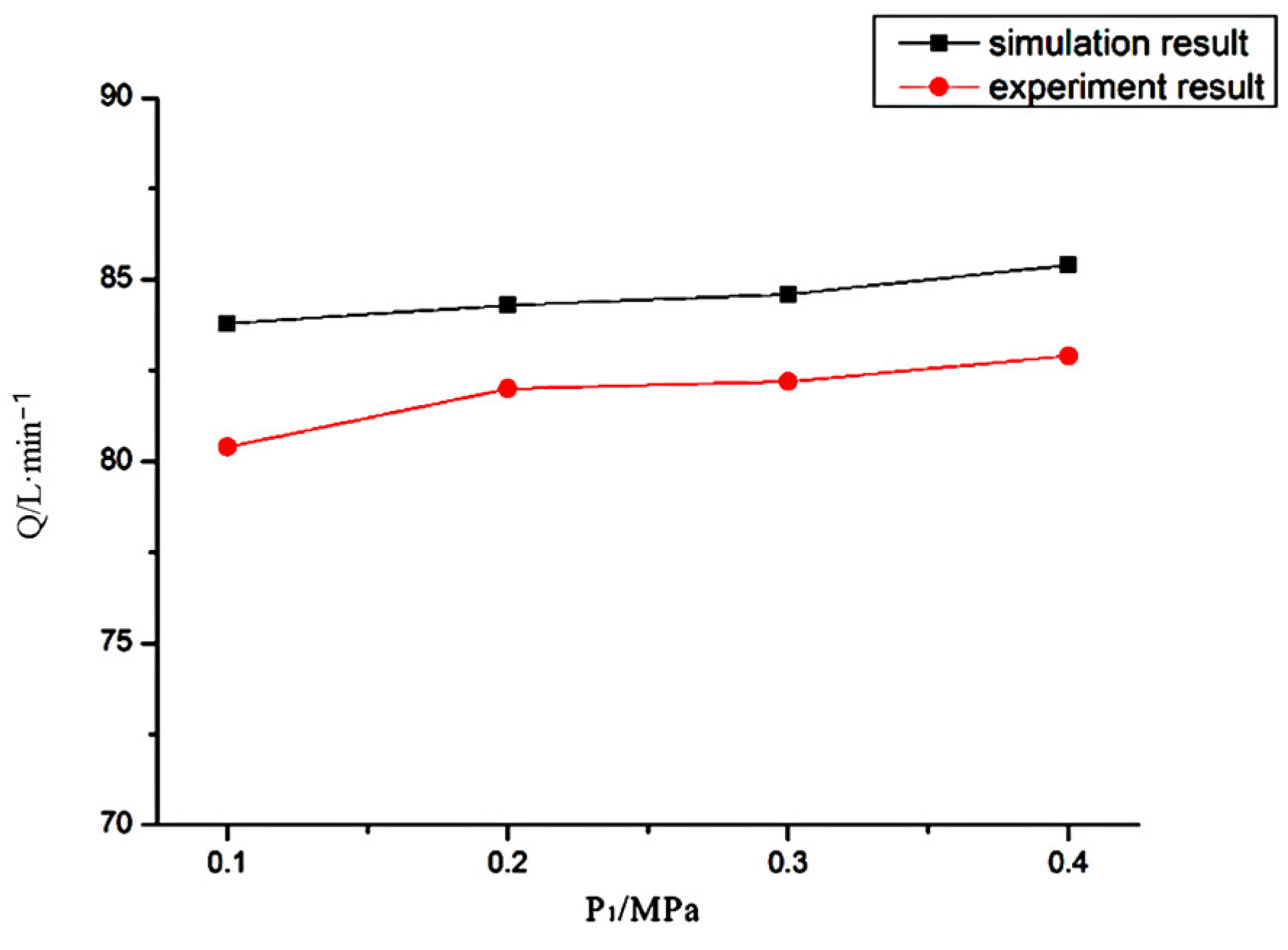
3.1. External Characteristic Analysis
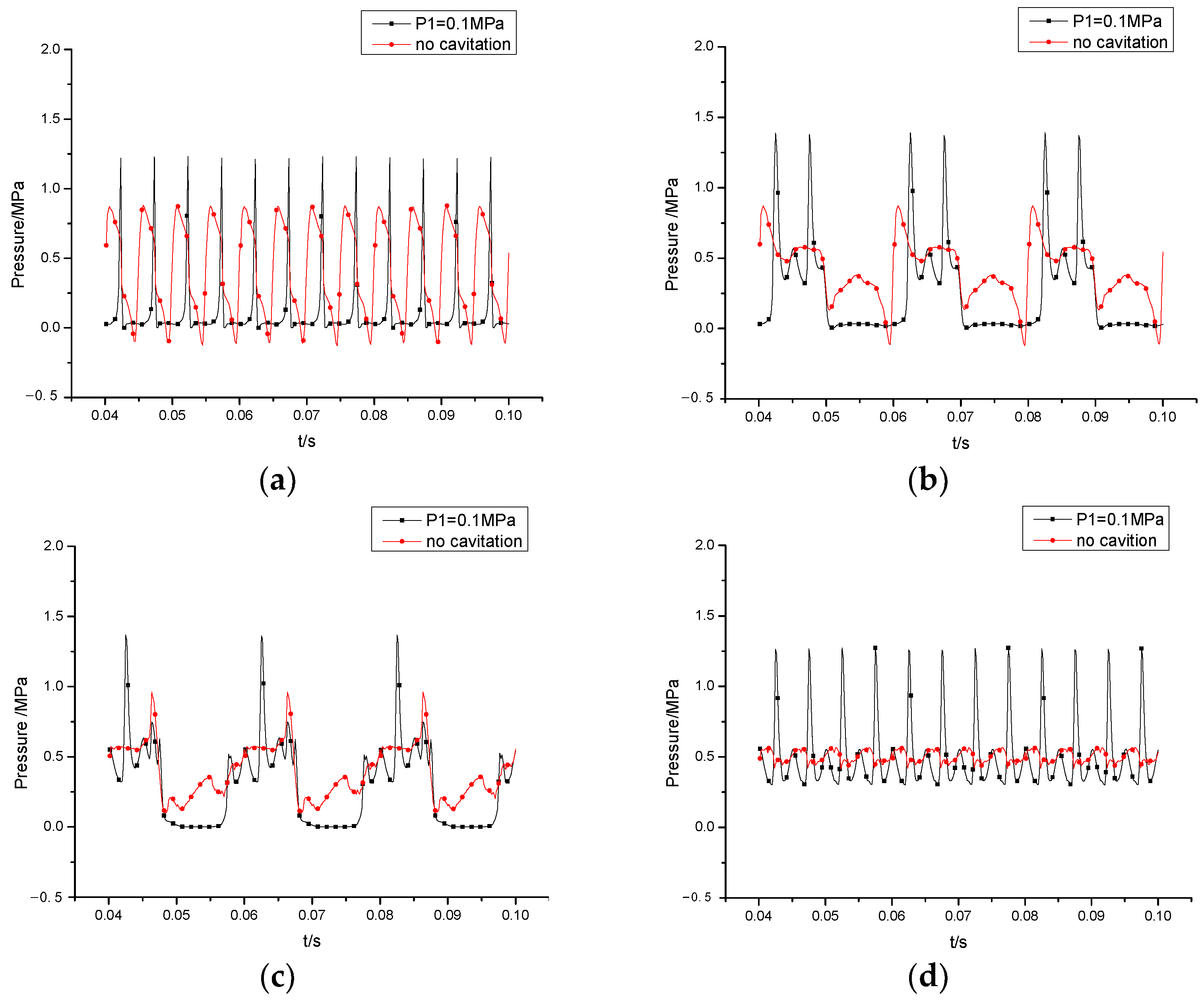
3.2. Effect of Cavitation on Pressure Fluctuation
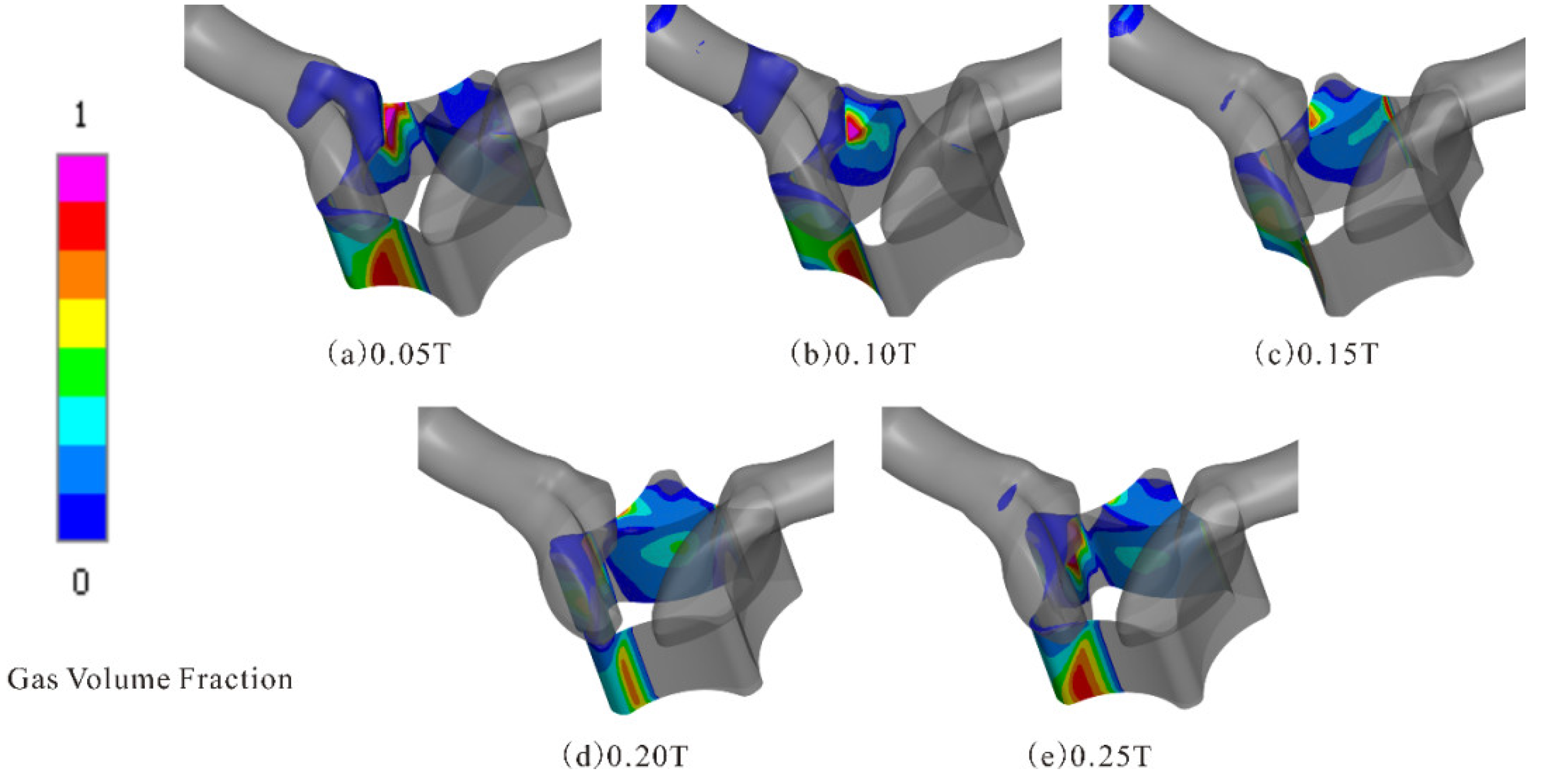
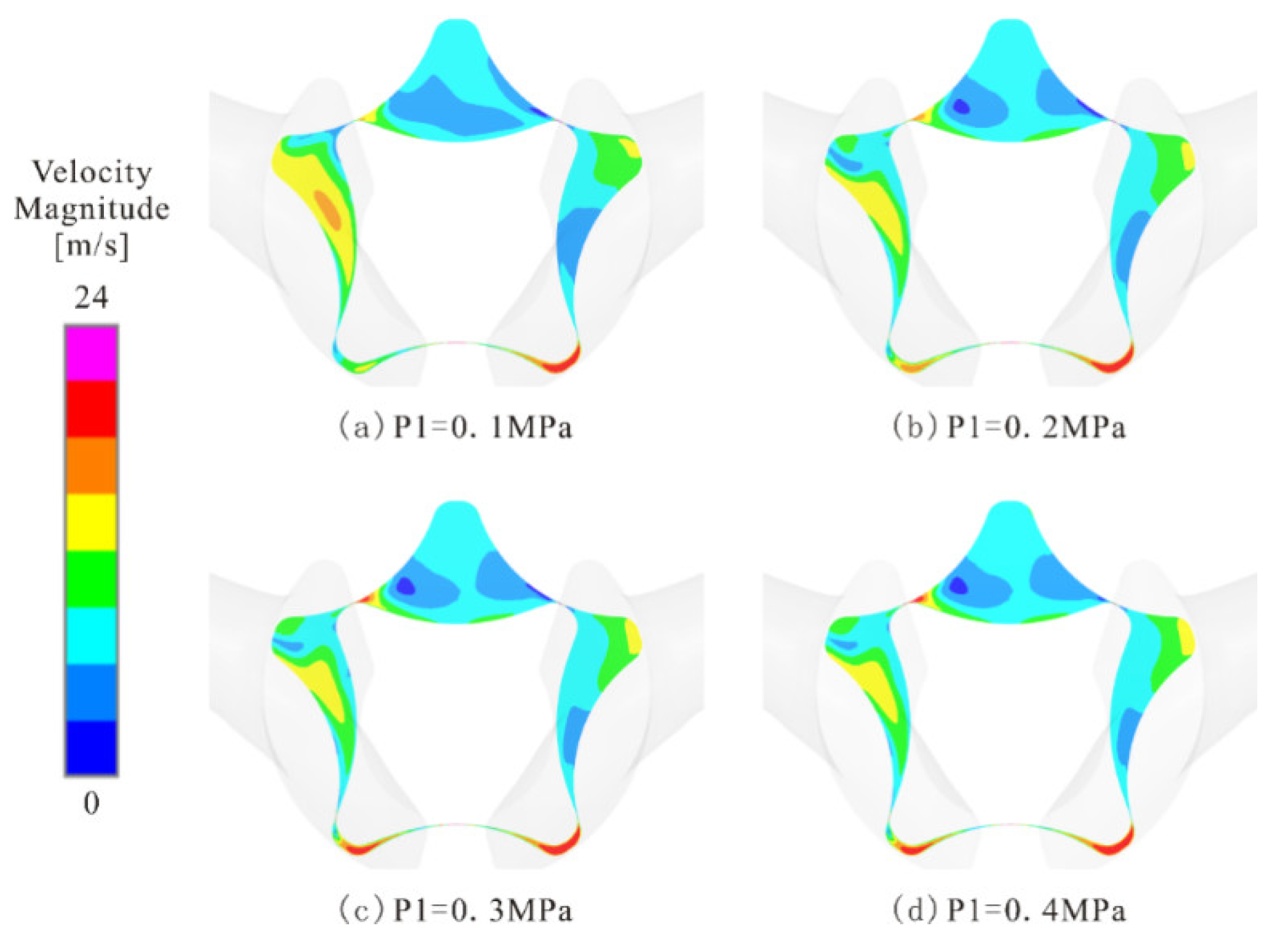
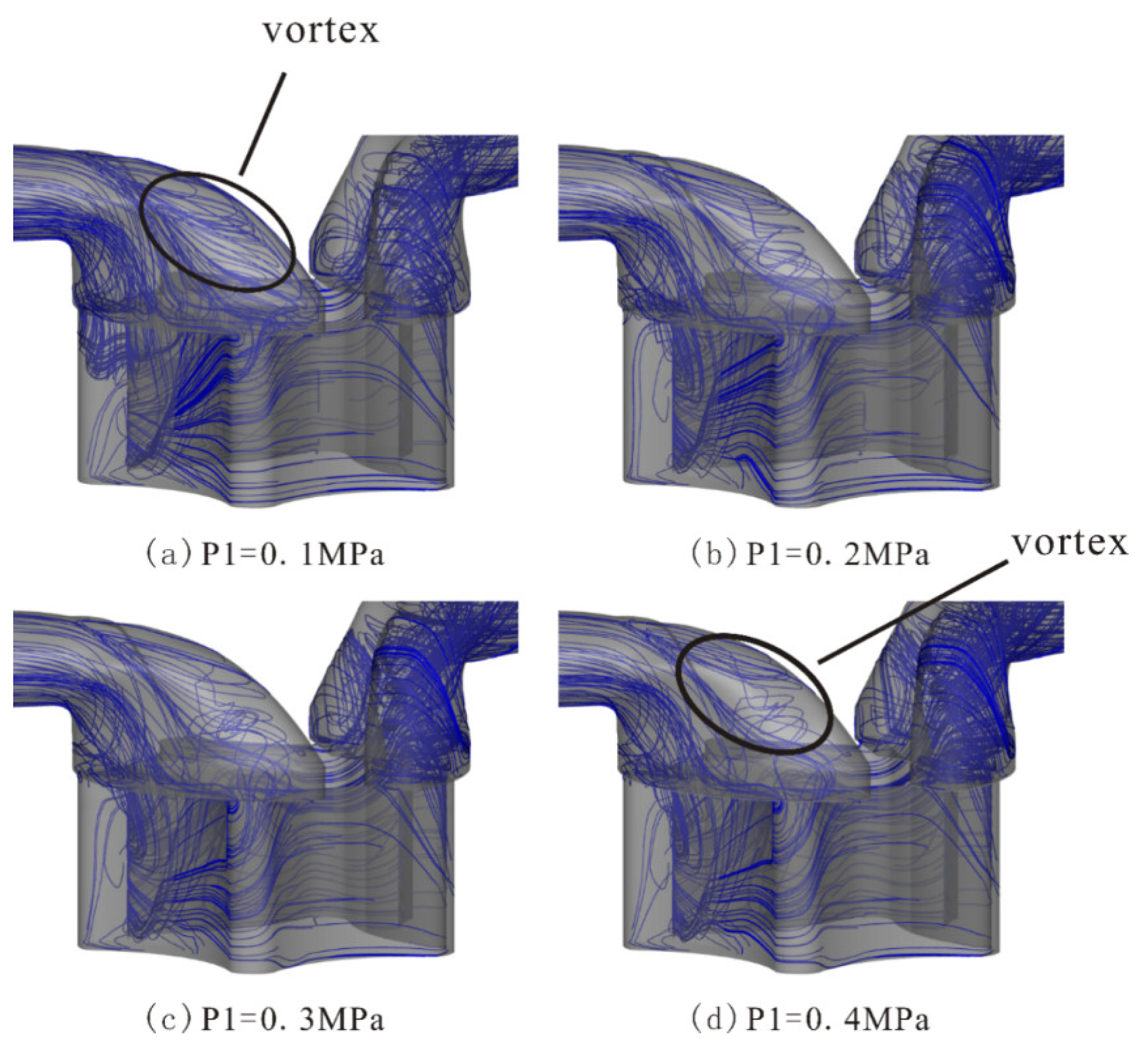
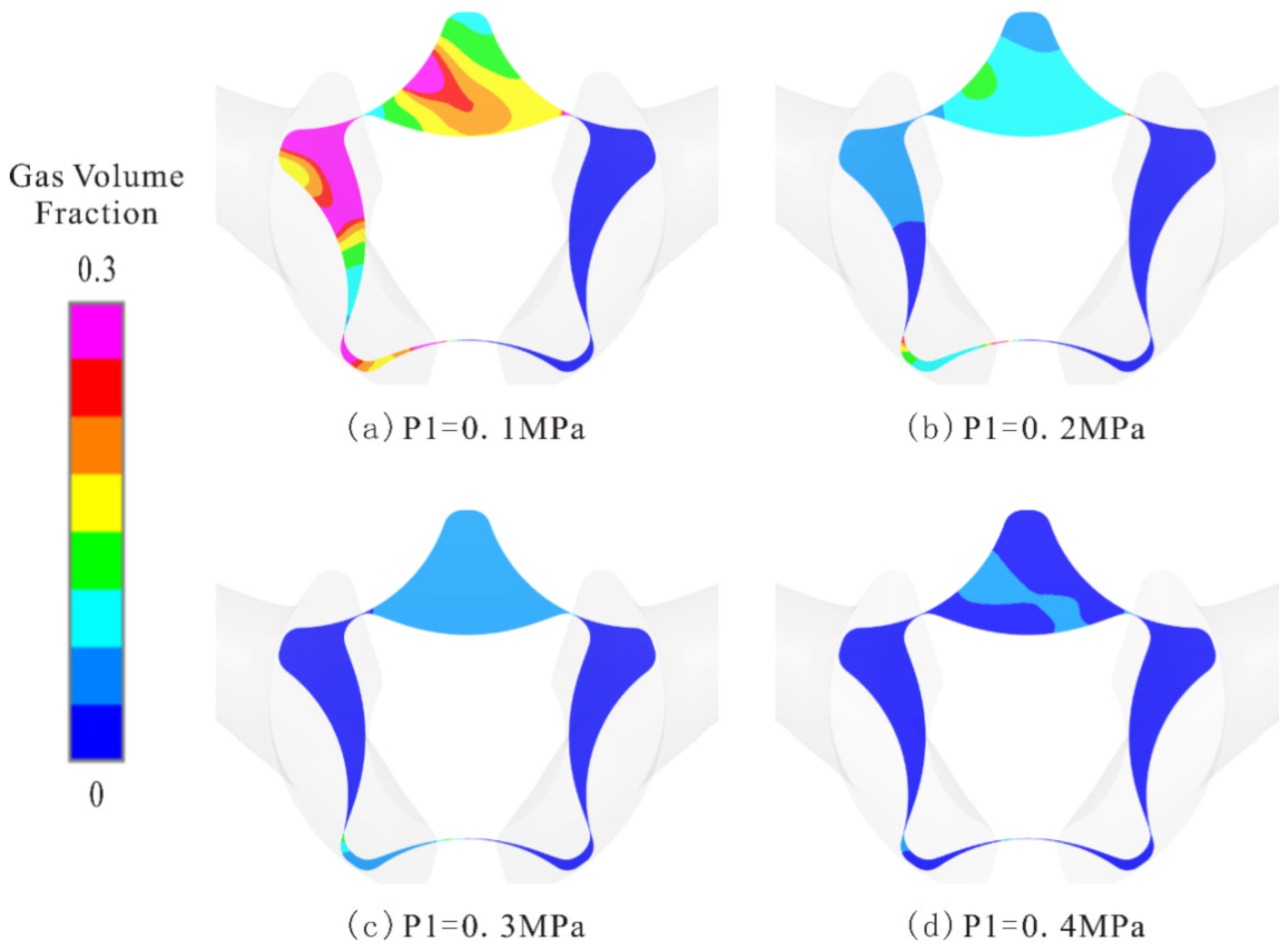
3.3. Unsteady Flow Analysis
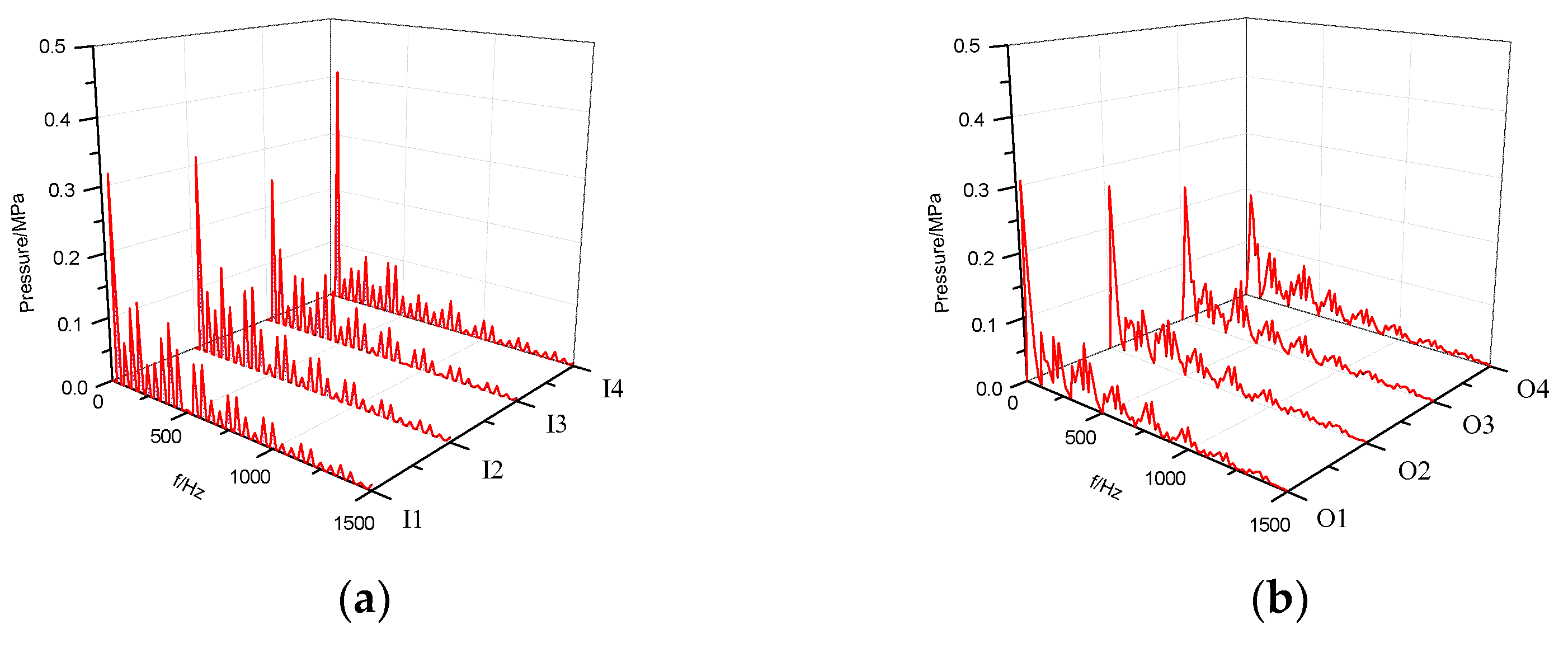
3.4. Analysis of Pressure Fluctuation at Different Positions
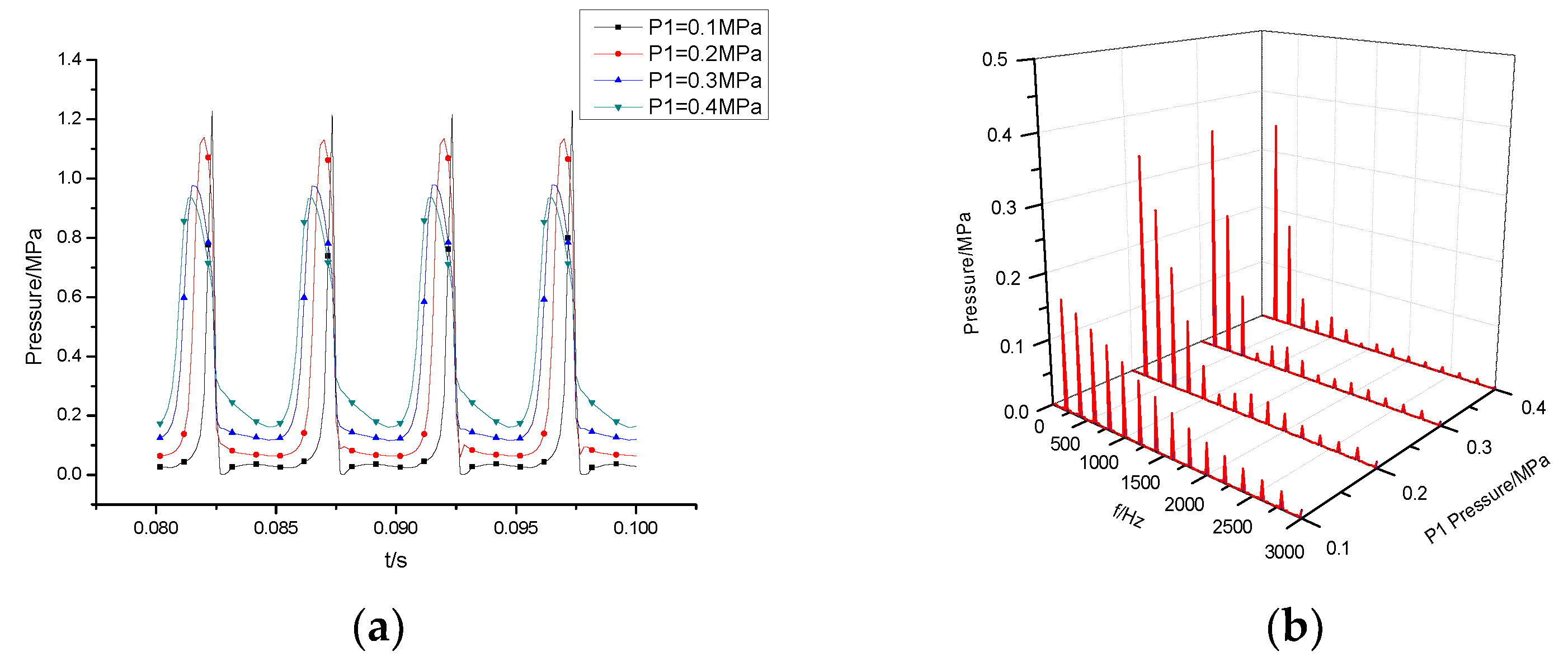
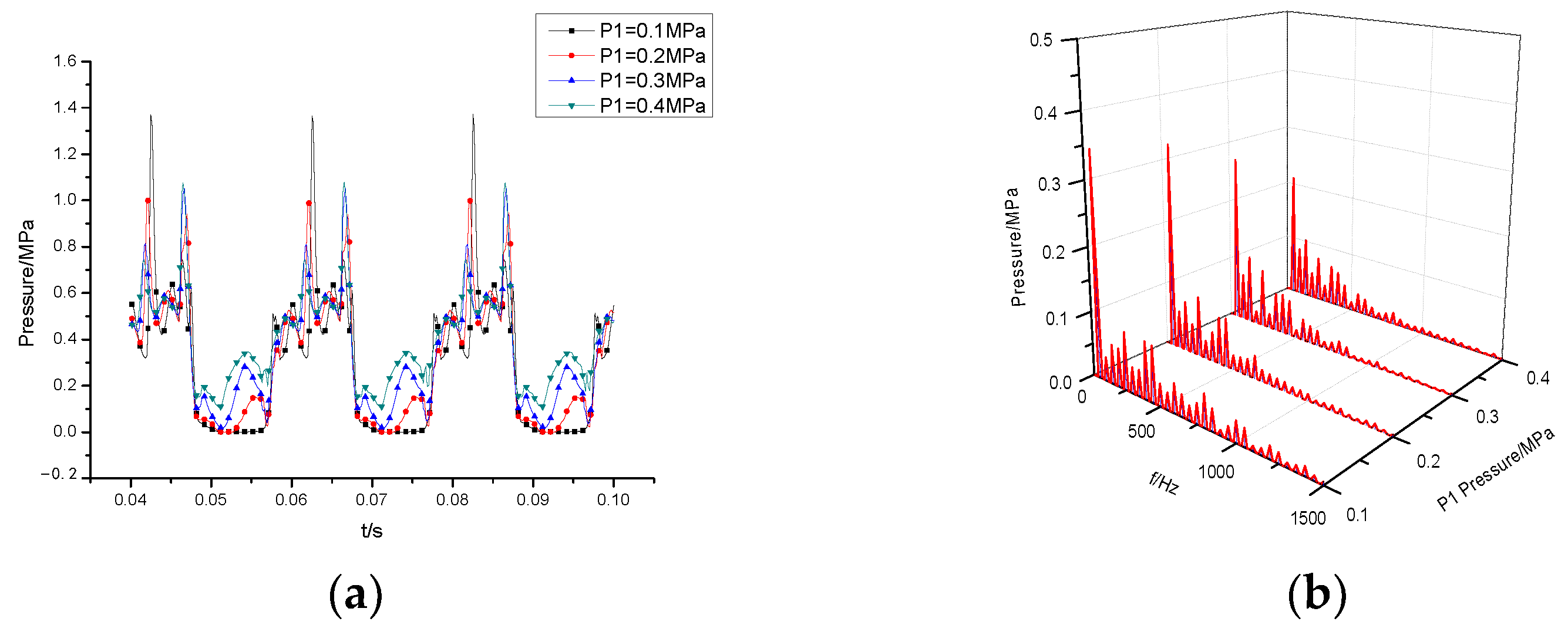
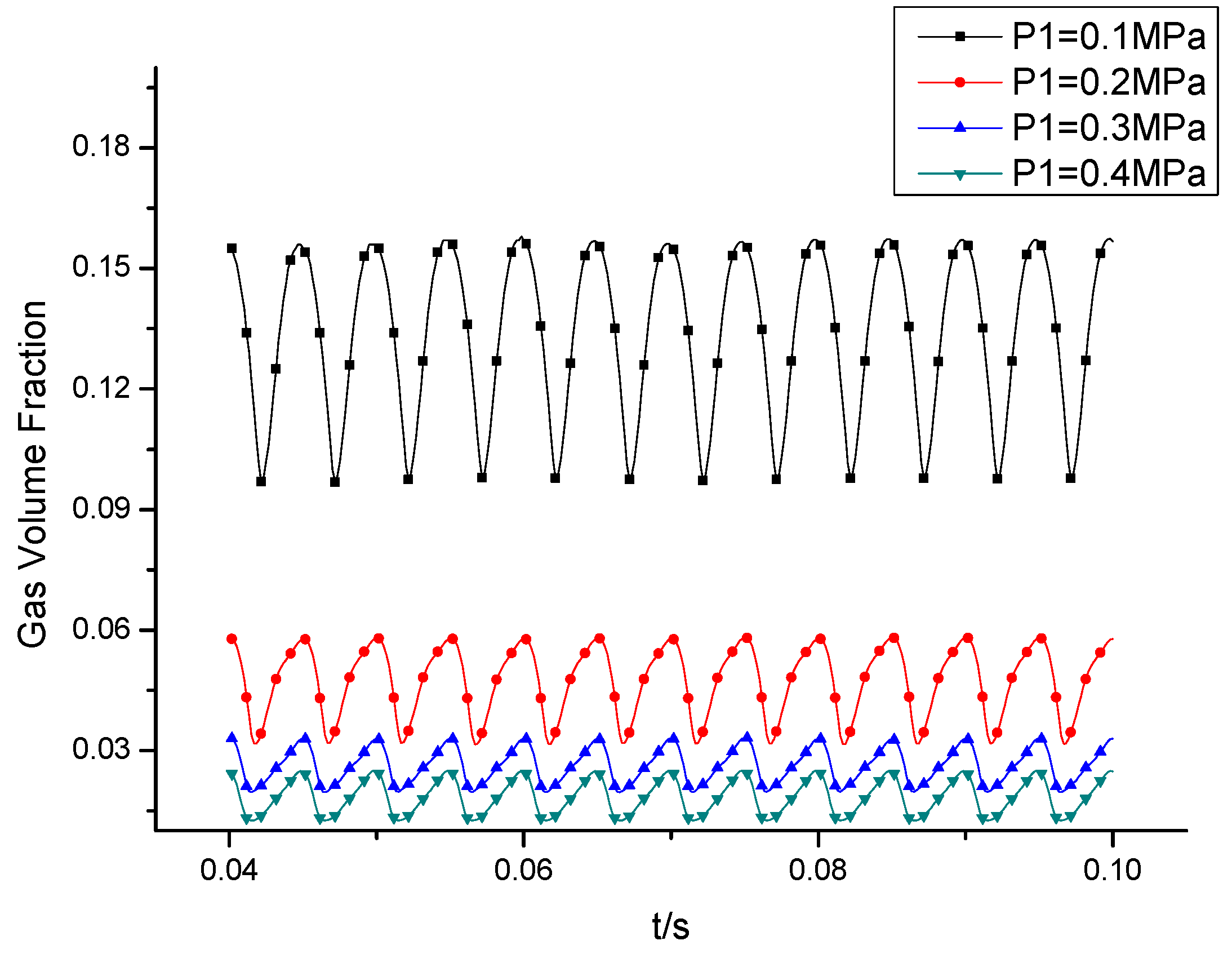
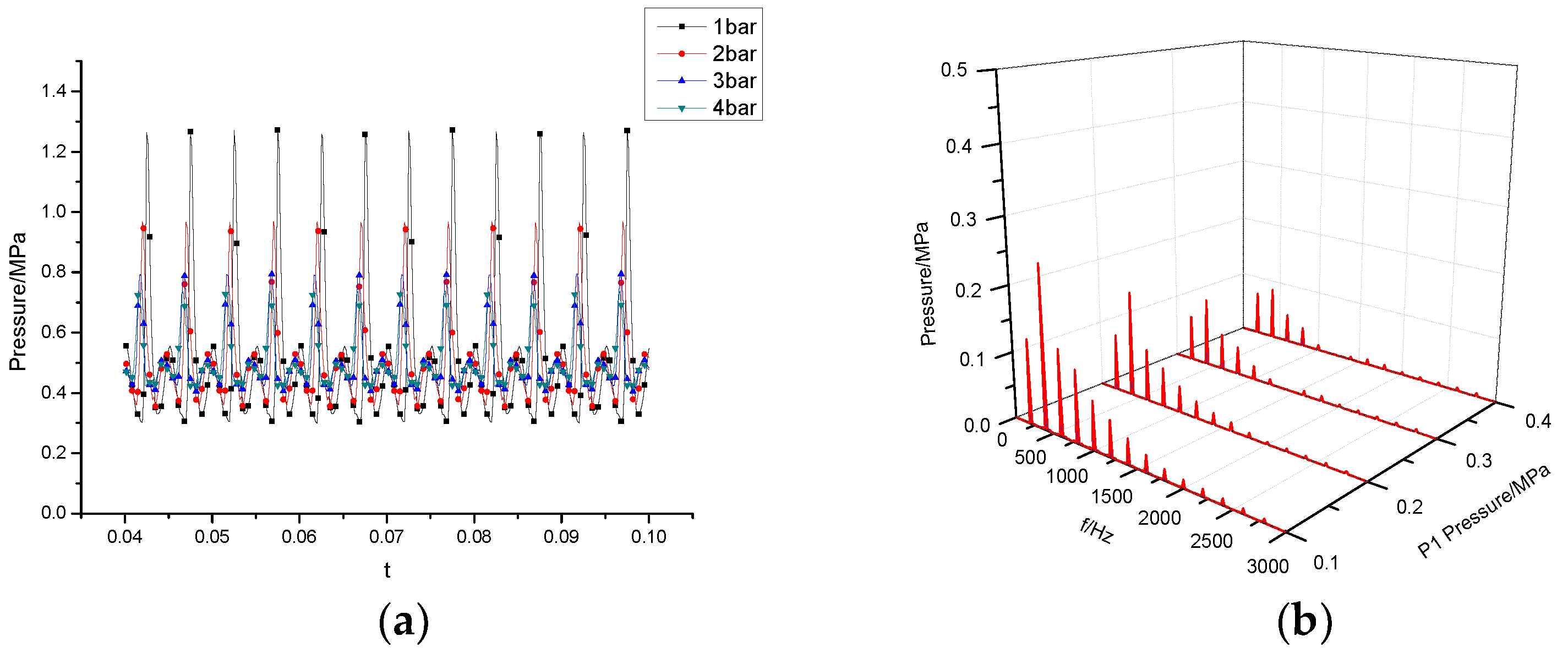
3.5. Analysis of Pressure Fluctuation under Different Cavitation Conditions
4. Conclusions
- (a)
- The range of cavitation in the rotor increases as the inlet pressure decreases, with vapor even spreading into the oil inlet groove. Unsteady flow analysis predicts the development characteristics of the cavity at an inlet pressure of P1 = 0.1 MPa. The results indicate that the vapor content in the interdental volume in contact with the oil inlet groove is higher and that the cavity collapses under compression, resulting in a greater pressure impact;
- (b)
- As the inlet pressure decreases, cavitation becomes more severe, and the pressure fluctuation caused by cavity collapse increases. The main frequency of pressure fluctuation is not affected by cavitation conditions. The main frequency of monitoring points on the rotor appears at the rotating frequency , and the bandwidth decreases with increasing inlet pressure. The main frequencies of the static monitoring points occur at blade passing frequency ;
- (c)
- The pressure fluctuation amplitude is strongest at point , which is located in the demarcation zone between the low-pressure zone and the high-pressure zone in the chamber. On the outer rotor side, the pressure fluctuation gradually decreases from the top to the root of the tooth, with the reduction increasing gradually. On the inner rotor side, the pressure fluctuation amplitude at point is the smallest, and the pressure fluctuation amplitude at point is the largest. The volume between the oil inlet groove and the oil outlet groove is the main vibration source in the rotor pump.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ham, J.; Kim, S.; Oh, J.; Cho, H. Theoretical Investigation of the effect of a relief groove on the performance of a gerotor oil pump. J. Mech. Sci. Technol. 2018, 32, 3687–3698. [Google Scholar] [CrossRef]
- Zhou, P.; Dai, J.; Li, Y.; Chen, T.; Mou, J. Unsteady flow structures in centrifugal pump under two types of stall conditions. J. Hydrodyn. 2018, 30, 1038–1044. [Google Scholar] [CrossRef]
- Pradhan, A.R.; Sahoo, V. Inter-chamber leakage flow through the transition contacts in epitrochoid generated star and ring hydrostatic units. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2023, 237, 1244–1263. [Google Scholar] [CrossRef]
- Zhou, P.; Dai, J.; Yan, C.; Zheng, S.; Ye, C.; Zhang, X. Effect of stall cells on pressure fluctuations characteristics in a centrifugal pump. Symmetry 2019, 11, 1116. [Google Scholar] [CrossRef]
- Ye, C.; Tang, Y.; An, D.; Wang, F.; Zheng, Y.; van Esch, B.P.M. Investigation on stall characteristics of marine centrifugal pump considering transition effect. Ocean. Eng. 2023, 280, 114823. [Google Scholar] [CrossRef]
- Zhang, S.; Yao, Z.; Wu, H.; Zhong, Q.; Tao, R.; Wang, F. A new turbulent viscosity correction model with URANS solver for unsteady turbulent cavitation flow computations. J. Fluids Eng. 2022, 144, 091403. [Google Scholar] [CrossRef]
- Zhou, W.; Zhou, P.; Xiang, C.; Wang, Y.; Mou, J.; Cui, J. A review of bionic structures in control of aerodynamic noise of centrifugal fans. Energies 2023, 16, 4331. [Google Scholar] [CrossRef]
- Jacazio, G.; De Martin, A. Influence of rotor profile geometry on the performance of an original low-pressure gerotor pump. Mech. Mach. Theory. 2016, 100, 296–312. [Google Scholar] [CrossRef]
- Zhang, D.; Perng, C.; Laverty, M. Gerotor oil pump performance and flow/pressure ripple study. SAE Trans. 2006, 115, 204–209. [Google Scholar]
- Suresh Kumar, M.; Manonmani, K. Numerical and experimental investigation of lubricating oil flow in a gerotor pump. Int. J. Automot. Technol. 2011, 12, 903–911. [Google Scholar] [CrossRef]
- Frosina, E.; Senatore, A.; Buono, D. A tridimensional CFD analysis of the oil pump of a high performance motorbike engine. Energy Procedia 2014, 45, 938–948. [Google Scholar] [CrossRef]
- Sung, H.J.; Min, H.K.; Nam, Y.J.; Park, M.K. Design and experimental verification of a port plate in a gerotor pump to reduce pressure pulsation. J. Mech. Sci. Technol. 2018, 32, 671–678. [Google Scholar] [CrossRef]
- Kwak, H.S.; Li, S.H.; Kim, C. Performance improvement of an oil pump: Design of port assembled with gerotor (2-ellipses-combined lobe). Int. J. Precis. Eng. Manuf. 2016, 17, 1017–1024. [Google Scholar] [CrossRef]
- Kim, S.Y.; Nam, Y.J.; Park, M.K. Design of port plate in gerotor pump for reduction of pressure pulsation. J. Mech. Sci. Technol. 2006, 20, 1626–1637. [Google Scholar] [CrossRef]
- Zhou, J.; Vacca, A.; Casoli, P. A novel approach for predicting the operation of external gear pumps under cavitating conditions. Simul. Model. Pract. Ther. 2014, 45, 35–49. [Google Scholar] [CrossRef]
- Ivanović, L.; Matejić, M. Improving gerotor pump performance trough design, modeling and simulation. Int. J. Fluid Power 2020, 327–346. [Google Scholar] [CrossRef]
- Buono, D.; Cola, F.D.S.D.; Senatore, A. Modelling approach on a gerotor pump working in cavitation conditions. Energy Procedia 2016, 101, 701–709. [Google Scholar] [CrossRef]
- Rituraj, R.; Vacca, A. Investigation of flow through curved constrictions for leakage flow modelling in hydraulic gear pumps. Mech. Syst. Signal Process. 2021, 153, 107503. [Google Scholar] [CrossRef]
- Tian, S.; Zhao, Y.; Dong, K.; Liu, G.; Yang, Q.; Li, L. Internal flow and cavitation analysis of scroll oil pump by CFD method. Processes 2021, 9, 1705. [Google Scholar] [CrossRef]
- Ivanovic, L.; Devedzic, G.; Cukovic, S.; Miric, N. Modeling of the meshing of trochoidal profiles with clearances. J. Mech. Des. 2012, 134, 041003. [Google Scholar] [CrossRef]
- Cao, W.; Liu, Y.; Dong, J.; Niu, Z.; Shi, Y. Research on pressure pulsation characteristics of gerotor pump for active vibration damping system. IEEE Access 2019, 7, 116567–116577. [Google Scholar] [CrossRef]
- Li, Z.H.; Liu, Z.X.; Lv, Y.G.; Li, G.Q. Numerical investigation on altitude performance for aeroengine gerotor pump. J. Propul. Technol. 2015, 36, 846–851. [Google Scholar]
- Gamez-Montero, P.J.; Codina, E.; Castilla, R. A review of gerotor technology in hydraulic machines. Energies 2019, 12, 2423. [Google Scholar] [CrossRef]
- Ivanovic, L.; Velickovic, S.; Stojanovic, B.; Kandeva, M.; Jakimovska, K. The selection of optimal parameters of gerotor pump by application of factorial experimental design. FME Trans. 2017, 45, 159–164. [Google Scholar] [CrossRef]
- Wu, T.; Wu, D.; Ren, Y.; Song, Y.; Gu, Y.; Mou, J. Multi-objective optimization on diffuser of multistage centrifugal pump base on ANN-GA. Struct. Multidisc. Optim. 2022, 65, 182. [Google Scholar] [CrossRef]
- Xu, M.; Zeng, G.; Wu, D.; Mou, J.; Zhao, J.; Zheng, S.; Huang, B.; Ren, Y. Structural optimization of jet fish pump design based on a multi-objective genetic algorithm. Energies 2022, 15, 4104. [Google Scholar] [CrossRef]
- Wang, H.; Zhou, P.; Chen, T.; Mou, J.; Cui, J.; Zhang, H. Optimization of liquid−liquid mixing in a novel mixer based on hybrid SVR-DE model. Energies 2023, 16, 1808. [Google Scholar] [CrossRef]
- Huang, R.; Zhang, Z.; Zhang, W.; Mou, J.; Zhou, P. Energy performance prediction of the centrifugal pumps by using a hybrid neural network. Energy 2020, 213, 119005. [Google Scholar] [CrossRef]
- Luo, H.; Zhou, P.; Shu, L.; Mou, J.; Zheng, H.; Jiang, C.; Wang, Y. Energy performance curves prediction of centrifugal pumps based on constrained PSO-SVR model. Energies 2022, 15, 3309. [Google Scholar] [CrossRef]
- Prosperetti, A. A generalization of the Rayleigh–Plesset equation of bubble dynamics. Phys. Fluids 1982, 25, 409–410. [Google Scholar] [CrossRef]
- Ashok, K.; Athavale, M.; Li, H.Y.; Jiang, Y. Mathematical basis and validation of the full cavitation model. J. Fluid. Eng. 2002, 124, 617–624. [Google Scholar]
- Yu, L.; Gu, Y.; Mou, J.; Wu, D.; Chen, Z.; Ren, Y.A. A numerical investigation on the characteristics of the radial force in a cycloid gerotor pump. Fluid Dyn. Mater. Proc. 2022, 16, 1007–1018. [Google Scholar] [CrossRef]
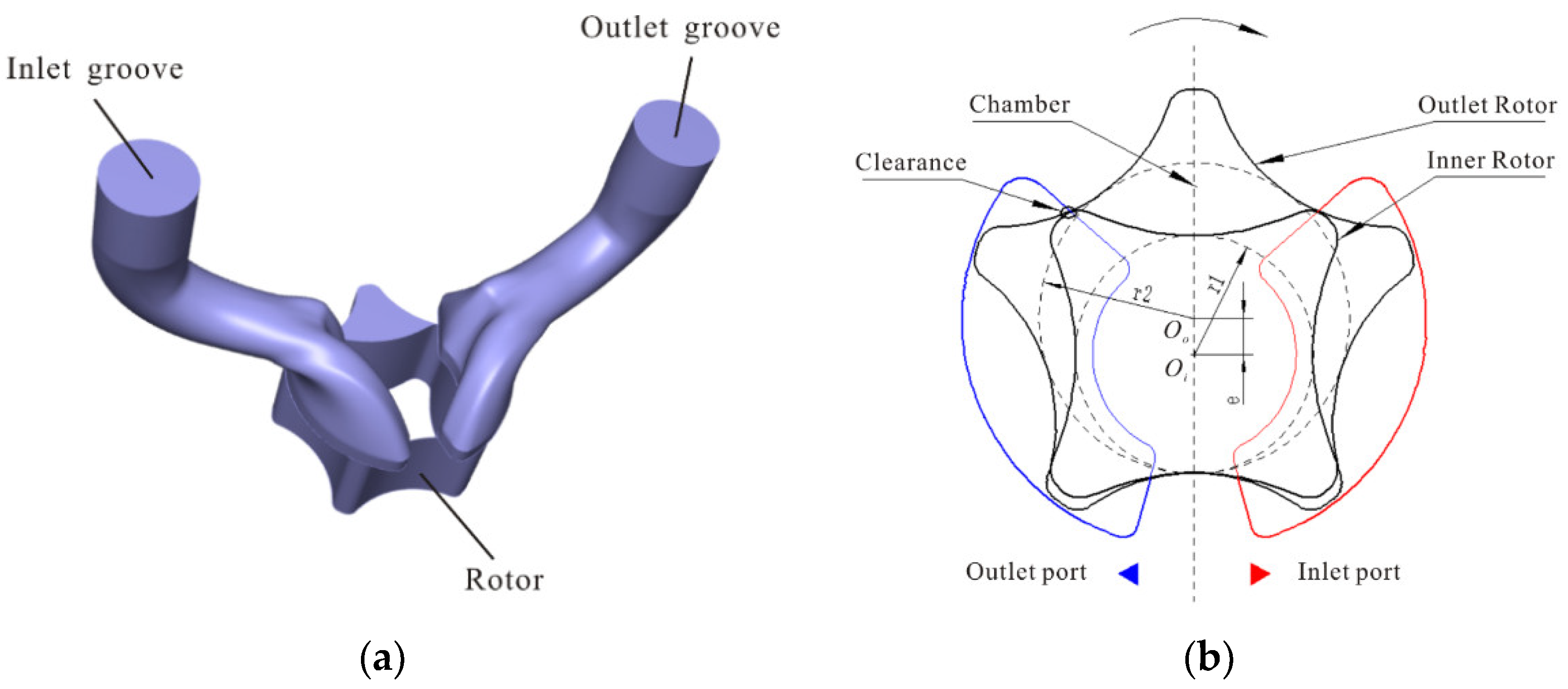
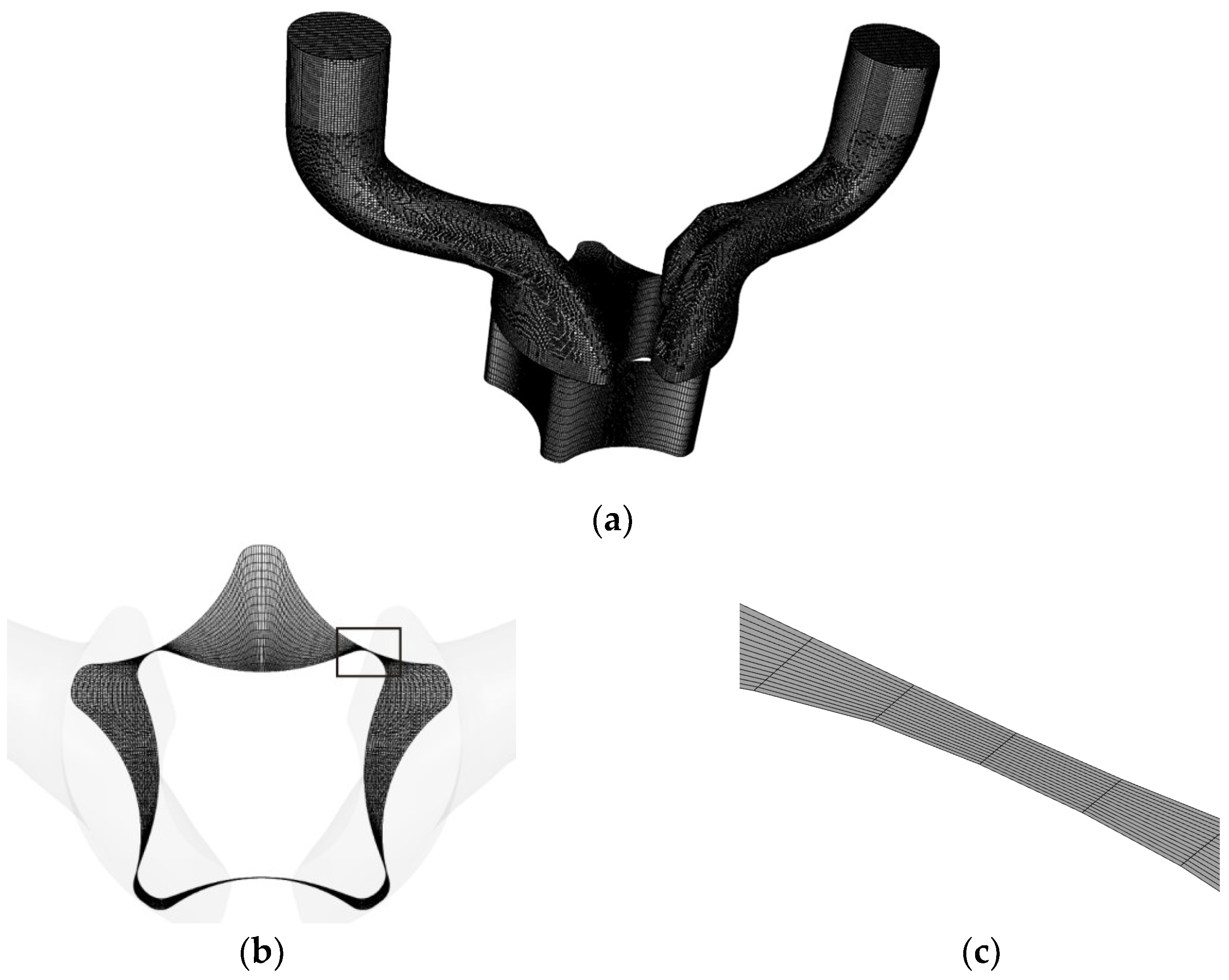

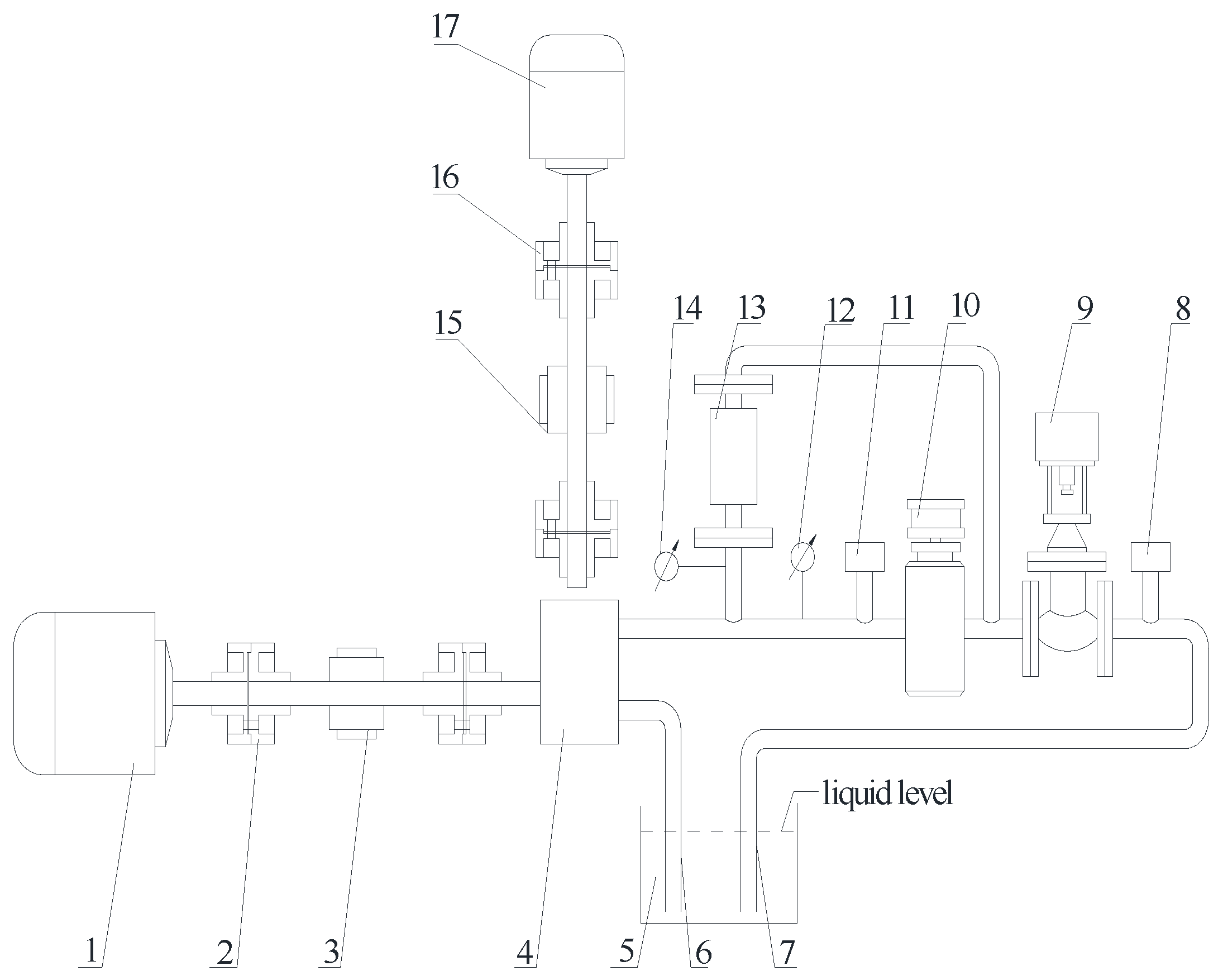
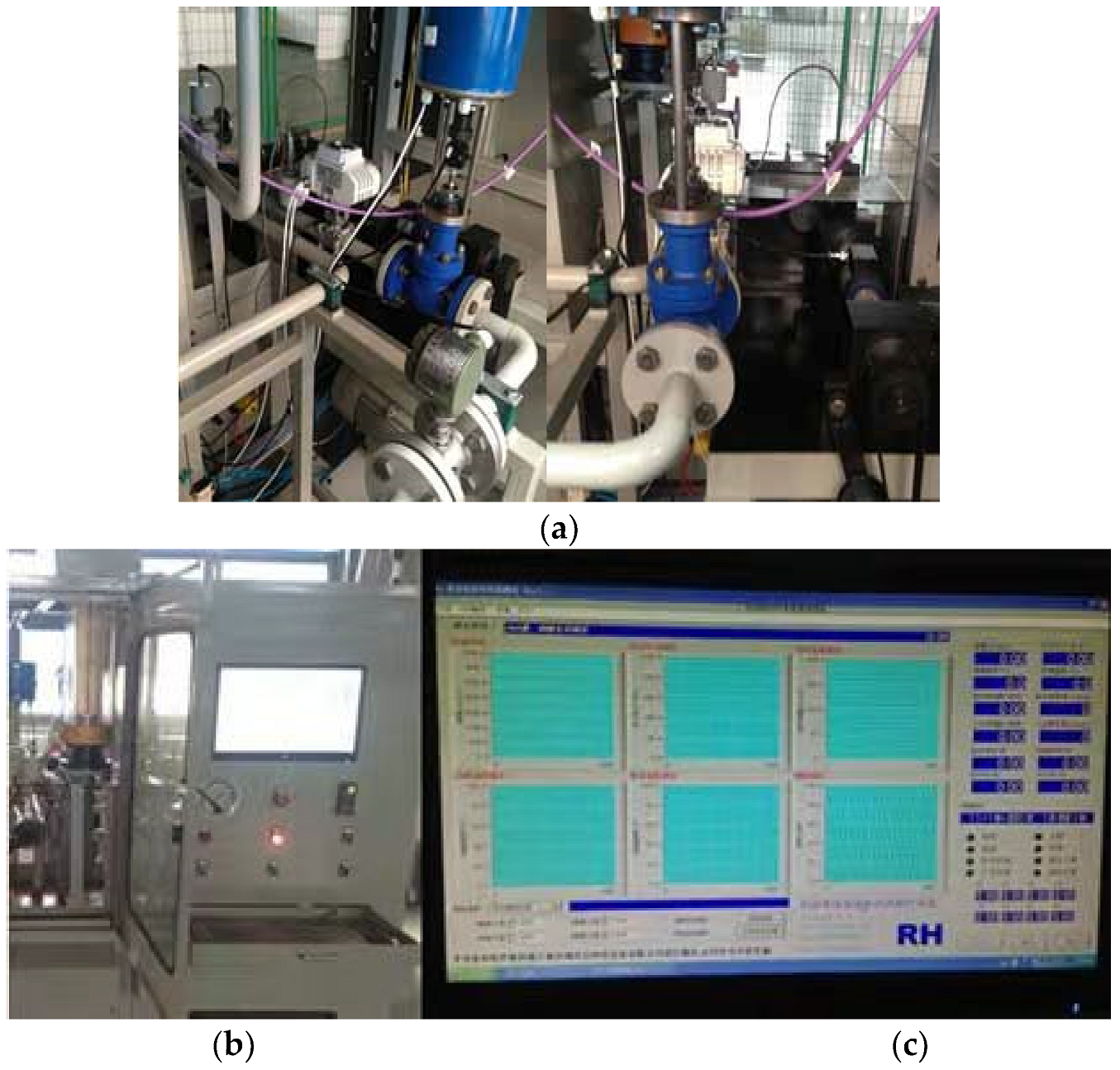




| External diameter of outer rotor (mm) | D2 = 69.7 |
| External diameter of inner rotor (mm) | D1 = 52.6 |
| Eccentricity (mm) | e = 4.95 |
| Gerotor height (mm) | B = 28 |
| Number of lobes in the outer rotor | Z2= 5 |
| Number of lobes in the inner rotor | Z1= 4 |
| Tip clearance (mm) | 0.05 |
| Number of Grids/10,000 | Simulation Value of Exit Flow /L·min−1 | Volumetric Efficiency/% |
|---|---|---|
| 20 | 96.34 | 87.28 |
| 30 | 97.25 | 88.04 |
| 40 | 98.87 | 89.39 |
| 50 | 98.92 | 89.43 |
| 60 | 98.73 | 89.28 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, P.; Cui, J.; Xiao, G.; Xiang, C.; Dai, J.; Zheng, S. Numerical Study on Cavitating Flow-Induced Pressure Fluctuations in a Gerotor Pump. Energies 2023, 16, 7301. https://doi.org/10.3390/en16217301
Zhou P, Cui J, Xiao G, Xiang C, Dai J, Zheng S. Numerical Study on Cavitating Flow-Induced Pressure Fluctuations in a Gerotor Pump. Energies. 2023; 16(21):7301. https://doi.org/10.3390/en16217301
Chicago/Turabian StyleZhou, Peijian, Jiayi Cui, Gang Xiao, Chun Xiang, Jiacheng Dai, and Shuihua Zheng. 2023. "Numerical Study on Cavitating Flow-Induced Pressure Fluctuations in a Gerotor Pump" Energies 16, no. 21: 7301. https://doi.org/10.3390/en16217301
APA StyleZhou, P., Cui, J., Xiao, G., Xiang, C., Dai, J., & Zheng, S. (2023). Numerical Study on Cavitating Flow-Induced Pressure Fluctuations in a Gerotor Pump. Energies, 16(21), 7301. https://doi.org/10.3390/en16217301






