Experimental Study of the Corrugation Profile Effect on the Local Heat Transfer Coefficient
Abstract
:1. Introduction
2. Experimental Setup
2.1. Pipe Geometries
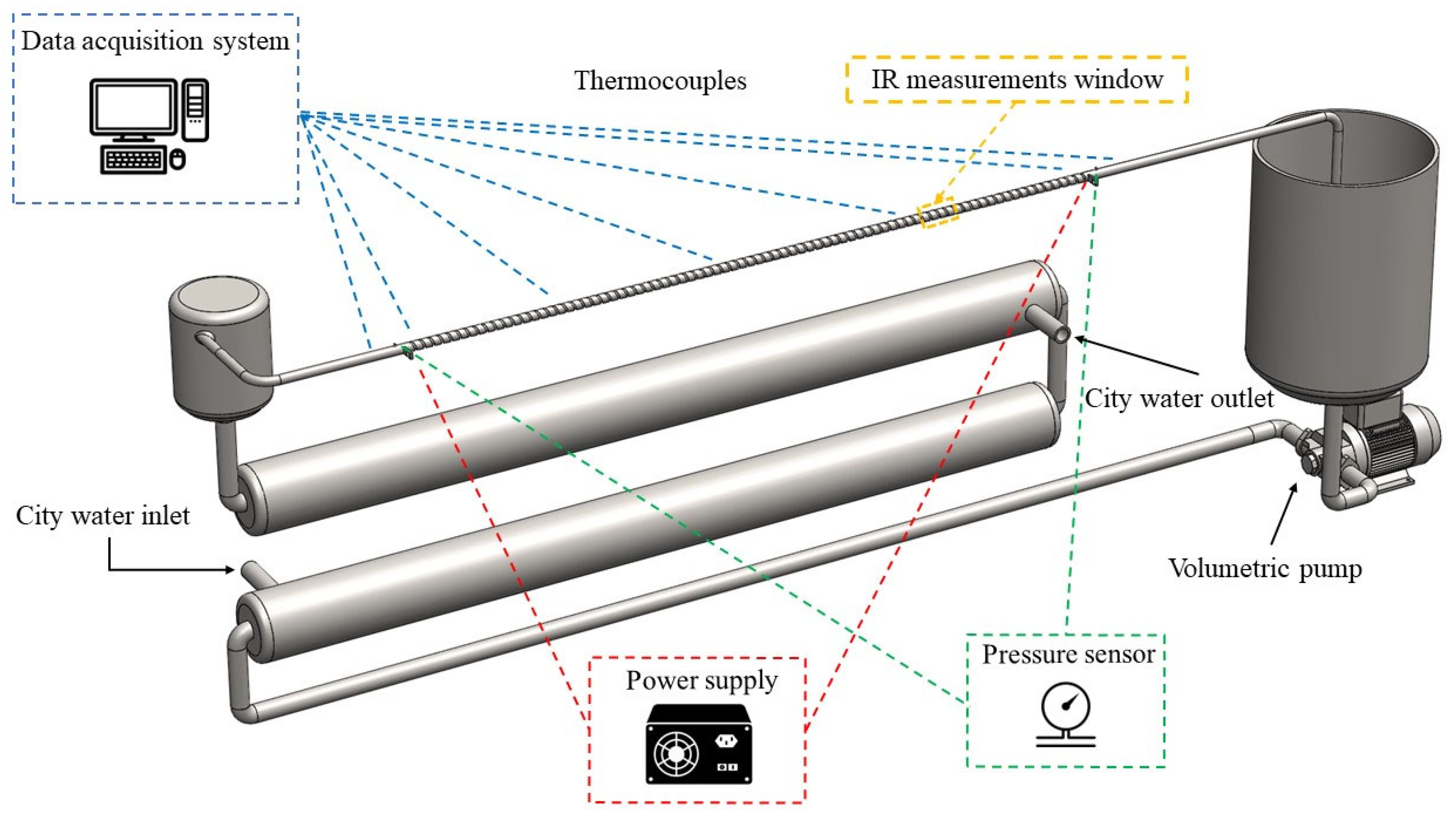
2.2. Test Circuit
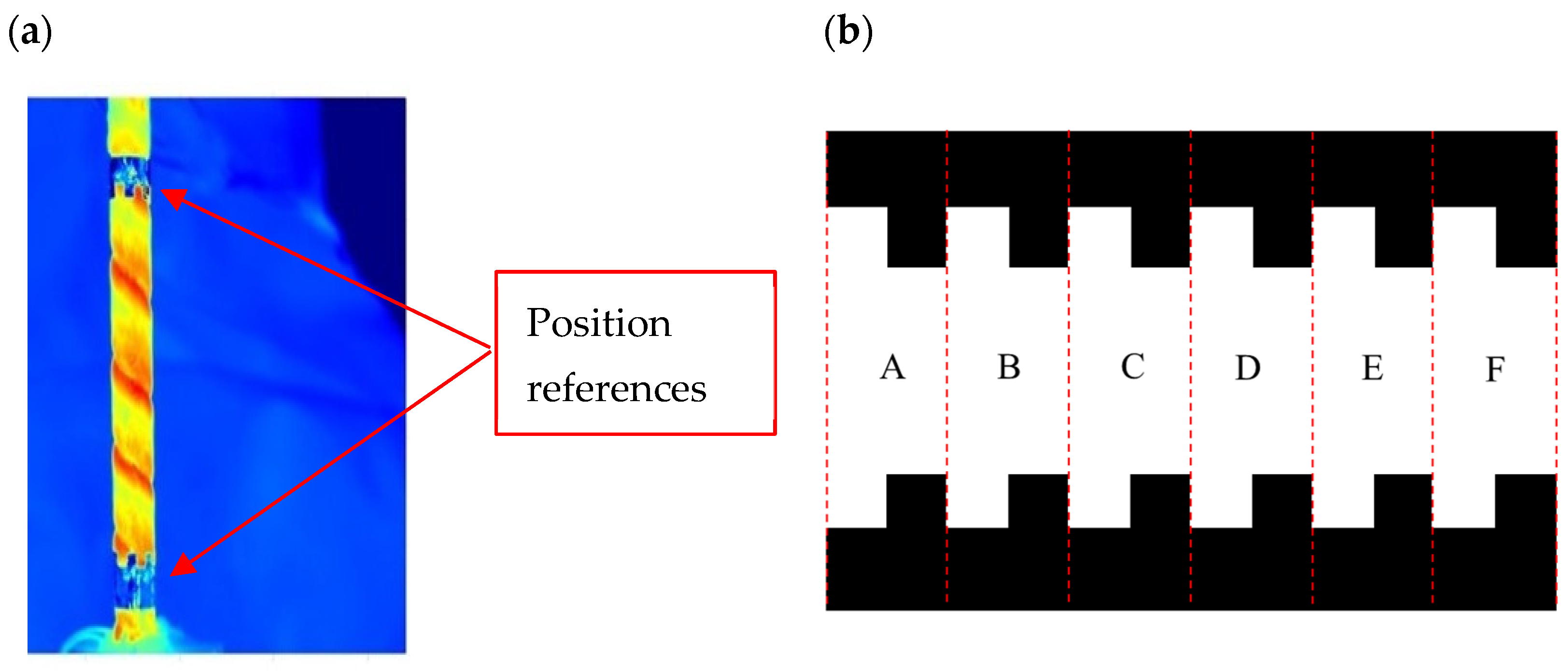
3. Estimation Procedure
4. Results
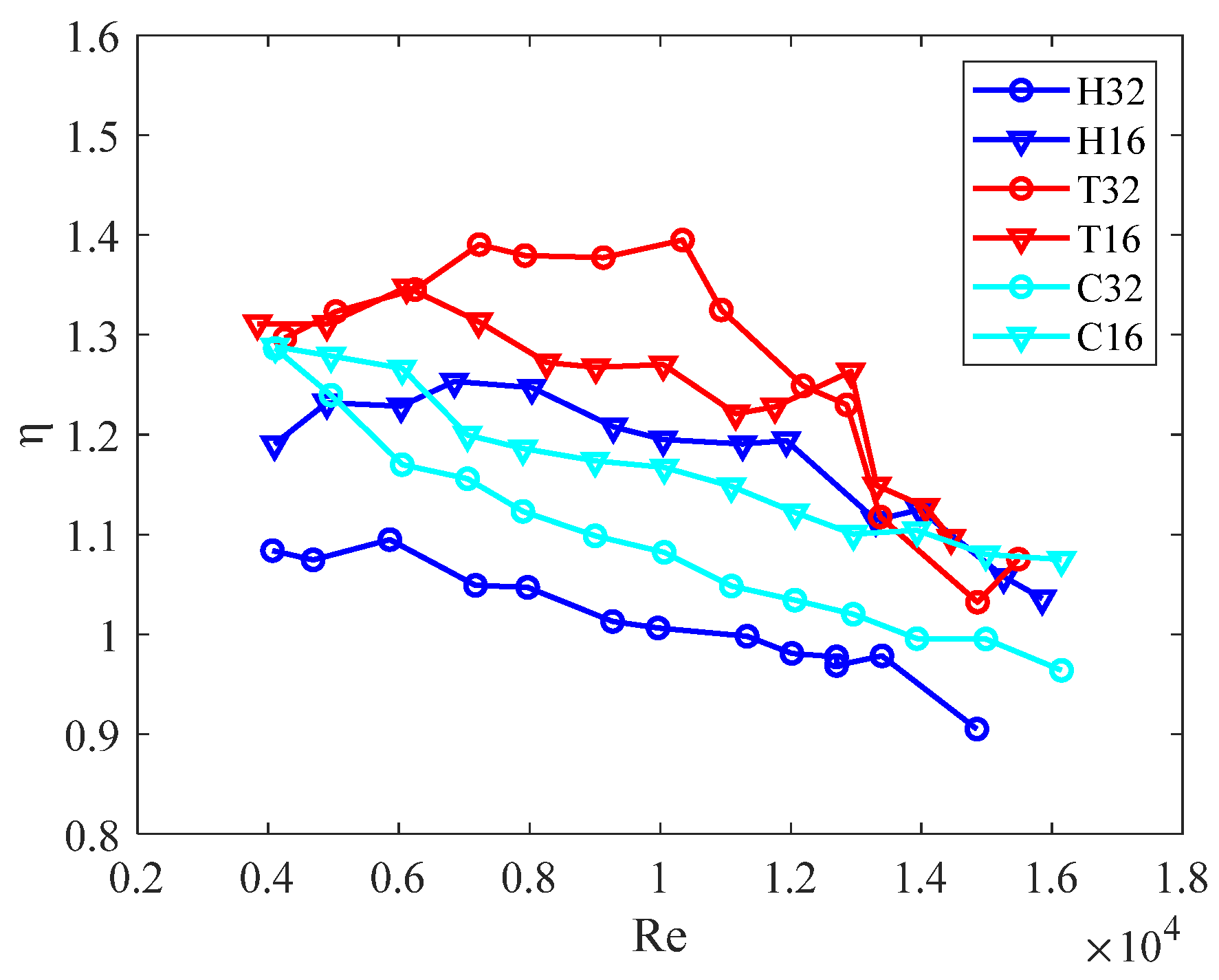
4.1. Average Results
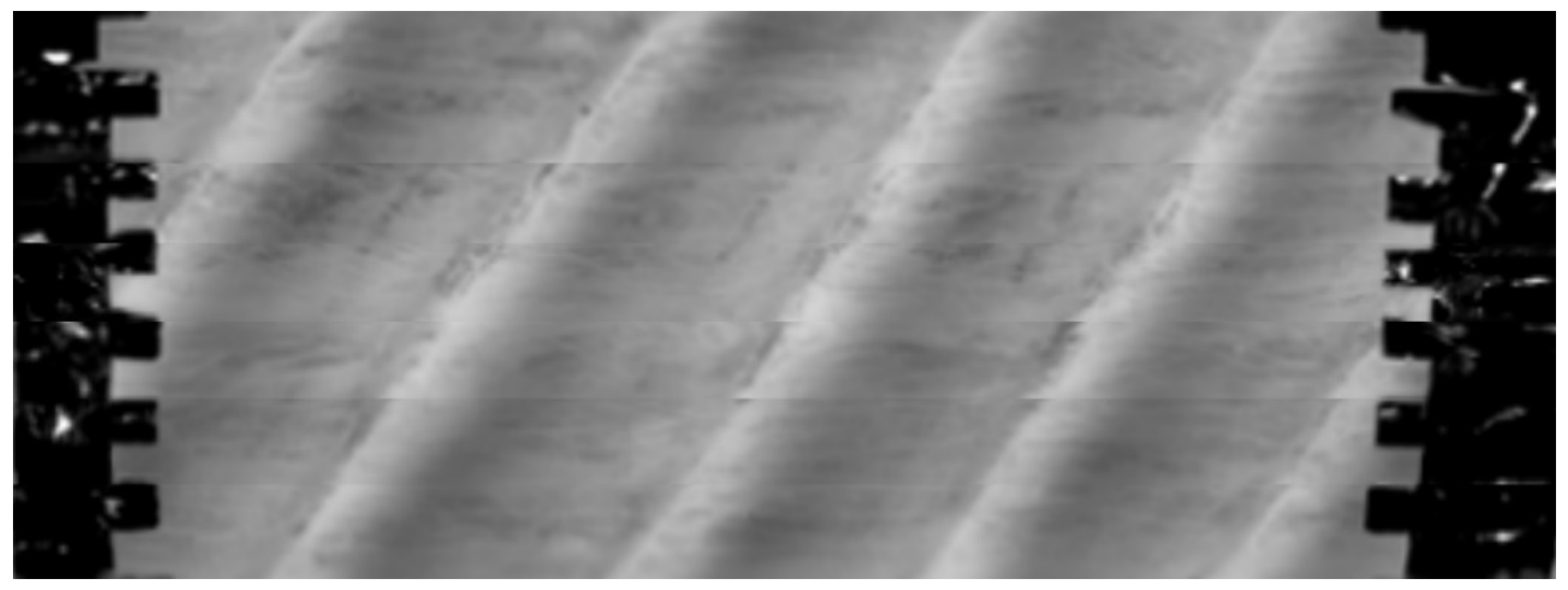
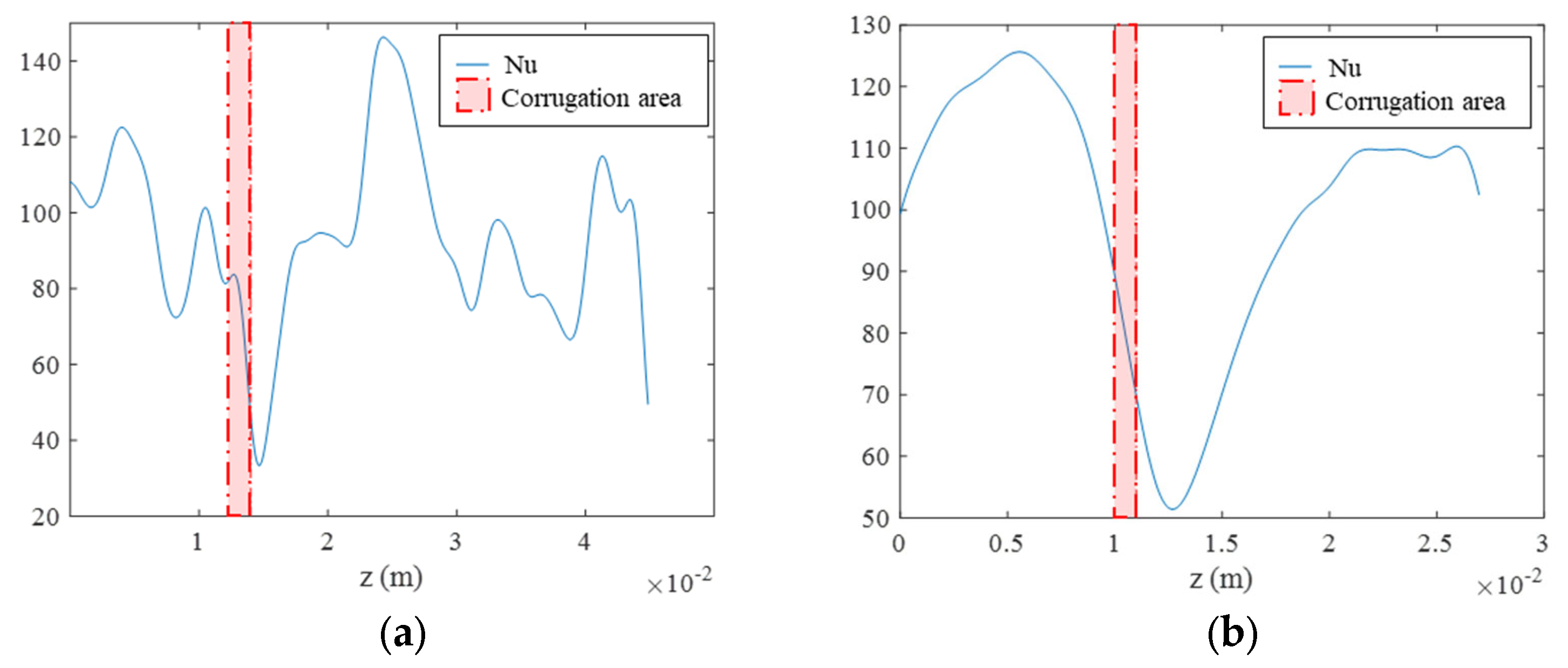
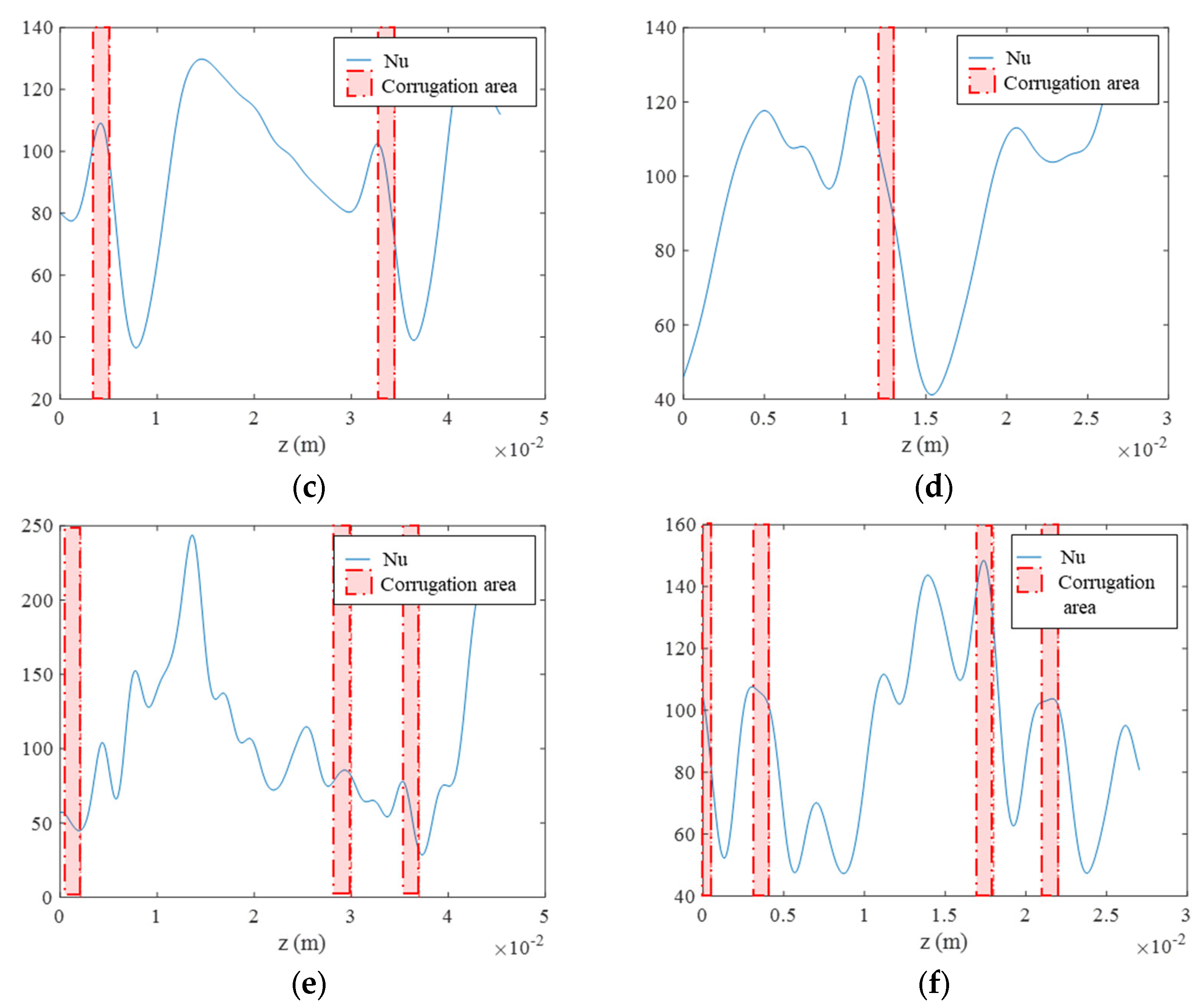
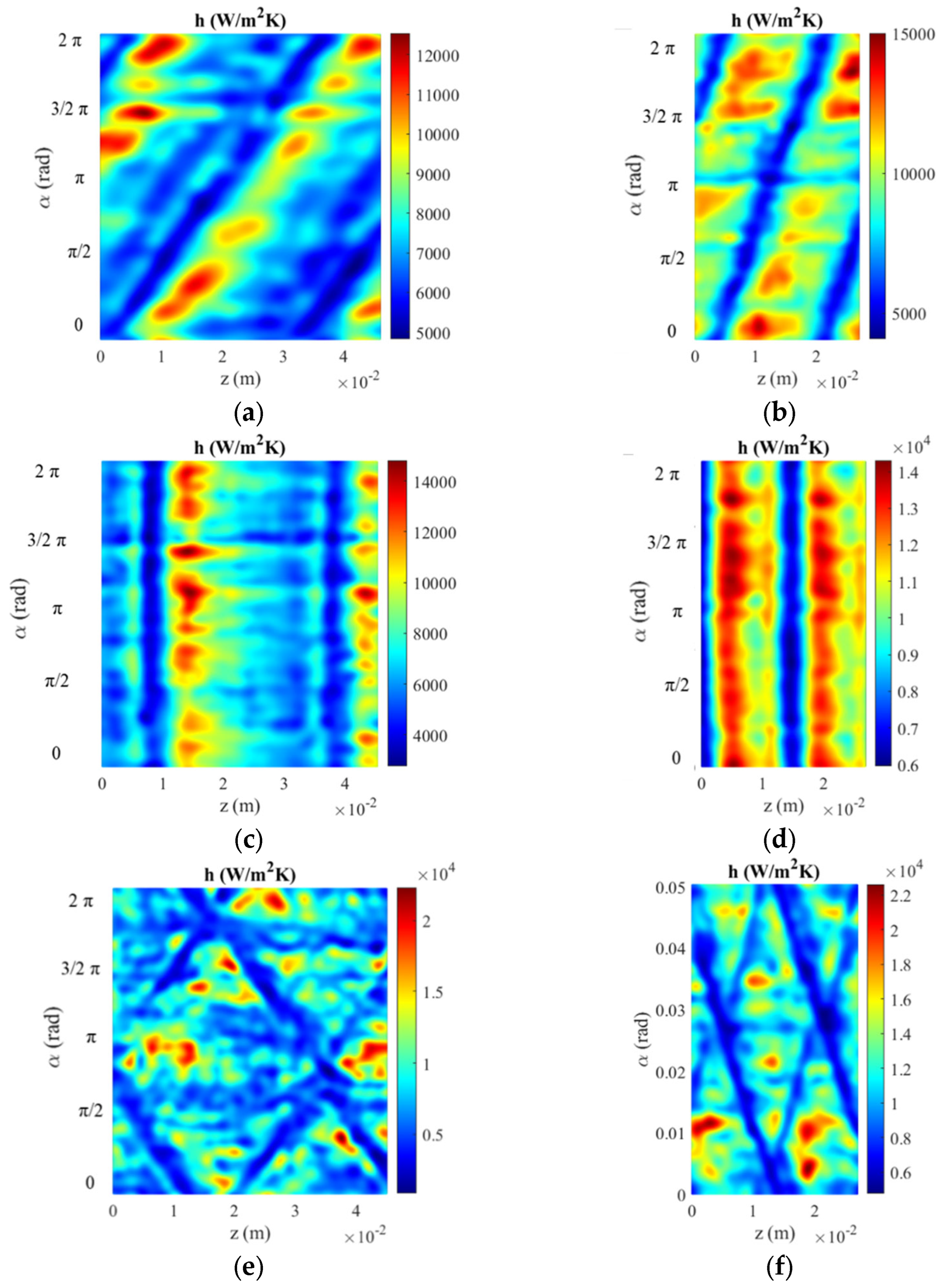
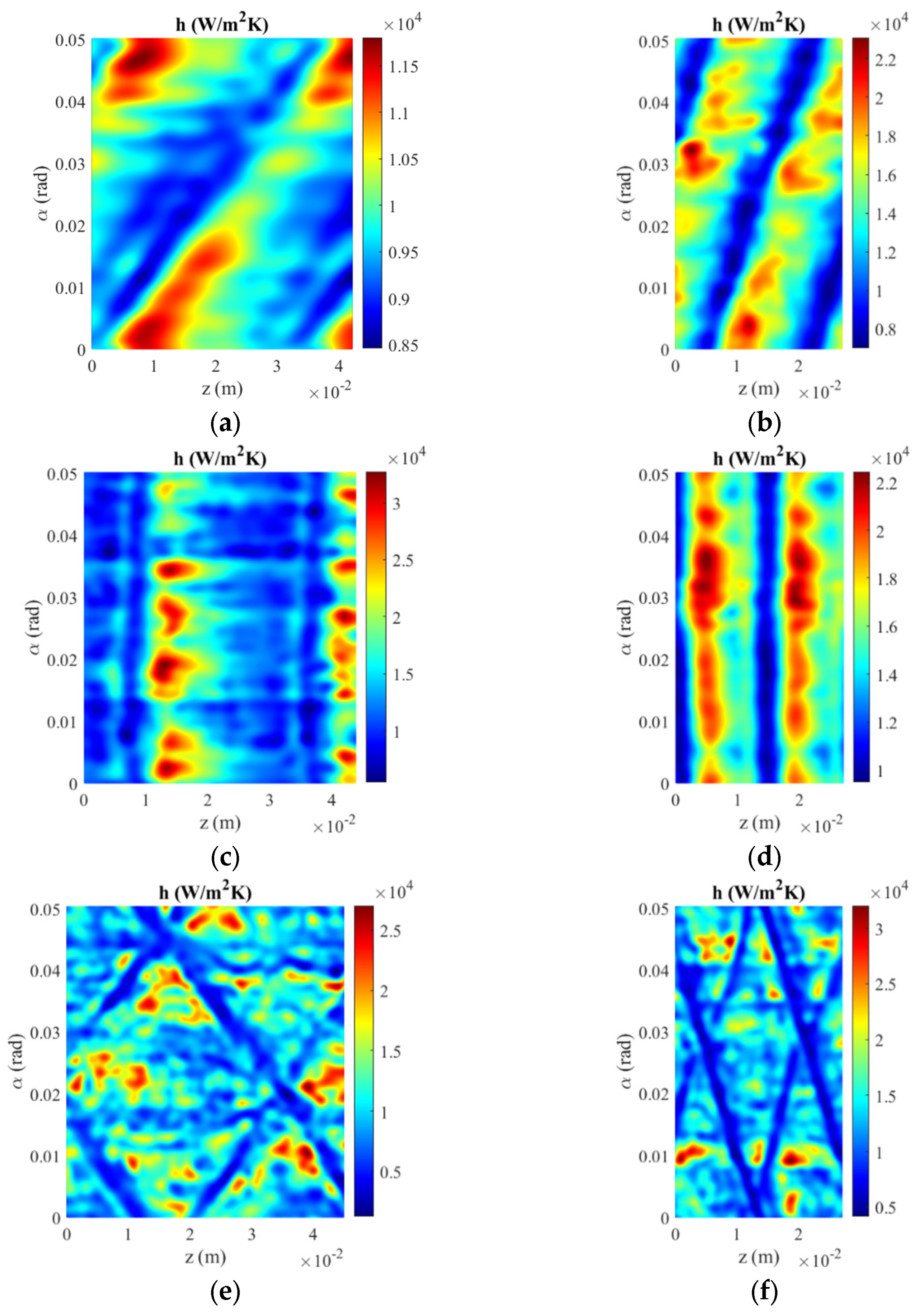
4.2. Local Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| cp | Fluid specific heat at constant pressure | J·kg−1 K−1 |
| Do | Tube outer diameter | m |
| Di | Tube internal diameter | m |
| e | Corrugation depth | m |
| f | Darcy friction factor | - |
| h | Convective heat transfer coefficient | W·m−2K−1 |
| l | Corrugation pitch | m |
| L | Length of pipe | m |
| Nu | Nusselt number | - |
| p | Pressure | Pa |
| r | Radial coordinate | m |
| Pr | Prandlt number, Pr = (cp·μ)/λ | - |
| q | Heat flux exchanged per unit surface | W·m−2 |
| qg | Internal heat generation per unit volume | W·m−3 |
| Re | Reynolds number | - |
| Renv | Overall heat transfer resistance between the external wall tube and the surrounding environment | - |
| t | Wall thickness | m |
| T | Temperature | |
| x | Axial coordinate | m |
| w | Mean fluid axial velocity | m/s |
| Greek Symbols | ||
| α | Angular coordinate | rad |
| εf | Friction factor enhancement | - |
| εh | Heat transfer enhancement | - |
| η | Enhancement efficiency | - |
| λ | Thermal conductivity | W·m−1·K−1 |
| μ | Dynamic viscosity | Pa·s |
| ν | Kinematic viscosity | m2·s−1 |
| ρ | Density | kg·m−3 |
| Subscripts | ||
| b | Bulk | |
| e | Enhanced geometry | |
| env | Environment | |
| i | Inlet section | |
| w | Wall | |
| x | Local value along the curvilinear coordinate x | |
| 0 | Reference geometry | |
| Superscripts | ||
| Averaged | ||
References
- Shank, K.; Tiari, S. A Review on Active Heat Transfer Enhancement Techniques within Latent Heat Ther-mal Energy Storage Systems. Energies 2023, 16, 4165. [Google Scholar] [CrossRef]
- Ajarostaghi, S.S.M.; Zaboli, M.; Javadi, H.; Badenes, B.; Urchueguia, J.F. A Review of Recent Passive Heat Transfer Enhancement Methods. Energies 2022, 15, 986. [Google Scholar] [CrossRef]
- Webb, R.L. Principles of Enhanced Heat Transfer; Routledge: London, UK, 1994. [Google Scholar]
- Thulukkanam, K. Heat Exchanger Design Handbook; CRC Press: Boca Raton, FL, USA, 2013. [Google Scholar]
- Kareem, Z.S.; Jaafar, M.M.; Lazim, T.M.; Abdullah, S.; Abdulwahid, A.F. Passive heat transfer enhancement review in corrugation. Exp. Therm. Fluid Sci. 2015, 68, 22–38. [Google Scholar] [CrossRef]
- Hong, K.-B.; Kim, D.-W.; Kwark, J.; Nam, J.-S.; Ryou, H.-S. Numerical Study on the Effect of the Pipe Groove Height and Pitch on the Flow Characteristics of Corrugated Pipe. Energies 2021, 14, 2614. [Google Scholar] [CrossRef]
- Chen, C.; Wu, Y.-T.; Wang, S.-T.; Ma, C.-F. Experimental investigation on enhanced heat transfer in transversally corrugated tube with molten salt. Exp. Therm. Fluid Sci. 2013, 47, 108–116. [Google Scholar] [CrossRef]
- Bozzoli, F.; Pagliarini, S.R.G.; Vocale, P.; Mocerino, A. Second principal approach to the analysis of un-steady flow and heat transfer in a tube with arc- shaped corrugation. J. Phys. Conf. Ser. 2016, 755, 011001. [Google Scholar] [CrossRef]
- Ahmad, F.; Mahmud, S.; Ehsan, M.M.; Salehin, M. Thermo-hydrodynamic performance evaluation of double-dimpled corrugated tube using single and hybrid nanofluids. Int. J. Thermofluids 2023, 17, 100283. [Google Scholar] [CrossRef]
- Qin, S.-Y.; Xiao, H.; Xiao, Y.; Liu, P.; Zhou, F.-Y.; Liu, W.; Liu, Z.-C.; Shan, F. Experimental investigation of the coherent struc-tures in a spirally corrugated pipe. Int. J. Heat Fluid Flow 2020, 84, 108601. [Google Scholar] [CrossRef]
- Li, X.; Liu, S.; Mo, X.; Sun, Z.; Tian, G.; Xin, Y.; Zhu, D. Investigation on Convection Heat Transfer Augment in Spirally Corrugated Pipe. Energies 2023, 16, 1063. [Google Scholar] [CrossRef]
- Bilen, K.; Cetin, M.; Gul, H.; Balta, T. The investigation of groove geometry effect on heat transfer for internally grooved tubes. Appl. Therm. Eng. 2009, 29, 753–761. [Google Scholar] [CrossRef]
- Zhang, L.; Duan, R.; Che, Y.; Lu, Z.; Cui, X.; Wei, L.; Jin, L. A numerical analysis of fluid flow and heat transfer in wavy and curved wavy channels. Int. J. Therm. Sci. 2021, 171, 107248. [Google Scholar] [CrossRef]
- Mateo-Gabín, A.; Chávez, M.; Garicano-Mena, J.; Valero, E. Wavy walls, a passive way to control the transi-tion to turbulence. detailed simulation and physical explanation. Energies 2021, 14, 3937. [Google Scholar] [CrossRef]
- Al-Obaidi, A.R.; Alhamid, J. Investigation of flow pattern, thermohydraulic performance and heat transfer improvement in 3D corrugated circular pipe under varying structure configuration parameters with development different correlations. Int. Commun. Heat Mass Transf. 2021, 126, 105394. [Google Scholar] [CrossRef]
- Al-Obaidi, A.R. Investigation on effects of varying geometrical configurations on thermal hydraulics flow in a 3D corrugated pipe. Int. J. Therm. Sci. 2022, 171, 107237. [Google Scholar] [CrossRef]
- Rinaldi, M.; Malavasi, M.; Cordioli, M.; Barbanti, D. Computation fluid dynamics design of a plate fin heat exchanger with different transverse vortex generators for food processing. J. Food Process. Eng. 2019, 42, e13140. [Google Scholar] [CrossRef]
- Duda, P. Heat Transfer Coefficient Distribution—A Review of Calculation Methods. Energies 2023, 16, 3683. [Google Scholar] [CrossRef]
- Beck, J.V.; Balckwell, B.; Clair, C.R., Jr. Inverse Heat Conduction—Ill-Posed Problems; John Wiley & Sons Inc.: New York, NY, USA, 1985. [Google Scholar]
- Rogalski, A. Recent progress in infrared detector technologies. Infrared Phys. Technol. 2011, 54, 136–154. [Google Scholar] [CrossRef]
- Rainieri, S.; Bozzoli, F.; Pagliarini, G. Characterization of an uncooled infrared thermographic system suitable for the solution of the 2-D inverse heat conduction problem. Exp. Therm. Fluid Sci. 2008, 32, 1492–1498. [Google Scholar] [CrossRef]
- Murio, A.D. The Mollification Method and the Numerical Solution of Ill-Posed Problems; John Wiley and Sons: New York, NY, USA, 1993. [Google Scholar]
- Cattani, L.; Maillet, D.; Bozzoli, F.; Rainieri, S. Estimation of the local convective heat transfer coefficient in pipe flow using a 2D thermal Quadrupole model and Truncated Singular Value Decomposition. Int. J. Heat Mass Transf. 2015, 91, 1034–1045. [Google Scholar] [CrossRef]
- Xiong, P.; Qiu, Z.; Lu, Q.; Lu, T.; Deng, J.; Liu, Y.; Zhang, Y. Simultaneous estimation of fluid temperature and convective heat transfer coefficient by sequential function specification method. Prog. Nucl. Energy 2020, 131, 103588. [Google Scholar] [CrossRef]
- Delpueyo, D.; Balandraud, X.; Grédiac, M. Heat source reconstruction from noisy temperature fields using an opti-mised derivative Gaussian filter. Infrared Phys. Technol. 2013, 60, 312–322. [Google Scholar] [CrossRef]
- Colaço, M.J.; Alves, C.J.; Bozzoli, F. The reciprocity function approach applied to the non-intrusive estimation of spatially varying internal heat transfer coefficients in ducts: Numerical and experimental results. Int. J. Heat Mass Transf. 2015, 90, 1221–1231. [Google Scholar] [CrossRef]
- Ngendahayo, J.P.; Niyobuhungiro, J.; Berntsson, F. Estimation of surface temperatures from interior measurements using Tikhonov regularization. Results Appl. Math. 2021, 9, 100140. [Google Scholar] [CrossRef]
- Bozzoli, F.; Cattani, L.; Pagliarini, G.; Rainieri, S. Infrared image filtering applied to the restoration of the convective heat transfer coefficient distribution in coiled tubes. Opto-Electron. Rev. 2015, 23, 107–115. [Google Scholar] [CrossRef]
- Carlomagno, G.M.; Cardone, G. Infrared thermography for convective heat transfer measurements. Exp. Fluids 2010, 49, 1187–1218. [Google Scholar] [CrossRef]
- Bergman, T.L.; Incropera, F.P.; DeWitt, D.P.; Lavine, A.S. Fundamentals of Heat and Mass Transfer; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Zimparov, V. Extended performance evaluation criteria for enhanced heat transfer surfaces: Heat transfer through ducts with constant heat flux. Int. J. Heat Mass Transf. 2001, 44, 169–180. [Google Scholar] [CrossRef]
- Yilmaz, M.; Sara, O.; Karsli, S. Performance evaluation criteria for heat exchangers based on second law analysis. Exergy Int. J. 2001, 1, 278–294. [Google Scholar] [CrossRef]
- Astarita, T.; Carlomagno, G.M. Infrared Thermography for Thermo-Fluid-Dynamics; Springer: Berlin/Heidelberg, Germany, 2013; ISBN 9783642295072. [Google Scholar]
- Bozzoli, F.; Cattani, L.; Rainieri, S. Effect of wall corrugation on local convective heat transfer in coiled tubes. Int. J. Heat Mass Transf. 2016, 101, 76–90. [Google Scholar] [CrossRef]
- Beck, J. Inverse Heat Conduction Ill-Posed Problems; John Wiley & Sons: Hoboken, NJ, USA, 2011; pp. 137–157, 179–180, 198–211. [Google Scholar]
- Morozov, V.A. Methods for Solving Incorrectly Posed Problems; Springer: Berlin/Heidelberg, Germany, 1984; ISBN 9780387960593. [Google Scholar]
- Bevington, P.R. Data Reduction and Error Analysis for the Physical Sciences; McGraw-Hill: New York, NY, USA, 1969. [Google Scholar]
- Vicente, P.G.; Garcıa, A.; Viedma, A. Experimental investigation on heat transfer and frictional characteristics of spirally corrugated tubes in turbulent flow at different Prandtl numbers. Int. J. Heat Mass Transf. 2004, 47, 671–681. [Google Scholar] [CrossRef]
- Mohammed, H.; Abbas, A.K.; Sheriff, J. Influence of geometrical parameters and forced convective heat transfer in transversely corrugated circular tubes. Int. Commun. Heat Mass Transf. 2013, 44, 116–126. [Google Scholar] [CrossRef]
- Bozzoli, F.; Cattani, L.; Rainieri, S. Cross-helix corrugation: The optimal geometry for effective food thermal processing. Int. J. Heat Mass Transf. 2020, 147, 118874. [Google Scholar] [CrossRef]

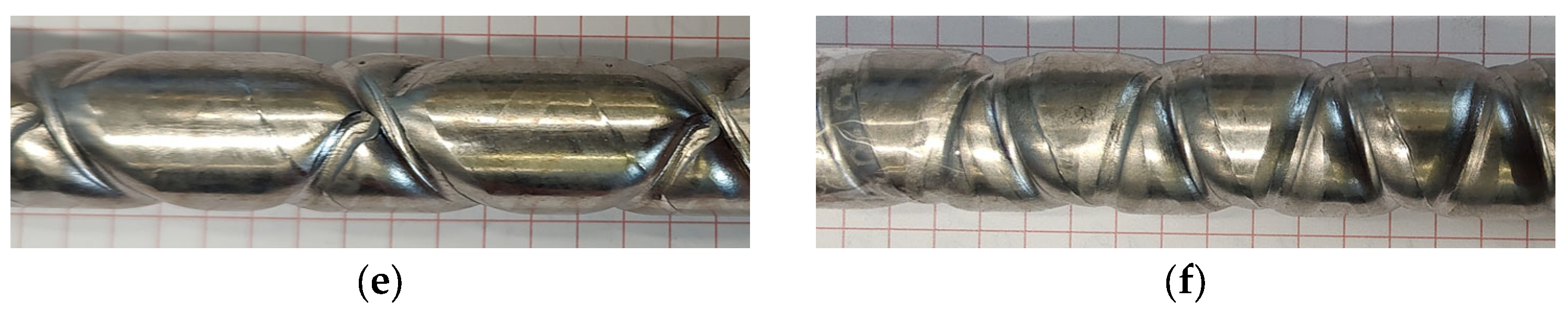


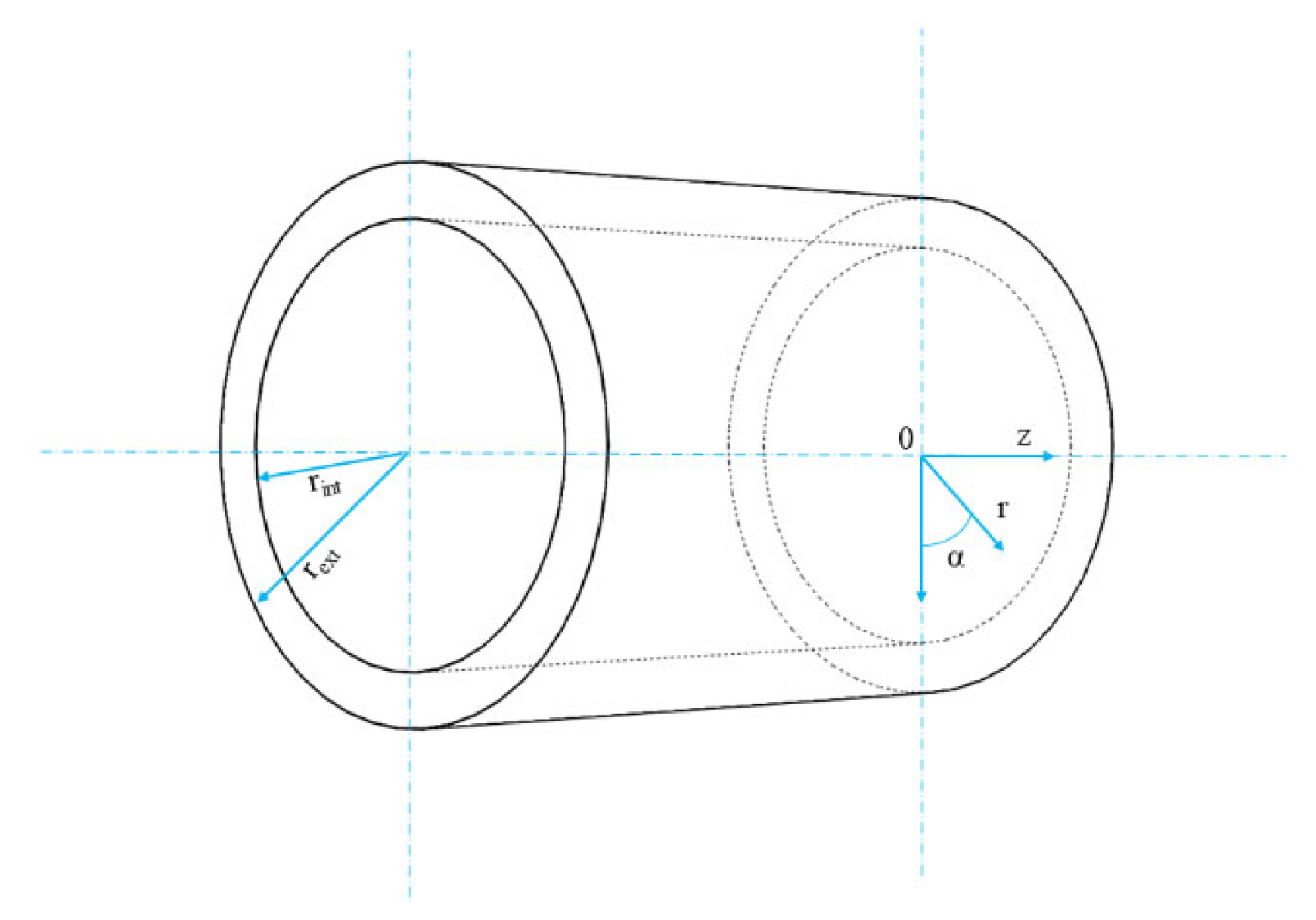
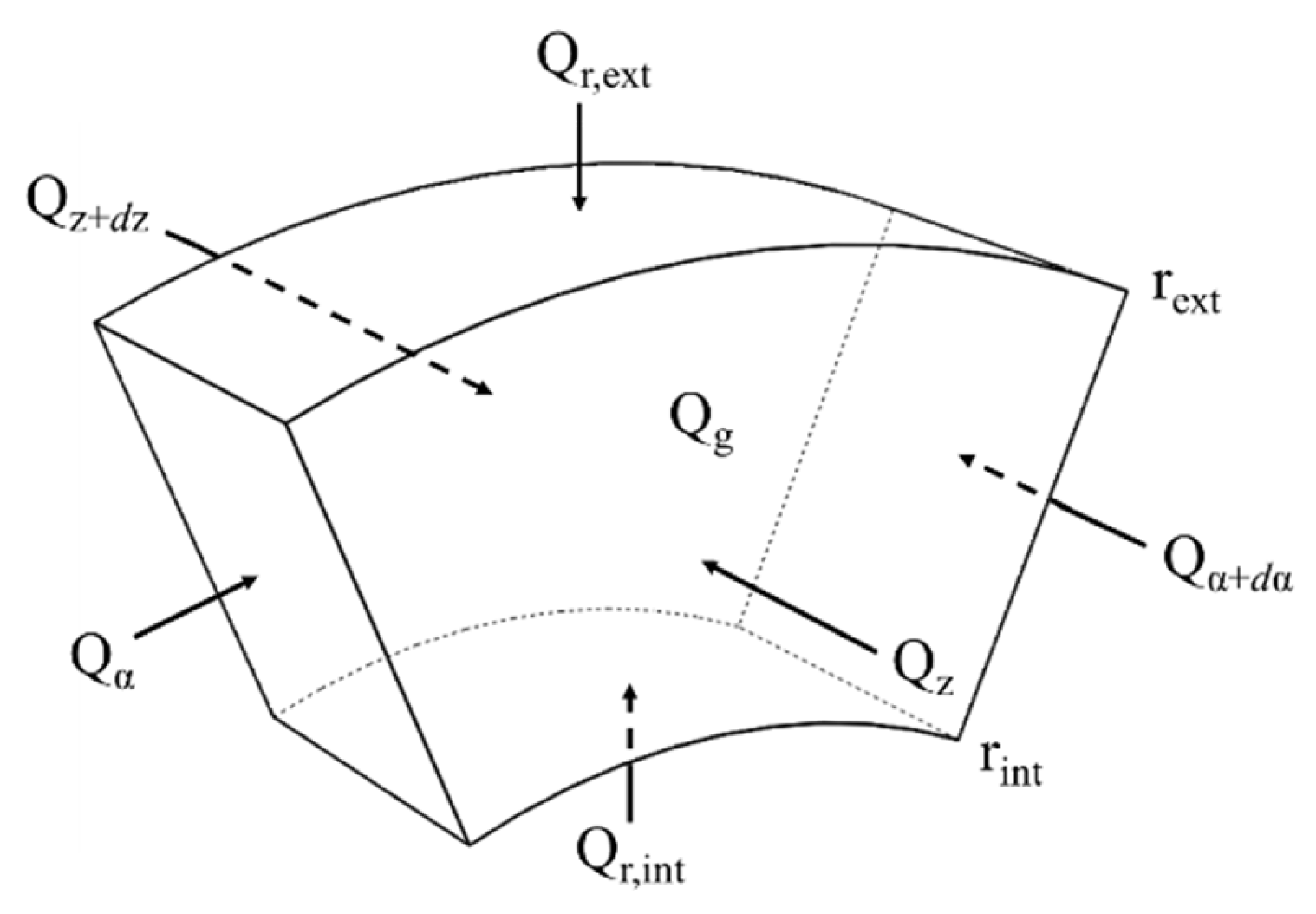




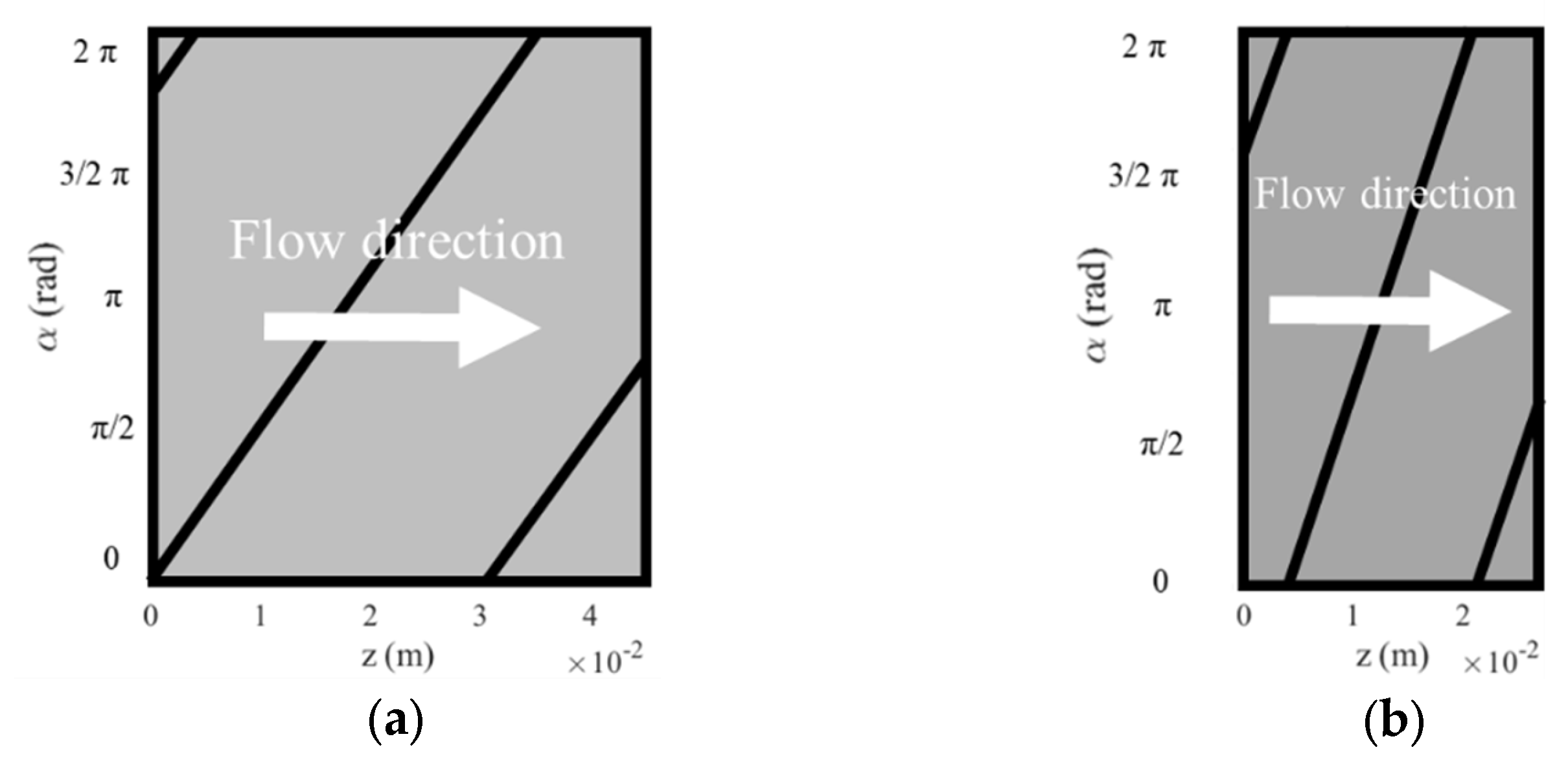





| Pipe Name | Corrugation Profile | Pitch (p) (mm) | Depth (e) (mm) | Di (mm) | Do (mm) |
|---|---|---|---|---|---|
| T32 | Transversal | 32 | 1 | 14 | 16 |
| T16 | Transversal | 16 | 1 | 14 | 16 |
| H32 | Helical | 32 | 1 | 14 | 16 |
| H16 | Helical | 16 | 1 | 14 | 16 |
| C32 | Cross-helix | 32 | 1 | 14 | 16 |
| C16 | Cross-helix | 16 | 1 | 14 | 16 |
| T {K} | ΔT {K} | ρ {kg/m3} | µ {Pa·s} | Di {m} | qg {W/m3} | {m3/s} | Δp {Pa} |
|---|---|---|---|---|---|---|---|
| ±0.1 | ±0.2 | ±1% | ±1% | ±2% | ±3% | ±3% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Azam, M.W.; Cattani, L.; Malavasi, M.; Bozzoli, F. Experimental Study of the Corrugation Profile Effect on the Local Heat Transfer Coefficient. Energies 2023, 16, 7181. https://doi.org/10.3390/en16207181
Azam MW, Cattani L, Malavasi M, Bozzoli F. Experimental Study of the Corrugation Profile Effect on the Local Heat Transfer Coefficient. Energies. 2023; 16(20):7181. https://doi.org/10.3390/en16207181
Chicago/Turabian StyleAzam, Muhammad Waheed, Luca Cattani, Matteo Malavasi, and Fabio Bozzoli. 2023. "Experimental Study of the Corrugation Profile Effect on the Local Heat Transfer Coefficient" Energies 16, no. 20: 7181. https://doi.org/10.3390/en16207181
APA StyleAzam, M. W., Cattani, L., Malavasi, M., & Bozzoli, F. (2023). Experimental Study of the Corrugation Profile Effect on the Local Heat Transfer Coefficient. Energies, 16(20), 7181. https://doi.org/10.3390/en16207181








