Coupling Simulation of Longitudinal Vibration of Rod String and Multi-Phase Pipe Flow in Wellbore and Research on Downhole Energy Efficiency
Abstract
:1. Introduction
2. Mathematical Model of Unit Simulation
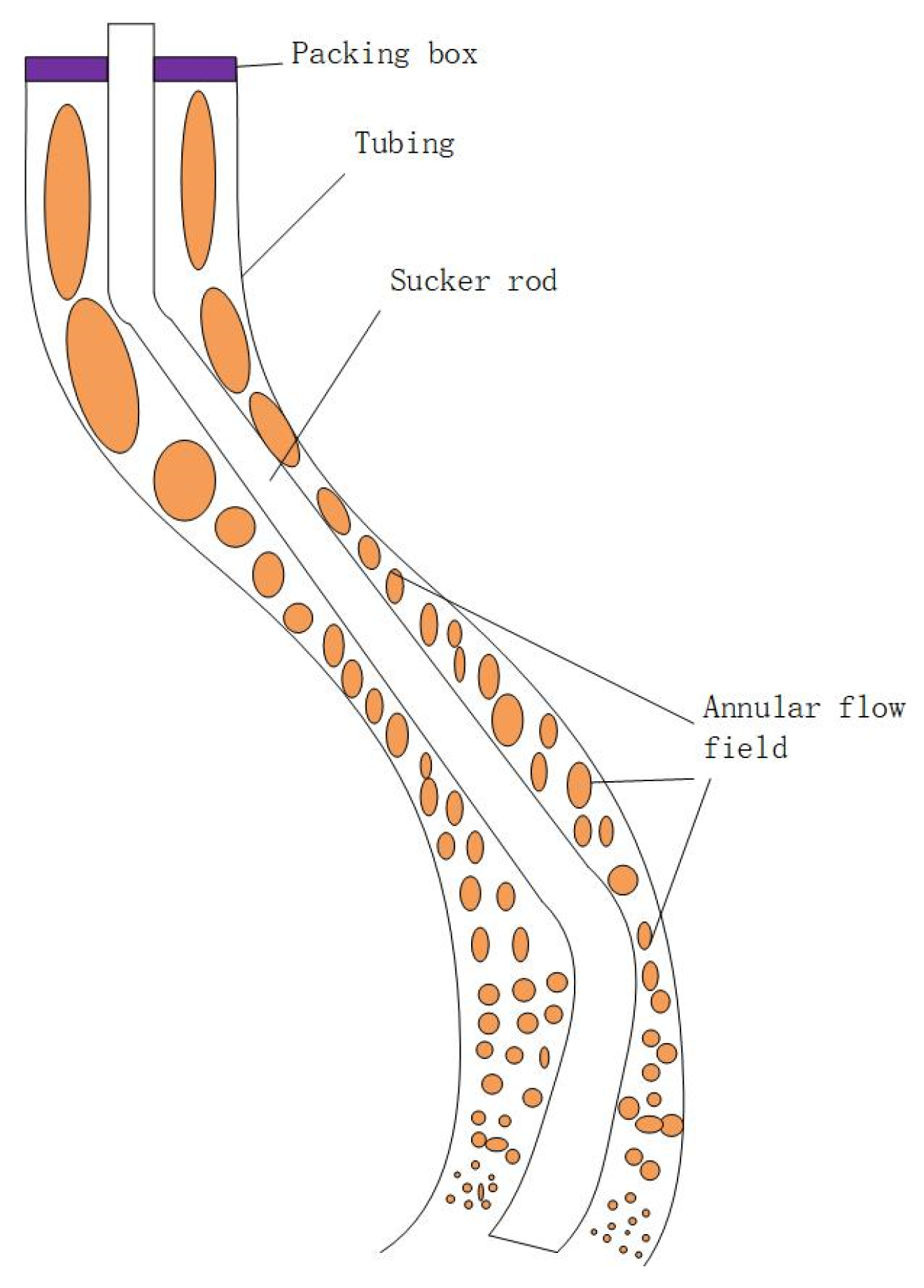
2.1. Mathematical Model of Longitudinal Vibration of Sucker Rod String
2.2. Model of Sinking Pressure and Discharge Pressure
2.3. Coupling Model of Discharge Pressure and Oil Production Based on Pump Outflow Characteristics
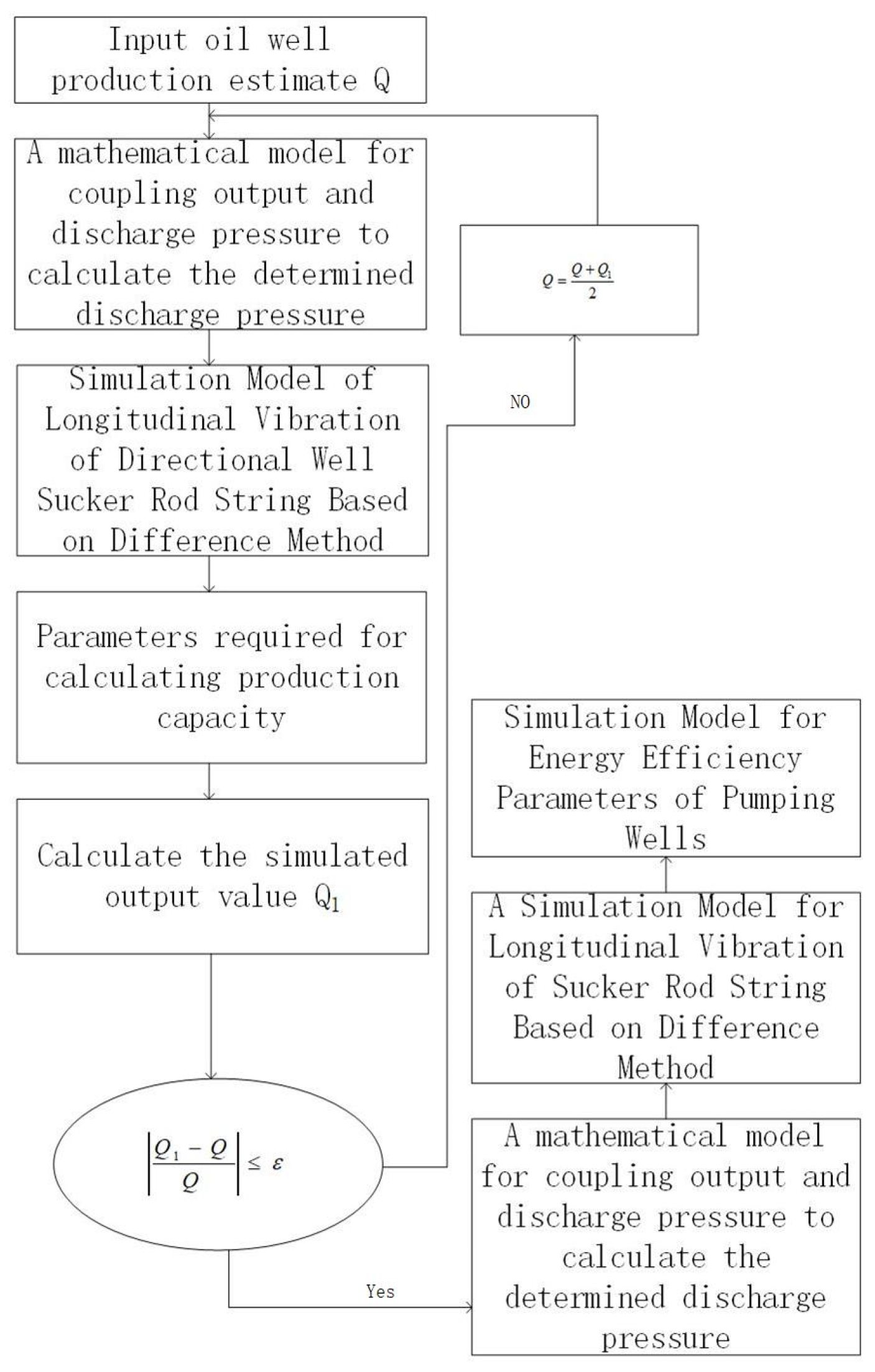
3. System Coupling Model and Numerical Simulation Algorithm
- (1)
- Input the parameters of the oil production, , and the oil well fluid parameters;
- (2)
- Based on the oil production, , the oil well fluid parameters, and the multi-phase pipe flow model in the wellbore, calculate the discharge pressure, , using the Runge–Kutta method;
- (3)
- Based on the longitudinal vibration model of the sucker-rod string and the discharge pressure, , calculate the displacement of any interface of the sucker-rod string and the downhole energy efficiency parameters using the finite difference method;
- (4)
- Calculate the oil production simulation results, ;
- (5)
- If ( is the calculation error check value), output the required parameters and indicator diagram. If , correct , and return to Steps (2)–(4) until the result is met.
4. Mathematical Model of Downhole Energy Efficiency Parameters
4.1. Mathematical Model of Polished Rod Power
4.2. Mathematical Model of Pump Input Power and Pump Internal Loss Power
4.3. Mathematical Model for the Effective Power of a System
4.3.1. Industry Standard Effective Power Model
4.3.2. Effective Power Calculation Model Based on Multiphase Flow
4.3.3. Effective Power Model Based on Pumping Pump Energy Balance Relationship
4.3.4. Calculation Model of Downhole Efficiency and Sub-Efficiency
5. Precision Verification
5.1. Analysis of Calculation Results of Discharge Pressure
- (1)
- When the HC is used to calculate the discharge pressure, the production does not affect the discharge pressure. However, when the DC is used, the discharge pressure decreases as oil production increases. As the gas-oil ratio (GOR) increases and the water content decreases, the degree of variation in the discharge pressure also increases;
- (2)
- The discharge pressure calculated by the HC is lower than that calculated by the DC. The difference between the calculated results of the two models is greater when the oil well production is higher, the gas-oil ratio (GOR) is higher, and the water content is lower. This is due to the presence of not only bubbly flows but also slug flow, agitation flow, and annular flow in the fluid flow inside the oil pipe. These flow patterns result in a decrease in the pressure gradient of the fluid and in the discharge pressure.
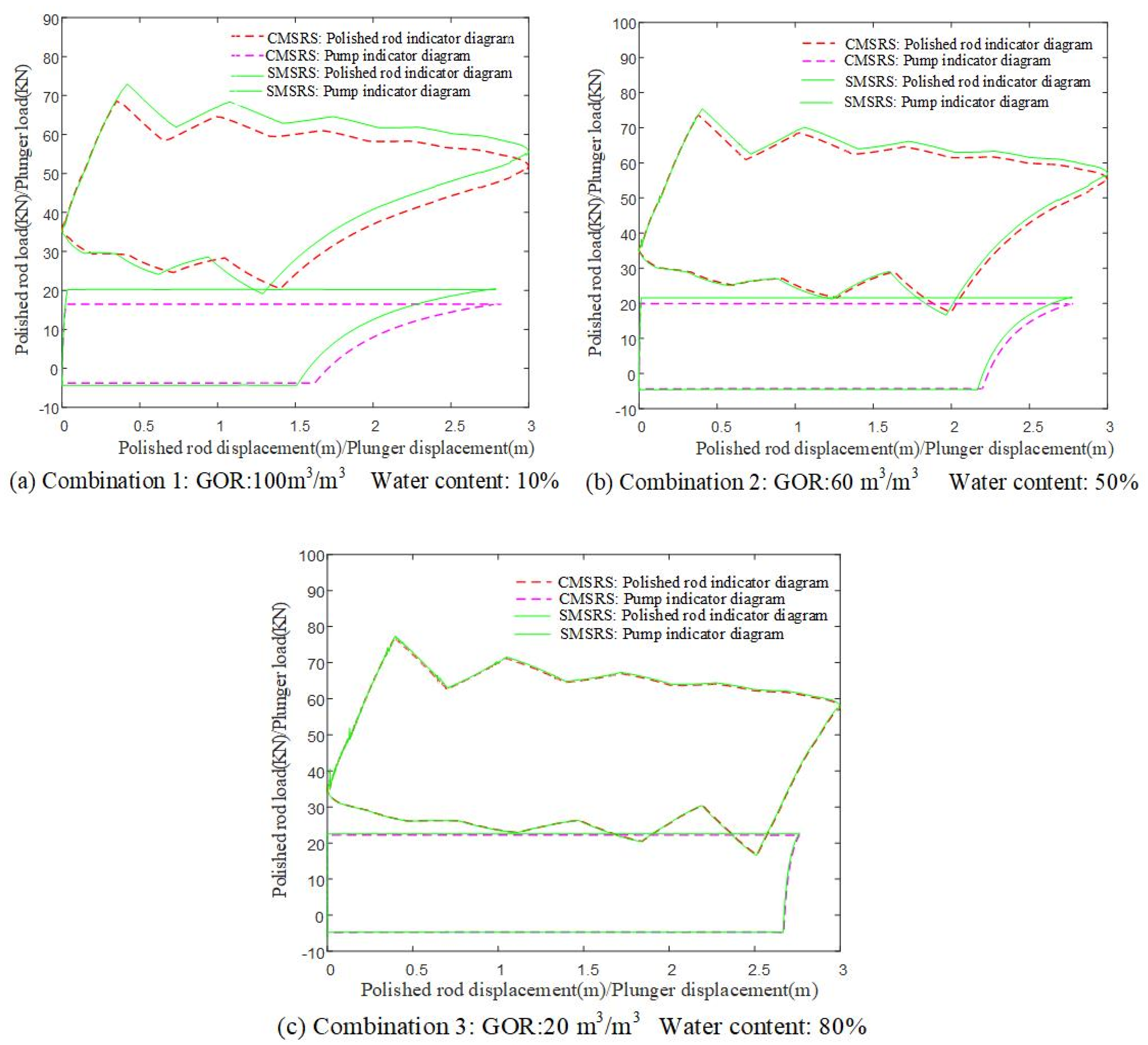
5.2. Comparison Analysis of Simulation Results of the Indicator Diagram
- (1)
- The simulation indicator diagram of the CMSRS model differs from that of the SMSRS model. The discrepancy between the two models is larger when the water content is lower, and the gas-oil ratio (GOR) is higher;
- (2)
- The maximum load on the polished rod, as calculated by the CMSRS, is lower than the load calculated by the SMSRS. Conversely, the minimum load on the polished rod, as calculated by the CMSRS, is higher than the load calculated by the SMSRS. This discrepancy is primarily due to variations in the water content and gas-oil ratio. At a gas-oil ratio (GOR) of 100 and water content of 10%, the maximum and minimum polished rod load differences are −17.07% and 20.30%, respectively. Similarly, at a gas-oil ratio (GOR) of 20 and water content of 80%, the maximum and minimum polished rod load differences are only −1.16% and 1.78%, respectively;
- (3)
- The average power of the polished rod calculated using the CMSRS is less than that calculated using the SMSRS. Similarly, the average pump input power, calculated using the CMSRS, was also less than that calculated using the SMSRS. However, the oil production calculated using the CMSRS was larger than that calculated using the SMSRS. When the gas-oil ratio (GOR) is 100, and the water content is 10%, the average power of the polished rod difference is −18.10% and the oil production difference is 4.43%. When the gas-oil ratio is 20, and the water content is 80%, the average power of the polished rod and the oil production difference are only −1.87% and 0.33%, respectively;
- (4)
- The main reason for the above results is that the discharge pressure calculated by the DC is smaller than that calculated by the HC. When the gas-oil ratio (GOR) is 100 and the water content is 10%, the difference in the discharge pressure is −33.52%. When the gas-oil ratio (GOR) is 20, and the water content is 80%, the difference in the discharge pressure is only −2.74%.
5.3. Comparison of Effective Power Simulation Results of Different Models
- (1)
- The effective power of the multi-phase flow model is equivalent to the effective power based on the energy balance equation of the pump. The difference between the calculated results of the available power under the three gas-oil ratios (GORs) and water content combination conditions is only 0.04–1.02%. This verifies the correctness of the effective power model of the multi-phase flow;
- (2)
- There are significant disparities between the industry standard calculated effective power and the pump energy balance simulation results. The effective power calculation results of the two models differ by −3.50–−17.90% under the combined conditions of three gas-oil ratios (GORs) and water content. Upon analyzing Equations (22) to (23), it became evident that the industry standard effective power calculation model has certain limitations. Specifically, the reciprocating pump transports oil, gas, and water, and its effective power is not only the power of the pump lifting the liquid phase but also the power of the pump lifting the gas phase. However, the industry standard only accounts for the output of the pump that lifts the oil-water mixture and does not consider the output of the pump that lifts the gas phase. The computation of the discharge pressure simplifies the fluid in the wellbore into the mixed liquid oil and water, which increases the discharge pressure and lifting height.
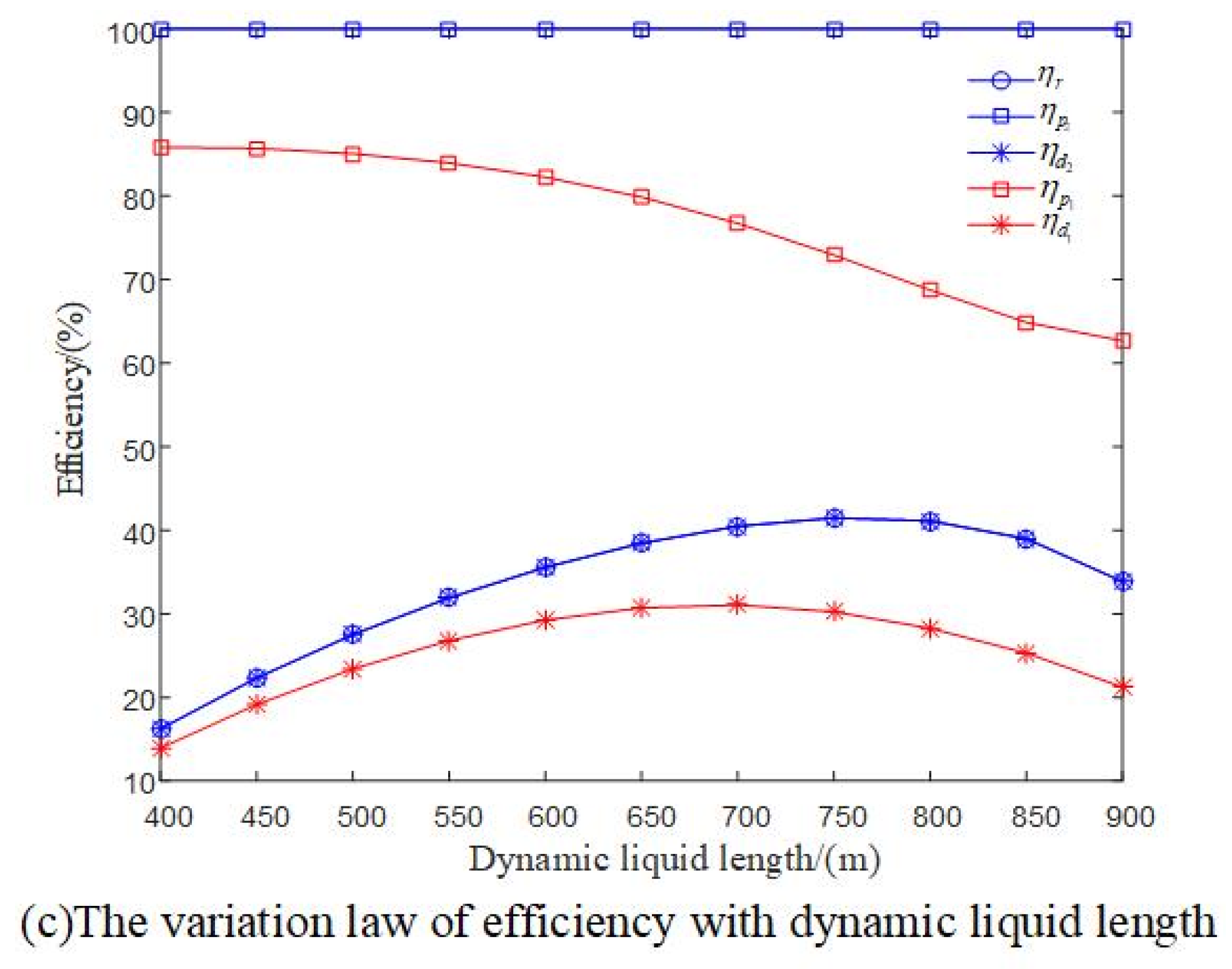
5.4. Simulation Analysis of Downhole Efficiency and Sub-Efficiency
- (1)
- When the effective power is calculated using the multi-phase pipe flow model, the pump’s efficiency approaches 100%, and the impacts of the gas-oil ratio, water content, and dynamic liquid length are minimal. The pump’s efficiency under the simulated thin oil conditions in this study was found to be nearly 100%, consistent with the experimental findings. When based on the industry standard model, the pump efficiency is below 100% and is highly dependent on various parameters. For example, the pump efficiency varies depending on the water content and gas-oil ratio (GOR). At a water content of 10%, the pump efficiency is 56.66%, while at a gas-oil ratio (GOR) of 100, the pump efficiency is 71.95%. However, at a gas-oil ratio (GOR) of 5 and a water content of 95%, the pump efficiency is nearly 100%. The results of the downhole efficiency calculation also reveal the constraints of the industry standard effective power model;
- (2)
- In the presence of thin oil and normal clearance leakage in the pump, the primary cause of downhole power loss in the pumping unit is frictional power loss in the rod. Pump power loss is negligible, resulting in downhole efficiency being nearly equal to the rod efficiency;
- (3)
- The impact of wellbore parameters on downhole efficiency is significant. An increase in the gas-oil ratio and water content results in a decrease in downhole efficiency. An inflection point exists in the relationship between downhole efficiency and submergence degree. This suggests that there is an optimal submergence degree in the pumping well that maximizes downhole efficiency. Hence, optimizing the parameters of swabbing can considerably enhance the downhole and system efficiency.
6. Conclusions
- (1)
- Significant differences are present between the discharge pressure obtained from the multi-phase pipe flow model and that obtained from the hydrostatic column model for oil and water. Given that the wellbore of the pumping unit involves a multi-phase flow of oil, gas, and water, it is imperative to compute the discharge pressure using a multi-phase pipe flow model. Additionally, it is crucial to develop a simulation model for the longitudinal vibration of the rod string;
- (2)
- The CMSRS model was developed by integrating the multi-phase pipe flow model with the longitudinal vibration of the sucker-rod string. This model was utilized to determine the discharge pressure in the system’s dynamic simulation. The experimental results demonstrated that the simulation model effectively improves the accuracy of the simulation for both indicators and energy efficiency;
- (3)
- Based on the simulation results of the pump indicator diagram and the energy balance relationship, a simulation model was established to determine the effective power of the system. The accuracy of the multi-phase flow effective power calculation model was demonstrated by comparing its simulation results with those of the industry standard and multi-phase flow models while also highlighting the limitations of the industry standard model;
- (4)
- The impact of wellbore parameters, including the gas-oil ratio, water content, and dynamic liquid level, on the efficiency of downhole, rod, and pump operations was analyzed. The simulation results indicated that the pump operates with high efficiency, and the efficiency of the rod primarily affects the downhole efficiency. Downhole efficiency is significantly affected by water content and the gas-oil ratio. Optimizing swabbing parameters and achieving appropriate sinking depths can greatly improve downhole efficiency.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Gibbs, S.G. Predicting the Behavior of Sucker Rod Pumping Systems; Shell Development Co.: Houston, TX, USA, 1963; Volume 6, pp. 1–10. [Google Scholar] [CrossRef]
- Doty, D.R.; Schmidt, Z. An improved model for sucker rod pumping. Soc. Pet. Eng. J. 1983, 23, 33–41. [Google Scholar] [CrossRef]
- Lekia, S.D.; Evans, R.D. A coupled rod and fluid Dynamic model for predicting the behavior of sucker-rod pumping system. SPE Prod. Oper. 1995, 10, 26–40. [Google Scholar] [CrossRef]
- Shardakov, I.N.; Wasserman, I.N. Numerical Modeling of Longitudinal Vibration of a Sucker Rod String. J. Sound Vib. 2010, 329, 317–327. [Google Scholar] [CrossRef]
- Everitt, T.A.; Jennings, J.W. An Improved Finite Difference Calculation of Downhole Dynamometer Cards for Sucker Rod Pumps. SPE Prod. Oper. 1992, 7, 121–127. [Google Scholar] [CrossRef]
- Schafer, D.J.; Jennings, J.W. An Investigation of Analytical and Numerical Sucker Rod Pumping Mathematical Models; SPE-16919-MS; Society of Petroleum Engineers: Richardson, TX, USA, 1987. [Google Scholar] [CrossRef]
- Xing, M.M. Response Analysis of Longitudinal Vibration of Sucker Rod String Considering Rod Buckling. Adv. Eng. Softw. 2016, 99, 49–58. [Google Scholar] [CrossRef]
- Li, W.C.; Dong, S.M.; Sun, X.R. An Improved Sucker Rod Pumping System Model and Swabbing Parameters Optimized Design. Math. Probl. Eng. 2018, 2018, 4746210. [Google Scholar] [CrossRef]
- Xing, M.; Zhou, L.; Zhang, C.; Xue, K. Simulation Analysis of Nonlinear Friction of Rod String in Sucker Rod Pumping System. J. Comput. Nonlinear Dyn. 2019, 14, 091008. [Google Scholar] [CrossRef]
- Li, Q.; Chen, B.; Huang, Z.; Tang, H. Study on Equivalent Viscous Damping Coefficient of Sucker Rod Based on the Principle of Equal Friction Loss. Math. Probl. Eng. 2019, 2019, 9272751. [Google Scholar] [CrossRef]
- Moreno, G.A.; Garriz, A.E. Sucker Rod String Dynamics in Deviated Wells. J. Pet. Sci. Eng. 2020, 184, 106534. [Google Scholar] [CrossRef]
- Wang, X.; Lv, L.; Li, S.; Pu, H. Longitudinal vibration analysis of sucker rod based on a simplified thermo-solid model. J. Comput. Nonlinear Dyn. 2021, 196, 107951. [Google Scholar] [CrossRef]
- Hasan, A.R.; Kabir, C.S. Two-phase flow in vertical and inclined annuli. Int. J. Multiph. Flow 1992, 18, 279–293. [Google Scholar] [CrossRef]
- Li, W.; Vaziri, V.; Aphale, S.S.; Dong, S.; Wiercigroch, M. Dynamics and frequency and voltage control of downhole oil pumping system. Mech. Syst. Signal Process. 2020, 139, 106562. [Google Scholar] [CrossRef]
- Xing, M.M.; Dong, S. An improved longitudinal vibration model and dynamic characteristic of sucker rod string. J. Vibro Eng. 2014, 16, 3432–3448. [Google Scholar]
- Li, W.; Vaziri, V.; Aphale, S.S.; Dong, S.; Wiercigroch, M. Energy saving by reducing motor rating of Sucker-Rod Pump systems. Energy 2021, 228, 120618. [Google Scholar] [CrossRef]
- Wang, H.; Dong, S.; Gan, Q.; Xin, H.; Zhu, G. Dynamic parameter simulation model of low-production pumping well and the ways to improve system efficiency. Shiyou Xuebao/Acta Pet. Sin. 2018, 39, 1299–1307. [Google Scholar] [CrossRef]
- Dong, S. Study on a new method for calculating effective power of pumping wells. Pet. Mach. 2003, 10, 19–22+75. (In Chinese) [Google Scholar]
- Lukasiewicz, S.A. Dynamic Behavior of the Sucker Rod String in the Inclined Well. In Proceedings of the SPE Production Operations Symposium, Oklahoma City, OK, USA, 7–9 April 1991; SPE 21665. Society of Petroleum Engineers: Richardson, TX, USA, 1991; pp. 313–321. [Google Scholar] [CrossRef]
- Zhang, B.; Gao, X.; Li, X. Complete Simulation and Fault Diagnosis of Sucker-Rod Pumping. SPE Prod. Oper. 2021, 36, 277–290. [Google Scholar] [CrossRef]
- Sun, X.; Dong, S.; Li, W.; Zhang, W. The numerical simulation of entire sucker rod string buckling with coupling in vertical wells. Clust. Comput. 2018, 22, 12283–12295. [Google Scholar] [CrossRef]
- Antonietti, P.; Formaggia, L.; Scotti, A.; Verani, M.; Verzott, N. Mimetic finite difference approximation of flows in fractured porous media. ESAIM Math. Model. Numer. Anal. 2016, 50, 809–832. [Google Scholar] [CrossRef] [Green Version]
- Ahmadianfar, I.; Heidari, A.A.; Gandomi, A.H.; Chu, X.; Chen, H. RUN beyond the metaphor: An efficient optimization algorithm based on Runge Kutta method. Expert Syst. Appl. 2021, 181, 2–22. [Google Scholar] [CrossRef]



| Parameter | TEST | CMSRS | SMSRS | ||
|---|---|---|---|---|---|
| Simulation | Error/% | Simulation | Error/% | ||
| PRLmax/kN | 68.85 | 66.46 | −3.47 | 75.54 | 9.72 |
| PRLmin/kN | 19.63 | 19.85 | 1.12 | 16.63 | −15.28 |
| Nprm/kW | 10.20 | 9.74 | −4.51 | 11.30 | 10.79 |
| Parameter | GOR 100 m3/m3 | Water Content 10% | GOR 60 m3/m3 | Water Content 50% | GOR 20 m3/m3 | Water Content 80% | |||
|---|---|---|---|---|---|---|---|---|---|
| CMSRS | SMSRS | Error/(%) | CMSRS | SMSRS | Error/(%) | CMSRS | SMSRS | Error/(%) | |
| pd/MPa | 5.91 | 8.89 | −33.52 | 8.19 | 9.43 | −13.15 | 9.57 | 9.84 | −2.74 |
| PRLmax/KN | 61.11 | 73.69 | −17.07 | 71.14 | 76.02 | −6.42 | 76.96 | 77.86 | −1.16 |
| PRLmin/KN | 21.93 | 18.23 | 20.30 | 18.01 | 16.07 | 12.07 | 16.62 | 16.33 | 1.78 |
| Pprm/KW | 8.56 | 10.44 | −18.01 | 10.80 | 11.79 | −8.40 | 12.59 | 12.83 | −1.87 |
| Ppm/KW | 2.94 | 4.61 | −36.23 | 5.19 | 6.12 | −15.20 | 7.11 | 7.36 | −3.40 |
| Q/m3/d | 42.02 | 40.24 | 4.43 | 67.13 | 65.96 | 1.77 | 87.31 | 87.02 | 0.33 |
| Parameter | Water Content 10%, GOR 100 m3/m3 | Water Content 50%, GOR 60 m3/m3 | Water Content 80%, GOR 20 m3/m3 |
|---|---|---|---|
| Ne1/kW | 3.12 | 6.12 | 7.36 |
| Ne2/kW | 2.91 | 5.16 | 7.10 |
| Ne3/kW | 2.94 | 5.19 | 7.11 |
| Ne2l/kW | 1.67 | 4.34 | 6.95 |
| Ne2g/kW | 1.24 | 0.82 | 0.15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ma, B.; Dong, S. Coupling Simulation of Longitudinal Vibration of Rod String and Multi-Phase Pipe Flow in Wellbore and Research on Downhole Energy Efficiency. Energies 2023, 16, 4988. https://doi.org/10.3390/en16134988
Ma B, Dong S. Coupling Simulation of Longitudinal Vibration of Rod String and Multi-Phase Pipe Flow in Wellbore and Research on Downhole Energy Efficiency. Energies. 2023; 16(13):4988. https://doi.org/10.3390/en16134988
Chicago/Turabian StyleMa, Biao, and Shimin Dong. 2023. "Coupling Simulation of Longitudinal Vibration of Rod String and Multi-Phase Pipe Flow in Wellbore and Research on Downhole Energy Efficiency" Energies 16, no. 13: 4988. https://doi.org/10.3390/en16134988
APA StyleMa, B., & Dong, S. (2023). Coupling Simulation of Longitudinal Vibration of Rod String and Multi-Phase Pipe Flow in Wellbore and Research on Downhole Energy Efficiency. Energies, 16(13), 4988. https://doi.org/10.3390/en16134988





