Numerical Simulation on Radial Well Deflagration Fracturing Based on Phase Field Method
Abstract
1. Introduction
2. Phase Field Fracture Theory and Model Verification
2.1. Phase Field Fracture Theory
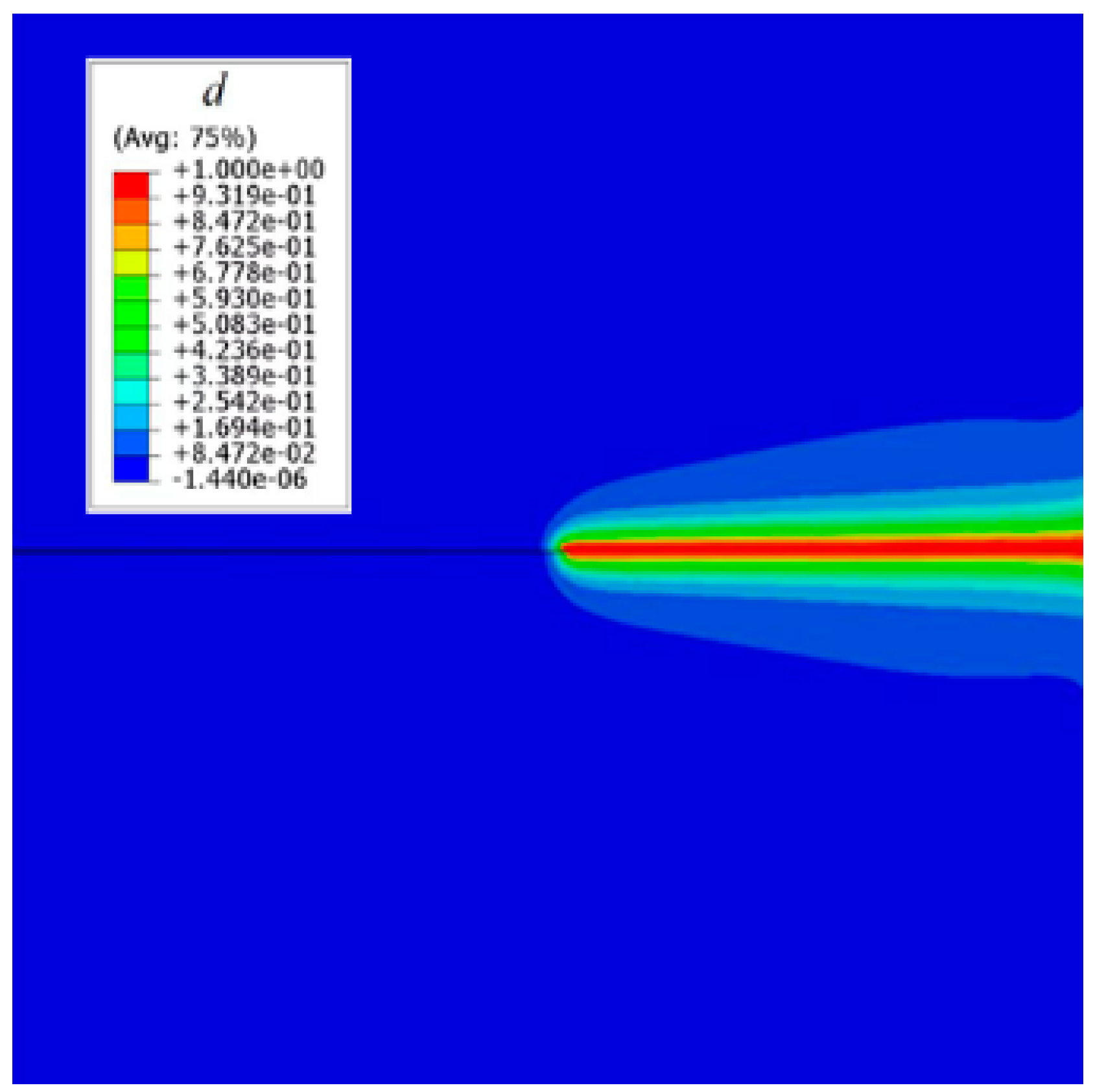
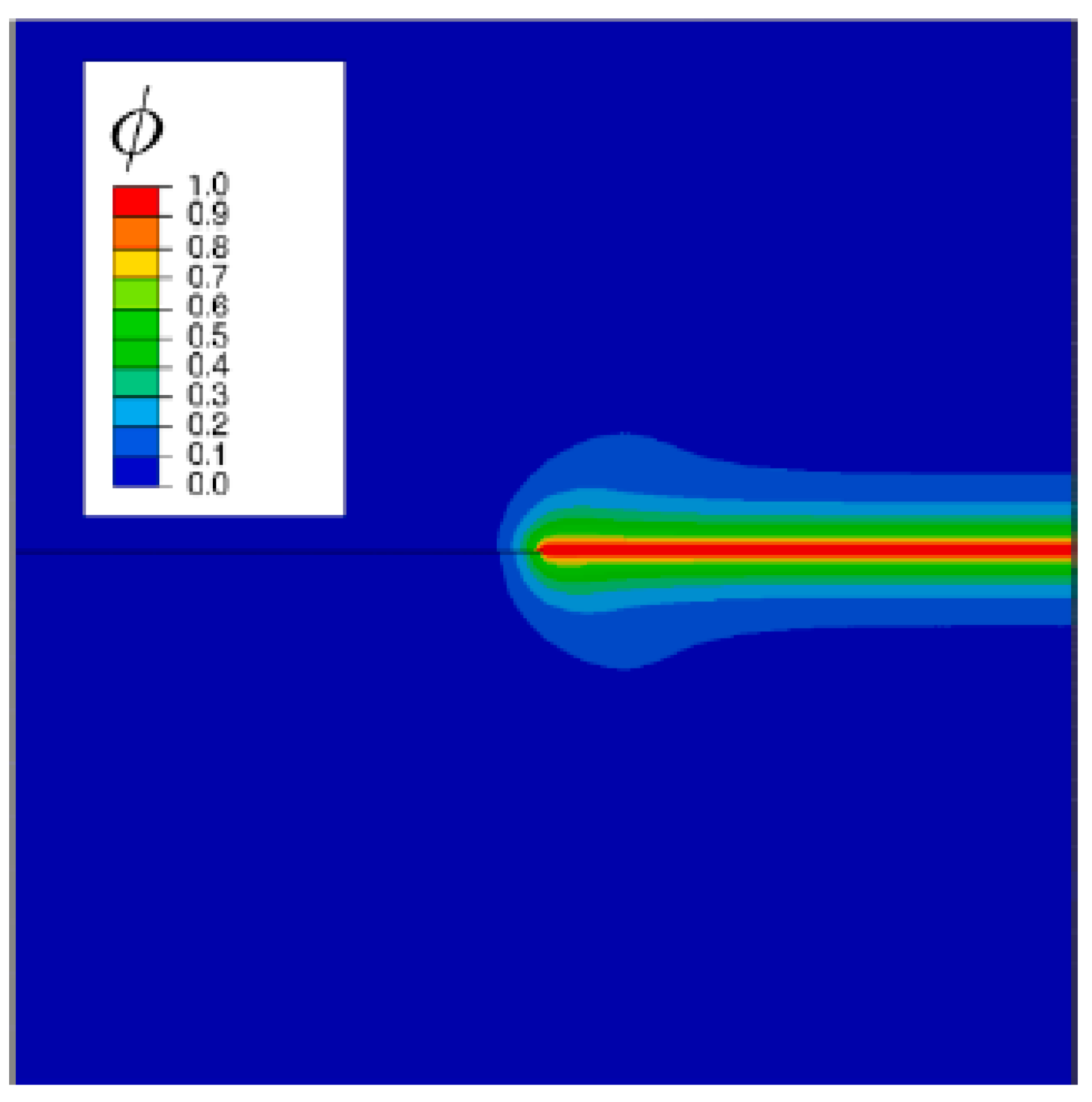
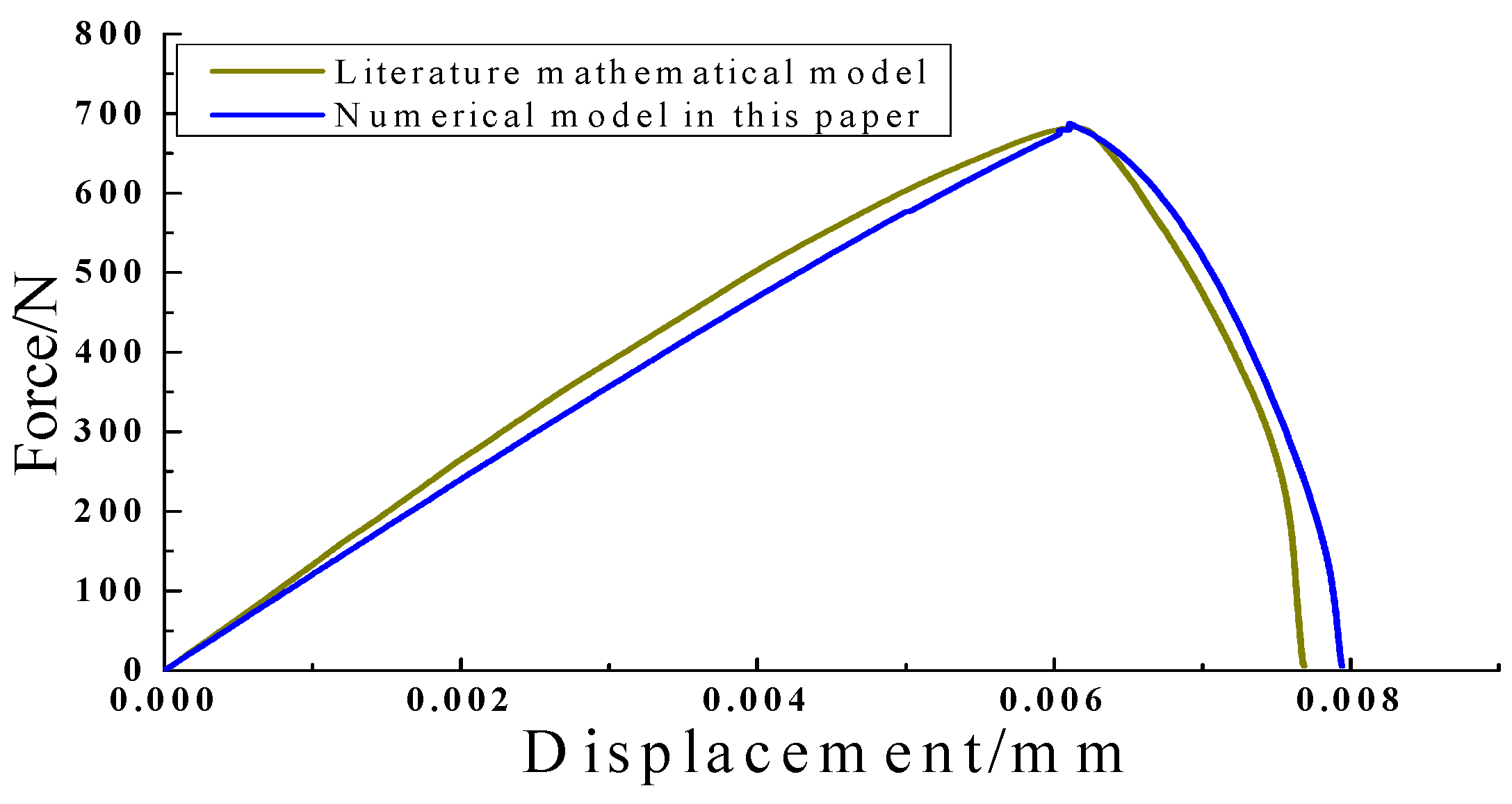
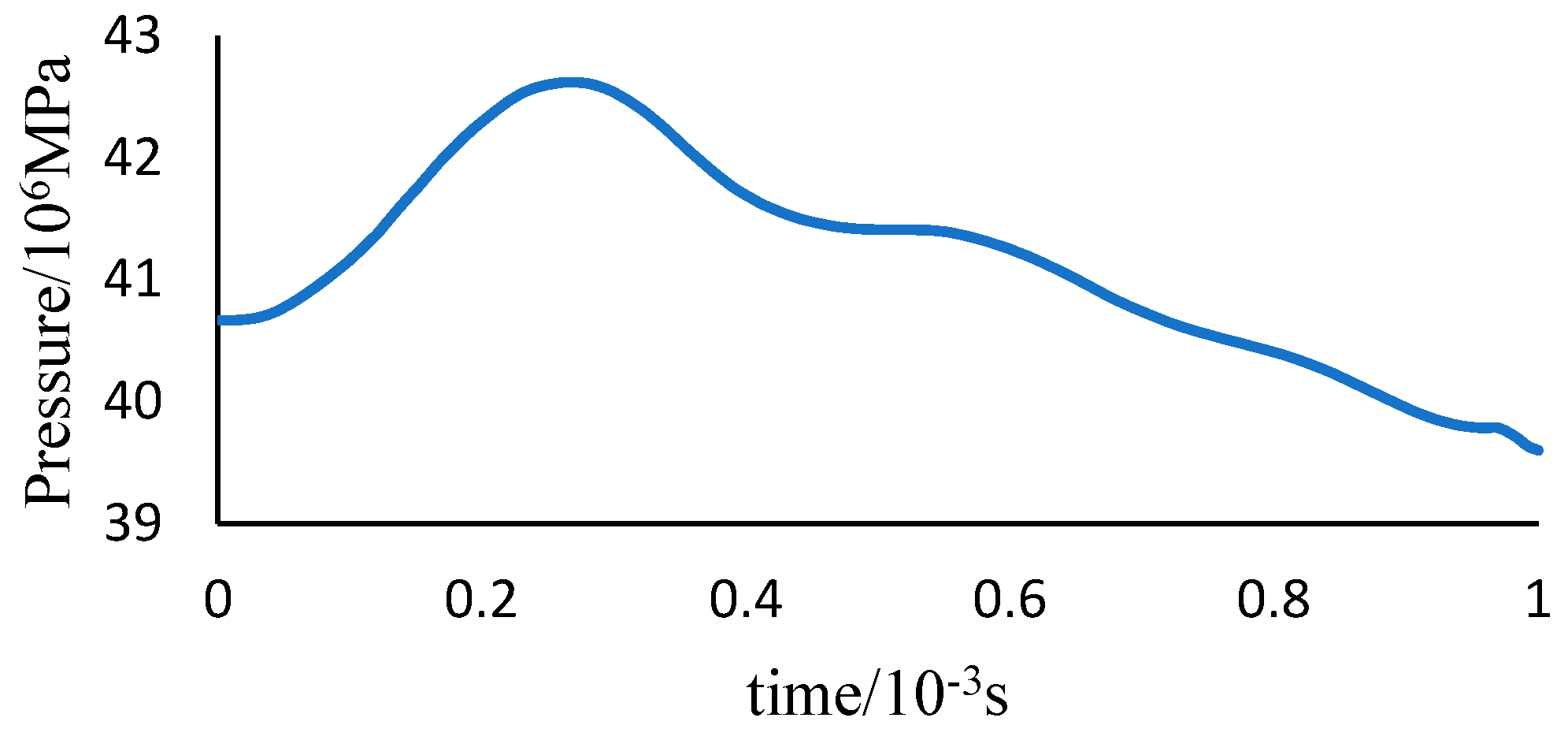
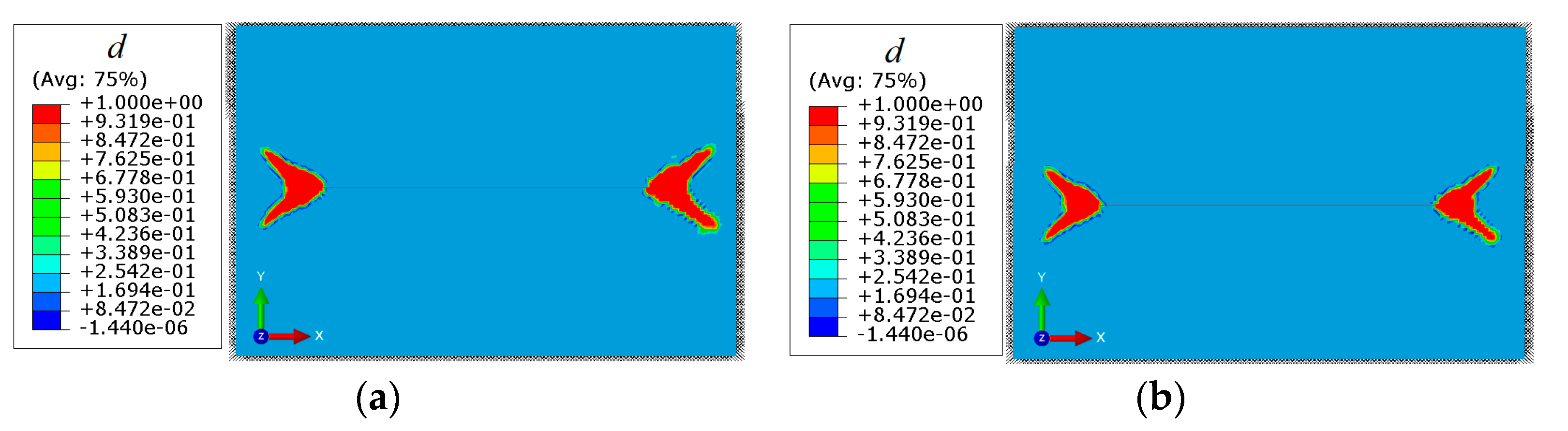
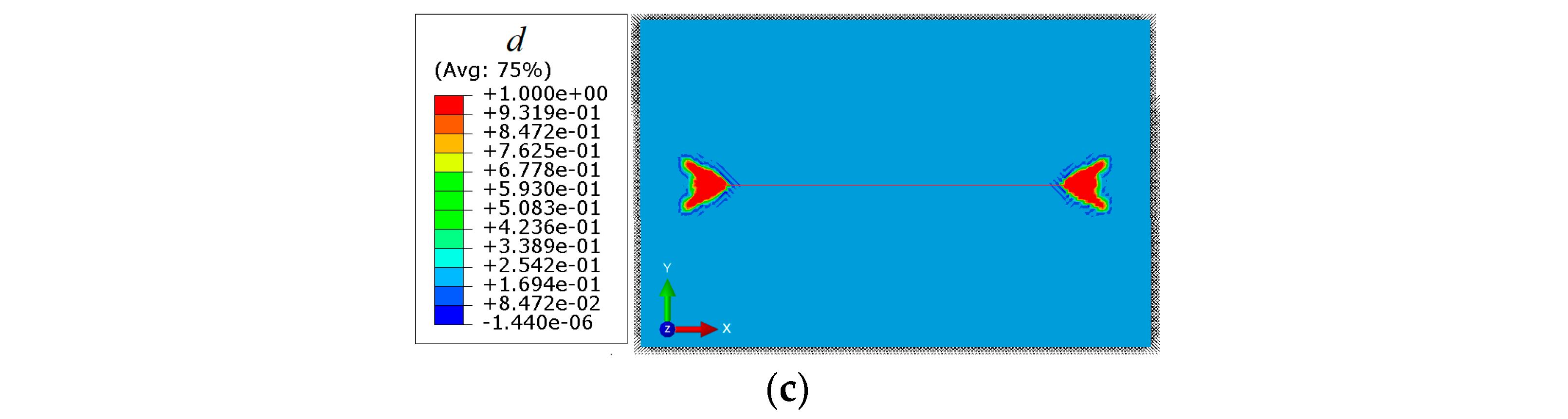
2.2. Numerical Model Verification
3. Analysis of Results
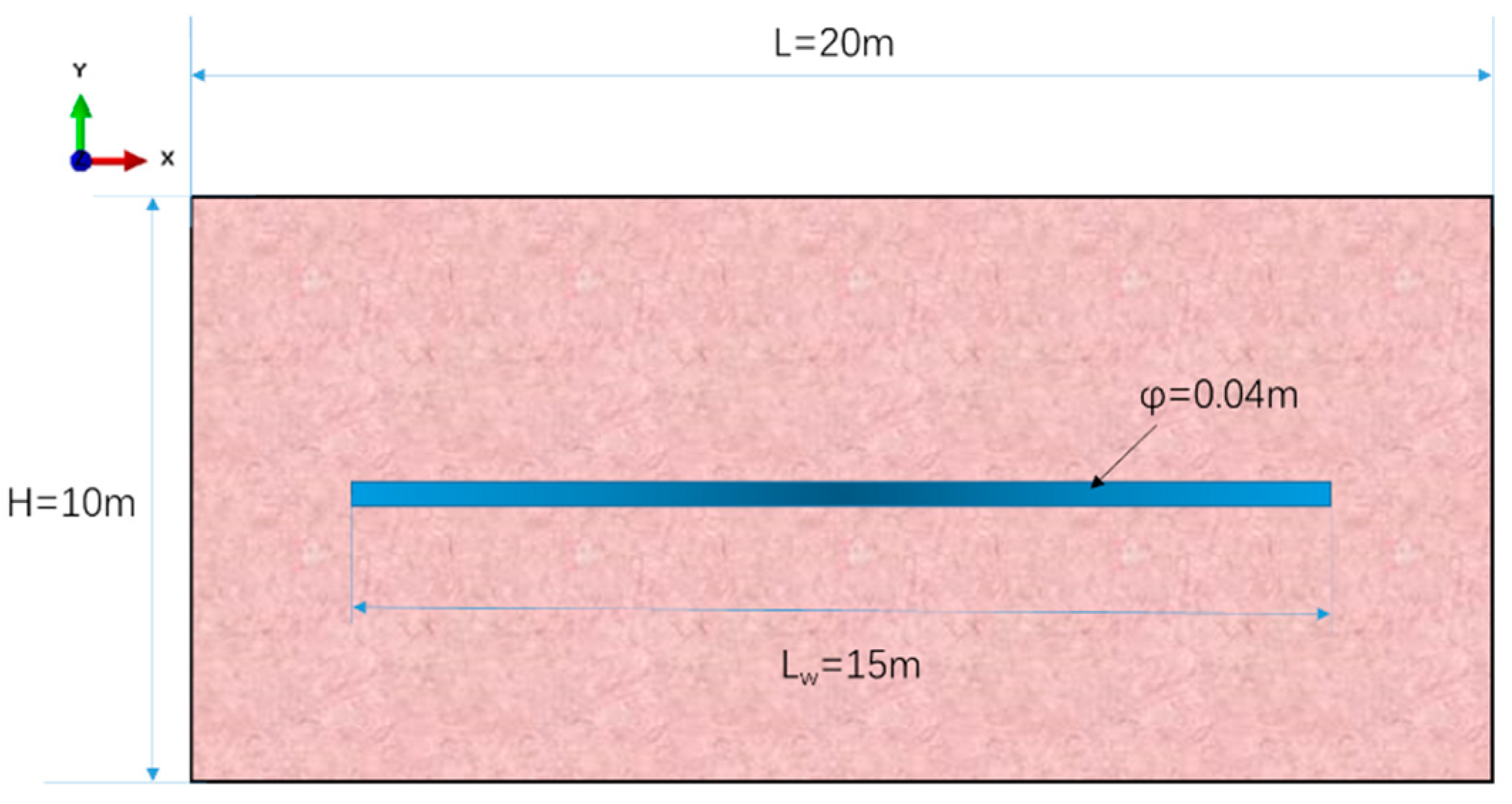
3.1. Establishment of Model of Radial Well Deflagration Fracturing
3.2. Fracture Propagation Mechanism in Deflagration Fracturing
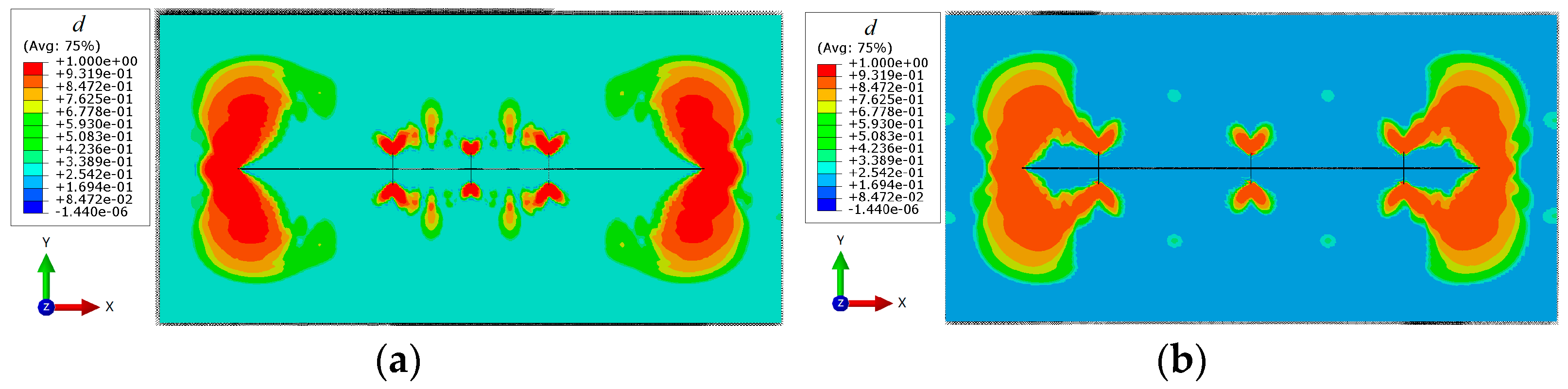
3.2.1. Effect of Horizontal Principal Stress Difference on Fracture Propagation in Case of No Natural Fractures
3.2.2. Effect of Natural Fracture Distribution on Fracture Propagation
3.2.3. Effect of Natural Fracture Density on Fracture Propagation
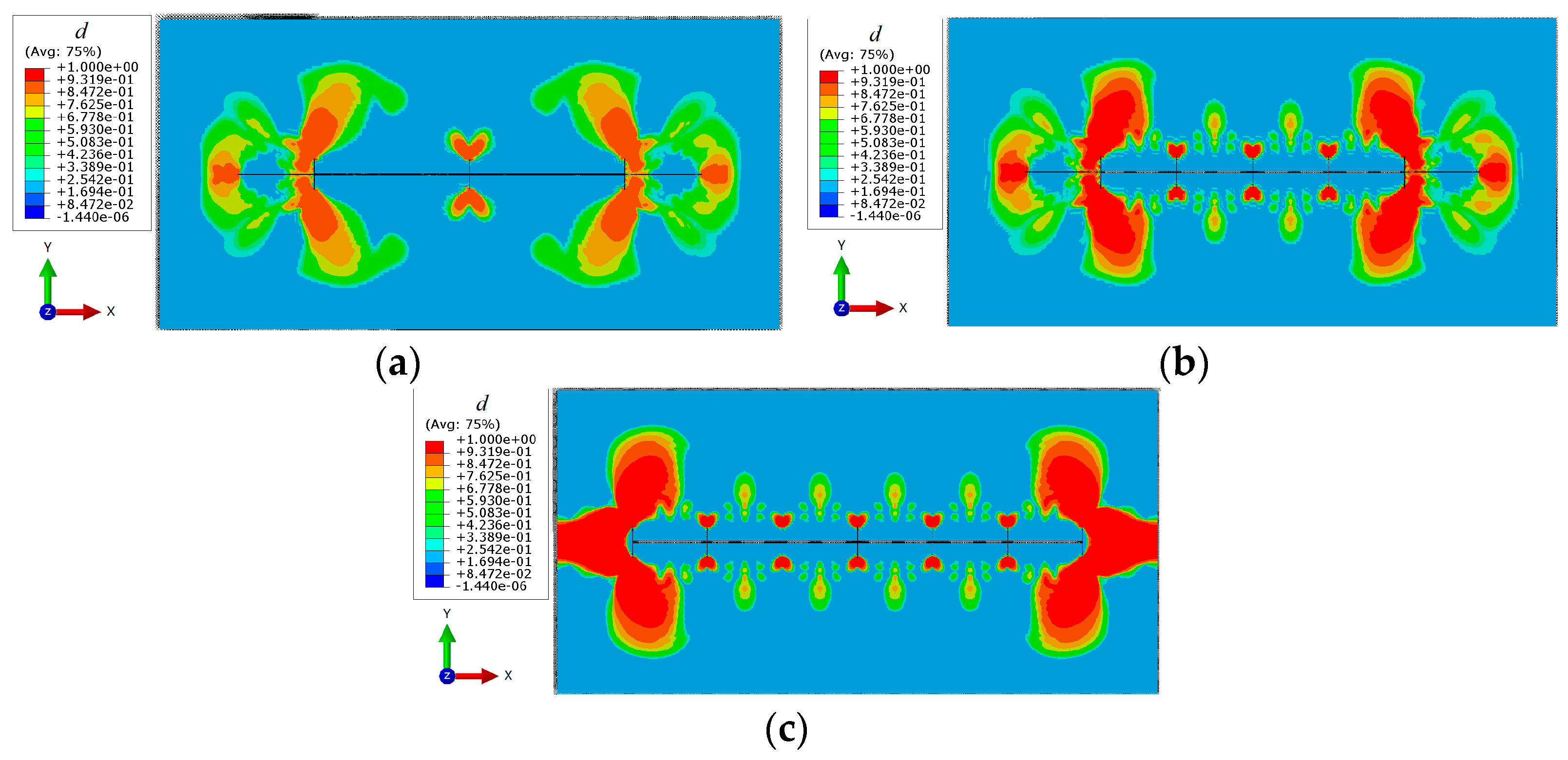
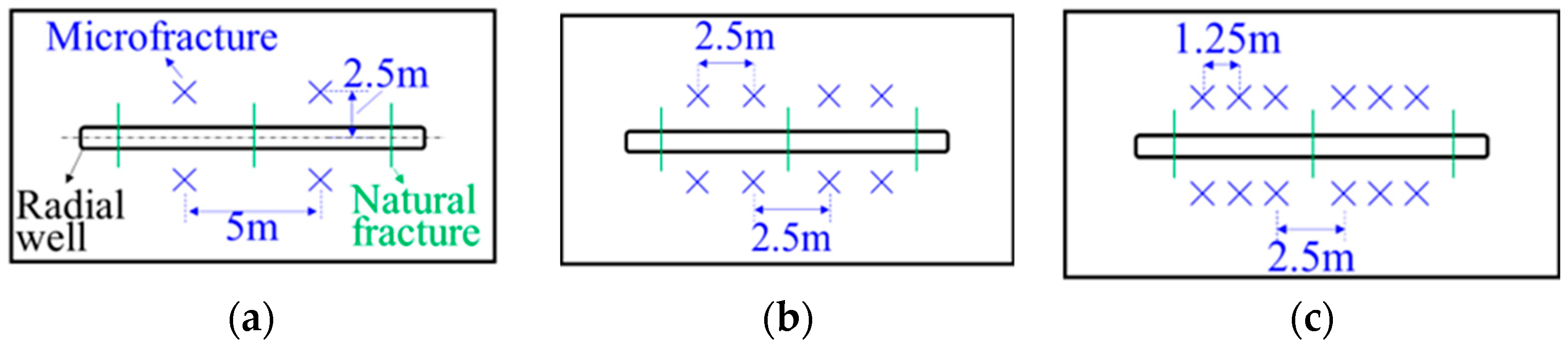
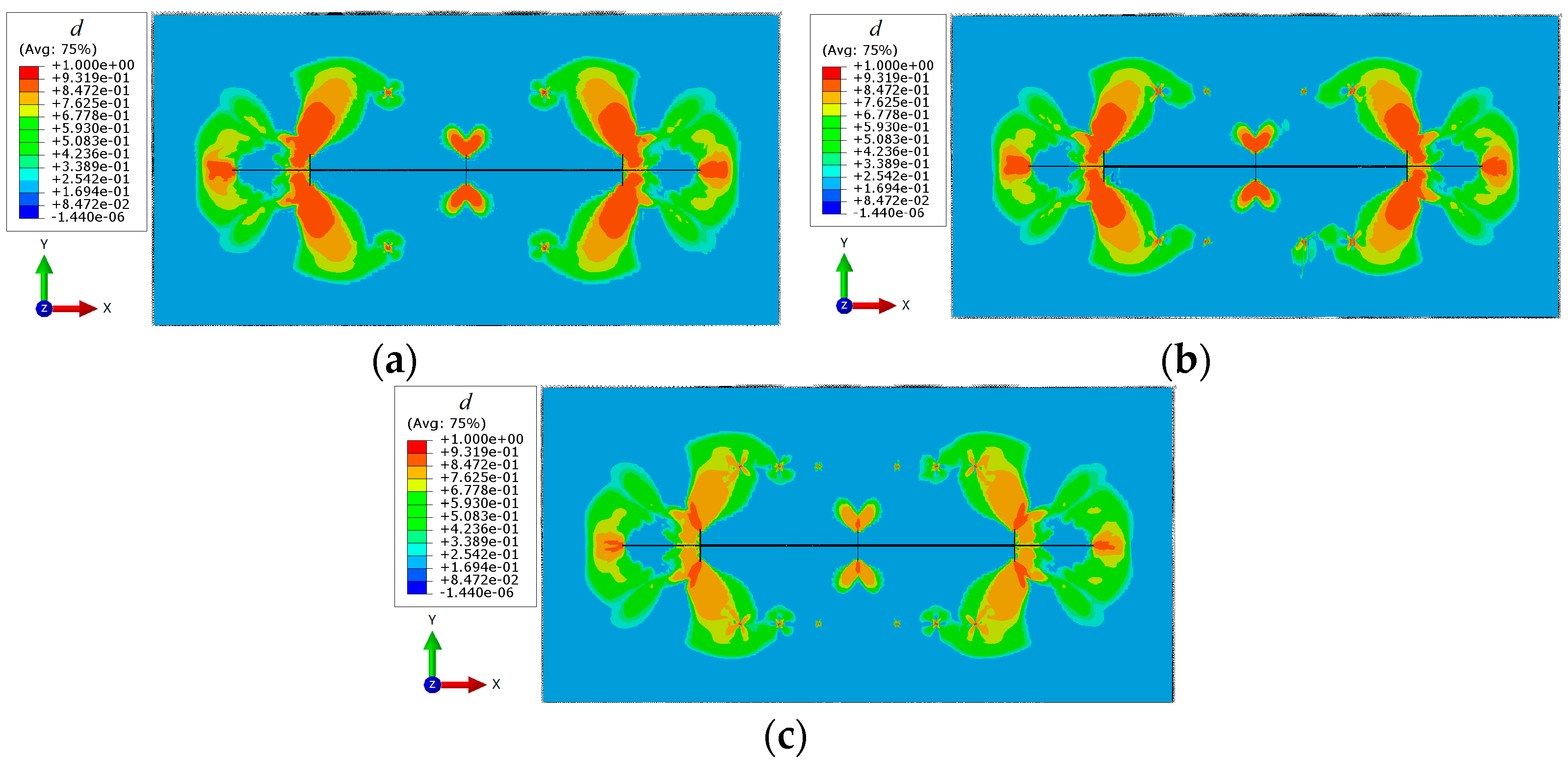
3.2.4. Effect of Micro-Fracture Distribution on Fracture Propagation
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Xin, J.; Shunhui, Y.; Zhiqiang, H.; Xianzhi, S.; Hongbao, Z.; Yang, C. The experimental study on the coal rock breaking efficiency of the self-rotating multi-orifice nozzle applied in URRS technique. IOP Conf. Ser. Earth Environ. Sci. 2021, 861, 062085. [Google Scholar]
- Tiankui, G.; Binyan, L.; Zhanqing, Q.; Diguang, G.; Lei, X. Study on Initiation Mechanisms of Hydraulic Fracture Guided by Vertical Multi-radial Boreholes. Rock Mech. Rock Eng. 2017, 50, 1767–1785. [Google Scholar]
- Houben, G.J.; Collins, S.; Bakker, M.; Daffner, T.; Triller, F.; Kacimov, A. Review: Horizontal, directionally drilled and radial collector wells. Hydrogeol. J. 2022, 30, 329–357. [Google Scholar] [CrossRef]
- Egberts, P.; Peters, E. Well testing of radial jet drilling wells in geothermal reservoirs. Comput. Geosci. 2022, 26, 1449–1463. [Google Scholar] [CrossRef]
- He, J.C.; Zhang, K.S.; Liu, H.B.; Tang, M.R.; Zheng, X.L.; Zhang, G.Q. Laboratory investigation on hydraulic fracture propagation in sandstone-mudstone-shale layers. Pet. Sci. 2022, 19, 1664–1673. [Google Scholar] [CrossRef]
- Abdelaziz, A.; Ha, J.; Li, M.; Magsipoc, E.; Sun, L.; Grasselli, G. Understanding hydraulic fracture mechanisms: From the laboratory to numerical modelling. Adv. Geo-Energy Res. 2023, 7, 66–68. [Google Scholar] [CrossRef]
- Song, J.H.; Wang, H.; Belytschko, T. A comparative study on finite element methods for dynamic fracture. Comput. Mech. 2008, 42, 239–250. [Google Scholar] [CrossRef]
- Bourdin, B.; Francfort, G.A.; Marigo, J.J. Numerical experiments in revisited brittle fracture. J. Mech. Phys. Solids 2000, 48, 797–826. [Google Scholar] [CrossRef]
- Gravouil, A.; Moës, N.; Belytschko, T. Non-planar 3D crack growth by the extended finite element and level sets-Part II: Level set update. Int. J. Numer. Methods Eng. 2002, 53, 2569–2586. [Google Scholar] [CrossRef]
- Sukumar, N.; Chopp, D.L.; Béchet, E.; Moës, N. Three-dimensional non-planar crack growth by a coupled extended finite element and fast marching method. Int. J. Numer. Methods Eng. 2008, 76, 727–748. [Google Scholar] [CrossRef]
- Li, J.; Dong, S.; Hua, W.; Li, X.; Pan, X. Numerical investigation of hydraulic fracture propagation based on cohesive zone model in naturally fractured formations. Processes 2019, 7, 28. [Google Scholar] [CrossRef]
- Li, J.; Hua, W.; Tang, H.; Huang, J.; Dong, S. Stress intensity factors and T-stress for an edge cracked Brazilian disk specimen under diametrically distributed load. Theor. Appl. Fract. Mech. 2022, 120, 103402. [Google Scholar] [CrossRef]
- Menouillard, T.; Belytschko, T. Dynamic fracture with meshfree enriched XFEM. Acta Mech. 2010, 213, 53–69. [Google Scholar] [CrossRef]
- Xu, D.; Liu, Z.; Liu, X.; Zeng, Q.; Zhuang, Z. Modeling of dynamic crack branching by enhanced extended finite element method. Comput. Mech. 2014, 54, 489–502. [Google Scholar] [CrossRef]
- Belytschko, T.; Chen, H.; Xu, J.; Zi, G. Dynamic crack propagation based on loss of hyperbolicity and a new discontinuous enrichment. Int. J. Numer. Methods Eng. 2003, 58, 1873–1905. [Google Scholar] [CrossRef]
- Guo, T.K.; Luo, Z.L.; Zhou, J.; Gong, Y.Z.; Dai, C.L.; Tang, J.; Yu, Y.; Xiao, B.; Niu, B.L.; Ge, J.J. Numerical simulation on proppant migration and placement within the rough and complex fractures. Pet. Sci. 2022, 19, 2268–2283. [Google Scholar] [CrossRef]
- Wu, S.; Xu, X. A study of three intrinsic problems of the classic discrete element method using flat-joint model. Rock Mech. Rock Eng. 2015, 49, 1813–1830. [Google Scholar] [CrossRef]
- Chen, F.; Li, Y.; Shi, T.; Yan, H. Numerical Simulation of Full Phases of Collapse of Three-dimensional Cylindrical Granular Pile. Chin. J. Theor. Appl. Mech. 2022, 54, 1572–1589. [Google Scholar]
- Ilyushin, Y.V.; Asadulagi, M.A.M. Development of a Distributed Control System for the Hydrodynamic Processes of Aquifers, Taking into Account Stochastic Disturbing Factors. Water 2023, 15, 770. [Google Scholar] [CrossRef]
- Wu, J.Y.; Nguyen, V.P.; Nguyen, C.T.; Sutula, D.; Sinaie, S.; Bordas, S.P. Chapter one-phase-field modeling of fracture. Adv. Appl. Mech. 2020, 53, 1–183. [Google Scholar]
- Diehl, P.; Lipton, R.; Wick, T.; Tyagi, M. A comparative review of peridynamics and phase-field models for engineering fracture mechanics. Comput. Mech. 2022, 69, 1259–1293. [Google Scholar] [CrossRef]
- Francfort, G.A.; Marigo, J.J. Revisiting brittle fracture as an energy minimization problem. J. Mech. Phys. Solids 1998, 46, 1319–1342. [Google Scholar] [CrossRef]
- Miehe, C.; Welschinger, F.; Hofacker, M. Thermodynamically consistent phase-field models of fracture: Variational principles and multi-field FE implementations. Int. J. Numer. Methods Eng. 2010, 83, 1273–1311. [Google Scholar] [CrossRef]
- Miehe, C.; Hofacker, M.; Welschinger, F. A phase field model for rate-independent crack propagation: Robust algorithmic implementation based on operator splits. Comput. Methods Appl. Mech. Eng. 2010, 199, 2765–2778. [Google Scholar] [CrossRef]
- Liu, G.; Li, Q.; Msekh, M.A.; Zuo, Z. Abaqus implementation of monolithic and staggered schemes for quasi-static and dynamic fracture phase-field model. Comput. Mater. Sci. 2016, 121, 35–47. [Google Scholar] [CrossRef]
- Chu, D.; Li, X.; Liu, Z. Study the dynamic crack path in brittle material under thermal shock loading by phase field modeling. Int. J. Fract. 2017, 208, 115–130. [Google Scholar] [CrossRef]
- Liu, G.; Li, Q.; Zuo, Z. Implementation of a staggered algorithm for a phase field model in ABAQUS. Chin. J. Rock Mech. Eng. 2016, 35, 1019–1030. [Google Scholar]
- Liang, K. Mechanics; Higher Education Press: Beijing, China, 1995. [Google Scholar]
- Kristensen, P.K.; Martínez-Pañeda, E. Phase field fracture modelling using quasi-Newton methods and a new adaptive step scheme. Theor. Appl. Fract. Mech. 2020, 107, 137–144. [Google Scholar] [CrossRef]
- Li, L.; Zhang, T. Estimation of HEGF Crack Length Using Measured P-t Curves. In Proceedings of the Theory and Practice of Rock Crushing—Selected Papers from the Fifth National Symposium on Rock Crushing, Xi’an, China, September 1992; pp. 291–296. [Google Scholar]
- Cai, W.; Liu, Y.; Lv, S. Influence of firing-circuitry polarity to igniter p-t curve. Aero Weapon. 2005, 06, 47–49. [Google Scholar]
- Martirosyan, A.V.; Ilyushin, Y.V. The Development of the Toxic and Flammable Gases Concentration Monitoring System for Coalmines. Energies 2022, 15, 8917. [Google Scholar] [CrossRef]
- Makarova, A.A.; Kaliberda, I.V.; Kovalev, D.A.; Pershin, I.M. Modeling a Production Well Flow Control System Using the Example of the Verkhneberezovskaya Area. In Proceedings of the 2022 Conference of Russian Young Researchers in Electrical and Electronic Engineering (ElConRus), Saint Petersburg, Russia, 25–28 January 2022; pp. 760–764. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gong, D.; Chen, J.; Cheng, C.; Kou, Y.; Jiang, H.; Zhu, J. Numerical Simulation on Radial Well Deflagration Fracturing Based on Phase Field Method. Energies 2023, 16, 4758. https://doi.org/10.3390/en16124758
Gong D, Chen J, Cheng C, Kou Y, Jiang H, Zhu J. Numerical Simulation on Radial Well Deflagration Fracturing Based on Phase Field Method. Energies. 2023; 16(12):4758. https://doi.org/10.3390/en16124758
Chicago/Turabian StyleGong, Diguang, Junbin Chen, Cheng Cheng, Yuanyuan Kou, Haiyan Jiang, and Jianhong Zhu. 2023. "Numerical Simulation on Radial Well Deflagration Fracturing Based on Phase Field Method" Energies 16, no. 12: 4758. https://doi.org/10.3390/en16124758
APA StyleGong, D., Chen, J., Cheng, C., Kou, Y., Jiang, H., & Zhu, J. (2023). Numerical Simulation on Radial Well Deflagration Fracturing Based on Phase Field Method. Energies, 16(12), 4758. https://doi.org/10.3390/en16124758





