Nonlinear Hydraulic Vibration Modeling and Dynamic Analysis of Hydro-Turbine Generator Unit with Multiple Faults
Abstract
1. Introduction
2. Mathematical Modeling of HGU
2.1. Modeling of Asymmetric Hydraulic Forces
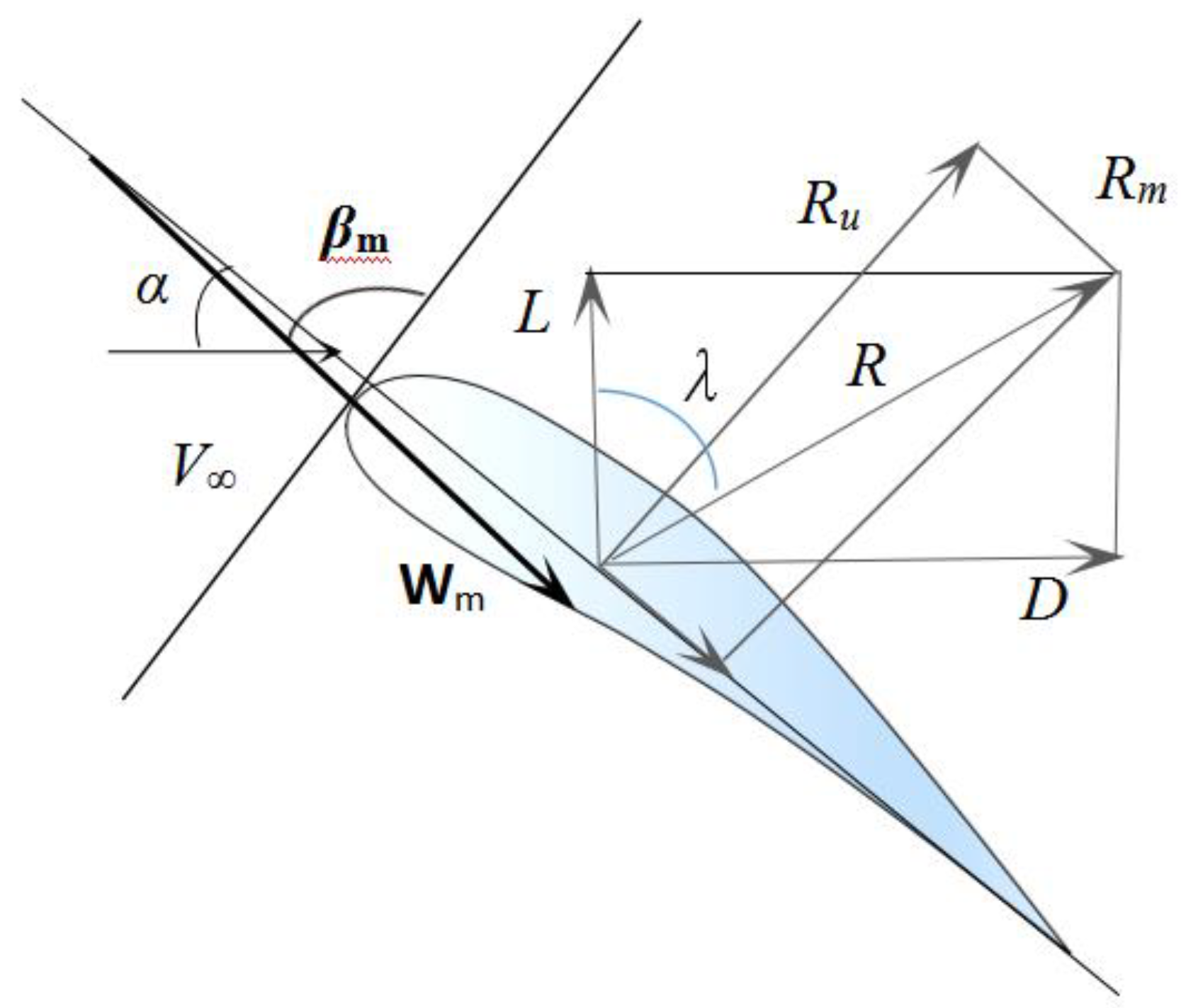
2.1.1. Radial Hydraulic Force of a Single Airfoil Blade
2.1.2. Lift and Drag Coefficients of the Asymmetric Airfoil Blade
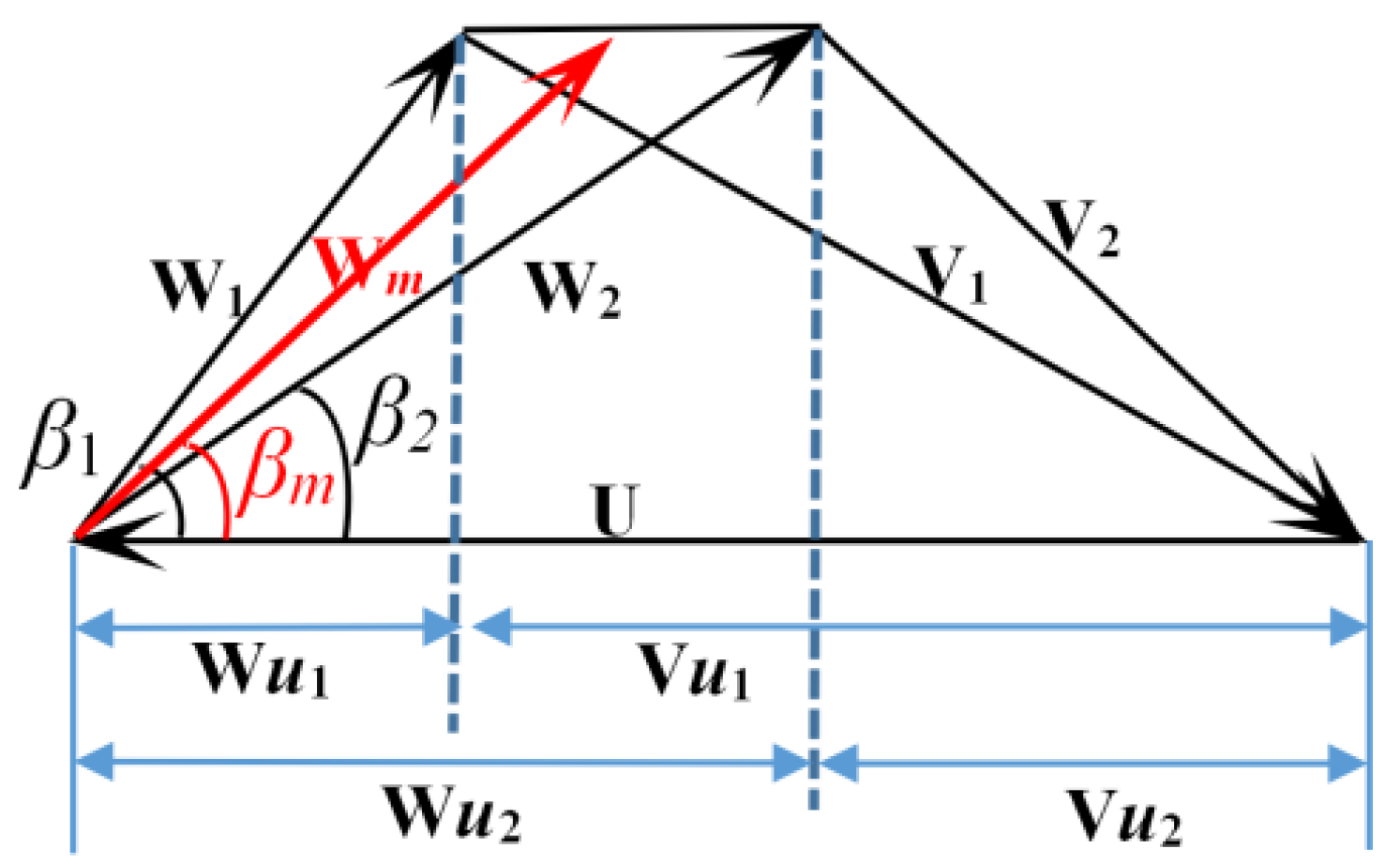
2.1.3. Average Relative Velocity of the Blade
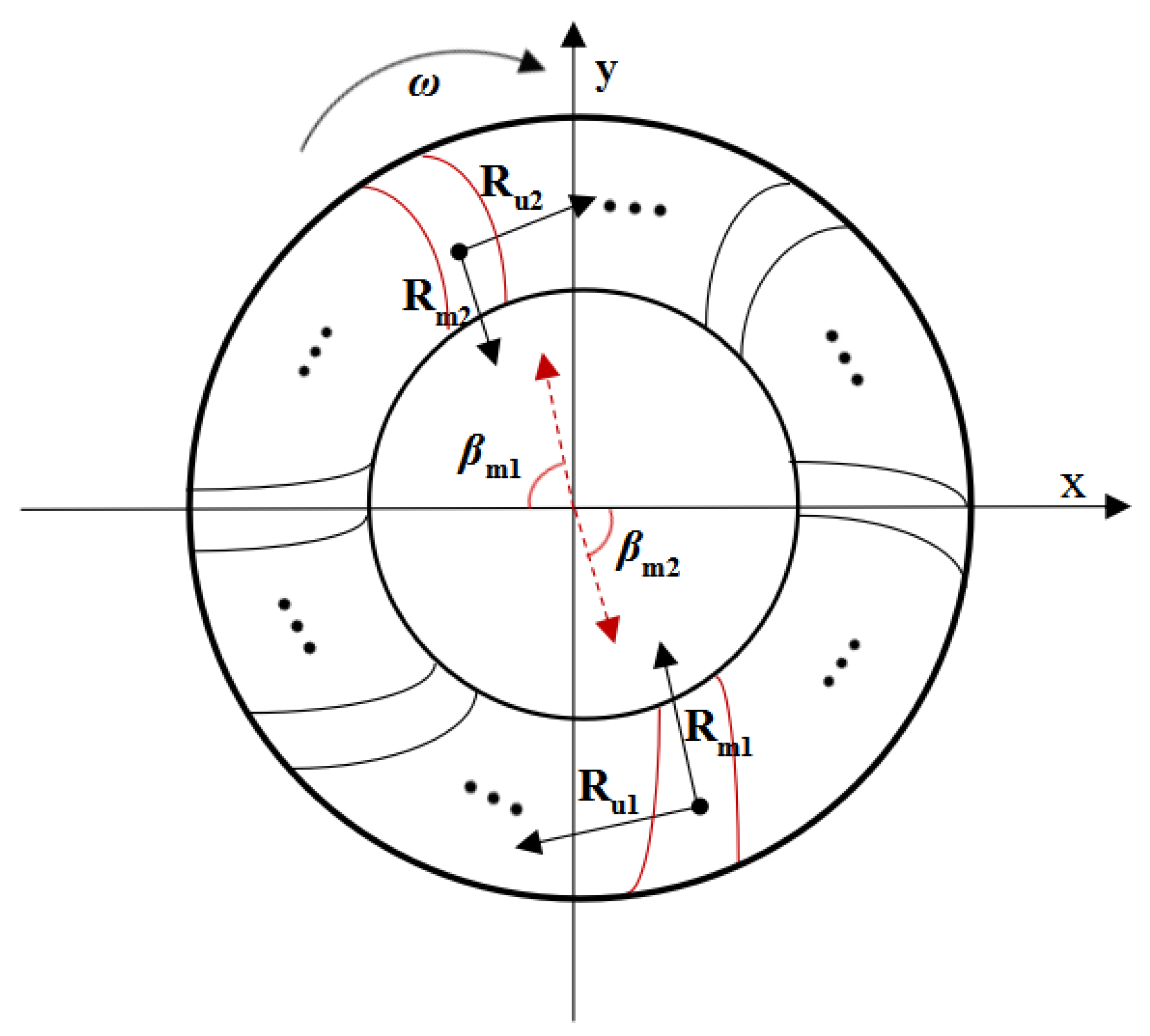
2.1.4. Asymmetric Hydraulic Forces of the Turbine Runner
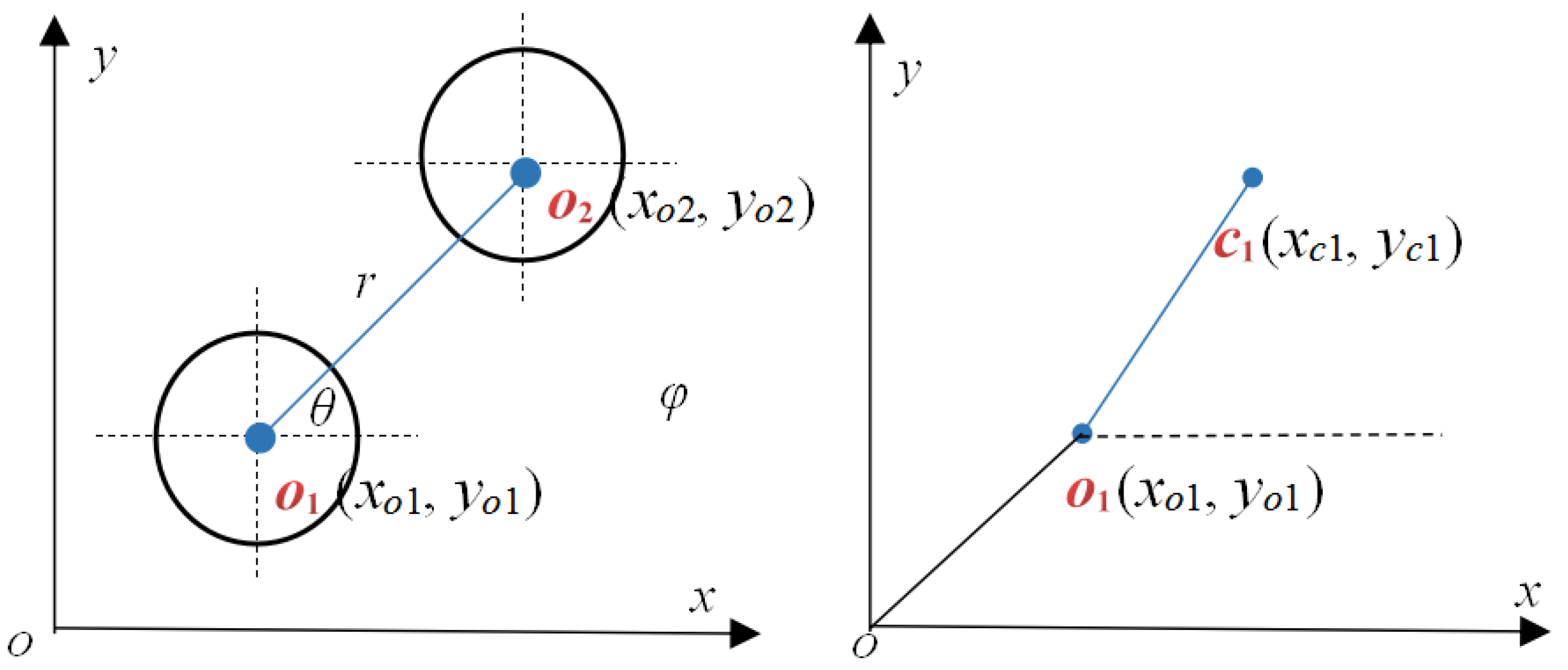
2.2. Modeling of the Mechanic and Electric Asymmetric Forces
2.3. Modeling of the HGU
3. Numerical Simulation and Analysis
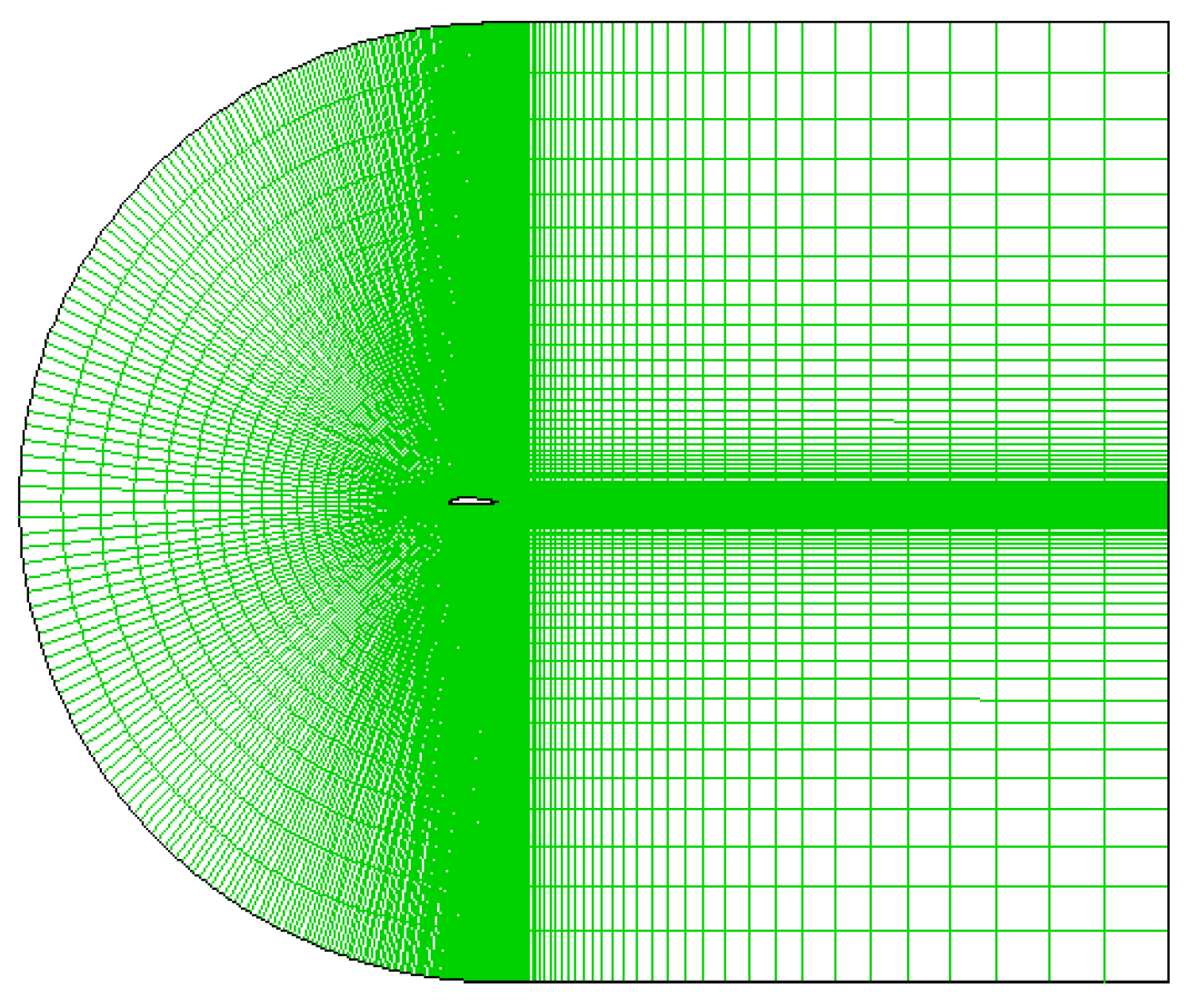
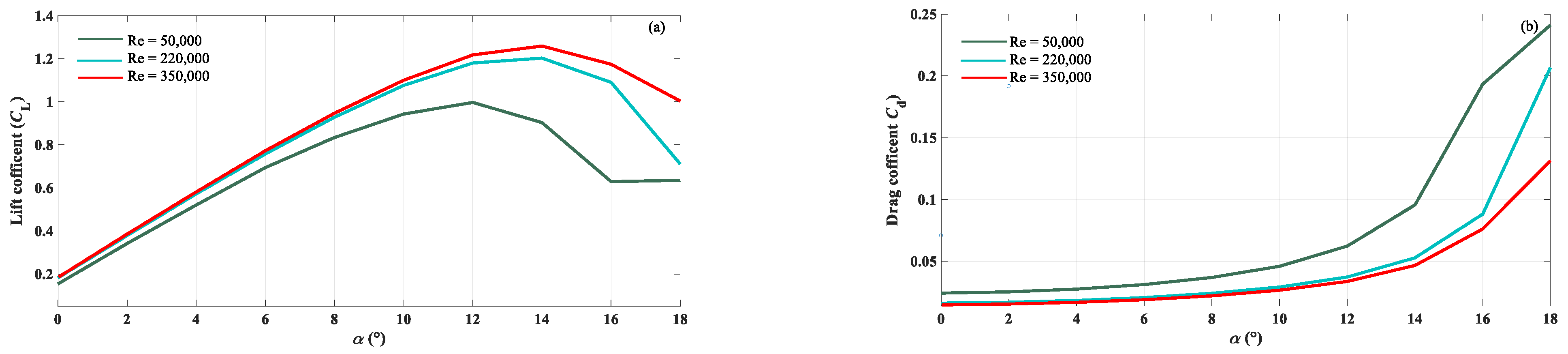
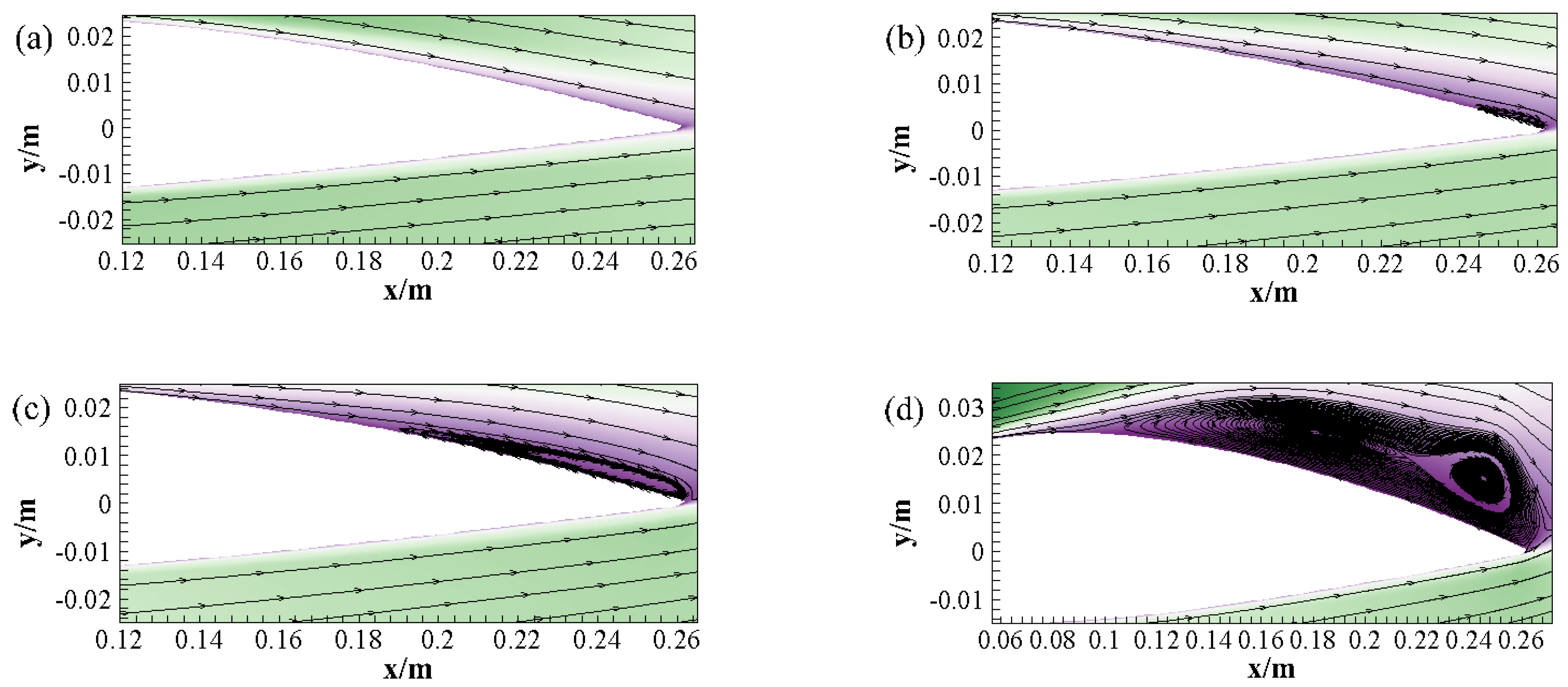
3.1. Calculation and Verification of the Lift and Drag Coefficients
3.2. Nonlinear Dynamic Analysis of the HGU
3.2.1. Comparison and Verification
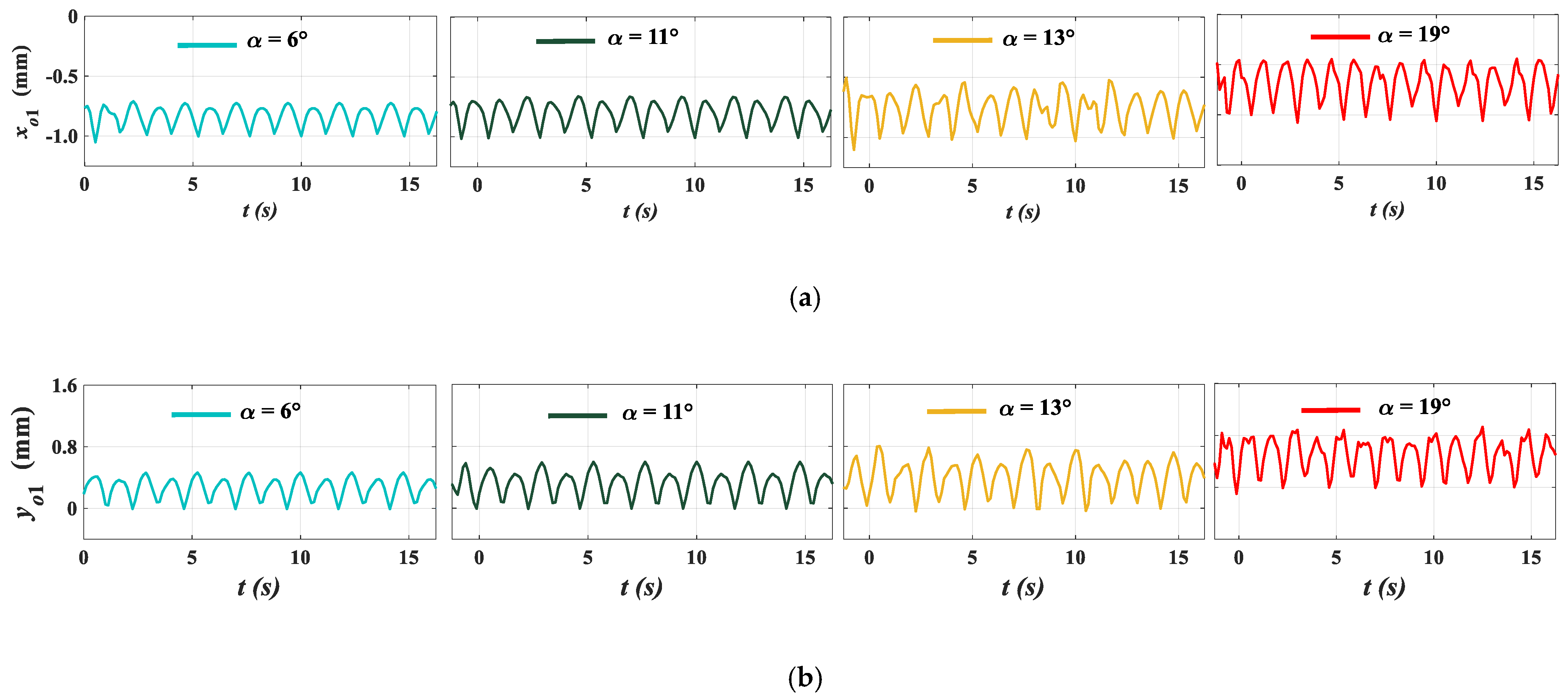
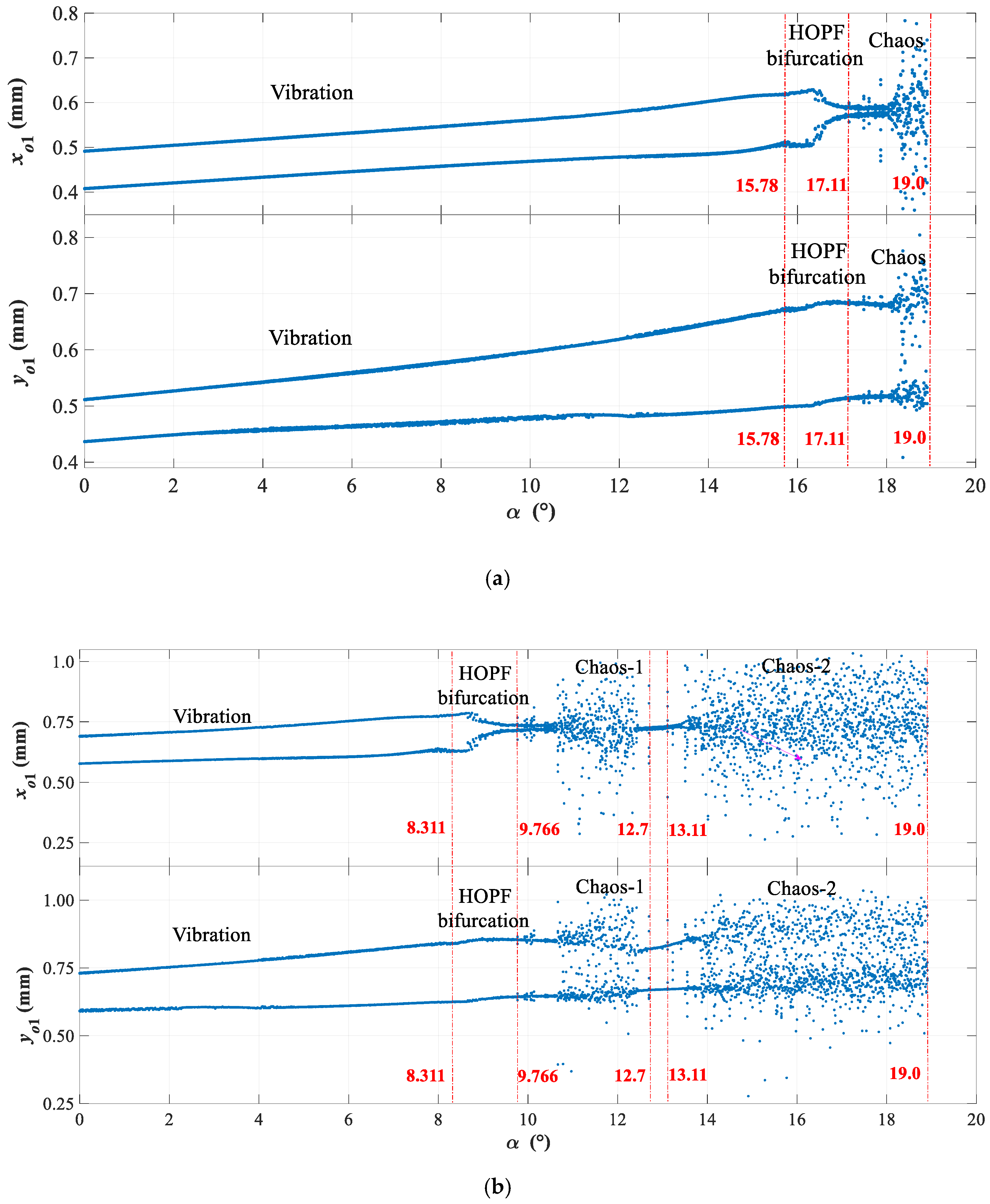
3.2.2. Effects of Attack Angle (α)
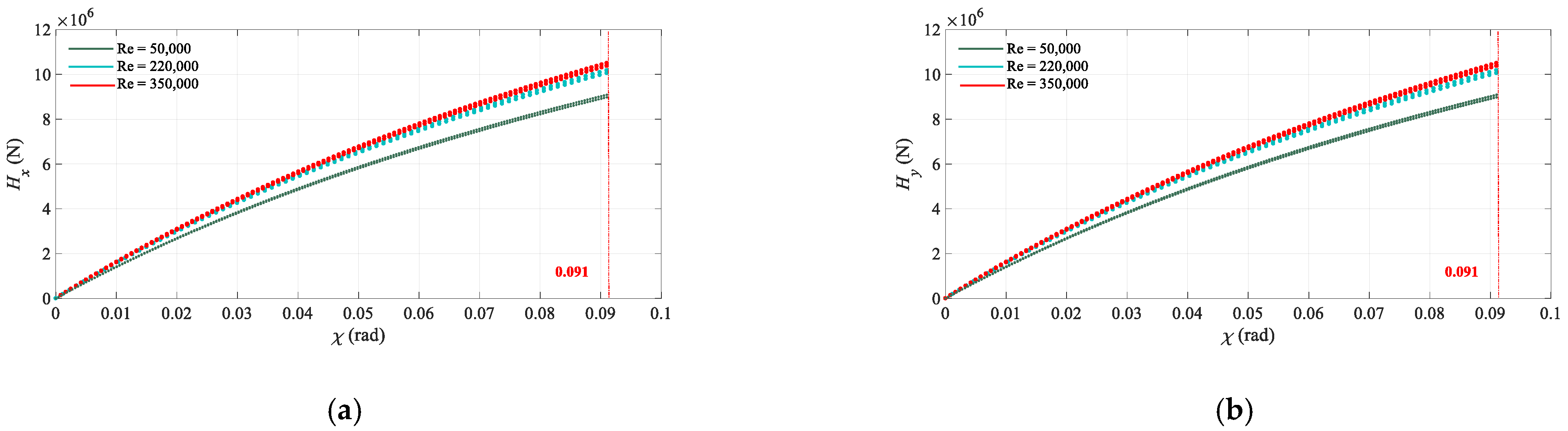
3.2.3. Effects of the Deviation of Outlet Blade Angle (χ)
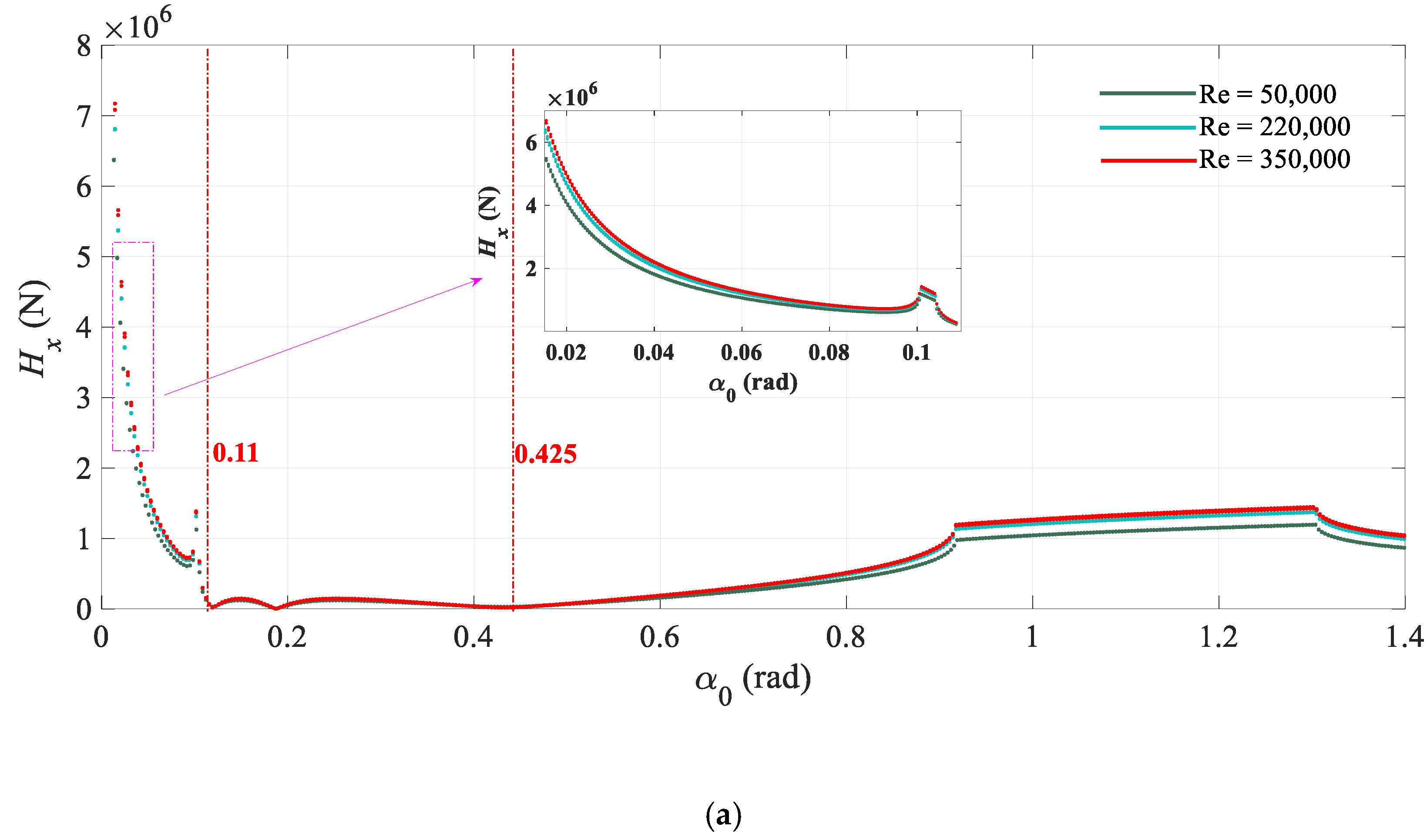
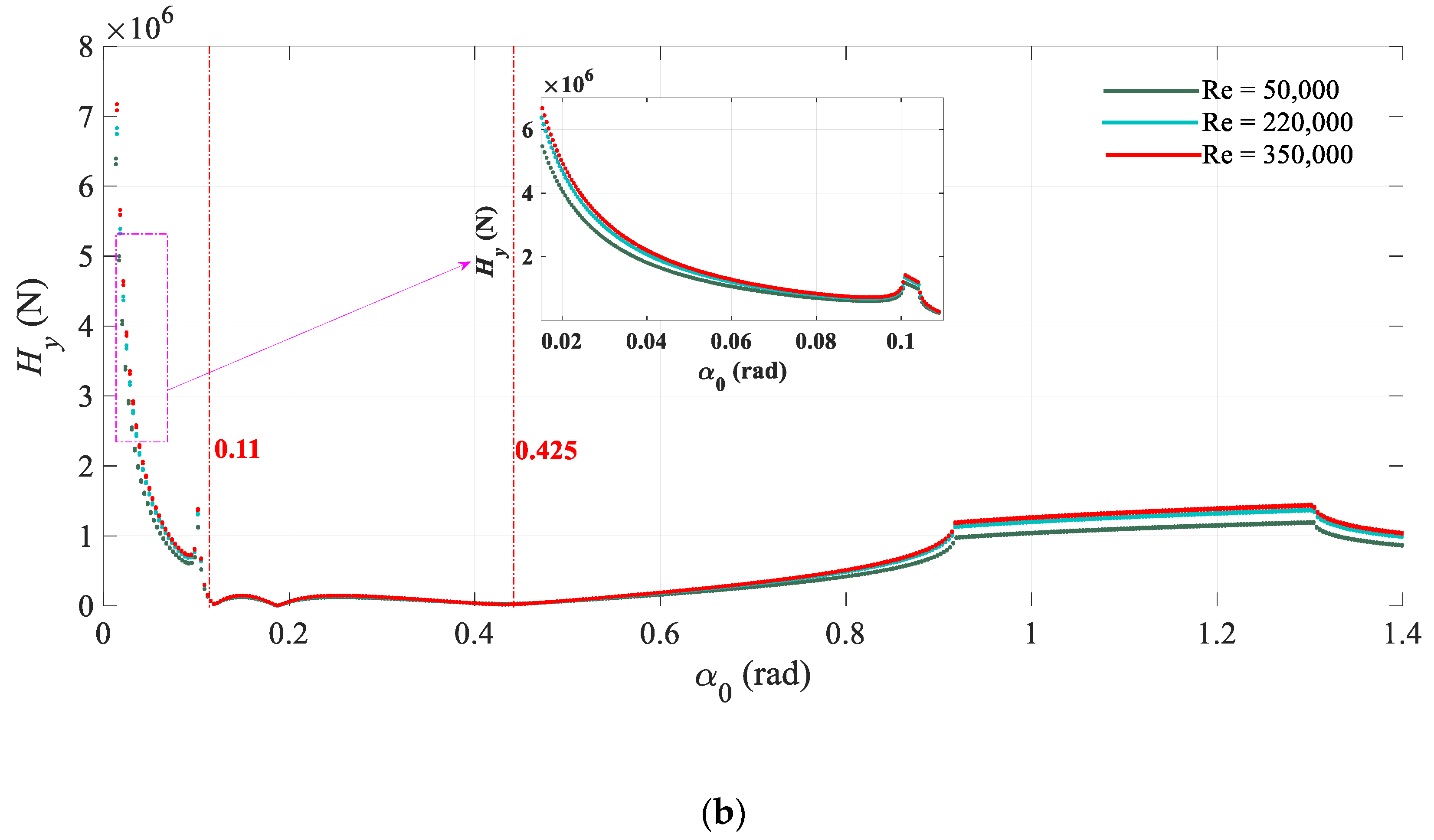
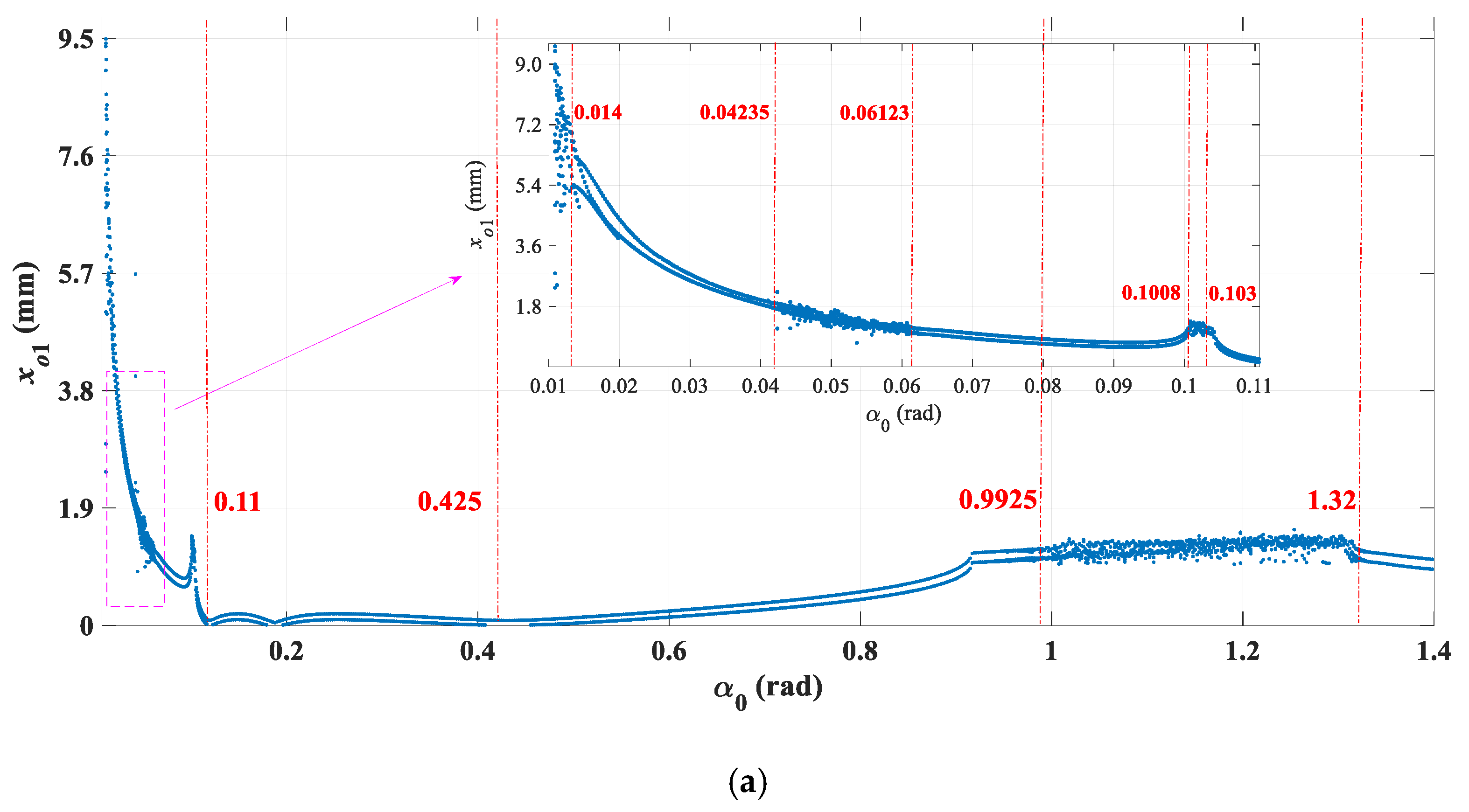
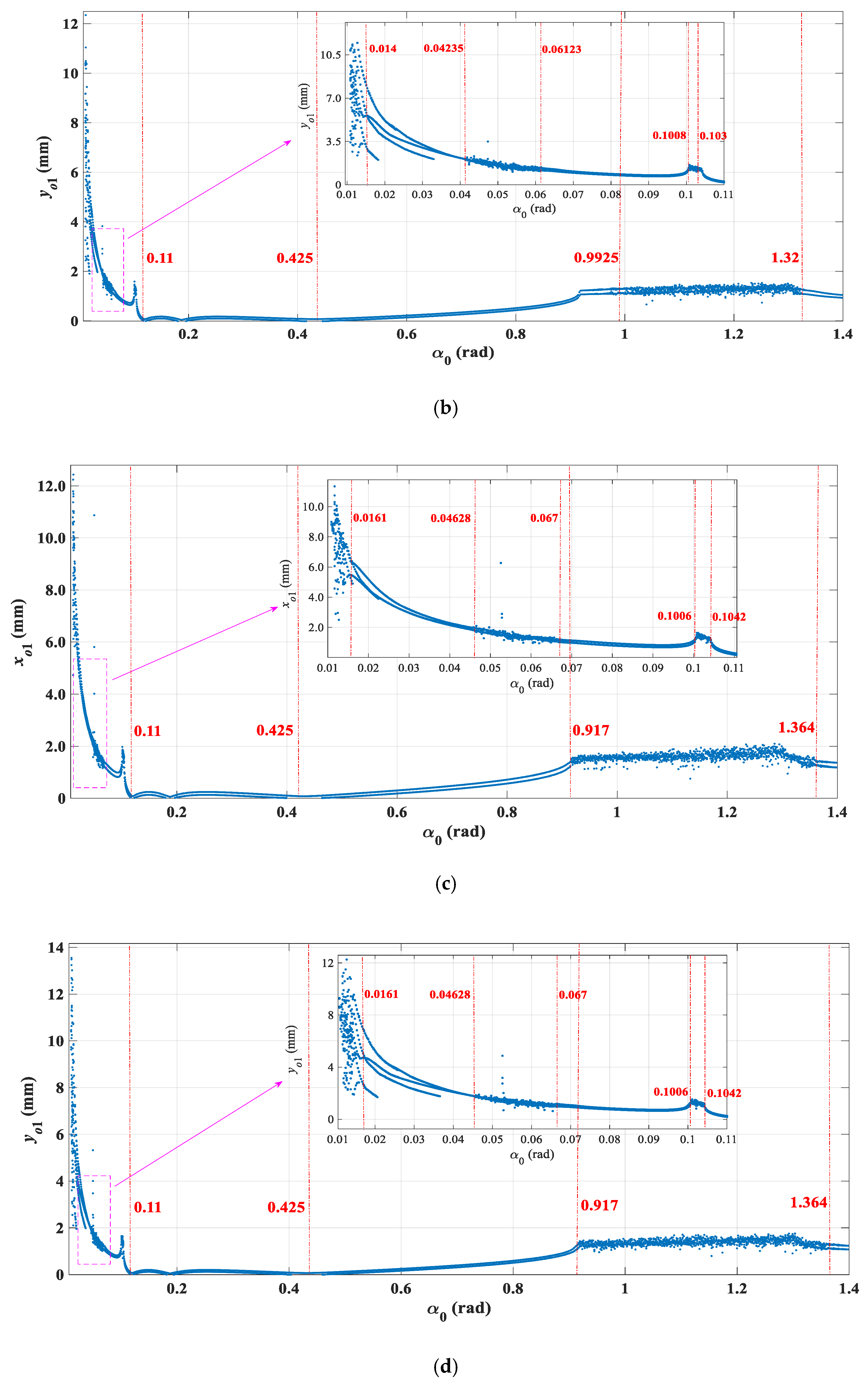
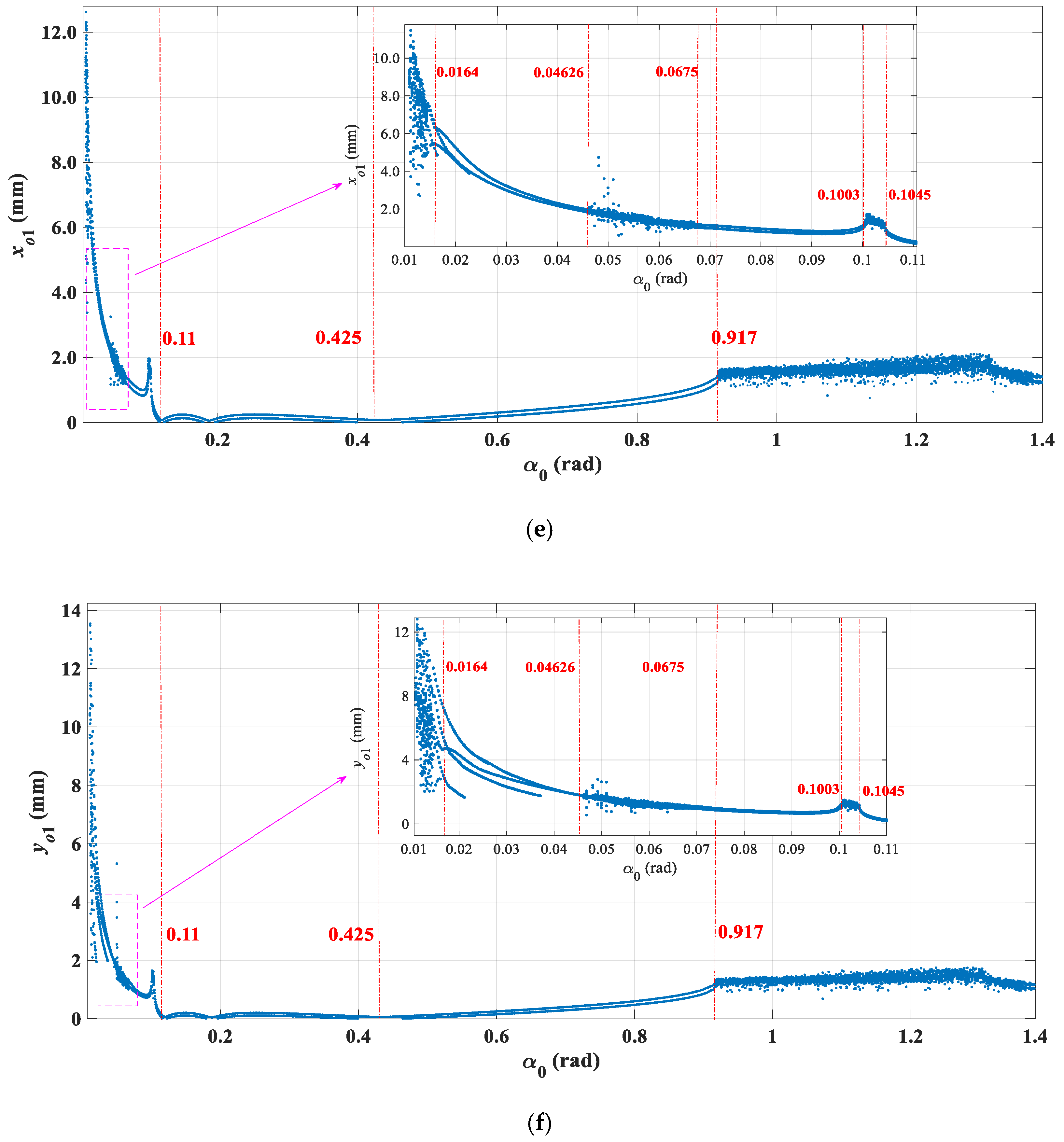
3.2.4. Effects of Outlet Guide Vane Angle (α1)
4. Conclusions
- (1)
- In the changing process of attack angle (α), deviation of the outlet blade angle (χ), and outlet guide vane angle (α1), asymmetric hydraulic forces have decisive effects on the vibration amplitude of the HGU.
- (2)
- The Reynolds number is less sensitive to the outlet guide vane angle (α1) than to the attack angle (α) and deviation of the outlet blade angle (χ), and the dynamic characteristics of the HGU with increasing outlet guide vane angle (α1) can only be effected by large changes in the Reynolds number.
- (3)
- Increasing the Reynolds number can enhance the effects of the attack angle (α) and the deviation of outlet blade angle (χ) on dynamic evolutions of the HGU, which leads to the points of the high variability state moving forward. Meanwhile, the stable ranges are respectively analyzed.
- (4)
- A larger or smaller outlet guide vane angle (α1) can both enhance the nonlinear hydraulic vibration, and the best range is from 0.11 rad to 0.425 rad. In addition, in the ranges of outlet guide vane angle (α1) from 0.917 rad to 1.4 rad, the Reynolds number can intensify the nonlinear hydraulic vibration.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Varney, P.; Green, I. Rough surface contact of curved conformal surfaces: An application to rotor-stator rub. J. Tribol. Trans. ASME 2016, 138, 041401. [Google Scholar] [CrossRef]
- Bovsunovsky, A.P. Efficiency analysis of vibration based crack diagnostics in rotating shafts. Eng. Fract. Mech. 2017, 173, 118–129. [Google Scholar] [CrossRef]
- Ramamurthy, S.T.; Wang, Y.X.; Hughes, T. Electromagnetic response of three-dimensional topological crystalline insulators. Phys. Rev. Lett. 2017, 118, 146602. [Google Scholar] [CrossRef] [PubMed]
- Si, G.Q.; Diao, L.; Zhu, J.W.; Lei, Y.H.; Zhang, Y.B. Attempt to generalize fractional-order electric elements to complex-order ones. Chin. Phys. B 2017, 26, 060503. [Google Scholar] [CrossRef]
- Trivedi, C.; Cervantes, M.J.; Gandhi, B.K. Investigation of a High Head Francis Turbine at Runaway Operating Conditions. Energies 2016, 9, 149. [Google Scholar] [CrossRef]
- Chu, S.; Majumdar, A. Opportunities, and challenges for a sustainable energy future. Nature 2012, 488, 294–303. [Google Scholar] [CrossRef]
- Giosio, D.R.; Henderson, A.D.; Walker, J.M.; Henderson, A.D. Rapid Reserve Generation from a Francis Turbine for System Frequency Control. Energies 2017, 10, 496. [Google Scholar] [CrossRef]
- Zhang, L.K.; Ma, Z.Y.; Wu, Q.Q.; Wang, X.N.; Wu, Q.Q.; Fan, Z. Vibration analysis of coupled bending-torsional rotor-bearing system for hydraulic generating set with rub-impact under electromagnetic excitation. Arch. Appl. Mech. 2016, 86, 1665–1679. [Google Scholar] [CrossRef]
- Martinez-Lucas, G.; Sarasua, J.I.; Sanchez-Fernandez, J.A.; Wilhelmi, J.R. Frequency control support of a wind-solar isolated system by a hydropower plant with long tail-race tunnel. Renew. Energy 2016, 90, 362–376. [Google Scholar] [CrossRef]
- Xu, B.B.; Chen, D.Y.; Tolo, S.; Patelli, E.; Jiang, Y.L. Model validation and stochastic stability of a hydro-turbine governing system under hydraulic excitations. Int. J. Electr. Power Energy Syst. 2018, 95, 156–165. [Google Scholar] [CrossRef]
- Williamson, S.J.; Stark, B.H.; Booker, J.D. Performance of a low-head pico-hydro Turgo turbine. Appl. Energy 2013, 102, 1114–1126. [Google Scholar] [CrossRef]
- Trivedi, C.; Cervantes, M.J.; Gandhi, B.K.; Dahlhaug, O.G. Transient pressure measurements on a high head model Francis turbine during emergency shutdown, total Load rejection, and runaway. J. Fluids Eng. 2014, 136, 121107. [Google Scholar] [CrossRef]
- Williamson, S.J.; Stark, B.H.; Booker, J.D. Low head pico hydro turbine selection using a multi-criteria analysis. Renew. Energy 2014, 61, 43–50. [Google Scholar] [CrossRef]
- Yan, D.L.; Chen, Q.J.; Zheng, Y.; Wang, W.Y.; An, Y.C. Dynamic evolution of a bulb hydroelectric generating unit considering effects of the blades. Energy Convers. Manag. 2019, 185, 183–201. [Google Scholar] [CrossRef]
- Zhang, J.S.; Xu, B.B.; Chen, D.Y. Global sensitivity analysis of hydro power generator unit system. J. Hydroelectr. Eng. 2019, 38, 146–159. (In Chinese) [Google Scholar]
- Qin, W.Y.; Chen, G.R.; Meng, G. Nonlinear responses of a rub-impact overhung rotor. Chaos Solitons Fractals 2004, 19, 1161–1172. [Google Scholar] [CrossRef]
- Yan, D.L.; Wang, W.Y.; Chen, Q.J. Nonlinear Modeling and Dynamic Analyses of the Hydro-Turbine Governing System in the Load Shedding Transient Regime. Energies 2018, 11, 1244. [Google Scholar] [CrossRef]
- Yan, D.L.; Chen, Q.J.; Zheng, Y.; Liu, W.Y. Parameter sensitivity and dynamic characteristic analysis of bulb hydro generating unit with shaft crack fault. Mech. Syst. Signal Process. 2021, 158, 107732. [Google Scholar] [CrossRef]
- Qu, J.X.; Zhang, Z.S. A novel intelligent method for mechanical fault diagnosis based on dual-tree complex wavelet packet transform and multiple classifier fusion. Chaos Solitons Fractals 2016, 171, 837–853. [Google Scholar] [CrossRef]
- Fu, X.Q.; Jia, W.T.; Xu, H.; Song, S.L. Imbalance-misalignment-rubbing coupling faults in hydraulic turbine vibration. Opt. Int. J. Light Electron Opt. 2016, 127, 3708–3712. [Google Scholar] [CrossRef]
- Gasch, R. A survey of the dynamic behaviour of a simple rotating shaft with a transverse crack. J. Sound Vib. 1993, 160, 313–332. [Google Scholar] [CrossRef]
- Darpe, A.K.; Chawla, A.; Gupta, K. Analysis of the response of a cracked Jeffcott rotor to axial excitation. J. Sound Vib. 2002, 249, 429–445. [Google Scholar] [CrossRef][Green Version]
- Moreno, C. Turbulence, Vibrations, Noise and Fluid Instabilities. Practical Approach. In Computational Fluid Dynamics; Intech: Rijeka, Croatia, 2010; p. 420. [Google Scholar]
- Lees, A.W. Misalignment rinrigidly coupled rotors. J. Sound Vib. 2007, 305, 261–271. [Google Scholar] [CrossRef]
- Slim, B.; Molka, A.H.; Mohamed, M.; Fakhfakh, T.; Haddar, M. Dynamic behavior of hydrodynamic journal bearings in presence of rotor spatial angular misalignment. Mech. Mach. Theory 2009, 44, 1548–1559. [Google Scholar]
- Khanlo, H.M.; Ghayour, M.; Ziaei-Rad, S. Chaotic vibration analysis of rotating, flexible, continuous shaft-disk system with a rub-impact between the disk and the stator. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 566–582. [Google Scholar] [CrossRef]
- Khanlo, H.M.; Ghayour, M.; Ziaei-Rad, S. Theeffectsof lateral-torsional couplingonthenonlineardynamicbehaviorofarotatingcontinuousflexible shaft-disk system with rub-impact. Commun. Nonlinear Sci. Numer. Simul. 2013, 18, 1524–1538. [Google Scholar] [CrossRef]
- Dirani, H.C.; Merkhouf, A.; Giroux, A.M.; Kedjar, B.; Al-Haddad, K. Impact of Real Air Gap Nonuniformity on the Electromagnetic Forces of a Large Hydro-Generator. IEEE Trans. Ind. Electron. 2018, 65, 8464–8475. [Google Scholar] [CrossRef]
- Yan, D.L.; Wang, W.Y.; Chen, Q.J. Fractional-order modeling and dynamic analyses of a bending-torsional coupling generator rotor shaft system with multiple faults. Chaos Solitons Fractals 2018, 110, 1–15. [Google Scholar] [CrossRef]
- Shi, Y.S.; Zhou, J.Z.; Lai, X.J.; Xu, Y.H.; Guo, W.C.; Liu, B.N. Stability and sensitivity analysis of the bending-torsional coupled vibration with the arcuate whirl of hydro-turbine generator unit. Mech. Syst. Signal Process. 2021, 149, 107306. [Google Scholar] [CrossRef]
- Xu, B.B.; Yan, D.L.; Chen, D.Y.; Gao, X.; Wu, C.Z. Sensitivity analysis of a Pelton hydropower station based on a novel approach of turbine torque. Energy Convers. Manag. 2017, 148, 785–801. [Google Scholar] [CrossRef]
- Su, W.Q.; Guo, Z.Q. Mathematical modeling and nonlinear vibration analysis of a coupled hydro-generator shaft-foundation system. Commun. Nonlinear Sci. Numer. Simul. 2021, 98, 105776. [Google Scholar]
- Xu, B.B.; Luo, X.Q.; Egusquiza, M.; Ye, W.; Liu, J.; Egusquiza, E.; Chen, D.Y.; Guo, P.C. Nonlinear modal interaction analysis and vibration characteristics of a francis hydro-turbine generator unit. Renew. Energy 2021, 168, 854–864. [Google Scholar] [CrossRef]
- Dimitriadis, P.; Koutsoyiannis, D.; Papanicolaou, P. Stochastic similarities between the microscale of turbulence and hydrometeorological processes. Hydrol. Sci. J. 2016, 61, 1623–1640. [Google Scholar] [CrossRef]
- Lorenz, E.N. Deterministic nonperiodic flow. J. Atmos. Sci. 1963, 20, 130–141. [Google Scholar] [CrossRef]
- Frisch, U. The Legacy of A.N. Kolmogorov; Cambridge University Press: Cambridge, UK, 2006. [Google Scholar]
- Shen, A.Y.; Chen, J.; Zhou, F.; Yang, H.; Cai, F.S. Hydraulic Vibration and Possible Exciting Sources Analysis in a Hydropower System. Appl. Sci. 2021, 11, 5529. [Google Scholar] [CrossRef]
- Zhuang, K.Y.; Gao, C.D.; Li, Z.; Yan, D.L.; Fu, X.Q. Dynamic Analyses of the Hydro-Turbine Generator Shafting System Considering the Hydraulic Instability. Energies 2018, 11, 2862. [Google Scholar] [CrossRef]
- Xu, B.B.; Chen, D.Y.; Behrens, P.; Ye, W.; Guo, P.C.; Luo, X.Q. Modeling oscillation modal interaction in a hydroelectric generating system. Energy Convers. Manag. 2018, 174, 208–217. [Google Scholar] [CrossRef]
- Zheng, Y.; Chen, X.D. Hydraulic Turbine; China Water Resources and Electric Power Press: Beijing, China, 2011. (In Chinese) [Google Scholar]
- Perers, R.; Lundin, U.; Leijon, M. Saturation effects on unbalanced magnetic pullin a hydroelectric generator with an eccentric rotor. IEEE Trans. Magn. 2007, 43, 3884–3890. [Google Scholar] [CrossRef]
- Xu, B.B.; Chen, D.Y.; Zhang, H.; Zhou, R. Dynamic analysis and modeling of a novel fractional-order hydro-turbine-generator unit. Nonlinear Dyn. 2015, 81, 1263–1274. [Google Scholar] [CrossRef]
- Zeng, Y.; Zhang, L.X.; Guo, Y.K.; Qian, J.; Zhang, C.L. The generalized Hamiltonian model for the shafting transient analysis of the hydro turbine generating sets. Nonlinear Dyn. 2014, 76, 1921–1933. [Google Scholar] [CrossRef]
- Genç, M.S.; Lock, G.; Kaynak, U. An Experimental and Computational Study of Low Re Number Transitional Flows over an Aerofoil with Leading Edge Slat, AIAA-2008-8877. In Proceedings of the The 26th Congress of ICAS and 8th AIAA ATIO, Anchorage, AK, USA, 14–19 September 2008. [Google Scholar]
- Genç, M.S. Kanat Profilleri Etrafındaki Düşük Reynolds Sayılı Akışın Kontrolü ve Aerodinamik Performansın İncelenmesi. Ph.D. Thesis, Fen Bilimleri Enstitüsü, Erciyes Üniversitesi, Kayseri, Türkiye, 2009. (In Turkish). [Google Scholar]
- He, J.X.; Tang, F.; Xiong, C.; Wang, Z.C. Experiment of Lift Coefficient measurement Based on Thin Airfoil Model of Simple Wind Tunnel. Phys. Eng. 2018, 28, 113–117. [Google Scholar]
- Feng, Y.R.; Gu, M.C.; Fang, R.Z. Modernization transformation of No. 4 generating unit of Fengman Hydropower Plant. Water Power 2000, 1, 38–40. (In Chinese) [Google Scholar]

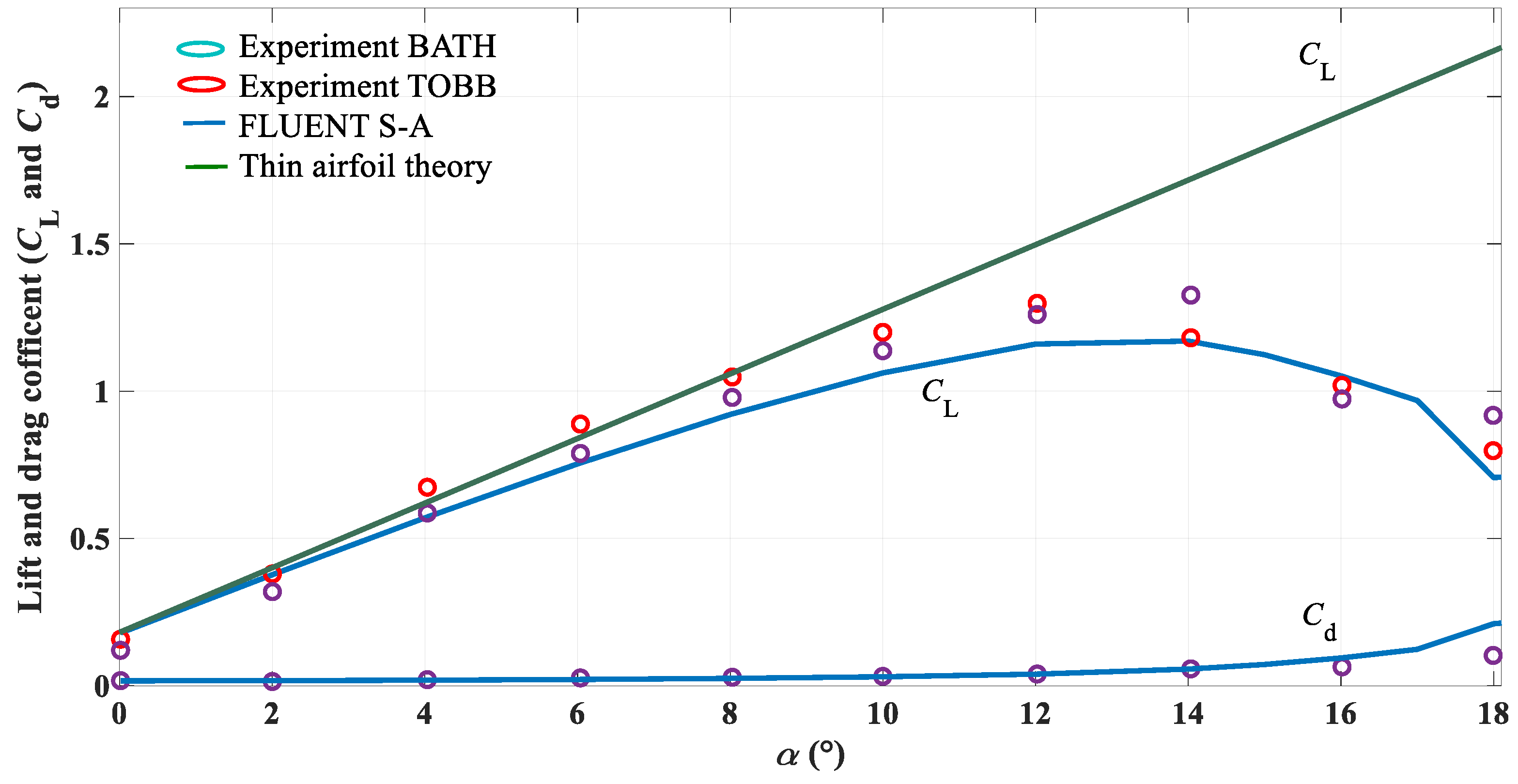


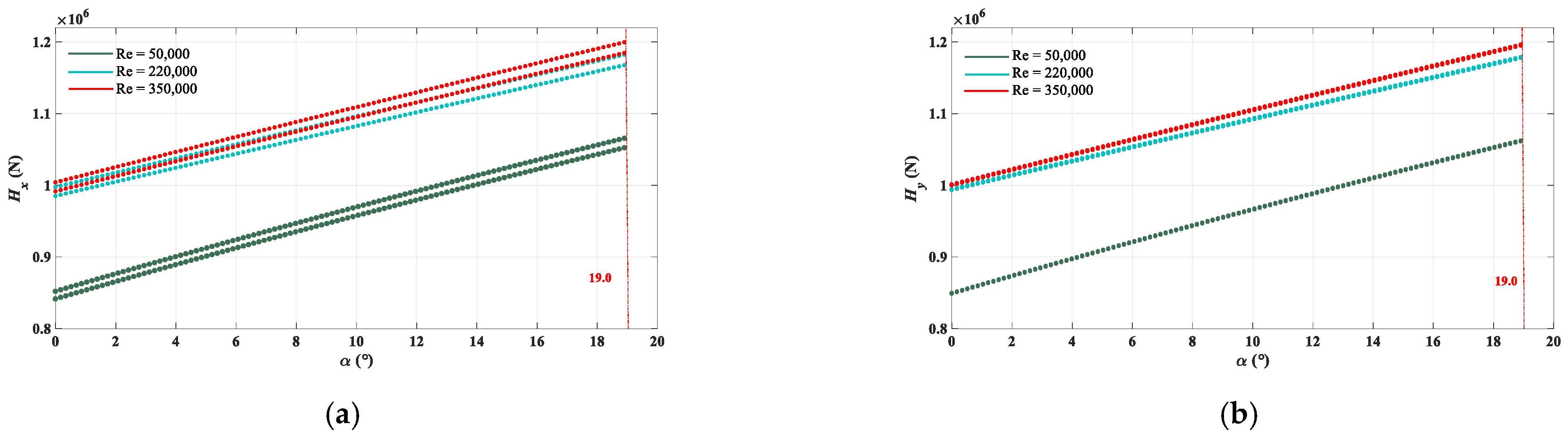



| Component. | Damping Force | Oil-Film Force | Asymmetric Magnetic Pull | Hydraulic Asymmetric Force | Rub-Impact Force |
|---|---|---|---|---|---|
| Generator rotor | √, Ref. [17] | √, Ref. [41] | √, Ref. [31] | ||
| Turbine runner | √, Ref. [17] | √ | |||
| Generator bearing | √, Ref. [42] | ||||
| Water guide bearing | √, Ref. [42] |
| Conditions | Coefficients of the Power Series | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| i or j | 7 | 6 | 5 | 4 | 3 | 2 | 1 | 0 | |
| (1) | pi | - | - | 0.0003 | −0.0027 | 0.0141 | −0.0365 | 0.1278 | 0.1541 |
| qj | −0.0004 | 0.0035 | −0.0174 | 0.0489 | −0.0608 | 0 | 0 | 0.1578 | |
| (2) | pi | - | - | 0.0001 | −0.0009 | 0.0039 | −0.0083 | 0.1049 | 0.1824 |
| qj | −0.0002 | 0.0018 | −0.009 | 0.0254 | −0.0317 | 0 | 0 | 0.0889 | |
| (3) | pi | - | - | 0.0001 | −0.0008 | 0.0043 | −0.0115 | 0.112 | 0.1839 |
| qj | −0.0002 | 0.0016 | −0.0079 | 0.0225 | −0.0281 | 0 | 0 | 0.0793 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhuang, K.; Huang, S.; Fu, X.; Chen, L. Nonlinear Hydraulic Vibration Modeling and Dynamic Analysis of Hydro-Turbine Generator Unit with Multiple Faults. Energies 2022, 15, 3386. https://doi.org/10.3390/en15093386
Zhuang K, Huang S, Fu X, Chen L. Nonlinear Hydraulic Vibration Modeling and Dynamic Analysis of Hydro-Turbine Generator Unit with Multiple Faults. Energies. 2022; 15(9):3386. https://doi.org/10.3390/en15093386
Chicago/Turabian StyleZhuang, Keyun, Shehua Huang, Xiangqian Fu, and Li Chen. 2022. "Nonlinear Hydraulic Vibration Modeling and Dynamic Analysis of Hydro-Turbine Generator Unit with Multiple Faults" Energies 15, no. 9: 3386. https://doi.org/10.3390/en15093386
APA StyleZhuang, K., Huang, S., Fu, X., & Chen, L. (2022). Nonlinear Hydraulic Vibration Modeling and Dynamic Analysis of Hydro-Turbine Generator Unit with Multiple Faults. Energies, 15(9), 3386. https://doi.org/10.3390/en15093386





