Design Optimization of a Cross-Flow Air Turbine for an Oscillating Water Column Wave Energy Converter
Abstract
:1. Introduction
2. CFD Setup
2.1. Numerical Model
2.2. Data Analysis
3. Experimental Setup
3.1. Experimental Apparatus
3.2. Measurement Instruments and Experiment Procedures
4. Results and Discussion
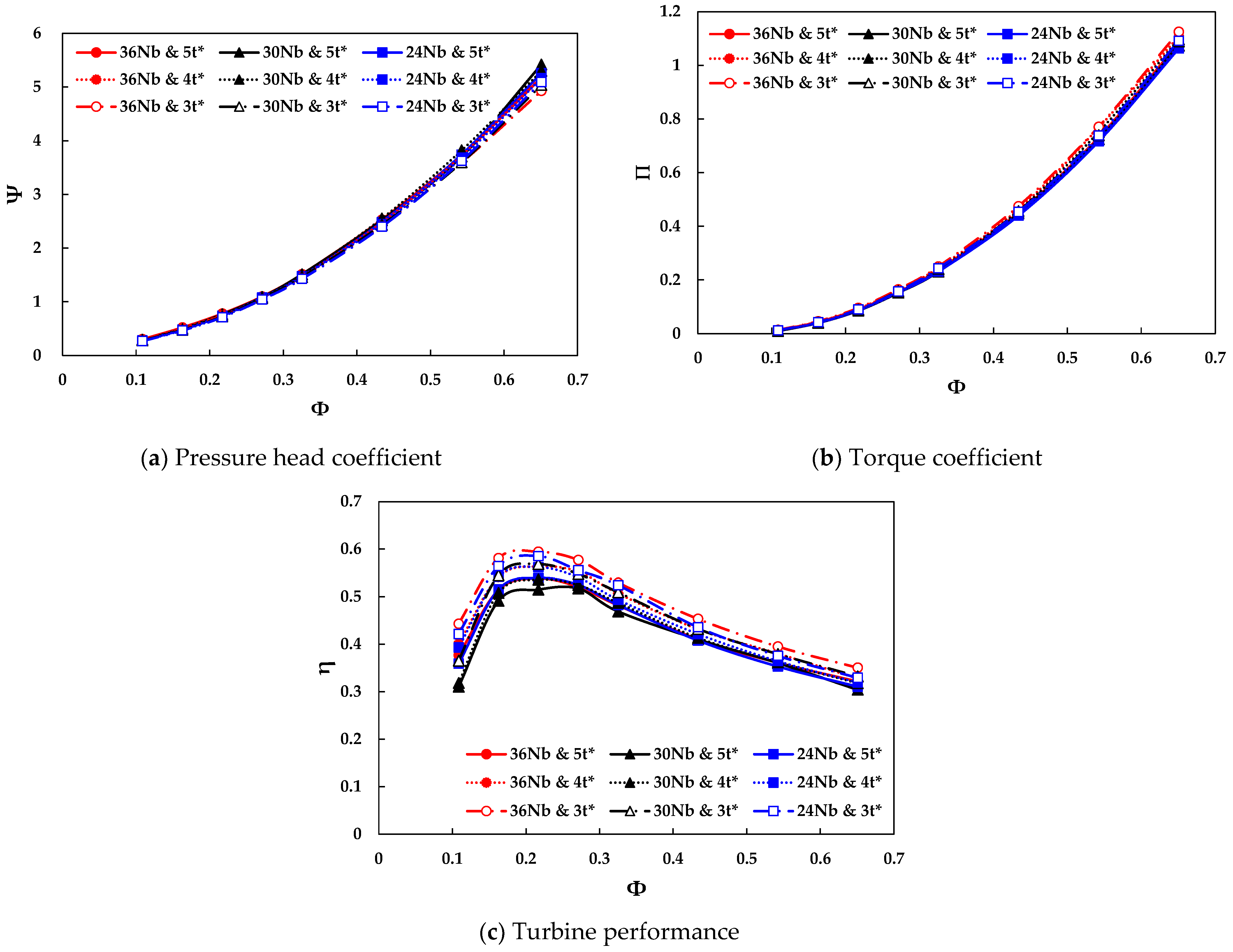
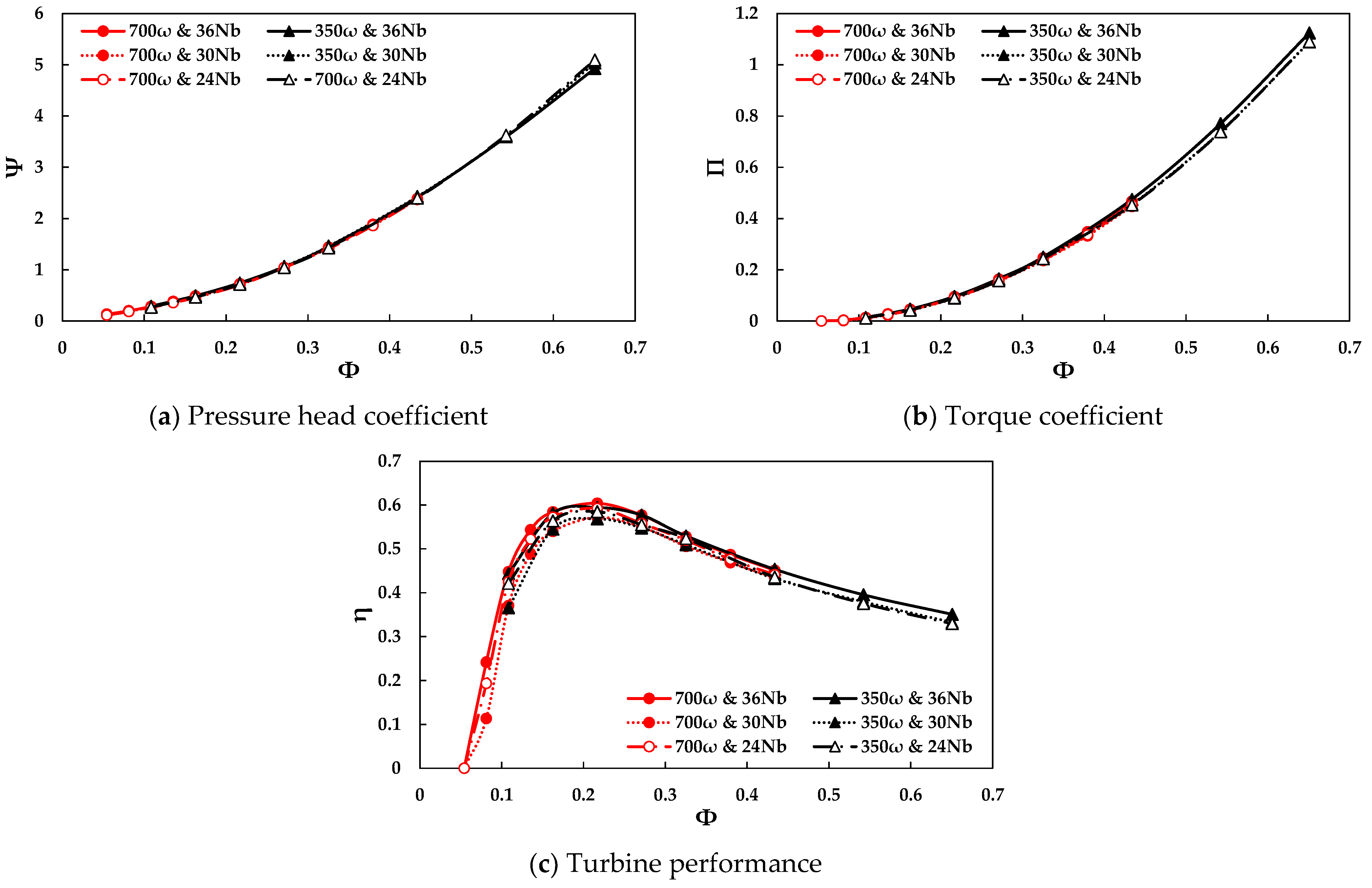
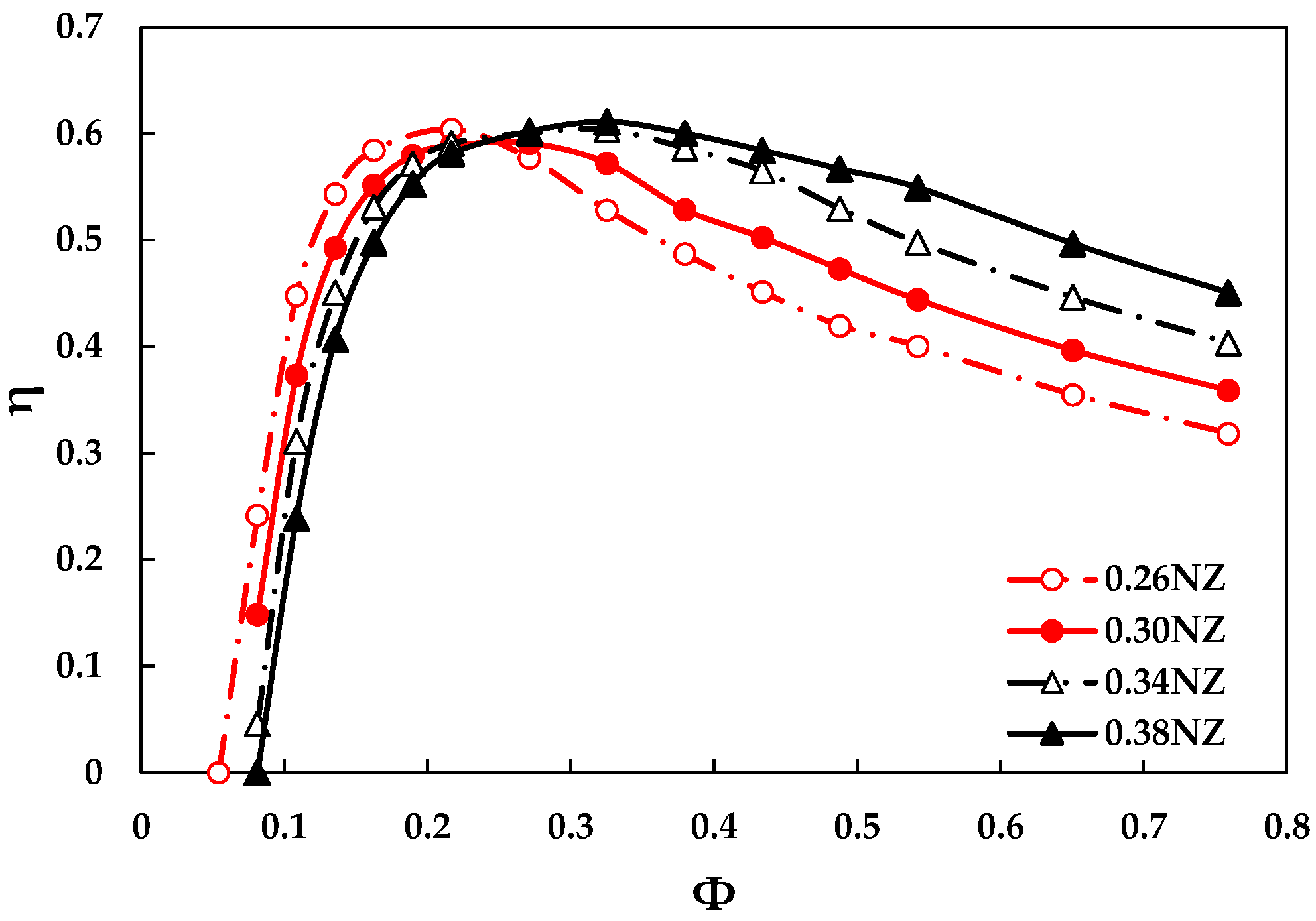
4.1. Geometric Optimization
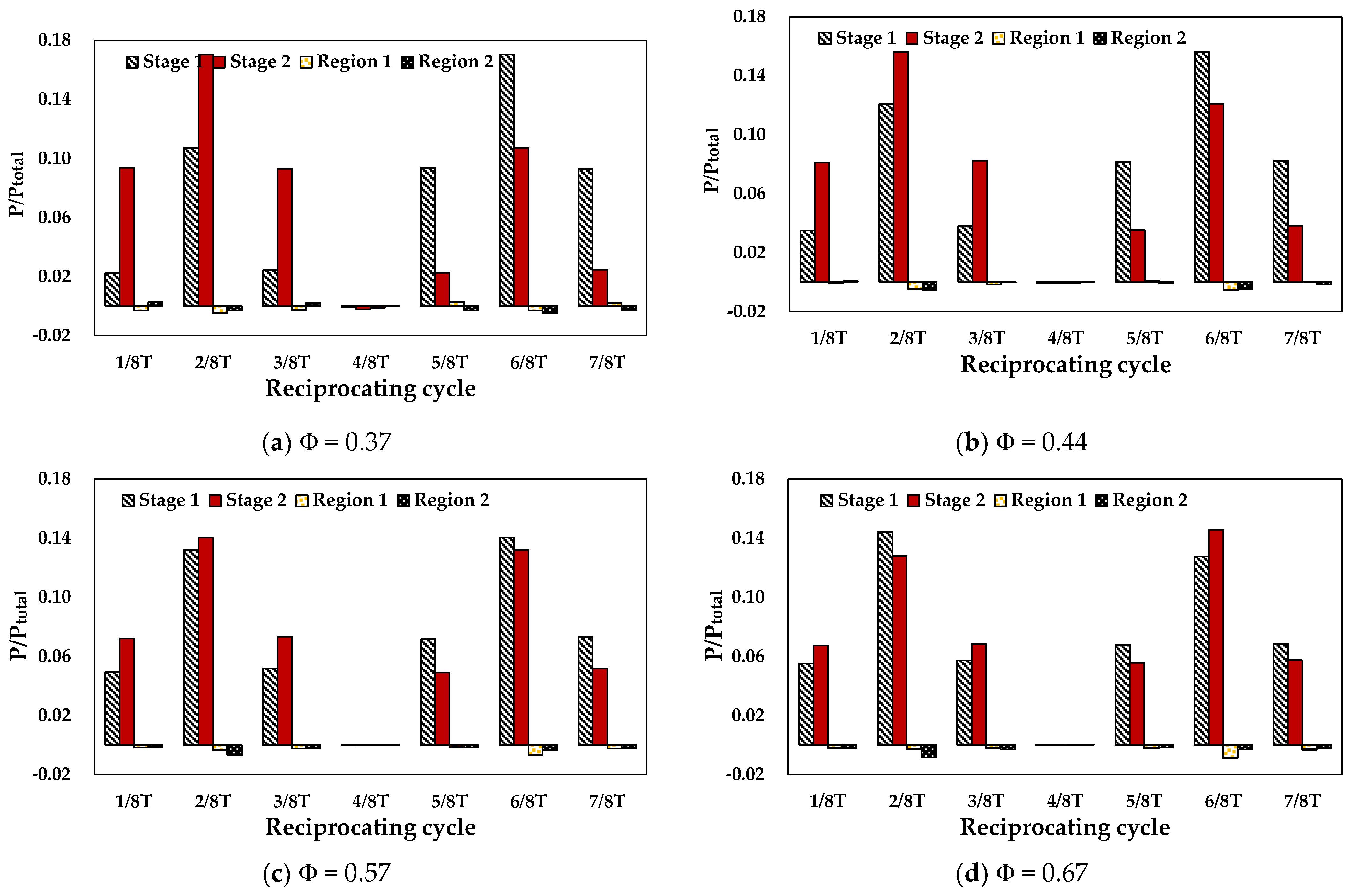
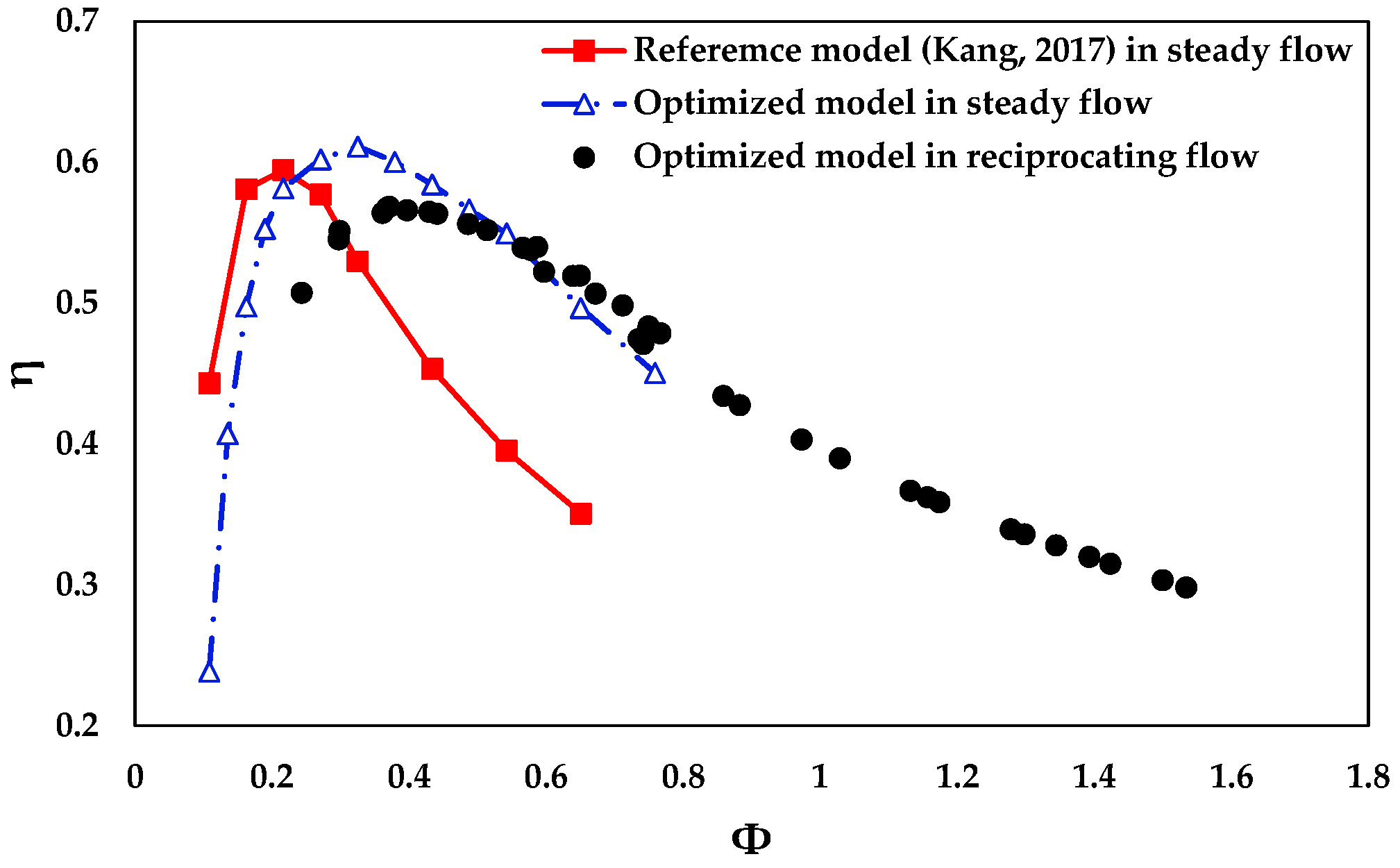
4.2. Overall Performance in Reciprocating Flows
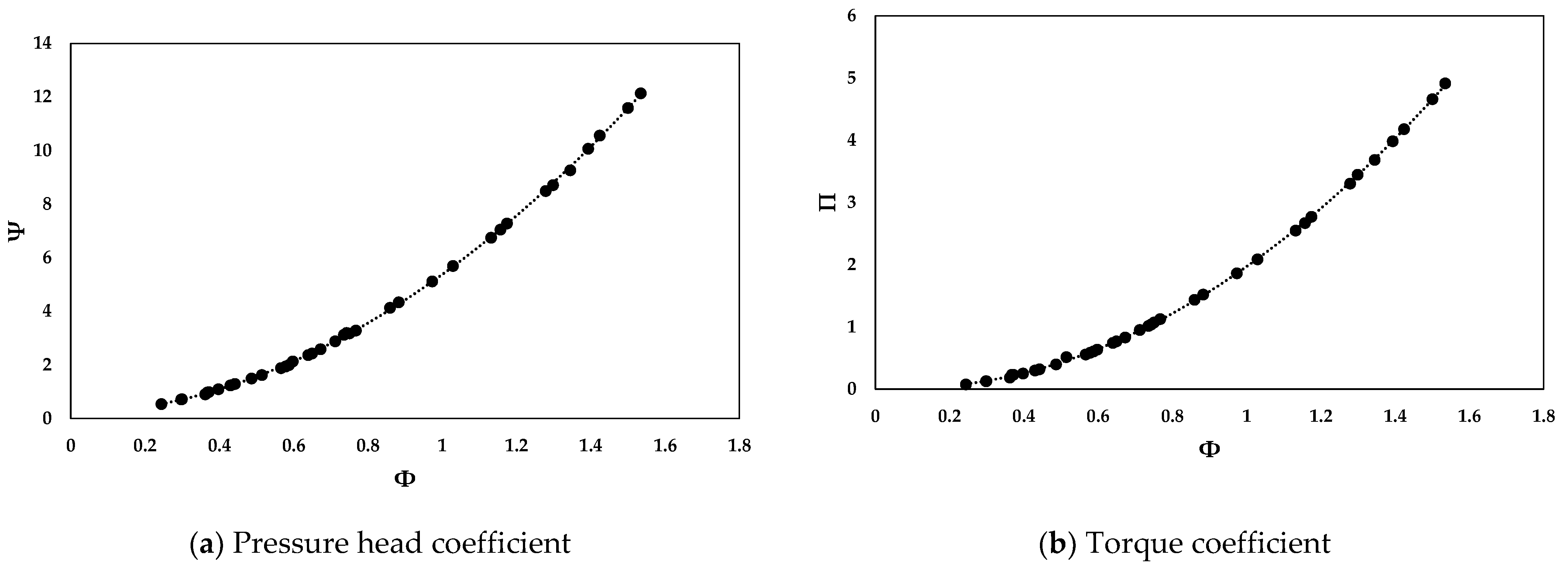
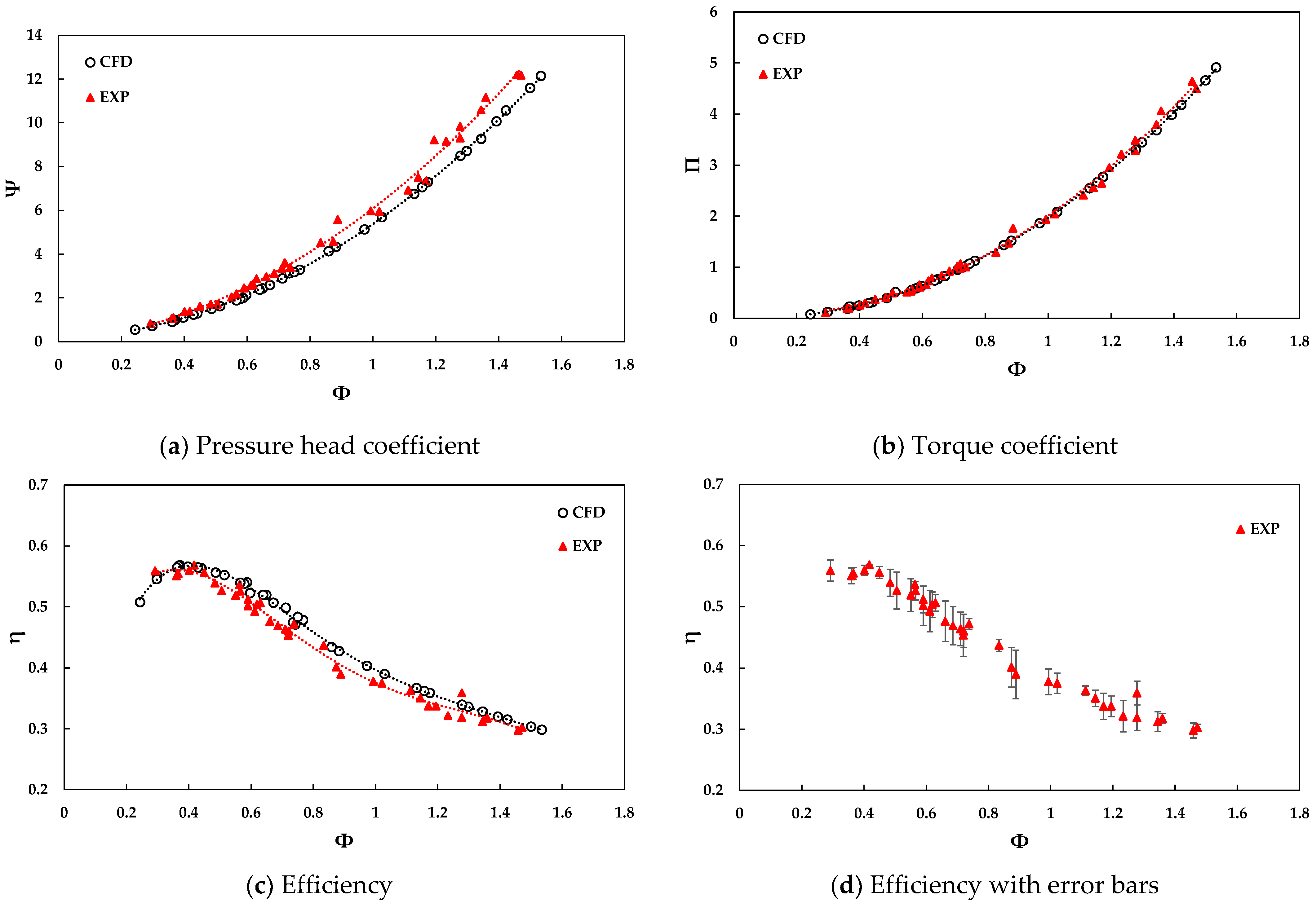
4.3. Validation of Numerical Model with Experiment Result
5. Conclusions
- The optimized model had 36 blades of the rotor with 3 mm thickness and 0.38 throat width of the nozzle.
- The geometric optimization of the nozzle was the most sensitive among the selected design variables. This indicates greater possibility for enhancing its performance in future work.
- The maximum efficiency of the optimized model was 0.611, which was 1.7% larger than that of the reference model.
- The band width of the model significantly widened as the flow coefficient increased.
- The optimized model in reciprocating flows had more improved operating range with higher efficiency than the steady-state performance, but the peak performance decreased by 4.3%.
- The averaged difference between the numerical result and the experimental result was 3.5%, which indicates that the numerical model was able to predict the turbine performance with high accuracy.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- PDrew, B.; Plummer, A.R.; Sahinkaya, M.N. A review of wave energy converter technology. Proc. Inst. Mech. Eng. Part A J. Power Energy 2009, 223, 887–902. [Google Scholar]
- Vining, J.G.; Muetze, A. Economic factors and incentives for ocean wave energy conversion. IEEE Trans. Ind. Appl. 2009, 45, 547–554. [Google Scholar] [CrossRef]
- Aderinto, T.; Li, H. Review on power performance and efficiency of wave energy converters. Energies 2019, 12, 4329. [Google Scholar] [CrossRef] [Green Version]
- Weerakoon, A.S.; Kim, B.H.; Cho, Y.J.; Prasad, D.D.; Ahmed, M.R.; Lee, Y.H. Design optimization of a novel vertical augmentation channel housing a cross-flow turbine and performance evaluation as a wave energy converter. Renew. Energy 2021, 180, 1300–1314. [Google Scholar] [CrossRef]
- Falcão, A.F.; Henriques, J.C. Oscillating-water-column wave energy converters and air turbines: A review. Renew. Energy 2016, 85, 1391–1424. [Google Scholar] [CrossRef]
- Masuda, Y.; McCormick, M.E. Experiences in pneumatic wave energy conversion in Japan. In Utilization of Ocean Waves—Wave to Energy Conversion; ASCE: Reston, VA, USA, 1986; pp. 1–33. [Google Scholar]
- Torresi, M.; Stefanizzi, M.; Fornarelli, F.; Gurnari, L.; Filianoti, P.G.F.; Camporeale, S.M. Performance characterization of a wells turbine under unsteady flow conditions. In the AIP Conference Proceedings; AIP Publishing: Melville, NY, USA, 2019; p. 020149. [Google Scholar]
- Setoguchi, T.; Takao, M. Current status of self rectifying air turbines for wave energy conversion. Energy Convers. Manag. 2006, 47, 2382–2396. [Google Scholar] [CrossRef]
- Curran, R.; Folley, M. Air turbine design for OWCs. In Ocean Wave Energy-Current Status and Future Prospects; Springer: Manhattan, NY, USA, 2008; pp. 189–219. [Google Scholar]
- Falcão, A.F.O.; Gato, L.M.C. 8.05—Air Turbines. In Comprehensive Renewable Energy, 2nd ed.; Elsevier: Amsterdam, The Netherlands, 2012; pp. 111–149. [Google Scholar]
- Das, T.K.; Halder, P.; Samad, A. Optimal design of air turbines for oscillating water column wave energy systems: A review. Int. J. Ocean Clim. Syst. 2017, 8, 37–49. [Google Scholar] [CrossRef]
- Thakker, A.; Dhanasekaran, T.S. Computed effect of guide vane shape on performance of impulse turbine for wave energy conversion. Int. J. Energy Res. 2005, 29, 1245–1260. [Google Scholar] [CrossRef]
- Kang, H.G.; Kim, B.H.; Lee, Y.H. A Performance Study of a Cross-flow Air Turbine Utilizing an Orifice for OWC WEC. J. Korean Soc. Fluid Mach. 2017, 20, 54–62. [Google Scholar] [CrossRef]
- Mockmore, C.A.; Merryfield, F. The Banki water turbine. Or. Eng. Exp. Stn. Bull. Ser. 1949, 25, 1–27. [Google Scholar]
- Durali, M. Design of Small Water Turbines for Farms and Small Communities. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1976. [Google Scholar]
- Nakase, Y. A study of cross-flow turbine (effects of nozzle shape on its performance). In ASME 103rd Winter Annual Meeting; ASME 103rd Winter Annual Meeting: Scottsdale, AZ, USA, 1982; Volume 13. [Google Scholar]
- Khosrowpanah, S.; Fiuzat, A.A.; Albertson, M.L. Experimental study of cross-flow turbine. J. Hydraul. Eng. 1988, 114, 299–314. [Google Scholar] [CrossRef]
- Fiuzat, A.A.; Akerkar, B. The use of interior guide tube in cross flow turbines. In Waterpower’89; ASCE: Reston, VA, USA, 1989; pp. 1111–1119. [Google Scholar]
- Desai, V.R. A Parametric Study of the Cross-Flow Turbine Performance. Ph.D. Thesis, Clemson University, Clemson, SC, USA, 1993. [Google Scholar]
- Totapally, H.G.; Aziz, N.M. Refinement of cross-flow turbine design parameters. J. Energy Eng. 1994, 120, 133–147. [Google Scholar] [CrossRef]
- Fukutomi, J.; Nakase, Y.; Watanabe, T. A numerical method of free jet from a cross-flow turbine nozzle. Bull. JSME 1985, 28, 1436–1440. [Google Scholar] [CrossRef]
- Adhikari, R.C.; Wood, D.H. A new nozzle design methodology for high efficiency crossflow hydro turbines. Energy Sustain. Dev. 2017, 41, 139–148. [Google Scholar] [CrossRef]
- Prasad, D.D.; Ahmed, M.R.; Lee, Y.H. Performance studies on a direct drive turbine for wave power generation in a numerical wave tank. In Proceedings of the Asian Wave and Tidal Energy Conference, Jeju, Korea, 27–30 November 2012; pp. 185–192. [Google Scholar]
- Choi, Y.D.; Kim, C.G.; Kim, Y.T.; Song, J.I.; Lee, Y.H. A performance study on a direct drive hydro turbine for wave energy converter. J. Mech. Sci. Technol. 2010, 24, 2197–2206. [Google Scholar] [CrossRef]
- Prasad, D.D.; Kim, C.G.; Kang, H.G.; Ahmed, M.R.; Lee, Y.H. Performance and flow characteristics of single and a novel double oscillating water column devices. J. Mech. Sci. Technol. 2017, 31, 5879–5886. [Google Scholar] [CrossRef]
- Akabane, M.; Suzuki, H.; Yamauchi, K. On the Cross Flow Turbine for Wave Power Plant. In Proceedings of the 1st Symposium on Wave Energy Utilization in Japan, Tokyo, Japan, 27–28 November 1984. [Google Scholar]
- Paderi, M.; Puddu, P. Experimental investigation in a Wells turbine under bi-directional flow. Renew. Energy 2013, 57, 570–576. [Google Scholar] [CrossRef]
- Puddu, P.; Paderi, M.; Manca, C. Aerodynamic characterization of a Wells turbine under bi-directional airflow. Energy Procedia 2014, 45, 278–287. [Google Scholar] [CrossRef]
- Ghisu, T.; Puddu, P.; Cambuli, F. Physical explanation of the hysteresis in Wells turbines: A critical reconsideration. J. Fluids Eng. 2016, 138, 11. [Google Scholar] [CrossRef]
- Ghisu, T.; Puddu, P.; Cambuli, F. Numerical analysis of a wells turbine at different non-dimensional piston frequencies. J. Therm. Sci. 2015, 24, 535–543. [Google Scholar] [CrossRef]
- Liu, Z.; Cui, Y.; Xu, C.; Sun, L.; Li, M.; Jin, J. Experimental and numerical studies on an OWC axial-flow impulse turbine in reciprocating air flows. Renew. Sustain. Energy Rev. 2019, 113, 109272. [Google Scholar] [CrossRef]
- Elhanafi, A.; Fleming, A.; Macfarlane, G.; Leong, Z. Numerical energy balance analysis for an onshore oscillating water column–wave energy converter. Energy 2016, 116, 539–557. [Google Scholar] [CrossRef]
- Choi, Y.D.; Lim, J.I.; Kim, Y.T.; Lee, Y.H. Performance and internal flow characteristics of a cross-flow hydro turbine by the shapes of nozzle and runner blade. J. Fluid Sci. Technol. 2008, 3, 398–409. [Google Scholar] [CrossRef] [Green Version]
- De Andrade, J.; Curiel, C.; Kenyery, F.; Aguillón, O.; Vásquez, A.; Asuaje, M. Numerical investigation of the internal flow in a Banki turbine. Int. J. Rotating Mach. 2011, 2011, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Sammartano, V.; Aricò, C.; Carravetta, A.; Fecarotta, O.; Tucciarelli, T. Banki-Michell optimal design by computational fluid dynamics testing and hydrodynamic analysis. Energies 2013, 6, 2362–2385. [Google Scholar] [CrossRef]
- ANSYS, Inc. ANSYS CFX-Solver Theory Guide, ANSYS, Inc.: Canonsburg, PA, USA, 2016.
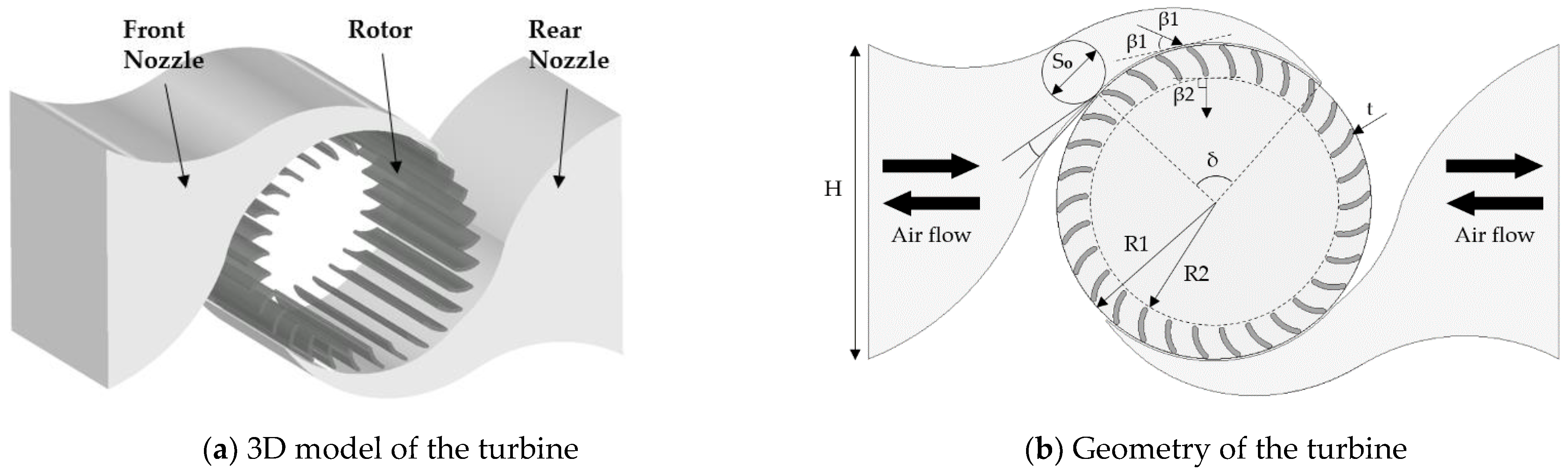

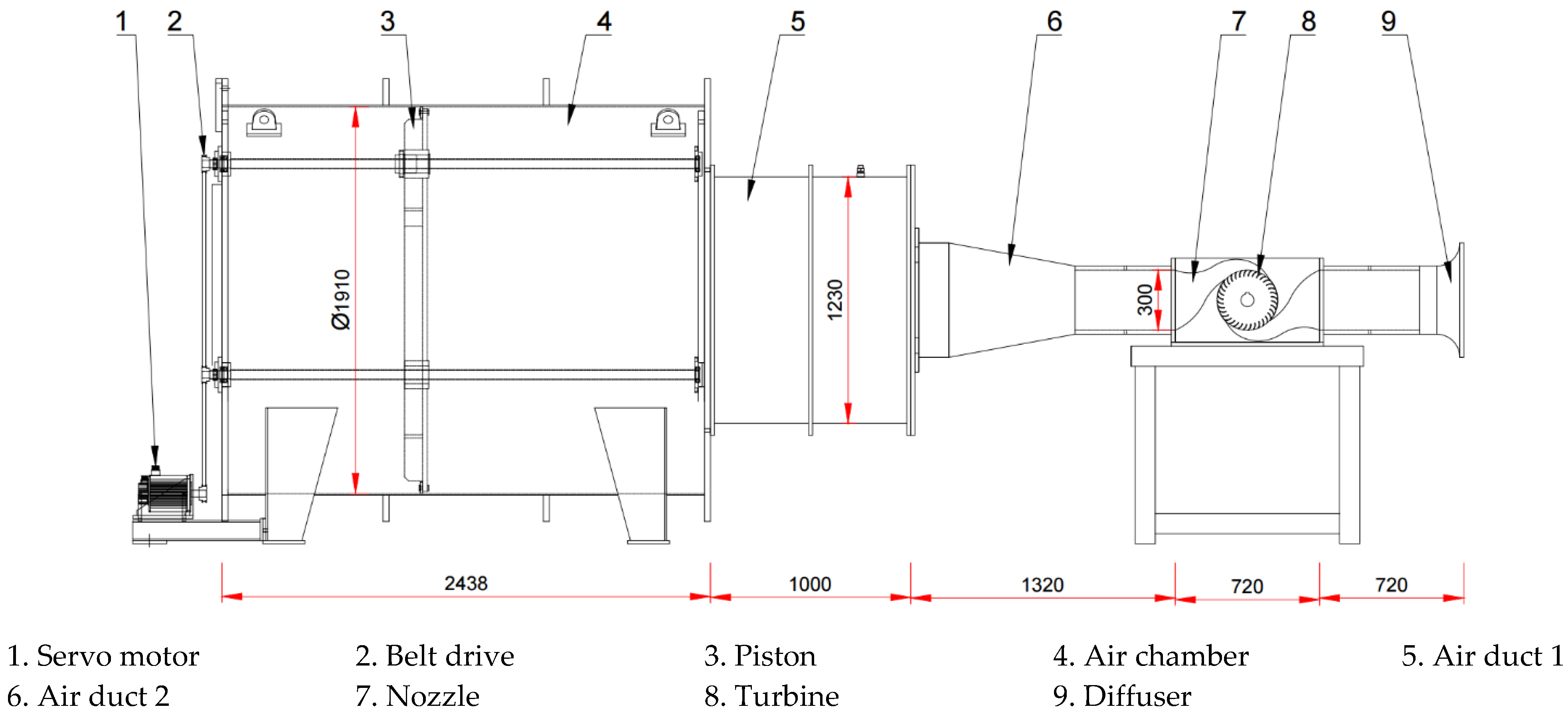
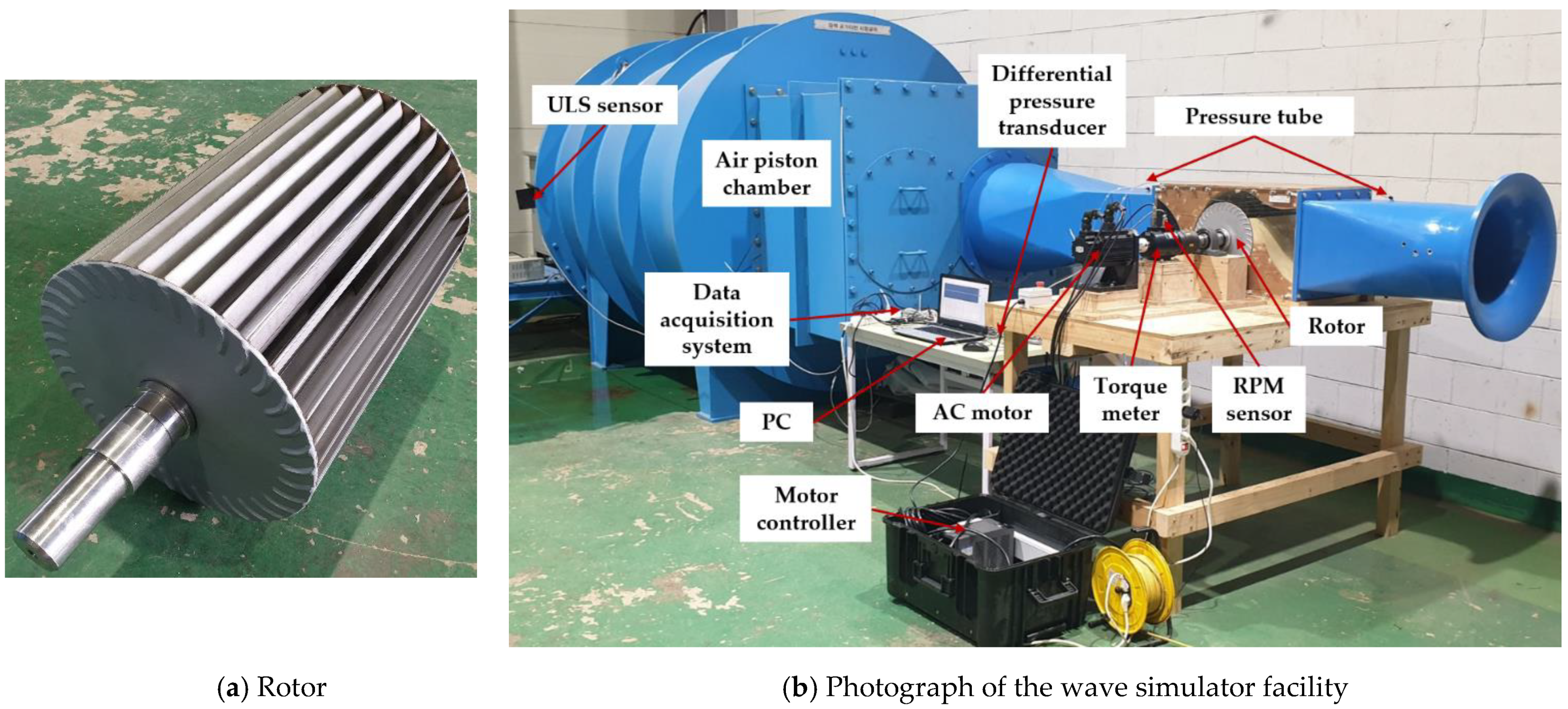



| Design Parameter | Value |
|---|---|
| Outer diameter, D1, (m) | 0.3 |
| Inner diameter, D2, (m) | 0.24 |
| No. of blades, Nb | 24, 30, 36 |
| Thickness of blades, t*, (mm) | 3, 4, 5 |
| Nozzle entry angle, δ, (°) | 90 |
| Angle of attack, α, (°) | 18 |
| Blade inlet angle, β1, (°) | 30 |
| Blade exit angle, β2, (°) | 90 |
| Rotor and nozzle width, W, (m) | 0.4 |
| Rotational speed, ω, (rpm) | 350 and 700 |
| 0.26 (original), 0.3, 0.34, 0.38 |
| Design Parameter | Value |
|---|---|
| Outer diameter, D1, (m) | 0.3 |
| Inner diameter, D2, (m) | 0.24 |
| Width of turbine, W, (m) | 0.4 |
| Tip clearance, (mm) | 1 |
| No. of blades, Nb | 36 |
| Thickness of blades, t*, (mm) | 3 |
| Nozzle entry angle, δ, (°) | 90 |
| Angle of attack, α, (°) | 18 |
| Blade inlet angle, β1, (°) | 30 |
| Blade exit angle, β2, (°) | 90 |
| Rotor and nozzle width, W, (m) | 0.4 |
| Rotational speed, ω, (rpm) | 350 and 700 |
| 0.38 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kang, H.-G.; Lee, Y.-H.; Kim, C.-J.; Kang, H.-D. Design Optimization of a Cross-Flow Air Turbine for an Oscillating Water Column Wave Energy Converter. Energies 2022, 15, 2444. https://doi.org/10.3390/en15072444
Kang H-G, Lee Y-H, Kim C-J, Kang H-D. Design Optimization of a Cross-Flow Air Turbine for an Oscillating Water Column Wave Energy Converter. Energies. 2022; 15(7):2444. https://doi.org/10.3390/en15072444
Chicago/Turabian StyleKang, Hong-Goo, Young-Ho Lee, Chan-Joo Kim, and Hyo-Dong Kang. 2022. "Design Optimization of a Cross-Flow Air Turbine for an Oscillating Water Column Wave Energy Converter" Energies 15, no. 7: 2444. https://doi.org/10.3390/en15072444
APA StyleKang, H.-G., Lee, Y.-H., Kim, C.-J., & Kang, H.-D. (2022). Design Optimization of a Cross-Flow Air Turbine for an Oscillating Water Column Wave Energy Converter. Energies, 15(7), 2444. https://doi.org/10.3390/en15072444






