Study on the Characteristics of Water Jet Injection and Temperature Spatial Distribution in the Process of Hot Water Deicing for Insulators
Abstract
:1. Introduction
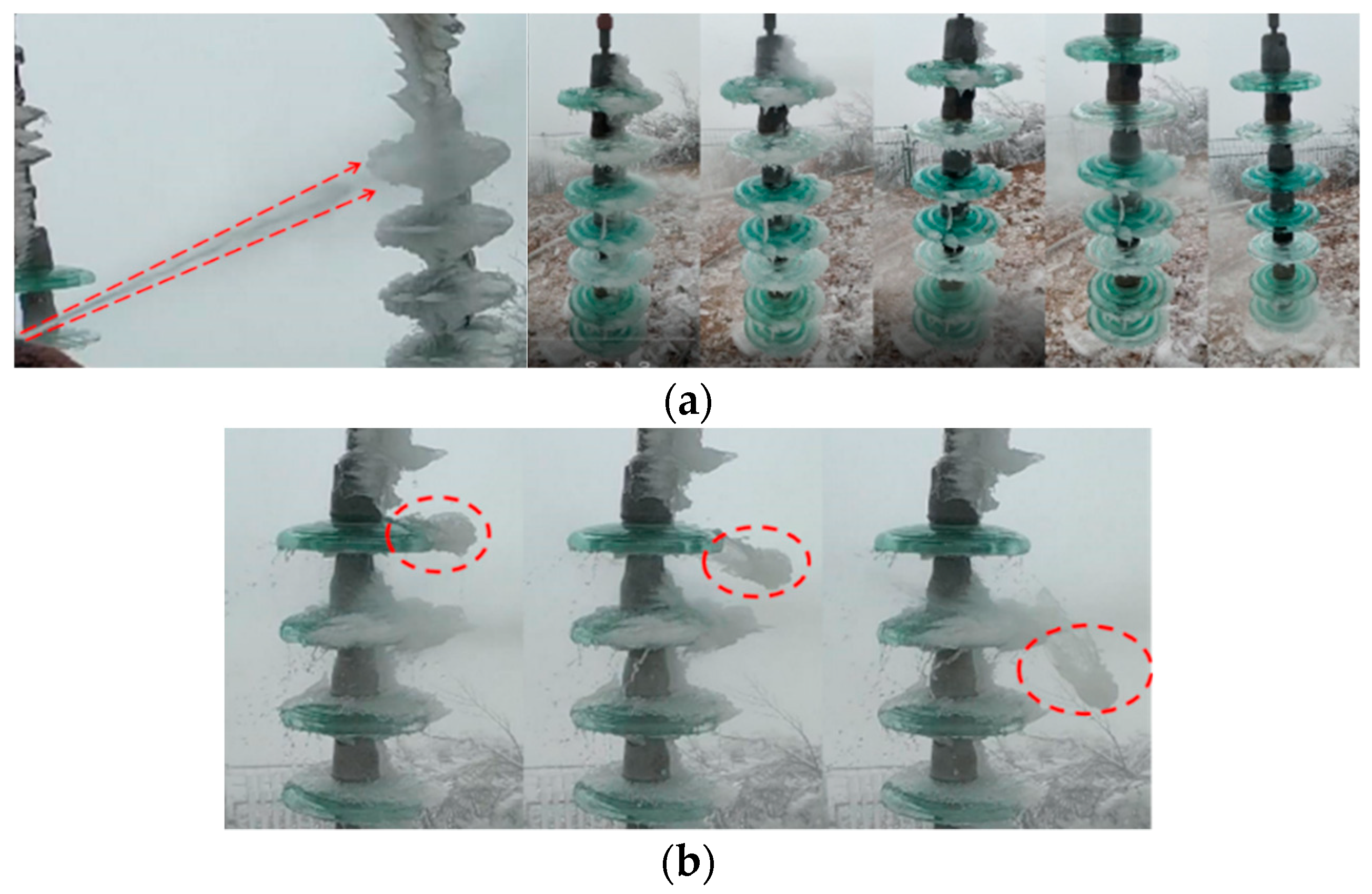
2. Physical Process of Hot Water Deicing
- (1)
- High-speed hot water jets from the nozzle outlet to the ice layer (Figure 1a). The reference temperature, wind speed, nozzle diameter, ambient temperature, injection distance, and outlet pressure on the spatial distribution of water jet temperature will determine the technical parameters of the hot water deicing device. This paper will focus on this process;
- (2)
- The hot water causes the ice to melt and shed through convective heat transfer and impact force (Figure 1b). The insulation performance of the water jet needs to be considered in the actual deicing operation. It is necessary to investigate the influence of jet distance, water conductivity, and outlet pressure on the water jet’s leakage current in order to validate the shortest deicing length under various combinations of water conductivity and outlet pressure.
3. Numerical Model of Water Jet Flow Field
3.1. The Physical Structure of the Nozzle
3.2. Geometric Model and Meshing of the Water Jet Flow Field
3.3. Calculation Model and Governing Equation
3.4. Boundary Conditions
4. Numerical Calculation Results and Validation of the Water Jet Flow Field
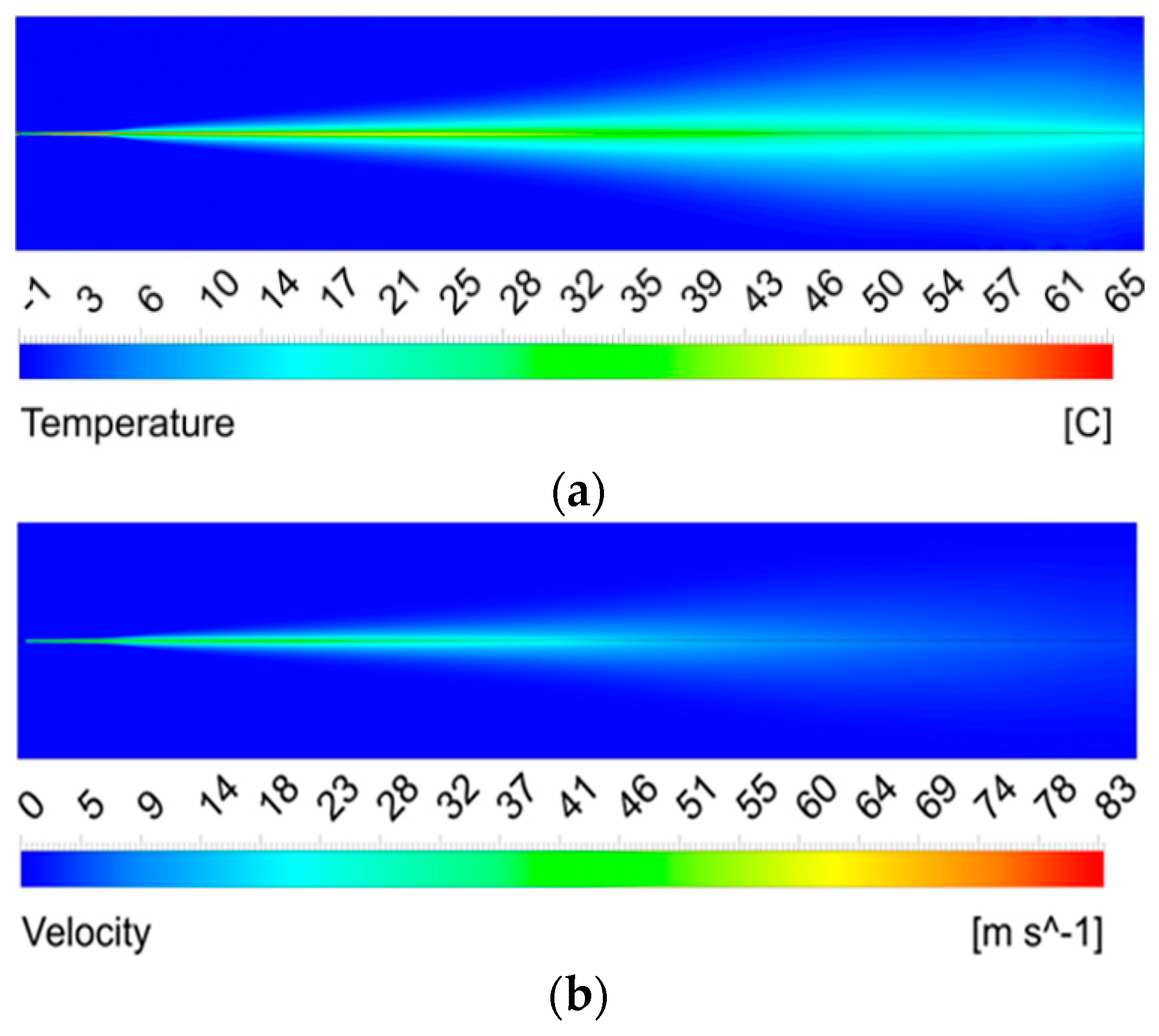
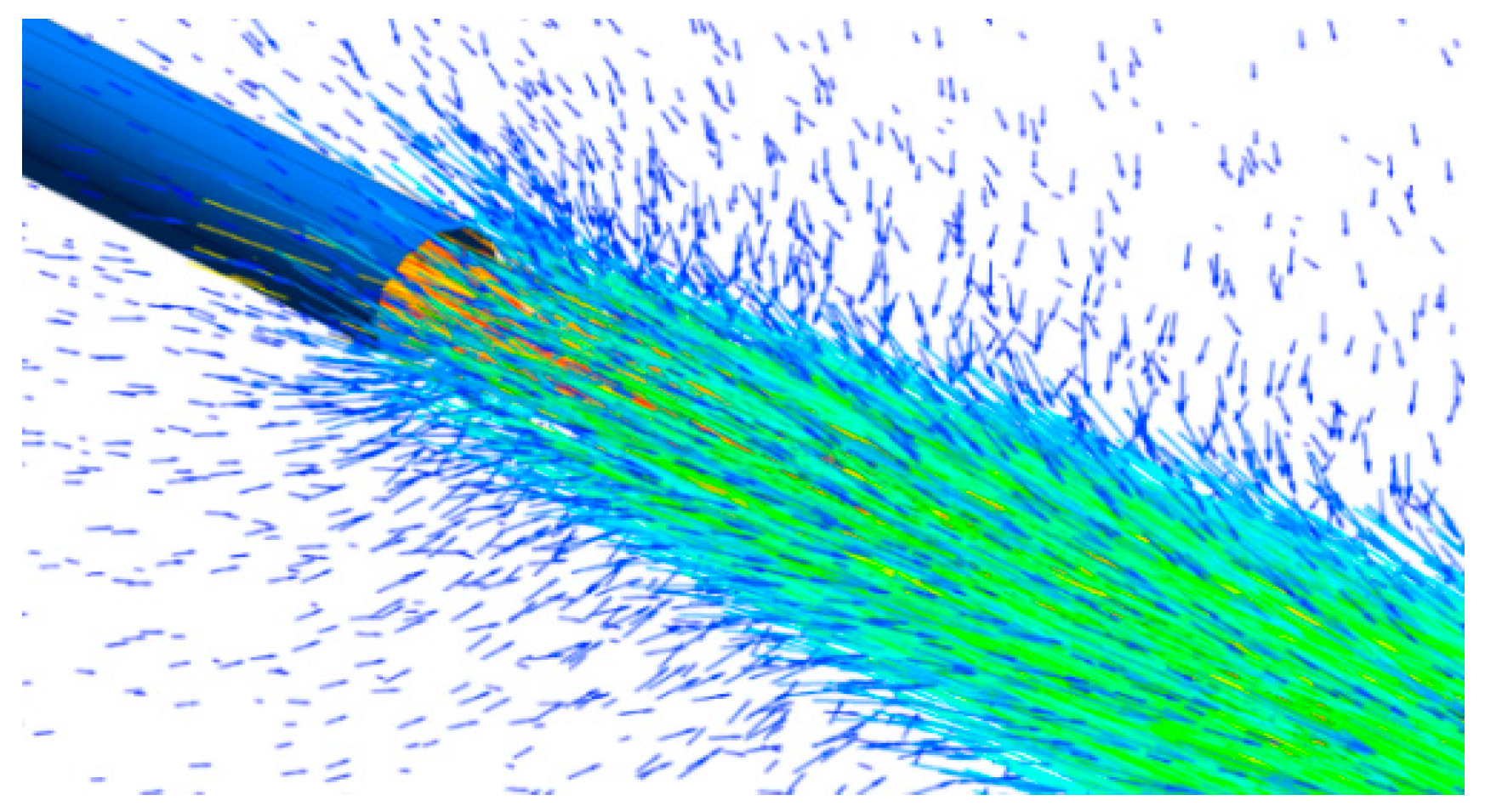
4.1. Numerical Calculation Results of Water Jet Flow Field
4.2. Experimental Validation
- (1)
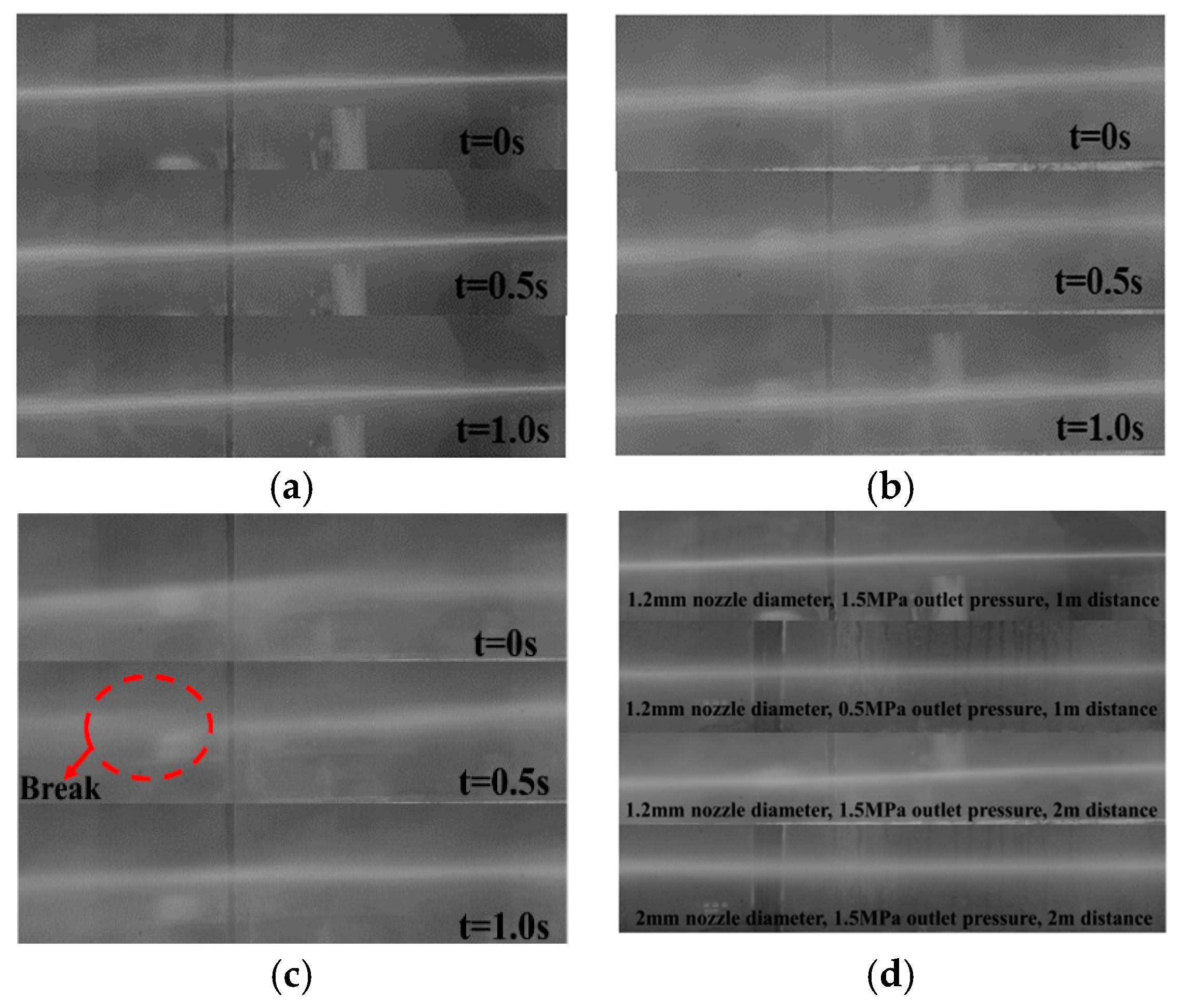
- The distance affects the shape of the water jet. The water jet within 1 m is compact in Figure 9a, and the water column is straight, transparent, and dense. The cross-section width of the water column is clear, and the air is less mixed. According to the experiment results in Figure 9d, the compact segment’s length is related to the nozzle diameter and outlet pressure. To be specific, by comparing the water column states in the first two pictures, we can see that the compactness of the water column of 1.5 MPa is better, and the cross-section width of the water column is clearer than that at 0.5 MPa. Therefore, the length of the compact segment grows larger when the outlet pressure rises. Similarly, by comparing the water column states in the last two pictures, it can be seen that the water column of 2 mm nozzle diameter at 2 m is more compact than that of 1.2 mm nozzle diameter—that is, the compact segment extends as the nozzle diameter increases;
- (2)
- After the dense water column enters the air, it has strong friction with the air, the section widens, and the speed slows down. The water column is gradually doped with air, and the jet edge begins to diverge into water mist. The boundary layer of the water column gradually blurs, as shown in Figure 9b;
- (3)
- With the increase in distance, the jet entrains more air, causing the randomness of movement to become greater, and the water column diverges accordingly. For example, “break” occurs after the jet distance reaches 3 m in the condition of 1.2 mm nozzle diameter and 1.5 MPa outlet pressure, as shown in Figure 9c. With the increase in water jet injection distance, air entrainment intensifies, and the water column gradually absorbs air. Due to the doping of air, the motion of the water jet is slow and random, and the velocity is reduced to a slower speed. With the increase in jet length, the jet absorbs more and more air and is isolated into multiple water blocks by the air, resulting in a “break”;
- (4)
- Figure 9d shows that we have carried out experiments under different nozzle outlet conditions, and the test results show that the length of the water jet compact section is related to the nozzle diameter and the outlet pressure. Because the jet direction is from the right side to the left side of the picture, the water jet’s gravity effect is more obvious after the speed is reduced, resulting in the water jet state showing a low left and high right state.
- (1)
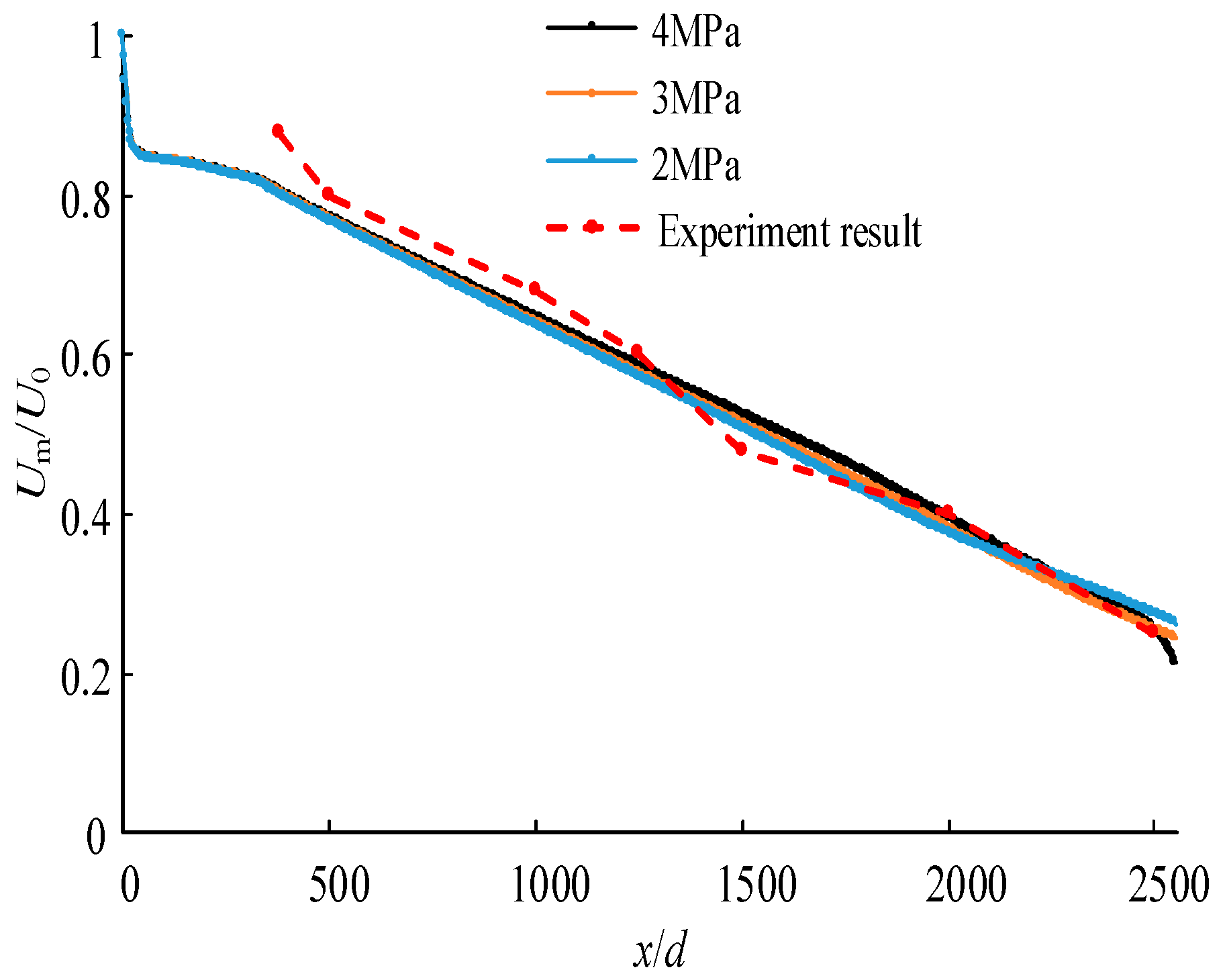
- Under the three outlet pressures, although the velocity at the nozzle outlet is different, the attenuation law of the normalized axial velocity along the axial direction is virtually the same;
- (2)
- The fluid velocity at the nozzle inlet is low. The velocity increases sharply after the conical contraction section, and reaches its maximum at the nozzle outlet. The jet velocity gradually decreases after leaving the nozzle, and the velocity decays rapidly within a short distance from the nozzle outlet, and then approximately declines linearly;
- (3)
- Taking the numerical result of 4 MPa as an example, when x = 1000d—that is, 1.5 m away from the nozzle outlet—the axial velocity Um = 57.11 m/s, which decreases to 64.73% of the outlet velocity (U0 = 88.23 m/s). When x = 2500d—that is, 3.75 m away from the nozzle outlet—the axial velocity Um = 22.25 m/s, which decreases to 25.22% of the outlet velocity U0. The numerical simulation results agree well with the experimental results in the literature [36,37]; that is, the axial velocity of the water jet shows linear attenuation, and the axial velocity is ~0.7U0 at 1000d and ~0.25U0 at 2500d.
5. Analysis of Factors Affecting the Spatial Distribution of Water Jet Temperature
5.1. The Effect of the Initial Temperature of the Hot Water
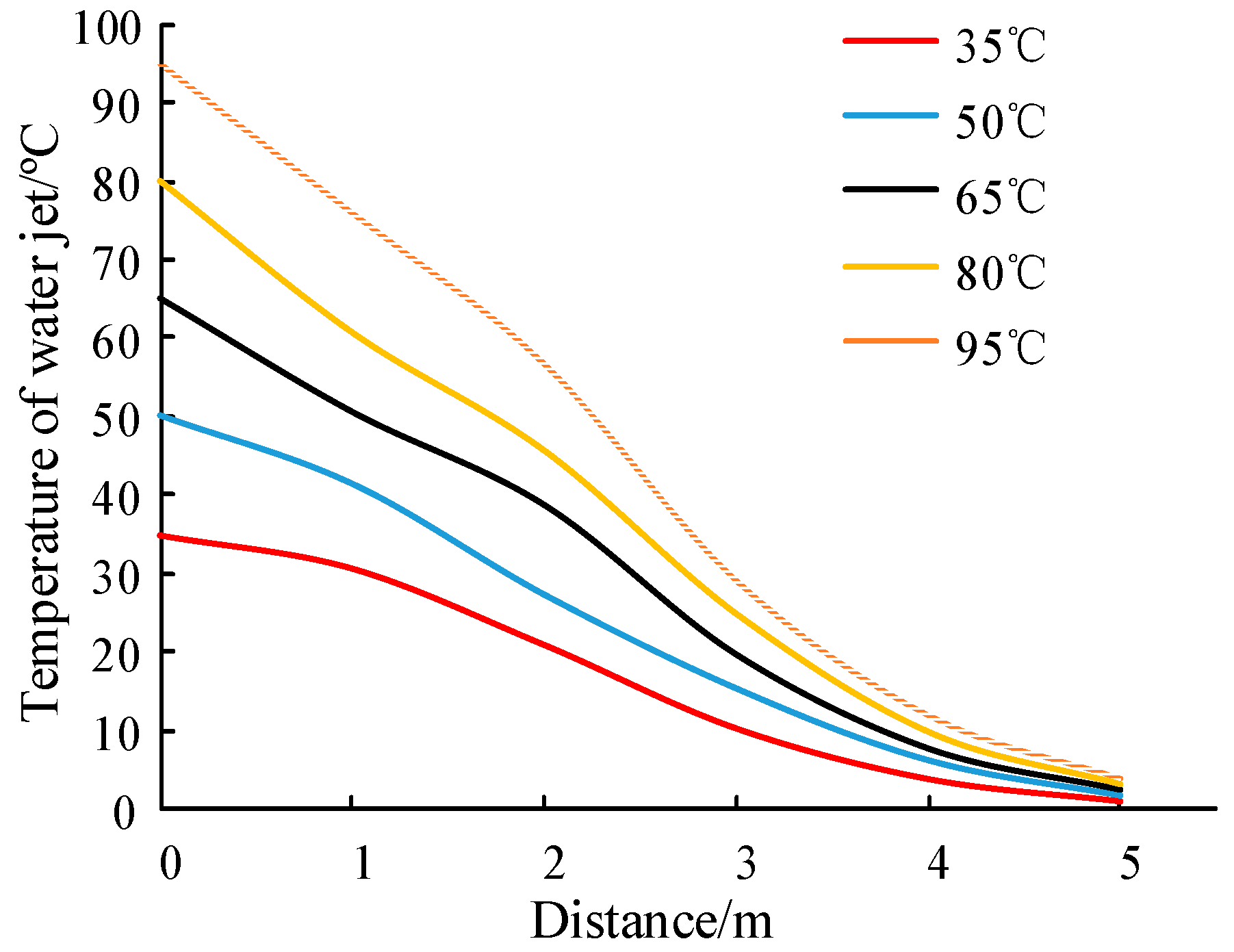
- (1)
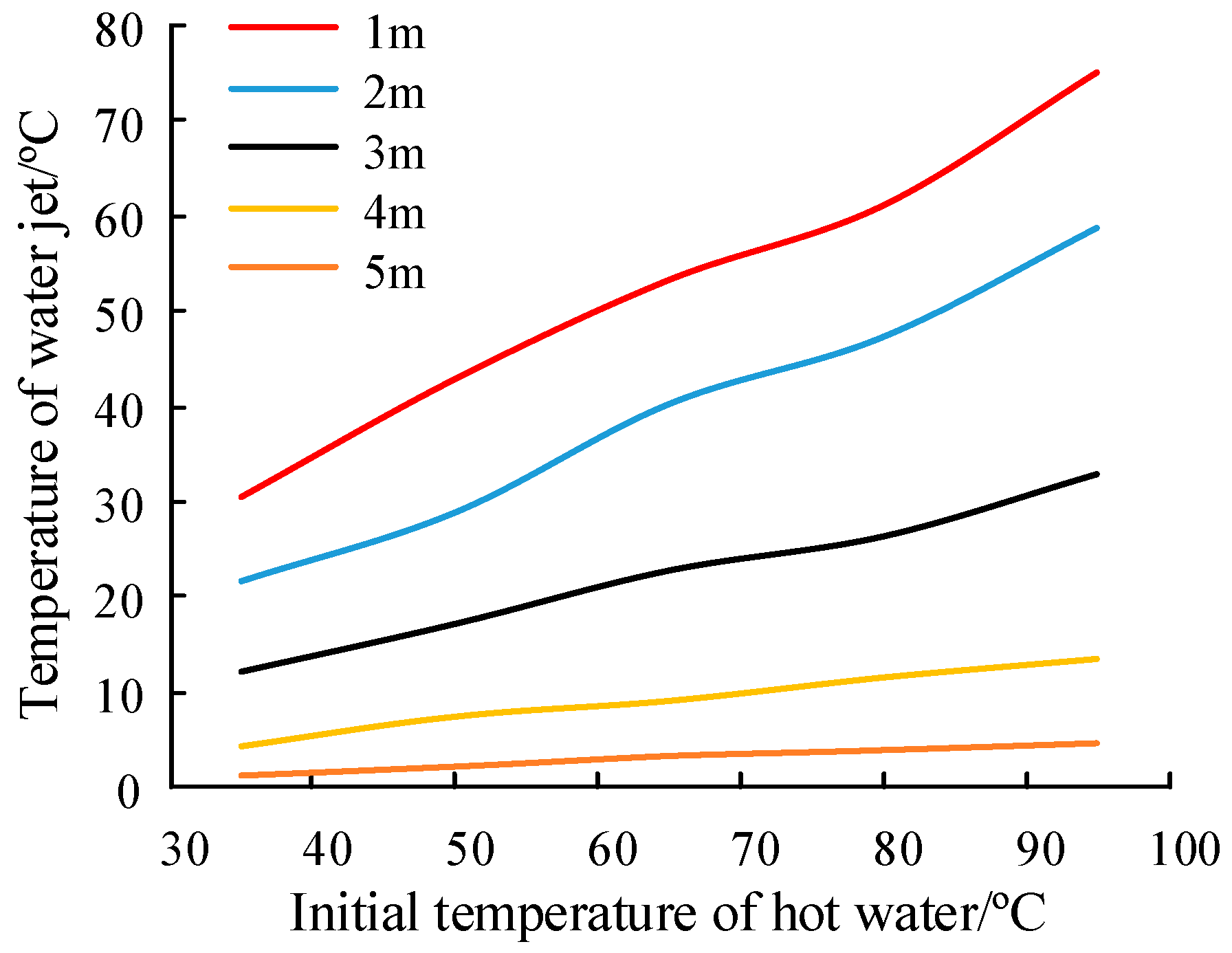
- The temperature of the water jet is positively correlated with the hot water’s initial temperature. Compared with 35 °C, the water temperatures of 50–95 °C increased the jet temperature by 33.67%, 86.73%, 119.48%, and 172.82%, showing an approximately linear increase;
- (2)
- The rate of water jet temperature increase with the initial temperature of the hot water is negatively correlated with distance—that is, the longer the distance, the lower the growth rate. As shown in Figure 12, when the distance increases from 1 m to 5 m, the increase rate of the jet temperature decreases from 0.72 to 0.06. With the increase in the jet distance, the difference between the initial temperature of the hot water and the temperature when it reaches the ice layer becomes larger—that is, the effect of the former weakens.
5.2. The Effect of Jet Distance
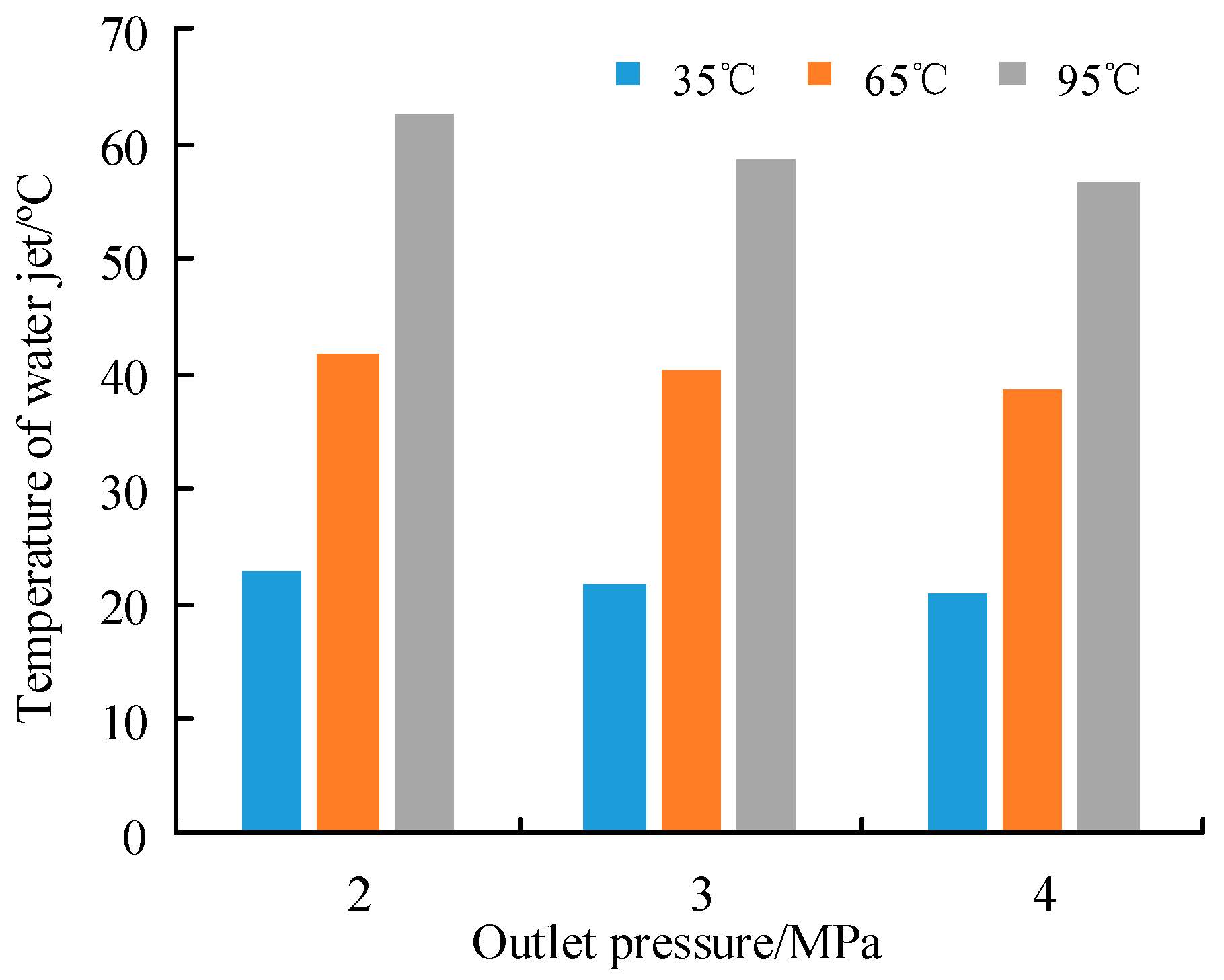
5.3. The Effect of Outlet Pressure
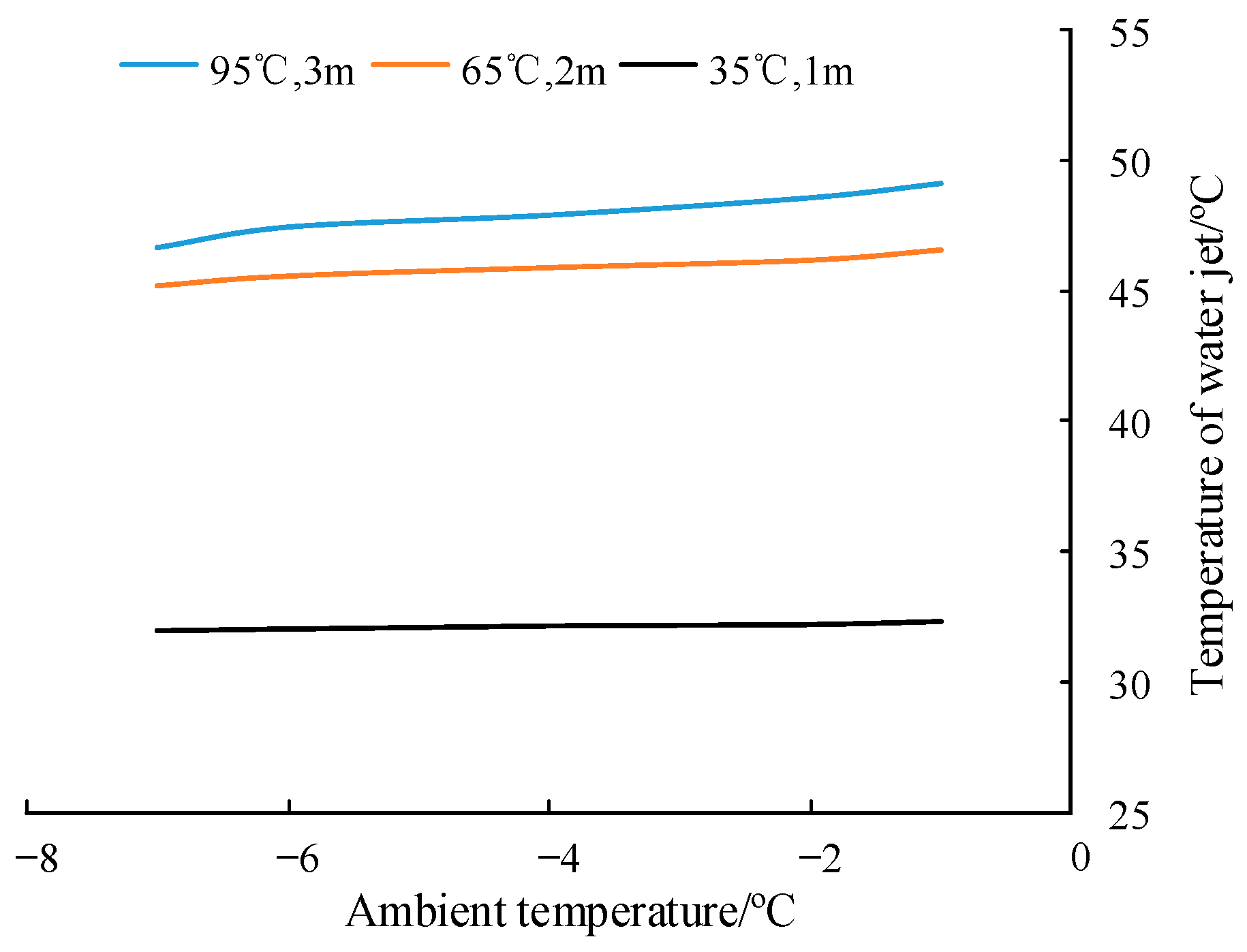
5.4. The Effect of Ambient Temperature
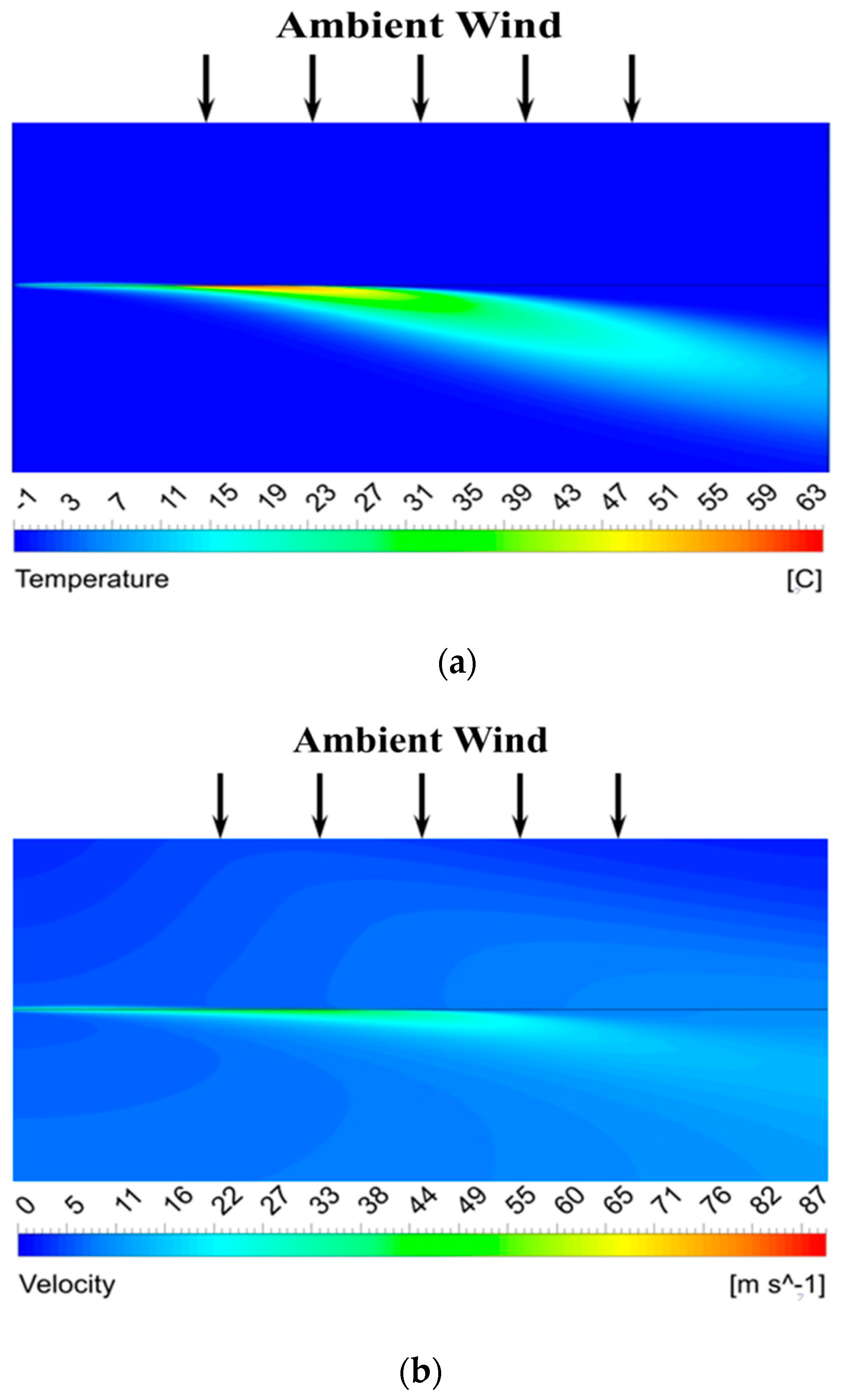
5.5. The Effect of Environmental Wind Speed
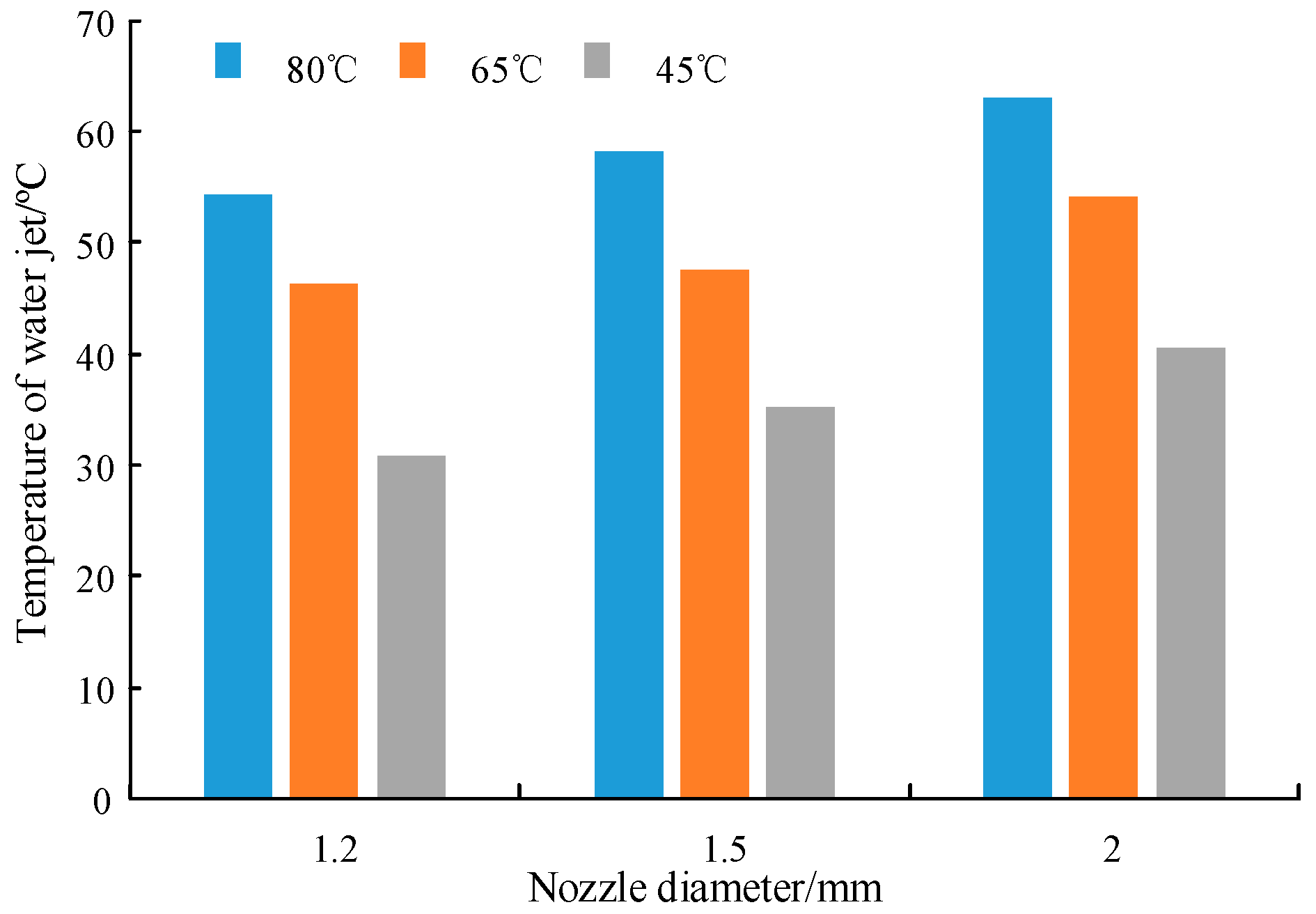
5.6. The Effect of Nozzle Diameter
6. Conclusions
- (1)
- The water column in the initial segment of the water jet is compact. The increase in distance will cause the water jet to diverge gradually, and the boundary layer will become blurred. After reaching a certain distance, the water jet “breaks”;
- (2)
- The numerical results of the hot water jet calculations are consistent with the results of the field studies. The mass entrainment of the water jet increases with the increasing distance. Under the three outlet pressures, the attenuation law of normalized axial velocity of the water jet along the axial direction is the same, and decreases approximately linearly. When the distance x = 1000d, Um = 0.63U0, and when the distance x = 2500d, Um = 0.25U0;
- (3)
- The water jet temperature is negatively correlated with distance and outlet pressure, and the distance has a greater impact. The water jet temperature is positively correlated with the initial temperature of the hot water, and the increase in distance will weaken the influence of the initial temperature of the hot water. When the ambient temperature and wind speed change the same value, the wind speed is greater than the ambient temperature. Increasing the nozzle diameter can increase the flow rate, effectively increasing the water jet temperature. According to the effective laws of the above factors on the spatial distribution of water jet temperature, the optimal parameter for ice melting can be obtained theoretically, which will be discussed in future work.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Abbreviations
| EMUs | Electric multiple units |
| VOF | Volume of fluid |
Appendix A
- (1)
- The governing equations of the VOF model
- (1)
- Volume fraction equation
- (2)
- Momentum equation
- (3)
- Energy equation
- (2)
- Experimental equipment
References
- Yin, F.; Farzaneh, M.; Jiang, X. Electrical characteristics of an energized conductor under various weather conditions. High Volt. 2017, 2, 102–109. [Google Scholar] [CrossRef]
- Ale-Emran, S.M.; Farzaneh, M. Flashover performance of ice-covered post insulators with booster sheds using experiments and partial arc modeling. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 979–986. [Google Scholar] [CrossRef]
- Hu, Q.; Wang, S.; Shu, L.; Jiang, X.; Liang, J.; Qiu, G. Comparison of AC icing flashover performances of 220 kV composite insulators with different shed configurations. IEEE Trans. Dielectr. Electr. Insul. 2016, 23, 995–1004. [Google Scholar] [CrossRef]
- Farzaneh, M.; Chisholm, W.A. 50 years in icing performance of outdoor insulators. IEEE Electr. Insul. Mag. 2014, 30, 14–24. [Google Scholar] [CrossRef]
- Jiang, X.; Fan, C.; Xie, Y. New method of preventing ice disaster in power grid using expanded conductors in heavy icing area. IET Gener. Transm. Distrib. 2019, 13, 536–542. [Google Scholar] [CrossRef]
- Jiang, X.; Xiang, Z.; Zhang, Z.; Hu, J.; Hu, Q.; Shu, L. Predictive Model for Equivalent Ice Thickness Load on Overhead Transmission Lines Based on Measured Insulator String Deviations. IEEE Trans. Power Deliv. 2014, 29, 1659–1665. [Google Scholar] [CrossRef]
- Hu, Q.; Yuan, W.; Shu, L.; Jiang, X.; Wang, S. Effects of electric field distribution on icing and flashover performance of 220 kV composite insulators. IEEE Trans. Dielectr. Electr. Insul. 2014, 21, 2181–2189. [Google Scholar] [CrossRef]
- Polhman, J.C.; Landers, P. Present state-of-the-art of transmission line icing. IEEE Trans. Power Appar. Syst. 1982, 101, 2443–2450. [Google Scholar] [CrossRef]
- Losowski, E.P.; Gayet, J.F. Atmospheric icing: A review. Process. IWAIS 1988, 3, 1–6. [Google Scholar]
- Laforte, J.; Allaire, M.; Laflamme, J. State-of-the-art on power line de-icing. Atmos. Res. 1998, 46, 143–158. [Google Scholar] [CrossRef]
- Chang, G.; Su, S.; Li, M.; Chao, D. Novel Deicing Approach of Overhead Bundled Conductors of EHV Transmission Systems. IEEE Trans. Power Deliv. 2009, 24, 1745–1747. [Google Scholar] [CrossRef]
- Farzaneh, M.; Jakl, F.; Arabani, M.P.; Eliasson, A.J.; Fikke, S.M.; Gallego, A.; Haldar, A.; Isozaki, M.; Lake, R.; Leblond, L. Systems for Prediction and Monitoring of Ice Shedding, Anti-Icing and De-Icing for Power Line Conductors and Ground Wires; CIGRE: Paris, France, 2010; p. 438. [Google Scholar]
- Yan, B.; Chen, K.; Guo, Y.; Liang, M.; Yuan, Q. Numerical Simulation Study on Jump Height of Iced Transmission Lines After Ice Shedding. IEEE Trans. Power Deliv. 2012, 28, 216–225. [Google Scholar] [CrossRef]
- Chen, Y.; Wang, Q.; Gu, B.; Cai, Y.; Wen, F. Research on the operating process of DC De-icer and its De-icing efficiency in China Southern Power Grid. In Proceedings of the 2012 10th International Power & Energy Conference (IPEC), Ho Chi Minh City, Vietnam, 12–14 December 2012; pp. 583–588. [Google Scholar]
- Wei, S.; Wang, Y.; Yang, Y.; Yin, F.; Cao, W.; Tang, Y. Applying Q-learning Algorithm to Study Line-Grasping Control Policy for Transmission Line Deicing Robot. In Proceedings of the 2010 International Conference on Intelligent System Design and Engineering Application, Changsha, China, 13–14 October 2010; pp. 382–387. [Google Scholar]
- Zhu, W. Research on De-Icing of Power Line Using CO2 Laser. Master’s Thesis, School of Optoelectronic Science and Engineering, Huazhong University of Science and Technology, Wuhan, China, 2007. [Google Scholar]
- Qi, L.; Zhu, X.; Guo, F.; Gu, S. Deicing with Nd:YAG and CO2 lasers. Opt. Eng. 2010, 49, 114301. [Google Scholar] [CrossRef]
- Xie, T.; Dong, J.; Chen, H.; Jiang, Y.; Yao, Y. Experiment investigation on de-icing characteristics and energy efficiency using infrared ray as heat source. Energy 2016, 116, 998–1005. [Google Scholar] [CrossRef]
- Li, B.; He, L.; Liu, Y.; Zhang, G. Influences of key factors in hot-air de-icing for live substation equipment. Cold Reg. Sci. Tech. 2019, 160, 89–96. [Google Scholar] [CrossRef]
- Xie, T.; Dong, J.; Chen, H.; Jiang, Y.; Yao, Y. Experimental investigation of de-icing characteristics using hot air as heat source. Appl. Therm. Eng. 2016, 107, 681–688. [Google Scholar] [CrossRef]
- Incropera, F.P.; Dewitt, D.P. Fundamentals of Heat and Mass Transfer; John Wiley & Sons Press: New York, NY, USA, 2007. [Google Scholar]
- Takahashi, K.; Usami, N.; Shibata, T.; Goto, K.; Uehara, T.; Kondo, H.; Ishikawa, R.; Saeki, H. Experimental study on a deicing method using a water jet. In Proceedings of the Oceans ′04 MTS/IEEE Techno-Ocean ′04 (IEEE Cat. No.04CH37600), Kobe, Japan, 9–12 November 2004; pp. 1668–1673. [Google Scholar]
- Guo, Z.; Long, X.; Liu, Q.; Zhang, M. Experimental study on water-jet de-icing. In Proceedings of the 2014 ISFMFE—6th International Symposium on Fluid Machinery and Fluid Engineering, Wuhan, China, 22–22 October 2014; pp. 1–4. [Google Scholar]
- Zhu, Z.; Zhang, X.; Wang, Q.; Chu, W. Research and experiment of thermal water de-icing device. Trans. Can. Soc. Mech. Eng. 2015, 39, 783–788. [Google Scholar] [CrossRef]
- Zhou, J.Z.; Xu, X.P.; Chu, W.J.; Dai, J.Y.; Chen, Y.H.; Zhu, Z.C.; Lai, S.W. R&D of a New De-Icing Device Based on Synthetically De-Icing Techniques. Appl. Mech. Mater. 2013, 372, 381–386. [Google Scholar] [CrossRef]
- Ji, C. Experimental Research and Simulation Analysis for EMU de-icing method. Master’s Thesis, Mechanical Engineering Tianjin University, Tianjin, China, 2012. [Google Scholar]
- Zhang, Z.; Yang, S.; Jiang, X.; Ma, X.; Huang, H.; Pang, G.; Ji, Y.; Dong, K. Hot Water Deicing Method for Insulators Part 2: Analysis of Ice Melting Process, Deicing Efficiency and Safety Distance. IEEE Access 2020, 8, 130729–130739. [Google Scholar]
- Wen, J.; Qi, Z.; Behbahani, S.S.; Pei, X.; Iseley, T. Research on the structures and hydraulic performances of the typical direct jet nozzles for water jet technology. J. Braz. Soc. Mech. Sci. Eng. 2019, 41, 570. [Google Scholar] [CrossRef]
- Zhou, J.Z.; Xu, X.P.; Chu, W.J.; Zhu, Z.C.; Chen, Y.H.; Lai, S.W. Analysis and Simulation of the Fluid Field in Thermal Water-Jet Nozzle Based on ANSYS FLUENT & ICEM CFD. Appl. Mech. Mater. 2013, 423–426, 1677–1684. [Google Scholar] [CrossRef]
- Guha, A.; Barron, R.M.; Balachandar, R. Numerical simulation of high-speed turbulent water jets in air. J. Hydraul. Res. 2010, 48, 119–124. [Google Scholar] [CrossRef]
- Srinivasan, V.; Salazar, A.J.; Saito, K. Modeling the disintegration of modulated liquid jets using volume-of-fluid (VOF) methodology. Appl. Math. Model. 2011, 35, 3710–3730. [Google Scholar] [CrossRef]
- Tikhomirov, R.A.; Petukhov, E.N.; Babanin, V.F. High-Pressure Jet Cutting; ASME Press: New York, NY, USA, 1992. [Google Scholar]
- Turner, J.S. Turbulent entrainment: The development of the entrainment assumption, and its application to geophysical flows. J. Fluid Mech. 1986, 173, 431–471. [Google Scholar] [CrossRef]
- Yu, Y.; Li, X.; Meng, H.; Wang, Y.; Song, M.; Wu, J. Jet flow and entrainment characteristics of nozzles with different shapes. Chin. J. Process Eng. 2014, 14, 549–555. [Google Scholar]
- Li, D.; Fan, J.; Cen, K. Direct numerical simulation of the entrainment coefficient and turbulence properties for compressible spatially evolving axisymmetric jets. Fuel 2012, 102, 470–477. [Google Scholar] [CrossRef]
- Rajaratnam, N.; Rizvi, S.A.H.; Steffler, P.M.; Smy, P.R. An experimental study of very high velocity circular water jets in air. J. Hydraul. Res. 1994, 32, 461–470. [Google Scholar] [CrossRef]
- Guha, A.; Barron, R.M.; Balachandar, R. An experimental and numerical study of water jet cleaning process. J. Mater. Process. Technol. 2011, 211, 610–618. [Google Scholar] [CrossRef] [Green Version]
- Holman, J.P. Heat Transfer; China Machine Press: Beijing, China, 2011. [Google Scholar]
- Pang, G.A.; Laufer, J.; Niessner, R. Photoacoustic Signal Generation in Gold Nanospheres in Aqueous Solution: Signal Generation Enhancement and Particle Diameter Effects. J. Phys. Chem. C 2016, 6, 09374. [Google Scholar] [CrossRef]
- Gandolfi, M.; Banfi, F.; Glorieux, C. Optical wavelength dependence of photoacoustic signal of gold nanofluid. Photoacoustics 2020, 20, 100199. [Google Scholar] [CrossRef]
- Chen, Y.S.; Frey, W.; Kim, S. Silica-Coated Gold Nanorods as Photoacoustic Signal Nanoamplifiers. Nano Lett. 2011, 11, 348–354. [Google Scholar] [CrossRef] [PubMed]
- Yuyong, L.; Puhua, T.; Daijun, J.; Kefu, L. Artificial neural network model of abrasive water jet cutting stainless steel process. In Proceedings of the 2010 International Conference on Mechanic Automation and Control Engineering, Wuhan, China, 26–28 June 2010; pp. 3507–3511. [Google Scholar] [CrossRef]
- Li, J.H.; Kim, J.T.; Lee, M.J.; Jee, S.C.; Kang, H.J.; Kim, M.K.; Kwak, H.W.; Kim, S.B.; Oh, T.W. Water jetting arm optimal design consideration for a ROV trencher. In Proceedings of the OCEANS 2015—Genova, Genova, Italy, 18–21 May 2015; pp. 1–5. [Google Scholar] [CrossRef]
- Wang, G.; Senocak, I.; Shyy, W.; Ikohagi, T.; Cao, S. Dynamics of attached turbulent cavitating flows. Prog. Aerosp. Sci. 2001, 37, 551–581. [Google Scholar] [CrossRef]
- Sun, Z.; Kang, X.; Wang, X. Experimental system of cavitation erosion with water-jet. Mater. Des. 2004, 26, 59–63. [Google Scholar] [CrossRef]
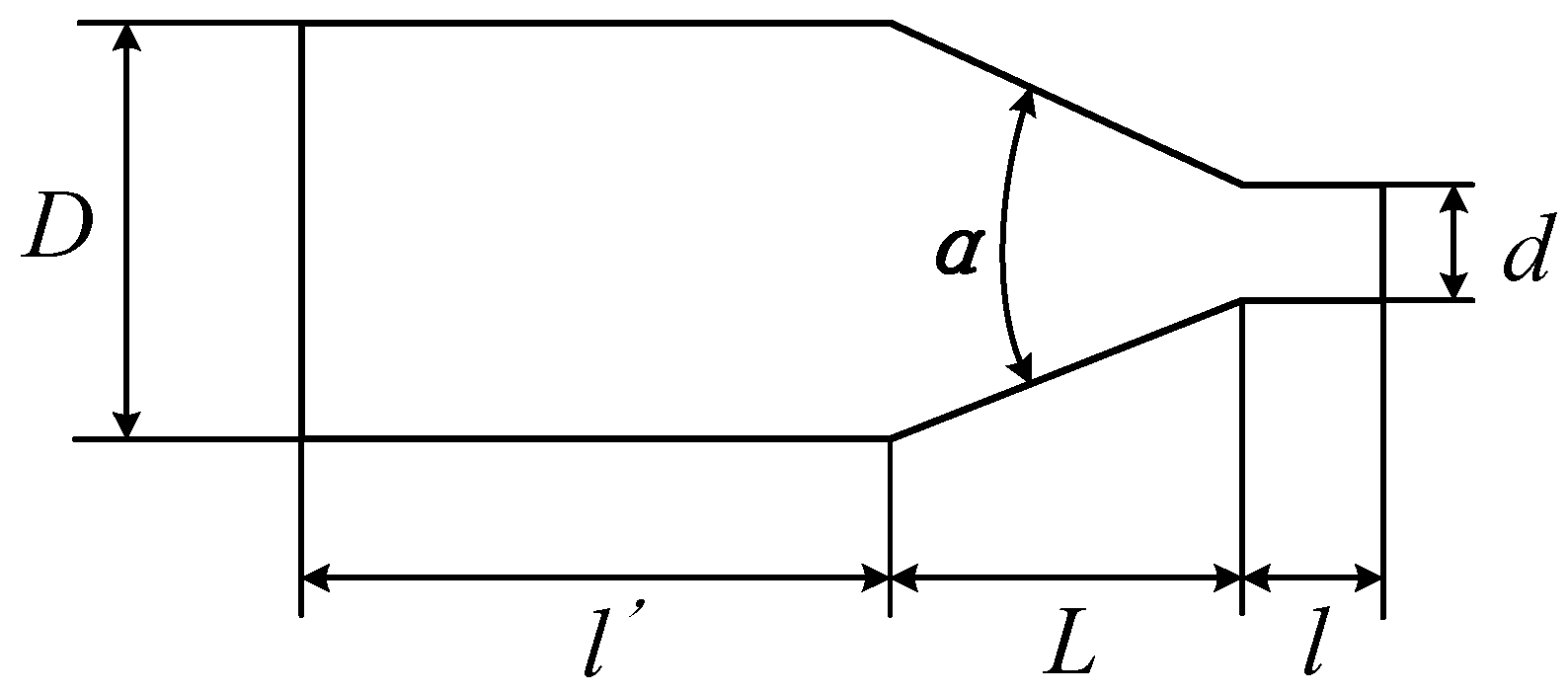





| Parameters | D (mm) | l′ (mm) | L (mm) | α (°) | d (mm) |
|---|---|---|---|---|---|
| 7 | 10 | 5 | 45 | 1–2 |
| Properties | Density (kg/m3) | Cp (J/(kg K)) | Thermal Conductivity (W/(m K)) | Viscosity (kg/(m s)) | Reference Temperature (°C) |
|---|---|---|---|---|---|
| Air | 1.225 | 1006.43 | 0.0242 | 1.7894e-05 | 25 |
| Water | 998.2 | 4182 | 0.65 | 0.001003 | 24.85 |
| Test Serial Number | Ambient Temperature (°C) | Average Wind Speed (m/s) | The Initial Temperature of Hot Water (°C) | Outlet Pressure (MPa) |
|---|---|---|---|---|
| 1 | 2 | 3.4 | 80 | 2.2 |
| 2 | 0.2 | 3.9 | 65 | 3.0 |
| 3 | 1.5 | 4.7 | 55 | 4.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Q.; Zhang, Z.; Yang, S.; Zeng, H.; Ma, X.; Huang, H.; Pang, G. Study on the Characteristics of Water Jet Injection and Temperature Spatial Distribution in the Process of Hot Water Deicing for Insulators. Energies 2022, 15, 2298. https://doi.org/10.3390/en15062298
Yang Q, Zhang Z, Yang S, Zeng H, Ma X, Huang H, Pang G. Study on the Characteristics of Water Jet Injection and Temperature Spatial Distribution in the Process of Hot Water Deicing for Insulators. Energies. 2022; 15(6):2298. https://doi.org/10.3390/en15062298
Chicago/Turabian StyleYang, Qi, Zhijin Zhang, Shenghuan Yang, Huarong Zeng, Xiaohong Ma, Huan Huang, and Guohui Pang. 2022. "Study on the Characteristics of Water Jet Injection and Temperature Spatial Distribution in the Process of Hot Water Deicing for Insulators" Energies 15, no. 6: 2298. https://doi.org/10.3390/en15062298
APA StyleYang, Q., Zhang, Z., Yang, S., Zeng, H., Ma, X., Huang, H., & Pang, G. (2022). Study on the Characteristics of Water Jet Injection and Temperature Spatial Distribution in the Process of Hot Water Deicing for Insulators. Energies, 15(6), 2298. https://doi.org/10.3390/en15062298






