Refurbishment of Natural Gas Pipelines towards 100% Hydrogen—A Thermodynamic-Based Analysis
Abstract
:1. Introduction
2. Materials and Methods
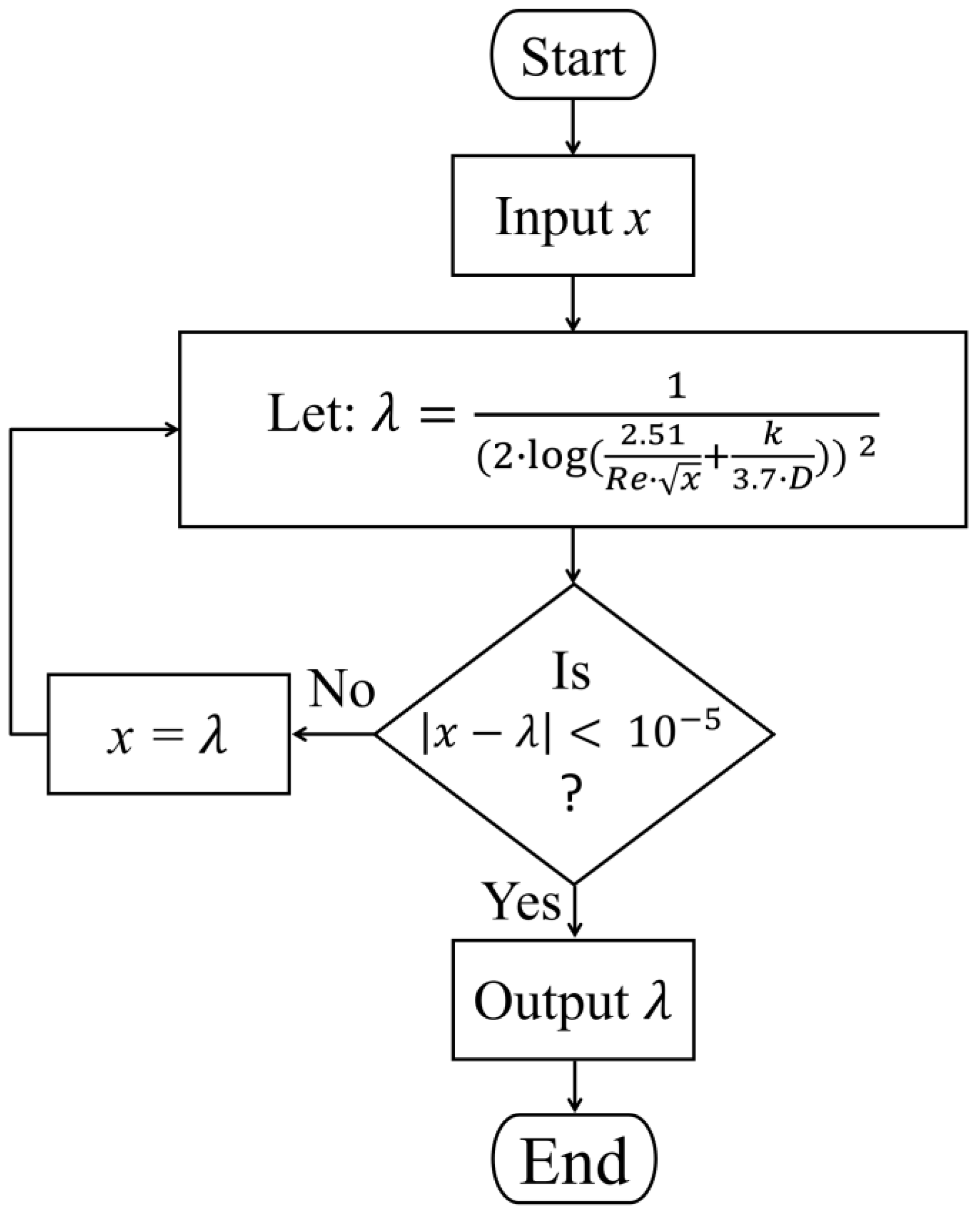
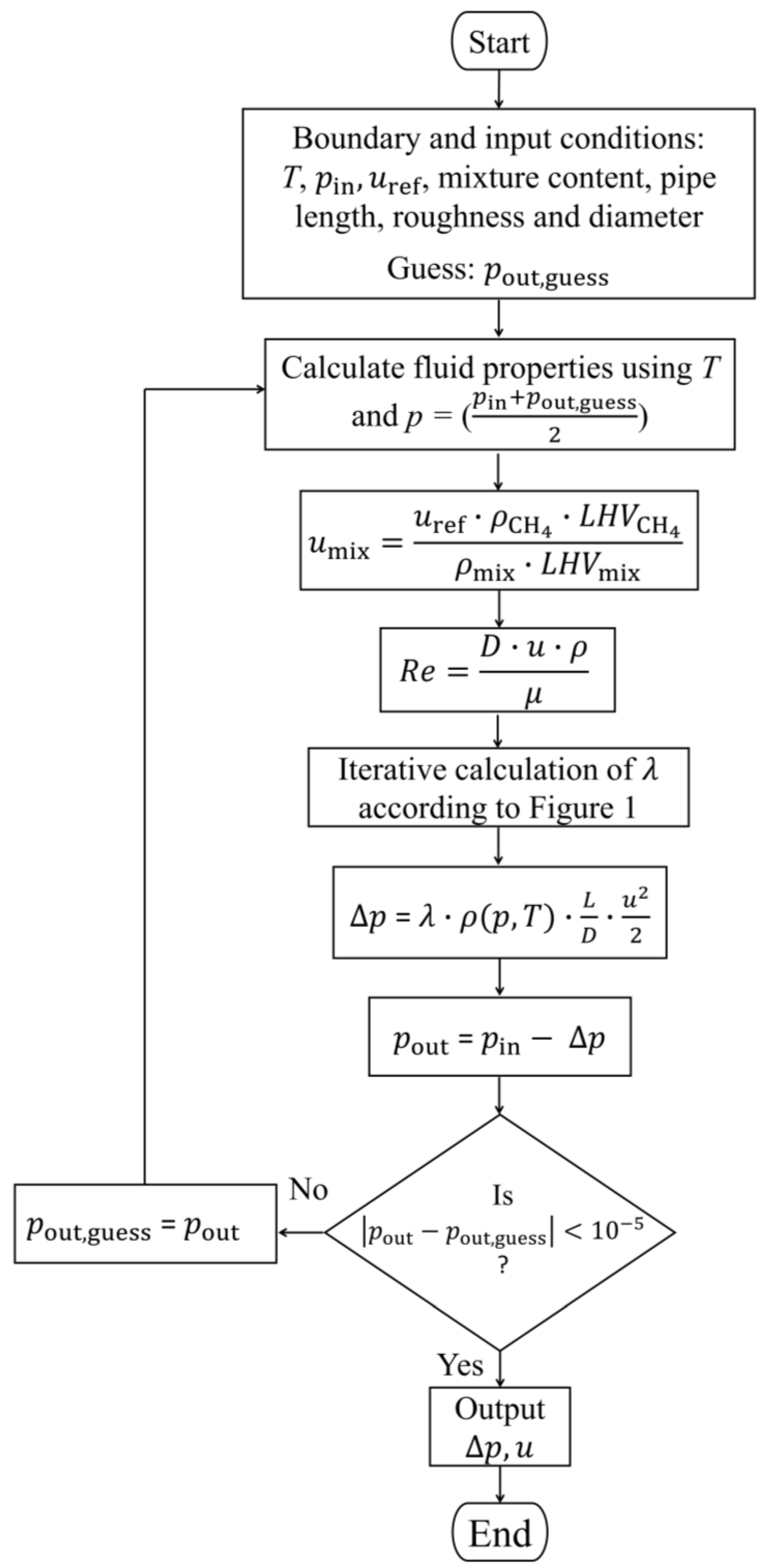
2.1. Pressure Loss Calculation
2.2. Mixture Properties
- Density:
- Lower heating value:
- Specific heat capacity isobaric:
- Specific heat capacity isochoric:
2.3. Scenario Building
- Scenario 1: constant energy flow at different hydrogen contents, with 100% NG as the reference case:
- Scenario 2: constant pressure drop over 100 km at different hydrogen contents, with 100% NG as the reference case;
2.3.1. Scenario 1-Constant Energy Flow
2.3.2. Scenario 2: Constant Pressure Drop Δp
2.4. Compressor Power and Energy Buffer
2.5. Boundary Conditions of the Reference Case
3. Results and Discussion
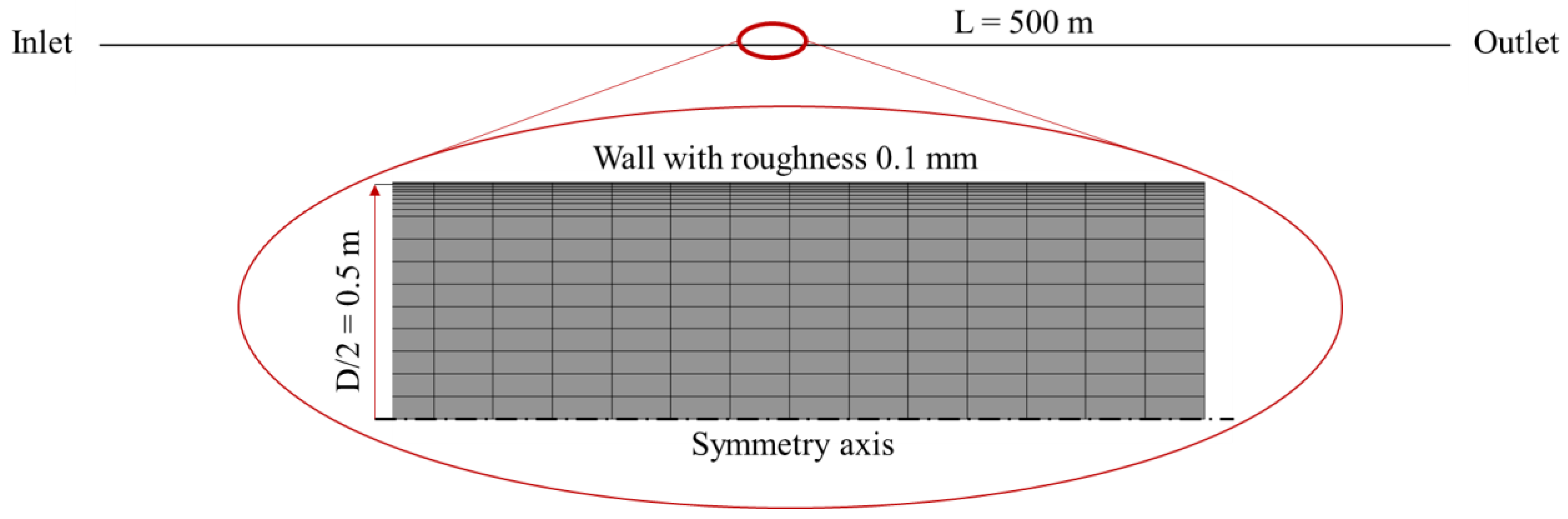
3.1. Validation of the Analytical Approach with CFD Simulation Models
3.2. Energy Transported Ė
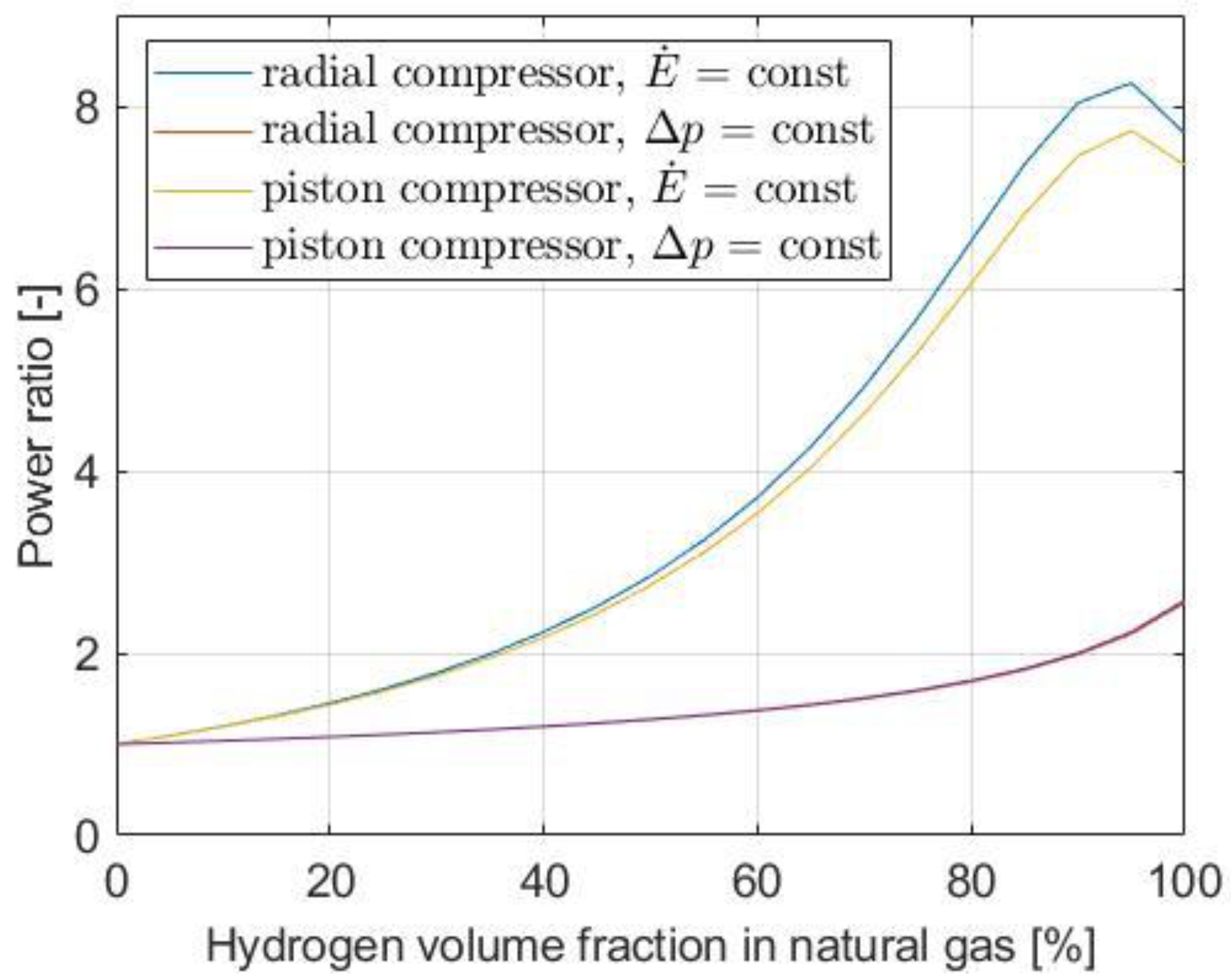
3.3. Compressor Power
3.4. Energy Buffer
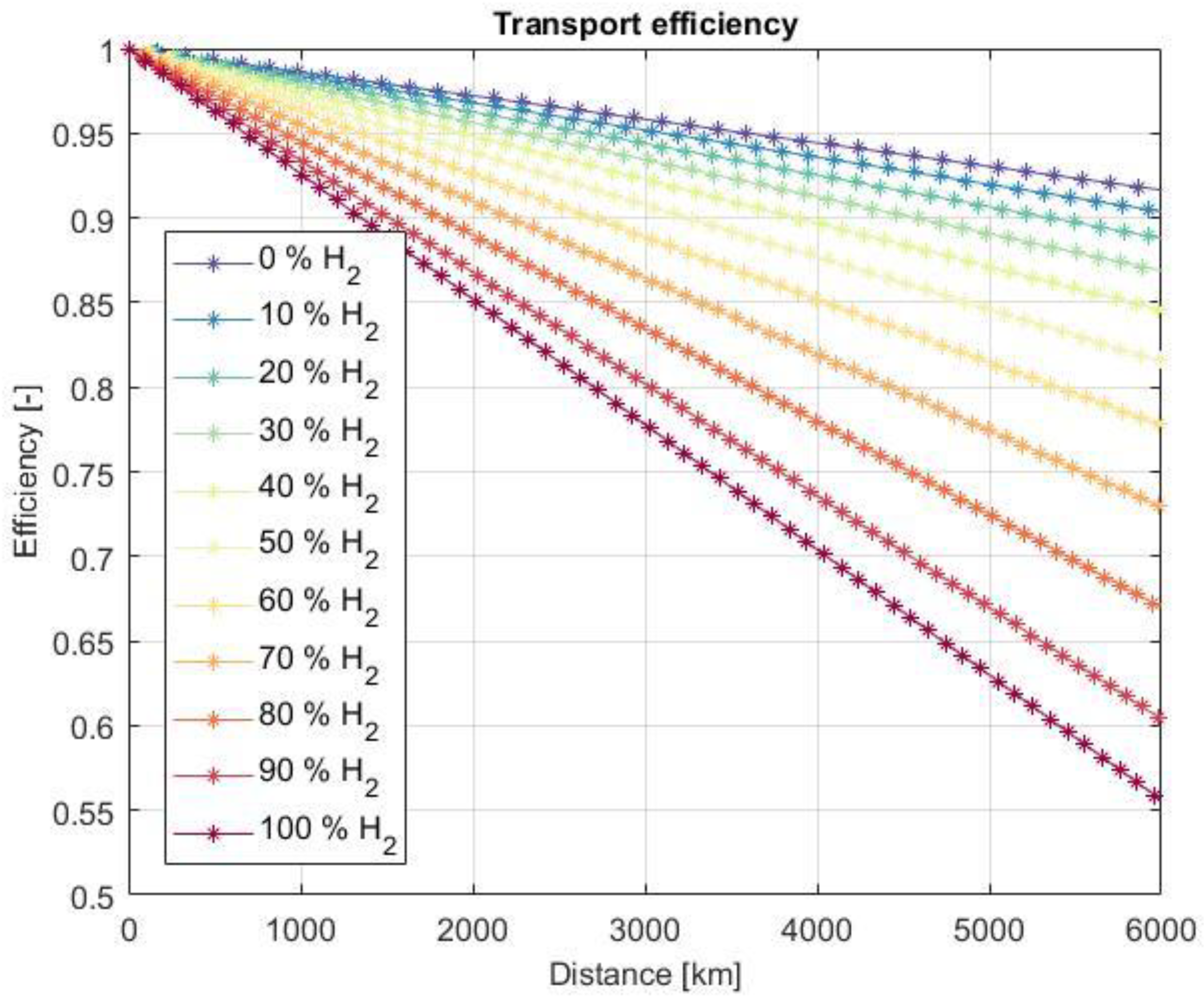
3.5. Pipeline Transport Efficiency
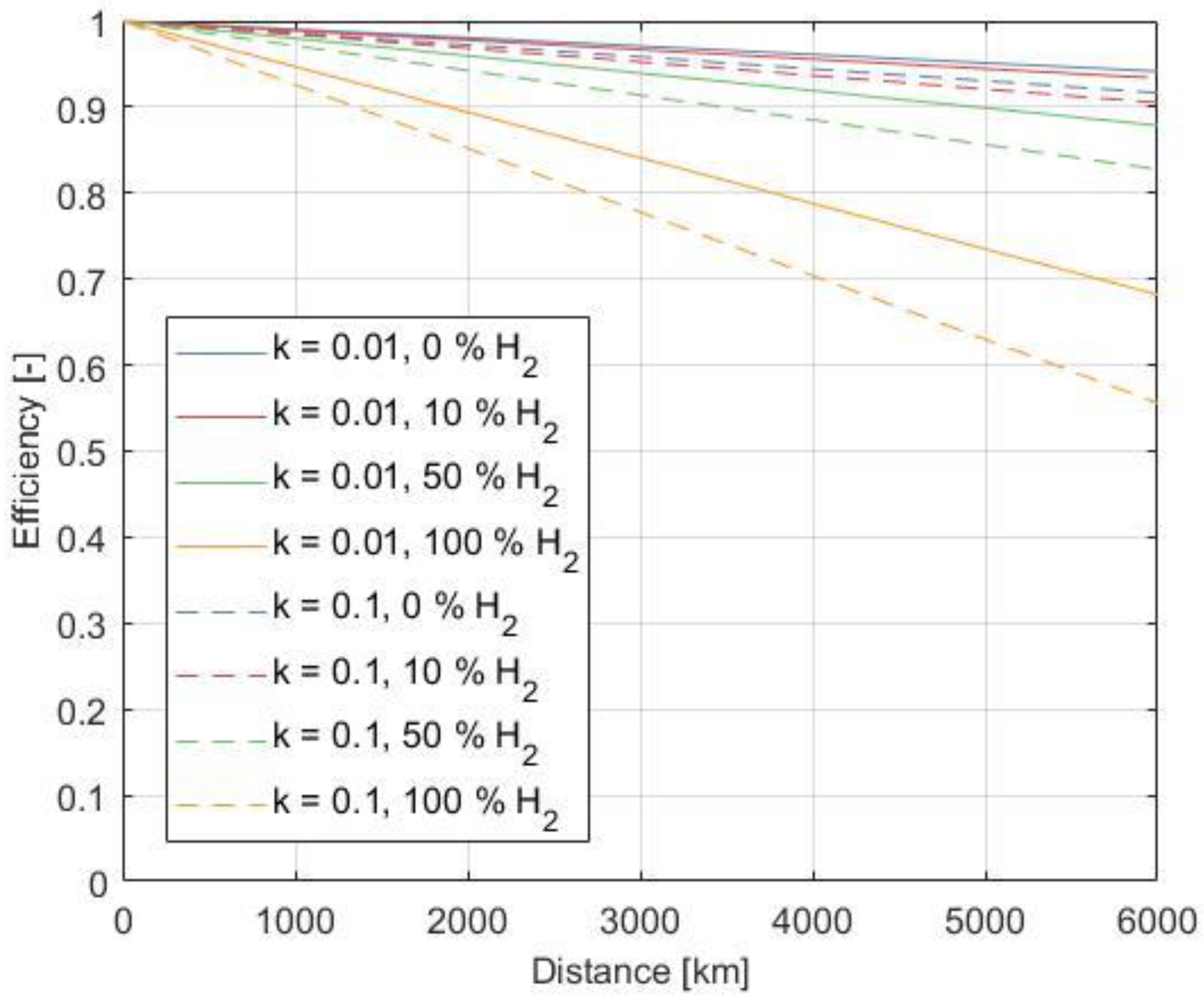
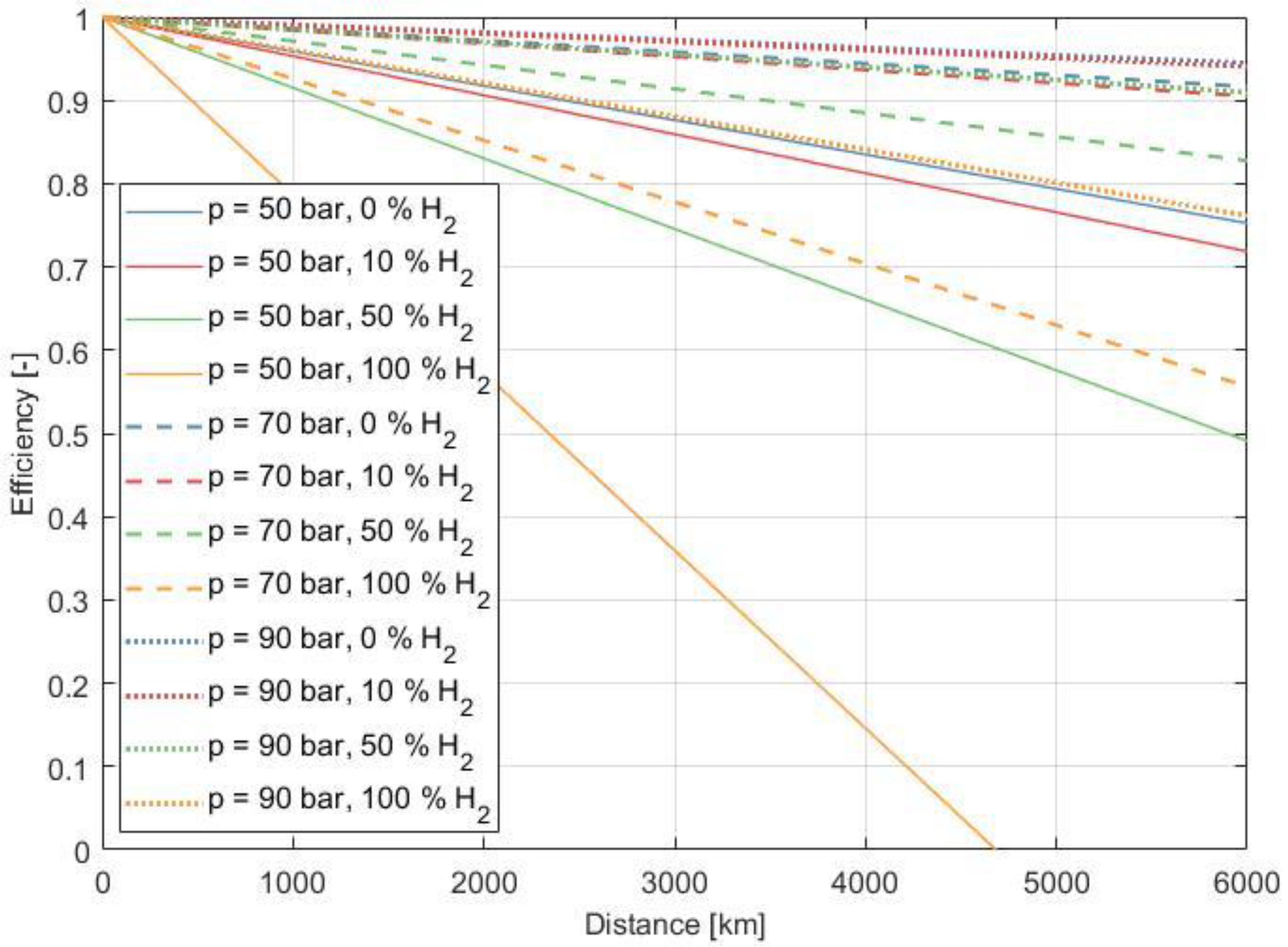
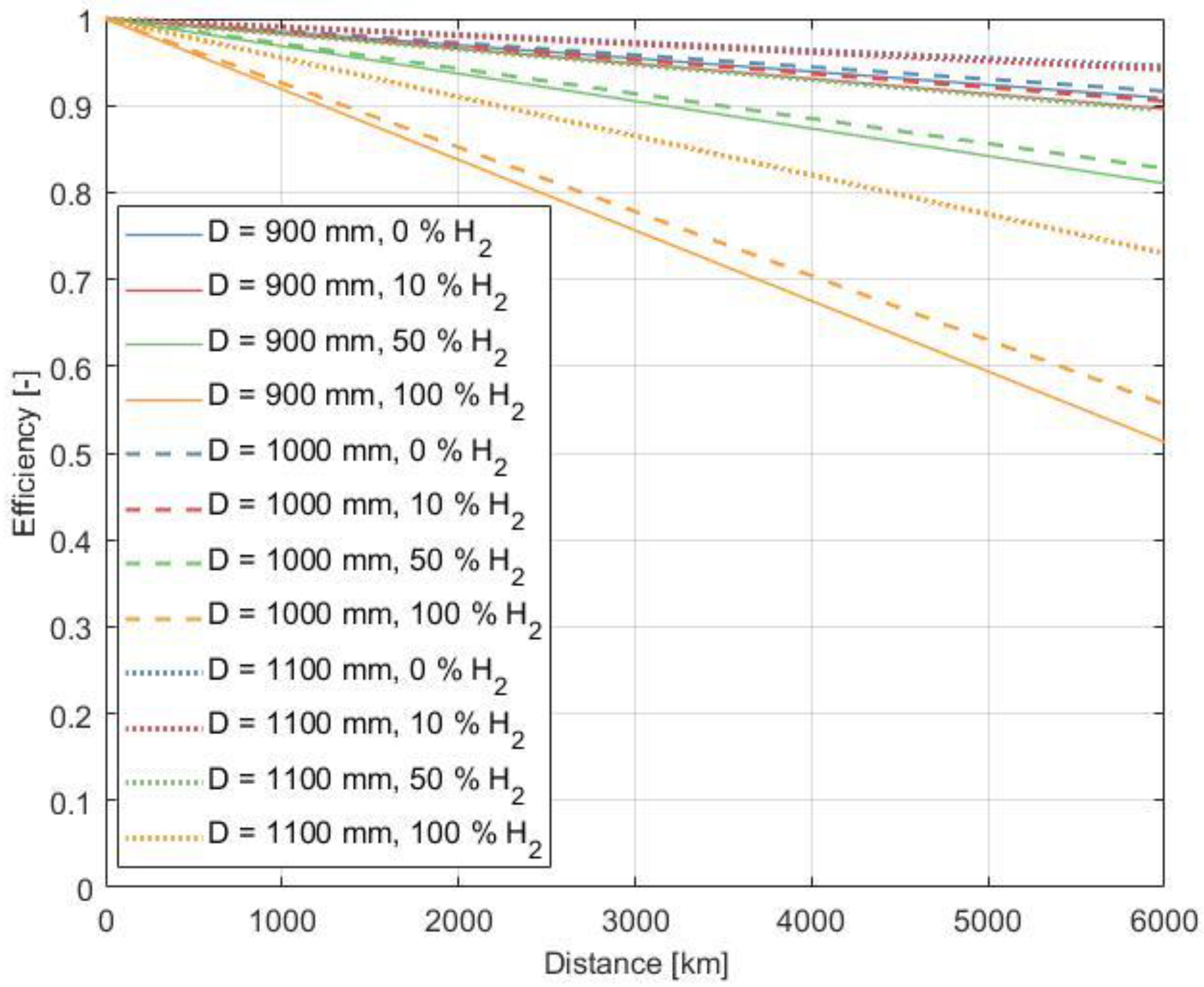
3.6. Influences on Transport Efficiency
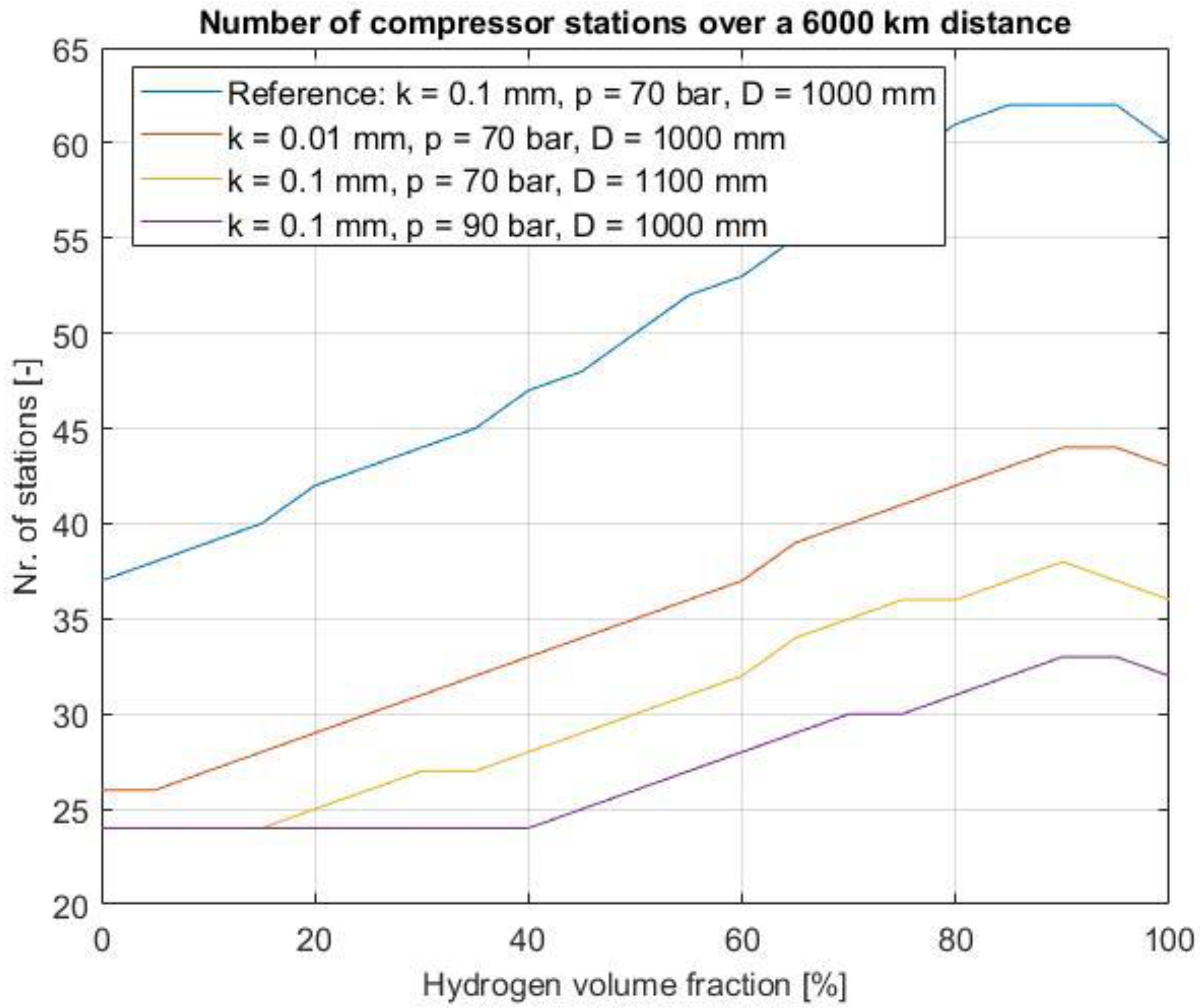
3.6.1. Wall Roughness k
3.6.2. Operating Pressure MOP
3.6.3. Pipeline Diameter
3.6.4. Distance between Compressor Stations for Hydrogen Pipeline Transport
3.7. H2 transport Efficiencies of Different Distribution Technologies
- Pipeline transport is the most energy-efficient method of transporting H2 on land in large quantities and over long distances.
- GH2 efficiency increases significantly with the pressure level. The losses for the initial compression are marginal compared to the transport losses.
- LH2 liquefaction efforts are amortized after approx. 2200 km of trailer transport.
4. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Myhre, G.; Shindell, D.; Bréon, F.-M.; Collins, W.; Fuglestvedt, J.; Huang, J.; Koch, D.; Lamarque, J.-F.; Lee, D.; Mendoza, B.; et al. Anthropogenic and Natural Radiative Forcing. In Climate Change 2013: The Physical Science Basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; Stocker, T.F., Quin, D., Plattner, G.-K., Tignor, M., Allen, S.K., Boschung, J., Nauels, A., Xia, Y., Bex, V., Midgley, P.M., Eds.; Cambridge University Press: Cambridge, UK; New York, NY, USA,, 2013; pp. 659–740. [Google Scholar]
- Lauvaux, T.; Giron, C.; Mazzolini, M.; d’Aspremont, A.; Duren, R.; Cusworth, D.; Ciais, P. Global assessment of oil and gas methane ultra-emitters. Science 2022, 375, 557–561. [Google Scholar] [CrossRef] [PubMed]
- Irakulis-Loitxate, I.; Guanter, L.; Liu, Y.-N.; Varon, D.J.; Maasakkers, J.D.; Zhang, Y.; Wofsy, S.C.; Thorpe, A.K.; Duren, R.M.; Frankenberg, C.; et al. Satellite-based survey of extreme methane emissions in the Permian basin. Sci. Adv. 2021, 7, eabf4507. [Google Scholar] [CrossRef] [PubMed]
- Statistical Review of World Energy. 2021|70th Edition. 2021. Available online: Bp.com/energyoutlook (accessed on 14 June 2022).
- Trattner, A.; Klell, M.; Radner, F. Sustainable hydrogen society–Vision, findings and development of a hydrogen economy using the example of Austria. Int. J. Hydrog. Energy 2022, 47, 2059–2079. [Google Scholar] [CrossRef]
- Klell, M.; Eichlseder, H.; Trattner, A. Wasserstoff in der Fahrzeugtechnik; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- International Energy Agency, I.E.A. The Future of Hydrogen: Seizing Today’s Opportunities. Report Prepared by the I.E. A for the G20, Japan. Available online: https://iea.blob.core.windows.net/assets/9e3a3493-b9a6-4b7d-b499-7ca48e357561/The_Future_of_Hydrogen.pdf (accessed on 7 June 2022).
- Winters, J. What Are the Colors of Hydrogen. Available online: https://www.asme.org/topics-resources/content/infographic-what-are-the-colors-of-hydrogen (accessed on 30 November 2022).
- World Energy Council. Hydrogen Demand and Cost Dynamics: Working Paper. Available online: https://www.worldenergy.org/publications/entry/working-paper-hydrogen-demand-and-cost-dynamics (accessed on 28 March 2022).
- Wang, A.; Jaro, D.; Mavins, D.; Moultak, M.; Schimmel, M.; van der Leun, K. Analysing Future Demand, Supply, and Transport of Hydrogen. European Hydrogen Backbone. Available online: https://gasforclimate2050.eu/?smd_process_download=1&download_id=718 (accessed on 9 November 2021).
- European Hydrogen Backbone. Five Hydrogen Supply Corridors for Europe in 2030, May 2022. Available online: https://ehb.eu/files/downloads/EHB-Supply-corridor-presentation-Full-version.pdf (accessed on 24 October 2022).
- Wang, A.; van der Leun, K.; Peters, D.; Buseman, M. European Hydrogen Backbone: How Dedicated Hydrogen Infrastructure Can Be Created. Available online: https://gasforclimate2050.eu/?smd_process_download=1&download_id=471 (accessed on 9 November 2021).
- ENTSOG, Gas Infrastructure Europe, Hydrogen Europe. Transport & Speicherung von Wasserstoff: Zahlen & Fakten. Available online: https://www.gasconnect.at/fileadmin/Broschueren-Folder/entsog_gie/entsog_gie_he_QA_hydrogen_transport_and_storage_DE_210630.pdf (accessed on 9 November 2021).
- Linke, G. Technical-Economic Considerations of Delivering Hydrogen via the Gas Grid: H2 Research, Standards and operational Support of the German Gas & Water Association (DVGW). 2021. Available online: https://www.irena.org/events/2021/Dec/Hydrogen-in-the-Gas-Grid-Workshop-Series (accessed on 15 November 2022).
- European Union Agency for the Cooperation of Energy Regulators. Transporting Pure Hydrogen by Repurposing Existing Gas Infrastructure: Overview of Existing Studies and Reflections on the Conditions for Repurposing. Available online: https://acer.europa.eu/Official_documents/Acts_of_the_Agency/Publication/Transporting%20Pure%20Hydrogen%20by%20Repurposing%20Existing%20Gas%20Infrastructure_Overview%20of%20studies.pdf (accessed on 30 September 2022).
- International Renewable Energy Agency, I.R. ENA. Geopolitics of the Energy Transformation: The Hydrogen Factor. Available online: https://www.irena.org/-/media/Files/IRENA/Agency/Publication/2022/Jan/IRENA_Geopolitics_Hydrogen_2022.pdf (accessed on 15 November 2022).
- Fink, S. Untersuchung zur Wasserstoffversprödung von Gasrohrleitungen; Technische Universität Graz: Graz, Austria, 2021. [Google Scholar]
- Gondal, I.A.; Sahir, M.H. Prospects of natural gas pipeline infrastructure in hydrogen transportation. Int. J. Energy Res. 2012, 36, 1338–1345. [Google Scholar] [CrossRef]
- Haeseldonckx, D.; D’haeseleer, W. The use of the natural-gas pipeline infrastructure for hydrogen transport in a changing market structure. Int. J. Hydrog. Energy 2007, 32, 1381–1386. [Google Scholar] [CrossRef]
- Horlacher, H.-B.; Helbig, U. Rohrleitungen 2; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Adam, P.; Heunemann, F.; Engelshove, S.; Bussche, C.; von dem Thiemann, T. Wasserstoffinfrastruktur–Tragende Säule der Energiewende: Umstellung von Ferngasnetzen auf Wasserstoffbetrieb in der Praxis. 2020. Available online: https://www.get-h2.de/wp-content/uploads/200915-whitepaper-h2-infrastruktur-DE.pdf (accessed on 15 November 2022).
- Bell, I.H.; Wronski, J.; Quoilin, S.; Lemort, V. CoolProp. Available online: www.coolprop.org (accessed on 26 June 2022).
- Mischner, J. Zur hydraulischen Berechnung von Niederdruckleitungen für Erdgas-Wasserstoff-Gemische: Teil 1. GWF Gas + Energie. 2021, 3, 58–69. [Google Scholar]
- Laureys, A.; Depraetere, R.; Cauwels, M.; Depover, T.; Hertelé, S.; Verbeken, K. Use of existing steel pipeline infrastructure for gaseous hydrogen storage and transport: A review of factors affecting hydrogen induced degradation. J. Nat. Gas Sci. Eng. 2022, 101, 104534. [Google Scholar] [CrossRef]
- Andrews, R.M.; Gallon, N.; Huising, O.J.C. Assessing Damaged Pipelines Transporting Hydrogen. J. Pipeline Sci. Eng. 2022, 100066. [Google Scholar] [CrossRef]
- Mohtadi-Bonab, M.A. Effect of different parameters on hydrogen affected fatigue failure in pipeline steels. Eng. Fail. Anal. 2022, 137, 106262. [Google Scholar] [CrossRef]
- Wu, X.; Zhang, H.; Yang, M.; Jia, W.; Qiu, Y.; Lan, L. From the perspective of new technology of blending hydrogen into natural gas pipelines transmission: Mechanism, experimental study, and suggestions for further work of hydrogen embrittlement in high-strength pipeline steels. Int. J. Hydrog. Energy 2022, 47, 8071–8090. [Google Scholar] [CrossRef]
- Sun, Y.; Frank Cheng, Y. Hydrogen-induced degradation of high-strength steel pipeline welds: A critical review. Eng. Fail. Anal. 2022, 133, 105985. [Google Scholar] [CrossRef]
- Bouledroua, O.; Hafsi, Z.; Djukic, M.B.; Elaoud, S. The synergistic effects of hydrogen embrittlement and transient gas flow conditions on integrity assessment of a precracked steel pipeline. Int. J. Hydrog. Energy 2020, 45, 18010–18020. [Google Scholar] [CrossRef]
- Li, H.; Niu, R.; Li, W.; Lu, H.; Cairney, J.; Chen, Y.-S. Hydrogen in pipeline steels: Recent advances in characterization and embrittlement mitigation. J. Nat. Gas Sci. Eng. 2022, 105, 104709. [Google Scholar] [CrossRef]
- Martin, M.L.; Connolly, M.; Buck, Z.N.; Bradley, P.E.; Lauria, D.; Slifka, A.J. Evaluating a natural gas pipeline steel for blended hydrogen service. J. Nat. Gas Sci. Eng. 2022, 101, 104529. [Google Scholar] [CrossRef]
- Wang, H.; Tong, Z.; Zhou, G.; Zhang, C.; Zhou, H.; Wang, Y.; Zheng, W. Research and demonstration on hydrogen compatibility of pipelines: A review of current status and challenges. Int. J. Hydrog. Energy 2022, 47, 28585–28604. [Google Scholar] [CrossRef]
- Liu, C.; Pei, Y.; Cui, Z.; Li, X.; Yang, H.; Xing, X.; Duan, P.; Li, L.; Li, Y. Study on the stratification of the blended gas in the pipeline with hydrogen into natural gas. Int. J. Hydrog. Energy, in press (corrected proof). 2022. [Google Scholar] [CrossRef]
- Eames, I.; Austin, M.; Wojcik, A. Injection of gaseous hydrogen into a natural gas pipeline. Int. J. Hydrog. Energy 2022, 47, 25745–25754. [Google Scholar] [CrossRef]
- Ekhtiari, A.; Flynn, D.; Syron, E. Investigation of the Multi-Point Injection of Green Hydrogen from Curtailed Renewable Power into a Gas Network. Energies 2020, 13, 6047. [Google Scholar] [CrossRef]
- Pellegrini, M.; Guzzini, A.; Saccani, C. A Preliminary Assessment of the Potential of Low Percentage Green Hydrogen Blending in the Italian Natural Gas Network. Energies 2020, 13, 5570. [Google Scholar] [CrossRef]
- Vaccariello, E.; Trinchero, R.; Stievano, I.S.; Leone, P. A Statistical Assessment of Blending Hydrogen into Gas Networks. Energies 2021, 14, 5055. [Google Scholar] [CrossRef]
- Melaina, M.W.; Antonia, O.; Penev, M. Blending Hydrogen into Natural Gas Pipeline Networks: A Review of Key Issues; National Renewable Energy Laboratory: Golden, CO, USA, 2013. [CrossRef]
- Mischner, J. Zur Frage der Strömungsgeschwindigkeiten in Gasleitungen. GWF Gas + Energie 2021, 2021, 44–63. [Google Scholar]
- Bschorer, S. Technische Strömungslehre; Springer: Berlin/Heidelberg, Germany, 2018. [Google Scholar]
- Moran, M.J.; Shapiro, H.N.; Boettner, D.D.; Bailey, M.B. Fundamentals of Engineering Thermodynamics, 9th ed.; Wiley: Hoboken, NJ, USA, 2018. [Google Scholar]
- Eichelseder, H.; Sturm, P. Thermodynamik; Institute of Internal Combustion Engines and Thermodynamics, TU Graz: Graz, Austria, 2018. [Google Scholar]
- Wilke, C.R. A Viscosity Equation for Gas Mixtures. J. Chem. Phys. 1950, 18, 517–519. [Google Scholar] [CrossRef]
- Bell, I.H.; Wronski, J.; Quoilin, S.; Lemort, V. Pure and Pseudo-pure Fluid Thermophysical Property Evaluation and the Open-Source Thermophysical Property Library CoolProp. Ind. Eng. Chem. Res. 2014, 53, 2498–2508. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- van Rossum, R.; Jens, J.; La Gaudia, G.; Wang, A.; Kühnen, L.; Overgaag, M. European Hydrogen Backbone: A European Hydrogen Infrastructure Vision Covering 28 Countries. Available online: https://gasforclimate2050.eu/?smd_process_download=1&download_id=1038 (accessed on 5 April 2022).
- Abbas, A.J.; Hassani, H.; Burby, M.; John, I.J. An Investigation into the Volumetric Flow Rate Requirement of Hydrogen Transportation in Existing Natural Gas Pipelines and Its Safety Implications. Gases 2021, 1, 156–179. [Google Scholar] [CrossRef]
- Krieg, D. Konzept und Kosten Eines Pipelinesystems zur Versorgung des Deutschen Straßenverkehrs mit Wasserstoff. RWTH Aachen University, Dissertation, 2012. Forschungszentrum Jülich GmbH: Jülich, 2012. Available online: https://juser.fz-juelich.de/record/136392/files/Energie% (accessed on 20 July 2022).
- ANSYS Inc. Ansys Fluent Theory Guide, 2022R1 2022. Available online: https://ansyshelp.ansys.com/account/secured?returnurl=/Views/Secured/prod_page.html?pn=Fluent&pid=Fluent&prodver=22.1&lang=en (accessed on 20 July 2022).
- Adam, P.; Barton, M.; Lake, K.; Schulz, M. Hydrogen Compression–An Integral Part of the H2 Value Chain: White Paper. Available online: https://www.siemens-energy.com/global/en/offerings/technical-papers/whitepaper-h2-compression.html (accessed on 25 May 2022).
- Österreichische Vereinigung für das Gas- und Wasserfach–ÖVGW. Richtlinie G B210 Gasbeschaffenheit; 2021. Available online: https://portal.ovgw.at/pls/f?p=101:203::::RP,203:P203_ID,P203_FROM_PAGE_ID:1075524,202 (accessed on 20 July 2022).
- Bender, B.; Göhlich, D. Dubbel Taschenbuch für den Maschinenbau 3: Maschinen und Systeme; Springer: Berlin, Heidelberg, 2020. [Google Scholar]
- Di Bella, F. Development of a Centrifugal Hydrogen Pipeline Compressor: Technical Memorandum No. 1785. Available online: https://www.osti.gov/servlets/purl/1227195 (accessed on 19 May 2022).
- United Pipeline System. Tite Liner Thermoplastic Pipeline Lining System. Available online: https://www.aegion.com/capabilities/hdpe-pipe-lining (accessed on 28 June 2022).
- Pertl, P. Well-to-Wheel Potentials of H2-Mobility; Wien, 2019. Available online: https://www.a3ps.at/sites/default/files/conferences/2014/papers/15_pertl_well-to-wheel_potentials_of_h2_mobility_a3ps_pertl.pdf;. (accessed on 20 July 2022).
- Rodriguez, F.; Delgado, O.; Muncrief, R. The International Council on Clean Transportation. Fuel consumption testing of tractor-trailers in the European Union and the United States 2018. Available online: https://theicct.org/wp-content/uploads/2021/06/EU_HDV_Testing_BriefingPaper_20180515a.pdf (accessed on 15 November 2022).




| Characteristic | H2 | CH4 |
|---|---|---|
| Molar mass [kg/kmol] | 2.016 | 16.04 |
| Gravimetric energy density [MJ/kg]/[kWh/kg] | 119.93/33.314 | 55.598/15.444 |
| Upper volumetric heating value [MJ/Nm3]/[kWh/Nm3] | 12.744/3.540 | 39.830/11.064 |
| Lower volumetric heating value [MJ/Nm3]/[kWh/Nm3] | 10.782/2.995 | 35.896/9.971 |
| Density [kg/Nm³] | 0.0898 | 0.7175 |
| Specific gravity [-] | 0.0696 | 0.5537 |
| Density at 70 bar, 10 °C [kg/m3] | 5.7459 | 55.353 |
| Upper Wobbe index [MJ/Nm3 | 48.34 | 53.45 |
| Lower Wobbe index [MJ/Nm3] | 40.90 | 48.17 |
| Specific heat capacity [kJ/(kg·K)] | 14.198 | 2.1810 |
| Kinematic viscosity [cm2/s] | 0.9342 | 0.1429 |
| Specifications and Assumptions | |
|---|---|
| Line type | Gas transport line |
| Natural gas composition | 100% CH4 |
| Transported energy amount | 14,856,000 kWh/h |
| Inner diameter | DN 1000 |
| Maximum operating pressure | MOP 70 |
| Material | L450MB |
| Line length | 100 km |
| Average gas temperature | 283 K |
| Initial pressure | 70 bar |
| Average gas flow velocity at 100% NG | 8 m/s |
| Average wall roughness k | 0.1 mm |
| Model Settings | |
|---|---|
| Energy equation | on |
| Turbulence model | Realizable- k-ε |
| Wall model | Enhanced wall treatment |
| Real gas model | Redlich-Kwong |
| Species transport | CH4 and H2 |
| Case 1 | Case 2 | Case 3 | |
|---|---|---|---|
| Gas composition | 100% CH4 | 50% CH4 50% H2 | 100% H2 |
| Temperature [K] | 283 | ||
| Inlet pressure [bar] | 70 | ||
| Outlet massflow [kg/s] | 297.12 | 256.88 | 123.80 |
| Wall flux [W/m2] | 0 | ||
| Wall roughness [mm] | 0.1 | ||
| Δp Fluent [Pa] | Δp Matlab [Pa] | Relative Error [%] | |
|---|---|---|---|
| Case 1: 100% CH4 | 7849 | 7800 | 0.6243 |
| Case 2: 50% CH4 50% H2 | 11008 | 10500 | 4.6148 |
| Case 3: 100% H2 | 13194 | 13100 | 0.7124 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Klopčič, N.; Stöhr, T.; Grimmer, I.; Sartory, M.; Trattner, A. Refurbishment of Natural Gas Pipelines towards 100% Hydrogen—A Thermodynamic-Based Analysis. Energies 2022, 15, 9370. https://doi.org/10.3390/en15249370
Klopčič N, Stöhr T, Grimmer I, Sartory M, Trattner A. Refurbishment of Natural Gas Pipelines towards 100% Hydrogen—A Thermodynamic-Based Analysis. Energies. 2022; 15(24):9370. https://doi.org/10.3390/en15249370
Chicago/Turabian StyleKlopčič, Nejc, Thomas Stöhr, Ilena Grimmer, Markus Sartory, and Alexander Trattner. 2022. "Refurbishment of Natural Gas Pipelines towards 100% Hydrogen—A Thermodynamic-Based Analysis" Energies 15, no. 24: 9370. https://doi.org/10.3390/en15249370
APA StyleKlopčič, N., Stöhr, T., Grimmer, I., Sartory, M., & Trattner, A. (2022). Refurbishment of Natural Gas Pipelines towards 100% Hydrogen—A Thermodynamic-Based Analysis. Energies, 15(24), 9370. https://doi.org/10.3390/en15249370








