A Comparison between Statistical Behaviours of Scalar Dissipation Rate between Homogeneous MILD Combustion and Premixed Turbulent Flames
Abstract
1. Introduction
- (a)
- to demonstrate the effects of turbulence intensity and O2 dilution on the statistical behaviour of the SDR and the terms of its transport equation in MILD turbulent combustion
- (b)
- to illustrate the differences in the SDR transport behaviour between homogeneous mixture MILD combustion and conventional premixed flames, and between homogeneous mixture MILD combustion and passive scalar mixing
- (c)
- to provide physical explanations for (a) and (b) and indicate the modelling implications.
2. Mathematical Background
3. Numerical Implementation
- 1D laminar premixed flames are simulated for the thermochemical conditions shown in Table 1. An initial bimodal distribution of based on CH4 mass fraction with prescribed integral length scale is then developed using the methodology by Eswaran and Pope [24]. The 1D laminar profiles of species mass fraction for are specified as a function of the progress variable based on the CH4 mass fraction. These functions alongside the bimodal distribution of are used to initialise the density and species mass fractions corresponding to atmospheric pressure and an unburned gas temperature of 1500 K (i.e., ).
- The generated bimodal scalar fields in the previous step are then allowed to evolve under the generated turbulence for about 1 eddy turnover time in a periodic domain mimicking the EGR in MILD combustion. At the end of this step, the mean and variance of the preprocessed field are and , respectively, where is the mean value evaluated over the whole domain. The temperature in the preprocessed mixture has a variation of about of its mean value.
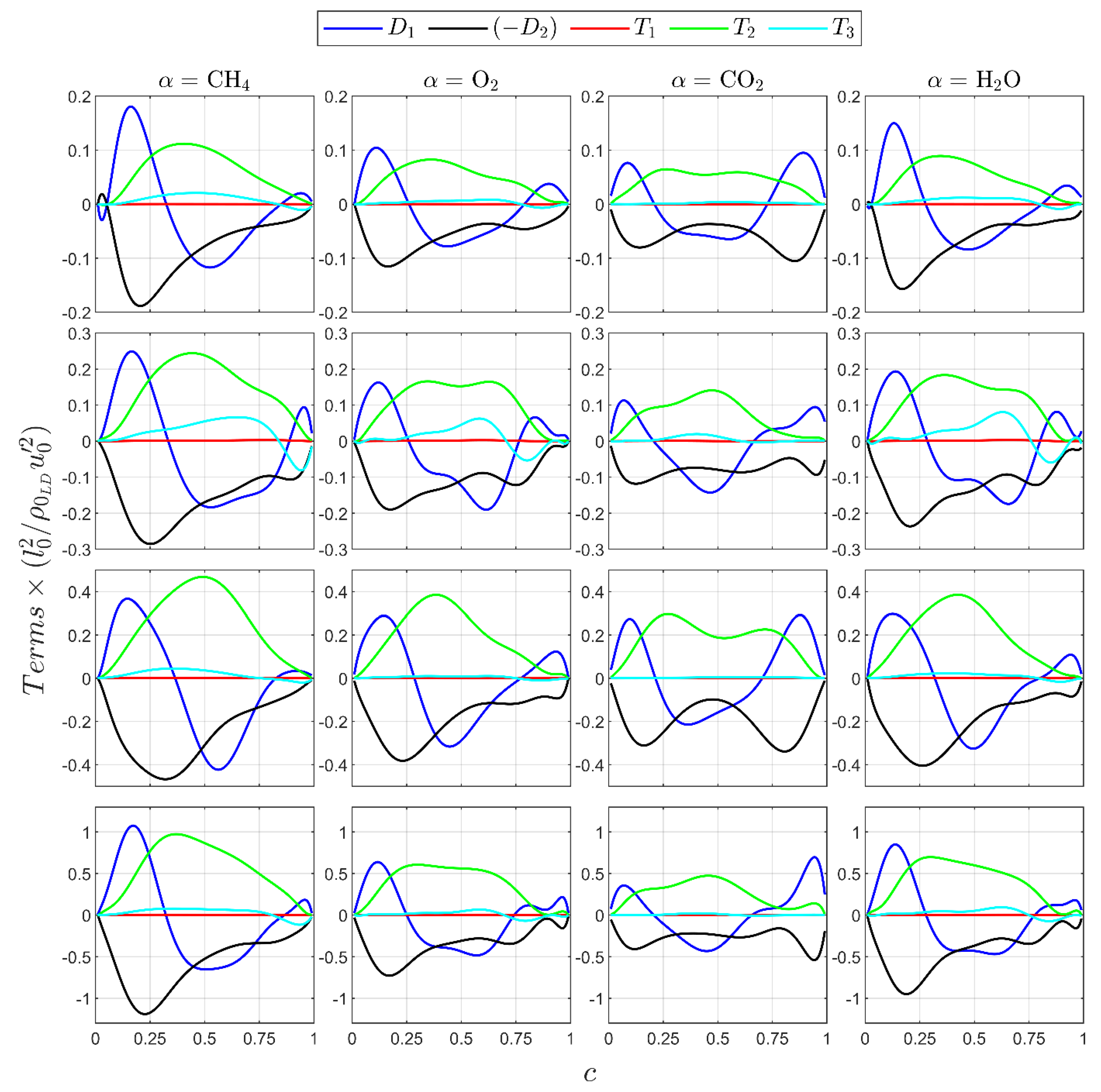
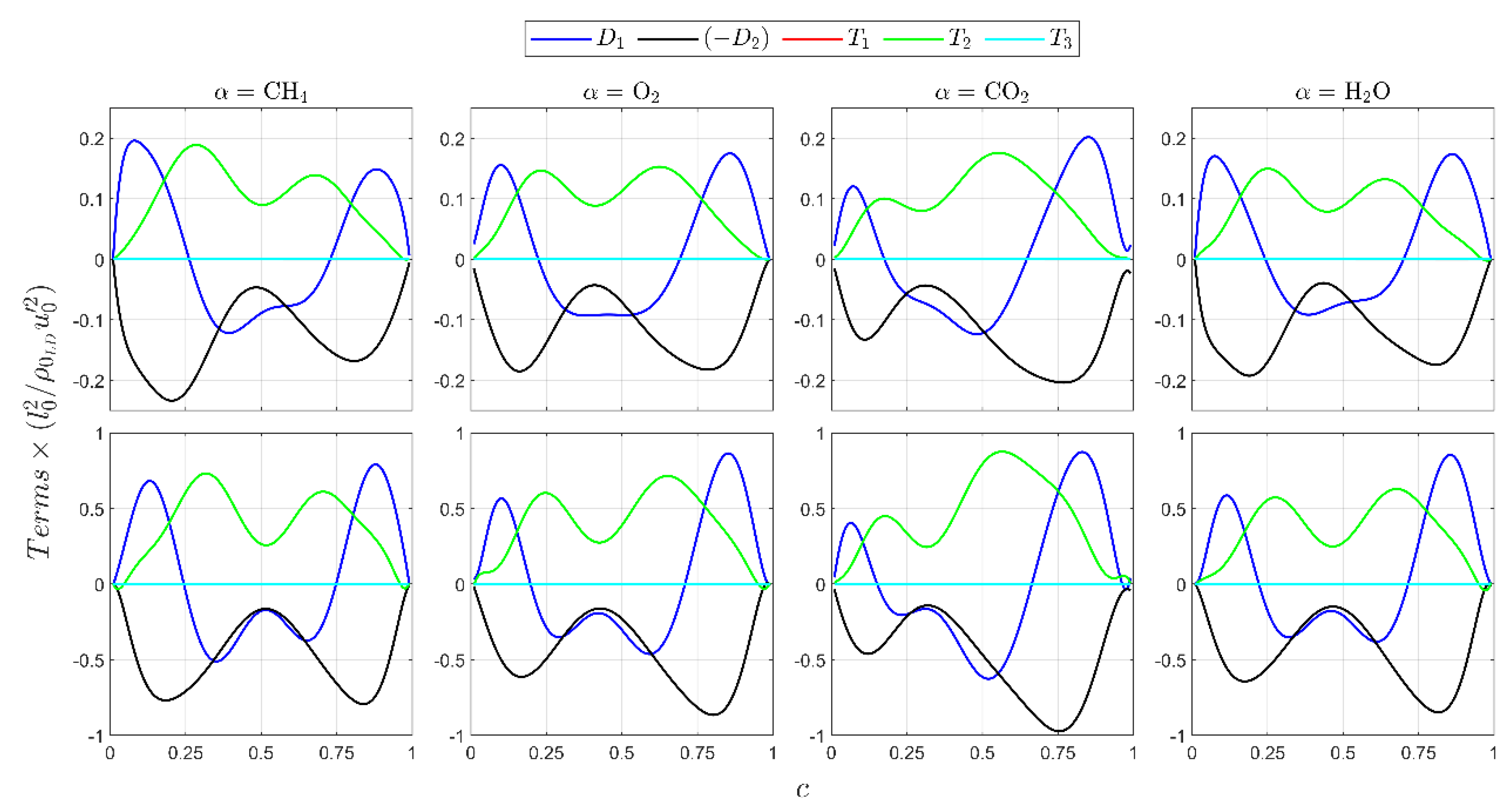
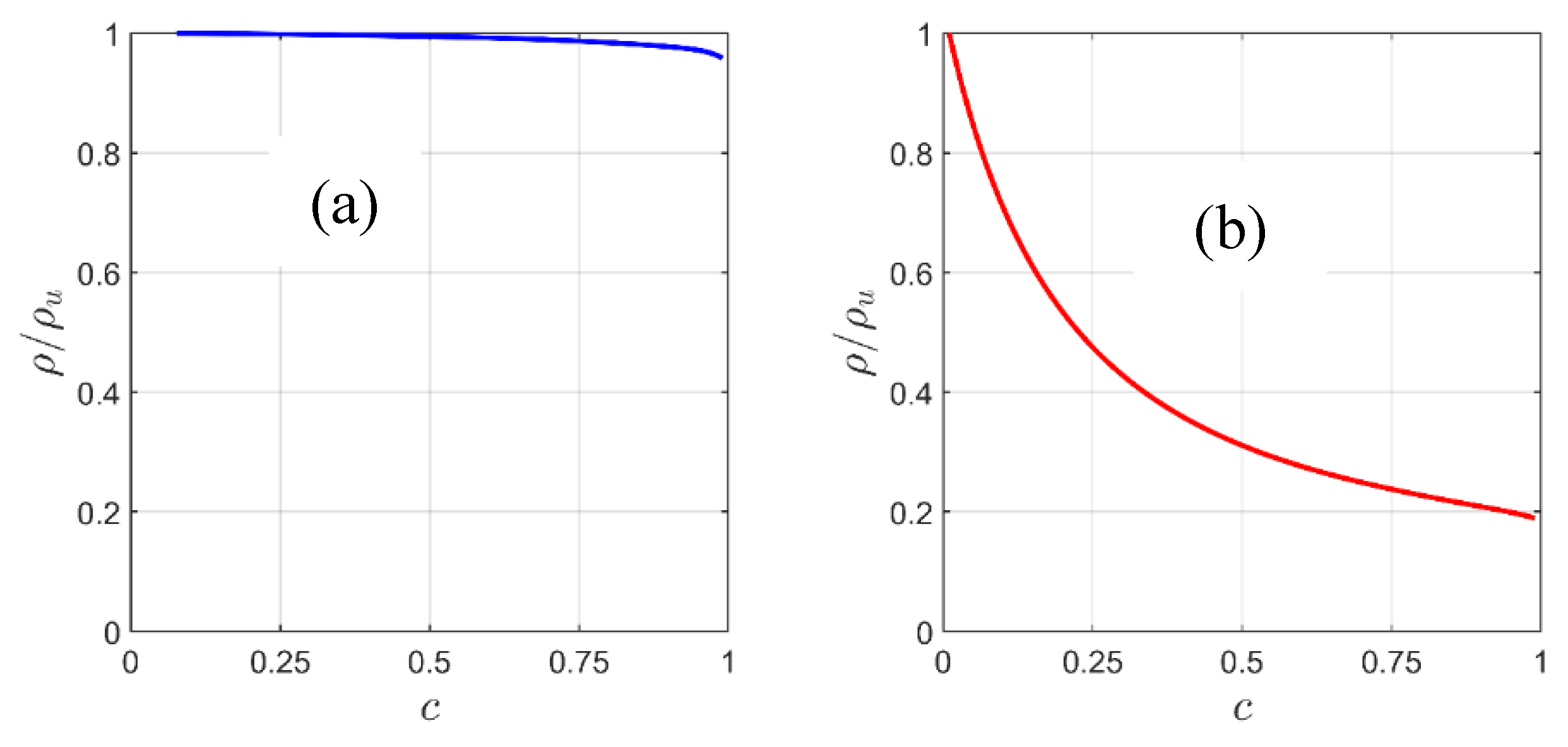
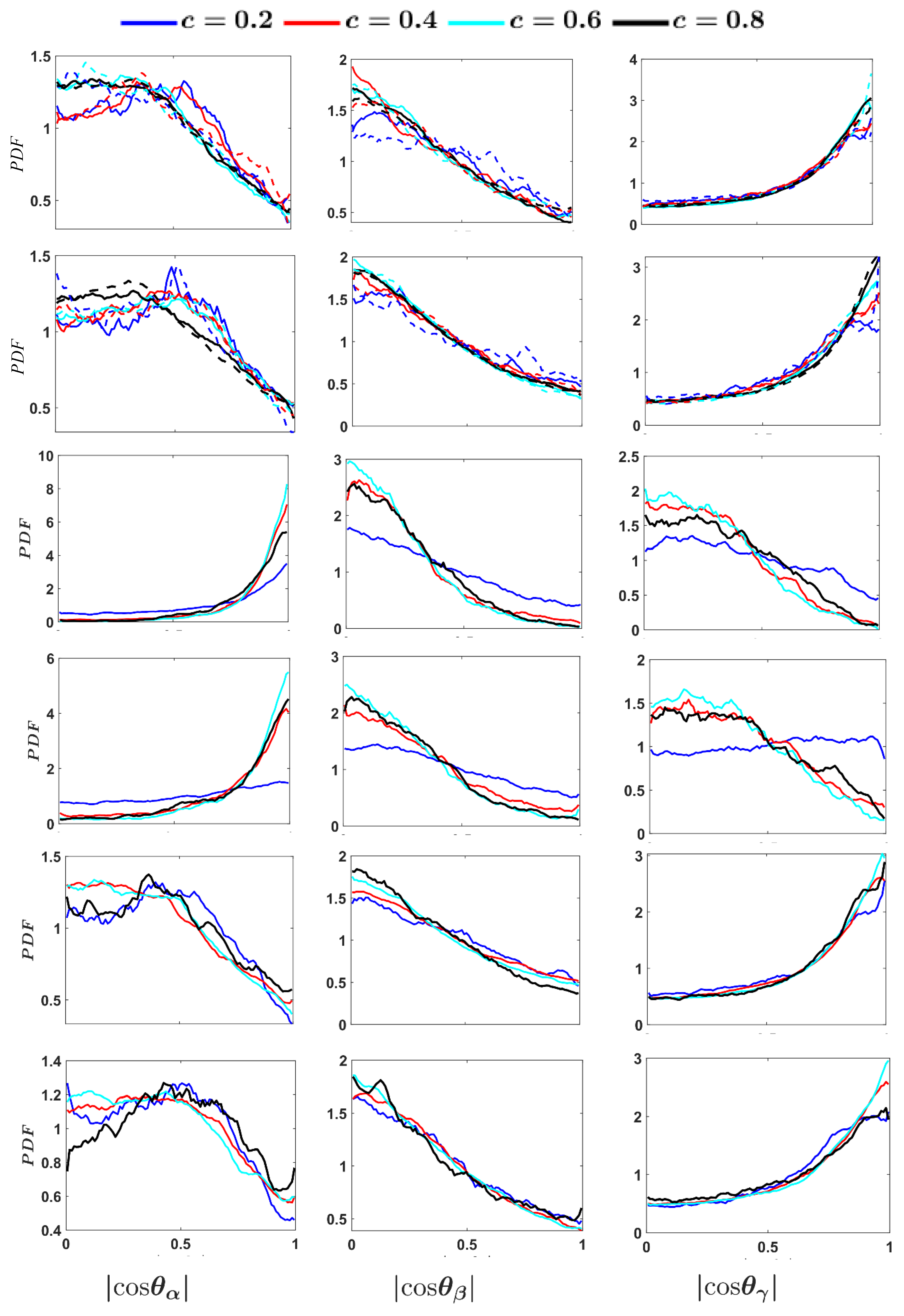
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fox, R.O. Computational Models of Turbulent Reacting Flows, 1st ed.; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Chakraborty, N.; Champion, M.; Mura, A.; Swaminathan, N. Scalar Dissipation Rate Approach to Reaction Rate Closure, Turbulent Premixed Flame, 1st ed.; Swaminathan, N., Bray, K.N.C., Eds.; Cambridge University Press: Cambridge, UK, 2011; pp. 74–102. [Google Scholar]
- Wang, G.; Clemens, N.; Varghese, P. High-repetition rate measurements of temperature and thermal dissipation in a nonpremixed turbulent jet flames. Proc. Combust. Inst. 2004, 30, 691–699. [Google Scholar] [CrossRef]
- Ihme, M.; See, Y.C. LES flamelet modeling of a three-stream MILD combustor: Analysis of flame sensitivity to scalar inflow conditions. Proc. Combust. Inst. 2011, 33, 1309–1317. [Google Scholar] [CrossRef]
- Langella, I.; Swaminathan, N.; Pitz, R.W. Application of unstrained flamelet SGS closure for multi-regime premixed combustion. Combust. Flame 2016, 173, 161–178. [Google Scholar] [CrossRef]
- Chen, Z.; Reddy, V.; Ruan, S.; Doan, N.A.K.; Roberts, W.; Swaminathan, S. Simulation of MILD combustion using Perfectly Stirred Reactor model. Proc. Combust. Inst. 2017, 36, 4279–4286. [Google Scholar] [CrossRef]
- Ferrarotti, M.; Li, Z.; Parente, A. On the role of mixing models in the simulation of MILD combustion using finite-rate chemistry combustion models. Proc. Combust. Inst. 2019, 37, 4531–4538. [Google Scholar] [CrossRef]
- Huang, X.; Tummers, M.J.; van Veen, E.H.; Roekaerts, D.J. Modelling of MILD combustion in a lab-scale furnace with an extended FGM model including turbulence–radiation interaction. Combust. Flame 2022, 237, 111634. [Google Scholar] [CrossRef]
- Cavaliere, A.; de Joannon, M. Mild Combustion. Prog. Energy Combust. Sci. 2004, 30, 329–366. [Google Scholar] [CrossRef]
- Awad, H.S.; Abo-Amsha, K.; Ahmed, U.; Chakraborty, N. Comparison of the reactive scalar gradient evolution between homogeneous MILD combustion and premixed turbulent flames. Energies 2021, 14, 7677. [Google Scholar] [CrossRef]
- Papapostolou, V.; Chakraborty, N.; Klein, M.; Im, H.G. Effects of reaction progress variable definition on the Flame Surface Density transport statistics and closure for different combustion regimes. Combust. Sci. Technol. 2019, 191, 1276–1293. [Google Scholar] [CrossRef]
- Chakraborty, N.; Wacks, D.H.; Ketterl, S.; Klein, M.; Im, H.G. Scalar dissipation rate transport conditional on flow topologies in different regimes of premixed turbulent combustion. Proc. Combust. Inst. 2019, 37, 2353–2361. [Google Scholar] [CrossRef]
- Cant, R.S. Technical Report CUED/A–THERMO/TR67; Cambridge University Engineering Department: Cambridge, UK, 2012. [Google Scholar]
- Poinsot, T.J.; Lele, S.K. Boundary conditions for direct simulations of compressible viscous flows. J. Comput. Phys. 1992, 101, 104–129. [Google Scholar] [CrossRef]
- Smooke, M.D.; Giovangigli, V. Premixed and Nonpremixed Test Flame Results, Reduced Kinetic Mechanisms and Asymptotic Approximations for Methane-Air Flames; Springer: Berlin/Heidelberg, Germany, 1991; pp. 29–47. [Google Scholar]
- Minamoto, Y.; Swaminathan, N.; Cant, R.S.; Leung, T. Reaction zones and their structure in MILD combustion. Combust. Sci. Technol. 2014, 186, 1075–1096. [Google Scholar] [CrossRef]
- Minamoto, Y.; Dunstan, T.; Swaminathan, N.; Cant, R. DNS of EGR-type turbulent flame in MILD condition. Proc. Combust. Inst. 2013, 34, 3231–3238. [Google Scholar] [CrossRef]
- Minamoto, Y.; Swaminathan, N.; Cant, S.R.; Leung, T. Morphological and statistical features of reaction zones in MILD and premixed combustion. Combust. Flame 2014, 161, 2801–2814. [Google Scholar] [CrossRef]
- Minamoto, Y.; Swaminathan, N. Scalar gradient behavior in MILD combustion. Combust. Flame 2014, 161, 1063–1075. [Google Scholar] [CrossRef]
- Minamoto, Y.; Swaminathan, N. Subgrid scale modelling for MILD combustion. Proc. Combust. Inst. 2015, 35, 3529–3536. [Google Scholar] [CrossRef]
- Peters, N. Turbulent Combustion, Cambridge Monograph on Mechanics; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Rogallo, R.S. Numerical Experiments in Homogeneous Turbulence, NASA Technical Memorandum 81315; NASA Ames Research Center: Mountain View, CA, USA, 1981.
- Batchelor, G.K.; Townsend, A.A. Decay of turbulence in the final period. Proc. Royal Soc. London A 1948, 194, 527–543. [Google Scholar]
- Eswaran, V.; Pope, S.B. Direct numerical simulations of the turbulent mixing of a passive scalar. Phys. Fluids 1988, 31, 506–520. [Google Scholar] [CrossRef]
- Doan, N.A.K.; Swaminathan, N.; Minamoto, Y. DNS of MILD combustion with mixture fraction variations. Combust. Flame 2018, 189, 173–189. [Google Scholar] [CrossRef]
- Doan, N.; Swaminathan, N. Role of radicals on MILD combustion inception. Proc. Combust. Inst. 2019, 37, 4539–4546. [Google Scholar] [CrossRef]
- Doan, N.; Swaminathan, N. Analysis of markers for combustion mode and heat release in MILD combustion using DNS data, Combust. Sci. Technol. 2019, 191, 1059–1078. [Google Scholar]
- Swaminathan, N. Physical insights on MILD combustion from DNS. Front. Mech. Eng. 2019, 5, 549. [Google Scholar] [CrossRef]
- Boger, M.; Veynante, D.; Boughanem, H.; Trouvé, A. Direct Numerical Simulation analysis of flame surface density concept for Large Eddy Simulation of turbulent premixed combustion. Proc. Comb. Inst. 1998, 27, 917–925. [Google Scholar] [CrossRef]
- Kollman, W.; Chen, J. Pocket formation and the flame surface density equation. Proc. Combust. Inst. 1998, 27, 927–934. [Google Scholar] [CrossRef]
- Peters, N.; Terhoeven, P.; Chen, J.H.; Echekki, T. Statistics of Flame Displacement Speeds from Computations of 2-D Unsteady Methane-Air Flames. Proc. Combust. Inst. 1998, 27, 833–839. [Google Scholar] [CrossRef]
- Echekki, T.; Chen, J.H. Analysis of the contribution of curvature to premixed flame propagation. Combust. Flame 1999, 118, 308–311. [Google Scholar] [CrossRef]
- Chakraborty, N.; Swaminathan, N. Influence of the Damköhler number on turbulence-scalar interaction in premixed flames. I. Physical insight. Phys. Fluids 2007, 19, 045103. [Google Scholar] [CrossRef]
- Chakraborty, N.; Klein, M.; Swaminathan, N. Effects of Lewis number on reactive scalar gradient alignment with local strain rate in turbulent premixed flames. Proc. Combust. Inst. 2009, 32, 1409–1417. [Google Scholar] [CrossRef]
- Malkeson, S.P.; Chakraborty, N. Alignment statistics of active and passive scalar gradients in turbulent stratified flames. Phys. Rev. E 2011, 86, 046308. [Google Scholar] [CrossRef]
- Sandeep, A.; Proch, F.; Kempf, A.M.; Chakraborty, N. Chakraborty, Statistics of strain rates and Surface Density Function in a flame-resolved high-fidelity simulation of a turbulent premixed bluff body burner. Phys. Fluids 2018, 30, 065101. [Google Scholar] [CrossRef]
- Dopazo, C.; Cifuentes, L.; Alwazzan, D.; Chakraborty, N. Influence of the Lewis number on effective strain rates in weakly turbulent premixed combustion. Combust. Sci. Technol. 2018, 190, 591–614. [Google Scholar] [CrossRef]
- Chakraborty, N.; Klein, M.; Alwazzan, D.; Im, H.G. Surface Density Function statistics in Hydrogen-air flames for different turbulent premixed combustion regimes. Combust. Sci. Technol. 2018, 190, 1988–2002. [Google Scholar] [CrossRef]
- Kolla, H.; Rogerson, J.W.; Chakraborty, N.; Swaminathan, N. Scalar dissipation rate modelling and its validation. Combust. Sci. Technol. 2009, 181, 518–535. [Google Scholar] [CrossRef]
- Dunstan, T.; Minamoto, Y.; Chakraborty, N.; Swaminathan, N. Scalar dissipation rate modelling for Large Eddy Simulation of turbulent premixed flames. Proc. Combust. Inst. 2013, 34, 1193–1201. [Google Scholar] [CrossRef]
- Gao, Y.; Chakraborty, N.; Swaminathan, N. Algebraic closure of scalar dissipation rate for Large Eddy Simulations of turbulent premixed combustion. Combust. Sci. Technol. 2014, 186, 1309–1337. [Google Scholar] [CrossRef]
- Gao, Y.; Chakraborty, N.; Swaminathan, N. Dynamic scalar dissipation rate closure for Large Eddy Simulations of turbulent premixed combustion: A Direct Numerical Simulations analysis. Flow Turb. Combust. 2015, 95, 775–802. [Google Scholar] [CrossRef]
- Kolla, H.; Swaminathan, N. Strained flamelets for turbulent premixed flames II: Laboratory flame results. Combust. Flame 2010, 157, 1274–1289. [Google Scholar] [CrossRef]
- Sadasivuni, S.; Bulat, G.; Senderson, V.; Swaminathan, N. Application of Scalar Dissipation Rate Model to Siemens DLE Combustors, Paper no. GT2012-68483. In Proceedings of the ASME TURBO EXPO 2012: Power for Land, Sea and Air GT2012, Bella Center, Copenhagen, Denmark, 11–15 June 2012. [Google Scholar]
- Ahmed, I.; Swaminathan, N. Simulation of spherically expanding turbulent premixed flames. Combust. Sci. Technol. 2013, 185, 1509–1540. [Google Scholar] [CrossRef]
- Chen, Z.; Ruan, S.; Swaminathan, N. Simulation of turbulent lifted methane jet flames: Effects of air-dilution and transient flame propagation. Combust. Flame 2015, 162, 703–716. [Google Scholar] [CrossRef]
- Ma, T.; Gao, Y.; Kempf, A.M.; Chakraborty, N. Validation and Implementation of algebraic LES modelling of Scalar Dissipation Rate for reaction rate closure in turbulent premixed combustion. Combust. Flame 2014, 161, 3134–3153. [Google Scholar] [CrossRef]
- Butz, D.; Gao, Y.; Kempf, A.M.; Chakraborty, N. Large Eddy Simulations of a turbulent premixed swirl flame using an algebraic Scalar Dissipation Rate closure. Combust. Flame 2015, 162, 3180–3196. [Google Scholar] [CrossRef]
- Langella, I.; Swaminathan, N.; Gao, Y.; Chakraborty, N. Assessment of dynamic closure for premixed combustion LES. Combust. Theory Model. 2015, 19, 628–656. [Google Scholar] [CrossRef]
- Langella, I.; Swaminathan, N.; Gao, Y.; Chakraborty, N. LES of premixed combustion using an algebraic closure involving scalar dissipation rate. Combust. Sci. Technol. 2017, 189, 43–77. [Google Scholar] [CrossRef]
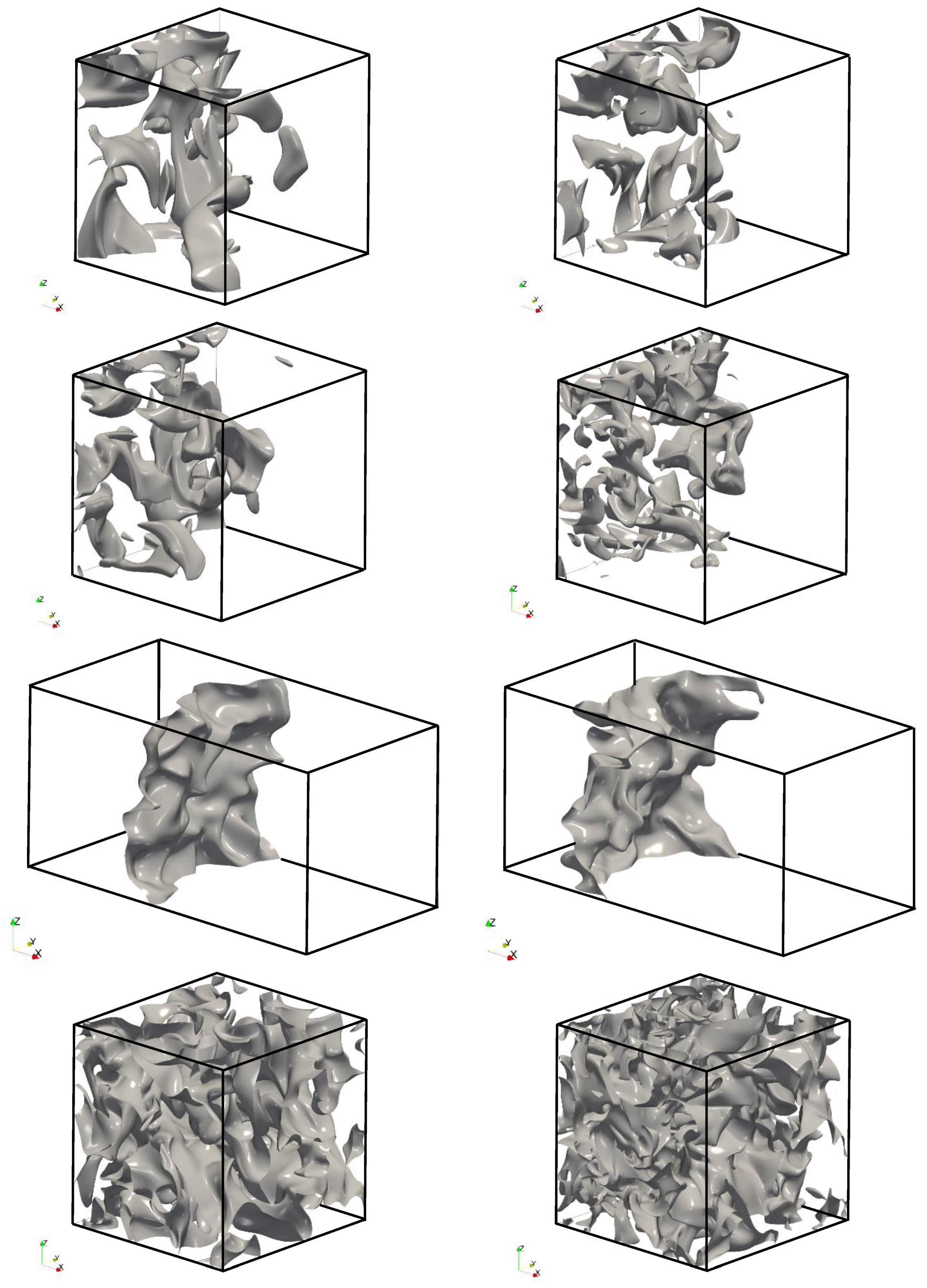
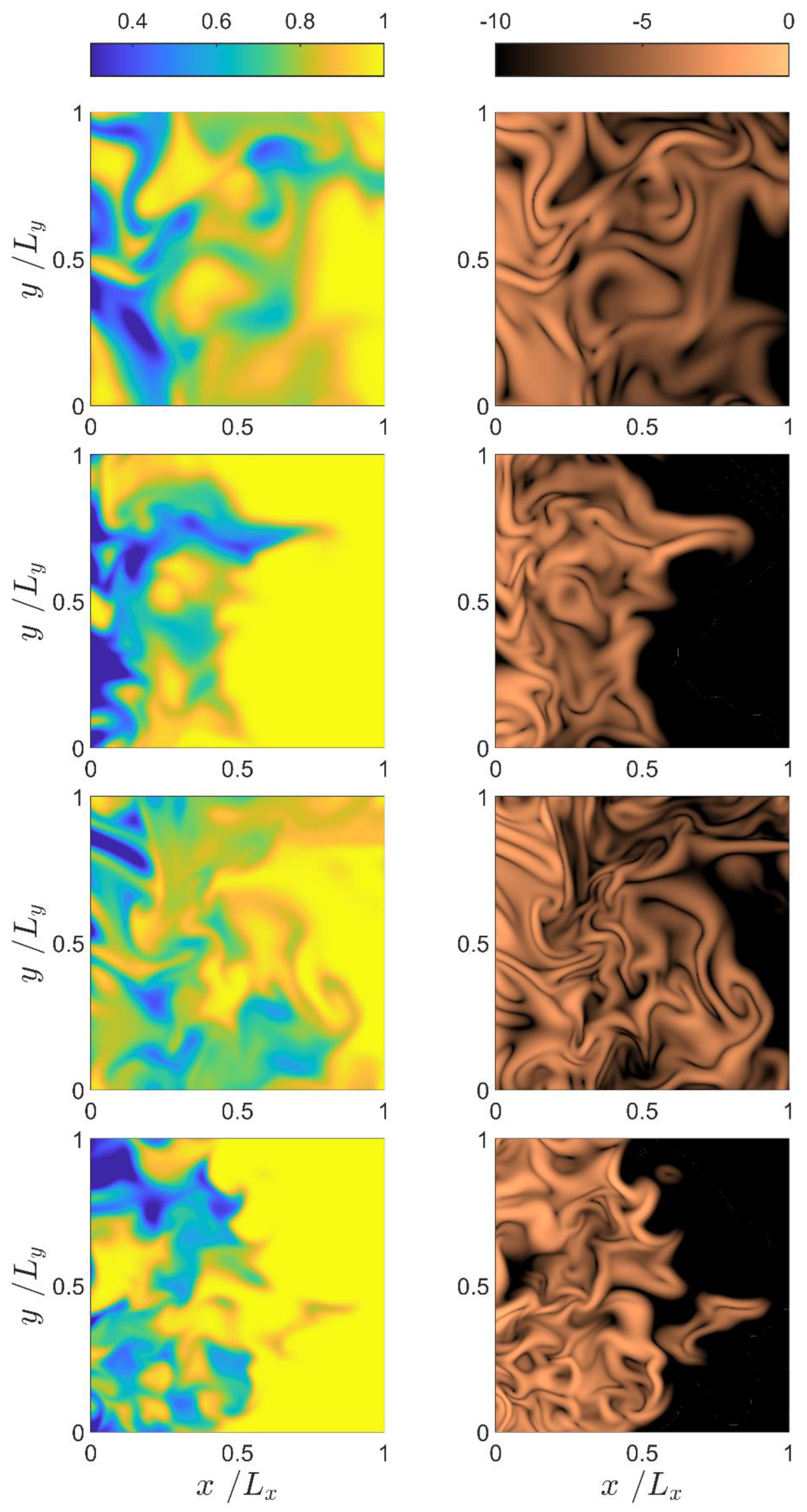
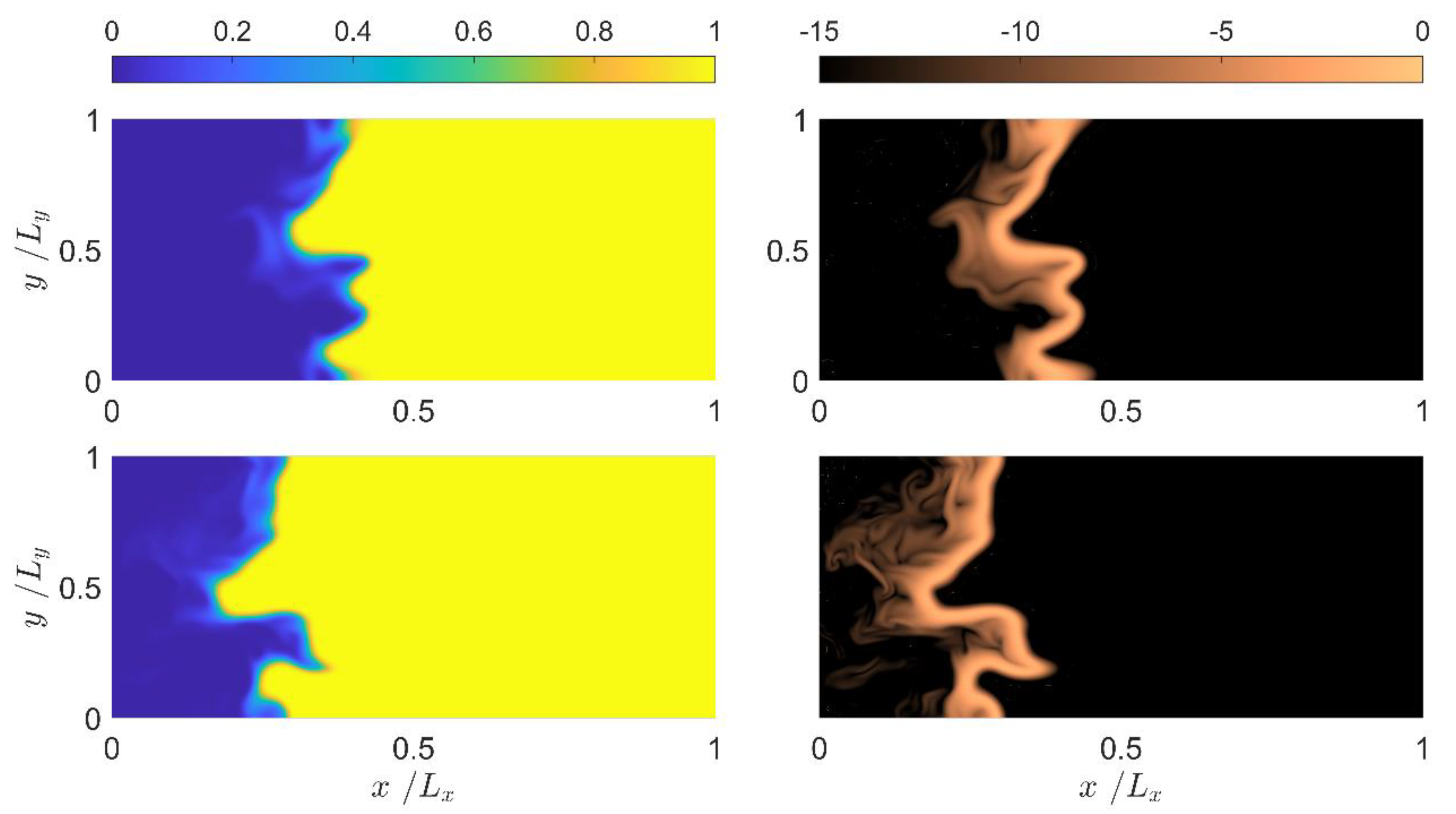
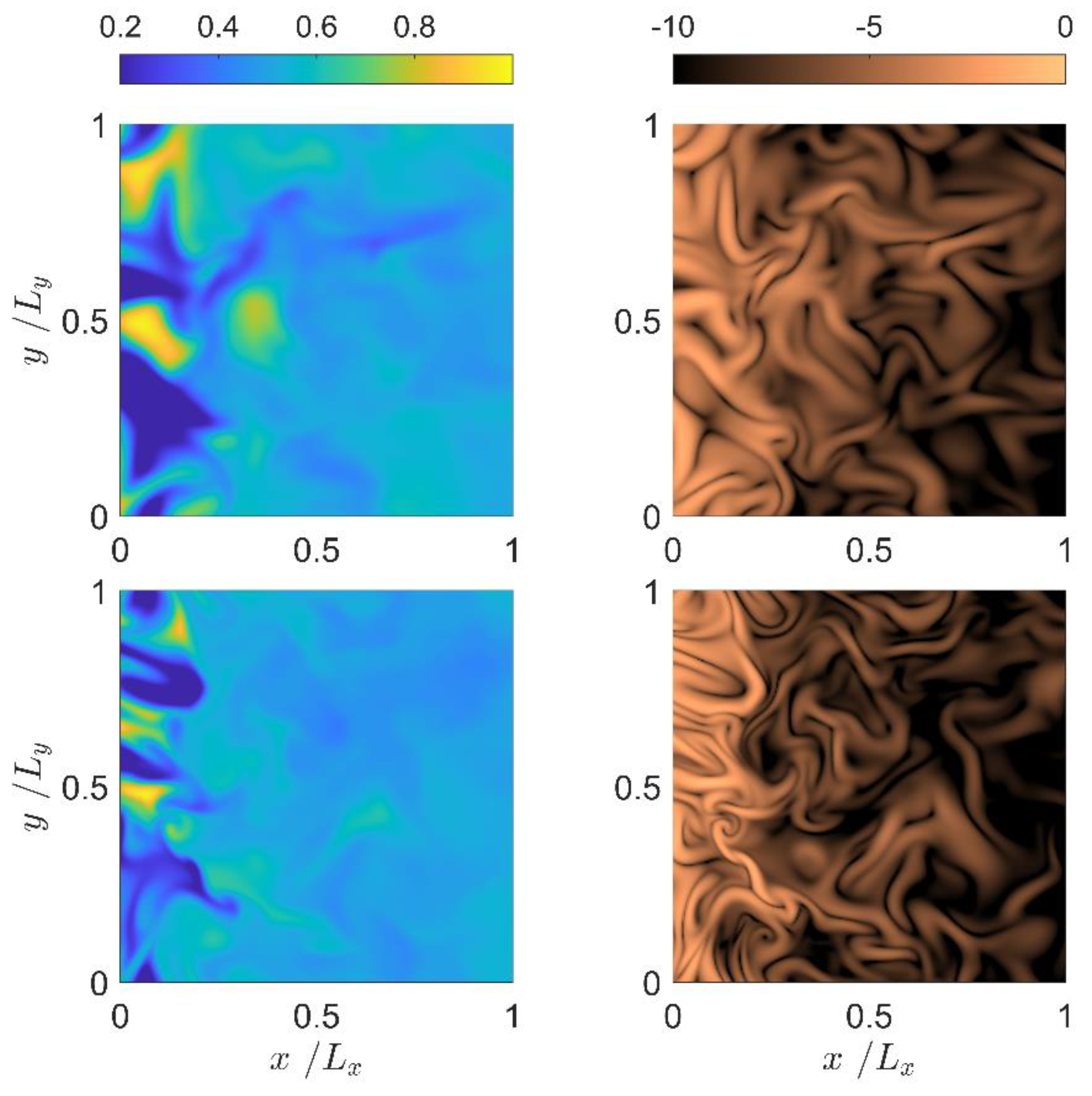


| Case | ||||||||
|---|---|---|---|---|---|---|---|---|
| MILD–3.5% O2 | 0.035 | 0.066 | 0.132 | 0.014 | 0.753 | 2.3 | 1500 | 0.8 |
| MILD–4.8% O2 | 0.048 | 0.061 | 0.121 | 0.019 | 0.751 | 3.2 | 1500 | 0.6 |
| Premixed | 0.194 | 0.0 | 0.0 | 0.077 | 0.729 | 0.3 | 300 | 0.5 |
| Case | ||||
|---|---|---|---|---|
| MILD–3.5% O2 | 4.0 | 2.5 | 0.62 | 5.06 |
| 8.0 | 2.5 | 0.31 | 14.31 | |
| MILD–4.8% O2 | 4.0 | 2.5 | 0.62 | 5.06 |
| 8.0 | 2.5 | 0.31 | 14.31 | |
| Premixed | 4.0 | 2.5 | 0.62 | 5.06 |
| 8.0 | 2.5 | 0.31 | 14.31 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Young, F.W.; Awad, H.S.A.M.; Abo-Amsha, K.; Ahmed, U.; Chakraborty, N. A Comparison between Statistical Behaviours of Scalar Dissipation Rate between Homogeneous MILD Combustion and Premixed Turbulent Flames. Energies 2022, 15, 9188. https://doi.org/10.3390/en15239188
Young FW, Awad HSAM, Abo-Amsha K, Ahmed U, Chakraborty N. A Comparison between Statistical Behaviours of Scalar Dissipation Rate between Homogeneous MILD Combustion and Premixed Turbulent Flames. Energies. 2022; 15(23):9188. https://doi.org/10.3390/en15239188
Chicago/Turabian StyleYoung, Frederick W., Hazem S. A. M. Awad, Khalil Abo-Amsha, Umair Ahmed, and Nilanjan Chakraborty. 2022. "A Comparison between Statistical Behaviours of Scalar Dissipation Rate between Homogeneous MILD Combustion and Premixed Turbulent Flames" Energies 15, no. 23: 9188. https://doi.org/10.3390/en15239188
APA StyleYoung, F. W., Awad, H. S. A. M., Abo-Amsha, K., Ahmed, U., & Chakraborty, N. (2022). A Comparison between Statistical Behaviours of Scalar Dissipation Rate between Homogeneous MILD Combustion and Premixed Turbulent Flames. Energies, 15(23), 9188. https://doi.org/10.3390/en15239188








