Effectiveness of the Chebyshev Approximation in Magnetic Field Line Tracking
Abstract
1. Introduction
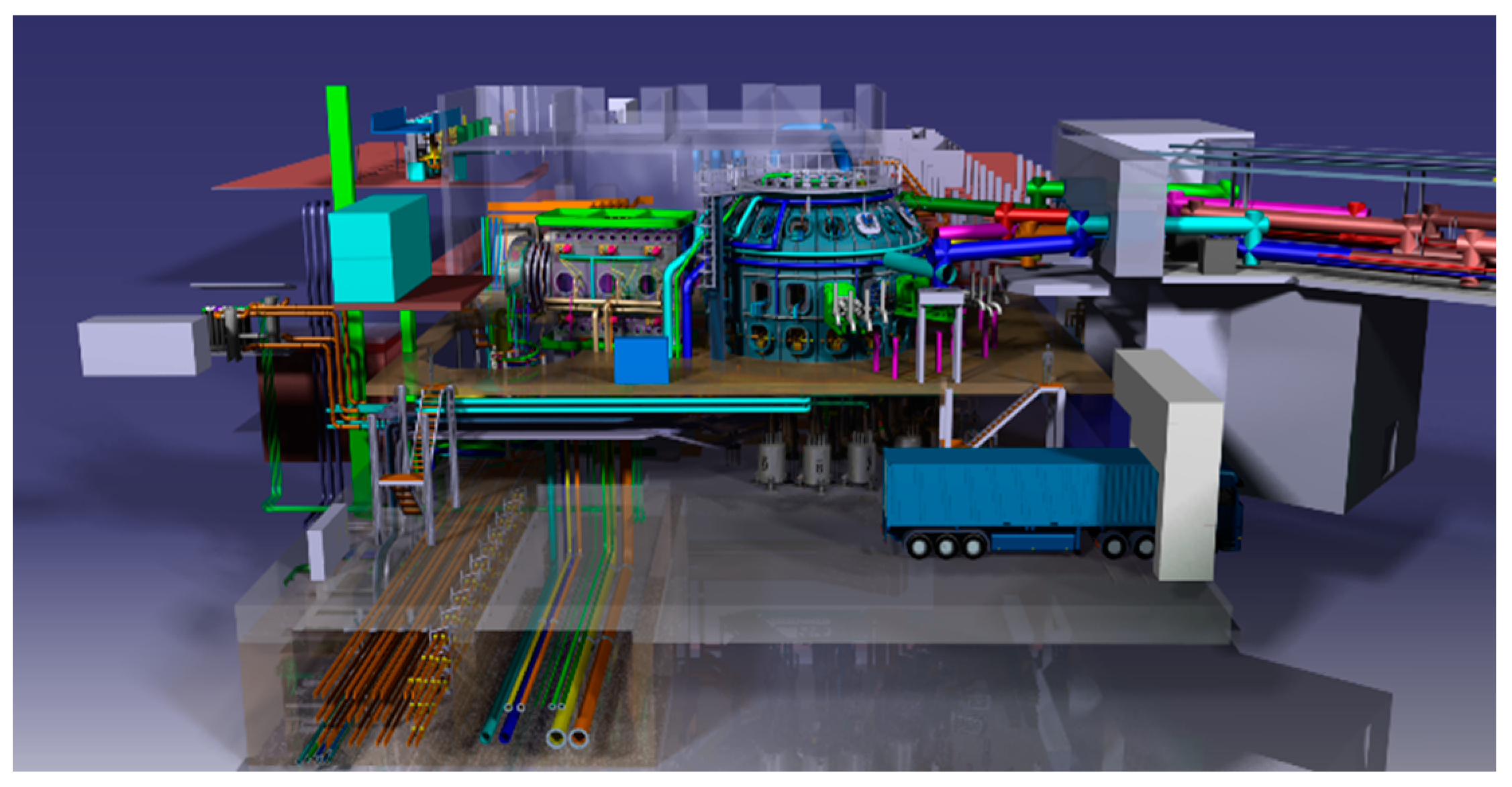
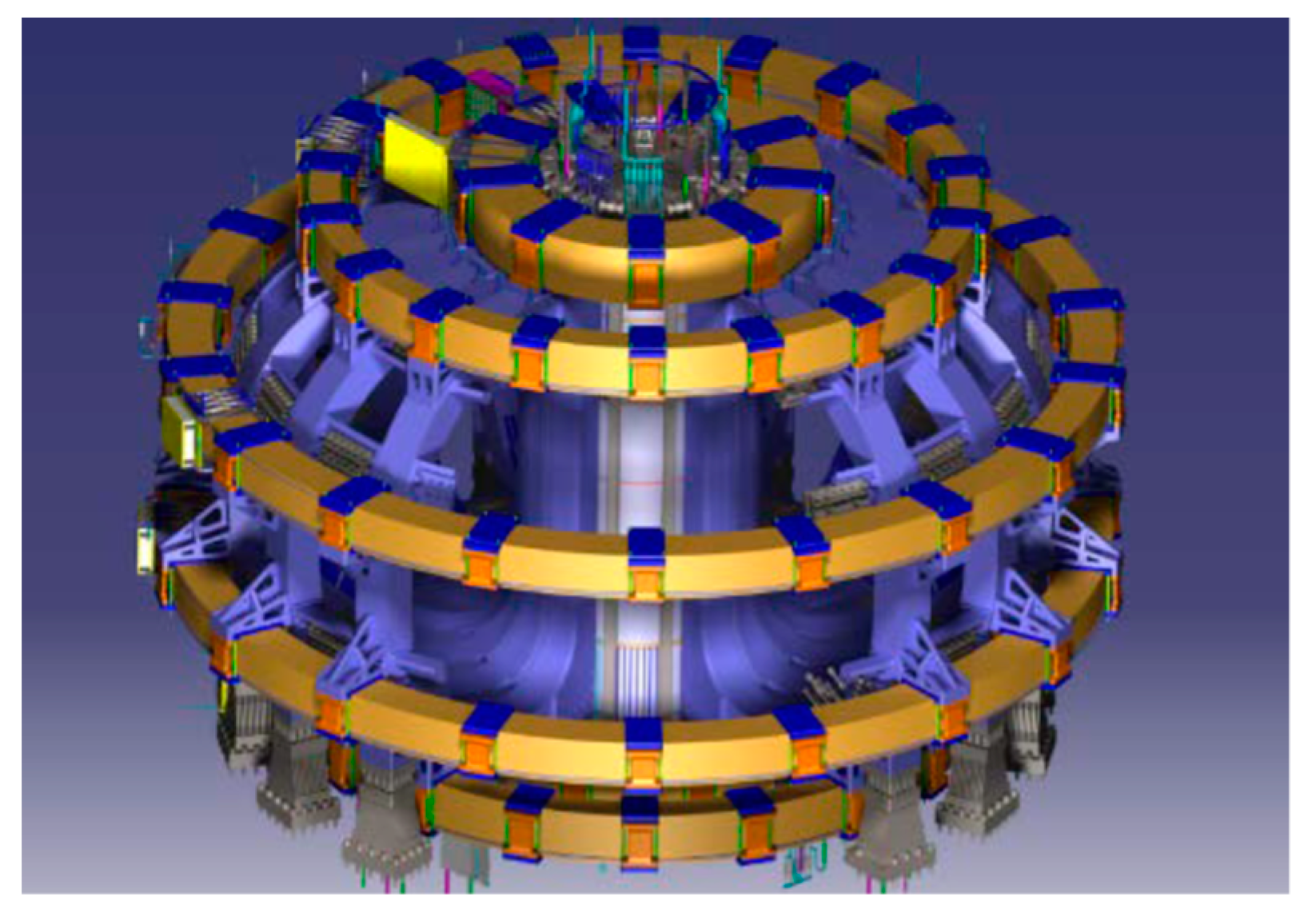
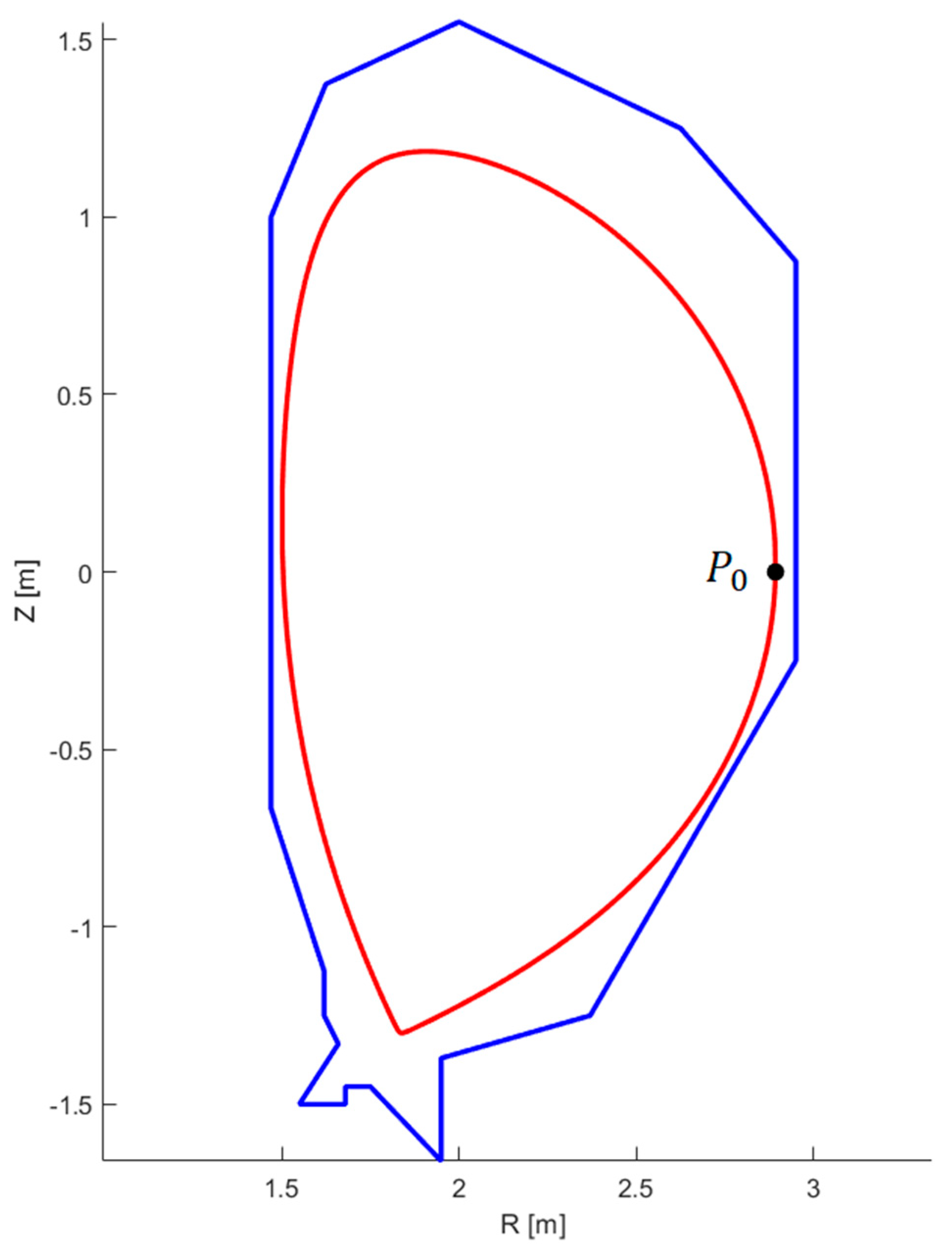
2. The DTT Tokamak
3. Mathematical Formulation
3.1. FL Tracking
3.2. The Field Approximation
4. Results
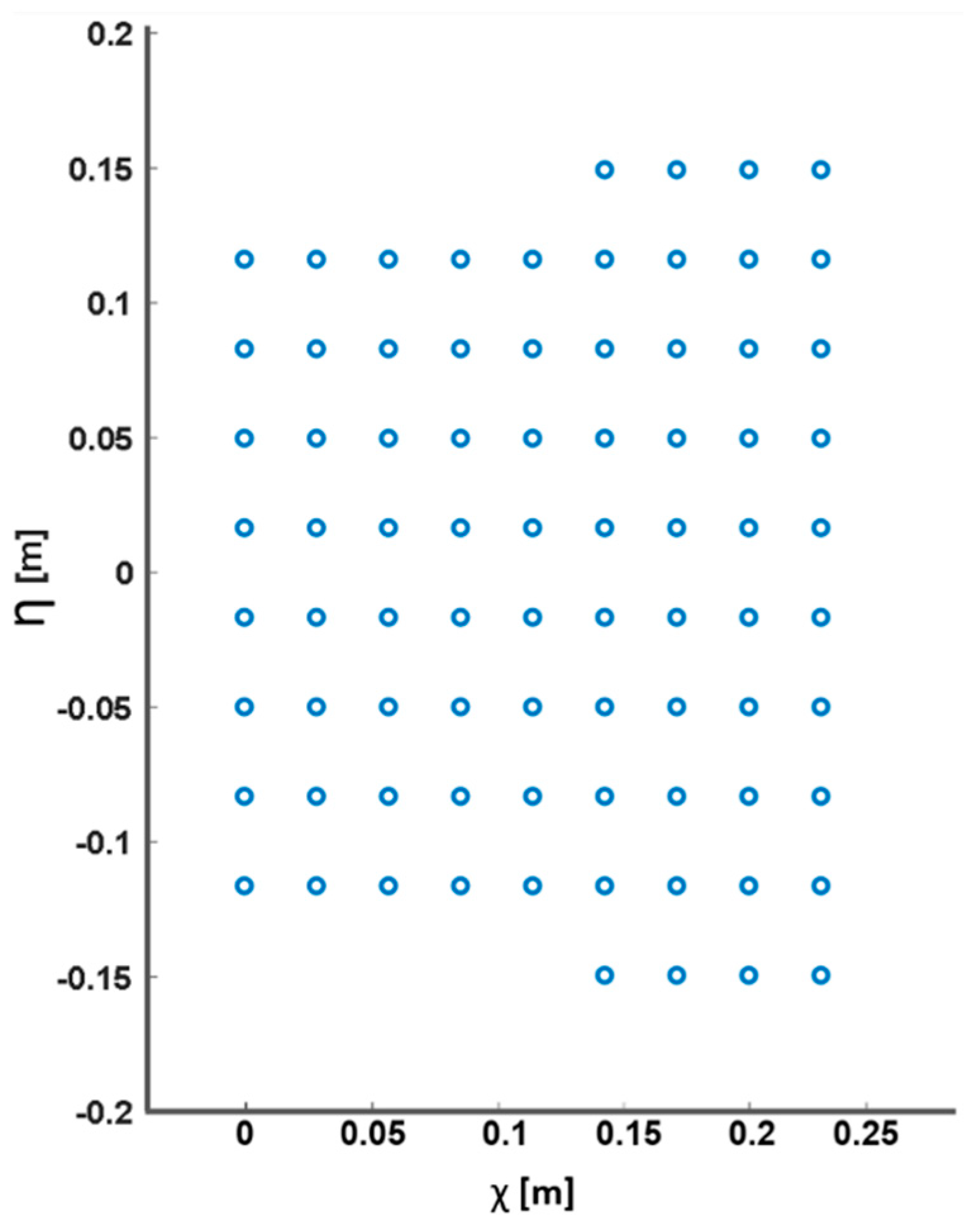
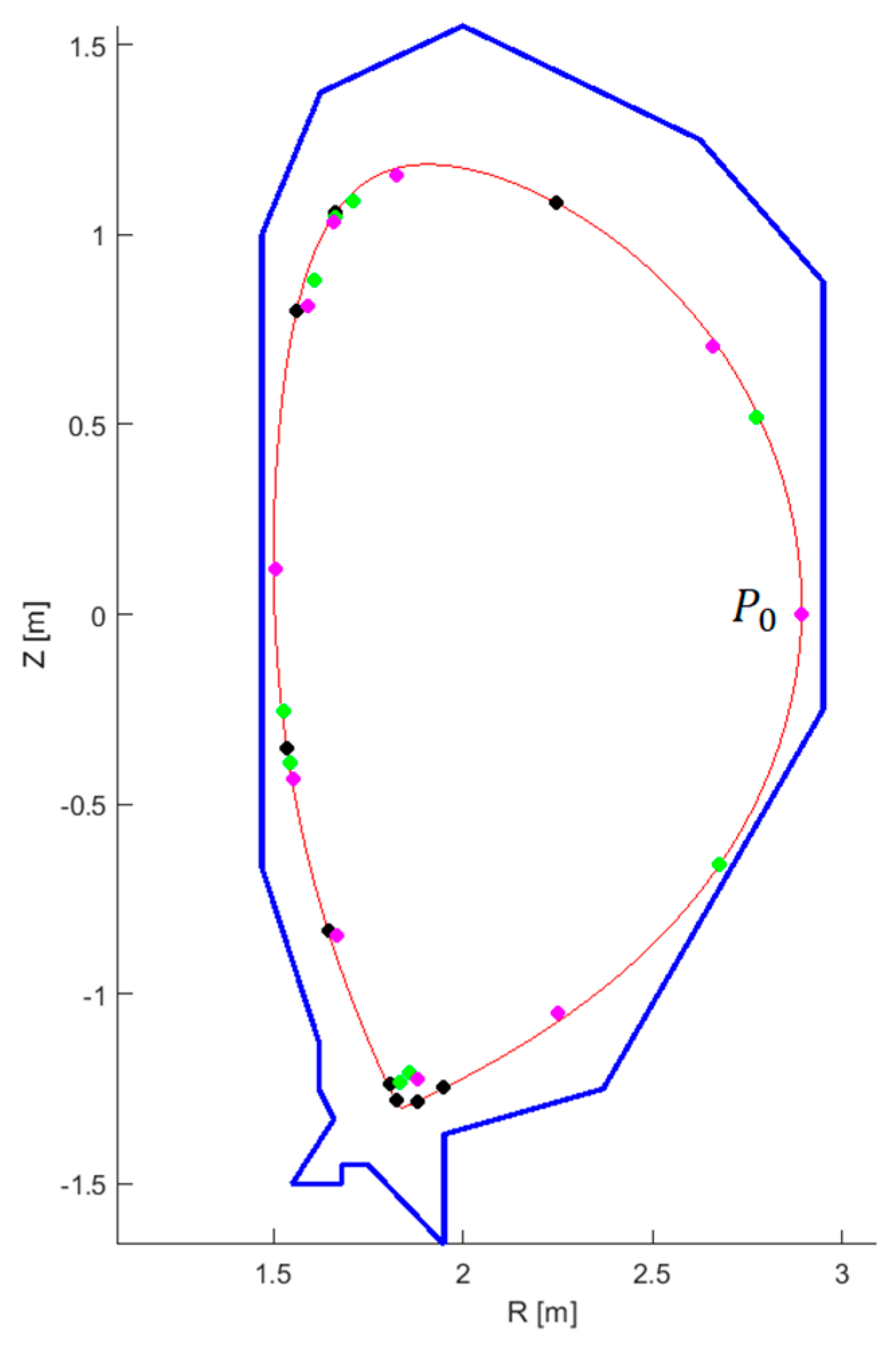
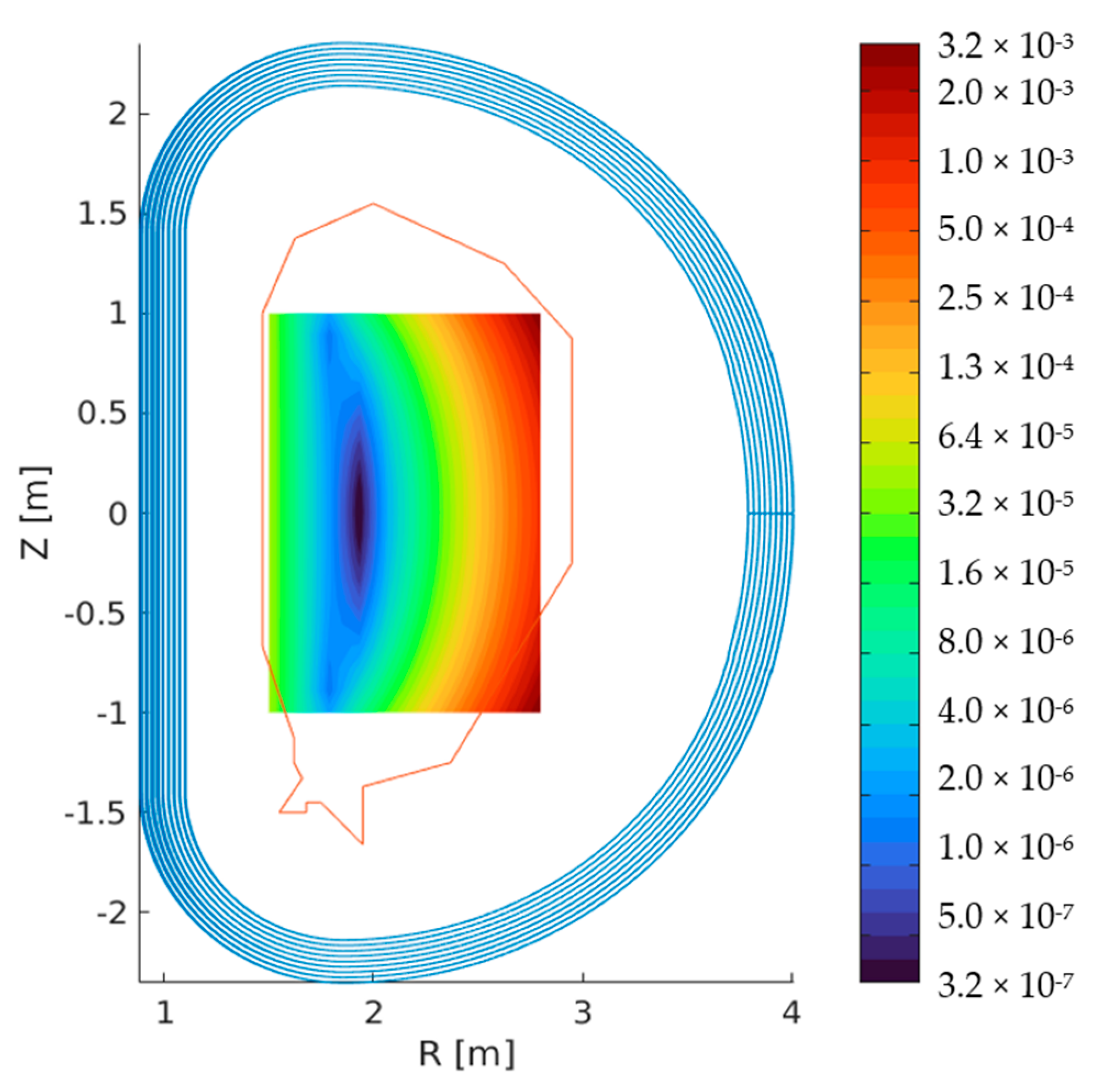
4.1. Accuracy Assessment: Magnetic Field Reconstruction Inside a Domain
4.2. Performance of the CP Approach in Magnetic FL Tracking
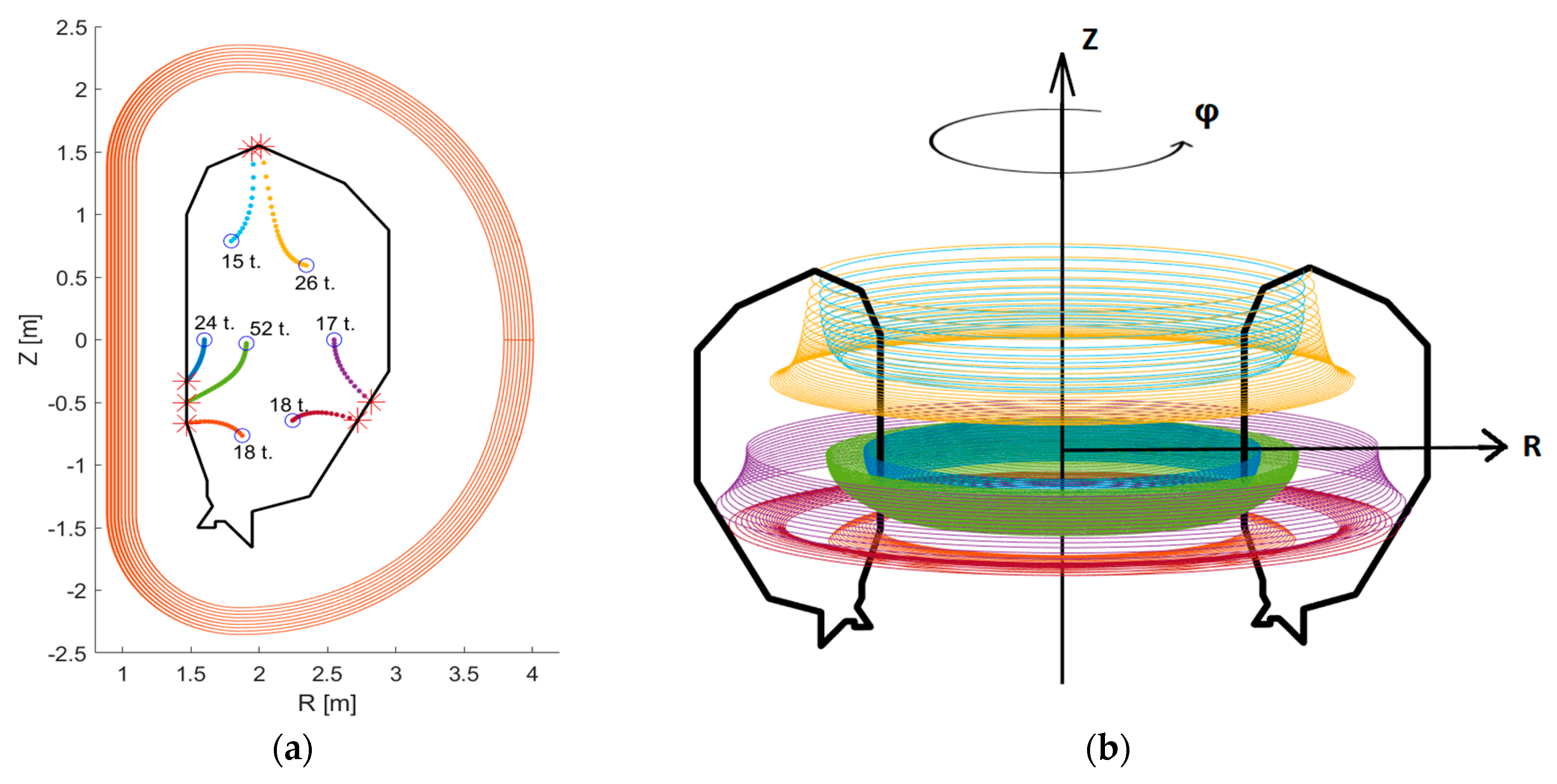
4.3. Applicative Examples of Magnetic Field Lines Tracking
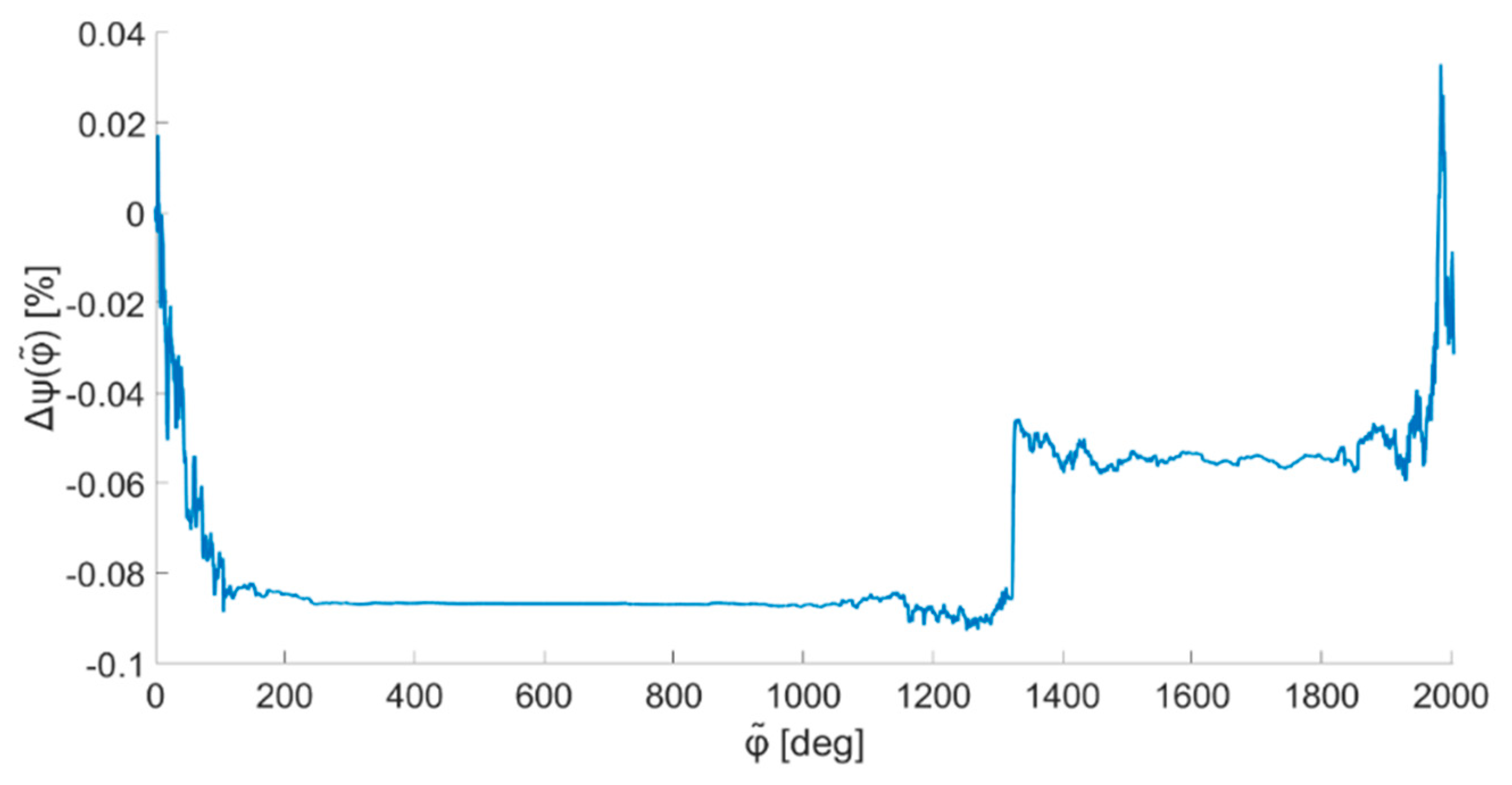
4.4. Treatment of Periodicity
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- McLachlan, R.I.; Reinout, G.; Quispel, G.R.W. Geometric Integrators for ODEs. J. Phys. A Math. Gen. 2006, 39, 5251. [Google Scholar] [CrossRef]
- Sanz-Serna, J.M. Symplectic Runge-Kutta schemes for adjoint equations, automatic differentiation, optimal control, and more. SIAM Rev. 2016, 58, 3–33. [Google Scholar] [CrossRef]
- Finn, J.M.; Chacón, L. Volume preserving integrators for solenoidal fields on a grid. Phys. Plasmas 2005, 12, 054503. [Google Scholar] [CrossRef]
- Blackwell, B.D.; McMillan, B.F.; Searle, A.C.; Gardner, H.J. Algorithms for real time magnetic field tracing and optimization. Comput. Phys. Commun. 2001, 142, 243–247. [Google Scholar] [CrossRef]
- Albanese, R.; De Magistris, M.; Fresa, R.; Maviglia, F.; Minucci, S. Accuracy assessment of numerical tracing of three-dimensional magnetic field lines in tokamaks with analytical invariants. Fusion Sci. Technol. 2015, 68, 741–749. [Google Scholar] [CrossRef]
- Chiariello, A.G.; Formisano, A.; Ledda, F.; Martone, R.; Pizzo, F. Effective tracing of magnetic field lines. In Proceedings of the IGTE Symposium on Numerical Field Calculation, Graz, Austria, 14–17 September 2014; pp. 237–242. [Google Scholar]
- Trefethen, L.N. Approximation Theory and Approximation Practice; SIAM: Philadelphia, PA, USA, 2019. [Google Scholar]
- Wesson, J.; Campbell, D.J. Tokamaks; Oxford University Press: Oxford, UK, 2011; Volume 149. [Google Scholar]
- Martone, R.; Albanese, R.; Crisanti, F.; Martin, P.; Pizzuto, A. DTT Divertor Tokamak Test Facility Interim Design Report; ENEA April 2019 (“Green Book”); ISBN 978-88-8286-378-4. Available online: https://www.dtt-dms.enea.it/share/s/avvglhVQT2aSkSgV9vuEtw (accessed on 15 June 2022).
- Portone, A.; Roccella, M.; Roccella, R.; Lucca, F.; Ramogida, G. The ITER TF coil ripple: Evaluation of ripple attenuation using Fe insert and of ripple enhancement produced by TBM. Fusion Eng. Des. 2008, 83, 1619–1624. [Google Scholar] [CrossRef]
- Butcher, J.C. Numerical Methods for Ordinary Differential Equations; John Wiley: Hoboken, NJ, USA, 2003. [Google Scholar]
- Kang, F.; Shang, Z.J. Volume-preserving algorithms for source-free dynamical systems. Numer. Math. 1995, 71, 451–463. [Google Scholar] [CrossRef]
- Hairer, E.; Lubich, C.; Wanner, G. Geometric Numerical Integration; Springer Series in Computational Mathematics; Springer: Berlin/Heidelberg, Germany, 2010; Volume 31. [Google Scholar]
- Chiariello, A.G.; Formisano, A.; Ledda, F.; Martone, R.; Pizzo, F. Effectiveness in 3-D magnetic field evaluation of complex magnets. IEEE Trans. Magn. 2015, 51, 7093459. [Google Scholar] [CrossRef]
- Chiariello, A.G.; Formisano, A.; Martone, R. Fast magnetic field computation in fusion technology using GPU technology. Fusion Eng. Des. 2013, 88, 1635–1639. [Google Scholar] [CrossRef]
- Chiariello, A.G.; Mastrostefano, S.; Nicolazzo, M.; Rubinacci, G.; Tamburrino, A.; Ventre, S.; Villone, F. Speedup of Magnetic-Electric Matrices Assembly Computation by Means of a Multi-GPUs Environment. IEEE Trans. Magn. 2016, 52, 7209204. [Google Scholar] [CrossRef]
- Ventre, S.; Cau, F.; Chiariello, A.; Giovinco, G.; Maffucci, A.; Villone, F. Fast and Accurate Solution of Integral Formulations of Large MQS Problems Based on Hybrid OpenMP–MPI Parallelization. Appl. Sci. 2022, 12, 627. [Google Scholar] [CrossRef]
- Cau, F.; Chiariello, A.G.; Rubinacci, G.; Scalera, V.; Tamburrino, A.; Ventre, S.; Villone, F. A Fast Matrix Compression Method for Large Scale Numerical Modelling of Rotationally Symmetric 3D Passive Structures in Fusion Devices. Energies 2022, 15, 3214. [Google Scholar] [CrossRef]
- Kalling, R.C.; Evans, T.E.; Orlov, D.M.; Schissel, D.P.; Maingi, R.; Menard, J.E.; Sabbagh, S.A. Accelerating the numerical simulation of magnetic field lines in tokamaks using the GPU. Fusion Eng. Des. 2011, 86, 399–406. [Google Scholar] [CrossRef]
- Abate, D.; Carpentieri, B.; Chiariello, A.G.; Marchiori, G.; Marconato, N.; Mastrostefano, S.; Rubinacci, G.; Ventre, S.; Villone, F. Fast and parallel computational techniques applied to numerical modeling of RFX-mod fusion device. Appl. Comput. Electromagn. Soc. J. 2018, 33, 176–179. [Google Scholar]
- Castaldo, A.; Albanese, R.; Ambrosino, R.; Crisanti, F. Plasma Scenarios for the DTT Tokamak with Optimized Poloidal Field Coil Current Waveforms. Energies 2022, 15, 1702. [Google Scholar] [CrossRef]
- Oliva, A.B.; Chiariello, A.G.; Formisano, A.; Martone, R.; Portone, A.; Testoni, P. Estimation of error fields from ferromagnetic parts in ITER. Fusion Eng. Des. 2013, 88, 1576–1580. [Google Scholar] [CrossRef]
- Knaster, J.; Amoskov, V.; Formisano, A.; Gribov, Y.; Lamzin, E.; Martone, L.; Maximenkova, N.; Mitchell, N.; Portone, A.; Sytchevsky, S.; et al. ITER non-axisymmetric error fields induced by its magnet system. Fusion Eng. Des. 2011, 86, 1053–1056. [Google Scholar] [CrossRef]
- Chiariello, A.G.; Formisano, A.; Martone, R.; Arshad, S.; Vayakis, G. Error Field in Tokamaks: A Plasmaless Measurement Approach. In Proceedings of the 1st EPS conference on Plasma Diagnostics, ECPD2015, Villa Mondragone, Frascati, Italy, 14–17 April 2015. [Google Scholar]
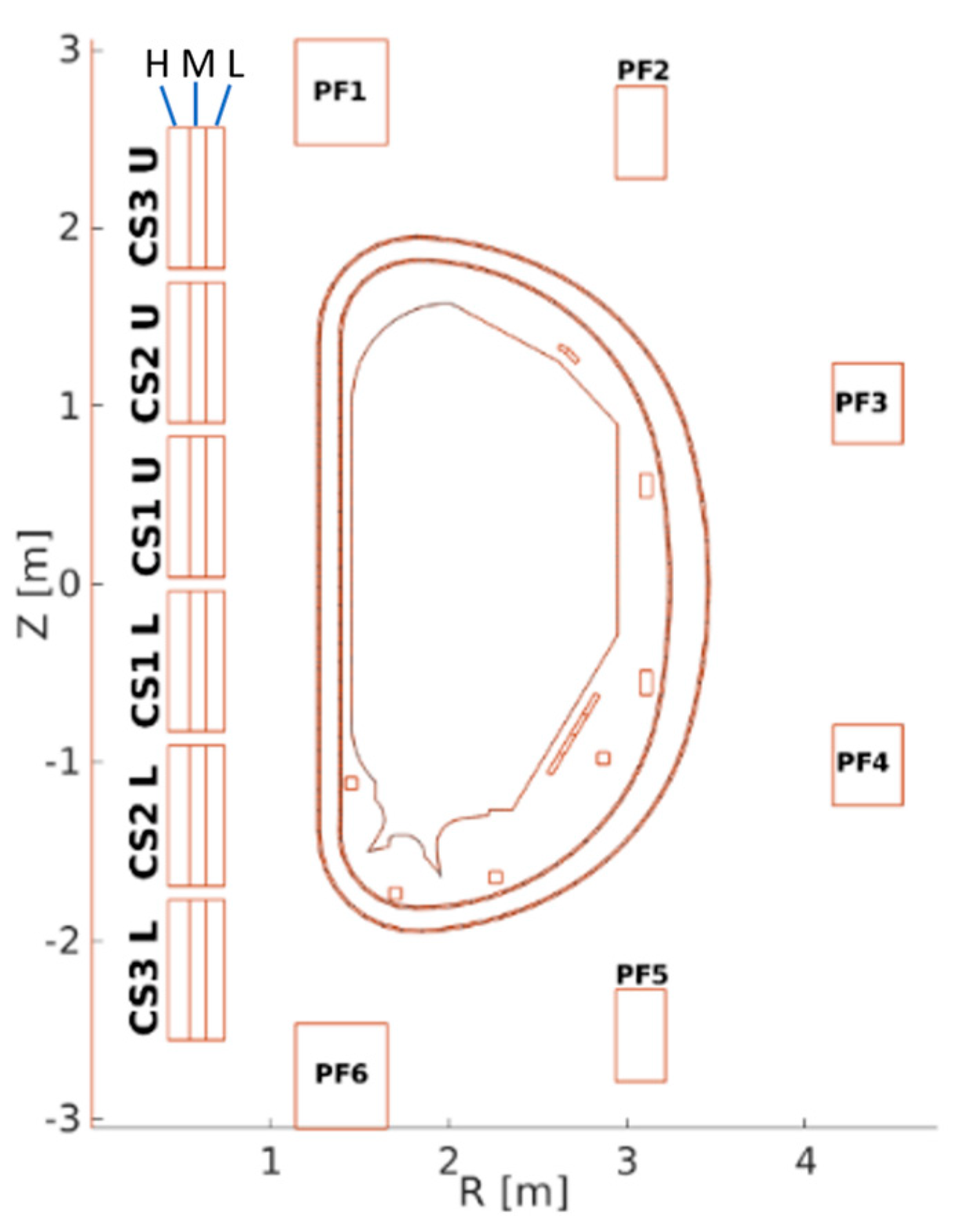
| Coil Name | Center R (m) | Center Z (m) | DR (m) | DZ (m) | nR | nZ |
|---|---|---|---|---|---|---|
| PF1 | 1.4000 | 2.7600 | 0.5100 | 0.5904 | 18 | 20 |
| PF2 | 3.0795 | 2.5340 | 0.2790 | 0.5168 | 10 | 16 |
| PF3 | 4.3511 | 1.0150 | 0.3898 | 0.4522 | 14 | 14 |
| CS3U-H | 0.4896 | 2.1658 | 0.1213 | 0.7880 | 4 | 17 |
| CS3U-M | 0.5960 | 2.1658 | 0.0915 | 0.7880 | 4 | 20 |
| CS3U-L | 0.6935 | 2.1658 | 0.1035 | 0.7880 | 6 | 24 |
| CS2U-H | 0.4896 | 1.2994 | 0.1213 | 0.7880 | 4 | 17 |
| CS2U-M | 0.5960 | 1.2994 | 0.0915 | 0.7880 | 4 | 20 |
| CS2U-L | 0.6935 | 1.2994 | 0.1035 | 0.7880 | 6 | 24 |
| CS1U-H | 0.4896 | 0.4331 | 0.1213 | 0.7880 | 4 | 17 |
| CS1U-M | 0.5960 | 0.4331 | 0.0915 | 0.7880 | 4 | 20 |
| CS1U-L | 0.6935 | 0.4331 | 0.1035 | 0.7880 | 6 | 24 |
| 3 | 4 | 5 | 6 | |
|---|---|---|---|---|
| 2 | 1.44 × 10−6 | 9.10 × 10−7 | 7.90 × 10−7 | 6.67 × 10−7 |
| 3 | / | 1.74 × 10−8 | 1.52 × 10−8 | 1.32 × 10−8 |
| 4 | / | / | 6.42 × 10−11 | 5.74 × 10−11 |
| 5 | / | / | / | 2.21 × 10−12 |
| Tracking Time (s) without GPU | Tracking Time (s) with GPU | |||
|---|---|---|---|---|
| 3 | 297 | 13.2 | 0.1 × 103 | 7.1 |
| 4 | 704 | 5.6 | 0.3 × 103 | 7.2 |
| 5 | 1375 | 2.8 | 0.5 × 103 | 7.6 |
| 6 | 2376 | 1.6 | 0.9 × 103 | 8.8 |
| 7 | 3773 | 1.0 | 1.5 × 103 | 10.6 |
| 8 | 5632 | 0.7 | 2.2 × 103 | 22.6 |
| Coil Name | Coil Current (MAturns) | Coil Name | Coil Current (MAturns) |
|---|---|---|---|
| PF1 | 4.110 | PF6 | 9.331 |
| PF2 | −1.910 | PF5 | −3.211 |
| PF3 | −2.047 | PF4 | −1.815 |
| CS3U-H | −0.071 | CS3L-H | 0.295 |
| CS3U-M | −0.083 | CS3L-M | 0.347 |
| CS3U-L | −0.150 | CS3L-L | 0.624 |
| CS2U-H | 0.398 | CS2L-H | −0.933 |
| CS2U-M | 0.468 | CS2L-M | −1.097 |
| CS2U-L | 0.842 | CS2L-L | −1.975 |
| CS1U-H | −1.596 | CS1L-H | −1.345 |
| CS1U-M | −1.878 | CS1L-M | −1.583 |
| CS1U-L | −3.380 | CS1L-L | −2.850 |
| Coil Name | Coil Current (MAturns) |
|---|---|
| PF1 | 2.484 |
| PF2 | 0.816 |
| PF3 | 0 |
| CS3U-H | 1.700 |
| CS3U-M | 2.000 |
| CS3U-L | 3.600 |
| CS2U-H | 2.135 |
| CS2U-M | 2.512 |
| CS2U-L | 4.522 |
| CS1U-H | 2.135 |
| CS1U-M | 2.512 |
| CS1U-L | 4.522 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Albanese, R.; Chiariello, A.G.; Fresa, R.; Iaiunese, A.; Martone, R.; Zumbolo, P. Effectiveness of the Chebyshev Approximation in Magnetic Field Line Tracking. Energies 2022, 15, 7619. https://doi.org/10.3390/en15207619
Albanese R, Chiariello AG, Fresa R, Iaiunese A, Martone R, Zumbolo P. Effectiveness of the Chebyshev Approximation in Magnetic Field Line Tracking. Energies. 2022; 15(20):7619. https://doi.org/10.3390/en15207619
Chicago/Turabian StyleAlbanese, Raffaele, Andrea Gaetano Chiariello, Raffaele Fresa, Antonio Iaiunese, Raffaele Martone, and Pasquale Zumbolo. 2022. "Effectiveness of the Chebyshev Approximation in Magnetic Field Line Tracking" Energies 15, no. 20: 7619. https://doi.org/10.3390/en15207619
APA StyleAlbanese, R., Chiariello, A. G., Fresa, R., Iaiunese, A., Martone, R., & Zumbolo, P. (2022). Effectiveness of the Chebyshev Approximation in Magnetic Field Line Tracking. Energies, 15(20), 7619. https://doi.org/10.3390/en15207619






