State Feedback Speed Control with Periodic Disturbances Attenuation for PMSM Drive
Abstract
:1. Introduction
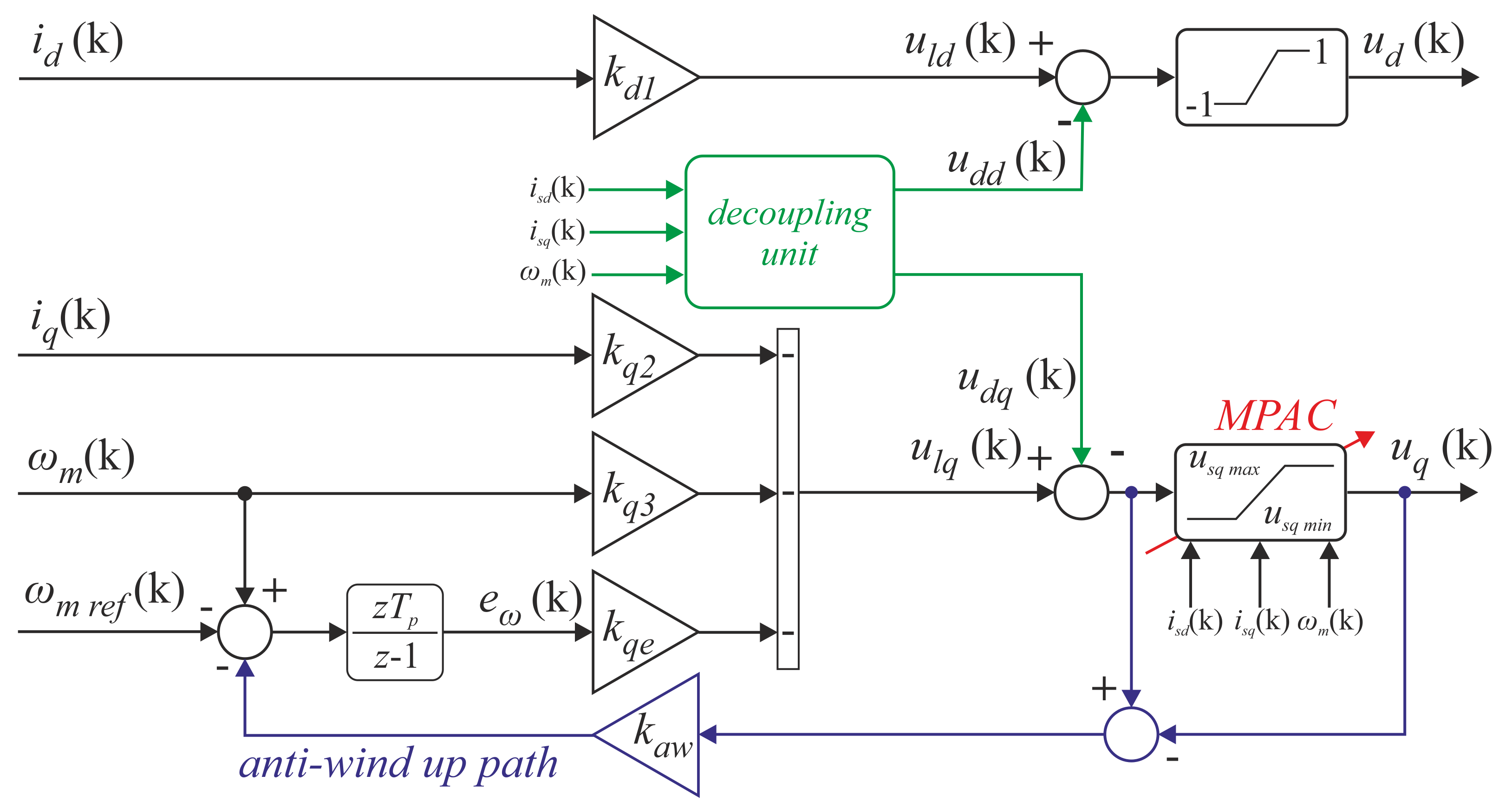
2. Proposed Control System
2.1. Employed Control Structure
2.2. Auto-Tuning Algorithm
| Algorithm 1 Artificial Bee Colony algorithm. |
|
3. Experimental Results
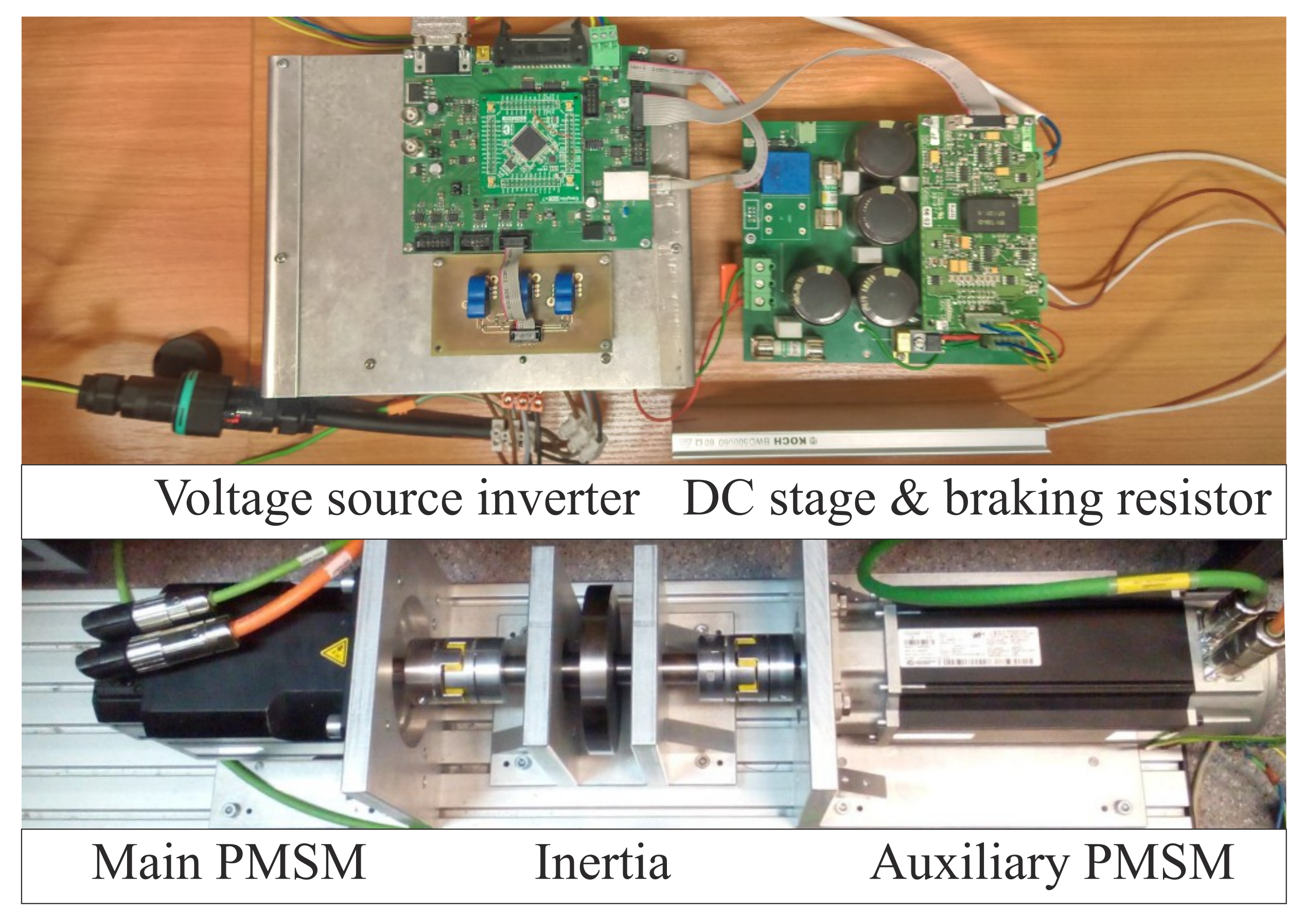
3.1. Laboratory Stand
3.2. Tuning Experiment
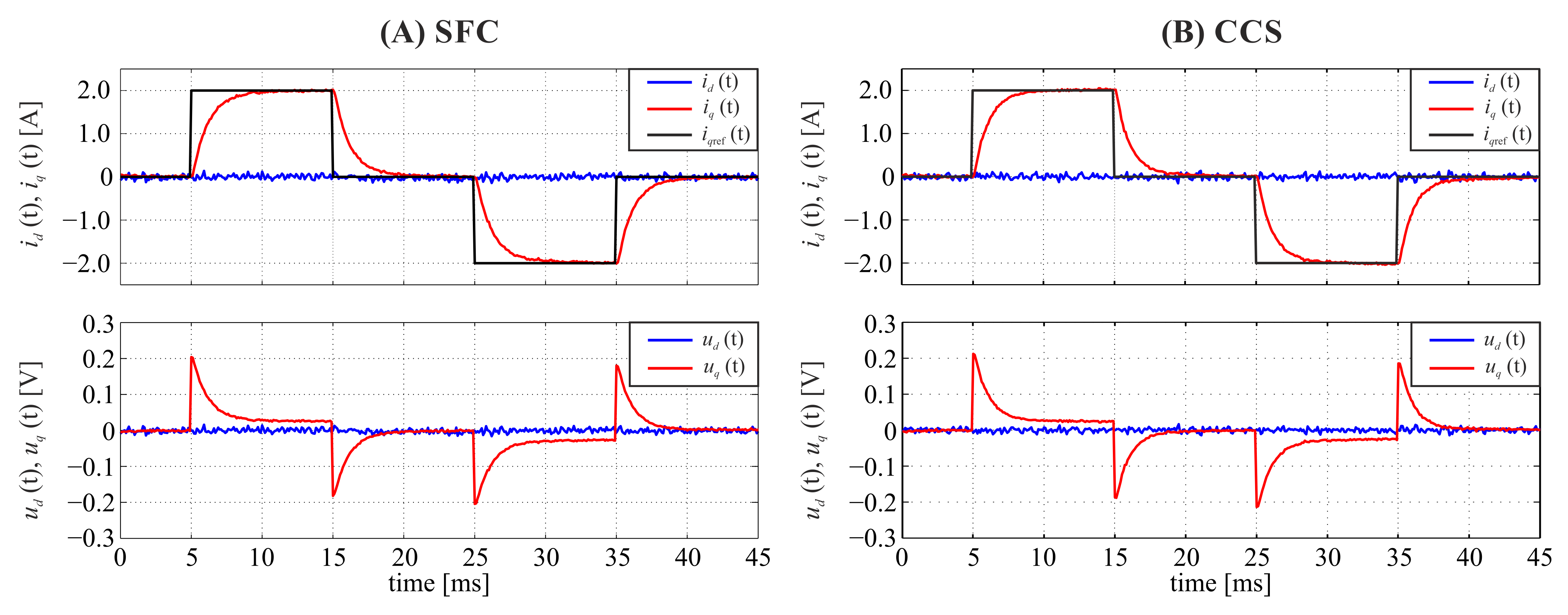
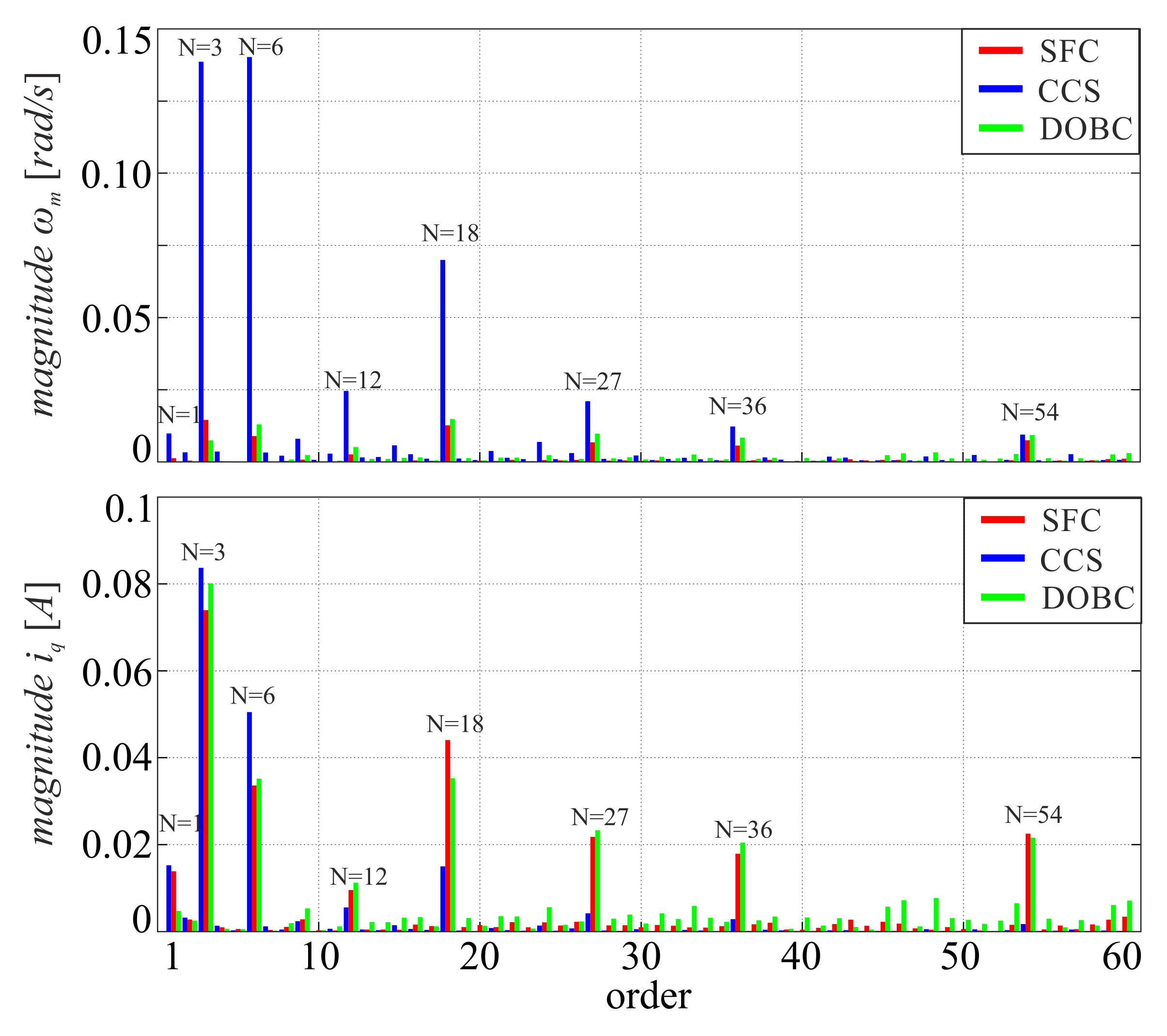
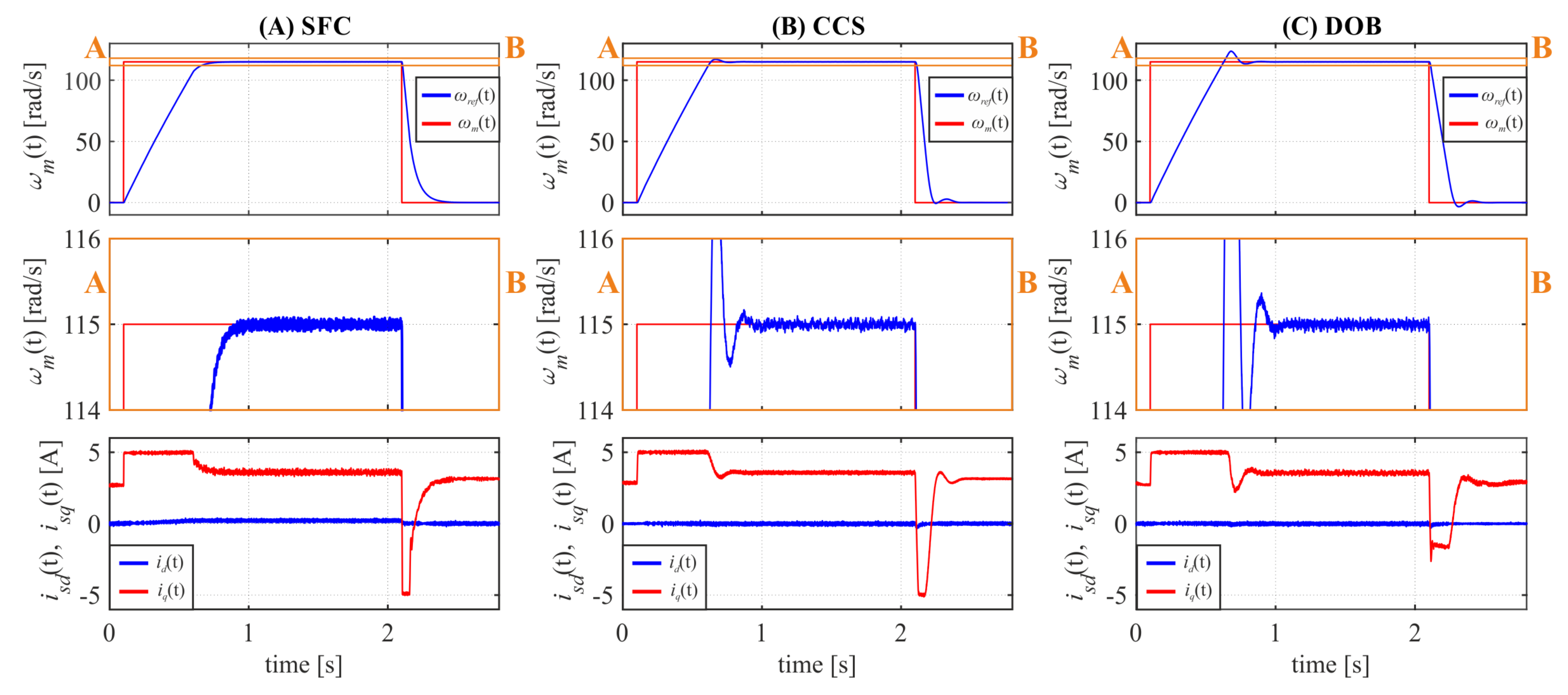
3.3. Quadrature-Current Step Response
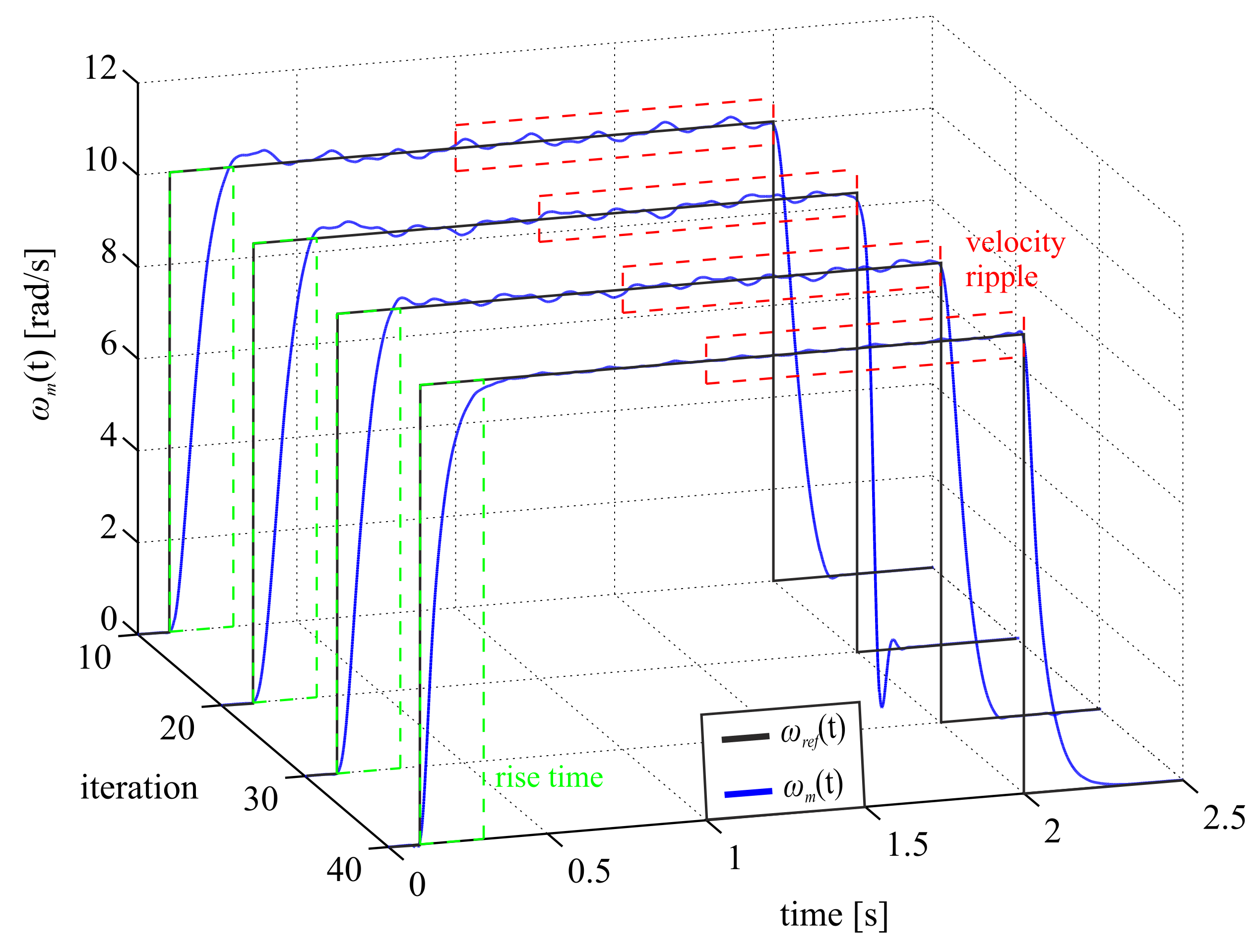
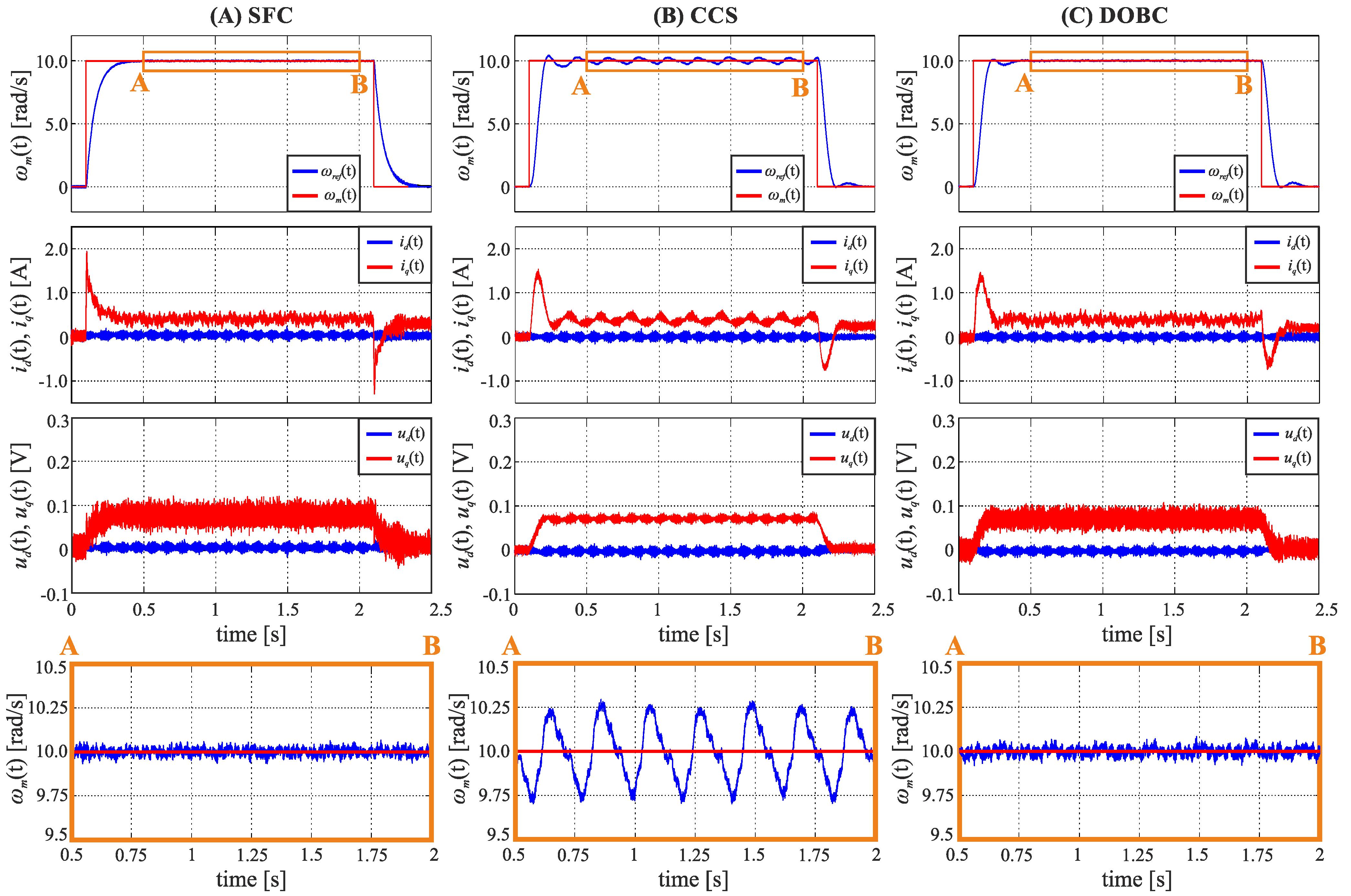
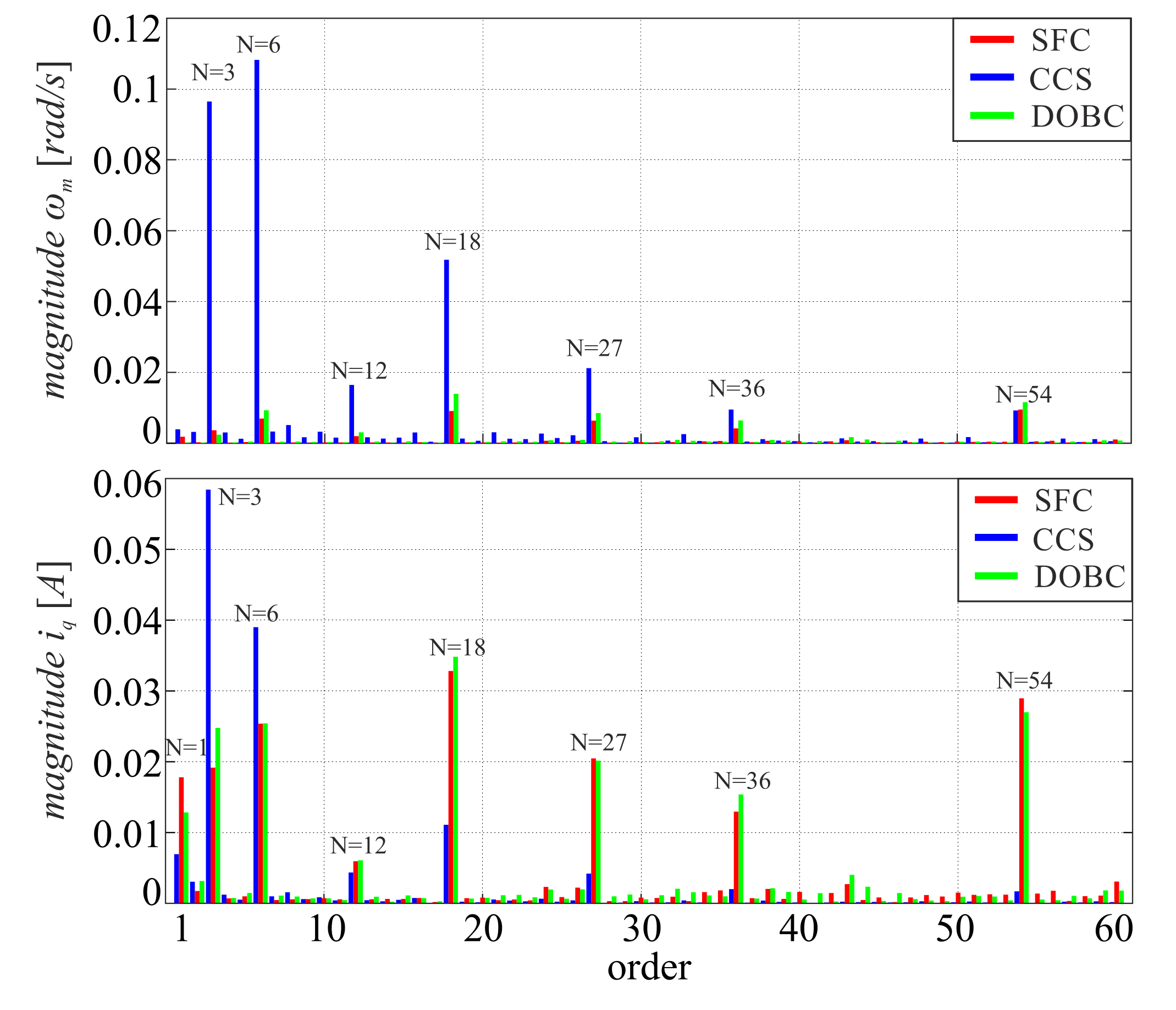
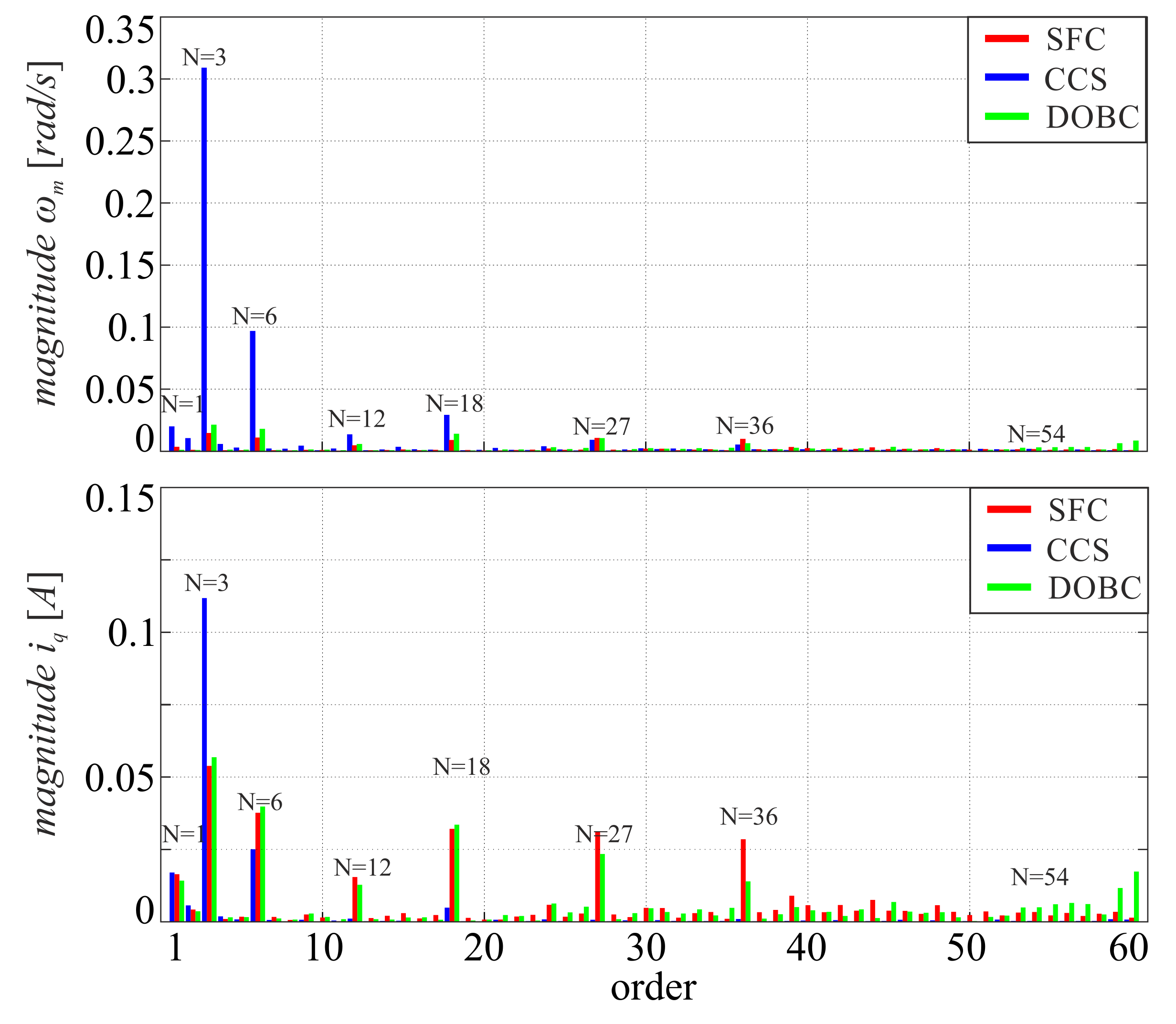
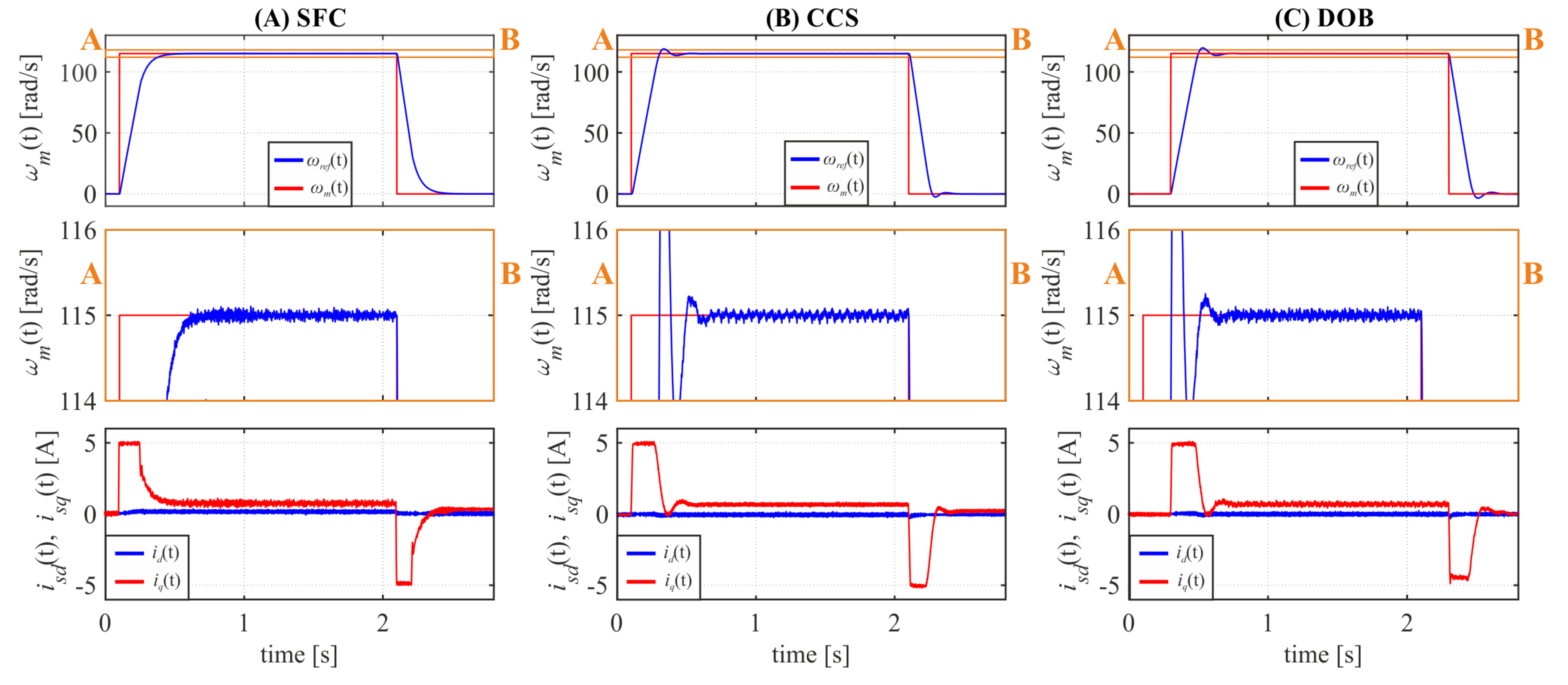
3.4. Velocity Step Response
- 1.
- The regulation time of compared control structures is equal to 178.5 ms for SFC and 103.3 ms for CCS;
- 2.
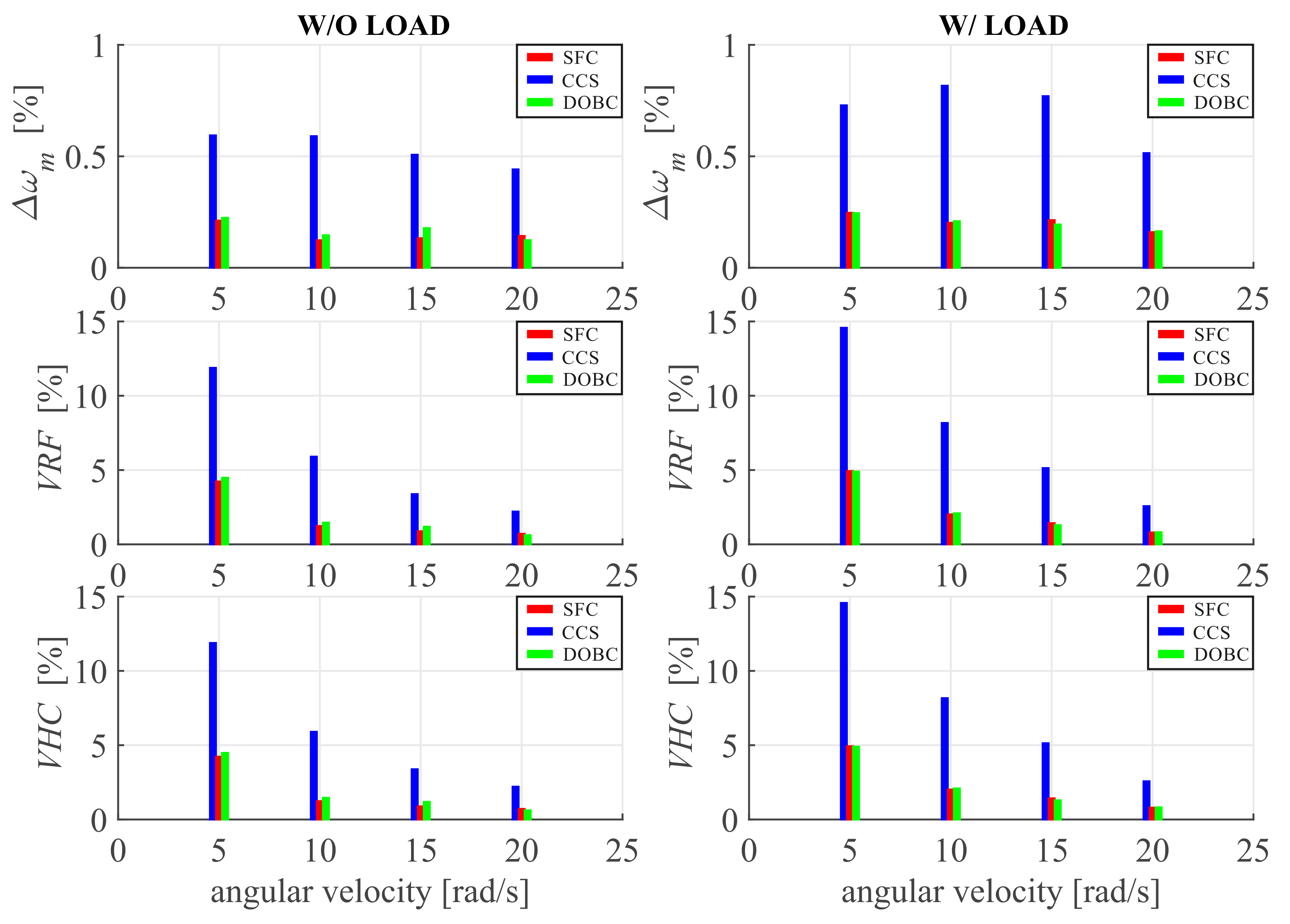
- The level of velocity ripple for a classical CCS with PI controllers is much higher than for a system based on SFC (0.591 rad/s vs. 0.173 rad/s);
- 3.
- In respect to (2) the attenuation of periodic disturbances present in the investigated PMSM drive is much higher in the SFC based control system;
- 4.
- An ABC based tuning procedure can be successfully employed for the proposed SFC structure in order to obtain smooth operation of the drive.
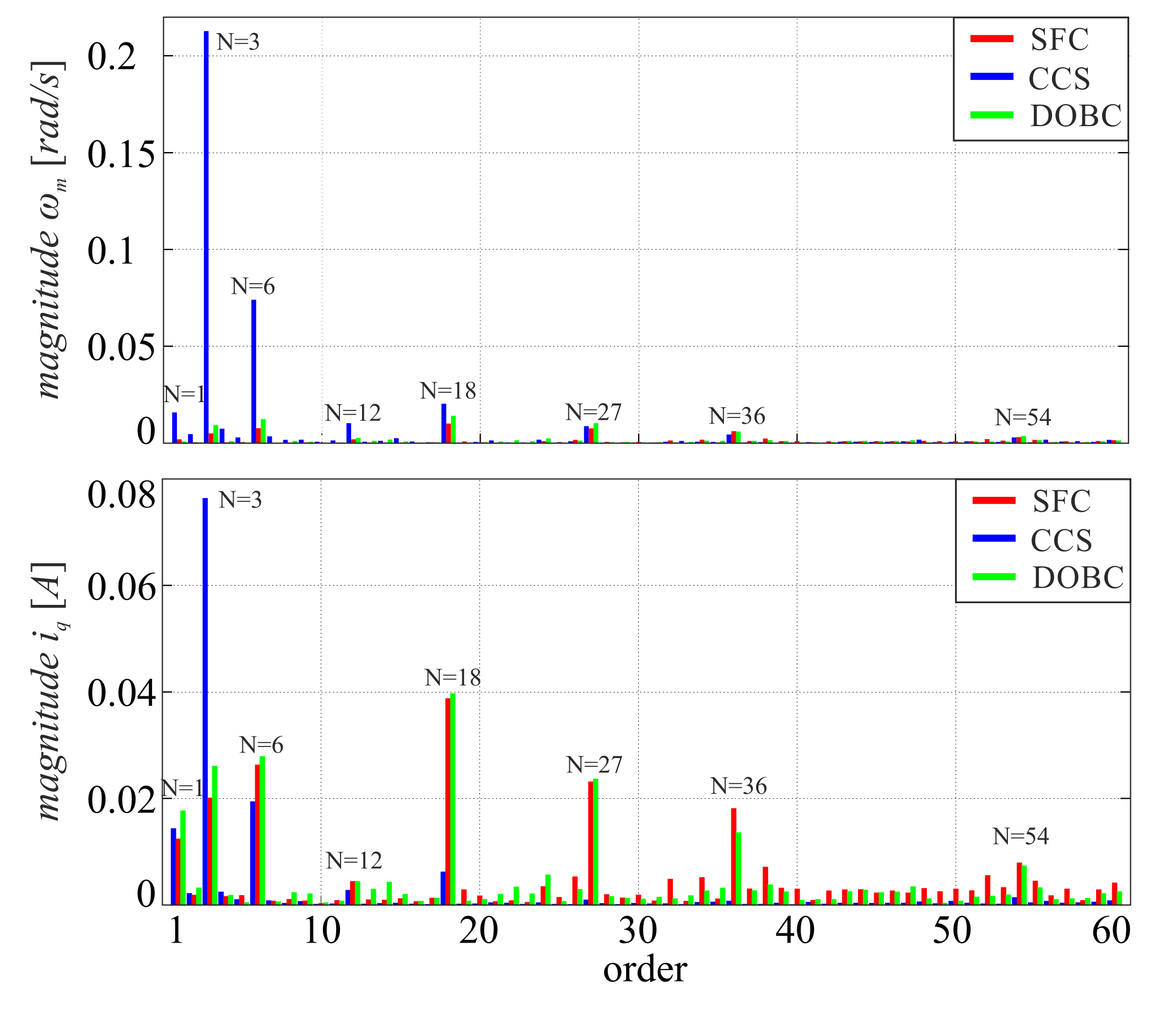
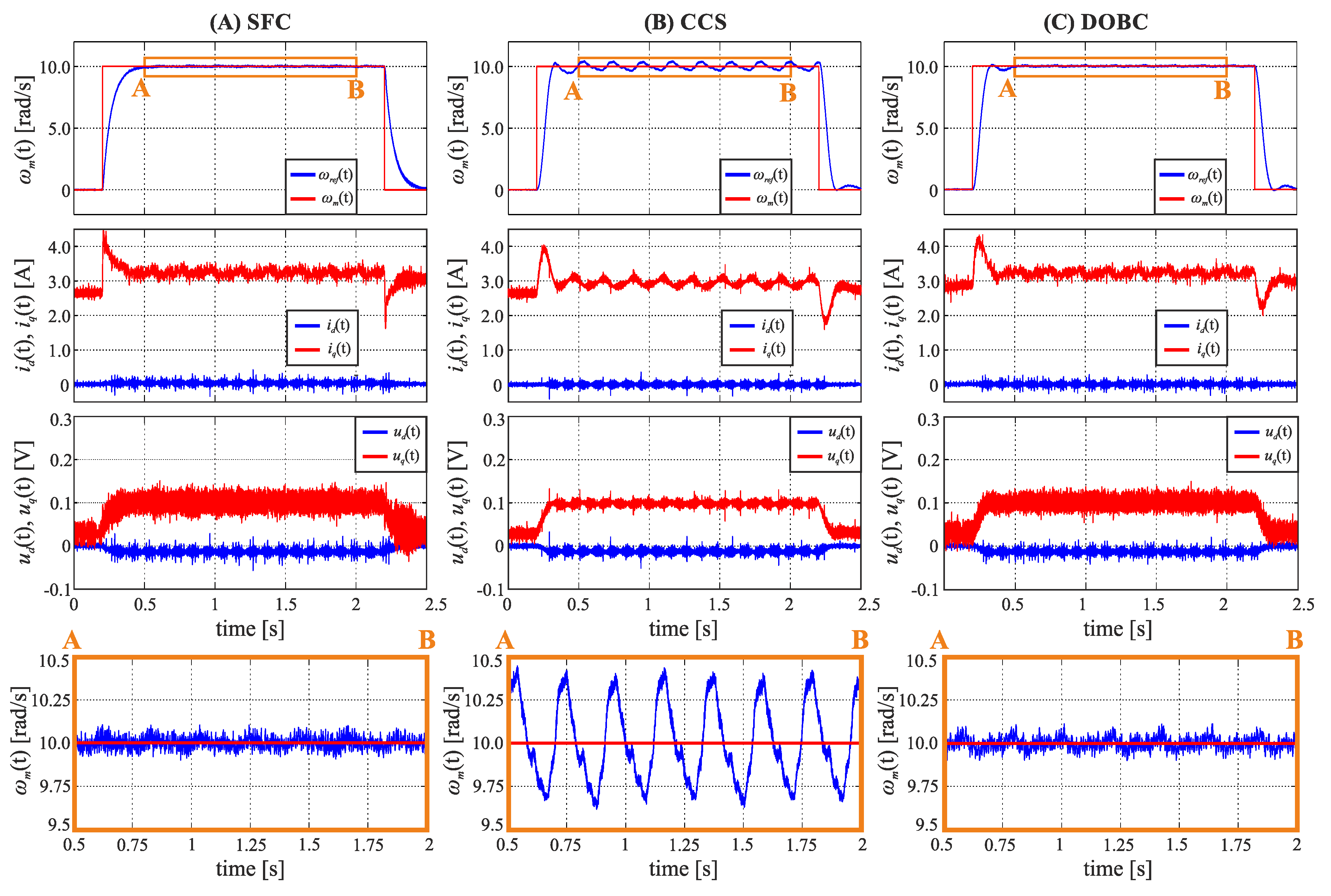
- 1.
- The regulation time of compared control structures is equal to 183.4 ms for SFC and 104.1 ms for CCS at constant load operation;
- 2.
- The level of velocity ripple for classical CCS with PI controllers is also much higher than for a SFC based system (0.817 rad/s vs. 0.202 rad/s);
- 3.
- The proposed control structure (SFC) ensures smooth operation of the drive with constant load torque;
- 4.
- With respect to (2) the attenuation of periodic disturbances is much better in the SFC based control system.
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tarczewski, T.; Grzesiak, L.M. Constrained State Feedback Speed Control of PMSM Based on Model Predictive Approach. IEEE Trans. Ind. Electron. 2016, 63, 3867–3875. [Google Scholar] [CrossRef]
- Yang, J.; Chen, W.; Li, S.; Guo, L.; Yan, Y. Disturbance/Uncertainty Estimation and Attenuation Techniques in PMSM Drives-A Survey. IEEE Trans. Ind. Electron. 2017, 64, 3273–3285. [Google Scholar] [CrossRef] [Green Version]
- Zhao, K.; Yin, T.; Zhang, C.; Li, X.; Chen, Y.; Li, T.; He, J. Sliding mode-based velocity and torque controllers for permanent magnet synchronous motor drives system. J. Eng. 2019, 2019, 8604–8608. [Google Scholar] [CrossRef]
- Adase, L.A.; Alsofyani, I.M.; Lee, K. Predictive Torque Control With Simple Duty-Ratio Regulator of PMSM for Minimizing Torque and Flux Ripples. IEEE Access 2020, 8, 2373–2381. [Google Scholar] [CrossRef]
- Shi, T.; Yan, Y.; Zhou, Z.; Xiao, M.; Xia, C. Linear Quadratic Regulator Control for PMSM Drive Systems Using Nonlinear Disturbance Observer. IEEE Trans. Power Electron. 2020, 35, 5093–5101. [Google Scholar] [CrossRef]
- Sandre-Hernandez, O.; Rangel-Magdaleno, J.; Morales-Caporal, R. Modified model predictive torque control for a PMSM-drive with torque ripple minimisation. IET Power Electron. 2019, 12, 1033–1042. [Google Scholar] [CrossRef]
- Niewiara, Ł.J.; Tarczewski, T.; Grzesiak, L.M. Application of extended Kalman filter for estimation of periodic disturbance and velocity ripple reduction in PMSM drive. Bull. Pol. Acad. Sci. Tech. Sci. 2020, 68, 983–995. [CrossRef]
- Chung, D.-W.; Sul, S.-K. Analysis and compensation of current measurement error in vector-controlled AC motor drives. IEEE Trans. Ind. Appl. 1998, 34, 340–345. [Google Scholar] [CrossRef]
- Houari, A.; Bouabdallah, A.; Djerioui, A.; Machmoum, M.; Auger, F.; Darkawi, A.; Olivier, J.; Benkhoris, M.F. An Effective Compensation Technique for Speed Smoothness at Low-Speed Operation of PMSM Drives. IEEE Trans. Ind. Appl. 2018, 54, 647–655. [Google Scholar] [CrossRef]
- Tang, M.; Formentini, A.; Odhano, S.A.; Zanchetta, P. Torque Ripple Reduction of PMSMs Using a Novel Angle-Based Repetitive Observer. IEEE Trans. Ind. Electron. 2020, 67, 2689–2699. [Google Scholar] [CrossRef] [Green Version]
- Jo, I.; Lee, H.; Jeong, G.; Ji, W.; Park, C. A Study on the Reduction of Cogging Torque for the Skew of a Magnetic Geared Synchronous Motor. IEEE Trans. Magn. 2019, 55, 1–5. [Google Scholar] [CrossRef]
- Bozorgi, A.M.; Farasat, M.; Jafarishiadeh, S. Model predictive current control of surface-mounted permanent magnet synchronous motor with low torque and current ripple. IET Power Electron. 2017, 10, 1120–1128. [Google Scholar] [CrossRef]
- Feng, G.; Lai, C.; Kar, N.C. A Closed-Loop Fuzzy-Logic-Based Current Controller for PMSM Torque Ripple Minimization Using the Magnitude of Speed Harmonic as the Feedback Control Signal. IEEE Trans. Ind. Electron. 2017, 64, 2642–2653. [Google Scholar] [CrossRef]
- Pajchrowski, T. Application of neural networks for compensation of torque ripple in high performance PMSM motor. In Proceedings of the 2017 19th European Conference on Power Electronics and Applications (EPE’17 ECCE Europe), Warsaw, Poland, 11–14 September 2017; pp. P1–P8. [Google Scholar]
- Shahruz, S.M. Performance enhancement of a class of nonlinear systems by disturbance observers. IEEE/ASME Trans. Mechatron. 2000, 5, 319–323. [Google Scholar] [CrossRef]
- Tarczewski, T.; Skiwski, M.; Niewiara, L.J.; Grzesiak, L.M. High-performance PMSM servo-drive with constrained state feedback position controller. Bull. Pol. Acad. Sci. 2018, 66, 49–58. [Google Scholar] [CrossRef]
- Szczepanski, R.; Kaminski, M.; Tarczewski, T. Auto-Tuning Process of State Feedback Speed Controller Applied for Two-Mass System. Energies 2020, 13, 3067. [Google Scholar] [CrossRef]
- Tarczewski, T.; Grzesiak, L.M. An Application of Novel Nature-Inspired Optimization Algorithms to Auto-Tuning State Feedback Speed Controller for PMSM. IEEE Trans. Ind. Appl. 2018, 54, 2913–2925. [Google Scholar] [CrossRef]
- Deb, K. An efficient constraint handling method for genetic algorithms. Comput. Methods Appl. Mech. Eng. 2000, 186, 311–338. [Google Scholar] [CrossRef]
- Harnefors, L.; Nee, H. Model-based current control of AC machines using the internal model control method. IEEE Trans. Ind. Appl. 1998, 34, 133–141. [Google Scholar] [CrossRef]
- Brock, S.; Łuczak, D. Speed control in direct drive with non-stiff load. In Proceedings of the 2011 IEEE International Symposium on Industrial Electronics, Gdansk, Poland, 27–30 June 2011; pp. 1937–1942. [Google Scholar] [CrossRef]
- Kim, J.; Yoon, M.; Hong, J.; Kim, S. Analysis of cogging torque caused by manufacturing tolerances of surface-mounted permanent magnet synchronous motor for electric power steering. IET Electr. Power Appl. 2016, 10, 691–696. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Ruangsinchaiwanich, S.; Howe, D. Synthesis of cogging-torque waveform from analysis of a single stator slot. IEEE Trans. Ind. Appl. 2006, 42, 650–657. [Google Scholar] [CrossRef]




| Parameter | Symbol | Value |
|---|---|---|
| Dimension of optimized parameters | D | 6 |
| Colony size | NP | 10 |
| Number of food sources | FN | 5 (NP/2) |
| Maximum number of iterations | MNI | 90 |
| Limit of trials | limit | 30 (FN×D) |
| Scout production period | SPP | 30 (FN×D) |
| Modification rate | MR | 0.8 |
| Lower bounds of parameters | ||
| Upper bounds of parameters | ||
| Ripple gain coefficient | 5.0 | |
| Rise time limit | 100 ms | |
| Maximum current | 5.0 A |
| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Rated power | 2.76 | kW | |
| Rated current | 5.8 | A | |
| Rated torque | 8.8 | Nm | |
| Rated speed | 314 | rad/s | |
| Switching frequency | 10.0 | kHz | |
| Stator inductance | 12.7 | mH | |
| Stator resistance | 1.05 | ||
| Flux linkage | 0.254 | Wb | |
| Inverter gain | 115.5 | V/V | |
| Number of poles | 6 | - | |
| Number of stator slots | 27 | - | |
| Torque constant | 1.15 | Nm/A | |
| Moment of inertia | kg m | ||
| Friction coefficient | Nms/rad |
| Parameter | Symbol | Value | Unit |
|---|---|---|---|
| Proportional gain of velocity controller | 0.229 | As/rad | |
| Integral gain of velocity controller | 36.620 | A/rad | |
| Proportional gain of current controllers | 0.104 | V/A | |
| Integral gain of current controllers | 82.847 | V/As |
| Velocity | CCS | SFC | DOBC | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| [rad/s] | [%] | [%] | [rad/s] | [%] | [%] | [rad/s] | [%] | [%] | ||
| w/o load | 5 rad/s | 0.59 | 11.9 | 3.13 | 0.21 | 4.23 | 0.35 | 0.22 | 4.48 | 0.46 |
| 10 rad/s | 0.59 | 5.90 | 2.29 | 0.12 | 1.23 | 0.19 | 0.15 | 1.46 | 0.27 | |
| 15 rad/s | 0.51 | 3.38 | 1.37 | 0.13 | 0.88 | 0.15 | 0.18 | 1.19 | 0.19 | |
| 20 rad/s | 0.44 | 2.21 | 0.77 | 0.14 | 0.71 | 0.12 | 0.12 | 0.62 | 0.13 | |
| w/load | 5 rad/s | 0.73 | 14.6 | 4.26 | 0.25 | 4.93 | 0.49 | 0.25 | 4.90 | 0.58 |
| 10 rad/s | 0.82 | 8.17 | 3.26 | 0.20 | 2.03 | 0.30 | 0.21 | 2.09 | 0.36 | |
| 15 rad/s | 0.77 | 5.13 | 2.14 | 0.21 | 1.42 | 0.21 | 0.19 | 1.29 | 0.27 | |
| 20 rad/s | 0.51 | 2.57 | 1.02 | 0.16 | 0.80 | 0.16 | 0.16 | 0.82 | 0.21 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Niewiara, Ł.J.; Szczepański, R.; Tarczewski, T.; Grzesiak, L.M. State Feedback Speed Control with Periodic Disturbances Attenuation for PMSM Drive. Energies 2022, 15, 587. https://doi.org/10.3390/en15020587
Niewiara ŁJ, Szczepański R, Tarczewski T, Grzesiak LM. State Feedback Speed Control with Periodic Disturbances Attenuation for PMSM Drive. Energies. 2022; 15(2):587. https://doi.org/10.3390/en15020587
Chicago/Turabian StyleNiewiara, Łukasz J., Rafał Szczepański, Tomasz Tarczewski, and Lech M. Grzesiak. 2022. "State Feedback Speed Control with Periodic Disturbances Attenuation for PMSM Drive" Energies 15, no. 2: 587. https://doi.org/10.3390/en15020587
APA StyleNiewiara, Ł. J., Szczepański, R., Tarczewski, T., & Grzesiak, L. M. (2022). State Feedback Speed Control with Periodic Disturbances Attenuation for PMSM Drive. Energies, 15(2), 587. https://doi.org/10.3390/en15020587








