Research on Rock Damage Evolution Based on Fractal Theory-Improved Dynamic Tensile-Compression Damage Model
Abstract
:1. Introduction
2. Establishment of Tension-Compression Damage Model
2.1. Improved Damage Model
2.2. Determination of Parameters and Damage Threshold
3. Model Verification
3.1. Model Validation Scheme
3.2. Field Test Scheme of Blasting Operation
3.3. Numerical Validation
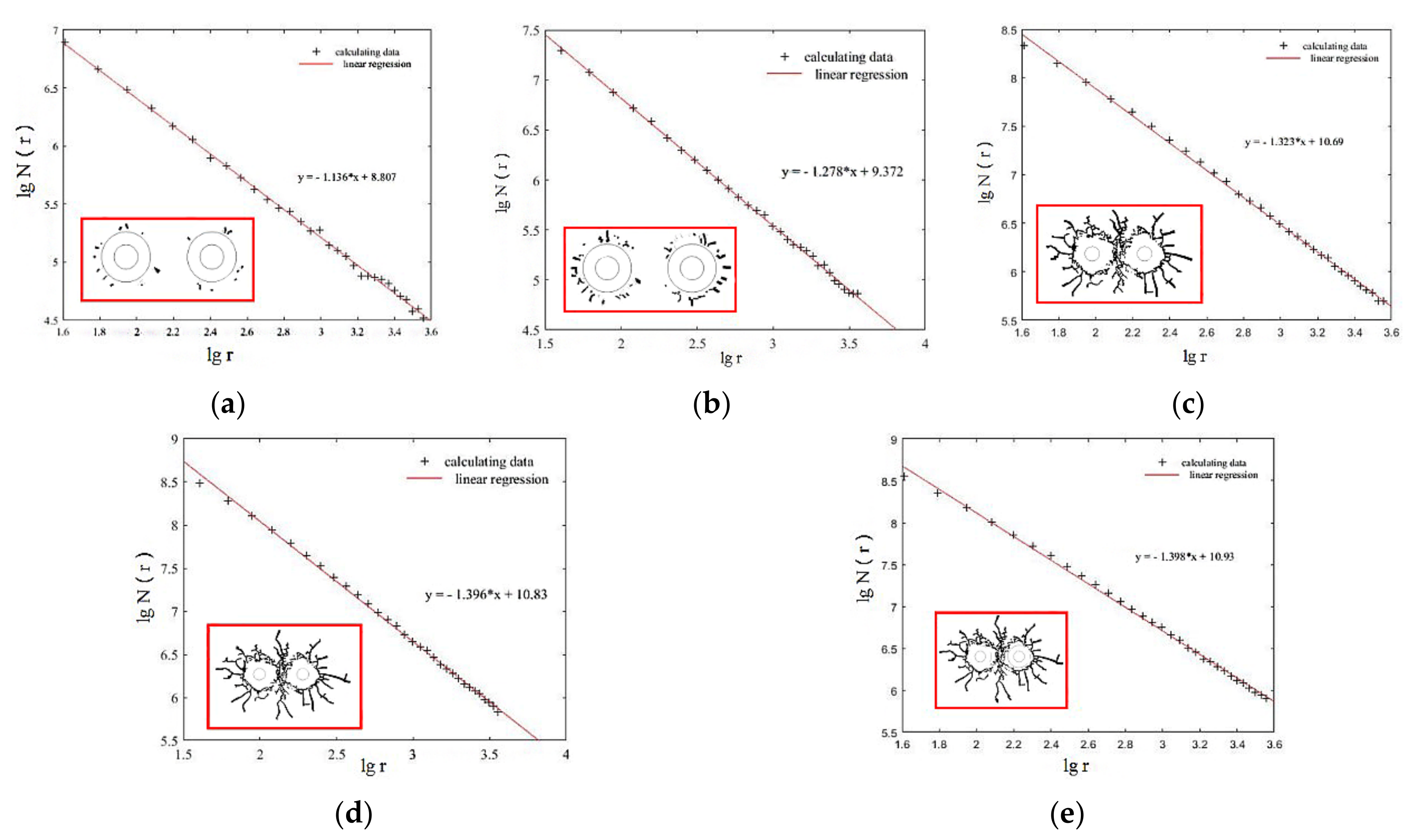
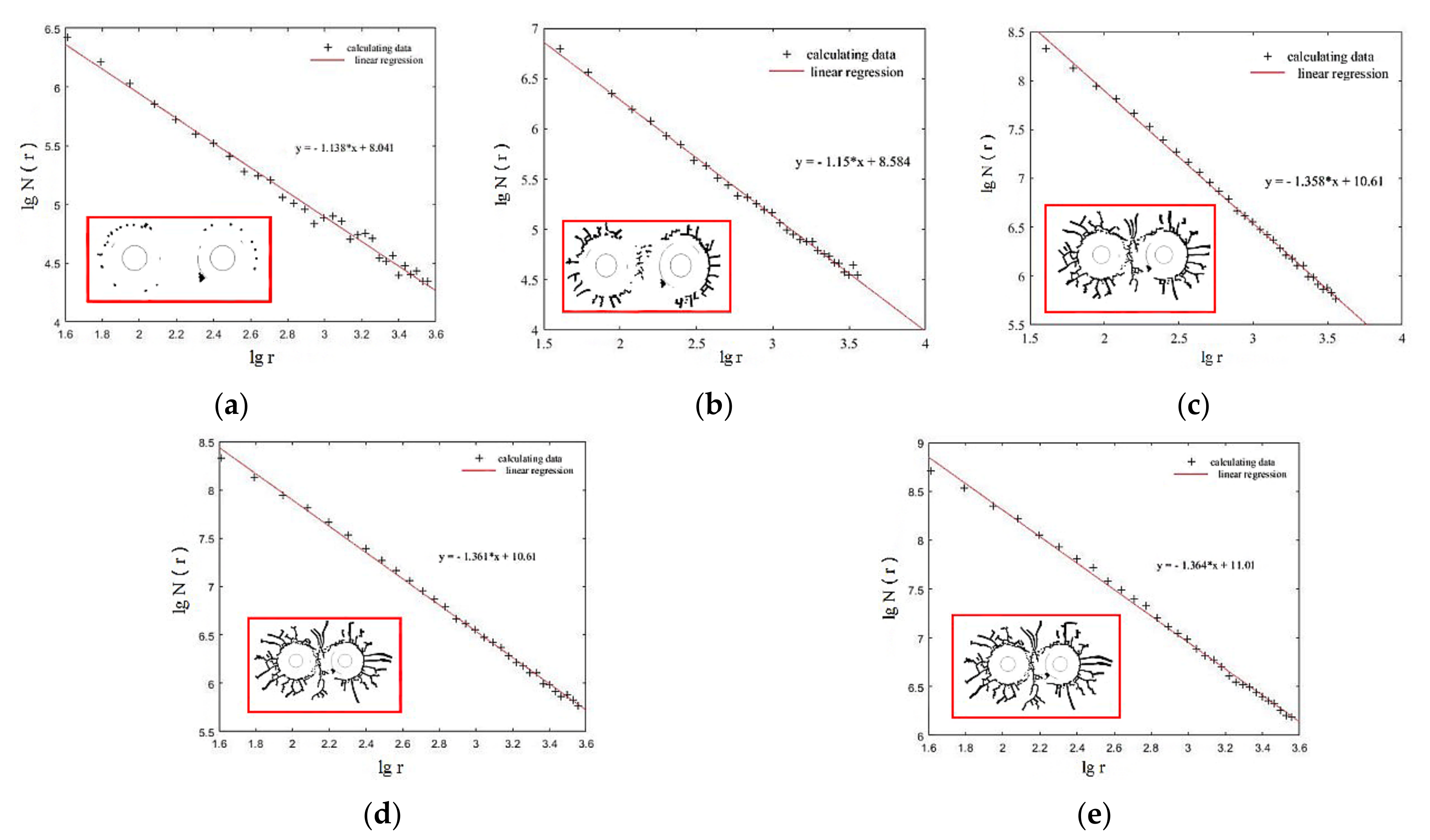
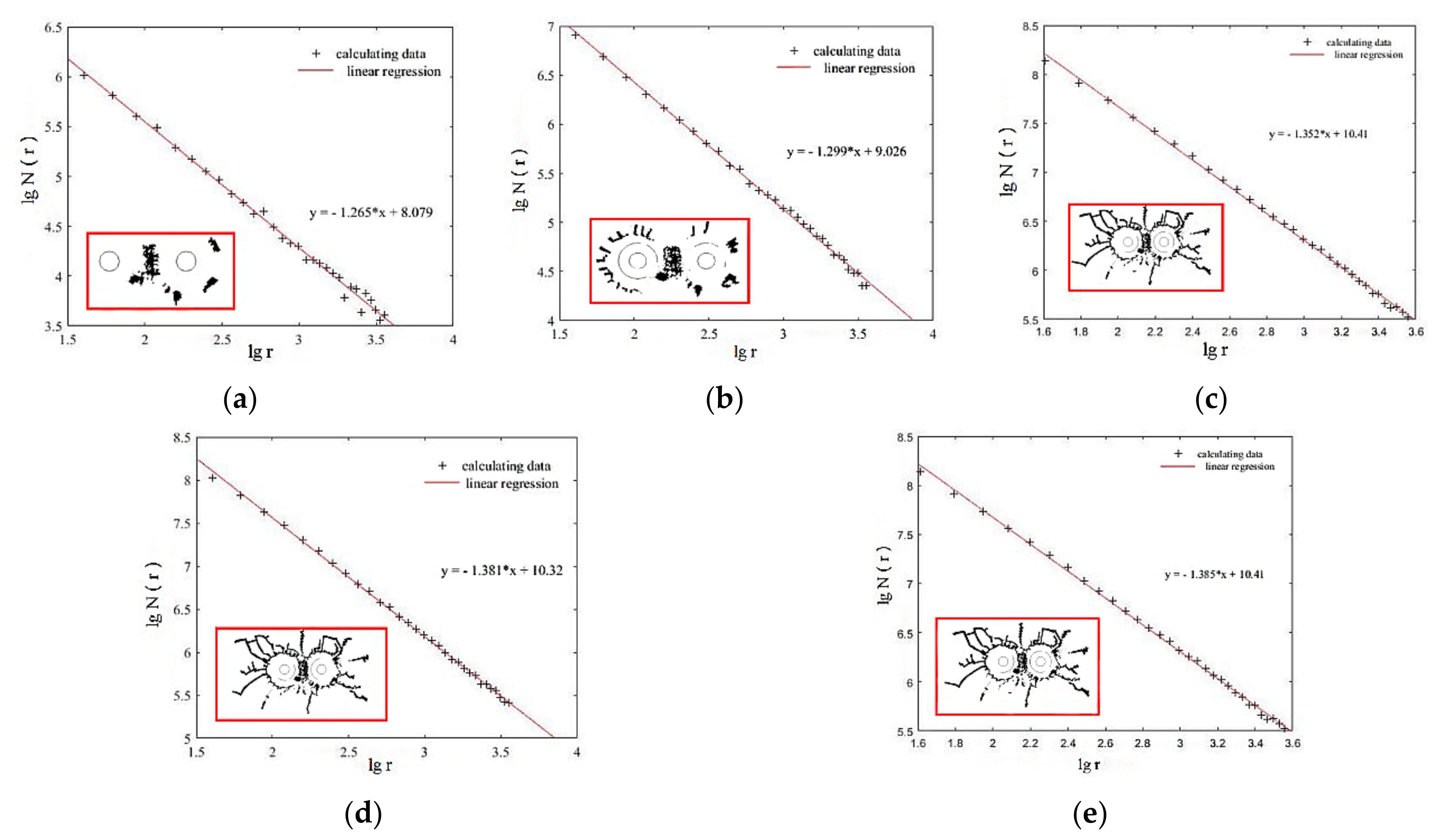
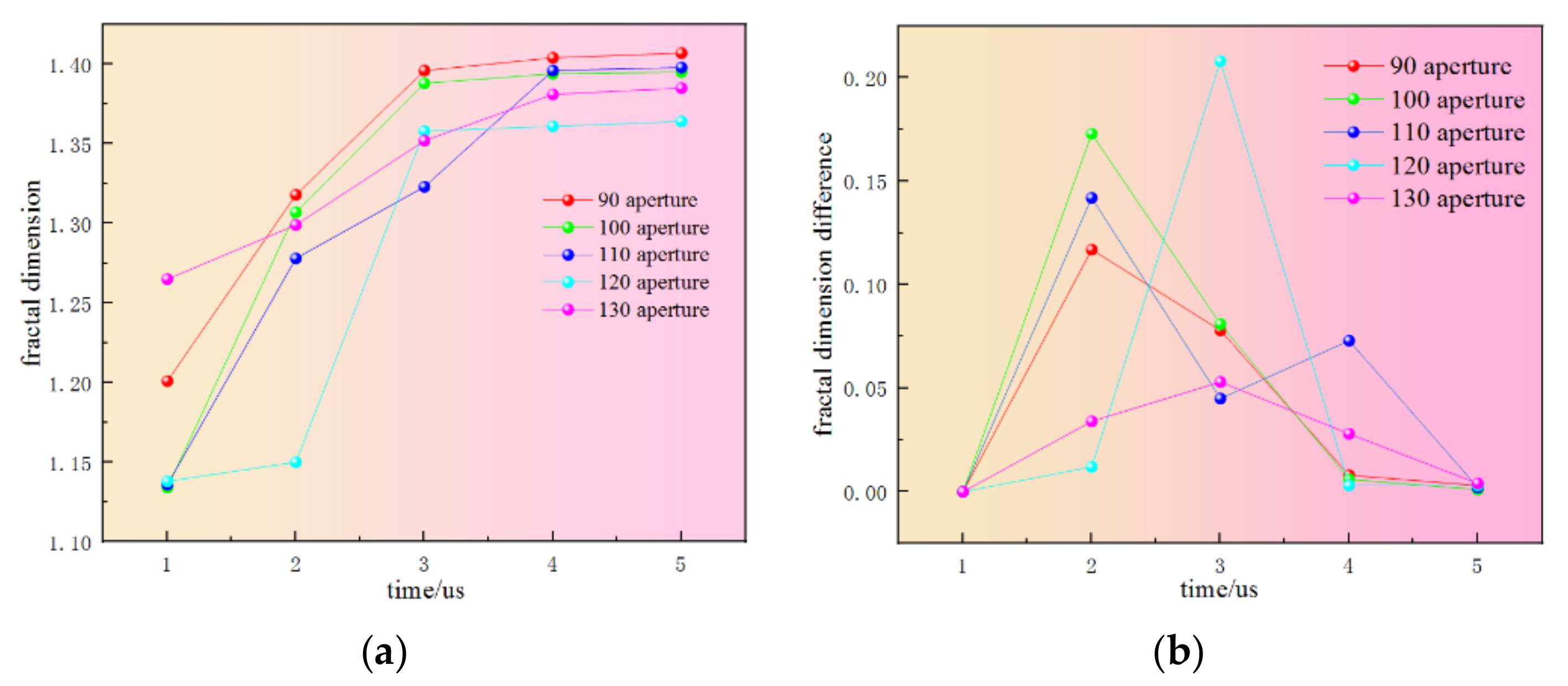
4. Damage Characteristics
5. Conclusions
- The tension-compression model of blasting damage proposed in this paper includes the tensile damage and compression damage of rock, which can reflect the different characteristics of rock tensile and compression damage to a certain extent. Based on previous studies, the expression of rock stiffness degradation caused by compression damage and tensile damage was established. By analyzing the uniaxial tensile test of rock, the physical meaning of the material constants defining the crack density function in the tensile damage model was clarified, and the method for determining the constants was given. Compared with other models, the proposed model parameters are less and the physical meaning is clear, and the application is more convenient.
- The tensile and compressive damage models proposed in this paper consider the influence of initial damage, and the initial damage can be obtained by the acoustic test in the engineering site, which overcomes the deficiency of the existing models that seldom consider the initial damage. Compared with other models, the numerical calculation results of the blasting damage range of the proposed model were closest to the field measurement results, which proves the rationality of the proposed model and its suitability for practical blast engineering analysis.
- Based on the tensile and compressive damage model proposed in this paper, the fractal theory was introduced to further elaborate the damage characteristics of rock under blasting load. With the increase in shock wave propagation distance and propagation time, the energy gradually weakened, the crack growth rate decreased, and the crack density growth rate decreased. At the early stages after initiation, the crack density of 90 aperture and 130 aperture was larger than that of other working conditions, and the crack density of 130 aperture was the largest, followed by 90 aperture. In the middle stage after initiation, obvious cracks were formed around the two blastholes; at the end of initiation, the shock wave front decreased rapidly and continued to propagate outward in the form of a compression wave; the crack propagation velocity was much smaller than that at the early and middle stages after initiation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Tatone, B.S.A.; Abdelaziz, A.; Grasselli, G. Novel Mechanical Classification Method of Rock Based on the Uniaxial Compressive Strength and Brazilian Disc Strength. Rock Mech. Rock Eng. 2022, 55, 2503–2507. [Google Scholar]
- Wei, J.; Zhou, J.; Song, J.-J.; Chen, Y.; Kulatilake, P.H.S.W. Estimation of tensile strength and moduli of a tension-compression bi-modular rock. Geomech. Eng. 2021, 24, 349–358. [Google Scholar]
- Grady, D.E.; Kipp, M.E. Continuum modeling of explosive fracture in oil shale. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1980, 17, 147–157. [Google Scholar]
- Taylor, L.M.; Chen, E.P.; Kuszmau, J.S. Microcrack-induced damage accumulation in brittle rock under dynamic loading. Comput. Methods Appl. Mech. Eng. 1986, 55, 301–320. [Google Scholar] [CrossRef]
- Budiansky, B.; O’connell, R.J. Elastic moduli of acracked solid. Int. J. Solids Struct. 1976, 12, 81–97. [Google Scholar] [CrossRef]
- Kuszmaul, J.S. A new constitution model for fragmentation of rock under dynamic loading. In Proceedings of the 2nd International Symposium on Rock Fragmentation by Blasting, Keystone, CO, USA, 23–26 August 1987; pp. 412–423. [Google Scholar]
- Hu, Y.; Lu, W.; Chen, M.; Yan, P.; Zhou, C. Comparison and improvement of blasting damage models for rock. Rock Soil Mech. 2012, 33, 3278–3284. [Google Scholar]
- Thorne, B.J.; Hommert, P.J.; Brown, B. Experimentaland computational investigation of the fundamentalmechanisms of cratering. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1992, 29, 309. [Google Scholar]
- Yang, R.; Brwden, W.F.; Katsabanis, P.D. A new constitutive model for blast damage. Int. J. Rock Mech. Min. Sci. 1996, 33, 245–254. [Google Scholar] [CrossRef]
- Hu, X.; Qu, S.; Li, K. Study on elastoplastic damage model of rock based on unified strength theory. J. China Univ. Min. Technol. 2019, 48, 305–312. [Google Scholar]
- Sun, Z.; Yu, Q.; Yang, J. Numerical simulation of bench blasting vibration based on continuous damage constitutive model. J. Mil. Eng. 2016, 37, 232–235. [Google Scholar]
- Xu, M.; Jiang, M.; Jiang, T.; Zhang, Z.; Yu, H. Hoek-Brown elastoplastic damage model considering cyclic blasting effect and its engineering application. Rock Mech. Eng. 2020, 39, 2683–2692. [Google Scholar]
- Liu, L.; Katsabanis, P.D. Development of acontinuum damage model for blasting analysis. Int. J. Rock Mech. Min. 1997, 34, 217–231. [Google Scholar] [CrossRef]
- Yang, R.; Xu, P. Fractal study of media damageunder blasting loading. J. China Coal Soc. 2017, 42, 3065–3071. [Google Scholar]
- Xie, F.; Zhang, J.; Chen, J. Dynamic damage model of rock under impact loads of compression and tension. J. Cent. South Univ. Sci. Technol. 2019, 50, 420–427. [Google Scholar]
- Liu, Y.; Yang, G.; Wang, J.-X.; Jiang, A.-N. Mohr-Coulomb elastoplastic damage constitutive model of rockand implicit return mapping algorithm in principal stressspace. Rock Soil Mech. 2017, 38, 418–428. [Google Scholar]
- Zhang, W.; Wang, J.; Fu, H. Damage Mechanics Fundamentals of Geotechnical Engineering; Science Press: Beijing, China, 2018; pp. 55–71. [Google Scholar]
- Liang, R.; Zhou, W.; Yu, J.; Li, Z.; Du, C.; Wang, D. Numerical simulation of rock tension-compression fracture caused by impact load during slope casting blast. Chin. J. High Press. Phys. 2019, 33, 82–91. [Google Scholar]
- Cao, W.; Lin, X.; Zhang, C.; Yang, S. A statistical damage simulation method of dynamic deformation process for rocks based on nonlinear dynamic strength criterion. Chin. J. Rock Mech. Eng. 2017, 36, 794–802. [Google Scholar]
- Chen, J.; Zhang, J.; Li, X. Model of rock blasting-induced damage and its application based on the integrity of rock mass. Chin. J. Geotech. Eng. 2016, 38, 857–866. [Google Scholar]
- Shan, R.; Song, L.; Bai, Y.; Song, Y.; Li, Z.; Wei, L.; Cao, J. Model test study on damage assessment of frozen rock wall under blasting. Rock Mech. Eng. 2014, 33, 1945–1952. [Google Scholar]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Su, H.; Wang, Z.; Ma, S. Research on Rock Damage Evolution Based on Fractal Theory-Improved Dynamic Tensile-Compression Damage Model. Energies 2022, 15, 6194. https://doi.org/10.3390/en15176194
Su H, Wang Z, Ma S. Research on Rock Damage Evolution Based on Fractal Theory-Improved Dynamic Tensile-Compression Damage Model. Energies. 2022; 15(17):6194. https://doi.org/10.3390/en15176194
Chicago/Turabian StyleSu, Hengyu, Ziyi Wang, and Shu Ma. 2022. "Research on Rock Damage Evolution Based on Fractal Theory-Improved Dynamic Tensile-Compression Damage Model" Energies 15, no. 17: 6194. https://doi.org/10.3390/en15176194
APA StyleSu, H., Wang, Z., & Ma, S. (2022). Research on Rock Damage Evolution Based on Fractal Theory-Improved Dynamic Tensile-Compression Damage Model. Energies, 15(17), 6194. https://doi.org/10.3390/en15176194






