Cavitation Flow Characteristics of Water and Liquid Oxygen in the Inducer Considering Thermodynamic Effect
Abstract
:1. Introduction
2. Numerical Calculation Method
2.1. Basic Governing Equation
2.2. The Turbulence Model
2.3. Zwart Cavitation Model
2.4. Thermodynamic Modified Zwart Cavitation Model
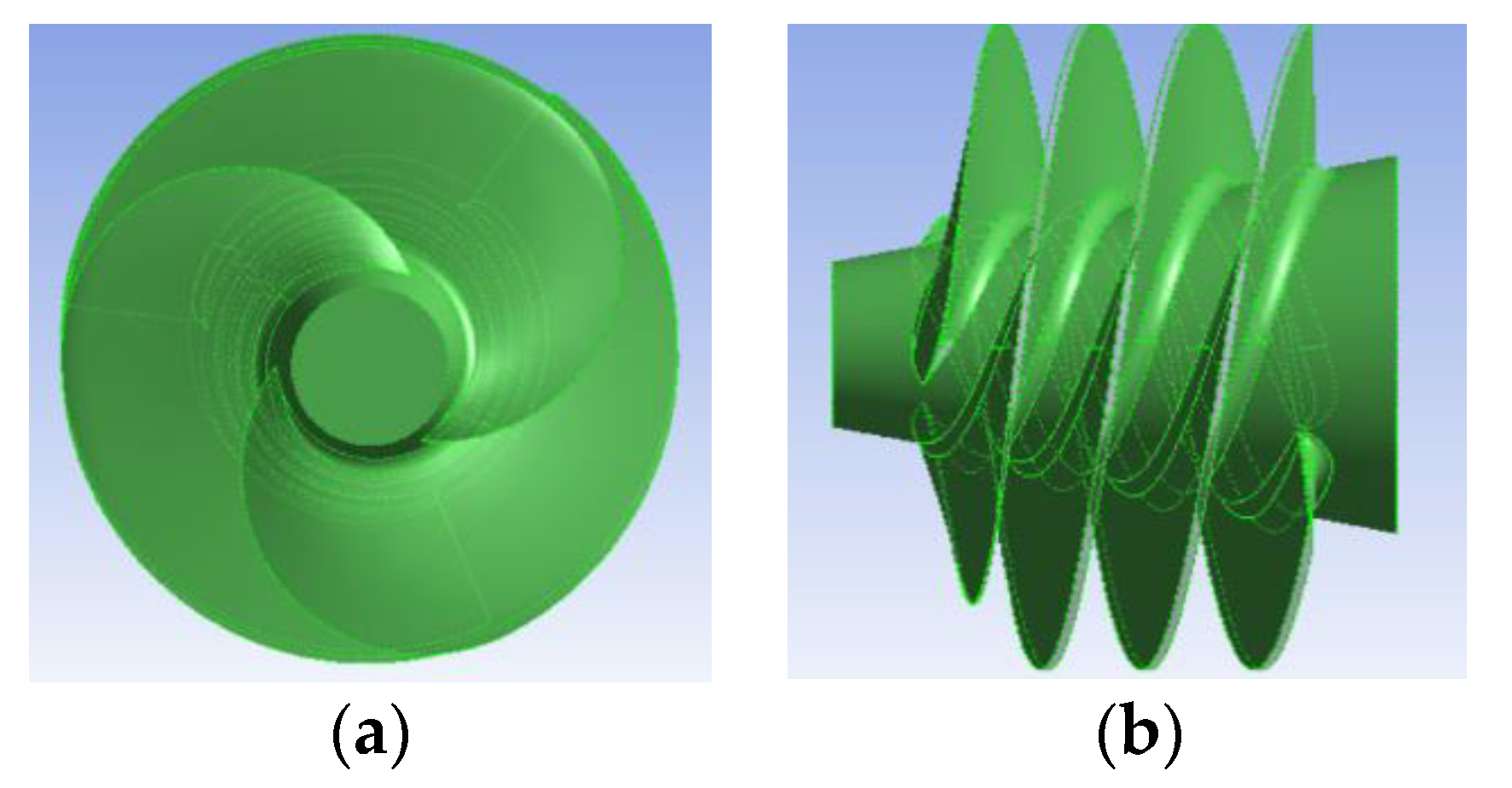
2.5. Three Blades Inducer Model of Turbopump
2.6. The Boundary Condition
- The mass flow inlet and pressure outlet are adopted. This setting makes the model easier to converge;
- The initial liquid volume fraction at the inlet of the fluid domain is 1, and the initial gas volume fraction is 0;
- The wall of the fluid domain is set without slipping, the heat transfer mode is adiabatic and the wall roughness is smooth;
- The interface model of the contact surface between the stationary domain and rotating domain is a general connection, and the phase transition/mixing model is a frozen rotor; since it only involves the analysis of the cavitation flow characteristics in the steady state, different boundary conditions need to be set. In a steady-state flow, first select the steady state, the convergence accuracy is 1 × 10−4 of the default setting and the number of iterations is 100;
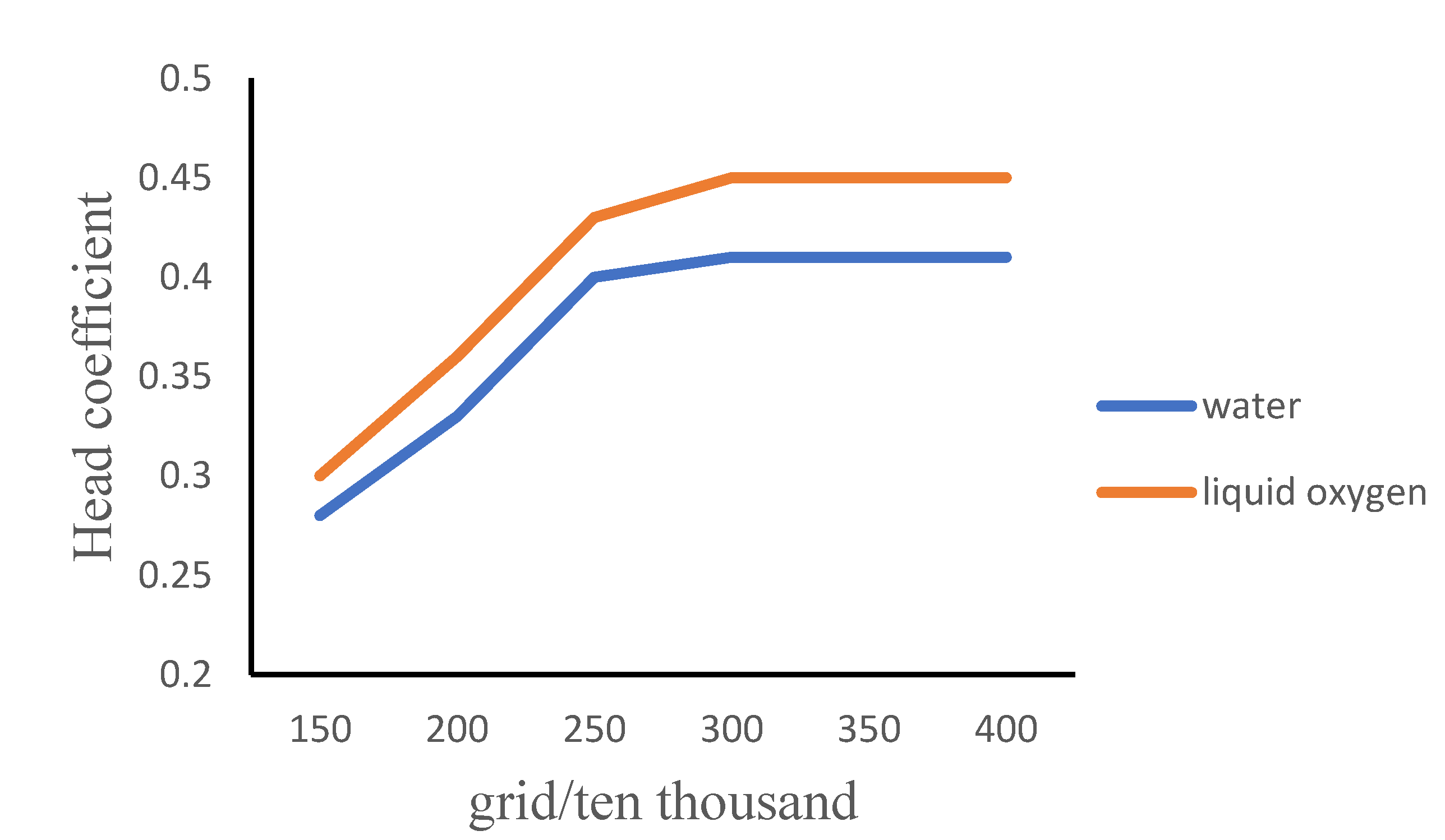
2.7. Grid Independence Verification
2.8. Verification of Numerical Calculation Method
3. Results and Discussion
3.1. Analysis of Steady-State Cavitation Characteristics of Liquid Water at Different Temperatures
3.1.1. Temperature Characteristic Analysis
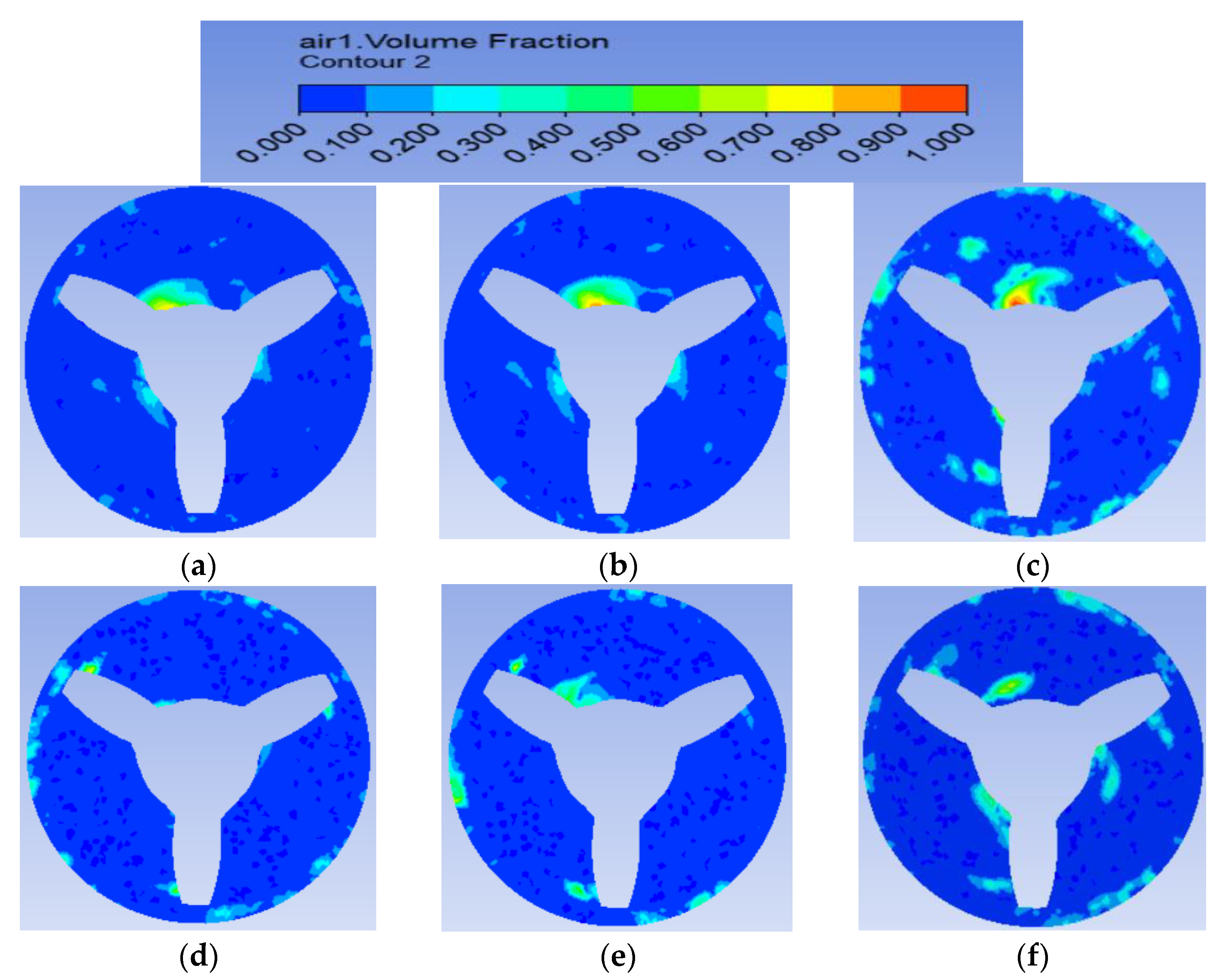
3.1.2. Pressure Characteristic Analysis
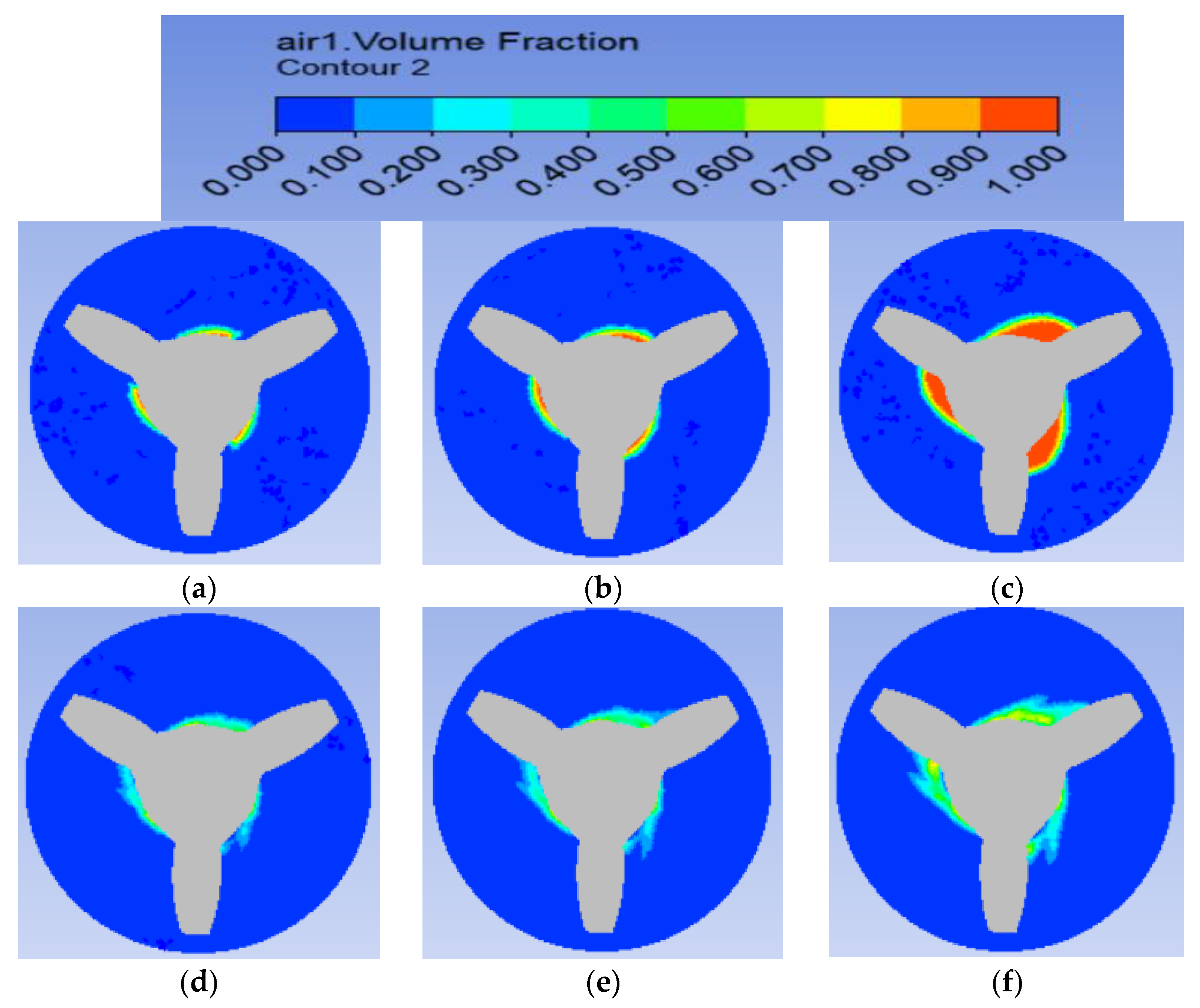
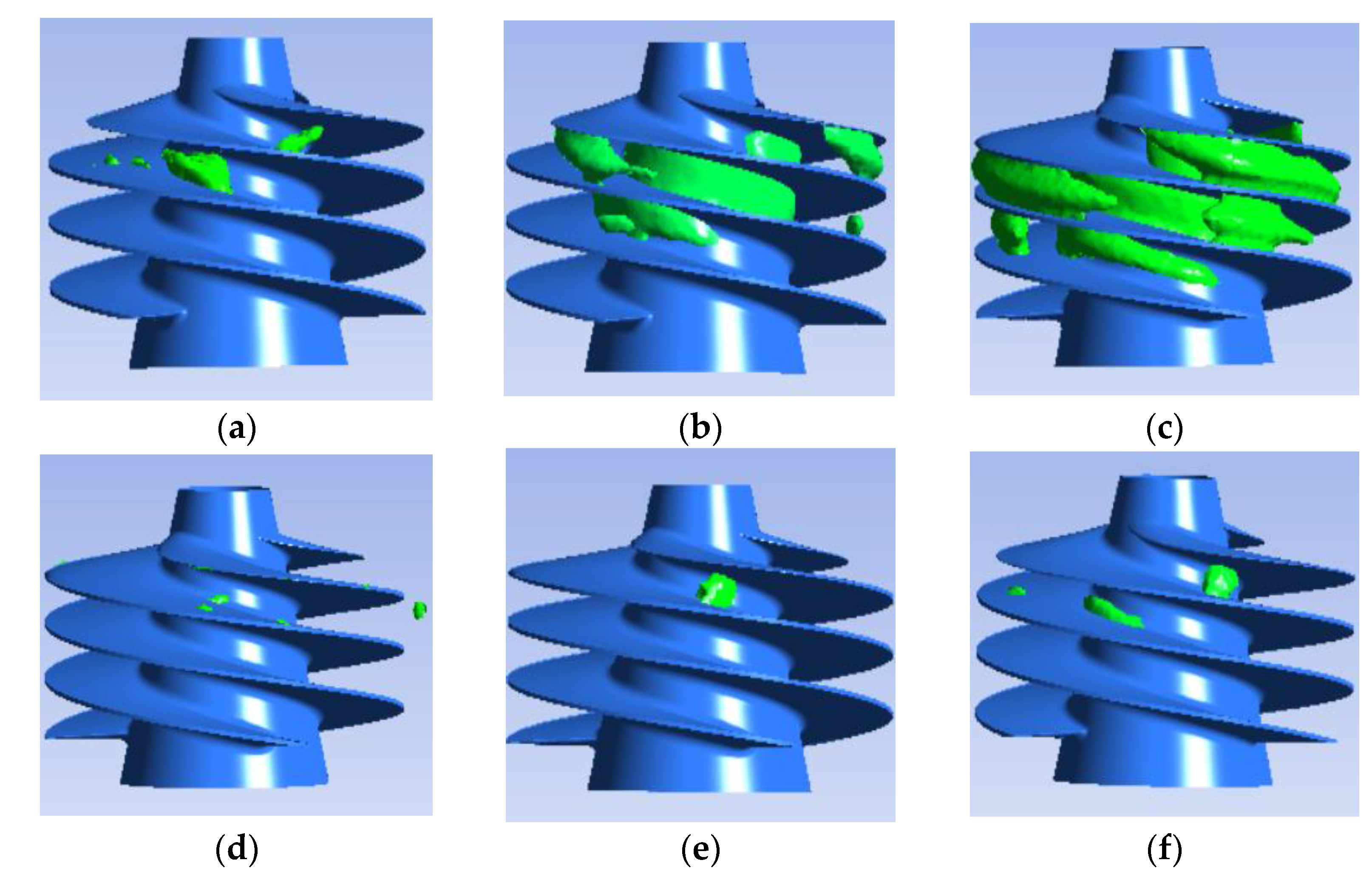
3.1.3. Cavitation and Bubble Characteristic Analysis
3.2. Analysis of Steady-State Cavitation Characteristics of Liquid Oxygen at Different Temperatures
3.2.1. Temperature Characteristic Analysis
3.2.2. Pressure Characteristic Analysis
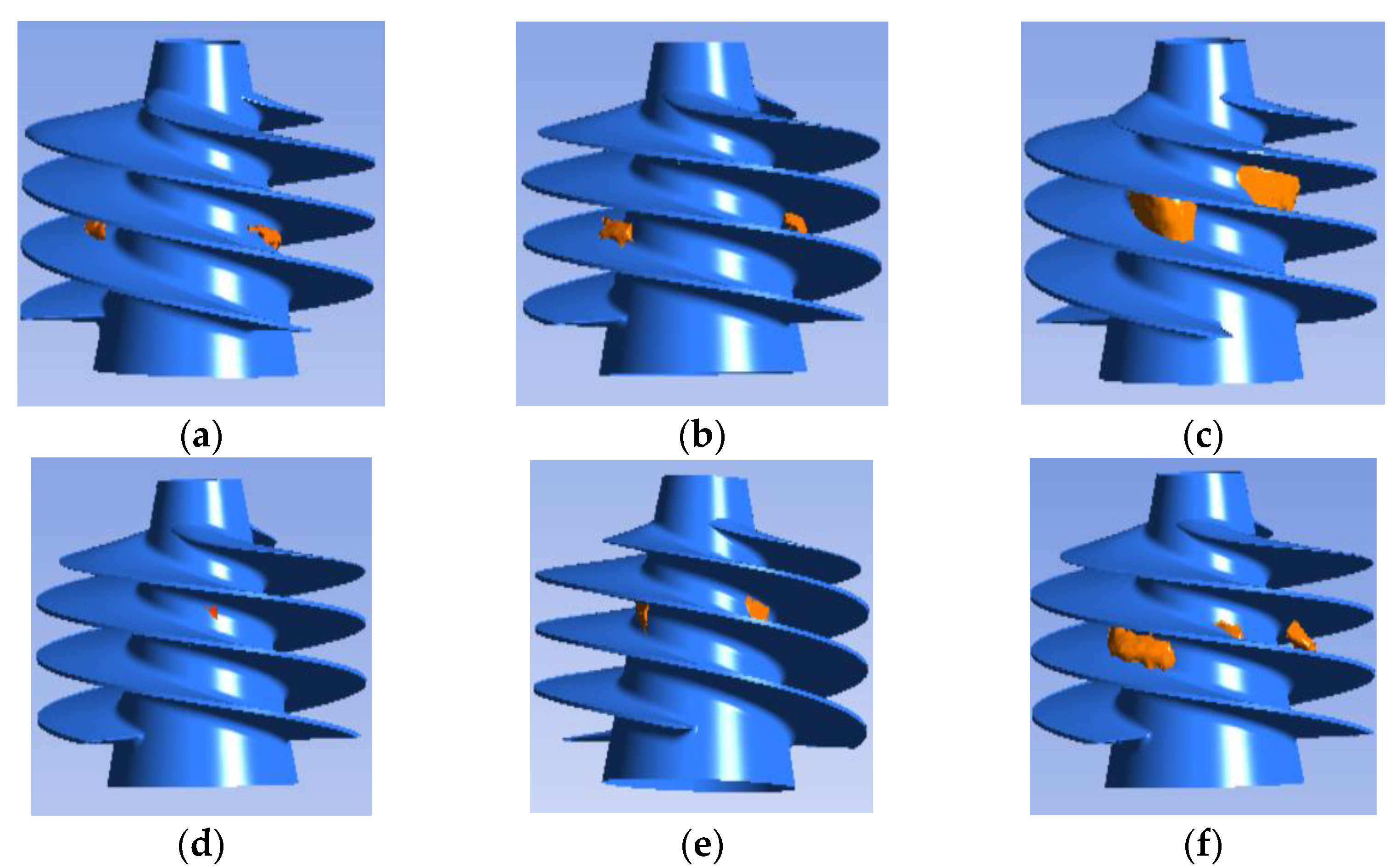
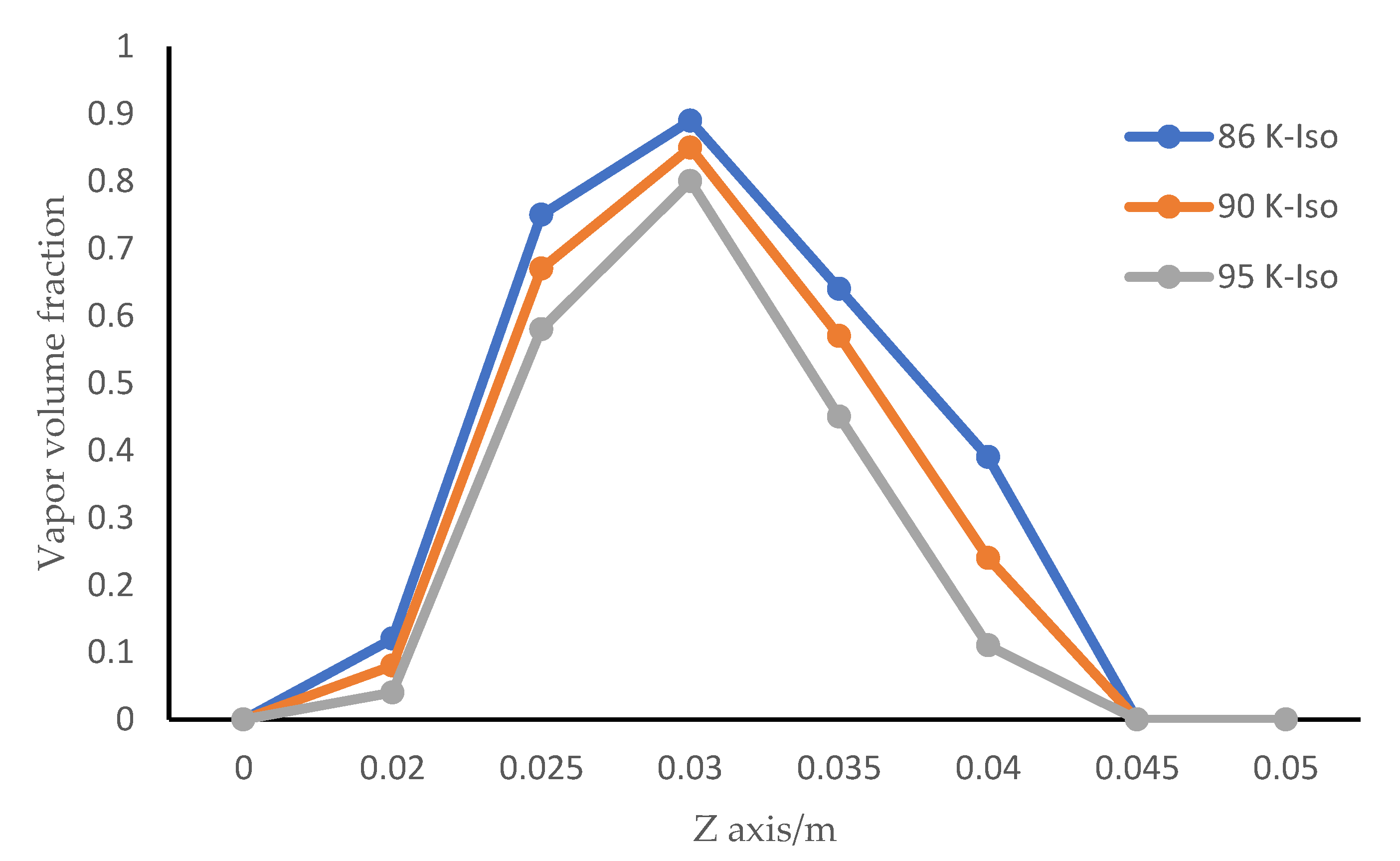
3.2.3. Cavitation and Bubble Characteristic Analysis
4. Conclusions
- The thermodynamic term of the Zwart isothermal cavitation model is modified, mainly considering the influence of the saturated vapor pressure, adding a turbulent kinetic energy term and considering the temperature difference in the cavitation region improved by the B-factor theory. For liquid oxygen in low-temperature medium, the empirical system of an evaporation term and condensation phase is modified.
- For liquid water, when the temperature increases, the liquid water cavitation deepens and the cavitation area becomes wider and bigger, but the position of the cavitation remains unchanged. In the Zwart modified cavitation model, the cavitation level of liquid water will be reduced. This shows that, when the cavitation is serious, the liquid absorbs more gasification latent heat from the cavitation area, and the saturated vapor pressure decreases, so the cavitation is inhibited to a certain extent. When the temperature of liquid water is high, it can show more obvious thermodynamic characteristics.
- For liquid oxygen, when the temperature increases, the temperature difference of liquid oxygen increases, the pressure difference also increases and the corresponding cavitation shape changes more obviously in the larger vapor volume fraction. It can be found that the Zwart modified cavitation model can reflect the thermodynamic effect of liquid oxygen on cavitation. The thermodynamic characteristics of liquid oxygen at different temperatures are obvious, but the higher temperature and more significant cavitation characteristics will inhibit the process of cavitation. Compared with liquid water, the temperature difference characteristics, pressure difference characteristics and cavitation of liquid oxygen change more significantly, which further verifies the thermodynamic characteristics of low-temperature medium liquid oxygen.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kim, D.; Sung, H.; Choi, C.; Kim, J. Cavitation instabilities of an inducer in a cryogenic pump. Acta Astronaut. 2017, 132, 19–24. [Google Scholar] [CrossRef]
- Utturkar, Y.; Wu, J.; Wang, G.; Shyy, W. Recent progress in modeling of cryogenic cavitation for liquid rocket propulsion. Progress Aerosp. Sci. 2005, 41, 558–608. [Google Scholar] [CrossRef]
- Long, X.; Liu, Q.; Ji, B.; Lu, Y. Numerical investigation of two typical cavitation shedding dynamics flow in liquid hydrogen with thermodynamic effects. Int. J. Heat Mass Transf. 2017, 109, 879–893. [Google Scholar] [CrossRef]
- Brennen, C.E. Cavitation and Bubble Dynamics; Oxford University Press: New York, NY, USA, 1995. [Google Scholar]
- Brennen, C.E. Hydrodynamics of Pumps; Oxford University Press: Oxford, UK, 1994. [Google Scholar]
- Chen, T.; Chen, H.; Liang, W.; Huang, B.; Xiang, L. Experimental investigation of liquid nitrogen cavitating flows in converging-diverging nozzle with special emphasis on thermal transition. Int. J. Heat Mass Transf. 2019, 132, 618–630. [Google Scholar] [CrossRef]
- Hord, J.; Anderson, L.M.; Hall, W.J. Cavitation in Liquid Cryogens I—Venturi; NASA Contractor Report, NASA CR-2045; NASA: Washington, DC, USA, 1972. [Google Scholar]
- Hord, J. Cavitation in Liquid Cryogens II—Hydrofoil; NASA Contractor Report, NASA CR-2156; NASA: Washington, DC, USA, 1973. [Google Scholar]
- Hord, J. Cavitation in Liquid Cryogens III—Ogives; NASA Contractor Report, NASA CR-2242; NASA: Washington, DC, USA, 1973. [Google Scholar]
- Merkle, C.L.; Feng, J.; Buelow, P.E.O. Computational modeling of dynamics of sheet cavitation. In Proceedings of the 3rd International Symposium on Cavitation, Grenoble, France, 7–10 April 1998. [Google Scholar]
- Singhal, A.K.; Athavale, M.M.; Li, H.; Jiang, Y. Mathematical basis and validation of the full cavitation model. J. Fluids Eng. 2002, 124, 617–624. [Google Scholar] [CrossRef]
- Kunz, R.F.; Boger, D.A.; Stinebring, D.R.; Chyczewski, T.S.; Lindau, J.W.; Gibeling, H.J.; Venkateswaran, S.; Govindan, T. A preconditioned Navier-Stokes method for two-phase flows with application to cavitation prediction. Comput. Fluids 2000, 29, 849–875. [Google Scholar] [CrossRef]
- Zwart, P.J.; Gerber, A.G.; Belamri, T. A two-phase flow model for predicting cavitation dynamics. In Proceedings of the Fifth International Conference on Multiphase Flow, Yokohama, Japan, 30 May–3 June 2004. [Google Scholar]
- Goncalvès, E.; Fortes Patella, R.; Rolland, J.; Pouffary, B.; Challier, G. Thermodynamic effect on a cavitating inducer in liquid hydrogen. J. Fluids Eng. 2010, 132, 111305. [Google Scholar] [CrossRef]
- Ji, B.; Luo, X.W.; Wu, Y.L.; Zhang, Y.; Xu, H.Y. Simulation of high temperature water cavitation considering thermodynamic effect. Tsinghua Univ. J. Nat. Sci. 2010, 50, 262–265. [Google Scholar]
- Xu, B.; Feng, J.; Shen, X.; Zhang, D.; Zhang, W. Numerical investigation of cavitation suppression in an inducer for water and liquid nitrogen with emphasis on thermodynamic effect. J. Braz. Soc. Mech. Sci. Eng. 2021, 43, 212. [Google Scholar] [CrossRef]
- Tseng, C.; Shyy, W. Modeling for isothermal and cryogenic cavitation. Int. J. Heat Mass Transf. 2010, 53, 513–525. [Google Scholar] [CrossRef]
- Li, W.Q. Study on Cavitation Mechanism of Engine Cooling Water Pump under Thermodynamic Effect; Jiangsu University: Zhenjiang, China, 2016. [Google Scholar]
- Franc, J.P.; Rebattet, C.; Coulon, A. An Experimental investigation of thermal effects in a cavitating inducer. In Proceedings of the Fifth International Symposium on Cavitation (Cav2003), Osaka, Japan, 1–4 November 2003. [Google Scholar]
- Ruggeri, R.S.; Moore, R.D. Method of Prediction of Pump Cavitation Performance for Various Liquids, Liquid Temperatures and Rotation Speeds; NASA Technical Note, NASA TN D-5292; NASA: Washington, DC, USA, 1969. [Google Scholar]
- Franc, J.P.; Janson, E.; Morel, P.; Rebattet, C.; Riondet, M. Visualizations of leading edge cavitation in an inducer at different temperatures. In Proceedings of the Fourth International Symposium on Cavitation, Pasadena, NL, Canada, 20–23 June 2001; Volume 1, pp. 24–130. [Google Scholar]
- Sonntag, R.E.; Borgnakke, C.; Wylen, G.J. Fundamentals of Thermodynamics; John Wiley and Sons, Inc.: Hoboken, NJ, USA, 2004. [Google Scholar]
- Tani, N.; Yanabushi, N.; Tsujimoto, Y. Influence of flow coefficient and flow structure on rotational cavitation in inducer. J. Fluids Eng. 2012, 134, 021302. [Google Scholar] [CrossRef]
- Yu, A.; Tang, Q.; Zhou, D. Entropy production analysis in thermodynamic cavitating flow with the consideration of local compressibility. Int. J. Heat Mass Transf. 2020, 153, 119604. [Google Scholar] [CrossRef]



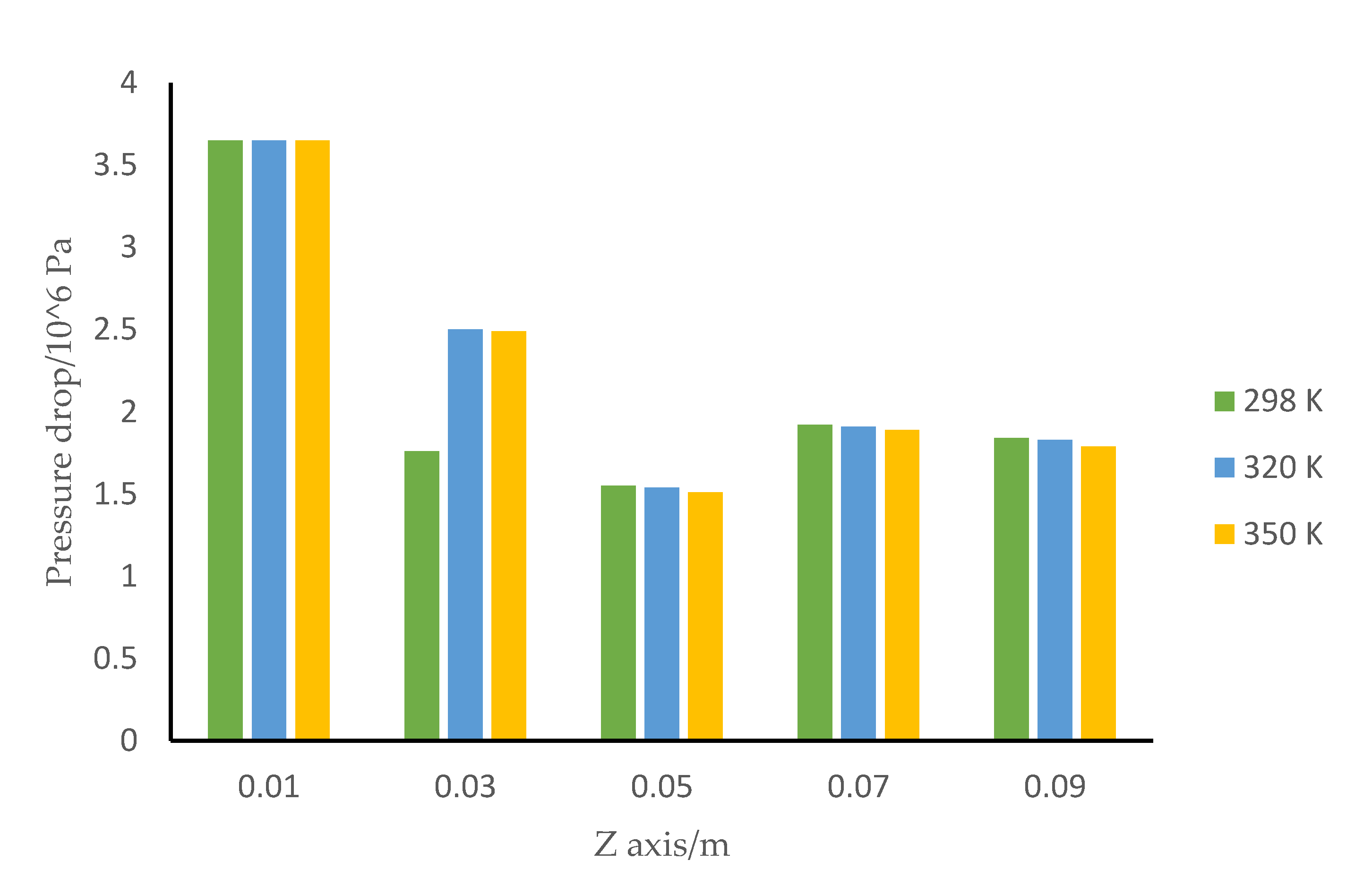
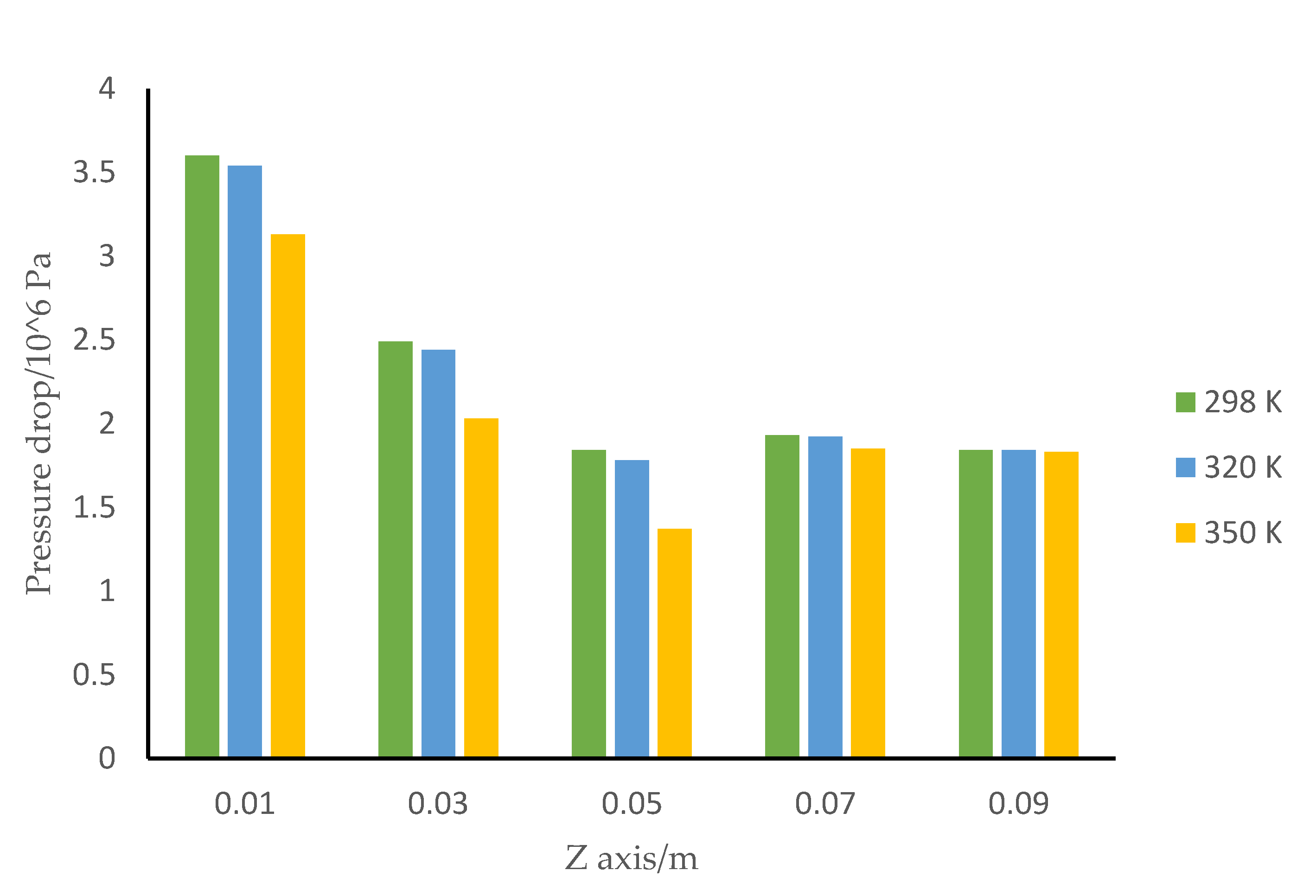

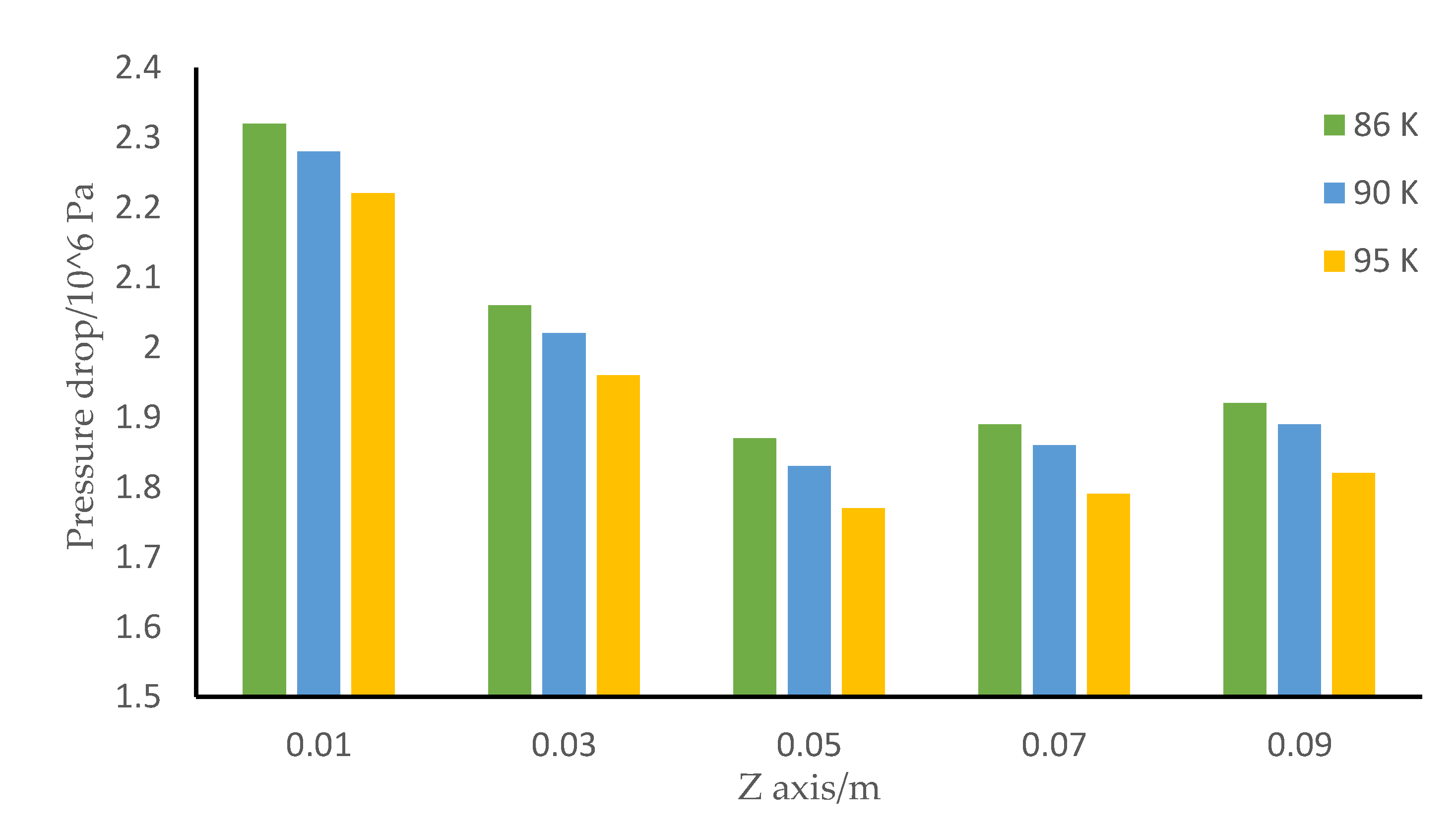

| Fluid | Temperature (°C) | A | B | C |
|---|---|---|---|---|
| Liquid water | 0~100 | 8.07131 | 1730.63 | 233.426 |
| Liquid oxygen | −210~−160 | 6.98983 | 370.757 | 273.200 |
| Parameter | Unit | Liquid Water | Liquid Oxygen |
|---|---|---|---|
| Mass flow | kg/s | 250 | 130 |
| Temperature | K | 298 | 90 |
| Inlet pressure | MPa | 0.2 | 0.2 |
| Speed | r/min | 5000 | 15,000 |
| Outlet pressure | MPa | 2.0 | 2.0 |
| Flow state | - | Steady | Steady |
| Fluid | Temperature (K) | Mass Flow (kg/s) | Efficiency | Outlet Pressure (MPa) |
|---|---|---|---|---|
| Liquid water | 298 | 250 | 0.72 | 2.0 |
| Liquid oxygen | 90 | 130 | 0.72 | 2.0 |
| Fluid | Cavitation Model | Rated Efficiency | Computational Efficiency | Difference |
|---|---|---|---|---|
| Liquid water | Iso-Zwart | 0.72 | 0.679 | 5.69% |
| Liquid water | Mod-Zwart | 0.72 | 0.745 | 3.47% |
| Liquid oxygen | Iso-Zwart | 0.72 | 0.685 | 4.86% |
| Liquid oxygen | Mod-Zwart | 0.72 | 0.735 | 1.67% |
| Temperature T(K) | Liquid Density (kg m−3) | Gas Density (kg m−3) | Latent Heat (J kg−1) | Specific Heat Capacity (J kg−1 K) |
|---|---|---|---|---|
| 298 | 996.9 | 0.0234 | 2,420,400 | 4182 |
| 320 | 989.4 | 0.0717 | 2,389,500 | 4181 |
| 350 | 937.7 | 0.2603 | 2,315,900 | 4195 |
| Temperature T(K) | Liquid Density (kg m−3) | Gas Density (kg m−3) | Latent Heat (J kg−1) | Specific Heat Capacity (J kg−1 K) |
|---|---|---|---|---|
| 86 | 1162 | 2.927 | 217,010 | 1690 |
| 90 | 1142 | 4.387 | 213,240 | 1699 |
| 95 | 1117 | 6.920 | 208,160 | 1715 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, G.; Wei, Y.; Liu, S. Cavitation Flow Characteristics of Water and Liquid Oxygen in the Inducer Considering Thermodynamic Effect. Energies 2022, 15, 4943. https://doi.org/10.3390/en15144943
Shi G, Wei Y, Liu S. Cavitation Flow Characteristics of Water and Liquid Oxygen in the Inducer Considering Thermodynamic Effect. Energies. 2022; 15(14):4943. https://doi.org/10.3390/en15144943
Chicago/Turabian StyleShi, Guangyuan, Yuan Wei, and Shulin Liu. 2022. "Cavitation Flow Characteristics of Water and Liquid Oxygen in the Inducer Considering Thermodynamic Effect" Energies 15, no. 14: 4943. https://doi.org/10.3390/en15144943
APA StyleShi, G., Wei, Y., & Liu, S. (2022). Cavitation Flow Characteristics of Water and Liquid Oxygen in the Inducer Considering Thermodynamic Effect. Energies, 15(14), 4943. https://doi.org/10.3390/en15144943






