Modeling of Passive and Forced Convection Heat Transfer in Channels with Rib Turbulators
Abstract
:1. Introduction
2. Materials and Methods
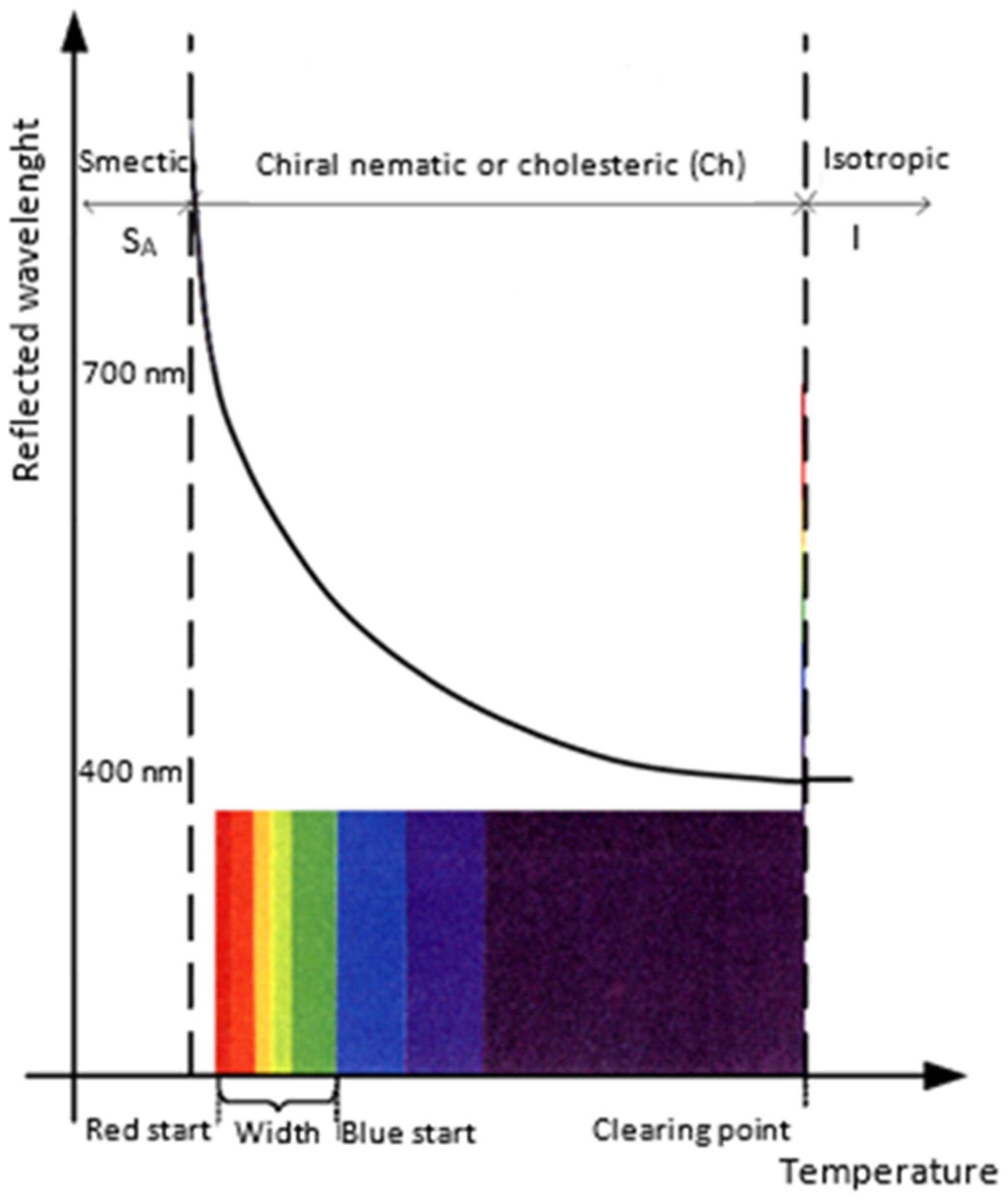
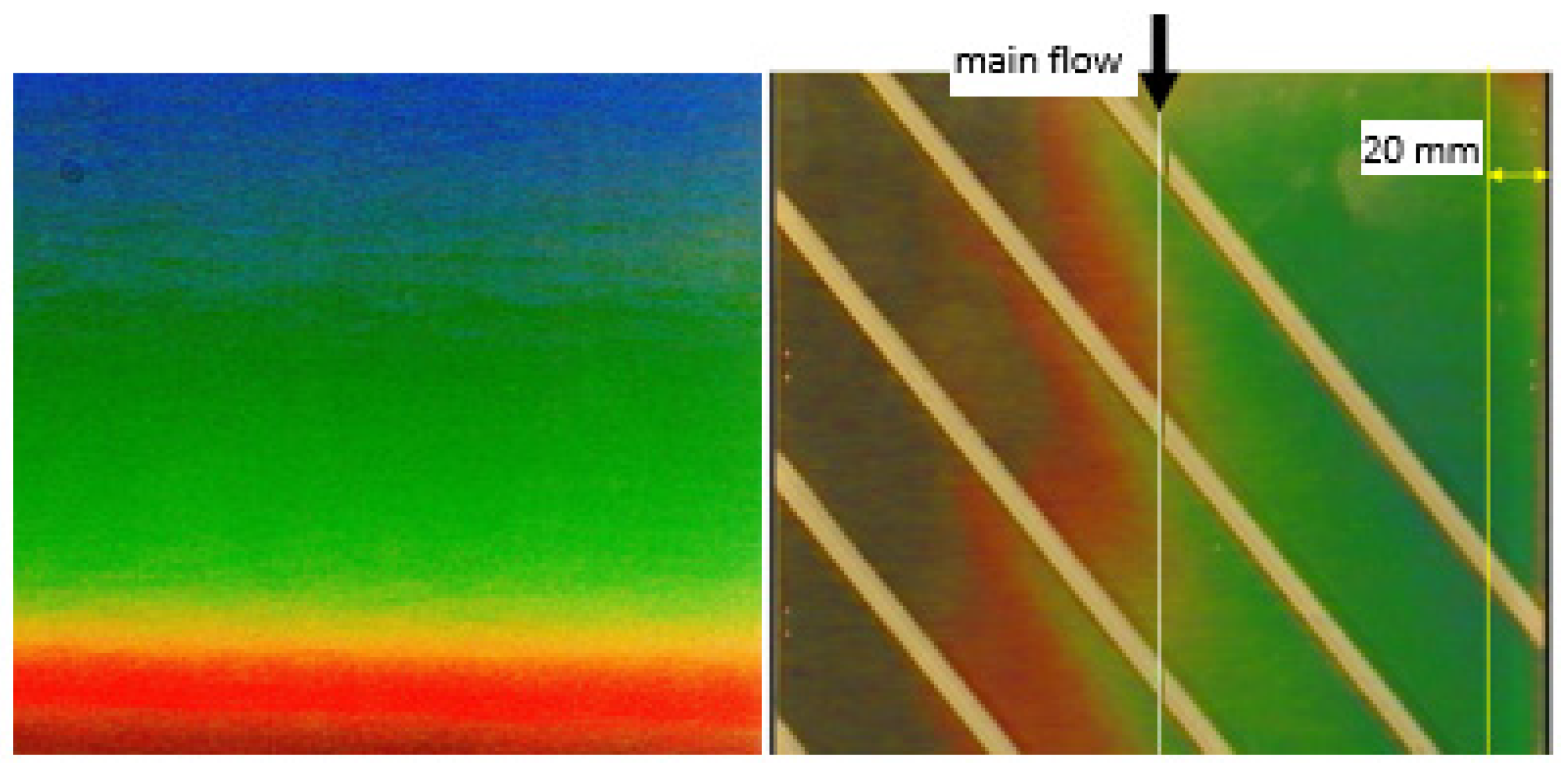
2.1. Liquid Crystals as a Temperature Meter
- By applying the liquid crystal to the test object as an alloy or solution in a suitable solvent,
- By using the encapsulated forms in the coated (printed) sheets or slurries.
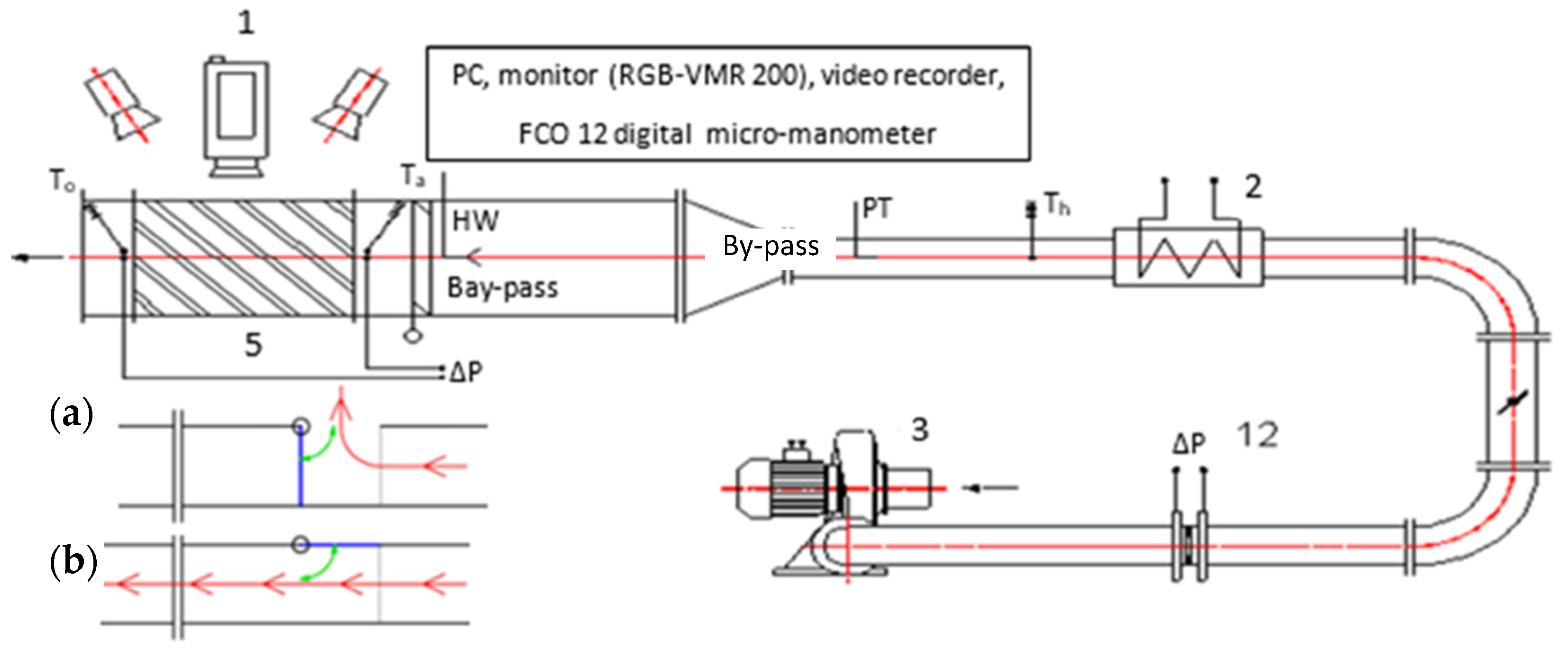
2.2. Experimental Stand
- (1)
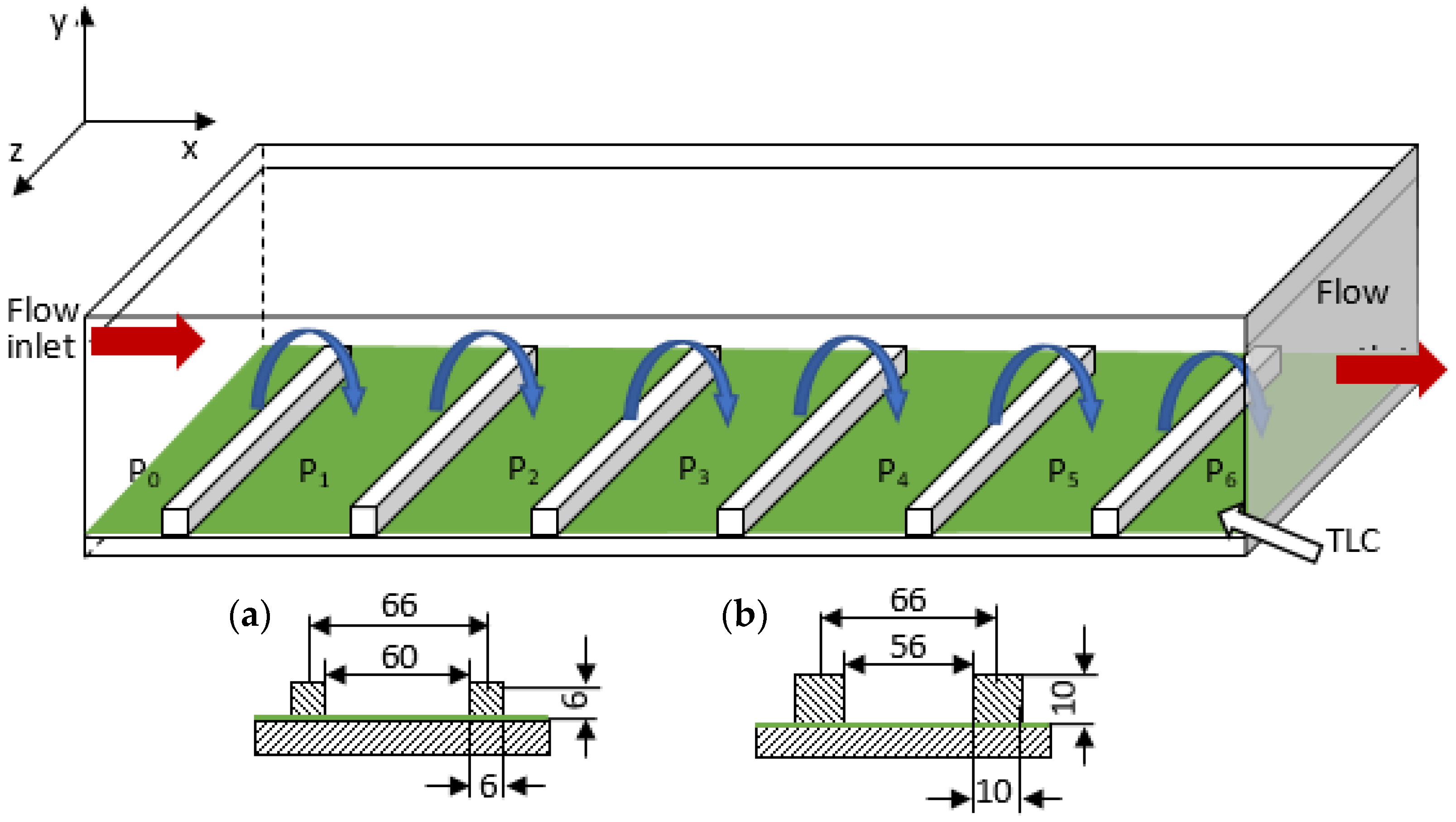
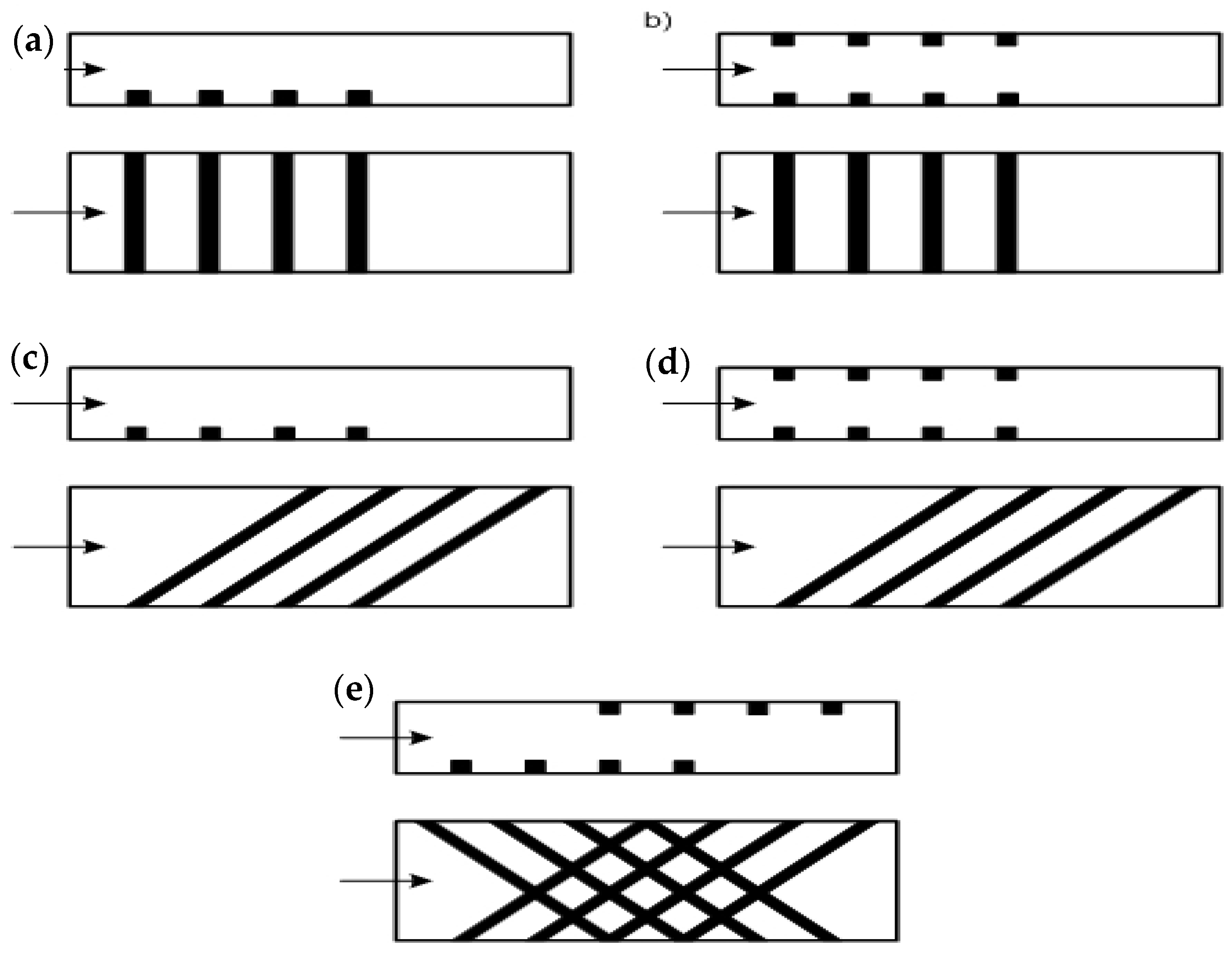
- configuration_1 (6 mm straight bottom): only the bottom wall of the duct ribbed with six ribs 6 mm wide and square cross-section; ribs perpendicular to the direction of incoming air; distance between rib centers: 66 mm (60 mm between rib edges), P/e = 11;
- (2)
- configuration_2 (6 mm straight bottom-up): bottom and top wall of the duct ribbed with six ribs (each wall) 6 mm wide and square; ribs perpendicular to the direction of incoming air; distance between rib centers: 66 mm (60 mm between rib edges) P/e = 11;
- (3)
- configuration_3 (6 mm 45°): only the bottom wall of the duct ribbed with six ribs 6 mm wide and square; ribs at an angle of 45° to the direction of incoming air; distance between rib centers: 66 mm (60 mm between rib edges) P/e = 11;
- (4)
- configuration_4 (6 mm 45°–45°): bottom and top wall of the duct ribbed with six ribs (each wall) 6 mm wide and square; ribs at an angle of 45° to the direction of incoming air and parallel to each other; distance between rib centers: 66 mm (60 mm between rib edges) pseudo laminar P/e = 11;
- (5)
- configuration_5 (6 mm 45°–135°): lower and upper duct wall ribbed with six ribs (each wall) 6 mm wide and square; ribs at an angle of 45° to the direction of incoming air and perpendicular to each other; distance between rib centers: 66 mm (60 mm between rib edges) P/e = 11;
- (6)
- configuration_6 (10 mm straight bottom): only the bottom wall of the duct ribbed with six ribs 10 mm wide and square in cross-section, ribs arranged perpendicular to the direction of incoming air; distance between rib centers: 66 mm (56 mm between rib edges) P/e = 6.6;
- (7)
- configuration_7 (10 mm straight bottom-up): bottom and top wall of the duct ribbed with six ribs (each wall) with a width of 10 mm and a square cross-section; ribs perpendicular to the direction of incoming air; distance between rib centers: 66 mm (56 mm between rib edges) P/e = 6.6;
- (8)
- configuration_8 (10 mm 45°): only the bottom wall of the duct ribbed with six ribs 10 mm wide and square; ribs at an angle of 45° to the direction of incoming air; distance between rib edges: 66 mm (56 mm between rib edges) P/e = 6.6;
- (9)
- configuration_9 (10 mm 45°–45°): bottom and top wall of the duct ribbed with six ribs (each wall) with a width of 10 mm and a square cross-section; ribs at an angle of 45° to the direction of incoming air and parallel to each other; distance between rib edges: 66 mm (56 mm between rib edges) P/e = 6.6;
- (10)
- configuration_10 (10 mm 45°–135°): bottom and top wall of the duct ribbed with six ribs (each wall) with a width of 10 mm and a square cross-section; ribs at an angle of 45° to the direction of incoming air and perpendicular to each other; distance between rib edges: 66 mm (56 mm between rib edges) P/e = 6.6.
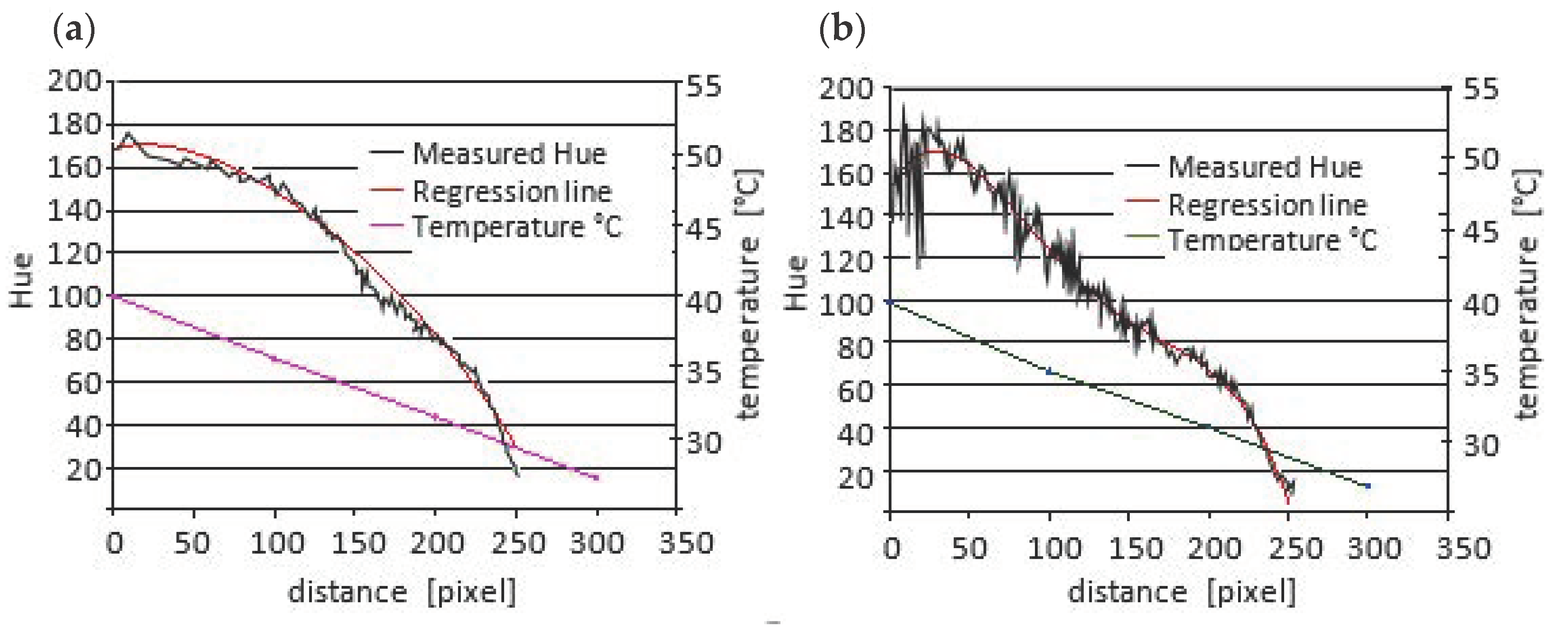
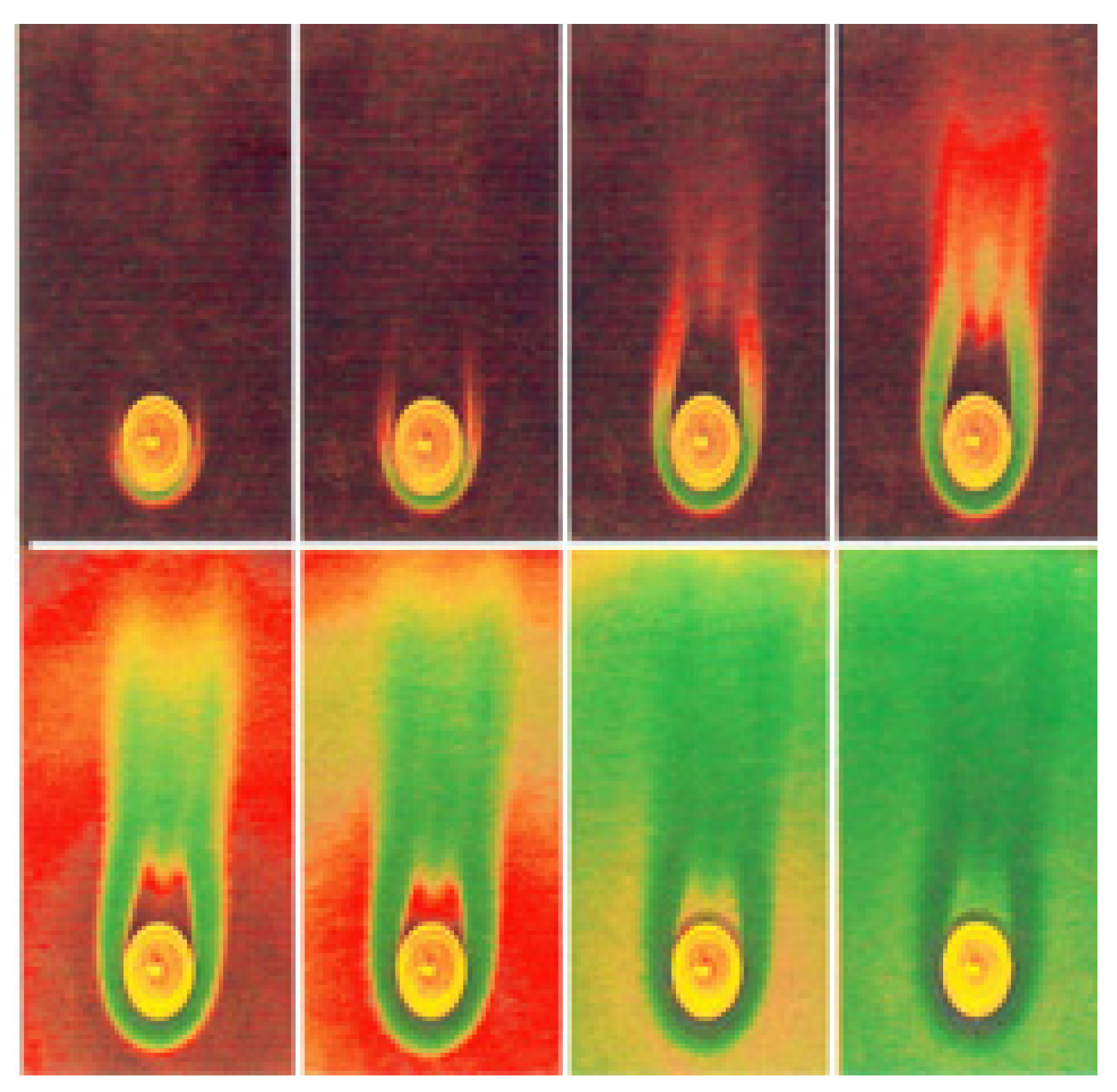
2.3. Calibration of Liquid Crystal-Coated Sheets
2.4. Steady State Analysis by the Constant Heat Flux Method
- Qel—is the measured input power to the plate heater,
- Qhal— is the heat supplied by halogen lighting,
- Qlos— is the heat losses to the environment by free convection,
- Qrad— is the radiative heat transfer rate to the surroundings.
2.5. Transient Method Analysis
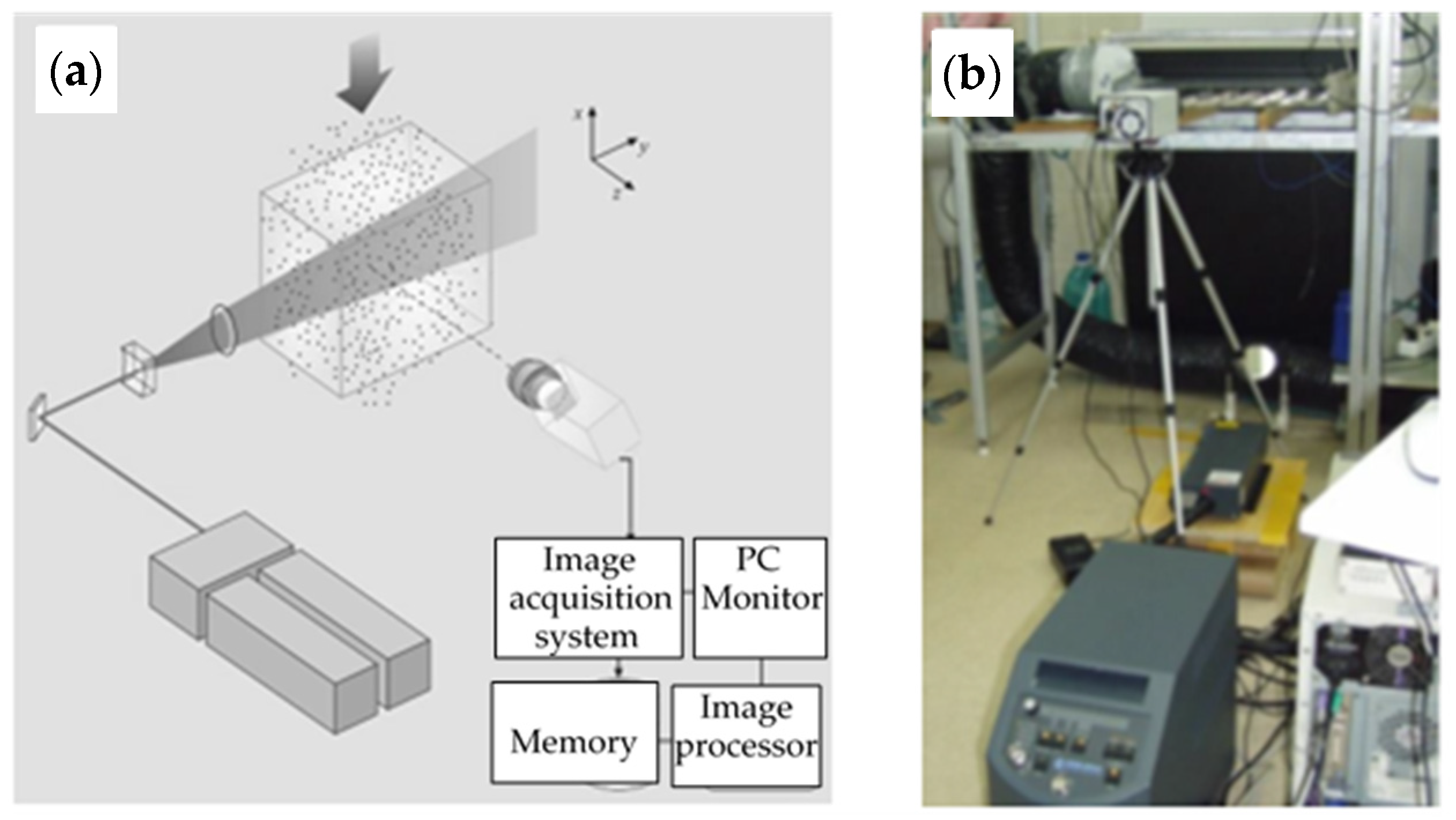
2.6. Particle Image Velocimetry Anemometry
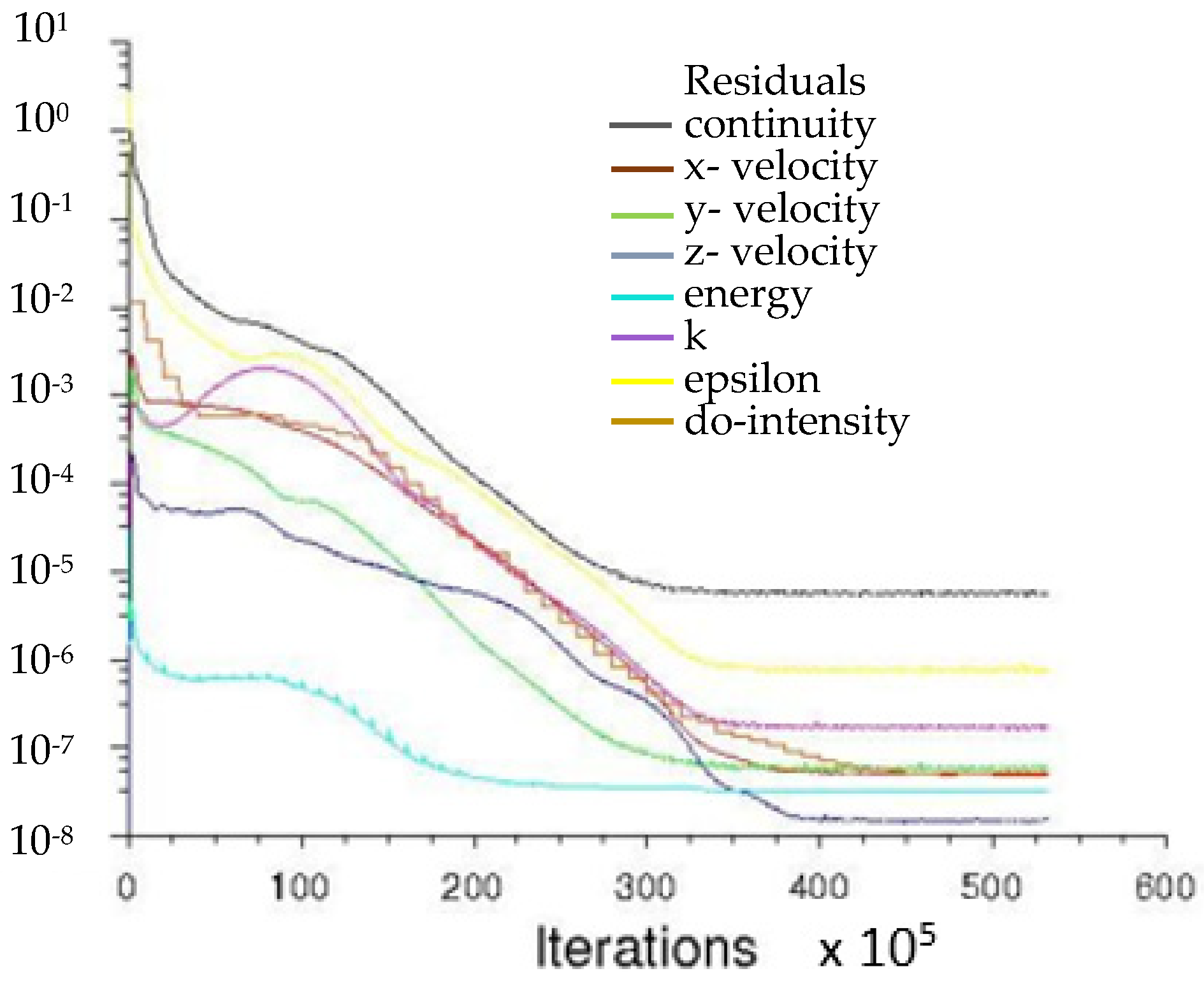
2.7. Computational Fluids Dynamics—CFD
3. Results
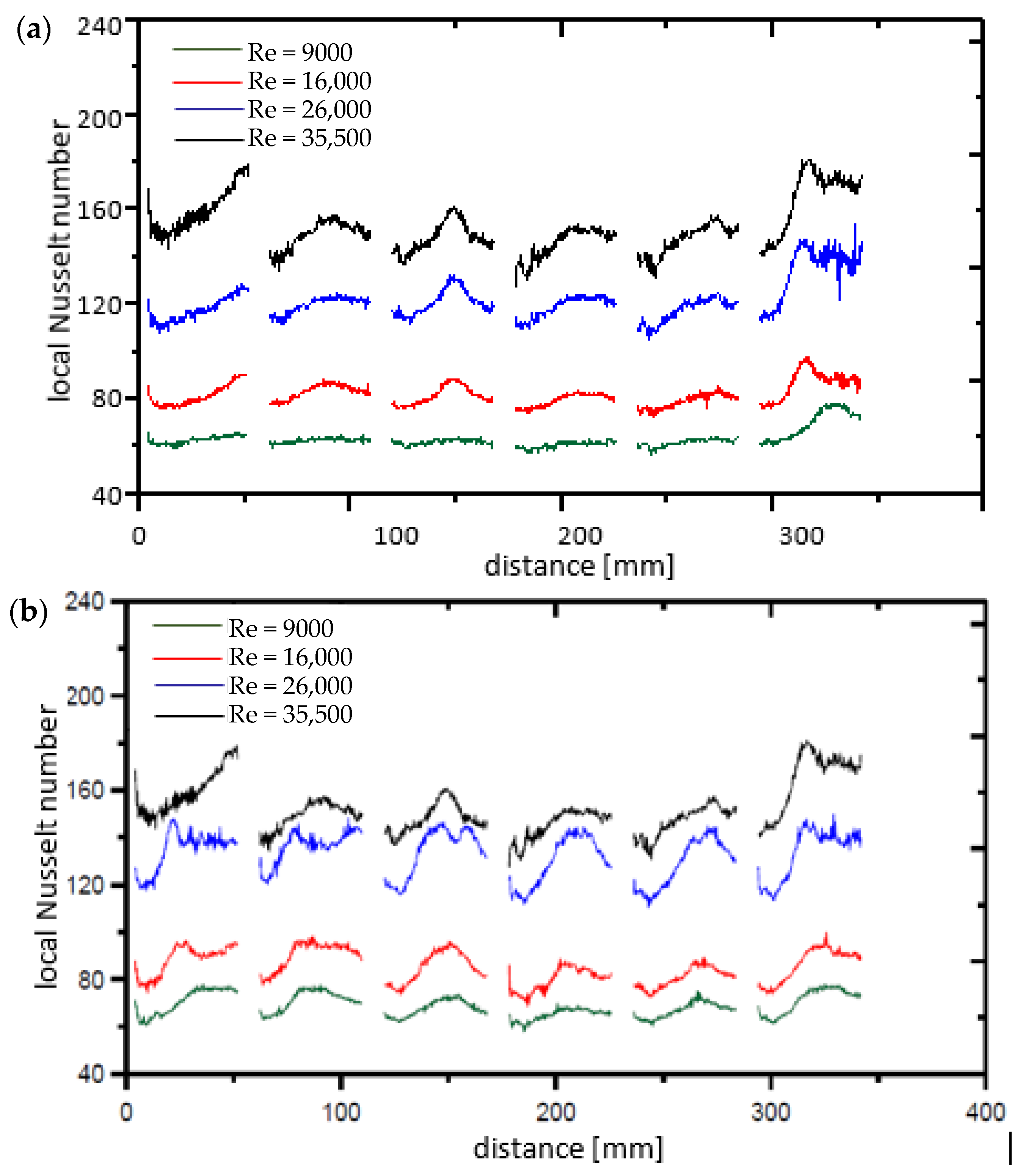
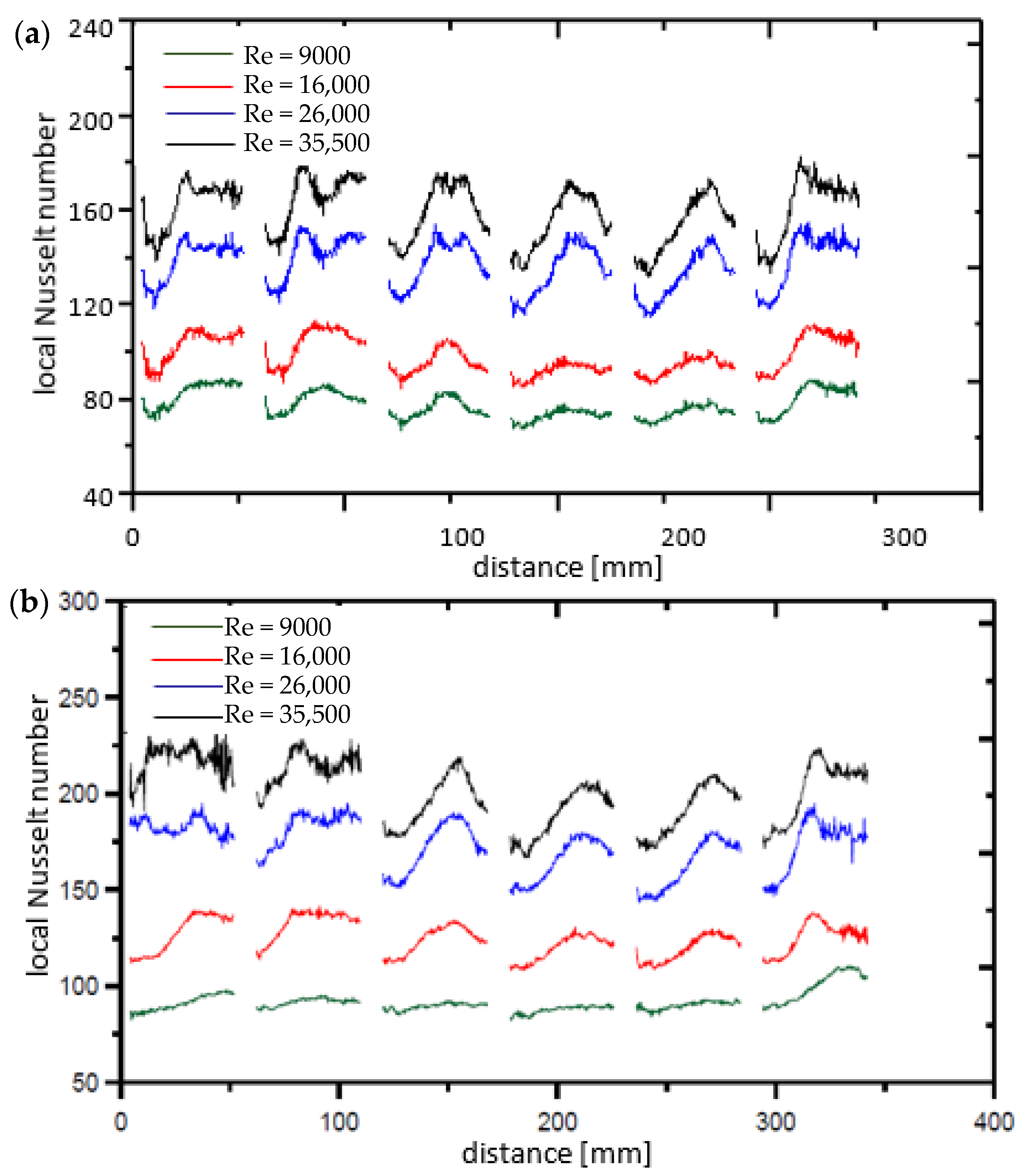
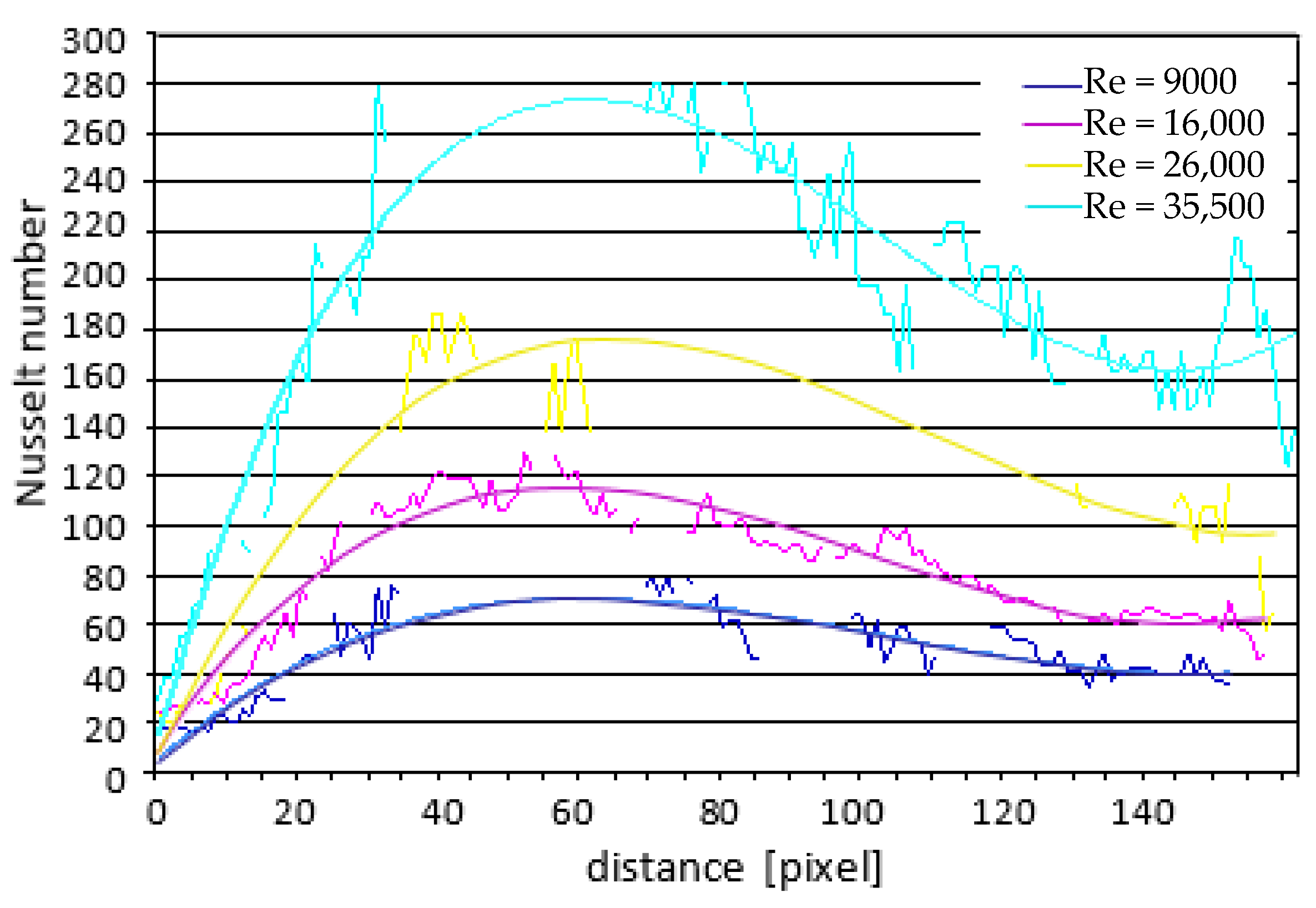
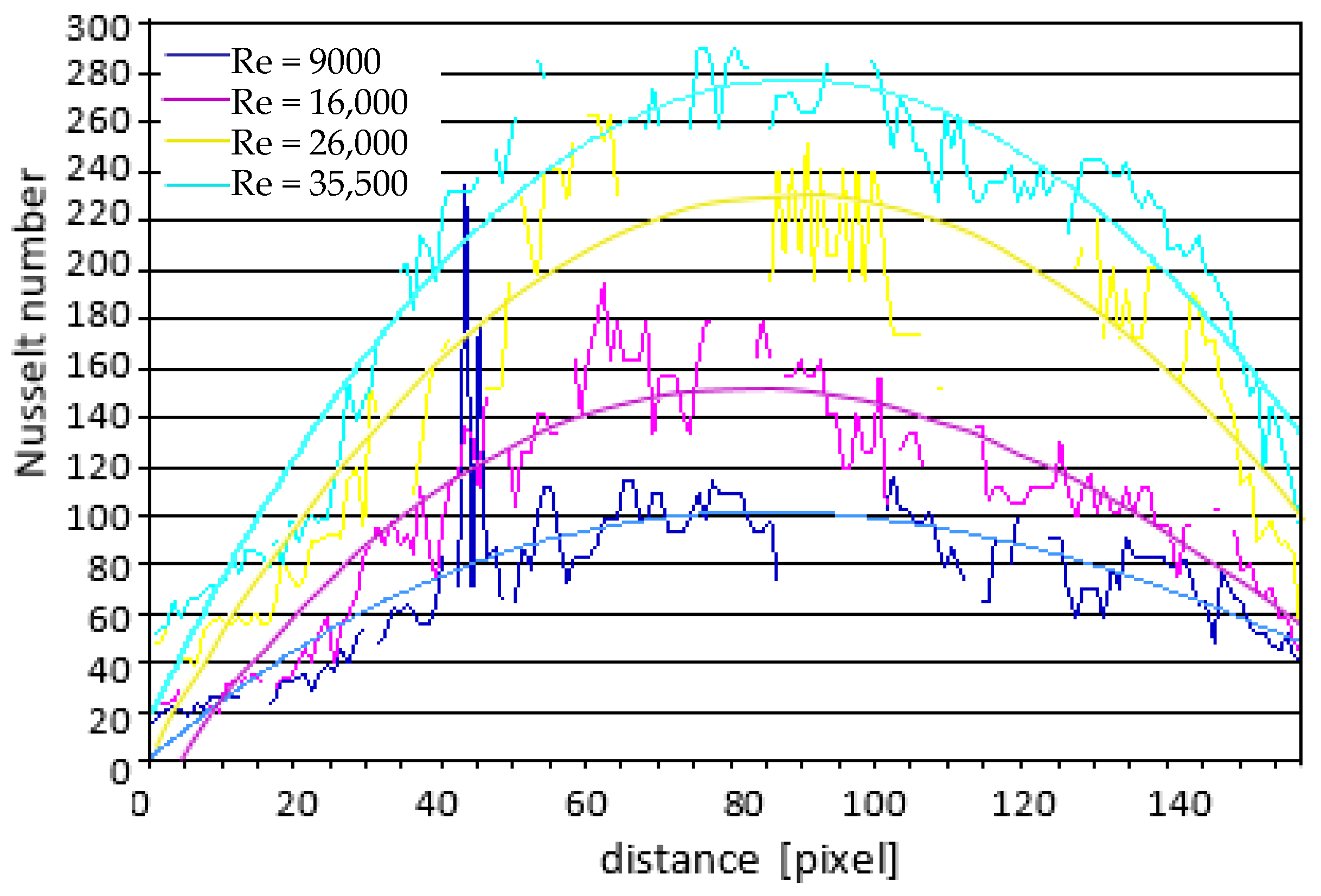
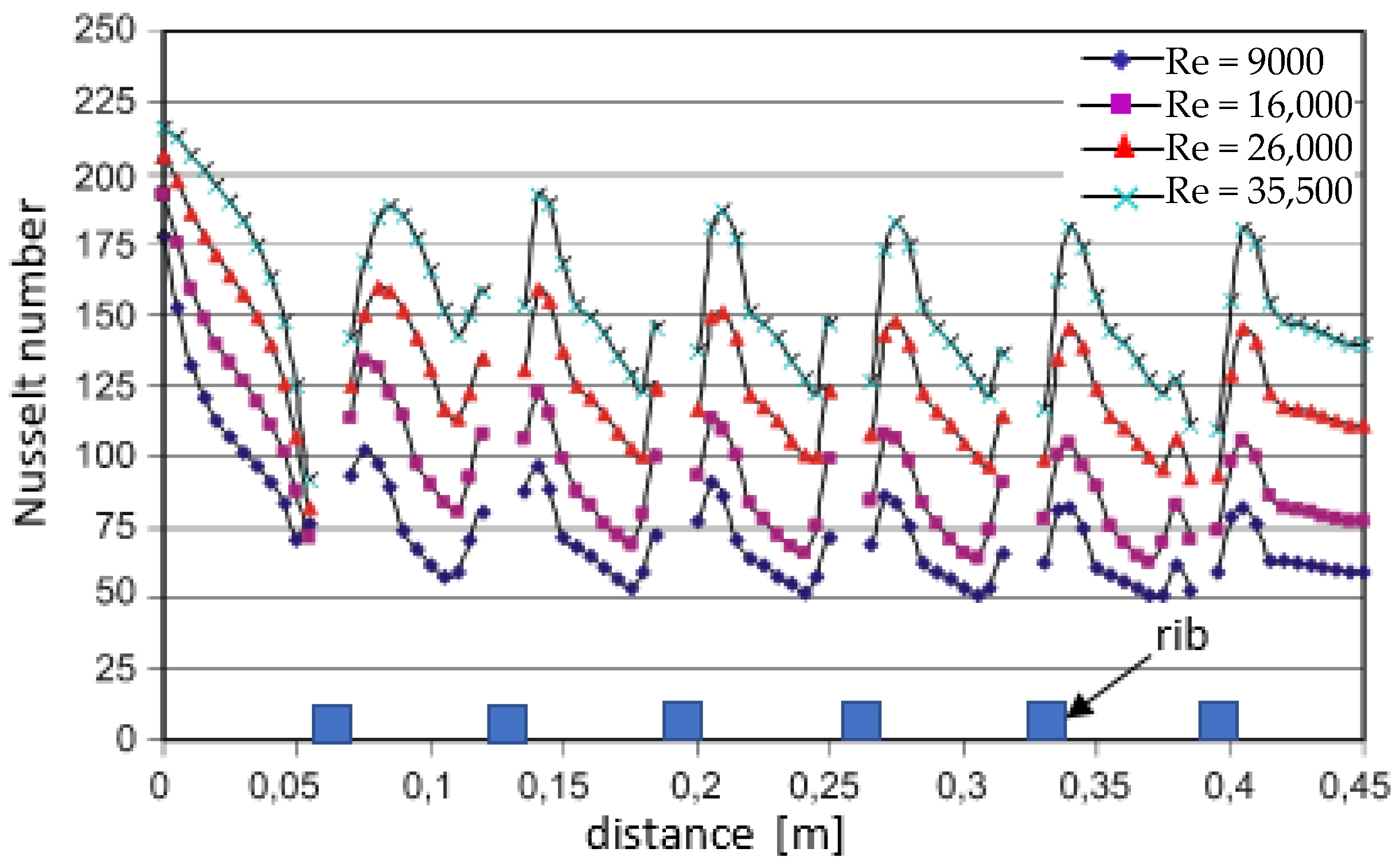
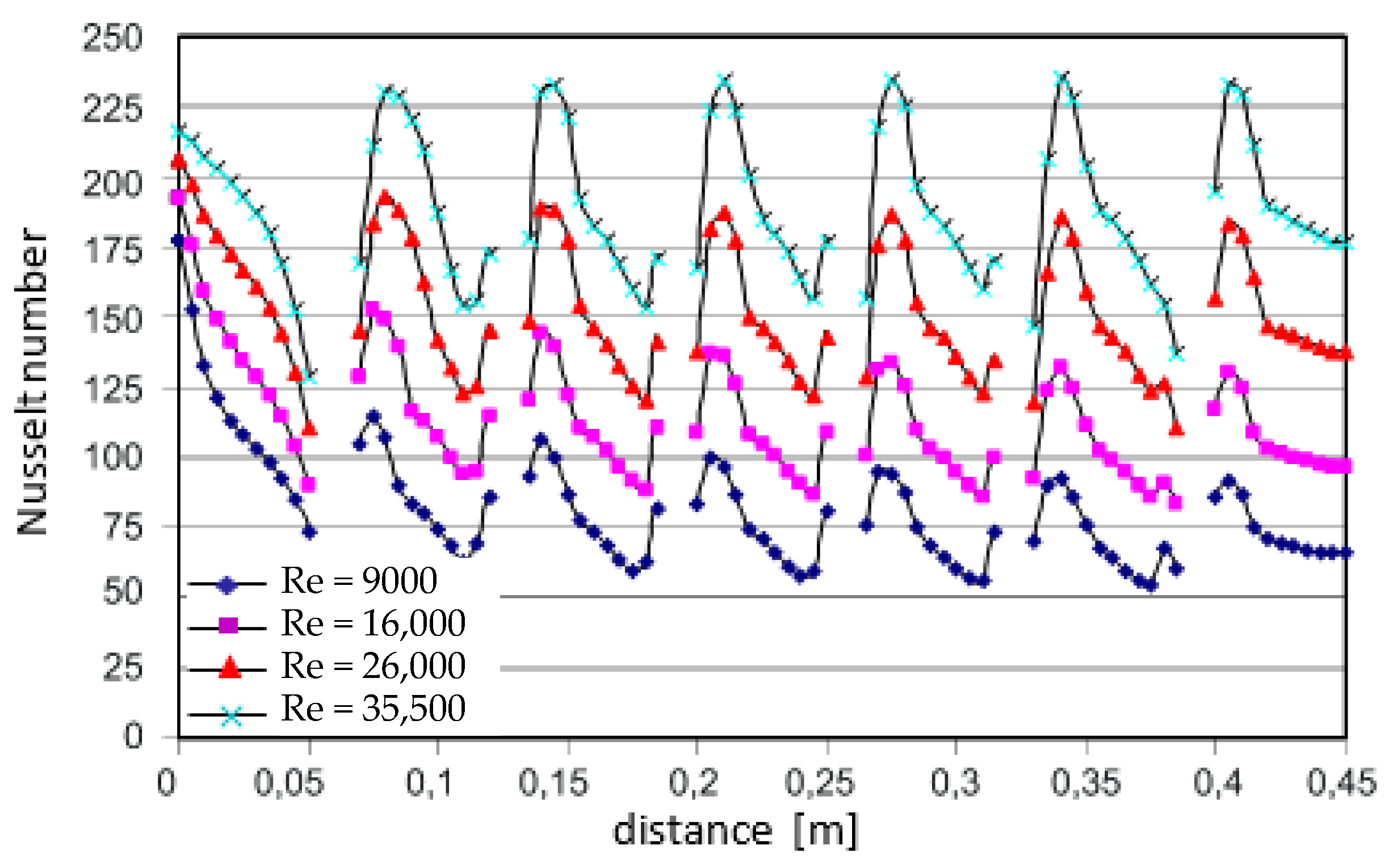
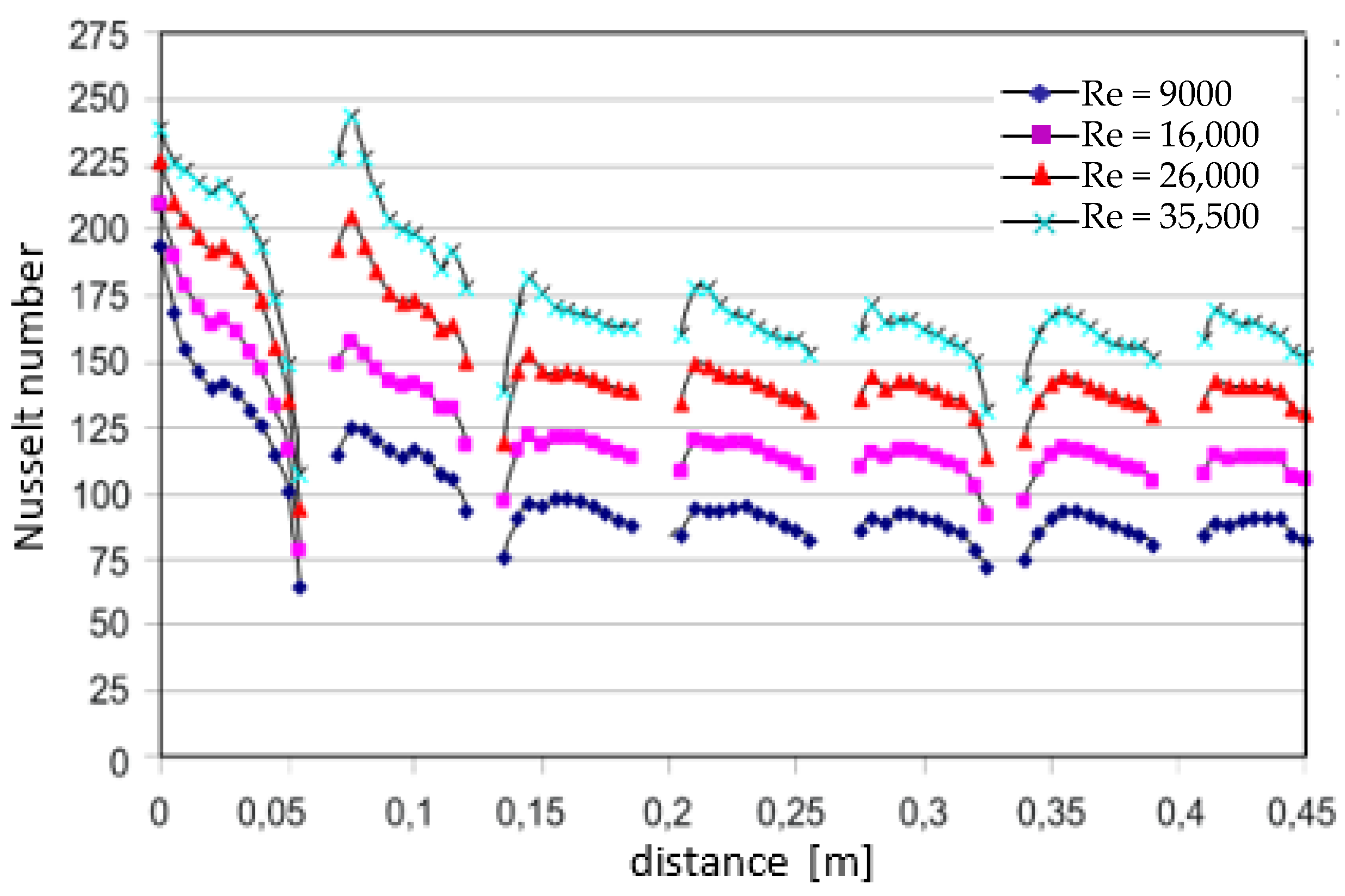
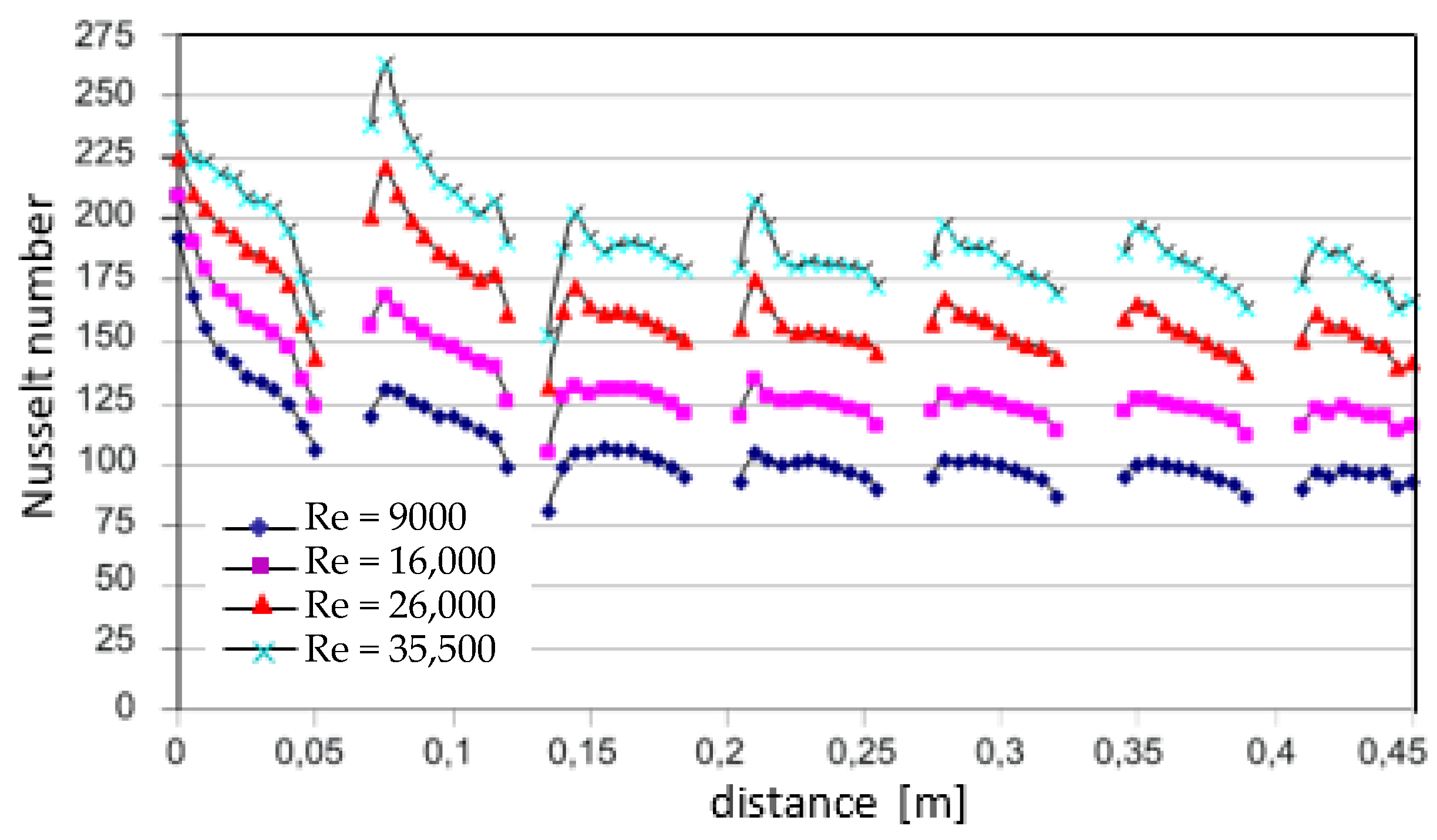
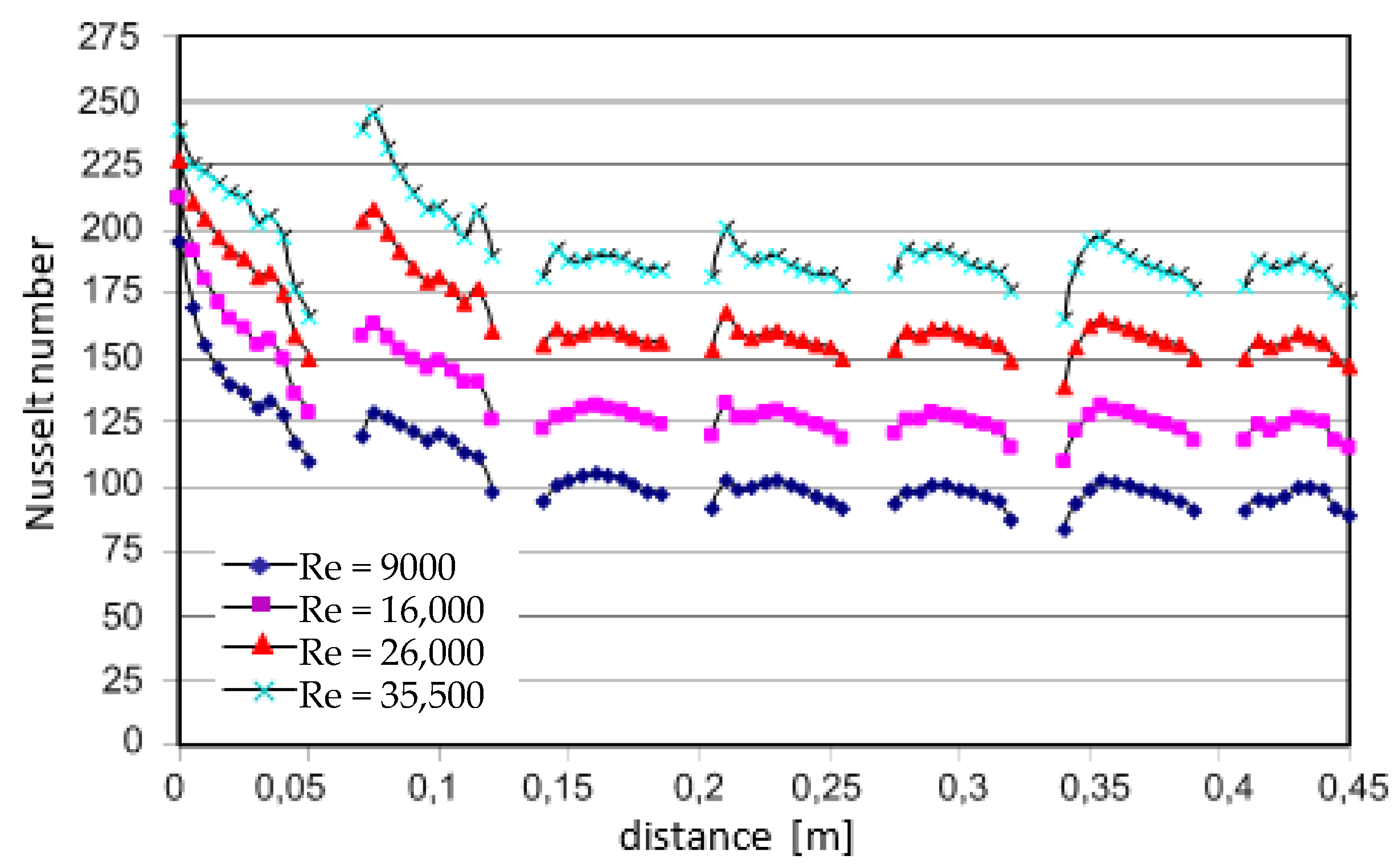
3.1. Experimental Results by the Steady States Method
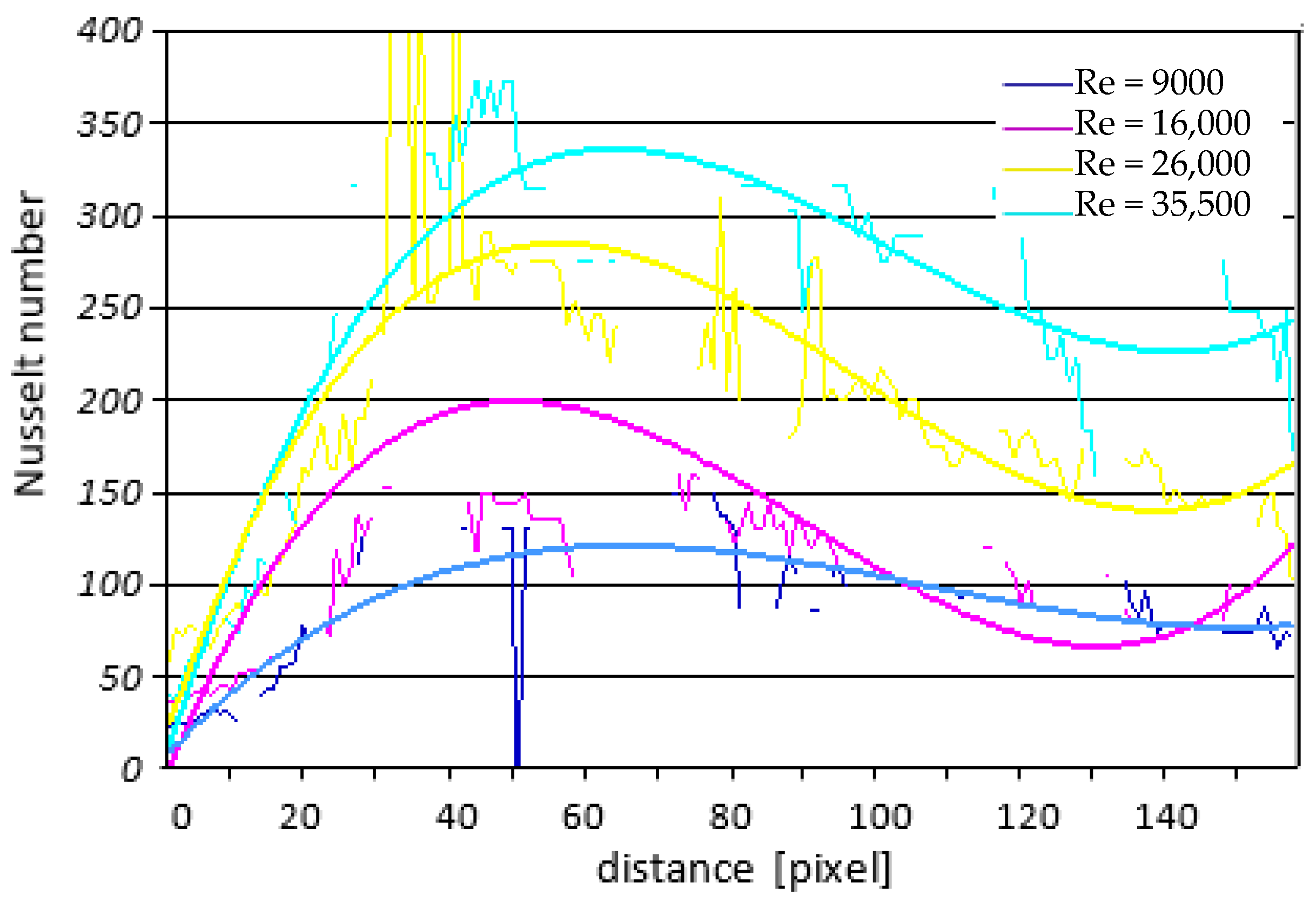
3.2. Results Obtained in the Transient Method
- Multiple increases of heat exchange and flow stabilization,
- Much greater influence on the increase of heat transfer at lower Reynolds numbers—this is confirmed by PIV measurements showing the absence of vortices and no blows or projected jets of air streams on the surfaces between the ribs,
- The influence of the ribs on the stabilization of heat transfer along the ribbed plate was also observed—higher heat transfer coefficients were obtained for the first section of the tested flat surface (entrance effect),
- For larger Reynolds numbers, the influence of the ribs is much smaller, which can be explained by the movement of the air stream above the turbulators—it looks like a pseudo laminar flow.
3.3. Data Reduction and Uncertainty Analysis
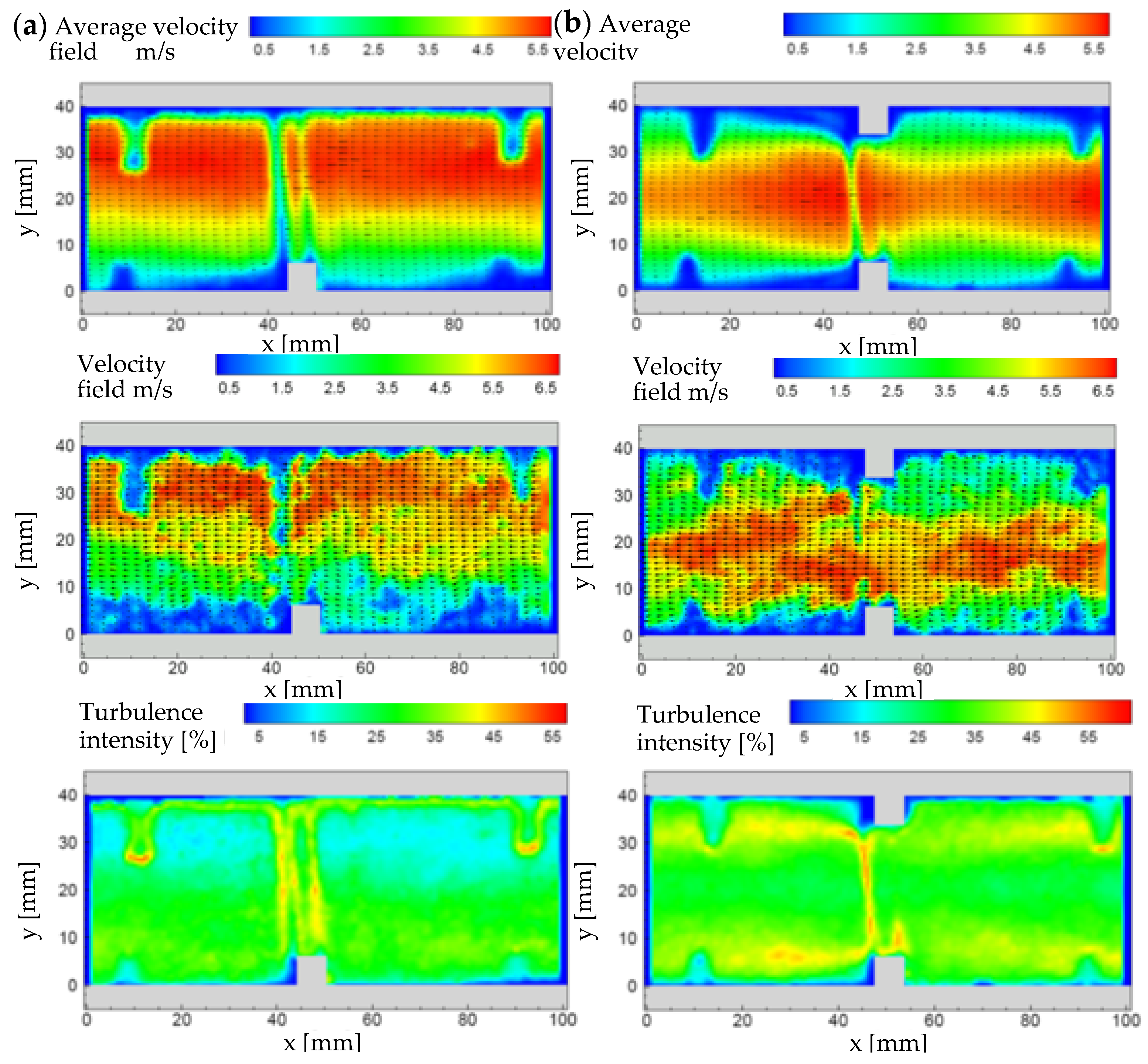
3.4. PIV Measurement Results
- Averaged velocity field: vectors and a colored contour of the velocity value,
- Selected instantaneous speed field: vectors and a colored contour of the speed value,
- Turbulence intensity field determined according to the relationship shown in Equation (11).
3.5. Numerical Results Obtained by CFD FLUENT
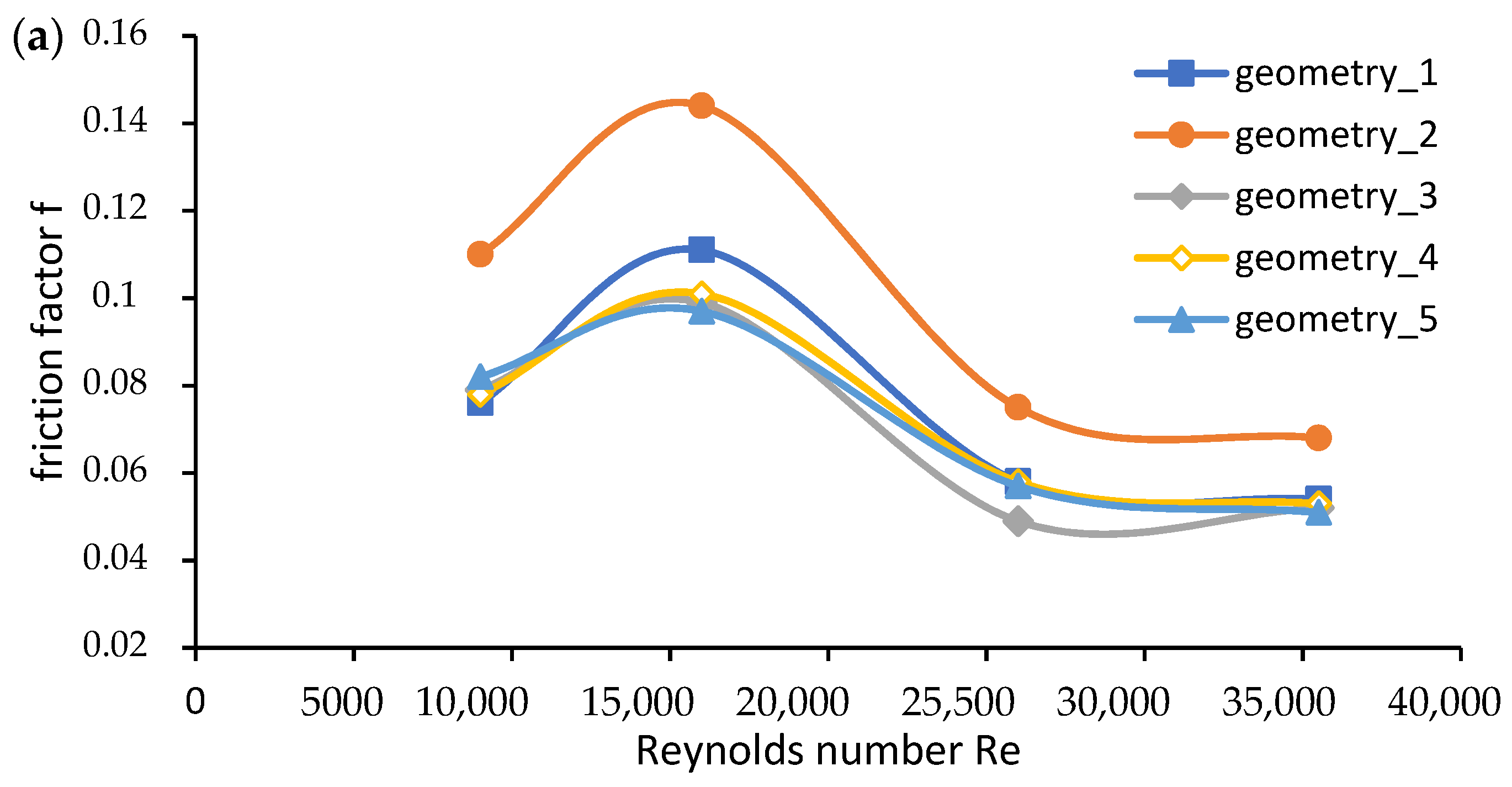
3.6. Friction Factor
4. Conclusions
- -
- Experimental studies of heat transfer and pressure drops (friction factors) in the model heat exchanger,
- -
- Visualization of velocity fields in the measurement section and their impact on the intensification of heat transfer by the application of PIV anemometry;
- -
- Numerical study of forced heat transfer on flat surfaces with mechanical flow turbulators (ribbed plate);
- -
- Comparison of the results of experimental tests in steady states, transient method and numerical calculations.
- High convergence of experimental and numerical methods was demonstrated for a higher Re number.
- The use of mechanical turbulators changed the location of the maximum and minimum values of the heat transfer coefficient or Nusselt numbers between the ribs.
- The effect of ribbing on the stabilization of heat transfer depending on the number of ribs was observed—higher heat transfer coefficients were obtained for the first measurement section and the so-called “entrance effect” was demonstrated.
- A good correlation between the turbulent momentum and heat transfer was shown.
- The designed test stand is burdened with small measurement errors calculated by the RSS method, which may prove the reliability of the results of the experimental research.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bergles, A.E. Augmentation of Heat Transfer, Single Phase. In International Encyclopedia of Heat and Mass Transfer; Hewitt, G.F., Shires, G.L., Polezhaev, Y.V., Eds.; CRC Press: London, UK, 1997; pp. 68–73. [Google Scholar]
- Tropea, C.; Yarin, A.L.; Foss, J.F. Springer Handbook of Experimental Fluid Mechanics Berlin; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Utriainen, E.; Sundén, B. Evaluation of the Cross Corrugated and Some Other Candidate Heat Transfer Surfaces for Microturbine Recuperators. J. Eng. Gas. Turbines Power 2002, 124, 550–560. [Google Scholar] [CrossRef]
- Borda, T.; Flaszyński, P.; Doerffer, P.; Jewartowski, M.; Stasiek, J. Flow visualization and heat transfer investigations on the flat plate with streamwise pressure gradient. In Proceedings of the 12th International Symposium on Experimental Computational Aerothermodynamics of Internal Flows, Lerici, Italy, 13–16 July 2015. [Google Scholar]
- Szwaba, R.; Kaczynski, P.; Doerffer, P.; Telega, J. Flow structure and heat exchange analysis in internal cooling channel of gas turbine blade. J. Therm. Sci. 2016, 25, 336–341. [Google Scholar] [CrossRef]
- Fiebig, M.; Chen, Y. Heat Transfer Enhancement by Wing-Type Longitudinal Vortex Generators and their Application to Finned Oval Tube Heat Exchanger Elements. In Heat Transfer Enhancement of Heat Exchangers; Kakaç, S., Bergles, A.E., Mayinger, F., Yüncü, H., Eds.; Springer: Dordrecht, The Netherlands, 1999; pp. 79–105. [Google Scholar]
- Stasiek, J.; Jewartowski, M.; Kowalewski, T.A. The Use of Liquid Crystal Thermography in Selected Technical and Medical Applications—Recent Development. J. Cryst. Process. Technol. 2014, 4, 46–59. [Google Scholar] [CrossRef] [Green Version]
- Satta, F.; Tanda, G. Measurement of local heat transfer coefficient on the endwall of a turbine blade cascade by liquid crystal thermography. Exp. Therm. Fluid Sci. 2014, 58, 209–215. [Google Scholar] [CrossRef]
- Raffel, M.; Willert, C.E.; Kompenhans, J. Particle Image Velocimetry; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Alkhamis, N.Y.; Rallabandi, A.P.; Han, J.-C. Heat Transfer and Pressure Drop Correlations for Square Channels With V-Shaped Ribs at High Reynolds Numbers. J. Heat Transf. 2011, 133, 111901. [Google Scholar] [CrossRef]
- Baughn, J.W.; Ireland, P.T.; Jones, T.V.; Saniei, N. A Comparison of the Transient and Heated-Coating Methods for the Measurement of Local Heat Transfer Coefficients on a Pin Fin. Volume 5: Manufacturing Materials and Metallurgy; Ceramics; Structures and Dynamics; Controls, Diagnostics and Instrumentation. Volume 4: Heat Transfer; Electric Power; Industrial and Cogeneration. Am. Soc. Mech. Eng. 1988, 111, 877–881. [Google Scholar] [CrossRef] [Green Version]
- Ciofalo, M.; Di Piazza, I.; Stasiek, J.A. Investigation of flow and heat transfer in corrugated-undulated plate heat exchangers. Heat Mass. Transf. 2000, 36, 449–462. [Google Scholar] [CrossRef]
- Ciofalo, M.; Di Liberto, M.; La Cerva, M.; Tamburini, A. Turbulent heat transfer in spacer-filled channels: Experimental and computational study and selection of turbulence models. Int. J. Therm. Sci. 2019, 145, 106040. [Google Scholar] [CrossRef] [Green Version]
- Ghorab, M.G. Film cooling effectiveness and net heat flux reduction of advanced cooling schemes using thermochromic liquid crystal. Appl. Therm. Eng. 2011, 31, 77–92. [Google Scholar] [CrossRef]
- Kumar, S.; Jakkareddy, P.S.; Balaji, C. A novel method to detect hot spots and estimate strengths of discrete heat sources using liquid crystal thermography. Int. J. Therm. Sci. 2020, 154, 106377. [Google Scholar] [CrossRef]
- Kumar, A.; Layek, A. Nusselt number and fluid flow analysis of solar air heater having transverse circular rib roughness on absorber plate using LCT and computational technique. Therm. Sci. Eng. Prog. 2019, 14, 100398. [Google Scholar] [CrossRef]
- Rao, Y.; Liu, Y.; Wan, C. Multiple-jet impingement heat transfer in double-wall cooling structures with pin fins and effusion holes. Int. J. Therm. Sci. 2018, 133, 106–119. [Google Scholar] [CrossRef]
- Rao, Y.; Zhang, P.; Xu, Y.; Ke, H. Experimental study and numerical analysis of heat transfer enhancement and turbulent flow over shallowly dimpled channel surfaces. Int. J. Heat Mass Transf. 2020, 160, 120195. [Google Scholar] [CrossRef]
- Mikielewicz, D.; Stasiek, A.; Jewartowski, M.; Stasiek, J. Measurements of heat transfer enhanced by the use of transverse vortex generators. Appl. Therm. Eng. 2012, 49, 61–72. [Google Scholar] [CrossRef]
- Stasiek, J. Experimental studies of heat transfer and fluid flow across corrugated-undulated heat exchanger surfaces. Int. J. Heat Mass Transf. 1998, 41, 899–914. [Google Scholar] [CrossRef]
- Stasiek, J.; Ciofalo, M.; Wierzbowski, M. Experimental and numerical simulations of flow and heat transfer in heat exchanger elements using liquid crystal thermography. J. Therm. Sci. 2004, 13, 133–137. [Google Scholar] [CrossRef]
- Stasiek, J.; Kowalewski, T. The use of thermochromic liquid crystals in heat transfer research. In Proceedings of the XIV Conference Liquid Crystals Chemistry, Physics and Application, Zakopane, Poland, 3–7 September 2001. [Google Scholar]
- Stąsiek, J.; Jewartowski, M.; Collins, M. Liquid crystal thermography and true-colour digital image processing. Opt. Laser Technol. 2006, 38, 243–256. [Google Scholar] [CrossRef]
- Tanda, G. Heat transfer in rectangular channels with transverse and V-shaped broken ribs. Int. J. Heat Mass Transf. 2004, 47, 229–243. [Google Scholar] [CrossRef]
- Tanda, G.; Abram, R. Forced Convection Heat Transfer in Channels with Rib Turbulators Inclined at 45 deg. J. Turbomach. 2009, 131, 021012. [Google Scholar] [CrossRef]
- Stasiek, J.; Jewartowski, M. LCT, PIV and IR Imaging Detection in Selected Technical and Biomedical Applications. In Proceedings of the Journal of Physics: Conference Series; IOP Publishing: Bristol, UK, 2019; Volume 1224. [Google Scholar]
- Stasiek, J.; Jewartowski, M. The use of liquid crystal thermography TLC and particle image velocimetry PIV in selected technical applications. Arch. Thermodyn. 2018, 39, 129–147. [Google Scholar]
- Stasiek, A. Passive Intensification of Heat Exchange on Ribbed Flat Surfaces. Ph.D. Thesis, Koszalin University of Technology, Koszalin, Poland, 2011. [Google Scholar]
- Jones, T.V.; Wang, Z.; Ireland, P.T. The use of liquid crystals in aerodynamic and heat transfer experiments. In Proceedings of the Seminar on optical methods and Data Processing in Heat and Fluid Flow, London, UK, 2–3 April 1992; pp. 51–65. [Google Scholar]
- Leiner, W.; Schulz, K.; Behle, M.; Lorenz, S. Imaging techniques to measure local heat and mass transfer. In Proceedings of the 3nd I Mech E Seminar Optical Methods and Data Processing in Heat and Fluid Flow, London, UK, 18–19 April 1996; pp. 1–13. [Google Scholar]
- Valencia, A.; Fiebig, M.; Mitra, N.K.; Leiner, W. Heat transfer and flow loss in a fin-tube heat exchanger element with wing-type vortex generators. In Proceedings of the Heat Transfer 3rd UK National Conference Incorporating 1st European Conference on Thermal Sciences, Birmingham, UK, 16–18 September 1992. [Google Scholar]
- Webb, R.L. Princeples of Enhanced Heat Transfer; John Willey & Sons Inc.: Hoboken, NJ, USA, 1994. [Google Scholar]
- Xie, P.; Zhang, X. A novel method of enhancing convective heat transfer by dynamic controlling rib. Int. Commun. Heat Mass Transf. 2020, 119, 104830. [Google Scholar] [CrossRef]
- Hayat, M.Z.; Nandan, G.; Tiwari, A.K.; Sharma, S.K.; Shrivastava, R.; Singh, A.K. Numerical study on heat transfer enhancement using twisted tape with trapezoidal ribs in an internal flow. Mater. Today Proc. 2021, 46, 5412–5419. [Google Scholar] [CrossRef]
- Xiao, H.; Liu, Z.; Liu, W. Turbulent heat transfer enhancement in the mini-channel by enhancing the original flow pattern with v-ribs. Int. J. Heat Mass Transf. 2020, 163, 120378. [Google Scholar] [CrossRef]
- Image Processing Handbook; Data Translation Ltd.: Boston, MA, USA, 1991.
- ANSYS. Modelling Turbulent Flows, Introductory FLUENT Training; ANSYS: Canonsburg, PA, USA, 2006. [Google Scholar]
- Handbook of Thermochromic Liquid Crystal Technology LCR; Hallcrest Ltd.: Glenview, IL, USA, 2020.
- Reinitzer, F. Beiträge zur Kenntniss des Cholesterins. Mon. Für Chem. Und Verwandte Teile Wiss. 1888, 9, 421–441. [Google Scholar] [CrossRef]
- Jones, T.V.; Hippensteele, S.A. High-resolution heat transfer coefficient mops applicable to compound-curve surfaces using liquid crystals in a transient wind tunnel. In Proceedings of the ASME National Heat Transfer Conference, Houston, TX, USA, 24–27 July 1988. [Google Scholar]
- Launder, B.E.; Spalding, D.B. The numerical computation of turbulent flows. Comput. Methods Appl. Mech. Eng. 1974, 3, 269–289. [Google Scholar] [CrossRef]
- Moffat, R.J. Describing the uncertainties in experimental results. Exp. Therm. Fluid Sci. 1988, 1, 3–17. [Google Scholar] [CrossRef] [Green Version]
- Moody, L.F. Friction factors for pipe flow. Trans. ASME 1944, 66, 671–684. [Google Scholar]
- Pigott, R.J.S. The flow of fluids in closed conduits. Mech. Eng. 1933, 55, 497–501. [Google Scholar]
- Zhang, X.; Stasiek, J.A.; Collins, M.W. Experimental and Numerical Analysis of Convective Heat Transfer in a Turbulent Channel Flow with Square and Circular Columns. Exp. Therm. Fluid Sci. 1995, 10, 229–237. [Google Scholar] [CrossRef]

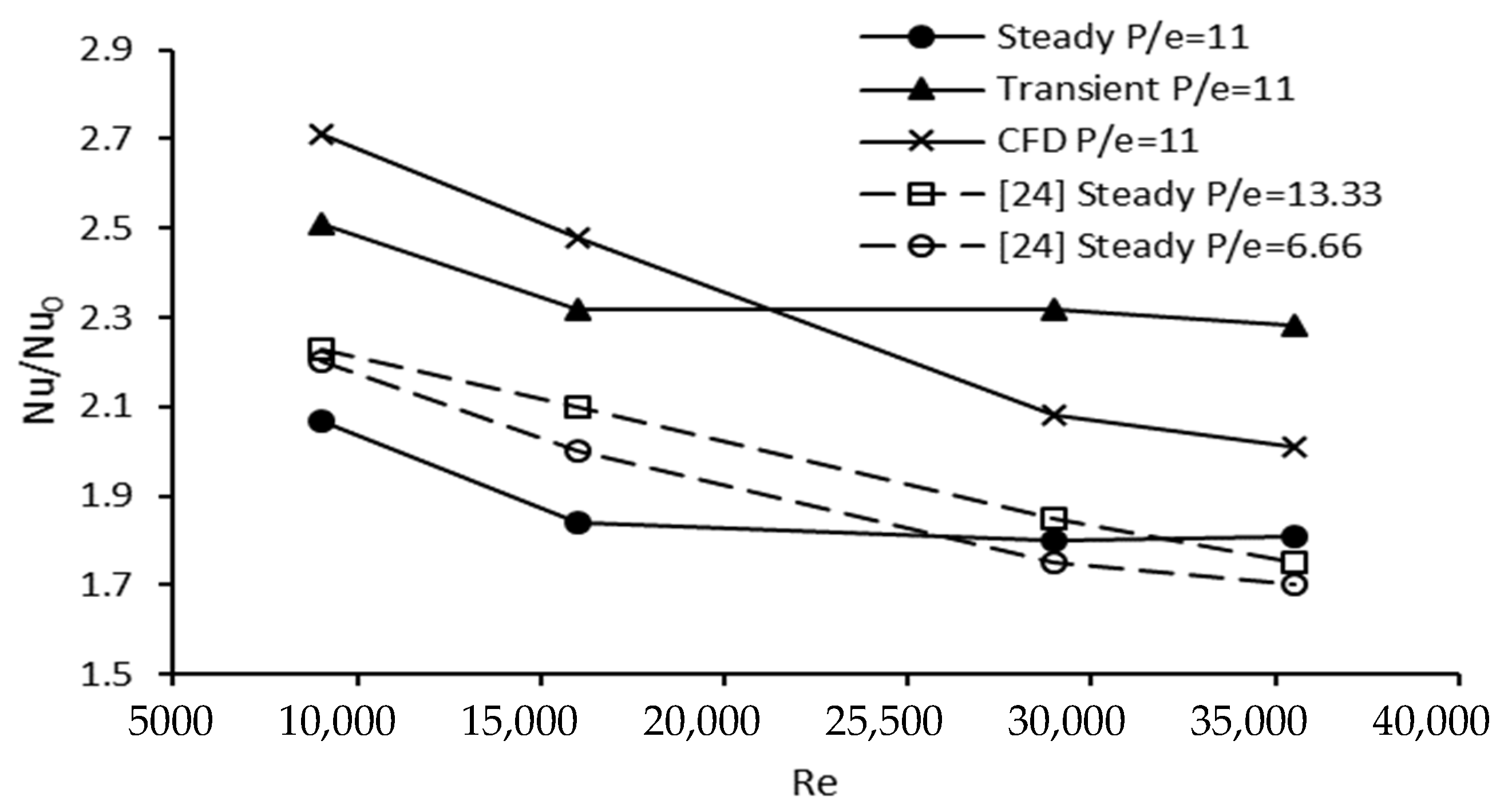
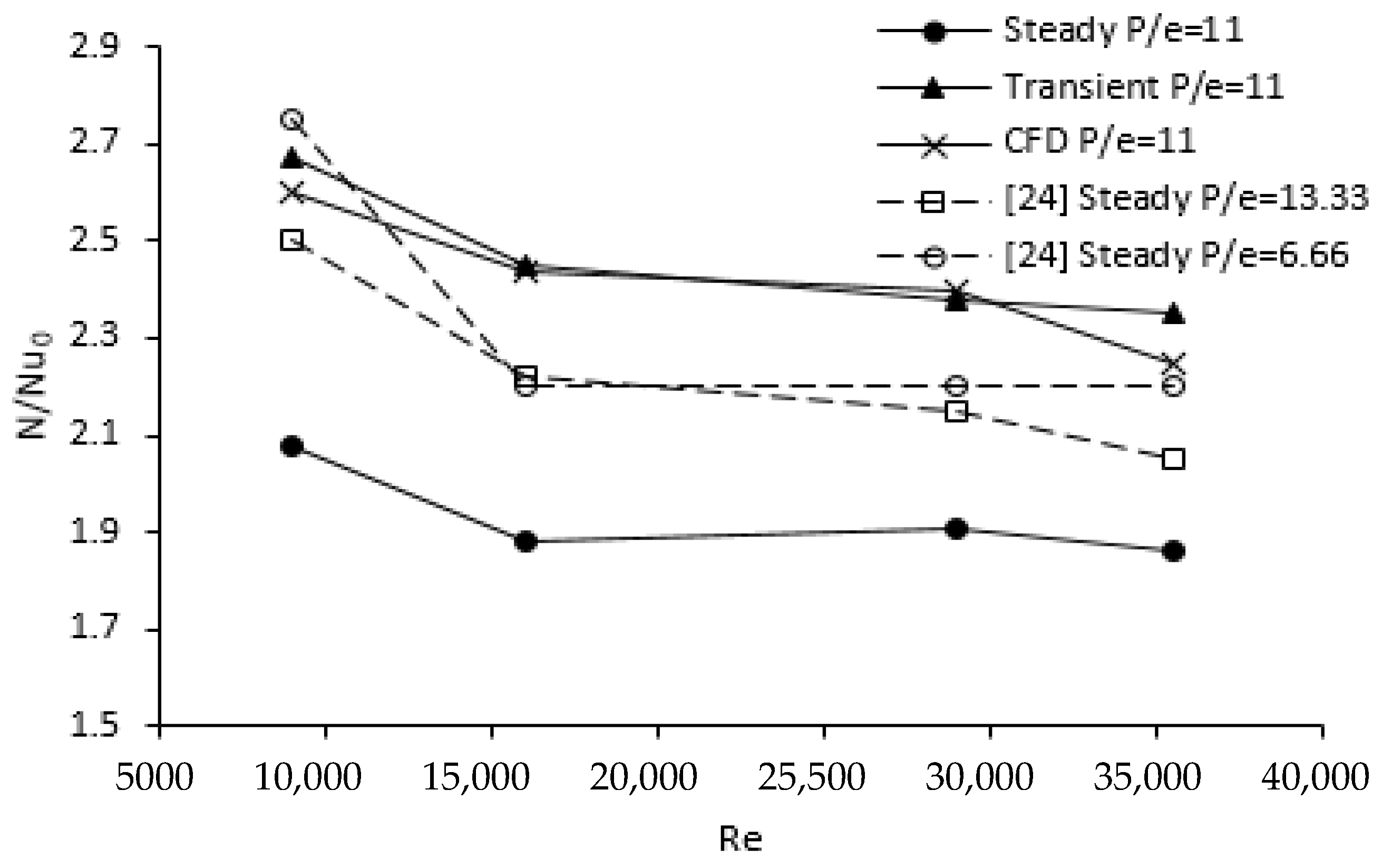

| Nuav | ||||||||
|---|---|---|---|---|---|---|---|---|
| Re | p1 | p2 | p3 | p4 | p5 | p6 | Nuav | |
| Conf_1 | 9000 | 66.53 | 65.40 | 62.34 | 58.11 | 57.41 | 65.18 | 62.49 |
| 16,000 | 102.58 | 100.27 | 97.51 | 95.05 | 95.11 | 108.28 | 99.80 | |
| 26,000 | 140.04 | 140.88 | 135.55 | 127.91 | 126.17 | 142.88 | 135.57 | |
| 35,500 | 172.41 | 175.69 | 171.68 | 162.48 | 162.39 | 184.31 | 171.49 | |
| Conf_2 | 9000 | 85.11 | 84.36 | 87.09 | 84.60 | 83.73 | 94.44 | 86.55 |
| 16,000 | 130.81 | 131.15 | 126.37 | 122.65 | 121.01 | 135.24 | 127.87 | |
| 26,000 | 172.57 | 172.98 | 172.25 | 168.10 | 167.63 | 185.68 | 173.20 | |
| 35,500 | 212.03 | 213.63 | 211.62 | 207.78 | 204.67 | 222.39 | 212.02 | |
| Conf_3 | 9000 | 60.15 | 60.88 | 60.88 | 60.21 | 57.59 | 64.96 | 60.78 |
| 16,000 | 88.26 | 87.31 | 86.14 | 84.05 | 83.73 | 96.23 | 87.62 | |
| 26,000 | 118.10 | 122.66 | 121.74 | 122.61 | 117.56 | 113.54 | 119.37 | |
| 35,500 | 153.72 | 160.76 | 156.67 | 157.25 | 154.14 | 155.94 | 156.41 | |
| Conf_4 | 9000 | 59.00 | 60.30 | 60.17 | 59.82 | 59.84 | 59.47 | 59.77 |
| 16,000 | 85.36 | 86.11 | 86.22 | 86.64 | 87.33 | 84.85 | 86.09 | |
| 26,000 | 126.10 | 132.26 | 129.21 | 129.93 | 127.14 | 129.13 | 128.96 | |
| 35,500 | 155.48 | 163.43 | 161.59 | 161.53 | 154.86 | 163.78 | 160.11 | |
| Conf_5 | 9000 | 62.18 | 64.90 | 61.76 | 60.80 | 58.68 | 61.74 | 61.68 |
| 16,000 | 86.60 | 88.70 | 84.89 | 83.00 | 80.90 | 87.21 | 85.22 | |
| 26,000 | 128.67 | 134.23 | 126.45 | 125.87 | 125.87 | 133.42 | 129.08 | |
| 35,500 | 152.97 | 155.70 | 154.04 | 155.49 | 152.15 | 155.48 | 154.30 | |
| Conf_6 | 9000 | 63.07 | 62.88 | 62.05 | 60.93 | 61.88 | 69.69 | 63.42 |
| 16,000 | 81.76 | 83.35 | 81.64 | 79.72 | 79.22 | 86.66 | 82.06 | |
| 26,000 | 117.90 | 119.95 | 121.04 | 119.07 | 117.37 | 134.27 | 121.60 | |
| 35,500 | 159.66 | 149.55 | 148.39 | 145.72 | 147.21 | 164.68 | 152.54 | |
| Conf_7 | 9000 | 103.57 | 100.07 | 95.16 | 91.49 | 93.18 | 100.96 | 97.40 |
| 16,000 | 128.73 | 129.84 | 119.80 | 115.13 | 117.27 | 127.39 | 123.03 | |
| 26,000 | 174.27 | 177.22 | 172.20 | 168.09 | 165.01 | 174.92 | 171.95 | |
| 35,500 | 203.60 | 207.21 | 199.56 | 194.44 | 191.83 | 202.54 | 199.87 | |
| Re | Nu0 | Conf._1 | Conf._2 | Conf._3 | Conf._4 | Conf._5 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Nu/Nu0 | Nu/Nu0 | Nu/Nu0 | Nu/Nu0 | Nu/Nu0 | ||||||||||||
| St | Tr | CFD | St | Tr | CFD | St | Tr | CFD | St | Tr | CFD | St | Tr | CFD | ||
| 9000 | 29 | 2.01 | 1.63 | 2.10 | 2.91 | 2.88 | 2.75 | 2.07 | 2.51 | 2.71 | 2.08 | 2.67 | 2.60 | 2.19 | 2.87 | 2.65 |
| 16,000 | 46 | 2.06 | 1.78 | 2.02 | 2.67 | 2.89 | 2.39 | 1.84 | 2.32 | 2.48 | 1.88 | 2.45 | 2.44 | 1.88 | 2.21 | 2.04 |
| 26,000 | 68 | 1.89 | 1.64 | 1.82 | 2.47 | 2.62 | 2.16 | 1.80 | 2.32 | 2.08 | 1.91 | 2.38 | 2.40 | 1.85 | 2.01 | 2.01 |
| 35,500 | 87 | 1.87 | 1.79 | 1.83 | 2.39 | 2.54 | 2.08 | 1.81 | 2.28 | 2.01 | 1.86 | 2.35 | 2.25 | 1.79 | 2.00 | 1.89 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stąsiek, J.; Stąsiek, A.; Szkodo, M. Modeling of Passive and Forced Convection Heat Transfer in Channels with Rib Turbulators. Energies 2021, 14, 7059. https://doi.org/10.3390/en14217059
Stąsiek J, Stąsiek A, Szkodo M. Modeling of Passive and Forced Convection Heat Transfer in Channels with Rib Turbulators. Energies. 2021; 14(21):7059. https://doi.org/10.3390/en14217059
Chicago/Turabian StyleStąsiek, Jan, Adam Stąsiek, and Marek Szkodo. 2021. "Modeling of Passive and Forced Convection Heat Transfer in Channels with Rib Turbulators" Energies 14, no. 21: 7059. https://doi.org/10.3390/en14217059
APA StyleStąsiek, J., Stąsiek, A., & Szkodo, M. (2021). Modeling of Passive and Forced Convection Heat Transfer in Channels with Rib Turbulators. Energies, 14(21), 7059. https://doi.org/10.3390/en14217059









